From Chaos to Ordering: New Studies in the Shannon Entropy of 2D Patterns
Abstract
:1. Introduction
2. Methods
2.1. Software
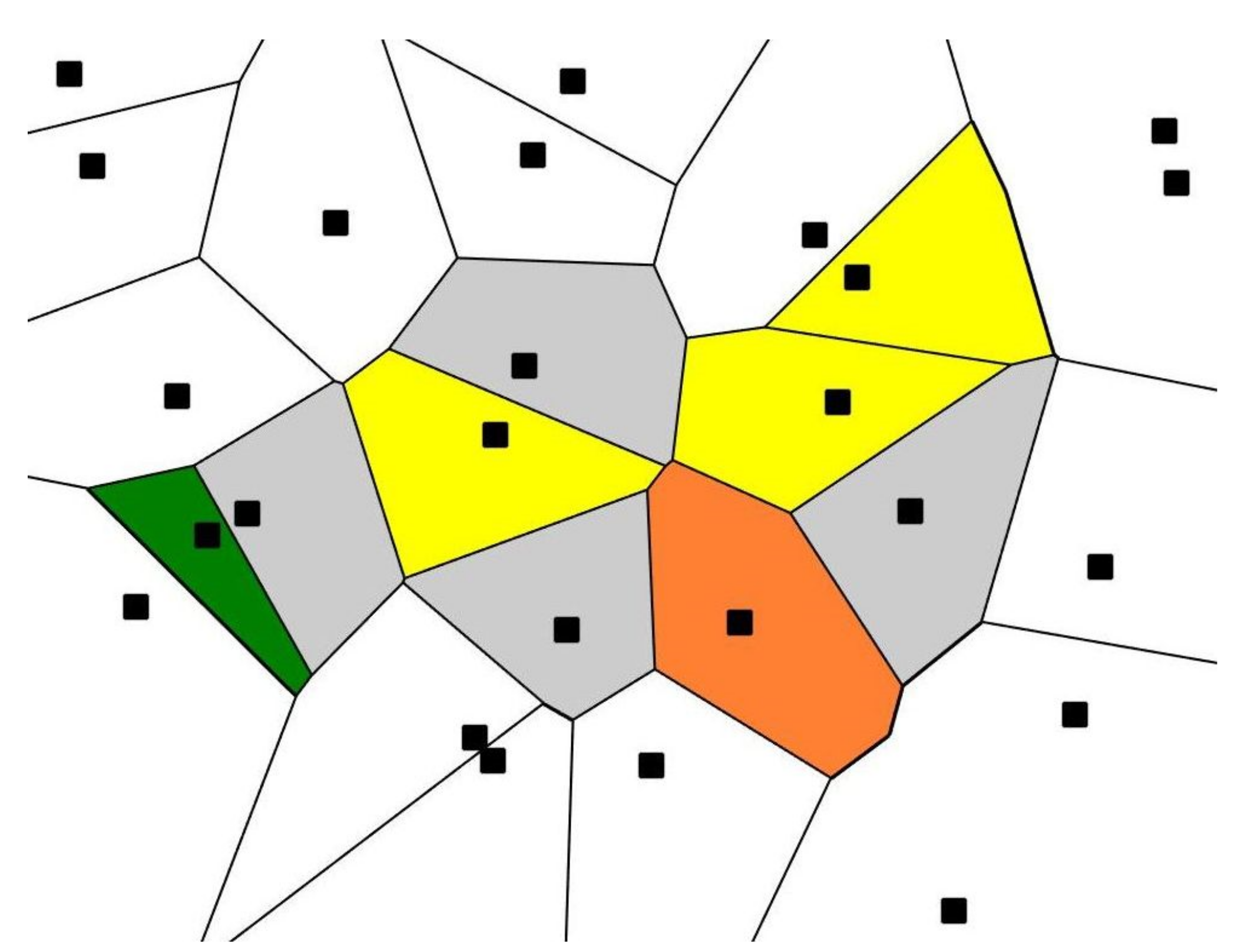
2.2. Procedure for Generation of Voronoi Diagrams
2.3. Procedure of Calculation of the Continuous Measure of Symmetry
3. Results
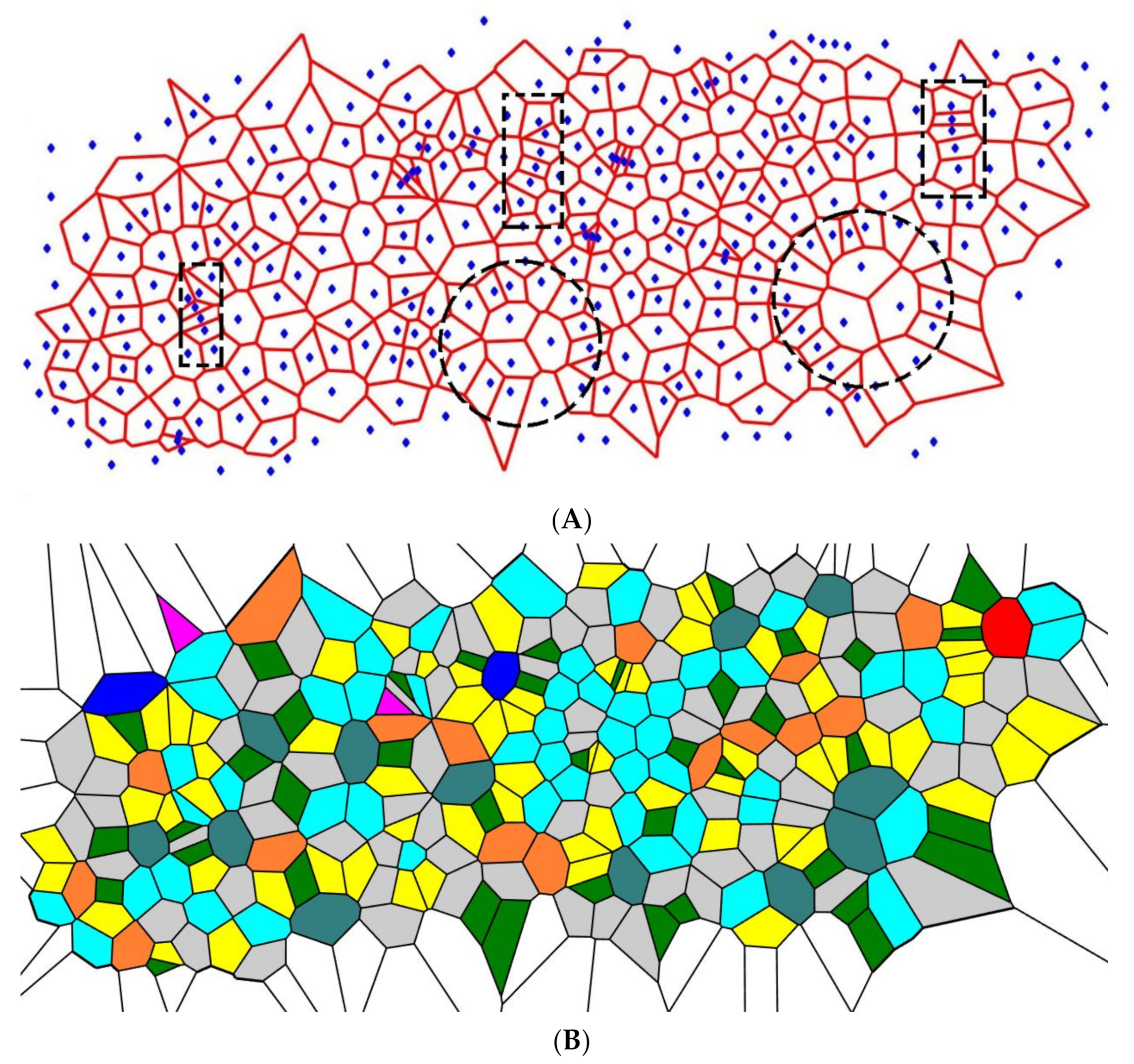
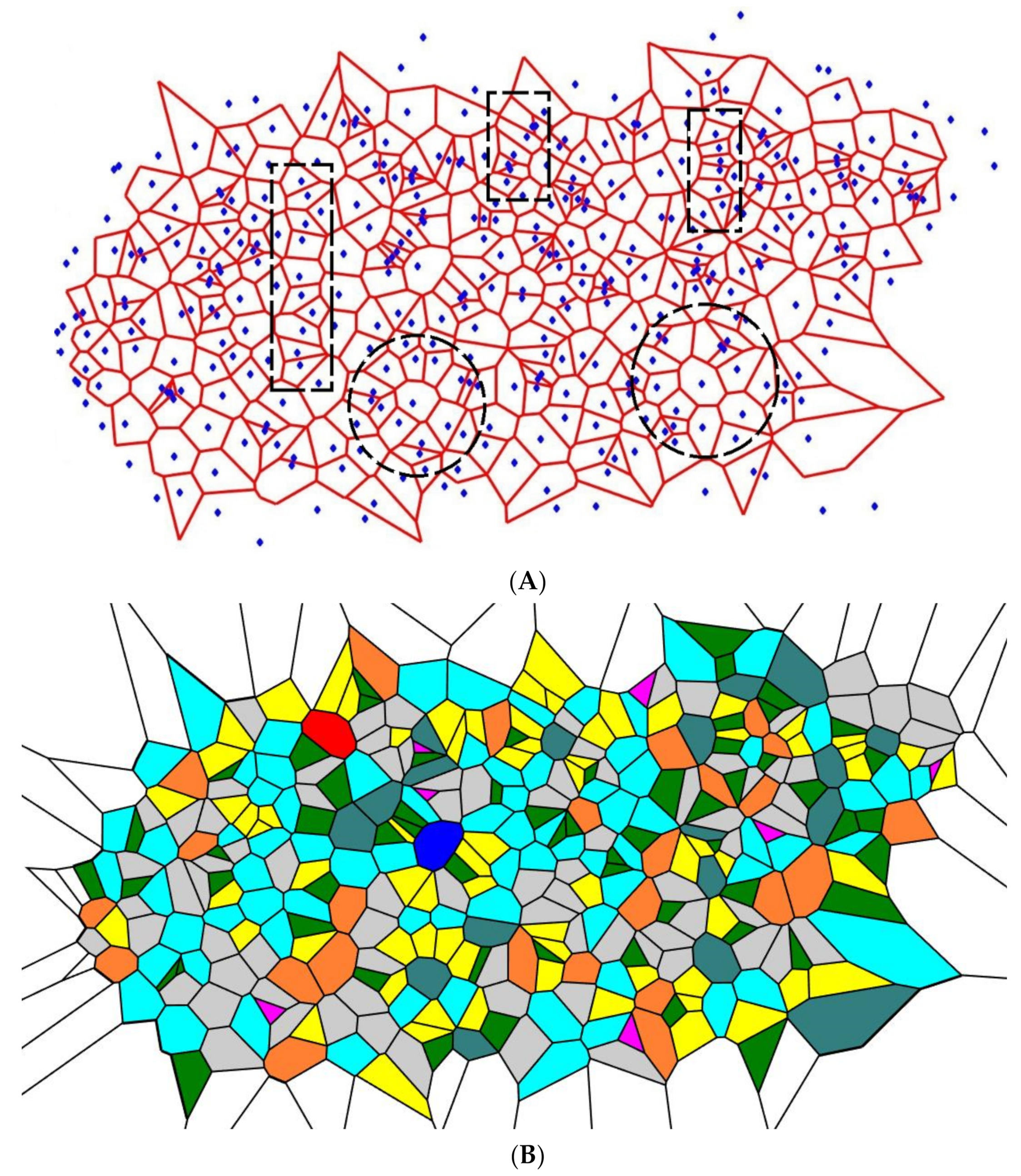
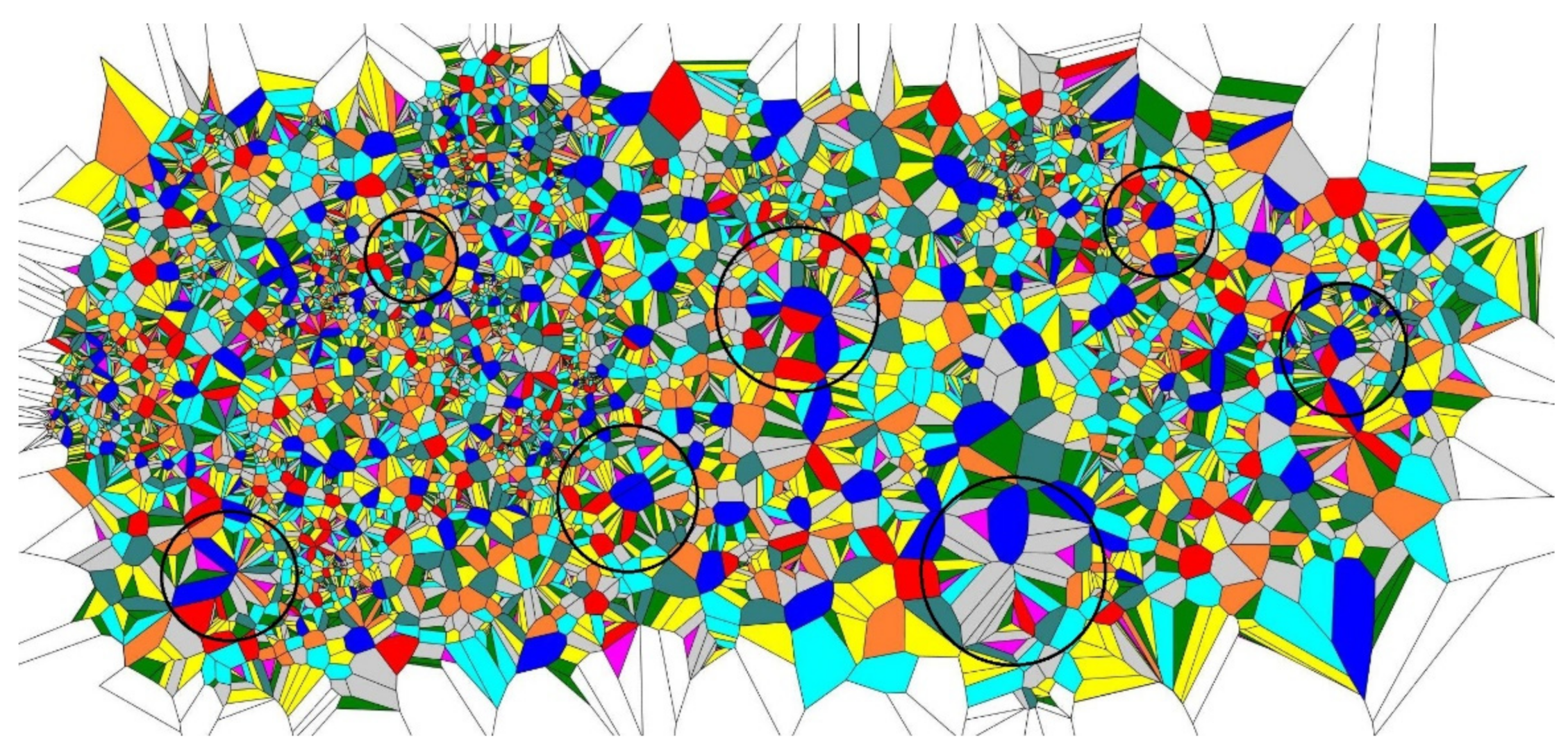
3.1. Ordering in Voronoi Diagrams: Qualitative Analysis
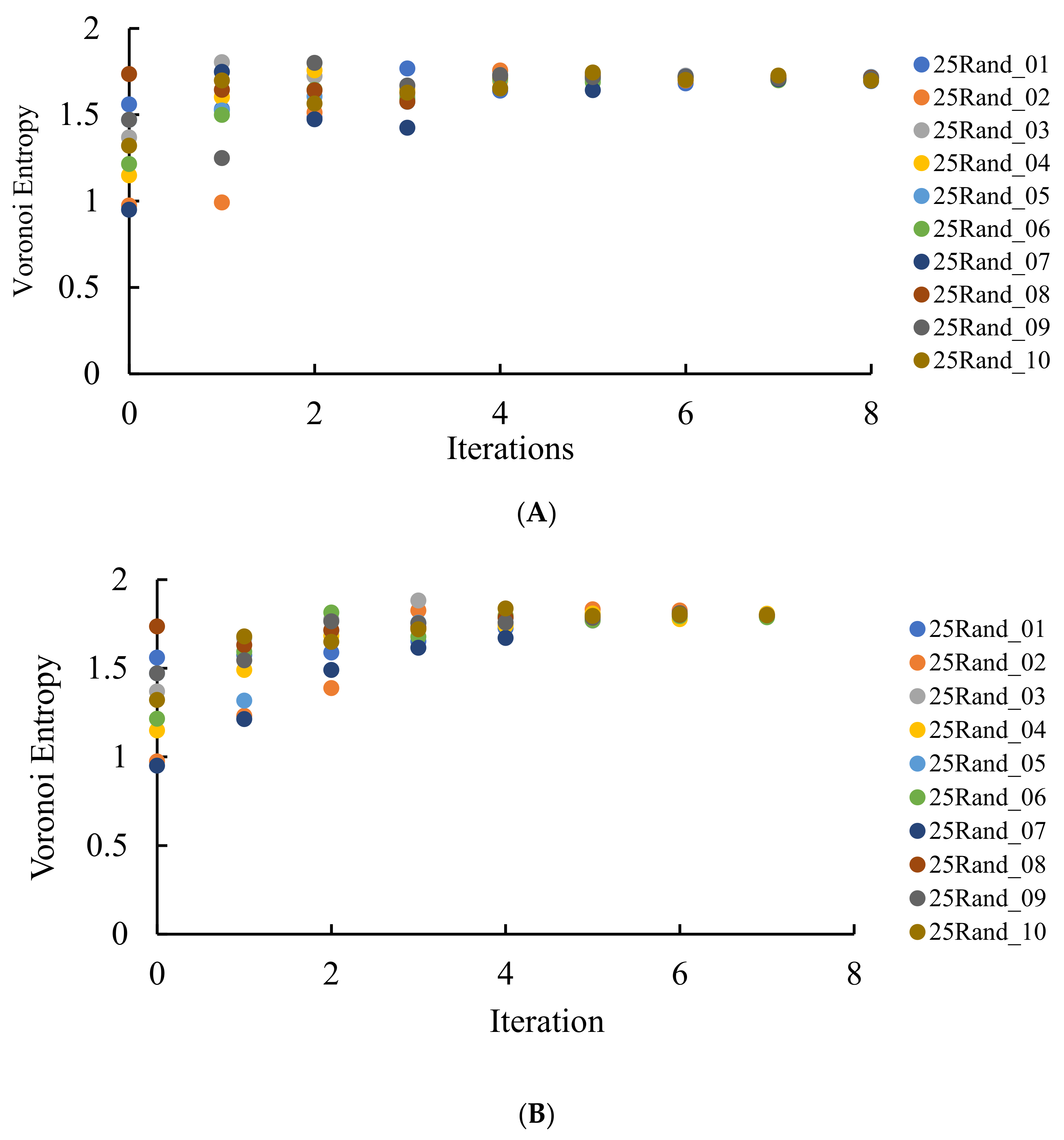
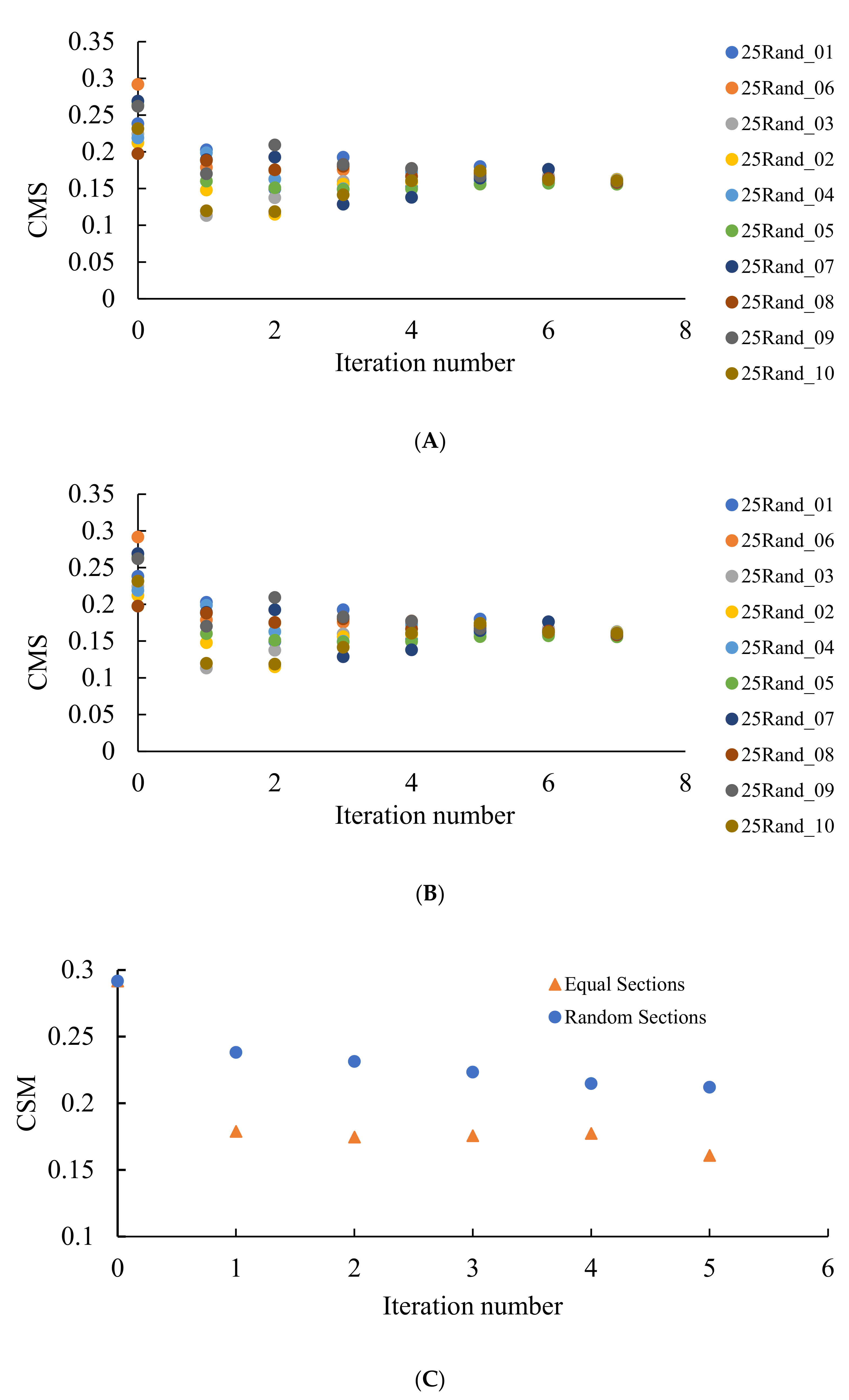
3.2. Quantitative Analysis of the Voronoi Diagrams
3.3. Physical Interpretation of the Self Organization
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mermin, N.D. Crystalline Order in Two Dimensions. Phys. Rev. 1968, 176, 250–254. [Google Scholar] [CrossRef]
- Onsager, L. Crystal Statistics. I. A Two-Dimensional Model with an Order-Disorder Transition. Phys. Rev. 1944, 65, 117–149. [Google Scholar] [CrossRef]
- Berezinskii, V.L. Destruction of long-range order in one-dimensional and two-dimensional systems having a continuous symmetry group I. Classical systems. Sov. Phys. JETP 1971, 32, 493–500. [Google Scholar]
- Berezinskii, V.L. Destruction of long-range order in one-dimensional and two-dimensional systems possessing a continuous symmetry group II, Quantum systems. Sov. Phys. JETP 1972, 34, 610–616. [Google Scholar]
- Hénon, M. A Two-dimensional Mapping with a Strange Attractor. In The Theory of Chaotic Attractors; Hunt, B.R., Li, T.Y., Kennedy, J.A., Nusse, H.E., Eds.; Springer: New York, NY, USA, 1976. [Google Scholar]
- Zabrodsky, H.; Peleg, S.; Avnir, D. Symmetry as a continuous feature. IEEE Trans. Pattern Anal. Mach. Intel. 1995, 17, 1154–1166. [Google Scholar] [CrossRef] [Green Version]
- Kovalerchuk, B. Visual Knowledge Discovery and Machine Learning; Springer International Publishing: Cham, Switzerland, 2018. [Google Scholar]
- Hu, W.; Singh, R.R.P.; Scalettar, R.T. Discovering phases, phase transitions, and crossovers through unsupervised machine learning: A critical examination. Phys. Rev. E 2017, 95, 062122. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, J.; Marques, M.R.G.; Botti, S.; Miguel, A.L.; Marques, M.A.L. Recent advances and applications of machine learning in solid-state materials science. NPJ Comput. Mater. 2019, 5, 83. [Google Scholar] [CrossRef]
- Voronoi, G. Nouvelles applications des paramètres continus à la théorie des formes quadratiques. Deuxième mémoire. Recherches sur les paralléloèdres primitifs. Reine Angew. Math. 1908, 134, 198–287. [Google Scholar] [CrossRef]
- Weaire, D.; Rivier, N. Soap, cells and statistics—Random patterns in two dimensions. Contemp. Phys. 1984, 25, 59–99. [Google Scholar] [CrossRef]
- Mayarani, M.; Basavaraj, M.G.; Satapathy, D.K. Colloidal monolayers with cell-like tessellations via interface assisted evaporative assembly. J. Colloid Interface Sci. 2021, 583, 683–691. [Google Scholar] [CrossRef]
- Borodin, E.; Jivkov, A.P.; Sheinerman, A.G.; Gutkin, M.Y. Optimisation of rGO-enriched nanoceramics by combinatorial analysis. Mater. Des. 2021, 212, 110191. [Google Scholar] [CrossRef]
- Liu, Y.T.; Tao, C.L.; Zhang, X.; Xia, W.; Shi, D.-Q.; Qi, L.; Xu, C.; Sun, R.; Li, X.-W.; Lau, P.-M.; et al. Mesophasic organization of GABAA receptors in hippocampal inhibitory synapses. Nat. Neurosci. 2020, 23, 1589–1596. [Google Scholar] [CrossRef]
- Bormashenko, E.; Frenkel, M.; Vilk, A.; Legchenkova, I.; Fedorets, A.A.; Aktaev, N.E.; Dombrovsky, L.A.; Nosonovsky, M. Characterization of Self-Assembled 2D Patterns with Voronoi Entropy. Entropy 2018, 20, 956. [Google Scholar] [CrossRef] [Green Version]
- Frenkel, M.; Arya, P.; Bormashenko, E.; Santer, S. Quantification of ordering in active light driven colloids. J. Colloid Interface Sci. 2021, 586, 866–875. [Google Scholar] [CrossRef]
- Barthélemy, M. Spatial networks. Phys. Rep. 2011, 499, 1–101. [Google Scholar] [CrossRef] [Green Version]
- Matsubara, T.; Hikage, C.; Kuriki, S. Minkovski functionals and the nonlinear perturbation theory in the large-scale structure: Second-order effects. Phys. Rev. D 2022, 105, 023527. [Google Scholar] [CrossRef]
- Parker, J.; Sherman, E.; van de Raa, M.; van der Meer, D.; Samelson, L.E.; Losert, W. Automatic sorting of point pattern sets using Minkowski functionals. Phys. Rev. E 2013, 88, 022720. [Google Scholar] [CrossRef] [Green Version]
- Mantz, H.; Jacobs, K.; Mecke, K. Utilizing Minkowski functionals for image analysis: A marching square algorithm. J. Stat. Mech. Theor. Exp. 2008, 2008, P12015. [Google Scholar] [CrossRef]
- Kürsten, R.; Stroteich, S.; Hernández, M.Z.; Ihle, T. Multiple Particle Correlation Analysis of Many-Particle Systems: Formalism and Application to Active Matter. Phys. Rev. Lett. 2020, 124, 088002. [Google Scholar] [CrossRef] [Green Version]
- Bormashenko, E.; Malkin, A.; Musin, A. Mesoscopic patterning in evaporated Polymer solutions: Poly(ethylene glycol) and room-temperature-vulcanized Polyorganosilanes/-siloxanes Promote formation of honeycomb structures. Macromol. Chem. Phys. 2008, 209, 567–576. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, J.; Zhu, X.; Lu, T. Testing symmetry based on empirical likelihood. J. Appl. Stat. 2018, 45, 2429–2454. [Google Scholar] [CrossRef]
- Pinsky, M.; Dryzun, C.; Casanova, D.; Alemany, P.; Avnir, D. Analytical methods for calculating Continuous Symmetry Measures and the Chirality Measure. J. Comput. Chem. 2008, 29, 2712–2721. [Google Scholar] [CrossRef]
- Bonjack, M.; Avnir, D. The near-symmetry of protein oligomers: NMR-derived structures. Sci. Rep. 2020, 10, 8367. [Google Scholar] [CrossRef]
- Shalit, Y.; Tuvi-Arad, I. Side chain flexibility and the symmetry of protein homodimers. PLoS ONE 2020, 15, e0235863. [Google Scholar] [CrossRef]
- Bormashenko, E.; Legchenkova, I.; Frenkel, M.; Shvalb, N.; Shoval, S. Shannon (Information) Measures of Symmetry for 1D and 2D Shapes and Patterns. Appl. Sci. 2022, 12, 1127. [Google Scholar] [CrossRef]
- Frenkel, M.; Fedorets, A.A.; Dombrovsky, L.A.; Nosonovsky, M.; Legchenkova, I.; Bormashenko, E. Continuous Symmetry Measure vs Voronoi Entropy of Droplet Clusters. J. Phys. Chem. C 2021, 125, 2431–2436. [Google Scholar] [CrossRef]
- Bormashenko, E.; Legchenkova, I.; Frenkel, M.; Shvalb, N.; Shoval, S. Voronoi Entropy vs. Continuous Measure of Symmetry of the Penrose Tiling: Part I. Analysis of the Voronoi Diagrams. Symmetry 2021, 13, 1659. [Google Scholar] [CrossRef]
- Bassett, D.C.; Olle, R.H. On the lamellar morphology of isotactic polypropylene spherulites. Polymer 1984, 25, 935–943. [Google Scholar] [CrossRef]
- Bassett, D.C. Polymer Spherulites: A Modern Assessment. J. Macromol. Sci. B 2003, 42, 227–256. [Google Scholar] [CrossRef]
- Chafetz, H.; Barth, J.; Cook, M.; Zhou, G. Origins of carbonate spherulites: Implications for Brazilian Aptian pre-salt reservoir. Sediment. Geol. 2018, 365, 21–33. [Google Scholar] [CrossRef]
- Befus, K.S.; Watkins, J.; Gardner, J.E.; Richard, D.; Befus, K.M.; Miller, N.R.; Dingwell, D.B. Spherulites as in-situ recorders of thermal history in lava flows. Geology 2015, 43, 647–650. [Google Scholar] [CrossRef] [Green Version]
- Kirkham, A.; Tucker, M.E. Thrombolites, spherulites and fibrous crusts (Holkerian, Purbeckian, Aptian): Context, fabrics and origins. Sediment. Geol. 2018, 374, 69–84. [Google Scholar] [CrossRef]
- Canti, M.G. The Production and Preservation of Faecal Spherulites: Animals, Environment and Taphonomy. J. Archaeol. Sci. 1999, 26, 251–258. [Google Scholar] [CrossRef]
- Cong, Y.; Hong, Z.; Qi, Z.; Zhou, W.; Li, H.; Liu, H.; Chen, W.; Wang, X.; Li, L. Conformational Ordering in Growing Spherulites of Isotactic Polypropylene. Macromolecules 2010, 43, 9859–9864. [Google Scholar] [CrossRef]
- Limaye, A.V.; Narhe, R.D.; Dhote, A.M.; Ogale, S.B. Evidence for convective effects in breath figure formation on volatile fluid surfaces. Phys. Rev. Lett. 1996, 76, 3762–3765. [Google Scholar] [CrossRef] [Green Version]
- Lauritzen, J.I., Jr.; Hoffman, J.D. Theory of Formation of Polymer Crystals with Folded Chains in Dilute Solution. J. Res. Natl. Bur. Stand. 1960, 64, 73–102. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, Z.; Guo, B.; Xu, J. Secondary nucleation in polymer crystallization: A kinetic view. Polym. Cryst. 2021, 4, e10173. [Google Scholar] [CrossRef]
- Köster, U.; Jastrow, L. Oxidation of Zr-based metallic glasses and nanocrystalline alloys. Mater. Sci. Eng. A 2007, 449, 57–62. [Google Scholar] [CrossRef]
- Liu, X.D.; Liu, X.B.; Altounian, Z. Structural evolution of Fe33Zr67 and Fe90Zr10 metallic glasses. J. Non-Cryst. Solids 2005, 351, 604–611. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Legchenkova, I.; Frenkel, M.; Shvalb, N.; Shoval, S.; Gendelman, O.V.; Bormashenko, E. From Chaos to Ordering: New Studies in the Shannon Entropy of 2D Patterns. Entropy 2022, 24, 802. https://doi.org/10.3390/e24060802
Legchenkova I, Frenkel M, Shvalb N, Shoval S, Gendelman OV, Bormashenko E. From Chaos to Ordering: New Studies in the Shannon Entropy of 2D Patterns. Entropy. 2022; 24(6):802. https://doi.org/10.3390/e24060802
Chicago/Turabian StyleLegchenkova, Irina, Mark Frenkel, Nir Shvalb, Shraga Shoval, Oleg V. Gendelman, and Edward Bormashenko. 2022. "From Chaos to Ordering: New Studies in the Shannon Entropy of 2D Patterns" Entropy 24, no. 6: 802. https://doi.org/10.3390/e24060802
APA StyleLegchenkova, I., Frenkel, M., Shvalb, N., Shoval, S., Gendelman, O. V., & Bormashenko, E. (2022). From Chaos to Ordering: New Studies in the Shannon Entropy of 2D Patterns. Entropy, 24(6), 802. https://doi.org/10.3390/e24060802








