Constructal Optimizations of Line-to-Line Vascular Channels with Turbulent Convection Heat Transfer
Abstract
:1. Introduction
2. Geometric Model of LVCs
3. Constructal Optimizations of LVCs with Minimum EGR
3.1. Constructal Optimizations of the First Order LVCs
3.2. Constructal Optimizations of the Second Order LVCs
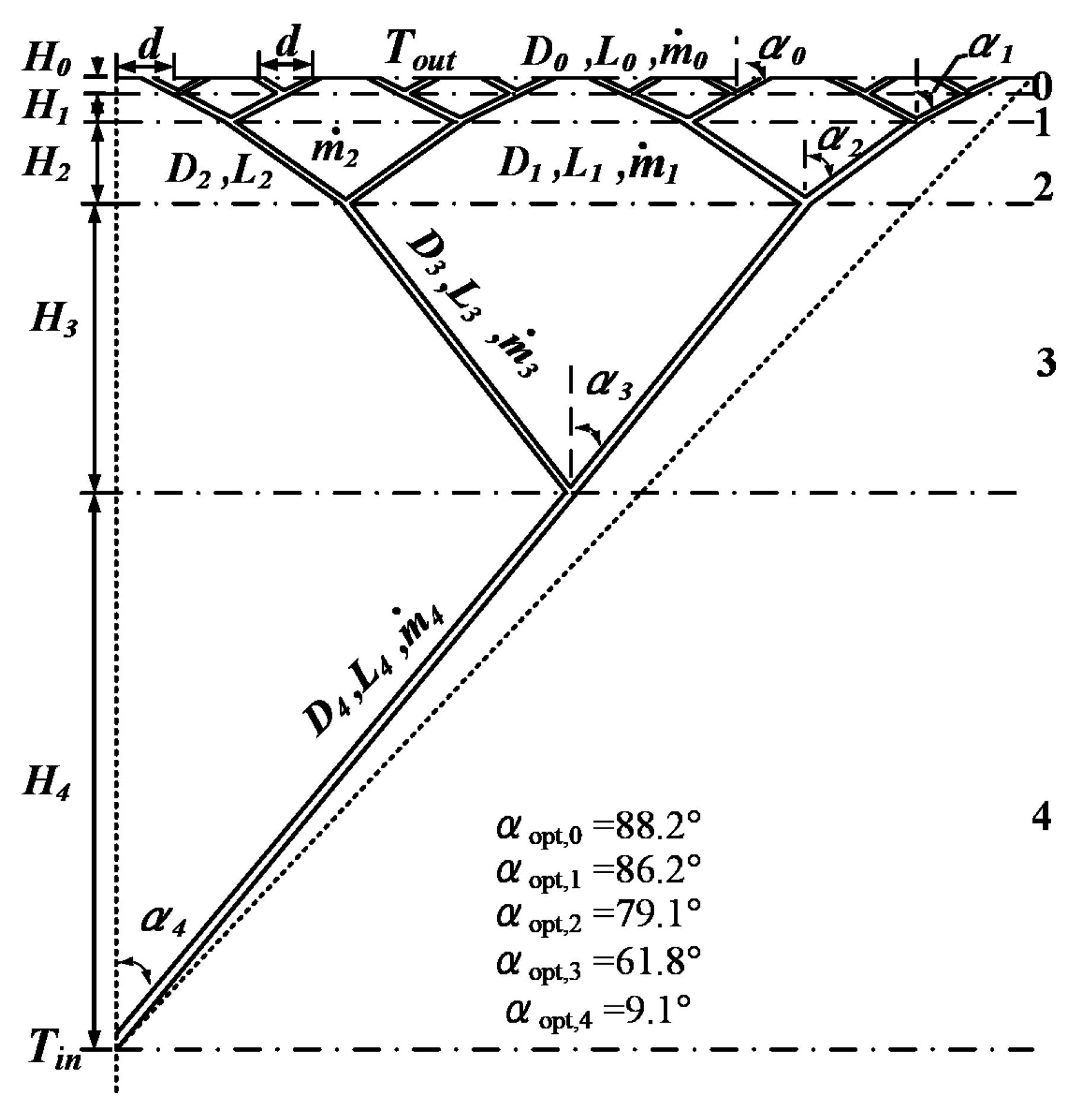
3.3. Constructal Optimizations of the Third and Higher Order LVCs
4. Effects of the Dimensionless Mass Flow Rate on Constructal Optimizations, Dimensionless Total Entropy Generation Rate and EGN
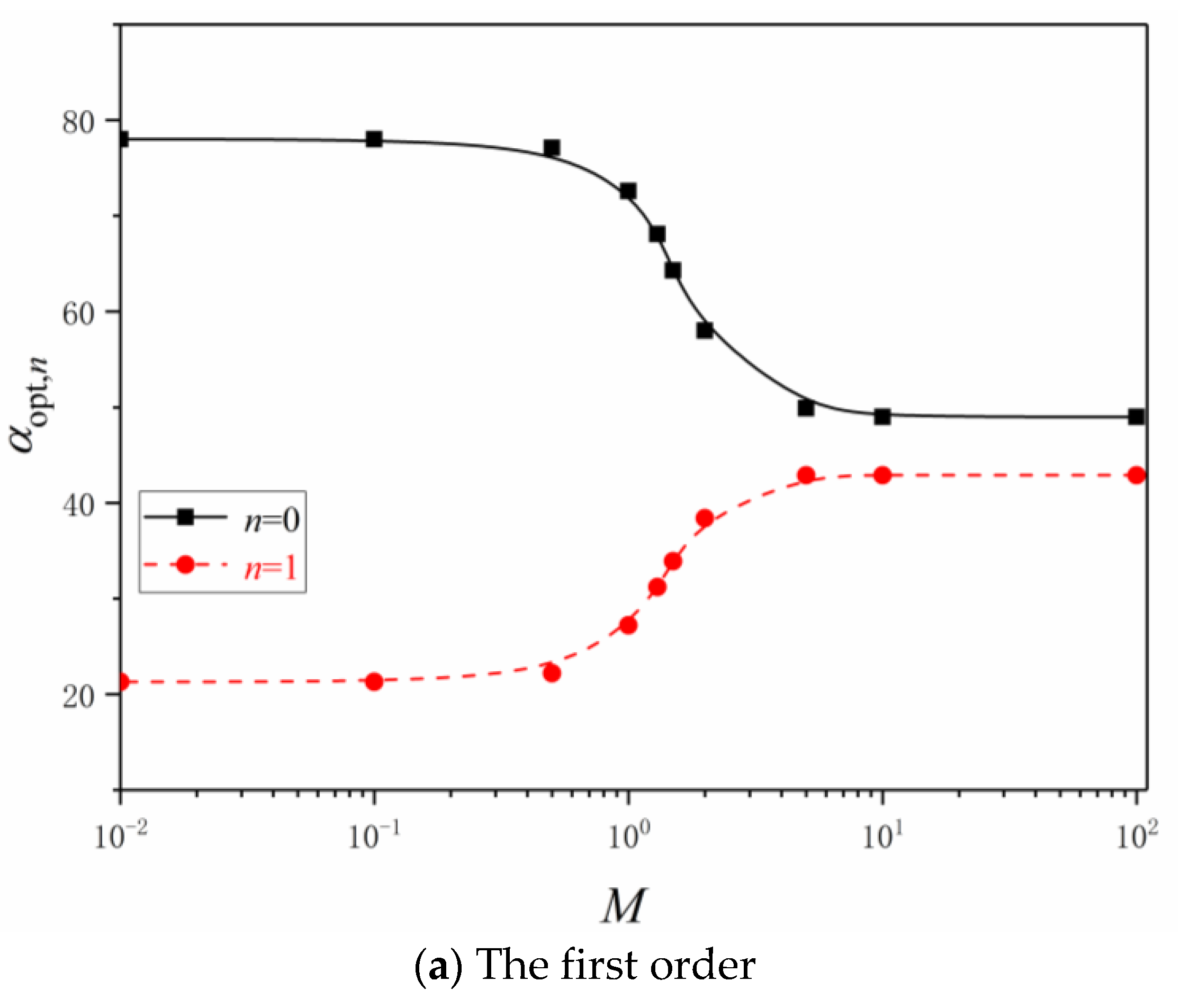
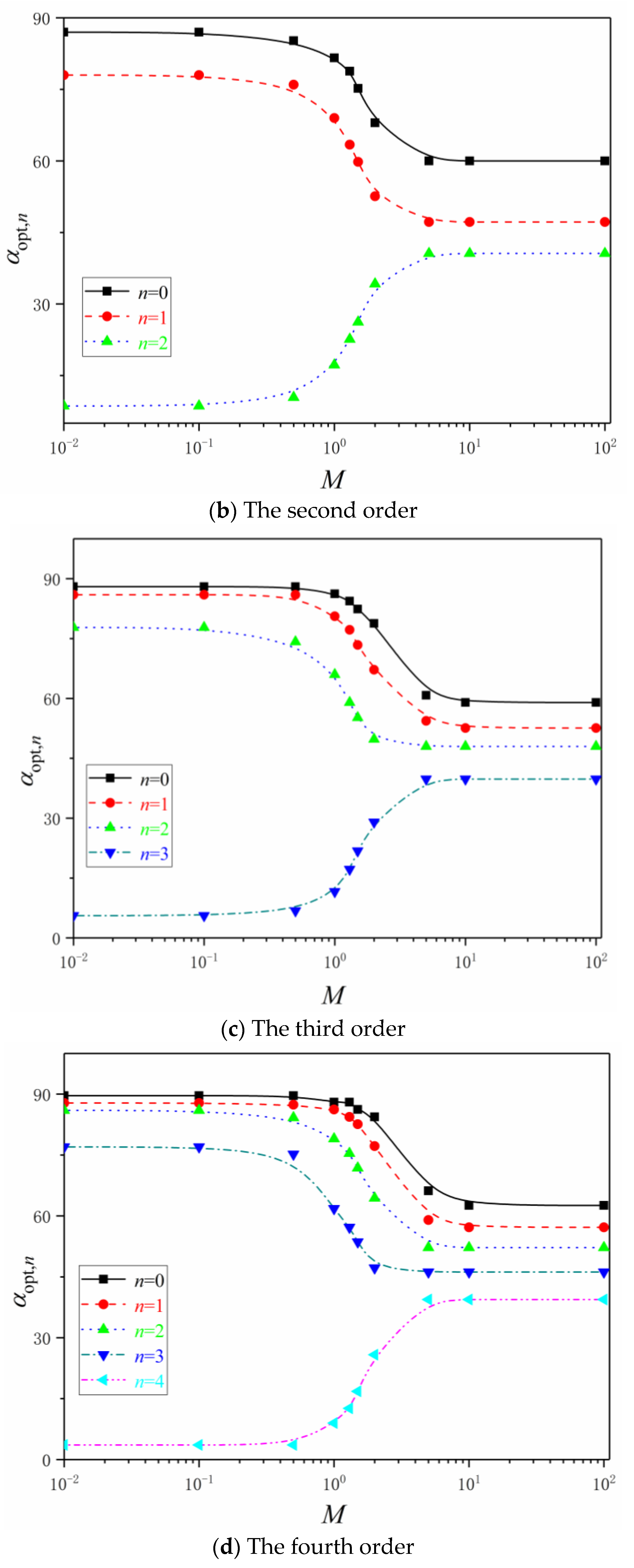
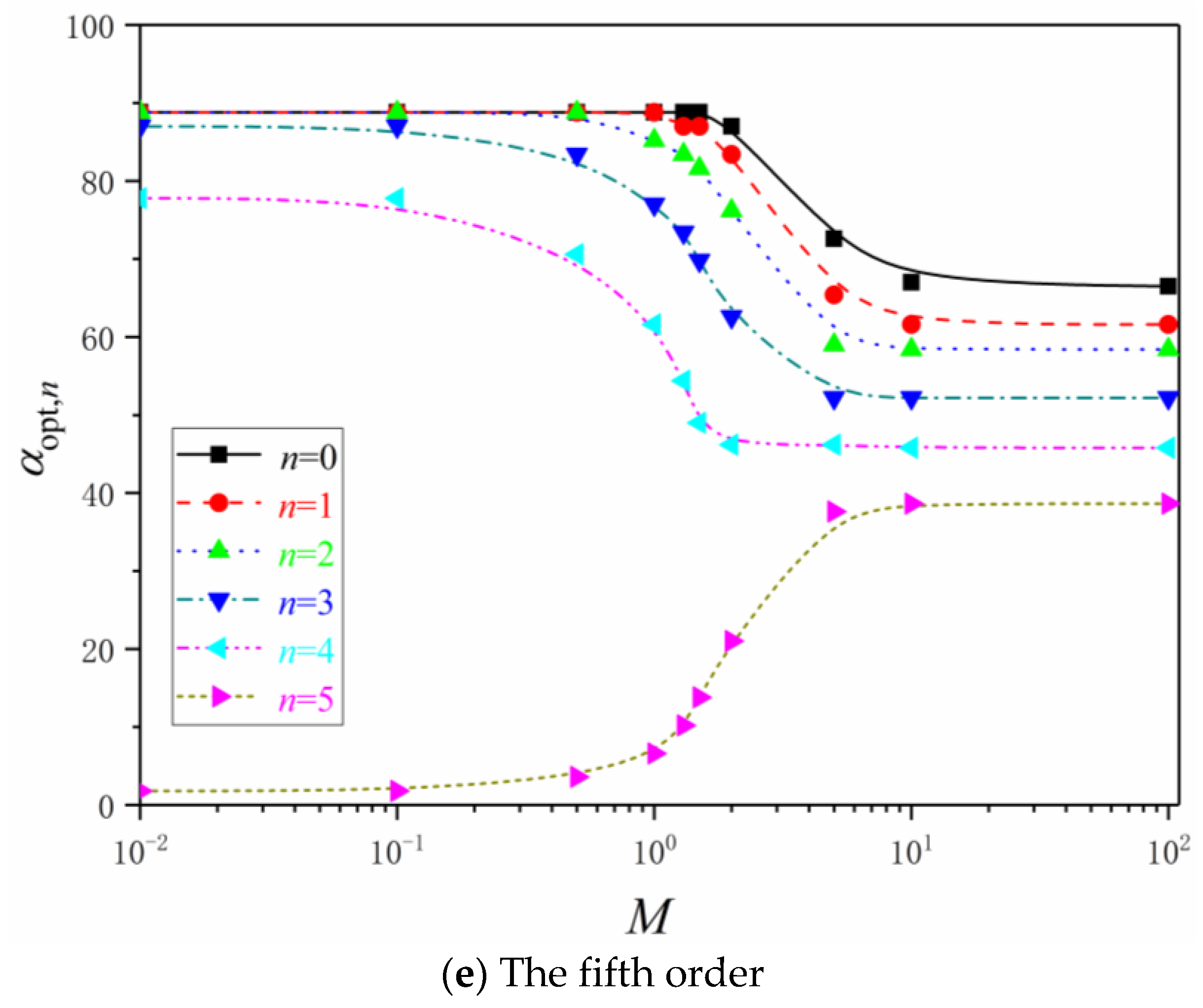
4.1. Effects of the Dimensionless Mass Flow Rate on Constructal Optimizations
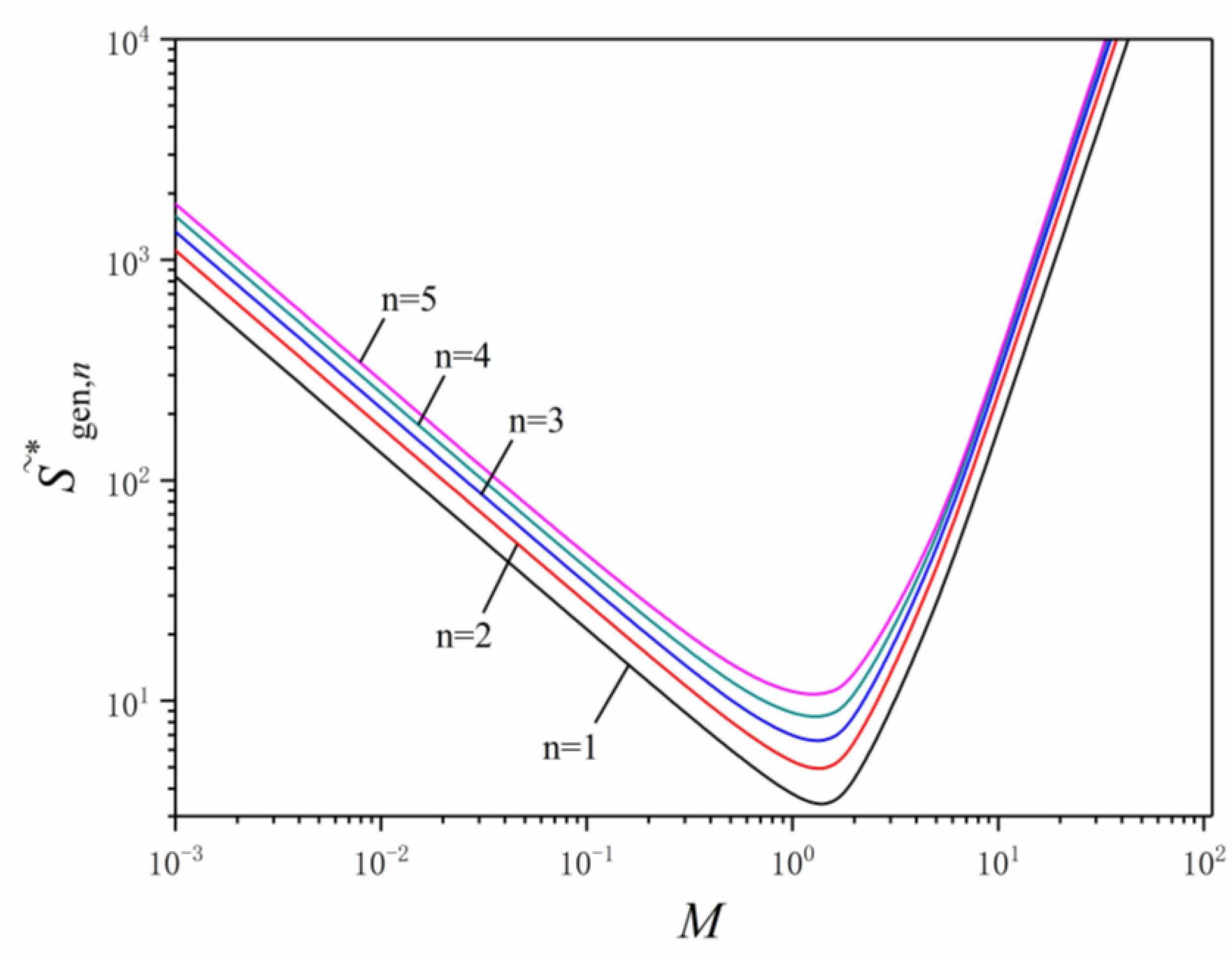
4.2. Effects of the Dimensionless Mass Flow Rate on the Dimensionless Total Entropy Generation Rate
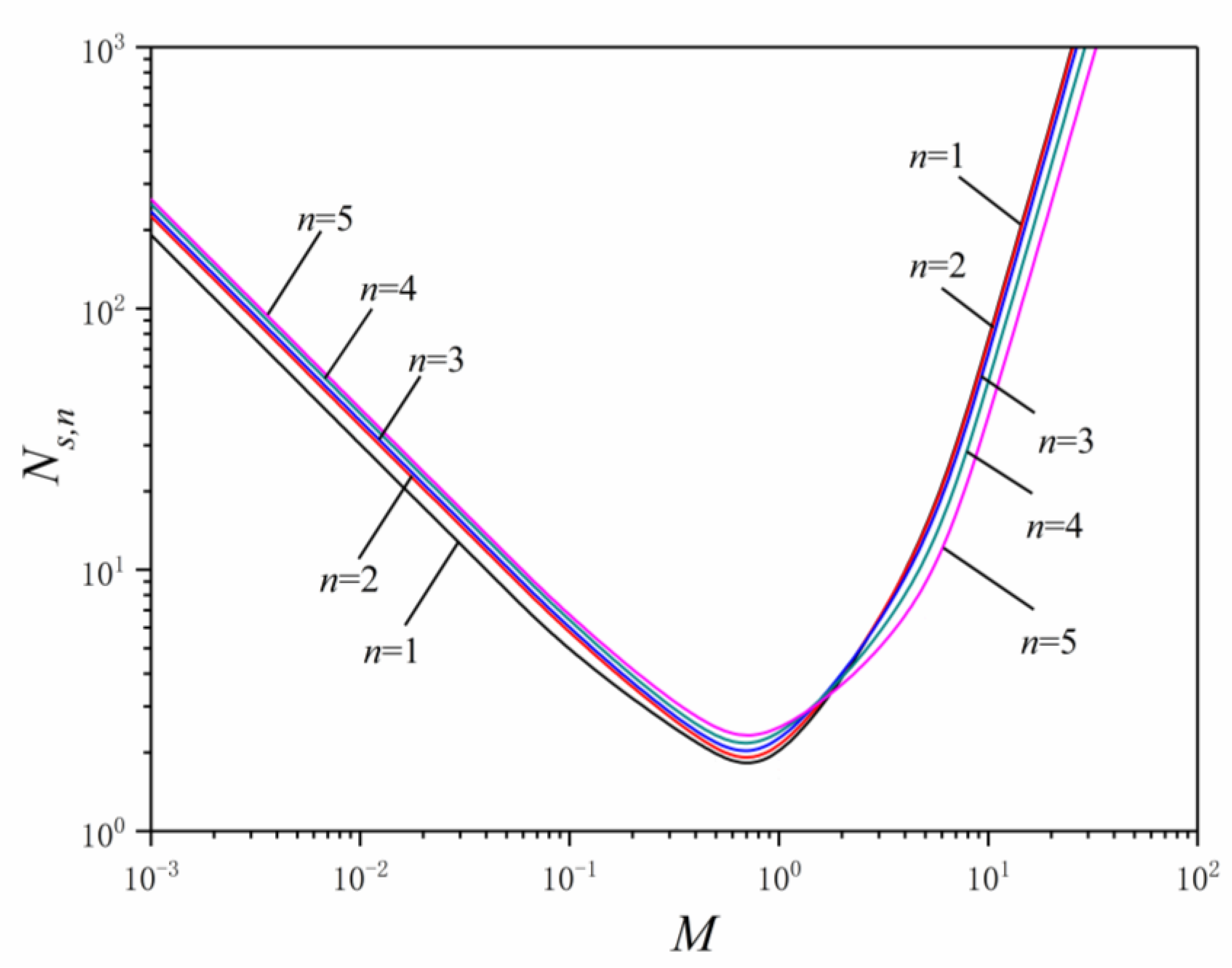
4.3. Effects of the Dimensionless Mass Flow Rate on EGN
5. Conclusions
- (1)
- The dimensionless total entropy generation rate of LVCs with any order can be significantly decreased by optimizing the angles of LVCs. From the first to fifth order, the dimensionless total entropy generation rate of LVCs with optimal angles were 10.65%, 24.54%, 43.75%, 66.99% and 93.67% smaller than those with fixed angles (), respectively. As the order of LVCs is higher, the dimensionless total entropy generation rate of LVCs decreases significantly more.
- (2)
- Based on the minimum dimensionless total entropy generation rate, as the dimensionless mass flow rate increases, the optimal angles of LVCs with any order remain unchanged first, then the optimal angles of LVCs at the entrance increase, and the other optimal angles of LVCs decrease continuously and finally tend to respective stable values. The optimal angles of LVCs continue to increase from the entrance to the outlet, i.e., the LVCs with a certain order gradually spread out from the root to the crown.
- (3)
- As the dimensionless mass flow rate increases, the dimensionless total entropy generation rate and EGN of LVCs with turbulent convection heat transfer decrease first and then increase sharply. There is optimal dimensionless mass flow rate can make the dimensionless total entropy generation rate and EGN of LVCs with any order obtain their respective minimums.
- (4)
- The dimensionless total entropy generation rate of LVCs increases gradually as the order of LVC increases for the same dimensionless mass flow rate . When the dimensionless mass flow rate is less than 2, the EGN of LVCs increases as the order of LVC increases; however, when the dimensionless mass flow rate is greater than 2, this is simply reversed.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclatures
| areas (m2) | |
| coefficient | |
| coefficient | |
| D | diameter (m) |
| d | distance between adjacent outlets (m) |
| H | height (m) |
| k | thermal conductivity (W·(m·K)−1) |
| L | length (m) |
| dimensionless mass flow rate | |
| mass flow rate (kg·s−1) | |
| dimensionless entropy generation number | |
| linear heat flux (W·m−1) | |
| entropy generation rate (J·(K·s)−1) | |
| dimensionless entropy generation rate | |
| T | temperature (K) |
| V | volume (m3) |
| Greek letters | |
| angel (°) | |
| density (kg·m−3) | |
| kinematic viscosity (m·s−2) | |
| Superscripts | |
| ~ | dimensionless |
| transform of physical quantity | |
| Subscripts | |
| i | channel rank |
| in | inlet |
| n | number of construction orders |
| out | outlet |
| opt | optimal |
| Abbreviation | |
| EGN | entropy generation number |
| EGR | entropy generation rate |
| LVC | line-to-line vascular channel |
| Nu | Nusselt number |
| Pr | Prandtl number |
References
- Van Erp, R.; Soleimanzadeh, R.; Nela, L.; Georgios, K.; Elison, M. Co-designing electronics with microfluidics for more sustainable cooling. Nature 2020, 585, 211–216. [Google Scholar] [CrossRef] [PubMed]
- Ahmed, H.E.; Salman, B.H.; Kherbeet, A.S.; Ahmed, M.I. Optimization of thermal design of heat sinks: A review. Int. J. Heat Mass Transf. 2018, 118, 129–153. [Google Scholar] [CrossRef]
- Xiao, H.; Liu, Z.C.; Liu, W. Conjugate heat transfer enhancement in the mini-channel heat sink by realizing the optimized flow pattern. Appl. Therm. Eng. 2021, 182, 116131. [Google Scholar] [CrossRef]
- Khoshvaght-Aliabadi, M.; Hosseinirad, E.; Farsi, M.; Hormozi, F. Heat transfer and flow characteristics of novel patterns of chevron minichannel heat sink: An insight into thermal management of microelectronic devices. Int. Comm. Heat Mass Transf. 2021, 122, 105044. [Google Scholar] [CrossRef]
- Bejan, A. Street network theory of organization in nature. J. Adv. Transp. 1996, 30, 85–107. [Google Scholar] [CrossRef]
- Bejan, A. Constructal-theory network of conducting paths for cooling a heat generating volume. Int. J. Heat Mass Tranf. 1997, 40, 799–816. [Google Scholar] [CrossRef]
- Bejan, A. Fundamentals of exergy analysis, entropy generation minimization, and the generation of flow architecture. Int. J. Energy Res. 2002, 26, 545–565. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S. Constructal theory of generation of configuration in nature and engineering. J. Appl. Phys. 2006, 100, 041301. [Google Scholar] [CrossRef] [Green Version]
- Bejan, A.; Lorente, S. Constructal tree-shaped flow structures. Appl. Therm. Eng. 2007, 27, 755–761. [Google Scholar] [CrossRef]
- Chen, L.G. Progress in study on constructal theory and its applications. Sci. China Technol. Sci. 2012, 55, 802–820. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S. Constructal law of design and evolution: Physics, biology, technology, and society. J. Appl. Phys. 2013, 113, 151301. [Google Scholar] [CrossRef] [Green Version]
- Bejan, A.; Errera, M.R. Complexity, organization, evolution, and constructal law. J. Appl. Phys. 2016, 119, 074901. [Google Scholar] [CrossRef]
- Chen, L.G.; Feng, H.J.; Xie, Z.H. Generalized thermodynamic optimization for iron and steel production processes: Theoretical exploration and application cases. Entropy 2016, 18, 353. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Feng, H.J. Multi-Objective Constructal Optimization for Flow and Heat and Mass Transfer Processes; Science Press: Beijing, China, 2016. (In Chinese) [Google Scholar]
- Lorente, S. The constructal law: From microscale to urban-scale design. Annual Rev. Heat Transf. 2017, 19, 335–368. [Google Scholar] [CrossRef]
- Miguel, A.F.; Rocha, L.A.O. Tree-Shaped Fluid Flow and Heat Transfer; Springer: New York, NY, USA, 2018. [Google Scholar]
- Feng, H.J.; Chen, L.G.; Xie, Z.H. Multi-disciplinary, multi-objective and multi-scale constructal optimizations for heat and mass transfer processes performed in Naval University of Engineering, a review. Int. J. Heat Mass Tranf. 2017, 115, 86–98. [Google Scholar] [CrossRef]
- Chen, L.G.; Feng, H.J.; Xie, Z.H.; Sun, F.R. Progress of constructal theory in China over the past decade. Int. J. Heat Mass Tranf. 2019, 130, 393–419. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Parsa, H.; Najafian, J. Proposing an optimal tree-like design of highly conductive material configuration with unequal branches for maximum cooling a heat generating piece. Int. J. Heat Mass Tranf. 2019, 142, 118422. [Google Scholar] [CrossRef]
- Estrada, E.S.D.; Barreto, E.X.; Isoldi, L.A.; dos Santos, E.D.; Lorente, S.; Rocha, L.A.O. Constructal design of tree shaped cavities inserted into a cylindrical body with heat generation. Int. J. Therm. Sci. 2020, 152, 106342. [Google Scholar] [CrossRef]
- Chen, L.G.; Wu, W.J.; Feng, H.J. Constructal Design for Heat Conduction; Book Publisher International: London, UK, 2021. [Google Scholar]
- Razera, A.L.; da Fonseca, R.J.C.; Isoldi, L.A.; dos Santos, E.D.; Rocha, L.A.O.; Biserni, C.A. Constructal approach applied to the cooling of semi-elliptical blocks assembled into a rectangular channel under forced convection. Int. J. Heat Mass Transf. 2022, 184, 122293. [Google Scholar] [CrossRef]
- Gong, S.W.; Chen, L.G.; Feng, H.J.; Xie, Z.H.; Sun, F.R. Constructal optimization of cylindrical heat sources surrounded with a fin based on minimization of hot spot temperature. Int. Comm. Heat Mass Transf. 2015, 68, 1–7. [Google Scholar] [CrossRef]
- Aslan, Y.; Puskely, J.; Yarovoy, A. Heat source layout optimization for two-dimensional heat conduction using iterative reweighted L1-norm convex minimization. Int. J. Heat Mass Transf. 2018, 122, 432–441. [Google Scholar] [CrossRef] [Green Version]
- Wang, R.; Xie, Z.H.; Yin, Y.; Chen, L.G. Constructal design of elliptical cylinders with heat generating for entropy generation minimization. Entropy 2020, 22, 651. [Google Scholar] [CrossRef]
- Birinci, S.; Saglam, M.; Sarper, B.; Aydin, O. Constructal design of heat sources with different heat generation rates for the hot spot mitigation. Int. J. Heat Mass Tranf. 2020, 163, 120472. [Google Scholar] [CrossRef]
- You, J.; Feng, H.J.; Chen, L.G.; Xie, Z.H. Heat conduction constructal optimization for nonuniform heat generation body based on triangular element. Int. J. Heat Mass Tranf. 2018, 117, 896–902. [Google Scholar] [CrossRef]
- Avendaño, P.A.; Souza, J.A.; Adamatti, D.F. Construction of conductive pathways using genetic algorithms and constructal theory. Int. J. Therm. Sci. 2018, 134, 200–207. [Google Scholar] [CrossRef]
- Chen, L.G.; You, J.; Feng, H.J.; Xie, Z.H. Constructal optimization for “disc-point” heat conduction with nonuniform heat generating. Int. J. Heat Mass Tranf. 2019, 134, 1191–1198. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Rezaei, E. Proposing a new algorithm for the optimization of conduction pathways based on a recursive localization. Appl. Therm. Eng. 2019, 151, 146–153. [Google Scholar] [CrossRef]
- Bejan, A.; Errera, M.R. Convective trees of fluid channels for volumetric cooling. Int. J. Heat Mass Transf. 2000, 43, 3105–3118. [Google Scholar] [CrossRef]
- Bello-Ochende, T.; Olakoyejo, O.T.; Meyer, J.P.; Bejan, A.; Lorente, S. Constructal flow orientation in conjugate cooling channels with internal heat generation. Int. J. Heat Mass Transf. 2013, 57, 241–249. [Google Scholar] [CrossRef] [Green Version]
- Zou, H.W.; Chen, Y.H.; Zhang, J.; Tang, Z.Y.; Wu, Q. Characteristics of liquid-liquid two-phase flow in a constructal honeycomb reactor. Chem. Eng. J. 2021, 409, 128179. [Google Scholar] [CrossRef]
- Mirzaei, M.; Hajabdollahi, H.; Fadakar, H. Multi-objective optimization of shell-and-tube heat exchanger by constructal theory. Appl. Therm. Eng. 2017, 125, 9–19. [Google Scholar] [CrossRef]
- Xie, Z.J.; Feng, H.J.; Chen, L.G.; Wu, Z.X. Constructal design for supercharged boiler evaporator. Int. J. Heat Mass Transf. 2019, 138, 571–579. [Google Scholar] [CrossRef]
- Hajabdollahi, H. Multi-objective optimization of plate fin heat exchanger using constructal theory. Int. Comm. Heat Mass Transf. 2019, 108, 104283. [Google Scholar] [CrossRef]
- Ganjehkaviri, A.; Mohd Jaafar, M.N. Multi-objective particle swarm optimization of flat plate solar collector using constructal theory. Energy 2020, 194, 116846. [Google Scholar] [CrossRef]
- Feng, H.J.; Xie, Z.J.; Chen, L.G.; Wu, Z.X.; Xia, S.J. Constructal design for supercharged boiler superheater. Energy 2020, 191, 116484. [Google Scholar] [CrossRef]
- Lorente, S.; Bejan, A. Heterogeneous porous media as multiscale structures for maximum flow access. J. Appl. Phys. 2006, 100, 114909. [Google Scholar] [CrossRef]
- Zhang, H.; Lorente, S.; Bejan, A. Vascularization with line-to-line trees in counterflow heat exchange. Int. J. Heat Mass Tranf. 2009, 52, 4327–4342. [Google Scholar] [CrossRef]
- Xie, Z.H.; Chen, L.G.; Sun, F.R. Constructal entropy generation rate minimization of line-to-line vascular networks with convective heat transfer. Int. J. Therm. Sci. 2013, 74, 72–80. [Google Scholar] [CrossRef]
- Yenigun, O.; Cetkin, E. Experimental and numerical investigation of constructal vascular channels for self-cooling: Parallel channels, tree-shaped and hybrid designs. Int. J. Heat Mass Tranf. 2016, 103, 1155–1165. [Google Scholar] [CrossRef] [Green Version]
- Fan, X.D.; Xie, Z.H.; Sun, F.R.; Chen, L.G. Convective heat transfer characteristics of line-to-line vascular microchannel heat sink with temperature-dependent fluid properties. Appl. Therm. Eng. 2016, 93, 606–613. [Google Scholar] [CrossRef]
- Jing, D.L.; Song, S.Y.; He, L. Reexamination of Murray’s law for tree-like rectangular microchannel network with constant channel height. Int. J. Heat Mass Transf. 2019, 128, 1344–1350. [Google Scholar] [CrossRef]
- Lorente, S.; Hautefeuille, M.; Sanchez-Cedillo, A. The liver, a functionalized vascular. Sci. Rep. 2020, 10, 16194. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Lorente, S.; Bejan, A. Vascularized materials: Tree-shaped flow architectures matched canopy to canopy. J. Appl. Phys. 2006, 100, 063525. [Google Scholar] [CrossRef]
- Wang, K.M.; Lorente, S.; Bejan, A. Vascularized networks with two optimized channel sizes. J. Phys. D Appl. Phys. 2006, 39, 3086–3096. [Google Scholar] [CrossRef]
- Wang, K.M.; Lorente, S.; Bejan, A. Vascularization with grids of channels: Multiple scales, loops and body shapes. J. Phys. D Appl. Phys. 2007, 40, 4740–4749. [Google Scholar] [CrossRef]
- Lee, J.; Kim, S.; Lorente, S.; Bejan, A. Vascularization with trees matched canopy to canopy: Diagonal channels with multiple sizes. Int. J. Heat Mass Transf. 2007, 51, 2029–2040. [Google Scholar] [CrossRef]
- Liu, H.L.; An, X.K.; Wang, C.S. Heat transfer performance of T-Y type micro-channel heat sink with liquid GaInSn coolant. Int. J. Therm. Sci. 2017, 120, 203–219. [Google Scholar] [CrossRef]
- Miguel, A.F. Constructal branching design for fluid flow and heat transfer. Int. J. Heat Mass Transf. 2018, 122, 204–211. [Google Scholar] [CrossRef]
- Hu, L.G.; Wang, D.T.; Zhu, H.X.; Fan, T.X. Extended efficient network-matrix model inspired by natural palmate leaves. Int. J. Heat Mass Transf. 2018, 119, 20–25. [Google Scholar] [CrossRef]
- Jing, D.L.; Song, J. Comparison on the hydraulic and thermal performances of two tree-like channel networks with different size constraints. Int. J. Heat Mass Transf. 2019, 130, 1070–1074. [Google Scholar] [CrossRef]
- Lu, Z.H.; Zhang, K.; Liu, J.X.; Li, F. Effect of branching level on the performance of constructal theory based Y-shaped liquid cooling heat sink. Appl. Therm. Eng. 2020, 168, 114824. [Google Scholar] [CrossRef]
- Wechsatol, W.; Lorente, S.; Bejan, A. Tree-shaped flow structures with local junction losses. Int. J. Heat Mass Transf. 2006, 49, 2957–2964. [Google Scholar] [CrossRef]
- Zimparov, V.D.; da Silva, A.K.; Bejan, A. Thermodynamic optimization of tree-shaped flow geometries. Int. J. Heat Mass Transf. 2006, 49, 1619–1630. [Google Scholar] [CrossRef]
- Zimparov, V.D.; da Silva, A.K.; Bejan, A. Thermodynamic optimization of tree-shaped flow geometries with constant channel wall temperature. Int. J. Heat Mass Transf. 2006, 49, 4839–4849. [Google Scholar] [CrossRef]
- Zimparov, V.D.; Angelov, M.S.; Petkov, V.M. Performance evaluation of turbulent tree-shaped flow. Part 1: T-shaped assembles. Int. Rev. Chem. Eng. 2017, 9, 31–36. [Google Scholar]
- Zimparov, V.D.; Angelov, M.S.; Petkov, V.M. Performance evaluation of turbulent tree-shaped flow. Part 2: Y-Shaped Assembles. Int. Rev. Chem. Eng. 2017, 9, 37–42. [Google Scholar]
- Feng, H.J.; Chen, L.G.; Xie, Z.H.; Sun, F.R. Constructal entropy generation rate minimization for asymmetric vascular networks in a disc-shaped body. Int. J. Heat Mass Tranf. 2015, 91, 1010–1017. [Google Scholar] [CrossRef]
- Feng, H.J.; Chen, L.G.; Xie, Z.H.; Sun, F.R. Constructal entropy generation rate minimization for X-shaped vascular networks. Int. J. Therm. Sci. 2015, 92, 129–137. [Google Scholar] [CrossRef]
- Miguel, A.F. Heat transfer in flow through branching tubes. Int. J. Hydromechatronics 2018, 1, 172–181. [Google Scholar] [CrossRef]
- Miguel, A.F. Optimal Y-shaped constructs heat sinks under different size constraints. Int. J. Heat Mass Transf. 2019, 131, 64–71. [Google Scholar] [CrossRef]
- Shi, H.N.; Xie, Z.H.; Chen, L.G.; Sun, F.R. Constructal optimization for line-to-line vascular based on entropy generation minimization principle. Int. J. Heat Mass Transf. 2018, 126, 848–854. [Google Scholar] [CrossRef]

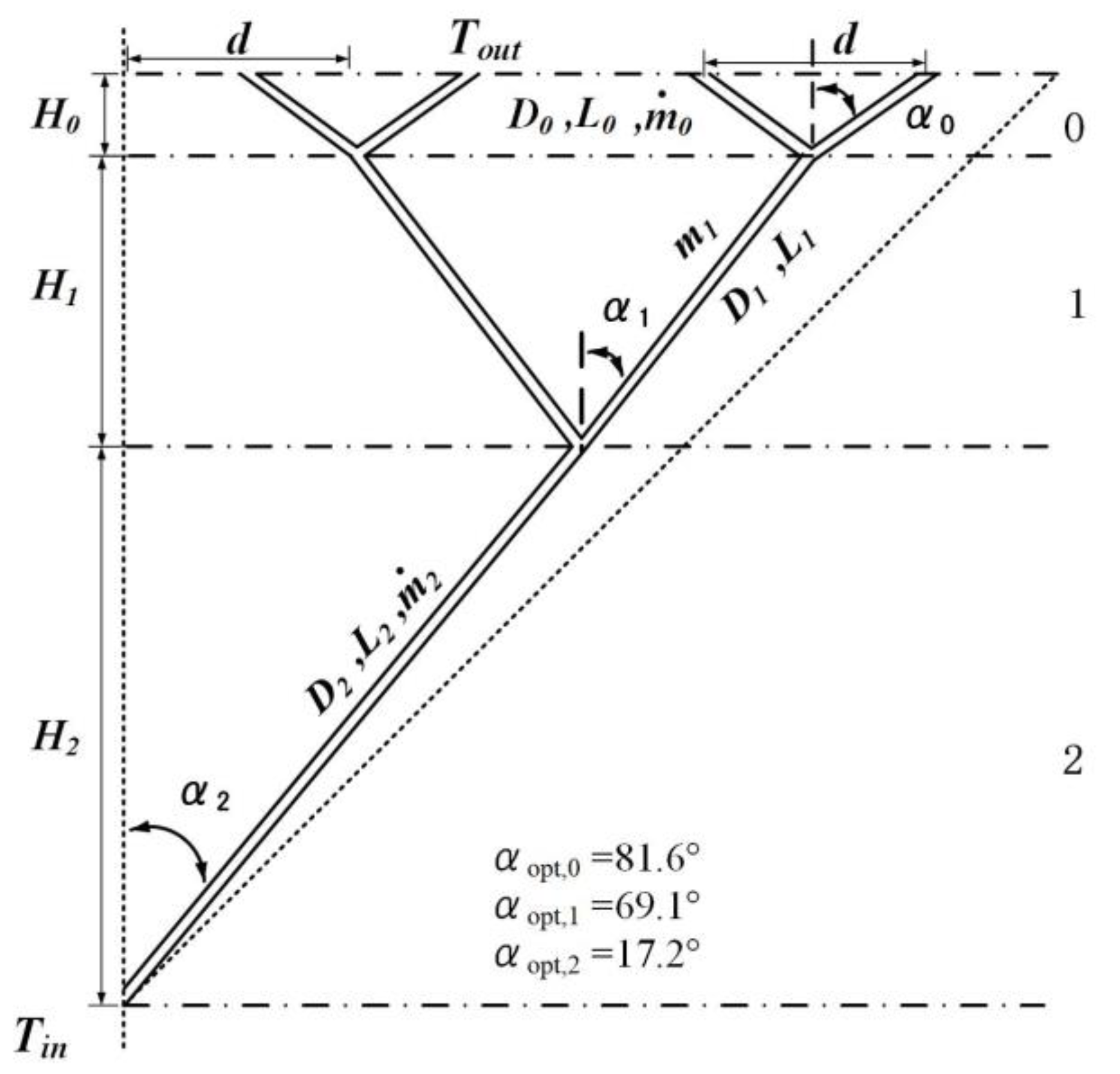

| 78.0 | 21.3 | ||||||
| 87.0 | 78.0 | 8.6 | |||||
| 88.0 | 86.0 | 77.8 | 5.6 | ||||
| 88.6 | 87.8 | 86.0 | 77.0 | 3.6 | |||
| 88.8 | 88.8 | 88.8 | 87.0 | 77.8 | 1.8 | ||
| 72.6 | 27.2 | ||||||
| 81.6 | 69.1 | 17.2 | |||||
| 86.2 | 80.6 | 66.1 | 11.6 | ||||
| 88.2 | 86.2 | 79.1 | 61.8 | 9.1 | |||
| 88.8 | 88.8 | 85.2 | 77.1 | 61.6 | 6.6 | ||
| 49.9 | 42.9 | ||||||
| 60.0 | 47.2 | 40.6 | |||||
| 60.8 | 54.4 | 48 | 39.8 | ||||
| 66.2 | 59.0 | 52.2 | 46.2 | 39.4 | |||
| 72.6 | 65.4 | 59.0 | 52.2 | 46.2 | 37.6 |
| 23.918 | 24.973 | 29.448 | 21.105 | 4.059 | 4.301 | 5.539 | 3.701 | 25.373 | 32.158 | 80.325 | 25.215 | |
| 38.356 | 40.048 | 47.223 | 27.710 | 6.468 | 6.843 | 8.747 | 5.193 | 36.880 | 46.706 | 116.439 | 36.407 | |
| 58.785 | 61.377 | 72.374 | 33.715 | 9.788 | 10.330 | 13.003 | 6.809 | 45.311 | 57.264 | 142.027 | 44.202 | |
| 87.645 | 91.510 | 107.905 | 39.582 | 14.417 | 15.175 | 18.810 | 8.633 | 51.473 | 64.840 | 159.503 | 49.689 | |
| 128.261 | 133.917 | 157.910 | 45.052 | 20.888 | 21.939 | 26.845 | 10.785 | 56.317 | 70.612 | 171.657 | 53.726 | |
| 10.357 | 10.063 | 9.017 | 4.807 | 1.758 | 1.733 | 1.696 | 1.585 | 11.528 | 13.553 | 25.672 | 10.987 | |
| 11.960 | 11.621 | 10.412 | 5.656 | 2.017 | 1.986 | 1.928 | 1.711 | 11.499 | 12.958 | 24.594 | 10.937 | |
| 14.230 | 13.826 | 12.388 | 5.904 | 2.369 | 2.327 | 2.226 | 1.871 | 10.968 | 12.910 | 24.311 | 10.089 | |
| 17.253 | 16.764 | 15.020 | 6.303 | 2.838 | 2.780 | 2.618 | 2.061 | 10.133 | 11.878 | 22.202 | 8.217 | |
| 21.209 | 20.608 | 18.464 | 6.579 | 3.454 | 3.376 | 3.139 | 2.217 | 9.313 | 10.866 | 20.071 | 6.528 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, D.; Xie, Z.; Nan, G.; Jiang, P.; Ge, Y. Constructal Optimizations of Line-to-Line Vascular Channels with Turbulent Convection Heat Transfer. Entropy 2022, 24, 999. https://doi.org/10.3390/e24070999
Lin D, Xie Z, Nan G, Jiang P, Ge Y. Constructal Optimizations of Line-to-Line Vascular Channels with Turbulent Convection Heat Transfer. Entropy. 2022; 24(7):999. https://doi.org/10.3390/e24070999
Chicago/Turabian StyleLin, Daoguang, Zhihui Xie, Gang Nan, Pan Jiang, and Yanlin Ge. 2022. "Constructal Optimizations of Line-to-Line Vascular Channels with Turbulent Convection Heat Transfer" Entropy 24, no. 7: 999. https://doi.org/10.3390/e24070999
APA StyleLin, D., Xie, Z., Nan, G., Jiang, P., & Ge, Y. (2022). Constructal Optimizations of Line-to-Line Vascular Channels with Turbulent Convection Heat Transfer. Entropy, 24(7), 999. https://doi.org/10.3390/e24070999






