Synchronization Induced by Layer Mismatch in Multiplex Networks
Abstract
:1. Introduction
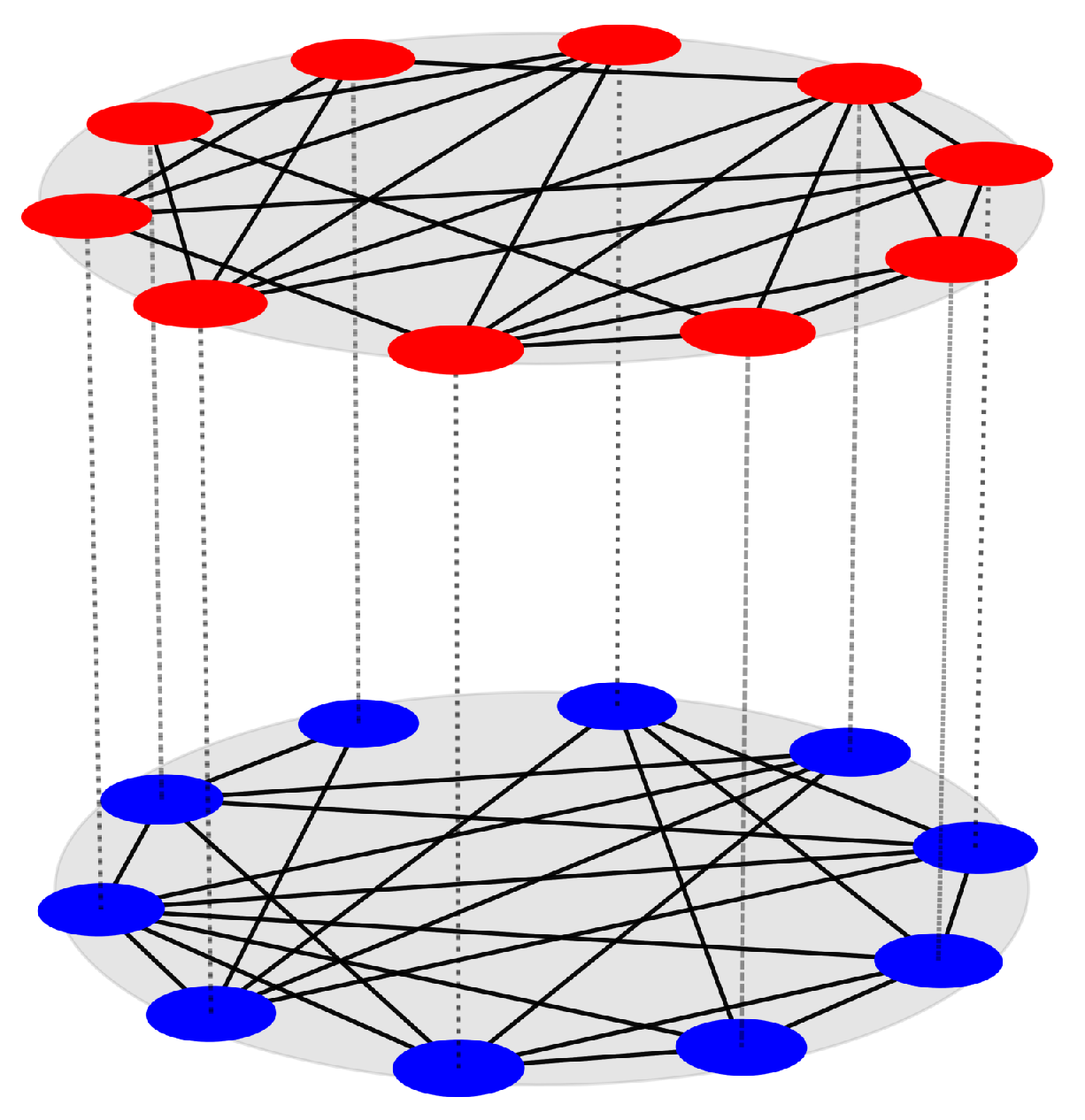
2. Mathematical Model
3. Results
3.1. Analytical Results
3.1.1. Invariance Condition
3.1.2. Stability Analysis
- If the connectivity structure of all the layers is identical, then all of them share the same Laplacian matrix. Without loss of generality, we choose the Laplacian matrix to be . Then, the master stability equation becomes
- If , i.e., the total number of layers is two, and the corresponding Laplacian matrices are and . Furthermore, suppose that the intralayer connection in any one layer is globally coupled—for example, say layer-1 is globally coupled—then the eigenvalues of the corresponding Laplacian matrix are 0 with algebraic multiplicity 1 and N with algebraic multiplicity . Then, in this scenario, the master stability Equation (11) can be fully decoupled into a low dimensional form, by projecting the transverse error components onto the basis of eigenvectors of , which eventually gives the decoupled master stability equation as
- Using a similar concept as in 2, we can infer that if the connectivity structures of the layers of our multiplex framework are such that the Laplacian matrix of each layer is either or , and is the Laplacian matrix associated with globally connected networks, then the master stability equation can be fully decoupled into a lower dimensional form. For example, we say that the first number of layers have an identical Laplacian matrix , and that number of layers have identical Laplacian matrices . Then, following 2, the master stability equation can be represented as
- When none of the intralayer connectivity structure is globally coupled but the Laplacian matrices and are commutative with each other, then also the master stability equation can be reduced to a lower dimensional form, because the commutative Laplacian matrices share the same set of the basis of eigenvectors that diagonalizes them. Hence, the reduced master stability equation becomes
3.1.3. Simultaneous Achievement of Layer-Wise Synchronization
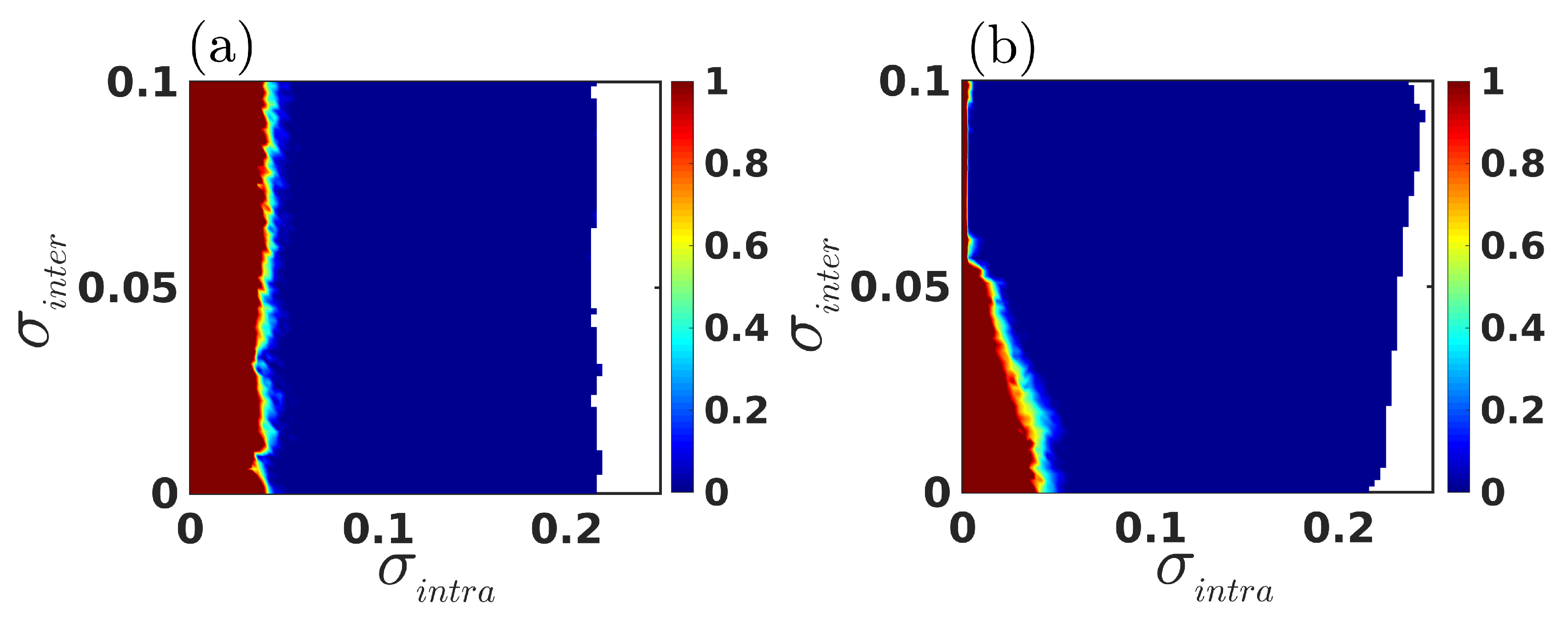
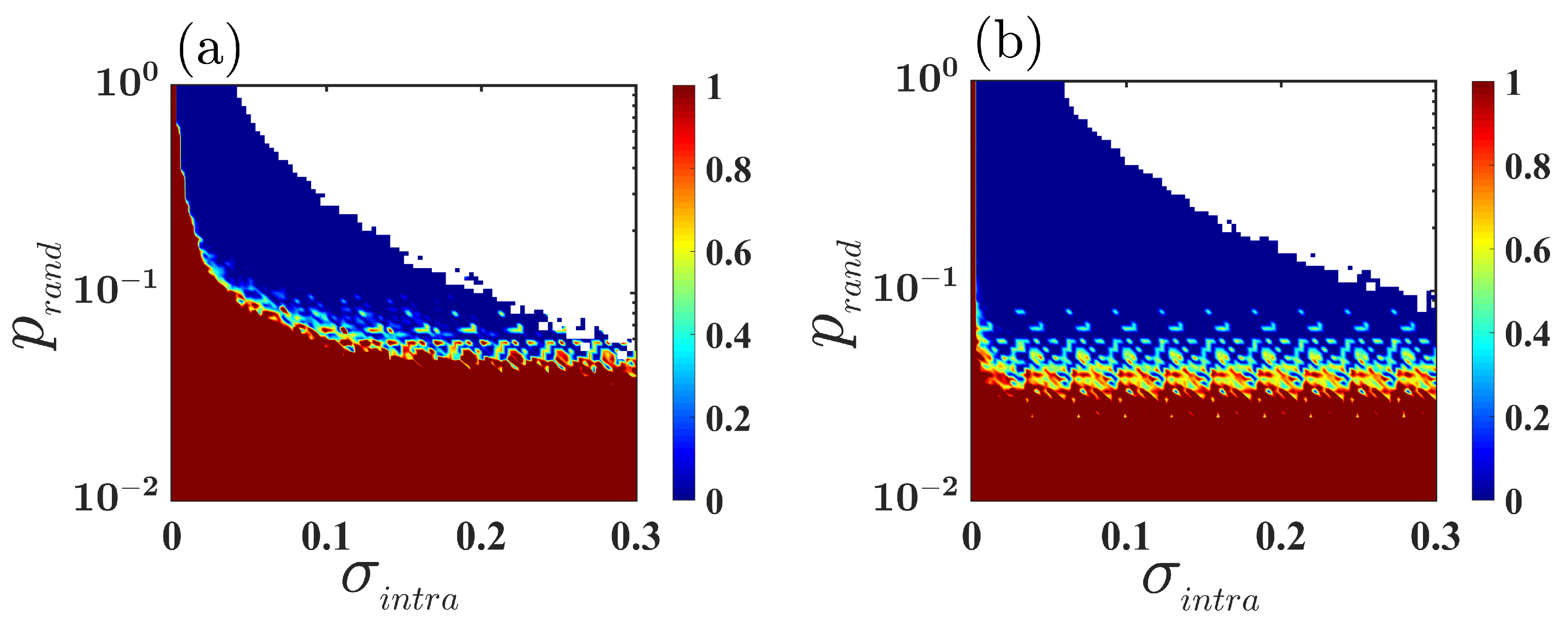
3.2. Numerical Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Newman, M. Networks; Oxford University Press: Oxford, UK, 2018. [Google Scholar]
- Latora, V.; Nicosia, V.; Russo, G. Complex Networks: Principles, Methods and Applications; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Watts, D.J.; Strogatz, S.H. Collective dynamics of ‘small-world’networks. Nature 1998, 393, 440–442. [Google Scholar] [CrossRef]
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
- Newman, M.E. The structure and function of complex networks. SIAM Rev. 2003, 45, 167–256. [Google Scholar] [CrossRef] [Green Version]
- Strogatz, S.H. Exploring complex networks. Nature 2001, 410, 268–276. [Google Scholar] [CrossRef] [Green Version]
- Kivelä, M.; Arenas, A.; Barthelemy, M.; Gleeson, J.P.; Moreno, Y.; Porter, M.A. Multilayer networks. J. Complex Netw. 2014, 2, 203–271. [Google Scholar] [CrossRef]
- Bianconi, G. Multilayer Networks: Structure and Function; Oxford University Press: Oxford, UK, 2018. [Google Scholar]
- Boccaletti, S.; Bianconi, G.; Criado, R.; Del Genio, C.I.; Gómez-Gardenes, J.; Romance, M.; Sendina-Nadal, I.; Wang, Z.; Zanin, M. The structure and dynamics of multilayer networks. Phys. Rep. 2014, 544, 1–122. [Google Scholar] [CrossRef] [Green Version]
- Cardillo, A.; Gómez-Gardenes, J.; Zanin, M.; Romance, M.; Papo, D.; Del Pozo, F.; Boccaletti, S. Emergence of network features from multiplexity. Sci. Rep. 2013, 3, 1344. [Google Scholar] [CrossRef] [Green Version]
- Brummitt, C.D.; D’Souza, R.M.; Leicht, E.A. Suppressing cascades of load in interdependent networks. Proc. Natl. Acad. Sci. USA 2012, 109, E680–E689. [Google Scholar] [CrossRef] [PubMed]
- Szell, M.; Lambiotte, R.; Thurner, S. Multirelational organization of large-scale social networks in an online world. Proc. Natl. Acad. Sci. USA 2010, 107, 13636–13641. [Google Scholar] [CrossRef]
- Pilosof, S.; Porter, M.A.; Pascual, M.; Kéfi, S. The multilayer nature of ecological networks. Nat. Ecol. Evol. 2017, 1, 0101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gomez, S.; Diaz-Guilera, A.; Gomez-Gardenes, J.; Perez-Vicente, C.J.; Moreno, Y.; Arenas, A. Diffusion dynamics on multiplex networks. Phys. Rev. Lett. 2013, 110, 028701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Battiston, F.; Nicosia, V.; Latora, V. Structural measures for multiplex networks. Phys. Rev. E 2014, 89, 032804. [Google Scholar] [CrossRef] [Green Version]
- Lee, K.M.; Min, B.; Goh, K.I. Towards real-world complexity: An introduction to multiplex networks. Eur. Phys. J. B 2015, 88, 48. [Google Scholar] [CrossRef] [Green Version]
- Barrat, A.; Barthelemy, M.; Vespignani, A. Dynamical Processes on Complex Networks; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Dorogovtsev, S.N.; Goltsev, A.V.; Mendes, J.F. Critical phenomena in complex networks. Rev. Mod. Phys. 2008, 80, 1275. [Google Scholar] [CrossRef] [Green Version]
- Nicosia, V.; Skardal, P.S.; Arenas, A.; Latora, V. Collective phenomena emerging from the interactions between dynamical processes in multiplex networks. Phys. Rev. Lett. 2017, 118, 138302. [Google Scholar] [CrossRef] [Green Version]
- Pikovsky, A.; Kurths, J.; Rosenblum, M. Synchronization: A Universal Concept in Nonlinear Sciences; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Strogatz, S. Sync: The emerging science of spontaneous order. Phys. Today 2004, 41, 172. [Google Scholar]
- Pecora, L.M.; Carroll, T.L. Synchronization in chaotic systems. Phys. Rev. Lett. 1990, 64, 821. [Google Scholar] [CrossRef]
- Gómez-Gardenes, J.; Moreno, Y.; Arenas, A. Paths to synchronization on complex networks. Phys. Rev. Lett. 2007, 98, 034101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, X.; Wan, X.; Zunshui, C.; Cao, J.; Liu, Y.; Rutkowski, L. Synchronization of switched discrete-time neural networks via quantized output control with actuator fault. IEEE Trans. Neural Netw. Learn. Syst. 2020, 32, 4191–4201. [Google Scholar] [CrossRef]
- Wang, H.; Yang, X.; Xiang, Z.; Tang, R.; Ning, Q. Synchronization of switched neural networks via attacked mode-dependent event-triggered control and its application in image encryption. IEEE Trans. Cybern. 2022, 1–10. [Google Scholar] [CrossRef]
- Qi, Q.; Yang, X.; Xu, Z.; Zhang, M.; Huang, T. Novel LKF Method on H∞ Synchronization of Switched Time-Delay Systems. IEEE Trans. Cybern. 2023, 53, 4545–4554. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Yang, X.; Xiang, Z.; Sun, Y. Monotone decreasing LKF method for secure consensus of second-order mass with delay and switching topology. Syst. Control Lett. 2023, 172, 105436. [Google Scholar] [CrossRef]
- Della Rossa, F.; Pecora, L.; Blaha, K.; Shirin, A.; Klickstein, I.; Sorrentino, F. Symmetries and cluster synchronization in multilayer networks. Nat. Commun. 2020, 11, 3179. [Google Scholar] [CrossRef] [PubMed]
- Jalan, S.; Singh, A. Cluster synchronization in multiplex networks. Europhys. Lett. 2016, 113, 30002. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Boccaletti, S.; Guan, S.; Liu, Z. Explosive synchronization in adaptive and multilayer networks. Phys. Rev. Lett. 2015, 114, 038701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Majhi, S.; Perc, M.; Ghosh, D. Chimera states in a multilayer network of coupled and uncoupled neurons. Chaos: Interdiscip. J. Nonlinear Sci. 2017, 27, 073109. [Google Scholar] [CrossRef] [Green Version]
- Gambuzza, L.V.; Frasca, M.; Gomez-Gardenes, J. Intra-layer synchronization in multiplex networks. EPL Europhys. Lett. 2015, 110, 20010. [Google Scholar] [CrossRef] [Green Version]
- Anwar, M.S.; Rakshit, S.; Ghosh, D.; Bollt, E.M. Stability analysis of intralayer synchronization in time-varying multilayer networks with generic coupling functions. Phys. Rev. E 2022, 105, 024303. [Google Scholar] [CrossRef]
- Leyva, I.; Sevilla-Escoboza, R.; Sendiña-Nadal, I.; Gutiérrez, R.; Buldú, J.; Boccaletti, S. Inter-layer synchronization in non-identical multi-layer networks. Sci. Rep. 2017, 7, 45475. [Google Scholar] [CrossRef] [Green Version]
- Sevilla-Escoboza, R.; Sendiña-Nadal, I.; Leyva, I.; Gutiérrez, R.; Buldú, J.; Boccaletti, S. Inter-layer synchronization in multiplex networks of identical layers. Chaos Interdiscip. J. Nonlinear Sci. 2016, 26, 065304. [Google Scholar] [CrossRef]
- Anwar, M.S.; Ghosh, D. Intralayer and interlayer synchronization in multiplex network with higher-order interactions. Chaos Interdiscip. J. Nonlinear Sci. 2022, 32, 033125. [Google Scholar] [CrossRef] [PubMed]
- Anwar, M.S.; Ghosh, D.; Frolov, N. Relay synchronization in a weighted triplex network. Mathematics 2021, 9, 2135. [Google Scholar] [CrossRef]
- Leyva, I.; Sendiña-Nadal, I.; Sevilla-Escoboza, R.; Vera-Avila, V.; Chholak, P.; Boccaletti, S. Relay synchronization in multiplex networks. Sci. Rep. 2018, 8, 8629. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Drauschke, F.; Sawicki, J.; Berner, R.; Omelchenko, I.; Schöll, E. Effect of topology upon relay synchronization in triplex neuronal networks. Chaos Interdiscip. J. Nonlinear Sci. 2020, 30, 051104. [Google Scholar] [CrossRef]
- Anwar, M.S.; Ghosh, D. Stability of synchronization in simplicial complexes with multiple interaction layers. Phys. Rev. E 2022, 106, 034314. [Google Scholar] [CrossRef] [PubMed]
- Rakshit, S.; Parastesh, F.; Chowdhury, S.N.; Jafari, S.; Kurths, J.; Ghosh, D. Relay interlayer synchronisation: Invariance and stability conditions. Nonlinearity 2022, 35, 681. [Google Scholar] [CrossRef]
- Kheowan, O.U.; Mihaliuk, E.; Blasius, B.; Sendina-Nadal, I.; Showalter, K. Wave mediated synchronization of nonuniform oscillatory media. Phys. Rev. Lett. 2007, 98, 074101. [Google Scholar] [CrossRef] [Green Version]
- Osipov, G.V.; Pikovsky, A.S.; Rosenblum, M.G.; Kurths, J. Phase synchronization effects in a lattice of nonidentical Rössler oscillators. Phys. Rev. E 1997, 55, 2353. [Google Scholar] [CrossRef] [Green Version]
- So, P.; Cotton, B.C.; Barreto, E. Synchronization in interacting populations of heterogeneous oscillators with time-varying coupling. Chaos Interdiscip. J. Nonlinear Sci. 2008, 18, 037114. [Google Scholar] [CrossRef] [Green Version]
- Plotnikov, S.; Lehnert, J.; Fradkov, A.; Schöll, E. Synchronization in heterogeneous FitzHugh-Nagumo networks with hierarchical architecture. Phys. Rev. E 2016, 94, 012203. [Google Scholar] [CrossRef] [Green Version]
- Barreto, E.; Hunt, B.; Ott, E.; So, P. Synchronization in networks of networks: The onset of coherent collective behavior in systems of interacting populations of heterogeneous oscillators. Phys. Rev. E 2008, 77, 036107. [Google Scholar] [CrossRef] [Green Version]
- Sun, J.; Bollt, E.M.; Nishikawa, T. Master stability functions for coupled nearly identical dynamical systems. EPL Europhys. Lett. 2009, 85, 60011. [Google Scholar] [CrossRef] [Green Version]
- Yang, F.; Wang, Y.; Ma, J. Creation of heterogeneity or defects in a memristive neural network under energy flow. Commun. Nonlinear Sci. Numer. Simul. 2023, 119, 107127. [Google Scholar] [CrossRef]
- Xie, Y.; Yao, Z.; Ma, J. Formation of local heterogeneity under energy collection in neural networks. Sci. China Technol. Sci. 2023, 66, 439–455. [Google Scholar] [CrossRef]
- Pecora, L.M.; Carroll, T.L. Master stability functions for synchronized coupled systems. Phys. Rev. Lett. 1998, 80, 2109. [Google Scholar] [CrossRef] [Green Version]
- Tang, L.; Wu, X.; Lü, J.; Lu, J.a.; D’Souza, R.M. Master stability functions for complete, intralayer, and interlayer synchronization in multiplex networks of coupled Rössler oscillators. Phys. Rev. E 2019, 99, 012304. [Google Scholar] [CrossRef] [Green Version]
- Gambuzza, L.V.; Di Patti, F.; Gallo, L.; Lepri, S.; Romance, M.; Criado, R.; Frasca, M.; Latora, V.; Boccaletti, S. Stability of synchronization in simplicial complexes. Nat. Commun. 2021, 12, 1255. [Google Scholar] [CrossRef]
- Erdös, P.; Rényi, A. On the evolution of random graphs. In The Structure and Dynamics of Networks; Princeton University Press: Princeton, NJ, USA, 2011; pp. 38–82. [Google Scholar]
- Rössler, O.E. An equation for continuous chaos. Phys. Lett. A 1976, 57, 397–398. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anwar, M.S.; Rakshit, S.; Kurths, J.; Ghosh, D. Synchronization Induced by Layer Mismatch in Multiplex Networks. Entropy 2023, 25, 1083. https://doi.org/10.3390/e25071083
Anwar MS, Rakshit S, Kurths J, Ghosh D. Synchronization Induced by Layer Mismatch in Multiplex Networks. Entropy. 2023; 25(7):1083. https://doi.org/10.3390/e25071083
Chicago/Turabian StyleAnwar, Md Sayeed, Sarbendu Rakshit, Jürgen Kurths, and Dibakar Ghosh. 2023. "Synchronization Induced by Layer Mismatch in Multiplex Networks" Entropy 25, no. 7: 1083. https://doi.org/10.3390/e25071083
APA StyleAnwar, M. S., Rakshit, S., Kurths, J., & Ghosh, D. (2023). Synchronization Induced by Layer Mismatch in Multiplex Networks. Entropy, 25(7), 1083. https://doi.org/10.3390/e25071083





