Analysis of Entropy Generation via Non-Similar Numerical Approach for Magnetohydrodynamics Casson Fluid Flow with Joule Heating
Abstract
:1. Introduction
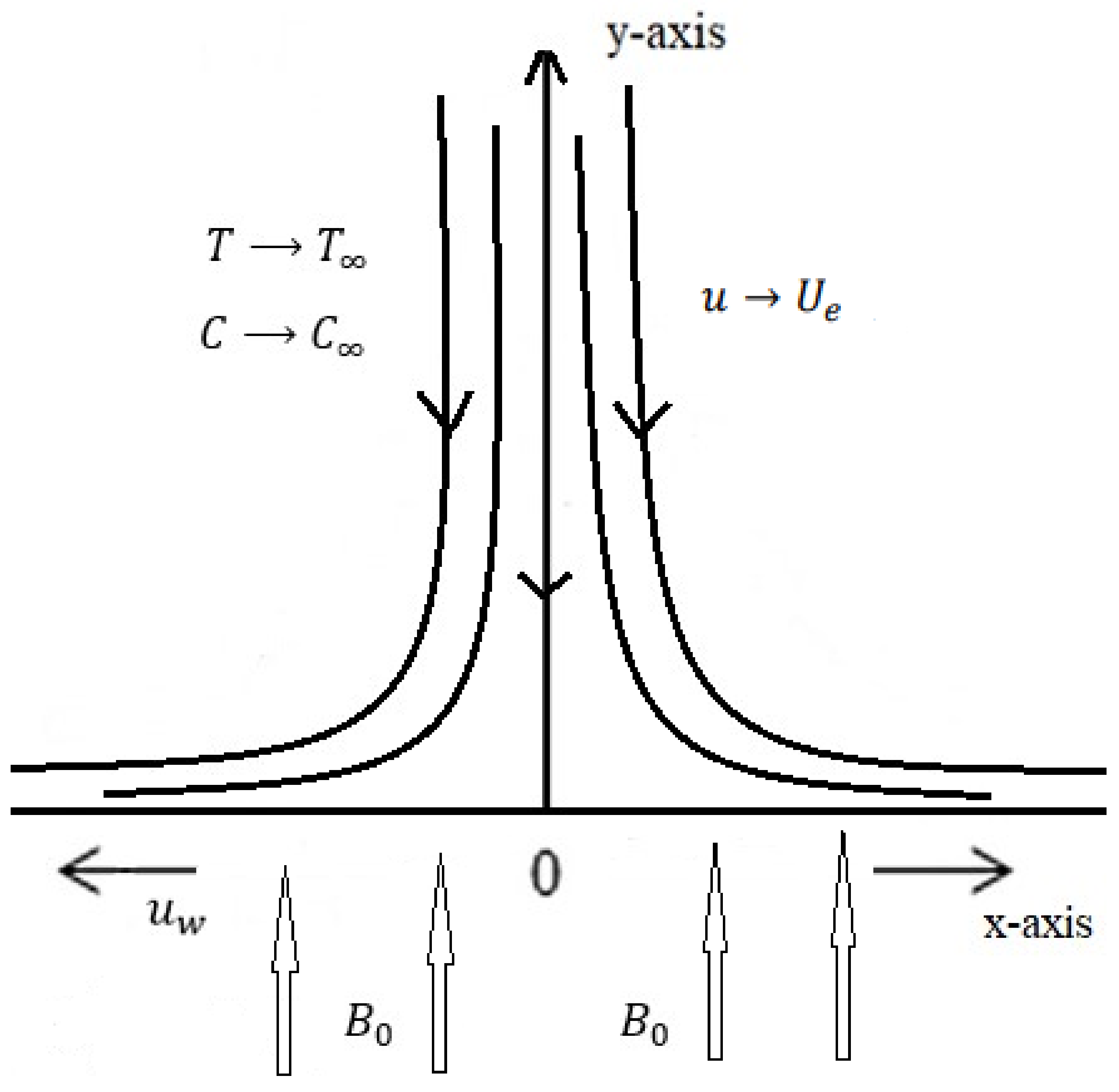
2. Problem Formulations
2.1. Non-Similar Transformation
2.2. Non-Similarity Technique
2.2.1. Truncation Level 1
2.2.2. Truncation Level 2
2.3. Entropy Analysis
2.4. Solution Procedure
3. Results and Discussions
4. Conclusions
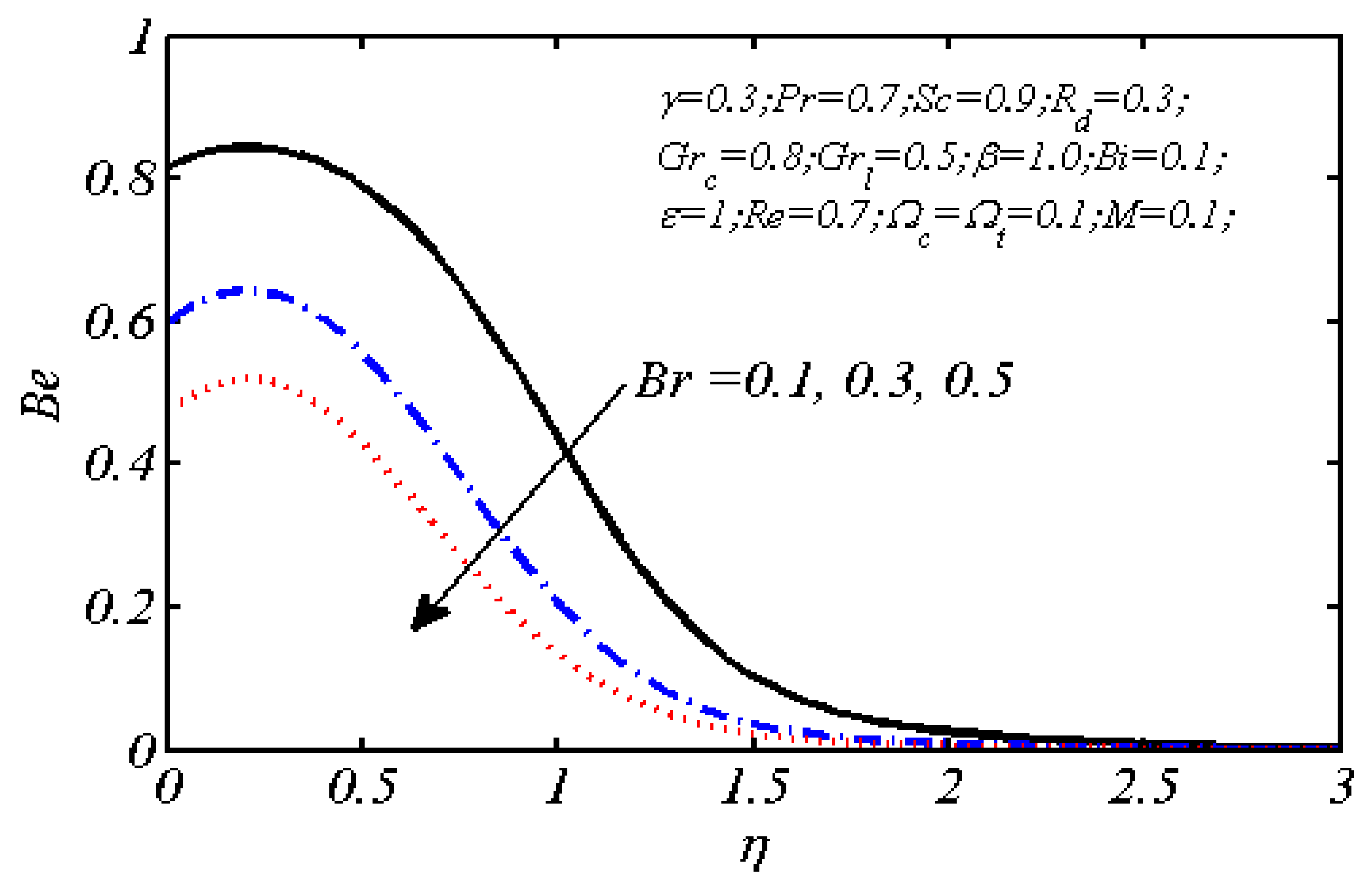
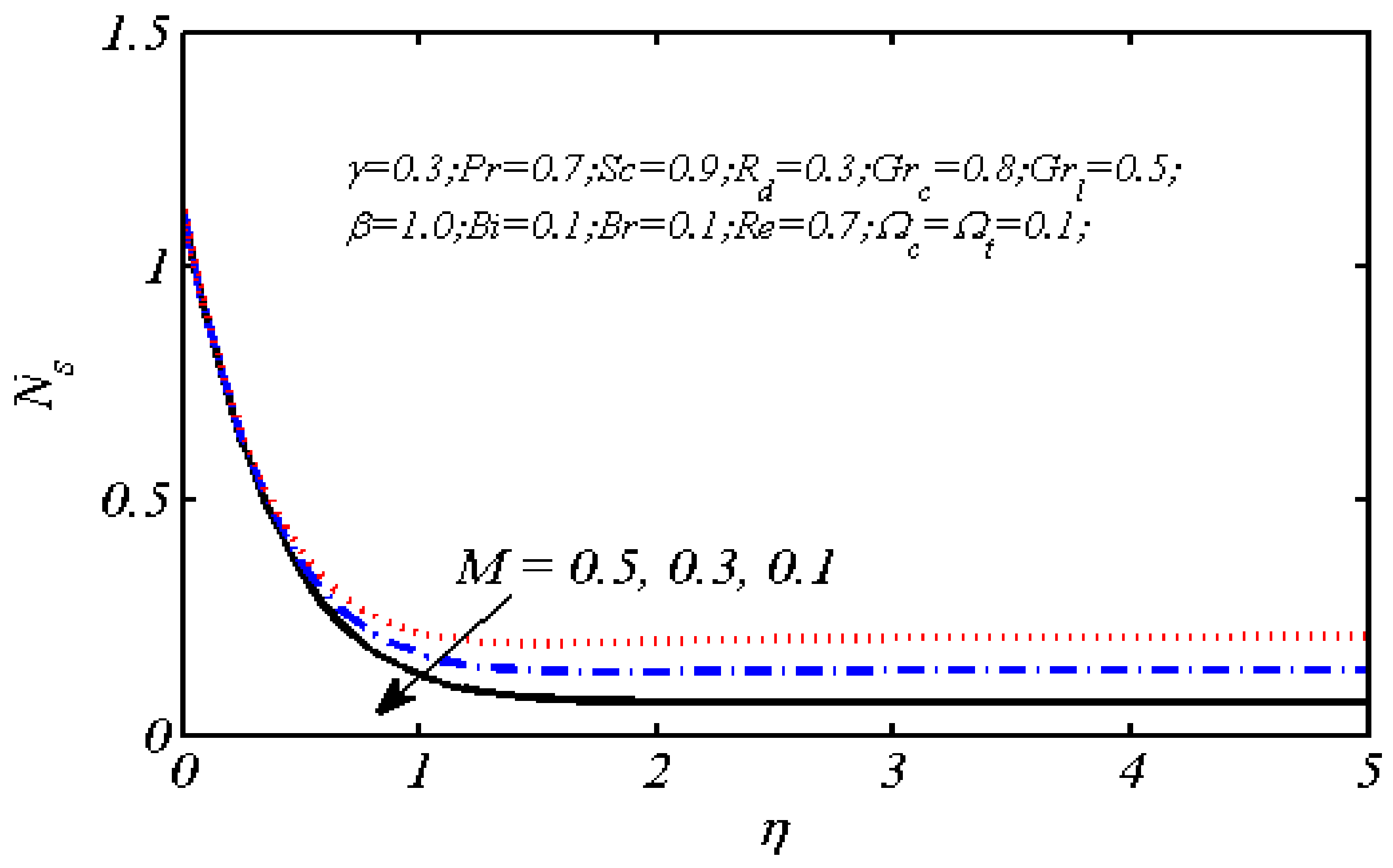
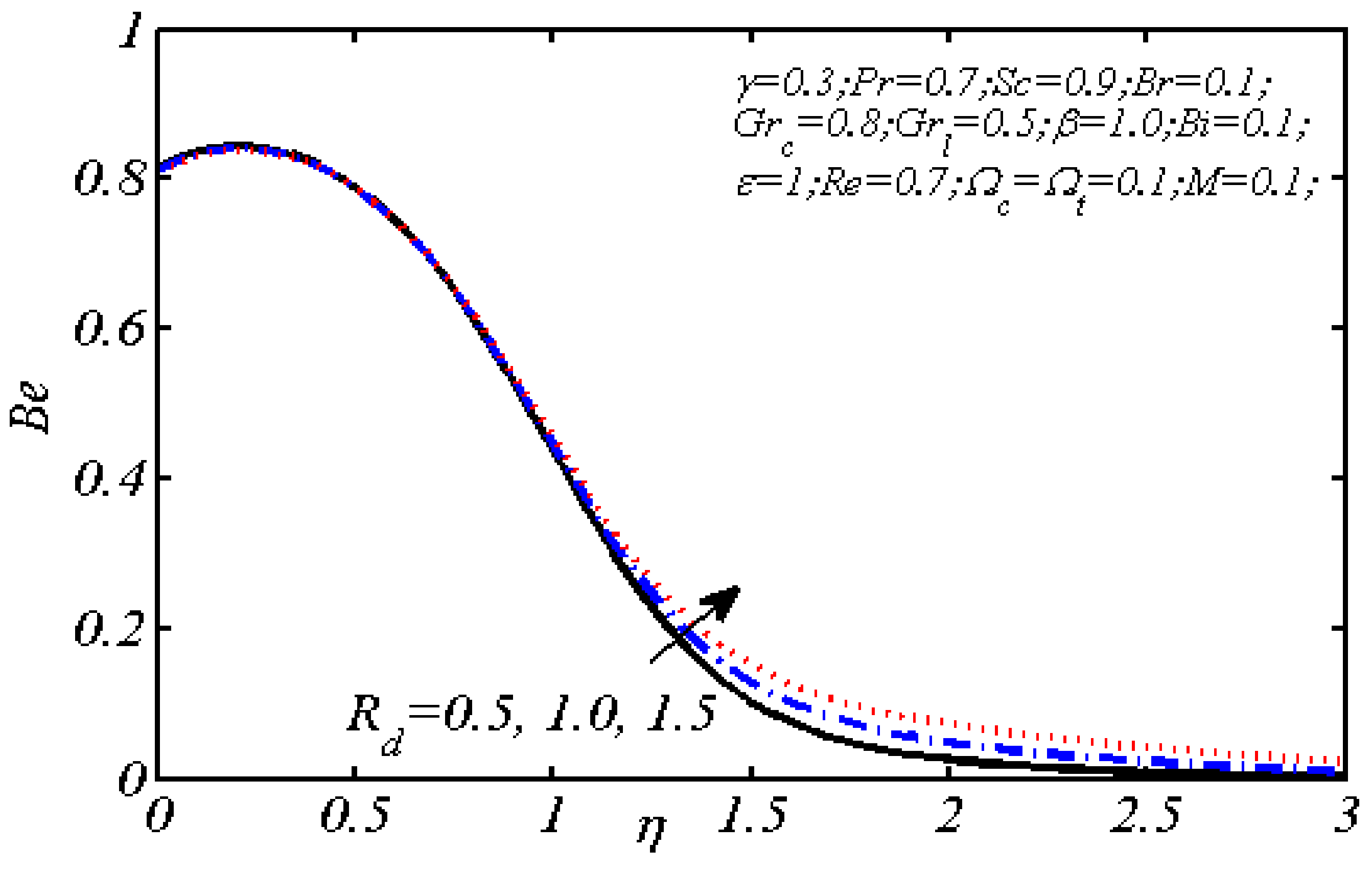
- The local entropy generation number is enhanced by increases in the Brinkman number, magnetic field, and radiation parameter.
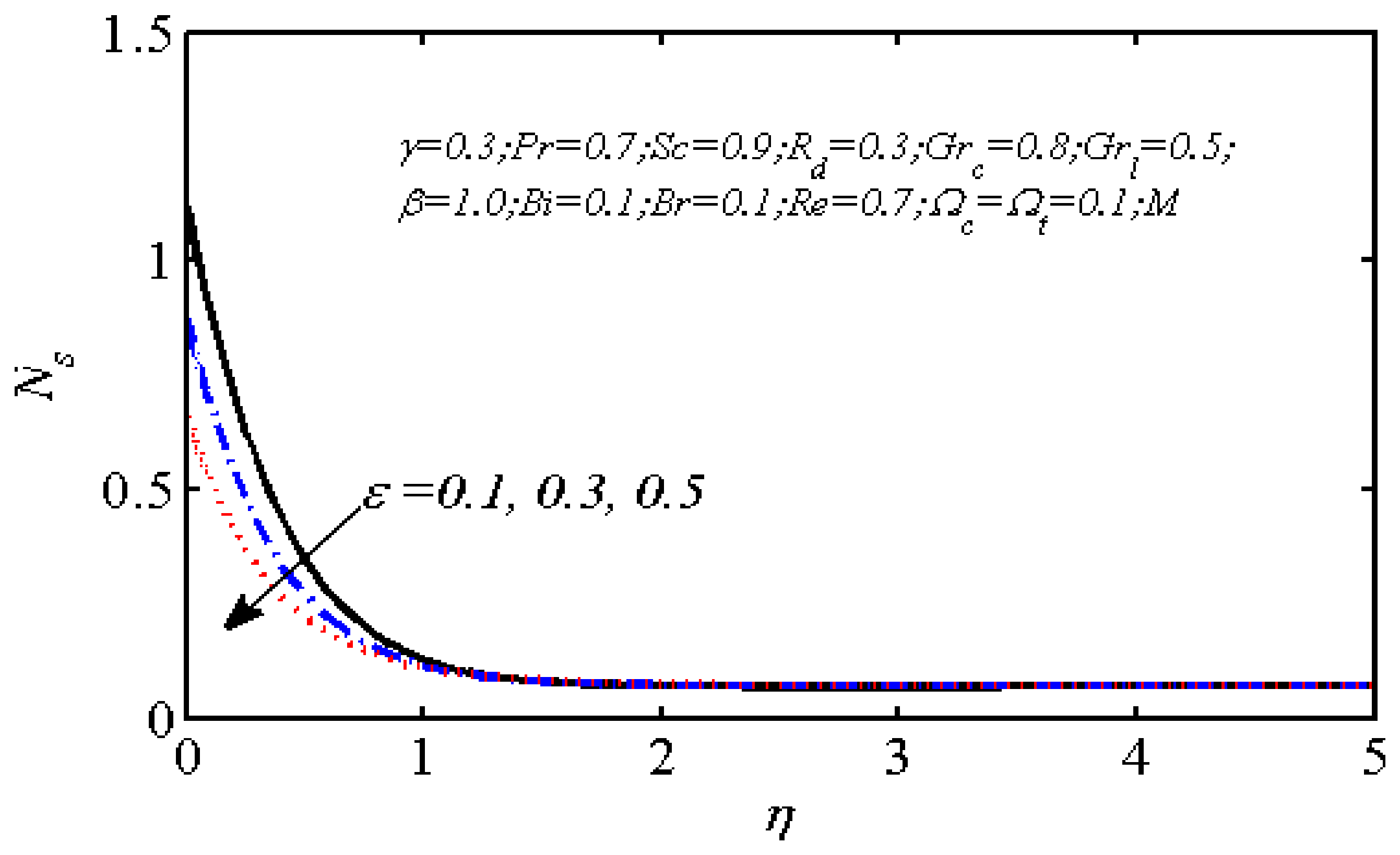
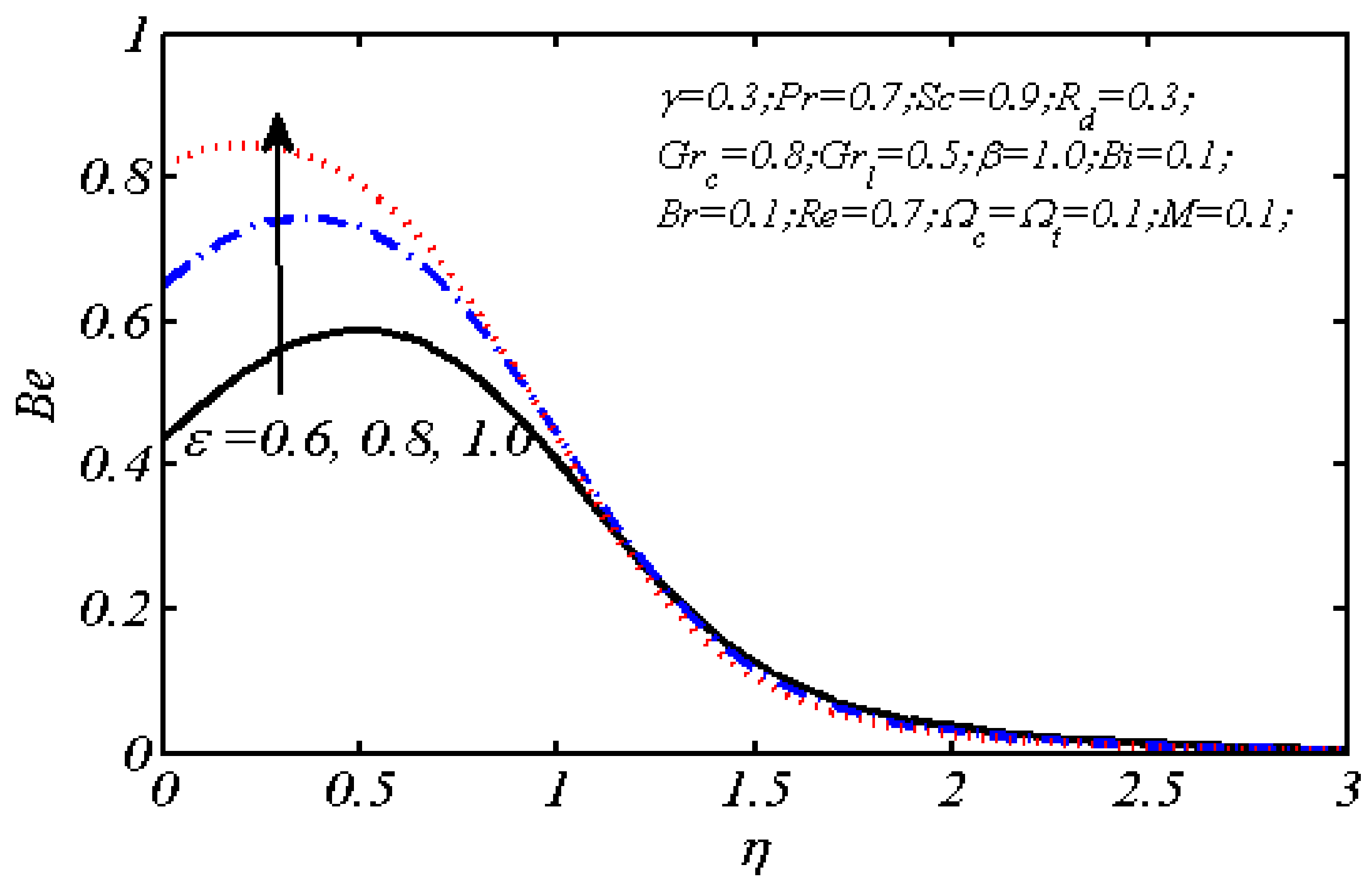
- An increase in the value of the Casson parameter and mass transfer parameter causes the local entropy generation number to decrease in the vicinity of the convective stretching surface.
- The average entropy generation number ( increases up to 10.43 times as changes from 0.1 to 1.1, while the average Bejan number decreases up to 3.60 times.
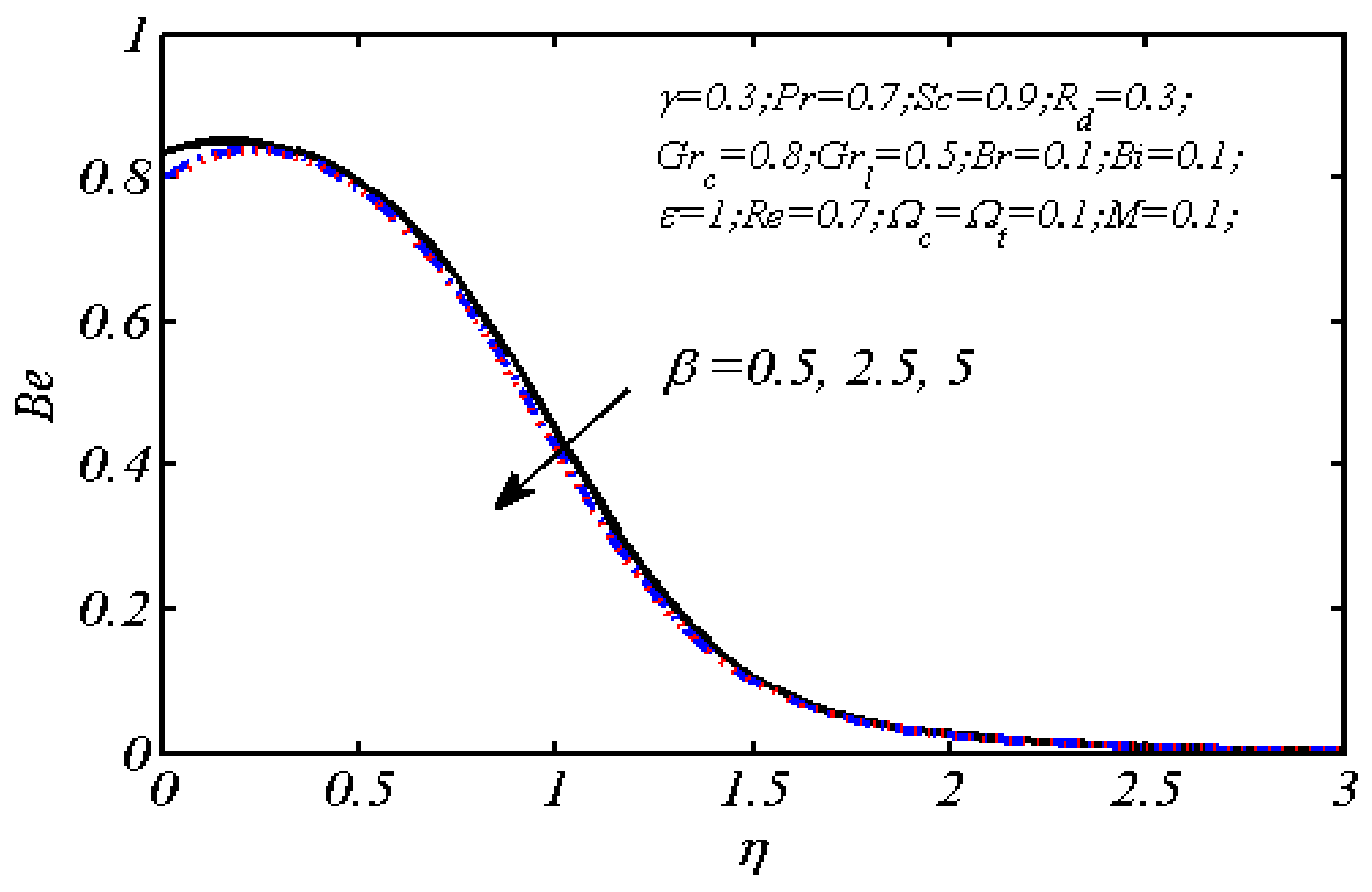
- The Bejan number graphs indicate that near the stretching surface, the effects of heat transfer and diffusion entropy are predominant, while in regions farther away, the entropy effects due to fluid friction and the magnetic field become more significant.
- At the surface of the plate, the average Bejan number decreased up to 3.21 times as the magnetic parameter varied from 0.1 to 1.1.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Vijayaragavan, R. Magnetohydrodynamic radiative casson fluid flow over a stretching sheet with heat source/sink. Adv. Phys. Theo. Appl. 2016, 55, 13–23. [Google Scholar]
- Mernone, A.V.; Mazumdar, J.N. A mathematical study of peristaltic transport of a casson fluid. Math. Comput. Model. 2002, 35, 895–912. [Google Scholar] [CrossRef]
- Raju, C.; Sandeep, N.; Sugunamma, V.; Babu, M.J.; Reddy, J.R. Heat and mass transfer in magnetohydrodynamic Casson fluid over an exponentially permeable stretching surface. Eng. Sci. Technol. Int. J. 2016, 19, 45–52. [Google Scholar] [CrossRef]
- Rehman, K.U.; Algehyne, E.A.; Shahzad, F.; Sherif, E.-S.M.; Chu, Y.-M. On thermally corrugated porous enclosure (TCPE) equipped with casson liquid suspension: Finite element thermal analysis. Case Stud. Therm. Eng. 2021, 25, 100873. [Google Scholar] [CrossRef]
- Hussain, F.; Nazeer, M.; Altanji, M.; Saleem, A.; Ghafar, M. Thermal analysis of Casson rheological fluid with gold nanoparticles under the impact of gravitational and magnetic forces. Case Stud. Therm. Eng. 2021, 28, 101433. [Google Scholar] [CrossRef]
- Nazeer, M.; Hussain, F.; Hameed, M.K.; Khan, M.I.; Ahmad, F.; Malik, M.Y.; Shi, Q.H. Development of mathematical modeling of multi-phase flow of Casson rheological fluid: Theoretical approach. Chaos Solitons Fractals 2021, 150, 111198. [Google Scholar] [CrossRef]
- Kempannagari, A.K.; Buruju, R.R.; Naramgari, S.; Vangala, S. Effect of Joule heating on MHD non-Newtonian fluid flow past an exponentially stretching curved surface. Heat Transf. 2020, 49, 3575–3592. [Google Scholar] [CrossRef]
- Gopal, D.; Kishan, N.; Raju, C.S.K. Viscous and Joule’s dissipation on Casson fluid over a chemically reacting stretching sheet with inclined magnetic field and multiple slips. Inform. Med. Unlocked 2017, 9, 154–160. [Google Scholar] [CrossRef]
- Muthtamilselvan, M.; Suganya, S.; Al-Mdallal, Q.M. Stagnation-Point Flow of the Williamson Nanofluid Containing Gyrotactic Micro-organisms. Proc. Natl. Acad. Sci. India Sect. A Phys. Sci. 2021, 91, 633–648. [Google Scholar] [CrossRef]
- Al-Zubaidi, A.; Nazeer, M.; Subia, G.S.; Hussain, F.; Saleem, S.; Ghafar, M. Mathematical modeling and simulation of MHD electro-osmotic flow of Jeffrey fluid in convergent geometry. Waves Random Complex Media 2021, 1–17. [Google Scholar] [CrossRef]
- Nazeer, M.; Hussain, F.; Shabbir, L.; Saleem, A.; Khan, M.I.; Malik, M.Y.; Sun, T.-C.; Hussain, A. A comparative study of MHD fluid-particle suspension induced by metachronal wave under the effects of lubricated walls. Int. J. Mod. Phys. B 2021, 35, 2150204. [Google Scholar] [CrossRef]
- Nazeer, M.; Saleem, S.; Hussain, F.; Zia, Z.; Khalid, K.; Feroz, N. Heat transmission in a magnetohydrodynamic multiphase flow induced by metachronal propulsion through porous media with thermal radiation. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2022, 09544089221075299. [Google Scholar] [CrossRef]
- Kumar, A.; Sugunamma, V.; Sandeep, N. Impact of non-linear radiation on MHD non-aligned stagnation point flow of micropolar fluid over a convective surface. J. Non-Equilib. Thermodyn. 2018, 43, 327–345. [Google Scholar]
- Khan, N.M.; Chu, Y.M.; Khan, M.I.; Kadry, S.; Qayyum, S. Modeling and dual solutions for magnetized mixed convective stagnation point flow of upper convected Maxwell fluid model with second-order velocity slip. Math. Methods Appl. Sci. 2020. [Google Scholar] [CrossRef]
- Bhattacharyya, K. Dual solutions in boundary layer stagnation-point flow and mass transfer with chemical reaction past a stretching/shrinking sheet. Int. Commun. Heat Mass Transf. 2011, 38, 917–922. [Google Scholar] [CrossRef]
- Bhattacharyya, K. Dual solutions in unsteady stagnation-point flow over a shrinking sheet. Chin. Phys. Lett. 2011, 28, 084702. [Google Scholar] [CrossRef]
- Bhattacharyya, K. MHD stagnation-point flow of Casson fluid and heat transfer over a stretching sheet with thermal radiation. J. Thermodyn. 2013, 2013, 169674. [Google Scholar] [CrossRef]
- Bhattacharyya, K. Heat transfer analysis in unsteady boundary layer stagnation-point flow towards a shrinking/stretching sheet. Ain Shams Eng. J. 2013, 4, 259–264. [Google Scholar] [CrossRef]
- Bhattacharyya, K.; Mukhopadhyay, S.; Layek, G. Slip effects on an unsteady boundary layer stagnation-point flow and heat transfer towards a stretching sheet. Chin. Phys. Lett. 2011, 28, 094702. [Google Scholar] [CrossRef]
- Mahapatra, T.R.; Gupta, A. Magnetohydrodynamic stagnation-point flow towards a stretching sheet. Acta Mech. 2001, 152, 191–196. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation Through Heat and Fluid Flow; Wiley and Sons: Hoboken, NJ, USA, 1994. [Google Scholar]
- Usha, R.; Uma, R. Entropy generation in a Casson fluid flow in a stenosed artery. J. Mech. Med. Biol. 2013, 13, 1350045. [Google Scholar]
- Rizvi, S.A.A.; Ansari, A.K. Heat transfer and entropy generation in Casson fluid flow over a stretching sheet with thermal radiation and viscous dissipation. J. Mol. Liq. 2016, 223, 1202–1210. [Google Scholar]
- Shevchuk, I.V. Convective Heat and Mass Transfer in Rotating Disk Systems; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Shevchuk, I.V. A Self-Similar Solution of Navier–Stokes and Energy Equations for Rotating Flows Between a Cone and a Disk. High Temp. 2004, 42, 104–110. [Google Scholar] [CrossRef]
- Gul, A.; Khan, I.; Shafie, S.; Khalid, A.; Khan, A. Heat transfer in MHD mixed convection flow of a ferrofluid along a vertical channel. PLoS ONE 2015, 10, e0141213. [Google Scholar] [CrossRef]
- Khalid, A.; Khan, I.; Khan, A.; Shafie, S. Unsteady MHD free convection flow of Casson fluid past over an oscillating vertical plate embedded in a porous medium. Eng. Sci. Technol. Int. J. 2015, 18, 309–317. [Google Scholar] [CrossRef]
- Gul, A.; Khan, I.; Shafie, S. Energy transfer in mixed convection MHD flow of nanofluid containing different shapes of nanoparticles in a channel filled with saturated porous medium. Nanoscale Res. Lett. 2015, 10, 490. [Google Scholar]
- Sparrow, E.; Quack, H.; Boerner, C. Local nonsimilarity boundary-layer solutions. AIAA J. 1970, 8, 1936–1942. [Google Scholar] [CrossRef]
- Shevchuk, I.V.; Saniei, N.; Yan, X.T. Impingement Heat Transfer over a Rotating Disk: Integral Method. J. Thermophys. Heat Transf. 2003, 17, 291–293. [Google Scholar] [CrossRef]
- Butt, A.S.; Ali, A.; Mehmood, A. Entropy analysis in MHD nanofluid flow near a convectively heated stretching surface. Int. J. Exergy 2016, 20, 318–342. [Google Scholar] [CrossRef]
- Kefayati, G.H.R. Simulation of natural convection and entropy generation of non-Newtonian nanofluid in a porous cavity using Buongiorno’s mathematical model. Int. J. Heat Mass Transf. 2017, 112, 709–744. [Google Scholar] [CrossRef]
- Butt, A.S.; Maqbool, K.; Imran, S.M.; Ahmad, B. Entropy generation effects in MHD Casson nanofluid past a permeable stretching surface. Int. J. Exergy 2020, 31, 150–171. [Google Scholar] [CrossRef]



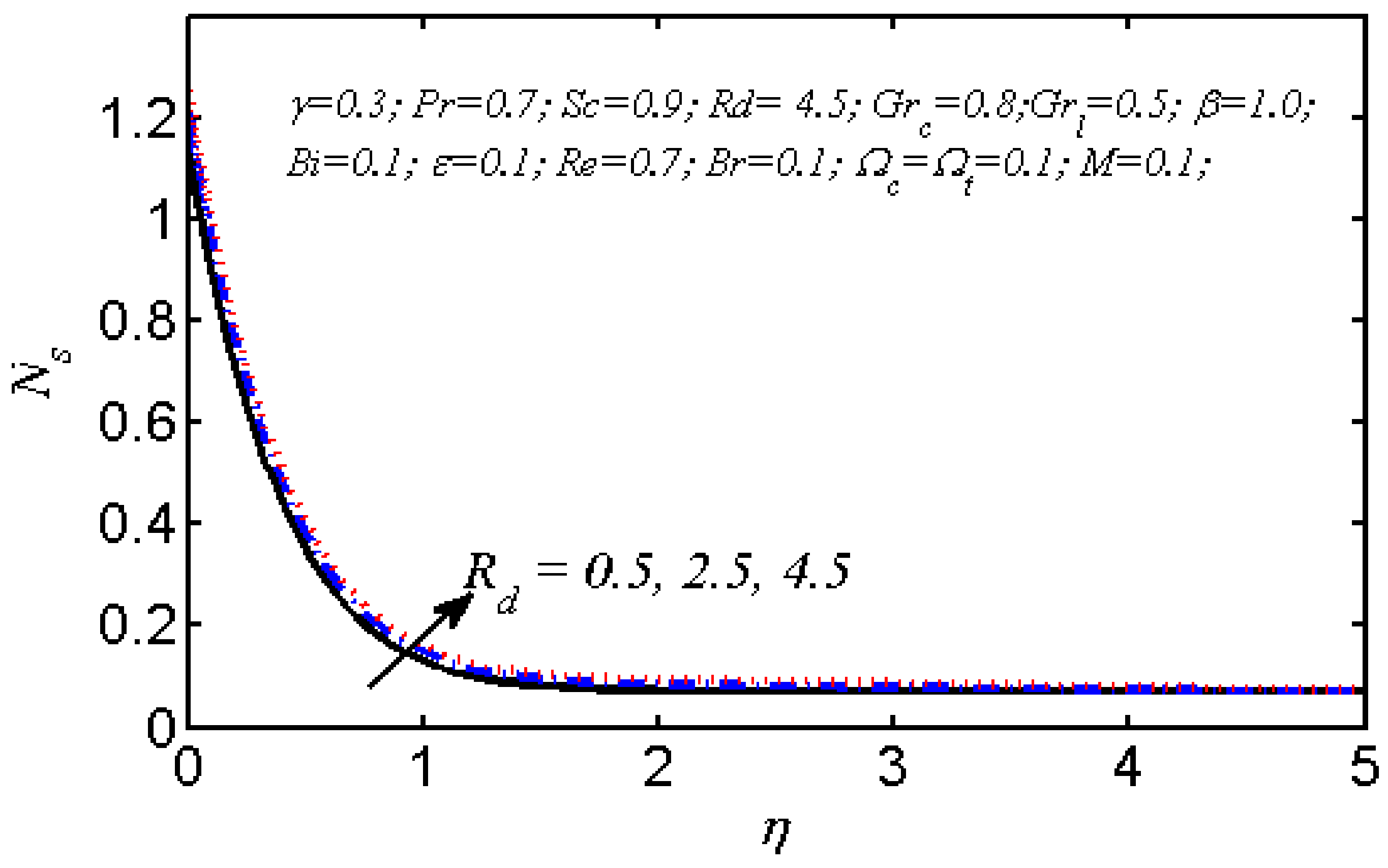

| 1.5759 | |||||||||
| 0.1 | 0.3 | 0.5 | 0.7 | 0.9 | 1.1 | |
|---|---|---|---|---|---|---|
| 0.0753 | 0.2174 | 0.3594 | 0.5015 | 0.6435 | 0.7856 | |
| 0.0891 | 0.0563 | 0.0423 | 0.0341 | 0.0287 | 0.0247 |
| 1 | 2 | 3 | 4 | 5 | 6 | |
|---|---|---|---|---|---|---|
| 0.0753 | 0.0732 | 0.0724 | 0.0719 | 0.0717 | 0.0715 | |
| 0.0891 | 0.0881 | 0.0877 | 0.0875 | 0.0873 | 0.0872 |
| 0.1 | 0.3 | 0.5 | 0.7 | 0.9 | 1.1 | |
|---|---|---|---|---|---|---|
| 0.0753 | 0.1327 | 0.1901 | 0.2475 | 0.3049 | 0.3623 | |
| 0.0891 | 0.0584 | 0.0452 | 0.0372 | 0.0317 | 0.0277 |
| 0.5 | 2.5 | 4.5 | 6.5 | 8.5 | 10.5 | |
|---|---|---|---|---|---|---|
| 0.0753 | 0.0826 | 0.0878 | 0.0925 | 0.0968 | 0.1010 | |
| 0.0891 | 0.1079 | 0.1296 | 0.1500 | 0.1682 | 0.1841 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Louati, H.; Khan, S.; Mansoor, M.; Hilali, S.O.; Gargouri, A. Analysis of Entropy Generation via Non-Similar Numerical Approach for Magnetohydrodynamics Casson Fluid Flow with Joule Heating. Entropy 2024, 26, 702. https://doi.org/10.3390/e26080702
Louati H, Khan S, Mansoor M, Hilali SO, Gargouri A. Analysis of Entropy Generation via Non-Similar Numerical Approach for Magnetohydrodynamics Casson Fluid Flow with Joule Heating. Entropy. 2024; 26(8):702. https://doi.org/10.3390/e26080702
Chicago/Turabian StyleLouati, Hanen, Sajid Khan, Muavia Mansoor, Shreefa O. Hilali, and Ameni Gargouri. 2024. "Analysis of Entropy Generation via Non-Similar Numerical Approach for Magnetohydrodynamics Casson Fluid Flow with Joule Heating" Entropy 26, no. 8: 702. https://doi.org/10.3390/e26080702





