The Analysis of Fractional-Order Kersten–Krasil Shchik Coupled KdV System, via a New Integral Transform
Abstract
:1. Introduction
2. Basic Definitions
3. The General Methodology of HPTM
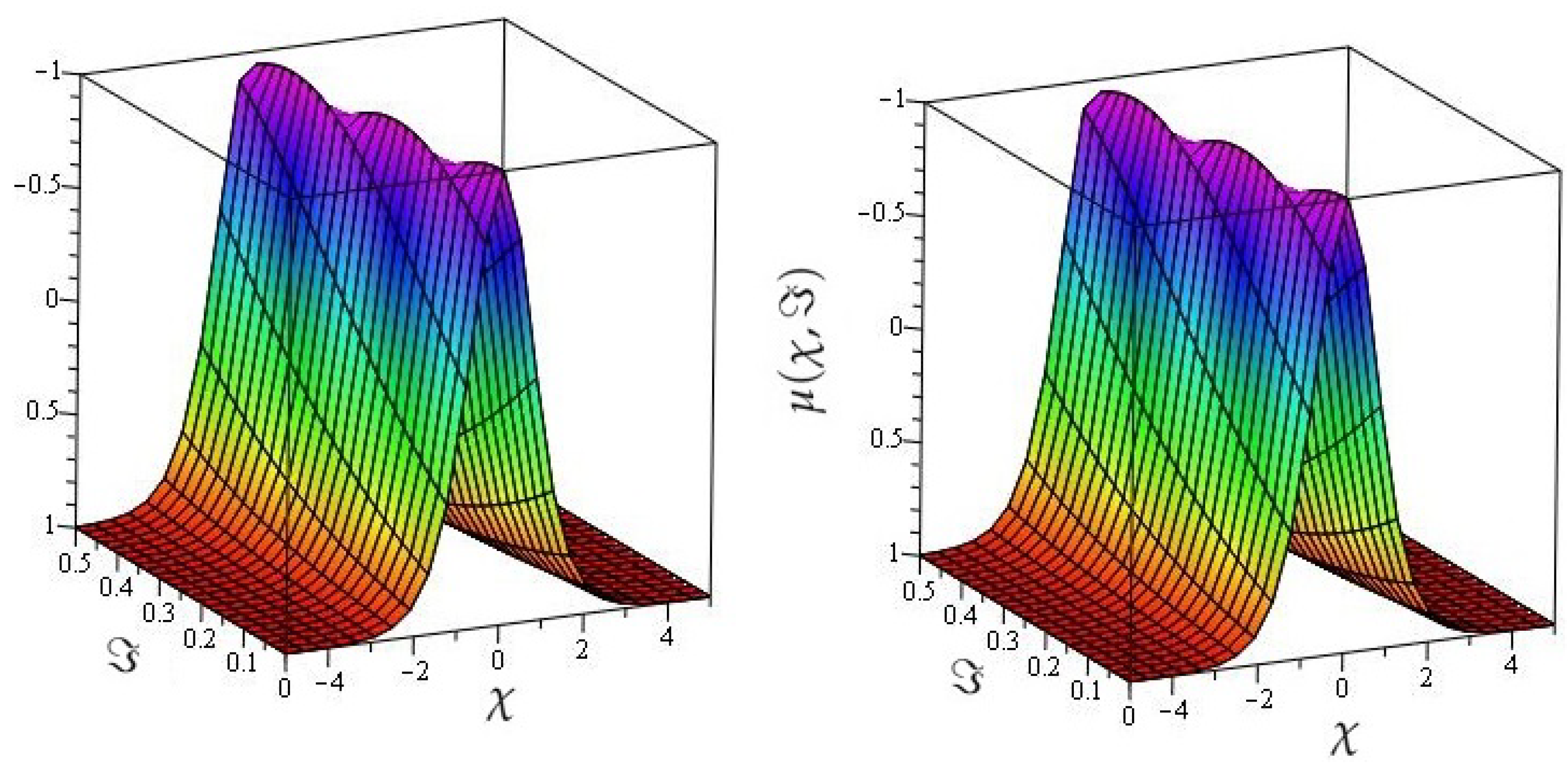
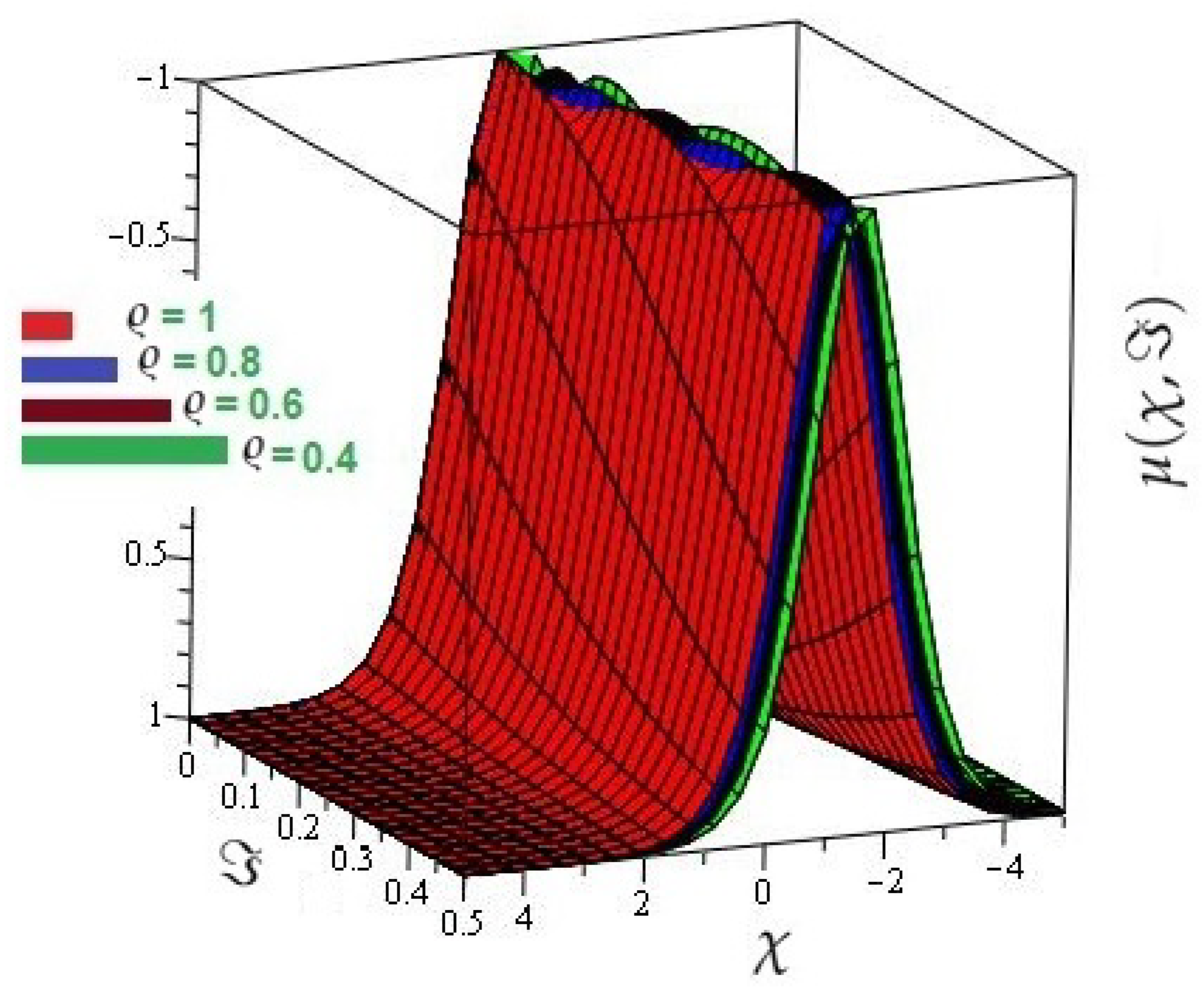
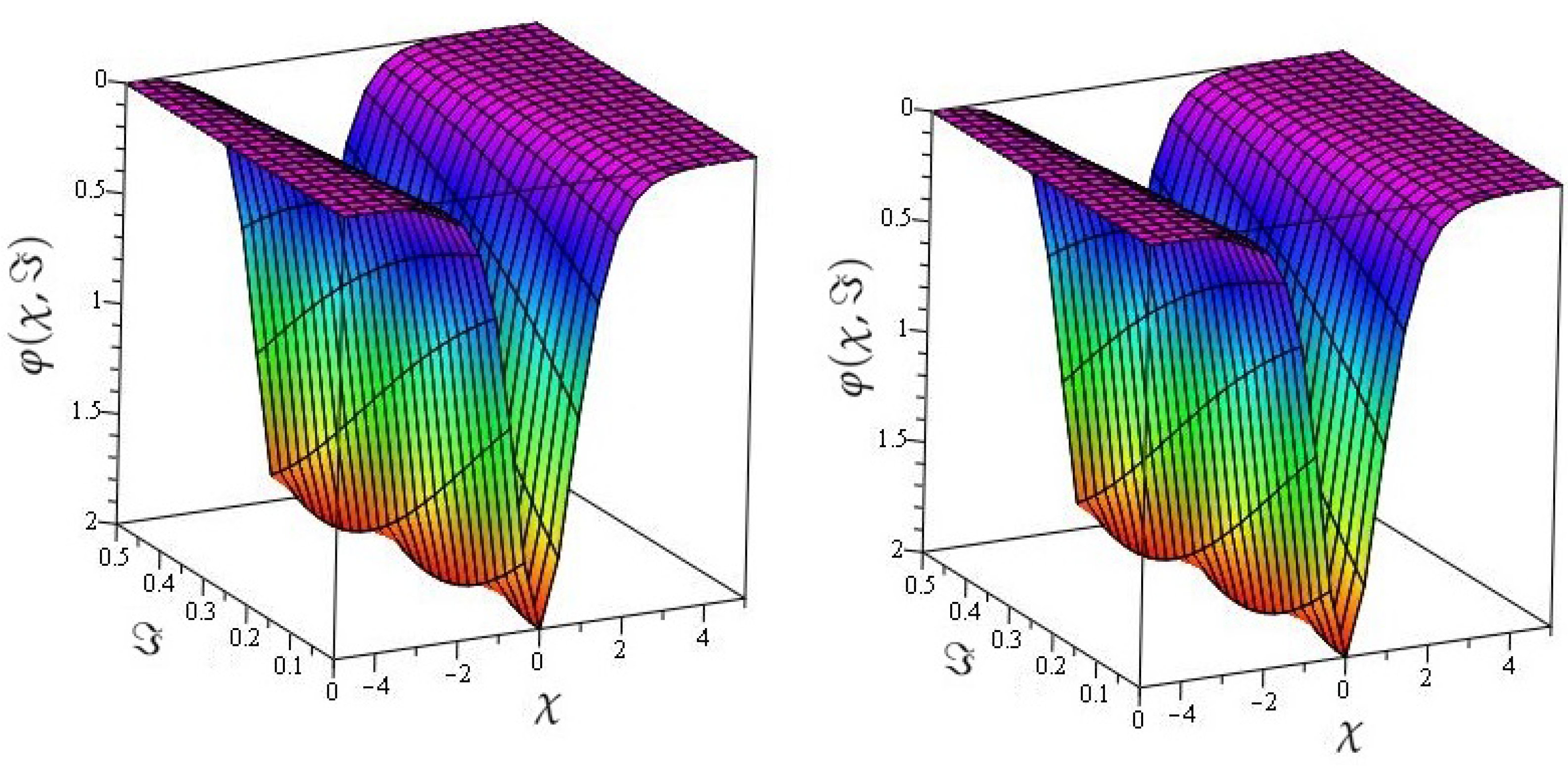
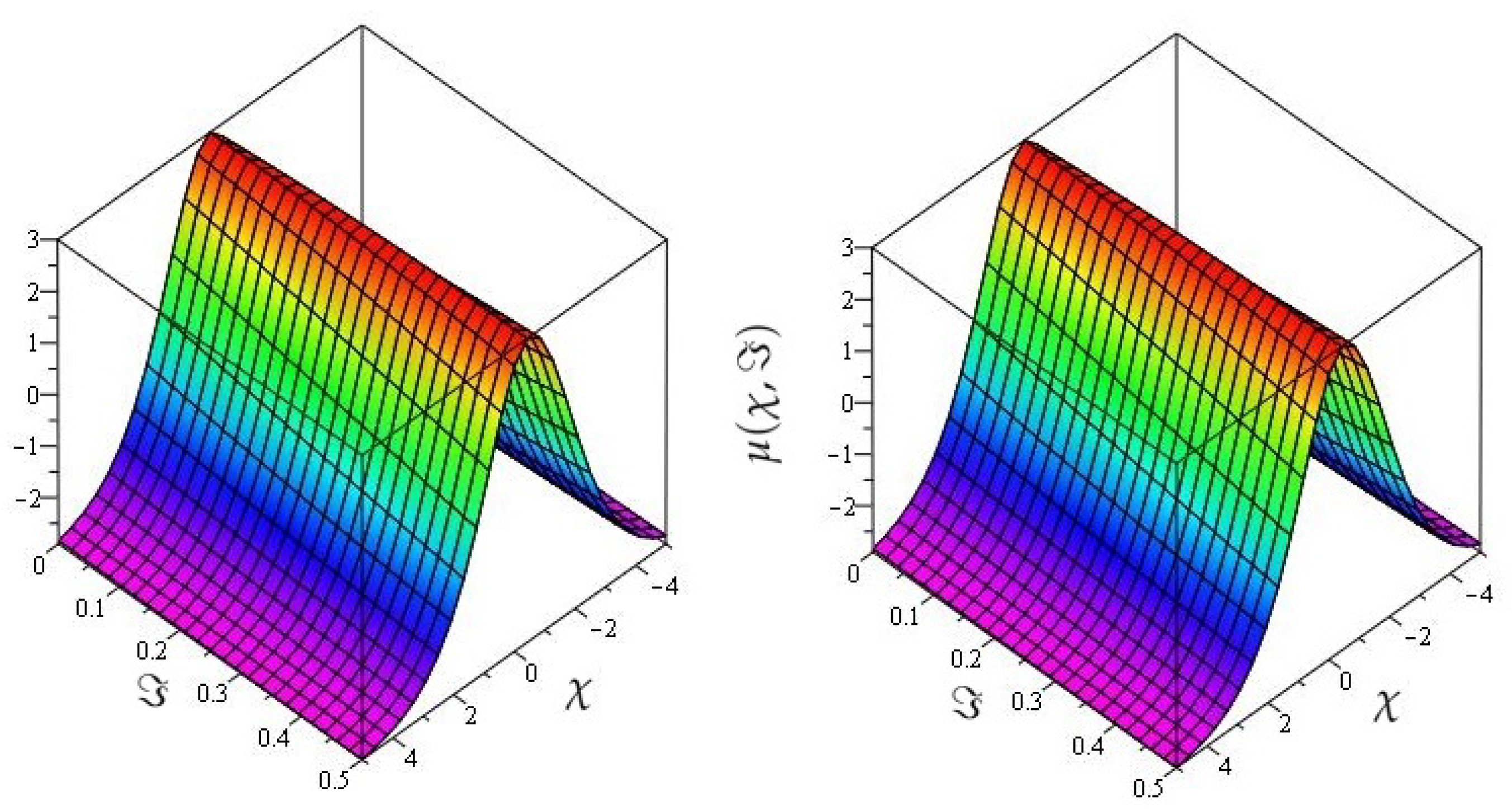
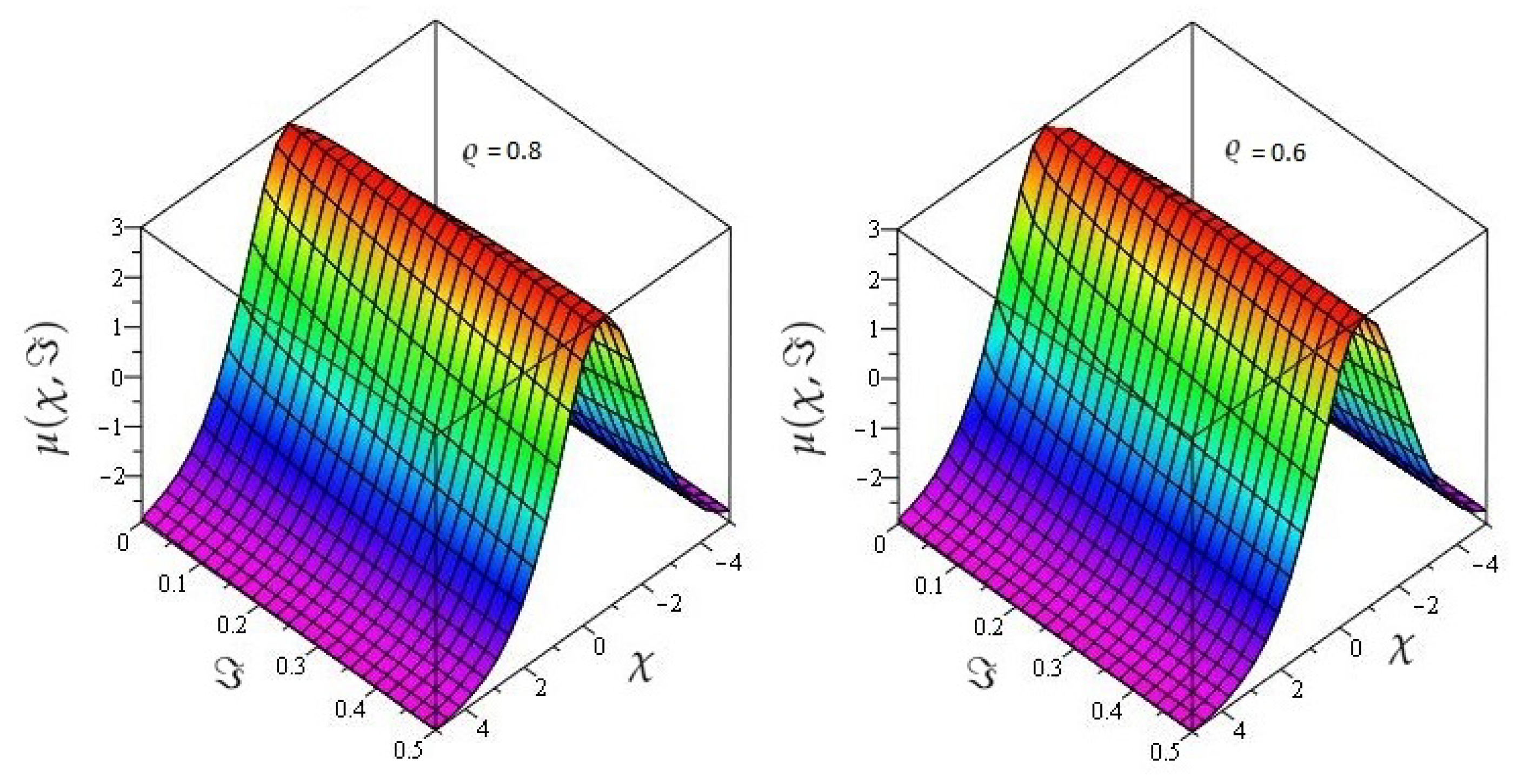
4. Numerical Experiments
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zaslavsky, G. Book Review: “Theory and Applications of Fractional Differential Equations” by Anatoly A. Kilbas, Hari M. Srivastava and Juan J. Trujillo. Fractals 2007, 15, 101–102. [Google Scholar] [CrossRef]
- Kilbas, A. Partial fractional differential equations and some of their applications. Analysis 2010, 30. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Calculus: Theory and Applications. Mathematics 2018, 6, 145. [Google Scholar] [CrossRef] [Green Version]
- Mu’lla, M. Fractional Calculus, Fractional Differential Equations and Applications. Oalib 2020, 7, 1–9. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Baleanu, D.; Kumam, P.; Arif, M. A semi-analytical method to solve family of Kuramoto–Sivashinsky equations. J. Taibah Univ. Sci. 2020, 14, 402–411. [Google Scholar] [CrossRef]
- Sakar, M.; Erdogan, F.; Yildirim, A. Variational iteration method for the time-fractional Fornberg-Whitham equation. Comput. Math. Appl. 2012, 63, 1382–1388. [Google Scholar] [CrossRef] [Green Version]
- El-Sayed, A.; Behiry, S.; Raslan, W. Adomian’s decomposition method for solving an intermediate fractional advection-dispersion equation. Comput. Math. Appl. 2010, 59, 1759–1765. [Google Scholar] [CrossRef] [Green Version]
- Nonlaopon, K.; Alsharif, A.; Zidan, A.; Khan, A.; Hamed, Y.; Shah, R. Numerical Investigation of Fractional-Order Swift-Hohenberg Equations via a Novel Transform. Symmetry 2021, 13, 1263. [Google Scholar] [CrossRef]
- Zhang, X.; Zhao, J.; Liu, J.; Tang, B. Homotopy perturbation method for two dimensional time-fractional wave equation. Appl. Math. Model. 2014, 38, 5545–5552. [Google Scholar] [CrossRef]
- Srivastava, V.; Awasthi, M.; Kumar, S. Analytical approximations of two and three dimensional time-fractional telegraphic equation by reduced differential transform method. Egypt. J. Basic Appl. Sci. 2014, 1, 60–66. [Google Scholar] [CrossRef] [Green Version]
- Pandey, R.; Singh, O.; Baranwal, V. An analytic algorithm for the space-time fractional advection-dispersion equation. Comput. Phys. Commun. 2011, 182, 1134–1144. [Google Scholar] [CrossRef]
- Wei, L.; He, Y. Analysis of a fully discrete local discontinuous Galerkin method for time-fractional fourth-order problems. Appl. Math. Model. 2014, 38, 1511–1522. [Google Scholar] [CrossRef]
- Rui, W.; Qi, X. Bilinear approach to quasi-periodic wave solutions of the Kersten–Krasil’shchik coupled KdV-mKdV system. Bound. Value Probl. 2016, 2016, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Keskin, Y.; Oturanc, G. Reduced Differential Transform Method for Partial Differential Equations. Int. J. Nonlinear Sci. Numer. Simul. 2009, 10, 741–750. [Google Scholar] [CrossRef]
- Kalkanli, A.; Sakovich, S.; Yurdusen, I. Integrability of Kersten–Krasil’shchik coupled KdV-mKdV equations: Singularity analysis and Lax pair. J. Math. Phys. 2003, 44, 1703–1708. [Google Scholar] [CrossRef] [Green Version]
- Goswami, A.; Sushila; Singh, J.; Kumar, D. Numerical computation of fractional Kersten–Krasil’shchik coupled KdV-mKdV system occurring in multi-component plasmas. AIMS Math. 2020, 5, 2346–2368. [Google Scholar]
- Jafari, H.; Prasad, J.; Goswami, P.; Dubey, R. Solution of the Local Fractional Generalized KDV Equation Using Homotopy Analysis Method. Fractals 2021, 29, 2140014. [Google Scholar] [CrossRef]
- Yang, Y.; Qi, J.; Tang, X.; Gu, Y. Further Results about Traveling Wave Exact Solutions of the (2 + 1)-Dimensional Modified KdV Equation. Adv. Math. Phys. 2019, 2019, 1–10. [Google Scholar] [CrossRef]
- Bhrawy, A.; Doha, E.; Ezz-Eldien, S.; Abdelkawy, M. A numerical technique based on the shifted Legendre polynomials for solving the time-fractional coupled KdV equations. Calcolo 2015, 53, 1–17. [Google Scholar] [CrossRef]
- Elbadri, M.; Ahmed, S.; Abdalla, Y.; Hdidi, W. A New Solution of Time-Fractional Coupled KdV Equation by Using Natural Decomposition Method. Abstr. Appl. Anal. 2020, 2020, 1–9. [Google Scholar] [CrossRef]
- He, J.H. Homotopy perturbation method: A new nonlinear analytical technique. Appl. Math. Comput. 2003, 135, 73–79. [Google Scholar] [CrossRef]
- Bhangale, N.; Kachhia, K.B.; Gomez-Aguilar, J.F. A new iterative method with ρ-Laplace transform for solving fractional differential equations with Caputo generalized fractional derivative. Eng. Comput. 2020, 1–14. [Google Scholar] [CrossRef]
- Wang, C. Hyers-Ulam-Rassias Stability of the Generalized Fractional Systems and the ρ-Laplace Transform Method. Mediterr. J. Math. 2021, 18, 1–22. [Google Scholar] [CrossRef]
- Jena, R.; Chakraverty, S. Solving time-fractional Navier-Stokes equations using homotopy perturbation Elzaki transform. SN Appl. Sci. 2018, 1, 16. [Google Scholar] [CrossRef] [Green Version]
- Mahgoub, M.; Sedeeg, A. A Comparative Study for Solving Nonlinear Fractional Heat-Like Equations via Elzaki Transform. Br. J. Math. Comput. Sci. 2016, 19, 1–12. [Google Scholar] [CrossRef]
- Das, S.; Gupta, P. An Approximate Analytical Solution of the Fractional Diffusion Equation with Absorbent Term and External Force by Homotopy Perturbation Method. Z. Fur Naturforschung A 2010, 65, 182–190. [Google Scholar] [CrossRef]
- Jan, R.; Khan, H.; Kumam, P.; Tchier, F.; Shah, R.; Bin Jebreen, H. The Investigation of the Fractional-View Dynamics of Helmholtz Equations within Caputo Operator. Comput. Mater. Contin. 2021, 68, 3185–3201. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shah, N.A.; Seikh, A.H.; Chung, J.D. The Analysis of Fractional-Order Kersten–Krasil Shchik Coupled KdV System, via a New Integral Transform. Symmetry 2021, 13, 1592. https://doi.org/10.3390/sym13091592
Shah NA, Seikh AH, Chung JD. The Analysis of Fractional-Order Kersten–Krasil Shchik Coupled KdV System, via a New Integral Transform. Symmetry. 2021; 13(9):1592. https://doi.org/10.3390/sym13091592
Chicago/Turabian StyleShah, Nehad Ali, Asiful H. Seikh, and Jae Dong Chung. 2021. "The Analysis of Fractional-Order Kersten–Krasil Shchik Coupled KdV System, via a New Integral Transform" Symmetry 13, no. 9: 1592. https://doi.org/10.3390/sym13091592






