Next Article in Journal
Investigation of Chemomarkers of Astragali Radix of Different Ages and Geographical Origin by NMR Profiling
Next Article in Special Issue
Imaging the Ultrafast Photoelectron Transfer Process in Alizarin-TiO2
Previous Article in Journal
Investigation of Structural Mimetics of Natural Phosphate Ion Binding Motifs
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 20
Issue 2
10.3390/molecules20023371
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

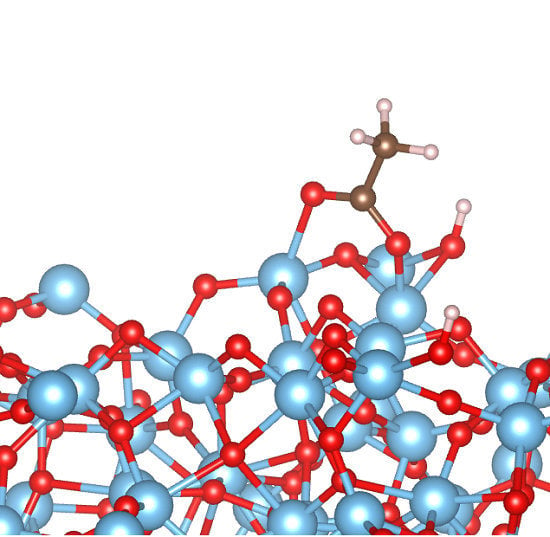
A Density Functional Tight Binding Study of Acetic Acid Adsorption on Crystalline and Amorphous Surfaces of Titania

by
Sergei Manzhos
1,*,
Giacomo Giorgi
2,3 and
Koichi Yamashita
2,3
1
Department of Mechanical Engineering, National University of Singapore, Block EA #07-08, 9 Engineering Drive 1, Singapore 117576, Singapore
2
Department of Chemical System Engineering, School of Engineering, University of Tokyo, 7-3-1, Hongo, Bunkyo-ku, Tokyo 113-8656, Japan
3
CREST-JST, 7 Gobancho, Chiyoda-ku, Tokyo 102-0076, Japan
*
Author to whom correspondence should be addressed.
Molecules 2015, 20(2), 3371-3388; https://doi.org/10.3390/molecules20023371
Submission received: 4 January 2015 / Revised: 12 February 2015 / Accepted: 13 February 2015 / Published: 17 February 2015
(This article belongs to the Special Issue Molecular Engineering for Electrochemical Power Sources)
Browse Figures
Versions Notes

Abstract

:
We present a comparative density functional tight binding study of an organic molecule attachment to TiO2 via a carboxylic group, with the example of acetic acid. For the first time, binding to low-energy surfaces of crystalline anatase (101), rutile (110) and (B)-TiO2 (001), as well as to the surface of amorphous (a-) TiO2 is compared with the same computational setup. On all surfaces, bidentate configurations are identified as providing the strongest adsorption energy, Eads = −1.93, −2.49 and −1.09 eV for anatase, rutile and (B)-TiO2, respectively. For monodentate configurations, the strongest Eads = −1.06, −1.11 and −0.86 eV for anatase, rutile and (B)-TiO2, respectively. Multiple monodentate and bidentate configurations are identified on a-TiO2 with a distribution of adsorption energies and with the lowest energy configuration having stronger bonding than that of the crystalline counterparts, with Eads up to −4.92 eV for bidentate and −1.83 eV for monodentate adsorption. Amorphous TiO2 can therefore be used to achieve strong anchoring of organic molecules, such as dyes, that bind via a -COOH group. While the presence of the surface leads to a contraction of the band gap vs. the bulk, molecular adsorption caused no appreciable effect on the band structure around the gap in any of the systems.

Graphical Abstract

1. Introduction

Titanium oxide is widely used in various electrochemical technologies, namely heterogeneous catalysis and photocatalysis [1,2], fuel cells [3,4], solar cells (dye-sensitized, DSSC [5,6,7,8,9], and perovskite-sensitized, PSSC [10,11]) and electrochemical batteries [12,13,14,15,16,17,18]. It is relatively abundant, inexpensive, safe and possesses a band structure suitable for these electrochemical and photocatalytic applications [19]. The three most stable phases are usually employed: anatase, rutile and (B). In all of these applications, molecules interact with titania surfaces. While some higher-energy, higher-index surfaces are sometimes explored [20,21], most applications have relied on low-energy anatase (101), rutile (110) and (B) (001) surfaces [1,2,22,23,24], which is justified by the ease of synthesis and stability.
In the applications in DSSC anodes, as well as in studies of carboxylic acids on TiO2, molecules typically bind to TiO2 via the -COOH group, with bidentate bridging and monodentate configurations dominating [9,25,26,27,28,29,30]. Bidentate chelating configurations are sometimes considered, but are usually much less stable [9,25,31,32]. Adsorption of organic molecules via a carboxylic moiety is also important for other applications. For example, the 21 amino acids possess the -COOH group, which plays a role in adsorption on TiO2. These interactions are of interest for the development of biocompatible and bio-inspired nanostructured materials [33]. A key parameter of the interaction of molecules with TiO2 surfaces is binding strength. It controls the stability of DSSC anodes, as well as the degree of electronic coupling, which directly influences the electron transfer rate [26,34]. It also controls the reaction rates in catalytic applications [1,2]. The ability to control or to strengthen binding by the choice of a specific TiO2 surface is therefore important in all of these applications.
Applications of these crystalline phases and surfaces have also been supported by extensive theoretical and computational studies [35,36,37,38,39] and, specifically, studies of molecular adsorption via the carboxylic group onto selected crystalline phases [9,25,26,27,28,29,31,32]. Amorphous titania (a-TiO2) has recently attracted interest for its use in the dye-sensitized technology [40,41,42], as well as in electrochemical batteries [43,44,45,46,47,48] and in photocatalysis [49]. These studies indicate that a-TiO2 may be advantageous in these applications for several reasons. For example, the rate capability of battery electrodes made of nanostructured amorphous TiO2 was found to be higher than that of nanostructured anatase TiO2 due to the higher Li-diffusion coefficient in amorphous TiO2 [44,47]. a-TiO2 has also been shown to possess a distribution of binding energies to Li, Na and Mg, with the lowest binding energy stronger than that of the anatase, rutile and (B) phases [50,51]. The same might hold for molecular adsorption and needs to be quantified. The presence of amorphous TiO2 in the anodes of DSSC has been shown to improve conversion efficiency [42]. Low-crystallinity TiO2 DSSC anodes have shown a high open circuit voltage and low recombination [41]. a-TiO2 could also be a cheaper alternative to crystalline phases for photocatalytic applications, as it possesses similar band gap characteristics to anatase [49,52].
There are still few computational studies of the properties of a-TiO2 relevant for these applications [40,49,50,51,52,53]. Specifically, there are no ab initio studies comparing at the same level of theory and with the same computational parameters the adsorption of organic molecules on the surfaces of all of the phases of TiO2 that are finding use in electrochemical technologies, i.e., anatase, rutile, (B) and amorphous. As ab initio methods still possess only semi-quantitative accuracy, results obtained with different phases in different studies using different computational setups are not directly comparable. Here, we present a study that compares with the same computational setup the adsorption of a prototypical organic molecule endowed with a -COOH anchoring group (acetic acid) to low energy crystalline surfaces, namely, anatase (101), rutile (110) and (B) (001), and to amorphous surfaces. We therefore aim to compare for the first time the adsorption modes among different phases and to understand the effect of amorphization on the geometries and binding strength of molecules.

2. Theoretical and Computational Methods

Calculations were performed using the self-consistent charge density functional tight binding scheme (SCC-DFTB) [54] and the DFTB + code [55]. SCC-DFTB is an approximate DFT approach derived from a second-order expansion of the DFT energy with respect to charge density fluctuations and provides ab initio accuracy for systems for which it is parameterized. We used the parameter set (Slater–Koster files) “matsci-0-3”, which has been benchmarked, in particular, for TiO2 and for organic molecules interacting with TiO2 [56].
For bulk TiO2 calculations, we used conventional standard cells with 4, 2 and 8 formula units for anatase, rutile and (B), respectively. The Brillouin zone was sampled with a k-point density no less than one point per 30−1 Å−1. Converged results were obtained with 10 × 10 × 5, 8 × 8 × 12, and 3 × 10 × 6 k-points for anatase, rutile and (B), respectively. The initial structure of a-TiO2 was taken from [52] and re-optimized within the present setup. A 192-atom supercell of a size of about 13 × 13 × 13 Å was used, and converged results were obtained with 3 × 3 × 3 k-points.
Anatase (101), rutile (110) and B (001) low-energy surfaces were modeled with slabs of a thickness of at least 13 Å, which are shown in Figure 2, Figure 3 and Figure 4. The lateral (xy) extent of the supercell was about 10.5 × 11.5 Å, 12 × 13 Å and 12.5 × 11.3 Å, for anatase, rutile and (B), respectively. The top half of the slab (in the z direction) was optimized, and the bottom half held at bulk positions during optimization. The amorphous surfaces were obtained from the bulk supercell by adding a vacuum in the z direction. Two surfaces were obtained by optimizing the top/bottom half and fixing the bottom/top half of the slab; these are labeled as “top” and “bottom” surfaces. This is a similar approach to that used in [53]. All surface supercells were 30 Å in the z direction, and 3 × 3 × 1 k-points were used. Acetic acid molecules were then positioned above the surfaces in various configurations with the COOH group in monodentate or bidentate binding to the surface and optimized with DFTB. We here focus on bidentate binding in bridging configurations, which are known to be much more stable than chelating configurations [9,25,31,32].
The adsorption energy (Eads) of AcOH on top of all of the surfaces here considered has been calculated as:
E a d s = E s u r f   +   A c O H − ( E s u r f + E A c O H )
where Esurf, EAcOH and Esurf + AcOH represent the energy of, respectively, the optimized surface, a free optimized acetic acid molecule and of the final optimized anchored system formed by the surface and acetic acid.

3. Results and Discussion

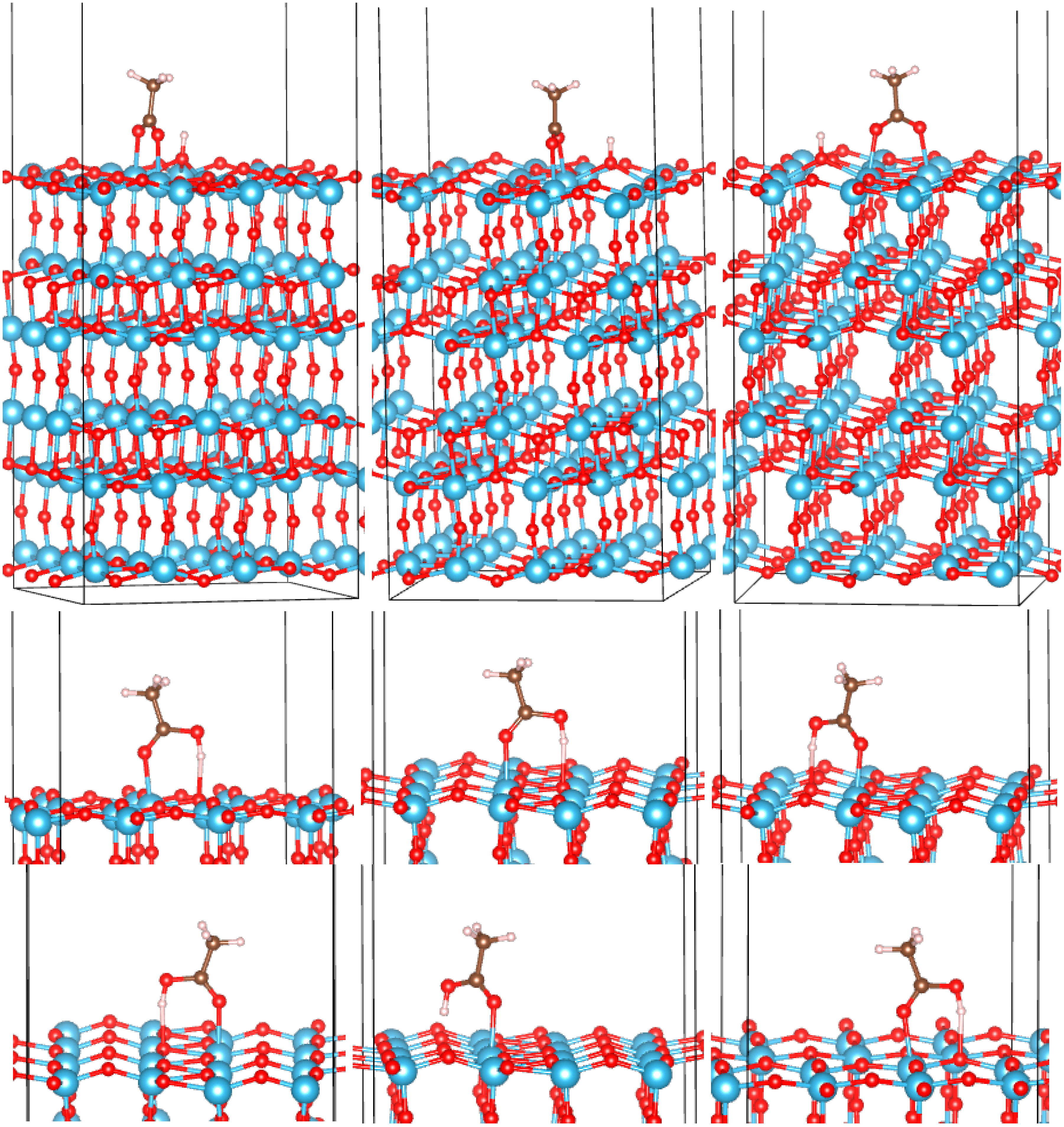
3.1. TiO2 Bulk and Surfaces

Anatase: The cell vectors of the optimized bulk anatase (conventional standard cell) are a = b = 3.81 Å, c = 9.73 Å, in good agreement with previously reported experimental and computed values [57,58,59,60,61]. The energy per formula unit is −215.181 eV. The density of states is shown in Figure 1 and shows a band gap of about 3.2 eV, in good agreement with experimental values of 3.2–3.3 eV [62] and prior DFTB calculations [63]. The presence of the (101) surface causes a contraction of the band gap by about 0.5 eV.
Molecules 20 03371 g001 550
Figure 1. The densities of states (DOSs) of bulk anatase, rutile, (B) and a-TiO2 and their surfaces, as well as surfaces with AcOH adsorbed in different configurations (described in Section 3.1). For each phase, the zero of the energy axis corresponds to the Fermi energy in the bulk, and the DOSs of surfaces are plotted to match the valence band maximum. Gaussian broadening of 0.1 eV. For a-TiO2, only the “top” surface data are shown. The curves for the “bottom” surface are qualitatively similar. For (B)-TiO2 and a-TiO2, curves for the selected adsorption configurations are shown; the curves for other adsorption configurations are similar. For anatase and rutile, the DOSs for nanosheets (“NS”) are also shown.
Figure 1. The densities of states (DOSs) of bulk anatase, rutile, (B) and a-TiO2 and their surfaces, as well as surfaces with AcOH adsorbed in different configurations (described in Section 3.1). For each phase, the zero of the energy axis corresponds to the Fermi energy in the bulk, and the DOSs of surfaces are plotted to match the valence band maximum. Gaussian broadening of 0.1 eV. For a-TiO2, only the “top” surface data are shown. The curves for the “bottom” surface are qualitatively similar. For (B)-TiO2 and a-TiO2, curves for the selected adsorption configurations are shown; the curves for other adsorption configurations are similar. For anatase and rutile, the DOSs for nanosheets (“NS”) are also shown.
Molecules 20 03371 g001
Rutile: The cell vectors of the optimized rutile phase are a = b = 4.61 Å, c = 2.97 Å, in good agreement with previously reported values [57,58,61]. The density of states is shown in Figure 1 and shows a band gap of about 3.1 eV, in good agreement with reported values of about 3 eV [22,64,65,66] and consistent with that of anatase. The energy per formula unit is −215.481 eV. The calculations therefore correctly reproduce the anatase-rutile phase ordering, albeit with a difference of 0.1 eV per atom, which is higher than the reported values of 0.012–0.015 eV [67,68]. This, however, is not critical for the present purpose of studying molecular adsorption. This accuracy is also comparable with that of previously reported DFT values for cohesive energies ranging from −18.77 to −21.44 eV [69,70] for rutile and from −21.54 to −21.60 eV [70,71] for anatase. The presence of the interface ((110) surface) causes a contraction of the band gap by about 1.5 eV.
(B): The cell parameters of the optimized (B) phase are a = 12.45 Å, b = 3.76 Å, c = 6.69 Å, α = γ = 90°, β = 107.3°, in good agreement with previously reported values [22,72]. The density of states is also shown in Figure 1 and shows a band gap of about 3.1 eV, in good agreement with the experimental value of 3.2 eV [73]. The energy per formula unit is −215.365 eV, i.e., similar to previous ab initio calculations, we obtain that (B) is more stable than anatase, although this is in contradiction with the low abundance of (B) relative to anatase and rutile [22,57]. The effect of the (001) surface on the band structure is the least significant among the three crystalline phases, decreasing the band gap by about 0.3 eV. This can be rationalized by the low surface energy of this surface [22], as well as by the substantial slab thickness we used (6 Ti layers vs. 4 Ti layers for the rutile (110) slab, which shows the strongest effect of the surface on the gap; see Figure 2, Figure 3 and Figure 4).
a-TiO2: The optimization in DFTB+ resulted in some atomic relaxation, but largely preserved the original structure of [52]. The energy per formula unit is −214.795 eV, i.e., metastability by about 0.13 eV per atom vs. anatase, similar to that reported in [52]. The density of states shown in Figure 1 for bulk a-TiO2 shows a similar gap of about 3 eV to that of the crystalline phases, similar to the report of [52]. The introduction of the surface gives rise to multiple states in the band gap, also shown in Figure 1.
The reduction of the bandgap is partly due to the limited thickness of the slab and the fixation of the bottom layers. The reduction is much less severe if all atoms are allowed to relax (i.e., a nanosheet model), as shown in Figure 1 for anatase and rutile (orange lines). We have also performed a plane wave DFT calculation on the same slab of anatase (surface and nanosheet) using the Vienna ab initio simulation package (VASP) code [74,75,76,77], the PBE functional [78], PAW pseudopotentials [79] and a cutoff energy of 600 eV and confirmed that the same effect is observed. Similar to DFTB results, no significant effect on the densities of state (DOS) of molecular adsorption was observed.

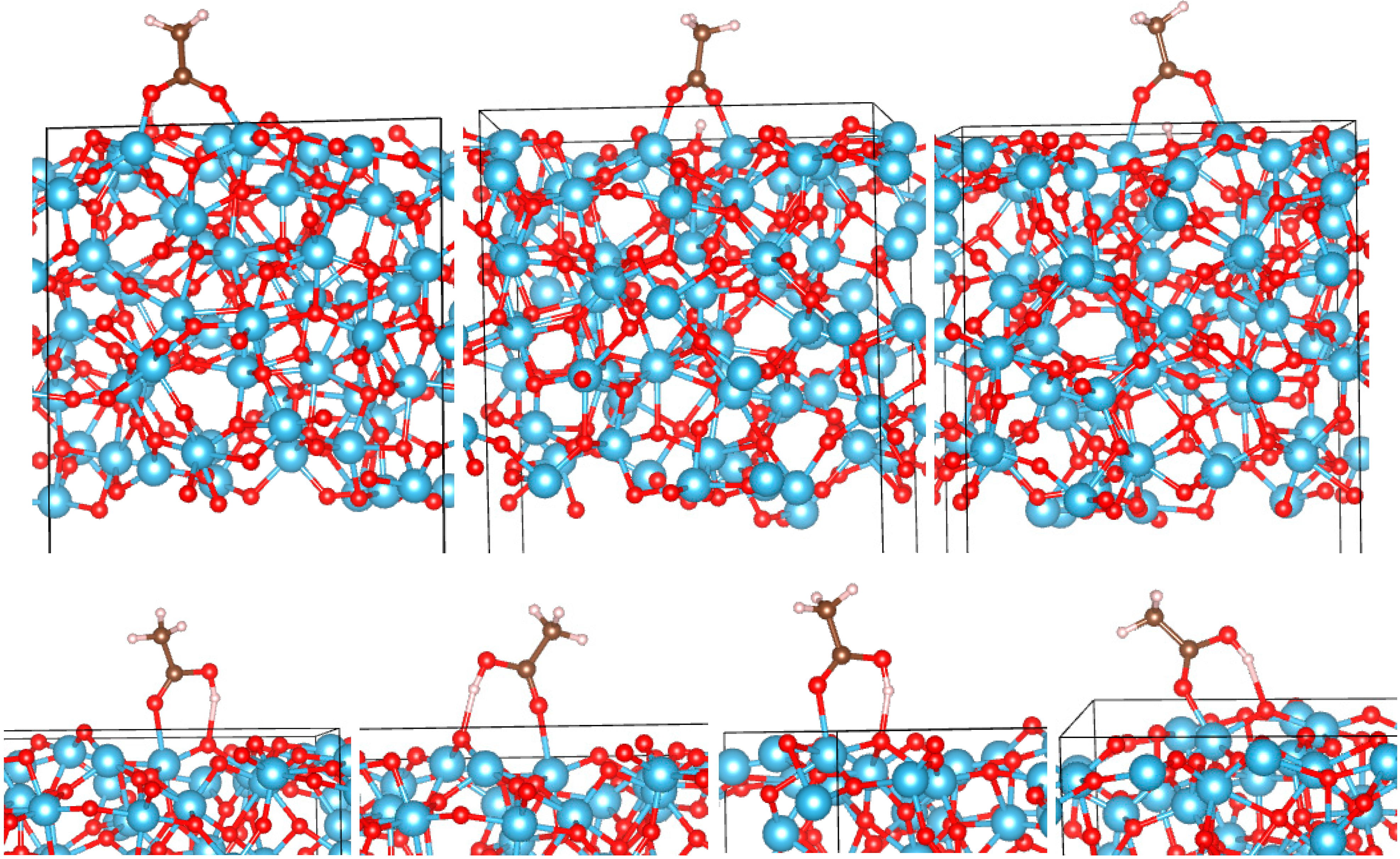
3.2. Adsorption Configurations of AcOH and Energetics

Anatase (101): Similar to previous works [31,80], a bidentate (BB) and two monodentate configurations (M1 and M2) were identified. These are shown in Figure 2, and their adsorption energies are listed in Table 1. The BB mode is therefore preferred. In the bidentate configuration, both oxygen atoms of the carboxylic group bind to surface Ti atoms with both Omol-Ti bonds equal to 2.22 Å. The H atom is dissociated from the molecule and is bound to a surface oxygen. In the monodentate configurations, the molecules are undissociated, with Omol-Ti bonds of 2.26 Å and hydrogen bonds between the H atom and a surface oxygen of 1.62 Å, in both M1 and M2 configurations. Molecular adsorption has no appreciable effect on the band structure around the gap regardless of the configuration (Figure 1).
Molecules 20 03371 g002 550
Figure 2. Adsorption configurations of AcOH on the anatase (101) surface of TiO2: bidentate (BB) (left), M1 (monodentate) (middle) and M2 (right). The atom color code here and elsewhere: Ti, blue; O, red; C, brown; H, light grey. Visualization here and elsewhere by VESTA [87].
Figure 2. Adsorption configurations of AcOH on the anatase (101) surface of TiO2: bidentate (BB) (left), M1 (monodentate) (middle) and M2 (right). The atom color code here and elsewhere: Ti, blue; O, red; C, brown; H, light grey. Visualization here and elsewhere by VESTA [87].
Molecules 20 03371 g002
For AcOH on anatase (101), we can compare the adsorption energies computed with DFTB (Table 1) to previous DFT calculations. Using the SIESTA code [81], the PBE functional [78], broad DZP basis functions and Troullier–Martins pseudopotentials [82], Chan et al. computed Eads of −1.71, −1.56 and −1.50 eV for the BB, M1 and M2 configurations, respectively, using a thinner slab than in the present study [80]. Spreafico et al. [30] computed Eads values of −1.25 and −1.27 eV for the BB and M1 configurations, respectively, by using the CP2K code [83], the PBE functional, Goedecker pseudopotentials [84], a mixed Gaussian-plane wave (GPW) basis [85], also employing the dispersion correction scheme of Grimme [86]. The values were also dependent on slab size [30]. In [31], the adsorption strength of the M1, M2 and BB modes was compared with different DFT setups, including a cluster of slab TiO2 models, localized or plane wave basis functions and GGA or hybrid functionals. The stability of M1 and M2 relative to BB was ± 0.5 eV [31]. We have also made a plane-wave DFT calculation with VASP [74,75,76,77], using the PBE functional, PAW pseudopotentials [79] and a plane wave cutoff of 600 eV. We obtained Eads values of −0.91, −0.95 and −0.91 eV for M1, M2 and BB configurations, respectively. The differences in the values of Eads of the order of half an eV observed among different commonly accepted DFT setups, including differences of the order of 0.5 eV in relative Eads for different adsorption configurations, highlight the need to use one and the same setup to obtain truly comparative values of Eads among different phases and configurations. Interestingly, a measured infrared spectrum of AcOH on the anatase (101) surface pointed to bidentate adsorption [31].
Table
Table 1. Adsorption energies Eads of AcOH in different configurations on anatase (101), rutile (110) and (B) (001) surfaces of TiO2, as well as on two amorphous surfaces. The bond length for bonding between the molecule’s and surface atoms, as well as charges on the molecule q are also given. For bidentate configurations, the two bond lengths are Omol-Ti; for monodentate, they are Omol-Ti and H-Osurf.
Table 1. Adsorption energies Eads of AcOH in different configurations on anatase (101), rutile (110) and (B) (001) surfaces of TiO2, as well as on two amorphous surfaces. The bond length for bonding between the molecule’s and surface atoms, as well as charges on the molecule q are also given. For bidentate configurations, the two bond lengths are Omol-Ti; for monodentate, they are Omol-Ti and H-Osurf.
SystemEads, eVq, |e|Omol-Ti, ÅOmol-Ti/H-Osurf, Å
Anatase (101)
BB−1.93−0.122.222.22
M1−0.930.162.261.62
M2−1.060.172.261.62
Rutile (110)
BB−2.49−0.112.212.21
M1−1.040.172.261.60
M2B−1.110.342.272.29
B (001)
BB1−0.74−0.172.242.25
BB2−0.41−0.182.252.25
BB3−1.07−0.162.232.24
M1−0.780.172.271.83
M2−0.790.182.271.87
M3−0.860.192.271.97
M4−0.700.172.271.87
M5−0.790.192.272.08
M6−0.750.182.271.86
a-TiO2
top BB1−2.600.031.932.25
top BB2−3.560.001.942.24
top BB3−2.96−0.031.932.27
top M1−2.200.331.961.60
top M2−2.400.351.951.60
top M3−2.380.351.951.63
top M4−0.970.182.261.63
top M5−0.800.162.261.62
bottom BB1−4.430.041.911.95
bottom BB2−4.920.081.972.00
bottom BB3−0.28−0.072.212.22
bottom M1−1.180.182.251.69
bottom M2−0.840.142.251.63
bottom M3−1.530.192.231.62
bottom M4−1.830.141.931.77
Rutile (110): Two bidentate (BB and M2B) and a monodentate configuration (M1) are identified. These are shown in Figure 3, and their adsorption energies are listed in Table 1. In the bidentate BB configuration, both oxygen atoms of the carboxylic group bind to surface Ti atoms with both Omol-Ti bonds equal to 2.21 Å. The H atom is dissociated from the molecule and is bound to a surface oxygen. In the monodentate configuration (M1), the molecule is undissociated, with Omol-Ti bonds of 2.26 Å and hydrogen bonds between the H atom and a surface oxygen of 1.60 Å. The configuration M2B was attempted as a monodentate configuration, where the molecular hydrogen coordinates to an in-plane oxygen atom of TiO2. This configuration, however, converged to a bidentate configuration in which AcOH preserves the H atom and in which a hydrogen atom of the CH3 group is 2.21 Å away from a surface O atom. This configuration is less stable than the dissociated bidentate configuration by more than 1 eV and has larger Omol-Ti bond lengths (2.27 and 2.29 Å) than the dissociated configuration. Similar to anatase, molecular adsorption has no appreciable effect on the band structure regardless of the configuration (Figure 1). The energetic preference of AcOH for bidentate configurations computed here (Table 1) is in agreement with known preference for bidentate bridging adsorption on the clean (110) surface identified experimentally [88,89].
Molecules 20 03371 g003 550
Figure 3. Adsorption configurations of AcOH on the rutile (110) surface of TiO2: BB (left), M1 (middle) and M2B (right).
Figure 3. Adsorption configurations of AcOH on the rutile (110) surface of TiO2: BB (left), M1 (middle) and M2B (right).
Molecules 20 03371 g003
(B) (001): Three bidentate (BB1 to BB3) and six monodentate configurations (M1 to M6) were identified and are shown in Figure 4. Their adsorption energies are listed in Table 1. BB1 and BB2 configurations, while visually similar, differ in that the COO group straddles different types of surface oxygen atoms, located in a slightly more “sagged” or slightly more protruding oxygen rows, respectively. This corresponds to significantly different Eads (−0.74 and −0.41 eV, respectively). The strongest bound configuration (Eads = −1.11 eV) is BB3, which straddles different Ti rows. In the BB configurations, both oxygen atoms of the carboxylic group bind to surface Ti atoms with both Omol-Ti bond lengths within 2.23–2.25 Å, with the shortest lengths corresponding to the strongest-bound BB3 configuration. In all monodentate configurations, the Omol-Ti bond length is 2.27 Å; the Hmol-Osurf bonds are spread within 1.86–2.08 Å. Similar to anatase and rutile, molecular adsorption has no appreciable effect on the band structure regardless of the configuration (Figure 1; not all M configurations are shown to prevent clutter, but no configuration changed the DOS).
Molecules 20 03371 g004 550
Figure 4. Adsorption configurations of AcOH on the (B) (001) surface of TiO2. Top row: BB1 to BB3 (left to right). Bottom rows: M1 to M6 (left to right and top to bottom).
Figure 4. Adsorption configurations of AcOH on the (B) (001) surface of TiO2. Top row: BB1 to BB3 (left to right). Bottom rows: M1 to M6 (left to right and top to bottom).
Molecules 20 03371 g004
a-TiO2: On both surfaces of a-TiO2 (“top” and “bottom”), we have identified a total of six bidentate and nine monodentate configurations. They are shown in Figure 5 and Figure 6, and their adsorption energies are listed in Table 1. While it is possible that other adsorption configurations exist on these surfaces, the 15 that we identified already provide an understanding of the strength of binding and the spread of adsorption energies on different sites of amorphous TiO2. Similar to the crystalline surfaces, bidentate binding is energetically preferred. The Omol-Ti bonds in BB configurations are generally shorter than on crystalline surfaces, the shortest reaching 1.91 Å (Table 1). While there is a dispersion of Eads values among both monodentate and bidentate configurations, the strongest Eads is larger than that of any crystalline surface in either the monodentate (reaching −1.83 eV) or bidentate (reaching −4.92 eV) regimes. Amorphous TiO2 can therefore be used to achieve stable anchoring of organic molecules, such as dyes, that bind via a -COOH group.
Molecules 20 03371 g005 550
Figure 5. Adsorption configurations of AcOH on the “top” surface of a-TiO2. Top row: BB1 to BB3 (left to right). Bottom rows: M1 to M5 (left to right and top to bottom).
Figure 5. Adsorption configurations of AcOH on the “top” surface of a-TiO2. Top row: BB1 to BB3 (left to right). Bottom rows: M1 to M5 (left to right and top to bottom).
Molecules 20 03371 g005

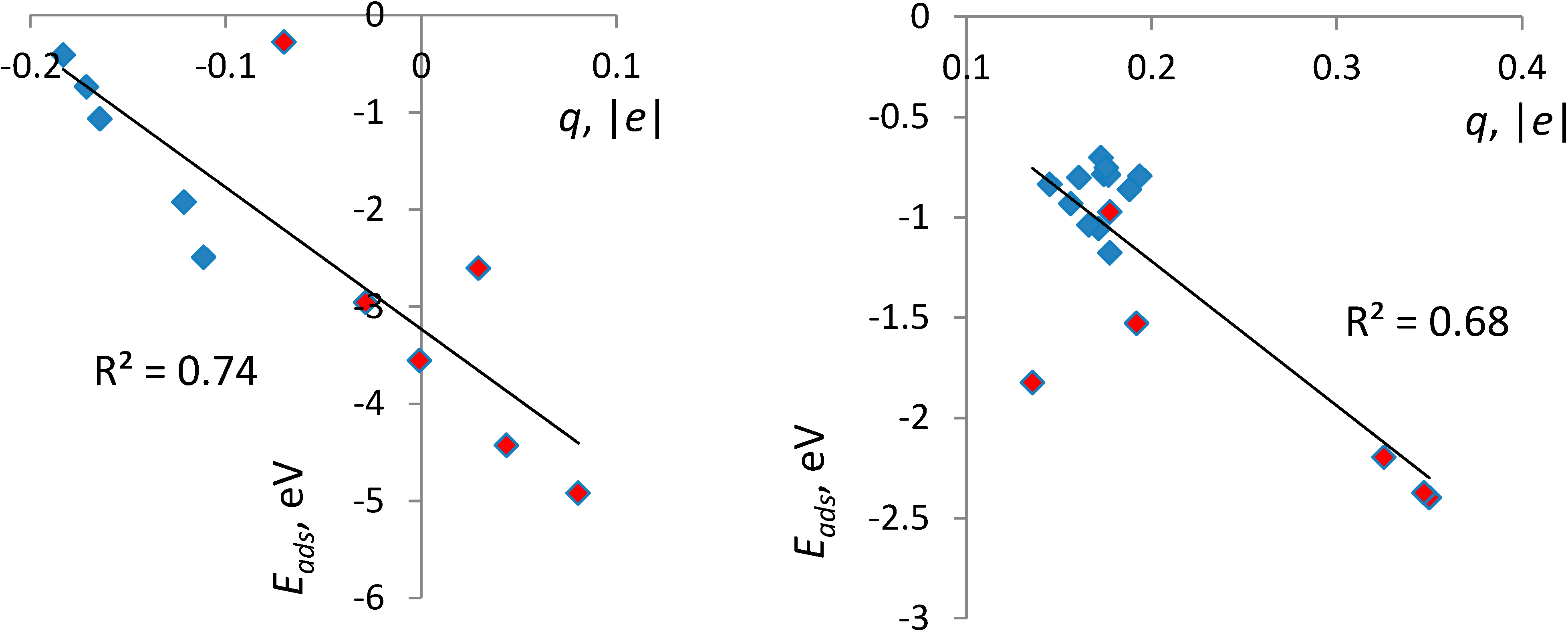
3.3. Correlates of Adsorption Strength

On all surfaces, bidentate configurations are more strongly bound to the surface than monodentate, and from Table 1, it can be seen that increased binding strength generally corresponds to shorter molecule-surface distances and more positive charge on the molecule. In Figure 7, we quantify this dependence by plotting Eads vs. the molecular charge q. Points corresponding to adsorption on amorphous surfaces are highlighted. There is a significant linear trend, with only two outlier points for bidentate and one outlier for monodentate configurations, with all outliers corresponding to a-TiO2. This is not surprising given the expected differences of the chemical environments of the adsorbed molecule in different parts of an amorphous surface. Even so, the correlation is significant, with Pearson correlation coefficients (R2) of 0.69 and 0.88 for bidentate and monodentate configurations, respectively. Figure 7 also highlights that Eads in excess of −3 eV is only achieved in bidentate modes on a-TiO2 and only with positive q values, in contrast with bidentate binding on crystalline surfaces, where the molecule accepts charge (we note that the scales of q should not be directly compared for BB and M configurations, as in one case, H is dissociated and in the other, it is not; see Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6).
Molecules 20 03371 g006 550
Figure 6. Adsorption configurations of AcOH on the “bottom” surface of a-TiO2. Top row: BB1 to BB3 (left to right). Bottom row: M1 to M4 (left to right).
Figure 6. Adsorption configurations of AcOH on the “bottom” surface of a-TiO2. Top row: BB1 to BB3 (left to right). Bottom row: M1 to M4 (left to right).
Molecules 20 03371 g006
Molecules 20 03371 g007 550
Figure 7. Dependence of the adsorption energy Eads on the molecular charge q in bidentate (left) and monodentate (right) configurations on all surfaces and their linear regressions. Points corresponding to a-TiO2 are highlighted in red.
Figure 7. Dependence of the adsorption energy Eads on the molecular charge q in bidentate (left) and monodentate (right) configurations on all surfaces and their linear regressions. Points corresponding to a-TiO2 are highlighted in red.
Molecules 20 03371 g007
Trends can also be identified between the adsorption energy and the shorter Omol-Ti distance, with correlation coefficients (R2) of 0.69 and 0.88 for bidentate and monodentate configurations, respectively. A combined linear regression in these two variables (i.e., charge and bond length) unveils a significant correlation with the correlation coefficient of 0.75 and 0.93 for bidentate and monodentate configurations, respectively.

4. Conclusions

We have presented the first comparative density functional tight binding study of acetic acid adsorption on anatase (101), rutile (110), B (001) and amorphous surfaces of TiO2. On all surfaces, bidentate bridging adsorption configurations are preferred, consistent with available data. The computed adsorption energies of the lowest-energy bidentate configurations Eads = −1.93, −2.49 and −1.09 eV on anatase, rutile and (B)-TiO2, respectively. For monodentate configurations, the strongest Eads = −1.06, −1.11 and −0.86 eV on anatase, rutile and (B)-TiO2, respectively. The comparison with previously reported DFT values for Eads computed with different computational setups highlights the need to use one and the same setup to obtain truly comparative values of Eads among different phases and configurations.
Multiple monodentate and bidentate configurations are identified on a-TiO2 with a distribution of adsorption energies and with the lowest binding energy stronger than that of the crystalline counterparts, with Eads up to −4.92 eV for bidentate and −1.83 eV for monodentate adsorption. Amorphous TiO2 can therefore be used to achieve strong anchoring of organic molecules, such as dyes, that bind via a -COOH group. While the presence of the surface leads to a contraction of the band gap vs. bulk, molecular adsorption caused no appreciable effect on the band structure around the gap in any of the systems.
Then, comparison among five surfaces (three crystalline and two amorphous) and a total of 30 binding geometries and energies allowed us to identify correlations between the binding strength, the charge donation at the interface and bond lengths between molecular and surface atoms. There is a strong positive correlation between the adsorption strength and the positive charge on the molecule, as well as between Eads and the shortest bond length between molecular O and surface Ti atoms.

Acknowledgments

This work was supported by an AcRF Tier 1 grant by the Ministry of Education of Singapore (WBS R-265-000-494-112). S.M. thanks Fleur Legrain for technical assistance and Thomas Frauenheim and Balint Aradi, the University of Bremen, for the discussion about the DFTB method. K.Y. acknowledges the funding from the Ministry of Education, Culture, Sports, Science and Technology, Japan (MEXT).

Author Contributions

S.M. and G.G. did the calculations and wrote the text. S.M. conceived the project. K.Y. provided project guidance and input factors.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Sorescu, D.C.; Civiš, S.; Jordan, K.D. Mechanism of oxygen exchange between CO2 and TiO2(101) anatase. J. Phys. Chem. C 2014, 118, 1628–1639. [Google Scholar] [CrossRef]
  2. Müller, K.; Lu, D.; Senanayake, S.D.; Starr, D.E. Monoethanolamine adsorption on TiO2(110): Bonding, structure, and implications for use as a model solid-supported CO2 capture material. J. Phys. Chem. C 2013, 118, 1576–1586. [Google Scholar] [CrossRef]
  3. Drew, K.; Girishkumar, G.; Vinodgopal, K.; Kamat, P.V. Boosting fuel cell performance with a semiconductor photocatalyst: TiO2/Pt-Ru hybrid catalyst for methanol oxidation. J. Phys. Chem. B 2005, 109, 11851–11857. [Google Scholar] [CrossRef] [PubMed]
  4. Liu, Y.; Li, J.; Zhou, B.; Chen, H.; Wang, Z.; Cai, W. A TiO2-nanotube-array-based photocatalytic fuel cell using refractory organic compounds as substrates for electricity generation. Chem. Commun. 2011, 47, 10314–10316. [Google Scholar] [CrossRef]
  5. Upadhyaya, H.M.; Senthilarasu, S.; Hsu, M.-H.; Kumar, D.K. Recent progress and the status of dye-sensitised solar cell (DSSC) technology with state-of-the-art conversion efficiencies. Sol. Energy Mater. Sol. Cells 2013, 119, 291–295. [Google Scholar] [CrossRef]
  6. Ning, Z.; Fu, Y.; Tian, H. Improvement of dye-sensitized solar cells: What we know and what we need to know. Energy Environ. Sci. 2010, 3, 1170–1181. [Google Scholar] [CrossRef]
  7. Jung, H.S.; Lee, J.-K. Dye sensitized solar cells for economically viable photovoltaic systems. J. Phys. Chem. Lett. 2013, 4, 1682–1693. [Google Scholar] [CrossRef]
  8. Hagfeldt, A.; Boschloo, G.; Sun, L.; Kloo, L.; Pettersson, H. Dye-sensitized solar cells. Chem. Rev. 2010, 110, 6595–6663. [Google Scholar] [CrossRef] [PubMed]
  9. Bai, Y.; Mora-Sero, I.; de Angelis, F.; Bisquert, J.; Wang, P. Titanium dioxide for photovoltaic applications. Chem. Rev. 2014, 114, 10095–10130. [Google Scholar] [CrossRef] [PubMed]
  10. Kojima, A.; Teshima, K.; Shirai, Y.; Miyasaka, T. Organometallic halide perovskites as visible-light sensitizers for photovoltaic cells. J. Am. Chem. Soc. 2009, 131, 6050–6051. [Google Scholar] [CrossRef] [PubMed]
  11. Kim, H.-S.; Lee, C.-R.; Im, J.-H.; Lee, K.-B.; Moehl, T.; Marchioro, A.; Moon, S.-J.; Humphry-Baker, R.; Yum, J.-H.; Moser, J.E.; et al. Lead iodide perovskite sensitized all-solid-state submicron thin film mesoscopic solar cell with efficiency exceeding 9%. Sci. Rep. 2012, 2, 591. [Google Scholar] [CrossRef] [PubMed]
  12. Deng, D.; Kim, M.G.; Lee, J.Y.; Chi, J. Green energy storage materials: Nanostructured TiO2 and Sn-based anodes for lithium-ion batteries. Energy Environ. Sci. 2009, 2, 818–837. [Google Scholar] [CrossRef]
  13. Yang, Z.; Choi, D.; Kerisit, S.; Rosso, K.M.; Wang, D.; Zhang, J.; Graff, G.; Liu, J. Nanostructures and lithium electrochemical reactivity of lithium titanites and titanium oxides: A review. J. Power Sources 2009, 192, 588–598. [Google Scholar] [CrossRef]
  14. Liu, S.; Hu, J.J.; Yan, N.F.; Pan, G.L.; Li, G.R.; Gao, X.P. Aluminum storage behavior of anatase TiO2 nanotube arrays in aqueous solution for aluminum ion batteries. Energy Environ. Sci. 2012, 5, 9743–9746. [Google Scholar] [CrossRef]
  15. Dylla, A.G.; Henkelman, G.; Stevenson, K.J. Lithium insertion in nanostructured TiO2(B) architectures. Acc. Chem. Res. 2013, 46, 1104–1112. [Google Scholar] [CrossRef] [PubMed]
  16. Myung, S.-T.; Kikuchi, M.; Yoon, C.S.; Yashiro, H.; Kim, S.-J.; Sun, Y.-K.; Scrosati, B. Black anatase titania enabling ultra high cycling rates for rechargeable lithium batteries. Energy Environ. Sci. 2013, 6, 2609–2614. [Google Scholar] [CrossRef]
  17. Li, W.; Wang, F.; Feng, S.; Wang, J.; Sun, Z.; Li, B.; Li, Y.; Yang, J.; Elzatahry, A.; Xia, Y.; et al. Sol–gel design strategy for ultradispersed TiO2 nanoparticles on graphene for high-performance lithium ion batteries. J. Am. Chem. Soc. 2013, 135, 18300–18303. [Google Scholar] [CrossRef] [PubMed]
  18. Kim, K.-T.; Ali, G.; Chung, K.Y.; Yoon, C.S.; Yashiro, H.; Sun, Y.-K.; Lu, J.; Amine, K.; Myung, S.-T. Anatase titania nanorods as an intercalation anode material for rechargeable sodium batteries. Nano Lett. 2014, 14, 416–422. [Google Scholar] [CrossRef] [PubMed]
  19. Xu, H.; Zhang, R.Q.; Ng, A.M.C.; Djurisic, A.B.; Chan, H.T.; Chan, W.K.; Tong, S.Y. Splitting water on metal oxide surfaces. J. Phys. Chem. C 2011, 115, 19710–19715. [Google Scholar] [CrossRef]
  20. Xiang, G.; Wang, Y.-G.; Li, J.; Zhuang, J.; Wang, X. Surface-specific interaction by structure-match confined pure high-energy facet of unstable TiO2(B) polymorph. Sci. Rep. 2013, 3. [Google Scholar] [CrossRef]
  21. Niu, M.; Cheng, D.; Cao, D. Fluorite TiO2(111) surface phase for enhanced visible-light solar energy conversion. J. Phys. Chem. C 2014, 118, 20107–20111. [Google Scholar] [CrossRef]
  22. Vittadini, A.; Casarin, M.; Selloni, A. Structure and stability of TiO2-B surfaces: A Density Functional study. J. Phys. Chem. C 2009, 113, 18973–18977. [Google Scholar] [CrossRef]
  23. Perron, H.; Domain, C.; Roques, J.; Drot, R.; Simoni, E.; Catalette, H. Optimisation of accurate rutile TiO2 (110), (100), (101) and (001) surface models from periodic DFT calculations. Theor. Chem. Acc. 2007, 117, 565–574. [Google Scholar] [CrossRef] [Green Version]
  24. Giorgi, G.; Fujisawa, J.-I.; Segawa, H.; Yamashita, K. Unraveling the adsorption mechanism of aromatic and aliphatic diols on TiO2 surface: a density functional theory analysis. Phys. Chem. Chem. Phys. 2013, 15, 9761–9767. [Google Scholar] [CrossRef] [PubMed]
  25. Heckel, W.; Elsner, B.A.M.; Schulz, C.; Müller, S. The role of hydrogen on the adsorption behavior of carboxylic acid on TiO2 surfaces. J. Phys. Chem. C 2014, 118, 10771–10779. [Google Scholar] [CrossRef]
  26. Manzhos, S.; Segawa, H.; Yamashita, K. The effect of ligand substitution and water co-adsorption on the adsorption dynamics and energy level matching of amino-phenyl acid dyes on TiO2. Phys. Chem. Chem. Phys. 2012, 14, 1749–1755. [Google Scholar] [CrossRef] [PubMed]
  27. O’Rurke, C.; Bowler, D.R. Adsorption of thiophene-conjugated sensitizers on TiO2 anatase (101). J. Phys. Chem. C 2010, 114, 20240–20248. [Google Scholar] [CrossRef]
  28. Pastore, M.; de Angelis, F. Aggregation of organic dyes on TiO2 in dye-sensitized solar cells models: an ab initio investigation. ACS Nano 2010, 4, 556–562. [Google Scholar] [CrossRef] [PubMed]
  29. De Angelis, F.; Fantacci, S.; Gebauer, R. Simulating dye-sensitized TiO2 heterointerfaces in explicit solvent: Absorption spectra, energy levels, and dye desorption. J. Phys. Chem. Lett. 2011, 2, 813–817. [Google Scholar] [CrossRef]
  30. Spreafico, C.; Schiffmann, F.; VandeVondele, J. Structure and mobility of acetic acid at the anatase (101)/acetonitrile interface. J. Phys. Chem. C 2014, 118, 6251–6260. [Google Scholar] [CrossRef]
  31. Anselmi, C.; Mosconi, E.; Pastore, M.; Ronca, E.; de Angelis, F. Adsorption of organic dyes on TiO2 surfaces in dye-sensitized solar cells: interplay of theory and experiment. Phys. Chem. Chem. Phys. 2012, 14, 15963–15974. [Google Scholar] [CrossRef] [PubMed]
  32. Vittadini, A.; Selloni, A.; Rotzinger, F.P.; Gra1tzel, M. Formic acid adsorption on dry and hydrated TiO2 Anatase (101) surfaces by DFT calculations. J. Phys. Chem. B 2000, 104, 1300–1306. [Google Scholar] [CrossRef]
  33. Agosta, L.; Zollo, G.; Arcangeli, C.; Buonocore, F.; Gala, F.; Celino, M. Water driven adsorption of amino acids on the (101) anatase TiO2 surface: An ab initio study. Phys. Chem. Chem. Phys. 2015, 17, 1556–1561. [Google Scholar] [CrossRef] [PubMed]
  34. Coropceanu, V.; Cornil, J.; da Silva Filho, D.A.; Olivier, Y.; Silbey, R.; Brédas, J.L. Charge transport in organic semiconductors. Chem. Rev. 2007, 107, 926–952. [Google Scholar] [CrossRef]
  35. Borghols, W.J.H.; Wagemaker, M.; Lafont, U.; Kelder, E.M.; Mulder, F.M. Impact of nanosizing on lithiated rutile TiO2. Chem. Mater. 2008, 20, 2949–2955. [Google Scholar] [CrossRef]
  36. Armstrong, A.R.; Arrouvel, C.; Gentili, V.; Parker, S.C.; Saiful Islam, M.; Bruce, P. Lithium coordination sites in LixTiO2(B): A structural and computational study. Chem. Mater. 2010, 22, 6426–6432. [Google Scholar] [CrossRef]
  37. Borghols, W.J.H.; Lützenkirchen-Hecht, D.; Haake, U.; van Eck, E.R.H.; Muldera, F.M.; Wagemaker, M. The electronic structure and ionic diffusion of nanoscale LiTiO2 anatase. Phys. Chem. Chem. Phys. 2009, 11, 5742–5748. [Google Scholar] [CrossRef] [PubMed]
  38. Kang, J.; Wei, S.-H.; Kim, Y.-H. First-principles theory of electrochemical capacitance of nanostructured materials: Dipole-assisted subsurface intercalation of lithium in pseudocapacitive TiO2 anatase nanosheets. J. Phys. Chem. C 2011, 115, 4909–4915. [Google Scholar] [CrossRef]
  39. Sushko, M.L.; Rosso, K.M.; Liu, J. Mechanism of Li+/electron conductivity in rutile and anatase TiO2 nanoparticles. J. Phys. Chem. C 2010, 114, 20277–20283. [Google Scholar] [CrossRef]
  40. Eithiraj, R.D.; Geethalakshmi, K.R. Suitability of amorphous TiO2 nanoparticles as a photoelectrode in dye sensitized solar cells: A DFT–TDDFT study. Chem. Phys. Lett. 2013, 585, 138–142. [Google Scholar] [CrossRef]
  41. Tang, X.; Liu, X.; Zhang, L.; Xing, Y.; Tian, Y. Low crystallinity TiO2 film with inherent low oxygen vacancy for sensitized solar cells. Chem. Phys. 2014, 441, 121–127. [Google Scholar] [CrossRef]
  42. Kakuta, N.; Oku, T.; Suzuki, A.; Kikuchi, K.; Kikuchi, S. Effect of an amorphous TiO2 addition on dye-sensitized solar cells with organic dyes. J. Ceram. Process. Res. 2012, 13, 28–31. [Google Scholar]
  43. Xiong, H.; Slater, M.D.; Balasubramanian, M.; Johnson, C.S.; Rajh, T. Amorphous TiO2 nanotube anode for rechargeable sodium ion batteries. J. Phys. Chem. Lett. 2011, 2, 2560–2565. [Google Scholar] [CrossRef]
  44. Borghols, W.J.H.; Lützenkirchen-Hecht, D.; Haake, U.; Chan, W.; Lafont, U.; Kelder, E.M.; van Eck, E.R.H.; Kentgens, A.P.M.; Mulder, F.M.; Wagemaker, M. Lithium storage in amorphous TiO2 nanoparticles. J. Electrochem. Soc. 2010, 157, A582–A588. [Google Scholar] [CrossRef]
  45. Hibino, M.; Abe, K.; Mochizuki, M.; Miyayama, M. Amorphous titanium oxide electrode for high-rate discharge and charge. J. Power Sources 2004, 126, 139–143. [Google Scholar] [CrossRef]
  46. Sun, X.; Xie, M.; Travis, J.J.; Wang, G.; Sun, H.; Lian, J.; George, S.M. Pseudocapacitance of amorphous TiO2 thin films anchored to graphene and carbon nanotubes using atomic layer deposition. J. Phys. Chem. C 2013, 117, 22497–22508. [Google Scholar] [CrossRef]
  47. Hai-Tao, F.; Min, L.; Da-Wei, W.; Tao, S.; Dong-Sheng, G.; Feng, L.; Jigang, Z.; Tsun-Kong, S.; Hui-Ming, C. Comparison of the rate capability of nanostructured amorphous and anatase TiO2 for lithium insertion using anodic TiO2 nanotube arrays. Nanotechnology 2009, 20. [Google Scholar] [CrossRef] [PubMed]
  48. Yildirim, H.; Greeley, J.P.; Sankaranarayanan, S.K.R.S. Localized order–disorder transitions induced by Li segregation in amorphous TiO2 nanoparticles. ACS Appl. Mater. Interfaces 2014, 6, 18962–18970. [Google Scholar] [CrossRef] [PubMed]
  49. Kaur, K.; Singh, C.V. Amorphous TiO2 as a photocatalyst for hydrogen production: A DFT study of structural and electronic properties. Energy Procedia 2012, 29, 291–299. [Google Scholar] [CrossRef]
  50. Legrain, F.; Malyi, O.; Manzhos, S. Insertion energetics of lithium, sodium, and magnesium in crystalline and amorphous titanium dioxide: A comparative first-principles study. J. Power Sources 2015, 278, 197–202. [Google Scholar] [CrossRef]
  51. Legrain, F.; Malyi, O.; Manzhos, S. Comparative ab initio study of lithium storage in amorphous and crystalline TiO2. In Proceedings of the 14th Asian Conference on Solid State Ionics, Singapore, Singapore, 24–27 June 2014.
  52. Prasai, B.; Cai, B.; Underwood, M.K.; Lewis, J.P.; Drabold, D.A. Properties of amorphous and crystalline titanium dioxide from first principles. J. Mater. Sci. 2012, 47, 7515–7521. [Google Scholar] [CrossRef]
  53. Dolgonos, G. Self-consistent-charge density-functional tight-binding molecular dynamics simulations of amorphous TiO2 growth. Surf. Sci. 2014, 621, 51–54. [Google Scholar] [CrossRef]
  54. Elstner, M.; Porezag, D.; Jungnickel, G.; Elsner, J.; Haugk, M.; Frauenheim, T.; Suhai, S.; Seifert, G. Self-consistent-charge density-functional tight-binding method for simulations of complex materials properties. Phys. Rev. B 1998, 58, 7260–7268. [Google Scholar] [CrossRef]
  55. Aradi, B.; Hourahine, B.; Frauenheim, T. DFTB+, a sparse matrix-based implementation of the DFTB method. J. Phys. Chem. A 2007, 111, 5678–5684. [Google Scholar] [CrossRef] [PubMed]
  56. Gemming, S.; Enyashin, A.; Frenzel, J.; Seifert, G. Adsorption of nucleotides on the rutile (110) surface. Int. J. Mater. Res. 2010, 101, 758–764. [Google Scholar] [CrossRef]
  57. Zhu, T.; Gao, S.-P. The stability, electronic structure, and optical property of TiO2 polymorphs. J. Phys. Chem. C 2014, 118, 11385–11396. [Google Scholar] [CrossRef]
  58. Burdett, J.K.; Hughbanks, T.; Miller, G.J.; Richardson, J.W.; Smith, J.V. Structural-electronic relationships in inorganic solids: Powder neutron diffraction studies of the rutile and anatase polymorphs of titanium dioxide at 15 and 295 K. J. Am. Chem. Soc. 1987, 109, 3639–3646. [Google Scholar] [CrossRef]
  59. Horn, M.; Schwerdtfeger, C.F.; Meagher, E.P. Refinement of the structure of anatase at several temperatures. Z. Kristallogr. 1972, 136, 273–281. [Google Scholar] [CrossRef]
  60. Asahi, R.; Taga, Y.; Mannstadt, W.; Freeman, A.J. Electronic and optical properties of anatase TiO2. Phys. Rev. B 2000, 61, 7459–7465. [Google Scholar] [CrossRef]
  61. Labat, F.; Baranek, P.; Domain, C.; Minot, C.; Adamo, C. Density functional theory analysis of the structural and electronic properties of TiO2 rutile and anatase polytypes: Performances of different exchange-correlation functionals. J. Chem. Phys. 2007, 126, 154703–12. [Google Scholar] [CrossRef] [PubMed]
  62. Tang, H.; Berger, H.; Schmid, P.E.; Lévy, F.; Burri, G. Photoluminescence in TiO2 anatase single crystals. Solid State Commun. 1993, 87, 847–850. [Google Scholar] [CrossRef]
  63. Dolgonos, G.; Aradi, B.; Moreira, N.H.; Frauenheim, T. An improved self-consistent-charge Density-Functional Tight-Binding (SCC-DFTB) set of parameters for simulation of bulk and molecular systems involving titanium. J. Chem. Theory Comput. 2010, 6, 266–278. [Google Scholar] [CrossRef]
  64. Tezuka, Y.; Shin, S.; Lshii, T.; Ejima, T.; Suzuki, S.; Sato, S. Photoemission and Bremsstrahlung Isochromat spectroscopy studies of TiO2 (Rutile) and SrTiO3. J. Phys. Soc. Jpn. 1994, 63, 347–357. [Google Scholar] [CrossRef]
  65. Pascual, J.; Camassel, J.; Mathieu, H. Resolved quadrupolar transition in TiO2. Phys. Rev. Lett. 1977, 39, 1490–1493. [Google Scholar] [CrossRef]
  66. Pascual, J.; Camassel, J.; Mathieu, H. Fine structure in the intrinsic absorption edge of TiO2. Phys. Rev. B 1978, 18, 5606–5614. [Google Scholar] [CrossRef]
  67. CRC Handbook of Chemistry and Physics, 67th ed.; CRC: Cleveland, OH, USA, 1986.
  68. Chase, M.W.; Davies, C.A.; Downey, J.R.; Frurip, D.J.; McDonald, R.A.; Syverud, A.N. JANAF Thermodynamical Tables, 3rd ed. J. Phys. Chem. Ref. Data 1985, 14 (Suppl. 1), 1680. [Google Scholar]
  69. Hamad, B.A. First-principle calculations of structural and electronic properties of rutile-phase dioxides (MO2), M = Ti, V, Ru, Ir and Sn. Eur. Phys. J. B 2009, 70, 163–169. [Google Scholar] [CrossRef]
  70. Lazzeri, M.; Vittadini, A.; Selloni, A. Structure and energetics of stoichiometric TiO2 anatase surfaces. Phys. Rev. B 2001, 63, 155409. [Google Scholar] [CrossRef]
  71. Yang, H.G.; Sun, C.H.; Qiao, S.Z.; Zou, J.; Liu, G.; Smith, S.C.; Cheng, H.M.; Lu, G.Q. Anatase TiO2 single crystals with a large percentage of reactive facets. Nature 2008, 453, 638–641. [Google Scholar] [CrossRef] [PubMed]
  72. Yahia, M.B.; Lemoigno, F.; Beuvier, T.; Filhol, J.-S.; Richard-Plouet, M.; Brohan, L.; Doublet, M.-L. Updated references for the structural, electronic, and vibrational properties of TiO2(B) bulk using first-principles density functional theory calculations. J. Chem. Phys. 2009, 130, 204501–204511. [Google Scholar] [CrossRef] [PubMed]
  73. Betz, G.; Tributsch, H.; Marchand, R. Hydrogen insertion (intercalation) and light induced proton exchange at TiO2(B) -electrodes. J. Appl. Electrochem. 1984, 14, 315–322. [Google Scholar] [CrossRef]
  74. Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558. [Google Scholar] [CrossRef]
  75. Kresse, G.; Hafner, J. Ab initio molecular-dynamics simulation of the liquid-metal-amorphous-semiconductor transition in germanium. Phys. Rev. B: Condens. Matter. 1994, 49, 14251–14269. [Google Scholar] [CrossRef]
  76. Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mat. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
  77. Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
  78. Perdew, J.P.; Burke, K.; Ernzerhoff, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
  79. Blochl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17978. [Google Scholar] [CrossRef]
  80. Chan, M.; Carrington, T.; Manzhos, S. Anharmonic vibrations of the carboxyl group in acetic acid on TiO2: implications for adsorption mode assignment in dye-sensitized solar cells. Phys. Chem. Chem. Phys. 2013, 15, 10028–10034. [Google Scholar] [CrossRef] [PubMed]
  81. Soler, J.M.; Artacho, E.; Dale, J.D.; Garcia, A.; Junquera, J.; Ordejon, P.; Sanchez-Portal, D. The SIESTA method for ab initio order-N materials simulation. J. Phys.: Condens. Matter 2002, 14, 2745–2779. [Google Scholar] [CrossRef]
  82. Troullier, N.; Martins, J.L. Efficient pseudopotentials for plane-wave calculations. Phys. Rev. B 1991, 43, 1993–2006. [Google Scholar] [CrossRef]
  83. Hutter, J.; Iannuzzi, M.; Schiffmann, F.; VandeVondele, J. CP2K: Atomistic simulations of condensed matter systems. WIREs Comput. Mol. Sci. 2014, 4, 15–25. [Google Scholar] [CrossRef]
  84. Hartwigsen, C.; Goedecker, S.; Hutter, J. Relativistic separable dual-space Gaussian pseudopotentials from H to Rn. Phys. Rev. B 1998, 58, 3641–3662. [Google Scholar] [CrossRef]
  85. Lippert, G.; Hutter, J.; Parrinello, M. A hybrid Gaussian and plane wave Density Functional scheme. Mol. Phys. 1997, 92, 477–487. [Google Scholar] [CrossRef]
  86. Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed]
  87. Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
  88. Tao, J.; Luttrell, T.; Bylsma, J.; Batzill, M. Adsorption of acetic acid on rutile TiO2(110) vs. (011)-2 × 1 surfaces. J. Phys. Chem. C 2011, 115, 3434–3442. [Google Scholar] [CrossRef]
  89. Diebold, U. The surface science of titanium dioxide. Surf. Sci. Rep. 2003, 48, 53–229. [Google Scholar] [CrossRef]

Share and Cite

MDPI and ACS Style

Manzhos, S.; Giorgi, G.; Yamashita, K. A Density Functional Tight Binding Study of Acetic Acid Adsorption on Crystalline and Amorphous Surfaces of Titania. Molecules 2015, 20, 3371-3388. https://doi.org/10.3390/molecules20023371

AMA Style

Manzhos S, Giorgi G, Yamashita K. A Density Functional Tight Binding Study of Acetic Acid Adsorption on Crystalline and Amorphous Surfaces of Titania. Molecules. 2015; 20(2):3371-3388. https://doi.org/10.3390/molecules20023371

Chicago/Turabian Style

Manzhos, Sergei, Giacomo Giorgi, and Koichi Yamashita. 2015. "A Density Functional Tight Binding Study of Acetic Acid Adsorption on Crystalline and Amorphous Surfaces of Titania" Molecules 20, no. 2: 3371-3388. https://doi.org/10.3390/molecules20023371

Article Metrics

Back to TopTop