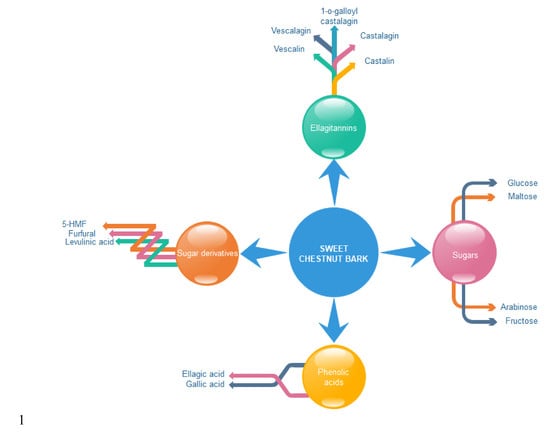
Subcritical Water Extraction of Chestnut Bark and Optimization of Process Parameters
Abstract
:1. Introduction
2. Results
2.1. Extraction Yield
2.2. Total Phenols, Total Tannins and Antioxidant Activity
2.3. Ellagic Acid, Gallic Acid and Ellagitannins
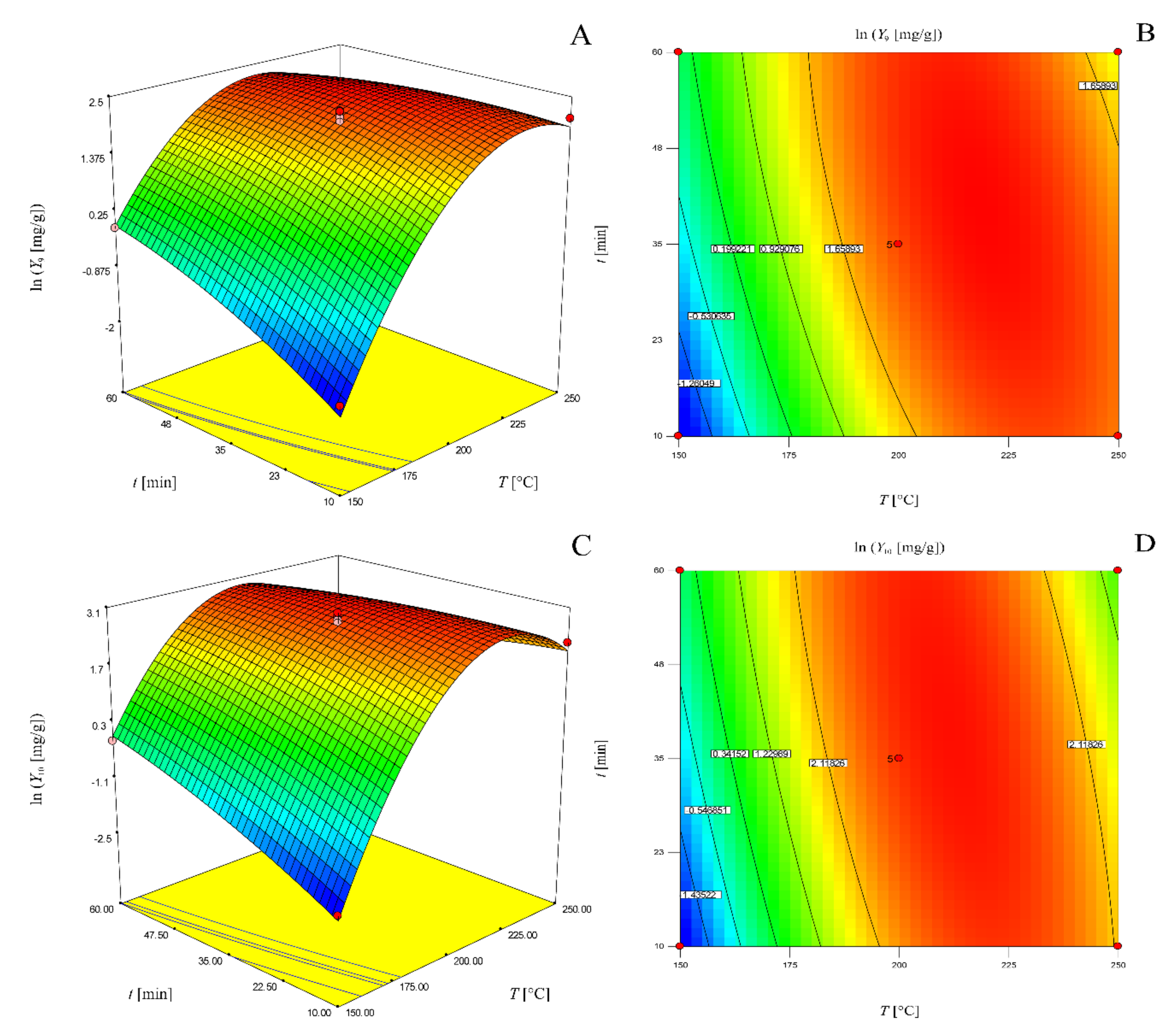
2.4. Sugars and Their Derivatives
2.5. Optimal Conditions
3. Materials and Methods
3.1. Materials
3.2. Subcritical Water Extraction
3.3. Total Phenols
3.4. Total Tannins
3.5. Antioxidant Activity
3.6. HPLC Analysis
3.7. Statistical Analysis
3.8. Optimization of Reaction Parameters
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gagić, T.; Knez, Ž.; Škerget, M. Hydrothermal hydrolysis of sweet chestnut (Castanea sativa) tannins. Serb. Chem. Soc. 2019, 84, 1–14. [Google Scholar]
- Squillaci, G.; Apone, F.; Sena, L.M.; Carola, A.; Tito, A.; Bimonte, M.; Lucia, A.D.; Colucci, G.; Cara, F.L.; Morana, A. Chestnut (Castanea sativa Mill.) industrial wastes as a valued bioresource for the production of active ingredients. Process. Biochem. 2018, 64, 228–236. [Google Scholar] [CrossRef]
- Zivkovic, J.; Mujic, I.; Zekovic, Z.; Nikolić, G.; Vidovic, S.; Mujić, A. Extraction and analysis of condensed tannins in castanea sativa mill. J. Cent. Eur. Agric. 2009, 10, 283–288. [Google Scholar]
- García-Niño, W.R.; Zazueta, C. Ellagic acid: Pharmacological activities and molecular mechanisms involved in liver protection. Pharm. Res. 2015, 97, 84–103. [Google Scholar] [CrossRef] [PubMed]
- De Vasconcelos, M.d.C.B.M.; Bennett, R.N.; Quideau, S.; Jacquet, R.; Rosa, E.A.S.; Ferreira-Cardoso, J.V. Evaluating the potential of chestnut (Castanea sativa Mill.) fruit pericarp and integument as a source of tocopherols, pigments and polyphenols. Ind. Crop. Prod. 2010, 31, 301–311. [Google Scholar] [CrossRef]
- De Vasconcelos, M.d.C.B.M.; Bennett, R.N.; Rosa, E.A.S.; Ferreira-Cardoso, J.V. Composition of European chestnut (Castanea sativa Mill.) and association with health effects: Fresh and processed products. J. Sci. Food Agric. 2010, 90, 1578–1589. [Google Scholar] [CrossRef] [PubMed]
- Chiarini, A.; Micucci, M.; Malaguti, M.; Budriesi, R.; Ioan, P.; Lenzi, M.; Fimognari, C.; Gallina Toschi, T.; Comandini, P.; Hrelia, S. Sweet chestnut (Castanea sativa Mill.) bark extract: Cardiovascular activity and myocyte protection against oxidative damage. Oxid. Med. Cell. Longev. 2013, 2013, 471790. [Google Scholar] [CrossRef] [Green Version]
- Bhat, T.K.; Singh, B.; Sharma, O.P. Microbial degradation of tannins—A current perspective. Biodegradation 1998, 9, 343–357. [Google Scholar] [CrossRef]
- Comandini, P.; Lerma-García, M.J.; Simó-Alfonso, E.F.; Toschi, T.G. Tannin analysis of chestnut bark samples (Castanea sativa Mill.) by HPLC-DAD–MS. Food Chem. 2014, 157, 290–295. [Google Scholar] [CrossRef]
- Landete, J.M. Ellagitannins, ellagic acid and their derived metabolites: A review about source, metabolism, functions and health. Food Res. Int. 2011, 44, 1150–1160. [Google Scholar] [CrossRef]
- Larrosa, M.; García-Conesa, M.T.; Espín, J.C.; Tomás-Barberán, F.A. Ellagitannins, ellagic acid and vascular health. Mol. Asp. Med. 2010, 31, 513–539. [Google Scholar] [CrossRef]
- Dinis, L.-T.; Oliveira, M.M.; Almeida, J.; Costa, R.; Gomes-Laranjo, J.; Peixoto, F. Antioxidant activities of chestnut nut of Castanea sativa Mill. (cultivar ‘Judia’) as function of origin ecosystem. Food Chem. 2012, 132, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Rodrigues, F.; Santos, J.; Pimentel, F.B.; Braga, N.; Palmeira-de-Oliveira, A.; Oliveira, M.B.P.P. Promising new applications of Castanea sativa shell: Nutritional composition, antioxidant activity, amino acids and vitamin E profile. Food Funct. 2015, 6, 2854–2860. [Google Scholar] [CrossRef] [PubMed]
- Vázquez, G.; Fontenla, E.; Santos, J.; Freire, M.S.; González-Álvarez, J.; Antorrena, G. Antioxidant activity and phenolic content of chestnut (Castanea sativa) shell and eucalyptus (Eucalyptus globulus) bark extracts. Ind. Crop. Prod. 2008, 28, 279–285. [Google Scholar] [CrossRef]
- Vázquez, G.; González-Alvarez, J.; Santos, J.; Freire, M.S.; Antorrena, G. Evaluation of potential applications for chestnut (Castanea sativa) shell and eucalyptus (Eucalyptus globulus) bark extracts. Ind. Crop. Prod. 2009, 29, 364–370. [Google Scholar] [CrossRef]
- Tanaka, T.; Ueda, N.; Shinohara, H.; Nonaka, G.-I.; Fujioka, T.; Mihashi, K.; Kouno, I. C-glycosidic ellagitannin metabolites in the heartwood of Japanese chestnut tree (Castanea crenata SIEB. et Zucc.). Chem. Pharm. Bull. 1996, 44, 2236–2242. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, T.; Ueda, N.; Shinohara, H.; Nonaka, G.-i.; Kouno, I. Four New-C-glycosidic ellagitannins, castacrenins D-G, from Japanese chestnut wood (Castanea crenata SIEB. et ZUCC.). Chem. Pharm. Bull. 1997, 45, 1751–1755. [Google Scholar] [CrossRef] [Green Version]
- Neri, L.; Dimitri, G.; Sacchetti, G. Chemical composition and antioxidant activity of cured chestnuts from three sweet chestnut (Castanea sativa Mill.) ecotypes from Italy. J. Food Compos. Anal. 2010, 23, 23–29. [Google Scholar] [CrossRef]
- Vekiari, S.A.; Gordon, M.H.; García-Macías, P.; Labrinea, H. Extraction and determination of ellagic acid contentin chestnut bark and fruit. Food Chem. 2008, 110, 1007–1011. [Google Scholar] [CrossRef]
- Viriot, C.; Scalbert, A.; Hervé du Penhoat, C.L.M.; Moutounet, M. Ellagitannins in woods of sessile oak and sweet chestnut dimerization and hydrolysis during wood ageing. Phytochemistry 1994, 36, 1253–1260. [Google Scholar] [CrossRef]
- Nicolas, V.; Guy, B.; Christiane, V.; Yves, G.; de, F.V. Determination of the composition of commercial tannin extracts by liquid secondary ion mass spectrometry (LSIMS). J. Sci. Food Agric. 1996, 72, 309–317. [Google Scholar]
- Maurelli, L.; Ionata, E.; La Cara, F.; Morana, A. Chestnut shell as unexploited source of fermentable sugars: Effect of different pretreatment methods on enzymatic saccharification. Appl. Biochem. Biotechnol. 2013, 170, 1104–1118. [Google Scholar] [CrossRef] [PubMed]
- Lampire, O.; Mila, I.; Raminosoa, M.; Michon, V.; Penhoat, C.H.D.; Faucheur, N.; Laprevote, O.; Scalbert, A. Polyphenols isolated from the bark of castanea sativa Mill. Chemical structures and auto-association in honour of professor G. H. Neil Towers 75th birthday. Phytochemistry 1998, 49, 623–631. [Google Scholar] [CrossRef]
- Moure, A.; Conde, E.; Falqué, E.; Domínguez, H.; Parajó, J.C. Production of nutraceutics from chestnut burs by hydrolytic treatment. Food Res. Int. 2014, 65, 359–366. [Google Scholar] [CrossRef]
- Díaz Reinoso, B.; Couto, D.; Moure, A.; Fernandes, E.; Domínguez, H.; Parajó, J.C. Optimization of antioxidants—Extraction from Castanea sativa leaves. Chem. Eng. J. 2012, 203, 101–109. [Google Scholar] [CrossRef]
- Fuente-Maqueda, F.; Rodríguez, A.; Majada, J.; Fernández, B.; Feito, I. Methodology optimization for the analysis of phenolic compounds in chestnut (Castanea sativa Mill.). Food Sci. Technol. Int. 2020, 1–15. [Google Scholar] [CrossRef]
- Aires, A.; Carvalho, R.; Saavedra, M.J. Valorization of solid wastes from chestnut industry processing: Extraction and optimization of polyphenols, tannins and ellagitannins and its potential for adhesives, cosmetic and pharmaceutical industry. Waste Manage. 2016, 48, 457–464. [Google Scholar] [CrossRef]
- Yamada, H.; Wakamori, S.; Hirokane, T.; Ikeuchi, K.; Matsumoto, S. Structural revisions in natural ellagitannins. Molecules 2018, 23, 1901. [Google Scholar] [CrossRef] [Green Version]
- Garro Galvez, J.M.; Fechtal, M.; Riedl, B. Gallic acid as a model of tannins in condensation with formaldehyde. Thermochim. Acta 1996, 274, 149–163. [Google Scholar] [CrossRef]
- Nagamori, M.; Funazukuri, T. Glucose production by hydrolysis of starch under hydrothermal conditions. J. Chem. Technol. Biotechnol. 2004, 79, 229–233. [Google Scholar] [CrossRef]
- Gagić, T.; Perva-Uzunalić, A.; Knez, Ž.; Škerget, M. Hydrothermal degradation of cellulose at temperature from 200 to 300 °C. Ind. Eng. Chem. Res. 2018, 57, 6576–6584. [Google Scholar] [CrossRef]
- Lavarack, B.P.; Griffin, G.J.; Rodman, D. The acid hydrolysis of sugarcane bagasse hemicellulose to produce xylose, arabinose, glucose and other products. Biomass Bioenergy 2002, 23, 367–380. [Google Scholar] [CrossRef]
- Gullón, B.; Eibes, G.; Dávila, I.; Moreira, M.T.; Labidi, J.; Gullón, P. Hydrothermal treatment of chestnut shells (Castanea sativa) to produce oligosaccharides and antioxidant compounds. Carbohydr. Polym. 2018, 192, 75–83. [Google Scholar] [CrossRef]
- Talmaciu, A.I.; Ravber, M.; Volf, I.; Knez, Ž.; Popa, V.I. Isolation of bioactive compounds from spruce bark waste using sub- and supercritical fluids. J. Supercrit. Fluids 2016, 117, 243–251. [Google Scholar] [CrossRef]
- Ravber, M.; Knez, Ž.; Škerget, M. Simultaneous extraction of oil- and water-soluble phase from sunflower seeds with subcritical water. Food Chem. 2015, 166, 316–323. [Google Scholar] [CrossRef]
- Bizuayehu, D.; Atlabachew, M.; Ali, M.T. Determination of some selected secondary metabolites and their invitro antioxidant activity in commercially available Ethiopian tea (Camellia sinensis). SpringerPlus 2016, 5, 412. [Google Scholar] [CrossRef] [Green Version]
- Jokić, S.; Gagić, T.; Knez, Ž.; Šubarić, D.; Škerget, M. Separation of active compounds from food by-product (cocoa shell) using subcritical water extraction. Molecules 2018, 23, 1408. [Google Scholar] [CrossRef] [Green Version]
- Arapitsas, P. Hydrolyzable tannin analysis in food. Food Chem. 2012, 135, 1708–1717. [Google Scholar] [CrossRef]
Sample Availability: Samples of the compounds are not available from the authors. |
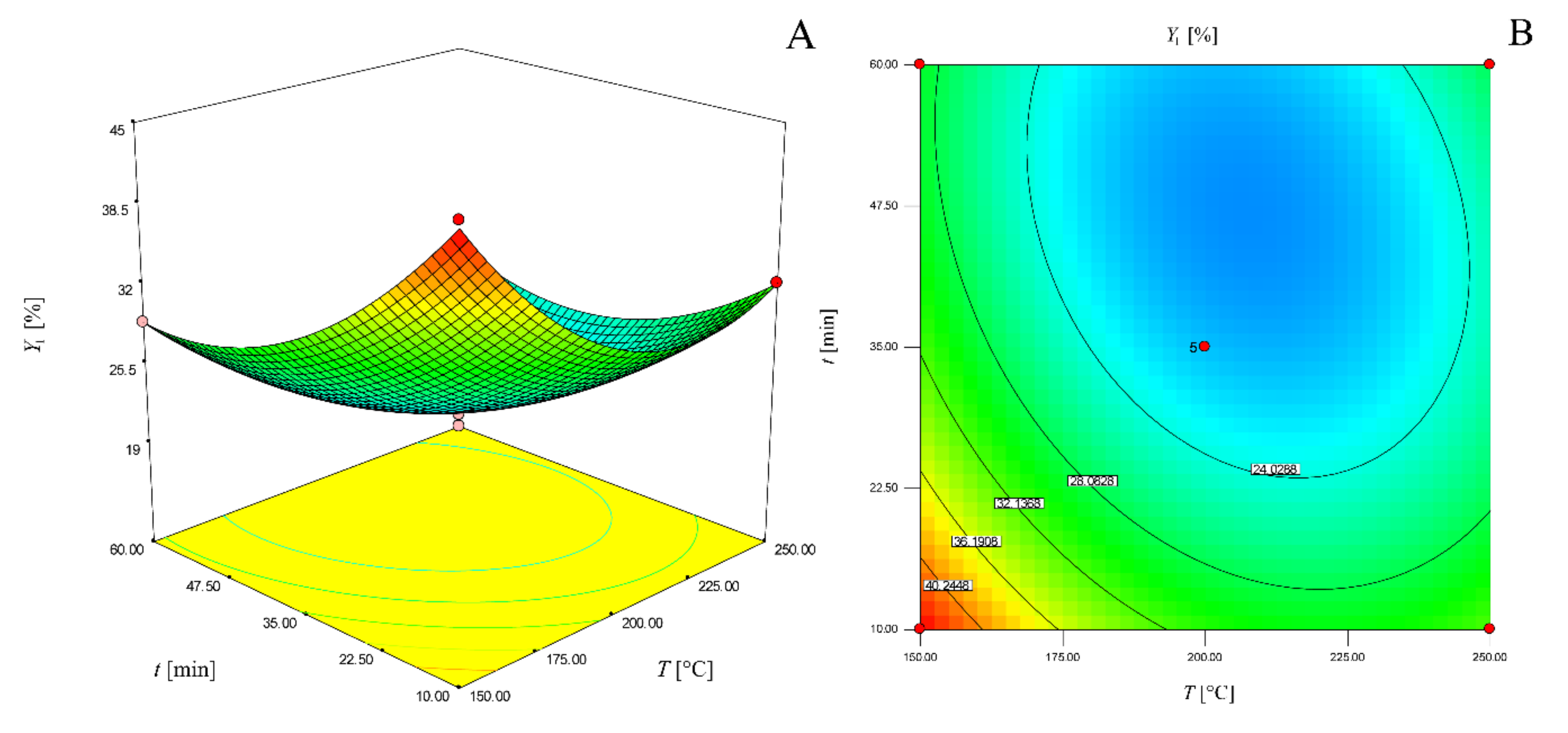

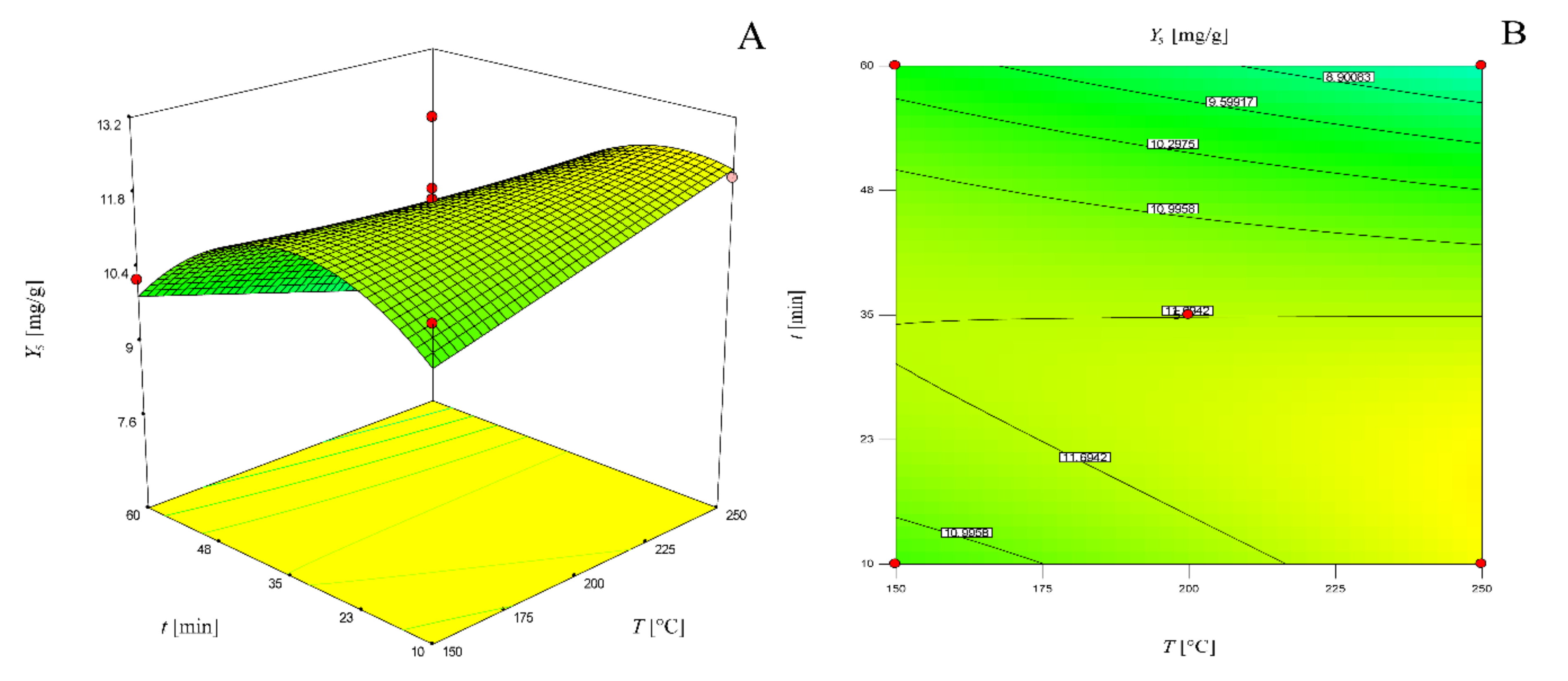
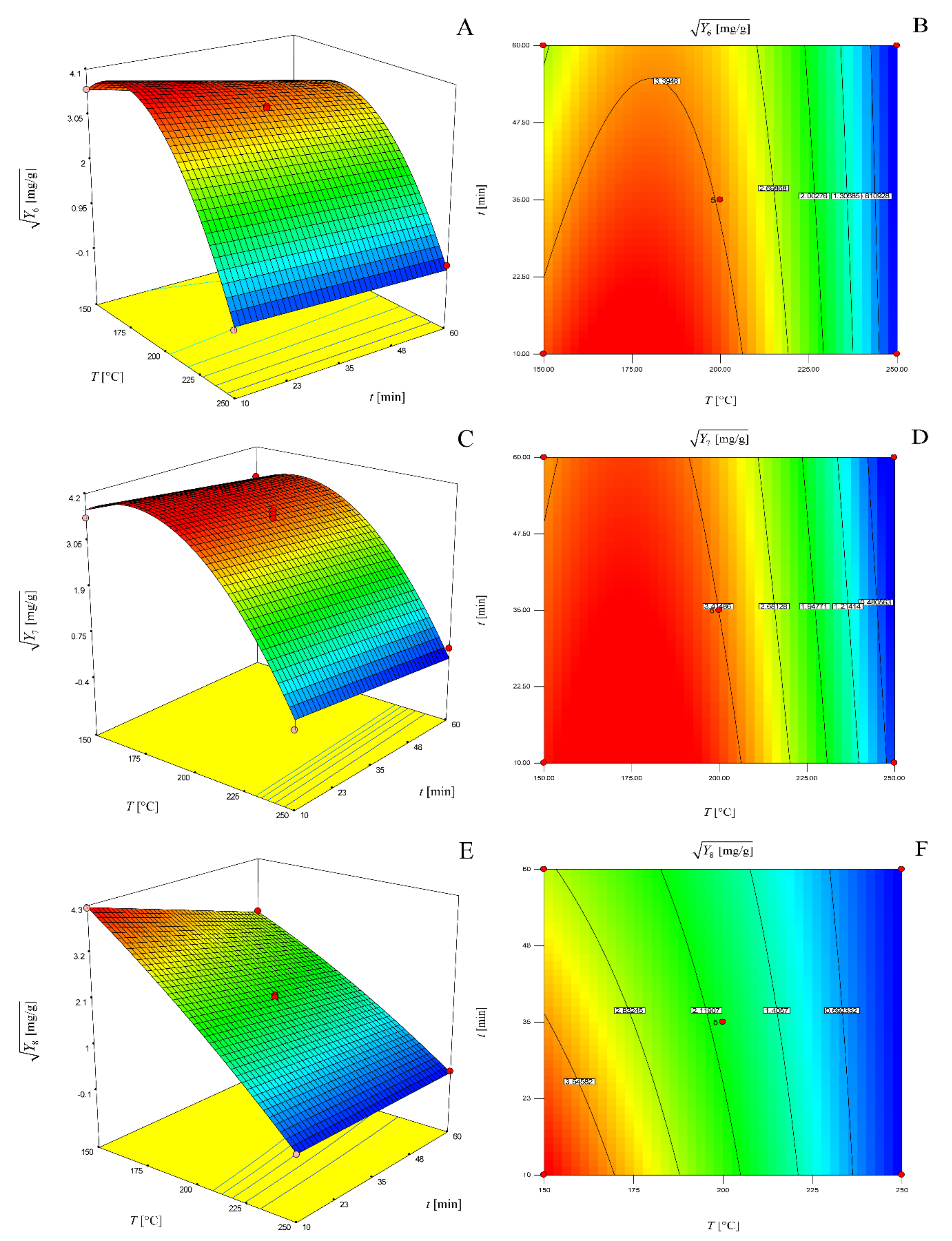
| Run | Temp. (X1), °C | Time (X2), min | Solvent-Solid Ratio (X3), mL/g | Extraction Yield, % | |
|---|---|---|---|---|---|
| Experimental | Predicted | ||||
| 1 | 150 (−1) | 10 (−1) | 20 (0) | 44.9 | 44.3 |
| 2 | 250 (1) | 10 (−1) | 20 (0) | 32.2 | 32.2 |
| 3 | 150 (−1) | 60 (1) | 20 (0) | 29.0 | 29.0 |
| 4 | 250 (1) | 60 (1) | 20 (0) | 26.9 | 27.5 |
| 5 | 150 (−1) | 35 (0) | 10 (−1) | 25.6 | 25.6 |
| 6 | 250 (1) | 35 (0) | 10 (−1) | 24.4 | 23.8 |
| 7 | 150 (−1) | 35 (0) | 30 (1) | 33.9 | 34.5 |
| 8 | 250 (1) | 35 (0) | 30 (1) | 22.6 | 22.6 |
| 9 | 200 (0) | 10 (−1) | 10 (−1) | 28.1 | 28.7 |
| 10 | 200 (0) | 60 (1) | 10 (−1) | 15.9 | 16.0 |
| 11 | 200 (0) | 10 (−1) | 30 (1) | 29.9 | 29.9 |
| 12 | 200 (0) | 60 (1) | 30 (1) | 23.1 | 22.6 |
| 13 | 200 (0) | 35 (0) | 20 (0) | 20.3 | 21.5 |
| 14 | 200 (0) | 35 (0) | 20 (0) | 23.3 | 21.5 |
| 15 | 200 (0) | 35 (0) | 20 (0) | 21.3 | 21.5 |
| 16 | 200 (0) | 35 (0) | 20 (0) | 22.1 | 21.5 |
| 17 | 200 (0) | 35 (0) | 20 (0) | 20.3 | 21.5 |
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
|---|---|---|---|---|---|---|---|
| Model | 710.81 | 9 | 78.98 | 64.84 | <0.0001 | ||
| Intercept | 21.46 | 0.49 | 1 | ||||
| X1 | −3.41 | 0.39 | 93.02 | 1 | 93.02 | 76.37 | <0.0001 |
| X2 | −5.01 | 0.39 | 200.70 | 1 | 200.70 | 164.77 | <0.0001 |
| X3 | 1.94 | 0.39 | 29.99 | 1 | 29.99 | 24.62 | 0.0016 |
| X1 X2 | 2.63 | 0.55 | 27.72 | 1 | 27.72 | 22.76 | 0.0020 |
| X1 X3 | −2.52 | 0.55 | 25.35 | 1 | 25.35 | 20.81 | 0.0026 |
| X2 X3 | 1.35 | 0.55 | 7.24 | 1 | 7.24 | 5.94 | 0.0449 |
| X12 | 7.08 | 0.54 | 211.03 | 1 | 211.03 | 173.25 | <0.0001 |
| X22 | 4.71 | 0.54 | 93.49 | 1 | 93.49 | 76.75 | <0.0001 |
| X32 | −1.90 | 0.54 | 15.25 | 1 | 15.25 | 12.52 | 0.0095 |
| Residual | 8.53 | 7 | 1.22 | ||||
| Lack of fit | 2.07 | 3 | 0.69 | 0.43 | 0.7440 | ||
| Pure error | 6.45 | 4 | 1.61 | ||||
| R2 | 0.9881 | Adj R2 | 0.9729 | ||||
| C.V. % | 4.23 | Pred R2 | 0.9399 | ||||
| PRESS | 43.25 | Adeq Precision | 33.461 |
| Run | Temp. (X1), °C | Time (X2), min | Solvent-Solid Ratio (X3), mL/g | Total Phenol Content, mg/g of Bark | Total Tannin Content, mg/g of Bark | Antioxidant Activity, % | |||
|---|---|---|---|---|---|---|---|---|---|
| Experimental | Predicted | Experimental | Predicted | Experimental | Predicted | ||||
| 1 | 150 (−1) | 10 (−1) | 20 (0) | 77.7 | 80.0 | 84.4 | 88.4 | 80.5 | 79.9 |
| 2 | 250 (1) | 10 (−1) | 20 (0) | 68.8 | 67.5 | 75.5 | 76.7 | 90.6 | 90.3 |
| 3 | 150 (−1) | 60 (1) | 20 (0) | 75.7 | 68.9 | 83.6 | 81.4 | 81.0 | 79.9 |
| 4 | 250 (1) | 60 (1) | 20 (0) | 57.8 | 56.4 | 73.9 | 69.6 | 88.2 | 90.3 |
| 5 | 150 (−1) | 35 (0) | 10 (−1) | 59.1 | 62.1 | 66.6 | 66.3 | 79.2 | 80.4 |
| 6 | 250 (1) | 35 (0) | 10 (−1) | 47.3 | 49.6 | 53.3 | 54.5 | 86.2 | 85.7 |
| 7 | 150 (−1) | 35 (0) | 30 (1) | 85.2 | 86.7 | 98.3 | 96.8 | 72.8 | 73.3 |
| 8 | 250 (1) | 35 (0) | 30 (1) | 73.9 | 74.2 | 83.0 | 85.0 | 90.0 | 88.9 |
| 9 | 200 (0) | 10 (−1) | 10 (−1) | 53.7 | 50.8 | 60.3 | 54.6 | 88.1 | 87.4 |
| 10 | 200 (0) | 60 (1) | 10 (−1) | 38.3 | 39.6 | 42.8 | 47.5 | 90.0 | 90.1 |
| 11 | 200 (0) | 10 (−1) | 30 (1) | 76.9 | 75.4 | 86.0 | 85.0 | 88.3 | 88.2 |
| 12 | 200 (0) | 60 (1) | 30 (1) | 60.8 | 64.2 | 77.5 | 78.0 | 84.7 | 85.5 |
| 13 | 200 (0) | 35 (0) | 20 (0) | 59.6 | 57.5 | 66.7 | 69.6 | 85.3 | 87.6 |
| 14 | 200 (0) | 35 (0) | 20 (0) | 48.1 | 57.5 | 72.6 | 69.6 | 87.3 | 87.6 |
| 15 | 200 (0) | 35 (0) | 20 (0) | 61.9 | 57.5 | 67.1 | 69.6 | 88.9 | 87.6 |
| 16 | 200 (0) | 35 (0) | 20 (0) | 60.2 | 57.5 | 68.9 | 69.6 | 88.3 | 87.6 |
| 17 | 200 (0) | 35 (0) | 20 (0) | 58.1 | 57.5 | 71.5 | 69.6 | 88.1 | 87.6 |
| TOTAL PHENOLS | |||||||
|---|---|---|---|---|---|---|---|
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
| Model | 2256.04 | 4 | 78.98 | 31.11 | <0.0001 | ||
| Intercept | 57.50 | 1.42 | 1 | ||||
| X1 | −6.25 | 1.51 | 312.50 | 1 | 312.50 | 17.24 | 0.0013 |
| X2 | −5.58 | 1.51 | 248.98 | 1 | 248.98 | 13.73 | 0.0030 |
| X3 | 12.31 | 1.51 | 1211.55 | 1 | 1211.55 | 66.83 | <0.0001 |
| X12 | 10.68 | 2.07 | 483.01 | 1 | 483.01 | 26.64 | 0.0002 |
| Residual | 217.54 | 12 | 18.13 | ||||
| Lack of fit | 96.80 | 8 | 12.10 | 0.40 | 0.8738 | ||
| Pure error | 120.74 | 4 | 30.19 | ||||
| R2 | 0.9121 | Adj R2 | 0.8827 | ||||
| C.V. % | 6.81 | Pred R2 | 0.8392 | ||||
| PRESS | 397.87 | Adeq Precision | 20.407 | ||||
| TOTAL TANNINS | |||||||
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-value | p-value Probability > F |
| Model | 2641.11 | 5 | 528.22 | 43.73 | <0.0001 | ||
| Intercept | 69.63 | 1.38 | 1 | ||||
| X1 | −5.89 | 1.23 | 277.18 | 1 | 277.18 | 22.95 | 0.0006 |
| X2 | −3.54 | 1.23 | 100.32 | 1 | 100.32 | 8.31 | 0.0149 |
| X3 | 15.24 | 1.23 | 1858.06 | 1 | 1858.06 | 153.82 | <0.0001 |
| X12 | 9.38 | 1.69 | 371.28 | 1 | 371.28 | 30.74 | 0.0002 |
| X32 | −3.37 | 1.69 | 47.81 | 1 | 47.81 | 3.96 | 0.0721 |
| Residual | 132.88 | 11 | 12.08 | ||||
| Lack of fit | 105.72 | 7 | 15.10 | 2.22 | 0.2294 | ||
| Pure error | 27.16 | 4 | 6.79 | ||||
| R2 | 0.9521 | Adj R2 | 0.9303 | ||||
| C.V. % | 4.80 | Pred R2 | 0.8674 | ||||
| PRESS | 367.89 | Adeq Precision | 23.869 | ||||
| ANTIOXIDANT ACTIVITY | |||||||
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-value | p-value Probability > F |
| Model | 346.89 | 7 | 49.56 | 24.39 | <0.0001 | ||
| Intercept | 87.56 | 0.64 | 1 | ||||
| X1 | 5.20 | 0.50 | 216.32 | 1 | 216.32 | 106.48 | <0.0001 |
| X3 | −0.96 | 0.50 | 7.32 | 1 | 7.32 | 3.60 | 0.0902 |
| X1 X3 | 2.56 | 0.71 | 26.16 | 1 | 26.16 | 12.88 | 0.0059 |
| X2 X3 | −1.38 | 0.71 | 7.62 | 1 | 7.62 | 3.75 | 0.0848 |
| X12 | −4.11 | 0.69 | 71.19 | 1 | 71.19 | 35.04 | 0.0002 |
| X22 | 1.62 | 0.69 | 11.06 | 1 | 11.06 | 5.44 | 0.0445 |
| X32 | −1.39 | 0.69 | 8.19 | 1 | 8.19 | 4.03 | 0.0756 |
| Residual | 18.28 | 9 | 2.03 | ||||
| Lack of fit | 10.37 | 5 | 2.07 | 1.05 | 0.4959 | ||
| Pure error | 7.92 | 4 | 1.98 | ||||
| R2 | 0.9499 | Adj R2 | 0.9110 | ||||
| C.V. % | 1.66 | Pred R2 | 0.7557 | ||||
| PRESS | 89.20 | Adeq Precision | 17.313 | ||||
| Run | Temp. (X1), °C | Time (X2), min | Solvent-Solid Ratio (X3), mL/g | Ellagic Acid, mg/g of Bark | Gallic Acid, mg/g of Bark | Vescalagin, mg/g of Bark | Castalagin, mg/g of Bark | 1-o-galloyl-Castalagin, mg/g of Bark | |
|---|---|---|---|---|---|---|---|---|---|
| Experimental | Predicted | Experimental | Experimental | Experimental | Experimental | ||||
| 1 | 150 (−1) | 10 (−1) | 20 (0) | 11.3 | 10.6 | 2.8 | 1.1 | 5.3 | 1.6 |
| 2 | 250 (1) | 10 (−1) | 20 (0) | 12.1 | 12.3 | 0 | 0 | 0 | 0 |
| 3 | 150 (−1) | 60 (1) | 20 (0) | 10.2 | 9.9 | 3.3 | traces | 2.3 | 0.4 |
| 4 | 250 (1) | 60 (1) | 20 (0) | 7.6 | 8.2 | 0 | 0 | 0 | 0 |
| 5 | 150 (−1) | 35 (0) | 10 (−1) | 9.3 | 8.6 | 3.2 | traces | 3.4 | 0.3 |
| 6 | 250 (1) | 35 (0) | 10 (−1) | 8.5 | 8.6 | 0 | 0 | 0 | 0 |
| 7 | 150 (−1) | 35 (0) | 30 (1) | 14.2 | 14.8 | 3.5 | 3.1 | 5.3 | 0.4 |
| 8 | 250 (1) | 35 (0) | 30 (1) | 14.8 | 14.8 | 0 | 0 | 0 | 0 |
| 9 | 200 (0) | 10 (−1) | 10 (−1) | 8.7 | 9.2 | 2.8 | traces | 0.09 | 0.01 |
| 10 | 200 (0) | 60 (1) | 10 (−1) | 5.0 | 5.1 | 0.6 | 0 | 0 | 0 |
| 11 | 200 (0) | 10 (−1) | 30 (1) | 13.6 | 13.6 | 3.7 | traces | 0.2 | trace |
| 12 | 200 (0) | 60 (1) | 30 (1) | 13.4 | 13.0 | Traces | 0 | 0 | 0 |
| 13 | 200 (0) | 35 (0) | 20 (0) | 11.7 | 11.7 | 1.2 | 0 | 0 | 0 |
| 14 | 200 (0) | 35 (0) | 20 (0) | 11.9 | 11.7 | 1.2 | 0 | 0 | 0 |
| 15 | 200 (0) | 35 (0) | 20 (0) | 10.9 | 11.7 | 1.2 | 0 | 0 | 0 |
| 16 | 200 (0) | 35 (0) | 20 (0) | 13.2 | 11.7 | 1.2 | 0 | 0 | 0 |
| 17 | 200 (0) | 35 (0) | 20 (0) | 10.7 | 11.7 | 0.9 | 0 | 0 | 0 |
| Compounds | Concentration in Extract g/100 g of Extract (Chiarini et al. [7]) | Concentration in Extract (TAN 1) g/100 g of Extract (Comandini et al. [9]) | Concentration in Extract g/100 g of Extract (Present Work at Conditions of 150 °C, 35 min and 30 mL/g) |
|---|---|---|---|
| Vescalagin | 2.31 | 4.08 | 0.91 |
| Castalagin | 2.26 | 3.80 | 1.56 |
| 1-o-galloyl-castalagin | / | 3.20 | 0.13 |
| Gallic acid | 1.25 | 2.80 | 1.03 |
| Ellagic acid | 1.70 | 0.93 | 4.19 |
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
|---|---|---|---|---|---|---|---|
| Model | 101.37 | 5 | 20.27 | 35.97 | <0.0001 | ||
| Intercept | 11.68 | 0.25 | 1 | ||||
| X2 | −1.18 | 0.27 | 11.16 | 1 | 11.16 | 19.81 | 0.0010 |
| X3 | 3.07 | 0.27 | 75.40 | 1 | 75.40 | 133.78 | <0.0001 |
| X1 X2 | −0.85 | 0.38 | 2.87 | 1 | 2.87 | 5.10 | 0.0453 |
| X2 X3 | 0.87 | 0.38 | 2.99 | 1 | 2.99 | 5.31 | 0.0417 |
| X22 | −1.45 | 0.36 | 8.94 | 1 | 8.94 | 15.87 | 0.0021 |
| Residual | 6.20 | 11 | 0.56 | ||||
| Lack of fit | 2.27 | 7 | 0.32 | 0.33 | 0.9042 | ||
| Pure error | 3.93 | 4 | 0.98 | ||||
| R2 | 0.9424 | Adj R2 | 0.9162 | ||||
| C.V. % | 6.82 | Pred R2 | 0.8711 | ||||
| PRESS | 13.86 | Adeq Precision | 21.613 |
| Run | Temp. (X1), °C | Time (X2), min | Solvent-Solid Ratio (X3), mL/g | Glucose Yield, mg/g of Bark | Fructose Yield, mg/g of Bark | Maltose Yield, mg/g of Bark | Arabinose Yield, mg/g of Bark | |||
|---|---|---|---|---|---|---|---|---|---|---|
| Experimental | Predicted | Experimental | Predicted | Experimental | Predicted | Experimental | ||||
| 1 | 150 (−1) | 10 (−1) | 20 (0) | 13.2 | 13.3 | 13.0 | 14.5 | 17.9 | 18.1 | 9.2 |
| 2 | 250 (1) | 10 (−1) | 20 (0) | 0 | 0.008 | 0 | 0.06 | 0 | 0.0004 | 0 |
| 3 | 150 (−1) | 60 (1) | 20 (0) | 7.8 | 6.9 | 11.4 | 11.0 | 8.5 | 8.4 | 12.5 |
| 4 | 250 (1) | 60 (1) | 20 (0) | 0 | 0.008 | 0 | 0.06 | 0 | 0.0004 | 0 |
| 5 | 150 (−1) | 35 (0) | 10 (−1) | 9.5 | 10.6 | 13.9 | 12.7 | 11.2 | 11.4 | 16.5 |
| 6 | 250 (1) | 35 (0) | 10 (−1) | 0 | 0.01 | 0 | 0 | 0 | 0.002 | 0 |
| 7 | 150 (−1) | 35 (0) | 30 (1) | 9.3 | 9.2 | 12.5 | 12.7 | 14.7 | 14.3 | 11.4 |
| 8 | 250 (1) | 35 (0) | 30 (1) | 0 | 0.01 | 0 | 0 | 0 | 0.002 | 0 |
| 9 | 200 (0) | 10 (−1) | 10 (−1) | 15.3 | 14.1 | 14.9 | 13.4 | 5.9 | 5.1 | 5.9 |
| 10 | 200 (0) | 60 (1) | 10 (−1) | 10.4 | 10.0 | 10.1 | 10.0 | 2.7 | 2.4 | 0 |
| 11 | 200 (0) | 10 (−1) | 30 (1) | 12.8 | 12.5 | 13.8 | 13.4 | 5.9 | 5.8 | 9.7 |
| 12 | 200 (0) | 60 (1) | 30 (1) | 7.5 | 8.6 | 6.8 | 10.0 | 3.0 | 2.9 | 0 |
| 13 | 200 (0) | 35 (0) | 20 (0) | 11.7 | 11.2 | 13.4 | 11.6 | 4.2 | 3.9 | 0 |
| 14 | 200 (0) | 35 (0) | 20 (0) | 11.8 | 11.2 | 12.5 | 11.6 | 3.6 | 3.9 | 0 |
| 15 | 200 (0) | 35 (0) | 20 (0) | 10.8 | 11.2 | 12.7 | 11.6 | 3.7 | 3.9 | 0 |
| 16 | 200 (0) | 35 (0) | 20 (0) | 11.5 | 11.2 | 12.0 | 11.6 | 4.0 | 3.9 | 0 |
| 17 | 200 (0) | 35 (0) | 20 (0) | 10.0 | 11.2 | 9.6 | 11.6 | 2.9 | 3.9 | 0 |
| GLUCOSE YIELD | |||||||
|---|---|---|---|---|---|---|---|
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
| Model | 34.11 | 5 | 6.82 | 350.71 | <0.0001 | ||
| Intercept | 3.35 | 0.046 | 1 | ||||
| X1 | −1.57 | 0.049 | 19.72 | 1 | 19.72 | 1013.91 | <0.0001 |
| X2 | −0.30 | 0.049 | 0.70 | 1 | 0.70 | 35.81 | <0.0001 |
| X3 | −0.11 | 0.049 | 0.091 | 1 | 0.091 | 4.68 | 0.0533 |
| X1 X2 | 0.21 | 0.070 | 0.18 | 1 | 0.18 | 9.08 | 0.0118 |
| X12 | −1.78 | 0.068 | 13.42 | 1 | 13.42 | 690.07 | <0.0001 |
| Residual | 0.21 | 11 | 0.019 | ||||
| Lack of fit | 0.16 | 7 | 0.023 | 1.75 | 0.3071 | ||
| Pure error | 0.053 | 4 | 0.013 | ||||
| R2 | 0.9938 | Adj R2 | 0.9909 | ||||
| C.V. % | 5.55 | Pred R2 | 0.9810 | ||||
| PRESS | 0.65 | Adeq Precision | 46.573 | ||||
| FRUCTOSE YIELD | |||||||
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
| Model | 37.10 | 3 | 12.37 | 212.08 | <0.0001 | ||
| Intercept | 3.41 | 0.080 | 1 | ||||
| X1 | −1.78 | 0.085 | 25.37 | 1 | 25.37 | 435.04 | <0.0001 |
| X2 | −0.25 | 0.085 | 0.51 | 1 | 0.51 | 8.78 | 0.0110 |
| X12 | −1.63 | 0.12 | 11.22 | 1 | 11.22 | 192.43 | <0.0001 |
| Residual | 0.76 | 13 | 0.058 | ||||
| Lack of fit | 0.57 | 9 | 0.063 | 1.34 | 0.4149 | ||
| Pure error | 0.19 | 4 | 0.047 | ||||
| R2 | 0.9800 | Adj R2 | 0.9754 | ||||
| C.V. % | 9.14 | Pred R2 | 0.9641 | ||||
| PRESS | 1.36 | Adeq Precision | 34.725 | ||||
| MALTOSE YIELD | |||||||
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
| Model | 27.33 | 6 | 4.56 | 338.9 | <0.0001 | ||
| Intercept | 1.98 | 0.039 | 1 | ||||
| X1 | −1.79 | 0.041 | 25.68 | 1 | 25.68 | 1910.07 | <0.0001 |
| X2 | −0.35 | 0.041 | 0.97 | 1 | 0.97 | 72.32 | <0.0001 |
| X3 | 0.077 | 0.041 | 0.047 | 1 | 0.047 | 3.50 | 0.0910 |
| X1 X2 | 0.33 | 0.058 | 0.43 | 1 | 0.43 | 31.93 | 0.0002 |
| X1 X3 | −0.12 | 0.058 | 0.059 | 1 | 0.059 | 4.42 | 0.0619 |
| X12 | −0.19 | 0.056 | 0.15 | 1 | 0.15 | 11.16 | 0.0075 |
| Residual | 0.13 | 10 | 0.013 | ||||
| Lack of fit | 0.067 | 6 | 0.011 | 0.67 | 0.6865 | ||
| Pure error | 0.067 | 4 | 0.017 | ||||
| R2 | 0.9951 | Adj R2 | 0.9922 | ||||
| C.V. % | 6.13 | Pred R2 | 0.9900 | ||||
| PRESS | 0.27 | Adeq Precision | 57.856 | ||||
| Run | Temp. (X1), °C | Time (X2), min | Solvent-Solid Ratio (X3), mL/g | 5-HMF Yield, mg/g of Bark | Furfural Yield, mg/g of Bark | Levulinic Acid Yield, mg/g of Bark | ||
|---|---|---|---|---|---|---|---|---|
| Experimental | Predicted | Experimental | Predicted | Experimental | ||||
| 1 | 150 (−1) | 10 (−1) | 20 (0) | 0.2 | 0.1 | 0.1 | 0.1 | 0 |
| 2 | 250 (1) | 10 (−1) | 20 (0) | 8.0 | 6.8 | 9.5 | 7.8 | 18.6 |
| 3 | 150 (−1) | 60 (1) | 20 (0) | 0.9 | 1.0 | 0.8 | 1.0 | 0 |
| 4 | 250 (1) | 60 (1) | 20 (0) | 3.5 | 3.7 | 2.3 | 2.4 | 25.0 |
| 5 | 150 (−1) | 35 (0) | 10 (−1) | 0.7 | 0.7 | 0.7 | 0.6 | 0 |
| 6 | 250 (1) | 35 (0) | 10 (−1) | 5.1 | 5.2 | 4.0 | 4.0 | 22.3 |
| 7 | 150 (−1) | 35 (0) | 30 (1) | 0.3 | 0.3 | 0.2 | 0.2 | 0 |
| 8 | 250 (1) | 35 (0) | 30 (1) | 7.8 | 8.2 | 6.3 | 7.0 | 20.1 |
| 9 | 200 (0) | 10 (−1) | 10 (−1) | 4.1 | 5.1 | 9.3 | 11.6 | 0 |
| 10 | 200 (0) | 60 (1) | 10 (−1) | 11.0 | 10.1 | 21.6 | 20.3 | 20.2 |
| 11 | 200 (0) | 10 (−1) | 30 (1) | 3.5 | 4.0 | 8.6 | 9.1 | 0 |
| 12 | 200 (0) | 60 (1) | 30 (1) | 8.5 | 7.9 | 18.5 | 16.0 | 19.4 |
| 13 | 200 (0) | 35 (0) | 20 (0) | 9.0 | 8.2 | 17.3 | 16.8 | 16.9 |
| 14 | 200 (0) | 35 (0) | 20 (0) | 9.2 | 8.2 | 18.1 | 16.8 | 15.6 |
| 15 | 200 (0) | 35 (0) | 20 (0) | 8.1 | 8.2 | 16.3 | 16.8 | 13.5 |
| 16 | 200 (0) | 35 (0) | 20 (0) | 9.1 | 8.2 | 19.4 | 16.8 | 14.8 |
| 17 | 200 (0) | 35 (0) | 20 (0) | 7.4 | 8.2 | 15.2 | 16.8 | 11.4 |
| 5-HMF YIELD | |||||||
|---|---|---|---|---|---|---|---|
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
| Model | 27.61 | 7 | 3.94 | 173.74 | <0.0001 | ||
| Intercept | 2.10 | 0.060 | 1 | ||||
| X1 | 1.32 | 0.053 | 13.88 | 1 | 13.88 | 611.54 | <0.0001 |
| X2 | 0.34 | 0.053 | 0.95 | 1 | 0.95 | 41.83 | 0.0001 |
| X3 | −0.12 | 0.053 | 0.11 | 1 | 0.11 | 4.98 | 0.0525 |
| X1 X2 | −0.64 | 0.075 | 1.63 | 1 | 1.63 | 71.83 | <0.0001 |
| X1 X3 | 0.35 | 0.075 | 0.48 | 1 | 0.48 | 21.18 | 0.0013 |
| X12 | −1.55 | 0.073 | 10.09 | 1 | 10.09 | 444.33 | <0.0001 |
| X22 | −0.25 | 0.073 | 0.25 | 1 | 0.25 | 11.20 | 0.0086 |
| Residual | 0.20 | 9 | 0.023 | ||||
| Lack of fit | 0.17 | 5 | 0.034 | 3.91 | 0.1053 | ||
| Pure error | 0.035 | 4 | 8.670 × 10−3 | ||||
| R2 | 0.9927 | Adj R2 | 0.9869 | ||||
| C.V. % | 11.97 | Pred R2 | 0.9583 | ||||
| PRESS | 1.16 | Adeq Precision | 41.694 | ||||
| FURFURAL YIELD | |||||||
| Parameter | Coefficient Estimate | Standard Error | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value Probability > F |
| Model | 44.65 | 7 | 6.38 | 281.09 | <0.0001 | ||
| Intercept | 2.82 | 0.060 | 1 | ||||
| X1 | 1.32 | 0.053 | 14.04 | 1 | 14.04 | 618.64 | <0.0001 |
| X2 | 0.28 | 0.053 | 0.63 | 1 | 0.63 | 27.65 | 0.0005 |
| X3 | −0.12 | 0.053 | 0.11 | 1 | 0.11 | 5.01 | 0.0520 |
| X1 X2 | −0.87 | 0.075 | 3.03 | 1 | 3.03 | 133.69 | <0.0001 |
| X1 X3 | 0.40 | 0.075 | 0.65 | 1 | 0.65 | 28.61 | 0.0005 |
| X12 | −2.47 | 0.073 | 25.69 | 1 | 25.69 | 1131.99 | <0.0001 |
| X22 | −0.21 | 0.073 | 0.18 | 1 | 0.18 | 7.93 | 0.0202 |
| Residual | 0.20 | 9 | 0.023 | ||||
| Lack of fit | 0.17 | 5 | 0.034 | 3.83 | 0.1088 | ||
| Pure error | 0.035 | 4 | 8.823 × 10−3 | ||||
| R2 | 0.9954 | Adj R2 | 0.9919 | ||||
| C.V. % | 9.61 | Pred R2 | 0.9733 | ||||
| PRESS | 1.20 | Adeq Precision | 51.689 | ||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gagić, T.; Knez, Ž.; Škerget, M. Subcritical Water Extraction of Chestnut Bark and Optimization of Process Parameters. Molecules 2020, 25, 2774. https://doi.org/10.3390/molecules25122774
Gagić T, Knez Ž, Škerget M. Subcritical Water Extraction of Chestnut Bark and Optimization of Process Parameters. Molecules. 2020; 25(12):2774. https://doi.org/10.3390/molecules25122774
Chicago/Turabian StyleGagić, Tanja, Željko Knez, and Mojca Škerget. 2020. "Subcritical Water Extraction of Chestnut Bark and Optimization of Process Parameters" Molecules 25, no. 12: 2774. https://doi.org/10.3390/molecules25122774
APA StyleGagić, T., Knez, Ž., & Škerget, M. (2020). Subcritical Water Extraction of Chestnut Bark and Optimization of Process Parameters. Molecules, 25(12), 2774. https://doi.org/10.3390/molecules25122774