Cellulose Dissolution in Mixtures of Ionic Liquids and Dimethyl Sulfoxide: A Quantitative Assessment of the Relative Importance of Temperature and Composition of the Binary Solvent
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Synthesis of the Ionic Liquids
2.3. Determination of the Concentration Dissolved Cellulose in IL–DMSO
2.4. Calculation of the Empirical Solution Polarity, ET(WB)
2.5. Statistical Design of the Cellulose Dissolution Experiment
3. Results and Discussion
Design of the Cellulose Dissolution Experiments
- In order to compare the regression coefficients directly, the values of T and χDMSO were reduced, so that they varied between 0 and 1, before being subjected to the regression analysis;
- The magnitudes of the regression coefficients indicate the susceptibility/response of the phenomenon studied (i.e., Cel dissolution) to the experimental variables. In addition to expressing the concentration of dissolved Cel as MCC-m%, we also report our data as MCC-χ. The latter scale is more fundamental because differences in the molar masses of mixture components (Cel, CB, IL, co-solvent) do not affect the numerical value of the component. Additionally, the mole fraction scale permits calculation of the number of IL and DMSO molecules required to dissolve Cel. For example, maximum MCC dissolution was observed at 80 °C and χDMSO approximately 0.6 (Figure 6). Under these conditions, the molar ratios IL:DMSO per AGU were: AlBzMe2NAcO, 3.1:4.7; C3OMeImAcO, 1.7:2.5; DBUHAcO, 5.4:8.1, TMGHAcO, 9.7:14.9. Thus, the most efficient solvent system was that based on imidazole because it required smaller numbers of IL and DMSO molecules to dissolve Cel. We recommend that the mole fraction scale should be employed to compare the efficiency of different Cel solvents;
- As argued above, we concentrated on the regression coefficient of the second (T) and the fourth (χDMSO) terms of the equations listed in Table 2. These showed that both variables had comparable effects on MCC dissolution, at least in the range studied, in agreement with Pareto’s chart (Figure 5) and the abovementioned data in the literature where only one variable at a time was changed. Although the two concentration scales (i.e., MCC-m% and MCC-χ) resulted in different values of regression coefficients, the trends were qualitatively similar;
- The excellent calculated correlation coefficients (R2) were satisfactory and show the importance of using dedicated dissolution equipment and a consistent dissolution protocol, and both ensure reproducible results as argued elsewhere [15];
- Regarding the aprotic ILs, C3OMeImAcO showed a larger decrease of MCC-m% than AlBzMe2AcO as a function of increasing χDMSO, from 0.6 to 0.8. As discussed elsewhere [21], the oxygen atom of the C3O- moiety is a Lewis base, capable of forming intramolecular hydrogen bonds with C2-H of the imidazolium ring, in addition to intermolecular bonds with the hydroxyl groups of the AGU. It is possible that the efficiency of intermolecular hydrogen bonding is impaired at higher T due to the concomitant large increase of entropy;
- To test the robustness of these correlations, we calculated MCC-m% under conditions other than those employed to generate the statistical models, and also determined the concentration of dissolved MCC experimentally. Table 3 shows the excellent agreement between the predicted and experimental values with the difference ranging between 0.7% and 6.2%. Entry 4 is interesting because the temperature employed was outside the T range investigated.
- Many authors used solvatochromic parameters to assess the efficiency of Cel solvents. In this regard we note:
- −
- We used ET(WB) because values of λmax of the corresponding solvatochromic peak showed a much larger dependence on the experimental conditions than other probes employed for calculation of, for example, Lewis basicity (SB; S = solvent). Consider the following values of Δλmax of WB that we observed for CB/TMGHAcO-DMSO on changing χDMSO from 0.4 → 0.8: 10 nm (T = 40 °C) and 16.7 nm (T = 60 °C). The corresponding Δλmax for N,N-dimethyl-4-nitroaniline (one of the homomorphic pair employed to calculate SB) were 0.1 nm (T = 40 °C) and 0.6 nm (T = 60 °C). In the present case, therefore, WB is much more sensitive to changes in the experimental variables than other solvatochromic probes that are used to calculate specific Cel-solvent interactions. Note that empirical solvent polarity is related to the parameters that describe the specific solute–solvent interactions by Equation (5) [22,23,24,25,26,27,28,29,30,31,32]:where SB is as defined before; SA, SD, and SP refer to Lewis acidity, dipolarity, and polarizability, respectively. That is, the dependence of ET(probe) on the experimental variables reflects collectively the dependence (on the same variables) of hydrogen bonding (as given by SA and SB) and the hydrophobic interactions (related to SP). These two mechanisms of Cel–solvent interactions are central to dissolution of the biopolymer [33,34].ET(probe) = ET(probe)0 + a SA + b SB + d SD + p SP
- −
- ET(probe) is, however, a dependent variable, i.e., its value is determined by T and χDMSO. Indeed, correlations of the values of ET(WB) listed in the footer of Table 1(d) with T at fixed χDMSO and with χDMSO at fixed T (correlations not listed) show perfect second-order polynomials with R2 = 1. Therefore, it is possible, in principle, to use ET(WB) instead of T or χDMSO in Table 2, because the medium empirical polarity is strongly correlated with the independent variables studied. The relevant point, however, is that there is theoretical and experimental ground for using ET(probe) to assess the efficiency of cellulose solvents.
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
List of Abbreviations and Acronyms
| AGU | Anhydroglucose unit |
| AlBzMe2NAcO | Allylbenzyldimethylammonium acetate |
| BM | Binary mixture of solvents |
| CB | Cellobiose |
| Cel | Cellulose |
| DBUAHcO | 1,8-Diazabicyclo[5.4.0]undec-7-ene-8-ium acetate |
| DMSO | Dimethyl sulfoxide |
| IL | Ionic liquid |
| MCC | Microcrystalline cellulose |
| TMGHAcO | Tetramethylguanidinium acetate |
| ET(WB) | Empirical polarity of the medium in kcal/mole, calculated from the UV-Vis spectra of the solvatochromic probe 2,6-dichloro-4-(2,4,6-triphenylpyridinium-1-yl)phenolate (WB) |
| SA | Lewis acidity of the medium |
| SB | Lewis basicity of the medium |
| SD | Medium dipolarity |
| SP | Medium polarizability |
| C3OMeImAcO | 1-(2-methoxyethyl)-3-methylimidazolium acetate |
References
- Zhang, J.; Wu, J.; Yu, J.; Zhang, X.; He, J.; Zhang, J. Application of ionic liquids for dissolving cellulose and fabricating cellulose-based materials: State of the art and future trends. Mater. Chem. Front. 2017, 1, 1273–1290. [Google Scholar] [CrossRef]
- Hermanutz, F.; Vocht, M.P.; Panzier, N.; Buchmeiser, M.R. Processing of Cellulose Using Ionic Liquids. Macromol. Mater. Eng. 2018, 302. [Google Scholar] [CrossRef] [Green Version]
- Reyes, G.; Aguayo, M.G.; Fernández-Pérez, A.; Pääkkönen, T.; Gacitúa, W.; Rojas, O.J. Dissolution and Hydrolysis of Bleached Kraft Pulp Using Ionic Liquids. Polymers 2019, 11, 673. [Google Scholar] [CrossRef] [PubMed]
- El Seoud, O.A.; Kostag, M.; Jedvert, K.; Malek, N.I. Cellulose Regeneration and Chemical Recycling: Closing the “Cellulose Gap” Using Environmentally Benign Solvents. Macromol. Mater. Eng. 2020, 305. [Google Scholar] [CrossRef]
- Kostag, M.; Gericke, M.; Heinze, T.; El Seoud, O.A. Twenty-five years of cellulose chemistry: Innovations in the dissolution of the biopolymer and its transformation into esters and ethers. Cellulose 2019, 26, 139–184. [Google Scholar] [CrossRef]
- El Seoud, O.A.; Koschella, A.; Fidale, L.C.; Dorn, A.S.; Heinze, T. Applications of Ionic Liquids in Carbohydrate Chemistry: A Window of Opportunities. Biomacromolecules 2007, 8, 2629–2647. [Google Scholar] [CrossRef]
- Heinze, T.; El Seoud, O.A.; Koschella, A. Cellulose Activation and Dissolution. In Cellulose Derivatives; Springer: Cham, Switzerland, 2018; pp. 173–257. ISBN 978-3-319-73168-1. [Google Scholar]
- Fidale, L.C.; Ruiz, N.; Heinze, T.; El Seoud, O.A. Cellulose Swelling by Aprotic and Protic Solvents: What are the Similarities and Differences? Macromol. Chem. Phys. 2008, 209, 1240–1254. [Google Scholar] [CrossRef]
- Gericke, M.; Fardim, P.; Heinze, T. Ionic Liquids—Promising but Challenging Solvents for Homogeneous Derivatization of Cellulose. Molecule 2012, 17, 7458–7502. [Google Scholar] [CrossRef] [Green Version]
- Huang, Y.-B.; Xin, P.-P.; Li, J.-X.; Shao, Y.-Y.; Huang, C.; Pan, H. Room-Temperature Dissolution and Mechanistic Investigation of Cellulose in a Tetra-Butylammonium Acetate/Dimethyl Sulfoxide System. ACS Sustain. Chem. Eng. 2016, 4, 2286–2294. [Google Scholar] [CrossRef]
- Idström, A.; Gentile, L.; Gubitosi, M.; Olsson, C.; Stenqvist, B.; Lund, M.; Bergquist, K.-E.; Olsson, U.; Köhnke, T.; Bialik, E. On the dissolution of cellulose in tetrabutylammonium acetate/dimethyl sulfoxide: A frustrated solvent. Cellulose 2017, 24, 3645–3657. [Google Scholar] [CrossRef]
- Kostag, M.; Jedvert, K.; Achtel, C.; Heinze, T.; El Seoud, O.A. Recent Advances in Solvents for the Dissolution, Shaping and Derivatization of Cellulose: Quaternary Ammonium Electrolytes and their Solutions in Water and Molecular Solvents. Molecules 2018, 23, 511. [Google Scholar] [CrossRef] [Green Version]
- Kostag, M.; Pires, P.A.R.; El Seoud, O.A. Dependence of cellulose dissolution in quaternary ammonium acetates/DMSO on the molecular structure of the electrolyte: Use of solvatochromism, micro-calorimetry, and molecular dynamics simulations. Cellulose 2020, 27, 3565–3580. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments, 8th ed.; Wiley: Hoboken, NJ, USA, 2013; pp. 233, 394, 478. ISBN 978-1118-14692-7. [Google Scholar]
- Kostag, M.; Dignani, M.T.; Lourenço, M.C.; Bioni, T.D.A.; El Seoud, O.A. Assessing cellulose dissolution efficiency in solvent systems based on a robust experimental quantification protocol and enthalpy data. Holzforschung 2019, 73, 1103–1112. [Google Scholar] [CrossRef]
- Hauru, L.K.J.; Hummel, M.; King, A.W.T.; Kilpeläinen, I.; Sixta, H. Role of Solvent Parameters in the Regeneration of Cellulose from Ionic Liquid Solutions. Biomacromolecules 2012, 13, 2896–2905. [Google Scholar] [CrossRef] [PubMed]
- Parviainen, A.; King, A.; Mutikainen, I.; Hummel, M.; Selg, C.; Hauru, L.K.J.; Sixta, H.; Kilpeläinen, I. Predicting Cellulose Solvating Capabilities of Acid-Base Conjugate Ionic Liquids. ChemSusChem 2013, 6, 2161–2169. [Google Scholar] [CrossRef]
- NBR ISO 5351:2012. Brazilian Association of Technical Norms (ABNT). In Pulps—Determination of Limiting Viscosity Number in Cupri-Ethylenediamine (CED) Solution; Brazilian Standards: Rio de Janeiro, Brazil, 2012.
- Kostag, M.; El Seoud, O.A. Dependence of cellulose dissolution in quaternary ammonium-based ionic liquids/DMSO on the molecular structure of the electrolyte. Carbohydr. Polym. 2019, 205, 524–532. [Google Scholar] [CrossRef]
- El Seoud, O.A.; Da Silva, V.C.; Possidonio, S.; Casarano, R.; Arêas, E.P.G.; Gimenes, P. Microwave-Assisted Derivatization of Cellulose, 2—The Surprising Effect of the Structure of Ionic Liquids on the Dissolution and Acylation of the Biopolymer. Macromol. Chem. Phys. 2011, 212, 2541–2550. [Google Scholar] [CrossRef]
- De Jesus, J.C.; Pires, P.A.R.; Mustafa, R.; Riaz, N.; El Seoud, O.A. Experimental and theoretical studies on solvation in aqueous solutions of ionic liquids carrying different side chains: The n-butyl-group versus the methoxyethyl group. RSC Adv. 2017, 7, 15952–15963. [Google Scholar] [CrossRef] [Green Version]
- Reichardt, C.; Welton, T. Solvents and Solvent Effects in Organic Chemistry, 4th ed.; Updated and Enlarged Edition; Wiley: Hoboken, NJ, USA, 2010; pp. 425–508. ISBN 978-3-527-32473-6. [Google Scholar]
- De Oliveira, H.F.N.; Rinaldi, R. Understanding Cellulose Dissolution: Energetics of Interactions of Ionic Liquids and Cellobiose Revealed by Solution Microcalorimetry. ChemSusChem 2015, 8, 1577–1584. [Google Scholar] [CrossRef]
- Lan, G.; Zhang, C.; Lu, B.; Yu, K.; Liu, J.; Kang, H.; Liu, R.; Lan, G. Cellobiose as a model compound for cellulose to study the interactions in cellulose/lithium chloride/N-methyl-2-pyrrolidone systems. Cellulose 2017, 24, 1621–1629. [Google Scholar] [CrossRef]
- Weissman, S.A.; Anderson, N.G. Design of Experiments (DoE) and Process Optimization. A Review of Recent Publications. Org. Process. Res. Dev. 2015, 19, 1605–1633. [Google Scholar] [CrossRef]
- Lendrem, D.; Owen, M.; Godbert, S. DOE (Design of Experiments) in Development Chemistry: Potential Obstacles. Org. Process. Res. Dev. 2001, 5, 324–327. [Google Scholar] [CrossRef]
- Bei, D.; Marszalek, J.M.; Youan, B.-B.C. Formulation of Dacarbazine-loaded Cubosomes—Part II: Influence of Process Parameters. AAPS PharmSciTech 2009, 10, 1040–1047. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Khuri, A.I.; Cornell, J.A. Response Surfaces: Designs and Analyses, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1996; pp. 1, 107. ISBN 9780367401252. [Google Scholar]
- Minnick, D.L.; Flores, R.A.; DeStefano, M.R.; Scurto, A.M. Cellulose Solubility in Ionic Liquid Mixtures: Temperature, Cosolvent, and Antisolvent Effects. J. Phys. Chem. B 2016, 120, 7906–7919. [Google Scholar] [CrossRef] [PubMed]
- Tomimatsu, Y.; Suetsugu, H.; Yoshimura, Y.; Shimizu, A. The solubility of cellulose in binary mixtures of ionic liquids and dimethyl sulfoxide: Influence of the anion. J. Mol. Liq. 2019, 278, 120–126. [Google Scholar] [CrossRef]
- El Seoud, O.A.; Kostag, M.; Jedvert, K.; Malek, N.I. Cellulose in Ionic Liquids and Alkaline Solutions: Advances in the Mechanisms of Biopolymer Dissolution and Regeneration. Polymers 2019, 11, 1917. [Google Scholar] [CrossRef] [Green Version]
- Catalán, J. Toward a Generalized Treatment of the Solvent Effect Based on Four Empirical Scales: Dipolarity (SdP, a New Scale), Polarizability (SP), Acidity (SA), and Basicity (SB) of the Medium. J. Phys. Chem. B 2009, 113, 5951–5960. [Google Scholar] [CrossRef]
- Lindman, B.; Karlström, G.; Stigsson, L. On the mechanism of dissolution of cellulose. J. Mol. Liq. 2010, 156, 76–81. [Google Scholar] [CrossRef]
- Lindman, B.; Medronho, B.; Alves, L.; Costa, C.; Edlund, H.; Norgren, M. The relevance of structural features of cellulose and its interactions to dissolution, regeneration, gelation and plasticization phenomena. Phys. Chem. Chem. Phys. 2017, 19, 23704–23718. [Google Scholar] [CrossRef] [Green Version]
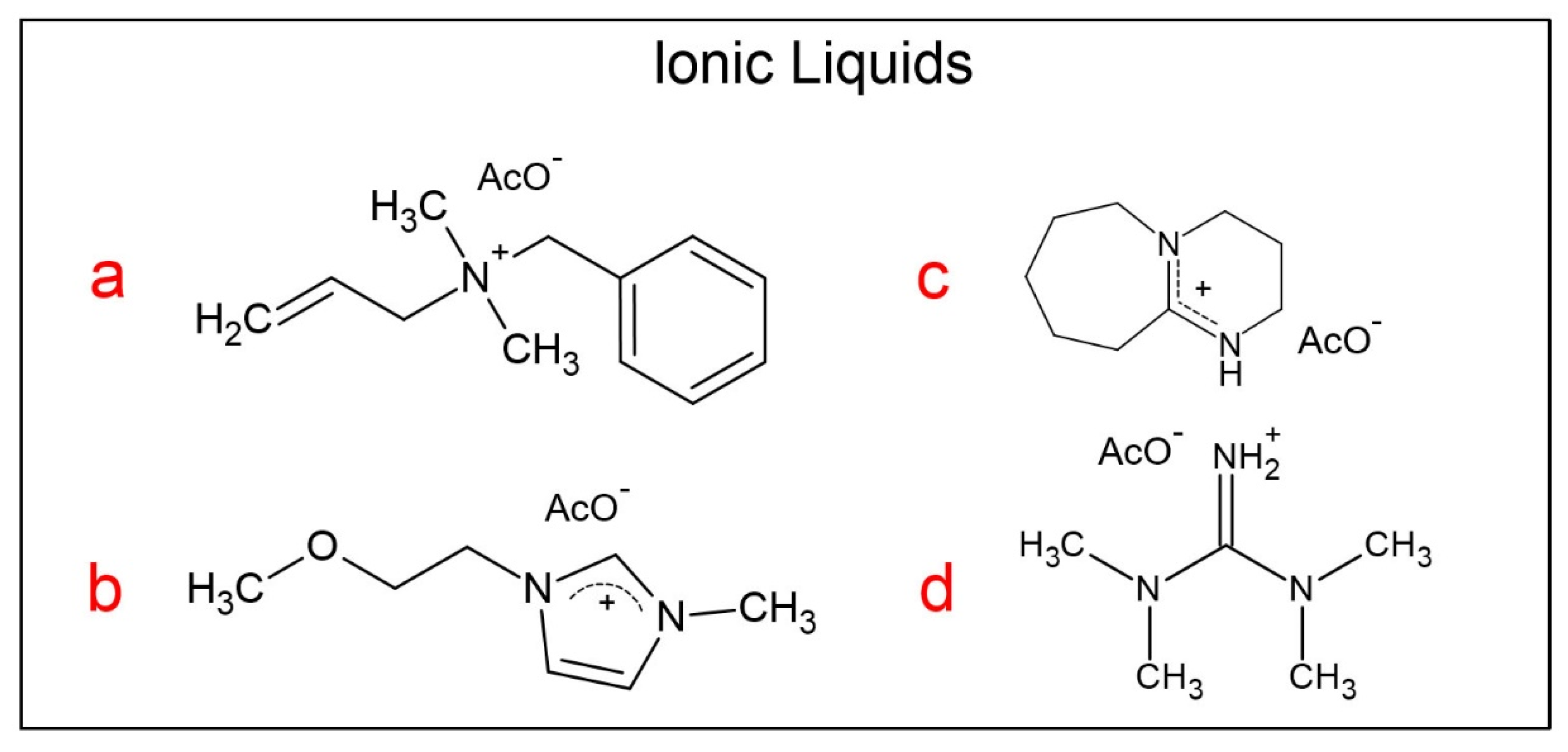

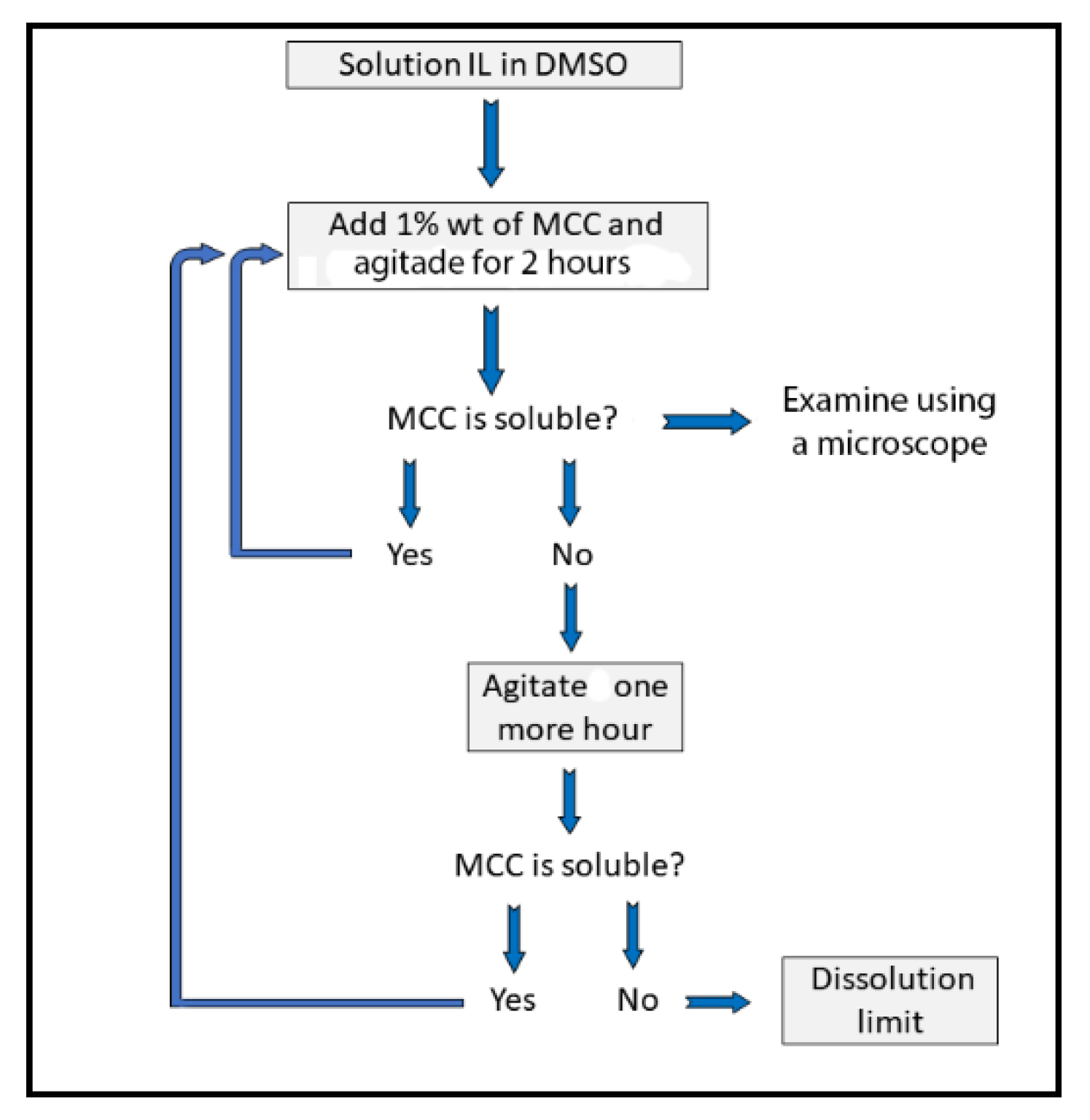
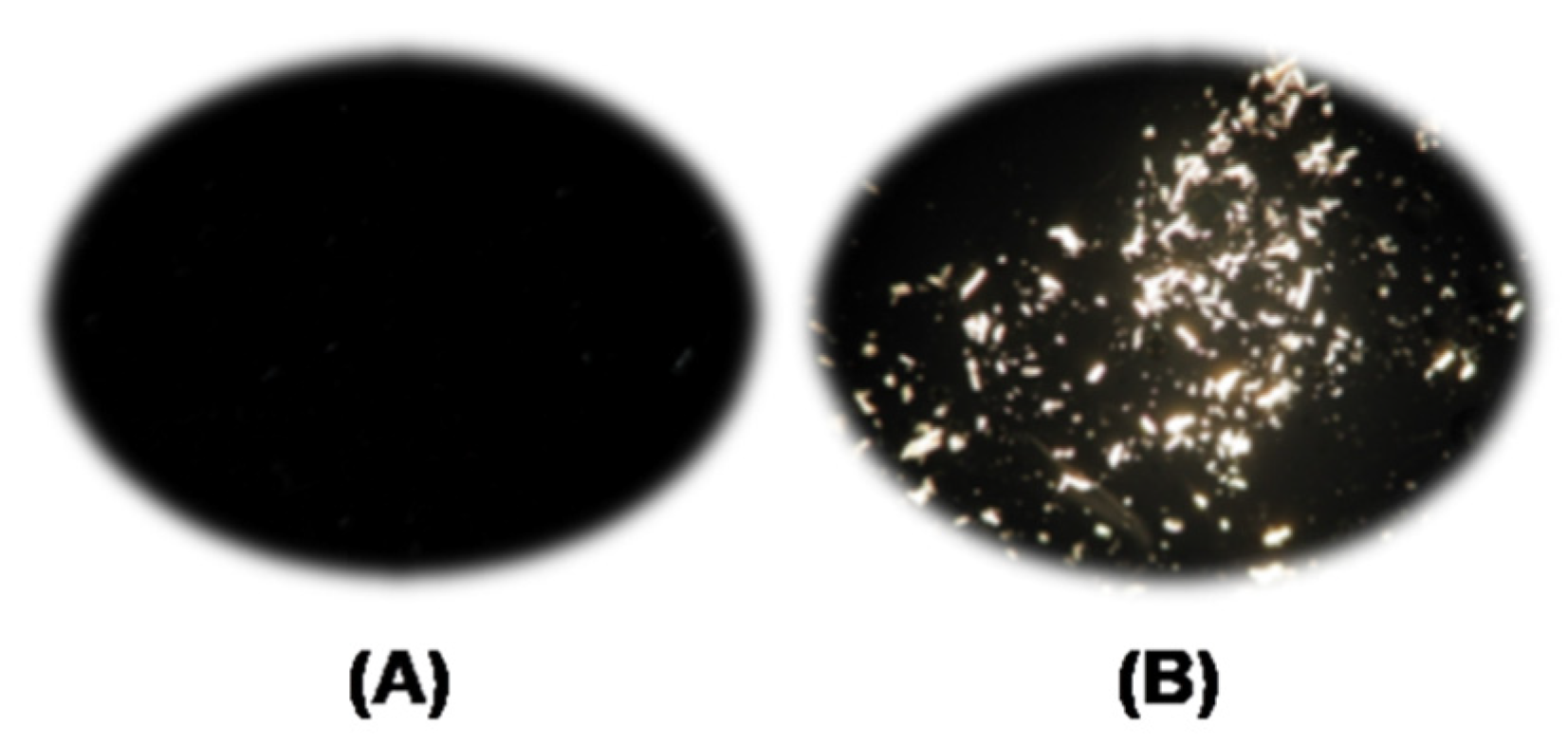


| AlBzMe2NAcO | C3OMeImAcO | DBUHAcO | TMGHAcO | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Temperature (°C) | χDMSO | MCC-m% b | MCC-χ b | MCC-m% b | MCC-χ b | MCC-m% b | MCC-χ b | MCC-m% b | MCC-χ b |
| 40 | 0.4 | 0.0 c,d | 0.0 | 14.5 c,d | 13.7 | 0.0 c,d | 0.0 | 1.3 c,d | 1.1 |
| 40 | 0.4 | 0.0 c | 0.0 c | 14.8 | 13.8 | 0.0 c | 0.0 c | 1.4 | 1.1 |
| 60 | 0.4 | 2.6 | 2.5 | 18.0 | 16.9 | 3.2 | 2.9 | 4.5 | 3.8 |
| 80 | 0.4 | 4.0 | 4.3 | 22.0 | 20.8 | 5.8 | 5.4 | 7.4 | 6.3 |
| 80 | 0.4 | 4.3 | 4.5 | 22.2 | 21.0 | 5.5 | 5.0 | 7.1 | 6.0 |
| 40 | 0.6 | 6.2 | 5.5 | 15.3 | 12.4 | 2.2 | 1.7 | 3.4 | 2.4 |
| 60 | 0.6 | 9.3 | 8.2 | 19.9 | 16.2 | 7.5 | 5.9 | 6.9 | 5.1 |
| 60 | 0.6 | 9.6 | 8.4 | 19.0 | 15.4 | 7.6 | 6.1 | 6.7 | 4.9 |
| 60 | 0.6 | 9.5 | 8.5 | 19.9 | 16.3 | 7.6 | 6.1 | 6.9 | 5.1 |
| 60 | 0.6 | 9.6 | 8.4 | 19.0 | 15.4 | 7.9 | 6.2 | 7.0 | 5.1 |
| 80 | 0.6 | 12.8 | 11.3 | 23.5 | 19.4 | 8.7 | 6.9 | 5.4 | 3.9 |
| 40 | 0.8 | 10.3 | 7.2 | 7.0 | 4.5 | 1.9 | 1.2 | 1.4 | 0.8 |
| 40 | 0.8 | 10.0 | 7.0 | 6.7 | 4.3 | 1.4 | 0.9 | 1.4 | 0.8 |
| 60 | 0.8 | 9.3 | 6.4 | 13.0 | 8.7 | 2.9 | 1.8 | 1.9 | 1.1 |
| 80 | 0.8 | 10.2 | 7.1 | 15.0 | 10.1 | 4.1 | 2.7 | 3.4 | 2.0 |
| 80 | 0.8 | 10.0 | 7.2 | 15.8 | 10.6 | 4.0 | 2.6 | 3.4 | 2.0 |
| Dependence of MCC-m% on T and χDMSO | |||
| Entry | Ionic Liquid | R2 a | |
| 1 | AlBzMe2AcO | MCC-m% = −0.59 + 5.23 T + 0.46 T2 + 23.63 (χDMSO) − 13.94 (χDMSO)2 − 4.20 T × (χDMSO) | 0.946 |
| 2 | C3OMeImAcO | MCC-m% = 14.38 + 9.57 T − 2.10 T2 + 10.18 (χDMSO) − 17.20 (χDMSO)2 + 1.10 T × (χDMSO) | 0.986 |
| 3 | DBUHAcO | MCC-m% = −0.65 + 10.54 T − 4.08 T2 + 15.04 (χDMSO) − 13.49 (χDMSO)2 − 3.25 T × (χDMSO) | 0.936 |
| 4 | TMGHAcO | MCC-m% = +1.43 + 9.35 T − 4.09 T2 + 8.25 (χDMSO) − 8.49 (χDMSO)2 − 3.90 T × (χDMSO) | 0.883 |
| Dependence of MCC-χ on T and χDMSO | |||
| Entry | Ionic Liquid | R2 a | |
| 5 | AlBzMe2AcO | MCC-χ = −0.45 + 5.33 T + 0.30 T2 + 21.78 (χDMSO) − 15.05 (χDMSO)2 − 4.46 T × (χDMSO) | 0.944 |
| 6 | C3OMeImAcO | MCC-χ = 13.51 + 8.39 T − 1.14 T2 + 4.36 (χDMSO) − 13.14 (χDMSO)2 + 1.20 T × (χDMSO) | 0.992 |
| 7 | DBUHAcO | MCC-χ = −0.43 + 8.48 T − 2.70 T2 + 12.23 (χDMSO) − 10.80 (χDMSO)2 −4.20 T × (χDMSO) | 0.933 |
| 8 | TMGHAcO | MCC-χ = +1.18 + 7.82 T − 3.29 T2 + 5.19 (χDMSO) − 5.69 (χDMSO)2 − 3.80 T × (χDMSO) | 0.903 |
| Entry | Ionic Liquid | Variable Employed | (WT%) Calculated | (WT%) Experimental | Δwt% |
|---|---|---|---|---|---|
| 1 | AlBzMe2NAcO | 60 °C/0.70 χDMSO | 10.4 | 10.0 | −4.0 |
| 2 | AlBzMe2NAcO | 55 °C/0.50 χDMSO | 6.1 | 5.8 | −5.1 |
| 3 | C3OMeImAcO | 70 °C/0.80 χDMSO | 14.2 | 14.1 | −0.7 |
| 4 | C3OMeImAcO | 35 °C/0.30 χDMSO | 10.5 | 11.0 | 4.5 |
| 5 | DBUHAcO | 55 °C/0.70 χDMSO | 5.5 | 5.8 | 5.2 |
| 6 | DBUHAcO | 70 °C/0.50 χDMSO | 7.3 | 7.5 | 2.7 |
| 7 | TMGHAcO | 55 °C/0.50 χDMSO | 5.5 | 5.2 | −5.8 |
| 8 | TMGHAcO | 80 °C/0.70 χDMSO | 5.1 | 4.8 | −6.2 |
Sample Availability: Samples of the compounds are not available from the authors. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dignani, M.T.; Bioni, T.A.; Paixão, T.R.L.C.; El Seoud, O.A. Cellulose Dissolution in Mixtures of Ionic Liquids and Dimethyl Sulfoxide: A Quantitative Assessment of the Relative Importance of Temperature and Composition of the Binary Solvent. Molecules 2020, 25, 5975. https://doi.org/10.3390/molecules25245975
Dignani MT, Bioni TA, Paixão TRLC, El Seoud OA. Cellulose Dissolution in Mixtures of Ionic Liquids and Dimethyl Sulfoxide: A Quantitative Assessment of the Relative Importance of Temperature and Composition of the Binary Solvent. Molecules. 2020; 25(24):5975. https://doi.org/10.3390/molecules25245975
Chicago/Turabian StyleDignani, Marcella T., Thaís A. Bioni, Thiago R. L. C. Paixão, and Omar A. El Seoud. 2020. "Cellulose Dissolution in Mixtures of Ionic Liquids and Dimethyl Sulfoxide: A Quantitative Assessment of the Relative Importance of Temperature and Composition of the Binary Solvent" Molecules 25, no. 24: 5975. https://doi.org/10.3390/molecules25245975
APA StyleDignani, M. T., Bioni, T. A., Paixão, T. R. L. C., & El Seoud, O. A. (2020). Cellulose Dissolution in Mixtures of Ionic Liquids and Dimethyl Sulfoxide: A Quantitative Assessment of the Relative Importance of Temperature and Composition of the Binary Solvent. Molecules, 25(24), 5975. https://doi.org/10.3390/molecules25245975








