Volumetric, Compressibility and Viscometric Approach to Study the Interactional Behaviour of Sodium Cholate and Sodium Deoxycholate in Aqueous Glycyl Glycine
Abstract
:1. Introduction
2. Results and Discussion
2.1. Volumetric and Compressibility Studies
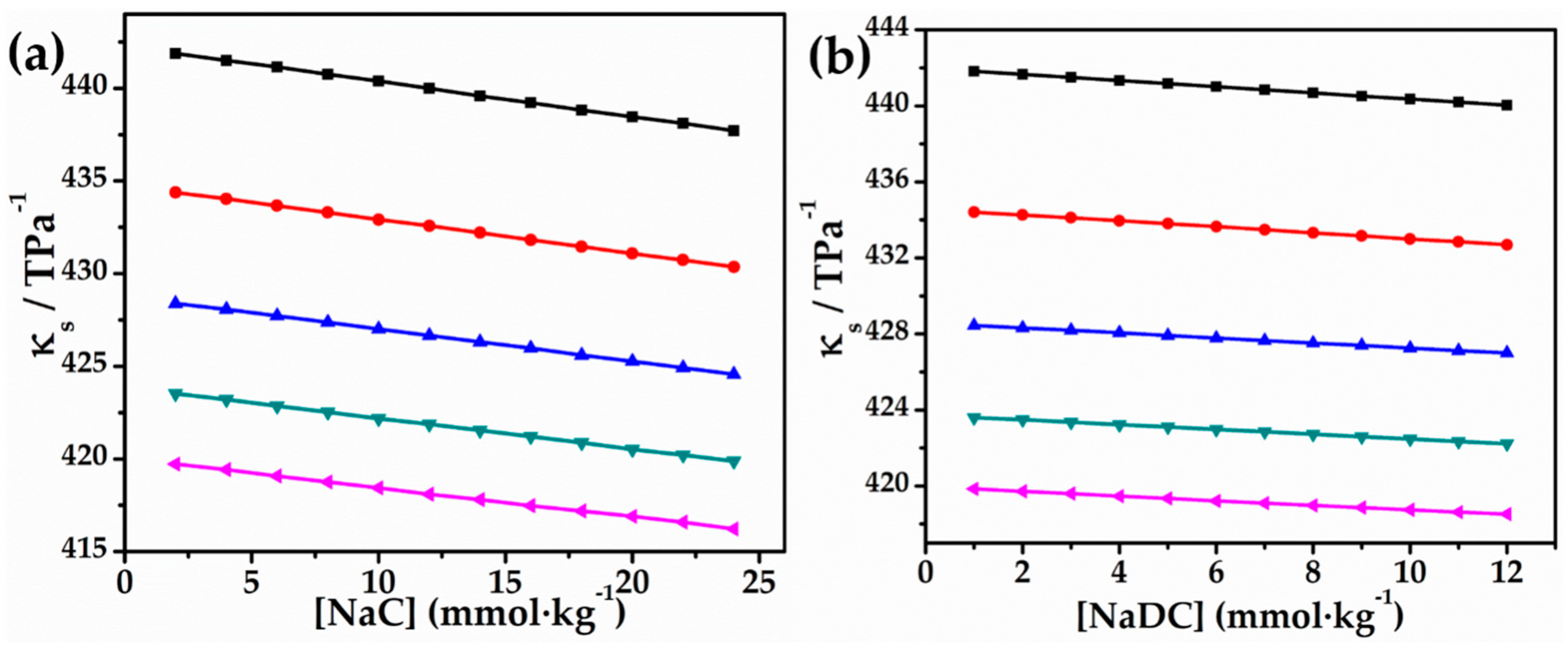
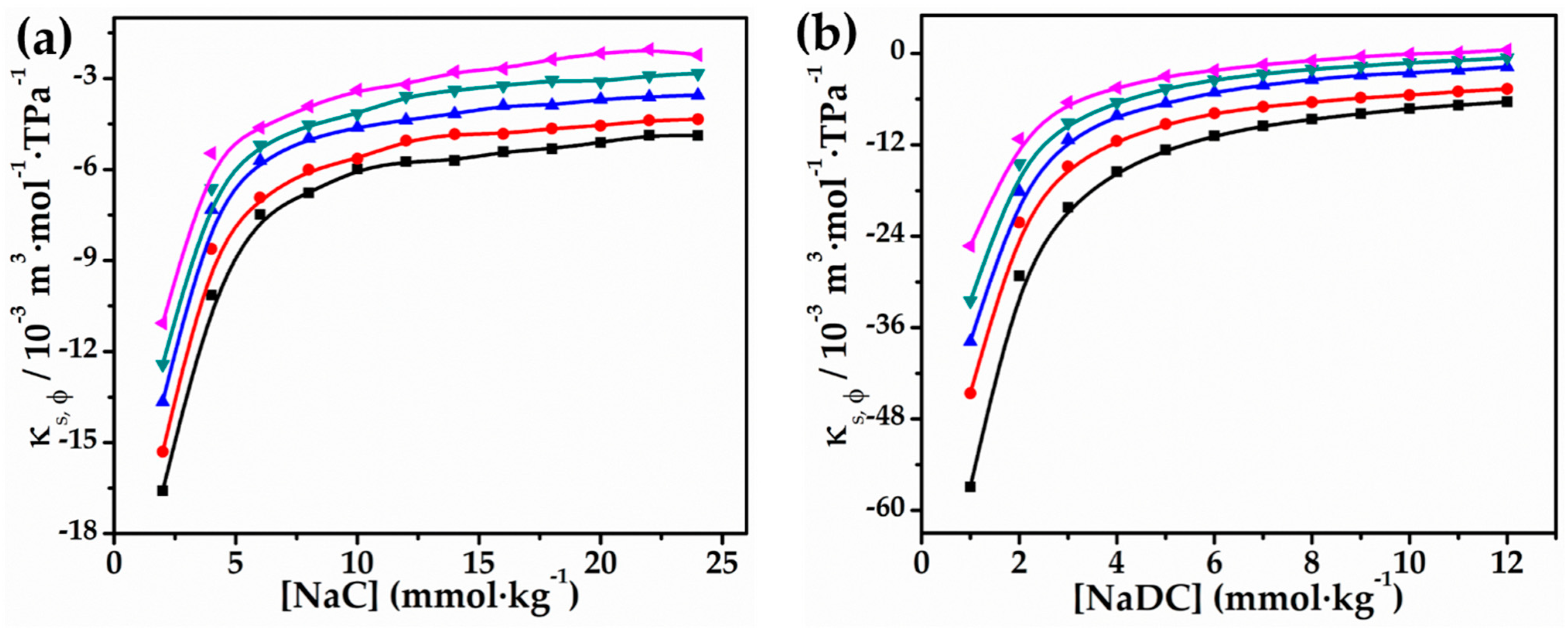
- (a)
- Hydrophobic–hydrophobic interactions between the hydrophobic part of NaC/NaDC and the non-polar part of the glycylglycine,
- (b)
- Hydrophobic–hydrophilic interactions between the hydrophobic part NaC/NaDC and the hydrophilic groups of glycyl glycineor viceversa,
- (c)
- Hydrogen bonding and other hydrophilic–hydrophilic interactions link the hydrophilic groups of NaC/NaDC to the hydrophilic groups of gylycyl glycine,
- (d)
- Ion–ion interactions between the glycylglycine –COO−/NH3+ ions and the polar region of NaC/NaDC.
2.2. Viscometric Studies
3. Experimental Details
3.1. Materials
3.2. Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Shah, S.K.; Chattergee, S.K.; Bhattarai, A. The Effect of Methanol on the Micellar Properties of Dodecyltrimethylammonium Bromide (DTAB) in Aqueous Medium at Different Temperatures. J. Dsurfact. Detrerg. 2016, 19, 201–207. [Google Scholar] [CrossRef]
- Jha, K.; Bhattarai, A.; Chatterjee, S.K. Determination of critical micelle concentration of cetyltrimethylammonium bromide in presence and absence of KCl and NaCl in aqueous media at room temperature by viscosity measurement. Bibechana 2014, 11, 131–135. [Google Scholar] [CrossRef] [Green Version]
- Lowe, M.E. The triglyceride lipases of the pancreas. J. Lipid Res. 2002, 43, 2007–2016. [Google Scholar] [CrossRef] [Green Version]
- Waldmann, T.A.; Rosenoer, I.V.M.; Oratz, M.; Rotschild, M.A. Albumin: Structure, Function and Uses; Pergamon Press: London, UK, 1977. [Google Scholar]
- Tochtrop, G.P.; Dekoster, G.T.; Covey, D.F.; Cistola, D.P. A single hydroxyl group governs ligand site selectivity in human ilea bile acid binding protein. J. Am. Chem. Soc. 2004, 126, 11024–11029. [Google Scholar] [CrossRef]
- Antonelli, M.A.; Capalbi, A.; Genta, G.; Palacios, A.D.; Sallustio, S.; Mesa, C.L. Thermodynamic properties of the bovine serum albumin–sodium taurodeoxycholate system. Colloids Surf. A 2004, 246, 127–134. [Google Scholar] [CrossRef]
- Liu, C.L. Interactions and Molecular Weights of Simple Micelles and Mixed Micelles in Taurocholate and Taurocholate−Lecithin Solutions. J. Phys. Chem. B 1997, 101, 7055–7059. [Google Scholar] [CrossRef]
- Funasaki, N.; Hada, S.; Neya, S. Self-Association Patterns of Sodium Taurocholate and Taurodeoxycholate As Studied by Frontal Derivative Chromatography. J. Phys. Chem. B 1999, 103, 169–172. [Google Scholar] [CrossRef]
- Funasaki, N.; Nomura, M.; Ishikawa, S.; Neya, S. Hydrophobic Self-Association of Sodium Taurochenodeoxycholate and Tauroursodeoxycholate. J. Phys. Chem. B 2000, 104, 7745–7751. [Google Scholar] [CrossRef]
- Singh, S.K.; Kishore, N. Partial Molar Volumes of Amino Acids and Peptides in Aqueous Salt Solutions at 25 °C and a Correlation with Stability of Proteins in the Presence of Salts. J. Solut. Chem. 2003, 32, 117–135. [Google Scholar] [CrossRef]
- Wang, J.J.; Yan, Z.N.; Lu, J.S. Effect of sodium caproate on the volumetric and viscometric properties of glycine, dl-α-alanine, and dl-α-amino-n-butyric acid in aqueous solutions. J. Chem. Thermodyn. 2004, 36, 281–288. [Google Scholar] [CrossRef]
- Wang, J.J.; Yan, Z.N.; Zhuo, K.L.; Lu, J.S. Partial molar volumes of some α-amino acids in aqueous sodium acetate solutions at 308.15 K. Biophys. Chem. 1999, 80, 179–188. [Google Scholar] [CrossRef]
- Yan, Z.N.; Wang, J.J.; Lu, J.S. Apparent Molar Volumes and Viscosities of Some α-Amino Acids in Aqueous Sodium Butyrate Solutions at 298.15 K. J. Chem. Eng. Data 2001, 46, 217–222. [Google Scholar] [CrossRef]
- Wang, J.J.; Yan, Z.N.; Zhuo, K.L.; Liu, D.Z. Standard Volumes of Transfer for Some α-Amino Acids from Water to Aqueous Sodium Acetate Solutions at 298.15 K. Z. Phys. Chem. 2000, 214, 333–345. [Google Scholar] [CrossRef]
- Yan, Z.N.; Wang, J.J.; Zhang, H.L.; Xuan, X.P. Volumetric and Viscosity Properties of α-Amino Acids and Their Groups in Aqueous Sodium Caproate Solutions. J. Chem. Eng. Data 2005, 50, 1864–1870. [Google Scholar] [CrossRef]
- Yan, Z.N.; Wang, X.G.; Zhao, Y.; Wang, J.J. Volumetric Properties of Glycyl Dipeptides in Aqueous Sodium Acetate Solutions at 298.15 K. Acta Chim. Sin. 2009, 67, 115–121. [Google Scholar]
- Yan, Z.N.; Zhao, Y.; Xing, R.H.; Wang, X.G.; Wang, J.J. Volumetric and Conductometric Behavior at T = 298.15 K of 2-[(2-Aminoacetyl)amino]acetic Acid, 2-[(2-Aminoacetyl)amino]-3-methylbutanoic Acid, and (2 S)-2-[(2-Aminoacetyl)amino]-4-methylpentanoic Acid with Sodium Hexanoate. J. Chem. Eng. Data 2010, 55, 759–764. [Google Scholar] [CrossRef]
- Yan, Z.N.; Wang, X.G.; Xing, R.H.; Wang, J.J. Interactions of Some Glycyl Dipeptides with Sodium Butyrate in Aqueous Solutions at 298.15 K: A Volumetric and Conductometric Study. J. Chem. Eng. Data 2009, 54, 1787–1792. [Google Scholar] [CrossRef]
- Yan, Z.N.; Wang, X.G.; Xing, R.H.; Wang, J.J. Volumetric and conductometric studies on the interactions of dipeptides with sodium acetate and sodium butyrate in aqueous solutions at T = 298.15 K. J. Chem. Thermodyn. 2009, 41, 1343–1349. [Google Scholar] [CrossRef]
- Yan, Z.N.; Li, W.W.; Zhang, Q.; Wang, X.G.; Wang, J.J. Effect of sodium caproate on the volumetric and conductometric properties of glycyl-L-glutamine and L-alanyl-L-glutamine in aqueous solution at 298.15 K. Fluid Phase Equilib. 2011, 301, 156–162. [Google Scholar] [CrossRef]
- Gumpen, S.; Hegg, P.O.; Martens, H. Thermal stability of fatty acid-serum albumin complexes studied by differential scanning calorimetry. Biochem. Biophys. Acta 1979, 574, 189–196. [Google Scholar] [CrossRef]
- Waninge, R.; Paulsson, M.; Nylander, T.; Ninham, B.; Sellers, P. Binding of Sodium Dodecyl Sulphate and Dodecyl Trimethyl Ammonium Chloride to β-Lactoglobulin: A Calorimetric Study. Int. Dairy J. 1998, 8, 141–148. [Google Scholar] [CrossRef]
- Nozaki, Y.; Reynolds, J.A.; Tanford, C. The interaction of a cationic detergent with bovine serum albumin and other proteins. J. Biol. Chem. 1974, 249, 4452–4459. [Google Scholar] [CrossRef]
- Yan, Z.; Liu, R.; Wu, S.; Bai, X.; Wang, J. Effect of temperature on the interactions of glycyl dipeptides with sodium perfluorooctanoate in aqueous solution: Volumetric, conductometric, and spectroscopic study. J. Chem. Thermodyn. 2013, 57, 360–366. [Google Scholar] [CrossRef]
- Chauhan, S.; Sharma, K.; Kumar, K.; Kumar, G. A comparative study of micellization behavior of an ethoxylated alkylphenol in aqueous solutions of glycine and leucine. J. Surfact. Deterg. 2014, 17, 161–168. [Google Scholar] [CrossRef]
- Bhuiyan, M.M.H.; Hakin, A.W.; Liu, J.L. Densities, Specific Heat Capacities, Apparent and Partial Molar Volumes and Heat Capacities of Glycine in Aqueous Solutions of Formamide, Acetamide, and N,N-Dimethylacetamide at T=298.15 K and Ambient Pressure. J. Sol. Chem. 2010, 39, 877–896. [Google Scholar] [CrossRef]
- Zafarani-Moattar, C.M.T.; Sarmad, S. Apparent molar volumes, apparent isentropic compressibilities, and viscosity B-coefficients of 1-ethyl-3-methylimidazolium bromide in aqueous di-potassium hydrogen phosphate and potassium di-hydrogen phosphate solutions at T = (298.15, 303.15, 308.15, 313.15, and 318.15) K. J. Chem. Thermodyn. 2012, 54, 192–203. [Google Scholar]
- Warminska, D.; Fuchs, A.; Lundberg, D. Apparent molar volumes and compressibilities of lanthanum, gadolinium, lutetium and sodium trifluoromethanesulfonates in N,N-dimethylformamide and N,N-dimethylacetamide. J. Chem. Thermodyn. 2013, 58, 46–54. [Google Scholar] [CrossRef]
- Singh, K.; Chauhan, S. Interactional Behavior of Sodium Cholate and Sodium Deoxycholate in the Presence of Ceftriaxone Sodium: Volumetric, Compressibility, Viscometric, and Proton Nuclear Magnetic Resonance Studies. J. Chem. Eng. Data 2020, 5, 4536–4546. [Google Scholar] [CrossRef]
- Gill, D.S.; Kaur, T.; Joshi, I.M.; Singh, J. Ultrasonic velocity, permittivity, density, viscosity and proton nuclear magnetic resonance measurements of binary mixtures of benzonitrile with organic solvents. J. Chem. Soc. Faraday Trans. 1993, 89, 1737–1740. [Google Scholar] [CrossRef]
- Chauhan, S.; Sharma, V.; Sharma, K. Maltodextrin–SDS interactions: Volumetric, viscometric and surface tension study. Fluid Phase Equilib. 2013, 354, 236–244. [Google Scholar] [CrossRef]
- Chauhan, S.; Chauhan, M.S.; Sharma, P.; Rana, D.S. Thermodynamics and micellization of cetyltrimethyl ammonium bromide in the presence of lysozyme. J. Mol. Liq. 2013, 187, 1–6. [Google Scholar] [CrossRef]
- Chauhan, S.; Chauhan, M.S.; Sharma, P.; Rana, D.S.; Umar, A. Physico-chemical studies of oppositely charged protein–surfactant system in aqueous solutions: Sodium dodecyl sulphate (SDS)–lysozyme. Fluid Phase Equilib. 2013, 337, 39–46. [Google Scholar] [CrossRef]
- Sahin, M.; Ayranci, E. Volumetric properties of (ascorbic acid+ polyethylene glycol 3350+ water) systems at T = (288.15, 298.15, and 308.15) K. J. Chem. Thermodyn. 2011, 43, 177–185. [Google Scholar] [CrossRef]
- Fried Man, H.; Krishnan, C.V. Water: A Comprehensive Treatise; Plenum Press: New York, NY, USA, 1973. [Google Scholar]
- Lee, Y.S.; Woo, K.W. Micellization of Aqueous Cationic Surfactant Solutions at the Micellar Structure Transition Concentration—Based upon the Concept of the Pseudophase Separation. Colloids Interface Sci. 1995, 169, 34–38. [Google Scholar] [CrossRef]
- Mehrian, T.; de Keizer, A.; Korteweg, A. Lyklema Thermodynamics of micellization of n-alkylpyridinium chlorides. J. Colloids Surf. 1993, 71, 255–267. [Google Scholar] [CrossRef]
- Moren, A.K.; Khan, A. Surfactant Hydrophobic Effect on the Phase Behavior of Oppositely Charged Protein and Surfactant Mixtures: Lysozyme and Sodium Alkyl Sulfates. Langmuir 1998, 14, 6818–6826. [Google Scholar] [CrossRef]
- Lomesh, S.K.; Nathan, V.; Bala, M.; Thakur, P. Volumetric and acoustic methods for investigating molecular interactions of antibiotic drug doxycycline hyclate in water and in aqueous solution of sodium chloride and potassium chloride at different temperatures (293.15–313.15) K. J. Mol.Liq. 2019, 284, 241–251. [Google Scholar] [CrossRef]
- Kumar, K.; Chauhan, S. Volumetric, compressibility and viscometric studies on sodium cholate/sodium deoxycholate–amino acid interactions in aqueous medium. Thermochim. Acta 2015, 606, 12–24. [Google Scholar] [CrossRef]
- Chauhan, S.; Sharma, V.; Kaur, M.; Chaudhary, P. Temperature-dependent aggregation of bio-surfactants in aqueous solutions of galactose and lactose: Volumetric and viscometric approach. Chin. J. Chem. Eng. 2018, 26, 1119–1131. [Google Scholar] [CrossRef]
- Bakshi, M.S.; Crisantino, R.; Lisi, R.D.; Milioto, S. Volume and heat capacity of sodium dodecyl sulfate-dodecyldimethylamine oxide mixed micelles. J. Phys. Chem. 1993, 97, 6914–6919. [Google Scholar] [CrossRef]
- Bakshi, M.S. Host-guest interactions. I. Volumes of the sucrose +β-cyclodextrin+water ternary systems at 25 °C. J. Solut. Chem. 1996, 25, 411–420. [Google Scholar] [CrossRef]
- Sadeghi, A.; Shahabi, S. A comparison study between sodium dodecyl sulfate and sodium dodecyl sulfonate with respect to the thermodynamic properties, micellization, and interaction with poly(ethylene glycol) in aqueous solutions. J. Chem. Thermodyn. 2011, 43, 1361–1370. [Google Scholar] [CrossRef]
- Brun, T.S.; Hoiland, H.; Vikingstand, E. Partial molal volumes and isentropic partial molal compressibilities of surface-active agents in aqueous solution. J. Colloid Interface Sci. 1978, 63, 89–96. [Google Scholar] [CrossRef]
- Rajagopal, K.; Gladson, S.E. Partial molar volume and partial molar compressibility of four homologous α-amino acids in aqueous sodium fluoride solutions at different temperatures. J. Chem. Thermodyn. 2011, 43, 852–867. [Google Scholar] [CrossRef]
- Chauhan, S.; Singh, K. Volumetric, Compressibility, Viscometric, and 1H NMR Analysis on Drug–Bile Salts Interactions in Aqueous Medium: Temperature and Concentration Effect. J. Chem. Eng. Data 2019, 64, 69–82. [Google Scholar] [CrossRef]
- Ananthapadmanabham, K.P. Interaction of Surfactant with Polymers and Proteins; CRC Press: Boca Raton, FL, USA, 1993; pp. 319–365. [Google Scholar]
- Nain, A.K.; Pal, R. Physicochemical study of solute–solute and solute–solvent interactions of l-phenylalanine in (water+ arabinose/glucose/sucrose) solutions at different temperatures. J. Chem. Thermodyn. 2014, 68, 169–182. [Google Scholar] [CrossRef]
- Riyazuddeen; Usmani, M.A. Densities, Speeds of Sound, and Viscosities of (ʟ-Proline + Aqueous Glucose) and (ʟ-Proline + Aqueous Sucrose) Solutions in the Temperature Range (298.15 to 323.15) K. J. Chem. Eng. Data 2011, 56, 3504–3509. [Google Scholar] [CrossRef]
- Syal, V.K.; Kumari, U.; Chauhan, S.; Chauhan, M.S.; Sud, S.P.; Singh, B. Ultrasonic Studies of Alkali Bromides in Dimethylsulfoxide + Dioxane Solvent Mixtures At 25-Degrees-C. Ind. J. Pure Appl. Phys. 1992, 30, 719–723. [Google Scholar]
- Syal, V.K.; Bhalla, V.; Chauhan, S. Ultrasonic studies of some tetraalkylammonium salts in acetonitrile+dioxane mixtures at 35 °C. Acustica 1995, 81, 276–278. [Google Scholar]
- Syal, V.K.; Lal, G.; Bisht, P.; Chauhan, S. Ultrasonic measurements of some 1:1 electrolytes in chlorobenzene + methanol mixtures. J. Mol. Liq. 1995, 63, 317–328. [Google Scholar] [CrossRef]
- Iqbal, M.; Verrall, R.E. Volumetric properties of aqueous solutions of bovine serum albumin, human serum albumin, and human hemoglobin. J. Phys. Chem. 1987, 91, 1935–1941. [Google Scholar] [CrossRef]
- Sadeghi, R.; Ziaii, M.J. Thermodynamic investigation of the systems poly(ethylene glycol) + sodium pentane-1-sulfonate + water and poly(vinyl pyrrolidone) + sodium pentane-1-sulfonate + water. Colloid Interface Sci. 2010, 346, 107–117. [Google Scholar] [CrossRef]
- Cabani, S.; Conti, G.; Matteoli, E. Adiabatic and isothermal apparent molal compressibilities of organic compounds in water. I. Cyclic and open-chain secondary alcohols and ethers. J. Sol. Chem. 1979, 8, 11–23. [Google Scholar] [CrossRef]
- Ravichandran, G.; Lakshiminarayanan, G.; Ragouramane, D. Apparent molar volume and ultrasonic studies on some bile salts in water–aprotic solvent mixtures. Fluid Phase Equilb. 2013, 356, 256–263. [Google Scholar] [CrossRef]
- Lindmann, B.; Wennerstrom, H. Topics in Current Chemistry; Dewar, M.J.S., Hafner, K., Heilbronner, E., Ito, S., Lehn, J.M., Niedenzu, K., Rees, C.W., Schafer, K., Wittig, G., Eds.; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1980. [Google Scholar]
- Chauhan, S.; Chauhan, M.S.; Kaushal, D.; Syal, V.K.; Jyoti, J. Study of micellar behavior of SDS and CTAB in aqueous media containing furosemide—A cardiovascular drug. J. Solut. Chem. 2010, 39, 622–638. [Google Scholar] [CrossRef]
- Chauhan, S.; Jyoti, J.; Kumar, G. Non-ionic surfactant interactions in aqueous gelatin solution: A physico-chemical investigation. J. Mol. Liq. 2011, 159, 196–200. [Google Scholar] [CrossRef]
- Manna, K.; Chang, C.H.; Panda, A.K. Physicochemical studies on the catanionics of alkyltrimethylammonium bromides and bile salts in aqueous media. Colloids Surf. A 2012, 415, 10–21. [Google Scholar] [CrossRef]
- Iqbal, M.J.; Chaudhary, M.A. Volumetric and Viscometric Studies of Antidepressant Drugs in Aqueous Medium at Different Temperatures. J. Chem. Eng. Data 2009, 54, 2772–2776. [Google Scholar] [CrossRef]
- Kaur, K.; Kumar, H. Investigation on molecular interaction of amino acids in antibacterial drug ampicillin solutions with reference to volumetric and compressibility measurements. J. Mol. Liq. 2012, 173, 130–136. [Google Scholar]
- Naik, A.B. Densities, viscosities, speed of sound and some acoustical parameter studies of substituted pyrazoline compounds at different temperatures. Indian J. Pure Appl. Phys. 2015, 53, 27–34. [Google Scholar]
- Abezgauz, L.; Kuperkar, K.; Hassan, P.A.; Ramon, O.; Bahadur, P.; Danino, D. Effect of Hofmeister anions on micellization and micellar growth of the surfactant cetylpyridinium chloride. J. Colloid Interface Sci. 2010, 342, 83–92. [Google Scholar] [CrossRef]
- Kumar, K.; Patial, B.S.; Chauhan, S. Interactions of saccharides in aqueous glycine and leucine solutions at different temperatures of (293.15 to 313.15) K: A viscometric study. J. Chem. Eng. Data 2015, 60, 47–56. [Google Scholar] [CrossRef]
- Sharma, K.; Chauhan, S.; Priya, B. Surface, compressibility and viscometric measurements of binary system containing cationic surfactant with achiral amino acid at different temperatures. J. Mol. Liq. 2016, 222, 407–414. [Google Scholar] [CrossRef]
- George, J.; Nair, S.M.; Sreejith, L. Interactions of Sodium Dodecyl Benzene Sulfonate and Sodium Dodecyl Sulfate with Gelatin: A Comparison. J. Surfactants Deterg. 2008, 11, 29–32. [Google Scholar] [CrossRef]
- Praharaj, M.K.; Mishra, S.; Satapathy, A. Ultrasonic study of ternary liquid mixture containing substituted benzene. Arch. Phys. Res. 2012, 3, 192–200. [Google Scholar]
- Dhondge, S.S.; Zodape, S.P.; Parwate, D.V. Volumetric and viscometric studies of some drugs in aqueous solutions at different temperatures. J. Chem. Thermodyn. 2012, 48, 207–212. [Google Scholar] [CrossRef]
- Chauhan, S.; Kumari, S.; Singh, K. Conductometric and fluorescence probe analysis on molecular interactions between cationic surfactants in aqueous medium of glycyl dipeptide: Concentration and temperature effect. J. Chem. Thermodyn. 2017, 105, 337–344. [Google Scholar] [CrossRef]
- Chauhan, S.; Kumar, K. Partial molar volumes and isentropic compressibilities of some saccharides in aqueous solutions of leucine at different temperatures. J. Chem. Eng. Data 2014, 59, 1375–1384. [Google Scholar] [CrossRef]


| NaC | NaDC | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| [NaC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K | [NaDC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K |
| [Pure Water] | |||||||||||
| 2 | 30.43 | 30.70 | 30.98 | 31.21 | 31.45 | 1 | 23.76 | 23.97 | 24.38 | 25.10 | 25.93 |
| 4 | 31.50 | 31.70 | 32.01 | 32.17 | 32.28 | 2 | 27.07 | 27.94 | 28.06 | 29.20 | 29.08 |
| 6 | 31.84 | 32.10 | 32.33 | 32.45 | 32.52 | 3 | 28.84 | 29.76 | 29.99 | 30.53 | 30.39 |
| 8 | 31.85 | 32.25 | 32.49 | 32.57 | 32.68 | 4 | 29.90 | 30.57 | 30.80 | 31.14 | 31.25 |
| 10 | 31.91 | 32.23 | 32.48 | 32.58 | 32.75 | 5 | 30.57 | 31.08 | 31.45 | 31.65 | 31.83 |
| 12 | 31.99 | 32.27 | 32.48 | 32.58 | 32.74 | 6 | 31.09 | 31.40 | 31.92 | 32.04 | 32.16 |
| 14 | 31.97 | 32.23 | 32.48 | 32.59 | 32.75 | 7 | 31.28 | 31.63 | 32.06 | 32.21 | 32.41 |
| 16 | 31.99 | 32.28 | 32.46 | 32.64 | 32.82 | 8 | 31.42 | 31.61 | 32.14 | 32.24 | 32.42 |
| 18 | 31.95 | 32.34 | 32.53 | 32.68 | 32.87 | 9 | 31.35 | 31.73 | 32.13 | 32.43 | 32.58 |
| 20 | 32.00 | 32.30 | 32.60 | 32.70 | 32.96 | 10 | 31.45 | 31.78 | 32.14 | 32.45 | 32.57 |
| 22 | 31.98 | 32.39 | 32.60 | 32.79 | 32.93 | 11 | 31.38 | 31.77 | 32.27 | 32.52 | 32.51 |
| 24 | 32.06 | 32.40 | 32.65 | 32.80 | 32.99 | 12 | 31.50 | 31.81 | 32.27 | 32.54 | 32.54 |
| [Glycyl glycine] = 0.001 mol∙kg−1 | |||||||||||
| 2 | 30.58 | 30.90 | 31.13 | 31.26 | 31.45 | 1 | 23.96 | 24.87 | 25.49 | 26.42 | 28.06 |
| 4 | 31.63 | 32.18 | 32.26 | 32.32 | 32.28 | 2 | 27.92 | 28.44 | 28.82 | 29.85 | 31.06 |
| 6 | 31.90 | 32.35 | 32.51 | 32.58 | 32.72 | 3 | 29.04 | 29.66 | 30.33 | 31.10 | 31.85 |
| 8 | 31.98 | 32.49 | 32.58 | 32.75 | 32.84 | 4 | 30.00 | 30.50 | 31.33 | 31.62 | 32.24 |
| 10 | 32.02 | 32.53 | 32.58 | 32.76 | 32.91 | 5 | 30.63 | 31.08 | 31.69 | 31.93 | 32.44 |
| 12 | 32.00 | 32.53 | 32.63 | 32.71 | 32.96 | 6 | 31.14 | 31.31 | 32.02 | 32.31 | 32.64 |
| 14 | 32.00 | 32.51 | 32.63 | 32.80 | 32.98 | 7 | 31.41 | 31.51 | 32.19 | 32.34 | 32.67 |
| 16 | 31.99 | 32.50 | 32.58 | 32.71 | 33.00 | 8 | 31.71 | 31.67 | 32.28 | 32.39 | 32.65 |
| 18 | 31.96 | 32.52 | 32.62 | 32.68 | 32.94 | 9 | 31.75 | 31.89 | 32.42 | 32.52 | 32.78 |
| 20 | 32.04 | 32.44 | 32.65 | 32.70 | 32.99 | 10 | 31.81 | 32.05 | 32.54 | 32.59 | 32.92 |
| 22 | 31.94 | 32.49 | 32.63 | 32.75 | 32.94 | 11 | 31.88 | 32.03 | 32.58 | 32.75 | 33.09 |
| 24 | 32.02 | 32.48 | 32.60 | 32.78 | 32.94 | 12 | 31.98 | 32.06 | 32.57 | 32.82 | 33.04 |
| [Glycyl glycine] = 0.005 mol∙kg−1 | |||||||||||
| 2 | 30.78 | 30.95 | 31.23 | 31.31 | 31.70 | 1 | 24.16 | 25.17 | 26.70 | 27.83 | 28.97 |
| 4 | 31.67 | 32.15 | 32.36 | 32.59 | 32.76 | 2 | 27.72 | 28.74 | 29.62 | 30.41 | 31.05 |
| 6 | 31.97 | 32.41 | 32.63 | 32.63 | 32.74 | 3 | 29.24 | 30.16 | 30.49 | 31.40 | 32.08 |
| 8 | 32.00 | 32.58 | 32.65 | 32.69 | 32.82 | 4 | 30.02 | 30.59 | 31.40 | 31.82 | 32.54 |
| 10 | 32.04 | 32.57 | 32.63 | 32.69 | 32.92 | 5 | 30.55 | 31.07 | 31.93 | 32.43 | 32.76 |
| 12 | 32.03 | 32.54 | 32.62 | 32.71 | 32.95 | 6 | 31.00 | 31.51 | 32.26 | 32.66 | 33.14 |
| 14 | 32.00 | 32.52 | 32.61 | 32.77 | 32.95 | 7 | 31.25 | 31.62 | 32.60 | 32.71 | 33.15 |
| 16 | 32.06 | 32.51 | 32.64 | 32.73 | 32.98 | 8 | 31.55 | 32.04 | 32.64 | 32.79 | 33.23 |
| 18 | 32.06 | 32.47 | 32.61 | 32.78 | 32.92 | 9 | 31.84 | 32.46 | 32.79 | 33.09 | 33.36 |
| 20 | 32.01 | 32.55 | 32.60 | 32.75 | 32.98 | 10 | 31.98 | 32.45 | 32.98 | 33.01 | 33.51 |
| 22 | 32.01 | 32.49 | 32.57 | 32.75 | 32.98 | 11 | 32.07 | 32.57 | 33.05 | 33.20 | 33.58 |
| 24 | 32.05 | 32.47 | 32.63 | 32.73 | 32.99 | 12 | 32.19 | 32.76 | 33.31 | 33.52 | 33.81 |
| [Glycyl glycine] = 0.010 mol∙kg−1 | |||||||||||
| 2 | 30.87 | 31.09 | 31.27 | 31.45 | 31.64 | 1 | 24.76 | 25.37 | 26.89 | 27.92 | 29.17 |
| 4 | 31.84 | 32.19 | 32.50 | 32.61 | 32.75 | 2 | 28.26 | 29.03 | 30.16 | 30.90 | 31.40 |
| 6 | 32.06 | 32.47 | 32.75 | 32.87 | 33.00 | 3 | 29.83 | 30.35 | 31.19 | 31.52 | 32.24 |
| 8 | 32.15 | 32.51 | 32.72 | 32.87 | 33.03 | 4 | 30.46 | 30.84 | 31.57 | 32.19 | 32.73 |
| 10 | 32.12 | 32.59 | 32.77 | 32.91 | 33.02 | 5 | 30.88 | 31.24 | 32.06 | 32.62 | 32.93 |
| 12 | 32.12 | 32.56 | 32.80 | 32.95 | 33.08 | 6 | 31.28 | 31.65 | 32.40 | 32.76 | 33.26 |
| 14 | 32.14 | 32.61 | 32.90 | 33.06 | 33.10 | 7 | 31.66 | 31.93 | 32.71 | 32.89 | 33.31 |
| 16 | 32.11 | 32.60 | 32.95 | 33.04 | 33.14 | 8 | 31.80 | 32.11 | 32.81 | 33.10 | 33.49 |
| 18 | 32.05 | 32.60 | 32.87 | 33.09 | 33.16 | 9 | 31.99 | 32.28 | 32.99 | 33.26 | 33.54 |
| 20 | 32.15 | 32.67 | 32.96 | 33.09 | 33.18 | 10 | 32.14 | 32.48 | 33.09 | 33.38 | 33.71 |
| 22 | 32.14 | 32.68 | 32.95 | 33.09 | 33.16 | 11 | 32.27 | 32.59 | 33.23 | 33.53 | 33.81 |
| 24 | 32.09 | 32.65 | 32.93 | 33.03 | 33.21 | 12 | 32.34 | 32.72 | 33.37 | 33.65 | 33.89 |
| NaC | NaDC | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| [NaC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K | [NaDC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K |
| [Pure water] | |||||||||||
| 2 | 455.03 | 447.12 | 440.49 | 435.06 | 430.72 | 1 | 454.99 | 447.25 | 440.68 | 435.28 | 430.93 |
| 4 | 454.48 | 446.73 | 440.17 | 434.74 | 430.44 | 2 | 454.68 | 447.05 | 440.45 | 435.10 | 430.79 |
| 6 | 454.09 | 446.29 | 439.76 | 434.35 | 430.10 | 3 | 454.45 | 446.85 | 440.29 | 434.94 | 430.65 |
| 8 | 453.66 | 445.82 | 439.35 | 434.00 | 429.75 | 4 | 454.24 | 446.69 | 440.14 | 434.79 | 430.51 |
| 10 | 453.16 | 445.35 | 438.95 | 433.61 | 429.40 | 5 | 454.07 | 446.51 | 439.96 | 434.65 | 430.37 |
| 12 | 452.65 | 444.89 | 438.55 | 433.23 | 429.04 | 6 | 453.89 | 446.35 | 439.85 | 434.49 | 430.23 |
| 14 | 452.18 | 444.49 | 438.14 | 432.85 | 428.69 | 7 | 453.72 | 446.17 | 439.66 | 434.31 | 430.09 |
| 16 | 451.75 | 444.06 | 437.78 | 432.48 | 428.35 | 8 | 453.56 | 445.98 | 439.47 | 434.16 | 429.95 |
| 18 | 451.38 | 443.75 | 437.47 | 432.11 | 428.00 | 9 | 453.39 | 445.76 | 439.32 | 434.01 | 429.81 |
| 20 | 450.94 | 443.47 | 437.13 | 431.80 | 427.70 | 10 | 453.22 | 445.59 | 439.14 | 433.87 | 429.66 |
| 22 | 450.74 | 443.04 | 436.78 | 431.47 | 427.43 | 11 | 453.03 | 445.43 | 438.97 | 433.73 | 429.54 |
| 24 | 450.32 | 442.68 | 436.42 | 431.16 | 427.13 | 12 | 452.87 | 445.29 | 438.85 | 433.56 | 429.41 |
| [Glycyl glycine] = 0.005 mol∙kg−1 | |||||||||||
| 2 | 454.62 | 446.73 | 440.10 | 434.70 | 430.40 | 1 | 454.58 | 446.86 | 440.26 | 434.86 | 430.53 |
| 4 | 454.08 | 446.35 | 439.77 | 434.38 | 430.10 | 2 | 454.39 | 446.64 | 440.07 | 434.70 | 430.39 |
| 6 | 453.65 | 445.90 | 439.37 | 434.01 | 429.79 | 3 | 454.14 | 446.48 | 439.93 | 434.56 | 430.26 |
| 8 | 453.24 | 445.43 | 438.98 | 433.66 | 429.43 | 4 | 453.92 | 446.32 | 439.78 | 434.41 | 430.13 |
| 10 | 452.73 | 444.95 | 438.57 | 433.28 | 429.07 | 5 | 453.73 | 446.13 | 439.61 | 434.29 | 429.98 |
| 12 | 452.25 | 444.48 | 438.16 | 432.90 | 428.71 | 6 | 453.52 | 445.93 | 439.45 | 434.15 | 429.84 |
| 14 | 451.80 | 444.08 | 437.77 | 432.52 | 428.37 | 7 | 453.32 | 445.75 | 439.32 | 434.00 | 429.71 |
| 16 | 451.34 | 443.67 | 437.37 | 432.18 | 428.02 | 8 | 453.13 | 445.58 | 439.14 | 433.85 | 429.54 |
| 18 | 450.96 | 443.37 | 437.12 | 431.82 | 427.69 | 9 | 452.93 | 445.41 | 438.99 | 433.70 | 429.40 |
| 20 | 450.52 | 443.05 | 436.78 | 431.47 | 427.35 | 10 | 452.73 | 445.26 | 438.85 | 433.54 | 429.26 |
| 22 | 450.35 | 442.70 | 436.41 | 431.14 | 427.01 | 11 | 452.57 | 445.09 | 438.70 | 433.42 | 429.14 |
| 24 | 449.91 | 442.31 | 436.06 | 430.83 | 426.68 | 12 | 452.40 | 444.91 | 438.55 | 433.29 | 429.00 |
| [Glycyl glycine] = 0.010 mol∙kg−1 | |||||||||||
| 2 | 454.18 | 446.30 | 439.67 | 434.28 | 430.00 | 1 | 454.18 | 446.40 | 439.81 | 434.40 | 430.12 |
| 4 | 453.63 | 445.90 | 439.34 | 433.94 | 429.70 | 2 | 453.98 | 446.26 | 439.65 | 434.27 | 429.98 |
| 6 | 453.18 | 445.46 | 438.93 | 433.57 | 429.32 | 3 | 453.78 | 446.08 | 439.48 | 434.12 | 429.86 |
| 8 | 452.78 | 445.01 | 438.55 | 433.22 | 429.02 | 4 | 453.63 | 445.91 | 439.34 | 433.98 | 429.73 |
| 10 | 452.27 | 444.56 | 438.15 | 432.84 | 428.66 | 5 | 453.46 | 445.72 | 439.17 | 433.85 | 429.58 |
| 12 | 451.86 | 444.12 | 437.75 | 432.50 | 428.31 | 6 | 453.28 | 445.57 | 439.01 | 433.70 | 429.44 |
| 14 | 451.37 | 443.70 | 437.36 | 432.08 | 427.98 | 7 | 453.12 | 445.40 | 438.88 | 433.57 | 429.31 |
| 16 | 451.00 | 443.26 | 436.96 | 431.72 | 427.64 | 8 | 452.97 | 445.23 | 438.70 | 433.40 | 429.18 |
| 18 | 450.57 | 442.91 | 436.69 | 431.35 | 427.34 | 9 | 452.82 | 445.09 | 438.56 | 433.25 | 429.07 |
| 20 | 450.11 | 442.66 | 436.33 | 431.02 | 427.00 | 10 | 452.65 | 444.92 | 438.40 | 433.12 | 428.94 |
| 22 | 449.90 | 442.26 | 435.94 | 430.69 | 426.70 | 11 | 452.50 | 444.76 | 438.26 | 432.99 | 428.80 |
| 24 | 449.49 | 441.89 | 435.65 | 430.44 | 426.37 | 12 | 452.31 | 444.59 | 438.11 | 432.87 | 428.67 |
| NaC | NaDC | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| [NaC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K | [NaDC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K |
| [Pure Water] | |||||||||||
| 2 | −21.46 | −16.73 | −14.63 | −13.29 | −11.48 | 1 | −63.10 | −36.73 | −26.78 | −20.51 | −17.25 |
| 4 | −16.94 | −10.74 | −8.15 | −7.56 | −5.65 | 2 | −40.38 | −21.48 | −18.00 | −12.33 | −8.85 |
| 6 | −12.89 | −9.68 | −7.38 | −6.74 | −4.68 | 3 | −29.74 | −15.95 | −12.44 | −8.67 | −6.01 |
| 8 | −11.46 | −9.48 | −7.06 | −5.98 | −4.46 | 4 | −23.81 | −12.22 | −9.41 | −6.60 | −4.42 |
| 10 | −11.30 | −9.47 | −6.91 | −5.81 | −4.22 | 5 | −19.35 | −10.45 | −8.11 | −5.35 | −3.41 |
| 12 | −11.24 | −9.31 | −6.74 | −5.75 | −4.21 | 6 | −16.62 | −8.99 | −6.05 | −4.67 | −2.69 |
| 14 | −10.93 | −8.83 | −6.67 | −5.62 | −4.15 | 7 | −14.57 | −8.20 | −5.84 | −4.43 | −2.24 |
| 16 | −10.47 | −8.66 | −6.35 | −5.48 | −4.01 | 8 | −12.89 | −7.78 | −5.71 | −4.06 | −1.99 |
| 18 | −9.78 | −7.81 | −5.75 | −5.34 | −3.93 | 9 | −11.80 | −7.79 | −5.28 | −3.62 | −1.73 |
| 20 | −9.56 | −7.03 | −5.47 | −4.98 | −3.64 | 10 | −10.92 | −7.21 | −5.09 | −3.27 | −1.64 |
| 22 | −8.32 | −6.99 | −5.30 | −4.70 | −3.28 | 11 | −10.34 | −6.79 | −4.89 | −2.94 | −1.35 |
| 24 | −8.17 | −6.74 | −5.12 | −4.45 | −3.09 | 12 | −9.59 | −6.17 | −4.28 | −2.93 | −1.23 |
| [Glycyl glycine] = 0.005 mol∙kg−1 | |||||||||||
| 2 | −19.88 | −15.88 | −14.09 | −12.89 | −10.11 | 1 | −56.48 | −30.80 | −24.11 | −21.55 | −19.14 |
| 4 | −16.13 | −10.01 | −7.97 | −7.17 | −5.36 | 2 | −30.82 | −19.22 | −14.50 | −11.54 | −9.40 |
| 6 | −12.94 | −9.18 | −7.19 | −6.37 | −4.20 | 3 | −24.01 | −13.16 | −9.83 | −7.51 | −5.68 |
| 8 | −11.22 | −9.10 | −6.76 | −5.64 | −4.17 | 4 | −19.82 | −10.38 | −7.37 | −5.85 | −3.86 |
| 10 | −11.21 | −9.26 | −6.63 | −5.47 | −4.11 | 5 | −16.75 | −9.23 | −6.26 | −4.08 | −3.16 |
| 12 | −10.93 | −9.24 | −6.60 | −5.38 | −4.06 | 6 | −14.83 | −8.46 | −5.34 | −3.35 | −2.50 |
| 14 | −10.53 | −8.72 | −6.42 | −5.28 | −3.92 | 7 | −13.52 | −7.75 | −4.34 | −3.00 | −2.08 |
| 16 | −10.30 | −8.43 | −6.39 | −5.06 | −3.85 | 8 | −12.28 | −6.99 | −4.23 | −2.70 | −2.13 |
| 18 | −9.66 | −7.61 | −5.50 | −4.92 | −3.75 | 9 | −11.40 | −6.32 | −3.77 | −2.39 | −1.80 |
| 20 | −9.50 | −6.97 | −5.25 | −4.81 | −3.67 | 10 | −10.78 | −5.85 | −3.35 | −2.31 | −1.50 |
| 22 | −8.11 | −6.69 | −5.20 | −4.57 | −3.61 | 11 | −9.96 | −5.46 | −3.01 | −1.86 | −1.19 |
| 24 | −8.05 | −6.58 | −5.00 | −4.33 | −3.52 | 12 | −9.24 | −5.20 | −2.71 | −1.40 | −0.93 |
| [Glycyl glycine] = 0.010 mol∙kg−1 | |||||||||||
| 2 | −18.21 | −14.51 | −13.43 | −11.58 | −9.84 | 1 | −49.70 | −32.35 | −24.46 | −21.99 | −18.92 |
| 4 | −15.63 | −9.94 | −7.67 | −6.99 | −5.21 | 2 | −27.50 | −15.93 | −12.81 | −10.50 | −9.07 |
| 6 | −12.92 | −9.09 | −7.05 | −6.13 | −5.18 | 3 | −20.15 | −11.56 | −9.38 | −7.18 | −5.33 |
| 8 | −11.13 | −8.90 | −6.51 | −5.39 | −4.11 | 4 | −15.23 | −9.39 | −7.04 | −5.36 | −3.67 |
| 10 | −11.04 | −8.68 | −6.37 | −5.25 | −4.06 | 5 | −12.61 | −8.33 | −6.11 | −3.89 | −3.00 |
| 12 | −10.25 | −8.50 | −6.22 | −4.91 | −3.97 | 6 | −10.97 | −7.01 | −5.19 | −3.23 | −2.28 |
| 14 | −10.19 | −8.26 | −6.04 | −5.12 | −3.73 | 7 | −9.53 | −6.26 | −4.20 | −2.66 | −1.76 |
| 16 | −9.50 | −8.17 | −6.02 | −4.95 | −3.62 | 8 | −8.34 | −5.79 | −4.12 | −2.49 | −1.33 |
| 18 | −9.23 | −7.59 | −5.28 | −4.88 | −3.34 | 9 | −7.36 | −5.06 | −3.48 | −2.29 | −0.87 |
| 20 | −9.13 | −6.62 | −5.12 | −4.66 | −3.30 | 10 | −6.87 | −4.72 | −3.29 | −1.86 | −0.57 |
| 22 | −7.96 | −6.55 | −5.10 | −4.45 | −3.12 | 11 | −6.24 | −4.38 | −2.87 | −1.56 | −0.46 |
| 24 | −7.84 | −6.39 | −4.71 | −3.98 | −3.03 | 12 | −6.06 | −4.17 | −2.59 | −1.17 | −0.23 |
| NaC | NaDC | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| [NaC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K | [NaDC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K |
| [Pure water] | |||||||||||
| 2 | 1.002 | 1.003 | 1.004 | 1.004 | 1.004 | 1 | 1.001 | 1.001 | 1.002 | 1.002 | 1.003 |
| 4 | 1.005 | 1.006 | 1.007 | 1.008 | 1.008 | 2 | 1.003 | 1.003 | 1.003 | 1.004 | 1.004 |
| 6 | 1.009 | 1.010 | 1.011 | 1.011 | 1.012 | 3 | 1.004 | 1.004 | 1.005 | 1.005 | 1.006 |
| 8 | 1.014 | 1.014 | 1.015 | 1.016 | 1.017 | 4 | 1.007 | 1.007 | 1.008 | 1.009 | 1.010 |
| 10 | 1.019 | 1.018 | 1.020 | 1.020 | 1.020 | 5 | 1.012 | 1.013 | 1.015 | 1.016 | 1.018 |
| 12 | 1.023 | 1.024 | 1.025 | 1.025 | 1.026 | 6 | 1.025 | 1.028 | 1.031 | 1.034 | 1.038 |
| 14 | 1.030 | 1.031 | 1.032 | 1.033 | 1.034 | 7 | 1.034 | 1.038 | 1.041 | 1.046 | 1.051 |
| 16 | 1.035 | 1.036 | 1.038 | 1.039 | 1.041 | 8 | 1.038 | 1.043 | 1.046 | 1.052 | 1.057 |
| 18 | 1.038 | 1.040 | 1.042 | 1.045 | 1.048 | 9 | 1.043 | 1.047 | 1.053 | 1.057 | 1.063 |
| 20 | 1.042 | 1.044 | 1.047 | 1.050 | 1.052 | 10 | 1.045 | 1.049 | 1.055 | 1.061 | 1.065 |
| 22 | 1.046 | 1.048 | 1.051 | 1.054 | 1.056 | 11 | 1.048 | 1.052 | 1.058 | 1.063 | 1.069 |
| 24 | 1.049 | 1.052 | 1.055 | 1.057 | 1.059 | 12 | 1.049 | 1.054 | 1.061 | 1.066 | 1.071 |
| [Glycyl glycine] = 0.001 mol∙kg−1 | |||||||||||
| 2 | 1.006 | 1.007 | 1.007 | 1.008 | 1.010 | 1 | 1.005 | 1.005 | 1.006 | 1.006 | 1.007 |
| 4 | 1.009 | 1.011 | 1.012 | 1.013 | 1.014 | 2 | 1.006 | 1.007 | 1.007 | 1.008 | 1.008 |
| 6 | 1.013 | 1.014 | 1.016 | 1.016 | 1.018 | 3 | 1.008 | 1.008 | 1.008 | 1.009 | 1.010 |
| 8 | 1.017 | 1.018 | 1.019 | 1.021 | 1.022 | 4 | 1.010 | 1.011 | 1.012 | 1.012 | 1.014 |
| 10 | 1.022 | 1.023 | 1.025 | 1.027 | 1.028 | 5 | 1.013 | 1.017 | 1.019 | 1.019 | 1.022 |
| 12 | 1.028 | 1.029 | 1.031 | 1.032 | 1.034 | 6 | 1.026 | 1.031 | 1.035 | 1.037 | 1.047 |
| 14 | 1.035 | 1.036 | 1.039 | 1.041 | 1.042 | 7 | 1.035 | 1.041 | 1.045 | 1.049 | 1.054 |
| 16 | 1.040 | 1.041 | 1.044 | 1.046 | 1.049 | 8 | 1.039 | 1.047 | 1.050 | 1.055 | 1.060 |
| 18 | 1.043 | 1.045 | 1.048 | 1.052 | 1.054 | 9 | 1.043 | 1.051 | 1.056 | 1.061 | 1.066 |
| 20 | 1.047 | 1.049 | 1.054 | 1.056 | 1.059 | 10 | 1.046 | 1.053 | 1.059 | 1.064 | 1.069 |
| 22 | 1.051 | 1.053 | 1.057 | 1.061 | 1.063 | 11 | 1.048 | 1.056 | 1.061 | 1.067 | 1.073 |
| 24 | 1.054 | 1.057 | 1.061 | 1.064 | 1.067 | 12 | 1.049 | 1.057 | 1.065 | 1.069 | 1.074 |
| [Glycyl glycine] = 0.005 mol∙kg−1 | |||||||||||
| 2 | 1.006 | 1.007 | 1.007 | 1.009 | 1.010 | 1 | 1.001 | 1.002 | 1.003 | 1.003 | 1.003 |
| 4 | 1.009 | 1.011 | 1.012 | 1.013 | 1.014 | 2 | 1.003 | 1.004 | 1.004 | 1.004 | 1.005 |
| 6 | 1.013 | 1.014 | 1.016 | 1.017 | 1.018 | 3 | 1.005 | 1.005 | 1.005 | 1.006 | 1.006 |
| 8 | 1.017 | 1.019 | 1.020 | 1.021 | 1.022 | 4 | 1.007 | 1.008 | 1.009 | 1.009 | 1.010 |
| 10 | 1.022 | 1.024 | 1.026 | 1.027 | 1.028 | 5 | 1.010 | 1.014 | 1.016 | 1.016 | 1.018 |
| 12 | 1.028 | 1.030 | 1.031 | 1.033 | 1.034 | 6 | 1.023 | 1.028 | 1.032 | 1.034 | 1.043 |
| 14 | 1.035 | 1.037 | 1.039 | 1.041 | 1.042 | 7 | 1.032 | 1.038 | 1.042 | 1.046 | 1.050 |
| 16 | 1.040 | 1.042 | 1.044 | 1.046 | 1.048 | 8 | 1.037 | 1.043 | 1.047 | 1.052 | 1.056 |
| 18 | 1.043 | 1.046 | 1.049 | 1.052 | 1.055 | 9 | 1.040 | 1.048 | 1.052 | 1.057 | 1.062 |
| 20 | 1.047 | 1.050 | 1.054 | 1.056 | 1.060 | 10 | 1.043 | 1.050 | 1.055 | 1.060 | 1.065 |
| 22 | 1.051 | 1.054 | 1.058 | 1.061 | 1.064 | 11 | 1.045 | 1.053 | 1.058 | 1.063 | 1.068 |
| 24 | 1.054 | 1.059 | 1.061 | 1.064 | 1.068 | 12 | 1.046 | 1.054 | 1.061 | 1.066 | 1.072 |
| [Glycyl glycine] = 0.010 mol∙kg−1 | |||||||||||
| 2 | 1.007 | 1.007 | 1.007 | 1.009 | 1.010 | 1 | 1.002 | 1.002 | 1.003 | 1.003 | 1.003 |
| 4 | 1.009 | 1.011 | 1.011 | 1.013 | 1.014 | 2 | 1.003 | 1.004 | 1.004 | 1.005 | 1.005 |
| 6 | 1.013 | 1.015 | 1.016 | 1.018 | 1.018 | 3 | 1.004 | 1.005 | 1.006 | 1.006 | 1.007 |
| 8 | 1.017 | 1.020 | 1.019 | 1.022 | 1.022 | 4 | 1.007 | 1.008 | 1.009 | 1.010 | 1.011 |
| 10 | 1.022 | 1.025 | 1.025 | 1.028 | 1.028 | 5 | 1.010 | 1.014 | 1.016 | 1.016 | 1.018 |
| 12 | 1.028 | 1.030 | 1.031 | 1.033 | 1.034 | 6 | 1.020 | 1.028 | 1.032 | 1.034 | 1.043 |
| 14 | 1.035 | 1.037 | 1.039 | 1.042 | 1.042 | 7 | 1.032 | 1.039 | 1.042 | 1.046 | 1.050 |
| 16 | 1.040 | 1.042 | 1.044 | 1.046 | 1.049 | 8 | 1.037 | 1.043 | 1.047 | 1.052 | 1.056 |
| 18 | 1.043 | 1.046 | 1.048 | 1.052 | 1.055 | 9 | 1.040 | 1.047 | 1.052 | 1.057 | 1.062 |
| 20 | 1.047 | 1.050 | 1.053 | 1.056 | 1.061 | 10 | 1.042 | 1.050 | 1.055 | 1.060 | 1.065 |
| 22 | 1.051 | 1.054 | 1.057 | 1.061 | 1.065 | 11 | 1.045 | 1.053 | 1.058 | 1.063 | 1.068 |
| 24 | 1.054 | 1.059 | 1.061 | 1.065 | 1.069 | 12 | 1.046 | 1.054 | 1.061 | 1.065 | 1.072 |
| NaC | NaDC | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| [NaC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K | [NaDC] mmol∙kg−1 | 293.15 K | 298.15 K | 303.15 K | 308.15 K | 313.15 K |
| [Pure Water] | |||||||||||
| 2 | 0.609 | 0.532 | 0.470 | 0.419 | 0.376 | 1 | 0.609 | 0.532 | 0.469 | 0.418 | 0.376 |
| 4 | 0.610 | 0.534 | 0.471 | 0.420 | 0.378 | 2 | 0.609 | 0.532 | 0.470 | 0.419 | 0.376 |
| 6 | 0.612 | 0.535 | 0.472 | 0.421 | 0.379 | 3 | 0.609 | 0.532 | 0.471 | 0.419 | 0.377 |
| 8 | 0.615 | 0.537 | 0.474 | 0.423 | 0.380 | 4 | 0.611 | 0.534 | 0.472 | 0.421 | 0.378 |
| 10 | 0.617 | 0.538 | 0.476 | 0.424 | 0.381 | 5 | 0.614 | 0.537 | 0.475 | 0.423 | 0.381 |
| 12 | 0.618 | 0.541 | 0.478 | 0.426 | 0.383 | 6 | 0.622 | 0.545 | 0.482 | 0.431 | 0.389 |
| 14 | 0.622 | 0.544 | 0.481 | 0.428 | 0.386 | 7 | 0.627 | 0.550 | 0.487 | 0.436 | 0.393 |
| 16 | 0.625 | 0.546 | 0.483 | 0.431 | 0.388 | 8 | 0.629 | 0.552 | 0.489 | 0.438 | 0.395 |
| 18 | 0.626 | 0.548 | 0.485 | 0.433 | 0.390 | 9 | 0.632 | 0.554 | 0.492 | 0.440 | 0.397 |
| 20 | 0.628 | 0.550 | 0.487 | 0.434 | 0.391 | 10 | 0.633 | 0.555 | 0.493 | 0.441 | 0.398 |
| 22 | 0.630 | 0.551 | 0.488 | 0.436 | 0.393 | 11 | 0.634 | 0.556 | 0.494 | 0.442 | 0.400 |
| 24 | 0.631 | 0.553 | 0.490 | 0.437 | 0.394 | 12 | 0.635 | 0.557 | 0.495 | 0.443 | 0.400 |
| [Glycyl glycine] = 0.001 mol∙kg−1 | |||||||||||
| 2 | 0.614 | 0.535 | 0.473 | 0.422 | 0.379 | 1 | 0.613 | 0.534 | 0.472 | 0.421 | 0.378 |
| 4 | 0.615 | 0.536 | 0.474 | 0.423 | 0.381 | 2 | 0.614 | 0.535 | 0.473 | 0.422 | 0.379 |
| 6 | 0.617 | 0.538 | 0.476 | 0.425 | 0.382 | 3 | 0.614 | 0.535 | 0.473 | 0.422 | 0.379 |
| 8 | 0.618 | 0.539 | 0.477 | 0.426 | 0.383 | 4 | 0.615 | 0.536 | 0.475 | 0.423 | 0.381 |
| 10 | 0.621 | 0.541 | 0.479 | 0.428 | 0.385 | 5 | 0.617 | 0.539 | 0.477 | 0.426 | 0.384 |
| 12 | 0.624 | 0.544 | 0.482 | 0.430 | 0.387 | 6 | 0.624 | 0.547 | 0.485 | 0.433 | 0.393 |
| 14 | 0.627 | 0.547 | 0.485 | 0.433 | 0.390 | 7 | 0.630 | 0.552 | 0.489 | 0.438 | 0.396 |
| 16 | 0.630 | 0.549 | 0.487 | 0.435 | 0.392 | 8 | 0.631 | 0.554 | 0.492 | 0.441 | 0.398 |
| 18 | 0.631 | 0.551 | 0.489 | 0.437 | 0.394 | 9 | 0.634 | 0.557 | 0.494 | 0.443 | 0.400 |
| 20 | 0.633 | 0.553 | 0.491 | 0.439 | 0.395 | 10 | 0.635 | 0.557 | 0.495 | 0.444 | 0.401 |
| 22 | 0.635 | 0.554 | 0.492 | 0.440 | 0.396 | 11 | 0.636 | 0.559 | 0.496 | 0.445 | 0.402 |
| 24 | 0.636 | 0.556 | 0.493 | 0.441 | 0.398 | 12 | 0.637 | 0.559 | 0.498 | 0.446 | 0.402 |
| [Glycyl glycine] = 0.005 mol∙kg−1 | |||||||||||
| 2 | 0.616 | 0.535 | 0.474 | 0.424 | 0.380 | 1 | 0.613 | 0.533 | 0.471 | 0.421 | 0.378 |
| 4 | 0.617 | 0.537 | 0.475 | 0.425 | 0.382 | 2 | 0.614 | 0.534 | 0.472 | 0.422 | 0.378 |
| 6 | 0.619 | 0.538 | 0.477 | 0.426 | 0.383 | 3 | 0.614 | 0.534 | 0.472 | 0.422 | 0.379 |
| 8 | 0.621 | 0.540 | 0.478 | 0.428 | 0.384 | 4 | 0.615 | 0.535 | 0.474 | 0.423 | 0.380 |
| 10 | 0.623 | 0.542 | 0.480 | 0.430 | 0.386 | 5 | 0.617 | 0.538 | 0.477 | 0.426 | 0.383 |
| 12 | 0.626 | 0.545 | 0.483 | 0.432 | 0.388 | 6 | 0.625 | 0.546 | 0.484 | 0.433 | 0.393 |
| 14 | 0.630 | 0.548 | 0.486 | 0.435 | 0.391 | 7 | 0.630 | 0.551 | 0.489 | 0.438 | 0.395 |
| 16 | 0.632 | 0.550 | 0.488 | 0.437 | 0.393 | 8 | 0.632 | 0.553 | 0.491 | 0.441 | 0.397 |
| 18 | 0.634 | 0.552 | 0.490 | 0.439 | 0.395 | 9 | 0.634 | 0.555 | 0.493 | 0.443 | 0.399 |
| 20 | 0.635 | 0.554 | 0.492 | 0.440 | 0.397 | 10 | 0.636 | 0.556 | 0.495 | 0.444 | 0.400 |
| 22 | 0.637 | 0.555 | 0.493 | 0.442 | 0.398 | 11 | 0.637 | 0.558 | 0.496 | 0.445 | 0.401 |
| 24 | 0.639 | 0.557 | 0.494 | 0.443 | 0.399 | 12 | 0.637 | 0.558 | 0.497 | 0.446 | 0.403 |
| [Glycyl glycine] = 0.010 mol∙kg−1 | |||||||||||
| 2 | 0.618 | 0.536 | 0.475 | 0.425 | 0.381 | 1 | 0.615 | 0.534 | 0.473 | 0.423 | 0.379 |
| 4 | 0.619 | 0.538 | 0.476 | 0.427 | 0.383 | 2 | 0.615 | 0.534 | 0.473 | 0.423 | 0.380 |
| 6 | 0.621 | 0.539 | 0.478 | 0.428 | 0.384 | 3 | 0.616 | 0.535 | 0.474 | 0.424 | 0.380 |
| 8 | 0.622 | 0.541 | 0.479 | 0.429 | 0.385 | 4 | 0.617 | 0.536 | 0.475 | 0.425 | 0.382 |
| 10 | 0.625 | 0.544 | 0.481 | 0.432 | 0.387 | 5 | 0.619 | 0.539 | 0.478 | 0.428 | 0.384 |
| 12 | 0.628 | 0.546 | 0.484 | 0.434 | 0.389 | 6 | 0.625 | 0.547 | 0.486 | 0.435 | 0.394 |
| 14 | 0.631 | 0.549 | 0.487 | 0.437 | 0.392 | 7 | 0.632 | 0.552 | 0.490 | 0.440 | 0.396 |
| 16 | 0.634 | 0.551 | 0.489 | 0.438 | 0.394 | 8 | 0.635 | 0.554 | 0.492 | 0.442 | 0.398 |
| 18 | 0.635 | 0.553 | 0.491 | 0.440 | 0.396 | 9 | 0.637 | 0.556 | 0.495 | 0.444 | 0.400 |
| 20 | 0.637 | 0.555 | 0.493 | 0.442 | 0.398 | 10 | 0.638 | 0.557 | 0.496 | 0.445 | 0.401 |
| 22 | 0.639 | 0.556 | 0.494 | 0.443 | 0.399 | 11 | 0.639 | 0.558 | 0.497 | 0.446 | 0.402 |
| 24 | 0.640 | 0.558 | 0.495 | 0.445 | 0.400 | 12 | 0.640 | 0.559 | 0.498 | 0.447 | 0.404 |
| Chemical Name | Source | CAS No. | Mol.Wt./kg∙mol−1 | Purification Method | Mass Fraction Purity a |
|---|---|---|---|---|---|
| Glycyl glycine | Spectrochem Pvt. Ltd. | 556–50–3 | 0.132 | None | 0.98 |
| Sodium cholate | Himedia Pvt. Ltd. | 361–09–1 | 0.431 | Recrystallization | 0.98 |
| Sodium deoxycholate | Himedia Pvt. Ltd. | 302–95–4 | 0.415 | Recrystallization | 0.98 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kumari, S.; Chauhan, S.; Singh, K.; Umar, A.; Fouad, H.; Alissawi, M.S.; Akhtar, M.S. Volumetric, Compressibility and Viscometric Approach to Study the Interactional Behaviour of Sodium Cholate and Sodium Deoxycholate in Aqueous Glycyl Glycine. Molecules 2022, 27, 8998. https://doi.org/10.3390/molecules27248998
Kumari S, Chauhan S, Singh K, Umar A, Fouad H, Alissawi MS, Akhtar MS. Volumetric, Compressibility and Viscometric Approach to Study the Interactional Behaviour of Sodium Cholate and Sodium Deoxycholate in Aqueous Glycyl Glycine. Molecules. 2022; 27(24):8998. https://doi.org/10.3390/molecules27248998
Chicago/Turabian StyleKumari, Santosh, Suvarcha Chauhan, Kuldeep Singh, Ahmad Umar, Hassan Fouad, Mohammed S. Alissawi, and Mohammad Shaheer Akhtar. 2022. "Volumetric, Compressibility and Viscometric Approach to Study the Interactional Behaviour of Sodium Cholate and Sodium Deoxycholate in Aqueous Glycyl Glycine" Molecules 27, no. 24: 8998. https://doi.org/10.3390/molecules27248998
APA StyleKumari, S., Chauhan, S., Singh, K., Umar, A., Fouad, H., Alissawi, M. S., & Akhtar, M. S. (2022). Volumetric, Compressibility and Viscometric Approach to Study the Interactional Behaviour of Sodium Cholate and Sodium Deoxycholate in Aqueous Glycyl Glycine. Molecules, 27(24), 8998. https://doi.org/10.3390/molecules27248998







