The Atomic Structure and Mechanical Properties of ZIF-4 under High Pressure: Ab Initio Calculations
Abstract
:1. Introduction
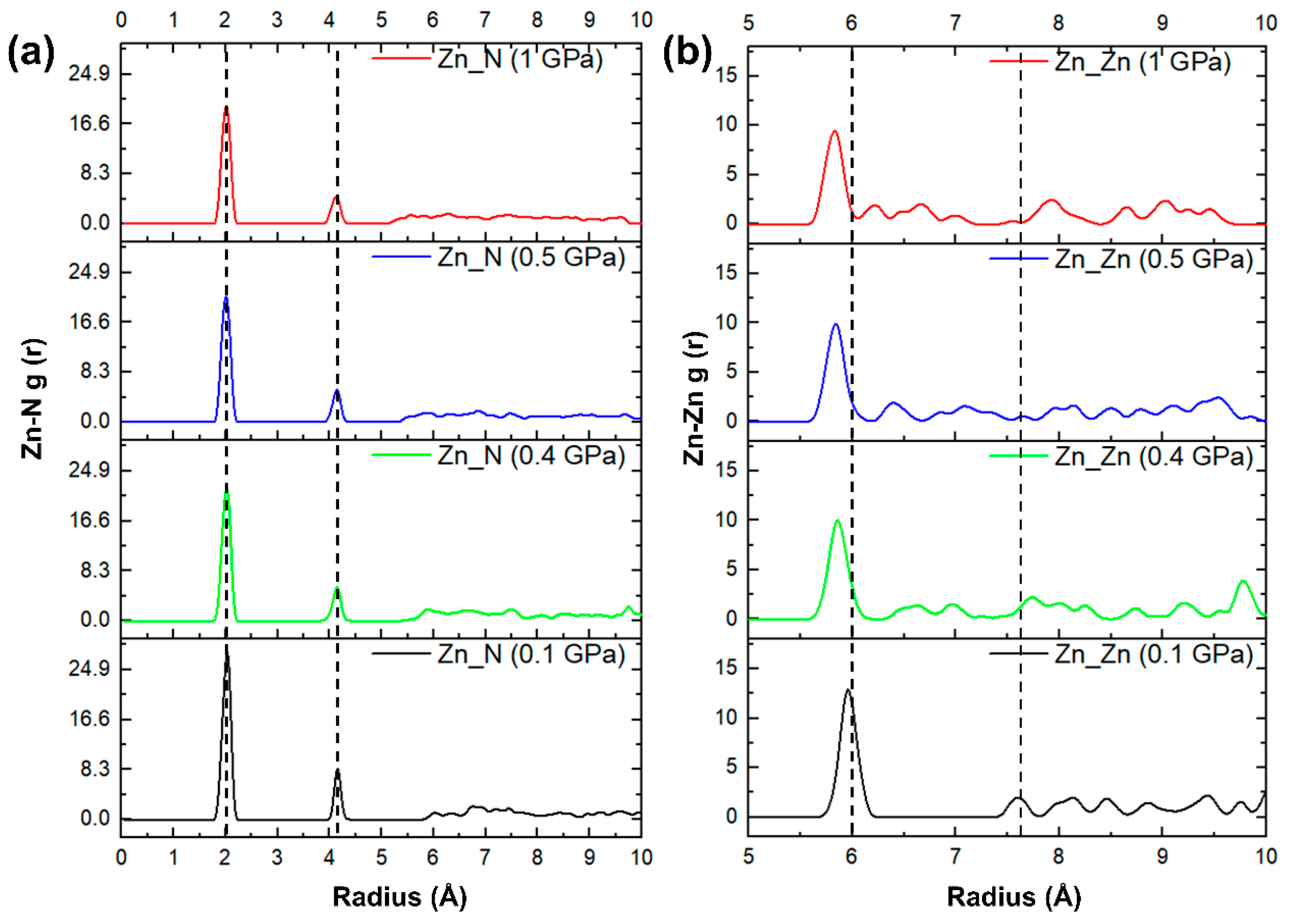
2. Results
3. Discussion
4. Materials and Methods
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Rao, C.N.R.; Cheetham, A.K.; Thirumurugan, A. Hybrid inorganic–organic materials: A new family in condensed matter physics. J. Phys. Condens. Matter 2008, 20, 083202. [Google Scholar] [CrossRef]
- Férey, G. Some suggested perspectives for multifunctional hybrid porous solids. Dalton Trans. 2009, 4400–4415. [Google Scholar] [CrossRef] [PubMed]
- Furukawa, H.; Cordova, K.E.; O’Keeffe, M.; Yaghi, O.M. The chemistry and applications of metal-organic frameworks. Science 2013, 341, 1230444. [Google Scholar] [CrossRef] [Green Version]
- Mondloch, J.E.; Katz, M.J.; Isley, W.C.; Ghosh, P.; Liao, P.; Bury, W.; Wagner, G.W.; Hall, M.G.; DeCoste, J.B.; Peterson, G.W.; et al. Destruction of chemical warfare agents using metal-organic frameworks. Nat. Mater. 2015, 14, 512–516. [Google Scholar] [CrossRef]
- Chen, M.-L.; Zhou, S.-Y.; Xu, Z.; Ding, L.; Cheng, Y.-H. Metal-Organic Frameworks of MIL-100(Fe, Cr) and MIL-101(Cr) for Aromatic Amines Adsorption from Aqueous Solutions. Molecules 2019, 24, 3718. [Google Scholar] [CrossRef] [Green Version]
- Li, R.; Ren, X.; Feng, X.; Li, X.; Hu, C.; Wang, B. A highly stable metal-and nitrogen-doped nanocomposite derived from Zn/Ni-ZIF-8 capable of CO 2 capture and separation. Chem. Commun. 2014, 50, 6894–6897. [Google Scholar] [CrossRef]
- Hasan, M.R.; Paseta, L.; Malankowska, M.; Téllez, C.; Coronas, J. Synthesis of ZIF-94 from Recycled Mother Liquors: Study of the Influence of Its Loading on Postcombustion CO2 Capture with Pebax Based Mixed Matrix Membranes. Adv. Sustain. Syst. 2022, 6, 2100317. [Google Scholar] [CrossRef]
- Liu, S.; Chen, F.; Li, S.; Peng, X.; Xiong, Y. Enhanced photocatalytic conversion of greenhouse gas CO2 into solar fuels over g-C3N4 nanotubes with decorated transparent ZIF-8 nanoclusters. Appl. Catal. B Environ. 2017, 211, 1–10. [Google Scholar] [CrossRef]
- Bensiradj, N.E.H.; Timón, V.; Boussessi, R.; Dalbouha, S.; Senent, M.L. DFT studies of single and multiple molecular adsorption of CH4, SF6 and H2O in Zeolitic-Imidazolate Framework (ZIF-4 and ZIF-6). Inorg. Chim. Acta 2019, 490, 272–281. [Google Scholar] [CrossRef]
- Phan, A.; Doonan, C.J.; Uribe-Romo, F.J.; Knobler, C.B.; O’Keeffe, M.; Yaghi, O.M. Synthesis, structure, and carbon dioxide capture properties of zeolitic imidazolate frameworks. Acc. Chem. Res. 2010, 43, 58–67. [Google Scholar] [CrossRef]
- Bumstead, A.M.; Gomez, M.L.R.; Thorne, M.F.; Sapnik, A.F.; Longley, L.; Tuffnell, J.M.; Keeble, D.S.; Keen, D.A.; Bennett, T.D. Investigating the melting behaviour of polymorphic zeolitic imidazolate frameworks. Crystengcomm 2020, 22, 3627–3637. [Google Scholar] [CrossRef]
- Gaillac, R.; Pullumbi, P.; Coudert, F.X. Melting of Zeolitic Imidazolate Frameworks with Different Topologies: Insight from First-Principles Molecular Dynamics. J. Phys. Chem. C 2018, 122, 6730–6736. [Google Scholar] [CrossRef] [Green Version]
- Longley, L.; Collins, S.M.; Li, S.C.; Smales, G.J.; Erucar, I.; Qiao, A.; Hou, J.; Doherty, C.M.; Thornton, A.W.; Hill, A.J.; et al. Flux melting of metal-organic frameworks. Chem. Sci. 2019, 10, 3592–3601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nozari, V.; Calahoo, C.; Tuffnell, J.M.; Keen, D.A.; Bennett, T.D.; Wondraczek, L. Ionic liquid facilitated melting of the metal-organic framework ZIF-8. Nat. Commun. 2021, 12, 13. [Google Scholar] [CrossRef] [PubMed]
- Bennett, T.D.; Simoncic, P.; Moggach, S.A.; Gozzo, F.; Macchi, P.; Keen, D.A.; Tan, J.-C.; Cheetham, A.K. Reversible pressure-induced amorphization of a zeolitic imidazolate framework (ZIF-4). Chem. Commun. 2011, 47, 7983–7985. [Google Scholar] [CrossRef] [PubMed]
- Celeste, A.; Paolone, A.; Itie, J.P.; Borondics, F.; Joseph, B.; Grad, O.; Blanita, G.; Zlotea, C.; Capitani, F. Mesoporous Metal-Organic Framework MIL-101 at High Pressure. J. Am. Chem. Soc. 2020, 142, 15012–15019. [Google Scholar] [CrossRef]
- Chapman, K.W.; Halder, G.J.; Chupas, P.J. Pressure-induced amorphization and porosity modification in a metal- organic framework. J. Am. Chem. Soc. 2009, 131, 17546–17547. [Google Scholar] [CrossRef]
- Bennett, T.D.; Cheetham, A.K. Amorphous Metal-Organic Frameworks. Acc. Chem. Res. 2014, 47, 1555–1562. [Google Scholar] [CrossRef]
- Cao, S.; Bennett, T.D.; Keen, D.A.; Goodwin, A.L.; Cheetham, A.K. Amorphization of the prototypical zeolitic imidazolate framework ZIF-8 by ball-milling. Chem. Commun. 2012, 48, 7805–7807. [Google Scholar] [CrossRef]
- Panda, T.; Horike, S.; Hagi, K.; Ogiwara, N.; Kadota, K.; Itakura, T.; Tsujimoto, M.; Kitagawa, S. Mechanical Alloying of Metal-Organic Frameworks. Angew. Chem. Int. Edit. 2017, 56, 2413–2417. [Google Scholar] [CrossRef]
- Knebel, A.; Geppert, B.; Volgmann, K.; Kolokolov, D.I.; Stepanov, A.G.; Twiefel, J.; Heitjans, P.; Volkmer, D.; Caro, J. Defibrillation of soft porous metal-organic frameworks with electric fields. Science 2017, 358, 347–351. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mottillo, C.; Friščić, T. Advances in Solid-State Transformations of Coordination Bonds: From the Ball Mill to the Aging Chamber. Molecules 2017, 22, 144. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Qiao, A.; To, T.; Stepniewska, M.; Tao, H.Z.; Calvez, L.; Zhang, X.H.; Smedskjaer, M.M.; Yue, Y.Z. Deformation mechanism of a metal-organic framework glass under indentation. Phys. Chem. Chem. Phys. 2021, 23, 16923–16931. [Google Scholar] [CrossRef] [PubMed]
- Madsen, R.S.K.; Qiao, A.; Sen, J.N.; Hung, I.; Chen, K.Z.; Gan, Z.H.; Sen, S.; Yue, Y.Z. Ultrahigh-field Zn-67 NMR reveals short-range disorder in zeolitic imidazolate framework glasses. Science 2020, 367, 1473–1476. [Google Scholar] [CrossRef]
- Wharmby, M.T.; Henke, S.; Bennett, T.D.; Bajpe, S.R.; Schwedler, I.; Thompson, S.P.; Gozzo, F.; Simoncic, P.; Mellot-Draznieks, C.; Tao, H.; et al. Extreme Flexibility in a Zeolitic Imidazolate Framework: Porous to Dense Phase Transition in Desolvated ZIF-4. Angew. Chem. Int. Ed. 2015, 54, 6447–6451. [Google Scholar] [CrossRef]
- Hartmann, M.; Böhme, U.; Hovestadt, M.; Paula, C. Adsorptive Separation of Olefin/Paraffin Mixtures with ZIF-4. Langmuir 2015, 31, 12382–12389. [Google Scholar] [CrossRef]
- Hwang, S.; Gopalan, A.; Hovestadt, M.; Piepenbreier, F.; Chmelik, C.; Hartmann, M.; Snurr, R.Q.; Kärger, J. Anomaly in the Chain Length Dependence of n-Alkane Diffusion in ZIF-4 Metal-Organic Frameworks. Molecules 2018, 23, 668. [Google Scholar] [CrossRef] [Green Version]
- Widmer, R.N.; Lampronti, G.I.; Chibani, S.; Wilson, C.W.; Anzellini, S.; Farsang, S.; Kleppe, A.K.; Casati, N.P.M.; MacLeod, S.G.; Redfern, S.A.T.; et al. Rich Polymorphism of a Metal–Organic Framework in Pressure–Temperature Space. J. Am. Chem. Soc. 2019, 141, 9330–9337. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.Y.; Qiao, A.; Tao, H.Z.; Yue, Y.Z. Synthesis, phase transitions and vitrification of the zeolitic imidazolate framework: ZIF-4. J. Non-Cryst. Solids 2019, 525, 5. [Google Scholar] [CrossRef]
- Tan, J.-C.; Civalleri, B.; Erba, A.; Albanese, E. Quantum mechanical predictions to elucidate the anisotropic elastic properties of zeolitic imidazolate frameworks: ZIF-4 vs. ZIF-zni. Crystengcomm 2015, 17, 375–382. [Google Scholar] [CrossRef]
- Henke, S.; Wharmby, M.T.; Kieslich, G.; Hante, I.; Schneemann, A.; Wu, Y.; Daisenberger, D.; Cheetham, A.K. Pore closure in zeolitic imidazolate frameworks under mechanical pressure. Chem. Sci. 2018, 9, 1654–1660. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vervoorts, P.; Hobday, C.L.; Ehrenreich, M.G.; Daisenberger, D.; Kieslich, G. The Zeolitic Imidazolate Framework ZIF-4 under Low Hydrostatic Pressures. Z. für Anorg. und Allg. Chemie 2019, 645, 970–974. [Google Scholar] [CrossRef]
- Hasmy, A.; Ispas, S.; Hehlen, B. Percolation transitions in compressed SiO2 glasses. Nature 2021, 599, 62–66. [Google Scholar] [CrossRef] [PubMed]
- Habasaki, J.; Ngai, K.L. Rigidity and soft percolation in the glass transition of an atomistic model of ionic liquid, 1-ethyl-3-methyl imidazolium nitrate, from molecular dynamics simulations—Existence of infinite overlapping networks in a fragile ionic liquid. J. Chem. Phys. 2015, 142, 1–4643. [Google Scholar] [CrossRef] [PubMed]
- Li, N.; Blankson, K.; Yang, Y.; Zhang, P.; Zhao, X. Unraveling the electronic structure, mechanical, and dielectric properties of ZnPurBr-MOF: Ab initio calculations. APL Mater. 2020, 8, 111101. [Google Scholar] [CrossRef]
- Shi, Z.; Arramel, A.; Bennett, T.D.; Yue, Y.; Li, N. The deformation of short-range order leading to rearrangement of topological network structure in zeolitic imidazolate framework glasses. Science 2022, 25, 104351. [Google Scholar] [CrossRef]
- Xiong, M.; Li, N.; Yin, G.; Ching, W.-Y.; Zhao, X. Effects of the halogenated imidazolate linker on the fundamental properties of amorphous zeolitic imidazolate frameworks. J. Non-Cryst. Solids 2020, 536, 120005. [Google Scholar] [CrossRef]
- Xiong, M.; Zhao, X.; Yin, G.; Ching, W.-Y.; Li, N. Unraveling the effects of linker substitution on structural, electronic and optical properties of amorphous zeolitic imidazolate frameworks-62 (a-ZIF-62) glasses: A DFT study. RSC Adv. 2020, 10, 14013–14024. [Google Scholar] [CrossRef] [Green Version]
- Timón, V.; Senent, M.L.; Hochlaf, M. Structural single and multiple molecular adsorption of CO2 and H2O in zeolitic imidazolate framework (ZIF) crystals. Microporous Mesoporous Mat. 2015, 218, 33–41. [Google Scholar] [CrossRef]
- Haas, P.; Tran, F.; Blaha, P. Calculation of the lattice constant of solids with semilocal functionals. Phys. Rev. B 2009, 79, 085104. [Google Scholar] [CrossRef]
- Tran, F.; Laskowski, R.; Blaha, P.; Schwarz, K. Performance on molecules, surfaces, and solids of the Wu-Cohen GGA exchange-correlation energy functional. Phys. Rev. B 2007, 75, 115131. [Google Scholar] [CrossRef] [Green Version]
- Colmenero, F.; Timón, V. ZIF-75 under Pressure: Negative Linear Compressibility and Pressure-Induced Instability. Appl. Sci. 2022, 12, 10413. [Google Scholar] [CrossRef]
- Li, W.; Probert, M.R.; Kosa, M.; Bennett, T.D.; Thirumurugan, A.; Burwood, R.P.; Parinello, M.; Howard, J.A.K.; Cheetham, A.K. Negative Linear Compressibility of a Metal–Organic Framework. J. Am. Chem. Soc. 2012, 134, 11940–11943. [Google Scholar] [CrossRef] [PubMed]
- Mouhat, F.; Coudert, F.-X. Necessary and sufficient elastic stability conditions in various crystal systems. Phys. Rev. B 2014, 90, 224104. [Google Scholar] [CrossRef] [Green Version]
- Tan, J.-C.; Civalleri, B.; Lin, C.-C.; Valenzano, L.; Galvelis, R.; Chen, P.-F.; Bennett, T.D.; Mellot-Draznieks, C.; Zicovich-Wilson, C.M.; Cheetham, A.K. Exceptionally low shear modulus in a prototypical imidazole-based metal-organic framework. Phys. Rev. Lett. 2012, 108, 095502. [Google Scholar] [CrossRef] [Green Version]
- Ortiz, A.U.; Boutin, A.; Fuchs, A.H.; Coudert, F.-X. Investigating the pressure-induced amorphization of zeolitic imidazolate framework ZIF-8: Mechanical instability due to shear mode softening. J. Phys. Chem. Lett. 2013, 4, 1861–1865. [Google Scholar] [CrossRef] [Green Version]
- Stepniewska, M.; Januchta, K.; Zhou, C.; Qiao, A.; Smedskjaer, M.M.; Yue, Y.Z. Observation of indentation-induced shear bands in a metal-organic framework glass. Proc. Natl. Acad. Sci. USA 2020, 117, 10149–10154. [Google Scholar] [CrossRef]
- Pugh, S.F. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1954, 45, 823–843. [Google Scholar] [CrossRef]
- Pettifor, D.G. Theoretical predictions of structure and related properties of intermetallics. Mater. Sci. Technol. 1992, 8, 345–349. [Google Scholar] [CrossRef]
- Greaves, G.N.; Greer, A.L.; Lakes, R.S.; Rouxel, T. Poisson’s ratio and modern materials. Nat. Mater. 2011, 10, 823–837. [Google Scholar] [CrossRef]
- Li, N.; Sakidja, R.; Aryal, S.; Ching, W.-Y. Densification of a continuous random network model of amorphous SiO2 glass. Phys. Chem. Chem. Phys. 2014, 16, 1500–1514. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Zhang, H.; Yuan, N.; Meng, L.; Geng, C.; Bai, H. Insights into reactive behaviors and mechanisms of nickel-based oxygen carriers doped by Fe/Co during chemical looping combustion from multiple-scale molecular modeling combined with experiments. Fuel Process. Technol. 2022, 229, 107181. [Google Scholar] [CrossRef]
- Kočí, P.; Novák, V.; Štěpánek, F.; Marek, M.; Kubíček, M.J.C.E.S. Multi-scale modelling of reaction and transport in porous catalysts. Chem. Eng. Sci. 2010, 65, 412–419. [Google Scholar] [CrossRef]
- Kennard, O.; Allen, F. 3D search and research using the Cambridge Structural Database. Chem. Des. Autom. 1993, 8, 31–37. [Google Scholar]
- Tian, Y.-Q.; Zhao, Y.-M.; Chen, Z.-X.; Zhang, G.-N.; Weng, L.-H.; Zhao, D.-Y. Design and Generation of Extended Zeolitic Metal–Organic Frameworks (ZMOFs): Synthesis and Crystal Structures of Zinc(II) Imidazolate Polymers with Zeolitic Topologies. Chem. A Eur. J. 2007, 13, 4146–4154. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B Condens Matter 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [Green Version]
- Le Page, Y.; Saxe, P. Symmetry-general least-squares extraction of elastic data for strained materials from ab initio calculations of stress. Phys. Rev. B 2002, 65, 104104. [Google Scholar] [CrossRef]
- Wu, X.; Vanderbilt, D.; Hamann, D.R. Systematic treatment of displacements, strains, and electric fields in density-functional perturbation theory. Phys. Rev. B 2005, 72, 035105. [Google Scholar] [CrossRef] [Green Version]
- Hill, R. The Elastic Behaviour of a Crystalline Aggregate. Proc. Phys. Soc. Sect. A 1952, 65, 349–354. [Google Scholar] [CrossRef]
- Wu, Z.-j.; Zhao, E.-j.; Xiang, H.-p.; Hao, X.-f.; Liu, X.-j.; Meng, J. Crystal structures and elastic properties of superhard IrN2 and IrN3 from first principles. Phys. Rev. B 2007, 76, 054115. [Google Scholar] [CrossRef]
- Ravindran, P.; Fast, L.; Korzhavyi, P.A.; Johansson, B.; Wills, J.; Eriksson, O. Density functional theory for calculation of elastic properties of orthorhombic crystals: Application to TiSi2. J. Appl. Phys. 1998, 84, 4891–4904. [Google Scholar] [CrossRef]
- Ranganathan, S.I.; Ostoja-Starzewski, M. Universal Elastic Anisotropy Index. Phys. Rev. Lett. 2008, 101, 055504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xing, M.-J.; Li, X.-Z.; Yu, S.-J.; Wang, F.-Y. Mechanical Anisotropic and Electronic Properties of Amm2-carbon under Pressure. Commun. Theor. Phys. 2017, 68, 395. [Google Scholar] [CrossRef]
- Anderson, O.L. A simplified method for calculating the debye temperature from elastic constants. J. Phys. Chem. Solids 1963, 24, 909–917. [Google Scholar] [CrossRef]



| Pressure (GPa) | a (Ǻ) | b (Ǻ) | c (Ǻ) | V (Ǻ3) |
|---|---|---|---|---|
| 0 | 15.350 | 15.811 | 18.519 | 4494.540 |
| Ref [15] | 15.403 | 15.459 | 18.408 | 4383.220 |
| Ref [39] | 15.370 | 15.080 | 18.610 | 4313.418 |
| 0.1 | 15.570 | 15.789 | 18.161 | 4464.605 |
| 0.2 | 15.546 | 15.539 | 17.970 | 4341.000 |
| 0.3 | 15.327 | 15.312 | 18.000 | 4224.366 |
| 0.4 | 15.958 | 15.544 | 14.631 | 3629.236 |
| 0.5 | 16.198 | 14.166 | 15.322 | 3515.799 |
| 0.6 | 16.160 | 14.042 | 15.253 | 3461.191 |
| 0.7 | 16.165 | 13.903 | 15.153 | 3405.515 |
| 0.8 | 16.194 | 13.805 | 15.025 | 3358.962 |
| 0.9 | 16.198 | 13.661 | 14.993 | 3317.664 |
| 1 | 16.215 | 13.582 | 14.888 | 3278.816 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Z.; Weng, K.; Li, N. The Atomic Structure and Mechanical Properties of ZIF-4 under High Pressure: Ab Initio Calculations. Molecules 2023, 28, 22. https://doi.org/10.3390/molecules28010022
Shi Z, Weng K, Li N. The Atomic Structure and Mechanical Properties of ZIF-4 under High Pressure: Ab Initio Calculations. Molecules. 2023; 28(1):22. https://doi.org/10.3390/molecules28010022
Chicago/Turabian StyleShi, Zuhao, Kaiyi Weng, and Neng Li. 2023. "The Atomic Structure and Mechanical Properties of ZIF-4 under High Pressure: Ab Initio Calculations" Molecules 28, no. 1: 22. https://doi.org/10.3390/molecules28010022
APA StyleShi, Z., Weng, K., & Li, N. (2023). The Atomic Structure and Mechanical Properties of ZIF-4 under High Pressure: Ab Initio Calculations. Molecules, 28(1), 22. https://doi.org/10.3390/molecules28010022






