Next Article in Journal
Impact of Pomegranate Juice on the Pharmacokinetics of CYP3A4- and CYP2C9-Mediated Drugs Metabolism: A Preclinical and Clinical Review
Next Article in Special Issue
Unlocking the Conformational Changes of P2Y12: Exploring an Acridinone Compound’s Effect on Receptor Activity and Conformation
Previous Article in Journal
Experimental Determination of a Chiral Ternary Solubility Diagram and Its Interpretation in Gas Antisolvent Fractionation
Previous Article in Special Issue
In Silico Computational Studies of Bioactive Secondary Metabolites from Wedelia trilobata against Anti-Apoptotic B-Cell Lymphoma-2 (Bcl-2) Protein Associated with Cancer Cell Survival and Resistance
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 28
Issue 5
10.3390/molecules28052116
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Quantum Computational, Spectroscopic (FT-IR, FT-Raman, NMR, and UV–Vis) Hirshfeld Surface and Molecular Docking-Dynamics Studies on 5-Hydroxymethyluracil (Monomer and Trimer)

by
Mohit Kumar
1,
Gautam Jaiswar
1,*,
Mohd. Afzal
2,*,
Mohd. Muddassir
2,
Abdullah Alarifi
2,
Aysha Fatima
3,
Nazia Siddiqui
4,*,
Rashid Ayub
5,
Naaser A. Y. Abduh
2,
Waseem Sharaf Saeed
6 and
Saleem Javed
7,*
1
Department of Chemistry, Institute of Basic Science, Khandari, Dr. Bhimrao Ambedkar University, Agra 282002, Uttar Pradesh, India
2
Department of Chemistry, College of Science, King Saud University, Riyadh 11451, Saudi Arabia
3
S.O.S in Chemistry, Jiwaji University, Gwalior 474011, Madhya Pradesh, India
4
Department of Chemistry, Dayal Bagh Educational Institute, Agra 282005, Uttar Pradesh, India
5
Department of Science Technology Unit, King Saud University, Riyadh 11451, Saudi Arabia
6
Restorative Dental Sciences Department, College of Dentistry, King Saud University, Riyadh 11545, Saudi Arabia
7
Department of Chemistry, University of Allahabad, Prayagraj 211002, Uttar Pradesh, India
*
Authors to whom correspondence should be addressed.
Molecules 2023, 28(5), 2116; https://doi.org/10.3390/molecules28052116
Submission received: 2 December 2022 / Revised: 15 February 2023 / Accepted: 16 February 2023 / Published: 24 February 2023
(This article belongs to the Special Issue Computational Studies on the Development and Characterization of Pharmaceutical Materials)
Browse Figures
Versions Notes

Abstract

:
For many decades, uracil has been an antineoplastic agent used in combination with tegafur to treat various human cancers, including breast, prostate, and liver cancer. Therefore, it is necessary to explore the molecular features of uracil and its derivatives. Herein, the molecule’s 5-hydroxymethyluracil has been thoroughly characterized by NMR, UV–Vis, and FT-IR spectroscopy by means of experimental and theoretical analysis. Density functional theory (DFT) using the B3LYP method at 6-311++G(d,p) was computed to achieve the optimized geometric parameters of the molecule in the ground state. For further investigation and computation of the NLO, NBO, NHO analysis, and FMO, the improved geometrical parameters were utilized. The potential energy distribution was used to allocate the vibrational frequencies using the VEDA 4 program. The NBO study determined the relationship between the donor and acceptor. The molecule’s charge distribution and reactive regions were highlighted using the MEP and Fukui functions. Maps of the hole and electron density distribution in the excited state were generated using the TD-DFT method and PCM solvent model in order to reveal electronic characteristics. The energies and diagrams for the lowest unoccupied molecular orbital (LUMO) and the highest occupied molecular orbital (HOMO) were also provided. The HOMO–LUMO band gap estimated the charge transport within the molecule. When examining the intermolecular interactions in 5-HMU, Hirshfeld surface analysis was used, and fingerprint plots were also produced. The molecular docking investigation involved docking 5-HMU with six different protein receptors. Molecular dynamic simulation has given a better idea of the binding of the ligand with protein.

1. Introduction

One of the important derivatives of methyl uracil is 5-hydroxymethyluracil (5-HMU). The heterocyclic category includes uracil and its derivatives. Since some of them are the primary building blocks of DNA and RNA such as pyrimidine, cytosine, and uracil, and their derivatives are of prime value. Researchers in the fields of spectroscopy, medicine, and industry have all paid close attention to pyridine-derived biomolecules because of their biological activities [1,2,3,4,5,6].
Reactive oxygen species are created by cells, which damage biomolecules and are crucial to the development of cancers and aging. The creation of 8-oxo-7,8-dihydroguanine, 5-HMU, 5-hydroxyuracil (5-HU), and other major forms occurs as a result of oxidative DNA damage when nucleotides are hydroxylated. As indicators of oxidative DNA damage, hydroxylated DNA adducts have been studied [7]. Although the biological effects of 5-HMU in humans are not completely known, certain glycosylase activities that remove 5-HMU from DNA have been found [8]. During the T-transition mutations, the conversion of 5-methylcytosine to 5-HMU is frequently detected in cancers. High level of 5-HMU formation in tissue DNA has been linked to human disorders and, hence, is regarded as a biomarker for breast cancer and oxidative DNA damage [9,10].
The pharmacological properties of uracil and its derivatives (Scheme 1) have served as the motivation for recent spectroscopic studies [11]. The formation of 5-HMU takes place by the attack of carbonyl oxygen on the DNA’s methyl group. Hydroxy radicals may bring on different modifications to DNA bases. A big aspect of the development of matters is to explore the hydrogen bonding interaction between the 5-HMU and base pairs of DNA [11]. The excited state processes (internal conversion and intersystem crossover) in the fields of intramolecular charge transfer have significantly contributed to analyzing their kinetics of hydrogen bonding resulting from vibrations of the hydrogen donor and acceptor groups [12,13,14].
As far as researchers know, there has not been any literature-reported computational vibrational research on the 5-HMU’s structure. In this study, DFT/ B3LYP theoretical methods with the “6-311++G(d,p) basis set” were employed to gain a comprehensive description of the molecular structure and vibrational pattern of 5-HMU along with experimental analysis (FT-IR, NMR and UV–Vis spectroscopy). Finally, the findings were compared with the theoretical outcomes. The chemicals ampyra, amine dimedone, sulfanilic acid, and sulfathiazole were the focus of similar experiments that we also conducted [15,16,17,18,19].

2. Results and Discussion

2.1. Optimized Geometry

The optimized geometry of 5-HMU was acquired using the B3LYP methods with the 6-311++G (d, p) basis set, and their comparisons have been made with the experimental analysis (Table 1A,B). The optimized molecular geometry of 5-HMU is shown in Figure 1A and the crystal structure analysis with the diamond program for a trimer (Figure 1B) and two dimers (Figure 1C,D), which was in close resemblance with the structural parameters of the refcode AYEBIP [20,21]. Cagrı et al. have calculated the bond length between C=O, N-C, N-H, O-H, C-C, and C-H as 1.2–1.4, 1.37–1.4, 1.009–1.01, 0.966, 1.3–1.5, and 1.08–1.09 Å, respectively [1]. The obtained values were very close to the experimental parameters which are 1.2–1.4, 1.37–1.4, 1.009–1.01, 0.963, 1.34–1.51, and 1.08–1.09 Å for C=O, N-C, N-H, O-H, C-C, and C-H, respectively. The experimental values for C=O, N-C, N-H, O-H, C-C, and C-H were found to be between 1.23 and 1.4, between 1.36 and 1.38, between 0.87 and 0.89, 0.8, between 1.34 and 1.51, and between 1.08 and 1.1 Å, respectively, which were close to the experimental values of uracil [22].
Each 5-HMU molecule is linked to each other via a hydrogen bond to form a trimer unit. In this case, the 2-N-H...O bonds hold within the 5-HMU trimer. The carbonyl and hydroxyl groups drive forming a trimer between monomer complexes in their close proximity. Figure 1 depicts the intermolecular hydrogen bond N-H...O formation based on the optimized structure (bond angles and distances) with the least amount of energy (Table 1B). Because of the inter-molecular interactions, the energy (E) of the trimer was found to be −1588.58 a.u. with N–H…O bond lengths of 1.94 and 1.92 Å (Supplementary Materials, Figure S1). The bond angle of 174.17° and 174.54° in the trimer unit is almost linear. Two independent dimers exhibited by 5-HMU are shown in Figure 1C,D, and the parameters were summarized in Supplementary Materials; Tables S1 and S2.

2.2. Vibrational Spectral Analysis

IR spectroscopy may be used to identify chemical groups, and it provides microscopic data on their conformations. IR spectroscopy is a vital method to locate the actual binding regions. Both observed and calculated FT-IR and FT-Raman spectra of 5-HMU were compared in Figure 2A,B. 5-HMU is composed of 14 atoms, resulting in 36 fundamental vibrations modes with C1 point group symmetry, all of which are active in FT-IR and FT-Raman. The estimated frequencies (scaled and unscaled) and PED assignments for 5-HMU on the gaseous phase were assigned by the VEDA software (Table 2).

2.2.1. N-H and C-H Vibrations

By using the B3LYP method and 6-311++G(d,p) method, the bands at 3495 and 3455 cm−1 correspond to N-H stretching frequencies, consistent with the experimental value at 3497 cm−1 [23]. These pure modes provide 50% and 50% PED, respectively.
The stretching vibration in the FT-Raman spectrum observed at 3068, 2980, and 2917 cm−1 for C-H was noticed to be in the range 3083, 2954, and 2919 cm−1 in the FT-IR by calculated methods, and their PED assignments contribute to 99%, 94%, and 95% (Table 2).

2.2.2. C-N, H-C-C-C, and C-C Vibrations

The C-N stretching frequency occurs in a composite, which permits the vibrational of various bands [24] In benzamide, the designated C-N stretching band at 1368 cm−1 [25]. The stretching vibrations of C-N with a maximum PED assignment of 34% are allocated to the theoretically scaled wavenumbers in FT-IR estimated at 1444, 1438, 1369, 1354, 1166, 1142, 937, 746, and 525 cm−1. The FT-Raman band of C-N vibrations was displayed at 1432, 1365, 1175, 1148, 1090, and 514 cm−1.
The H-C-C-C torsion vibrations were observed in this investigation, with FT-IR spectra maxima at 1626, 1369, 1290, and 1142 cm−1.
The bands in the range of 1400 and 1650 cm−1 have been ascribed to vibrations of the C-C heterocyclic ring [26]. In the range of 1300-1000 cm−1, stretching vibrations (C-C) are predictable [27]. The C-C stretching vibrations have theoretical frequencies of 1626, 1290, 1166, and 1091 cm−1. PED contributes 65%, 15%, 23%, and 11% to various modalities. In the present study, the FT-Raman spectrum shows the stretching vibration of C-C was observed at 1269 and 791 cm−1. It demonstrates that theoretical values and experimental data are in good accord.

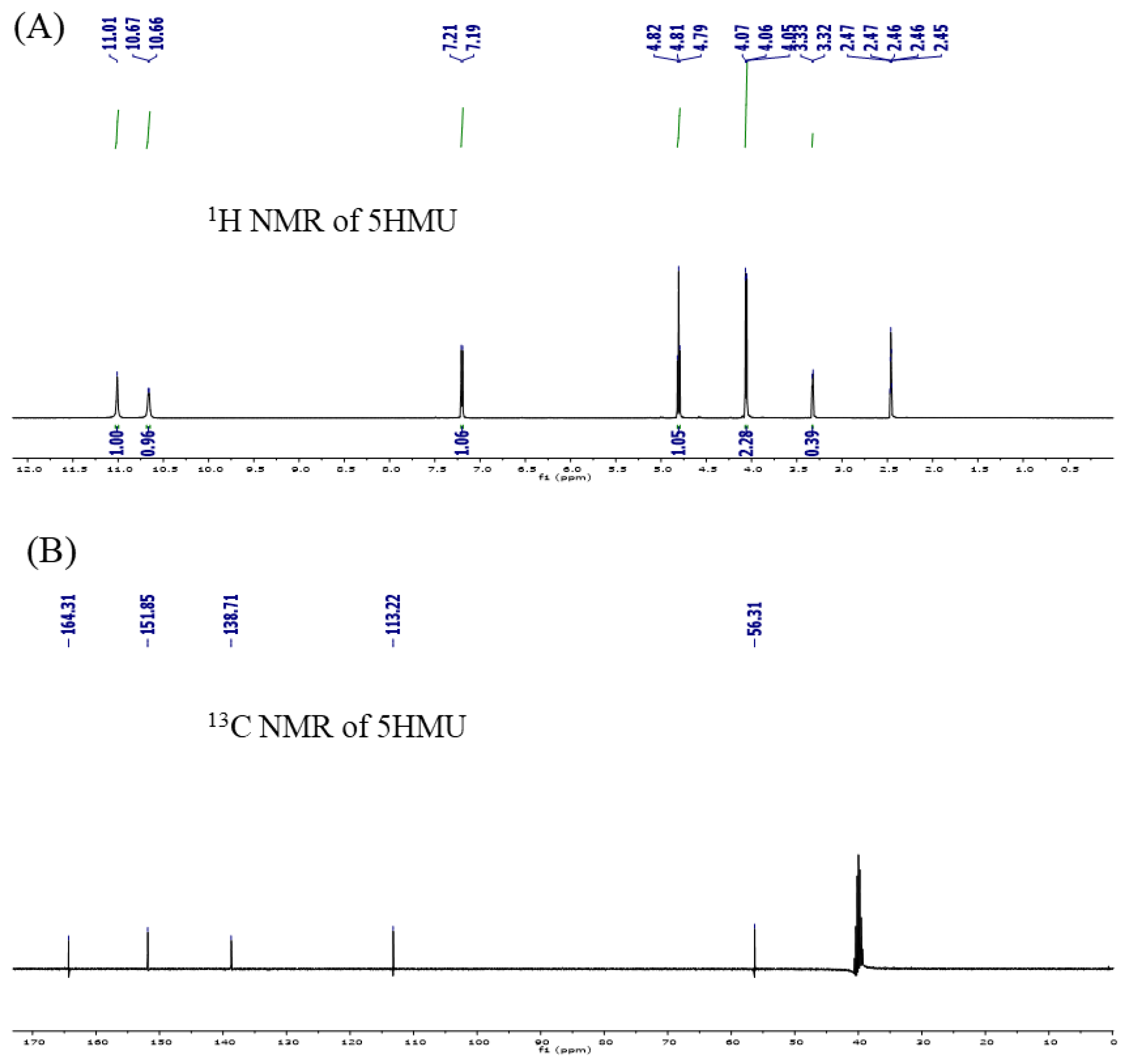
2.3. NMR Analysis

NMR is a reliable method for obtaining detailed information regarding the structure and chemical environment of organic compounds [28]. Hence, the overall geometry optimization of 5-HMU was analyzed employing the B3LYP method and 6-311++G(d,p) basis set, and with the help of GIAO methodology using the aug-cc-pV5Z def2/J basis set, their chemical shifts value was calculated. The input files have been created with the atomistica online tool [29]. Table 3 shows the actual and estimated chemical shifts for 1H and 13C NMR. In DMSO solvent, the experimental NMR of 5-HMU were recorded and displayed in Figure 3A,B. In 1H NMR, the peaks for hydrogen attached to nitrogen (H11 and H13) were observed at 10.66 and 7.20 ppm and theoretically appeared at 7.06 ppm and 6.75 ppm, respectively. In 13C NMR, the carbonyl carbons (C1 and C5) were observed in the area of 164.31 and 151.85 ppm while the peaks for C3 and C4 carbons were observed at 138.71 and 113.22 ppm, and the peak for C9 carbon appeared at 56.31 ppm, and theoretical spectral peaks are found at 165.94, 153.59, 141.71, 120.20, and 62.23 ppm.

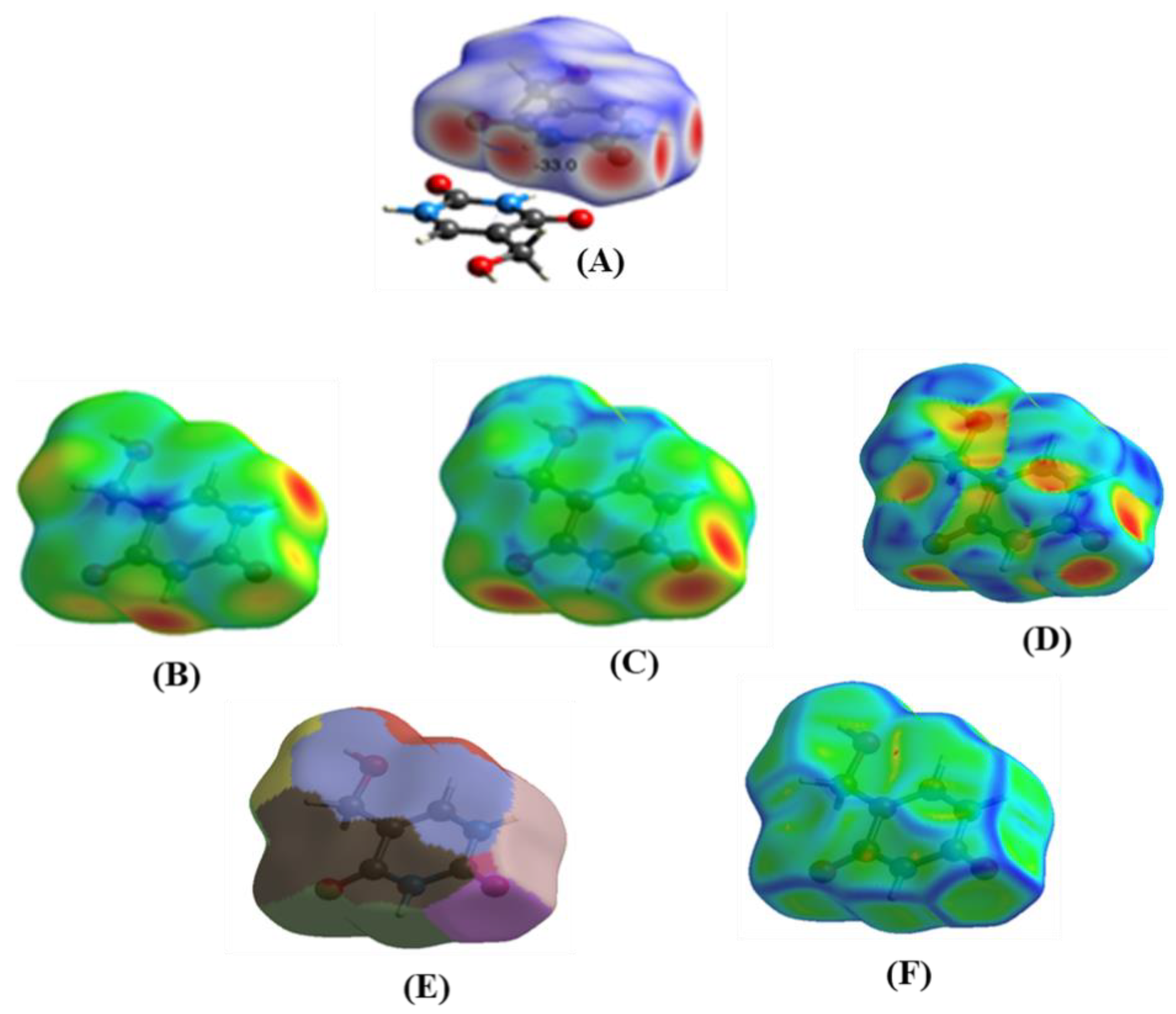
2.4. Hirshfeld Surface (HS) Analysis

HS analysis was established to divide the crystal’s electron density into covalently electron density pieces. The HS is plotted over dnorm, a property that has values between −0.6245 (red) and 0.9768 (blue) a.u. [30,31,32,33,34] along with di, de, shape index, curvedness, and fragment patch, respectively (Figure 4). The values of di ranged from 0.7259 to 2.3342, of de from 0.7281 to 2.2269, of shape index from −0.9922 to 0.9975, of curvedness from −4.043 to 0.306, and of fragment patch from 0 to 13 (Supplementary Material, Table S3). Intermolecular interactions could be seen using color labeling. Spots in red, white, and blue represent intermolecular interactions that occur at distances that are less than, equal to, and greater than the van der Waal radii, respectively.
The HS and its inside closest nucleus are separated by a distance of di, whereas the closest nucleus outside is separated by a distance of de. The type of weak interactions that the molecules in the crystal packing experience are also quantified using 2-D fingerprint designs [35,36,37]. When calculating individual interatomic interactions, the mutual contact for each interatomic contact is also taken into account. The spikes of 2D FP plot show the interatomic interactions that significantly influence crystal packing (Supplementary Material, Figure S2). The major percentage contributions in the crystal packing belonging to O...H, H...H, and C...O are 44, 27.9, and 10.1%, respectively. The other interatomic connections from C…O, N…H, N…O, C…C, N…C, and O…O are 5.25, 4.6, 4.1, 1.7, 1.3, and 1.2%, respectively, that contribute considerably less to crystal packing.

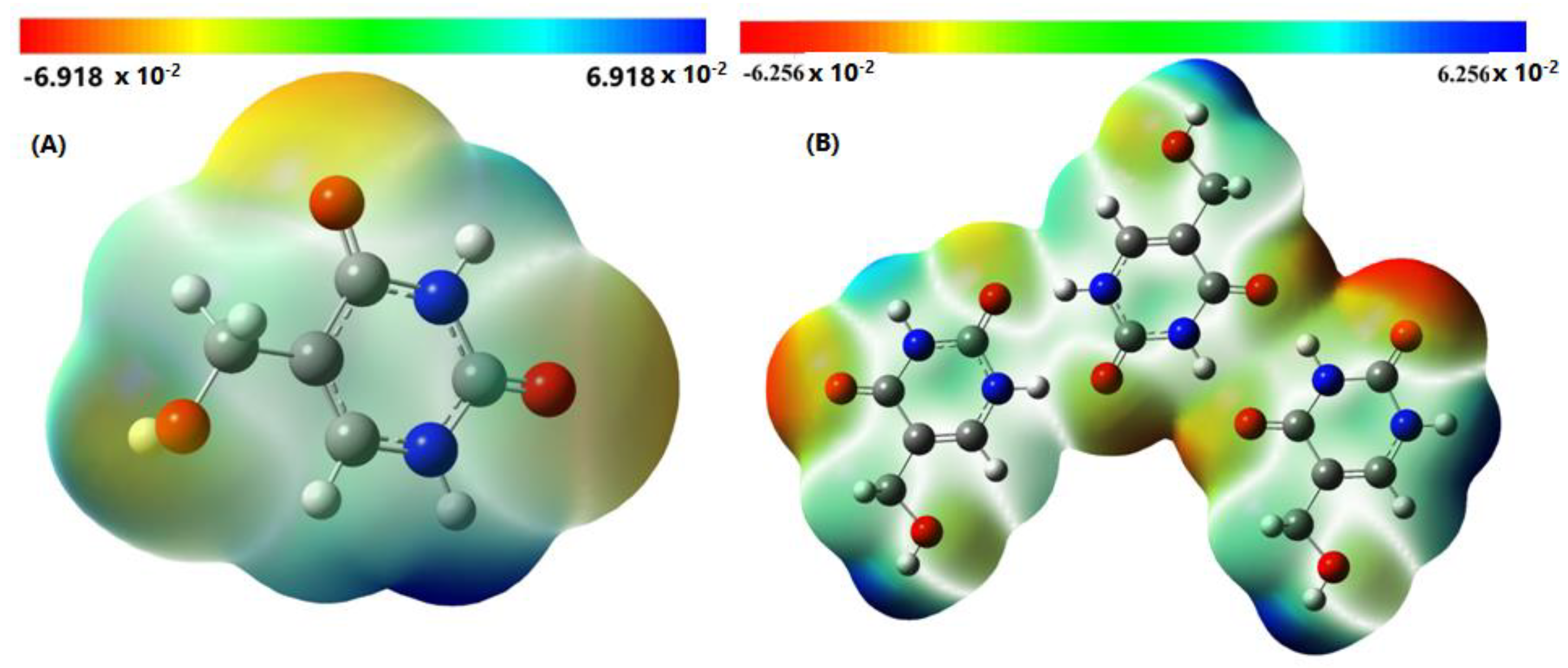
2.5. Molecular Electrostatic Potential Surface (MEPs)

The MEP’s crucial points can be utilized to identify both the structural and electronic characteristics of molecules. It also indicates the shape and size and its positive, negative, and neutral potentials of the molecular system with the help of a color gradient. It is a very useful factor for its electrophilic and nucleophilic properties as well as hydrogen bonding interactions [38]. It also holds importance to verify the proof of the drug’s reaction as an inhibitor and its reaction toward the electrophilic and nucleophilic attacks. This can also be used to predict the relationship between the physicochemical characteristics and molecular structure of the drug [39] such as (a) molecular recognition, drug-receptor interactions; (b) reactive properties of biomolecules; and (c) chemical carcinogenesis, etc. [40,41].
The negative and positive potential sites are preferred for an electrophilic and nucleophilic attack, respectively, corresponding to red and blue colors, respectively. The zero potential sites are encoded in a green color.
The MEP of 5-HMU and its trimer was calculated using DFT and B3LY/6-311++G(d,p) level of theory and ranged 6.918 × 10−2, -6.256 to 6.918 × 10−2, 6.256 × 10−2 a.u, respectively. The 3D plots of monomer and trimer show that the negatively charged potential. Which is a site of the electrophilic attack, is seen near the oxygen atom while the positive potential (blue color) is found near the carbon and hydrogen atoms (Figure 5A,B). It can be inferred that the reactive sites of the 5-HMU are C=O and nitrogen atoms.

2.6. Electron Localization Function

If an electron pair is localized, it means that there is a high possibility of paired electron system with the opposite spin in a certain area of space, leading to the low probability of interaction with outer electrons of this area. A surplus of kinetic energy density generated as a result of Pauli repulsion serves as the foundation for ELF measurement. The baseline of the electron localization function is formed by the electron pairs caused by covalent bonds in molecular space.
ELF can also reveal some important information regarding reactivity, molecular bonding, and chemical structure [42]. Tiny, localized electrons comprise atomic shells, chemical bonds, and lone pair electrons, which governed the strong Pauli repulsion zone. The graph represents high values in a red color (maximum Pauli repulsion) and lower values in a blue color (no Pauli repulsion). The ELF values can be represented graphically in two and three dimensions as a shaded surface map with projection exposing the hydrogen bonding (Figure 6). As we can see, the region around the N-H and C-H bonds has a high ELF value (red) and, hence, a greater chance of finding a spin pair electron while the region around the nucleus of the atoms in the rings shows blue rings.

2.7. Non-Linear Optical (NLO) Analysis

DFT methods employing B3LYP/6-311++G(d,p) basis set have been utilized extensively to study the NLO characteristics of organic materials [43,44]. For this purpose, the dipole moment (μD), linear polarizability (α), and first-order hyperpolarizability (βtot) were computed; electron correlation, which is dependent on the basis set used, can alter the hyperpolarizability value [45,46,47]. Table 4 lists the computed dipole moment as well as the linear polarizability and first-order hyperpolarizability. The values of α and β from the Gaussian output files are given in atomic units (a.u.), which were then translated into electrostatic units (e.s.u) using the formulas 1 a.u. = 0.184210−24 e.s.u for α and 1 a.u. = 8.639610−33 e.s.u for β.
The calculated dipole moment for 5-HMU was found to be 3.5115 Debye, and the highest dipole moment is seen in the μz component. The linear polarizability and first-order hyperpolarizabilities were −0.914 × 10−23 and 0.3545 × 10−30 e.s.u, respectively, compared to the values of urea which are 0.9771 × 10−23 and 0.927 × 10−30 e.s.u, respectively.

2.8. Natural Bond Orbital (NBO) and Natural Hybrid Orbital (NHO) Analysis

The NBO technique offers knowledge about intra- and inter-molecular interactions between the bonds, as well as a suitable framework for dealing interactions in each filled and virtual orbital area, as well as charge transfer and conjugative interactions in molecular systems [48]. The second-order Fock matrix was employed in the NBO analysis to evaluate the donor–acceptor interactions [49]. An empty non-Lewis orbital replaces the localized NBO of the perfect Lewis structure as a result of the interaction. The stabilization energy E(2) associated with the delocalization i → j is calculated for each donor (i) and acceptor (j) as:
E ( 2 ) = - n σ F i j 2 ε σ *   −   ε σ
where (Fij)2, εσ, εσ*, and nσ are the Fock matrix element between donor (i) and acceptor (j), the energies of bonding NBO, anti-bonding NBO, and population in the s (donor) orbital, respectively [50]. The more intense the engagement between electron donors and electron acceptors, or the greater the propensity for electron donors to donate to electron acceptors, the stronger the E(2) value, and the more conjugation throughout the system. A stabilizing donor–acceptor interaction takes place when electron density delocalizes between occupied Lewis type (bond or lone pair) NBO orbitals and formally unoccupied (antibonding and Rydberg) non-Lewis NBO orbitals. NBO analysis was analyzed on a Gaussian 09W [51] package at DFT-B3LYP/6-311++G(d,p) theory level (Supplementary Material, Table S4). The weak departure from precisely localized natural Lewis structure that signifies the primary noncovalent effect is designated by the delocalization of σ-σ* electrons. In 5-HMU, the highest stabilization is shown by the delocalization of π (C3–C4) to π*(C5–O7) of 22.5 kJ/mol. The electron contributing from the lone pair(1) of the nitrogen atom (N2) and N6 to the (C1–O8), which offers a good stabilizing energy of 53.48 and 58 kJ/mol, respectively, are another important interaction connected to the resonance in the molecule. Similarly, the electron contributing from lone pair(1) N6 to π*(C5–O7) gives a moderate stabilization energy of 48.98 kJ/mol.
The NBO, which is composed of its natural atomic hybrids (hA and hB), displays sp hybridization, their polarization coefficients (CA and CB), and atomic orbital (percentage s-character, p-character) (Supplementary Material, Table S5). The σ(C1-N2) is constituted by the sp2.16 of carbon (C1) and sp1.9 of nitrogen (N2). The C1 here has 31.61% s character and 68.28% p character, and N2 has 34.43% s character and 65.25% p character. As a result, the NBO C1-N2 is a bond between a C1 sp2.16 hybrid and a N2 sp1.9 hybrid. The larger polarization coefficient (CB = 0.7915) of the N2 atom compared to the C1 atom (CA = 0.6112) reflects its stronger electronegativity.
σCN = 0.6112(sp2.16) C1 + 0.7915(sp1.9) N2
The p-component of the hybrid described by the polar (ϴ) and azimuthal (Φ) angles is utilized to specify its orientation. The hybrid’s directionality is determined by comparing the central line direction between the two nuclei to the maximum angular amplitude. The bond bends, which is then stated as the angle of divergence between these two directions. Because of the optimization of geometry, the orientation of geometry changes (Supplementary Material, Tables S6 and S7). The carbon NHOs of NBO σ C1-N2 (NBO 1) and σ C1-N6 (NBO 2) are bent away from the line of centers of C-N by 5.3° and 5°, respectively, while the nitrogen NHOs are bent away by 1.2° and 1.4°, respectively.

2.9. Population Analysis

Analysis of atomic charge is essential because it determines the atoms NMR chemical shifts and impacts the system’s dipole moment, polarizability of molecules, electronic structure, and molecular reactivity. Using the B3LYP/6-311++G(d,p) method and basis set together with the Mulliken population analysis (MPA) method, the charges on the atoms of the 5-HMU were determined (Supplementary Material, Table S8 and Figure S3). There are five carbon atoms, three oxygen, and two nitrogen atoms as depicted in Figure S3. Almost all carbon atoms are positively charged except C9. Out of the carbon atoms, C1 attached with the hydroxy group has the highest positive charge due to the electron-withdrawing nature of oxygen. C9 was the most negatively charged of all the atoms. The oxygens are almost similar in charge, same with the case of nitrogen atoms. It is usual that all the hydrogen atoms have the same charges with a little difference due to the presence of different groups attached to them.
The Fukui function is a useful tool based on Mulliken population analysis. It gives quantitative information such as the electron densities and determines the most electrophilic and nucleophilic sites on a molecule. The electron density decreases upon addition of electron to some spots providing the negative value of the Fukui function. On the contrary, positive values mean an electron is removed and electron density is increased.
As per Parr and Yang [52], the Fukui function’s value can be used to determine the reactivity. As a result, the condensed Fukui function’s value increases and so does the atomic center’s level of reactivity. When the molecule is attacked by an electrophile or when it loses electrons, the reactivity is denoted by the symbol f-, whereas when it is attacked by a nucleophile or when it gains electrons, the reactivity is denoted by f+. The value of Fukui functions along with the local softness of each atom obtained using Mulliken population analysis has been summarized in Supplementary Material, Table S8. It can be seen that C9 and O8 have the highest values for f+ and f−, respectively. These sites are likely to undergo nucleophilic and electrophilic attacks, respectively. The reactivity order for electrophilic attack is O8 > C4 > O7 > N2 > O10 > N6 > C5 while the order for nucleophilic attack is C9 > C1 > N2 > C3 > O10 > N6, and the order for radical attack is C9 > C1 > C3. The electrophilic attack is shown to have higher reactivity than the other two.

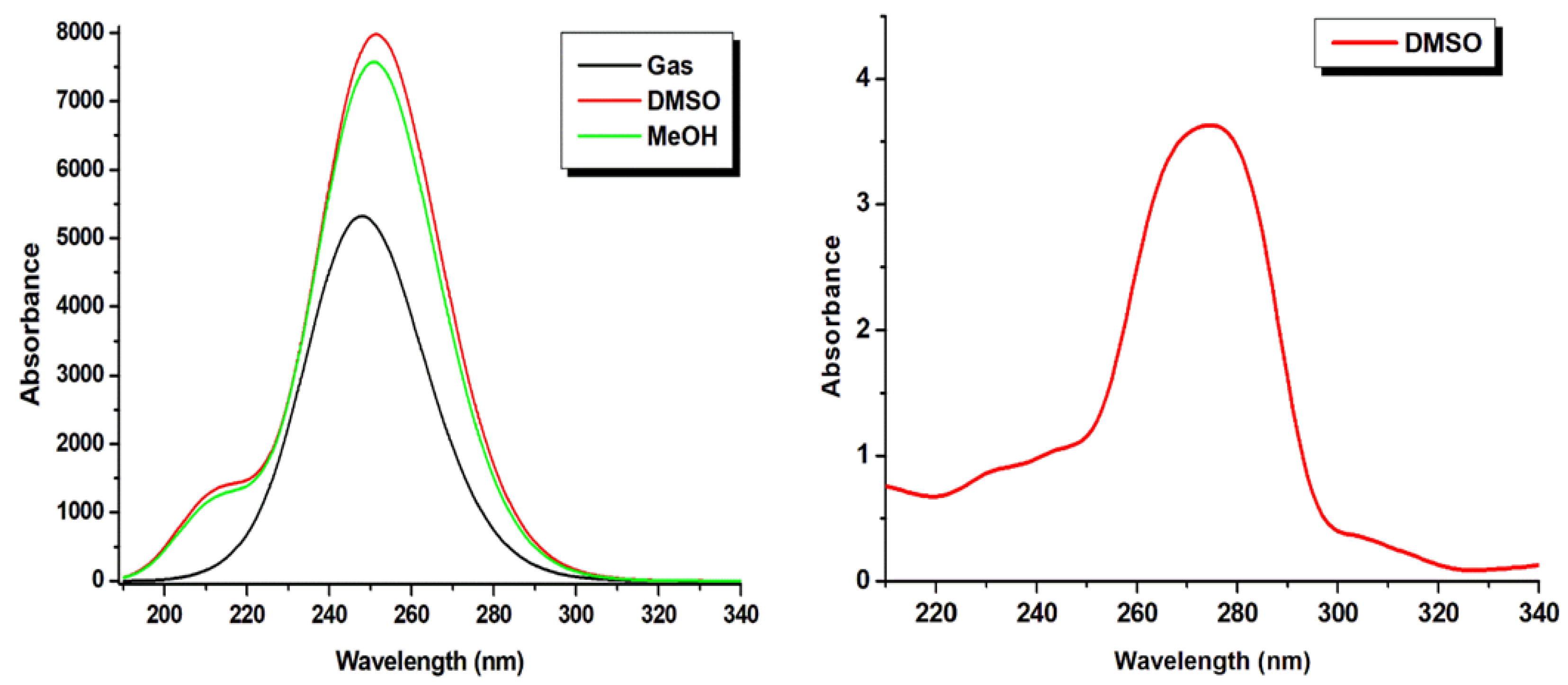
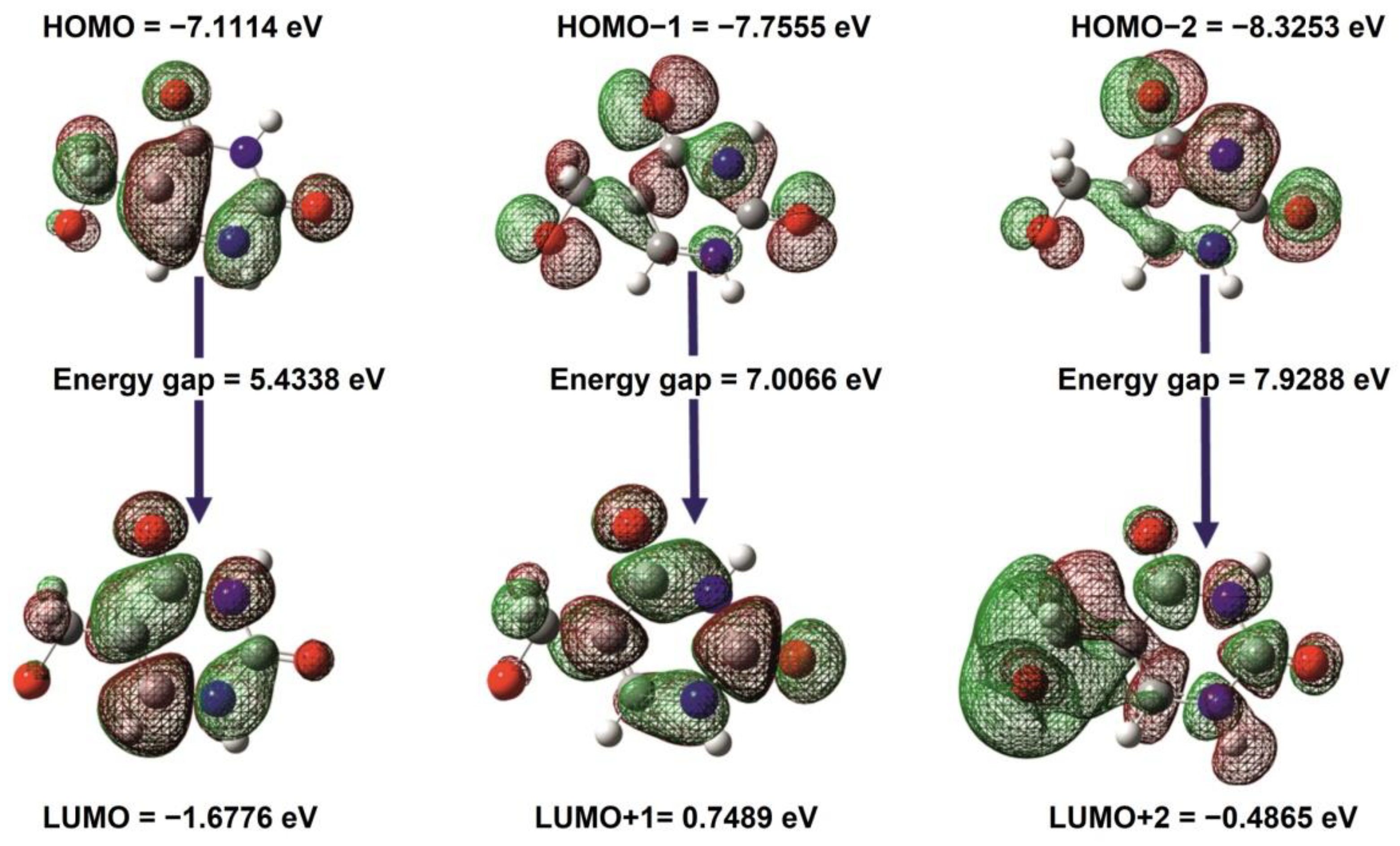
2.10. Frontier Molecular Orbital (FMO) and UV–Vis Spectra

The UV–Vis spectrum of 5-HMU was measured experimentally in DMSO solvent. Using the TD-DFT/B3LYP/6-311++G(d,p) basis set and the PCM model polarizable continuum model, the UV–Vis spectrum was estimated in the DMSO and methanol solvents. The excitation energy, oscillatory strength, and bandgap are summarized in Supplementary Materials; Table S9. The calculated absorption maximum in gas, DMSO, and methanol were 261.67, 252.24, and 251.81 nm, respectively, while the experimental value in DMSO was observed at 275 nm. The calculated and experimental UV–Vis spectra of the titled molecule were given in Figure 7. It is very much clear from the spectra that the molecule shows a (red and hyperchromic) shift in DMSO and MeOH because of secondary interaction with the solvent.
There are various methods for calculating the excitation energy. The first is the difference between the lowest unoccupied molecular orbital (LUMO) and highest occupied molecular orbital (HOMO) of a neutral system (LUMO). These orbitals regulate the manner in which medications interact with other molecules, such as interactions between pharmaceuticals and their receptors. The HOMO electrons’ propensity to transfer to the LUMO can be accurately determined qualitatively using the frontier molecular orbitals (FMO). The HOMO, LUMO, and FMO gaps, which are crucial aspects of quantum chemistry [53], serve as illustrations of the chemical reactivity and kinetic stability of molecules. While the LUMO orbital mostly functions as an electron acceptor, the HOMO orbital primarily serves as an electron donor. At B3LYP/6-311++G(d,p), the HOMO, LUMO, and orbital energy gaps for the 5-HMU were determined. The highest molecular orbital value that is occupied is −7.12 eV, and the lowest molecular orbital value that is empty is −1.68 eV (Supplementary Materials; Table S10). HOMO-LUMO, HOMO-1-LUMO+1, and HOMO-2-LUMO+2 have energy gaps of 5.4338 eV, 7.0066 eV, and 7.9288 eV, respectively (Figure 8). It can be seen from the plot of HOMO that the positive and negative regions are spread equally all over the molecule same as in the case of LUMO. The charge transfer interaction between the molecules is explained by the energy gap between HOMO and LUMO. The smaller the energy gap (5.44 eV), the easier the charge transfer between the two orbitals due to the electron acceptor’s strong electron-accepting capacity. The bioactivity of the molecule is due to the high charge transfer contact. There are some important tools such as ionization potential (I.E), electron affinity (E.A), electronegativity (χ), chemical hardness (ɳ), and chemical potential to check the order of stability of the molecular system (Supplementary Materials; Table S10). HOMO and LUMO energies are used to compute chemical hardness and chemical potential (μ). A negative chemical potential is defined as electronegativity. Chemical hardness could be a good predictor of chemical stability. Soft molecules are those with a little low energy gap, while hard molecules are those with a big energy gap [54,55].
When the chemical hardness of a substance is raised, it is said to have higher stability. A hard molecule is said to have a wide energy gap when compared to soft molecules [56]. The calculated chemical hardness of 5-HMU is 2.71. The electrophilicity index of can be used to describe a compound’s biological potential, and the calculated electrophilicity index of 5-HMU is 3.55. Furthermore, it has very low softness value of 0.369. It provides information on the toxicity of various contaminants based on their reactivity and site selectivity [57].

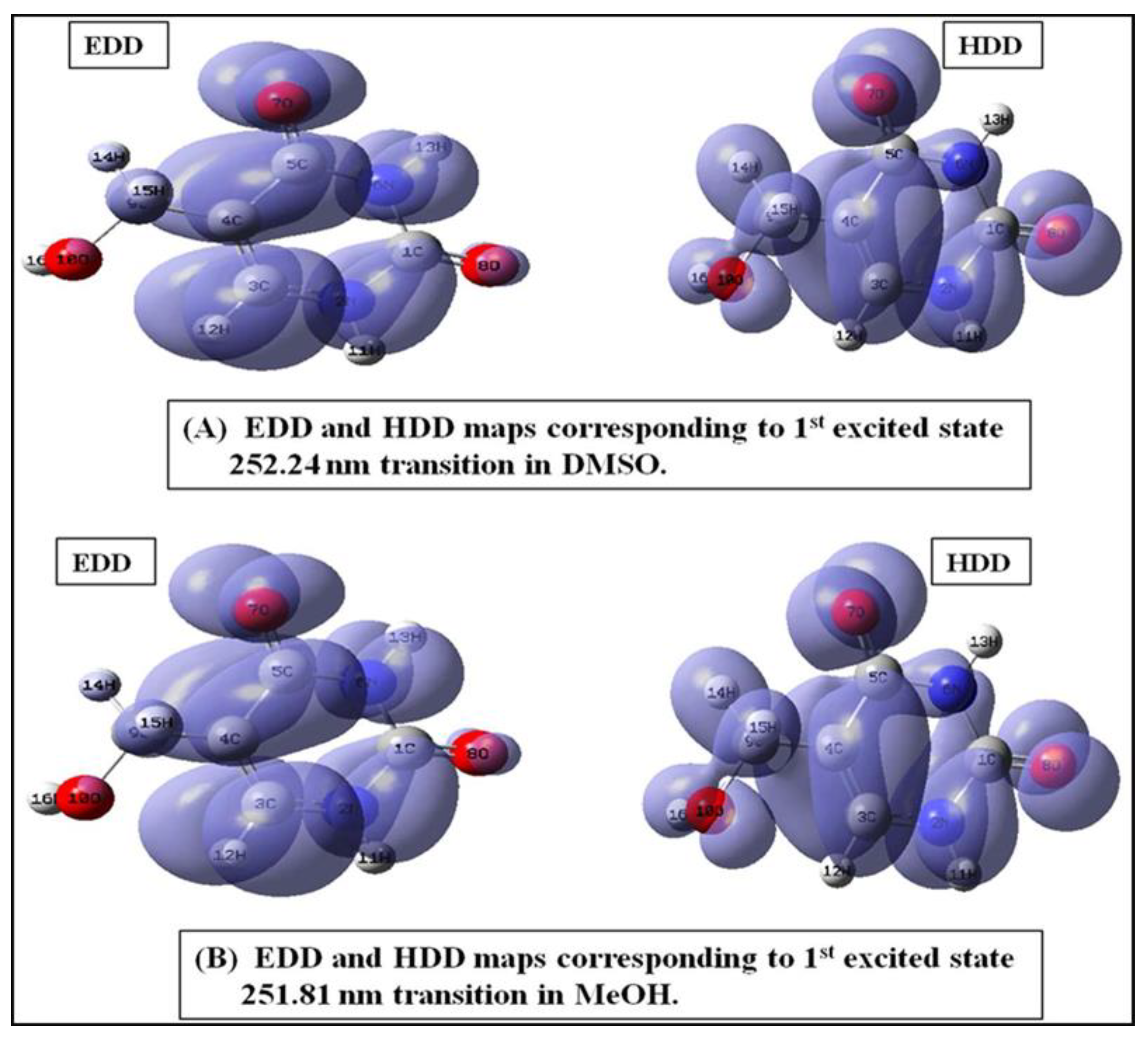
2.11. Electron Excitation (Electron and Hole Density Distribution) Analysis

Electron and hole density distribution provides the concept of multi-molecular orbital excitation that delivered obvious features of the excited state for the receptors and the corresponding receptor anion. Once an electron is delocalized from A to B as a part of single electron excitation processes (where A and B are real space functions), the excitation of an electron from an occupied to virtual molecular orbital (MO) is taken as a model for excited state. The ground state of MO can be compared to the various regions of photoexcited states shown by the density distribution maps for electrons (ρele(r)) and holes (ρhole(r)). According to T. Lu and F. Chen, the electron and hole density distribution (EDD and HDD) can be explained by MO wavefunction (Φ) and the configuration coefficient (w), which resembles to the transition of an electron from occupied MO(i) to a virtual MO (1) as shown in equations below [58]:
ρ ele ( r ) = ∑   W l i 2   Φ l   ( r )   Φ l   ( r ) + ∑   ∑   W l i W m i   Φ l   ( r )   Φ m   ( r )
i→l         i→li→m ≠ l
ρ hole ( r ) = ∑   W l i 2   Φ i   ( r )   Φ i   ( r ) + ∑   ∑   W l i W l j   Φ i   ( r )   Φ j   ( r )
i→l       i→l j≠i→l
The electron and hole density distribution maps for 5-HMU were calculated using the aforementioned method. The B3LYP/6-311++G(d,p) level calculations were performed using PCM in DMSO and MeOH and the Multiwfn 3.3.4 software [58].
The strong absorption band maxima at 275 nm (4.51 eV) corresponding to HOMO-LUMO were predicted by calculations for the electronic structure of 5-HMU at the TD-DFT- B3LYP/6-311++G(d,p) level with PCM in DMSO. This transition is shown in Figure 9, whereas the absorption at max = 251.81 nm (4.92 eV) for MeOH. The estimated EDD map in Figure 9A depicts a significantly thicker surface on the ring and C=O groups, while the HDD map depicts a denser isosurface on the whole molecule in DMSO. Figure 9B shows EDD and HDD maps created in MeOH; the EDD map exhibits a thicker surface on the entire ring and C=O groups, and the HDD map displays a denser surface on the entire molecule (Supplementary Materials; Table S11).

2.12. Thermodynamic Properties

The B3LYP/6-311++G(d,p) technique and basis set by ORCA software were used to calculate the thermodynamic characteristics of 5-HMU. The values of the enthalpy (H0), entropy, and Gibbs free energy were computed between 100 K and 700 K. The intensity of molecular oscillations also rises with temperature because of higher translational and rotational energy, which is supported by uniform distribution [59,60]. In light of the fact that thermal stability is crucial both at low and high temperatures, physicochemical effects are observed in the industry to preserve food or medicine storage. As a result, temperature is important in chemical thermodynamics and pharmacodynamics. From the Gibbs free energy, biological thermodynamic applications such as protein stability, membrane, DNA, and enzyme kinetics can be calculated.
The Gibbs free energy (G0) shows an inverse relation with temperature, and hence it decreases with an increase in temperature (Supplementary Materials; Figure S4 and Table S12). The correlation between the temperature and the thermodynamic function was determined using quadratic and linear equations. The correlation (R2) between the two for entropy, enthalpy, and Gibbs free energy are 1, 0.9999, and 0.9747, respectively. The relationships are plotted in Supplementary Material; Figure S5. The following is the thermodynamic correlation fitting equation:
G = 0.0012x2 − 1.3345x + 385.3434 (R2 = 0.9747)
S = −0.0001x2 + 0.5423x + 214.6058 (R2 = 1)
H = 0.0002x2 + 0.0298x + 310.3621 (R2 = 0.9999)
The thermodynamic properties also influence how non-covalent interactions such as electrostatic interactions, multiple hydrogen bonds, van der Waals interactions, and hydrophobic effects bind to one another [61]. Utilizing the relationship between thermodynamic functions and the second rule of thermodynamics, these features can be used in the thermochemical field to determine the flow of chemical reactions and identify other thermodynamic energy [62].

2.13. Molecular Docking

The ligand–protein docking method is used to predict the ligand’s binding sites in proteins using a three-dimensional network. Predicting chemical interactions between the lead compound and biologically relevant targets (proteins, enzymes, etc.) has become critical in the science of drug discovery. Furthermore, by analyzing the docking of the lead molecule with a variety of protein targets, one can gain insight into the underlying molecular mechanisms of selectivity [63].
The two fundamental steps of the docking procedure are the prediction of the ligand structure as well as its orientation within these sites and the evaluation of the binding affinity. The Swiss ADME online source predicted the target protein that needs to dock with the 5-HMU and downloaded the file in RCSB protein database (pdb) format. The relevant target protein ID (docked with a similar ligand as hydantoin) was picked from the Swiss ADME- target prediction site and retrieved from the protein data bank with the aid of a smile string of 5-HMU ligand, which was added to the site of the Swiss target prediction (PDB). The active sites for docking are ligands that have already docked inside proteins. The proteins 4P6T 4P6S, 6QXD, 6EI4, 5OAE, and 5M67 all belonging to the oxidoreductase class except 5M67 and were docked with 5-HMU showing the bond distance of hydrogen bond interaction between 5-HMU and the target (Figure 10A–F). In this docking, all the metal centers of the proteins are avoided in PDB’s. The binding energies have been calculated using AutoDock vina [64,65]. The ligand’s characteristics, including its binding energy and inhibition constant, are collected in Table 5. The lowest binding energy (∆G) and intermolecular energy were found to be −5.0 kcal/mol between 5-HMU and 6QXD in the protein–ligand interaction. The highest binding energy was found for 5M67. The calculated bond distance for the same object is 2.270. The solid lines in the figure depict the hydrogen bonds between the ligand and the targeted protein. Inhibition constants were calculated by Ki = exp(∆G/RT).

2.14. Drug-Likeness

To decide the structural appearances of ligands in order to achieve effective and efficient results, a set of rules known as “drug-likeness” is employed following the Lipinski’s rule of five [66,67]. We looked at the polar surface area (TPSA), molar refractivity, and the quantity of hydrogen bond donors and acceptors (HBD and HBA, respectively) (Supplementary Materials; Table S13), which shows that these metrics have values within acceptable ranges for the medication candidate under evaluation. The HBD and HBA should be less than 5. The title molecule satisfies these requirements. The MLogP, one of the most critical characteristics (showing the hydrophobic/lipophilic nature of the molecule), is equal to −1.22 which is far below Lipinski’s rule of five threshold value of 5. The TPSA value for a compound is 85.95 Å2, which is much below the maximum cut-off value of 140 Å2 (Supplementary Materials; Figure S6). The molar refractivity was calculated to be 33.81, which falls within the range of pharmaceutical candidates [68].

2.15. Molecular Dynamic (MD) Simulation

The efficacy of ligand binding on protein structure was analyzed by using MD modeling. The GROMACS 2022 software was utilized to study MD simulation [69]. The ‘CHARMM36 force field’ was used to generate the protein topology. Each system was solvated using the TIP3P water model before being neutralized with the proper concentrations of Na+ and Cl− ions [70]. The docked protein–ligand combination of the molecule with the PDB ID: 6QXD was used to evaluate the stability of the 5-HMU. To confirm the stability of the complex structures, the simulation was run for 20 nanoseconds at room temperature. The system was designed to use as little energy as possible, employing the steepest descent technique. Position restraint together with NVT and NPT ensembles were used after the energy minimization process. Following temperature stabilization, an isothermal isobaric ensemble (NPT) was performed. The trajectory indicates that the docked ligand was stable throughout the simulation in the protein’s binding site where the protein-ligand is relatively stable and exhibits minor conformational changes. The data were examined using the graphical tool origin once all of the MD simulation phases had been completed. The built-in functions of GROMACS 2022 were used to find the different vital parameters, such as root mean square deviation (RMSD), number of hydrogen bonds occurring between protein and the ligand, and interaction energy. The stability of the complex is represented by the RMSD stabilization (Figure 11A). After alignment, further RMSD values were determined for the protein with an inbuilt option in the VMD software; therefore, the protein showed a smaller variation in the RMSD values. Thirteen stable hydrogen bonds are formed between the protein and the ligand, and these bonds define how strong the complex is (Figure 11B). However, in this instance, the total interaction energy is useful. According to the common formula for adding two values, this value is equal to −137.34 ± 11.8 kJ mol−1 after propagating the error. According to every outcome of the MD simulation, 5-HMU can bind to the active sites of proteins to create a stable protein complex. The resultant values of MD simulations are more precise and correct in comparison to docking results. We may predict that there is more fluctuation in the RMSD values of ligand arisen due to movement all 13 hydrogen bonds of ligand during the simulation, but not at once. Hydrogen bonds occupancy with their donor–acceptor sides showed in Supplementary Materials; Table S14.

3. Experimental Section

3.1. Materials and Instrumentations

The spectral study was conducted using the 5-hydroxymethyl uracil (Sigma-Aldrich Delhi, India). The infrared spectrum was measured with FT-IR PerkinElmer (Serial No. 105627) using a KBr disc method (FT-RAMAN at IIT Kanpur, Kanpur, India). NMR spectra were achieved with the help of a Bruker Advance 400/Avll HD-300 (FT-NMR) apparatus. The UV–Vis analysis was carried out on a UV-1280 Spectrophotometer (Shimadzu, Mumbai, India).

3.2. Computational Analysis

The supporting information has provided computational details (See the Supplementary Materials).

4. Conclusions

The title chemical 5-HMU was examined through the spectroscopic analysis using FTIR, FT-Raman, NMR (1H and 13C), and UV–Visible in accordance with the theoretical DFT method. The optimized crystal structure of 5-HMU and its trimer that was produced with the least amount of energy were compared to the experimental crystal structure. The development of an intermolecular H-bond with adjacent monomers was found to stabilize the trimer, and its ideal molecular structure was established using the 6-311++G(d,p) basis set and the DFT-B3LYP approach. The complete vibrational assignment was investigated based on potential energy distribution (PED). The nucleophilic and electrophilic sites, as well as the H-bonding interactions of the 5-HMU, were predicted via MEP mapping. The positive potential sites were found on hydrogen and carbon atoms, whereas the negative potential sites were found on oxygen atoms. The electron localization function (ELF) was employed to represent the degree of electron localization. The resonance interaction energy of 5-HMU was 48.98 kJ/mol, as measured by the maximum stabilization of electron leading LP(1) N6—π* C5-O7. The deviation angle, coupled with the polar (θ) and azimuthal (ɸ) angles of the vector from the nucleus, were utilized to determine the orientation of a hybrid. The energy gap between highest occupied and lowest unoccupied was determined to be 5.4338 eV. Three metrics, such as dipole moment, polarizability, and hyperpolarizability, were used to validate a material nonlinear optical characteristic. High chemical hardness (2.72) indicated the compound stability, while low chemical softness (0.37) was designated as non-toxicity, and the electrophilicity index (3.56) indicated 5-HMU biological activity. EDD and HDD maps for the first excited-state were created with DMSO and MeOH to indicate single MO pair excitation. In the temperature range of 100 K to 700 K, the ORCA program was utilized to determine various thermodynamic features. It depicts how the thermodynamic properties change as the temperature increases. The Fukui function describes the intramolecular interaction between atoms in a molecule. The 5-HMU receptor has the lowest binding energy of −5.0 kcal/mol, indicating that further research into the therapeutic potential of the mentioned molecule is recommended. The molecular dynamic simulation gave better idea of binding and showed 11 stable hydrogen bonds.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules28052116/s1, Figure S1: Trimer of 5-HMU by a secondary interaction; Figure S2. 2-D fingerprint plot of different contributions for 5-HMU; Figure S3. Graph showing Mulliken atomic charges of 5-HMU; Figure S4. Graph representing the dependence of Entropy, Gibbs free energy, and enthalpy on the temperature of 5-HMU; Figure S5. The plot showing relationships of temperature with Gibbs free energy, and entropy, enthalpy; Graph representing the dependence of Entropy, Gibbs free energy, and enthalpy on the temperature of 5-HMU; Figure S6. Predicted Lipinski’s rule of five showing bioavailability radar plot of 5-HMU; Table S1: Some optimized “Geometrical parameters” Bond Length (pm) and Bond Angle (°) of dimer 1; Table S2. Some optimized “Geometrical parameters” Bond Length (pm) and Bond Angle (°) of dimer 2; Table S3. Surface property information in Hirshfeld for 5-HMU; Table S4. Second-order perturbation theory of the Fock matrix NBO analysis of 5HMU; Table S5. Hybrid, polarization coefficient and atomic orbital contribution in selected natural bond orbitals of 5HMU; Table S6. Natural hybrid orbital directionality and bond bending (deviations from line of nuclear centres) of 5HMU; Table S7. Natural Hybrid Orbital directionality and bond bending (deviations from line of nuclear centres) of 5HMU; Table S8. Mulliken charge distribution, Fukui function, and local softness corresponding to (0,1), (−1,2), and (1,2) charge and multiplicity of 5-HMU; Table S9. Comparison of calculated and experimental electronic properties of 5-HMU; Table S10. Calculated energy values of 5-HMU; Table S11. Calculated centroid coordinates and distances between EDD and HDD centroids for allowed excited state transition of 5-HMU in DMSO and methanol; Table S12. Temperature dependence of thermodynamic properties of 5-HMU at B3LYP/6–311++G(d,p); Table S13. ADME properties of 5-HMU; Table S14. Hydrogen bonds occupancy with their donor-acceptor sides [30,51,58,65,71,72,73,74,75,76,77,78,79,80,81,82].

Author Contributions

Conceptualization, S.J. and G.J.; methodology, N.S.; software, G.J.; validation, M.A., M.M., A.A., W.S.S. and R.A.; formal analysis, A.F.; investigation, M.K. and A.F.; resources, M.K.; data curation, A.F.; writing—original draft preparation, M.K.; writing—review and editing, S.J.; visualization, N.A.Y.A.; supervision, S.J. and G.J.; project administration, M.A.; funding acquisition, M.A. All authors have read and agreed to the published version of the manuscript.

Funding

The authors extend their appreciation to the Deputyship for Research & Innovation, Ministry of Education in Saudi Arabia for funding this research work through the project number IFKSURG-2-1666.

Informed Consent Statement

Not applicable.

Data Availability Statement

Data Availability Statements are available in section “MDPI Research Data Policies” at https://www.mdpi.com/ethics (accessed on 30 November 2022).

Conflicts of Interest

There are no conflict to declare.

References

  1. Çırak, Ç.; Sert, Y.; Ucun, F. Effect of intermolecular hydrogen bonding, vibrational analysis and molecular structure of a biomolecule: 5-Hydroxymethyluracil. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2014, 127, 41–46. [Google Scholar] [CrossRef] [PubMed]
  2. Bandekar, J.; Zundel, G. Normal coordinate analysis treatment on uracil in solid state. Spectrochim. Acta Part A Mol. Spectrosc. 1983, 39, 343–355. [Google Scholar] [CrossRef]
  3. Aruna, S.; Shanmugam, G. Vibrational assignments of six-membered heterocyclic compounds: Normal vibrations of 6-amino uracil and 6-amino 2-thio uracil. Spectrochim. Acta Part A Mol. Spectrosc. 1985, 41, 531–536. [Google Scholar] [CrossRef]
  4. Portalone, G.; Ballirano, P.; Maras, A. The crystal structure of 3-methyluracil from X-ray powder diffraction data. J. Mol. Struct. 2002, 608, 35–39. [Google Scholar] [CrossRef]
  5. Grosmaire, L.; Delarbre, J.-L. Vibrational spectra of 6-methyluracil, 6-methyl-2-thiouracil and their deuterated analogues. J. Mol. Struct. 2012, 1011, 42–49. [Google Scholar] [CrossRef]
  6. Yaniv, M.; Folk, W. The nucleotide sequences of the two glutamine transfer ribonucleic acids from Escherichia coli. J. Biol. Chem. 1975, 250, 3243–3253. [Google Scholar] [CrossRef]
  7. Amir, M.; Javed, S.; Kumar, H. Pyrimidine as antiinflammatory agent: A review. Indian J. Pharm. Sci. 2007, 69, 337. [Google Scholar] [CrossRef] [Green Version]
  8. Shigenaga, M.K.; Ames, B.N. Assays for 8-hydroxy-2′-deoxyguanosine: A biomarker of in vivo oxidative DNA damage. Free Radic. Biol. Med. 1991, 10, 211–216. [Google Scholar] [CrossRef]
  9. Rusmintratip, V.; Sowers, L.C. An unexpectedly high excision capacity for mispaired 5-hydroxymethyluracil in human cell extracts. Proc. Natl. Acad. Sci. USA 2000, 97, 14183–14187. [Google Scholar] [CrossRef] [Green Version]
  10. Djuric, Z.; Heilbrun, L.K.; Reading, B.A.; Boomer, A.; Valeriote, F.A.; Martino, S. Effects of a Low-Fat Diet on Levels of Oxidative Damage to DNA in Human Peripheral Nucleated Blood Cells. JNCI J. Natl. Cancer Inst. 1991, 83, 766–769. [Google Scholar] [CrossRef]
  11. Frenkel, K.; Karkoszka, J.; Kim, E.; Taioli, E. Recognition of oxidized DNA bases by sera of patients with inflammatory diseases. Free Radic. Biol. Med. 1993, 14, 483–494. [Google Scholar] [CrossRef] [PubMed]
  12. Zhao, G.-J.; Han, K.-L. Hydrogen Bonding in the Electronic Excited State. Acc. Chem. Res. 2012, 45, 404–413. [Google Scholar] [CrossRef] [PubMed]
  13. Zhao, G.-J.; Han, K.-L. Early Time Hydrogen-Bonding Dynamics of Photoexcited Coumarin 102 in Hydrogen-Donating Solvents: Theoretical Study. J. Phys. Chem. A 2007, 111, 2469–2474. [Google Scholar] [CrossRef] [PubMed]
  14. Zhao, G.-J.; Han, K.-L. Ultrafast Hydrogen Bond Strengthening of the Photoexcited Fluorenone in Alcohols for Facilitating the Fluorescence Quenching. J. Phys. Chem. A 2007, 111, 9218–9223. [Google Scholar] [CrossRef]
  15. Fatima, A.; Ali, A.; Shabbir, S.; Khan, M.; Mehkoom, M.; Afzal, S.M.; Ahmad, M.; Althubeiti, K.; Siddiqui, N.; Singh, M.; et al. Synthesis, crystal structure, characterization, Hirshfeld analysis, molecular docking and DFT calculations of 5-Phenylamino-isophthalic acid: A good NLO material. J. Mol. Struct. 2022, 1261, 132791. [Google Scholar] [CrossRef]
  16. Manhas, F.M.; Fatima, A.; Verma, I.; Siddiqui, N.; Muthu, S.; AlSalem, H.S.; Savita, S.; Singh, M.; Javed, S. Quantum computational, spectroscopic (FT-IR, NMR and UV–Vis) profiling, Hirshfeld surface, molecular docking and dynamics simulation studies on pyridine-2,6-dicarbonyl dichloride. J. Mol. Struct. 2022, 1265, 133374. [Google Scholar] [CrossRef]
  17. Fatima, A.; Singh, M.; Abualnaja, K.M.; Althubeiti, K.; Muthu, S.; Siddiqui, N.; Javed, S. Experimental Spectroscopic, Structural (Monomer and Dimer), Molecular Docking, Molecular Dynamics Simulation and Hirshfeld Surface Analysis of 2-Amino-6-Methylpyridine. Polycycl. Aromat. Compd. 2022, 1–31. [Google Scholar] [CrossRef]
  18. Kumar, M.; Fatima, A.; Singh, M.; Verma, I.; Khanum, G.; Muthu, S.; Althubeiti, K.; Abualnaja, K.M.; Ahmad, M.; Siddiqui, N.; et al. Experimental Spectroscopic, Quantum Computational, Hirshfeld Surface, Molecular Docking, and Electronic Excitation Studies on an Antibiotic Agent: SDZ. Polycycl. Aromat. Compd. 2022, 1–25. [Google Scholar] [CrossRef]
  19. Sharma, A.; Fatima, A.; Malla, M.A.; Khanum, G.; Kumar, A.; Singh, M.; Abualnaja, K.M.; Althubeiti, K.; Muthu, S.; Siddiqui, N.; et al. Molecular Docking, Dynamic Simulation and DFT Approach to Noble “2-Hydrazinobenzothiazole” Compound. Polycycl. Aromat. Compd. 2022, 1–28. [Google Scholar] [CrossRef]
  20. Law, G.-L.; Szeto, L.; Wong, W.-T. 2,4-Dioxo-1,2,3,4-tetrahydropyrimidine-5-carboxylic acid monohydrate. Acta Cryst. 2004, E60, o1072–o1074. [Google Scholar] [CrossRef] [Green Version]
  21. Seiler, V.K.; Hützler, W.M.; Bolte, M. Eight new crystal structures of 5-(hy¬droxy¬meth¬yl)uracil, 5-carb¬oxy¬uracil and 5-carb¬oxy-2-thio-uracil: Insights into the hydrogen-bonded networks and the predominant conformations of the C5-bound residues. Acta Crystallogr. C Struct. Chem. 2016, 72, 379. [Google Scholar] [CrossRef]
  22. Stewart, R.F.; Jensen, L.H. Redetermination of the crystal structure of uracil. Acta Crystallogr. 1967, 23, 1102–1105. [Google Scholar] [CrossRef]
  23. Long, D.A. Infrared and Raman Characteristic Group Frequencies: Tables and Charts, 3rd ed.; Socrates, S., Ed.; John Wiley and Sons, Ltd.: Chichester, UK, 2001. [Google Scholar]
  24. Jennings, K.R. Spectrometric Identification of Organic Compounds, 5th ed.; Silverstein, R.M., Bassler, G.C., Morrill, T.C., Eds.; Wiley: New York, NY, USA, 1991; 430p. [Google Scholar]
  25. Sameut, A.; Zanndouche, S.Y.; Boumaza, C.; Dikes, C.; Ziani, B.E.C. Chemical Synthesis and Hemi-Synthesis of Novel Benzimidazole Derivatives Using Microwave-Assisted Process: Chemical Characterization, Bioactivities and Molecular Docking. Chem. Proc. 2021, 3, 71. [Google Scholar]
  26. Fuson, N.; Josien, M.-L. Infrared Spectra of Polynuclear Aromatic Compounds. I. 1,2-Benzanthracene, the Monomethyl-1,2-benzanthracenes and Some Dimethyl-1,2-benzanthracenes 1. J. Am. Chem. Soc. 1956, 78, 3049–3060. [Google Scholar] [CrossRef]
  27. Barnes, A.J.; Majid, M.A.; Stuckey, M.A.; Gregory, P.; Stead, C.V. The resonance Raman spectra of Orange II and Para Red: Molecular structure and vibrational assignment. Spectrochim. Acta Part A Mol. Spectrosc. 1985, 41, 629–635. [Google Scholar] [CrossRef]
  28. Nerli, S.; McShan, A.C.; Sgourakis, N.G. Chemical shift-based methods in NMR structure determination. Prog. Nucl. Magn. Reson. Spectrosc. 2018, 106–107, 1–25. [Google Scholar] [CrossRef] [PubMed]
  29. Armaković, S.; Armaković, S.J. Atomistica.online—Web application for generating input files for ORCA molecular modelling package made with the Anvil platform. Mol. Simul. 2022, 49, 117–123. [Google Scholar] [CrossRef]
  30. Spackman, M.A.; Jayatilaka, D. Hirshfeld surface analysis. CrystEngComm 2009, 11, 19–32. [Google Scholar] [CrossRef]
  31. Ashfaq, M.; Tahir, M.N.; Kuznetsov, A.; Mirza, S.H.; Khalid, M.; Ali, A. DFT and single crystal analysis of the pyrimethamine-based novel co-crystal salt: 2,4-diamino-5-(4-chloro-phenyl)-6-ethylpyrimidin-1-ium:4-hydroxybenzoate:methanol:hydrate (1:1:1:1) (DEHMH). J. Mol. Struct. 2020, 1199, 127041. [Google Scholar] [CrossRef]
  32. Tahir, M.N.; Ashfaq, M.; de la Torre, A.F.; Caballero, J.; Hernández-Rodríguez, E.W.; Ali, A. Rationalizing the stability and interactions of 2,4-diamino-5-(4-chlorophenyl)-6-ethylpyrimidin-1-ium 2-hydroxy-3,5-dinitrobenzoate salt. J. Mol. Struct. 2019, 1193, 185–194. [Google Scholar] [CrossRef]
  33. Ali, A.; Zukerman-Schpector, J.; Weber Paixão, M.; Jotani, M.M.; Tiekink, E.R.T. 7-Methyl-5-[(4-methylbenzene)sulfonyl]-2H,5H-[1,3]dioxolo[4,5-f]indole: Crystal structure and Hirshfeld analysis. Acta Crystallogr. Sect. E Crystallogr. Commun. 2018, 74, 184–188. [Google Scholar] [CrossRef] [Green Version]
  34. Ali, A.; Khalid, M.; ur Rehman, M.F.; Haq, S.; Ali, A.; Tahir, M.N.; Ashfaq, M.; Rasool, F.; Braga, A.A.C. Efficient Synthesis, SC-XRD, and Theoretical Studies of O-Benzenesulfonylated Pyrimidines: Role of Noncovalent Interaction Influence in Their Supramolecular Network. ACS Omega 2020, 5, 15115–15128. [Google Scholar] [CrossRef]
  35. Ashfaq, M.; Bogdanov, G.; Ali, A.; Tahir, M.N.; Abdullah, S. Pyrimethamine-Based Novel Co-Crystal Salt: Synthesis, Single-Crystal Investigation, Hirshfeld surface analysis and DFT inspection of the 2,4-diamino-5-(4-chlorophenyl)-6-ethylpyrimidin-1-ium 2,4-dichlorobenzoate (1:1) (DECB). J. Mol. Struct. 2021, 1235, 130215. [Google Scholar] [CrossRef]
  36. Taia, A.; Ibrahimi, B.E.; Benhiba, F.; Ashfaq, M.; Tahir, M.N.; Essaber, M.; Aatif, A.; Hökelek, T.; Mague, J.T.; Sebbar, N.K.; et al. Syntheses, single crystal X-ray structure, Hirshfeld surface analyses, DFT computations and Monte Carlo simulations of New Eugenol derivatives bearing 1,2,3-triazole moiety. J. Mol. Struct. 2021, 1234, 130189. [Google Scholar] [CrossRef]
  37. Ali, A.; Khalid, M.; Tahir, M.N.; Imran, M.; Ashfaq, M.; Hussain, R.; Assiri, M.A.; Khan, I. Synthesis of Diaminopyrimidine Sulfonate Derivatives and Exploration of Their Structural and Quantum Chemical Insights via SC-XRD and the DFT Approach. ACS Omega 2021, 6, 7047–7057. [Google Scholar] [CrossRef] [PubMed]
  38. Muthu, S.; Renuga, S. Vibrational spectra and normal coordinate analysis of 2-hydroxy-3-(2-methoxyphenoxy) propyl carbamate. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2014, 132, 313–325. [Google Scholar] [CrossRef]
  39. Spackman, M.A.; Byrom, P.G. A novel definition of a molecule in a crystal. Chem. Phys. Lett. 1997, 267, 215–220. [Google Scholar] [CrossRef]
  40. Gadre, S.R.; Babu, K.; Rendell, A.P. Electrostatics for Exploring Hydration Patterns of Molecules. 3. Uracil. J. Phys. Chem. A 2000, 104, 8976–8982. [Google Scholar] [CrossRef]
  41. Prabavathi, N.; Nilufer, A.; Krishnakumar, V. Vibrational spectroscopic (FT-IR and FT-Raman) studies, natural bond orbital analysis and molecular electrostatic potential surface of Isoxanthopterin. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2013, 114, 101–113. [Google Scholar] [CrossRef]
  42. Poater, J.; Duran, M.; Solà, M.; Silvi, B. Theoretical Evaluation of Electron Delocalization in Aromatic Molecules by Means of Atoms in Molecules (AIM) and Electron Localization Function (ELF) Topological Approaches. Chem. Rev. 2005, 105, 3911–3947. [Google Scholar] [CrossRef]
  43. Hermann, J.P.; Ricard, D.; Ducuing, J. Optical nonlinearities in conjugated systems: β-carotene. Appl. Phys. Lett. 1973, 23, 178–180. [Google Scholar] [CrossRef]
  44. Ishow, E.; Bellaïche, C.; Bouteiller, L.; Nakatani, K.; Delaire, J.A. Versatile Synthesis of Small NLO-Active Molecules Forming Amorphous Materials with Spontaneous Second-Order NLO Response. J. Am. Chem. Soc. 2003, 125, 15744–15745. [Google Scholar] [CrossRef] [PubMed]
  45. Reed, A.E.; Curtiss, L.A.; Weinhold, F. Intermolecular interactions from a natural bond orbital, donor-acceptor viewpoint. Chem. Rev. 1988, 88, 899–926. [Google Scholar] [CrossRef]
  46. Tobey, S.W.; West, R. Pentachlorocyclopropane 1. J. Am. Chem. Soc. 1966, 88, 2478–2481. [Google Scholar] [CrossRef]
  47. Sekino, H.; Bartlett, R.J. Frequency dependent nonlinear optical properties of molecules. J. Chem. Phys. 1986, 85, 976–989. [Google Scholar] [CrossRef]
  48. Kosar, B.; Albayrak, C. Spectroscopic investigations and quantum chemical computational study of (E)-4-methoxy-2-[(p-tolylimino)methyl]phenol. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2011, 78, 160–167. [Google Scholar] [CrossRef]
  49. Zhang, Z.; Li, D.; Jiang, W.; Wang, Z. The electron density delocalization of hydrogen bond systems. Adv. Phys. X 2018, 3, 1428915. [Google Scholar] [CrossRef] [Green Version]
  50. Al-Ahmary, K.M.; Habeeb, M.M.; Aljahdali, S.H. Synthesis, spectroscopic studies and DFT/TD-DFT/PCM calculations of molecular structure, spectroscopic characterization and NBO of charge transfer complex between 5-amino-1,3-dimethylpyrazole (5-ADMP) with chloranilic acid (CLA) in different solvents. J. Mol. Liq. 2019, 277, 453–470. [Google Scholar] [CrossRef]
  51. Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 09, Revision A.02; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
  52. Parr, R.G.; Yang, W. Density functional approach to the frontier-electron theory of chemical reactivity. J. Am. Chem. Soc. 1984, 106, 4049–4050. [Google Scholar] [CrossRef]
  53. Karabacak, M.; Asiri, A.M.; Al-Youbi, A.O.; Qusti, A.H.; Cinar, M. Identification of structural and spectral features of synthesized cyano-stilbene dye derivatives: A comparative experimental and DFT study. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2014, 120, 144–150. [Google Scholar] [CrossRef]
  54. Hughbanks, T.; Hoffmann, R. Chains of trans-edge-sharing molybdenum octahedra: Metal-metal bonding in extended systems. J. Am. Chem. Soc. 1983, 105, 3528–3537. [Google Scholar] [CrossRef]
  55. Miller, G.J. Solids and Surfaces: A Chemist’s View of Bonding in Extended Structures; VonR. Hoffmann. VCH Publishers: New York, NY, USA; VCH Verlagsgesellschaft: Weinheim, Germany, 1988. [Google Scholar]
  56. Balachandran, V.; Karpagam, V.; Revathi, B.; Kavimani, M.; Santhi, G. Conformational stability, vibrational and NMR analysis, chemical potential and thermodynamical parameter of 3-tert-butyl-4-hydroxyanisole. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2015, 135, 1039–1051. [Google Scholar] [CrossRef] [PubMed]
  57. Saral, A.; Sudha, P.; Muthu, S.; Sevvanthi, S.; Sangeetha, P.; Selvakumari, S. Vibrational spectroscopy, quantum computational and molecular docking studies on 2-chloroquinoline-3-carboxaldehyde. Heliyon 2021, 7, e07529. [Google Scholar] [CrossRef] [PubMed]
  58. Lu, T.; Chen, F. Multiwfn: A multifunctional wavefunction analyzer. J. Comput. Chem. 2012, 33, 580–592. [Google Scholar] [CrossRef]
  59. Roy, D.R.; Parthasarathi, R.; Maiti, B.; Subramanian, V.; Chattaraj, P.K. Electrophilicity as a possible descriptor for toxicity prediction. Bioorg. Med. Chem. 2005, 13, 3405–3412. [Google Scholar] [CrossRef]
  60. Shahraki, S.; Saeidifar, M.; Shiri, F.; Heidari, A. Assessment of the interaction procedure between Pt(IV) prodrug [Pt(5,5′-dmbpy)Cl 4 and human serum albumin: Combination of spectroscopic and molecular modeling technique. J. Biomol. Struct. Dyn. 2017, 35, 3098–3106. [Google Scholar] [CrossRef]
  61. Ben Issa, T.; Sagaama, A.; Issaoui, N. Computational study of 3-thiophene acetic acid: Molecular docking, electronic and intermolecular interactions investigations. Comput. Biol. Chem. 2020, 86, 107268. [Google Scholar] [CrossRef]
  62. Zhang, R.; Du, B.; Sun, G.; Sun, Y. Experimental and theoretical studies on o-, m- and p-chlorobenzylideneaminoantipyrines. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2010, 75, 1115–1124. [Google Scholar] [CrossRef]
  63. Ruszkowski, M.; Sliwiak, J.; Ciesielska, A.; Barciszewski, J.; Sikorski, M.; Jaskolski, M. Specific binding of gibberellic acid by Cytokinin-Specific Binding Proteins: A new aspect of plant hormone-binding proteins with the PR-10 fold. Acta Crystallogr. Sect. D Biol. Crystallogr. 2014, 70, 2032–2041. [Google Scholar] [CrossRef]
  64. Eberhardt, J.; Santos-Martins, D.; Tillack, A.F.; Forli, S. AutoDock Vina 1.2.0: New Docking Methods, Expanded Force Field, and Python Bindings. J. Chem. Inf. Model. 2021, 61, 3891–3898. [Google Scholar] [CrossRef]
  65. Trott, O.; Olson, A.J. AutoDock Vina: Improving the speed and accuracy of docking with a new scoring function, efficient optimization, and multithreading. J. Comput. Chem. 2010, 31, 455–461. [Google Scholar] [CrossRef] [Green Version]
  66. Lipinski, C.A.; Lombardo, F.; Dominy, B.W.; Feeney, P.J. Experimental and computational approaches to estimate solubility and permeability in drug discovery and development settings. Adv. Drug Deliv. Rev. 1997, 23, 3–25. [Google Scholar] [CrossRef]
  67. Lipinski, C.A. Lead- and drug-like compounds: The rule-of-five revolution. Drug Discov. Today Technol. 2004, 1, 337–341. [Google Scholar] [CrossRef] [PubMed]
  68. Ghose, A.K.; Viswanadhan, V.N.; Wendoloski, J.J. A Knowledge-Based Approach in Designing Combinatorial or Medicinal Chemistry Libraries for Drug Discovery. 1. A Qualitative and Quantitative Characterization of Known Drug Databases. J. Comb. Chem. 1999, 1, 55–68. [Google Scholar] [CrossRef] [PubMed]
  69. Bekker, H.; Berendsen, H.J.C.; Dijkstra, E.J.; Achterop, S.; van Drunen, R.; van der Spoel, D.; Sijbers, A.; Keegstra, H.; Renardus, M.K.R. Gromacs: A parallel computer for molecular dynamics simulations. In Physics Computing 92, Proceedings of the 4th International Conference, Prague, Czech Republic, 24–28 August 1992; de Groot, R.A., Nadrchal, J., Eds.; World Scientific: Singapore, 1993; pp. 252–256. [Google Scholar]
  70. Jorgensen, W.L.; Chandrasekhar, J.; Madura, J.D. Comparison of simple potential functions for simulating liquid water. J. Chem. Phys. 1983, 79, 926–935. [Google Scholar] [CrossRef]
  71. Petersson, G.A.; Al-Laham, M.A. A complete basis set model chemistry. II. Open-shell systems and the total energies of the first-row atoms. J. Chem. Phys. 1991, 94, 6081–6090. [Google Scholar] [CrossRef]
  72. Petersson, G.A.; Bennett, A.; Tensfeldt, T.G.; Al-Laham, M.A.; Shirley, W.A.; Mantzaris, J. A complete basis set model chemistry. I. The total energies of closed-shell atoms and hydrides of the first-row elements. J. Chem. Phys. 1988, 89, 2193–2218. [Google Scholar] [CrossRef]
  73. Neese, F. The ORCA program system. WIREs Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
  74. Jamróz, M.H. Vibrational Energy Distribution Analysis (VEDA): Scopes and limitations. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2013, 114, 220–230. [Google Scholar] [CrossRef]
  75. Safin, D.A.; Robeyns, K.; Garcia, Y. 1,2,4-Triazole-based molecular switches: Crystal structures, Hirshfeld surface analysis and optical properties. CrystEngComm 2016, 18, 7284–7296. [Google Scholar] [CrossRef]
  76. Wang, W.; Ling, Y.; Yang, L.-J.; Liu, Q.-L.; Luo, Y.-H.; Sun, B.-W. Crystals of 4-(2-benzimidazole)-1,2,4-triazole and its hydrate: Preparations, crystal structure and Hirshfeld surfaces analysis. Res. Chem. Intermed. 2016, 42, 3157–3168. [Google Scholar] [CrossRef]
  77. Shyamapada, S.; Christoph, M.; Mitra, S. Synthesis, Crystal structure, and Hirshfeld Surface Analysis of a New Mixed Ligand Copper(II) Complex. Acta Chim. Slov. 2016, 63, 129–137. [Google Scholar] [CrossRef] [PubMed]
  78. Chohan, Z.H.; Youssoufi, M.H.; Jarrahpour, A.; Ben Hadda, T. Identification of antibacterial and antifungal pharmacophore sites for potent bacteria and fungi inhibition: Indolenyl sulfonamide derivatives. Eur. J. Med. Chem. 2010, 45, 1189–1199. [Google Scholar] [CrossRef] [PubMed]
  79. Ben M’leh, C.; Brandán, S.A.; Issaoui, N.; Roisnel, T.; Marouani, H. Synthesis, molecular structure, vibrational and theoretical studies of a new non-centrosymmetric organic sulphate with promising NLO properties. J. Mol. Struct. 2018, 1171, 771–785. [Google Scholar] [CrossRef]
  80. Pettersen, E.F.; Goddard, T.D.; Huang, C.C.; Couch, G.S.; Greenblatt, D.M.; Meng, E.C.; Ferrin, T.E. UCSF Chimera, A visualization system for exploratory research and analysis. J. Comput. Chem. 2004, 25, 1605–1612. [Google Scholar] [CrossRef] [Green Version]
  81. Daina, A.; Michielin, O.; Zoete, V. SwissADME: A free web tool to evaluate pharmacokinetics, drug-likeness and medicinal chemistry friendliness of small molecules. Sci. Rep. 2017, 7, 42717. [Google Scholar] [CrossRef] [Green Version]
  82. Yang, W.; Mortier, W.J. The use of global and local molecular parameters for the analysis of the gas-phase basicity of amines. J. Am. Chem. Soc. 1986, 108, 5708–5711. [Google Scholar] [CrossRef]
Molecules 28 02116 sch001 550
Scheme 1. Uracil and its derivatives.
Scheme 1. Uracil and its derivatives.
Molecules 28 02116 sch001
Molecules 28 02116 g001 550
Figure 1. (A) The optimized molecular structure of 5-HMU, (B) its trimer, (C) dimer 1, (D) dimer 2.
Figure 1. (A) The optimized molecular structure of 5-HMU, (B) its trimer, (C) dimer 1, (D) dimer 2.
Molecules 28 02116 g001
Molecules 28 02116 g002 550
Figure 2. Experimental and computational spectra of (A) FT-IR, and (B) FT-Raman.
Figure 2. Experimental and computational spectra of (A) FT-IR, and (B) FT-Raman.
Molecules 28 02116 g002
Molecules 28 02116 g003 550
Figure 3. (A) Experimental 1H and (B) 13C NMR of 5-HMU in DMSO-d6 as a solvent.
Figure 3. (A) Experimental 1H and (B) 13C NMR of 5-HMU in DMSO-d6 as a solvent.
Molecules 28 02116 g003
Molecules 28 02116 g004 550
Figure 4. Hirshfeld surface for 5-HMU: mapped with dnorm (A), di (B), de (C), shape index (D) curvedness (E), Fragment patch (F).
Figure 4. Hirshfeld surface for 5-HMU: mapped with dnorm (A), di (B), de (C), shape index (D) curvedness (E), Fragment patch (F).
Molecules 28 02116 g004
Molecules 28 02116 g005 550
Figure 5. MEP pattern of 5-HMU, (A) For Monomer (B) For Trimer.
Figure 5. MEP pattern of 5-HMU, (A) For Monomer (B) For Trimer.
Molecules 28 02116 g005
Molecules 28 02116 g006 550
Figure 6. ELF (A) Color filled map, (B) Shaded surface map with projection of 5-HMU.
Figure 6. ELF (A) Color filled map, (B) Shaded surface map with projection of 5-HMU.
Molecules 28 02116 g006
Molecules 28 02116 g007 550
Figure 7. UV–Vis spectra of 5-HMU: Theoretical in the gas phase, DMSO, and Methanol and Experimental in different solvents and experimental in DMSO.
Figure 7. UV–Vis spectra of 5-HMU: Theoretical in the gas phase, DMSO, and Methanol and Experimental in different solvents and experimental in DMSO.
Molecules 28 02116 g007
Molecules 28 02116 g008 550
Figure 8. HOMO–LUMO diagrams with band gap energy of 5-HMU.
Figure 8. HOMO–LUMO diagrams with band gap energy of 5-HMU.
Molecules 28 02116 g008
Molecules 28 02116 g009 550
Figure 9. EDD and HDD maps of the excited state of 5-HMU in (A) DMSO and (B) methanol.
Figure 9. EDD and HDD maps of the excited state of 5-HMU in (A) DMSO and (B) methanol.
Molecules 28 02116 g009
Molecules 28 02116 g010 550
Figure 10. Ligand 5-HMU: embedded in the active sites of (A) 4P6T, (B) 4P6S, (C) 6QXD, (D) 6EI4, (E) 5OAE, and (F) 5M67 proteins.
Figure 10. Ligand 5-HMU: embedded in the active sites of (A) 4P6T, (B) 4P6S, (C) 6QXD, (D) 6EI4, (E) 5OAE, and (F) 5M67 proteins.
Molecules 28 02116 g010
Molecules 28 02116 g011 550
Figure 11. (A) Root mean square deviation plot, and (B) Hydrogen bond formations.
Figure 11. (A) Root mean square deviation plot, and (B) Hydrogen bond formations.
Molecules 28 02116 g011
Table
Table 1. (A) Optimized geometrical parameters of 5-HMU showing bond length (Å); and bond angles (°). (B) Optimized geometrical parameters showing bond length (pm) and bond angle (°) of the trimer.
Table 1. (A) Optimized geometrical parameters of 5-HMU showing bond length (Å); and bond angles (°). (B) Optimized geometrical parameters showing bond length (pm) and bond angle (°) of the trimer.
(A)
Bond length (Å)Bond angle (˚)
ParameterB3LYP/6-311++G(d,p)ExperimentalParameterB3LYP/6-311++G(d,p)Experimental
C1-N21.391.35C1-N2-H11120.93117.15
C1-N61.381.36C3-N2-H11122.36120.83
C1-O81.211.23N2-C3-C4116.13122.70
N2-C31.371.37N2-C3-H12121.50118.62
N2-H111.000.87C4-C3-H12118.77118.66
C3-C41.341.35C3-C4-C5122.99118.01
C3-H121.080.95C3-C4-C9118.21122.87
C4-C51.461.45C5-C4-C9114.34119.09
C4-C91.511.50C4-C5-6125.20115.13
C5-N61.401.38C4-C5-O7120.45124.63
C5-O71.211.23N6-C5-O7128.00120.23
N6-H131.010.89C1-N6-C5115.70126.54
C9-O101.421.40C1-N6-H13116.28117.05
C9-H141.090.98C5-N6-H13112.81116.36
C9-H151.090.99C4-C9-O10109.30108.30
O10-H160.960.84C4-C9-H1C4109.94110.07
Bond angle (˚)C4-C9-H15111.89110.01
N2-C1-N6112.66115.48O10-C9-H14106.07110.04
N2-C1-O8123.22122.75O10-C9-H15106.58110.02
6-C1-O8124.11121.76H14-C9-H15108.72108.38
C1-N2-C3115.22122.01C9-O10-H16108.72109.83
R20.97 R20.91
(B)
Bond length Bond angle
ParameterB3LYP/6-311++G(d,p) ParameterB3LYP/6-311++G(d,p)
O17-H21.81 N1-H2-O17174.17
O5-H181.78 N22-H18-O5174.54
O12-H201.84 N24-H20-O12170.45
O19-H91.84 N6-H9-O19170.46
N22-C211.37 C21-O17-H2125.56
C21-O171.23 C3-O5-H18126.78
N22-H181.03 C23-O19-H9132.13
Table
Table 2. Calculated vibrational frequencies (cm−1) assignments of 5-HMU.
Table 2. Calculated vibrational frequencies (cm−1) assignments of 5-HMU.
Mode NoExperimentalTheoreticalIIRIRAMANAssignments (PED)
FT-IRFT-RamanUnscaledScaled
423651 38363686548γOH(100)
413491 363734951369γNH(50)
40 35963455954γNH(50)
393078306832083083151γCH(99)
382960298030742954261γCH(94)
37 2917303829193100γCH(95)
36 17321801173110017γOC(48) + βHNC(10)
3516581694174816798434γOC(44)
34 16921626726γCC(65) + βHCC(11)
331458 1503144426γNC(14) + βHNC(16) + βHCH(27)
321421143214971438416γNC(10) + βHNC(11) + βHCH(52)
31 13651425136911γNC(15) + βHNC(15) + βHCC(11)
30135713571409135441γNC(16) + βHNC(46)
29 1399134519γHC(10) + βHNC(11) + τHCCC(42)
28 1374132075βHOC(42) + βHCO(32)
271215126913421290312γCC(15) + βHCC(43)
26 11751213116673γNC(25) + γCC(23)
251151 1201115424βHOC(40) + βHCO(36) + τHCCC(11)
24 114811881142122γNC(24) + βHNC(14) +βHCC(19)
23104710901136109111γNC(27) + γCC(11) + γOC(13)
221013103510441003104γOC(72)
21 94598494551βHOC(10) + τHCCC(43)
20906 97593712γNC(13) + βCNC(23)
1980089892689031τHCCC(65)
1876179179976812γCC(28) + βCNC(12) + βCCN(10)
17 776746112γNC(34)
16 75872823τOCCC(30)
15 75572651τOCCC(10)
14659654668641111τHNCC(66)
13 63661123τHNCC(24)
1255953656354171τHNCC(69)
1152051454652523γNC(10) + βONC(25) + βCNC(29) + βCNC(10)
10451 52049931βCNC(12) + βNCN(32) + βOCC(22)
9 40739132βOCN(37) + βCNC(11)
8 39838231τCCNC(42)
7 33432122βCNC(12) + βOCN(16) + βCCN(14) + βNCN(10) + βOCC(15)
6 28227141τHOCC(20)
5 245235141τHOCC(75)
4 18217511βCCC(63) + βOCC(19)
3 14714111τCNCN(77)
2 11010611τCCNC(18) + τCNCN(58)
1 666421βCNCN(13) + βOCCC(67)
Table
Table 3. Experimental and theoretical 13C and 1H chemical shift values (ppm) of 5-HMU in DMSO.
Table 3. Experimental and theoretical 13C and 1H chemical shift values (ppm) of 5-HMU in DMSO.
AtomsExperimentalCalculated (B3LYP/6-311++G(d,p))Calculated (aug-cc-pV5Z def2/J)
C5164.31165.946151.95
C1151.85153.5959128.18
C3138.71141.7179102.59
C4113.22120.205670.18
C956.3162.234761.45
H1211.017.385719.54
H1310.667.0618.46
H117.206.75896.43
H144.814.62876.43
H154.064.26365.03
H163.330.53783.19
Table
Table 4. The calculated dipole moment μ(D), polarizability (α;0), and first-order hyperpolarizability (βtot) components of 5-HMU.
Table 4. The calculated dipole moment μ(D), polarizability (α;0), and first-order hyperpolarizability (βtot) components of 5-HMU.
ParametersB3LYP/6-311++G(d,p)
(5-HMU)		B3LYP
(Urcail)		ParametersB3LYP/6-311++G(d,p)
μx−1.4356 βxxx−41.6733
μy−3.0482 βyxx21.8308
μz−0.989 βxyy19.7825
μ(D)3.51151.7615βyyy−41.3496
αxx−71.175293.36βzxx−13.9748
αxy2.0043 βxyz−7.1467
αyy−58.188775.72βzyy−2.1224
αxz4.4834 βxzz−8.2926
αyz1.4686 βyzz−0.7765
αzz−55.604270.11βzzz−2.9145
α0 (e.s.u)−0.914 × 10−23 βtot (e.s.u)0.3545 × 10−30
Table
Table 5. Hydrogen bonding and molecular docking score of 5-HMU with protein targets.
Table 5. Hydrogen bonding and molecular docking score of 5-HMU with protein targets.
Protein (PDB ID)No of ResiduesBond Distance (Å)Inhibition Constant
(Micromolar)		Binding Energy (kcal/mol)Reference RMSD (Å)
4P6T32.092200.89−5.03.620
4P6S32.484101.67−5.43.690
6QXD32.27043.40−5.90.000
6EI432.54161.01−5.70.000
5OAE32.74761.01−5.72.256
5M6732.15661.01−5.74.035
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Kumar, M.; Jaiswar, G.; Afzal, M.; Muddassir, M.; Alarifi, A.; Fatima, A.; Siddiqui, N.; Ayub, R.; Abduh, N.A.Y.; Saeed, W.S.; et al. Quantum Computational, Spectroscopic (FT-IR, FT-Raman, NMR, and UV–Vis) Hirshfeld Surface and Molecular Docking-Dynamics Studies on 5-Hydroxymethyluracil (Monomer and Trimer). Molecules 2023, 28, 2116. https://doi.org/10.3390/molecules28052116

AMA Style

Kumar M, Jaiswar G, Afzal M, Muddassir M, Alarifi A, Fatima A, Siddiqui N, Ayub R, Abduh NAY, Saeed WS, et al. Quantum Computational, Spectroscopic (FT-IR, FT-Raman, NMR, and UV–Vis) Hirshfeld Surface and Molecular Docking-Dynamics Studies on 5-Hydroxymethyluracil (Monomer and Trimer). Molecules. 2023; 28(5):2116. https://doi.org/10.3390/molecules28052116

Chicago/Turabian Style

Kumar, Mohit, Gautam Jaiswar, Mohd. Afzal, Mohd. Muddassir, Abdullah Alarifi, Aysha Fatima, Nazia Siddiqui, Rashid Ayub, Naaser A. Y. Abduh, Waseem Sharaf Saeed, and et al. 2023. "Quantum Computational, Spectroscopic (FT-IR, FT-Raman, NMR, and UV–Vis) Hirshfeld Surface and Molecular Docking-Dynamics Studies on 5-Hydroxymethyluracil (Monomer and Trimer)" Molecules 28, no. 5: 2116. https://doi.org/10.3390/molecules28052116

APA Style

Kumar, M., Jaiswar, G., Afzal, M., Muddassir, M., Alarifi, A., Fatima, A., Siddiqui, N., Ayub, R., Abduh, N. A. Y., Saeed, W. S., & Javed, S. (2023). Quantum Computational, Spectroscopic (FT-IR, FT-Raman, NMR, and UV–Vis) Hirshfeld Surface and Molecular Docking-Dynamics Studies on 5-Hydroxymethyluracil (Monomer and Trimer). Molecules, 28(5), 2116. https://doi.org/10.3390/molecules28052116

Article Metrics

Back to TopTop