Assessment of Radio-Induced Damage in Endothelial Cells Irradiated with 40 kVp, 220 kVp, and 4 MV X-rays by Means of Micro and Nanodosimetric Calculations
Abstract
:1. Introduction
2. Results
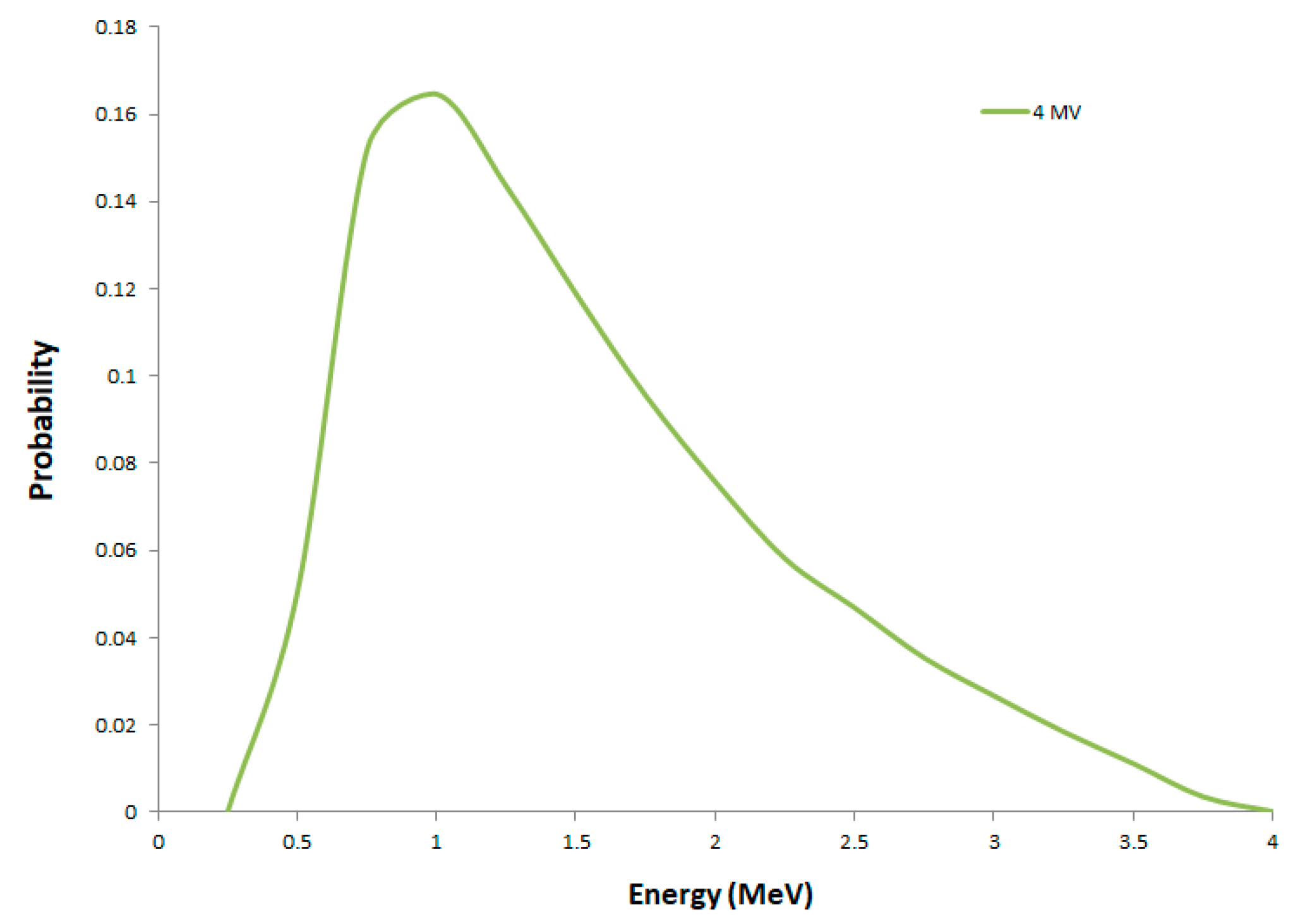
2.1. Secondary Electron Spectra
2.2. Microdosimetric Calculations
2.3. Yields of DSB/Gy/Gbp and DSB Complexity
2.4. Comparison between Simulated Results and Experimental Data
3. Discussion
4. Materials and Methods
4.1. Simulation of Experimental Cell Irradiations
4.1.1. Experimental Data on γ-H2AX Foci
4.1.2. SARRP Configuration
4.1.3. Medical Linear Accelerator Configuration
4.2. Phase Space of Secondary Electrons
4.3. Microdosimetric and Nanodosimetric Approaches
4.3.1. Microdosimetric Calculations in the Cell Population
4.3.2. Nanodosimetric Simulations for the Calculation of Double-Strand Breaks
Simulation Configuration
Computation Chain to Score Strand Breaks
Definition of a DSB and DSB Complexity
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gruel, G.; Villagrasa, C.; Voisin, P.; Clairand, I.; Benderitter, M.; Bottollier-Depois, J.F.; Barquinero, J.F. Cell to Cell Variability of Radiation-Induced Foci: Relation between Observed Damage and Energy Deposition. PLoS ONE 2016, 11, e0145786. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hunter, N.; Muirhead, C.R. Review of relative biological effectiveness dependence on linear energy transfer for low-LET radiations. J. Radiol. Prot. 2009, 29, 5–21. [Google Scholar] [CrossRef] [PubMed]
- Friedland, W.; Jacob, P.; Paretzke, H.G.; Stork, T. Monte Carlo Simulation of the Production of Short DNA Fragments by Low-Linear Energy Transfer Radiation Using Higher-Order DNA Models. Radiat. Res. 1998, 150, 170–182. [Google Scholar] [CrossRef] [PubMed]
- Friedland, W.; Jacob, P.; Paretzke, H.G.; Merzagora, M.; Ottolenghi, A. Simulation of DNA fragment distributions after irradiation with photons. Radiat Environ. Biophys. 1999, 38, 39–47. [Google Scholar] [CrossRef] [PubMed]
- Friedland, W.; Bernhardt, P.; Jacob, P.G.; Paretzke, H. Simulation of DNA Damage after Proton and Low LET Irradiation. Radiat. Prot. Dosim. 2002, 99, 99–102. [Google Scholar] [CrossRef]
- Göggelmann, W.; Jacobsen, C.; Panzer, W.; Walsh, L.; Roos, H.; Schmid, E. Re-evaluation of the RBE of 29 kV X-rays (mammography X-rays) relative to 220 kV X-rays using neoplastic transformation of human CGL1-hybrid cells. Radiat Environ. Biophys. 2003, 42, 175–182. [Google Scholar] [CrossRef] [PubMed]
- Brenner, D.J.; Amols, H.I. Enhanced risk from low-energy screen—Film mammography X rays. BJR 1989, 62, 910–914. [Google Scholar] [CrossRef]
- Frankenberg, D.; Brede, H.J.; Schrewe, U.J.; Steinmetz, C.; Frankenberg-Schwager, M.; Kasten, G.; Pralle, E. Induction of DNA double-strand breaks in mammalian cells and yeast. Adv. Space Res. 2000, 25, 2085–2094. [Google Scholar] [CrossRef]
- Frankenberg, D.; Kelnhofer, K.; Bär, K.; Frankenberg-Schwager, M. Enhanced Neoplastic Transformation by Mammography X Rays Relative to 200 kVp X Rays: Indication for a Strong Dependence on Photon Energy of the RBEM for Various End Points. Rare 2002, 157, 99–106. [Google Scholar] [CrossRef]
- Lehnert, A.; Lessmann, E.; Pawelke, J.; Dörr, W. RBE of 25 kV X-rays for the survival and induction of micronuclei in the human mammary epithelial cell line MCF-12A. Radiat Environ. Biophys. 2006, 45, 253–260. [Google Scholar] [CrossRef]
- Lehnert, A.; Lessmann, E.; Pawelke, J.; Dörr, W. RBE of 10 kV X rays determined for the human mammary epithelial cell line MCF-12A. Radiat Res. 2008, 169, 330–336. [Google Scholar] [CrossRef] [PubMed]
- Kühne, M.; Urban, G.; Frankenberg, D.; Löbrich, M. DNA Double-Strand Break Misrejoining after Exposure of Primary Human Fibroblasts to CK Characteristic X Rays, 29 kVp X Rays and 60Co γ Rays. Rare 2005, 164, 669–677. [Google Scholar] [CrossRef]
- Mestres, M.; Caballín, M.R.; Barrios, L.; Ribas, M.; Barquinero, J.F. RBE of X Rays of Different Energies: A Cytogenetic Evaluation by FISH. Radiat. Res. 2008, 170, 93–100. [Google Scholar] [CrossRef] [PubMed]
- Mestres, M.; Benkhaled, L.; Caballín, M.R.; Barrios, L.; Ribas, M.; Barquinero, J.F. Induction of Incomplete and Complex Chromosome Aberrations by 30 kVp X Rays. Rare 2010, 175, 201–208. [Google Scholar] [CrossRef]
- Schmid, E.; Regulla, D.; Kramer, H.M.; Harder, D. The Effect of 29 kV X Rays on the Dose Response of Chromosome Aberrations in Human Lymphocytes. Radiat. Res. 2002, 158, 771–777. [Google Scholar] [CrossRef] [Green Version]
- Schmid, E.; Krumrey, M.; Ulm, G.; Roos, H.; Regulla, D. The Maximum Low-Dose RBE of 17.4 and 40 keV Monochromatic X Rays for the Induction of Dicentric Chromosomes in Human Peripheral Lymphocytes. Radiat. Res. 2003, 160, 499–504. [Google Scholar] [CrossRef]
- Goodhead, D.T.; Nikjoo, H. Current Status of Ultrasoft X Rays and Track Structure Analysis as Tools for Testing and Developing Biophysical Models of Radiation Action. Radiat. Prot. Dosim. 1990, 31, 343–350. [Google Scholar] [CrossRef]
- Prise, K.M. A review of dsb induction data for varying quality radiations. Int. J. Radiat. Biol. 1998, 74, 173–184. [Google Scholar] [CrossRef]
- Kellerer, A.M. Electron Spectra and the RBE of X Rays. Rare 2002, 158, 13–23. [Google Scholar] [CrossRef]
- Nikjoo, H.; Lindborg, L. RBE of low energy electrons and photons. Phys. Med. Biol. 2010, 55, R65–R109. [Google Scholar] [CrossRef] [Green Version]
- Liang, Y.; Fu, Q.; Wang, X.; Liu, F.; Yang, G.; Luo, C.; Ouyang, Q.; Wang, Y. Relative biological effectiveness for photons: Implication of complex DNA double-strand breaks as critical lesions. Phys. Med. Biol. 2017, 62, 2153–2175. [Google Scholar] [CrossRef] [PubMed]
- Buch, T.; Scifoni, E.; Krämer, M.; Durante, M.; Scholz, M.; Friedrich, T. Modeling Radiation Effects of Ultrasoft X Rays on the Basis of Amorphous Track Structure. Radiat Res. 2018, 189, 32–43. [Google Scholar] [CrossRef] [PubMed]
- Hill, M.A. The variation in biological effectiveness of X-rays and gamma rays with energy. Radiat. Prot. Dosim. 2004, 112, 471–481. [Google Scholar] [CrossRef] [PubMed]
- Friedrich, T.; Durante, M.; Scholz, M. Simulation of DSB yield for high LET radiation. Radiat. Prot. Dosim. 2015, 166, 61–65. [Google Scholar] [CrossRef]
- Prise, K.M.; Pinto, M.; Newman, H.C.; Michael, B.D. A Review of Studies of Ionizing Radiation-Induced Double-Strand Break Clustering. Rare 2001, 156, 572–577. [Google Scholar] [CrossRef]
- Davis, A.J.; Chen, D.J. DNA double strand break repair via non-homologous end-joining. Transl. Cancer Res. 2013, 2, 130–143. [Google Scholar] [CrossRef]
- Ballarini, F.; Carante, M.P. Chromosome aberrations and cell death by ionizing radiation: Evolution of a biophysical model. Radiat. Phys. Chem. 2016, 128, 18–25. [Google Scholar] [CrossRef]
- Elsässer, T.; Scholz, M. Cluster effects within the local effect model. Radiat. Res. 2007, 167, 319–329. [Google Scholar] [CrossRef]
- Bernal, M.A.; de Almeida, C.E.; David, M.; Pires, E. Estimation of the RBE of mammography-quality beams using a combination of a Monte Carlo code with a B-DNA geometrical model. Phys. Med. Biol. 2011, 56, 7393–7403. [Google Scholar] [CrossRef]
- Freneau, A.; Dos Santos, M.; Voisin, P.; Tang, N.; Bueno Vizcarra, M.; Villagrasa, C.; Roy, L.; Vaurijoux, A.; Gruel, G. Relation between DNA double-strand breaks and energy spectra of secondary electrons produced by different X-ray energies. Int. J. Radiat. Biol. 2018, 9, 1–10. [Google Scholar] [CrossRef]
- Falk, M.; Lukasova, E.; Kozubek, S. Higher-order chromatin structure in DSB induction, repair and misrepair. Mutat. Res. 2010, 704, 88–100. [Google Scholar] [CrossRef] [PubMed]
- Rothkamm, K.; Horn, S. gamma-H2AX as protein biomarker for radiation exposure. Ann. Ist. Super. Sanita 2009, 45, 265–271. [Google Scholar] [PubMed]
- Tommasino, F.; Friedrich, T.; Jakob, B.; Meyer, B.; Durante, M.; Scholz, M. Induction and Processing of the Radiation-Induced Gamma-H2AX Signal and Its Link to the Underlying Pattern of DSB: A Combined Experimental and Modelling Study. PLoS ONE 2015, 10, e0129416. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Friedland, W.; Kundrát, P. Track structure based modelling of chromosome aberrations after photon and alpha-particle irradiation. Mutat. Res. Genet. Toxicol. Environ. Mutagen. 2013, 756, 213–223. [Google Scholar] [CrossRef] [PubMed]
- Meylan, S.; Incerti, S.; Karamitros, M.; Tang, N.; Bueno, M.; Clairand, I.; Villagrasa, C. Villagrasa. Simulation of early DNA damage after the irradiation of a fibroblast cell nucleus using Geant4-DNA. Sci. Rep. 2017, 7. [Google Scholar] [CrossRef]
- Agostinelli, S.; Allison, J.; Amako, K.A.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.D.; Axen, M.; Banerjee, S.; Barrand, G.; et al. Geant4—A simulation toolkit. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2003, 506, 250–303. [Google Scholar] [CrossRef] [Green Version]
- Allison, J.; Amako, K.; Apostolakis, J.E.A.; Araujo, H.A.A.H.; Dubois, P.A.; Asai Barrand, G.; Capra, R.; Chauvie, S.; Chytracek, R.; Cirrone, G.A. Geant4 developments and applications. IEEE Trans. Nucl. Sci. 2006, 53, 270–278. [Google Scholar] [CrossRef] [Green Version]
- Allison, J.; Amako, K.; Apostolakis, J.; Arce, P.; Asai, M.; Aso, T.; Bagli, E.; Bagulya, A.; Banerjee, S.; Barrand, G.; et al. Recent developments in Geant4. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2016, 835, 186–225. [Google Scholar] [CrossRef]
- Incerti, S.; Kyriakou, I.; Bernal, M.A.; Bordage, M.C.; Francis, Z.; Guatelli, S.; Ivanchenko, V.; Karamitros, M.; Lampe, N.; Lee, S.B.; et al. Geant4-DNA example applications for track structure simulations in liquid water: A report from the Geant4-DNA Project. Med. Phys. 2018, 6. [Google Scholar] [CrossRef] [Green Version]
- Bernal, M.A.; Bordage, M.C.; Brown, J.M.C.; Davídková, M.; Delage, E.; El Bitar, Z.; Enger, S.A.; Francis, Z.; Guatelli, S.; Ivanchenko, V.N.; et al. Track structure modeling in liquid water: A review of the Geant4-DNA very low energy extension of the Geant4 Monte Carlo simulation toolkit. Phys. Med. 2015, 31, 861–874. [Google Scholar] [CrossRef]
- Incerti, S.; Ivanchenko, A.; Karamitros, M.; Mantero, A.; Moretto, P.; Tran, H.N.; Mascialino, B.; Champion, C.; Ivanchenko, V.N.; Bernal, M.A.; et al. Comparison of GEANT4 very low energy cross section models with experimental data in water. Med. Phys. 2010, 37, 4692–4708. [Google Scholar] [CrossRef] [PubMed]
- Incerti, S.; Baldacchino, G.; Bernal, M.; Capra, R.; Champion, C.; Francis, Z.; Guèye, A.; Mantero, B.; Mascialino, P.; Moretto, P.; et al. THE GEANT4-DNA PROJECT. Int. J. Model. Simul. Sci. Comput. 2010, 1, 157–178. [Google Scholar] [CrossRef]
- Villegas, F.; Tilly, N.; Ahnesjö, A. Microdosimetric spread for cell-sized targets exposed to 60Co, 192Ir and 125I sources. Radiat. Prot. Dosim. 2015, 166, 365–368. [Google Scholar] [CrossRef] [PubMed]
- Stewart, R.D.; Yu, V.K.; Georgakilas, A.G.; Koumenis, C.; Park, J.H.; Carlson, D.J. Effects of radiation quality and oxygen on clustered DNA lesions and cell death. Radiat. Res. 2011, 176, 587–602; [Google Scholar] [CrossRef] [PubMed]
- Meylan, S.; Vimont, U.; Incerti, S.; Clairand, I.; Villagrasa, C. Geant4-DNA simulations using complex DNA geometries generated by the DnaFabric tool. Comput. Phys. Commun. 2016, 204, 159–169. [Google Scholar] [CrossRef]
- Tang, N.; Bueno, M.; Meylan, S.; Incerti, S.; Tran, H.N.; Vaurijoux, A.; Gruel, G.; Villagrasa, C. Influence of chromatin compaction on simulated early radiation-induced DNA damage using Geant4-DNA. Med. Phys. 2019, 46, 1501–1511. [Google Scholar] [CrossRef] [Green Version]
- Villagrasa, C.; Meylan, S.; Gonon, G.; Gruel, G.; Giesen, U.; Bueno, M.; Rabus, H. Geant4-DNA simulation of DNA damage caused by direct and indirect radiation effects and comparison with biological data. EPJ. Web. Conf. 2017, 153, 04019. [Google Scholar] [CrossRef] [Green Version]
- Georgakilas, A.G.; O’Neill, P.; Stewart, R.D. Induction and repair of clustered DNA lesions: What do we know so far? Radiat. Res. 2013, 180, 100–109. [Google Scholar] [CrossRef]
- Sutherland, B.M.; Bennett, P.V.; Sutherland, J.C.; Laval, J. Clustered DNA damages induced by x rays in human cells. Radiat. Res. 2002, 157, 611–616. [Google Scholar] [CrossRef]
- Hada, M.; Sutherland, B.M. Spectrum of complex DNA damages depends on the incident radiation. Radiat. Res. 2006, 165, 223–230. [Google Scholar] [CrossRef]
- Hada, M.; Georgakilas, A.G. Formation of clustered DNA damage after high-LET irradiation: A review. J. Radiat. Res. 2008, 49, 203–210. [Google Scholar] [CrossRef] [PubMed]
- Barbieri, S.; Baiocco, G.; Babini, G.; Morini, J.; Friedland, W.; Buonanno, M.; Grilj, V.; Brenner, D.J.; Ottolenghi, A. Modelling γ-H2AX foci induction to mimic limitations in the scoring technique. Radiat. Prot. Dosim. 2019, 183, 121–125. [Google Scholar] [CrossRef] [PubMed]
- Gonon, G.; Villagrasa, C.; Voisin, P.; Meylan, S.; Bueno, M.; Benadjaoud, M.A.; Tang, N.; Langner, F.; Rabus, H.; Barquinero, J.-F.; et al. From Energy Deposition of Ionizing Radiation to Cell Damage Signaling: Benchmarking Simulations by Measured Yields of Initial DNA Damage after Ion Microbeam Irradiation. Radiat. Res. 2019, 191, 566–584. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kirkby, C.; Ghasroddashti, E.; Poirier, Y.; Tambasco, M.; Stewart, R.D. RBE of kV CBCT radiation determined by Monte Carlo DNA damage simulations. Phys. Med. Biol. 2013, 58, 5693–5704. [Google Scholar] [CrossRef] [PubMed]
- Bistrović, M.; Bisćan, M.; Viculin, T. RBE of 20 kV and 70 kV X-rays determined for survival of V 79 cells. Radiother. Oncol. 1986, 7, 175–180. [Google Scholar] [CrossRef]
- Hoshi, M.; Antoku, S.; Nakamura, N.; Russell, W.J.; Miller, R.C.; Sawada, S.; Mizuno, M.; Nishio, S. Soft X-ray dosimetry and RBE for survival of Chinese hamster V79 cells. Int. J. Radiat. Biol. 1988, 54, 577–591. [Google Scholar] [CrossRef]
- Spadinger, I.; Palcic, B. The relative biological effectiveness of 60Co gamma-rays, 55 kVp X-rays, 250 kVp X-rays, and 11 MeV electrons at low doses. Int. J. Radiat. Biol. 1992, 61, 345–353. [Google Scholar] [CrossRef]
- Sinclair, W.K. The relative biological effectiveness of 22-Mevp X-rays, cobalt-60 gamma rays, and 200-Kvcp X-rays. VII. Summary of studies for five criteria of effect. Radiat. Res. 1962, 16, 394–398. [Google Scholar] [CrossRef]
- Schuemann, J.; McNamara, A.L.; Warmenhoven, J.W.; Henthorn, N.T.; Kirkby, K.J.; Merchant, M.J.; Ingram, S.; Paganetti, H.; Held, K.D.; Ramos-Mendez, J.; et al. A New Standard DNA Damage (SDD) Data Format. Radiat. Res. 2019, 191, 76–92; [Google Scholar] [CrossRef]
- Poludniowski, G.G.; Evans, P.M. Calculation of X-ray spectra emerging from an X-ray tube. Part, I. Electron penetration characteristics in X-ray targets. Med Phys. 2007, 34, 2164–2174. [Google Scholar] [CrossRef] [Green Version]
- Poludniowski, G.G. Calculation of X-ray spectra emerging from an X-ray tube. Part II. X-ray production and filtration in X-ray targets. Med Phys. 2007, 34, 2175–2186. [Google Scholar] [CrossRef] [PubMed]
- Poludniowski, G.; Landry, G.; DeBlois, F.; Evans, P.M.; Verhaegen, F. SpekCalc: A program to calculate photon spectra from tungsten anode X-ray tubes. Phys. Med. Biol. 2009, 54, N433–N438. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dos Santos, M.; Paget, V.; Ben Kacem, M.; Trompier, F.; Benadjaoud, M.A.; François, A.; Guipaud, O.; Benderitter, M.; Milliat, F. Importance of dosimetry protocol for cell irradiation on a low X-rays facility and consequences for the biological response. Int. J. Radiat. Biol. 2018, 94, 597–606. [Google Scholar] [CrossRef] [PubMed]
- Sheikh-Bagheri, D.; Rogers, D.W.O. Monte Carlo calculation of nine megavoltage photon beam spectra using the BEAM code. Med Phys. 2002, 29, 391–402. [Google Scholar] [CrossRef] [PubMed]
- Kellerer, A.M.; Chmelevsky, D. Concepts of microdosimetry II. Probability distributions of the microdosimetric variables. Radiat. Environ. Biophys. 1975, 12, 205–216. [Google Scholar] [CrossRef] [Green Version]
- Santa Cruz, G.A. Microdosimetry: Principles and applications. Rep. Pract. Oncol. Radiother. 2016, 21, 135–139. [Google Scholar] [CrossRef] [Green Version]
- Lobachevsky, P.N.; Martin, R.F. DNA strand breakage by 125I-decay in a synthetic oligodeoxynucleotide--2. Quantitative analysis of fragment distribution. Acta. Oncol. 1996, 35, 809–815. [Google Scholar] [CrossRef]
- Prise, K.M.; Folkard, M.; Michael, B.D.; Vojnovic, B.; Brocklehurst, B.; Hopkirk, A.; Munro, I.H. Critical energies for SSB and DSB induction in plasmid DNA by low-energy photons: Action spectra for strand-break induction in plasmid DNA irradiated in vacuum. Int. J. Radiat. Biol. 2000, 76, 881–890. [Google Scholar]
- Nikjoo, H.; O’Neill, P.; Wilson, W.E.; Goodhead, D.T. Computational approach for determining the spectrum of DNA damage induced by ionizing radiation. Radiat. Res. 2001, 156, 577–583. [Google Scholar] [CrossRef]
- Karamitros, M.; Mantero, A.; Incerti, S.; Friedland, W.; Baldacchino, G.; Barberet, P.; Bernal, M.; Capra, R.; Champion, C.; Bitar, Z.E.; et al. Modeling Radiation Chemistry in the Geant4 Toolkit. Prog. Nucl. Sci. Technol. 2011, 2, 503–508. [Google Scholar] [CrossRef]
- Karamitros, M.; Luan, S.; Bernal, M.A.; Allison, J.; Baldacchino, G.; Davidkova, M.; Francis, Z.; Friedland, W.; Ivantchenko, V.; Ivantchenko, A.; et al. Diffusion-controlled reactions modeling in Geant4-DNA. J. Comput. Phys. 2014, 274, 841–882. [Google Scholar] [CrossRef]
- Balasubramanian, B.; Pogozelski, W.K.; Tullius, T.D. DNA strand breaking by the hydroxyl radical is governed by the accessible surface areas of the hydrogen atoms of the DNA backbone. PNAS 1998, 95, 9738–9743. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dizdaroglu, M.; Jaruga, P. Mechanisms of free radical-induced damage to DNA. Free Radic. Res. 2012, 46, 382–419. [Google Scholar] [CrossRef] [PubMed]
- Nikjoo, H.; O’Neill, P.; Goodhead, D.T.; Terrissol, M. Computational modelling of low-energy electron-induced DNA damage by early physical and chemical events. Int. J. Radiat. Biol. 1997, 71, 467–483. [Google Scholar] [CrossRef]
- Ester, M.; Kriegel, H.-P.; Sander, J.; Xu, X. A density-based algorithm for discovering clusters in large spatial databases with noise. KDD 1996, 96, 226−231. [Google Scholar]






| Radiation Quality | 40 kVp X-rays | 220 kVp X-rays | 4 MV X-rays |
|---|---|---|---|
| % PE | 68.9 | 19.6 | 3.3 |
| % CE | 31.1 | 80.4 | 96.3 |
| % CONV | 0 | 0 | 0.4 |
| Mean energy of secondary electrons (keV) | 9.8 | 18.4 | 858.4 |
| Radiation Quality | 40 kVp X-rays | 220 kVp X-rays | 4 MV X-rays | |||
|---|---|---|---|---|---|---|
| Duration of the chemical stage | 2.5 ns | 10 ns | 2.5 ns | 10 ns | 2.5 ns | 10 ns |
| DSB/Gy/Gbp | 3.5 ± 0.3 | 4.7 ± 0.3 | 3.5 ± 0.3 | 4.7 ± 0.2 | 2.8 ± 0.3 | 3.6 ± 0.3 |
| Simple DSB (%) | 86.0 ± 3.3 | 86.3 ± 1.8 | 86.4 ± 2.1 | 86.2 ± 2.1 | 87.7 ± 2.9 | 86.5 ± 2.3 |
| Complex DSB (%) | 14.0 ± 3.3 | 13.7 ± 1.8 | 13.6 ± 2.1 | 13.8 ± 2.1 | 12.3 ± 2.9 | 13.5 ± 2.3 |
| Experimental Data | 40 kVp X-rays | 220 kVp X-rays | 4 MV X-rays |
| Mean number of γ-H2AX foci per nucleus (30 min post-irradiation) [30] | 0.25 Gy: 5.35 ± 1.13 | 0.25 Gy: 7.35 ± 2.17 | 0.25 Gy: 4.35 ± 0.21 |
| 0.5 Gy: 9.88 ± 0.87 | 0.5 Gy: 10.24 ± 1.73 | 0.5 Gy: 8.54 ± 1.42 | |
| 1 Gy: 18.59 ± 0.43 | 1 Gy: 18.64 ± 2.33 | 1 Gy: 16.46 ± 1.63 | |
| 2 Gy: 30.30 ± 2.21 | 2 Gy: 30.59 ± 2.96 | 2 Gy: 26.42 ± 0.87 | |
| Simulated DSBs and Experimental Foci at 1 Gy | 40 kVp X-rays | 220 kVp X-rays | 4 MV X-rays |
| Mean number of DSBs per nucleus (sim.) for a chemical simulation end-time of 2.5 ns | 21.0 ± 0.3 | 21.0 ± 0.3 | 16.8 ± 0.3 |
| Mean number of DSBs per nucleus (sim.) for a chemical simulation end-time of 10 ns | 28.2 ± 0.3 | 28.2 ± 0.2 | 21.6 ± 0.3 |
| Mean number of γ-H2AX foci per nucleus (exp.) | 18.59 ± 0.43 | 18.64 ± 2.33 | 16.46 ± 1.63 |
| (a) | |
| Reaction | Reaction Rate (1010·M−1s−1) |
| H● + e−aq + H2O → OH− + H2 | 2.65 |
| H● + OH● → H2O | 1.44 |
| H● + H● → H2 | 1.20 |
| H2 + OH● → H● + H2O | 4.17 × 10−3 |
| H2O2 + e−aq → OH− + OH● | 1.41 |
| H3O+ + e−aq → H● + H2O | 2.11 |
| H3O+ + OH− → 2H2O | 14.3 |
| OH● + e−aq → OH● | 2.95 |
| OH● + OH● → H2O2 | 0.44 |
| e−aq + e−aq + 2H2O → 2OH− + H2 | 0.50 |
| (b) | |
| Reaction | Reaction Rate (109·M−1s−1) |
| 2-deoxyribose + OH● | 1.8 |
| Adenine + OH● | 6.1 |
| Guanine + OH● | 9.2 |
| Thymine + OH● | 6.4 |
| Cytosine + OH● | 6.1 |
| 2-deoxyribose + e−aq | 0.01 |
| Adenine + e−aq | 9.0 |
| Guanine + e−aq | 14.0 |
| Thymine + e−aq | 18.0 |
| Cytosine + e−aq | 13.0 |
| 2-deoxyribose + H● | 0.029 |
| Adenine + H● | 0.10 |
| Thymine + H● | 0.57 |
| Cytosine + H● | 0.092 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, N.; Bueno, M.; Meylan, S.; Perrot, Y.; Tran, H.N.; Freneau, A.; Dos Santos, M.; Vaurijoux, A.; Gruel, G.; Bernal, M.A.; et al. Assessment of Radio-Induced Damage in Endothelial Cells Irradiated with 40 kVp, 220 kVp, and 4 MV X-rays by Means of Micro and Nanodosimetric Calculations. Int. J. Mol. Sci. 2019, 20, 6204. https://doi.org/10.3390/ijms20246204
Tang N, Bueno M, Meylan S, Perrot Y, Tran HN, Freneau A, Dos Santos M, Vaurijoux A, Gruel G, Bernal MA, et al. Assessment of Radio-Induced Damage in Endothelial Cells Irradiated with 40 kVp, 220 kVp, and 4 MV X-rays by Means of Micro and Nanodosimetric Calculations. International Journal of Molecular Sciences. 2019; 20(24):6204. https://doi.org/10.3390/ijms20246204
Chicago/Turabian StyleTang, Nicolas, Marta Bueno, Sylvain Meylan, Yann Perrot, Hoang N. Tran, Amélie Freneau, Morgane Dos Santos, Aurélie Vaurijoux, Gaëtan Gruel, Mario A. Bernal, and et al. 2019. "Assessment of Radio-Induced Damage in Endothelial Cells Irradiated with 40 kVp, 220 kVp, and 4 MV X-rays by Means of Micro and Nanodosimetric Calculations" International Journal of Molecular Sciences 20, no. 24: 6204. https://doi.org/10.3390/ijms20246204
APA StyleTang, N., Bueno, M., Meylan, S., Perrot, Y., Tran, H. N., Freneau, A., Dos Santos, M., Vaurijoux, A., Gruel, G., Bernal, M. A., Bordage, M.-C., Emfietzoglou, D., Francis, Z., Guatelli, S., Ivanchenko, V., Karamitros, M., Kyriakou, I., Shin, W.-G., Incerti, S., & Villagrasa, C. (2019). Assessment of Radio-Induced Damage in Endothelial Cells Irradiated with 40 kVp, 220 kVp, and 4 MV X-rays by Means of Micro and Nanodosimetric Calculations. International Journal of Molecular Sciences, 20(24), 6204. https://doi.org/10.3390/ijms20246204





