Can the Isothermal Calorimetric Curve Shapes Suggest the Structural Changes in Micellar Aggregates?
Abstract
:1. Introduction
2. Results and Discussion
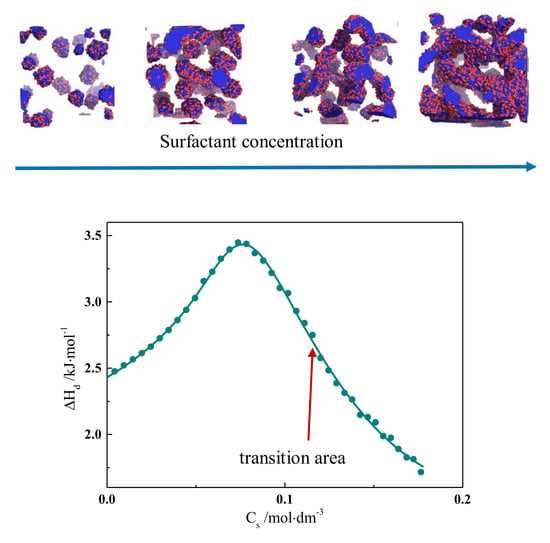
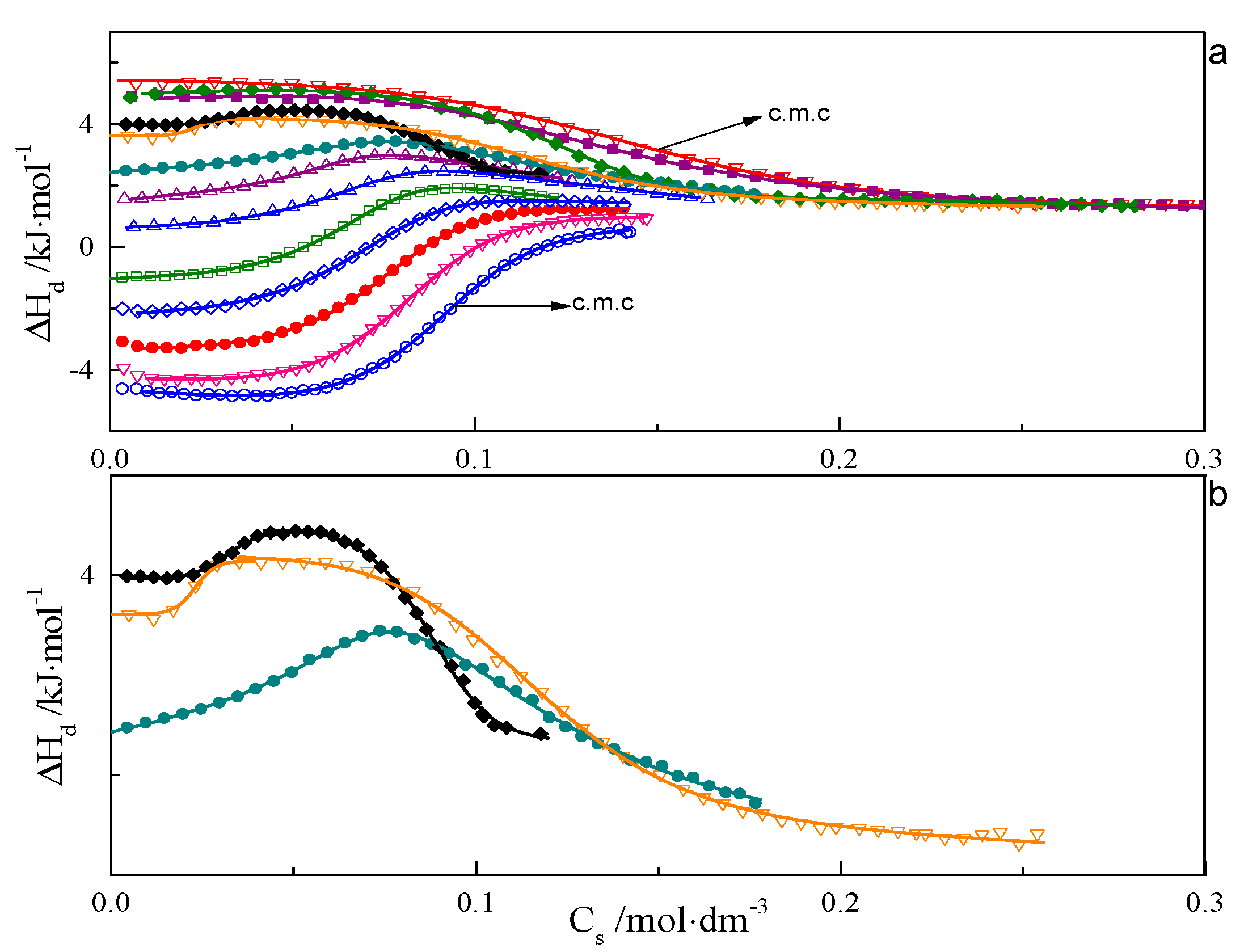
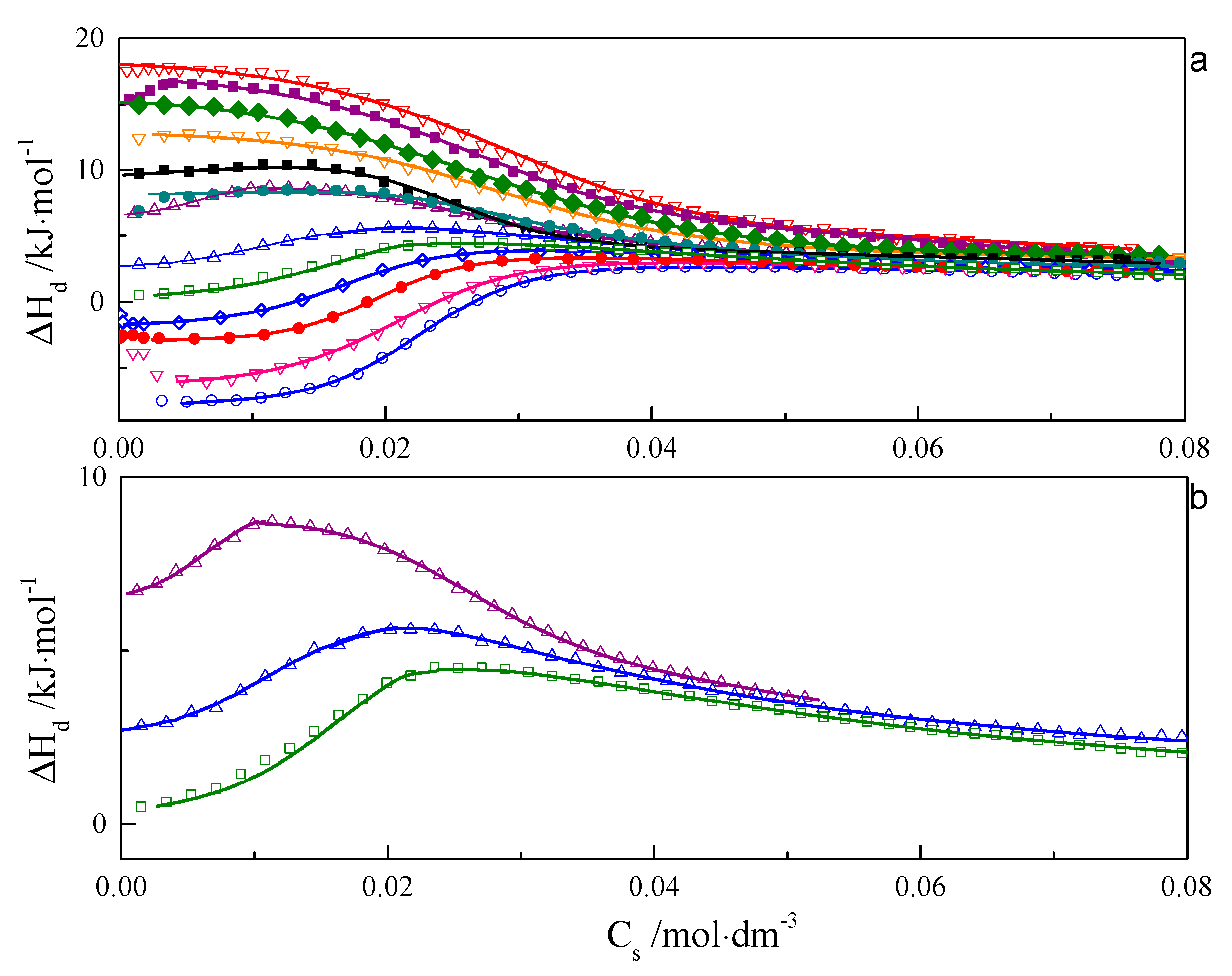
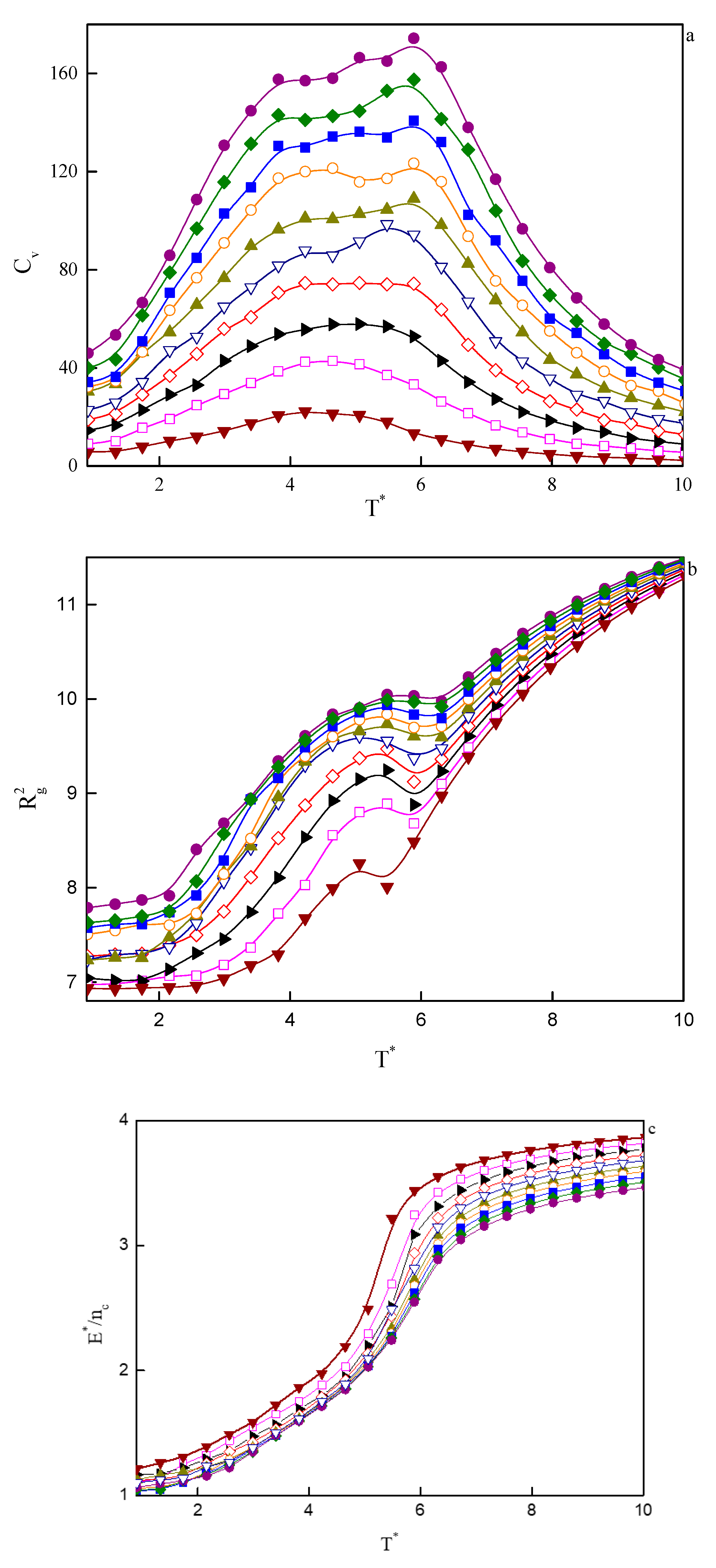
2.1. Calorimetric Data
2.2. Scanning Transmission Electron Microscopy
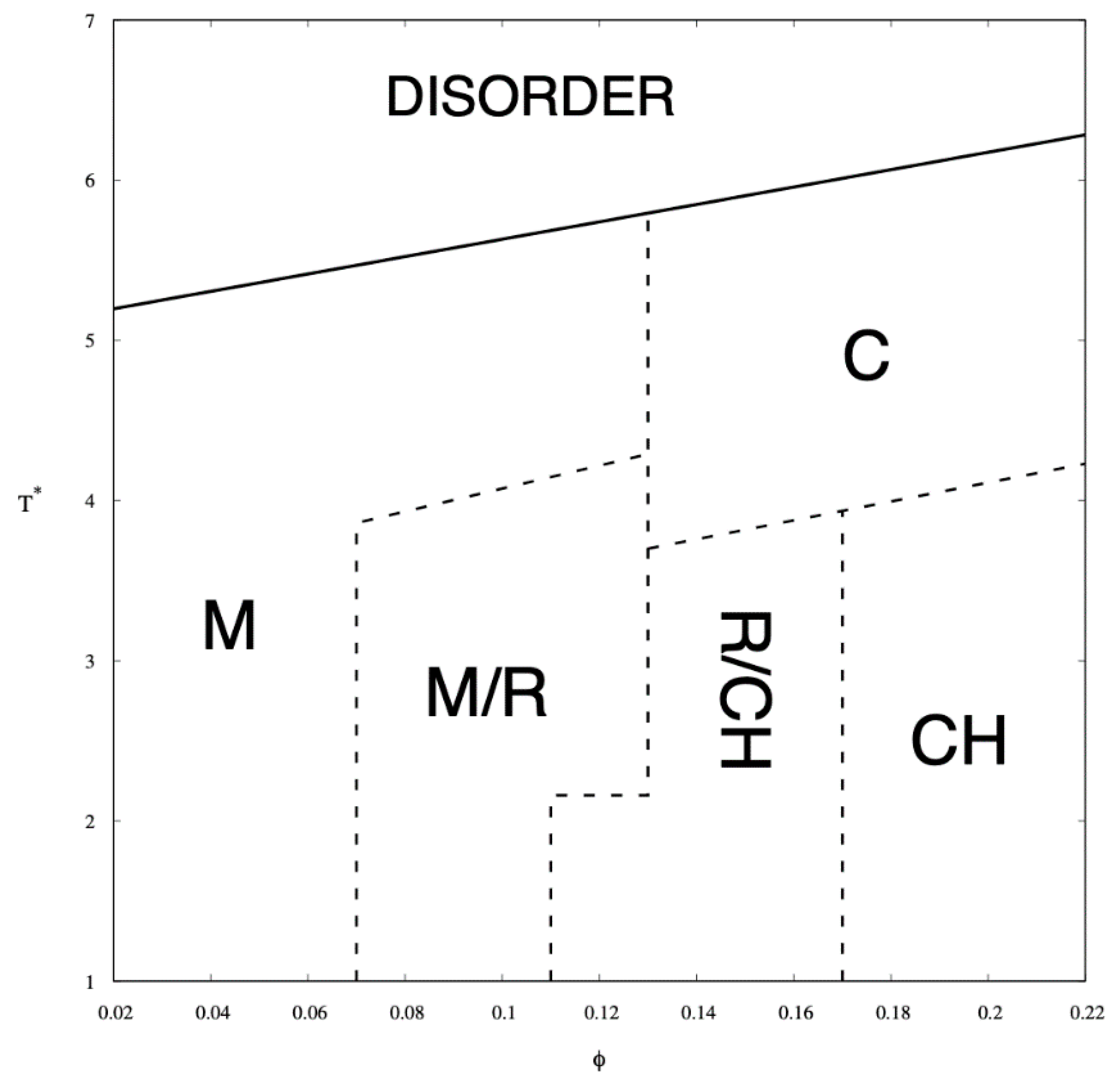
2.3. Computer Simulations
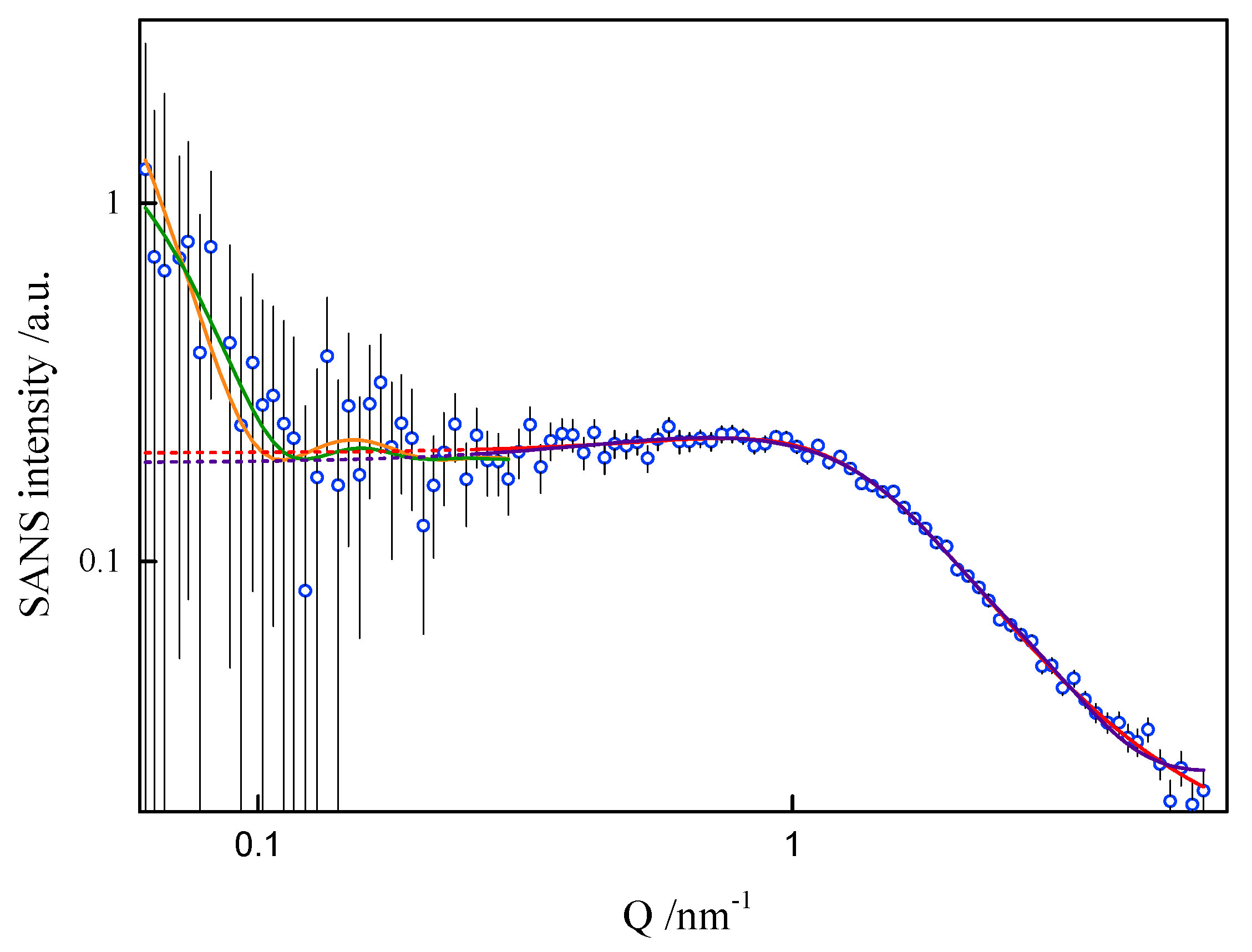
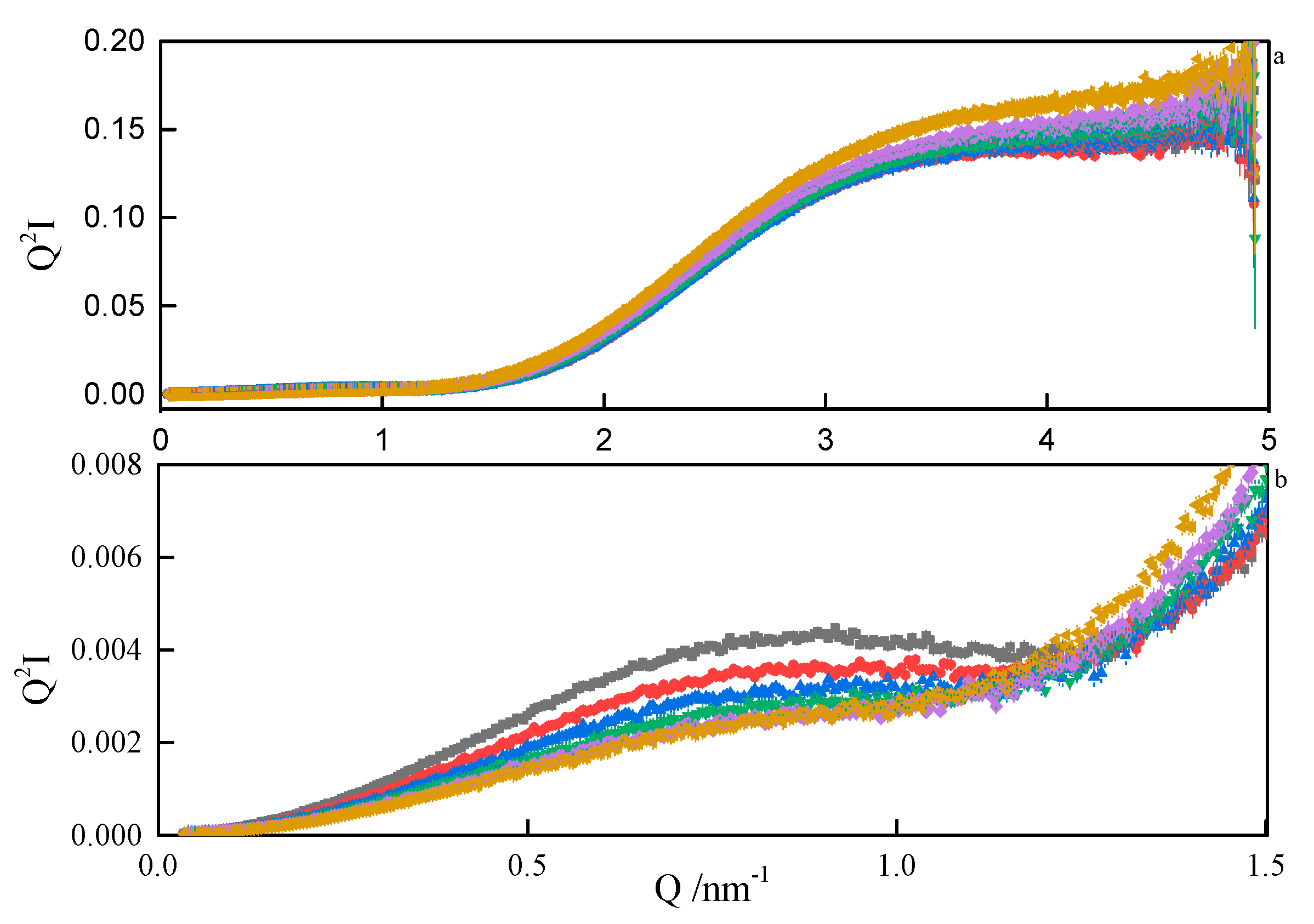
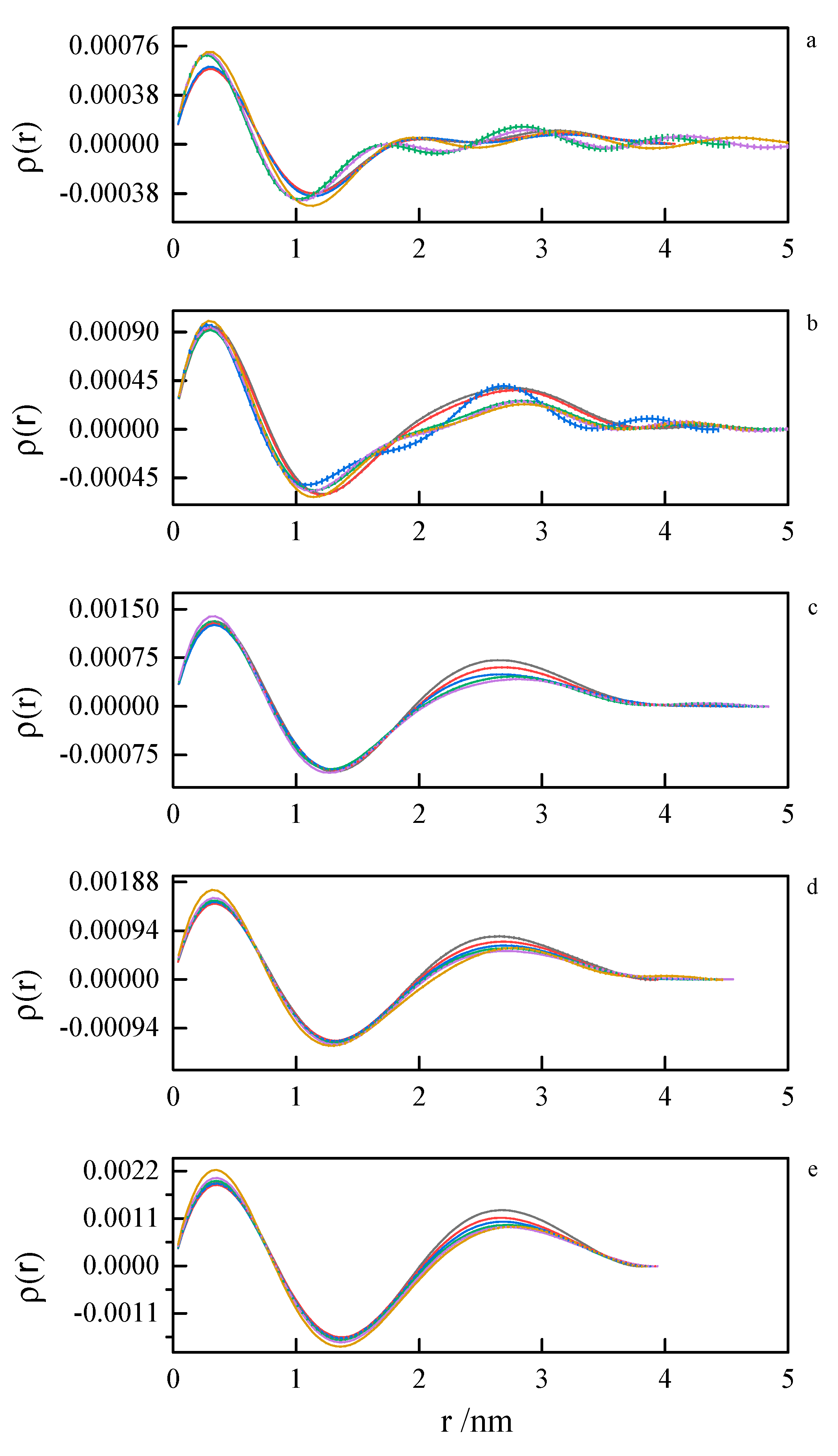
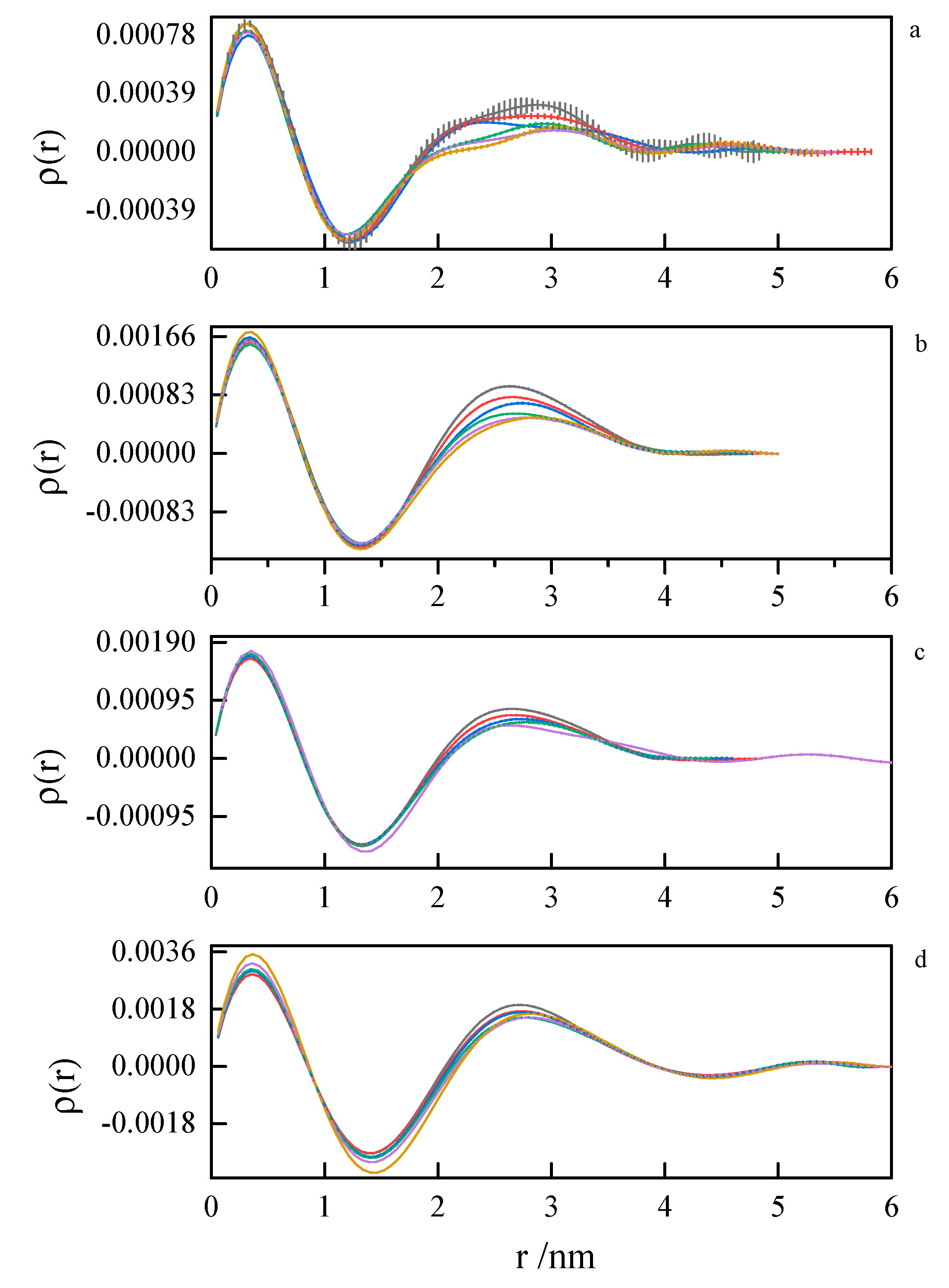
2.4. Small-Angle Neutron Scattering and Small-Angle X-ray Scattering
2.5. Interpretation of ITC Curves and Thermodynamics of Micellisation
3. Experimental Section
3.1. Materials
3.2. Methods
3.2.1. Calorimetric Measurements
3.2.2. Conductometric Studies
3.2.3. Small-Angle Neutron Scattering Measurements
3.2.4. SAXS Measurements
3.2.5. Scanning Transmission Electron Microscopy
3.2.6. Computer Simulations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kim, J.Y.; Kinoshita, M.; Kume, S.; Tomas, H.G.; Sugiki, T.; Ladbury, J.E.; Kojima, C.; Ikegami, T.; Kurisu, G.; Goto, Y.; et al. Noncovalent forces tune the electron transfer complex between ferredoxin and sulfite reductase to optimize enzymatic activity. Biochem. J. 2016, 473, 3837–3854. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ladbury, J.E. Calorimetry as a tool for understanding biomolecular interactions and an aid to drug design. Biochem. Soc. Trans. 2010, 38, 888–893. [Google Scholar]
- Sprakel, L.M.J.; Schuur, B. Improving understanding of solvent effects on intermolecular interactions in reactive liquid–liquid extraction with Isothermal Titration Calorimetry and molecular modeling. J. Ind. Eng. Chem. 2019, 72, 364–373. [Google Scholar] [CrossRef]
- Burnouf, D.; Ennifar, E.; Guedich, S.; Puffer, B.; Hoffmann, G.; Bec, G.; Disdier, F.; Baltzinger, M.; Dumas, P. A new method for obtaining joint thermodynamic and kinetic data by isothermal titration calorimetry. J. Am. Chem. Soc. 2012, 134, 559–565. [Google Scholar] [CrossRef] [PubMed]
- Gupta, S.; Tiwari, N.; Munde, M. A comprehensive biophysical analysis of the effect of DNA binding drugs on protamine-induced DNA condensation. Sci. Rep. 2019, 9, 5891. [Google Scholar] [CrossRef] [Green Version]
- Loh, W.; Brinatti, C.; Tam, K.C. Use of isothermal titration calorimetry to study surfactant aggregation in colloidal systems. Biochim. Biophys. Acta 2016, 1860, 999–1016. [Google Scholar] [CrossRef]
- Upendar, S.; Mani, E.; Basavaraj, M.G. Aggregation and Stabilization of Colloidal spheroids by oppositely charged spherical nanoparticles. Langmuir 2018, 34, 6511–6521. [Google Scholar] [CrossRef]
- Janeka, T.; Czyznikowska, Z.; Łuczynski, J.; Gudina, E.J.; Rodrigues, L.R.; Gałezowska, J. Physicochemical study of biomolecular interactions between lysosomotropic surfactants and bovine serum albumin. Coll. Surf. B Biointerfaces 2017, 159, 750–758. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.; Qiao, F.; Fan, Y.; Han, Y.; Wang, Y. Interactions of cationic/anionic mixed surfactant aggregates with phospholipid vesicles and their skin penetration ability. Langmuir 2017, 33, 2760–2769. [Google Scholar] [CrossRef]
- Sekhon, B. Surfactants: Pharmaceutical and medicinal aspects. J. Pharm. Technol. Res. Manag. 2013, 1, 43–68. [Google Scholar] [CrossRef] [Green Version]
- Pal, N.; Saxena, N.; Manda, A. Studies on the physicochemical properties of synthesized tailor-made gemini surfactants for application in enhanced oil recovery. J. Mol. Liq. 2018, 258, 211–224. [Google Scholar] [CrossRef]
- Tripathy, D.B.; Mishraa, A.; Clark, J.; Farmer, T. Synthesis, chemistry, physicochemical properties and industrial applications of amino acid surfactants: A review. Comptes Rendus Chim. 2018, 21, 112–130. [Google Scholar] [CrossRef]
- Hanafy, N.A.N.; Kemary, E.; Leporatti, S. Micelles structure development as a strategy to improve smart cancer therapy. Cancers 2018, 10, 238. [Google Scholar] [CrossRef] [Green Version]
- Long, J.A.; Rankin, B.M.; Ben-Amotz, D. Micelle structure and hydrophobic hydration. J. Am. Chem. Soc. 2015, 137, 10809–10815. [Google Scholar] [CrossRef] [PubMed]
- Patist, A.; Oh, S.G.; Leung, R.; Shah, D.O. Kinetics of micellisation; its significance to technological processes. Colloid Surf. A. Physicochem. Eng. Asp. 2001, 176, 3–16. [Google Scholar] [CrossRef]
- McLachlan, A.; Singh, K.; Piggott, E.; McAlduff, M.; MacLennan, S.; Sandre, V.; Reid, T.; Marangoni, D.G. msm Cationic Gemini and Zwitterionic Surfactants—Spacer-Dependent Synergistic Interactions. J. Phys. Chem. B 2019, 123, 1855–1868. [Google Scholar] [CrossRef]
- Andleeb, Z.N.; Noori, S.; Din, K. Effect of surfactant structure on the mixed micelle formation of cationic gemini-zwitterionic phospholipid systems. Colloids Surf. A Physicochem. Eng. Asp. 2015, 477, 9–18. [Google Scholar]
- Din, K.; Sheikh, M.S.; Dar, A.A. Analysis of mixed micellar and interfacial behavior of cationic gemini hexanediyl-1, 6-bis(dimethylcetylammonium bromide) with conventional ionic and nonionic surfactants in aqueous medium. J. Phys. Chem. B 2010, 114, 6023–6032. [Google Scholar] [CrossRef]
- Tehrani-Bagha, A.R. Cationic gemini surfactant with cleavable spacer: Emulsion stability. Colloids Surf. A Physicochem. Eng. Asp. 2016, 508, 79–84. [Google Scholar] [CrossRef]
- Taleb, K.; Mohamed-Benkadab, M.M.N.; Saidi-Besbesa, S.; Grohensc, Y.; Derdour, A. Benzene ring containing cationic gemini surfactants: Synthesis, surface properties and antibacterial activity. J. Mol. Liq. 2017, 241, 81–90. [Google Scholar] [CrossRef]
- Bao, Y.; Guo, J.; Ma, J.; Li, M.; Li, X. Physicochemical and antimicrobial activities of cationic gemini surfactants withpolyether siloxane linked group. J. Mol. Liq. 2017, 242, 8–15. [Google Scholar] [CrossRef]
- Shimizu, S.; Pires, P.A.R.; Loh, W.; El Seoud, O.A. Thermodynamics of micellization of cationic surfactants in aqueous solutions: Consequences of the presence of the 2-acylaminoethyl moiety in the surfactant head group. Colloid Polym. Sci. 2004, 282, 1026–1032. [Google Scholar] [CrossRef]
- Majhi, P.R.; Blume, A. Thermodynamic characterization of temperature-induced micellization and demicellization of detergents studied by Differential Scanning Calorimetry. Langmuir 2001, 17, 3844–3851. [Google Scholar] [CrossRef]
- Király, Z.; Dekány, I. A thermometric titration study on the micelleformation of sodium decyl sulfate in water. J. Colloid Interface Sci. 2001, 242, 214–219. [Google Scholar] [CrossRef]
- Olesen, N.E.; Westh, P.; Holm, R. Determination of thermodynamic potentials and the aggregation number for micelles with the mass-action model by isothermal titration calorimetry: A case study on bile salts. J. Colloid Interface Sci. 2015, 453, 79–89. [Google Scholar] [CrossRef] [PubMed]
- Bijma, K.; Engberts, J.; Blandamer, M.J.; Cullis, P.M.; Last, P.M.; Irlam, K.D.; Soldi, L.G. Classification of calorimetric titration plots for alkyltrimethylammonium and alkylpyridinium cationic surfactants inaqueous solutions. J. Chem. Soc. Faraday Trans. 1997, 93, 1579–1584. [Google Scholar] [CrossRef] [Green Version]
- Kresheck, G.C. Comparison of the calorimetric and van’t Hoff enthalpy of micelle formation for a nonionic surfactant in H2O and D2O solutions from 15 to 40 °C. J. Phys. Chem. B 1998, 102, 6596–6600. [Google Scholar] [CrossRef]
- Páhi, A.B.; Király, Z.; Dudás, Á.J.; Purkás, S.; Vágó, Á. Thermodynamics of micelle formation of the counterion coupled gemini surfactant bis(4-(2-dodecyl)benzenesulfonate)-jeffamine salt and its dynamic adsorption on sandstone. J. Phys. Chem. B 2008, 112, 15320–15326. [Google Scholar] [CrossRef]
- Grolier, J.P.E.; Del Río, J.M. Isothermal titration calorimetry: A thermodynamic interpretation of measurements. J. Chem. Thermodyn. 2012, 55, 193–202. [Google Scholar] [CrossRef]
- Heerklotz, H.; Tsamaloukas, A.; Kita-Tokarczyk, K.; Strunz, P.; Gutberlet, T. Structural, volumetric, and thermodynamic characterization of a micellar sphere-to-rod transition. J. Am. Chem. Soc. 2004, 126, 16544–16552. [Google Scholar] [CrossRef]
- Valentea, A.J.M.; López Cascales, J.J.; Fernández Romero, A.J. Thermodynamic analysis of unimer-micelle and sphere-to-rod micellar transitions of aqueous solutions of sodium dodecylbenzenesulfonate. J. Chem. Therm. 2014, 77, 54–62. [Google Scholar] [CrossRef]
- Rajput, S.M.; Kumar, S.; Aswal, V.K.; El Seoud, O.A.; Malek, N.I.; Kailasa, S.K. Drug-induced micelle-to-vesicle transition of a cationic gemini surfactant: Potential applications in drug delivery. Chem. Phys. Chem. 2018, 19, 865–872. [Google Scholar] [CrossRef] [PubMed]
- Du, C.; Cai, D.; Qin, M.; Zheng, P.; Hao, Z.; Yin, T.; Zhao, J.; Shen, W. Thermodynamics of mixed surfactant solutions of N,N′-Bis(dimethyldodecyl)-1,2-ethanediammoniumdibromide with 1-dodecyl-3-methylimidazolium bromide. J. Phys. Chem. B 2014, 118, 1168–1179. [Google Scholar] [CrossRef] [PubMed]
- Bergström, L.M.; Tehrani-Bagha, A.; Nagy, G. Growth behavior, geometrical shape, and second CMC of micelles formed by cationic gemini esterquat surfactants. Langmuir 2015, 31, 4644–4653. [Google Scholar] [CrossRef]
- Olofsson, G.; Loh, W. On the use of titration calorimetry to study the association of surfactants in aqueous solutions. J. Braz. Chem. Soc. 2009, 20, 577–593. [Google Scholar] [CrossRef] [Green Version]
- Ray, G.B.; Chakraborty, I.; Ghosh, S.; Moulik, S.P. A critical and comprehensive assessment of interfacial and bulk properties of aqueous binary mixtures of anionic surfactants, sodium dodecylsulfate, and sodium dodecylbenzenesulfonate. Colloid Polym. Sci. 2007, 285, 457–469. [Google Scholar]
- Karumbamkandathil, A.; Ghosh, S.; Anand, U.; Prithwidip, S.; Mukherjee, M.; Mukherjee, S. Micelles of benzethonium chloride undergoes spherical to cylindrical shape transformation: An intrinsic fluorescence and calorimetric approach. Chem. Phys. Lett. 2014, 593, 115–121. [Google Scholar] [CrossRef]
- James, J.P. Stewart, Stewart Computational Chemistry MOPAC2016. Version: 20.015W. Available online: HTTP://OpenMOPAC.net (accessed on 6 January 2020).
- Turbomole V.7.3, a development of University of Karlsruhe and Forschungszentrum Karlsruhe GmbH, 1989–2007. Available online: https://www.turbomole.org (accessed on 30 October 2015).
- Klamt, A.; Schüürmann, G. COSMO: A new approach to dielectric screening in solvents with explicit expressions for the screening energy and its gradient. J. Chem. Soc. Perkin Trans. 1993, 2, 799–805. [Google Scholar] [CrossRef]
- SasView, M. SasView Version 4.2.2. Zenodo 2019. [Google Scholar] [CrossRef]
- Franke, D.; Petoukhov, M.V.; Konarev, P.V.; Panjkovich, A.; Tuukkanen, A.; Mertens, H.D.T.; Kikhney, A.G.; Hajizadeh, N.R.; Franklin, J.M.; Jeffries, C.M.; et al. ATSAS 2.8: A comprehensive data analysis suite for small-angle scattering from macromolecular solutions. J. Appl. Crystallogr. 2017, 50, 1212–1225. [Google Scholar] [CrossRef] [Green Version]
- Putnam, C.; Hammel, M.; Hura, G.; Tainer, J. X-ray solution scattering (SAXS) combined with crystallography and computation: Defining accurate macromolecular structures, conformations and assemblies in solution. Q. Rev. Biophys. 2007, 40, 191–285. [Google Scholar] [CrossRef] [PubMed]
- Hura, G.; Menon, A.; Hammel, M.; Rambo, R.; Poole, F.; Tsutakawa, S.; Jenney, F.; Classen, S.; Frankel, K.; Hopkins, R.; et al. Robust, high-throughput solution structural analyses by small angle X-ray scattering (SAXS). Nat. Methods 2009, 6, 606–612. [Google Scholar] [CrossRef] [PubMed]
- Feigin, L.A.; Svergun, D.I. Structure Analysis by Small-Angle X-ray and Neutron Scattering; Plenum Press: New York, NY, USA, 1989. [Google Scholar]
- Chandler, D. Interfaces and the driving force of hydrophobic assembly. Nature 2005, 437, 640–647. [Google Scholar] [CrossRef] [PubMed]
- Diamant, H.; Andelman, D. Dimeric surfactants: Spacer chain conformation and specific area at the air/water interface. Langmuir 1994, 10, 2910–2916. [Google Scholar] [CrossRef]
- Hattori, N.; Hirata, H.; Okobayashi, H.; Furusaka, M.; O’Connor, C.J. Small-angle neutron scattering study of bis(quanternaryammonium bromide) surfactant micelles in water. Effect of the long spacer chain on micellar structure. Colloid Polym. Sci. 1999, 277, 95–100. [Google Scholar] [CrossRef]
- Hattori, N.; Yoshino, A.; Okabayashi, H.; O’Connor, C.J. Conformational analysis of (phenylenedimethylene)bis(n-octylammonium)dibromides in aqueous solution. Conformational change upon micellization. J. Phys. Chem. B 1998, 102, 8965–8973. [Google Scholar] [CrossRef]
- Zana, R.; Benrraou, M.; Rueff, R. Alkanediyl-α,ω-bis(dimethylammonium bromide) surfactants. Effect of the spacer chain length on the critical micelle concentration and micelle ionization degree. Langmuir 1991, 7, 1072–1075. [Google Scholar] [CrossRef]
- Piekarski, H.; Łudzik, K. A microcalorimetric titration study on the micelle formation of alkanediyl-α,ω-bis(dimethylalkylammonium bromide) surfactants at a 283.15–343.15 K temperature range. J. Therm. Anal. Calorim. 2012, 110, 263–271. [Google Scholar] [CrossRef] [Green Version]
- Zana, R. Critical micellization concentration of surfactants in aqueous solution and free energy of micellization. Langmuir 1996, 12, 1208–1211. [Google Scholar] [CrossRef]
- Ludzik, K.; Kustrzepa, K.; Piekarrski, H.; Jozwiak, M. Application of conductance study to analyze micellization behavior of cationic gemini surfactants in water and water−ethanol solvent mixed media. J. Chem. Eng. Data 2016, 61, 1047–1053. [Google Scholar] [CrossRef]
- Kuklin, A.I.; Islamov, A.K.; Kovalev, Y.S.; Utrobin, P.; Gordely, V.I. Optimization two-detector system small-angle neutron spectrometer YuMO for nanoobject investigation. J. Surf. Investig. X-ray Synchrotron Neutron Tech. 2006, 6, 74–83. [Google Scholar]
- Kuklin, A.I.; Islamov, A.K.; Gordeliy, V.I. Scientific Reviews: Two-detector system for small-angle neutron scattering instrument. Neutron News 2005, 16, 16–18. [Google Scholar] [CrossRef]
- Kuklin, A.I.; Soloviov, D.V.; Rogachev, A.V.; Utrobin, P.K.; Kovalev, Y.S.; Balasoiu, M.; Ivankov, O.I.; Sirotin, A.P.; Murugova, T.N.; Petukhova, T.B.; et al. New opportunities provided by modernized small-angle neutron scattering two-detector system instrument (YuMO). J. Phys. Conf. Ser. 2011, 291, 12–13. [Google Scholar] [CrossRef]
- Soloviev, A.G.; Solovjeva, T.M.; Ivankov, O.I.; Soloviov, D.V.; Rogachev, A.V.; Kuklin, A.I. SAS program for two-detector system: Seamless curve from both detectors. J. Phys. Conf. Ser. 2017, 848, 12020. [Google Scholar] [CrossRef]
- Murugova, T.N.; Vlasov, A.V.; Ivankov, O.I.; Rogachev, A.V.; Ryzhykau, Y.; Soloviov, D.V.; Gordeliy, V.I. Low resolution structural studies of apoferritin via SANS and SAXS: The effect of concentration. J. Optoelectron. Adv. Mater. 2015, 17, 1397–1402. [Google Scholar]
- Beardsley, T.; Matsen, M. Monte Carlo phase diagram for diblock copolymer melts. Eur. Phys. J. E 2010, 32, 255–264. [Google Scholar] [CrossRef]
- Lewandowski, K.; Knychala, P.; Banaszak, M. Parallel-tempering Monte-Carlo simulation with feedback-optimized algorithm applied to a coil-to-globule transition of a lattice homopolymer. Comput. Methods Sci. Technol. 2010, 16, 29–35. [Google Scholar] [CrossRef] [Green Version]
- Pakula, T.; Karatasos, K.; Anastasiadis, S.; Fytas, G. Computer simulation of static and dynamic behavior of diblock copolymer melts. Macromolecules 1997, 30, 8463–8472. [Google Scholar] [CrossRef]
- Pakula, T. Simulation Methods for Polymers; Marcel-Dekker: New York, NY, USA, 2004. [Google Scholar]
- Woloszczuk, S.; Tuhin, M.; Gade, S.; Pasquinelli, M.; Banaszak, M.; Spontak, R. Complex phase behavior and network characteristics of midblock-solvated triblock copolymers as physically cross-linked soft materials. ACS Appl. Mater. Interfaces 2017, 9, 39940–39944. [Google Scholar] [CrossRef]

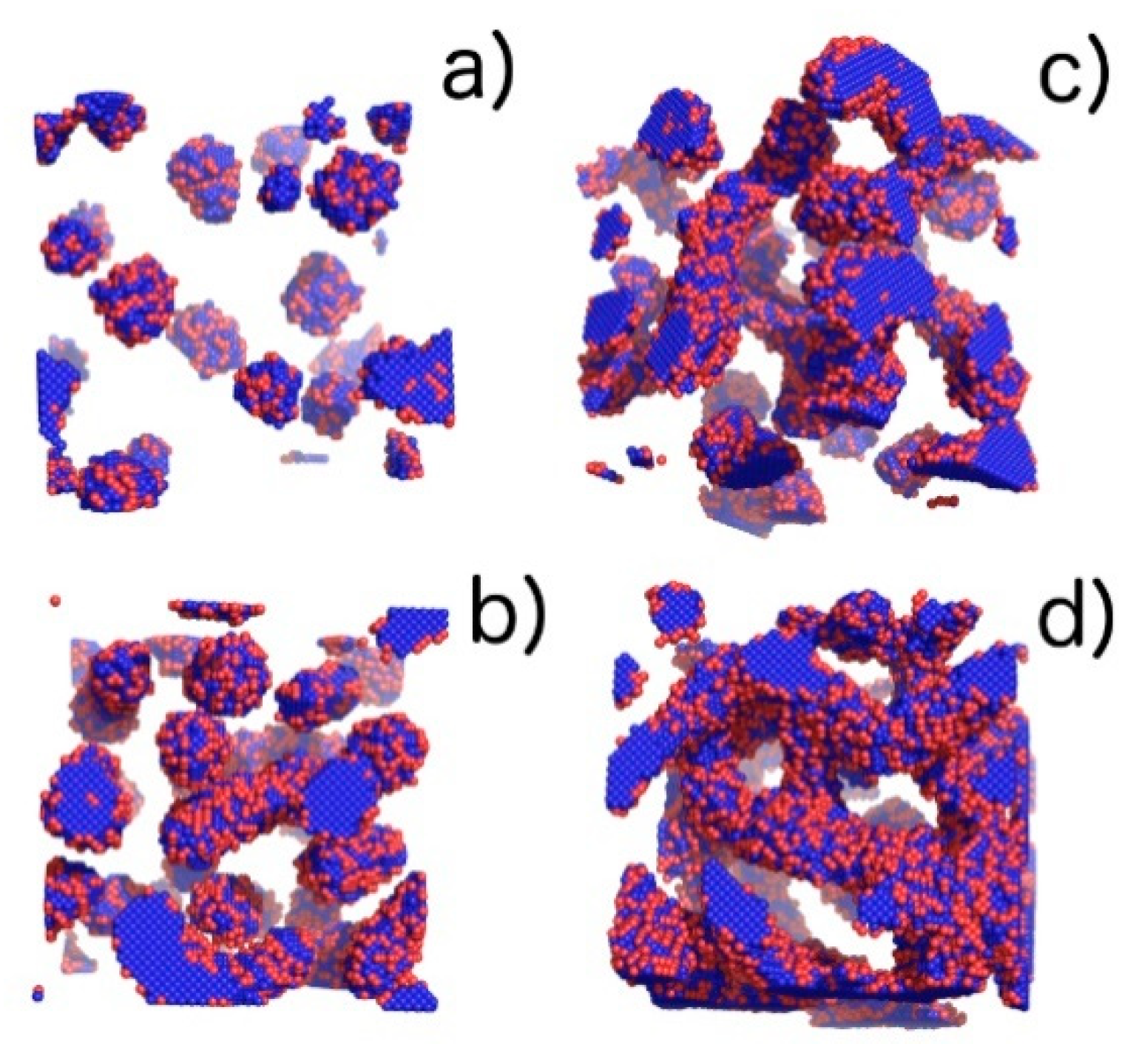




| 8-6-8 | 8-7-8 | 8-8-8 | 8-9-8 | ||||
|---|---|---|---|---|---|---|---|
| N | 6 | 9 | 6 | 8 | 8 | 7 | 13 |
| V/Å3 | 3724.9 | 5720.9 | 3860.0 | 5205.0 | 5409.8 | 4807.8 | 9133.3 |
| A/Å2 | 1502.5 | 2152.9 | 1559.7 | 1895.4 | 2020.2 | 1807.7 | 3103.6 |
| R/Å | 9.62 | 11.09 | 9.73 | 10.75 | 10.89 | 10.47 | 12.97 |
| d/g cm−3 | 1.0666 | 1.0417 | 1.0655 | 1.0535 | 1.0481 | 1.0658 | 1.0419 |
| Rg/Å | 7.47 | 9.16 | 7.71 | 8.61 | 8.72 | 8.20 | 10.52 |
| T /K | c.m.c/mol dm−3Cm.trans/mol dm−3 | Thermodynamic Functions for 8-8-8 | |||
|---|---|---|---|---|---|
/kJ mol−1 | /kJ mol−1 | /kJ mol−1 | /J mol−1 K−1 | ||
| 283.15 | 0.092 ± 0.09 | −17.12 | 5.66 | 22.78 | 80.45 |
| 288.15 | 0.082 ± 0.08 | −17.57 | 5.80 | 23.37 | 81.12 |
| 293.15 | 0.077 ± 0.007 | −17.90 | 4.52 | 22.42 | 76.45 |
| 298.15 | 0.071 ± 0.007 | −18.27 | 3.67 | 21.94 | 73.58 |
| 303.15 | 0.065 ± 0.006 | −18.64 | 3.29 | 21.93 | 72.33 |
| 308.15 | 0.062 ± 0.006 | −18.90 | 2.31 | 21.20 | 68.80 |
| 313.15 | 0.063 ± 0.006 | −19.02 | 1.39 | 20.41 | 65.17 |
| 318.15 | 0.049 ± 0.005 0.116 ± 0.008 | −19.83 −17.40 | 1.36 −2.10 | 21.19 15.30 | 66.60 48.09 |
| 323.15 | 0.032 ± 0.003 0.088 ± 0.005 | −21.15 −18.29 | 0.47 −2.16 | 21.62 16.14 | 66.91 49.95 |
| 328.15 | 0.023 ± 0.002 0.113 ± 0.01 | −22.22 −17.67 | 0.52 −2.82 | 22.74 14.85 | 69.29 45.25 |
| 333.15 | 0.122 ± 0.01 | −17.56 | −3.99 | 13.57 | 40.73 |
| 338.15 | 0.130 ± 0.01 | −17.46 | −4.10 | 13.36 | 39.52 |
| 343.15 | 0.145 ± 0.01 | −17.24 | −4.05 | 13.19 | 38.44 |
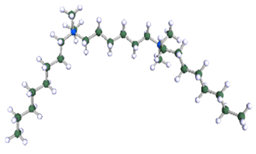
| Conformation | ESCF | ESCF-kinet | ESCF-pot. | |
|---|---|---|---|---|
| 8-6-8 | 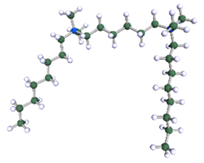 | −4.95 × 10−15 J | −4.92 × 10−15 J | −9.87 × 10−15 J |
| −2.98 × 106 KJ mol−1 | −2.96 × 106 KJ mol−1 | −5.94 × 10−15 KJ mol−1 | ||
 | −4.95 × 10−15 J | −4.92 × 10−15 J | −9.87 × 10−15 J | |
| −2.98 × 106 KJ mol−1 | −2.96 × 106 KJ mol−1 | −5.94 × 10−15 KJ mol−1 | ||
 | −4.95 × 10−15 J | −4.92 × 10−15 J | −9.87 × 10−15 J | |
| −2.98 × 106 KJ mol−1 | −2.96 × 106 KJ mol−1 | −5.94 × 10−15 KJ mol−1 | ||
| 8-7-8 | 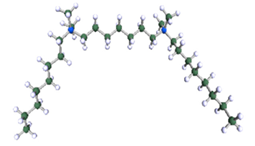 | −5.12 × 10−15 J | −5.09 × 10−15 J | −1.02 × 10−14 J |
| −3.08 × 106 KJ mol−1 | −3.07 × 106 KJ mol−1 | −6.15 × 106 KJ mol−1 | ||
 | −5.12 × 10−15 J | −5.09 × 10−15 J | −1.02 × 10−14 J | |
| −3.08 × 106 KJ mol−1 | −3.07 × 106 KJ mol−1 | −6.15 × 106 KJ mol−1 | ||
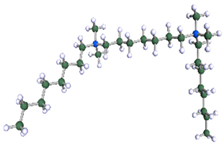 | −5.12 × 10−15 J | −5.09 × 10−15 J | −1.02 × 10−14 J | |
| −3.08 × 106 KJ mol−1 | −3.07 × 106 KJ mol−1 | −6.15 × 106 KJ mol−1 | ||
| 8-8-8 | 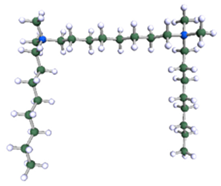 | −5.29 × 10−15 J | −5.26 × 10−15 J | −1.05 × 10−14 J |
| −3.18 × 106 KJ mol−1 | −3.17 × 106 KJ mol−1 | −6.35 × 106 KJ mol−1 | ||
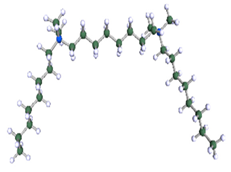 | −5.29 × 10−15 J | −5.26 × 10−15 J | −1.05 × 10−14 J | |
| −3.18 × 106 KJ mol−1 | −3.17 × 106 KJ mol−1 | −6.35 × 106 KJ mol−1 | ||
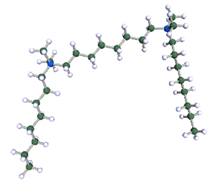 | −5.29 × 10−15 J | −5.26 × 10−15 J | −1.05 × 10−14 J | |
| −3.18 × 106 KJ mol−1 | −3.17 × 106 KJ mol−1 | −6.35 × 106 KJ mol−1 | ||
| 8-9-8 |  | −5.46 × 10−15 J | −5.43 × 10−15 J | −1.09 × 10−14 J |
| −3.29 × 106 KJ mol−1 | −3.27 × 106 KJ mol−1 | −6.56 × 106 KJ mol−1 | ||
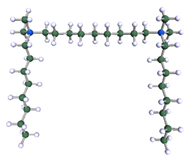 | −5.46 × 10−15 J | −5.43 × 10−15 J | −1.09 × 10−14 J | |
| −3.29 × 106 KJ mol−1 | −3.27 × 106 KJ mol−1 | −6.56 × 106 KJ mol−1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Łudzik, K.; Woloszczuk, S.; Zając, W.; Jazdzewska, M.; Rogachev, A.; Kuklin, A.I.; Zawisza, A.; Jóźwiak, M. Can the Isothermal Calorimetric Curve Shapes Suggest the Structural Changes in Micellar Aggregates? Int. J. Mol. Sci. 2020, 21, 5828. https://doi.org/10.3390/ijms21165828
Łudzik K, Woloszczuk S, Zając W, Jazdzewska M, Rogachev A, Kuklin AI, Zawisza A, Jóźwiak M. Can the Isothermal Calorimetric Curve Shapes Suggest the Structural Changes in Micellar Aggregates? International Journal of Molecular Sciences. 2020; 21(16):5828. https://doi.org/10.3390/ijms21165828
Chicago/Turabian StyleŁudzik, Katarzyna, Sebastian Woloszczuk, Wojciech Zając, Monika Jazdzewska, Andrey Rogachev, Alexander Ivanowicz Kuklin, Anna Zawisza, and Małgorzata Jóźwiak. 2020. "Can the Isothermal Calorimetric Curve Shapes Suggest the Structural Changes in Micellar Aggregates?" International Journal of Molecular Sciences 21, no. 16: 5828. https://doi.org/10.3390/ijms21165828
APA StyleŁudzik, K., Woloszczuk, S., Zając, W., Jazdzewska, M., Rogachev, A., Kuklin, A. I., Zawisza, A., & Jóźwiak, M. (2020). Can the Isothermal Calorimetric Curve Shapes Suggest the Structural Changes in Micellar Aggregates? International Journal of Molecular Sciences, 21(16), 5828. https://doi.org/10.3390/ijms21165828