Seismic Assessment of Footbridges under Spatial Variation of Earthquake Ground Motion (SVEGM): Experimental Testing and Finite Element Analyses
Abstract
1. Introduction
2. Theoretical Background of Spatial Variation of Earthquake Ground Motion
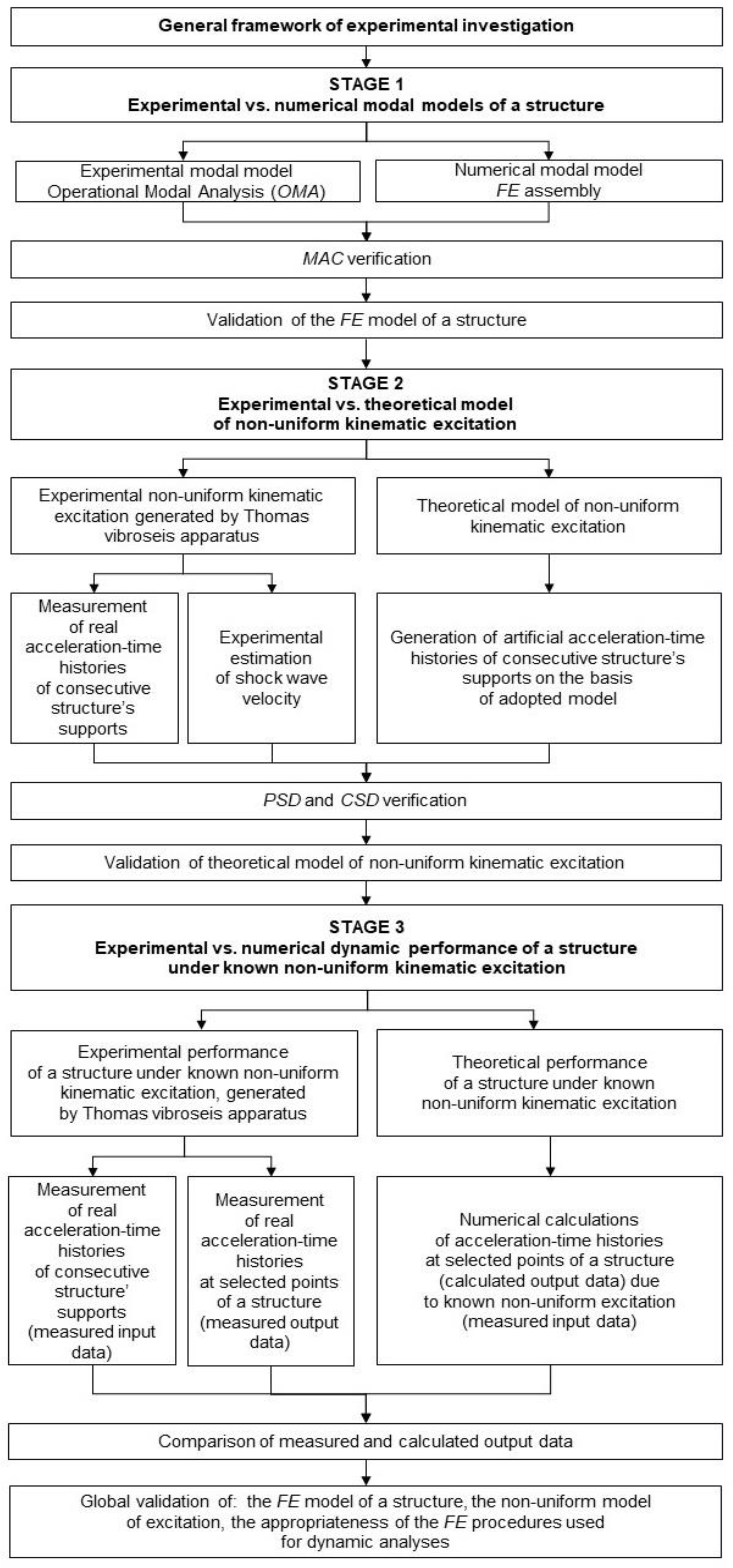
3. General Framework of the Research
- The experimental modal models of both footbridges were created employing the operational modal analysis (OMA) techniques.
- The numerical modal models of both footbridges were obtained through assembling the FE models of both footbridges and the calculation of dynamic properties of both structures.
- The validation of the FE models of both footbridges with regard to the results of in situ experiment was carried out using the modal assurance criterion (MAC) theory.
- The experimental measurements of bridges’ responses resulting from nonuniform kinematic excitation generated by the vibroseis, i.e., measurements of acceleration-time histories on the consecutive structural supports and estimation of shock wave velocities on the basis of the cross-correlation (CCr) functions of the acceleration-time histories registered at two consecutive structures’ supports.
- The generation of artificial acceleration-time histories for the consecutive supports of both footbridges, based on the adopted model of nonuniform kinematic excitation.
- The validation of the theoretical model of nonuniform kinematic excitation through the similarity assessment of the measured and artificial excitations using the power spectral density (PSD) and the cross-spectral density (CSD) functions.
- The experimental measurements of the dynamic responses of both footbridges (in terms of acceleration-time histories registered at selected output measurement points of the structures) under nonuniform excitation of known amplitudes and frequency range, generated by the vibroseis placed in close proximity to a footbridge.
- The numerical recognition of the dynamic responses of footbridges (in terms of acceleration-time histories calculated at selected output measurement points of the structures) under known nonuniform excitation generated by the vibroseis.
- The validation of both the FE models of both footbridges and the nonuniform model of excitation as well as the FE procedures used for dynamic analyses through the comparison of the measured and calculated dynamic responses of both footbridges.
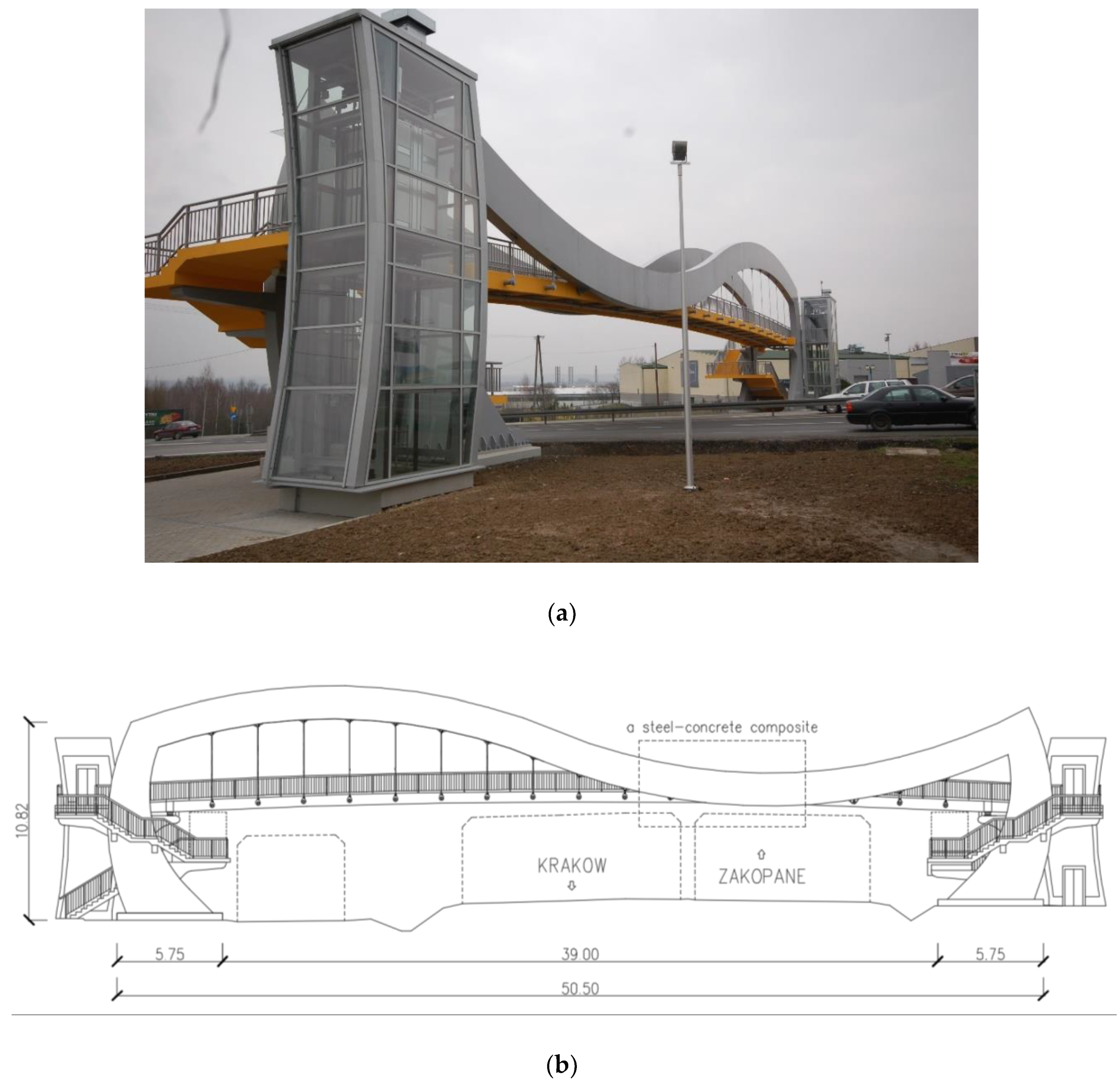
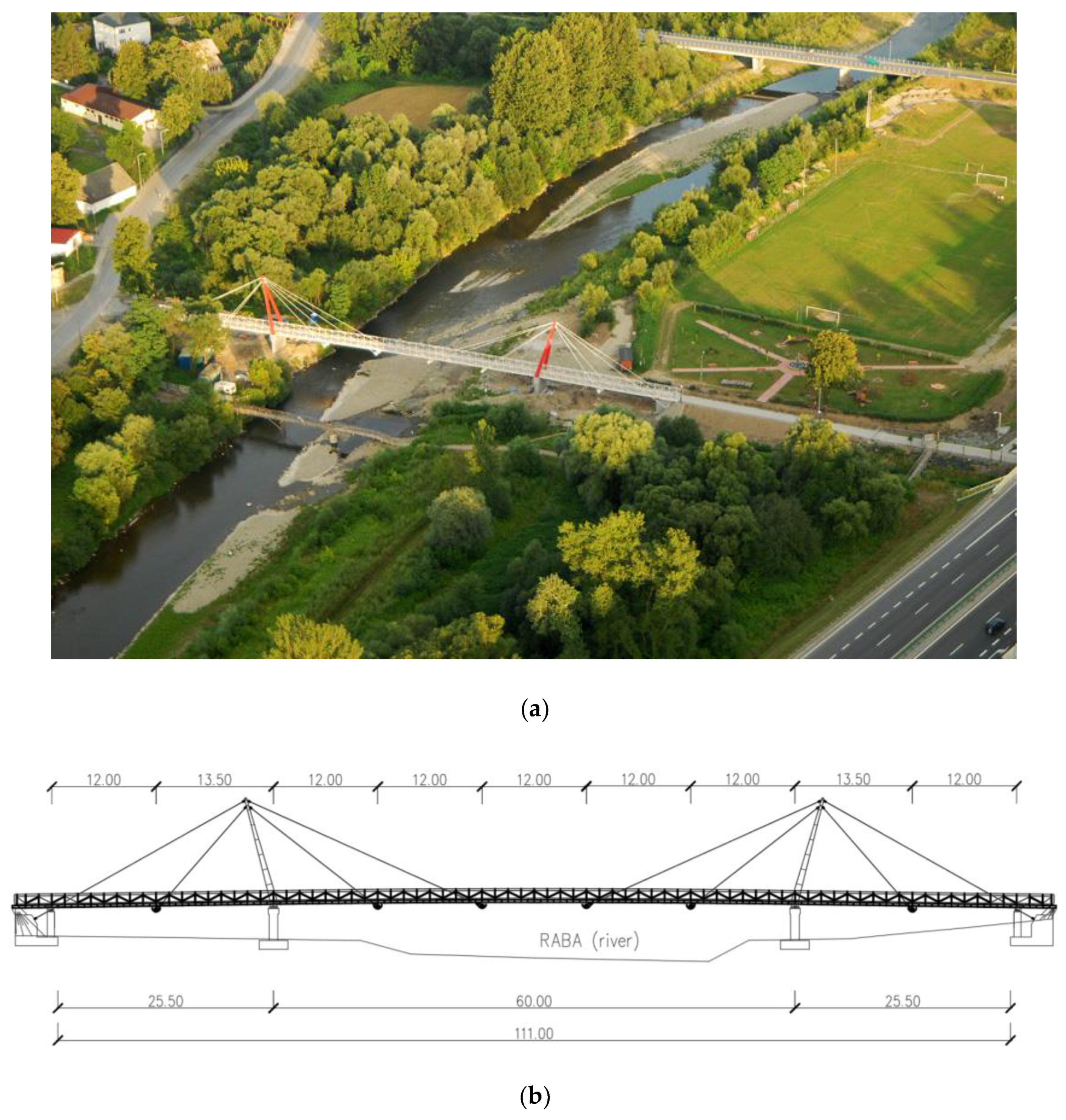
4. Structural Layouts and FE Models of the Footbridges
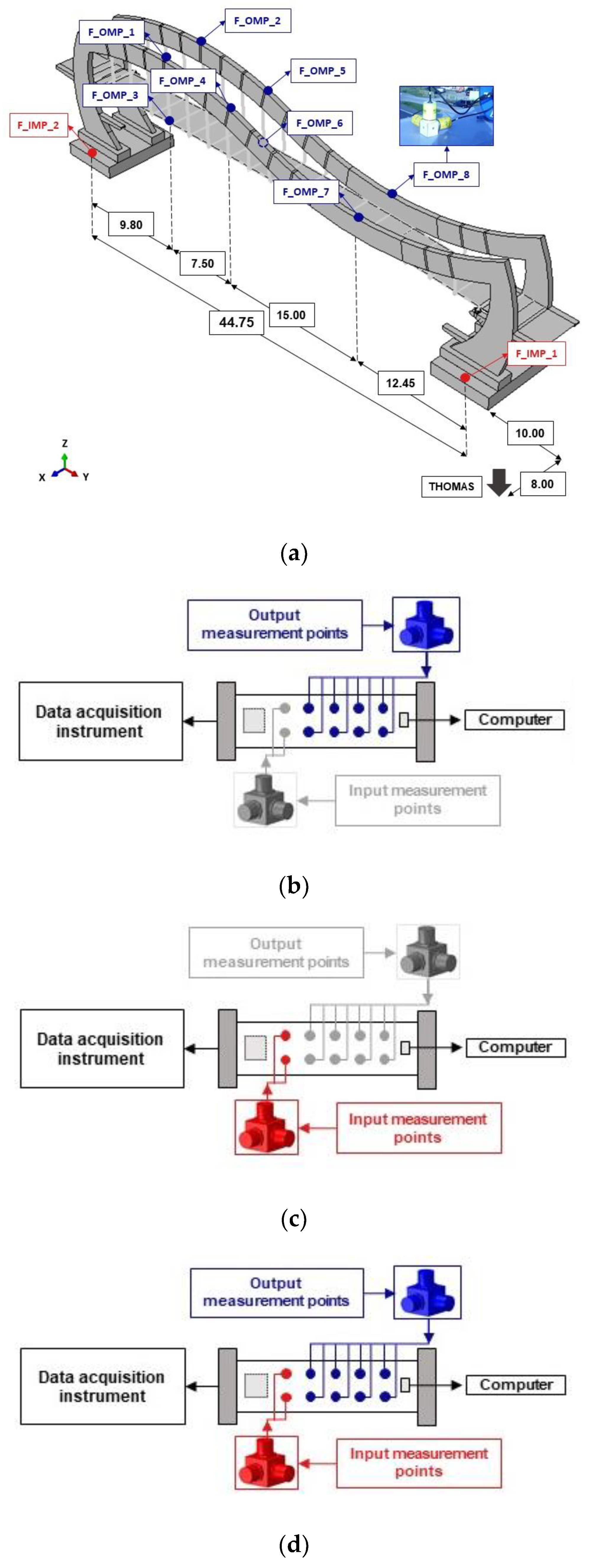
5. Experimental Set-Up
6. Theoretical Background for In Situ Tests
7. Results of in Situ Experiments and Discussion
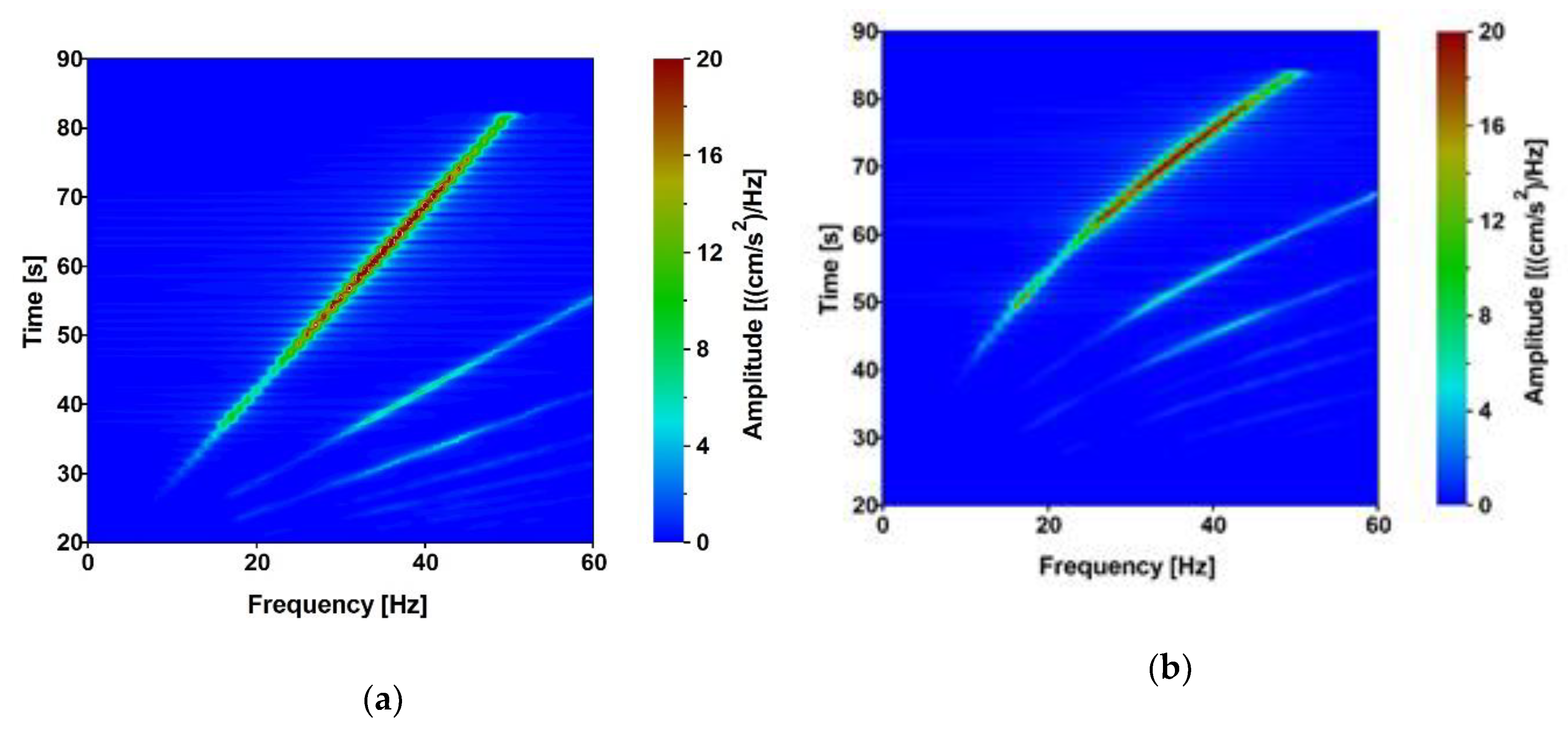
7.1. Stage 1: Experimental vs. Numerical Modal Models of the Structures
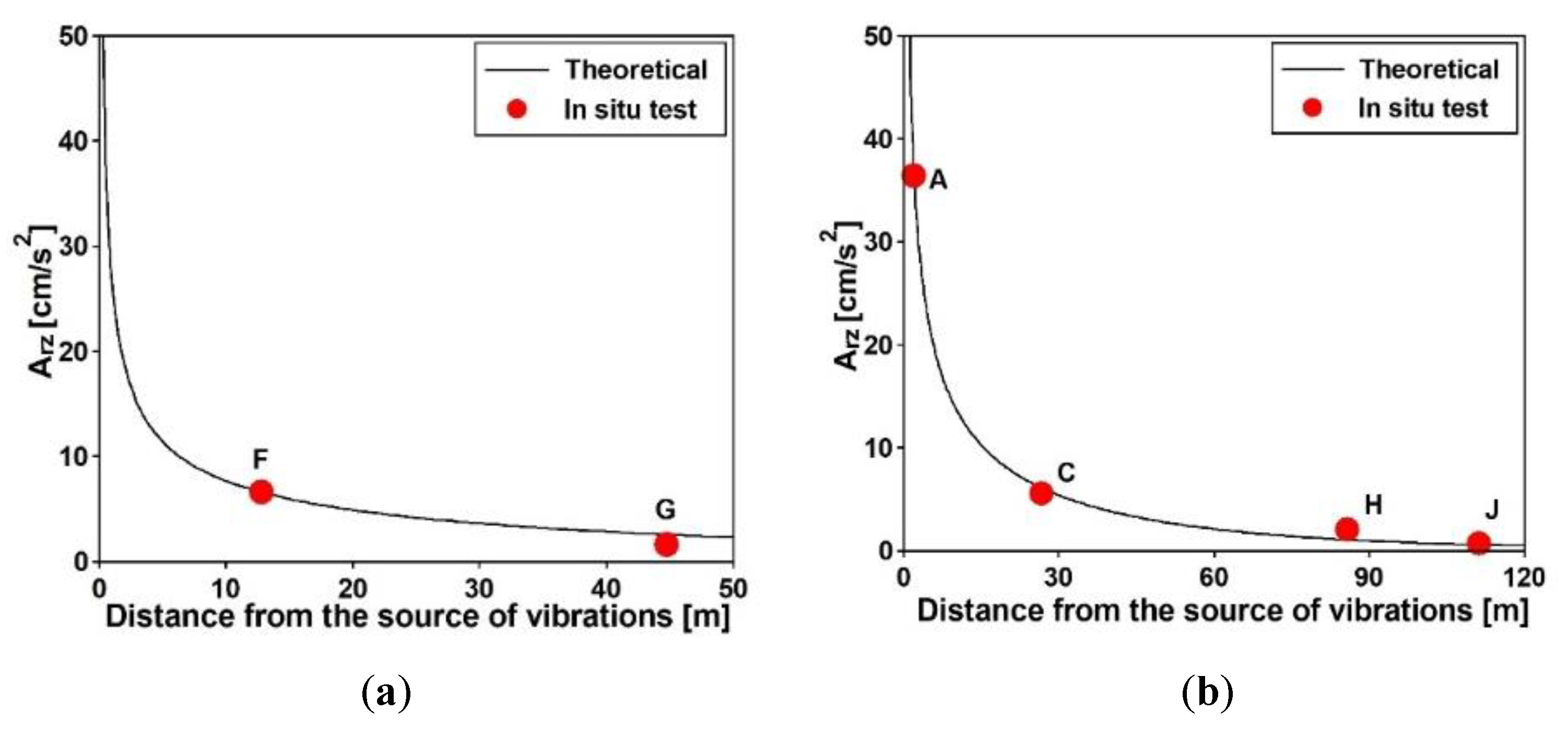
7.2. Stage 2: Experimental vs. Theoretical Model of Nonuniform Kinematic Excitation
7.3. Stage 3: Experimental vs. Numerical Dynamic Performance of Footbridges under Known Nonuniform Kinematic Excitation
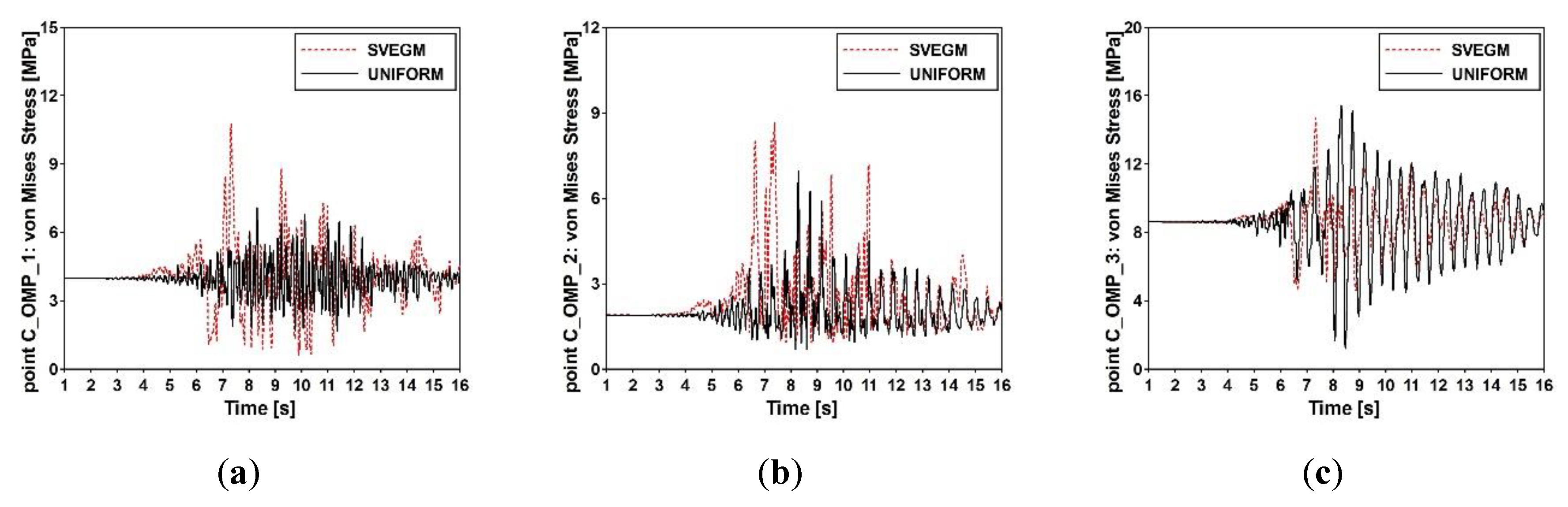
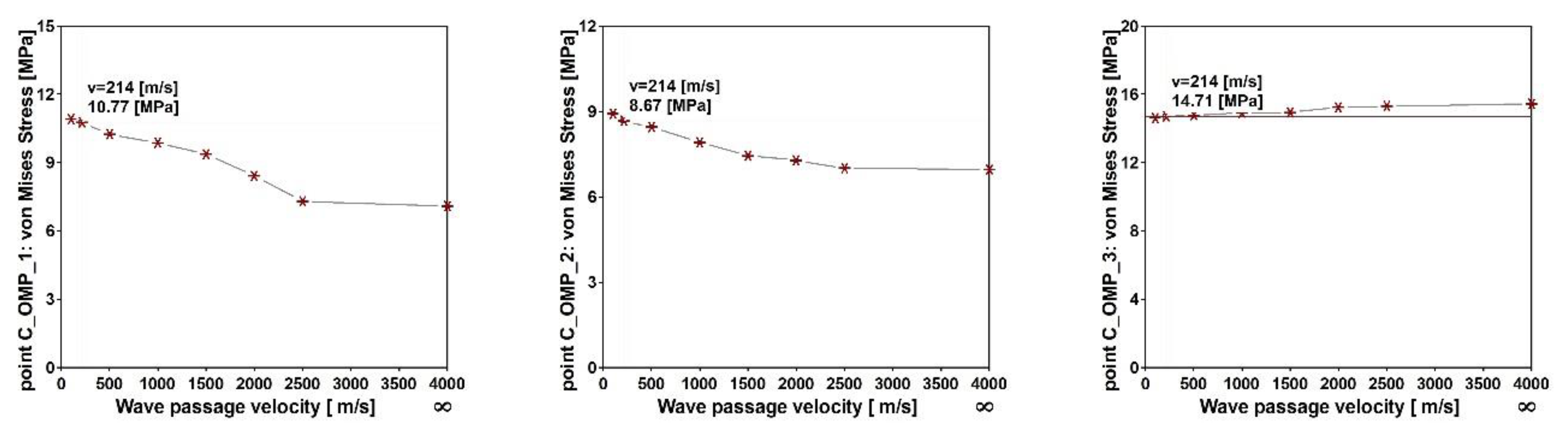
8. Dynamic Performance of the Footbridges under a Selected Seismic Shock—Practical Application of the Measurement Results of the SVEGM Effect
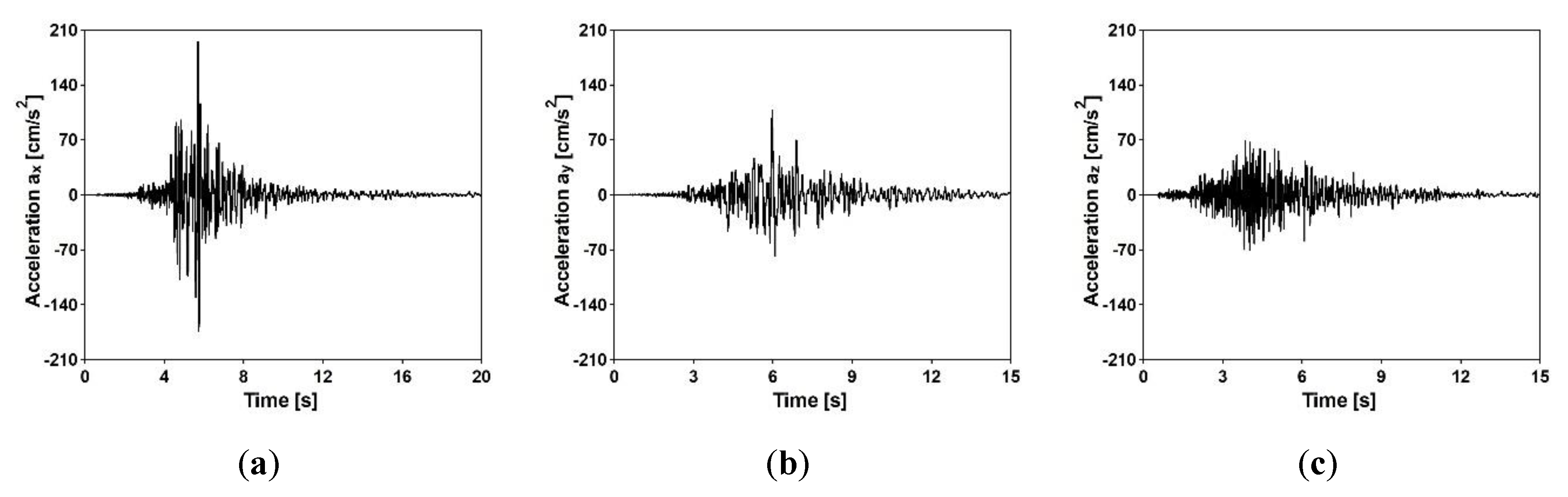
8.1. Seismic Event Chosen for Calculations
8.2. Dynamic Performance of the Single-Span Steel Frame Footbridge
8.3. Dynamic Performance of the Three-Span Cable-Stayed Footbridge
9. Conclusions
- The numerical modal models of both footbridges, obtained through assembling the FE models of footbridges and the through the calculation of the dynamic properties, were positively validated with regard to the experimental modal models obtained through the operational modal analysis (OMA) techniques. The differences between the experimental and numerical natural frequencies were generally less than 10%. The comparison of the experimental and numerical mode shapes also revealed a good agreement between them since the values of indices were satisfactory for both structures.
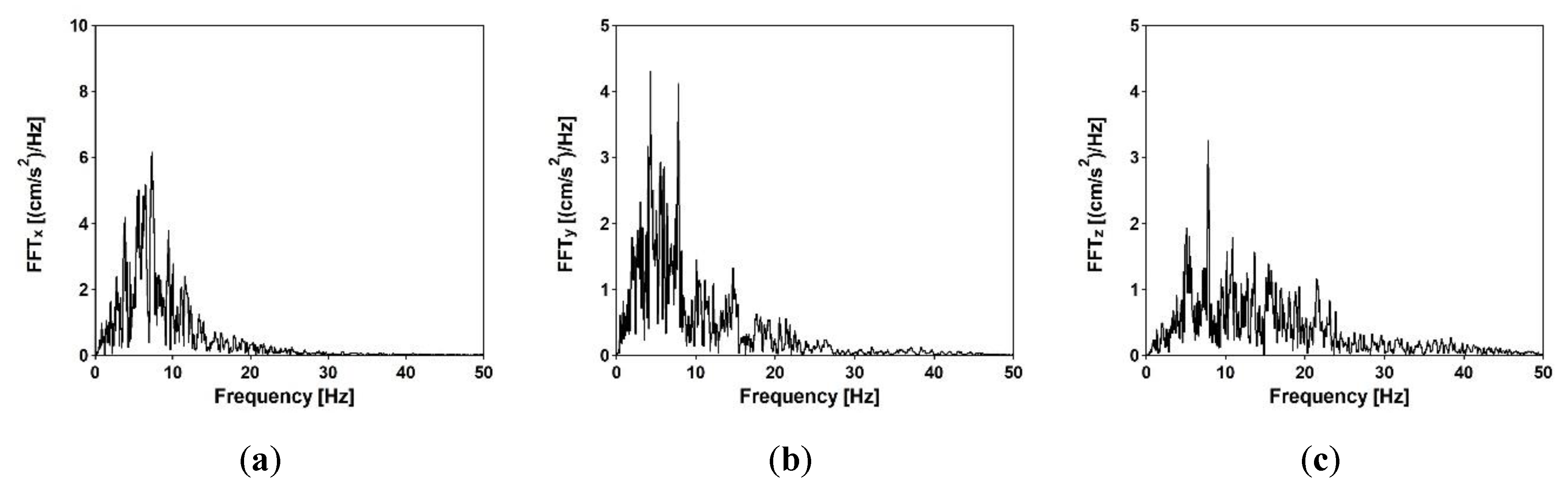
- The non-destructive test with the vibroseis generating kinematic excitation was carried out. On the basis of the signals collected at all footbridges’ supports, nonuniform kinematic excitation was detected. The apparent wave velocities, obtained on the basis of cross-correlation functions between the acceleration-time histories at the consecutive supports of structures, are representative for the clayey subsoils of both the frame and the cable-stayed footbridge. Also, the coherence functions between these signals were extracted. The similarity and the frequency consistency of the data recorded at the input points was satisfactory, especially for the frequency range of 5 to 15 Hz. Therefore, for seismic signals with such dominant frequency range, the adopted model of nonuniform kinematic excitation, in which the loss of coherence is neglected, can be appropriate.
- The artificial kinematic excitation was generated on the basis of the adopted model of nonuniform kinematic excitation. The obtained PSD functions of the acceleration-time histories registered at all supports as well as the CSD functions between the registered and artificial acceleration-time histories for all supports confirmed the strong similarity of the measured and numerical signals. Therefore, the adopted model of nonuniform kinematic excitation was positively validated with regard to the experimentally detected nonuniform excitation.
- The experimental and numerical assessments of the footbridges performance under the known dynamic excitation induced by the vibroseis were carried out. As the overall agreement is satisfactory, the FE models and procedures were positively validated by linking full-scale experiments and numerical calculations. Therefore, the numerical performance of structures reflects the real dynamic performance under known nonuniform excitation.
- For the stiff and relatively short single-span steel frame footbridge, the maximum structural response was obtained for the model of nonuniform kinematic excitation with the lowest seismic wave velocity. For the model of uniform seismic excitation, the response was the weakest. The most significant influence of the SVEGM effect was observed when the points were localized closely to the stiff node of the steel frame. The less significant differences were observed at points located in the middle of the upper beam of the frame. Therefore, in case of stiff frame footbridges the pseudo-static effects resulting from the nonuniformity of excitation enhance the dynamic response of a structure, especially disturbing zones located closely to stiff supports.
- For the flexible cable-stayed footbridge, two different scenarios were observed. For the output points located in the extreme spans, as well as in the midspan closely to the pillars, the maximum response occurred for the model of nonuniform kinematic excitation with the lowest wave velocity. For the output points located in the middle of the main span, the numerical simulations with the model of uniform excitation provided more conservative results. Therefore, in case of flexible cable-stayed footbridges the pseudo-static effects originated from SVEGM disturb zones located closely to the structure supports, whereas in the midspan of the structure the inertial effects caused by the uniform excitation are stronger.
- It is reasonable to consider the SVEGM effect for the seismic assessments of footbridges since this phenomenon may generate non-conservative results in comparison with results of classic analysis in which uniform seismic excitation is assumed.
Author Contributions
Funding
Conflicts of Interest
References
- Zivanovic, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Van Nimmen, K.; Lombaert, G.; De Roeck, G.; Van den Broeck, P. Vibration serviceability of footbridges: Evaluation of the current codes of practice. Eng. Struct. 2014, 59, 448–461. [Google Scholar] [CrossRef]
- Piccardo, G.; Tubino, F. Equivalent spectral model and maximum dynamic response for the serviceability analysis of footbridges. Eng. Struct. 2012, 40, 445–456. [Google Scholar] [CrossRef]
- Drygala, I.J.; Polak, M.A.; Dulinska, J.M. Vibration serviceability assessment of GFRP pedestrian bridges. Eng. Struct. 2019, 184, 176–185. [Google Scholar] [CrossRef]
- Sun, Q.; Yan, W.J.; Ren, W.X.; Liu, L.L. Application of transmissibility measurements to operational modal analysis of railway, highway, and pedestrian cable-stayed bridges. Measurement 2019, 148, 106880. [Google Scholar] [CrossRef]
- Drygala, I.J.; Dulinska, J.M. Full-scale experimental and numerical investigations on the modal parameters of a single-span steel-frame footbridge. Symmetry 2019, 11, 404. [Google Scholar] [CrossRef]
- Drygala, I.J.; Dulinska, J.M. A theoretical and experimental evaluation of the modal properties of a cable-stayed footbridge. Procedia Eng. 2017, 199, 2937–2942. [Google Scholar] [CrossRef]
- Wotherspoon, L.; Bradshaw, A.; Green, R.; Wood, C.; Palermo, A.; Cubrinovski, M.; Bradley, B. Performance of Bridges during the 2010 Darfield and 2011 Christchurch Earthquakes. Seismol. Res. Lett. 2011, 82, 950–964. [Google Scholar] [CrossRef]
- Zerva, A. Effect of spatial variability and propagation of seismic ground motions on the response of multiply supported structures. Probabilistic Eng. Mech. 1991, 6, 212–221. [Google Scholar] [CrossRef]
- Zerva, A. On the spatial variation of seismic ground motions and its effects on lifelines. Eng. Struct. 1994, 16, 534–546. [Google Scholar] [CrossRef]
- Zerva, A. Spatial Variation of Seismic Ground Motions: Modeling and Engineering Applications; CRC Press/Balkema–Taylor & Francis Group: Boca Raton, FL, USA, 2009. [Google Scholar]
- Jia, J. Modern Earthquake Engineering; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Abrahamson, N.A.; Bolt, B.A.; Darragh, R.B.; Penzien, J.; Tsai, Y.B. The SMART I Accelerograph Array (1980–1987): A Review. Earthq. Spectra 1987, 3, 263–287. [Google Scholar] [CrossRef]
- Burdette, N.J.; Elnashai, A.S.; Lupoi, A.; Sextos, A.G. Effect of asynchronous earthquake motion on complex bridges. I: Methodology and input motion. J. Bridge Eng. 2008, 13, 158–165. [Google Scholar] [CrossRef]
- Sextos, A.G.; Kappos, A.J. Evaluation of seismic response of bridges under asynchronous excitation and comparisons with Eurocode 8-2 provisions. Bull. Earthq. Eng. 2009, 7, 519–545. [Google Scholar] [CrossRef]
- Leger, P.; Idé, M.I.; Paultre, P. Multiple-support seismic analysis of large structures. Comput. Struct. 1990, 36, 1153–1158. [Google Scholar] [CrossRef]
- Zembaty, Z. Vibrations of bridge structure under kinematic wave excitations. J. Struct. Eng. 1997, 123, 479–487. [Google Scholar] [CrossRef]
- Sextos, A.G.; Karakostas, C.; Lekidis, V.; Papadopoulos, S. Multiple support seismic excitation of the Evripos bridge based on free-field and on-structure recordings. Struct. Infrastruct. Eng. 2015, 11, 1510–1523. [Google Scholar] [CrossRef]
- Davoodii, M.; Jafari, M.K.A.; Sadrolddini, S.M. Effect of multi support excitation on seismic response of embankment dams. Int. J. Civ. Eng. 2013, 11, 19–28. [Google Scholar]
- Tsinidis, G.; Di Sarno, L.; Sextos, A.; Furtner, P. A critical review on the vulnerability assessment of natural gas pipelines subjected to seismic wave propagation. Part 2: Pipe analysis aspects. Tunn. Undergr. Space Technol. 2019, 92, 103056. [Google Scholar] [CrossRef]
- Miao, Y.; Yao, E.; Ruan, B.; Zhuang, H. Seismic response of shield tunnel subjected to spatially varying earthquake ground motions. Tunn. Undergr. Space Technol. 2018, 77, 216–226. [Google Scholar] [CrossRef]
- Jankowski, R.; Wilde, K. A simple method of conditional random field simulation of ground motions for long structures. Eng. Struct. 2000, 22, 552–561. [Google Scholar] [CrossRef]
- Sextos, A. 22—Effect of soil–structure interaction and spatial variability of ground motion on seismic risk assessment of bridges. In Handbook of Seismic Risk Analysis and Management of Civil Infrastructure Systems; Woodhead Publishing Series in Civil and Structural Engineering: Cambridge, UK, 2013; pp. 597–625. [Google Scholar]
- Eurocode, C.E.N. 8: Design of Structures for Earthquake Resistance—Part 2: Bridges. EN 1998, 2, 2004. [Google Scholar]
- Clough, R.W.; Penzien, J. Dynamics of Structures, 2nd ed.; McGraw-Hill: New York, NY, USA, 1993. [Google Scholar]
- Richart, F.E.; Woods, R.D.; Hall, J.R. Vibrations of Soils and Foundations, Englewood Cliffs; Prentice-Hall: Upper Saddle River, NJ, USA, 1970. [Google Scholar]
- Simulia ABAQUS. 2013. Users’ Manual Version 6.13 Documentation; Dassault Systemes: Velizy-Villacoublay, France, 2015. [Google Scholar]
- Buckle, I.; Nagarajaiah, S.; Ferrell, K. Stability of Elastomeric Isolation Bearings: Experimental Study. J. Struct. Eng. 2002, 128, 3–11. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory, Practise and Application, 2nd ed.; Research Studies Press Ltd.: Philadelphia, PA, USA, 2000. [Google Scholar]
- Bianchi, G.; Sorrentino, R. Electronic Filter Simulation & Design; McGraw-Hill Education: New York, NY, USA, 2007. [Google Scholar]
- Luzi, L.; Pacor, F.; Puglia, R. Italian Accelerometric Archive v 2.1. Istituto Nazionale di Geofisica e Vulcanologia, Dipartimento della Protezione Civile Nazionale 2016. [Google Scholar] [CrossRef]
- Meroni, F.; Squarcina, T.; Pessina, V.; Locati, M.; Modica, M.; Zoboli, R. A damage scenario for the 2012 Northern Italy Earthquakes and estimation of the economic losses to residential buildings. Int. J. Disaster Risk Sci. 2017, 8, 326–341. [Google Scholar] [CrossRef]
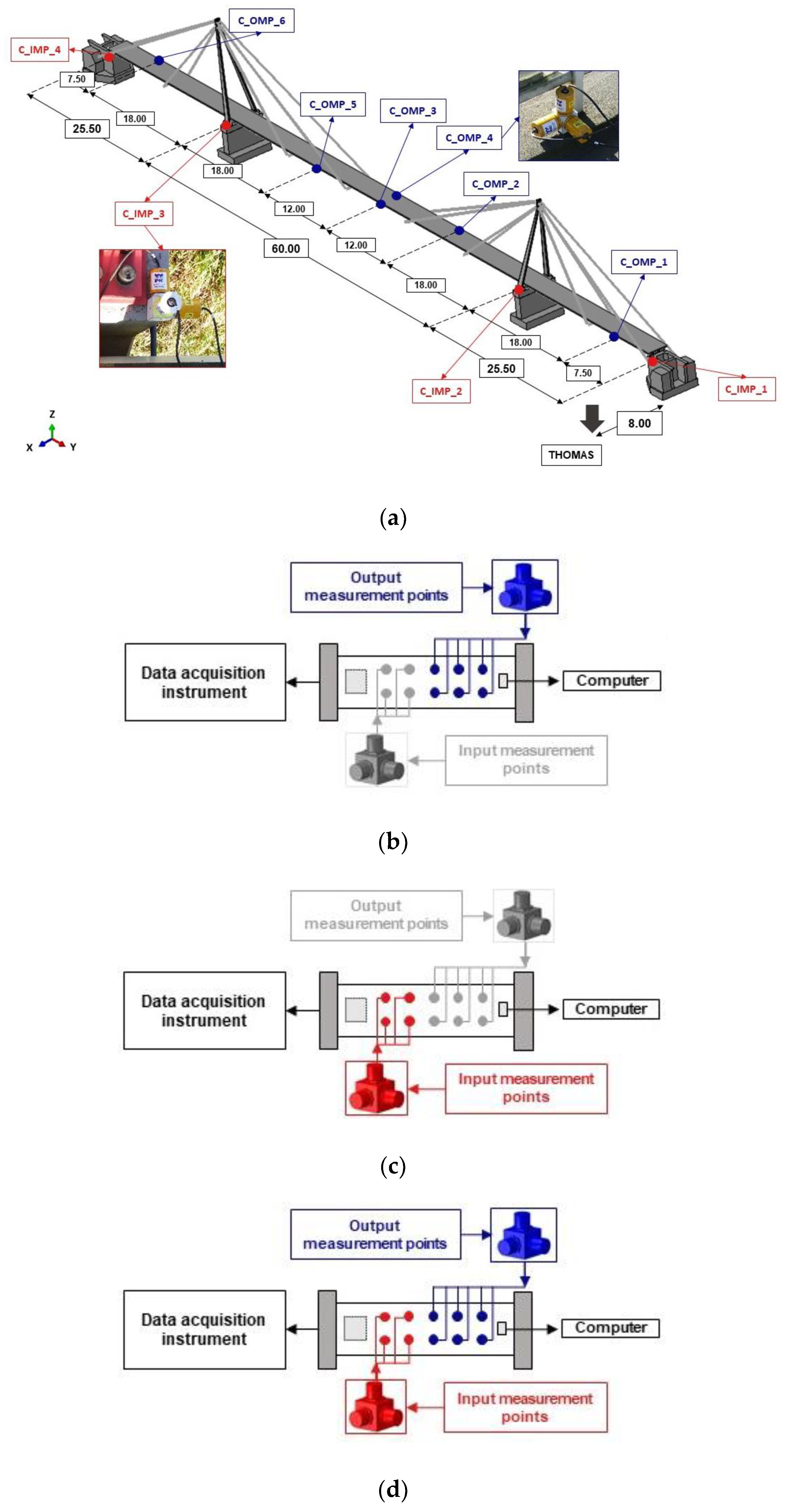















| The Single-Span Steel frame Footbridge—Bridge One | |||||
| Mode | Natural Frequency fi [Hz] | Differences [%] | MACii [–] | Logarithmic Decrement [–] | |
| FE Analysis | OMA | ||||
| 1 | 1.71 | 1.76 | 2.92 | 0.92 | 0.131 |
| 2 | 2.45 | 2.68 | 9.39 | 0.84 | 0.101 |
| 3 | 3.33 | 3.15 | 5.41 | 0.80 | 0.056 |
| 4 | 3.41 | 4.19 | 22.87 | 0.78 | 0.035 |
| 5 | 4.40 | 4.47 | 1.59 | 0.83 | 0.025 |
| The Three-Span Cable-Stayed Footbridge—Bridge Two | |||||
| Mode | Natural Frequency fi [Hz] | Differences [%] | MACii [–] | Logarithmic Decrement [–] | |
| FE Analysis | OMA | ||||
| 1 | 1.93 | 1.95 | 1.04 | 0.95 | 0.092 |
| 2 | 2.21 | 2.41 | 9.05 | 0.94 | 0.079 |
| 3 | 2.61 | 2.58 | 1.15 | 0.94 | 0.077 |
| 4 | 3.86 | 3.69 | 4.40 | 0.91 | 0.075 |
| 5 | 4.37 | 4.28 | 2.06 | 0.72 | 0.033 |
| Method | Acceleration [cm/s2] | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Axis | Output Measurement Point (F_OMP) | ||||||||
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | ||
| In situ experiment | X | 5.98 | 6.80 | 3.52 | 3.02 | 4.39 | 5.77 | 4.48 | 3.67 |
| Y | 3.49 | 2.75 | 4.05 | 3.29 | 6.90 | 5.23 | 6.91 | 5.69 | |
| Z | 12.49 | 9.14 | 21.08 | 6.88 | 9.82 | 5.67 | 4.89 | 4.79 | |
| FE analysis | X | 5.38 | 5.91 | 3.82 | 3.39 | 4.89 | 6.06 | 4.98 | 4.07 |
| Y | 3.91 | 3.04 | 3.93 | 3.15 | 7.54 | 5.38 | 6.31 | 5.97 | |
| Z | 12.32 | 11.59 | 18.37 | 7.94 | 8.27 | 6.91 | 5.59 | 5.54 | |
| Method | Acceleration [cm/s2] | ||||||
|---|---|---|---|---|---|---|---|
| Direction | Output Measurement Point (C_OMP) | ||||||
| 1 | 2 | 3 | 4 | 5 | 6 | ||
| In situ experiment | X | 7.14 | 8.04 | 6.81 | 6.17 | 9.35 | 5.74 |
| Y | 7.01 | 8.48 | 6.37 | 6.67 | 6.90 | 8.47 | |
| Z | 23.74 | 17.72 | 31.12 | 28.37 | 24.80 | 28.18 | |
| FE analysis | X | 8.23 | 9.87 | 7.55 | 7.49 | 11.42 | 7.38 |
| Y | 8.99 | 10.52 | 7.79 | 7.21 | 8.51 | 11.37 | |
| Z | 29.72 | 21.72 | 35.31 | 36.25 | 28.37 | 29.73 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Drygala, I.J.; Dulinska, J.M.; Polak, M.A. Seismic Assessment of Footbridges under Spatial Variation of Earthquake Ground Motion (SVEGM): Experimental Testing and Finite Element Analyses. Sensors 2020, 20, 1227. https://doi.org/10.3390/s20041227
Drygala IJ, Dulinska JM, Polak MA. Seismic Assessment of Footbridges under Spatial Variation of Earthquake Ground Motion (SVEGM): Experimental Testing and Finite Element Analyses. Sensors. 2020; 20(4):1227. https://doi.org/10.3390/s20041227
Chicago/Turabian StyleDrygala, Izabela Joanna, Joanna Maria Dulinska, and Maria Anna Polak. 2020. "Seismic Assessment of Footbridges under Spatial Variation of Earthquake Ground Motion (SVEGM): Experimental Testing and Finite Element Analyses" Sensors 20, no. 4: 1227. https://doi.org/10.3390/s20041227
APA StyleDrygala, I. J., Dulinska, J. M., & Polak, M. A. (2020). Seismic Assessment of Footbridges under Spatial Variation of Earthquake Ground Motion (SVEGM): Experimental Testing and Finite Element Analyses. Sensors, 20(4), 1227. https://doi.org/10.3390/s20041227







