Stability Analysis and Design of n-DOF Vibration Systems Containing Both Semi-Active and Passive Mechanical Controllers
Abstract
1. Introduction
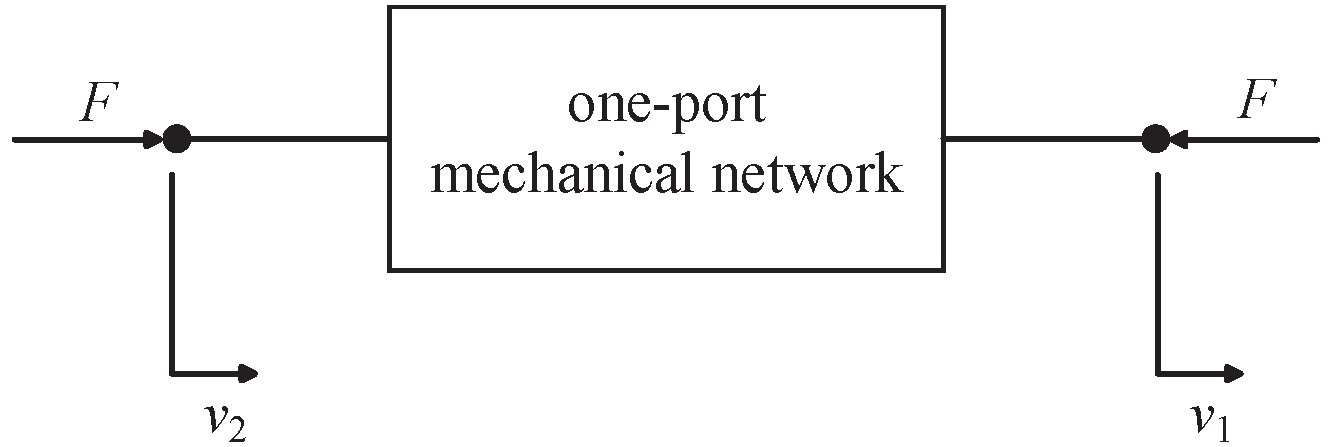
2. Preliminaries
- 1.
- and for any ,
- 2.
- ,
- 3.
- for any nonzero initial state, that is, ,
- 4.
- with ,
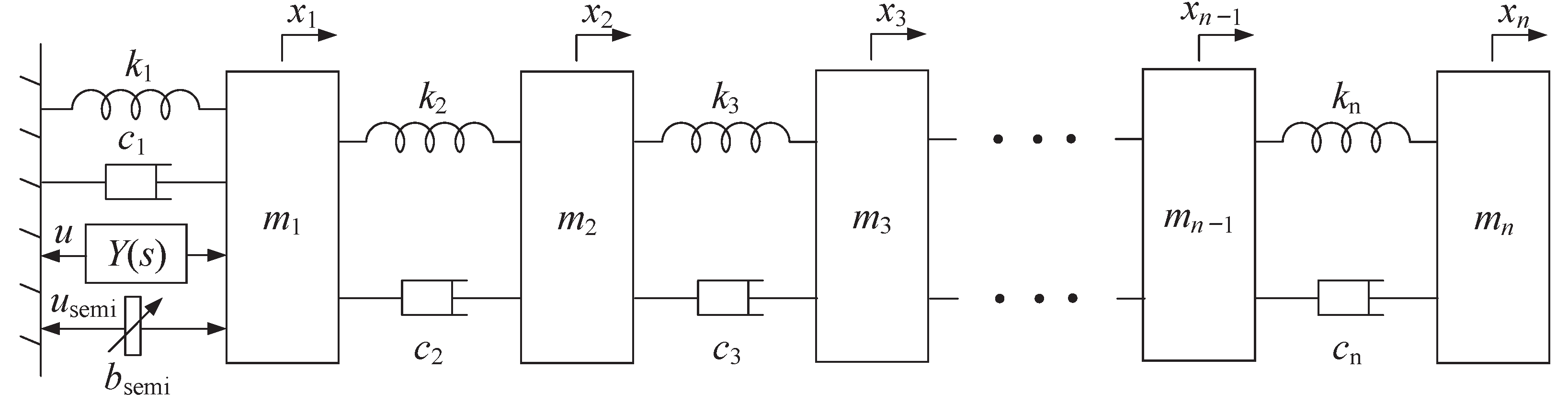
3. Model Formulation
4. Stability Analysis
5. Optimization Design
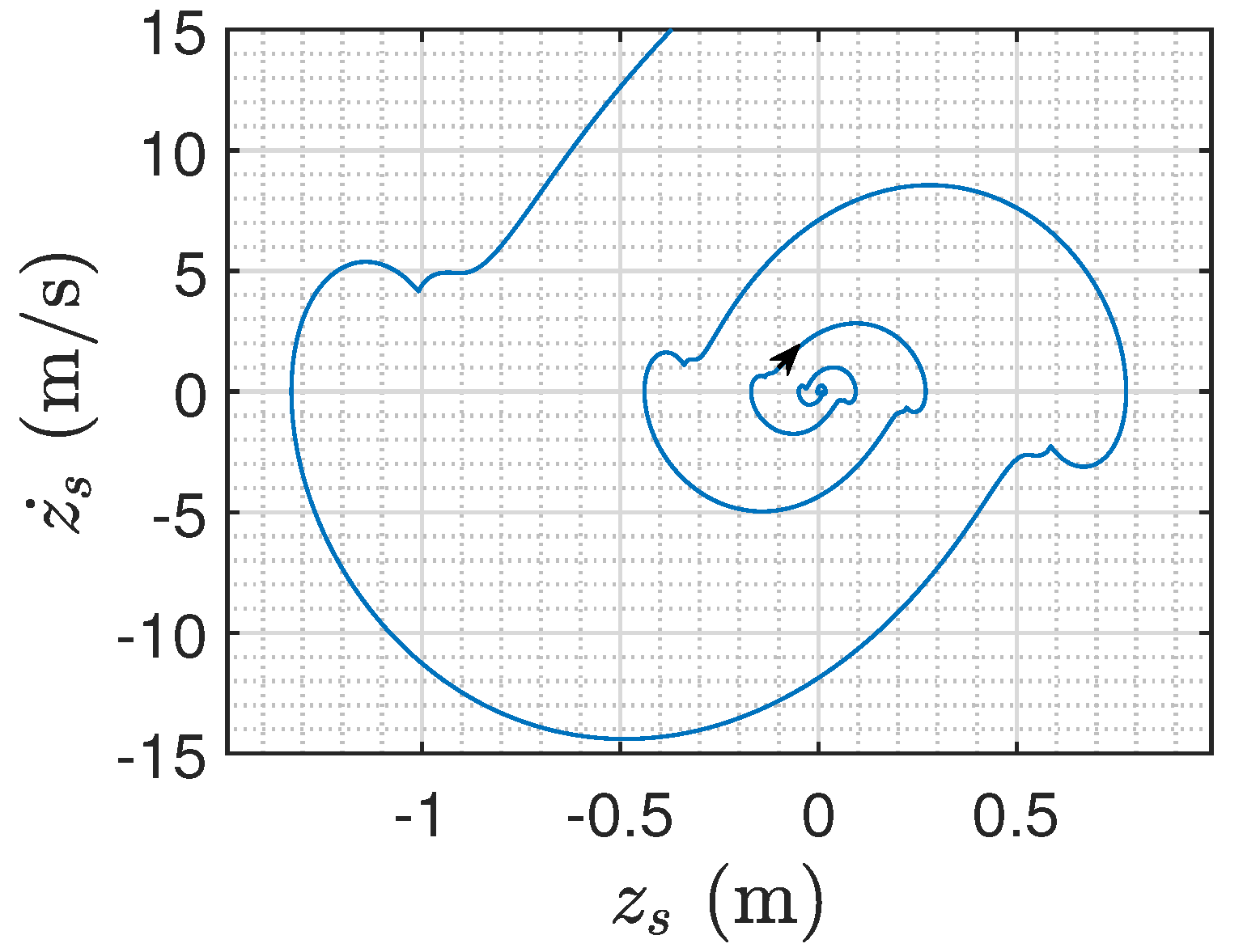
5.1. Quarter-Car Suspension Control System
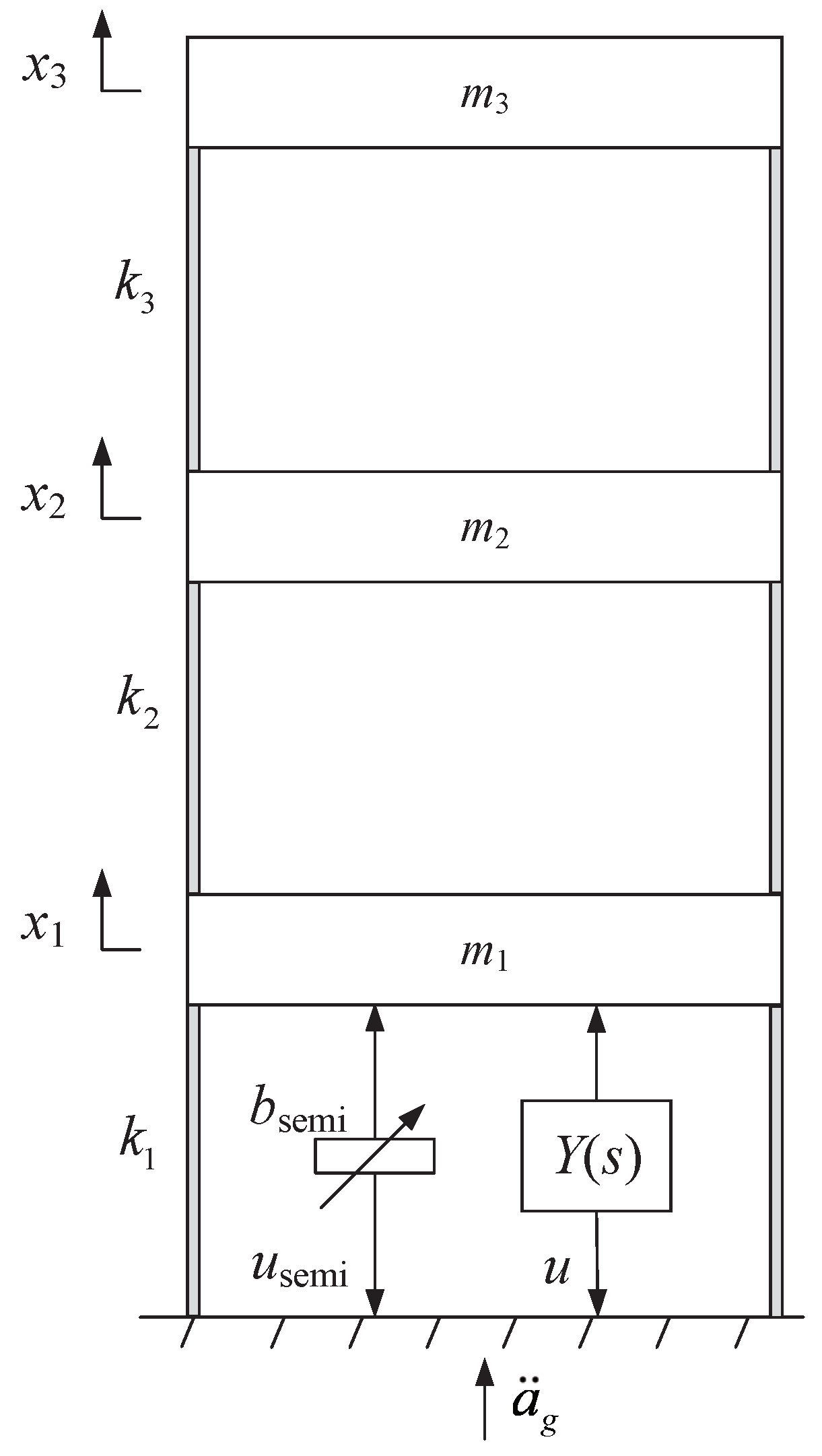
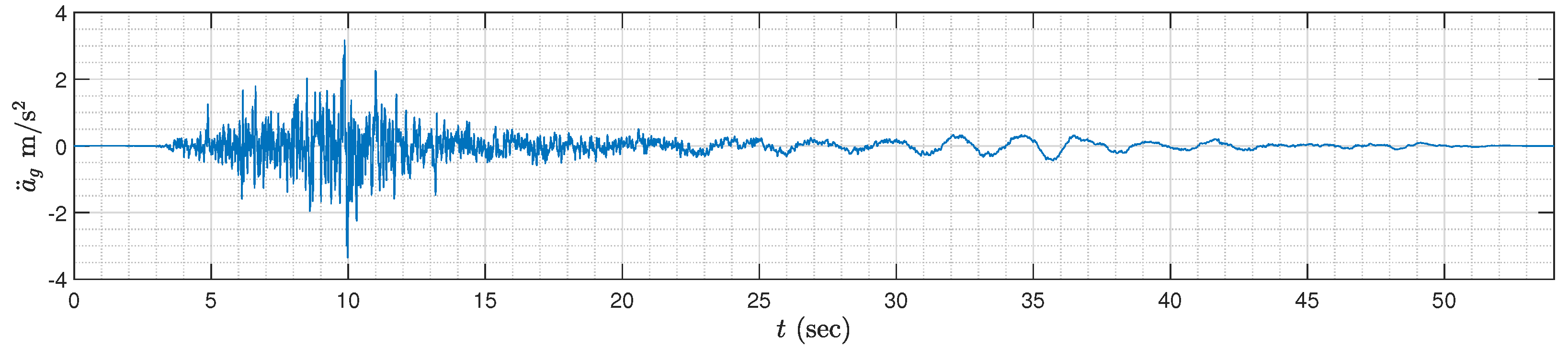
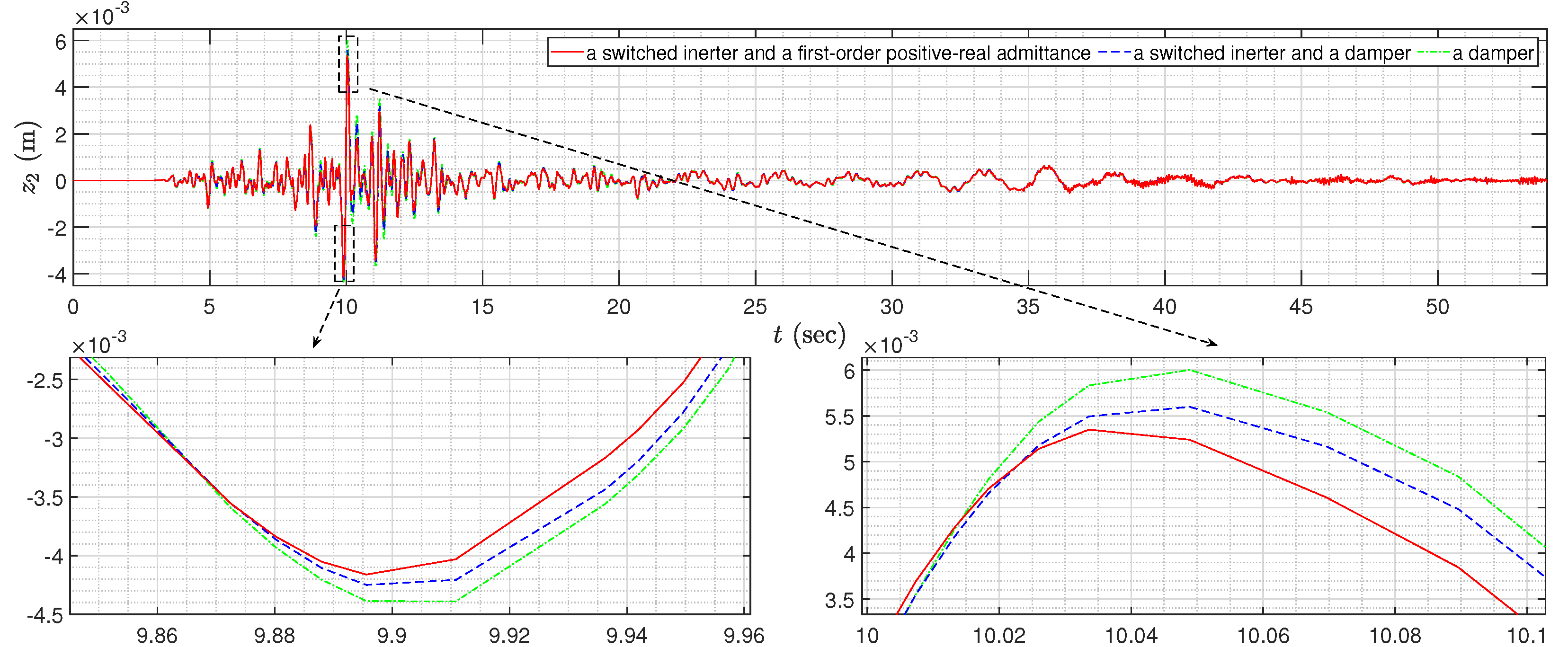
5.2. Three-Storey Building Vibration Control System
6. Conclusions
- Based on Lyapunov’s stability theory, a sufficient condition for the global asymptotic stability of a general n-DOF mass-chain vibration system, whose semi-active and passive controllers can be installed between any two adjacent masses, has been derived. Similarly, we have obtained a sufficient condition for a specific n-DOF mass-chain vibration system to be globally asymptotically stable.
- The stability conditions derived in this paper have been applied to the optimization designs of a vehicle suspension control system and a building vibration control system, respectively. The results reveal that simultaneously applying semi-active and passive mechanical controllers to vibration systems can clearly enhance system performances compared with the conventional semi-active or passive mechanical control methods.
- The research in this paper can provide important guidance for further investigation of the stability problems of more general systems, which is motivated by the designs of many vibration systems in practice.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Smith, M.C. Synthesis of mechanical networks: The inerter. IEEE Trans. Autom. Control 2002, 47, 1648–1662. [Google Scholar] [CrossRef]
- Smith, M.C. The inerter: A retrospective. Annu. Rev. Control Robot. Auton. Syst. 2020, 3, 361–391. [Google Scholar] [CrossRef]
- Wang, F.C.; Hong, M.F.; Lin, T.C. Designing and testing a hydraulic inerter. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 66–72. [Google Scholar] [CrossRef]
- Xu, S.; He, B. A compliance modeling method of flexible rotary joint for collaborative robot using passive network synthesis theory. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2022, 236, 4038–4048. [Google Scholar] [CrossRef]
- Wang, K.; Chen, M.Z.Q.; Liu, F. Series-parallel mechanical circuit synthesis of a positive-real third-order admittance using at most six passive elements for inerter-based control. J. Frankl. Inst. 2023, 360, 5442–5480. [Google Scholar] [CrossRef]
- Wang, K.; Chen, M.Z.Q.; Liu, F. Minimal seven-element series-parallel realizability of a certain positive-real biquadratic impedance. Int. J. Circuit Theory Appl. 2023, 51, 4043–4070. [Google Scholar] [CrossRef]
- Hughes, T.H. A theory of passive linear systems with no assumptions. Automatica 2017, 86, 87–97. [Google Scholar] [CrossRef]
- Hughes, T.H.; Branford, E.H. Dissipativity, Reciprocity, and Passive Network Synthesis: From the Seminal Dissipative Dynamical Systems Articles of Jan Willems to The Present Day. IEEE Control Syst. Mag. 2022, 42, 36–57. [Google Scholar] [CrossRef]
- Pates, R. Passive and reciprocal networks: From simple models to simple optimal controllers. IEEE Control Syst. Mag. 2022, 42, 73–92. [Google Scholar] [CrossRef]
- Luan, G.; Liu, P.; Ning, D.; Liu, G.; Du, H. Semi-Active Vibration Control of Seat Suspension Equipped with a Variable Equivalent Inertance-Variable Damping Device. Machines 2023, 11, 284. [Google Scholar] [CrossRef]
- Jin, X.; Chen, M.Z.Q.; Huang, Z. Minimization of the beam response using inerter-based passive vibration control configurations. Int. J. Mech. Sci. 2016, 119, 80–87. [Google Scholar] [CrossRef]
- Li, Y.; Han, S.; Xiong, J.; Wang, W. Comfort-Oriented Semi-Active Suspension Configuration with Inerter-Based Network Synthesis. Actuators 2023, 12, 290. [Google Scholar] [CrossRef]
- Jin, L.; Fan, J.; Du, F.; Zhan, M. Research on Two-Stage Semi-Active ISD Suspension Based on Improved Fuzzy Neural Network PID Control. Sensors 2023, 23, 8388. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Jia, M.; Yang, K.; Chen, Z.; Chen, L. Optimal design and dynamic performance analysis based on the asymmetric-damping vehicle ISD suspension. Shock Vib. 2021, 2021, 9996563. [Google Scholar] [CrossRef]
- Li, P.; Nguyen, A.T.; Du, H.; Wang, Y.; Zhang, H. Polytopic LPV approaches for intelligent automotive systems: State of the art and future challenges. Mech. Syst. Signal Process. 2021, 161, 107931. [Google Scholar] [CrossRef]
- Li, P.; Lam, J.; Cheung, K.C. Motion-based active disturbance rejection control for a non-linear full-car suspension system. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2018, 232, 616–631. [Google Scholar] [CrossRef]
- Chen, Y.; Tai, Y.; Xu, J.; Xu, X.; Chen, N. Vibration analysis of a 1-DOF system coupled with a nonlinear energy sink with a fractional order inerter. Sensors 2022, 22, 6408. [Google Scholar] [CrossRef]
- Wu, L.; Wang, K. Optimization design and stability analysis for a new class of inerter-based dynamic vibration absorbers with a spring of negative stiffness. J. Vib. Control 2024, 30, 822–836. [Google Scholar] [CrossRef]
- Dong, C.; Bas, S.; Catbas, F.N. Applications of Computer Vision-Based Structural Monitoring on Long-Span Bridges in Turkey. Sensors 2023, 23, 8161. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Chen, M.Z.Q. Relationships between vibrations of main device and mechanical network in a classical vibration control system. J. Sound Vib. 2023, 565, 117887. [Google Scholar] [CrossRef]
- Zhao, Z.; Hu, X.; Zhang, R.; Xie, M.; Liu, S. Advantages and design of inerters for isolated storage tanks incorporating soil conditions. Thin-Walled Struct. 2024, 195, 111356. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhao, Z.; Zhang, R.; De Domenico, D.; Pan, C. Optimal design based on analytical solution for storage tank with inerter isolation system. Soil Dyn. Earthq. Eng. 2020, 129, 105924. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Jiang, J.Z.; Neild, S. Optimal configurations for a linear vibration suppression device in a multi-storey building. Struct. Control Health Monit. 2017, 24, e1887. [Google Scholar] [CrossRef]
- Hu, Y.; Chen, M.Z.Q. Low-Complexity Passive Vehicle Suspension Design Based on Element-Number-Restricted Networks and Low-Order Admittance Networks. J. Dyn. Syst. Meas. Control 2018, 140, 101014. [Google Scholar] [CrossRef]
- Chen, M.Z.Q.; Wang, K.; Chen, G. Passive Network Synthesis: Advances with Inerter; World Scientific: Singapore, 2019. [Google Scholar]
- Hu, Y.; Chen, M.Z.Q.; Sun, Y. Comfort-oriented vehicle suspension design with skyhook inerter configuration. J. Sound Vib. 2017, 405, 34–47. [Google Scholar] [CrossRef]
- Wang, Y.; Meng, H.; Zhang, B.; Wang, R. Analytical research on the dynamic performance of semi-active inerter-based vibration isolator with acceleration–velocity-based control strategy. Struct. Control Health Monit. 2019, 26, e2336. [Google Scholar] [CrossRef]
- Wang, Y.; Ding, H.; Chen, L.Q. Averaging analysis on a semi-active inerter–based suspension system with relative-acceleration–relative-velocity control. J. Vib. Control 2020, 26, 1199–1215. [Google Scholar] [CrossRef]
- Chen, M.Z.Q.; Hu, Y. Inerter and Its Application in Vibration Control Systems; Springer: Beijing, China, 2019. [Google Scholar]
- Li, S.; Zou, W.; Chen, X.; Chen, C.; Xiang, Z. Adaptive Fully Distributed Consensus for a Class of Second-order Nonlinear Multi-agent Systems with Switching Networks. Int. J. Control Autom. Syst. 2023, 21, 2595–2604. [Google Scholar] [CrossRef]
- Zhang, J.; Xiang, Z. Event-Triggered Adaptive Neural Network Sensor Failure Compensation for Switched Interconnected Nonlinear Systems with Unknown Control Coefficients. IEEE Trans. Neural Netw. Learn. Syst. 2021, 33, 5241–5252. [Google Scholar] [CrossRef]
- Song, Y.; Yang, J.; Yang, T.; Fei, M. Almost Sure Stability of Switching Markov Jump Linear Systems. IEEE Trans. Autom. Control 2016, 61, 2638–2643. [Google Scholar] [CrossRef]
- Qu, H.; Hu, J.; Song, Y.; Yang, T. Mean square stabilization of discrete-time switching Markov jump linear systems. Optim. Control Appl. Methods 2019, 40, 141–151. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, L.; Liu, C.; Zhang, X. Two-parameter frequency tracker and its application of semi-active suspension with inerter. J. Vib. Eng. Technol. 2023, 11, 3593. [Google Scholar] [CrossRef]
- Ning, D.; Du, H.; Zhang, N.; Jia, Z.; Li, W.; Wang, Y. A semi-active variable equivalent stiffness and inertance device implemented by an electrical network. Mech. Syst. Signal Process. 2021, 156, 107676. [Google Scholar] [CrossRef]
- Corless, M.; Leitmann, G. Destabilization via active stiffness. Dyn. Control 1997, 7, 263–268. [Google Scholar] [CrossRef]
- Hu, Y.; Hua, T.; Chen, M.Z.Q.; Shi, S.; Sun, Y. Instability analysis for semi-active control systems with semi-active inerters. Nonlinear Dyn. 2021, 105, 99–112. [Google Scholar] [CrossRef]
- Xu, W.; Wang, K.; Chen, M.Z.Q. Stability analysis of inerter-based n-DOF vibration systems containing semi-active switched elements. Nonlinear Dyn. 2024; revision submitted. [Google Scholar]
- Ramaratnam, A.; Jalili, N. A switched stiffness approach for structural vibration control: Theory and real-time implementation. J. Sound Vib. 2006, 291, 258–274. [Google Scholar] [CrossRef]
- Yamamoto, K. Synthesis of passive interconnections in mass chains achieving a scale-free performance. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2021, 235, 1709–1717. [Google Scholar] [CrossRef]
- Li, H.; Chen, M.Z.Q. Achievable dynamic responses of general undamped mass-chain systems. Mech. Syst. Signal Process. 2022, 163, 108108. [Google Scholar] [CrossRef]
- Hu, Y.; Hua, T.; Chen, M.Z.Q.; Shi, S.; Sun, Y. Inherent stability analysis for multibody systems with semi-active inerters. J. Sound Vib. 2022, 535, 117073. [Google Scholar] [CrossRef]
- Chen, M.Z.Q.; Hu, Y.; Li, C.; Chen, G. Performance Benefits of Using Inerter in Semiactive Suspensions. IEEE Trans. Control Syst. Technol. 2015, 23, 1571–1577. [Google Scholar] [CrossRef]
- Bott, R.; Duffin, R. Impedance synthesis without use of transformers. J. Appl. Phys. 1949, 20, 816. [Google Scholar] [CrossRef]
- Hughes, T.H.; Smith, M.C. On the Minimality and Uniqueness of the Bott–Duffin Realization Procedure. IEEE Trans. Autom. Control 2014, 59, 1858–1873. [Google Scholar] [CrossRef]
- Liberzon, D. Switching in Systems and Control; Springer: Boston, USA, 2003. [Google Scholar]
- Khalil, H.K. Nonlinear Systems; Pearson Education Limited: Harlow, UK, 2013. [Google Scholar]
- Kwak, M.K. Dynamic Modeling and Active Vibration Control of Structures; Springer: Dordrecht, The Netherlands, 2022. [Google Scholar]
- Hu, Y.; Chen, M.Z.Q.; Shu, Z. Passive vehicle suspensions employing inerters with multiple performance requirements. J. Sound Vib. 2014, 333, 2212–2225. [Google Scholar] [CrossRef]
- Lazar, I.; Neild, S.; Wagg, D. Using an inerter-based device for structural vibration suppression. Earthq. Eng. Struct. Dyn. 2014, 43, 1129–1147. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Neild, S.; Jiang, J.Z. Optimal design of a pair of vibration suppression devices for a multi-storey building. Struct. Control Health Monit. 2020, 27, e2498. [Google Scholar] [CrossRef]
- Dai, K.; Lu, D.; Zhang, S.; Shi, Y.; Meng, J.; Huang, Z. Study on the damping ratios of reinforced concrete structures from seismic response records. Eng. Struct. 2020, 223, 111143. [Google Scholar] [CrossRef]
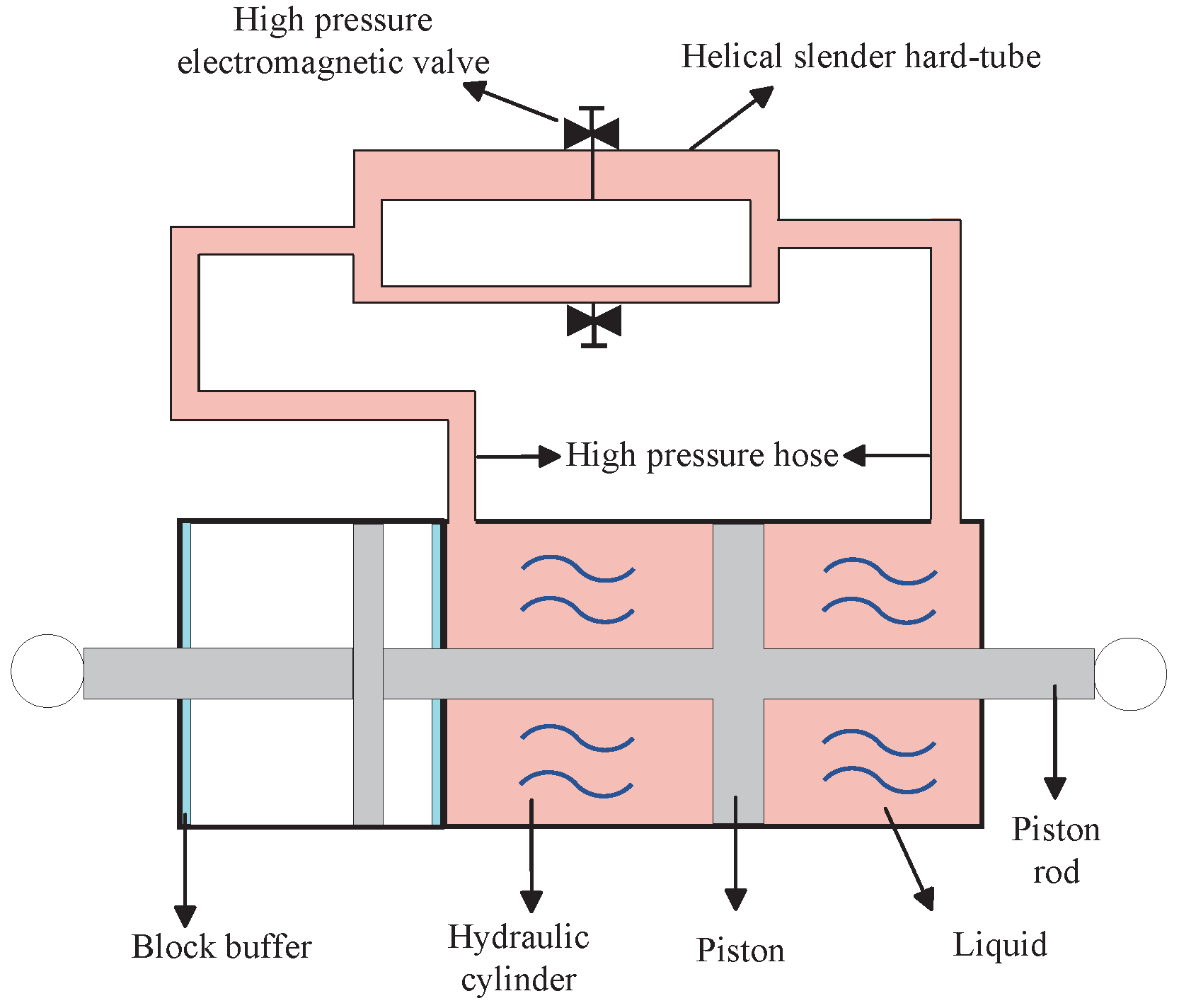





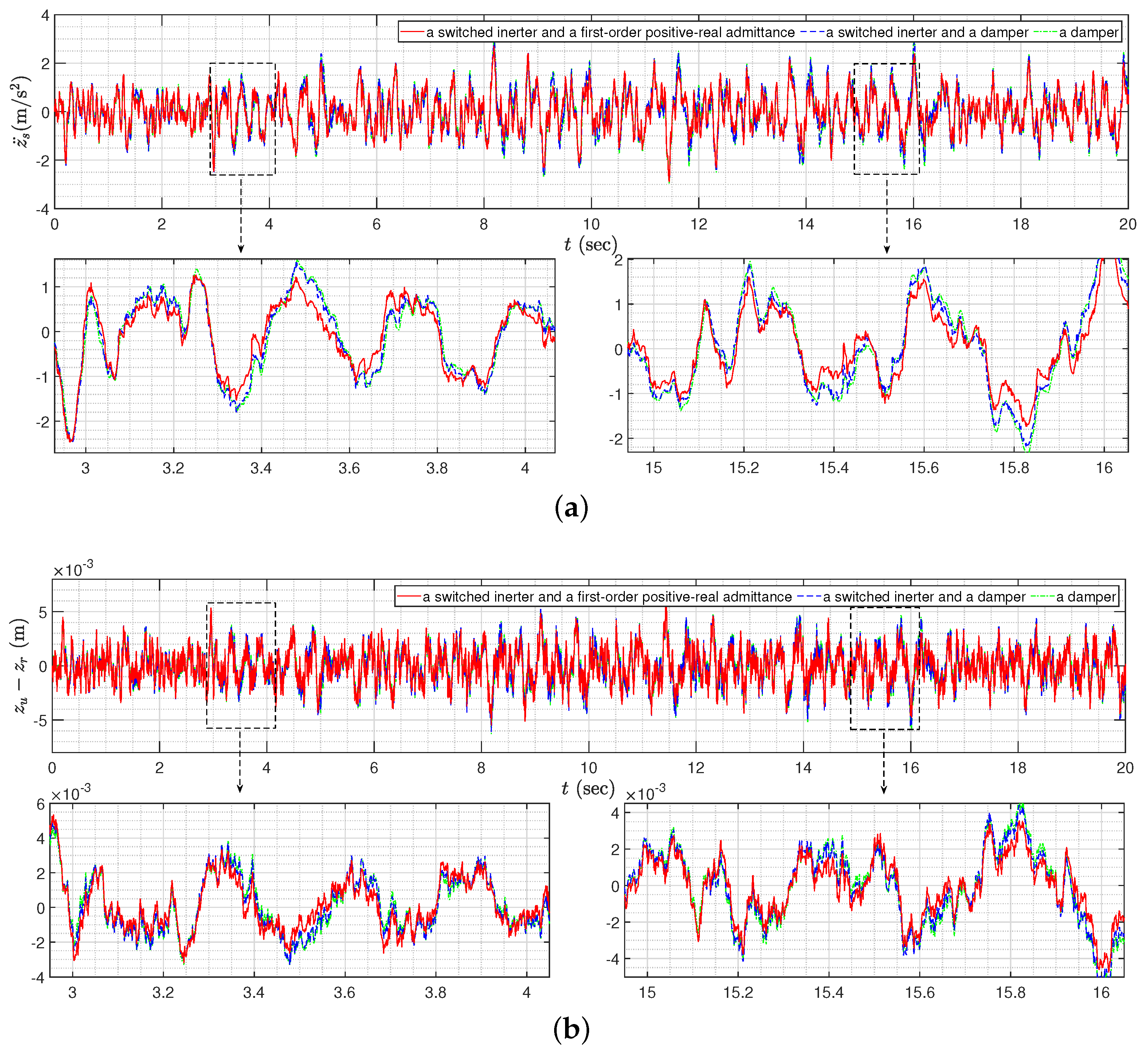

| Performance | A Switched Inerter and a First-Order Positive-Real Admittance (This Paper) | A Switched Inerter and a Damper | A Damper |
|---|---|---|---|
| J | |||
| RMS (m/) | |||
| RMS (mm) |
| Performance | A Switched Inerter and a First-Order Positive-Real Admittance (This Paper) | A Switched Inerter and a Damper | A Damper |
|---|---|---|---|
| J () | |||
| max (mm) | |||
| max (mm) | |||
| max (mm) | |||
| RMS (mm) | |||
| RMS (mm) | |||
| RMS (mm) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.; Xu, W. Stability Analysis and Design of n-DOF Vibration Systems Containing Both Semi-Active and Passive Mechanical Controllers. Sensors 2024, 24, 1600. https://doi.org/10.3390/s24051600
Wang K, Xu W. Stability Analysis and Design of n-DOF Vibration Systems Containing Both Semi-Active and Passive Mechanical Controllers. Sensors. 2024; 24(5):1600. https://doi.org/10.3390/s24051600
Chicago/Turabian StyleWang, Kai, and Wei Xu. 2024. "Stability Analysis and Design of n-DOF Vibration Systems Containing Both Semi-Active and Passive Mechanical Controllers" Sensors 24, no. 5: 1600. https://doi.org/10.3390/s24051600
APA StyleWang, K., & Xu, W. (2024). Stability Analysis and Design of n-DOF Vibration Systems Containing Both Semi-Active and Passive Mechanical Controllers. Sensors, 24(5), 1600. https://doi.org/10.3390/s24051600






