A Method to Design Compact MIMO Patch Antenna Using Self-Isolated Technique
Abstract
1. Introduction
2. Contribution of the Proposed Design
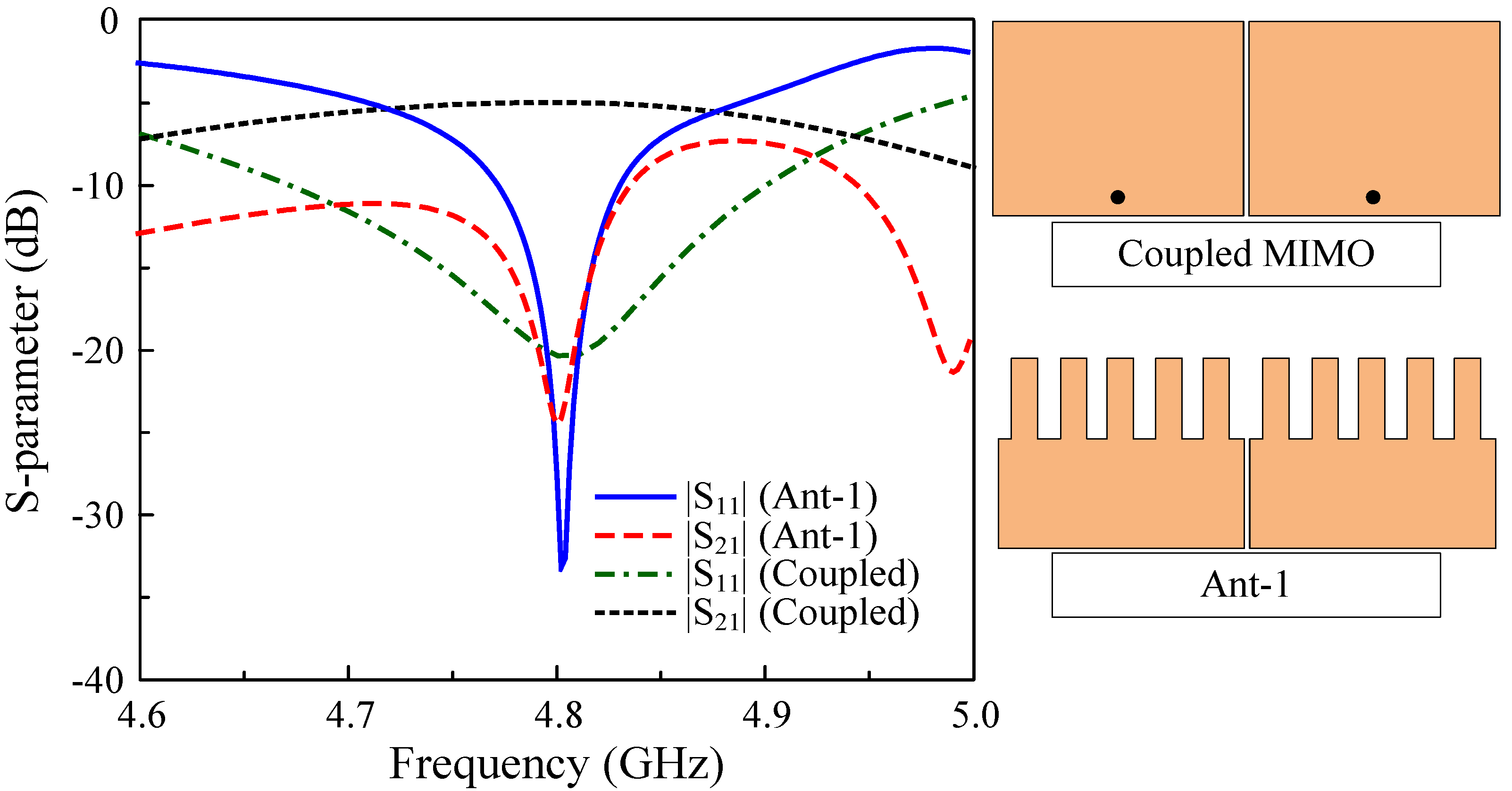
3. Compact MIMO Antenna
3.1. Patch Size Miniaturization
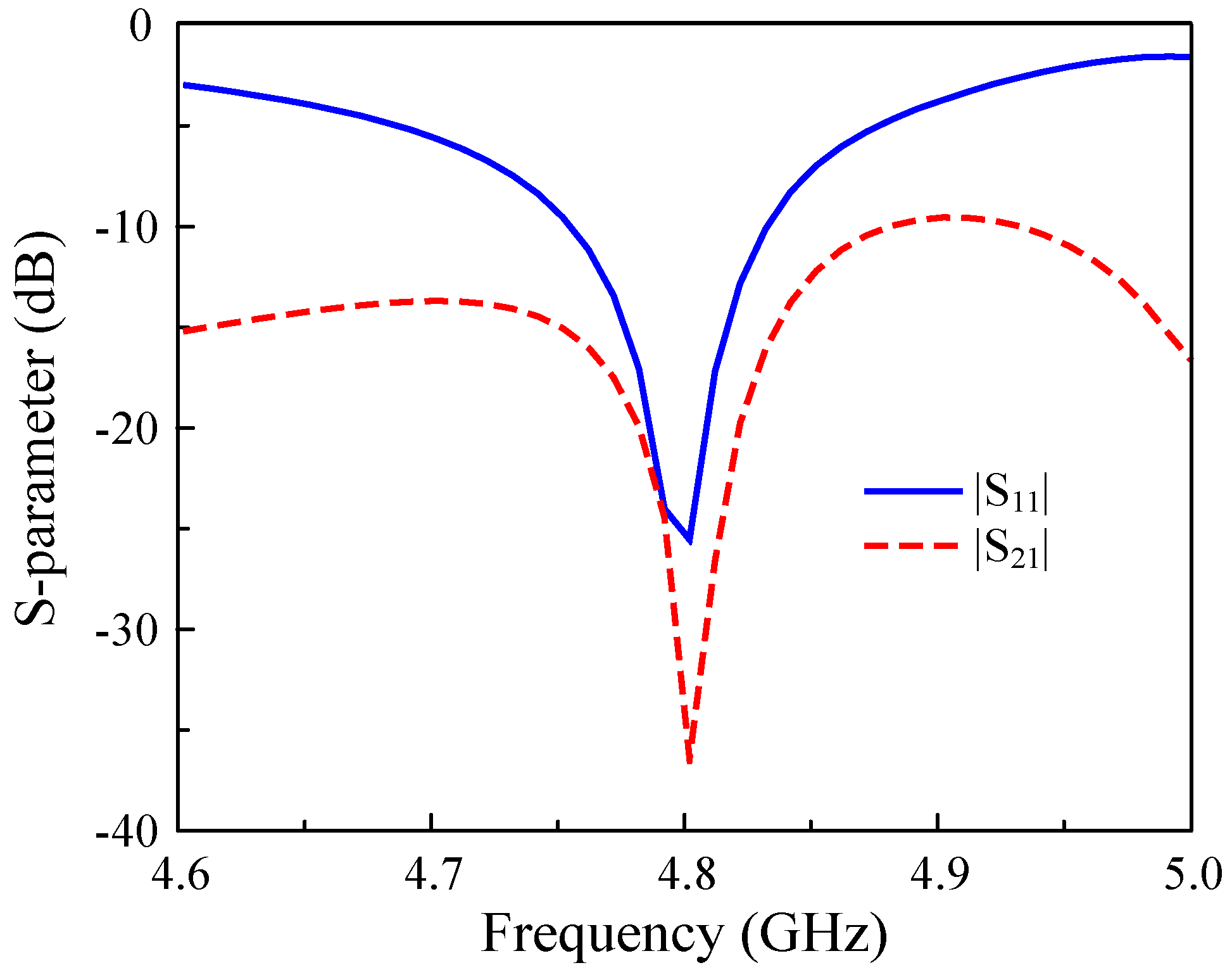
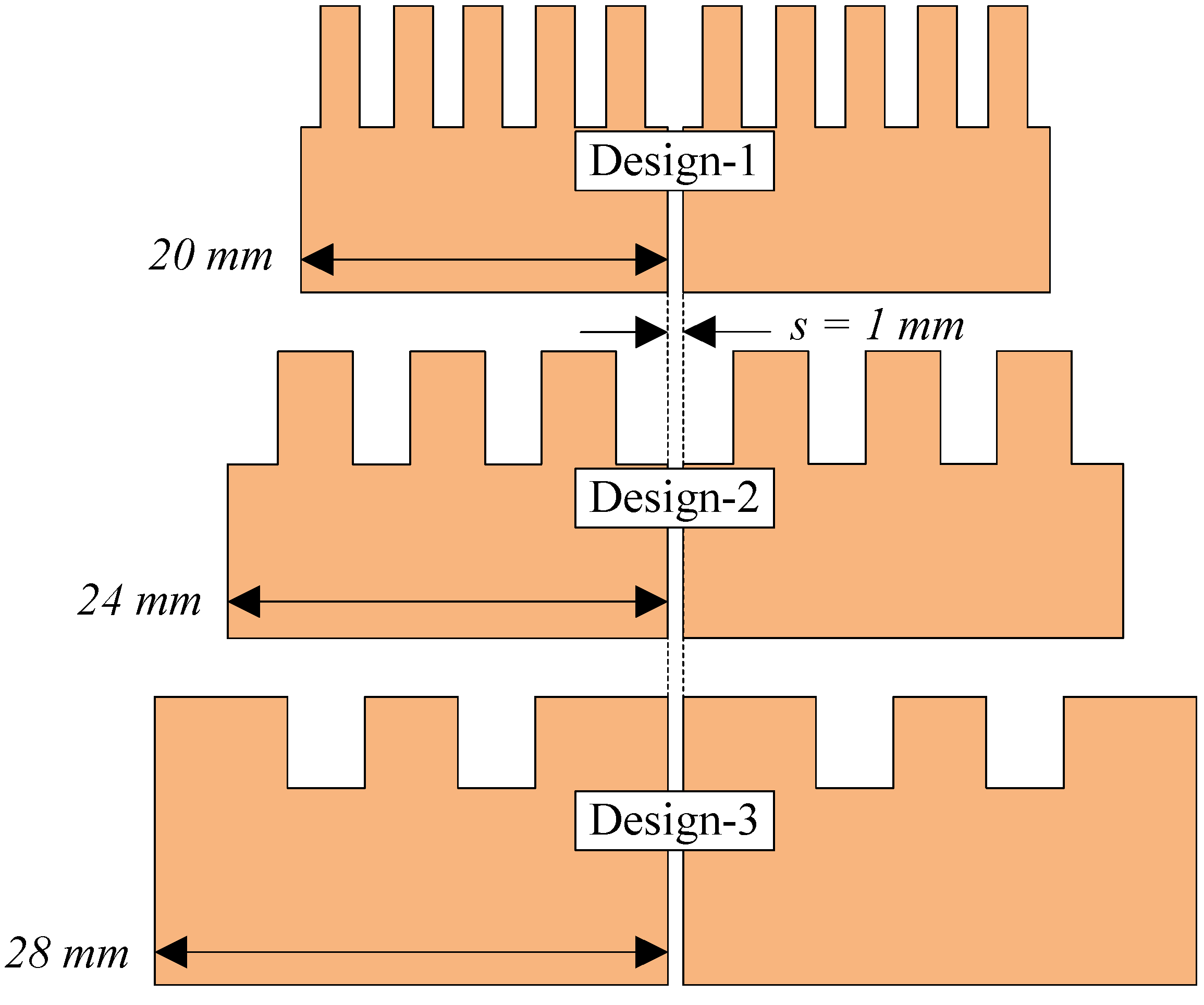
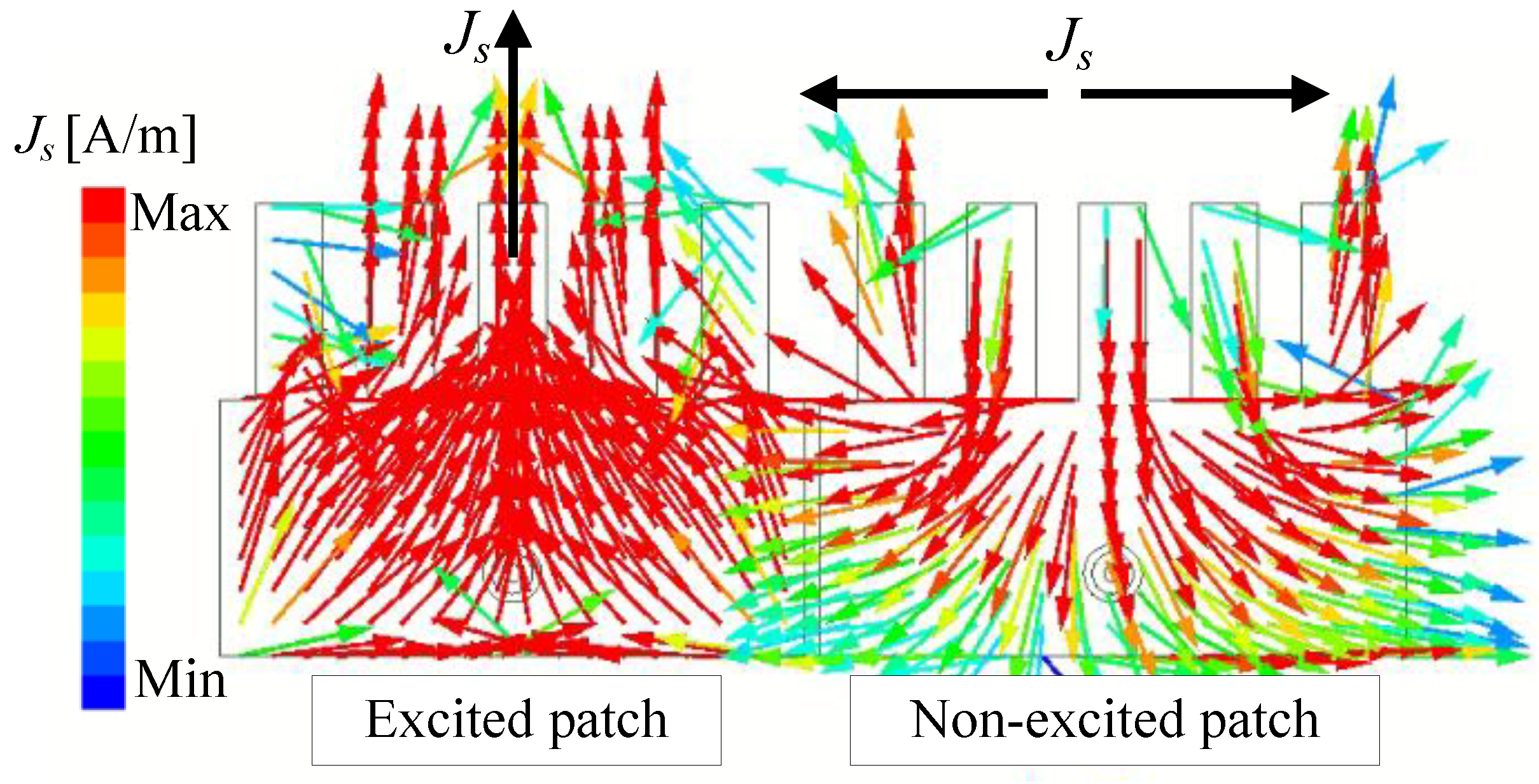
3.2. Element-Spacing Reduction
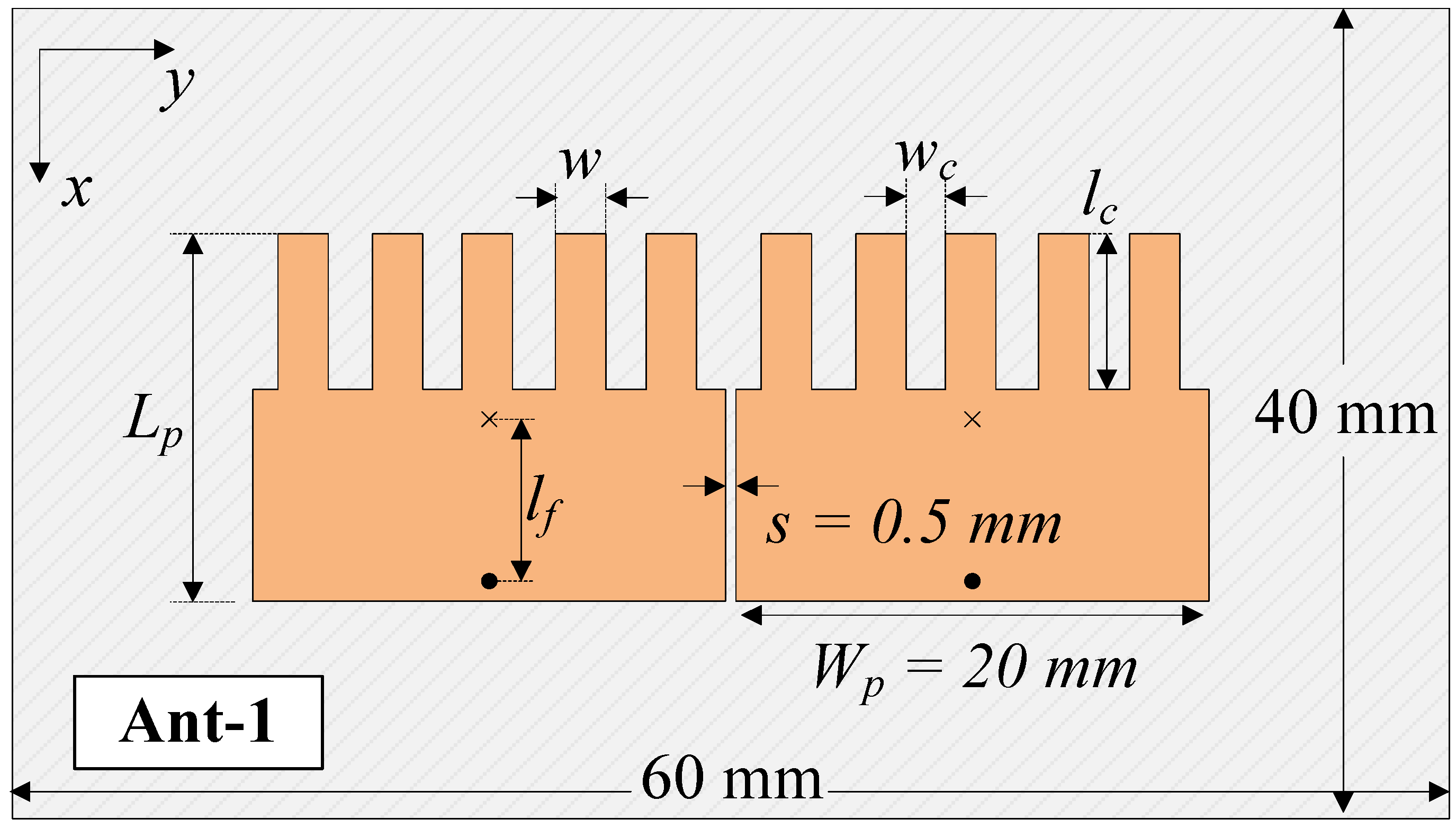
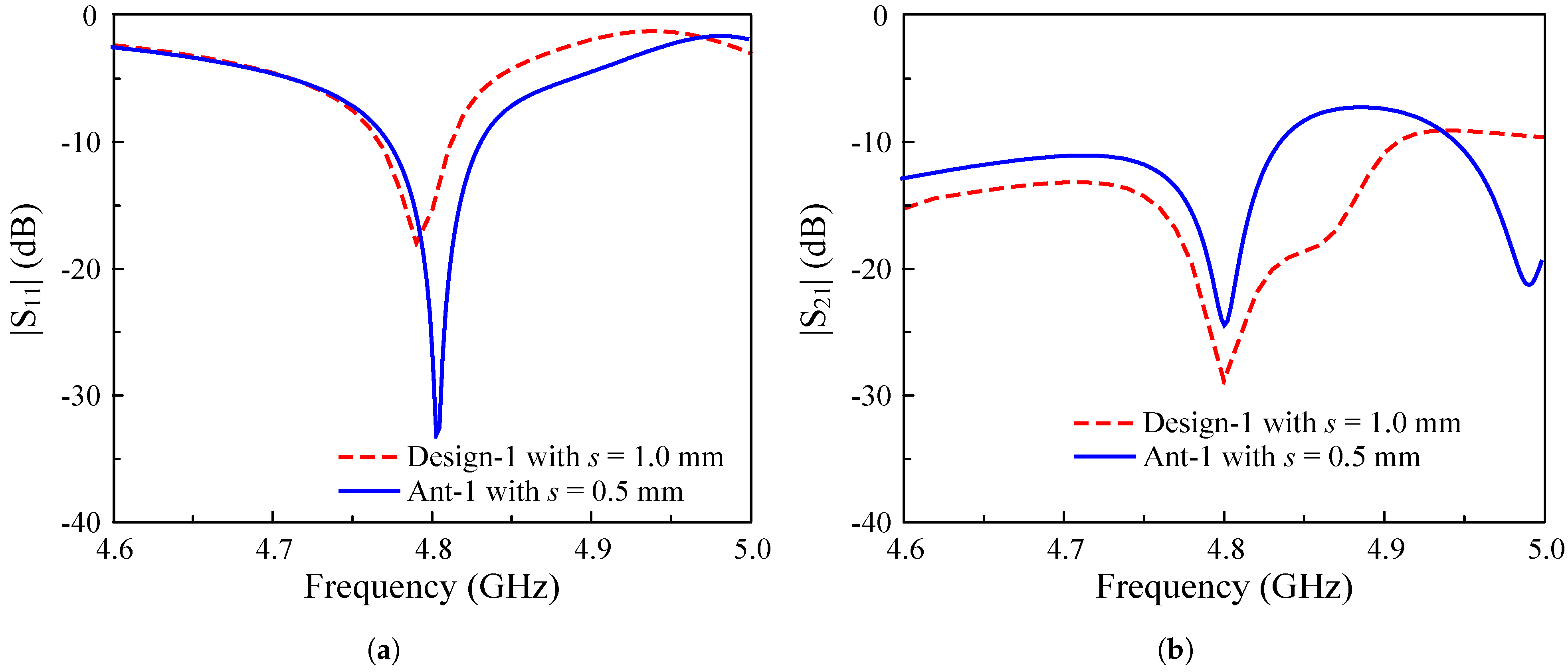
4. Compact and High-Gain MIMO Antenna
4.1. Design of High-Gain MIMO Element
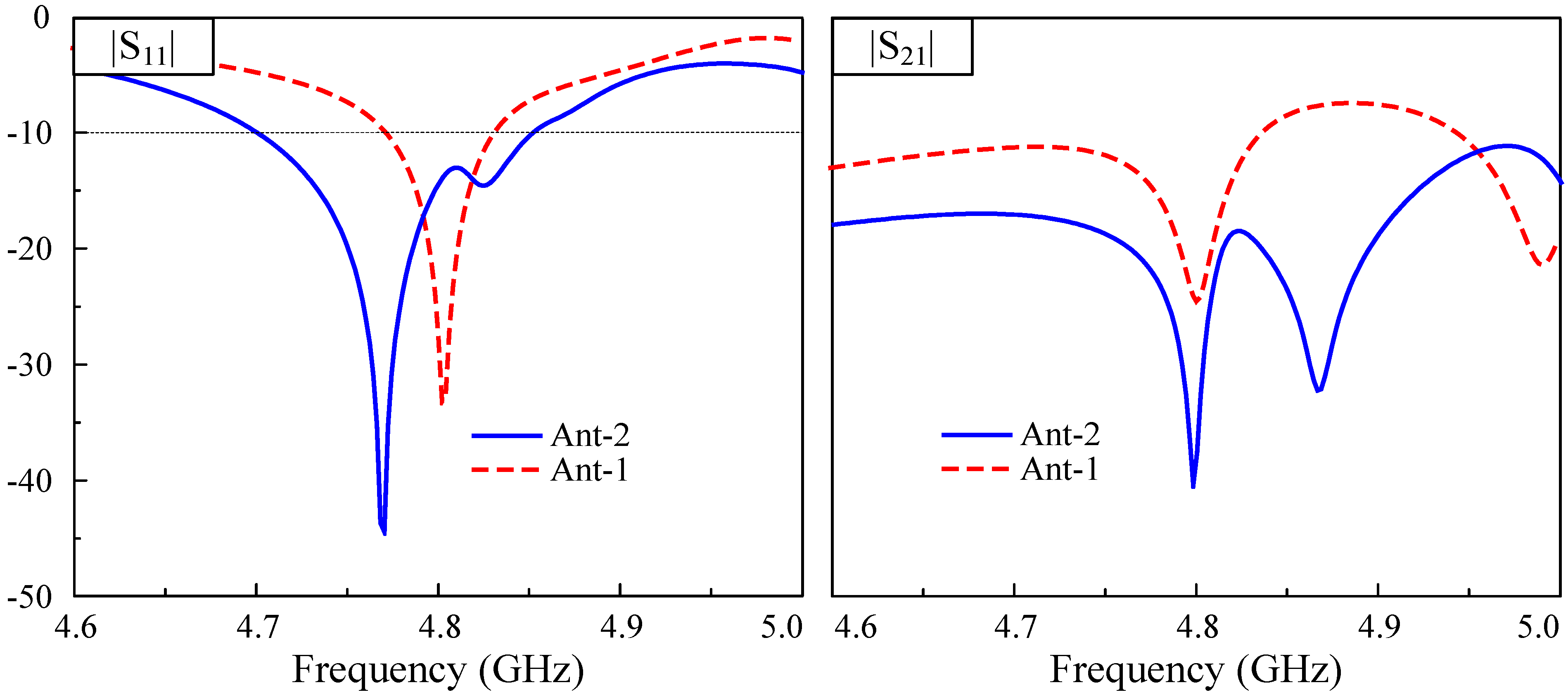
4.2. Design of Two-Port MIMO Antenna
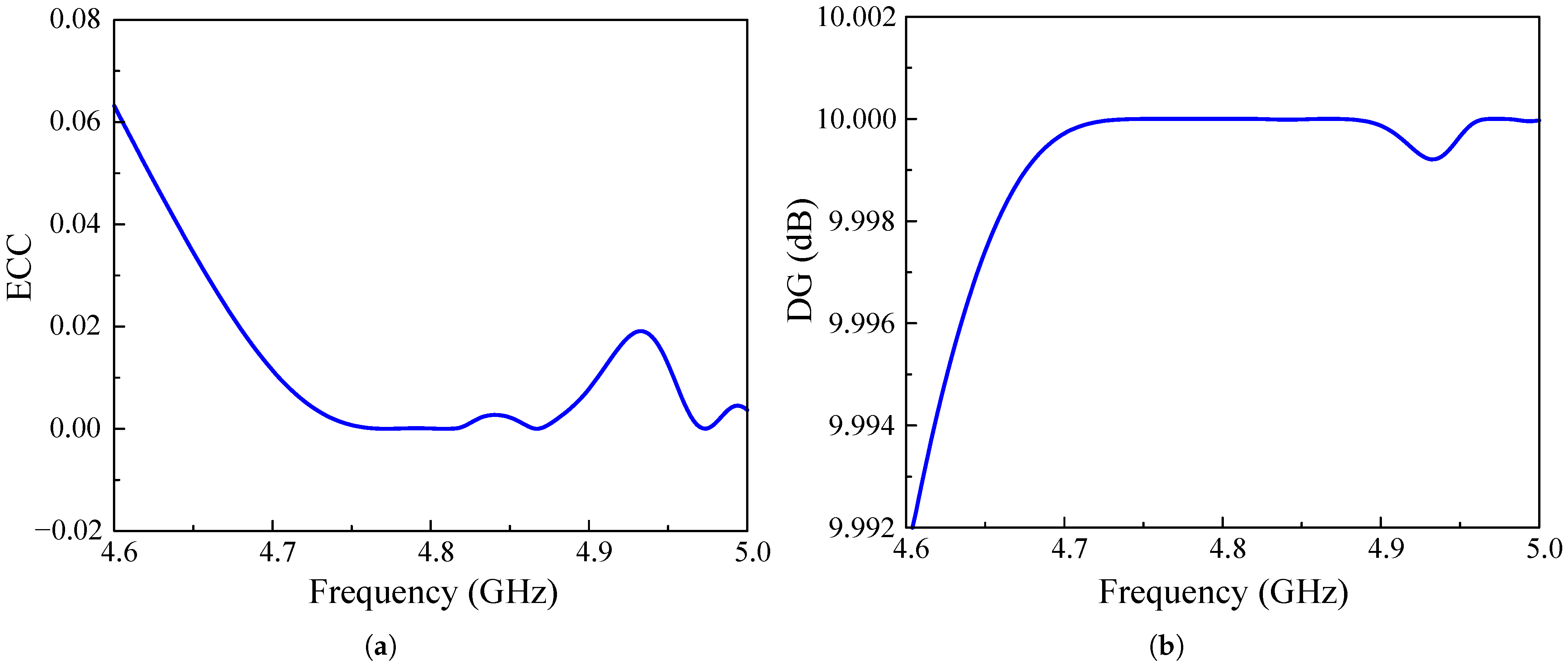
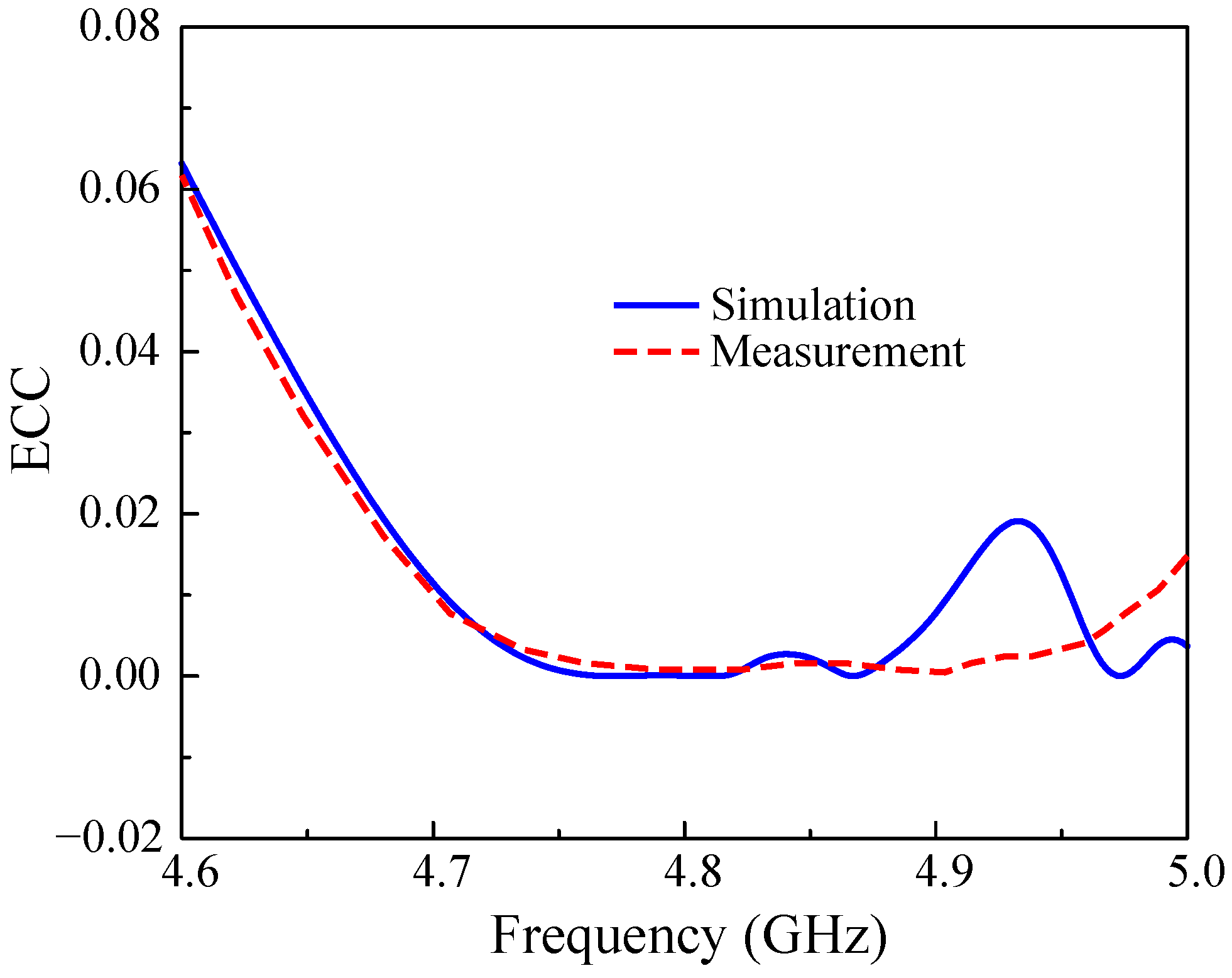
5. MIMO Diversity Performance
- where ;
- and
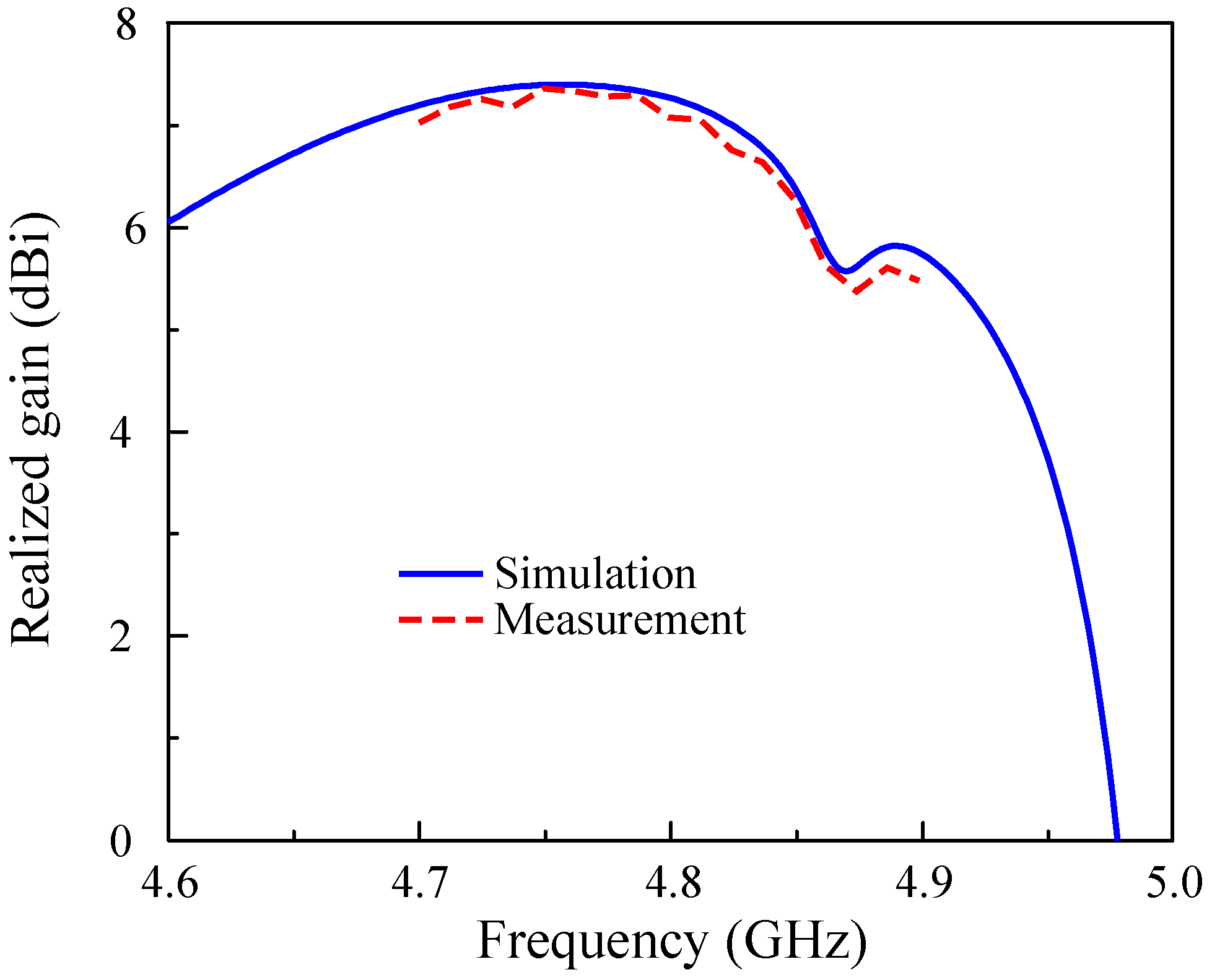
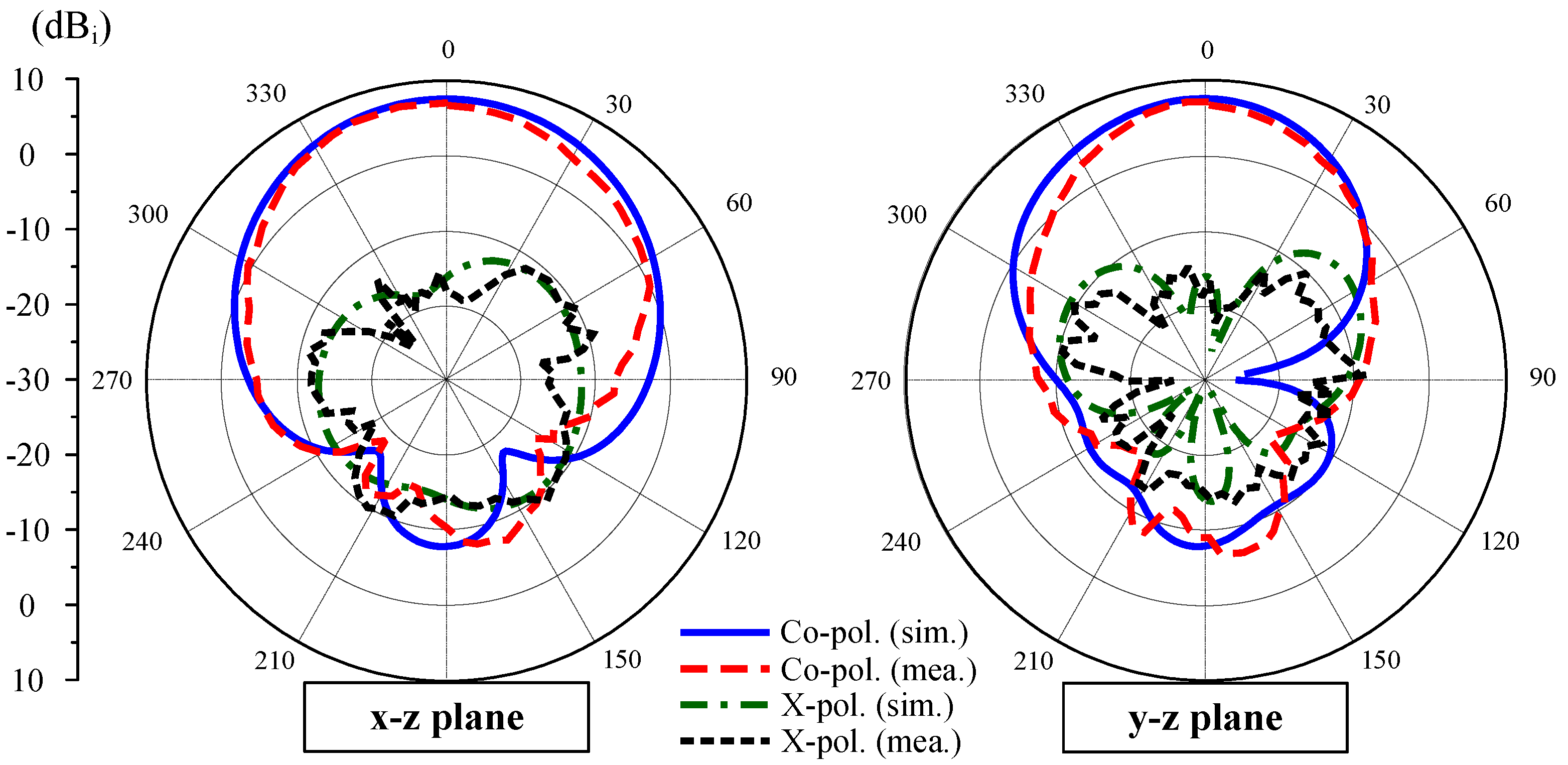
6. Measurement Results
7. Performance Comparison
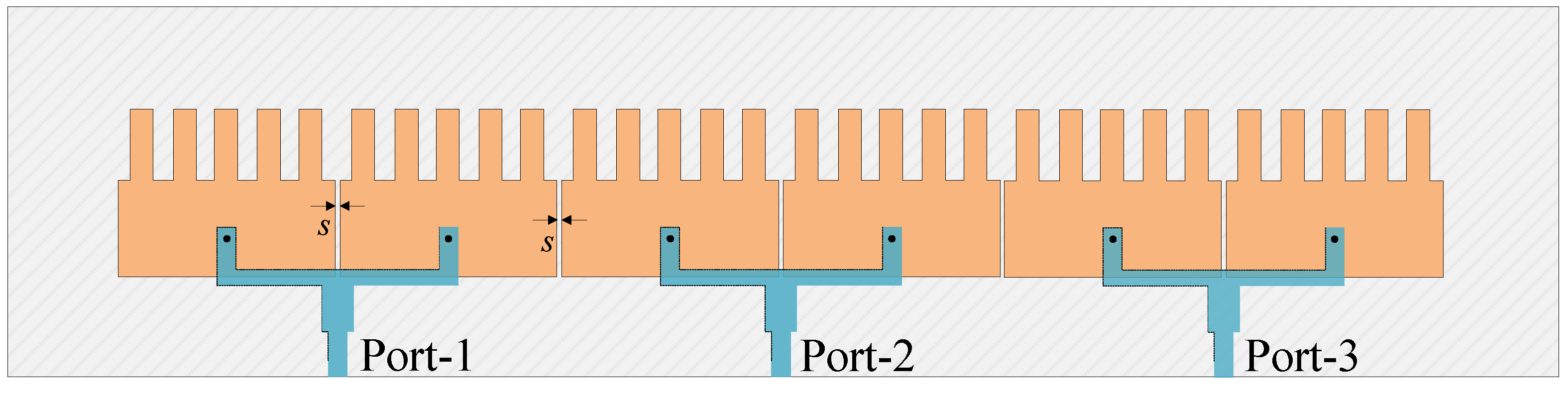
8. Multiple-Port MIMO Antenna
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jensen, M.A.; Wallace, J.W. A review of antennas and propagation for MIMO wireless communications. IEEE Trans. Antennas Propag. 2004, 52, 2810–2824. [Google Scholar] [CrossRef]
- Li, H.; Lau, B.K. MIMO systems and antennas for terminals. In Handbook of Antenna Technologies; Springer: Berlin/Heidelberg, Germany, 2016; pp. 2347–2388. [Google Scholar]
- Chen, X.; Zhang, S.; Li, Q. A Review of Mutual Coupling in MIMO Systems. IEEE Access 2018, 6, 24706–24719. [Google Scholar] [CrossRef]
- Balanis, C.A. Antenna Theory: Analysis and Design; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- Gao, D.; Cao, Z.X.; Fu, S.D.; Quan, X.; Chen, P. A Novel Slot-Array Defected Ground Structure for Decoupling Microstrip Antenna Array. IEEE Trans. Antennas Propag. 2020, 68, 7027–7038. [Google Scholar] [CrossRef]
- Xing, H.; Wang, X.; Gao, Z.; An, X.; Zheng, H.-X.; Wang, M.; Li, E. Efficient Isolation of an MIMO Antenna Using Defected Ground Structure. Electronics 2020, 9, 1265. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.; Xu, Y.X.; Gong, S.X. Isolation Enhancement in Patch Antenna Array With Fractal UC-EBG Structure and Cross Slot. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 2175–2178. [Google Scholar] [CrossRef]
- Fang, J.; Li, J.; Xiao, P.; Dong, J.; Li, G.; Du, S.; Joines, W.T. Coupling Mode Transformation-Based Dielectric Surface and Metasurface for Antenna Decoupler. IEEE Trans. Antennas Propag. 2023, 71, 1123–1128. [Google Scholar] [CrossRef]
- Rajo-Iglesias, E.; Quevedo-Teruel, O.; Inclan-Sanchez, L. Mutual Coupling Reduction in Patch Antenna Arrays by Using a Planar EBG Structure and a Multilayer Dielectric Substrate. IEEE Trans. Antennas Propag. 2008, 56, 1648–1655. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Zhang, S. A Novel Aperture-Loaded Decoupling Concept for Patch Antenna Arrays. IEEE Trans. Microw. Theory Tech. 2021, 69, 4272–4283. [Google Scholar] [CrossRef]
- Song, W.; Zhu, X.W.; Wang, L.; Hong, W. Simple Structure E-Plane Decoupled Millimeter Wave Antenna Based on Current Cancellation Model. IEEE Trans. Antennas Propag. 2022, 70, 9871–9876. [Google Scholar] [CrossRef]
- Pei, T.; Zhu, L.; Wang, J.; Wu, W. A Low-Profile Decoupling Structure for Mutual Coupling Suppression in MIMO Patch Antenna. IEEE Trans. Antennas Propag. 2021, 69, 6145–6153. [Google Scholar] [CrossRef]
- Qi, H.; Liu, L.; Yin, X.; Zhao, H.; Kulesza, W.J. Mutual Coupling Suppression Between Two Closely Spaced Microstrip Antennas With an Asymmetrical Coplanar Strip Wall. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 191–194. [Google Scholar] [CrossRef]
- Tang, M.-C.; Chen, Z.; Wang, H.; Li, M.; Luo, B.; Wang, J.; Shi, Z.; Ziolkowski, R.W. Mutual Coupling Reduction Using Meta-Structures for Wideband, Dual-Polarized, and High-Density Patch Arrays. IEEE Trans. Antennas Propag. 2017, 65, 3986–3998. [Google Scholar] [CrossRef]
- Li, M.; Zhong, B.G.; Cheung, S.W. Isolation Enhancement for MIMO Patch Antennas Using Near-Field Resonators as Coupling-Mode Transducers. IEEE Trans. Antennas Propag. 2019, 67, 755–764. [Google Scholar] [CrossRef]
- Cheng, Y.F.; Ding, X.; Shao, W.; Wang, B.Z. Reduction of Mutual Coupling Between Patch Antennas Using a Polarization-Conversion Isolator. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 1257–1260. [Google Scholar] [CrossRef]
- Niu, Z.; Zhang, H.; Chen, Q.; Zhong, T. Isolation Enhancement in Closely Coupled Dual-Band MIMO Patch Antennas. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1686–1690. [Google Scholar] [CrossRef]
- Qi, H.; Yin, X.; Liu, L.; Rong, Y.; Qian, H. Improving Isolation Between Closely Spaced Patch Antennas Using Interdigital Lines. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 286–289. [Google Scholar] [CrossRef]
- Das, P.; Mandal, K. Polarization Converter Surface Integrated MIMO Antenna for Simultaneous Reduction of RCS and Mutual Coupling. IEEE Antennas Wirel. Propag. Lett. 2022, 21, 1782–1786. [Google Scholar] [CrossRef]
- Lai, Q.X.; Pan, Y.M.; Zheng, S.Y. A Self-Decoupling Method for MIMO Antenna Array Using Characteristic Mode of Ground Plane. IEEE Trans. Antennas Propag. 2023, 71, 2126–2135. [Google Scholar] [CrossRef]
- Sun, L.; Li, Y.; Zhang, Z.; Wang, H. Antenna Decoupling by Common and Differential Modes Cancellation. IEEE Trans. Antennas Propag. 2021, 69, 672–682. [Google Scholar] [CrossRef]
- Lin, H.; Chen, Q.; Ji, Y.; Yang, X.; Wang, J.; Ge, L. Weak-Field-Based Self-Decoupling Patch Antennas. IEEE Trans. Antennas Propag. 2020, 68, 4208–4217. [Google Scholar] [CrossRef]
- Hong, K.D.; Zhang, X.; Weng, H.Y.; Zhu, L.; Yuan, T. A 2-D Self-Decoupling Method Based on Antenna-Field Redistribution for MIMO Patch Antenna Array. IEEE Antennas Wirel. Propag. Lett. 2024, 23, 940–944. [Google Scholar] [CrossRef]
- Lai, Q.X.; Pan, Y.M.; Zheng, S.Y.; Yang, W.J. Mutual Coupling Reduction in MIMO Microstrip Patch Array Using TM10 and TM02 Modes. IEEE Trans. Antennas Propag. 2021, 69, 7562–7571. [Google Scholar] [CrossRef]
- Zha, L.; Pan, Y.M.; Zheng, S.Y. Self-Decoupled Linear and Planar MIMO Microstrip Patch Antenna Arrays Operating in the Fundamental TM01 Mode. IEEE Trans. Antennas Propag. 2024, 72, 1224–1233. [Google Scholar] [CrossRef]
- Kim-Thi, P.; Van, T.N.; Thanh, T.B. A Self-Decoupling Technique for Isolation Enhancement in Closely-Spaced MIMO Patch Antennas. IEEE Antennas Wirel. Propag. Lett. 2024, 23, 1695–1699. [Google Scholar] [CrossRef]
- Francis, F.; Rosaline, S.I.; Kumar, R.S. A broadband metamaterial superstrate based MIMO antenna array for sub-6 GHz wireless applications. AEU-Int. J. Electron. Commun. 2024, 173, 155015. [Google Scholar]
- Tariq, S.; Naqvi, S.I.; Hussain, N.; Amin, Y. A Metasurface-Based MIMO Antenna for 5G Millimeter-Wave Applications. IEEE Access 2021, 9, 51805–51817. [Google Scholar] [CrossRef]
- Salehi, M.; Oraizi, H. Wideband high gain metasurface-based 4T4R MIMO antenna with highly isolated ports for sub-6 GHz 5G applications. Sci. Rep. 2024, 14, 14448. [Google Scholar] [CrossRef]
- Ojo, R.; Jamlos, M.F.; Soh, P.J.; Jamlos, M.A.; Bahari, N.; Lee, Y.S.; Al-Bawri, S.S.; Abdul Karim, M.S.; Khairi, K.A. A triangular MIMO array antenna with a double negative metamaterial superstrate to enhance bandwidth and gain. Int. J. Microw. Comput.-Aided Eng. 2020, 30, e22320. [Google Scholar] [CrossRef]
- Illahi, M.U.; Khan, M.U.; Hussain, R.; Tahir, F.A. A highly compact Fabry Perot cavity-based MIMO antenna with decorrelated fields. Sci. Rep. 2022, 12, 14021. [Google Scholar] [CrossRef]
- Hassan, T.; Khan, M.U.; Attia, H.; Sharawi, M.S. An FSS Based Correlation Reduction Technique for MIMO Antennas. IEEE Trans. Antennas Propag. 2018, 66, 4900–4905. [Google Scholar] [CrossRef]
- Khalid, M.; Naqvi, S.I.; Hussain, N.; Rahman, M.; Mirjavadi, S.S.; Khan, M.J.; Amin, Y. 4-Port MIMO antenna with defected ground structure for 5G millimeter wave applications. Electronics 2020, 9, 71. [Google Scholar] [CrossRef]
- Bilal, M.; Naqvi, S.I.; Hussain, N.; Amin, Y.; Kim, N. High-isolation MIMO antenna for 5G millimeter-wave communication systems. Electronics 2022, 11, 962. [Google Scholar] [CrossRef]
- Pant, M.; Malviya, L. High Gain and Low ECC MIMO Antenna Array for Millimeter Wave Communication Systems. Res. Sq. 2024; Preprint. [Google Scholar] [CrossRef]
- Haque, M.A.; Ahammed, M.S.; Ananta, R.A.; Aljaloud, K.; Jizat, N.M.; Abdulkawi, W.M.; Nahin, K.H.; Al-Bawri, S.S. Broadband high gain performance MIMO antenna array for 5G mm-wave applications-based gain prediction using machine learning approach. Alex. Eng. J. 2024, 104, 665–679. [Google Scholar] [CrossRef]









| Ref. | Overall Size () | Decoupling Network | No. of Elements for 1 Port | Center Spacing () | Edge Spacing () | Gain (dBi) | Isolation (dB) |
|---|---|---|---|---|---|---|---|
| [27] | 1.40 × 2.33 × 0.10 | No | 4 | 1.11 | 0.42 | 11.5 | 30 |
| [28] | 2.60 × 3.73 × 0.64 | Yes | 2 | 1.3 | 0.53 | 10.3 | 35 |
| [30] | 1.06 × 1.12 × 0.16 | No | 4 | 1.19 | 0.32 | 14.1 | 40 |
| [32] | 1.84 × 1.84 × 0.27 | No | 1 | 0.53 | 0.13 | 8.8 | 10 |
| [34] | 2.80 × 3.27 × 0.07 | Yes | 2 | 1.41 | 0.39 | 12 | 35 |
| [36] | 2.24 × 2.24 × 0.07 | No | 2 | 1.22 | 0.49 | 10.3 | 26 |
| Prop. | 0.64 × 1.60 × 0.02 | No | 2 | 0.33 | 0.01 | 7.1 | 18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Truong-Quang, N.; Dao-Duc, T.; Kim-Thi, P.; Le-Tuan, T.; Tran, H.; Nguyen-Tien, D.; Hussain, N. A Method to Design Compact MIMO Patch Antenna Using Self-Isolated Technique. Sensors 2025, 25, 2073. https://doi.org/10.3390/s25072073
Truong-Quang N, Dao-Duc T, Kim-Thi P, Le-Tuan T, Tran H, Nguyen-Tien D, Hussain N. A Method to Design Compact MIMO Patch Antenna Using Self-Isolated Technique. Sensors. 2025; 25(7):2073. https://doi.org/10.3390/s25072073
Chicago/Turabian StyleTruong-Quang, Noi, Tan Dao-Duc, Phuong Kim-Thi, Tu Le-Tuan, Hung Tran, Dat Nguyen-Tien, and Niamat Hussain. 2025. "A Method to Design Compact MIMO Patch Antenna Using Self-Isolated Technique" Sensors 25, no. 7: 2073. https://doi.org/10.3390/s25072073
APA StyleTruong-Quang, N., Dao-Duc, T., Kim-Thi, P., Le-Tuan, T., Tran, H., Nguyen-Tien, D., & Hussain, N. (2025). A Method to Design Compact MIMO Patch Antenna Using Self-Isolated Technique. Sensors, 25(7), 2073. https://doi.org/10.3390/s25072073






