The Management of Dental Practices in the Post-COVID 19 Era: An Economic and Operational Perspective
Abstract
:1. Introduction
2. Materials and Methods
- an increase in the time period between subsequent treatments (time Δt), reducing the time available in a given time period (generally one year) for the treatment, and
- an increase in fixed costs (e.g., the adapting of air-conditioning plants) and variable costs (e.g., personal protective equipment (PPE).
2.1. Methodology
- A.
- Leave the dental charges of single treatments unchanged with a resulting loss in markup;
- B.
- Increase the charge of single treatments in order to maintain unvaried markup; and
- C.
- Modify the dental treatment mix available (product mix) so as to contain the loss in economic performance.
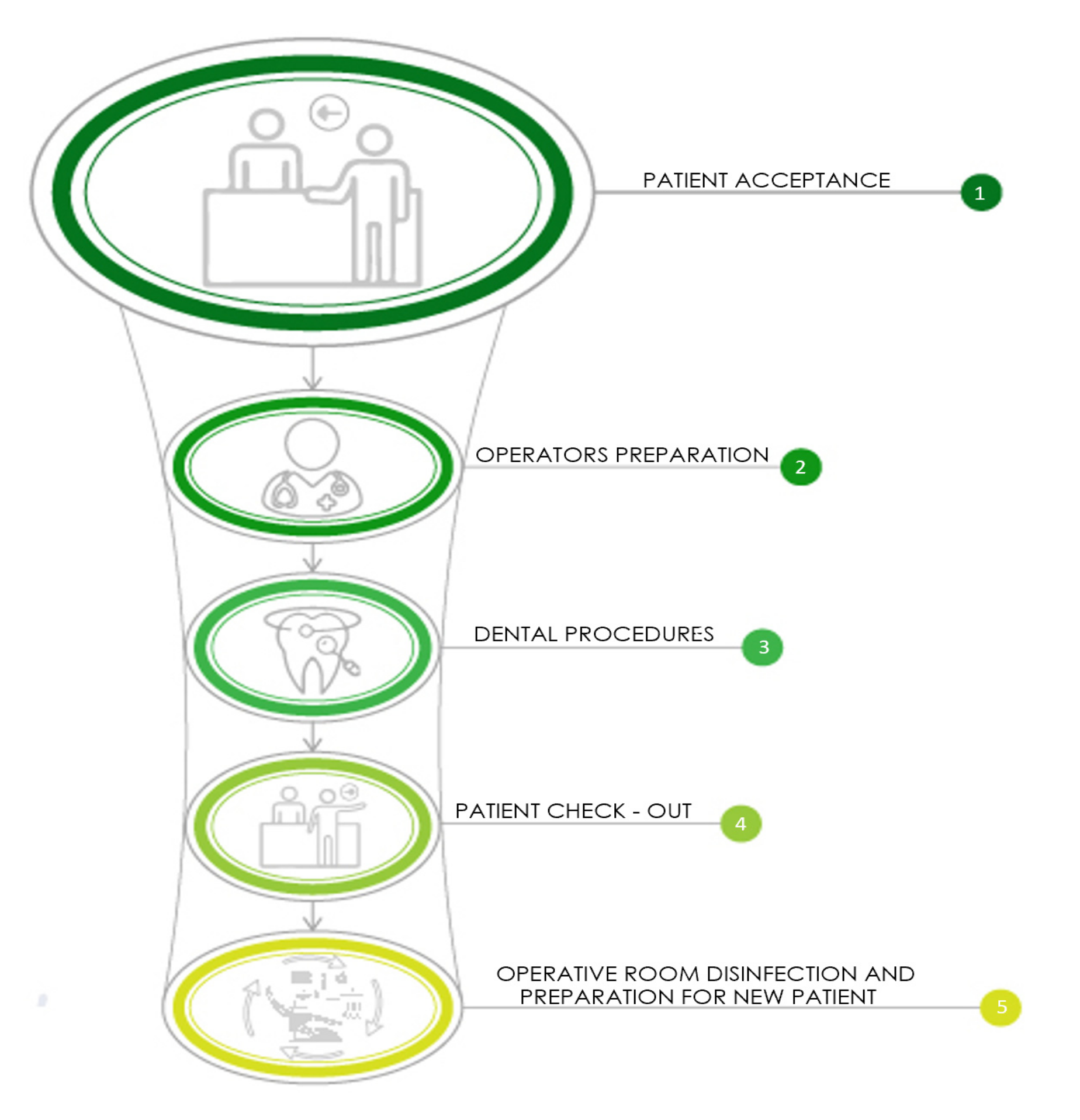
2.2. Operating Phases
3. Results
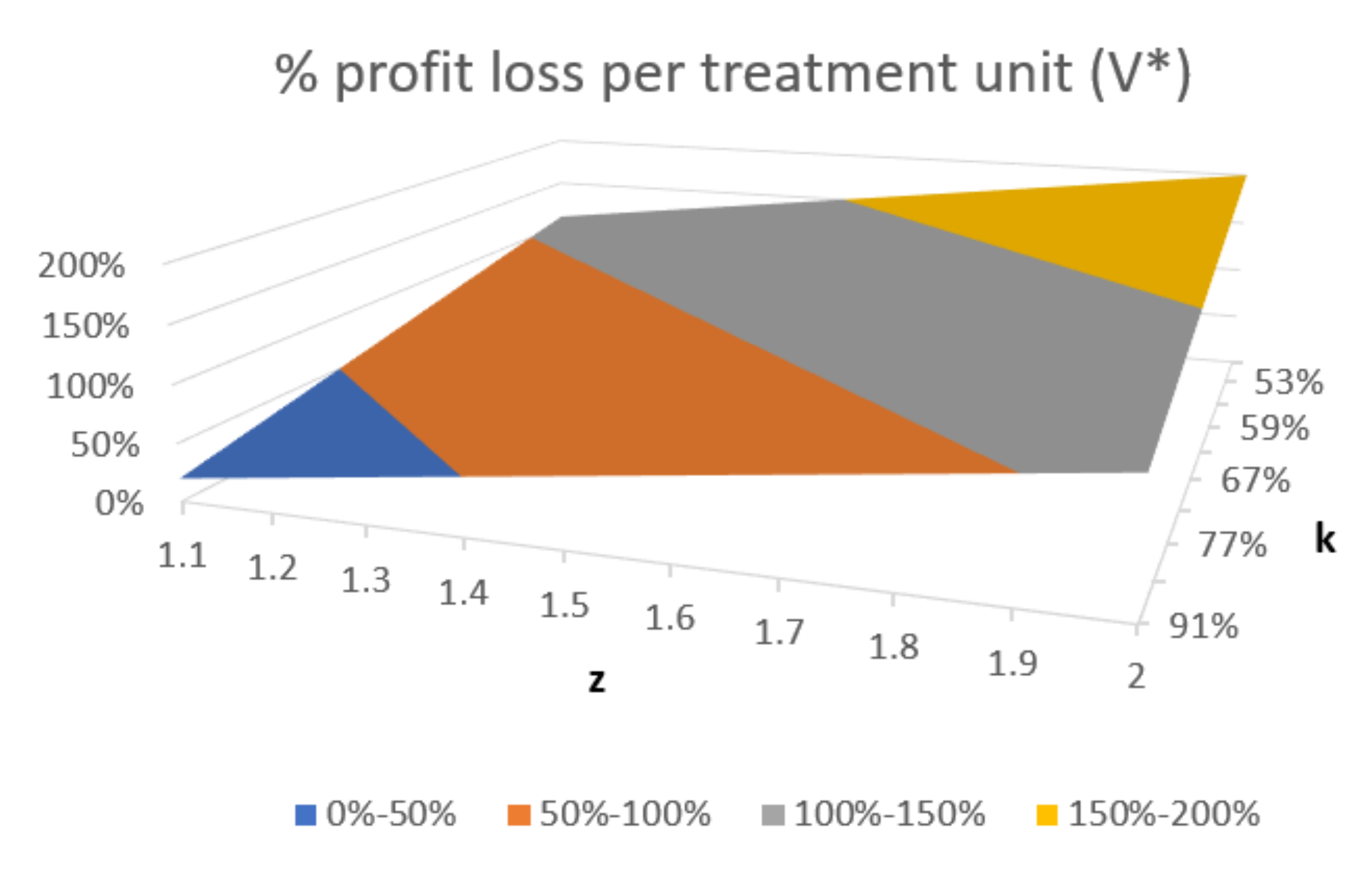
3.1. Approach A (Dental Charges and Product Mix Unchanged)
3.2. Approach B (Markup and Product Mix Unchanged)
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Operating Phases
Appendix A.2. An Increase in Time
Appendix A.3. Markup
Appendix A.4. Treatment Pricing
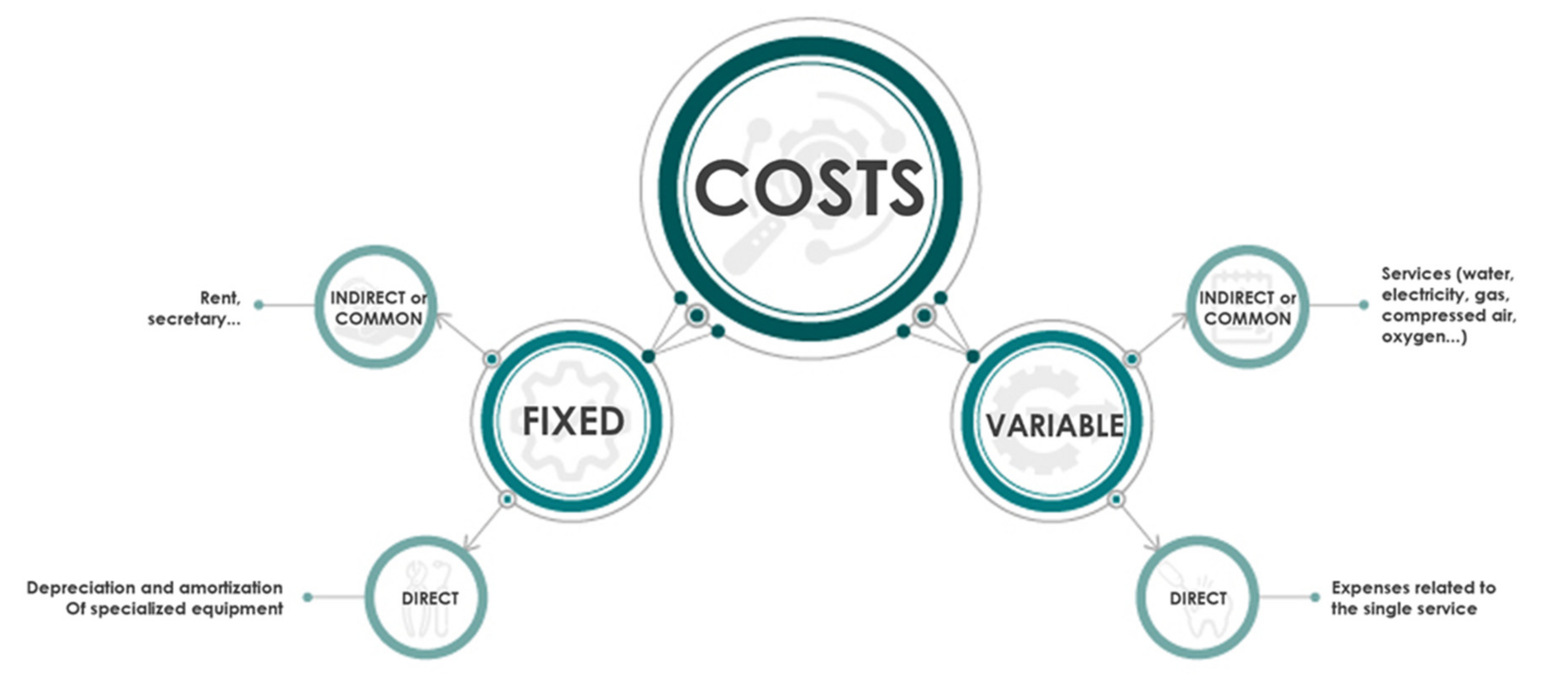
- Fixed common costs will increase following preventative recommendations (regarding, for example, the air-conditioning plant); and
- The number of treatments will diminish due to an increase in the working time, thereby changing the driver disproportionally. This implies that some treatments will absorb greater and lesser costs in percentage terms.
| Variables Used in the Analysis | Description |
|---|---|
| CF | pre-COVID fixed cost in period T |
| ΔCF | increase in fixed costs |
| CF* = CF + ΔCF | post-COVID fixed cost in period T |
| R | number of treatments |
| cfr | pre-COVID unit fixed cost of r-th treatment |
| cvr | pre-COVID variable unit cost of r-th treatment |
| cr = cfr+ cvr | pre-COVID unit cost of r-th treatment |
| Δcfr | increase in fixed unit costs of r-th treatment |
| cfr * = cfr+ Δcfr | post-COVID fixed unit cost of r-th treatment |
| Δcvr | increase in variable unit costs of r-th treatment |
| cvr * = cvr + Δcvr | post-COVID variable unit costs of r-th treatment |
| cr* = cfr*+ cVr* | post-COVID unit cost of r-th treatment |
| pr | pre-COVID dental charge of r-th treatment |
| pr* | post-COVID dental charge of r-th treatment |
| Vr | number of pre-COVID r-th treatment |
| Vr* | number of post-COVID r-th treatment |
| total volume of pre-COVID treatments | |
| total volume of post-COVID treatments | |
| total pre-COVID markup from the sum of markup for each treatment | |
| total post-COVID markup from the sum of markup for each treatment | |
| T | Timeframe for analysis: 1 year |
| percentage volume of r-th treatment on total volume | |
| percentage volume of r-th treatment on total post-COVID volume | |
| z | increase in post-COVID unit costs |
| K | reduction in post-COVID volumes |
| tb | preparation time for staff and treatment slot |
| ts | time necessary to render the OR operational between successive treatments |
| tw | total treatment duration |
| tc | cycle time or time interval between completing two successive treatments |
| Δt | increase in time ts, resulting from anti-COVID recommendations |
Appendix B
- On the one hand, the working time (which impacts on the volumes achievable, as previously demonstrated; refer to the Increase in time sub-section above), and the fixed unit cost (to be elaborated below)
- And, on the other, the risk level of the treatment (which impacts on the variable costs).
| j = l | j = m | j = h | |
|---|---|---|---|
| i = tw1 | mixtw1l | mixtw1m | mixtw1h |
| i = tw2 | mixtw2l | mixtw2m | mixtw2h |
| i = tw3 | mixtw3l | mixtw3m | mixtw3h |
| mixij | j = l | j = m | j = h |
|---|---|---|---|
| i = tw1 | 8% | 15% | 12% |
| i = tw2 | 10% | 11% | 11% |
| i = tw3 | 9% | 12% | 12% |
| subscript r | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
| subscript ij | tw1l | tw1m | tw1h | tw2l | tw2m | tw2h | tw3l | tw3m | tw3h |
| V*ij | j = l | j = m | j = h |
|---|---|---|---|
| i = tw1 | 335 | 213 | 156 |
| i = tw2 | 335 | 213 | 156 |
| i = tw3 | 335 | 213 | 156 |
| V*ij | j = l | j = m | j = h |
|---|---|---|---|
| i = tw1 | 181 | 138 | 112 |
| i = tw2 | 181 | 138 | 112 |
| i = tw3 | 181 | 138 | 112 |
| i = tw1 | i = tw2 | i = tw1 | |
|---|---|---|---|
| cvij (monetary units) | 54 | 85 | 116 |
| cij = cfij + cvij (monetary units) | 83 | 131 | 179 |
| i = tw1 | i = tw2 | i = tw3 | |
|---|---|---|---|
| pij (monetary units) | 125 | 196 | 268 |
| cij* | j = l | j = m | j = h |
|---|---|---|---|
| i = tw1 | 121 | 172 | 223 |
| i = tw2 | 131 | 182 | 233 |
| i = tw3 | 141 | 192 | 243 |
| pij* | j = l | j = m | j = h |
|---|---|---|---|
| i = tw1 | 181 | 258 | 335 |
| i = tw2 | 196 | 273 | 350 |
| i = tw2 | 211 | 288 | 365 |
| pi* average | 196 | 273 | 350 |
| Δpi* average % | 57% | 39% | 31% |
| MIX1 | MIX2 | ||||||
|---|---|---|---|---|---|---|---|
| mixil | 144% | 117% | 105% | mixil | 175% | 117% | 90% |
| mixim | 168% | 133% | 116% | mixim | 199% | 133% | 101% |
| mixih | 192% | 148% | 127% | mixih | 223% | 148% | 113% |
| average for i | 168% | 133% | 116% | average for i | 199% | 133% | 101% |
| MIX1 | MIX2 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| mixil | 9412 | 14,790 | 20,168 | 44,370 | mixil | 19,341 | 14,790 | 11,973 | 46,103 |
| mixim | 9412 | 14,790 | 20,168 | 44,370 | mixim | 19,341 | 14,790 | 11,973 | 46,103 |
| mixih | 9412 | 14,790 | 20,168 | 44,370 | mixih | 19,341 | 14,790 | 11,973 | 46,103 |
| total by column | 28,235 | 44,370 | 60,504 | 133,109 | total by column | 58,022 | 44,370 | 35,918 | 138,310 |
| average for i | 7% | 12% | 16% | 35% | average for i | 15% | 12% | 10% | 37% |
| MIX1 | MIX2 | ||||||
|---|---|---|---|---|---|---|---|
| mixil | 185 | 273 | 361 | mixil | 198 | 273 | 348 |
| mixim | 195 | 283 | 371 | mixim | 208 | 283 | 358 |
| mixih | 205 | 293 | 381 | mixih | 218 | 293 | 368 |
| average for i | 195 | 283 | 371 | average for i | 208 | 283 | 358 |
| MIX1 | MIX2 | ||||||
|---|---|---|---|---|---|---|---|
| mix1j | mix2j | mix3j | mix1j | mix2j | mix3j | ||
| mixil | 48% | 39% | 35% | mixil | 58% | 39% | 30% |
| mixim | 56% | 44% | 39% | mixim | 66% | 44% | 34% |
| mixih | 64% | 49% | 42% | mixih | 74% | 49% | 38% |
| average for i | 56% | 44% | 39% | average for i | 66% | 44% | 34% |
References
- A Cura del Tavolo Tecnico di Odontoiatria. In Indicazioni Operative per L‘attività Odontoiatrica Durante la Fase 2 Della Pandemia Covid-19. Versione 30 Maggio; 2020. Available online: http://www.salute.gov.it/imgs/C_17_pubblicazioni_2917_allegato.pdf (accessed on 30 May 2020).
- Consejo General de Dentistas de España. Plan Estratégico de Acción Para El Periodo Posterior A La Crisis Creada Por El COVID-19; 2020. Available online: https://www.consejodentistas.es/comunicacion/actualidad-consejo/notas-de-prensa-consejo/item/1763-plan-estrategico-de-accion-para-el-periodo-posterior-a-la-crisis-creada-por-el-covid-19.html (accessed on 13 April 2020).
- ADA. Interim Guidance for Minimizing Risk of COVID-19 Transmission; 2020. Available online: https://www.ada.org/en/publications/ada-news/2020-archive/april/ada-releases-interim-guidance-on-minimizing-covid-19-transmission-risk-when-treating-emergencies#:~:text=The%20ADA%20Interim%20Guidance%20for,precautions%2C%20including%20personal%20protective%20equipment%3B (accessed on 1 April 2020).
- Sneader, K.; Singhal, S. Beyond Coronavirus: The Path to the Next Normal. 2020. Available online: https://www.mckinsey.com/industries/healthcare-systems-and-services/our-insights/beyond-coronavirus-the-path-to-the-next-normal (accessed on 23 March 2020).
- Bartik, A.W.; Bertrand, B.; Cullen, Z.; Glaeser, E.L.; Luca, M.; Stanton, C. How Are Small Businesses Adjusting to COVID-19? Early Evidence from a Survey. SSRN Electron. J. 2020. [Google Scholar] [CrossRef] [Green Version]
- Schwendicke, F.; Krois, J.; Gomez, J. Impact of SARS-CoV2 (Covid-19) on Dental Practices. Econ. Anal. J. Dent. 2020, 99, 103387. [Google Scholar]
- Associazione Italiana Odontoiatri (AIO). Prospettive di Sviluppo per un Piano Nazionale per L’odontoiatria. Available online: https://www.camera.it/temiap/2016/11/25/OCD177-2533.pdf (accessed on 25 November 2016).
- Widstrom, E.; Eaton, K.A. Oral Healthcare Systems in The Extended European Union. Oral Health Prev. Dent. 2004, 2, 155–194. [Google Scholar] [PubMed]
- McLaughlin, D.B.; Olson, J.R. Healthcare Operations Management; Health Administration Press: Chicago, IL, USA, 2017. [Google Scholar]
- Warren, C.S.; Reeve, J.M.; Duchac, J. Financial & Managerial Accounting, 14th ed.; Cengage Learning: Andover, UK, 2016. [Google Scholar]
- Birkenshaw Garabelli, C. Revenue Cycle Management in Healthcare; Taylor & Francis: Milton Park, UK, 2016. [Google Scholar]
- Associazione Nazionale Dentisti Italiani (ANDI). Nomenclatore E Tariffario Andi. 2009. Available online: https://www.andipg.it/images/allegati/2016/ANDI_Tariffario_2009.pdf (accessed on 20 December 2016).
- Bizzoca, M.E.; Campisi, G.; Lo Muzio, L. An Innovative Risk‑Scoring System of Dental Procedures and Safety Protocols in the COVID‑19 Era. BMC Oral Health 2020, 20, 301. [Google Scholar] [CrossRef] [PubMed]
- Pyankov, O.V.; Bodnev, S.A.; Pyankova, O.G.; Agranovski, I.E. Survival of aerosolized coronavirus in the ambient air. J. Aerosol Sci. 2018, 115, 158–163. [Google Scholar] [CrossRef] [PubMed]
- Reeves, M.; Koehn, N.F.; Neeley, T.; Berinato, S. Coronavirus: Leadership and Recovery: The Insights You Need from Harvard Business Review; Harvard Business Review Press: Boston, MA, USA, 2020. [Google Scholar]
- ANSA. Fase 3: Il 20% Degli Italiani Teme di Non Potersi Permettere il Dentista. 2020. Available online: https://www.ansa.it/canale_saluteebenessere/notizie/salute_denti_gengive/2020/06/14/fase-3-20-italiani-teme-di-non-potersi-permettere-dentista_c7ef54c4-9068-4fd5-9594-f0765d4245d8.html (accessed on 3 June 2020).
- Scott, J.; Labno, A.; Rappl, B.; Kellar, J.; Rosenberg, B. Boston Consulting Group, Restoring Patients Confidence in Elective Health Care. 2020. Available online: https://www.bcg.com/it-it/publications/2020/elective-health-care-post-covid-19 (accessed on 27 May 2020).
- Monson, C. What Small Businesses Need to Survive the Coronavirus Crisis. 2020. Available online: https://hbr.org/2020/03/what-small-businesses-need-to-survive-the-coronavirus-crisis?referral=03759&cm_vc=rr_item_page.bottom (accessed on 27 March 2020).
- Anthony, R.N.; Hawkins, D.F.; Merchant, K.A. Accounting Text and Cases; McGraw Hill: New York, NY, USA, 2019. [Google Scholar]
- Bizzoca, M.E.; Campisi, G.; Lo Muzio, L. Covid-19 Pandemic: What Changes for Dentists and Oral Medicine Experts? A Review and Novel Approaches to Infection Containment. Int. J. Environ. Res. Public Health 2020, 17, 3793. [Google Scholar]


| Procedure | Timing and Risk-Level Post-COVID-19 | ||
|---|---|---|---|
| Duration of Procedure (Minutes) + 45 min after Each Patient | Risk-Level | Charges from the National Italian Price List (in Monetary Units) * | |
| Orthodontic checks | 30–60 | Low | 1 **: Orthodontic interceptive therapy: 1500/year Fixed orthodontic therapy: 2000/year Lingual orthodontics: 3000/yearInvisible aligners (complete therapy): 4500/year |
| Manual reduction of dislocation of the jaw | ≤30 | Low | 150 |
| Mobile/fixed orthodontic appliance positioning | >60 | Low | 1 |
| X-rays | ≤30 | Low | Intraoral X-ray: 30 Intraoral X-ray status: 200 Orthopantomography: 80 Lateral Teleradiograph: 80 |
| Periodontal therapy | ≤30 | Low | 100 |
| Topical treatment of dental hypersensitivity and caries prophylaxis | ≤30 | Low | 100 |
| Test of night guards | ≤30 | Low | 800 |
| Impression | ≤30 | Low | |
| Dental prosthesis tests, positioning and adaptation (temporary/definitive, removable/fixed) | 30–60 | Low | 2: Gold-porcelain/zirconia crown750 |
| Biopsy | ≤30 | Low | 200 |
| Bone graft (autogenous/biocompatible material) without rotating tools | >60 | Low | 400–700 (with membrane) |
| Mucogingival surgery (quadrant) | 30–60 | Low | 600 |
| Subgingival curettage without rotating tools (quadrant) | 30–60 | Low | 500 |
| Removal of cysts or small benign neoplasms | 30–60 | Low | 500 |
| Surgical medication | ≤30 | Low | |
| Oral minor surgery (e.g., abscess incision, frenulectomy, frenulotomy) | ≤30 | Low | 150–300 |
| Salivary stone removal | ≤30 | Low | 150–500 |
| Extraction without rotating tools | 30–60 | Low | 130 |
| Gingivectomy/gingivoplasty | ≤30 | Low | 300 |
| Endodontic treatment (1 root) with rubber dam (in subsequent appointment after access cavity) | ≤30 | Low | 3: 180 + 100 (for each additional canal) |
| Pulp-capping, pulpotomy, pulpectomy (in subsequent appointment after access cavity) with rubber dam | 30–60 | Low | 130 |
| Bleaching | >60 | Medium | 450 |
| Orthodontic splinting (1 dental arch) | ≤30 | Medium | 200 |
| Orthodontic splinting (2 dental arches) | 30–60 | Medium | 400 |
| Periodontal splinting (1 dental arch) | ≤30 | Medium | 200 |
| Periodontal splinting (2 dental arch) | 30–60 | Medium | 400 |
| Intra-oral examination | ≤30 | Medium | 100 |
| Tartar removal | 30–60 | High | 110 |
| Extraction with rotating tools | 30–60 | High | 150–300 |
| Sinus lift | >60 | High | 600–1200 |
| Cavity access (rotating instruments) | ≤30 | High | 3 |
| Implantology | >60 | High | 1200 |
| Subgingival curettage (quadrant) (rotating tools) | ≤30 | High | 500 |
| Resective/regenerative bone surgery (rotating tools) | >60 | High | 750–900 |
| Rhizectomy/rhizotomy (rotating tools) | 30–60 | High | 150–300 |
| Sealing of dental grooves | ≤30 | High | 50 (for each tooth) |
| Apicectomy with retrograde filling | >60 | High | 300–500 |
| Autologous bone harvest (rotating tools) | ≤30 | High | 300–450 |
| Abutment tooth preparation | ≤30 | High | 2 |
| Odontoplasty (1 tooth) | ≤30 | High | 100–250 (for each tooth) |
| Simple/complex filling using rotating tools | 30–60 | High | 150–250 |
| Extraction of impacted tooth with rotating tools | >60 | High | 300–500 |
| Dental Treatments | Risk-Level | |||
|---|---|---|---|---|
| Low | Medium | High | ||
| tw ≤ 45’(low) |
|
|
| |
| TIMING (tw) | 45’ < tw ≤ 75’(medium) |
|
|
|
| tw > 75’(high) |
|
|
| |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lo Nigro, G.; Bizzoca, M.E.; Lo Muzio, L.; Campisi, G. The Management of Dental Practices in the Post-COVID 19 Era: An Economic and Operational Perspective. Int. J. Environ. Res. Public Health 2020, 17, 8905. https://doi.org/10.3390/ijerph17238905
Lo Nigro G, Bizzoca ME, Lo Muzio L, Campisi G. The Management of Dental Practices in the Post-COVID 19 Era: An Economic and Operational Perspective. International Journal of Environmental Research and Public Health. 2020; 17(23):8905. https://doi.org/10.3390/ijerph17238905
Chicago/Turabian StyleLo Nigro, Giovanna, Maria Eleonora Bizzoca, Lorenzo Lo Muzio, and Giuseppina Campisi. 2020. "The Management of Dental Practices in the Post-COVID 19 Era: An Economic and Operational Perspective" International Journal of Environmental Research and Public Health 17, no. 23: 8905. https://doi.org/10.3390/ijerph17238905
APA StyleLo Nigro, G., Bizzoca, M. E., Lo Muzio, L., & Campisi, G. (2020). The Management of Dental Practices in the Post-COVID 19 Era: An Economic and Operational Perspective. International Journal of Environmental Research and Public Health, 17(23), 8905. https://doi.org/10.3390/ijerph17238905








