Re-Visiting Maximal Heart Rate Prediction Using Cross-Validation in Population Aged 7–55 Years
Abstract
1. Introduction
2. Methods
2.1. Study Protocol
2.2. Study Participants
2.3. Measures
2.3.1. Anthropometric Measurements
2.3.2. Metabolic Gas Analyzer Test
2.3.3. Maximal Heart Rate (HRmax)
2.4. Statistical Analysis
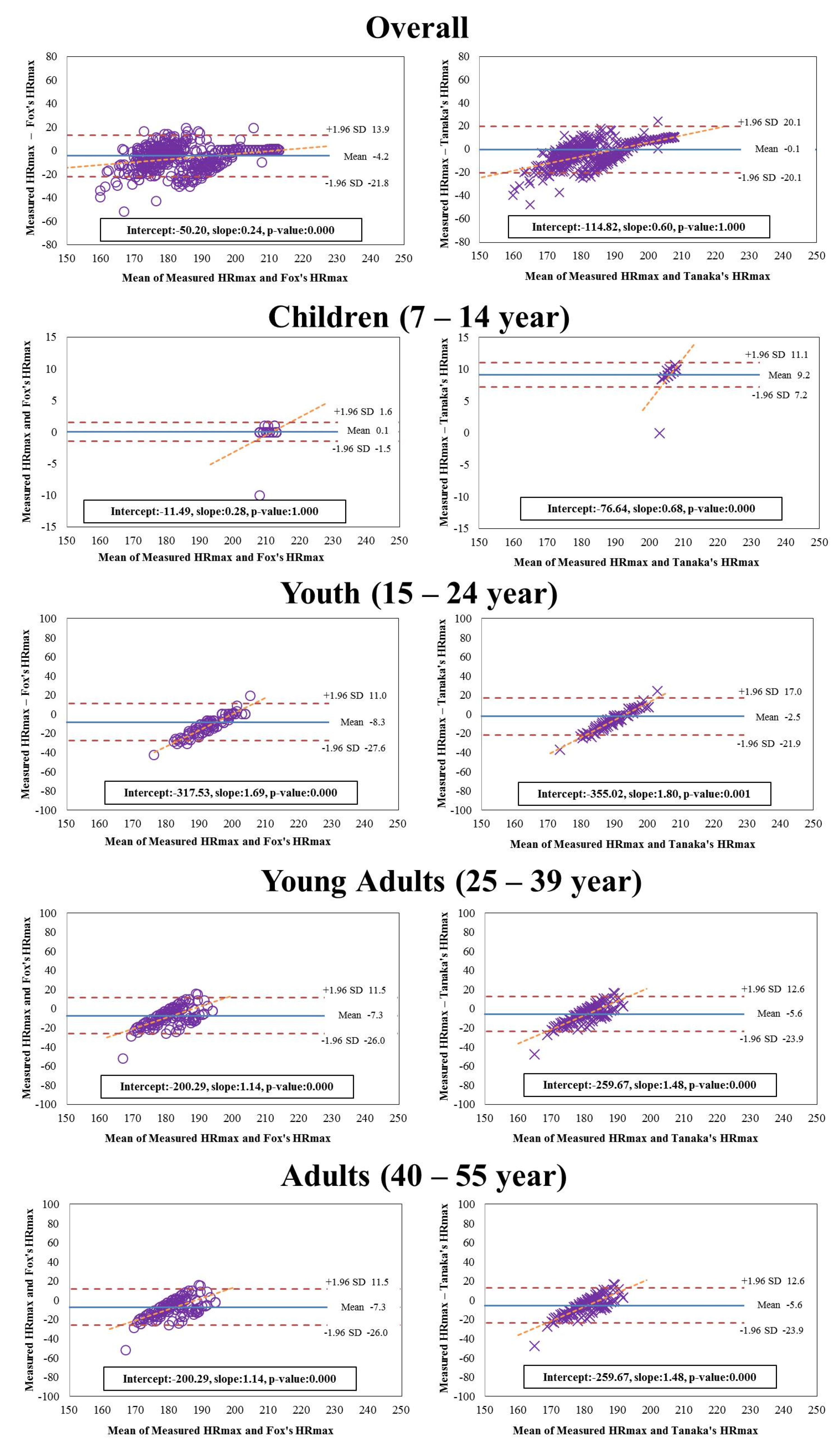
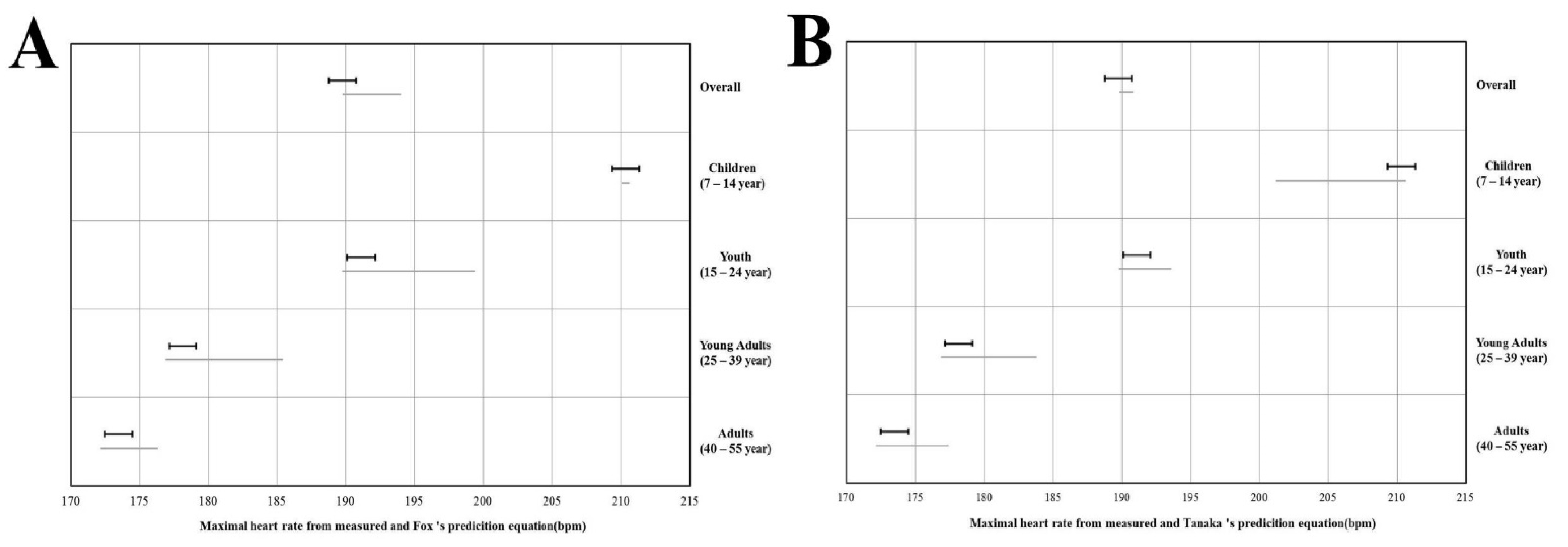
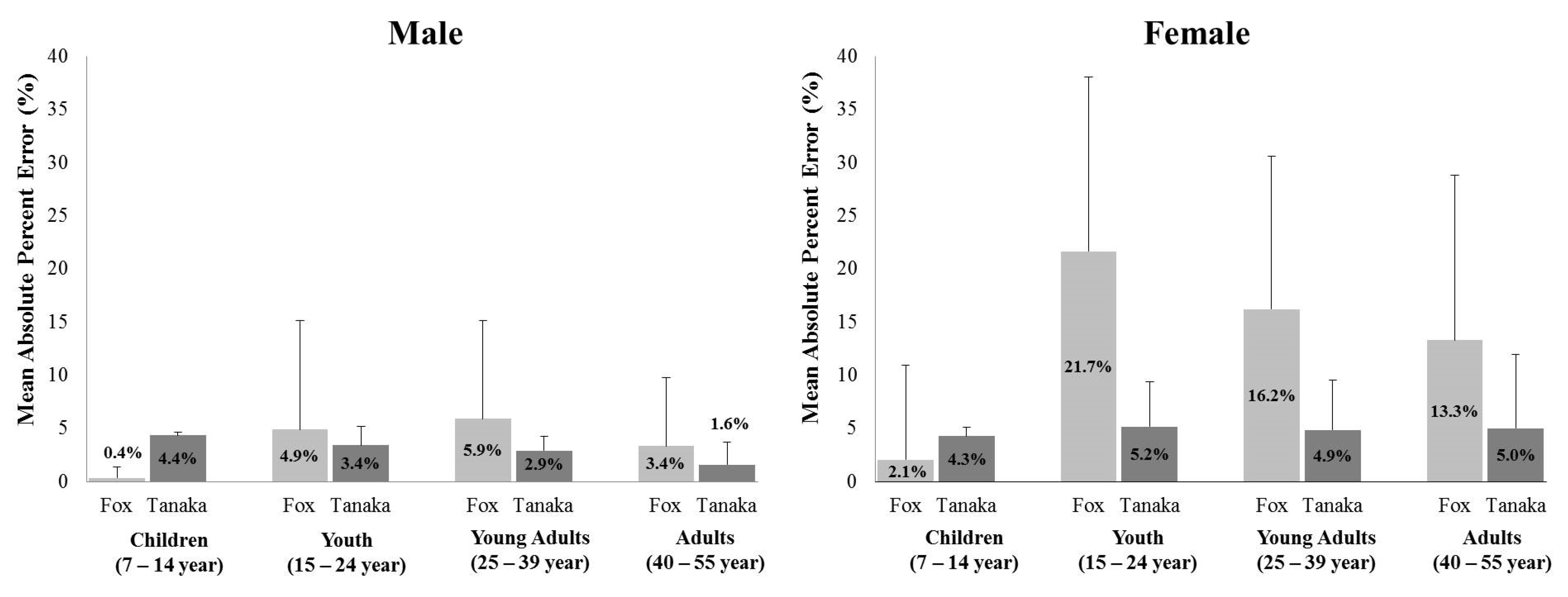
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hui, S.S.; Chan, J.W. The relationship between heart rate reserve and oxygen uptake reserve in children and adolescents. Res. Q Exerc. Sport 2006, 77, 41–49. [Google Scholar] [CrossRef] [PubMed]
- American College of Sports Medicine. ACSM’s Guidelines for Exercise Testing and Prescription; Lippincott Williams & Wilkins: Philadelphia, PA, USA, 2013. [Google Scholar]
- Mahon, A.D.; Marjerrison, A.D.; Lee, J.D.; Woodruff, M.E.; Hanna, L.E. Evaluating the prediction of maximal heart rate in children and adolescents. Res. Q. Exerc. Sport 2010, 81, 466–471. [Google Scholar] [CrossRef] [PubMed]
- Nes, B.M.; Janszky, I.; Wisløff, U.; Støylen, A.; Karlsen, T. Age-predicted maximal heart rate in healthy subjects: The HUNT fitness study. Scand. J. Med. Sci. Sports 2013, 23, 697–704. [Google Scholar] [CrossRef] [PubMed]
- Taylor, J.L.; Holland, D.J.; Spathis, J.G.; Beetham, K.S.; Wisløff, U.; Keating, S.E.; Coombes, J.S. Guidelines for the delivery and monitoring of high intensity interval training in clinical populations. Prog. Cardiovasc. Dis. 2019, 62, 140–146. [Google Scholar] [CrossRef] [PubMed]
- Albouaini, K.; Egred, M.; Alahmar, A.; Wright, D.J. Cardiopulmonary exercise testing and its application. Postgrad. Med. J. 2007, 83, 675–682. [Google Scholar] [CrossRef] [PubMed]
- Paridon, S.M.; Alpert, B.S.; Boas, S.R.; Cabrera, M.E.; Caldarera, L.L.; Daniels, S.R.; Kimball, T.R.; Knilans, T.K.; Nixon, P.A.; Rhodes, J. Clinical stress testing in the pediatric age group: A statement from the American Heart Association Council on Cardiovascular Disease in the Young, Committee on Atherosclerosis, Hypertension, and Obesity in Youth. Circulation 2006, 113, 1905–1920. [Google Scholar] [CrossRef]
- Washington, R.L.; Bricker, J.T.; Alpert, B.S.; Daniels, S.R.; Deckelbaum, R.J.; Fisher, E.A.; Gidding, S.S.; Isabel-Jones, J.; Kavey, R.E.; Marx, G.R. Guidelines for exercise testing in the pediatric age group. From the Committee on Atherosclerosis and Hypertension in Children, Council on Cardiovascular Disease in the Young, the American Heart Association. Circulation 1994, 90, 2166–2179. [Google Scholar] [CrossRef]
- Nikolaidis, P.T.; Rosemann, T.; Knechtle, B. Age-Predicted Maximal Heart Rate in Recreational Marathon Runners: A Cross-Sectional Study on Fox’s and Tanaka’s Equations. Front. Physiol. 2018, 9, 226. [Google Scholar] [CrossRef]
- Robinson, S. Experimental studies of physical fitness in relation to age. Arbeitsphysiologie 1938, 10, 251–323. [Google Scholar] [CrossRef]
- Åstrand, P.-O. Experimental Studies of Physical Working Capacity in Relation to Sex and Age; Munksgaard Forlag: Copenhagen, Denmark, 1952. [Google Scholar]
- Fairbarn, M.S.; Blackie, S.P.; McElvaney, N.G.; Wiggs, B.R.; Paré, P.D.; Pardy, R.L. Prediction of Heart Rate and Oxygen Uptake During Incremental and Maximal Exercise in Healthy Adults. Chest 1994, 105, 1365–1369. [Google Scholar] [CrossRef]
- Gulati, M.; Shaw, L.J.; Thisted, R.A.; Black, H.R.; Bairey Merz, C.N.; Arnsdorf, M.F. Heart rate response to exercise stress testing in asymptomatic women: The St. James women take heart project. Circulation 2010, 122, 130–137. [Google Scholar] [CrossRef] [PubMed]
- Blair, S.; Lavey, R.; Goodyear, N.; Gibbons, L.; Cooper, K. Physiologic responses to maximal graded exercise testing in apparently healthy white women aged 18 to 75 years. J. Card. Rehabil. 1984, 4, 459–468. [Google Scholar]
- Engels, H.-J.; Zhu, W.; Moffatt, R.J. An Empirical Evaluation of the Prediction of Maximal Heart Rate. Res. Q. Exerc. Sport 1998, 69, 94–98. [Google Scholar] [CrossRef] [PubMed]
- Whaley, M.H.; Kaminsky, L.A.; Dwyer, G.B.; Getchell, L.H.; Norton, J.A. Predictors of over-and underachievement of age-predicted maximal heart rate. Med. Sci. Sports Exerc. 1992, 24, 1173–1179. [Google Scholar] [CrossRef]
- Fox, S., 3rd; Naughton, J.P.; Haskell, W. Physical activity and the prevention of coronary heart disease. Ann. Clin. Res. 1971, 3, 404–432. [Google Scholar]
- Tanaka, H.; Monahan, K.D.; Seals, D.R. Age-predicted maximal heart rate revisited. J. Am. Coll. Cardiol. 2001, 37, 153–156. [Google Scholar] [CrossRef]
- Franckowiak, S.C.; Dobrosielski, D.A.; Reilley, S.M.; Walston, J.D.; Andersen, R.E. Maximal heart rate prediction in adults that are overweight or obese. J. Strength Cond. Res. Natl. Strength Cond. Assoc. 2011, 25, 1407. [Google Scholar] [CrossRef]
- Esco, M.R.; Chamberlain, N.; Flatt, A.A.; Snarr, R.L.; Bishop, P.A.; Williford, H.N. Cross-Validation of Age-Predicted Maximal Heart Rate Equations among Female Collegiate Athletes. J. Strength Cond. Res. 2015, 29, 3053–3059. [Google Scholar] [CrossRef]
- Robergs, R.A.; Landwehr, R. The surprising history of the “HRmax = 220-age” equation. J. Exerc. Physiol. Online 2002, 5, 1–10. [Google Scholar]
- Gelbart, M.; Ziv-Baran, T.; Williams, C.A.; Yarom, Y.; Dubnov-Raz, G. Prediction of Maximal Heart Rate in Children and Adolescents. Clin. J. Sport Med. Off. J. Can. Acad. Sport Med. 2017, 27, 139–144. [Google Scholar] [CrossRef]
- Paap, D.; Takken, T. Reference values for cardiopulmonary exercise testing in healthy adults: A systematic review. Expert Rev. Cardiovasc. Ther. 2014, 12, 1439–1453. [Google Scholar] [CrossRef]
- Machado, F.A.; Denadai, B.S. Validity of maximum heart rate prediction equations for children and adolescents. Arq. Bras. De Cardiol. 2011, 97, 136–140. [Google Scholar] [CrossRef] [PubMed]
- Powers, S.K.; Howley, E.T.; Quindry, J. Exercise Physiology: Theory and Application to Fitness and Performance; McGraw-Hill: New York, NY, USA, 2007. [Google Scholar]
- Hur, Y.-M.; Kaprio, J.; Iacono, W.G.; Boomsma, D.I.; McGue, M.; Silventoinen, K.; Martin, N.G.; Luciano, M.; Visscher, P.M.; Rose, R.J.; et al. Genetic influences on the difference in variability of height, weight and body mass index between Caucasian and East Asian adolescent twins. Int. J. Obes. 2008, 32, 1455–1467. [Google Scholar] [CrossRef] [PubMed]
- O’Neal, W.T.; Alam, A.B.; Sandesara, P.B.; Claxton, J.S.; MacLehose, R.F.; Chen, L.Y.; Bengtson, L.G.S.; Chamberlain, A.M.; Norby, F.L.; Lutsey, P.L.; et al. Sex and racial differences in cardiovascular disease risk in patients with atrial fibrillation. PLoS ONE 2019, 14, e0222147. [Google Scholar] [CrossRef]
- Egan, K.J.; Knutson, K.L.; Pereira, A.C.; von Schantz, M. The role of race and ethnicity in sleep, circadian rhythms and cardiovascular health. Sleep Med. Rev. 2017, 33, 70–78. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, O.B.; Boschi-Pinto, C.; Lopez, A.D.; Murray, C.J.; Lozano, R.; Inoue, M. Age Standardization of Rates: A New WHO Standard; World Health Organization: Geneva, Switzerland, 2001; Volume 9, pp. 1–14. [Google Scholar]
- Eisenmann, J.C.; Brisko, N.; Shadrick, D.; Welsh, S. Comparative analysis of the Cosmed Quark b2 and K4b2 gas analysis systems during submaximal exercise. J. Sports Med. Phys. Fit. 2003, 43, 150–155. [Google Scholar]
- Bruce, R.A.; Blackmon, J.R.; Jones, J.W.; Strait, G. Exercising testing in adult normal subjects and cardiac patients. Pediatrics 1963, 32, 742–756. [Google Scholar] [CrossRef]
- Engström, E.; Ottosson, E.; Wohlfart, B.; Grundström, N.; Wisén, A. Comparison of heart rate measured by Polar RS400 and ECG, validity and repeatability. Adv. Physiother. 2012, 14, 115–122. [Google Scholar] [CrossRef][Green Version]
- de Rezende Barbosa, M.P.d.C.; Silva, N.T.d.; de Azevedo, F.M.; Pastre, C.M.; Vanderlei, L.C.M. Comparison of Polar® RS 800G3™ heart rate monitor with P olar® S810i™ and electrocardiogram to obtain the series of RR intervals and analysis of heart rate variability at rest. Clin. Physiol. Funct. Imaging 2016, 36, 112–117. [Google Scholar] [CrossRef]
- Stahl, S.E.; An, H.-S.; Dinkel, D.M.; Noble, J.M.; Lee, J.-M. How accurate are the wrist-based heart rate monitors during walking and running activities? Are they accurate enough? BMJ Open Sport Exerc. Med. 2016, 2, e000106. [Google Scholar] [CrossRef] [PubMed]
- Cleary, M.A.; Hetzler, R.K.; Wages, J.J.; Lentz, M.A.; Stickley, C.D.; Kimura, I.F. Comparisons of Age-Predicted Maximum Heart Rate Equations in College-Aged Subjects. J. Strength Cond. Res. 2011, 25, 2591–2597. [Google Scholar] [CrossRef] [PubMed]
- Gellish, R.L.; Goslin, B.R.; Olson, R.E.; McDONALD, A.; Russi, G.D.; Moudgil, V.K. Longitudinal Modeling of the Relationship between Age and Maximal Heart Rate. Med. Sci. Sports Exerc. 2007, 39, 822–829. [Google Scholar] [CrossRef] [PubMed]
- Nikolaidis, P.; Padulo, J.; Chtourou, H.; Torres-Luque, G.; Afonso, J.; Heller, J. Estimating Maximal Heart Rate with the ‘220-Age’ Formula in Adolescent Female Volleyball Players: A Preliminary Study. Hum. Mov. 2014, 15, 166–170. [Google Scholar] [CrossRef]
- Sarzynski, M.; Rankinen, T.; Earnest, C.; Leon, A.; Rao, D.; Skinner, J.; Bouchard, C. Measured maximal heart rates compared to commonly used age-based prediction equations in the heritage family study. Am. J. Hum. Biol. 2013, 25, 695–701. [Google Scholar] [CrossRef]
- Cicone, Z.S.; Holmes, C.J.; Fedewa, M.V.; MacDonald, H.; Esco, M.R. Age-Based Prediction of Maximal Heart Rate in Children and Adolescents: A Systematic Review and Meta-Analysis. Res. Q. Exerc. Sport 2019, 90, 417–428. [Google Scholar] [CrossRef]
- Papadopoulou, S.D.; Papadopoulou, S.K.; Alipasali, F.; Hatzimanouil, D.; Rosemann, T.; Knechtle, B.; Nikolaidis, P.T. Validity of Prediction Equations of Maximal Heart Rate in Physically Active Female Adolescents and the Role of Maturation. Medicina 2019, 55, 735. [Google Scholar] [CrossRef]
- Fernhall, B.; McCubbin, J.A.; Pitetti, K.H.; Rintala, P.; Rimmer, J.H.; Millar, A.L.; De Silva, A. Prediction of maximal heart rate in individuals with mental retardation. Med. Sci. Sports Exerc. 2001, 33, 1655–1660. [Google Scholar] [CrossRef]
- Froelicher, V.; Myers, J. Exercise and the Heart, 4th ed.; WB Saunders: Philadelphia, PA, USA, 2000. [Google Scholar]
| Variables | All Participants | |||
|---|---|---|---|---|
| No. (%) | Mean ± SD | |||
| Anthropometrics × Age | Height (cm) | 7–14 | 144.22 ± 14.35 | |
| 15–24 | 167.69 ± 7.88 | |||
| 25–39 | 162.54 ± 5.55 | |||
| 40–55 | 163.45 ± 7.96 | |||
| Weight (kg) | 7–14 | 39.62 ± 17.96 | ||
| 15–24 | 62.66 ± 10.36 | |||
| 25–39 | 59.01 ± 9.21 | |||
| 40–55 | 64.35 ± 11.53 | |||
| BMI (kg·m−2) | 7–14 | 18.14 ± 4.27 | ||
| 15–24 | 22.13 ± 2.63 | |||
| 25–39 | 22.27 ± 3.21 | |||
| 40–55 | 23.92 ± 3.27 | |||
| maximal exercise Responses × Age | HRmax (bpm) | 7–14 | 209.89 ± 7.12 | |
| 15–24 | 201.12 ± 13.81 | |||
| 25–39 | 193.64 ± 18.96 | |||
| 40–55 | 189.90 ± 21.40 | |||
| VO2max (mL·kg−1·min−1) | 7–14 | 53.76 ± 9.20 | ||
| 15–24 | 46.05 ± 12.30 | |||
| 25–39 | 34.08 ± 6.45 | |||
| 40–55 | 33.22 ± 7.21 | |||
| Respiratory exchange ratio | 7–14 | 1.17 ± 0.13 | ||
| 15–24 | 1.22 ± 0.14 | |||
| 25–39 | 1.18 ± 0.08 | |||
| 40–55 | 1.18 ± 0.09 | |||
| Variables | Measured HRmax | Fox′s HRmax | Tanaka′s HRmax | ||
|---|---|---|---|---|---|
| Overall | 189.77 ± 17.17 | 193.99 ± 13.74 *** | 189.79 ± 9.67 | ||
| Gender | Male | 202.56 ± 11.96 | 202.98 ± 11.63 | 196.08 ± 8.14 *** | |
| Female | 180.63 ± 14.24 | 187.57 ± 11.31 *** | 185.29 ± 7.91 *** | ||
| Age (year) | 7–14 | 210.35 ± 2.22 | 210.29 ± 2.11 | 201.20 ± 1.47 *** | |
| 15–24 | 191.11 ± 10.34 | 199.41 ± 2.35 *** | 193.59 ± 1.64 ** | ||
| 25–39 | 178.15 ± 9.46 | 185.41 ± 4.57 *** | 183.79 ± 3.20 *** | ||
| 40–55 | 173.49 ± 10.40 | 176.29 ± 3.11 *** | 177.40 ± 2.18 *** | ||
| Gender × Age | Male | 7–14 | 210.32 ± 2.20 | 210.21 ± 2.13 | 201.14 ± 1.49 *** |
| 15–24 | 196.76 ± 7.21 | 200.05 ± 2.64 *** | 194.03 ± 1.85 ** | ||
| 25–39 | 189.13 ± 7.51 | 190.25 ± 5.97 | 187.17 ± 4.17 | ||
| 40–55 | 178.95 ± 5.22 | 176.86 ± 2.41 * | 177.80 ± 1.69 | ||
| Female | 7–14 | 210.57 ± 2.34 | 210.77 ± 1.87 | 201.53 ± 1.30 *** | |
| 15–24 | 187.38 ± 10.42 | 198.99 ± 2.03 *** | 193.29 ± 1.42 *** | ||
| 25–39 | 177.54 ± 9.20 | 185.15 ± 4.35 *** | 183.60 ± 3.04 *** | ||
| 40–55 | 171.86 ± 11.05 | 176.11 ± 3.27 *** | 177.27 ± 2.29 *** | ||
| Variables | Parameter | HRmax Regression Equation | New HRmax Prediction Equation | ||
|---|---|---|---|---|---|
| Coefficients (95% CI) | Standard Error | ||||
| Overall | Male | Intercept | 218.984 (218.117 to 219.851) | 0.440 | 219 − age |
| Age | −0.965 (−1.007 to −0.923) | 0.021 | |||
| Female | Intercept | 208.830 (205.715 to 211.945) | 1.584 | 209 − (0.9 × age) | |
| Age | −0.869 (−0.960 to −0.779) | 0.046 | |||
| Children | Male | Intercept | 220.310 (220.090 to 220.529) | 0.111 | 220 − age |
| Age | −1.020 (−1.042 to −0.998) | 0.011 | |||
| Female | Intercept | 217.760 (214.179 to 221.340) | 1.748 | 218 − (0.8 × age) | |
| Age | −0.779 (−1.159 to −0.399) | 0.186 | |||
| Youth | Male | Intercept | 213.619 (200.147 to 227.090) | 6.735 | 214 − (0.8 × age) |
| Age | −0.845 (−1.515 to −0.176) | 0.335 | |||
| Female | Intercept | 213.600 (191.871 to 235.328) | 10.940 | 214 − (1.2 × age) | |
| Age | −1.248 (−2.277 to −0.218) | 0.518 | |||
| Young Adults | Male | Intercept | 211.751 (181.448 to 242.053) | 12.384 | 212 − (0.8 × age) |
| Age | −0.761 (−1.762 to 0.241) | 0.409 | |||
| Female | Intercept | 187.700 (175.492 to 199.907) | 6.175 | 189 − (0.3 × age) | |
| Age | −0.291 (−0.639 to 0.056) | 0.176 | |||
| Adults | Male | Intercept | 210.269 (180.067 to 240.471) | 14.877 | 210 − (0.7 × age) |
| Age | −0.726 (−1.425 to −0.027) | 0.344 | |||
| Female | Intercept | 189.836 (163.521 to 216.150) | 13.293 | 190 − (0.4 × age) | |
| Age | −0.410 (−1.007 to 0.188) | 0.302 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.-H.; Jung, H.C.; Jung, Y.-S.; Song, J.-K.; Lee, J.-M. Re-Visiting Maximal Heart Rate Prediction Using Cross-Validation in Population Aged 7–55 Years. Int. J. Environ. Res. Public Health 2022, 19, 8509. https://doi.org/10.3390/ijerph19148509
Park J-H, Jung HC, Jung Y-S, Song J-K, Lee J-M. Re-Visiting Maximal Heart Rate Prediction Using Cross-Validation in Population Aged 7–55 Years. International Journal of Environmental Research and Public Health. 2022; 19(14):8509. https://doi.org/10.3390/ijerph19148509
Chicago/Turabian StylePark, Jeong-Hui, Hyun Chul Jung, Yeon-Sung Jung, Jong-Kook Song, and Jung-Min Lee. 2022. "Re-Visiting Maximal Heart Rate Prediction Using Cross-Validation in Population Aged 7–55 Years" International Journal of Environmental Research and Public Health 19, no. 14: 8509. https://doi.org/10.3390/ijerph19148509
APA StylePark, J.-H., Jung, H. C., Jung, Y.-S., Song, J.-K., & Lee, J.-M. (2022). Re-Visiting Maximal Heart Rate Prediction Using Cross-Validation in Population Aged 7–55 Years. International Journal of Environmental Research and Public Health, 19(14), 8509. https://doi.org/10.3390/ijerph19148509








