Study on Safety Coefficient of Sedimentary Bauxite Strip Pillar under Valley Terrain
Abstract
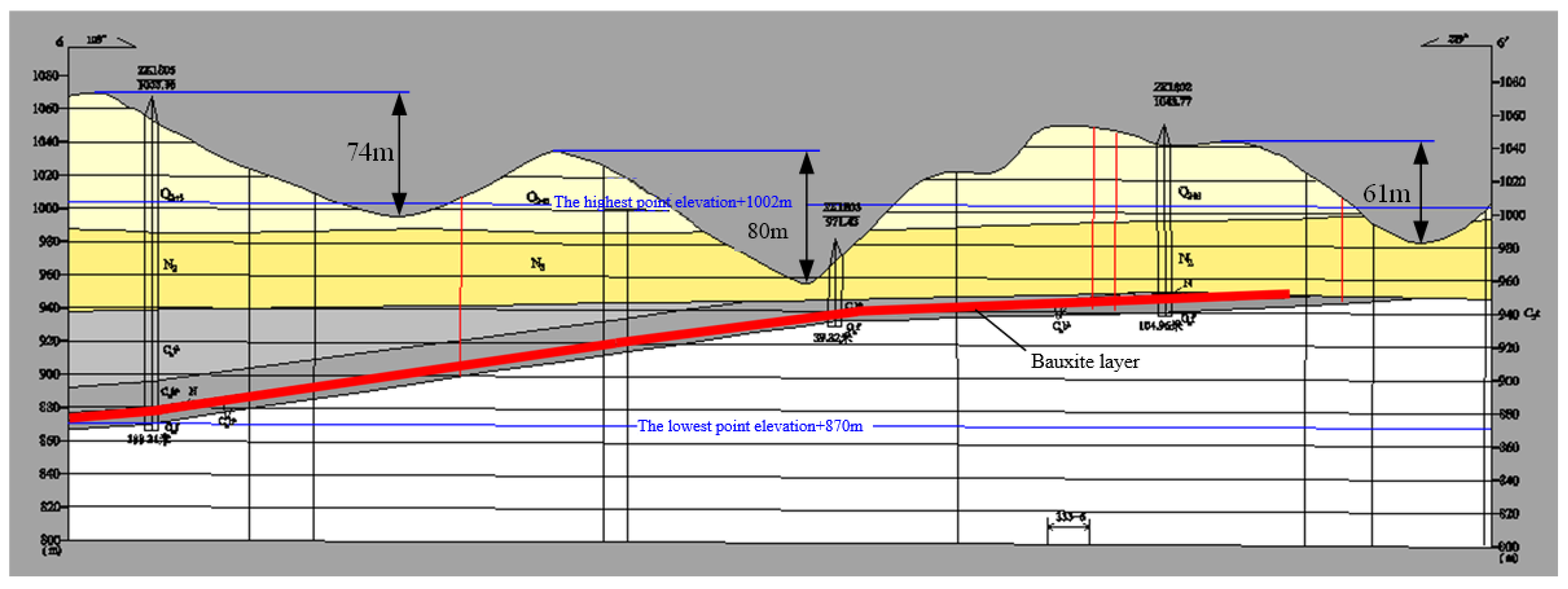
:1. Introduction
2. Mechanical Model of Pillar under Valley Terrain
3. Pillar Safety Coefficient under Valley Terrain
4. Parameter Influence Analysis
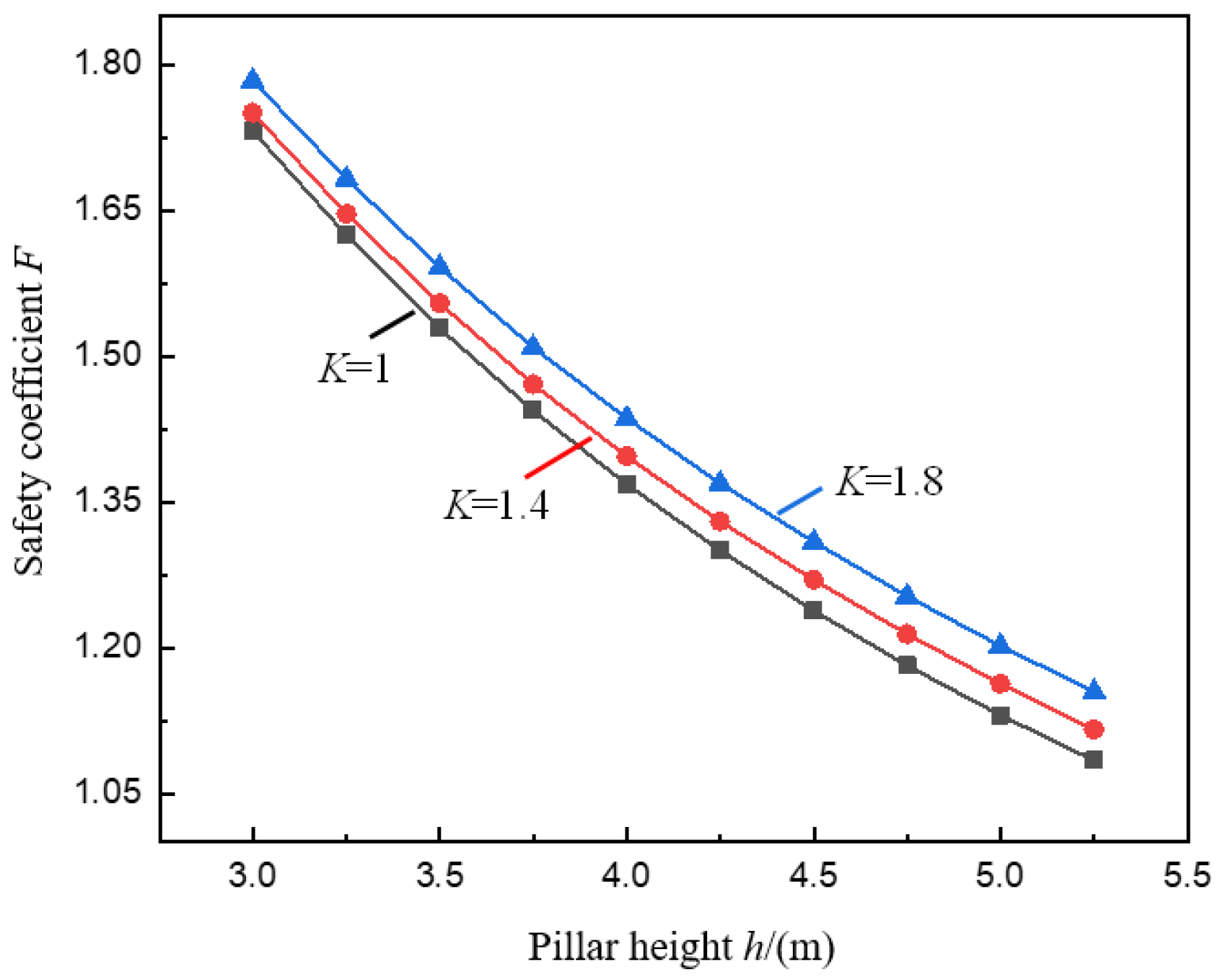
4.1. Influence Analysis of Structural Parameters of the Goaf
| Parameters | Cohesion c/MPa | Internal Friction Angle φ/° | Test Weight γ/kN·m−3 | Poisson’s Ratio μ | Constant m | Constant s | Uniaxial Compressive Strength σm/MPa |
|---|---|---|---|---|---|---|---|
| Value | 1.29 | 31.7 | 22.5 | 0.24 | 6.7 | 0.3 | 26.86 |
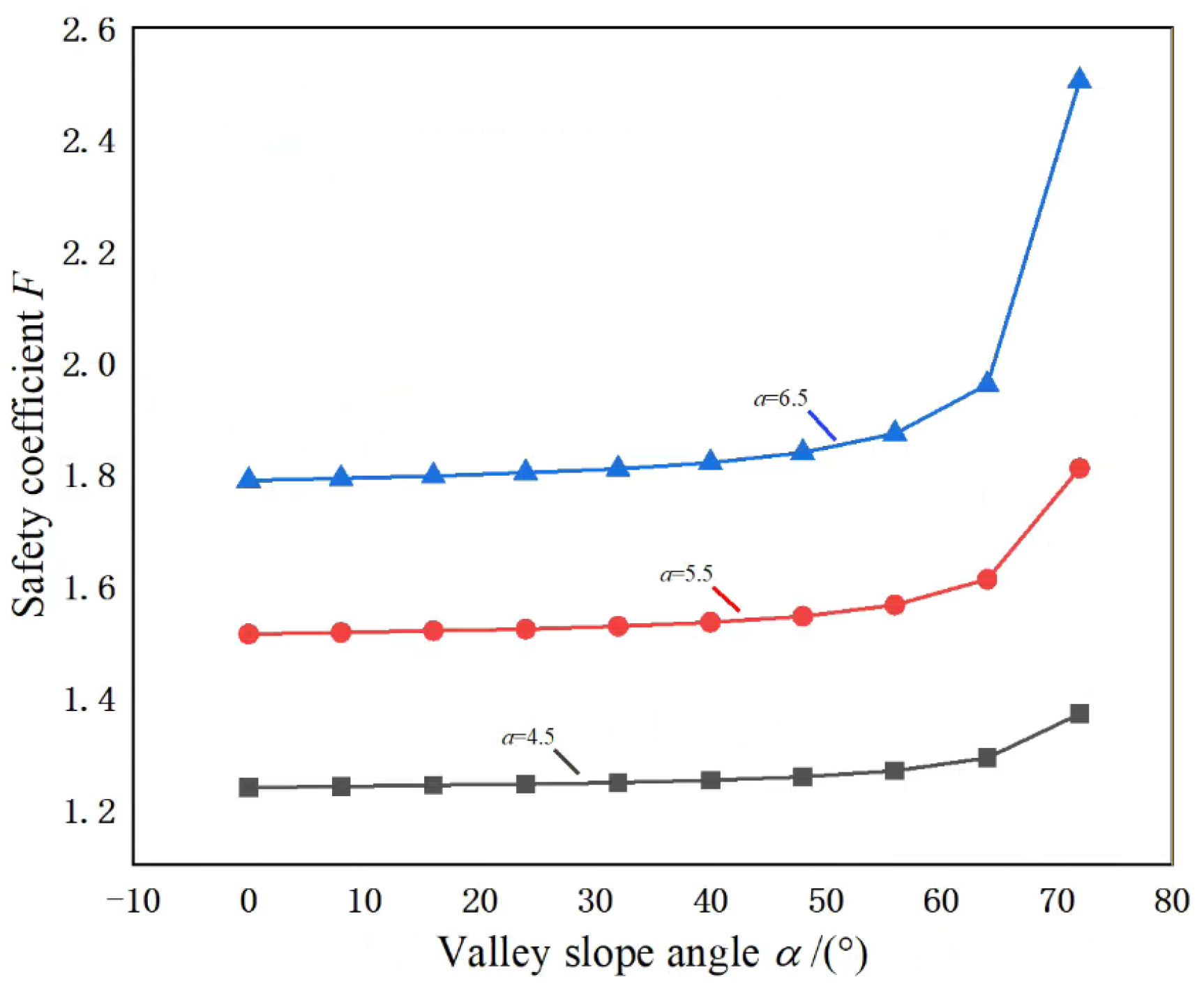
4.2. Influence Analysis of Valley Terrain Parameters
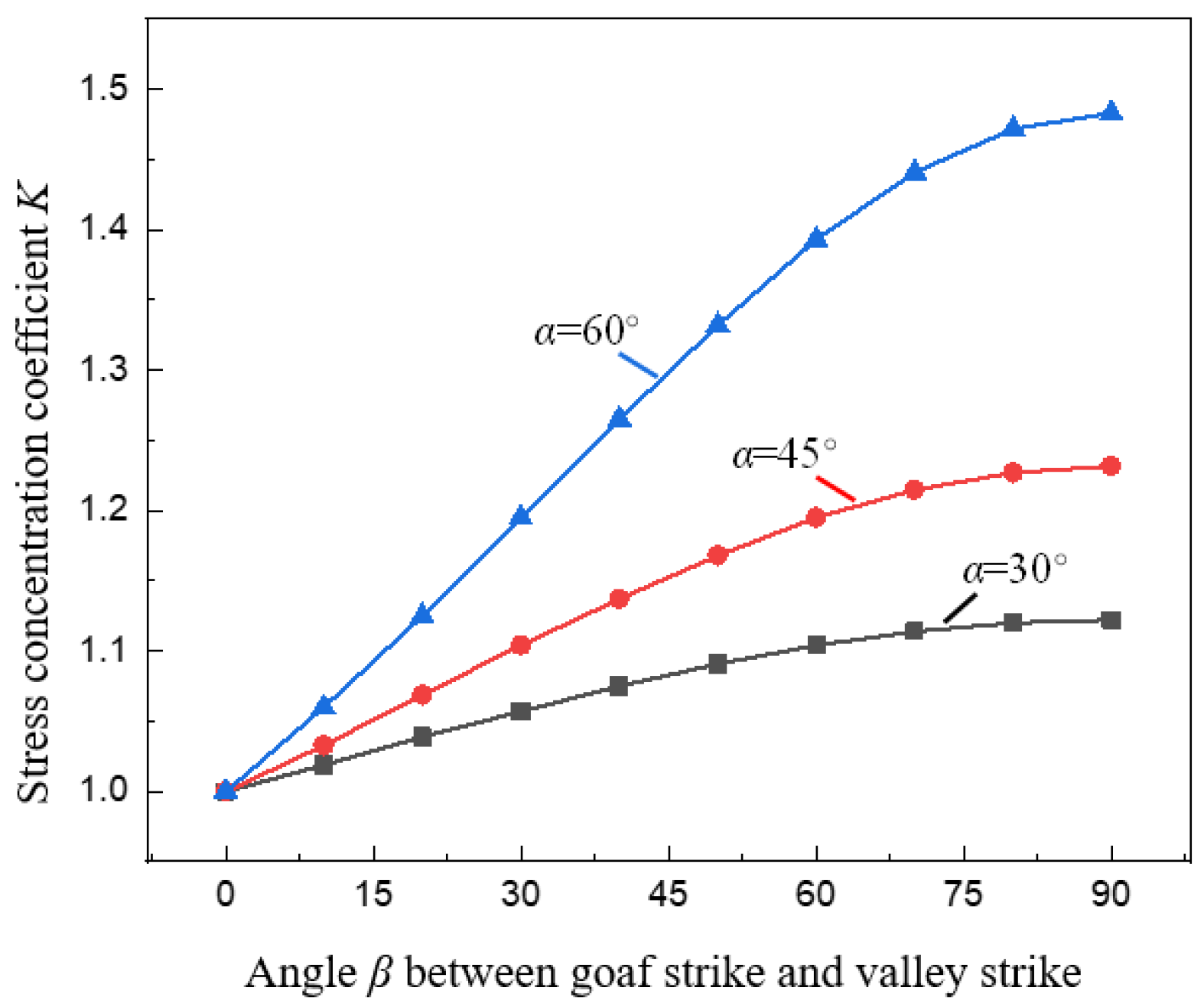
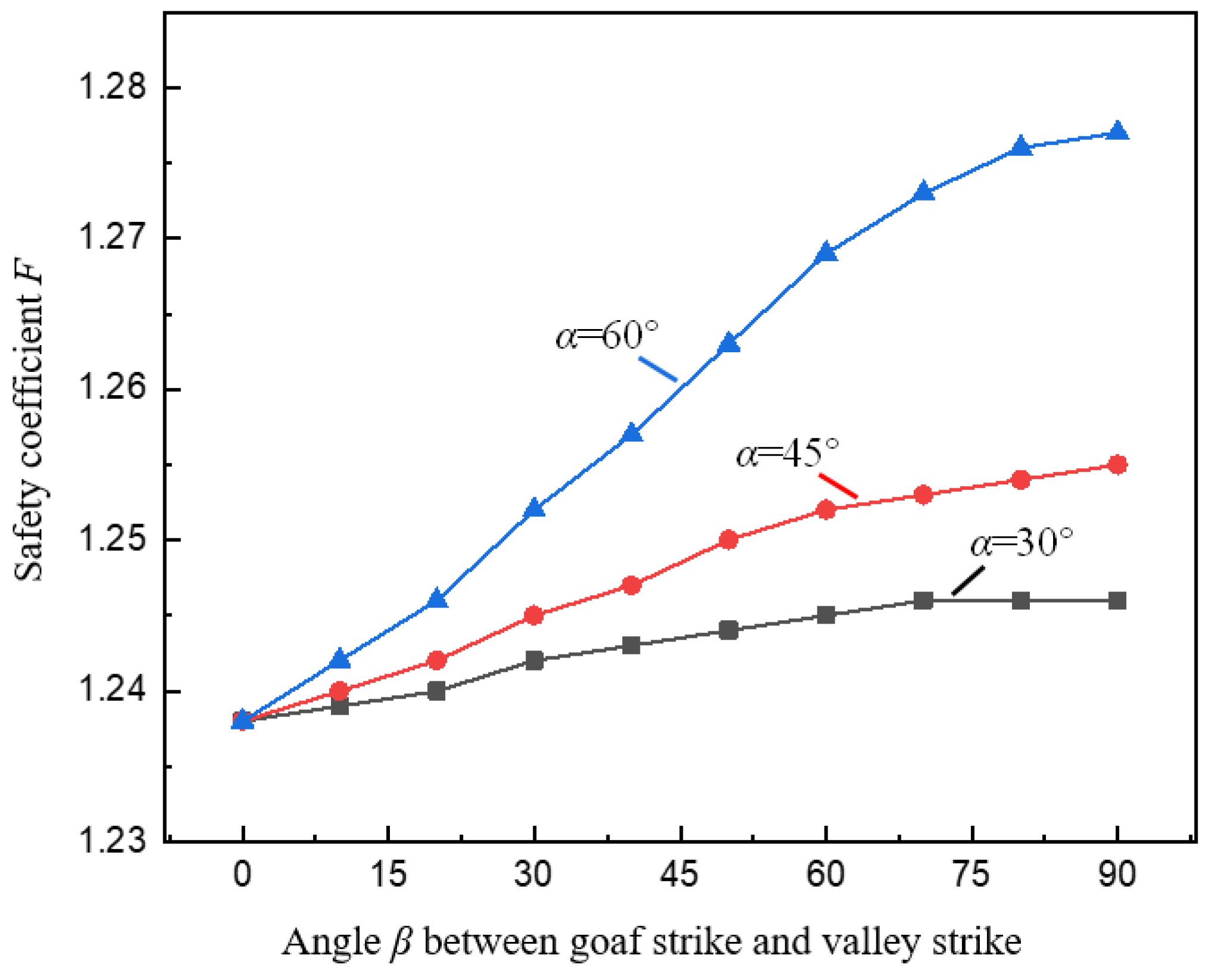
4.3. Influence Analysis of the Goaf Strike
5. Engineering Verification
6. Conclusions
- (1)
- The overlying valley terrain in the sedimentary bauxite mining area causes stress concentration in the pillar, reduces the effective bearing capacity, and reduces the stability of the pillar. The influence of the surface valley terrain should be considered when studying the stability of the pillar;
- (2)
- The analysis of the influence of the structural parameters of the goaf shows that, due to the stress concentration caused by the valley terrain, the mine pillar is more likely to induce instability when the span b of the goaf rise; the more significant the stress concentration effect is, the more likely the instability phenomenon will occur. The pillar is more prone to instability when the pillar height h increases, but the stress concentration effect is less resistant to the pillar instability caused by the increase of h;
- (3)
- The analysis of the influence of the valley terrain parameters shows that with the increase of the burial depth D of the pillar, the stability of the pillar is weakened, and the influence of the valley terrain gradually decreases; The more significant the valley slope angle α, the greater the stress concentration effect of the pillar, the more significant the safety coefficient F of the pillar under the same burial depth, and the greater the influence of α on F;
- (4)
- The analysis of the influence of the goaf strike shows that, compared with when the angle between the goaf strike and the valley strike is β > 50°, when β < 50°, the result of β on the stress concentration and safety coefficient of the pillar is greater, and the result is gradually weakened when β is larger. In addition, the steeper the slope of the valley, the more significant the influence of the goaf strike on the stability of the pillar under the valley terrain;
- (5)
- The safety coefficient analysis results show that the safety coefficient of the pillar at the bottom of the valley on the 6# exploration line of a bauxite mine in Shanxi has too much margin, while the safety coefficient of the pillar at the top of the valley is close to the critical value, and the pillar is on the edge of instability, the field investigation results verify the reliability of the quantitative assessment method of pillar stability in this paper.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, L.; Li, J.H.; Wang, D.H.; Xiong, X.Y.; Yi, C.W.; Han, M.Z. Outline of Metallogenic Regularity of Bauxite Deposits in China. Acta Geol. Sin. Engl. Ed. 2015, 89, 2072–2084. [Google Scholar] [CrossRef]
- Jiang, L.C.; Yang, C.; Jiao, H.Z. Ultimately exposed roof area prediction of bauxite deposit goaf based on macro joint damage. Int. J. Min. Sci. Technol. 2020, 30, 699–704. [Google Scholar] [CrossRef]
- Kariminejad, N.; Hosseinalizadeh, M.; Pourghasemi, H.R.; Tiefenbacher, J.P. Change detection in piping, gully head forms, and mechanisms. Catena 2021, 206, 105550. [Google Scholar] [CrossRef]
- Li, D.; Li, Y.; Masoumi, H.; Chen, J. An analytical model for the load-displacement behaviour of rock bolts based on continuously yielding criterion. Tunn. Undergr. Space Technol. 2021, 113, 103955. [Google Scholar] [CrossRef]
- Garza-Cruz, T.; Pierce, M.; Board, M. Effect of shear stresses on pillar stability: A back analysis of the troy mine experience to predict pillar performance at Montanore Mine. Rock Mech. Rock Eng. 2019, 52, 4979–4996. [Google Scholar] [CrossRef]
- Gao, T.; Sun, W.; Liu, Z.; Cheng, H.Y. Investigation on fracture characteristics and failure pattern of inclined layered cemented tailings backfill. Constr. Build. Mater. 2022, 343, 128110. [Google Scholar] [CrossRef]
- Jiang, L.C.; Jiao, H.Z.; Wang, Y.D.; Wang, G.G. Comprehensive safety coefficient of roof in goaf underdeep high stress. J. Central South Univ. 2021, 28, 595–603. [Google Scholar] [CrossRef]
- Li, D.; Shirani Faradonbeh, R.; Lv, A.; Wang, X.; Roshan, H. A data-driven field-scale approach to estimate the permeability of fractured rocks. Int. J. Min. Reclam. Environ. 2022. [Google Scholar] [CrossRef]
- He, Q.; Zhu, L.; Li, Y.; Li, D.; Zhang, B. Simulating Hydraulic Fracture Re-orientation in Heterogeneous Rocks with an Improved Discrete Element Method. Rock Mech. Rock Eng. 2021, 54, 2859–2879. [Google Scholar] [CrossRef]
- González-Nicieza, C.; Menéndez-Díaz, A.; Álvarez-Vigil, A.E.; Álvarez-Fernández, M.I. Analysis of support by hydraulic props in a longwall working. Int. J. Coal Geol. 2008, 74, 67–92. [Google Scholar] [CrossRef]
- Esterhuizen, G.S.; Dolinar, D.R.; Ellenberger, J.L. Pillar strength in underground stone mines in the United States. Int. J. Rock Mech. Min. Sci. 2011, 48, 42–50. [Google Scholar] [CrossRef]
- Zhao, G.Y.; Zhou, L.; Li, J.Y.; Yu, P.P. Reasonable pillar size design and nugget structural parameters optimization in room-and-pillar mining. J. Cent. South Univ. 2014, 45, 3943–3948. [Google Scholar]
- Zhao, K.; Yan, H.B.; Feng, X.; Wang, X.J.; Zhang, J.P.; Zhao, K. Stability analysis of pillar based on energy law. J. Theor. App. Mech. Pol. 2016, 48, 976–983. [Google Scholar] [CrossRef]
- Luo, B.Y.; Ye, Y.C.; Hu, N.Y.; Li, P.C.; Chen, C.Z. Mohr’s circle analysis and numerical simulation of stress state of pillar under combined compression-shear loading. Rock Soil Mech. 2020, 41, 63–73. [Google Scholar] [CrossRef]
- Li, J.W.; Li, X.T.; Liu, C.Y.; Wu, X.Y. Dynamic changes in surface damage induced by high-intensity mining of shallow, thick coal seams in gully areas. Adv. Civ. Eng. 2020, 2020, 5151246. [Google Scholar] [CrossRef]
- Li, J.W.; Liu, C.Y.; Wang, W.C.; Wang, C.Y. Linkage-induced mechanism and control technology of pressure bump and surface geological damage in shallow coal seam mining of gully area. Arab. J. Geosci. 2019, 12, 349. [Google Scholar] [CrossRef]
- Wang, X.F.; Wang, Y.; Zhang, D.S.; Wang, H.Z.; Zhang, Y.; Qin, D.D.; Zhang, C.G. Characteristics of strata behavior during thick seam mining by fully-mechanized top coal caving in a loess-covered gullied region. Minerals 2017, 7, 63. [Google Scholar] [CrossRef]
- Wang, Z.Q.; Li, J.K.; Wu, C.; Lv, W.Y.; Zhang, J.; Wang, P.; Li, Z.; Qu, H.Z. Study on Influence Laws of Strata Behaviors for Shallow Coal Seam Mining beneath Gully Terrain. Shock Vib. 2021, 2021, 3954659. [Google Scholar] [CrossRef]
- Kumar, R.; Das, A.J.; Mandal, P.K.; Bhattacharjee, R.; Tewari, S. Probabilistic stability analysis of failed and stable cases of coal pillars. Int. J. Rock Mech. Min. 2021, 144, 104810. [Google Scholar] [CrossRef]
- Jiang, S.Y.; Fan, G.W.; Li, Q.Z.; Zhang, S.Z.; Chen, L. Effect of mining parameters on surface deformation and coal pillar stability under customized shortwall mining of deep extra-thick coal seams. Energy Rep. 2021, 7, 2138–2154. [Google Scholar] [CrossRef]
- Guo, W.J.; Wang, H.L.; Chen, S.J. Coal pillar safety and surface deformation characteristics of wide strip pillar mining in deep mine. Arab. J. Geosci. 2016, 9, 137. [Google Scholar] [CrossRef]
- Wagner, H.; Ladinig, T.; Blaha, H. Design considerations for pillar systems in deep mines. Geomech. Tunn. 2016, 9, 524–528. [Google Scholar] [CrossRef]
- Zhu, D.F.; Song, X.M.; Li, H.C.; Liu, Z.H.; Wang, C.; Huo, Y.M. Cooperative load-bearing characteristics of a pillar group and a gob pile in partially caved areas at shallow depth. Energy Sci. Eng. 2020, 8, 89–103. [Google Scholar] [CrossRef]
- Sherizadeh, T.; Kulatilake, P.H. Assessment of roof stability in a room and pillar coal mine in the U.S. using three-dimensional distinct element method. Tunn. Undergr. Space Technol. 2016, 59, 24–37. [Google Scholar] [CrossRef]
- Jiao, H.Z.; Chen, W.L.; Wu, A.X.; Yu, Y.; Ruan, Z.E.; Rick, H.; Chen, X.M.; Yu, J.X. Flocculated unclassified tailings settling efficiency improvement by particle collision optimization in the feedwell. Int. J. Miner. Metall. Mater. 2021. [Google Scholar] [CrossRef]
- Jiang, L.C.; Xie, B. Analysis on Catastrophic Sliding of Pillar-Roof Supporting System in a Sedimentary Bauxite Goaf Mine. J. Northeast. Univ. 2020, 41, 1767–1774. [Google Scholar] [CrossRef]
- Jiang, L.C.; Su, Y. Theoretical model and application of critical blasting vibration velocity for instability of cemented backfill pillar. Chin. J. Nonferrous Met. 2019, 29, 2663–2670. [Google Scholar] [CrossRef]
- Esterhuizen, G.S.; Gearhart, D.F.; Klemetti, T.; Dougherty, H.; van Dyke, M. Analysis of gateroad stability at two longwall mines based on field monitoring results and numerical model analysis. Int. J. Min. Sci. Techno. 2019, 29, 35–43. [Google Scholar] [CrossRef]
- Jiao, H.Z.; Wu, Y.C.; Wang, H.; Chen, X.M.; Li, Z.; Wang, Y.F.; Zhang, B.Y.; Liu, J.H. Micro-scale mechanism of sealed water seepage and thickening from tailings bed in rake shearing thickener. Miner. Eng. 2021, 173, 107043. [Google Scholar] [CrossRef]
- Rafiei, R.H.; Martin, C.D. Slope stability analysis using equivalent Mohr-Coulomb and Hoek-Brown criteria. Rock Mech. Rock Eng. 2020, 53, 13–21. [Google Scholar] [CrossRef]
- Wilson, A.H. The stability of underground workings in the soft rocks of the coal measures. Int. J. Min. Eng. 1983, 1, 91–187. [Google Scholar] [CrossRef]
- Zhu, D.F.; Tu, S.H.; Tu, H.S.; Li, X.Y.; Liang, N.N. Analysis of stress propagation mechanism in the interval coal pillars under the gully landform. J. Min. Saf. Eng. 2018, 35, 701–707. [Google Scholar] [CrossRef]
- Zhang, M.; He, H.; Zhang, Y.; Jin, X.; Liang, X.Y.; Zhang, Y.D. Research on the Deformation and Control Technology of Surrounding Rock in Entry Retaining along the Gob Side. Adv. Civ. Eng. 2020, 2020, 8885439. [Google Scholar] [CrossRef]
- Xu, X.H.; He, F.L.; Li, X.B.; He, W.R. Research on mechanism and control of asymmetric deformation of gob side coal roadway with fully mechanized caving mining. Eng. Fail. Anal. 2021, 120, 105097. [Google Scholar] [CrossRef]
- Sun, W.; Zhang, Q.; Luan, Y.Z.; Zhang, X.P. A study of surface subsidence and coal pillar safety for strip mining in a deep mine. Environ. Earth Sci. 2018, 77, 627. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, B.; Wang, L.; Liu, B.H.; Li, S.C.; Gao, H.K.; Wang, Y. Control mechanism of roof fracture in no-pillar roadways automatically formed by roof cutting and pressure releasing. Arab. J. Geosci. 2020, 13, 274. [Google Scholar] [CrossRef]
- Jiang, L.C.; Wang, Y.D. Comprehensive safety coefficient of residual mining pillar under complex loads. J. Cent. South Univ. 2018, 49, 1511–1518. [Google Scholar] [CrossRef]
- Zhang, K.; Wu, F.F.; Yue, X. Study on Reasonable Chain Pillar Size in a Thick Coal Seam. Geofluids 2022, 2022, 7290894. [Google Scholar] [CrossRef]
- Ghasemi, E.; Shahriar, K. A new coal pillars design method in order to enhance safety of the retreat mining in room and pillar mines. Saf. Sci. 2012, 50, 579–585. [Google Scholar] [CrossRef]
- Yang, T.; Yang, Y.R.; Zhang, J.; Gao, S.S.; Li, T. Study on Development Law of Mining-Induced Slope Fracture in Gully Mining Area. Adv. Civ. Eng. 2021, 2021, 9990465. [Google Scholar] [CrossRef]
- Liu, Y.J.; Qi, Q.J.; Wang, A.H. Influence of valleys terrain on pressure of fully mechanized working faces in shallow coal seams. Shock Vib. 2021, 2021, 8880041. [Google Scholar] [CrossRef]
- Wu, A.X.; Ruan, Z.E.; Wang, J.D. Rheological behavior of paste in metal mines. Int. J. Miner. Metall. Mater. 2022, 29, 717–726. [Google Scholar] [CrossRef]
- Zhang, J.X.; Huang, P.; Zhang, Q.; Li, M.; Chen, Z.W. Stability and control of room mining coal pillars-taking room mining coal pillars of solid backfill recovery as an example. J. Cent. South Univ. 2017, 24, 1121–1132. [Google Scholar] [CrossRef]
- Zhu, W.B.; Chen, L.; Zhou, Z.L.; Shen, B.T.; Xu, Y. Failure propagation of pillars and roof in a room and pillar mine induced by longwall mining in the lower seam. Rock Mech. Rock Eng. 2019, 52, 1193–1209. [Google Scholar] [CrossRef]
- Jiang, B.Y.; Wang, L.G.; Lu, Y.L.; Sun, X.K.; Jin, G. Ground pressure and overlying strata structure for a repeated mining face of residual coal after room and pillar mining. Int. J. Min. Sci. Techno. 2016, 26, 645–652. [Google Scholar] [CrossRef]










Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, L.; Jiao, H.; Xie, B.; Yang, H. Study on Safety Coefficient of Sedimentary Bauxite Strip Pillar under Valley Terrain. Int. J. Environ. Res. Public Health 2022, 19, 10991. https://doi.org/10.3390/ijerph191710991
Jiang L, Jiao H, Xie B, Yang H. Study on Safety Coefficient of Sedimentary Bauxite Strip Pillar under Valley Terrain. International Journal of Environmental Research and Public Health. 2022; 19(17):10991. https://doi.org/10.3390/ijerph191710991
Chicago/Turabian StyleJiang, Lichun, Huazhe Jiao, Bo Xie, and Han Yang. 2022. "Study on Safety Coefficient of Sedimentary Bauxite Strip Pillar under Valley Terrain" International Journal of Environmental Research and Public Health 19, no. 17: 10991. https://doi.org/10.3390/ijerph191710991
APA StyleJiang, L., Jiao, H., Xie, B., & Yang, H. (2022). Study on Safety Coefficient of Sedimentary Bauxite Strip Pillar under Valley Terrain. International Journal of Environmental Research and Public Health, 19(17), 10991. https://doi.org/10.3390/ijerph191710991







