A Quantitative Analysis of Risk Premia in the Corporate Bond Market
Abstract
1. Introduction
2. Two Components of Corporate Bonds Excess Return: The Model
2.1. Price of a Credit Default Swap
2.2. Distress Risk Premium
2.3. Jump-at-Default Risk Premium
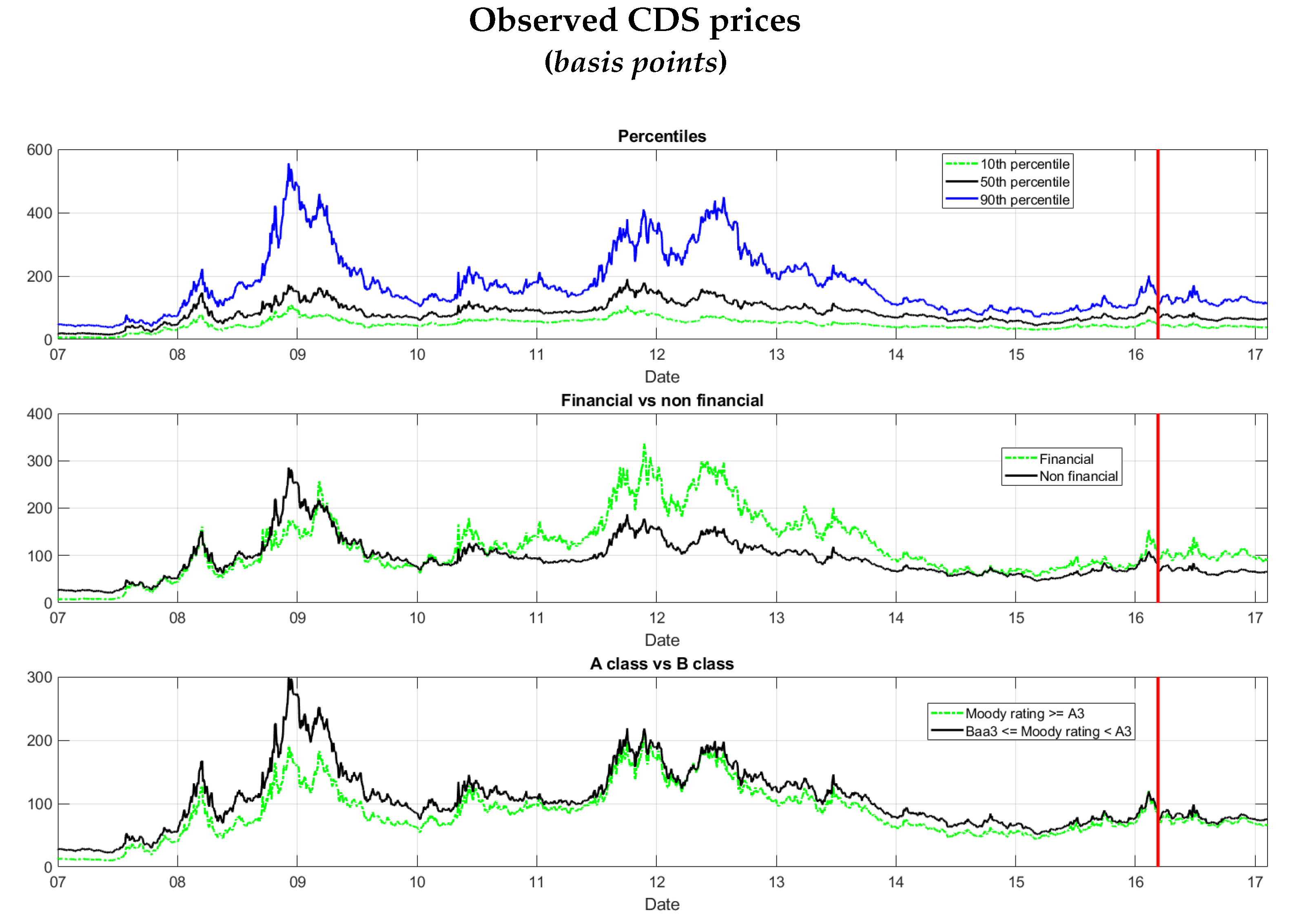
3. Data and Econometric Framework
- We get the maximum likelihood (ML) estimates of the risk-neutral mean of default arrival rates from the CDS spreads. Assuming that the default event is diversifiable, we get an estimate of the distress risk premium.
- We estimate the ML actual default intensity using information on the actual probabilities of default embedded in Moody’s KMV EDFs.22 We thus compute the jump-at-default risk premium in terms of the ratio , as shown by Driessen (2005); Pan and Singleton (2006).
3.1. Maximum Likelihood Estimates of Risk-Neutral Default Intensity
3.2. Maximum Likelihood Estimates of Actual Default Intensity
4. Estimates of the Two Risk Premia Components
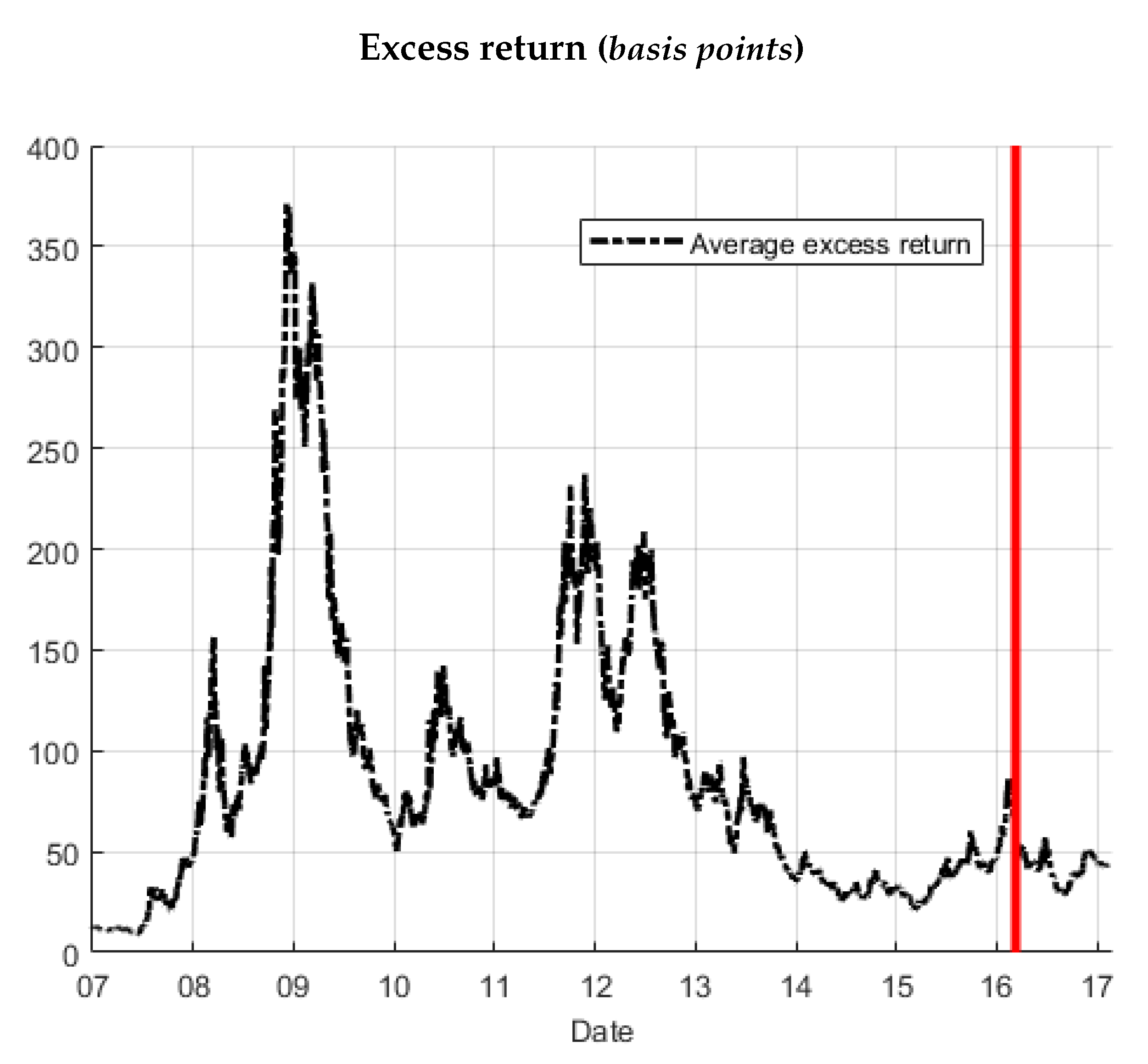
4.1. Estimation Results of the Distress Risk Premium
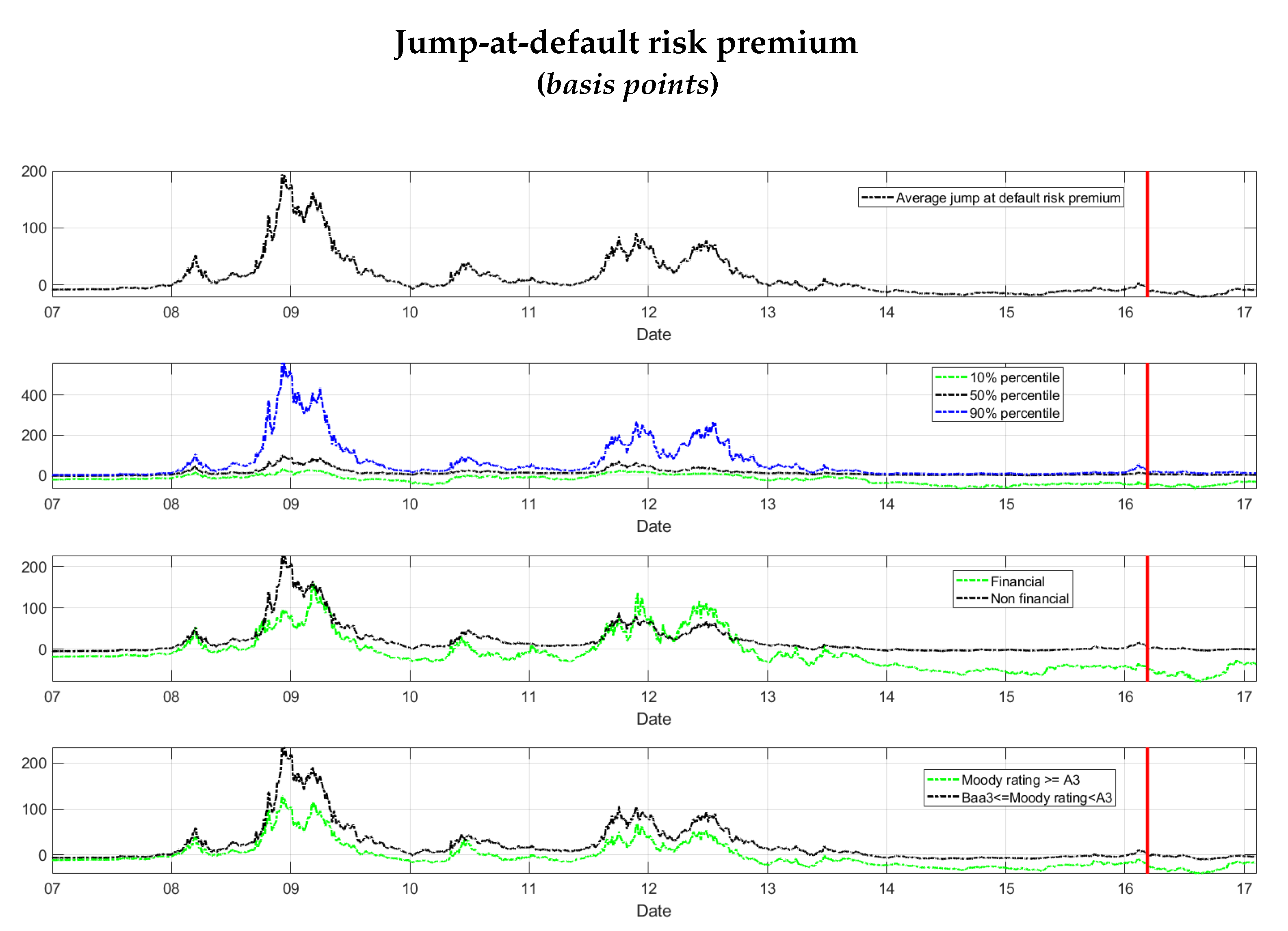
4.2. Estimation Results of the Jump-at-Default Risk Premium
5. Analysis of the Two Risk Premium Components and Event Study Exercise on the Risk Premia and the Expected Losses
- The (averaged) median distress risk premium is higher for financial firms (around 69 basis points, versus 49 basis points of the non financial counterparties);
- On the contrary, the (averaged) median jump-at-default risk premium is higher for non-financial firms (being around 4 basis points, versus a negative value of the financial counterparties);
- There is a greater heterogeneity among financial firms compared to non-financial counterparties, as both the dispersion in the distress risk premium and in the jump-at-default risk premium are higher for financial firms: in particular the average standard deviation of the distress risk premium is around 30 basis points for financial firms, compared to 22 for non-financial firms; the average standard deviation of the jump-at-default risk premium is around 73 basis points, compared to 19 for non-financial firms.
- 10 March 2016: the ECB adds corporate sector purchase programme (CSPP) to the asset purchase programme (APP);29
- 21 April 2016: Press Release: the ECB announces details of the corporate sector purchase programme (CSPP), mainly on the eligibility of bonds;30
- 2 June 2016: the ECB communicates the remaining details of the corporate sector purchase programme (CSPP) on the issuer’s eligibility.31
6. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Decomposition of the Excess Return into Two Components
Appendix A.2. Ito’s Lemma for a Process with Drift, Diffusion and Poisson Jumps
Appendix A.3. Ornstein Uhlenbeck Dynamics for the Risk-Neutral Default Intensity under the Objective Probability Measure
Appendix A.4. CIR Dynamics for the Risk-Neutral Default Intensity under the Objective Measure
Appendix A.5. Explicit Solution for the CDS Price in the CIR Model
Appendix A.6. PDE Associated with the Expectation and Crank Nicholson Scheme
Appendix A.7. Price of Jump-at-Default Risk
References
- Berndt, Antje, Rohan Douglas, Darrell Duffie, Mark Ferguson, and David Schranz. 2005. Measuring Default Risk Premia from Default Swap Rates and EDFs. Working Paper No. 173. Basel: BIS, March. [Google Scholar]
- Berndt, Antje, and Iulian Obreja. 2010. Decomposing European CDS returns. Review of Finance 14: 189–233. [Google Scholar] [CrossRef]
- Bharath, Sreedhar T., and Tyler Shumway. 2008. Forecasting Default with the Merton Distance to Default Model. The Review of Financial Studies 21: 1339–69. [Google Scholar] [CrossRef]
- Blanco, Roberto, Simon Brennan, and Ian W. Marsh. 2005. An empirical analysis of the dynamic relation between investment-grade bonds and credit defualt swaps. The Journal of Finance 60: 2255–81. [Google Scholar] [CrossRef]
- Cecchetti, Sara, and Marco Taboga. 2017. Assessing the Risks of Asset Overvaluation: Models and Challenges. Working Papers, No. 1114. Rome: Bank of Italy Temi di Discussione, April. [Google Scholar]
- Chen, Ren-Raw, and Louis Scott. 1993. Maximum likelihood estimation for a multifactor equilibrium model of the term structure of interest rates. The Journal of Fixed Income 3: 14–31. [Google Scholar] [CrossRef]
- Cheridito, Patrick, Damir Filipović, and Robert L. Kimmel. 2007. Market price of risk specifications for affine models: Theory and evidence. Journal of Financial Economics 83: 123–70. [Google Scholar] [CrossRef]
- Cox, John C., Jonathan E. Ingersoll, Jr., and Stephen A. Ross. 1985. A Theory of the Term Structure of Interest Rates. Econometrica 53: 385–407. [Google Scholar] [CrossRef]
- Cont, Rama, and Peter Tankov. 2004. Financial Modelling with Jump Processes. Financial Mathematics Series; Boca Raton: Chapman and Hall/CRC. [Google Scholar]
- Diaz, Antonio, Jonatan Groba, and Pedro Serrano. 2013. What drives corporate default risk premia? Evidence from the CDS market. Journal of International Money and Finance 37: 529–63. [Google Scholar] [CrossRef]
- Driessen, Joost. 2005. Is Default Event Risk Priced in Corporate Bonds? The Review of Financial Studies 18: 165–95. [Google Scholar] [CrossRef]
- Duffee, Gregory R. 2002. Term Premia and Interest Rate Forecasts in Affine Models. Journal of Finance 57: 405–43. [Google Scholar] [CrossRef]
- Duffie, Darrell, and Kenneth J. Singleton. 1999. Modeling Term Structures of Defaultable Bond Yields. The Review of Financial Studies 12: 687–720. [Google Scholar] [CrossRef]
- Duffie, Darrell, and Kenneth J. Singleton. 2003. Credit Risk: Pricing, Measurement and Management. Princeton: Princeton Series in Finance. [Google Scholar]
- European Central Bank. 2016. ECB Economic Bulletin. Issue 5/2016, Box 2. Available online: https://www.ecb.europa.eu/pub/pdf/ecbu/eb201605.en.pdf (accessed on 4 August 2016).
- Forte, Santiago, and Pena Juan Ignacio. 2009. Credit spreads: An empirical analysis on the informational content of stocks, bonds, and CDS. Journal of Banking & Finance 33: 2013–25. [Google Scholar]
- Gilchrist, S., and Zakrajsek Egon. 2012. Credit Spreads and Business Cycle Fluctuations. The American Economic Review 102: 1692–720. [Google Scholar] [CrossRef]
- Hamilton, James D. 1994. Time Series Analysis. Princeton: Princeton University Press. [Google Scholar]
- Hamilton, James D., and Wu Jing Cynthia. 2012. Identification and Estimation of Gaussian Affine Term Structure Models. Journal of Econometrics 168: 315–31. [Google Scholar] [CrossRef]
- Ishii, Hokuto. 2019. Forecasting Term Structure of Interest Rates in Japan. International Journal of Financial Studies 7: 39. [Google Scholar] [CrossRef]
- Jarrow, Robert A., David Lando, and Fan Yu. 2005. Default risk and diversification: Theory and empirical implications. Mathematical Finance 15: 1–26. [Google Scholar] [CrossRef]
- Kealhofer, Stephan. 2003. Quantifying credit risk I: Default prediction. Financial Analyst Journal 59: 30–44. [Google Scholar] [CrossRef]
- Kelly, Bryan, Hanno Lustig, and Stijn Van Nieuwerburgh. 2016. Too-Systemic-To-Fail: What Option Markets Imply About Sector-wide Government Guarantees. American Economic Review 106: 1278–319. [Google Scholar] [CrossRef]
- Korablev, Irina, and Douglas Dwyer. 2007. Power and Level Validation of Moody’s KMV EDFTM Credit Measures in North America, Europe and Asia. Moody’s KMV Technical Report 070930. New York: Moody’s KMV Company, pp. 17–18. [Google Scholar]
- Lando, David. 1998. On Cox Processes and Credit Risky Securities. Review of Derivative Research 2: 99–120. [Google Scholar] [CrossRef]
- Lando, David. 2004. Credit Risk Modeling. Princeton: Princeton Series in Finance. [Google Scholar]
- Li, Junye, and Gabriele Zinna. 2015. On Bank Credit Risk: Systemic or Bank-Specific? Evidence from the US and UK. Journal of Financial and Quantitative Analysis 49: 1403–42. [Google Scholar] [CrossRef]
- Longstaff, Francis A., Sanjay Mithal, and Eric Neis. 2005. Corporate yields spreads: Default risk or liquidity? New evidence from the the credit default swap market. The Journal of Finance 60: 2213–53. [Google Scholar] [CrossRef]
- Longstaff, Francis A., Jun Pan, Lasse H. Pedersen, and Kenneth J. Singleton. 2011. How Sovereign is Sovereign Credit Risk? American Economic Journal: Macroeconomics 3: 75–103. [Google Scholar] [CrossRef]
- Merton, Robert C. 1974. On the pricing of corporate debt: The risk structure of interest rates. The Journal of Finance 29: 449–70. [Google Scholar]
- Pan, Jun, and Kenneth J. Singleton. 2006. Interpreting Recent Changes in the Credit Spreads of Japanese Banks. Tokyo: Monetary and Economic Studies, Institute for Monetary and Economic Studies, Bank of Japan, vol. 24, pp. 129–41. [Google Scholar]
- Pan, Jun, and Kenneth J. Singleton. 2008. Default and recovery implicit in the term structure of sovereign CDS spread. The Journal of Finance 63: 2345–84. [Google Scholar] [CrossRef]
- Schonbucher, Philipp J. 2003. Credit Derivatives Pricing Models. Hoboken: Wiley Finance Series. [Google Scholar]
- Shreve, Steven E. 2008. Stochastic Calculus for Finance II. Continuous-Time Models. New York: Springer. [Google Scholar]
- Vassalou, Maria, and Yuhang Xing. 2004. Default risk in equity returns. The Journal of Finance 59: 831–68. [Google Scholar] [CrossRef]
- Vasicek, Oldrich. 1977. An equilibrium characterization of the term structure. Journal of Financial Economics 5: 177–88. [Google Scholar] [CrossRef]
- Yu, Fan. 2002. Modeling Expected Return on Defaultable Bonds. Journal of Fixed Income 12: 69–81. [Google Scholar] [CrossRef]
| 1. | For a discussion of the decomposition of corporate bond spreads in the expected losses and the risk premium components see, for example, Cecchetti and Taboga (2017). |
| 2. | In Berndt and Obreja (2010) the payments of a CDS can be reproduced by a replicating portfolio consisting of a long position in a defaultable bond and a short position in a risk-free bond. |
| 3. | |
| 4. | Meaning that price variations of CDSs anticipate variations in bond spreads. This evidence is consistent with the standard hypothesis whereby CDS prices adjust more rapidly to the release of new information and that adjustment, in turn, generates an informative signal to which bond spreads react with a time lag. |
| 5. | The same decomposition is employed by Pan and Singleton (2006) for the credit spreads of Japanese banks. |
| 6. | As in Pan and Singleton (2008) for sovereign CDS spreads. |
| 7. | This credit-risk literature can be related to the term structure of interest rate models where intuitively, survival probabilities take similar expressions to bond prices, with the default intensity replacing the short rate; in other words, risk-neutral (objective) bond prices relate to risk-neutral (objective) survival probabilities. A seminal paper in the literature of the term structure of interest rates is Vasicek (1977); recently Ishii (2019) examines and compares different models to forecast the term structure of interest rates. |
| 8. | For the dynamics of , we also studied a different model (starting from Li and Zinna (2015); Cheridito et al. (2007)) from the one used in those papers, but we found that this new model did not perform as well as the first: for this reason we continued our analysis using the consolidated model. |
| 9. | In the CSPP the object of the purchases are the investment grade bonds with residual life between 1 and 30 years, issued by non-financial firms or non-banking financial firms. The purchases can be made both in the primary and the secondary markets. |
| 10. | A completely different approach that also analysis the excess bond premium is in Gilchrist and Zakrajsek (2012). |
| 11. | Discounting by captures the survival-dependent nature of the payments. |
| 12. | These strong technical assumptions are standard in the litarature, and necessary to construct the discrete approximation for the solution of the CDS price introduced in Lando (2004), which we’ll see in Section 2.2. |
| 13. | See the Introduction for references and a brief explanation. |
| 14. | Technical proof can be found in Appendix A.3. |
| 15. | See Cox et al. (1985). |
| 16. | According to the authors, the motivation for this choice is to avoid to impose the Feller condition required by the CIR dynamcis to assure a strictly positive: . Such choice of market price of risk determines only one different parameter in the processes under the actual and the risk-neutral measure. |
| 17. | Technical proof can be found in Appendix A.4. |
| 18. | See Appendix A.6 for details of this method. |
| 19. | |
| 20. | See Appendix A.7 for a technical explanation. |
| 21. | Empirical studies corroborate the accuracy of EDFs for predicting default (see Kealhofer (2003); Bharath and Shumway (2008); Korablev and Dwyer (2007)) and using EDFs to determine the default premium has been also referred to in Berndt et al. (2005) for US corporate CDS spreads, Vassalou and Xing (2004) for stock prices and Pan and Singleton (2006) for CDS spreads of Japanese banks. |
| 22. | |
| 23. | See also Hamilton and Wu (2012) for a description of the method. |
| 24. | The same hypothesis is in Longstaff et al. (2011); Diaz et al. (2013), but is not related to different liquidity consitions of CDS contracts with different maturities. |
| 25. | We can consider this dynamics as the continuous time version of the corresponding AR(1) process, or
|
| 26. | A non-central distribution, defined in terms of the modified Bessel function of first kind, is instead involved in case of a CIR dynamics for (see Chen and Scott (1993)). |
| 27. | |
| 28. | Of course we expect that the main impact on the components of CDS prices arises at the date of the first announcement of the CSPP (as in fact is confirmed by the results), but we also consider the dates of the other two press releases in wich the ECB provides details of the programme. |
| 29. | |
| 30. | |
| 31. | |
| 32. | As often employed in the literature for event study exercises, see for instance Kelly et al. (2016). |
| 33. | Technical details can be also found in Cont and Tankov (2004). |
| 34. | Terms come from Ito’s lemma for a process with drift, diffusion and Poisson jumps (due to default) and Girsanov’s theorem; see Appendix A.2 for a brief overview and Cont and Tankov (2004) for details. |
| 35. | Technical details can be found in Cont and Tankov (2004). |
| 36. | could be a constant, a deterministic function of time, or a stochastic process. |
| 37. | |
| 38. |










| Mean | 0.3288 | −4.5333 | 1.1908 | 0.4314 | −6.6636 | 0.0016 | 0.0013 |
| Std | 0.0339 | 0.4705 | 0.0677 | 0.0047 | 0.0717 | 0.0016 | 0.0007 |
| Median | 0.3294 | −4.5036 | 1.2000 | 0.4304 | −6.6799 | 0.0012 | 0.0012 |
| Mean | 0.3035 | −12.5442 | 1.1360 | 0.0024 | 0.0012 |
| Std. | 0.0948 | 12.6319 | 0.1721 | 0.0046 | 0.0016 |
| Median | 0.3079 | −9.3997 | 1.1735 | 0.0011 | 0.0006 |
| −16.75 | −10.87 | −5.88 |
| 0.010 | 0.009 | 0.001 |
| −21.41 | −15.84 | −34.47 |
| −11.75 | −13.14 | −1.87 | −16.68 | −7.12 | −4.71 |
| −26.43 | −15.67 | −10.06 | −25.20 | −12.24 | −50.92 |
| 0.017 | 0.019 | −0.002 | 0.007 | 0.005 | 0.002 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cecchetti, S. A Quantitative Analysis of Risk Premia in the Corporate Bond Market. J. Risk Financial Manag. 2020, 13, 3. https://doi.org/10.3390/jrfm13010003
Cecchetti S. A Quantitative Analysis of Risk Premia in the Corporate Bond Market. Journal of Risk and Financial Management. 2020; 13(1):3. https://doi.org/10.3390/jrfm13010003
Chicago/Turabian StyleCecchetti, Sara. 2020. "A Quantitative Analysis of Risk Premia in the Corporate Bond Market" Journal of Risk and Financial Management 13, no. 1: 3. https://doi.org/10.3390/jrfm13010003
APA StyleCecchetti, S. (2020). A Quantitative Analysis of Risk Premia in the Corporate Bond Market. Journal of Risk and Financial Management, 13(1), 3. https://doi.org/10.3390/jrfm13010003





