Forecasting Commodity Prices Using the Term Structure

Abstract

The aim of this study is to test the ability of the yield curve on US government bonds to forecast the future evolution in the prices of commodities often used in as raw materials. We consider the monthly prices of nine commodities for more than 30 years. Our findings, confirmed by several parametric and non-parametric tests, are robust and indicate that the ability to forecast future performance changes over time. Specifically, between 1986 and the early 2000s the yield curve was quite successful in forecasting monthly changes in commodity prices, but that success diminished in the period following. One possible explanation for this outcome is the increased flow of capital into the commodity market resulting in stronger correlations with the equity markets and a breakdown of the obvious relationship between commodities and business cycle. Our findings are important for asset pricing, commodity traders and policy makers.

1. Introduction

The literature regards the term structure curve, which plots the yield of government bonds against their maturity, as an indicator with valuable information about the current and future states of the economy (e.g., Harvey 1989; Abdymomunov 2013; Gogas et al. 2015; McMillan 2021b). The U.S. Federal Reserve, among other policymakers and institutional market participants, has always looked at the difference between the yields on long- and short-term sovereign bonds as an indication of where the economy is heading. Thus, the forecasting ability of the yield spread has become something of a stylized fact among macroeconomists.

Many studies, detailed in the literature review section, have demonstrated the ability of yield spreads to predict future economic situations effectively. They have established that the spreads contain a great deal of information about future economic activity and are accurate predictors of economic growth. However, there have been fewer comprehensive attempts to understand the dynamic relationships between the evolution in the prices of commodities and the shape of the yield curve. This question has become particularly relevant in the wake of the unconventional monetary policy used in the last two decades, which has not been employed since the Great Depression during 1930s. Thus, our goal is to fill this gap by exploring the ability of the term structure to forecast the future evolution in the prices of commodities. To accomplish this task, we use 30 years of data about nine commodities often used as raw materials: coal, gold, silver, oil, platinum, palladium, zinc, ethanol, and natural gas. We also use various proxies for the yield spread combined from 30-year, 10-year, 2-year, 1-year and 3-month interest rates.

Our findings indicate that yield spreads are generally positively correlated with future changes in the price of commodities. Our results are robust to controlling for real economic and financial variables. Adding any or all of these potential alternative explanatory variables only marginally affects the coefficients or their statistical significance. In our analysis, we also utilize the Engle’s (2002) dynamic conditional correlation procedure (DCC). The overall picture confirms the existence of time-varying correlation between the yield spread and future price movements in commodities. To determine why this result emerged, we consider sub-samples that are determined endogenously using the Bai and Perron (2003) tests. These structural break tests confirm that the positive correlation is economically and statistically significant mainly for the period prior to 2004. On the other hand, in the period following (2004–2020) it seems that the yield spread has been unable to predict changes in commodities in any significant way.

The weakening correlation between the variables of interest may be related to the massive capital inflows from individual and institutional investors into the commodity market in the early 2000s (e.g., Tang and Xiong 2012). Many studies justified these inflows to commodities by their relatively low correlation with financial markets and, accordingly, the potential diversification benefits (e.g., Gorton and Rouwenhorst 2006; Daskalaki et al. 2017). However, the considerable inclusion of commodities in investors’ portfolios resulted in the financialization of commodities, yielding a strong correlation between commodity prices and equity markets (e.g., Hu et al. 2020) and a breakdown of the obvious relationship between commodities and cyclical phases of the economy.

Lastly, between 1986 and December 2020, we found eight periods during which the yield spread was negative or equal to zero. The non-parametric tests conducted to track the future evolution in commodity prices following flat or downward-sloped yield curves indicate that such situations can be a successful timing to embark on long positions in several commodities for investors planning to hold for a relatively long period of time. Recently, with the outbreak of the Coronavirus late in February 2020, the U.S. 1-year yield was 1.43%, and the 10-year was about flat (1.46%). Tracking the commodity prices in the few months following indicate significant shrink in prices. However, the prices recovered sharply after 2–4 quarters. This recent case, among the others observed, confirms that specific shapes of the yield curve may generate abnormal returns for investors (see

Table A1. Evolution in the commodity prices following the relatively flat curve witnessed in February 2020.

	Accumulated Returns after:
	1 Month	2 Months	3 Months	4 Months	5 Months	6 Months	7 Months	8 Months	9 Months	10 Months
Oil	−50.9%	−66.1%	−33.4%	−22.4%	−17.3%	−13.1%	−19.3%	−22.7%	−8.4%	−1.6%
Silver	−18.4%	−16.1%	−2.9%	−1.6%	25.4%	44.4%	26.5%	34.2%	28.4%	42.6%
Gold	−0.8%	4.7%	5.4%	7.0%	15.2%	16.1%	12.8%	15.5%	9.6%	14.5%
PLTNM	−20.0%	−17.0%	−4.9%	−13.9%	1.7%	0.2%	−9.7%	−6.4%	4.0%	11.9%
PLDM	−14.0%	−25.0%	−25.3%	−31.1%	−13.4%	−18.0%	−16.6%	−10.5%	−11.5%	−10.8%
Zinc	−9.4%	−7.1%	−1.6%	1.3%	9.9%	22.2%	16.9%	25.4%	35.9%	41.7%
Ethanol	−29.6%	−27.5%	−14.3%	−12.0%	−10.9%	−0.4%	1.6%	21.5%	6.2%	2.3%
Coal	−2.3%	−10.0%	−23.5%	−21.6%	−23.4%	−26.7%	−22.2%	−14.6%	−6.1%	17.8%
Nat.Gas	−10.2%	−5.5%	−6.3%	−19.8%	−2.1%	34.8%	15.8%	63.7%	56.8%	46.2%

Notes: With the outbreak of the COVID−19 pandemic in February 2020, the U.S. 1-year yield was 1.43% while the 10-year was 1.46%. That is, the yield spread was 0.03%. The table reports the evolution in the prices of commodities in the few months following this relatively flat curve. Though we discuss one case, the overall picture is clear and shows that the current yield spread is a relatively good predictor of the future evolution in commodity prices.

in the online Appendix A).

The mechanism underlying our conjecture here accords with the empirical evidence confirming a strong relationship between the business cycle and commodity prices (Labys et al. 1999; Chevallier et al. 2014). In addition, the literature has established that financial markets, including the yield curve, move more quickly than real markets (Saar and Yagil 2015). Accordingly, one should observe a causal relationship from this macro-financial predictor to the commodity market.

The paper contributes to the existing literature in several ways. First, our study sheds light on the link between yield spreads and long-term prices in the commodity market. While previous studies have focused on interest rates in level rather than the difference between long and short-term sovereign bond yields (e.g., Dai and Kang 2021), little is known about the information content of the term structure for commodities. Second, we add to the literature documenting the time-varying relationship between real economic and financial variables by examining the structural breaks in the long-term correlation between yield spreads and the commodity market (e.g., Chinn and Kucko 2015). Third, our findings are especially useful for policy makers and central banks because long-term predictions about commodity prices are essential in targeting inflation and promoting overall economic stability (e.g., Garner 1989; Orlowski 2017; Fasanya and Awodimila 2020). Finally, modeling and forecasting future innovations in commodity prices are important for both market participants and scholars. Predictions in this area play a vital role in portfolio optimization and risk management. Indeed, investors are attracted to commodities due to their inflation-hedging properties (e.g., Beckmann and Czudaj 2013; Bampinas and Panagiotidis 2015; Levine et al. 2018; Umar et al. 2019), and their possible contribution to diversifying risks (e.g., Gagnon et al. 2020).

The remainder of this study proceeds as follows. Section 2 reviews the literature. Section 3 describes the data and the construction of the key variables. Section 4 presents the methodology. Section 5 details the empirical findings and discusses the results. Section 6 checks the robustness of the findings, and Section 7 concludes.

2. Literature Review

The macroeconomic literature has established that future real economic growth is positively correlated with lagged interest spreads (e.g., Stock and Watson 1989; Estrella and Hardouvelis 1991; Plosser and Rouwenhorst 1994; McMillan 2021a). In parallel, the link between interest rates and commodities has been also investigated and can be classified into two categories. The first addresses the effect of the interest rate level on commodity prices. This line of literature has established that commodity prices increase significantly in response to reductions in real interest rates (Akram 2009; Arango et al. 2011). The second category explores the effect of shocks in the commodity market (mainly oil prices) on long-term interest rates (e.g., Ioannidis and Ka 2018). Recent studies use the Granger (1969) causality test and provide evidence that not only do interest rates drive commodity prices, but also that commodity prices drive income and interest rates (e.g., Harvey et al. 2017). Despite these extensive efforts, the examination of the ability of yield spreads to predict future innovations in commodity prices has attracted relatively less attention in the literature.

The literature points to several reasons why yield spreads forecast future real economic activity. One reason relates to the expectation theory, according to which when the yield curve flattens, market participants expect short-term interest rates to fall due to a recession. This expectation translates into a drop in long-term interest rates, as deteriorating market conditions during recessions might explain the decline in short-term rates. Indeed, economic depressions are often associated with job loss, increased uncertainty, business failures, and credit line contractions. Consequently, if people anticipate a slowdown in economic activity, there will likely be a drop in the demand for credit, which in turn leads to a decline in long-term interest rates. On the other hand, if market participants anticipate an upturn in the economy, future short-term interest rates will be expected to rise, leading to a steepening of the yield curve. Thus, while falling yield spreads preceding recessions are caused by both aforementioned factors, the decline in expectations about short-term rates is the more important one (Hamilton and Kim 2002).

The second explanation is related to the countercyclical monetary policy according to which economic expansion is accompanied by inflation. To control inflation, central banks follow a countercyclical monetary policy by raising short-term interest rates. Tight monetary policies are used to stabilize output growth and cause the yield spread to drop. This measure is aimed at reducing the anticipation of inflation to levels below the current inflation rate. Consequently, short-term interest rates rise more than long-term interest rates do, and the yield curve flattens. As real interest rates remain high, spending decreases, causing an economic slowdown. Estrella (2005) provided a theoretical model wherein the yield spread explains both output and inflation. The author showed that the predictive ability of the yield spread depends upon the reaction of the given monetary policy. By the same logic, in a recessionary economy, central banks will reduce short-term interest rates as part of a countercyclical monetary policy. Thus, a lower yield spread or a flat yield curve is a harbinger of economic downturn.

The third explanation of why the yield curve slope is a leading indicator of economic output is referred to as the inter-temporal consumption model. As per Harvey (1989), during expansionary periods people have a stable level of consumption, whereas during recessions, when income is falling, they tend to reduce their consumption. Hence, if people anticipate a decline in economic activity, they have an incentive to save in the current period by selling short-term assets and buying bonds, which will ensure a stable income during the low-income period. As a result, long-term bond prices rise, which in turn reduces their yields, and short-term bonds trade at increased rates.

Finally, there are various empirical works dealing with the relationship between the factors affecting macroeconomic fundamentals and commodity prices. Variables such as an increase in economic activity (e.g., Duarte et al. 2021), economic uncertainty (Qadan and Nama 2018), the exchange rate of the dollar (e.g., Churchill et al. 2019) and the market index (Kagraoka 2016) are capable of affecting commodity prices. Considering the ability of the spread in bond yields to anticipate future economic activity, it is very important to have some understanding of its role in providing information about the future prices of commodities.

3. Data

Our sample consists of monthly data on nine commodities—oil, silver, gold, platinum, palladium, zinc, ethanol, coal, and natural gas—obtained based on the availability of the data. These commodities are used in many industries as raw materials. Data about silver and gold come from the Chicago Mercantile Exchange (CME). Data about platinum palladium and natural gas come from the New York Mercantile Exchange (NYMEX). The data on zinc and copper come from the London Bullion Market Association (LBMA). Information about coal comes from the International Exchange (ICE), whereas the data on WTI oil are taken from the Federal Reserve Bank of St. Louis.

The largest sample period used is that for oil, gold and silver, and ranges from January 1986 to December 2020, while the smallest sample is that for coal and ranges from January 2009 to December 2020. Our starting point for each commodity is simply due to the availability of information about their prices. We use the International Monetary Fund’s International Financial Statistics database for the rates for the 3-month, 1-year, 2-year, 10-year and 30-year Treasury bills.

Table 1. Descriptive Statistics. Panel A—yield rates. Panel B—commodities.

Panel A
	Y3M		Y1		Y2		Y10		Y30
Mean	3.173		3.455		3.761		4.845		5.343
Median	3.055		3.390		3.920		4.680		5.155
Maximum	9.140		9.570		9.680		9.520		9.610
Minimum	0.010		0.100		0.130		0.620		1.270
Std. Dev.	2.557		2.623		2.653		2.280		2.052
Skewness	0.258		0.237		0.214		0.199		0.162
Kurtosis	1.845		1.824		1.793		1.981		2.046
J-Bera	28.001		28.118		28.716		20.950		17.767
#Obs.	420		420		420		420		420
Sample Period	1986:01 to 2020:12		1986:01 to 2020:12		1986:01 to 2020:12		1986:01 to 2020:12		1986:01 to 2020:12
Panel B
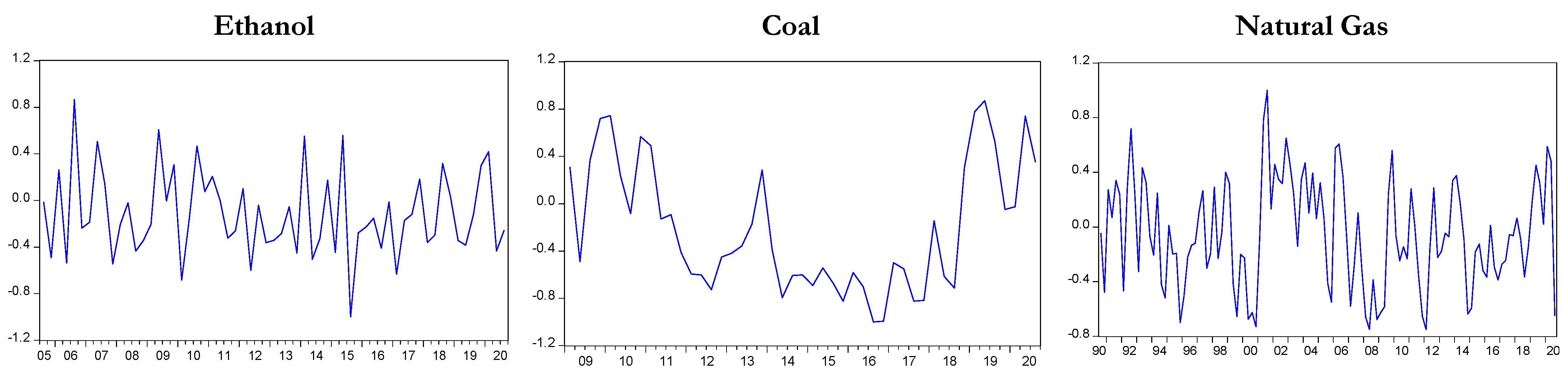
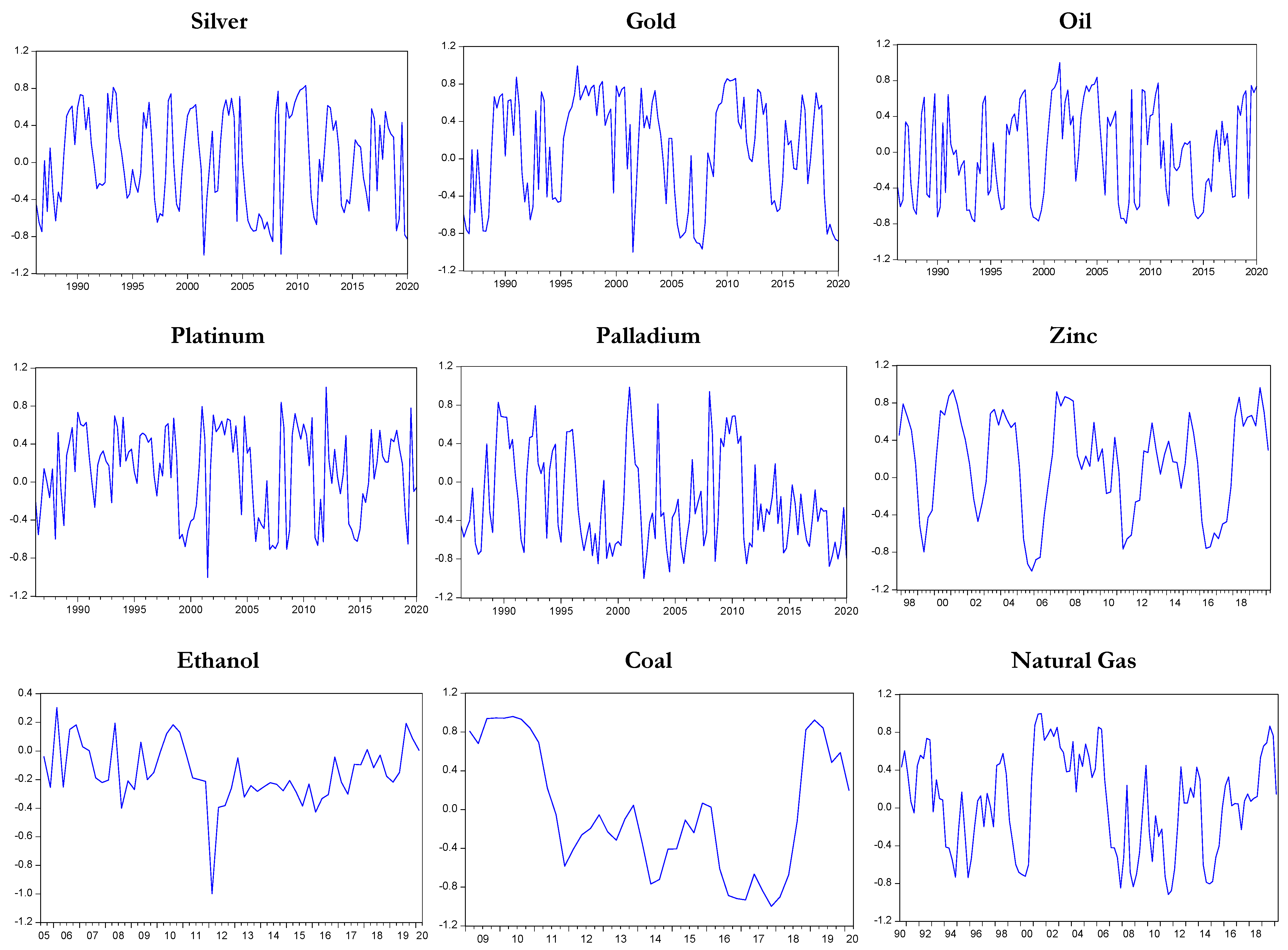
	COAL	ETHNL	GOLD	NGAZ	OIL	PLDM	PLTNM	SLVR	ZINC
Mean	83.85	1.89	730.10	3.75	44.15	459.31	822.34	11.20	1879.99
Median	82.65	1.77	425.55	2.92	31.90	309.75	680.50	6.72	1891.75
Maximum	130.90	3.62	1970.80	13.92	140.97	2508.80	2180.70	48.58	4474.00
Minimum	49.95	0.82	255.00	1.17	11.13	76.35	336.40	3.56	746.75
Std. Dev.	21.12	0.50	480.20	2.24	28.99	440.48	440.90	8.26	807.30
Skewness	0.280	0.672	0.792	1.721	0.879	2.111	0.813	1.418	0.528
Kurtosis	2.142	3.121	2.151	6.416	2.671	8.299	2.611	4.823	2.765
J-Bera	6.30	14.20	56.52	360.54	55.93	797.60	48.57	198.86	13.68
#Obs.	144	187	420	368	420	417	417	420	281
Sample Period	2009:01 to 2020:12	2005:06 to 2020:12	1986:01 to 2020:12	1990:05 to 2020:12	1986:01 to 2020:12	1986:04 to 2020:12	1986:04 to 2020:12	1986:01 to 2020:12	1997:08 to 2020:12

Notes: Panel A of the table reports the descriptive statistics of the Treasury yield rates, whereas Panel B reports those of the commodity prices. Y_3M, Y₁, Y₂, Y₁₀ and Y₃₀, are US treasury yields on 3-month, 1-year, 2-year, 10-year and 30-year bonds, respectively—all denominated in annual terms.

reports the descriptive statistics of the key variables used in this study and outlines the sample period. Panel A reports the descriptive statistics of the six proxies used to capture the yield spread, while Panel B reports the descriptive statistics of the commodities employed here.

4. Method

The empirical economic literature defines the yield spread as the difference between the yield rates on long-term and short-term government bonds. In fact, there is no precise theory that defines how the yield spread should be calculated, and the choice of creating a proxy for the yield spread is somewhat arbitrary. Indeed, the literature provides many proxies for the yield spread including the difference between the yields on 10-year bonds and 3-month bonds (Estrella and Hardouvelis 1991), the difference between 10-year and 1-year interest rates (Stock and Watson 1989) and the difference between yields on 30-year and 3-month bonds (Duffee 1998). Given the mixed definitions of the yield spread, we utilize as broad a spectrum of bonds as possible, specifically, the differences in the yields on 10-year and 3-month Treasury bonds, 10-year and 1-year bonds, 10-year and 2-year bonds, 30-year and 3-month bonds, 30-year and 1-year bonds and 30-year and 2-year bonds.

We formulate the following model to trace the effect of the current yield spread (at time t) on the cumulative rate of change in the “h” subsequent months or quarters.

$$R_{t+h}={\beta }_0^h+{\beta }_1^h\left(Y_{Long,t}-Y_{Short,t}\right)+B^{\prime }{X}_t+v_{t+h}$$

(1)

where

$$R_{t+h}=\left(\frac{12}{h}\times 100\right)\times \left(\ln \left(P_{t+h}\right)-\ln (P_t)\right)$$

(2)

$R_{t+h}$ is the rate of change in the price of the commodity in annual terms. If, for example, h = 1, then $R_{t+1}$ captures the cumulative return of one period (say, a quarter) ahead. If h = 4, then $R_{t+4}$ captures the cumulative returns for the coming twelve months (four quarters). The difference between the yield rates on long-term and short-term government bonds is given by $\left(Y_{Long,t}-Y_{Short,t}\right)$ and $v_{t+h}$ is the forecast error. Given the possibility that the forecast error might be correlated, we use Newey and West’s (1987) corrected covariance estimator. The estimated coefficients guarantee consistency in the presence of both heteroscedasticity and autocorrelation (HAC) of unknown form. $X_t$ denotes a matrix of additional explanatory variables. In line with the literature, we use the U.S. dollar exchange rate (Churchill et al. 2019), the S&P500 (Kagraoka 2016), the industrial production index (Duarte et al. 2021) and the economic policy uncertainty index (Huang et al. 2021).

To depict the dynamic correlation between the current yield spread and the future price direction of commodities, we use the established multivariate concept of dynamic conditional correlation generalized autoregressive conditional heteroscedasticity (DCC GARCH). Engle (2002) developed this state-of-the-art method, which has been used extensively to quantify dynamic relationships over time. In the following, we provide a very basic description of this methodology.1

The dynamic conditional correlation estimator is an extension of the constant conditional correlation model suggested by Bollerslev (1990). According to Bollerslev’s procedure, the correlation matrix ρ is constant. That is, $H_t=D_t\rho D_t,$ where $D_t=diag\left\{\sqrt{h_{i,t}}\right\}$ and $h_{i,t}$ represents the i-th univariate (G)ARCH(p, q) process. In other words,

$$D_t=\left(\begin{array}{ccccc}{h}_{1t}& 0& 0& \cdots & 0\\ 0& h_{2t}& 0& \cdots & 0\\ 0& 0& h_{3t}& & ⋮\\ ⋮& ⋮& & \ddots & 0\\ 0& 0& \cdots & & h_{nt}\end{array}\right)$$

(3)

According to Engle (2002), ρ is allowed to vary in time t. Thus,

$$H_t\equiv D_t{\rho }_t{D}_t$$

(4)

The correlation matrix is then given by:

$${\rho }_t=\left(\begin{array}{ccccc}1& q_{12,t}& q_{13,t}& \cdots & q_{1n,t}\\ q_{21,t}& 1& q_{23,t}& \cdots & q_{2n,t}\\ q_{31,t}& q_{32,t}& 1& \cdots & q_{3n,t}\\ ⋮& ⋮& ⋮& \ddots & ⋮\\ q_{n1,t}& q_{n2,t}& q_{n3,t}& \cdots & 1\end{array}\right)$$

(5)

The correlation matrix is a positive definite one because of the positive nature of H_t. Given that $Q_t=\left(q_{ij,t}\right)$, then:

$$Q_t=\left(1-\alpha -\beta \right)\overline{Q}+\alpha {\mathsf{\eta }}_{t-1}{\mathsf{\eta }}_{t-1}^{\prime }+\beta Q_{t-1}$$

(6)

where ${\mathsf{\eta }}_t={\epsilon }_{i,t}/\sqrt{h_{i,t}}$ are the standardized residuals from the (G)ARCH model, $\overline{Q}$ is a n × n matrix and represents the unconditional variance matrix of the standardized error terms η_t and computed as $\overline{Q}=E\left[{\mathsf{\eta }}_{\mathrm{t}}\times {\mathsf{\eta }}_{\mathrm{t}}^{\prime }\right]$. α and β are non-negative scalars and satisfy the mean-revering assumption (i.e., α + β < 1). $Q_t$ is a positive definite matrix that determines the structure of dynamics and $Q_t^{\ast -1}$ normalizes the elements in $Q_t$;

$$Q_t^{\ast -1}=\left(\begin{array}{ccccc}\frac{1}{\sqrt{q_{11t}}}& 0& 0& \cdots & 0\\ 0& \frac{1}{\sqrt{q_{22t}}}& 0& \cdots & 0\\ 0& 0& \frac{1}{\sqrt{q_{33t}}}& \cdots & 0\\ ⋮& ⋮& & \ddots & ⋮\\ 0& 0& 0& \cdots & \sqrt{q_{nnt}}\end{array}\right)$$

(7)

In order to estimate the parameters of $H_t$ specifically $\mathsf{\Phi }=\left(\alpha , \beta \right)$, the following log-likelihood function is maximized:

$$L\left(\mathsf{\Phi }\right)=-0.5{\displaystyle \sum }_{i=1}^T\left(n\log \left(2\pi \right)+\log \left(\left|H_t\right|\right)+y_t^{\prime }{H}_t^{-1}{y}_t\right)$$

(8)

5. Empirical Findings

Table 2. Estimation results of Equation (1) with the Y₁₀-Y_3M indicator. Panel A: entire sample. Panel B: sample period 1986–2003. Panel C: sample period 2004–2020.

Panel A
Forecast Horizon	Oil (1986:01–2020:12)				Silver (1986:01–2020:12)				Gold (1986:01–2020:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	10.61 (0.42)	−3.78 (0.56)	0.003	139	5.33 (0.48)	−0.37 (0.92)	0.000	139	4.89 (0.24)	0.08 (0.97)	0.000	138
2	9.03 (0.29)	−3.08 (0.46)	0.004	138	3.97 (0.45)	0.39 (0.88)	0.000	138	4.74 (0.10)	0.15 (0.92)	0.000	138
3	7.41 (0.24)	−2.30 (0.46)	0.004	137	1.85 (0.67)	1.40 (0.50)	0.003	137	4.56 * (0.06)	0.11 (0.93)	0.000	137
4	4.29 (0.41)	−0.91 (0.72)	0.001	136	−0.23 (0.95)	2.40 (0.17)	0.014	136	4.47 ** (0.04)	0.06 (0.95)	0.000	136
Forecast Horizon	Platinum (1986:04–2020:12)				Palladium (1986:04–2020:12)				Zinc (1997:08–2020:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	5.23 (0.45)	−1.48 (0.66)	0.001	139	19.38 * (0.06)	−6.14 (0.22)	0.011	139	3.18 (0.75)	−0.02 (0.99)	0.000	93
2	3.68 (0.44)	−0.72 (0.76)	0.000	138	15.63 ** (0.04)	−3.95 (0.28)	0.009	138	−1.03 (0.89)	2.62 (0.49)	0.005	92
3	1.27 (0.73)	0.45 (0.80)	0.0005	136	10.53 * (0.09)	−1.16 (0.70)	0.001	137	−4.31 (0.51)	4.53 (0.17)	0.021	91
4	−0.18 (0.95)	1.17 (0.43)	0.004	136	8.18 (0.14)	0.24 (0.93)	0.000	136	−6.91 (0.23)	6.04 ** (0.04)	0.05	90
Forecast Horizon	Ethanol (2005:06–2020:12)				Coal (2009:01–2020:12)				Natural Gas (1990:05–2020:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	3.52 (0.86)	−2.70 (0.79)	0.012	62	−7.04 (0.66)	4.08 (0.59)	0.006	48	4.24 (0.79)	−1.36 (0.86)	0.0003	122
2	1.49 (0.90)	−2.32 (0.70)	0.002	61	−10.92 (0.37)	5.85 (0.31)	0.0.23	47	2.11 (0.84)	−0.40 (0.94)	0.000	121
3	−1.69 (0.84)	−0.64 (0.88)	0.004	60	−15.40 (0.12)	7.67 (0.10)	0.06	46	−1.00 (0.91)	1.02 (0.80)	0.001	120
4	−5.01 (0.43)	0.61 (0.85)	0.001	59	−14.55 (0.11)	7.14 * (0.09)	0.06	45	−3.51 (0.61)	2.39 (0.47)	0.005	119
Panel B
Forecast Horizon	Oil (1986:01–2003:12)				Silver (1986:01–2003:12)				Gold (1986:01–2003:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	11.73 (0.42)	−3.38 (0.63)	0.003	71	−6.16 (0.40)	4.15 (0.25)	0.019	71	−4.99 (0.37)	3.60 (0.18)	0.025	70
2	10.25 (0.29)	−2.58 (0.58)	0.004	71	−8.19 (0.13)	5.85 ** (0.03)	0.07	71	−4.51 (0.18)	3.36 ** (0.04)	0.06	71
3	10.62 (0.13)	−2.91 (0.39)	0.01	71	−7.71 * (0.07)	5.28 *** (0.01)	0.09	71	−3.99 (0.13)	2.74 ** (0.03)	0.06	71
4	9.55 (0.12)	−2.26 (0.44)	0.009	71	−7.57 ** (0.04)	5.20 *** (0.004)	0.114	71	−3.54 (0.13)	2.40 ** (0.04)	0.06	71
Forecast Horizon	Platinum (1986:04–2003:12)				Palladium (1986:04–2003:12)				Zinc (1997:08–2003:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	−1.01 (0.89)	2.90 (0.44)	0.009	71	11.53 (0.40)	−4.63 (0.49)	0.007	71	−17.87 (0.11)	9.02 (0.12)	0.10	25
2	−3.28 (0.51)	4.35 * (0.07)	0.046	71	4.58 (0.66)	−0.001 (0.99)	0.000	71	−15.85 * (0.06)	9.50 ** (0.03)	0.18	25
3	−4.65 (0.23)	4.74 ** (0.014)	0.085	70	0.13 (0.99)	2.20 (0.60)	0.004	71	−14.08 ** (0.04)	8.39 ** (0.02)	0.20	25
4	−4.98 (0.15)	4.91 *** (0.004)	0.112	71	−1.30 (0.87)	2.99 (0.43)	0.009	71	−13.17 ** (0.03)	8.38 *** (0.01)	0.26	25
Forecast Horizon	Natural Gas (1990:05–2003:12)
h	C	(Y10-Y3M)	R2	N
1	8.41 (0.76)	1.11 (0.93)	0.0002	54
2	−0.57 (0.97)	5.51 (0.50)	0.009	54
3	−5.24 (0.69)	7.99 (0.19)	0.03	54
4	−7.17 (0.48)	9.42 * (0.05)	0.07	54
Panel C
Forecast Horizon	Oil (2004:01–2020:12)				Silver (2004:01–2020:12)				Gold (2004:01–2020:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	9.51 (0.67)	−4.22 (0.70)	0.002	68	17.23 (0.20)	−5.16 (0.44)	0.009	68	14.40 ** (0.02)	−3.29 (0.27)	0.019	68
2	7.77 (0.59)	−3.65 (0.60)	0.004	67	16.94 * (0.07)	−5.45 (0.23)	0.022	67	14.54 *** (0.002)	−3.29 (0.14)	0.033	67
3	3.90 (0.72)	−1.62 (0.76)	0.001	66	12.35 (0.11)	−2.87 (0.44)	0.009	66	13.92 *** (0.00)	−2.81 (0.14)	0.03	66
4	−1.66 (0.85)	0.66 (0.88)	0.0004	65	8.09 (0.20)	−0.78 (0.80)	0.001	65	13.53 *** (0.00)	−2.64 (0.12)	0.04	65
Forecast Horizon	Platinum (2004:01–2020:12)				Palladium (2004:01–2020:12)				Zinc (2004:01–2020:12)
h	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N	C	(Y10-Y3M)	R2	N
1	11.84 (0.31)	−6.18 (0.29)	0.017	68	27.41 * (0.07)	−7.64 (0.31)	0.016	68	13.99 (0.29)	−4.68 (0.48)	0.008	68
2	11.20 (0.17)	−6.21 (0.12)	0.04	67	27.36 ** (0.013)	−8.18 (0.13)	0.036	67	6.80 (0.52)	−0.91 (0.86)	0.0005	67
3	7.84 (0.21)	−4.25 (0.17)	0.029	66	21.92 ** (0.02)	−4.88 (0.26)	0.02	66	0.98 (2.38)	2.38 (0.59)	0.005	66
4	5.27 (0.30)	−2.97 (0.23)	0.023	65	18.91 ** (0.02)	−2.94 (0.43)	0.01	65	−3.42 (0.67)	4.67 (0.24)	0.022	65
Forecast Horizon	Natural Gas (2004:01–2020:12)
h	C	(Y10-Y3M)	R2	N
1	1.95 (0.92)	−4.07 (0.67)	0.003	68
2	5.14 (0.71)	−5.95 (0.38)	0.012	67
3	3.37 (0.76)	−5.48 (0.31)	0.016	66
4	0.49 (0.96)	−4.21 (0.32)	0.016	65

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y_3M denotes the yield spread calculated as the difference between the yield rates on 10-year and 3-month government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

reports the estimation results of the reduced form of Equation (1). That is, future commodity returns ($R_{t+h}$) are regressed against the current yield spread only. $R_{t+h}={\beta }_0^h+{\beta }_1^h\left(Y_{Long,t}-Y_{Short,t}\right)+v_t^h$. In this table, we utilize the difference between 10-year and 3-month bond yields (Y₁₀-Y_3M) as a proxy for the yield spread. We also use four forecasting horizons (h; h = 1, 2, 3 and 4) where h = 1 indicates forecasting of one quarter ahead and h = 4 indicates forecasting four quarters ahead. Panel A of the table reports the estimation results with respect to the entire sample, Panel B covers 1986 to 2003, and Panel C covers 2004 to 2020.

The picture that emerges indicates an insignificant positive tendency of the yield spread to forecast future changes in the commodities used. Panel B, however, presents a different picture. Except for oil and palladium, we find that, when considering 1986–2003, the yield spread has a positive effect on the future prices of the rest of the commodities. The ability of the current yield spread to predict future innovations in commodity prices is manifested in both the statically significant positive coefficients and the relatively high R² (for example, R² values are 6%, 7%, 11.2%, 11.4% and 26% for gold, natural gas, platinum, silver and zinc, respectively.). A steeper yield curve is always viewed as an indication that the growth in future output is about to rise. Thus, the positive correlation detected indicates that an increase in the slope at time t will have a positive impact on the future prices of commodities.

While Table 2 regresses the commodity returns against the yield spread only, in

Table 3. Estimation results of Equation (1) for the period 1986–2003.

Forecast Horizon	Oil (1986:01–2003:12)								Silver (1986:01–2003:12)
h	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N
1	11.80	−4.97	0.48	−2.20	11.89	0.03	−0.06	71	−8.22	4.93	0.58	−10.74	−1.92	0.00	−0.04	71
	(0.76)	(−0.62)	(0.06)	(−0.11)	(0.67)	(0.26)			(−1.06)	(1.23)	(0.15)	(−1.06)	(−0.22)	(−0.07)
2	10.61	−3.25	−9.41c	−5.24	10.78	−0.02	0.00	71	−9.31	6.50b	0.58	−5.78	−3.10	−0.01	0.01	71
	(1.06)	(−0.63)	(−1.82)	(−0.4)	(0.95)	(−0.19)			(−1.64)	(2.22)	(0.2)	(−0.78)	(−0.48)	(−0.27)
3	10.22	−2.73	−5.10	−4.14	3.21	0.00	−0.03	71	−9.37b	6.04a	0.53	−9.09	−2.79	−0.02	0.06	71
	(1.39)	(−0.72)	(−1.35)	(−0.43)	(0.38)	(−0.03)			(−2.11)	(2.64)	(0.23)	(−1.57)	(−0.55)	(−0.4)
4	8.01	−1.83	−2.85	−7.46	2.58	0.02	−0.03	71	−8.92b	5.92a	−0.02	−7.43	−2.73	−0.01	0.09	71
	(1.25)	(−0.56)	(−0.86)	(−0.9)	(0.35)	(0.44)			(−2.36)	(3.05)	(−0.01)	(−1.51)	(−0.63)	(−0.46)
Forecast Horizon	Gold (1986:01–2003:12)								Platinum (1986:04–2003:12)
h	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N
1	−7.28	3.49	4.47	−10.20	3.19	0.00	0.01	70	−2.64	2.25	−1.79	−22.72b	7.66	−0.10	0.06	71
	(−1.23)	(1.17)	(1.52)	(−1.39)	(0.5)	(0.1)			(−0.34)	(0.57)	(−0.45)	(−2.26)	(0.87)	(−1.57)
2	−4.01	4.01b	−1.50	3.21	−5.20	−0.02	0.04	70	−5.10	4.63c	−1.73	−14.33b	2.42	−0.03	0.07	71
	(−1.14)	(2.23)	(−0.83)	(0.7)	(−1.3)	(−0.72)			(−1.01)	(1.78)	(−0.66)	(−2.17)	(0.42)	(−0.75)
3	−3.74	3.04b	−0.49	0.67	−2.80	−0.02	0.02	70	−5.71	4.17b	0.32	−10.25c	5.89	−0.02	0.09	71
	(−1.35)	(2.14)	(−0.35)	(0.19)	(−0.89)	(−0.93)			(−1.42)	(2.02)	(0.16)	(−1.97)	(1.29)	(−0.49)
4	−3.62	2.94b	−0.87	−0.42	−3.48	−0.02	0.03	70	−5.19	4.32b	−0.21	−5.51	4.59	−0.02	0.09	71
	(−1.46)	(2.31)	(−0.68)	(−0.13)	(−1.24)	(−0.84)			(−1.44)	(2.33)	(−0.11)	(−1.17)	(1.12)	(−0.7)
Forecast Horizon	Palladium (1986:04–2003:12)								Zinc (1997:08–2003:12)
h	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N
1	15.89	−10.17	−14.00b	−13.30	38.73	−0.18	0.13	71	−19.18c	5.87	8.85	−27.86	9.80	−0.02	0.07	25
	(1.2)	(−1.49)	(−2.05)	(−0.77)	(2.57)	(−1.6)			(−1.76)	(1.00)	(1.59)	(−1.15)	(0.66)	(−0.18)
2	6.75	−4.82	−3.68	−12.06	32.29a	−0.08	0.06	71	−17.44b	7.48	5.42	−26.55	4.01	0.04	0.15	25
	(0.65)	(−0.9)	(−0.68)	(−0.89)	(2.72)	(−0.9)			(−2.13)	(1.7)	(1.3)	(−1.47)	(0.36)	(0.38)
3	3.16	−4.11	3.39	−4.46	37.41a	−0.02	0.13	71	−15.76b	7.29c	3.40	−24.21	−2.48	0.10	0.21	25
	(0.38)	(−0.95)	(0.78)	(−0.41)	(3.9)	(−0.35)			(−2.4)	(2.07)	(1.02)	(−1.67)	(−0.28)	(1.26)
4	0.88	−2.11	3.50	−4.59	30.30a	−0.01	0.10	71	−15.02b	7.85b	2.03	−12.48	1.41	0.09	0.22	25
	(0.11)	(−0.53)	(0.88)	(−0.46)	(3.46)	(−0.23)			(−2.59)	(2.52)	(0.69)	(−0.97)	(0.18)	(1.37)
Forecast Horizon	Natural gas (1990:05–2003:12)
h	C	(Y10-Y3M)	∆SP	∆EX	∆IP	∆EPU	R2	N
1	4.31	−3.20	18.12	−12.32	36.33	0.04	−0.04	54
	(0.15)	(−0.23)	(1.33)	(−0.33)	(1.14)	(0.16)
2	−6.06	4.49	4.89	−34.36	21.29	−0.03	−0.03	54
	(−0.34)	(0.52)	(0.57)	(−1.43)	(1.06)	(−0.18)
3	−16.59	9.11	5.02	−50.56a	20.50	0.12	0.16	54
	(−1.32)	(1.51)	(0.83)	(−3.02)	(1.46)	(1.15)
4	−15.38	10.68b	3.90	−32.98b	10.68	0.11	0.13	54
	(−1.51)	(2.19)	(0.8)	(−2.44)	(0.94)	(1.33)

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y_3M denotes the yield spread calculated as the difference between the yield rates on 10-year and 3-month government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. a, b and c denote statistical significance at the 1%, 5%, and 10% levels, respectively.

we present the full estimation of Equation (1) after controlling for additional explanatory variables. The sample period considered in Table 3 is for 1986–2003. The results for the entire sample and the period after 2004 appear in

Table A2. Estimation results of model 5 with the Y10-Y3M indicator. Panel A: entire sample. Panel B: sample period 2004–2020.

Panel A

Forecast Horizon

Oil (1986:01–2020:12)

Silver (1986:01–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

5.92

−0.79

−0.55

9.32

−14.07b

0.11

0.03

139

1.67

1.06

−0.85

−10.29

−4.90

0.06

−0.01

139

(0.45)

(−0.12)

(−0.08)

(0.56)

(−2.43)

(1.08)

(0.21)

(0.28)

(−0.21)

(−1.04)

(−1.44)

(0.99)

2

7.33

−1.74

−5.23

2.47

−3.76

0.04

−0.01

138

3.12

1.40

−3.50

−6.35

−4.05c

−0.05

0.02

138

(0.84)

(−0.41)

(−1.16)

(0.22)

(−0.99)

(0.66)

(0.58)

(0.54)

(−1.26)

(−0.93)

(−1.73)

(−1.10)

3

4.84

−0.54

−5.93

−9.86

−5.02

−0.02

0.04

137

−0.37

2.76

−2.12

−8.83

−5.06a

−0.03

0.05

137

(0.75)

(−0.17)

(−1.81)

(−1.23)

(−1.75)

(−0.35)

(−0.09)

(1.32)

(−0.96)

(−1.64)

(−2.62)

(−0.77)

4

4.49

−1.48

−2.40

−5.33

6.23

−0.01

−0.01

136

0.69

2.56

−3.33c

−0.55

−0.82

−0.04

0.02

136

(0.84)

(−0.56)

(−0.85)

(−0.80)

(1.17)

(−0.25)

(0.19)

(1.42)

(−1.71)

(−0.12)

(−0.23)

(−1.38)

Forecast Horizon

Gold (1986:01–2020:12)

Platinum (1986:04–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

3.73

0.54

1.61

−1.05

−2.52

0.01

−0.02

138

3.32

−0.56

−3.60

−8.25

−1.92

0.02

−0.02

139

(0.86)

(0.26)

(0.73)

(−0.20)

(−1.37)

(0.45)

(0.46)

(−0.16)

(−0.96)

(−0.91)

(−0.61)

(0.4)

2

5.19c

0.43

−2.09

2.21

−1.25

−0.03

0.01

137

3.83

−0.31

−6.05b

−5.14

0.50

−0.03

0.02

138

(1.76)

(0.30)

(−1.37)

(0.60)

(−0.97)

(−1.14)

(0.79)

(−0.13)

(−2.43)

(−0.84)

(0.24)

(−0.87)

3

4.68c

0.33

−0.91

1.45

−1.05

−0.02

−0.01

136

0.63

1.01

−2.32

−7.80c

−1.71

−0.04

0.02

137

(1.84)

(0.27)

(−0.71)

(0.46)

(−0.93)

(−0.85)

(0.17)

(0.55)

(−1.21)

(−1.66)

(−1.02)

(−1.44)

4

5.25b

0.38

−1.91

3.31

−2.83

−0.02

0.01

135

1.19

0.56

−1.71

−0.17

4.16

−0.04c

0.02

136

(2.34)

(0.34)

(−1.61)

(1.19)

(−1.27)

(−1.22)

(0.38)

(0.36)

(−1.03)

(−0.04)

(1.34)

(−1.8)

Forecast Horizon

Palladium (1986:04–2020:12)

Zinc (1997:08–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

19.89c

−6.40

−12.07b

−7.36

8.63c

0.03

0.03

139

−1.27

0.56

−6.60

−15.05

0.07

0.17b

0.01

93

(1.94)

(−1.29)

(−2.27)

(−0.57)

(1.93)

(0.37)

(−0.13)

(0.11)

(−1.3)

(−1.07)

(0.02)

(2.03)

2

16.52b

−4.10

−7.36c

−10.51

4.02

−0.05

0.02

138

−2.35

2.75

−2.46

−11.65

−0.71

0.03

−0.03

92

(2.16)

(−1.11)

(−1.87)

(−1.1)

(1.21)

(−0.92)

(−0.3)

(0.7)

(−0.61)

(−1.05)

(−0.23)

(0.45)

3

11.65c

−1.77

−0.97

−5.21

2.92

−0.03

−0.02

137

−6.15

5.27

−2.60

−5.86

−2.95

0.02

−0.01

91

(1.78)

(−0.56)

(−0.29)

(−0.64)

(1)

(−0.69)

(−0.91)

(1.59)

(−0.77)

(−0.63)

(−1.07)

(0.43)

4

9.71c

−1.60

0.49

−1.63

13.19b

−0.03

0.01

136

−6.83

6.04b

−1.16

7.09

3.45

0.02

0.01

90

(1.72)

(−0.57)

(0.16)

(−0.23)

(2.35)

(−0.72)

(−1.14)

(2.05)

(−0.37)

(0.86)

(0.57)

(0.38)

Forecast Horizon

Ethanol (2005:06–2020:12)

Coal (2009:01–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−1.39

−0.65

3.71

47.44c

−7.37

0.20

0.07

62

4.07

−0.18

−3.93

−14.93

9.82b

−0.12

0.10

48

(−0.07)

(−0.07)

(0.35)

(1.95)

(−1.12)

(1.35)

(0.26)

(−0.03)

(−0.54)

(−0.84)

(2.45)

(−1.11)

2

−5.25

−0.63

1.61

−2.20

−7.50c

0.13

0.02

61

−1.02

2.98

−5.38

−16.73

5.05c

−0.20b

0.15

47

(−0.43)

(−0.1)

(0.25)

(−0.15)

(−1.85)

(1.47)

(−0.09)

(0.55)

(−1.02)

(−1.29)

(1.72)

(−2.42)

3

−5.53

0.68

−0.35

−8.83

−5.12c

0.01

−0.03

60

−13.96

7.81

−1.61

−12.06

−1.60

−0.13c

0.07

46

(−0.63)

(0.16)

(−0.08)

(−0.83)

(−1.7)

(0.12)

(−1.34)

(1.66)

(−0.36)

(−1.1)

(−0.61)

(−1.84)

4

−3.89

0.66

−3.32

2.19

0.06

−0.02

−0.07

59

−12.55

6.48

−1.08

−7.51

1.56

−0.06

−0.01

45

(−0.58)

(0.2)

(−0.87)

(0.27)

(0.01)

(−0.33)

(−1.3)

(1.48)

(−0.24)

(−0.74)

(0.23)

(−0.93)

Forecast Horizon

Natural gas (1990:05–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

5.82

−3.09

15.11c

15.35

1.91

0.00

0.00

122

(0.36)

(−0.4)

(1.87)

(0.74)

(0.29)

(−0.01)

2

−0.62

0.29

2.48

−14.15

−3.37

0.02

−0.03

121

(−0.06)

(0.05)

(0.45)

(−1)

(−0.73)

(0.22)

3

−6.55

2.69

−0.25

−26.80b

−5.08

0.06

0.02

120

(−0.75)

(0.65)

(−0.06)

(−2.46)

(−1.38)

(0.85)

4

−5.93

1.55

1.67

−16.60c

12.51c

0.04

0.03

119

(−0.86)

(0.47)

(0.47)

(−1.93)

(1.85)

(0.7)

Panel B

Forecast Horizon

Oil (2004:01–2020:12)

Silver (2004:01–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−0.10

−0.53

0.86

16.77

−15.95b

0.17

0.04

68

11.98

−4.06

−2.96

−13.37

−4.65

0.11

−0.03

68

(0.00)

(−0.05)

(0.07)

(0.61)

(−2.11)

(1.01)

(0.86)

(−0.6)

(−0.4)

(−0.77)

(−0.98)

(1.09)

2

3.69

−2.15

0.72

9.54

−5.95

0.08

−0.03

67

16.38c

−4.37

−7.96

−11.18

−3.03

−0.06

0.06

67

(0.25)

(−0.3)

(0.09)

(0.52)

(−1.2)

(0.73)

(1.77)

(−0.98)

(−1.63)

(−0.98)

(−0.97)

(−0.9)

3

−0.32

0.45

−6.34

−16.19

−6.44c

−0.02

0.03

66

9.86

−1.47

−4.98

−11.62

−4.22

−0.03

0.05

66

(−0.03)

(0.08)

(−1.1)

(−1.21)

(−1.68)

(−0.27)

(1.29)

(−0.4)

(−1.26)

(−1.27)

(−1.6)

(−0.54)

4

−0.25

−0.17

−1.22

−1.81

8.51

−0.03

−0.05

65

10.70c

−0.62

−6.94c

3.41

−0.20

−0.06

0.03

65

(−0.03)

(−0.04)

(−0.24)

(−0.17)

(1.04)

(−0.44)

(1.7)

(−0.21)

(−1.98)

(0.45)

(−0.03)

(−1.23)

Forecast Horizon

Gold (2004:01–2020:12)

Platinum (2004:01–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

13.40b

−2.83

−0.20

3.97

−1.68

0.02

−0.03

68

7.99

−5.32

−5.15

0.76

−1.60

0.13

−0.02

68

(2.15)

(−0.94)

(−0.06)

(0.51)

(−0.79)

(0.45)

(0.66)

(−0.9)

(−0.79)

(0.05)

(−0.39)

(1.41)

2

15.01a

−2.91

−3.22

0.05

−0.50

−0.03

0.02

67

11.86

−5.76

−10.43b

−1.19

1.81

−0.01

0.05

67

(3.24)

(−1.31)

(−1.31)

(0.01)

(−0.32)

(−0.96)

(1.44)

(−1.45)

(−2.4)

(−0.12)

(0.65)

(−0.17)

3

14.24a

−2.59

−1.62

1.16

−0.16

−0.02

−0.02

66

7.39

−3.57

−4.49

−9.43

−1.74

−0.05

0.05

66

(3.44)

(−1.31)

(−0.76)

(0.23)

(−0.11)

(−0.67)

(1.16)

(−1.17)

(−1.36)

(−1.23)

(−0.79)

(−1.04)

4

15.03a

−2.38

−2.94

5.51

−1.90

−0.03

0.06

65

7.35

−3.13

−3.70

2.13

2.23

−0.05

0.02

65

(4.32)

(−1.42)

(−1.52)

(1.31)

(−0.6)

(−1.29)

(1.43)

(−1.26)

(−1.29)

(0.34)

(0.47)

(−1.31)

Forecast Horizon

Palladium (2004:01–2020:12)

Zinc (2004:01–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

25.75

−8.22

−8.27

−5.39

6.03

0.15

−0.01

68

8.68

−4.06

−15.79b

−15.38

2.54

0.22b

0.06

68

(1.66)

(−1.09)

(−0.99)

(−0.28)

(1.14)

(1.26)

(0.66)

(−0.63)

(−2.23)

(−0.93)

(0.56)

(2.19)

2

29.00a

−8.33

−8.79

−15.25

2.57

−0.06

0.03

67

5.83

−0.67

−6.85

−9.64

0.78

0.03

−0.05

67

(2.64)

(−1.58)

(−1.51)

(−1.13)

(0.69)

(−0.73)

(0.53)

(−0.13)

(−1.19)

(−0.72)

(0.21)

(0.37)

3

23.67b

−5.24

−2.35

−12.46

0.92

−0.07

−0.01

66

−0.61

3.31

−5.75

−3.17

−1.63

0.01

−0.04

66

(2.54)

(−1.18)

(−0.49)

(−1.11)

(0.29)

(−1.06)

(−0.06)

(0.73)

(−1.17)

(−0.28)

(−0.5)

(0.13)

4

21.04a

−3.07

−3.58

−4.10

1.08

−0.07

−0.02

65

−2.05

4.53

−2.82

10.35

3.08

0.00

−0.03

65

(2.65)

(−0.8)

(−0.81)

(−0.43)

(0.15)

(−1.26)

(−0.24)

(1.12)

(−0.6)

(1.02)

(0.4)

(0)

Forecast Horizon

Natural gas (2004:01–2020:12)

h

C

(Y10-Y3M)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

4.42

−5.06

17.53c

29.15

0.44

−0.03

−0.01

68

(0.23)

(−0.54)

(1.68)

(1.2)

(0.07)

(−0.2)

2

1.56

−5.31

2.90

−6.76

−4.44

0.06

−0.05

67

(0.11)

(−0.77)

(0.38)

(−0.38)

(−0.92)

(0.58)

3

−1.68

−4.13

−1.47

−18.22

−5.65

0.04

−0.01

66

(−0.15)

(−0.75)

(−0.25)

(−1.32)

(−1.43)

(0.51)

4

−0.06

−5.29

−0.68

−10.39

11.01

0.03

−0.01

65

(−0.01)

(−1.23)

(−0.14)

(−0.96)

(1.35)

(0.42)

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y_3M denotes the yield spread calculated as the difference between the yield rates on 10-year and 3-month government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. a, b and c denote statistical significance at the 1%, 5%, and 10% levels, respectively.

(in the online Appendix A). The overall picture is maintained as evident by the significant positive coefficients of the yield spreads even after controlling for real and financial economic variables in the period prior to 2004. The results hold true for all commodities except for oil and palladium.

The statistically significant positive coefficients accord with the empirical literature postulating that commodity prices (specifically silver, gold, platinum, zinc and natural gas) are tightly linked with the business cycle and the state of the economy (e.g., Batten et al. 2010; Kucher and McCoskey 2017; Jahan and Serletis 2019). This economically and statistically significant relationship confirms that these metals are used extensively in various industries, making them more exposed to the expected phases in the economic cycle.

In investigating whether the relationship between yield spreads and future innovations in commodity prices is stable over time, we made two major findings. The first is that the correlation between commodity prices and yield spreads is not stable over time. This finding is evident in Engle’s (2002) dynamic conditional correlations depicted in Figure 1, Figure 2 and Figure 3, which illustrate the dynamic conditional correlation between the yield spreads and the commodity prices two, three and four quarters ahead. This finding accords with recent studies maintaining time-varying relationship between yield spread and future economic output (e.g., Kuosmanen et al. 2019; Chinn and Kucko 2015).

Second, using the Bai and Perron (2003) structural break test we find that the early 2000s is the period associated with structural breaks in the relationship between the yield curve and the prices of commodities.

Table 4. Bai and Perron (2003) multiple break-point test.

h = 2 (Two Quarters Ahead)
	Silver	Oil	Gold	PLTNM	PLDM	Zinc	ETHNL	Coal	NatGas
Break Point #1	2003Q2	=	2001Q2	=	=	=	=	=	=
Break Point #2	2011Q2	=	2012Q3	=	=	=	=	=	=
Break Point #3	=	=	=	=	=	=	=	=	=
h = 3 (Three Quarters Ahead)
	Silver	Oil	Gold	PLTNM	PLDM	Zinc	ETHNL	Coal	NatGas
Break Point #1	2003Q2	=	2001Q1	=	1996Q3	=	=	2011Q1	=
Break Point #2	2011Q1	=	2012Q2	=	=	=	=	2015Q4	=
Break Point #3	=	=	=	=	=	=	=	=	=
h = 4 (Four Quarters Ahead)
	Silver	Oil	Gold	PLTNM	PLDM	Zinc	ETHNL	Coal	NatGas
Break Point #1	2003Q1	1995Q4	2001Q2	1998Q4	1996Q2	=	=	2011Q1	2000Q3
Break Point #2	2011Q1	=	2012Q2	2010Q4	2001Q2	=	=	2015Q4	2006Q2
Break Point #3	=	=	=	=	2008Q4	=	=	=	=

Notes: We tested for any structural break using the Bai and Perron (2003) multiple break-point test. The values listed in the table are those of the break dates. The vast majority of the commodities point to the 2000s as the structural break points. “=” denotes that no significant breakpoint was detected between the future return and the current yield spread.

presents the results of this test and indicates the dates detected as structural break points. In general, the findings of Bai and Perron (2003) test point to the 2003–3004 as the period in which there was a structural break in the relationship between yield spreads and future commodity prices. These findings accord with earlier studies that date the start of the financialization commodities to the early 2000s (Hamilton and Wu 2015; Henderson et al. 2015). In other words, this period marks the start of the increased exposure of portfolio managers, individuals and hedge funds to commodities.

This finding is in line with prior studies documenting the weakening ability of the term structure to predict future economic activity. Early on, Stock and Watson (2003) and Giacomini and Rossi (2006) maintained that the yield spread’s ability to forecast economic expansion has weakened since the 1980s, but its predictive ability remains strong only for recessions. Other works raise questions regarding the stability of the term spread’s predictive content (e.g., Wheelock and Wohar 2009). Evgenidis et al. (2020) confirm the time-varying nature of the yield spread’s predictive ability, mainly during the 2000s.

One possible factor explaining this break between commodities and the most reliable indicator of future economic activity is the financialization of commodities. For a long time, commodities were viewed as a segmented market offering significant diversification benefits in light of the low—even negative—correlation between their returns and the stock market (e.g., Bodie and Rosansky 1980; Demiralay et al. 2019). This characteristic prompted traders, financial institutions and institutional investors to consider this new asset class as a useful diversifier in their portfolios. A byproduct of this development is the acceleration in the financialization of these commodities, which in turn fueled a rapid increase in their co-movements with equity markets (e.g., Qadan et al. 2019). This evolution may explain the breakdown of the obvious relationship between commodities and the expected economic evolution.

We also test the extent to which the dynamic correlation of commodity “i” co-moves with that of commodity “j.” A quick glance at Figure 1, Figure 2 and Figure 3 shows the apparent co-movements between some of these commodities.

Table 5. Pearson’s correlation between the DCC values of the commodities.

	Coal	Ethanol	Gold	Nat.Gas	Oil	PLDM	PLTNM	Silver	Zinc
Coal	1.00
Ethanol	0.647 ***	1.00
Gold	0.090	−0.230 *	1.00
Nat.Gas	0.124	0.321 **	−0.153 *	1.00
Oil	0.692 ***	0.519 ***	0.167 *	0.627 ***	1.00
PLDM	0.407 ***	0.205	0.166 *	−0.222 **	−0.141 *	1.00
PLTNM	0.120	−0.037	0.534 ***	0.354 ***	0.406 ***	0.327 ***	1.00
Silver	0.249	−0.071	0.730 ***	−0.065	0.091	0.487 ***	0.630 ***	1.00
Zinc	0.493 ***	0.277 **	−0.154	0.086	0.196 *	0.278 ***	0.081	0.159	1.00

Notes: ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

presents the simple correlation between the DCC values. Some of the correlation values are negative and statistically significant. For example, we detect a negative correlation between the prices of ethanol and gold, gold and natural gas, natural gas and palladium, oil, and palladium. On the other hand, the majority of the other cases are associated with statistically significant positive correlations, particularly for precious metals. For example, the correlation between the DCC values of gold and silver is 0.73, and that between gold and platinum is 0.534. Overall, this picture reveals that the conditional slope among commodities is largely connected—a clear indication of their similar reaction to the current yield spread.

6. Robustness Checks

6.1. Additional Proxies for Yield

Previous studies suggested capturing the yield spread using different proxies. To develop a broader picture regarding the interaction between the yield spread and the future evolution in commodity prices, we depart from the standard yield spread used in the literature (Y₁₀-Y_3M) and test other proxies: the difference between 10-year and 1-year interest rates (Y₁₀-Y₁), between 10-year and 2-year yields (Y₁₀-Y₂), between 30-year and 3-months yields (Y₃₀-Y_3M), between 30-year and 1-year bond yields (Y₃₀-Y₁) and between 30-year and 2-year bond yields (Y₃₀-Y₂).

Table 6. Estimation results of Equation (1) with the Y₁₀-Y₁ indicator. Panel A: entire sample. Panel B: sample period 1986–2003. Panel C: sample period 2004–2020.

Panel A
Forecast Horizon	Oil (1986:01–2020:12)				Silver (1986:01–2020:12)				Gold (1986:01–2020:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	10.51 (0.25)	−4.45 (0.38)	0.003	139	3.28 (0.61)	1.01 (0.79)	0.000	139	3.82 (0.40)	0.84 (0.72)	0.001	138
2	8.59 (0.30)	−3.40 (0.47)	0.004	138	2.60 (0.67)	1.43 (0.70)	0.002	138	3.65 (0.41)	0.94 (0.68)	0.003	138
3	7.34 (0.35)	−2.71 (0.55)	0.005	137	1.03 (0.86)	2.26 (0.52)	0.007	137	3.41 (0.42)	0.94 (0.67)	0.004	137
4	4.95 (0.51)	−1.56 (0.72)	0.002	136	−0.65 (0.90)	3.19 (0.35)	0.021	136	3.30 (0.42)	0.89 (0.67)	0.004	136
Forecast Horizon	Platinum (1986:04–2020:12)				Palladium (1986:04–2020:12)				Zinc (1997:08–2020:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	3.98 (0.45)	−0.88 (0.78)	0.000	139	18.34 ** (0.04)	−6.63 (0.25)	0.01	139	3.24 (0.80)	−0.06 (0.99)	0.000	93
2	3.15 (0.54)	−0.49 (0.87)	0.0003	138	15.69 * (0.08)	−4.79 (0.39)	0.01	138	0.19 (0.99)	2.13 (0.71)	0.003	92
3	1.71 (0.73)	0.23 (0.94)	0.0001	136	12.24 (0.18)	−2.59 (0.63)	0.004	137	−2.26 (0.84)	3.74 (0.46)	0.01	91
4	0.49 (0.92)	0.94 (0.72)	0.002	136	10.16 (0.27)	−1.10 (0.83)	0.001	136	−4.33 (0.69)	5.12 (0.27)	0.03	90
Forecast Horizon	Ethanol (2005:06–2020:12)				Coal (2009:01–2020:12)				Natural gas (1990:05–2020:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	2.00 (0.88)	−1.97 (0.80)	0.001	62	−4.91 (0.79)	3.21 (0.71)	0.004	48	5.07 (0.71)	−2.16 (0.76)	0.001	122
2	0.20 (0.98)	−1.70 (0.78)	0.001	61	−8.31 (0.61)	4.90 (0.52)	0.02	47	2.75 (0.82)	−0.90 (0.89)	0.0002	121
3	−1.68 (0.84)	−0.72 (0.90)	0.000	60	−12.06 (0.40)	6.51 (0.34)	0.04	46	0.96 (0.93)	−0.12 (0.98)	0.000	120
4	−4.84 (0.46)	0.56 (0.91)	0.000	59	−11.26 (0.42)	5.98 (0.37)	0.04	45	−1.10 (0.91)	1.19 (0.82)	0.001	119
Panel B
Forecast Horizon	Oil (1986:01–2003:12)				Silver (1986:01–2003:12)				Gold (1986:01–2003:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	12.42 (0.31)	−4.79 (0.43)	0.005	71	−7.50 (0.15)	6.26 * (0.07)	0.03	71	−5.59 (0.14)	5.00 ** (0.03)	0.04	70
2	10.64 (0.33)	−3.56 (0.50)	0.006	71	−9.37 * (0.05)	8.28 ** (0.03)	0.11	71	−5.59 * (0.07)	5.06 *** (0.01)	0.10	71
3	9.74 (0.32)	−3.03 (0.53)	0.009	71	−8.99 ** (0.03)	7.64 ** (0.01)	0.14	71	−5.41 ** (0.04)	4.52 *** (0.004)	0.13	71
4	8.86 (0.34)	−2.35 (0.62)	0.007	71	−8.93 ** (0.01)	7.60 *** (0.003)	0.19	71	−5.18 ** (0.04)	4.26 *** (0.003)	0.15	71
Forecast Horizon	Platinum (1986:04–2003:12)				Palladium (1986:04–2003:12)				Zinc (1997:08–2003:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	−0.64 (0.93)	3.39 (0.35)	0.01	71	14.98 (0.29)	−8.42 (0.31)	0.02	71	−16.75 (0.14)	9.49 (0.12)	0.09	25
2	−2.72 (0.68)	5.09 (0.12)	0.05	71	8.46 (0.54)	−2.89 (0.72)	0.004	71	−14.68 (0.15)	10.01 * (0.09)	0.17	25
3	−3.93 (0.52)	5.45 * (0.07)	0.09	70	4.74 (0.73)	−0.63 (0.93)	0.000	71	−13.22 (0.18)	8.97 * (0.09)	0.20	25
4	−4.48 (0.43)	5.85 * (0.04)	0.12	71	2.72 (0.84)	0.80 (0.91)	0.001	71	−12.50 (0.17)	9.12 * (0.06)	0.26	25
Forecast Horizon	Natural Gas (1990:05–2003:12)
h	C	(Y10-Y1)	R2	N
1	10.73 (0.67)	−0.22 (0.99)	0.000	54
2	2.97 (0.89)	4.30 (0.66)	0.004	54
3	−0.41 (0.98)	6.44 (0.47)	0.02	54
4	−1.84 (0.92)	7.84 (0.30)	0.04	54
Panel C
Forecast Horizon	Oil (2004:01–2020:12)				Silver (2004:01–2020:12)				Gold (2004:01–2020:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	8.26 (0.60)	−3.95 (0.64)	0.002	68	15.22 (0.10)	−4.46 (0.46)	0.006	68	13.82 ** (0.01)	−3.34 (0.29)	0.02	68
2	6.04 (0.65)	−2.99 (0.69)	0.002	67	15.87 * (0.06)	−5.53 (0.34)	0.019	67	14.21 *** (0.01)	−3.52 (0.26)	0.03	67
3	4.29 (0.73)	−2.10 (0.76)	0.002	66	12.56 (0.12)	−3.41 (0.55)	0.012	66	13.88 *** (0.01)	−3.18 (0.29)	0.04	66
4	−0.09 (0.99)	−0.28 (0.97)	0.000	65	9.14 (0.25)	−1.59 (0.78)	0.004	65	13.71 *** (0.004)	−3.12 (0.26)	0.05	65
Forecast Horizon	Platinum (2004:01–2020:12)				Palladium (2004:01–2020:12)				Zinc (2004:01–2020:12)
h	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N	C	(Y10-Y1)	R2	N
1	8.60 (0.24)	−4.83 (0.30)	0.01	68	22.90 ** (0.01)	−5.63 (0.44)	0.01	68	13.37 (0.46)	−4.91 (0.58)	0.01	68
2	9.07 (0.19)	−5.64 (0.23)	0.03	67	24.29 *** (0.004)	−7.25 (0.32)	0.02	67	7.93 (0.65)	−1.80 (0.82)	0.002	67
3	7.55 (0.25)	−4.66 (0.27)	0.03	66	21.46 ** (0.01)	−5.25 (0.43)	0.02	66	3.58 (0.83)	0.97 (0.89)	0.000	66
4	5.67 (0.37)	−3.65 (0.33)	0.03	65	19.62 ** (0.03)	−3.82 (0.54)	0.01	65	0.15 (0.99)	2.97 (0.66)	0.01	65
Forecast Horizon	Natural Gas (2004:01–2020:12)
h	C	(Y10-Y1)	R2	N
1	0.56 (0.97)	−3.69 (0.63)	0.002	68
2	2.48 (0.86)	−4.99 (0.49)	0.01	67
3	1.93 (0.88)	−5.27 (0.41)	0.01	66
4	−0.77 (0.94)	−3.97 (0.47)	0.01	65

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y₁ denotes the yield spread calculated as the difference between the yield rates on 10-year and 1-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

,

Table 7. Estimation results of Equation (1) with the Y₁₀-Y₂ indicator. Panel A: entire sample. Panel B: sample period 1986–2003. Panel C: sample period 2004–2020.

Panel A
Forecast Horizon	Oil (1986:01–2020:12)				Silver (1986:01–2020:12)				Gold (1986:01–2020:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	11.27 (0.20)	−6.39 (0.30)	0.004	139	2.30 (0.70)	2.18 (0.65)	0.001	139	2.96 (0.48)	1.86 (0.50)	0.004	138
2	8.47 (0.27)	−4.25 (0.44)	0.004	138	1.81 (0.75)	2.56 (0.58)	0.004	138	2.87 (0.48)	1.91 (0.47)	0.01	138
3	7.27 (0.32)	−3.41 (0.52)	0.005	137	0.69 (0.90)	3.20 (0.47)	0.01	137	2.61 (0.51)	1.92 (0.45)	0.01	137
4	5.27 (0.45)	−2.29 (0.66)	0.003	136	−0.55 (0.91)	3.99 (0.35)	0.02	136	2.50 (0.51)	1.86 (0.45)	0.01	136
Forecast Horizon	Platinum (1986:04–2020:12)				Palladium (1986:04–2020:12)				Zinc (1997:08–2020:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	3.51 (0.50)	−0.71 (0.85)	0.0002	139	16.64 * (0.06)	−6.94 (0.33)	0.01	139	3.79 (0.76)	−0.54 (0.94)	0.000	93
2	2.90 (0.55)	−0.40 (0.91)	0.0001	138	15.04 * (0.09)	−5.55 (0.42)	0.01	138	1.10 (0.93)	1.78 (0.78)	0.002	92
3	2.18 (0.65)	−0.13 (0.97)	0.000	136	12.77 (0.15)	−3.80 (0.57)	0.01	137	−0.98 (0.93)	3.41 (0.56)	0.01	91
4	1.29 (0.77)	0.49 (0.88)	0.0005	136	10.88 (0.21)	−2.05 (0.74)	0.003	136	−2.64 (0.80)	4.73 (0.38)	0.02	90
Forecast Horizon	Ethanol (2005:06–2020:12)				Coal (2009:01–2020:12)				Natural gas (1990:05–2020:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	1.64 (0.91)	−1.94 (0.84)	0.0004	62	−4.20 (0.82)	3.23 (0.74)	0.003	48	6.92 (0.59)	−4.28 (0.62)	0.002	122
2	−0.35 (0.97)	−1.50 (0.84)	0.001	61	−6.71 (0.69)	4.59 (0.62)	0.01	47	5.23 (0.65)	−3.24 (0.67)	0.002	121
3	−1.33 (0.88)	−1.11 (0.87)	0.001	60	−9.94 (0.51)	6.12 (0.47)	0.03	46	3.88 (0.72)	−2.62 (0.71)	0.002	120
4	−4.08 (0.55)	0.05 (0.99)	0.000	59	−8.80 (0.56)	5.31 (0.53)	0.03	45	1.61 (0.87)	−0.77 (0.90)	0.0003	119
Panel B
Forecast Horizon	Oil (1986:01–2003:12)				Silver (1986:01–2003:12)				Gold (1986:01–2003:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	12.81 (0.24)	−7.33 (0.31)	0.01	71	−6.76 (0.17)	8.23 * (0.07)	0.04	71	−5.11 (0.15)	6.71 ** (0.02)	0.05	70
2	10.36 (0.30)	−4.83 (0.46)	0.01	71	−8.65 * (0.06)	11.18 ** (0.02)	0.13	71	−5.24 * (0.07)	6.93 *** (0.004)	0.13	71
3	8.78 (0.34)	−3.34 (0.57)	0.01	71	−8.73 ** (0.03)	10.73 *** (0.01)	0.18	71	−5.34 ** (0.03)	6.45 *** (0.001)	0.18	71
4	7.32 (0.39)	−1.74 (0.77)	0.003	71	−8.63 *** (0.01)	10.65 *** (0.001)	0.24	71	−5.25 ** (0.03)	6.22 *** (0.00)	0.21	71
Forecast Horizon	Platinum (1986:04–2003:12)				Palladium (1986:04–2003:12)				Zinc (1997:08–2003:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	−0.19 (0.98)	4.41 (0.37)	0.01	71	14.57 (0.25)	−11.71 (0.27)	0.02	71	−14.39 (0.22)	10.23 (0.17)	0.08	25
2	−1.92 (0.77)	6.49 (0.15)	0.05	71	9.81 (0.44)	−5.60 (0.58)	0.01	71	−13.13 (0.19)	11.82 * (0.09)	0.18	25
3	−2.94 (0.62)	6.78 * (0.09)	0.09	70	6.75 (0.59)	−3.07 (0.75)	0.004	71	−12.20 (0.18)	10.99 * (0.06)	0.22	25
4	−3.43 (0.54)	7.32 ** (0.05)	0.13	71	4.49 (0.71)	−0.74 (0.93)	0.0003	71	−11.53 (0.16)	11.25 ** (0.02)	0.29	25
Forecast Horizon	Natural Gas (1990:05–2003:12)
h	C	(Y10-Y2)	R2	N
1	12.09 (0.59)	−1.61 (0.91)	0.0002	54
2	7.03 (0.72)	2.16 (0.85)	0.001	54
3	3.90 (0.83)	4.94 (0.64)	0.01	54
4	1.86 (0.91)	7.49 (0.40)	0.02	54
Panel C
Forecast Horizon	Oil (2004:01–2020:12)				Silver (2004:01–2020:12)				Gold (2004:01–2020:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	9.12 (0.58)	−5.25 (0.61)	0.002	68	14.73 (0.13)	−4.77 (0.53)	0.005	68	13.80 *** (0.01)	−3.85 (0.31)	0.02	68
2	5.56 (0.67)	−3.09 (0.71)	0.002	67	15.76 * (0.08)	−6.31 (0.38)	0.02	67	14.40 *** (0.01)	−4.22 (0.26)	0.03	67
3	4.61 (0.71)	−2.68 (0.73)	0.002	66	13.68 (0.10)	−4.82 (0.49)	0.02	66	14.39 *** (0.01)	−4.07 (0.25)	0.04	66
4	1.36 (0.91)	−1.44 (0.85)	0.001	65	10.82 (0.17)	−3.11 (0.64)	0.01	65	14.36 *** (0.00)	−4.11 (0.22)	0.06	65
Forecast Horizon	Platinum (2004:01–2020:12)				Palladium (2004:01–2020:12)				Zinc (2004:01–2020:12)
h	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N	C	(Y10-Y2)	R2	N
1	7.53 (0.31)	−4.74 (0.36)	0.01	68	21.92 ** (0.02)	−5.74 (0.50)	0.01	68	15.05 (0.41)	−6.99 (0.51)	0.01	68
2	8.12 (0.25)	−5.78 (0.26)	0.02	67	23.87 *** (0.01)	−8.05 (0.33)	0.02	67	10.20 (0.57)	−3.84 (0.69)	0.01	67
3	7.92 (0.23)	−5.67 (0.24)	0.03	66	22.88 *** (0.01)	−7.17 (0.36)	0.03	66	6.35 (0.71)	−1.01 (0.91)	0.001	66
4	6.56 (0.29)	−4.90 (0.25)	0.04	65	21.70 *** (0.01)	−6.01 (0.40)	0.03	65	3.36 (0.84)	0.99 (0.90)	0.001	65
Forecast Horizon	Natural Gas (2004:01–2020:12)
h	C	(Y10-Y2)	R2	N
1	0.76 (0.96)	−4.42 (0.63)	0.002	68
2	2.15 (0.88)	−5.52 (0.52)	0.01	67
3	2.29 (0.86)	−6.38 (0.40)	0.01	66
4	−0.56 (0.96)	−4.76 (0.47)	0.01	65

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y₂ denotes the yield spread calculated as the difference between the yield rates on 10-year and 2-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

,

Table 8. Estimation results of Equation (1) with the Y₃₀-Y_3M indicator. Panel A: entire sample. Panel B: sample period 1986–2003. Panel C: sample period 2004–2020.

Panel A
Forecast Horizon	Oil (1986:01–2020:12)				Silver (1986:01–2020:12)				Gold (1986:01–2020:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	10.14 (0.32)	−2.70 (0.48)	0.002	139	3.39 (0.63)	0.60 (0.84)	0.0003	139	4.43 (0.37)	0.27 (0.88)	0.0002	138
2	8.54 (0.36)	−2.16 (0.54)	0.003	138	2.55 (0.70)	0.95 (0.73)	0.001	138	4.26 (0.37)	0.33 (0.85)	0.001	138
3	7.38 (0.40)	−1.77 (0.60)	0.004	137	0.96 (0.88)	1.49 (0.57)	0.01	137	3.99 (0.38)	0.34 (0.83)	0.001	137
4	4.49 (0.59)	−0.80 (0.80)	0.001	136	−0.53 (0.93)	2.00 (0.42)	0.01	136	3.99 (0.36)	0.27 (0.87)	0.001	136
Forecast Horizon	Platinum (1986:04–2020:12)				Palladium (1986:04–2020:12)				Zinc (1997:08–2020:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	4.83 (0.43)	−0.96 (0.70)	0.001	139	19.20 * (0.07)	−4.65 (0.29)	0.01	139	1.46 (0.92)	0.76 (0.87)	0.0004	93
2	3.92 (0.51)	−0.67 (0.78)	0.001	138	16.41 (0.11)	−3.41 (0.42)	0.01	138	−2.14 (0.87)	2.40 (0.57)	0.01	92
3	1.72 (0.76)	0.15 (0.95)	0.000	136	11.49 (0.27)	−1.33 (0.74)	0.002	137	−5.11 (0.68)	3.66 (0.33)	0.02	91
4	0.34 (0.95)	0.68 (0.73)	0.002	136	8.62 (0.40)	−0.01 (0.99)	0.000	136	−7.57 (0.52)	4.71 (0.17)	0.05	90
Forecast Horizon	Ethanol (2005:06–2020:12)				Coal (2009:01–2020:12)				Natural gas (1990:05–2020:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	1.03 (0.95)	−0.81 (0.89)	0.0002	62	−10.26 (0.62)	4.11 (0.53)	0.01	48	4.35 (0.78)	−1.08 (0.85)	0.0002	122
2	−0.25 (0.98)	−0.87 (0.85)	0.001	61	−13.06 (0.47)	4.96 (0.39)	0.02	47	2.07 (0.88)	−0.28 (0.95)	0.000	121
3	−1.98 (0.84)	−0.33 (0.94)	0.0002	60	−17.59 (0.26)	6.28 (0.22)	0.06	46	−0.74 (0.95)	0.66 (0.89)	0.0003	120
4	−5.05 (0.51)	0.45 (0.90)	0.001	59	−16.06 (0.30)	5.65 (0.27)	0.06	45	−3.24 (0.77)	1.70 (0.68)	0.003	119
Panel B
Forecast Horizon	Oil (1986:01–2003:12)				Silver (1986:01–2003:12)				Gold (1986:01–2003:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	13.64 (0.31)	−3.74 (0.42)	0.01	71	−8.02 (0.14)	4.36 * (0.07)	0.03	71	−5.61 (0.19)	3.29 * (0.05)	0.03	70
2	10.91 (0.35)	−2.46 (0.52)	0.01	71	−10.57 ** (0.04)	6.02 ** (0.03)	0.11	71	−5.54 (0.11)	3.29 ** (0.02)	0.08	71
3	10.37 (0.32)	−2.29 (0.52)	0.01	71	−10.29 ** (0.02)	5.64 *** (0.01)	0.14	71	−5.37 * (0.06)	2.95 *** (0.01)	0.11	71
4	8.64 (0.38)	−1.44 (0.68)	0.005	71	−9.87 *** (0.01)	5.44 *** (0.004)	0.18	71	−4.89* (0.08)	2.65 ** (0.02)	0.11	71
Forecast Horizon	Platinum (1986:04–2003:12)				Palladium (1986:04–2003:12)				Zinc (1997:08–2003:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	−1.58 (0.85)	2.69 (0.34)	0.01	71	13.62 (0.40)	−4.86 (0.43)	0.01	71	−18.45 (0.11)	7.06 * (0.09)	0.10	25
2	−4.02 (0.57)	3.98 (0.10)	0.05	71	6.92 (0.65)	−1.14 (0.85)	0.001	71	−17.37 * (0.09)	7.92 * (0.05)	0.20	25
3	−5.57 (0.39)	4.38 ** (0.04)	0.10	70	1.89 (0.90)	0.97 (0.86)	0.001	71	−16.08 * (0.09)	7.34 ** (0.04)	0.25	25
4	−6.05 (0.30)	4.60 ** (0.02)	0.14	71	−0.58 (0.97)	2.13 (0.69)	0.01	71	−15.39 * (0.08)	7.45 ** (0.02)	0.33	25
Forecast Horizon	Natural gas (1990:05–2003:12)
h	C	(Y30-Y3M)	R2	N
1	10.84 (0.71)	−0.20 (0.98)	0.000	54
2	1.57 (0.95)	3.48 (0.67)	0.01	54
3	−3.79 (0.87)	5.79 (0.43)	0.02	54
4	−6.18 (0.75)	7.15 (0.24)	0.06	54
Panel C
Forecast Horizon	Oil (2004:01–2020:12)				Silver (2004:01–2020:12)				Gold (2004:01–2020:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	5.77 (0.75)	−1.43 (0.82)	0.0004	68	17.50 * (0.09)	−3.79 (0.42)	0.01	68	16.24 *** (0.01)	−3.14 (0.18)	0.03	68
2	5.29 (0.73)	−1.57 (0.78)	0.001	67	18.60 ** (0.04)	−4.66 (0.28)	0.02	67	16.74 *** (0.003)	−3.30 (0.16)	0.05	67
3	3.24 (0.82)	−0.89 (0.87)	0.001	66	15.15 * (0.07)	−3.26 (0.42)	0.02	66	16.34 *** (0.002)	−3.05 (0.16)	0.06	66
4	−1.50 (0.91)	0.41 (0.93)	0.0002	65	11.55 (0.15)	−2.02 (0.60)	0.01	65	16.08 *** (0.001)	−2.98 (0.13)	0.07	65
Forecast Horizon	Platinum (2004:01–2020:12)				Palladium (2004:01–2020:12)				Zinc (2004:01–2020:12)
h	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N	C	(Y30-Y3M)	R2	N
1	11.93 (0.14)	−4.47 (0.21)	0.01	68	27.18 *** (0.01)	−5.38 (0.33)	0.01	68	12.91 (0.54)	−2.90 (0.67)	0.004	68
2	12.74 (0.11)	−5.13 (0.16)	0.04	67	28.83 *** (0.003)	−6.52 (0.22)	0.03	67	6.80 (0.73)	−0.66 (0.92)	0.0004	67
3	9.95 (0.16)	−3.96 (0.19)	0.04	66	24.38 *** (0.01)	−4.56 (0.34)	0.03	66	1.45 (0.94)	1.52 (0.79)	0.003	66
4	7.52 (0.27)	−3.09 (0.24)	0.04	65	21.37 ** (0.04)	−3.16 (0.47)	0.02	65	−2.77 (0.88)	3.11 (0.55)	0.02	65
Forecast Horizon	Natural gas (2004:01–2020:12)
h	C	(Y30-Y3M)	R2	N
1	−1.68 (0.92)	−1.37 (0.81)	0.000	68
2	1.87 (0.91)	−2.89 (0.61)	0.004	67
3	1.14 (0.93)	−3.01 (0.55)	0.01	66
4	−1.64 (0.89)	−2.16 (0.62)	0.01	65

Notes: The forecast horizon (h) is in quarters. Y₃₀-Y_3M denotes the yield spread calculated as the difference between the yield rates on 10-year and 3-month government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

,

Table 9. Estimation results of Equation (1) with the Y₃₀-Y₁ indicator. Panel A: entire sample. Panel C: sample period 2004–2020.

Panel A
Forecast Horizon	Oil (1986:01–2020:12)				Silver (1986:01–2020:12)				Gold (1986:01–2020:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	9.77 (0.28)	−2.90 (0.43)	0.002	139	1.75 (0.79)	1.55 (0.63)	0.002	139	3.58 (0.44)	0.74 (0.69)	0.001	138
2	7.95 (0.34)	−2.18 (0.52)	0.003	138	1.51 (0.81)	1.63 (0.59)	0.004	138	3.40 (0.45)	0.83 (0.65)	0.003	138
3	7.13 (0.36)	−1.90 (0.57)	0.004	137	0.45 (0.94)	1.98 (0.49)	0.01	137	3.09 (0.47)	0.86 (0.62)	0.005	137
4	4.93 (0.51)	−1.15 (0.72)	0.002	136	−0.66 (0.90)	2.37 (0.39)	0.02	136	3.07 (0.46)	0.78 (0.64)	0.01	136
Forecast Horizon	Platinum (1986:04–2020:12)				Palladium (1986:04–2020:12)				Zinc (1997:08–2020:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	3.68 (0.52)	−0.50 (0.84)	0.0002	139	17.84 * (0.07)	−4.63 (0.32)	0.01	139	1.52 (0.91)	0.81 (0.87)	0.0004	93
2	3.41 (0.53)	−0.50 (0.84)	0.0005	138	16.10 * (0.09)	−3.75 (0.40)	0.01	138	−1.001 (0.94)	2.08 (0.64)	0.005	92
3	2.09 (0.69)	−0.03 (0.99)	0.000	136	12.74 (0.18)	−2.18 (0.60)	0.01	137	−3.21 (0.80)	3.09 (0.45)	0.01	91
4	0.96 (0.84)	0.46 (0.83)	0.001	136	10.23 (0.27)	−0.85 (0.83)	0.001	136	−5.19 (0.66)	4.02 (0.28)	0.03	90
Forecast Horizon	Ethanol (2005:06–2020:12)				Coal (2009:01–2020:12)				Natural gas (1990:05–2020:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	−0.37 (0.98)	−0.20 (0.97)	0.000	62	−7.99 (0.70)	3.47 (0.61)	0.01	48	4.98 (0.73)	−1.52 (0.79)	0.0005	122
2	−1.43 (0.90)	−0.38 (0.94)	0.000	61	−10.36 (0.57)	4.21 (0.50)	0.02	47	2.60 (0.84)	−0.58 (0.91)	0.0001	121
3	−1.98 (0.83)	−0.36 (0.93)	0.0002	60	−14.18 (0.38)	5.35 (0.34)	0.04	46	0.99 (0.93)	−0.10 (0.98)	0.000	120
4	−4.89 (0.51)	0.41 (0.91)	0.000	59	−12.75 (0.04)	4.74 (0.39)	0.04	45	−1.07 (0.92)	0.85 (0.84)	0.001	119
Panel B
Forecast Horizon	Oil (1986:01–2003:12)				Silver (1986:01–2003:12)				Gold (1986:01–2003:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	13.74 (0.24)	−4.59 (0.30)	0.01	71	−8.56 * (0.09)	5.60 ** (0.04)	0.04	71	−5.65 (0.16)	4.02 ** (0.03)	0.04	70
2	10.92 (0.30)	−2.99 (0.44)	0.01	71	−10.78 ** (0.03)	7.42 ** (0.02)	0.14	71	−6.00 * (0.07)	4.26 *** (0.005)	0.12	71
3	9.39 (0.32)	−2.20 (0.54)	0.01	71	−10.63 *** (0.01)	7.04 *** (0.003)	0.19	71	−6.14 ** (0.03)	4.03 *** (0.001)	0.17	71
4	7.91 (0.37)	−1.31 (0.71)	0.004	71	−10.29 *** (0.005)	6.85 *** (0.001)	0.24	71	−5.87 ** (0.03)	3.79 *** (0.001)	0.19	71
Forecast Horizon	Platinum (1986:04–2003:12)				Palladium (1986:04–2003:12)				Zinc (1997:08–2003:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	−0.97 (0.90)	2.89 (0.35)	0.01	71	15.75 (0.27)	−7.15 (0.30)	0.02	71	−17.19 (0.15)	7.11 (0.12)	0.09	25
2	−3.10 (0.65)	4.27 (0.12)	0.05	71	9.86 (0.48)	−3.11 (0.64)	0.01	71	−16.06 (0.12)	8.03 * (0.07)	0.19	25
3	−4.49 (0.47)	4.66 * (0.05)	0.10	70	5.65 (0.68)	−1.04 (0.86)	0.001	71	−15.05 (0.11)	7.54 ** (0.04)	0.24	25
4	−5.10 (0.36)	5.02 ** (0.02)	0.15	71	2.85 (0.83)	0.56 (0.92)	0.0004	71	−14.51* (0.09)	7.75 ** (0.02)	0.32	25
Forecast Horizon	Natural gas (1990:05–2003:12)
h	C	(Y30-Y1)	R2	N
1	12.74 (0.62)	−1.23 (0.90)	0.0002	54
2	4.83 (0.83)	2.35 (0.77)	0.002	54
3	0.74 (0.97)	4.38 (0.54)	0.01	54
4	−1.10 (0.95)	5.68 (0.34)	0.03	54
Panel C
Forecast Horizon	Oil (2004:01–2020:12)				Silver (2004:01–2020:12)				Gold (2004:01–2020:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	4.55 (0.79)	−0.99 (0.87)	0.0002	68	15.55 (0.13)	−3.23 (0.52)	0.01	68	15.62 *** (0.01)	−3.15 (0.23)	0.02	68
2	3.67 (0.80)	−0.97 (0.86)	0.0004	67	17.50 * (0.06)	−4.59 (0.33)	0.02	67	16.35 *** (0.003)	−3.44 (0.18)	0.05	67
3	3.55 (0.79)	−1.12 (0.83)	0.001	66	15.24 * (0.07)	−3.62 (0.41)	0.02	66	16.21 *** (0.002)	−3.29 (0.17)	0.06	66
4	−0.10 (0.99)	−0.19 (0.97)	0.000	65	12.42 (0.12)	−2.61 (0.53)	0.02	65	16.13 *** (0.001)	−3.29 (0.13)	0.08	65
Forecast Horizon	Platinum (2004:01–2020:12)				Palladium (2004:01–2020:12)				Zinc (2004:01–2020:12)
h	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N	C	(Y30-Y1)	R2	N
1	8.85 (0.27)	−3.45 (0.31)	0.01	68	22.91 ** (0.03)	−3.90 (0.48)	0.01	68	12.24 (0.55)	−2.86 (0.69)	0.004	68
2	10.67 (0.15)	−4.65 (0.18)	0.03	67	25.87 *** (0.01)	−5.76 (0.28)	0.02	67	7.79 (0.69)	−1.18 (0.86)	0.001	67
3	9.57 (0.17)	−4.17 (0.18)	0.04	66	23.83 *** (0.01)	−4.74 (0.33)	0.03	66	3.82 (0.84)	0.57 (0.93)	0.0004	66
4	7.76 (0.23)	−3.51 (0.19)	0.05	65	21.88 ** (0.03)	−3.70 (0.41)	0.02	65	0.47 (0.98)	1.92 (0.73)	0.01	65
Forecast Horizon	Natural Gas (2004:01–2020:12)
h	C	(Y30-Y1)	R2	N
1	−3.02 (0.86)	−0.87 (0.88)	0.0002	68
2	−0.63 (0.97)	−2.01 (0.71)	0.002	67
3	−0.26 (0.98)	−2.66 (0.59)	0.01	66
4	−2.82 (0.81)	−1.83 (0.68)	0.004	65

Notes: The forecast horizon (h) is in quarters. Y₃₀-Y₁ denotes the yield spread calculated as the difference between the yield rates on 10-year and 1-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

and

Table 10. Estimation results of Equation (1) with the Y₃₀-Y₂ indicator. Panel A: entire sample. Panel B: sample period 1986–2003. Panel C: sample period 2004–2020.

Panel A
Forecast Horizon	Oil (1986:01–2020:12)				Silver (1986:01–2020:12)				Gold (1986:01–2020:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	10.05 (0.24)	−3.63 (0.37)	0.003	139	0.99 (0.87)	2.32 (0.53)	0.003	139	2.96 (0.50)	1.28 (0.55)	0.003	138
2	7.63 (0.33)	−2.40 (0.52)	0.003	138	0.94 (0.87)	2.30 (0.51)	0.01	138	2.85 (0.49)	1.33 (0.51)	0.01	138
3	6.90 (0.34)	−2.12 (0.56)	0.004	137	0.30 (0.96)	2.45 (0.46)	0.01	137	2.52 (0.53)	1.38 (0.47)	0.01	137
4	5.09 (0.46)	−1.47 (0.68)	0.003	136	−0.39 (0.94)	2.66 (0.40)	0.02	136	2.51 (0.51)	1.29 (0.48)	0.01	136
Forecast Horizon	Platinum (1986:04–2020:12)				Palladium (1986:04–2020:12)				Zinc (1997:08–2020:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	3.25 (0.56)	−0.33 (0.91)	0.000	139	16.08 * (0.09)	−4.42 (0.40)	0.01	139	1.81 (0.89)	0.75 (0.89)	0.0003	93
2	3.20 (0.54)	−0.47 (0.87)	0.0003	138	15.32 * (0.09)	−4.00 (0.42)	0.01	138	−0.29 (0.98)	1.95 (0.69)	0.003	92
3	2.49 (0.62)	−0.28 (0.92)	0.0002	136	13.01 (0.15)	−2.77 (0.55)	0.01	137	−2.13 (0.86)	2.90 (0.52)	0.01	91
4	1.64 (0.72)	0.12 (0.96)	0.0001	136	10.71 (0.23)	−1.32 (0.76)	0.002	136	−3.72 (0.75)	3.73 (0.36)	0.02	90
Forecast Horizon	Ethanol (2005:06–2020:12)				Coal (2009:01–2020:12)				Natural Gas (1990:05–2020:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	−1.17 (0.94)	0.20 (0.98)	0.000	62	−7.47 (0.71)	3.59 (0.63)	0.01	48	6.34 (0.65)	−2.57 (0.68)	0.001	122
2	−2.25 (0.84)	0.01 (0.99)	0.000	61	−8.79 (0.64)	3.94 (0.58)	0.01	47	4.53 (0.72)	−1.80 (0.75)	0.001	121
3	−1.75 (0.85)	−0.51 (0.92)	0.0003	60	−12.05 (0.48)	4.97 (0.44)	0.03	46	3.33 (0.77)	−1.46 (0.78)	0.001	120
4	−4.24 (0.58)	0.12 (0.98)	0.000	59	−10.28 (0.54)	4.16 (0.52)	0.03	45	1.17 (0.91)	−0.28 (0.95)	0.000	119
Panel B
Forecast Horizon	Oil (1986:01–2003:12)				Silver (1986:01–2003:12)				Gold (1986:01–2003:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	13.76 (0.20)	−6.08 (0.22)	0.01	71	−7.65 (0.11)	6.70 ** (0.04)	0.05	71	−5.00 (0.20)	4.81 ** (0.02)	0.04	70
2	10.50 (0.28)	−3.63 (0.41)	0.01	71	−9.78 ** (0.03)	9.03 *** (0.01)	0.15	71	−5.45 * (0.08)	5.21 *** (0.002)	0.13	71
3	8.50 (0.33)	−2.22 (0.58)	0.01	71	−9.99 *** (0.01)	8.81 *** (0.001)	0.23	71	−5.83 ** (0.03)	5.09 *** (0.0001)	0.21	71
4	6.63 (0.41)	−0.73 (0.86)	0.001	71	−9.64 *** (0.01)	8.55 *** (0.000)	0.29	71	−5.68 ** (0.02)	4.87 *** (0.00)	0.23	71
Forecast Horizon	Platinum (1986:04–2003:12)				Palladium (1986:04–2003:12)				Zinc (1997:08–2003:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	−0.44 (0.95)	3.41 (0.37)	0.01	71	14.97 (0.25)	−8.85 (0.27)	0.02	71	−15.13 (0.20)	7.27 (0.16)	0.08	25
2	−2.22 (0.74)	4.96 (0.14)	0.06	71	10.69 (0.40)	−4.78 (0.54)	0.01	71	−14.62 (0.14)	8.84 * (0.06)	0.19	25
3	−3.44 (0.57)	5.33 * (0.07)	0.10	70	7.10 (0.56)	−2.52 (0.72)	0.005	71	−14.04 (0.12)	8.85 ** (0.02)	0.26	25
4	−3.99 (0.46)	5.77 ** (0.02)	0.14	71	4.21 (0.72)	−0.32 (0.96)	0.000	71	−13.54 * (0.09)	8.84 *** (0.004)	0.34	25
Forecast Horizon	Natural Gas (1990:05–2003:12)
h	C	(Y30-Y2)	R2	N
1	13.81 (0.55)	−2.31 (0.84)	0.001	54
2	8.26 (0.69)	0.71 (0.94)	0.0001	54
3	4.46 (0.81)	3.12 (0.69)	0.005	54
4	2.21 (0.89)	5.08 (0.43)	0.02	54
Panel C
Forecast Horizon	Oil (2004:01–2020:12)				Silver (2004:01–2020:12)				Gold (2004:01–2020:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	4.49 (0.79)	−1.07 (0.87)	0.000	68	15.00 (0.17)	−3.28 (0.59)	0.004	68	15.76 *** (0.01)	−3.55 (0.25)	0.02	68
2	2.74 (0.85)	−0.59 (0.92)	0.0001	67	17.44 * (0.07)	−5.04 (0.36)	0.02	67	16.72 *** (0.004)	−3.98 (0.18)	0.05	67
3	3.61 (0.78)	−1.27 (0.82)	0.001	66	16.49 * (0.06)	−4.63 (0.37)	0.03	66	16.89 *** (0.002)	−3.98 (0.15)	0.07	66
4	1.10 (0.93)	−0.82 (0.88)	0.001	65	14.29 * (0.07)	−3.83 (0.42)	0.03	65	16.95 *** (0.00)	−4.05 (0.11)	0.10	65
Forecast Horizon	Platinum (2004:01–2020:12)				Palladium (2004:01–2020:12)				Zinc (2004:01–2020:12)
h	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N	C	(Y30-Y2)	R2	N
1	7.76 (0.35)	−3.24 (0.37)	0.01	68	21.83 * (0.05)	−3.74 (0.54)	0.004	68	13.28 (0.52)	−3.69 (0.64)	0.01	68
2	9.88 (0.19)	−4.73 (0.19)	0.02	67	25.47 *** (0.01)	−6.15 (0.28)	0.02	67	9.62 (0.63)	−2.25 (0.76)	0.003	67
3	10.02 (0.16)	−4.84 (0.16)	0.04	66	25.20 *** (0.01)	−5.94 (0.27)	0.03	66	6.23 (0.75)	−0.61 (0.93)	0.0003	66
4	8.70 (0.17)	−4.35 (0.14)	0.05	65	23.84 *** (0.01)	−5.08 (0.29)	0.03	65	3.33 (0.86)	0.67 (0.92)	0.001	65
Forecast Horizon	Natural Gas (2004:01–2020:12)
h	C	(Y30-Y2)	R2	N
1	−3.61 (0.84)	−0.66 (0.92)	0.000	68
2	−1.65 (0.92)	−1.69 (0.78)	0.001	67
3	−0.53 (0.97)	−2.79 (0.62)	0.005	66
4	−3.15 (0.79)	−1.85 (0.71)	0.003	65

Notes: The forecast horizon (h) is in quarters. Y₃₀-Y₂ denotes the yield spread calculated as the difference between the yield rates on 10-year and 2-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

report the estimation results of the prediction model. Table 6 presents the estimation results given Y₁₀-Y₁ as the yield spread. In Table 7, Y₁₀-Y₂ proxies for the yield spread. In Table 8, Y₃₀-Y_3M proxies for the yield spread. Table 9 utilizes Y₃₀-Y₁ as the yield spread, and Table 10 utilizes Y₃₀-Y₂ to proxy for the yield spread. The overall picture is maintained as evident by the significant positive coefficients in the period prior to 2004 (Panel B in each table), but the insignificant results in the period that follows (Panels C). The regression results that include the other explanatory variables reflect very similar picture. They appear in

Table A3. Estimation results of Equation (1) with the Y₁₀-Y₁ indicator. Panel A: sample period 1986–2003. Panel B: sample period 2004–2020.

Panel A

Forecast Horizon

Oil (1986:01–2003:12)

Silver (1986:01–2003:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

10.68

−5.04

0.38

−2.13

10.18

0.04

−0.06

72

−12.36

10.33 ** (0.03)

0.09

−11.41

−3.41

0.06

0.02

72

2

9.29

−2.61

−9.56 * (0.07)

−5.23

9.22

0.00

0.00

72

−10.04 * (0.05)

8.40 *** (0.01)

0.54

−6.30

−1.82

−0.01

0.05

72

3

7.75

−0.90

−5.37

−4.32

1.16

0.03

−0.03

72

−10.75 *** (0.01)

8.55 *** (0.00)

0.40

−9.64 * (0.09)

−2.06

0.00

0.13

72

4

6.47

−0.83

−3.00

−7.62

1.38

0.04

−0.03

72

−10.23 *** (0.00)

8.30 *** (0.00)

−0.13

−7.98 * (0.09)

−1.94

0.00

0.18

72

Forecast Horizon

Gold (1986:01–2003:12)

Platinum (1986:04–2003:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−8.47

5.17

4.41

−10.64

3.55

0.01

0.03

70

−3.87

4.24

−1.97

−22.85

7.19

−0.07

0.05

72

2

−4.65

5.43 *** (0.01)

−1.55

2.89

−4.56

−0.01

0.07

70

−4.31

4.94 * (0.08)

−1.66

−14.42 ** (0.03)

3.87

−0.03

0.07

72

3

−4.99 * (0.05)

4.71 *** (0.00)

−0.59

0.26

−2.62

−0.01

0.09

70

−5.63

5.03 ** (0.02)

0.32

−10.42 * (0.05)

6.87

−0.01

0.11

72

4

−5.03 ** (0.03)

4.71 *** (0.00)

−0.98

−0.86

−3.38

−0.01

0.12

70

−5.16

5.25 *** (0.01)

−0.22

−5.71

5.58

−0.02

0.11

72

Forecast Horizon

Palladium (1986:04–2003:12)

Zinc (1997:08–2003:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

11.59

−7.16

−14.61 * (0.05)

−12.90

33.00 ** (0.04)

−0.09

0.08

72

−21.33 * (0.06)

9.16

8.23

−25.35

11.31

0.03

0.10

26

2

7.05

−5.81

−3.70

−11.63

31.04 *** (0.01)

−0.07

0.06

72

−16.97 ** (0.04)

7.48

6.01

−27.80

6.11

0.01

0.16

26

3

3.32

−4.93

3.38

−4.13

36.35 *** (0.00)

−0.02

0.14

72

−15.35 ** (0.02)

7.55 ** (0.04)

3.84

−25.00 * (0.09)

−0.50

0.08

0.23

26

4

1.79

−3.31

3.58

−4.30

30.21 *** (0.00)

−0.02

0.11

72

−15.03 *** (0.01)

8.66 *** (0.01)

2.34

−12.80

3.54

0.09

0.28

26

Forecast Horizon

Natural gas (1990:05–2003:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

4.59

−4.38

18.18

−12.43

36.19

0.01

−0.04

55

2

−3.53

3.48

5.06

−33.81

22.93

−0.03

−0.03

55

3

−12.73

7.90

5.30

−49.78 *** (0.00)

23.53 * (0.09)

0.11

0.15

55

4

−9.98

8.53

4.29

−31.91 ** (0.02)

14.60

0.09

0.10

55

Panel B

Forecast Horizon

Oil (2004:01–2020:12)

Silver (2004:01–2020:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

0.01

−0.71

0.87

16.72

−15.96 ** (0.04)

0.17

0.04

67

13.07

−6.08

−2.52

−14.07

−4.75

0.08

−0.03

67

2

3.06

−2.22

0.81

9.33

−6.02 * (0.09)

0.07

−0.03

66

15.64 * (0.09)

−4.56

−7.99

−11.44

−3.10

−0.06

0.06

66

3

2.19

−1.56

−6.05

−16.46

−6.38

−0.04

0.04

65

10.99

−2.58

−4.89

−11.82

−4.20

−0.04

0.06

65

4

1.64

−1.67

−1.05

−1.99

8.65

−0.04

−0.05

64

11.94 * (0.05)

−1.64

−6.86 * (0.06)

3.29

−0.10

−0.06

0.03

64

Forecast Horizon

Gold (2004:01–2020:12)

Platinum (2004:01–2020:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

12.91 ** (0.03)

−2.92

−0.23

3.82

−1.73

0.02

−0.03

67

6.12

−5.08

−5.07

0.34

−1.76

0.12

−0.03

67

2

14.64 *** (0.00)

−3.10

−3.25

−0.10

−0.54

−0.03

0.02

66

9.76

−5.21

−10.51 ** (0.02)

−1.47

1.68

−0.01

0.04

66

3

14.14 *** (0.00)

−2.91

−1.65

1.01

−0.19

−0.02

−0.02

65

7.63

−4.39

−4.44

−9.72

−1.78

−0.05

0.06

65

4

15.12 *** (0.00)

−2.78

−2.98

5.36

−2.01

−0.03

0.07

64

7.83

−4.00

−3.69

1.85

2.06

−0.05

0.03

64

Forecast Horizon

Palladium (2004:01–2020:12)

Zinc (2004:01–2020:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

24.57 * (0.09)

−9.53

−7.76

−6.41

5.78

0.10

−0.02

67

9.45

−5.33

−15.72 ** (0.03)

−15.73

2.51

0.21 ** (0.04)

0.06

67

2

26.18 ** (0.02)

−7.80

−8.83

−15.73

2.36

−0.06

0.02

66

6.95

−1.46

−6.89

−9.66

0.83

0.03

−0.05

66

3

23.33 *** (0.01)

−5.92

−2.31

−12.83

0.82

−0.07

−0.01

65

2.55

1.68

−5.68

−3.06

−1.41

0.01

−0.05

65

4

21.35 *** (0.00)

−3.68

−3.65

−4.26

1.01

−0.07

−0.02

64

1.63

2.63

−2.57

10.56

3.89

−0.01

−0.05

64

Forecast Horizon

Natural Gas (2004:01–2020:12)

h

C

(Y10-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

1.86

−3.96

17.37

28.99

0.32

−0.02

−0.01

67

2

−0.63

−4.66

2.84

−7.03

−4.58

0.06

−0.05

66

3

−2.01

−4.66

−1.41

−18.54

−5.74

0.04

−0.01

65

4

−1.42

−5.19

−0.79

−10.76

10.45

0.03

−0.01

64

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y₁ denotes the yield spread calculated as the difference between the yield rates on 10-year and 1-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

,

Table A4. Estimation results of Equation (1) with the Y₁₀-Y₂ indicator. Panel A: sample period 1986–2003. Panel B: sample period 2004–2020.

Panel A

Forecast Horizon

Oil (1986:01–2003:12)

Silver (1986:01–2003:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

10.51

−6.94

0.47

−2.11

9.52

0.04

−0.06

72

−11.02

13.14 ** (0.02)

−0.01

−11.22

−1.76

0.06

0.03

72

2

8.54

−2.87

−9.57 * (0.07)

−5.37

8.69

0.00

0.00

72

−9.15 * (0.05)

10.90 *** (0.00)

0.44

−6.19

−0.53

−0.01

0.07

72

3

6.92

−0.37

−5.42

−4.51

0.81

0.03

−0.03

72

−10.21 *** (0.01)

11.50 *** (0.00)

0.26

−9.61 * (0.08)

−0.86

0.00

0.17

72

4

5.07

0.34

−3.11

−7.94

0.88

0.04

−0.03

72

−9.70*** (0.00)

11.15 *** (0.00)

−0.26

−7.95 * (0.08)

−0.77

0.00

0.23

72

Forecast Horizon

Gold (1986:01–2003:12)

Platinum (1986:04–2003:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−8.04

6.88 * (0.08)

4.32

−10.59

4.28

0.01

0.04

70

−3.70

5.81

−2.05

−22.86 ** (0.03)

7.76

−0.07

0.05

72

2

−4.12

7.13 *** (0.00)

−1.63

2.96

−3.76

−0.01

0.09

70

−3.63

6.24 * (0.07)

−1.71

−14.32 ** (0.03)

4.67

−0.03

0.07

72

3

−4.85 ** (0.03)

6.54 *** (0.00)

−0.69

0.25

−2.02

−0.01

0.13

70

−5.00

6.41 ** (0.02)

0.27

−10.32 * (0.05)

7.67 * (0.07)

−0.01

0.12

72

4

−4.94 *** (0.01)

6.59 *** (0.00)

−1.08

−0.89

−2.80

−0.01

0.18

70

−4.48

6.69 *** (0.01)

−0.27

−5.61

6.42 * (0.09)

−0.02

0.12

72

Forecast Horizon

Palladium (1986:04–2003:12)

Zinc (1997:08–2003:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

9.00

−7.31

−14.69

−13.43

31.39

−0.09

0.07

72

−19.80 * (0.06)

10.45

8.30

−24.92

12.87

0.03

0.10

26

2

6.24

−7.35

−3.65

−11.75

30.10

−0.07

0.06

72

−16.40 ** (0.04)

9.25 * (0.08)

5.98

−27.08

7.58

0.00

0.17

26

3

2.72

−6.32

3.43

−4.21

35.57

−0.02

0.14

72

−14.85 ** (0.02)

9.42 ** (0.03)

3.79

−24.23 * (0.09)

1.01

0.08

0.26

26

4

1.38

−4.23

3.61

−4.36

29.68

−0.02

0.11

72

−14.53 *** (0.01)

10.91 *** (0.00)

2.28

−11.87

5.29

0.08

0.33

26

Forecast Horizon

Natural Gas (1990:05–2003:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

4.28

−5.76

18.25

−12.59

35.60

0.01

−0.04

55

2

−0.05

1.51

5.22

−32.94

23.99

−0.04

−0.03

55

3

−8.32

6.74

5.43

−48.61 *** (0.01)

25.29 * (0.07)

0.10

0.13

55

4

−6.13

8.14

4.38

−30.86 ** (0.03)

16.34

0.08

0.08

55

Panel B

Forecast Horizon

Oil (2004:01–2020:12)

Silver (2004:01–2020:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

0.64

−1.32

0.90

16.67

−15.94 ** (0.04)

0.17

0.04

67

12.44

−6.54

−2.47

−14.23

−4.82

0.08

−0.03

67

2

2.39

−2.04

0.81

9.29

−6.07

0.07

−0.03

66

15.43 * (0.09)

−5.12

−7.94

−11.58

−3.15

−0.06

0.05

66

3

2.44

−2.01

−6.02

−16.53

−6.38 * (0.09)

−0.04

0.04

65

12.12

−3.87

−4.83

−11.96

−4.17

−0.04

0.06

65

4

2.98

−2.99

−0.97

−2.09

8.80

−0.04

−0.05

64

13.44 ** (0.03)

−3.08

−6.78 * (0.06)

3.18

0.08

−0.06

0.04

64

Forecast Horizon

Gold (2004:01–2020:12)

Platinum (2004:01–2020:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

12.82 ** (0.04)

−3.31

−0.20

3.73

−1.76

0.02

−0.03

67

4.78

−4.82

−5.04

0.25

−1.84

0.12

−0.03

67

2

14.76 *** (0.00)

−3.68

−3.21

−0.21

−0.56

−0.03

0.02

66

8.53

−5.06

−10.48 ** (0.02)

−1.57

1.59

−0.01

0.04

66

3

14.61 *** (0.00)

−3.74

−1.60

0.89

−0.20

−0.02

−0.01

65

7.96

−5.35

−4.37

−9.88

−1.82

−0.05

0.06

65

4

15.67 *** (0.00)

−3.66 * (0.09)

−2.93

5.24

−2.01

−0.03

0.08

64

8.58 * (0.09)

−5.23

−3.62

1.69

2.05

−0.05

0.04

64

Forecast Horizon

Palladium (2004:01–2020:12)

Zinc (2004:01–2020:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

23.20

−9.94

−7.69

−6.65

5.65

0.10

−0.02

67

10.90

−7.32

−15.61 ** (0.03)

−15.98

2.51

0.21 ** (0.04)

0.06

67

2

25.65 ** (0.02)

−8.61

−8.75

−15.96

2.27

−0.06

0.02

66

9.24

−3.49

−6.80

−9.83

0.89

0.03

−0.05

66

3

24.84 *** (0.01)

−8.04

−2.20

−13.10

0.83

−0.08

0.00

65

5.34

−0.22

−5.64

−3.14

−1.25

0.01

−0.05

65

4

23.42 *** (0.00)

−5.90

−3.52

−4.47

1.21

−0.07

−0.01

64

4.68

0.63

−2.45

10.55

4.42

−0.01

−0.06

64

Forecast Horizon

Natural Gas (2004:01–2020:12)

h

C

(Y10-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

2.17

−4.83

17.42

28.85

0.30

−0.02

−0.01

67

2

−0.89

−5.19

2.89

−7.17

−4.63

0.06

−0.05

66

3

−1.55

−5.77

−1.34

−18.72

−5.77

0.04

−0.01

65

4

−1.25

−6.14

−0.74

−10.95

10.32

0.03

−0.01

64

Notes: The forecast horizon (h) is in quarters. Y₁₀-Y₂ denotes the yield spread calculated as the difference between the yield rates on 10-year and 2-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

,

Table A5. Estimation results of Equation (1) with the Y₃₀-Y_3M indicator. Panel A: sample period 1986–2003. Panel B: sample period 2004–2020.

Panel A

Forecast Horizon

Oil (1986:01–2003:12)

Silver (1986:01–2003:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

12.77

−4.45

0.56

−1.91

11.52

0.04

−0.06

72

−13.32

7.41 ** (0.03)

−0.04

−11.21

−4.69

0.05

0.01

72

2

9.63

−1.93

−9.52 * (0.07)

−5.25

9.60

0.00

0.00

72

−11.32 ** (0.04)

6.28 *** (0.01)

0.39

−6.24

−3.08

−0.02

0.05

72

3

8.10

−0.78

−5.34

−4.29

1.39

0.03

−0.03

72

−12.25 *** (0.01)

6.50 *** (0.00)

0.24

−9.61 * (0.09)

−3.43

−0.01

0.14

72

4

6.17

−0.41

−3.02

−7.71

1.32

0.04

−0.03

72

−11.34 *** (0.00)

6.13 *** (0.00)

−0.26

−7.89 * (0.09)

−3.12

−0.01

0.17

72

Forecast Horizon

Gold (1986:01–2003:12)

Platinum (1986:04–2003:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−7.98

3.22

4.39

−10.34

3.31

0.01

0.02

70

−5.10

3.47

−2.09

−22.94

6.29

−0.07

0.05

72

2

−4.92

3.77 *** (0.01)

−1.60

3.03

−5.13

−0.02

0.06

70

−5.62

3.98 * (0.06)

−1.79

−14.49 ** (0.03)

2.88

−0.04

0.08

72

3

−5.12 * (0.06)

3.22 *** (0.01)

−0.63

0.41

−3.06

−0.02

0.07

70

−6.89 * (0.08)

4.02 *** (0.01)

0.20

−10.48 ** (0.04)

5.88

−0.01

0.12

72

4

−4.96 ** (0.04)

3.11 *** (0.00)

−1.00

−0.68

−3.73

−0.01

0.09

70

−6.42 * (0.07)

4.17 *** (0.01)

−0.34

−5.76

4.59

−0.02

0.12

72

Forecast Horizon

Palladium (1986:04–2003:12)

Zinc (1997:08–2003:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

10.62

−4.30

−14.65 * (0.05)

−13.36

33.17 ** (0.04)

−0.08

0.07

72

−21.63 * (0.07)

6.28

7.90

−25.51

9.97

0.03

0.09

26

2

7.04

−3.89

−3.67

−11.85

31.52 *** (0.01)

−0.07

0.06

72

−18.24 ** (0.04)

5.68 * (0.09)

5.59

−27.52

5.02

0.01

0.17

26

3

3.00

−3.14

3.38

−4.38

36.62 *** (0.00)

−0.01

0.13

72

−17.30 *** (0.01)

6.09 ** (0.02)

3.31

−24.44 * (0.09)

−1.61

0.08

0.27

26

4

0.66

−1.64

3.51

−4.65

29.98 *** (0.00)

−0.02

0.11

72

−17.16 *** (0.01)

6.94 *** (0.00)

1.75

−12.20

2.27

0.09

0.34

26

Forecast Horizon

Natural Gas (1990:05–2003:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

7.71

−4.40

18.39

−11.95

37.52

0.01

−0.04

55

2

−3.67

2.41

5.02

−33.69

22.62

−0.03

−0.03

55

3

−14.50

6.16

5.13

−49.82 *** (0.00)

22.36

0.10

0.15

55

4

−13.07

7.20 * (0.07)

4.04

−32.21 ** (0.02)

12.97

0.09

0.11

55

Panel B

Forecast Horizon

Oil (2004:01–2020:12)

Silver (2004:01–2020:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−3.50

1.08

0.76

16.84

−16.06 ** (0.04)

0.17

0.04

67

15.85

−5.05

−2.31

−14.20

−4.77

0.08

−0.03

67

2

2.08

−0.98

0.81

9.35

−6.09

0.07

−0.03

66

17.66 * (0.08)

−3.76

−7.83

−11.53

−3.12

−0.06

0.06

66

3

0.66

−0.34

−6.05

−16.43

−6.48 * (0.09)

−0.04

0.04

65

13.20

−2.58

−4.77

−11.91

−4.17

−0.04

0.06

65

4

0.65

−0.63

−1.06

−1.98

8.48

−0.04

−0.05

64

13.85 * (0.05)

−1.87

−6.70 * (0.06)

3.26

0.23

−0.06

0.04

64

Forecast Horizon

Gold (2004:01–2020:12)

Platinum (2004:01–2020:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

15.19 ** (0.03)

−2.83

−0.11

3.73

−1.71

0.02

−0.03

67

9.44

−4.65

−4.86

0.20

−1.75

0.12

−0.02

67

2

16.84 *** (0.00)

−2.91

−3.12

−0.19

−0.52

−0.03

0.04

66

12.79

−4.61

−10.31 ** (0.02)

−1.60

1.68

−0.01

0.05

66

3

16.44 *** (0.00)

−2.83 * (0.08)

−1.52

0.92

−0.16

−0.02

0.01

65

9.74

−3.69

−4.28

−9.82

−1.80

−0.05

0.07

65

4

17.09 *** (0.00)

−2.63 * (0.05)

−2.79

5.32

−1.67

−0.03

0.09

64

9.50 * (0.09)

−3.28

−3.48

1.81

2.35

−0.05

0.04

64

Forecast Horizon

Palladium (2004:01–2020:12)

Zinc (2004:01–2020:12)

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

29.84 * (0.07)

−8.31

−7.40

−6.65

5.77

0.10

−0.01

67

8.58

−2.99

−15.64 ** (0.03)

−15.73

2.41

0.21

0.05

67

2

30.47 *** (0.01)

−6.79

−8.53

−15.93

2.35

−0.06

0.03

66

5.39

−0.25

−6.91

−9.61

0.76

0.03

−0.05

66

3

26.31 *** (0.01)

−5.03

−2.10

−12.97

0.81

−0.07

0.00

65

−0.13

2.21

−5.79

−2.97

−1.49

0.01

−0.04

65

4

22.79 *** (0.01)

−2.97

−3.46

−4.30

1.26

−0.07

−0.02

64

−1.41

3.00

−2.82

10.61

3.38

−0.01

−0.04

64

Forecast Horizon

Natural gas (2004:01–2020:12))

h

C

(Y30-M3)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

0.34

−1.84

17.39

29.02

0.22

−0.02

−0.01

67

2

−1.16

−2.72

2.91

−7.04

−4.67

0.06

−0.06

66

3

−2.99

−2.55

−1.33

−18.55

−5.89

0.04

−0.01

65

4

−1.73

−3.17

−0.66

−10.78

10.40

0.03

−0.02

64

Notes: The forecast horizon (h) is in quarters. Y₃₀-Y_3M denotes the yield spread calculated as the difference between the yield rates on 10-year and 3-month government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

,

Table A6. Estimation results of Equation (1) with the Y₃₀-Y₁ indicator. Panel A: sample period 1986–2003. Panel B: sample period 2004–2020.

Panel A

Forecast Horizon

Oil (1986:01–2003:12)

Silver (1986:01–2003:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

11.73

−4.60

0.50

−1.93

10.11

0.04

−0.06

72

−13.51 * (0.08)

8.82 *** (0.01)

−0.08

−11.60

−2.95

0.06

0.03

72

2

9.08

−1.93

−9.55 * (0.07)

−5.29

8.95

0.00

0.00

72

−11.34 ** (0.03)

7.39 *** (0.00)

0.37

−6.54

−1.56

−0.01

0.08

72

3

7.17

−0.36

−5.41

−4.46

0.90

0.03

−0.03

72

−12.32 *** (0.00)

7.68 *** (0.00)

0.21

−9.93 * (0.07)

−1.88

0.00

0.19

72

4

5.40

−0.01

−3.08

−7.86

0.97

0.04

−0.04

72

−11.52 *** (0.00)

7.31 *** (0.00)

−0.30

−8.22 * (0.07)

−1.69

0.00

0.24

72

Forecast Horizon

Gold (1986:01–2003:12)

Platinum (1986:04–2003:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−8.48

4.09

4.35

−10.60

3.93

0.01

0.03

70

−4.59

3.77

−2.06

−22.99 ** (0.03)

7.30

−0.07

0.06

72

2

−4.99

4.49 *** (0.00)

−1.62

2.85

−4.25

−0.01

0.09

70

−4.77

4.17 * (0.06)

−1.74

−14.49 ** (0.03)

4.12

−0.03

0.08

72

3

−5.71 ** (0.02)

4.16 *** (0.00)

−0.69

0.14

−2.48

−0.01

0.13

70

−6.35 * (0.08)

4.39 *** (0.01)

0.22

−10.54 ** (0.04)

7.05 * (0.09)

−0.01

0.13

72

4

−5.69 *** (0.01)

4.11 *** (0.00)

−1.07

−0.97

−3.23

−0.01

0.16

70

−5.93 * (0.07)

4.60 *** (0.00)

−0.32

−5.85

5.76

−0.02

0.13

72

Forecast Horizon

Palladium (1986:04–2003:12)

Zinc (1997:08–2003:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

10.57

−5.02

−14.64 * (0.05)

−13.17

32.11 ** (0.04)

−0.09

0.08

72

−21.84 * (0.06)

6.90

8.09

−25.65

11.71

0.03

0.10

26

2

7.38

−4.78

−3.63

−11.59

30.69 *** (0.01)

−0.07

0.07

72

−18.30 ** (0.03)

6.16 * (0.08)

5.78

−27.69

6.57

0.00

0.18

26

3

3.17

−3.79

3.40

−4.19

35.91 *** (0.00)

−0.02

0.14

72

−17.09 *** (0.01)

6.44 ** (0.02)

3.55

−24.73 * (0.08)

0.02

0.08

0.28

26

4

1.10

−2.19

3.55

−4.48

29.72 *** (0.00)

−0.02

0.11

72

−17.03 *** (0.00)

7.41 *** (0.00)

2.01

−12.49

4.14

0.08

0.35

26

Forecast Horizon

Natural gas (1990:05–2003:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

6.68

−4.48

18.32

−12.18

36.38

0.01

−0.04

55

2

−1.62

1.67

5.15

−33.25

23.58

−0.04

−0.03

55

3

−11.31

5.34

5.33

−49.12 *** (0.01)

24.34 * (0.08)

0.10

0.14

55

4

−8.91

6.01

4.30

−31.30 ** (0.03)

15.38

0.09

0.09

55

Panel B

Forecast Horizon

Oil (2004:01–2020:12)

Silver (2004:01–2020:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−3.42

1.15

0.77

16.91

−16.04 ** (0.04)

0.17

0.04

67

14.08

−4.73

−2.40

−14.42

−4.86

0.08

−0.03

67

2

0.85

−0.50

0.77

9.36

−6.13

0.07

−0.03

66

16.73 * (0.09)

−3.71

−7.89

−11.72

−3.19

−0.06

0.06

66

3

1.44

−0.75

−6.04

−16.50

−6.46 * (0.09)

−0.04

0.04

65

13.60 * (0.09)

−3.04

−4.77

−12.10

−4.19

−0.03

0.06

65

4

1.53

−1.12

−1.02

−2.06

8.57

−0.04

−0.05

64

14.52 ** (0.03)

−2.39

−6.70 * (0.06)

3.10

0.22

−0.06

0.04

64

Forecast Horizon

Gold (2004:01–2020:12)

Platinum (2004:01–2020:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

14.67 ** (0.03)

−2.87

−0.14

3.57

−1.76

0.02

−0.03

67

6.63

−3.78

−4.97

0.07

−1.86

0.12

−0.03

67

2

16.43 *** (0.00)

−3.02

−3.15

−0.36

−0.57

−0.03

0.04

66

10.72

−4.10

−10.40 ** (0.02)

−1.77

1.58

−0.01

0.04

66

3

16.26 *** (0.00)

−3.04 * (0.07)

−1.54

0.74

−0.21

−0.02

0.01

65

9.37

−3.91

−4.31

−10.04

−1.87

−0.05

0.07

65

4

17.07 *** (0.00)

−2.89 ** (0.04)

−2.83

5.14

−1.82

−0.03

0.10

64

9.44 * (0.08)

−3.58 * (0.09)

−3.53

1.58

2.15

−0.05

0.05

64

Forecast Horizon

Palladium (2004:01–2020:12)

Zinc (2004:01–2020:12)

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

25.07

−6.88

−7.61

−6.89

5.57

0.11

−0.02

67

8.66

−3.34

−15.66 ** (0.03)

−15.93

2.38

0.21 ** (0.04)

0.05

67

2

27.23 ** (0.02)

−5.95

−8.68

−16.16

2.20

−0.06

0.02

66

6.70

−0.90

−6.87

−9.71

0.79

0.03

−0.05

66

3

25.42 *** (0.01)

−5.14

−2.15

−13.24

0.70

−0.07

0.00

65

2.66

1.13

−5.71

−2.99

−1.35

0.01

−0.05

65

4

23.11 *** (0.00)

−3.43

−3.49

−4.53

1.13

−0.07

−0.02

64

1.69

1.82

−2.61

10.69

4.00

−0.01

−0.05

64

Forecast Horizon

Natural gas (2004:01–2020:12))

h

C

(Y30-Y1)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−1.61

−1.10

17.31

29.03

0.16

−0.02

−0.01

67

2

−3.34

−1.97

2.82

−7.08

−4.75

0.06

−0.06

66

3

−3.84

−2.42

−1.37

−18.66

−5.96

0.04

−0.01

65

4

−3.23

−2.78

−0.79

−10.94

10.00

0.03

−0.02

64

Notes: The forecast horizon (h) is in quarters. Y₃₀-Y₁ denotes the yield spread calculated as the difference between the yield rates on 10-year and 1-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

and

Table A7. Estimation results of Equation (1) with the Y₃₀-Y₂ indicator. Panel A: sample period 1986–2003. Panel B: sample period 2004–2020.

Panel A

Forecast Horizon

Oil (1986:01–2003:12)

Silver (1986:01–2003:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

11.32

−5.65

0.56

−1.99

9.36

0.04

−0.06

72

−11.97 * (0.09)

10.26 *** (0.01)

−0.13

−11.33

−1.33

0.06

0.04

72

2

8.40

−1.97

−9.57 * (0.07)

−5.43

8.52

0.00

0.00

72

−10.23 ** (0.03)

8.73 *** (0.00)

0.31

−6.35

−0.24

−0.01

0.09

72

3

6.51

0.05

−5.46

−4.60

0.70

0.03

−0.03

72

−11.45 *** (0.00)

9.29 *** (0.00)

0.12

−9.80 * (0.07)

−0.58

0.00

0.22

72

4

4.35

0.81

−3.17

−8.10

0.73

0.04

−0.03

72

−10.68 *** (0.00)

8.84 *** (0.00)

−0.38

−8.09 * (0.07)

−0.44

0.00

0.28

72

Forecast Horizon

Gold (1986:01–2003:12)

Platinum (1986:04–2003:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−7.86

4.87 * (0.09)

4.29

−10.48

4.62

0.01

0.03

70

−4.23

4.62

−2.11

−22.94 ** (0.03)

7.92

−0.07

0.06

72

2

−4.30

5.30 *** (0.00)

−1.66

2.97

−3.45

−0.01

0.10

70

−4.00

4.81 * (0.05)

−1.76

−14.35 ** (0.03)

4.89

−0.04

0.08

72

3

−5.34 ** (0.02)

5.13 *** (0.00)

−0.75

0.19

−1.82

−0.01

0.16

70

−5.61 * (0.09)

5.12 *** (0.01)

0.19

−10.41 ** (0.04)

7.85 * (0.06)

−0.01

0.13

72

4

−5.36 *** (0.00)

5.10 *** (0.00)

−1.14

−0.92

−2.58

−0.01

0.21

70

−5.15 * (0.09)

5.36 *** (0.00)

−0.35

−5.71

6.60 * (0.08)

−0.02

0.14

72

Forecast Horizon

Palladium (1986:04–2003:12)

Zinc (1997:08–2003:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

8.32

−4.76

−14.73 ** (0.04)

−13.64

30.87 ** (0.04)

−0.08

0.07

72

−20.44 * (0.07)

7.38

8.17

−25.51

12.79

0.03

0.09

26

2

6.49

−5.51

−3.60

−11.75

29.80 *** (0.01)

−0.07

0.07

72

−17.71 ** (0.03)

7.05 * (0.07)

5.76

−27.27

7.72

0.00

0.19

26

3

2.48

−4.39

3.43

−4.32

35.21 *** (0.00)

−0.02

0.14

72

−16.55 *** (0.01)

7.43 ** (0.02)

3.52

−24.26 * (0.09)

1.24

0.08

0.30

26

4

0.64

−2.48

3.56

−4.56

29.30 *** (0.00)

−0.02

0.11

72

−16.49 *** (0.00)

8.59 *** (0.00)

1.96

−11.91

5.56

0.08

0.39

26

Forecast Horizon

Natural Gas (1990:05–2003:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

6.22

−5.39

18.38

−12.43

35.71

0.01

−0.04

55

2

1.23

0.21

5.31

−32.64

24.24

−0.04

−0.03

55

3

−7.52

4.24

5.48

−48.20 *** (0.01)

25.63 * (0.07)

0.10

0.13

55

4

−5.53

5.36

4.41

−30.44 ** (0.03)

16.70

0.08

0.07

55

Panel B

Forecast Horizon

Oil (2004:01–2020:12)

Silver (2004:01–2020:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−3.48

1.30

0.75

16.95

−16.03 ** (0.04)

0.17

0.04

67

13.47

−4.90

−2.36

−14.55

−4.93

0.08

−0.03

67

2

−0.15

−0.03

0.74

9.42

−6.15

0.07

−0.03

66

16.55 * (0.09)

−4.01

−7.84

−11.85

−3.23

−0.06

0.06

66

3

1.47

−0.84

−6.02

−16.53

−6.47 * (0.09)

−0.04

0.04

65

14.86 * (0.07)

−4.02

−4.68

−12.32

−4.20

−0.03

0.07

65

4

2.57

−1.79

−0.95

−2.18

8.67

−0.04

−0.05

64

16.11 ** (0.02)

−3.48

−6.58 * (0.06)

2.90

0.34

−0.06

0.05

64

Forecast Horizon

Gold (2004:01–2020:12)

Platinum (2004:01–2020:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

14.78 ** (0.03)

−3.23

−0.09

3.45

−1.79

0.02

−0.03

67

5.38

−3.52

−4.97

0.03

−1.93

0.12

−0.03

67

2

16.70 *** (0.00)

−3.48

−3.10

−0.50

−0.60

−0.03

0.04

66

9.64

−3.96

−10.39 ** (0.02)

−1.84

1.51

−0.01

0.04

66

3

16.88 *** (0.00)

−3.69 * (0.05)

−1.48

0.58

−0.24

−0.02

0.02

65

9.76

−4.53

−4.24

−10.22

−1.92

−0.05

0.07

65

4

17.75 *** (0.00)

−3.55 ** (0.03)

−2.76

4.97

−1.83

−0.03

0.11

64

10.18 * (0.06)

−4.35 * (0.07)

−3.45

1.39

2.12

−0.05

0.05

64

Forecast Horizon

Palladium (2004:01–2020:12)

Zinc (2004:01–2020:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

23.62

−6.84

−7.57

−7.03

5.47

0.11

−0.03

67

9.55

−4.16

−15.58 ** (0.03)

−16.12

2.35

0.21 ** (0.04)

0.05

67

2

26.66 ** (0.03)

−6.28

−8.62

−16.34

2.11

−0.06

0.02

66

8.53

−1.96

−6.79

−9.89

0.81

0.04

−0.05

66

3

26.79 *** (0.01)

−6.41

−2.03

−13.55

0.66

−0.07

0.01

65

5.05

0.00

−5.65

−3.13

−1.27

0.01

−0.05

65

4

24.98 *** (0.00)

−4.78

−3.35

−4.79

1.26

−0.07

−0.01

64

4.33

0.61

−2.48

10.59

4.39

−0.01

−0.06

64

Forecast Horizon

Natural Gas (2004:01–2020:12)

h

C

(Y30-Y2)

∆SP

∆EX

∆IP

∆EPU

R2

N

1

−2.07

−0.97

17.31

29.02

0.14

−0.02

−0.01

67

2

−4.18

−1.73

2.81

−7.08

−4.79

0.06

−0.06

66

3

−3.91

−2.64

−1.33

−18.75

−6.00

0.04

−0.01

65

4

−3.57

−2.90

−0.78

−11.03

9.87

0.03

−0.03

64

Notes: The forecast horizon (h) is in quarters. Y₃₀-Y₂ denotes the yield spread calculated as the difference between the yield rates on 10-year and 2-year government bonds. The table reports the estimation results of Equation (1) with the Newey and West (1987) procedure. The sample period appears separately for each commodity. Figures in parentheses denote estimated standard errors. ***, ** and * denote statistical significance at the 1%, 5%, and 10% levels, respectively.

in the online Appendix A.

A closer glance at the results in Panel B of Table 6 confirms that the yield spread, defined as Y₁₀-Y₁, is an efficient predictor of the future prices of silver, gold, platinum and zinc. The resulting R² for silver ranges between 0.03 when forecasting one quarter ahead (h = 1), and 0.19 when forecasting the prices one year ahead (h = 4). We find that the regression R² for gold ranges between 0.04 (for h = 1) and 0.15 (for h = 4), for platinum it ranges between 0.01 (for h = 1) and 0.12 (for h = 4), and finally it ranges between 0.09 (for h = 1) and 0.26 for zinc (h = 4). This picture is essentially replicated in Table 7, Table 8, Table 9 and Table 10. Moreover, Panel B of Table 10 provides strong support for these findings. The resulting R² for zinc ranges between 0.08 (for h = 1), and 0.34 (for h = 4). By and large, these findings confirm the premise that metal prices are positively correlated with macroeconomic activity (e.g., Fama and French 1988).

6.2. Commodity Prices following Periods of Non-Positive Yield Spreads

What happens to the price of commodities in the months following downward-sloped or flat yield curves? To answer this question, we track the evolution in the price of the sampled commodities throughout the sample period. The period between 1986 and December 2020 witnessed eight periods during which the yield spread (10-year minus 1-year yields) was negative or equal to zero. The left-hand side of

Table 11. Commodity prices in the periods following non-positive yield spread. Panel A: oil. Panel B: silver. Panel C: gold. Panel D: platinum. Panel E: palladium. Panel F: zinc. Panel G: ethanol. Panel H: coal. Panel I: natural gas.

Panel A
Periods Associated with Non-Positive Yield Spreads		Return 1Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
25/01/1989	30/06/1989	−0.69%	7.65%	0.05%	−15.79%	82.98%	40.31%
04/08/1989	13/10/1989	10.72%	−14.89%	−12.11%	90%	47.34%	2.82%
17/03/2000	28/04/2000	9.48%	27.2%	12.9%	9.83%	4.97%	−13.95%
27/12/2005	29/03/2006	10.64%	−5.33%	−8.13%	−0.63%	6.37%	22.89%
05/06/2006	05/06/2007	15.42%	33.35%	59.3%	94.77%	61.91%	−37.8%
20/07/2007	08/08/2007	32.31%	27.19%	71.43%	59.67%	−15.4%	−45.17%
23/05/2019	03/06/2019	1.3%	5.35%	−11.4%	−30.87%	−22.31%	−14.29%
05/08/2019	08/10/2019	13.26%	−52.33%	−22.29%	−21.74%
	Average	11.56%	3.52%	11.22%	23.15%	23.69%	−6.46%
Panel B
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
25/01/1989	30/06/1989	3.01%	2.29%	−3.39%	−5.2%	−8.63%	−17.61%
04/08/1989	13/10/1989	4.82%	−0.62%	−4.51%	−15.27%	−17.18%	−22.41%
17/03/2000	28/04/2000	0.81%	−3.63%	−2.76%	−12.16%	−15.28%	−14.46%
27/12/2005	29/03/2006	−6.68%	4.22%	16.82%	20.47%	11.56%	25.71%
05/06/2006	05/06/2007	−11.74%	3.32%	49.76%	24.31%	−11.35%	−31.94%
20/07/2007	08/08/2007	17.81%	29.92%	27.52%	16.4%	−24.35%	−2.58%
23/05/2019	03/06/2019	29.44%	16.18%	16.21%	23.88%	81.38%	63.41%
05/08/2019	08/10/2019	2.64%	−14.1%	7.8%	34.89%
	Average	5.01%	4.7%	13.43%	10.92%	2.31%	0.02%
Panel C
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
25/01/1989	30/06/1989	−2.68%	7.39%	−1.88%	−4.61%	2.46%	5.01%
04/08/1989	13/10/1989	14.15%	3.47%	0.66%	6.66%	10.54%	−0.55%
17/03/2000	28/04/2000	1.09%	−3.75%	−4.33%	−3.79%	−2.66%	1.6%
27/12/2005	29/03/2006	2.72%	4.41%	11.29%	15.38%	13.54%	29.57%
05/06/2006	05/06/2007	2.2%	19.1%	47.63%	30.2%	19.3%	12.08%
20/07/2007	08/08/2007	24.17%	36.16%	30.78%	27.18%	8.85%	32.31%
23/05/2019	03/06/2019	17.15%	11.76%	24.32%	30.43%	45.93%	38.87%
05/08/2019	08/10/2019	4.21%	11.23%	21.6%	26.14%
	Average	7.88%	11.22%	16.26%	15.95%	13.99%	16.98%
Panel D
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
25/01/1989	30/06/1989	−0.42%	−1.73%	−4.32%	−2.18%	−13.93%	−17.58%
04/08/1989	13/10/1989	3.49%	−0.83%	−0.99%	−13.79%	−13.63%	−17.16%
17/03/2000	28/04/2000	13.43%	13.6%	19.99%	18.93%	1%	−15.14%
27/12/2005	29/03/2006	11.96%	5.97%	5.79%	15.39%	18.77%	29.56%
05/06/2006	05/06/2007	−1.99%	13.05%	75.25%	54.95%	5.31%	−39.39%
20/07/2007	08/08/2007	14.14%	45.92%	58.18%	20.8%	−34.01%	−22.86%
23/05/2019	03/06/2019	16.41%	11.05%	5.9%	5.82%	8.37%	26.52%
05/08/2019	08/10/2019	8.32%	−17.59%	−1.28%	−3.06%
	Average	8.17%	8.68%	19.82%	12.11%	−4.02%	−8.01%
Panel E
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
25/01/1989	30/06/1989	−8.94%	−13.75%	−17.41%	−25.11%	−38.73%	−48.28%
04/08/1989	13/10/1989	−0.93%	−6.91%	−16.05%	−32.23%	−35.82%	−29.44%
17/03/2000	28/04/2000	24.56%	21.82%	69.84%	10.91%	−26.72%	−44.09%
27/12/2005	29/03/2006	−6.35%	−5.45%	1.15%	6.38%	10.12%	5.17%
05/06/2006	05/06/2007	−9.21%	−4.44%	52.74%	15.44%	−26.12%	−55.75%
20/07/2007	08/08/2007	3.72%	21.13%	19.77%	−9.12%	−38.45%	−43.44%
23/05/2019	03/06/2019	16.71%	39.16%	83.09%	48.74%	74.85%	75.24%
05/08/2019	08/10/2019	24.88%	26.93%	17.42%	45.57%
	Average	5.55%	9.81%	26.32%	7.57%	−11.55%	−20.08%
Panel F
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
17/03/2000	28/04/2000	0.02%	−9.09%	−9.56%	−17.29%	−27%	−34.66%
27/12/2005	29/03/2006	19.53%	27.26%	64.03%	24.16%	28%	17.24%
05/06/2006	05/06/2007	−25.82%	−36.91%	−26.28%	−49.13%	−54.42%	−72.24%
20/07/2007	08/08/2007	−20.16%	−30.17%	−37.26%	−52.14%	−69.52%	−66.48%
23/05/2019	03/06/2019	−14.05%	−14.01%	−23.99%	−21.75%	−3.41%	6.25%
05/08/2019	08/10/2019	4.25%	−17.65%	−8.67%	1.14%
	Average	−6.04%	−13.43%	−6.96%	−19.17%	−25.27%	−29.98%
Panel G
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
27/12/2005	29/03/2006	35.07%	−29.26%	−0.08%	−9.02%	−21.84%	−37.88%
05/06/2006	05/06/2007	−22.17%	−7.31%	11.04%	10.47%	2.36%	−34.06%
20/07/2007	08/08/2007	0.54%	14.36%	40.65%	10.57%	−6.67%	−12.47%
23/05/2019	03/06/2019	−12.49%	−2.91%	−16.66%	−23.33%	−9.78%	−7.47%
05/08/2019	08/10/2019	−7.42%	−38.07%	−5.69%	−3.26%
	Average	−1.29%	−12.64%	5.85%	−2.91%	−8.98%	−22.97%
Panel H
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
23/05/2019	03/06/2019	−9.22%	−7.99%	−9.62%	−24.16%	−32.15%	0.41%
05/08/2019	08/10/2019	0.3%	−6.35%	−21.45%	−13.3%
	Average	−4.46%	−7.17%	−15.54%	−18.73%	−32.15%	0.41%
Panel I
Periods Associated with Non-Positive Yield Spreads		Return 1 Q Later	Return 2 Qs. Later	Return 3Qs. Later	Return 4Qs. Later	Return 5Qs. Later	Return 6Qs. Later
Start	End
17/03/2000	28/04/2000	22.41%	44.57%	100.35%	54.95%	0.83%	1.94%
27/12/2005	29/03/2006	−15.18%	−22.3%	−12.91%	5.2%	−6.36%	−5.02%
05/06/2006	05/06/2007	−28.01%	−10.9%	20.8%	55.25%	−7.63%	−28.79%
20/07/2007	08/08/2007	24%	33.46%	81.08%	32.6%	8.63%	−22.72%
23/05/2019	03/06/2019	−1.87%	1.58%	−25.09%	−26.05%	3.5%	4.33%
05/08/2019	08/10/2019	−6.42%	−22.07%	−20.28%	14.82%
	Average	−0.85%	4.06%	23.99%	22.79%	−0.21%	−10.05%

Notes: the tables report the commodity returns accumulated after 1, 2, 3, 4, 5 and 6 quarters following downward-sloped or flat yield curves.

lists the dates when the yield spread became non-positive. For each commodity, we calculate the returns accumulated 1, 2, 3, 4, 5 and 6 quarters following these non-positive yield spread periods.

Oil, silver, gold, platinum, palladium, and natural gas prices surged strongly in the quarters following the periods associated with equality in long and short-term Treasury yields. For example, tracking the prices of these commodities three quarters after an end in the zero slope in the bond term structure reveals significant positive returns on average (oil 11.22%; silver 13.43%; gold 16.26%; platinum 19.82%; palladium 26.32%; ethanol 5.85%; natural gas 24%). In contrast, coal and zinc prices present a mixed and inconclusive picture with a tendency to negative returns. This finding emphasizes that investors should note that flat or downward-sloped yield curves seem to be reasonable points at which to take long positions in several commodities that they plan to hold for a relatively long period of time.

Our findings are even more pronounced if we consider the recent relatively flat yield curve observed during the last week in February 2020 due to the outbreak of the coronavirus. However, we did not include the findings in the table because the difference between the 10-year and 1-year bond interest rates was 0.03% (0.0003). While quite small, it is not a non-positive yield. In addition, prices recovered sharply after two to four quarters. Nevertheless, the findings in Table A1 in the online Appendix A lend support to our conjecture.

7. Conclusions

We investigated an important, yet barely discussed, issue: Can yield spreads forecast future innovations in the commodity market? If so, is this long-term correlation stable over time? Despite the extensive research linking economic real activity to lagged yield spreads, the predictive ability of the yield curve has not been proven with regard to commodities often used in as raw materials.

Our findings can be summarized as follows. First, the prediction ability of the yield curve is evident mainly in the period before the financialization of commodities era, but is absent between 2004 and 2020. Second, structural break tests confirm the changes in the correlation between the six yield spreads proposed and future commodity prices. Third, the findings of the dynamic conditional correlation confirm the time-varying nature of the yield spread in predicting the future evolution in commodity prices. One explanation might be the increased flow of money into the commodity market and the increased correlation between it and equity markets. These changes disconnected the prices of commodities from the economic cycle.

The structural breaks and the fading correlation between the variables of interest are critical for those involved in risk management and investment diversification. Furthermore, our results may be useful for policy makers who must make decisions about policies to target and control inflation. Future research can extend the standing literature by addressing the interplay between the shape of the term structure and future evolution of asset prices in the wake of pandemic outbreaks and the massive monetary intervention conducted by central banks under severe economic conditions.