A Fractal Approach to Nonlinear Topographical Features of Healthy and Keratoconus Corneas Pre- and Post-Operation of Intracorneal Implants
Abstract
1. Introduction
2. Materials and Methods
2.1. Inclusion/Exclusion Criteria
2.2. Pentacam-Derived Topometric Data
2.3. Fractal Dimension Analysis
2.3.1. Higuchi Fractal Dimension
2.3.2. Katz Fractal Dimension
2.3.3. Generalized Hurst Exponent
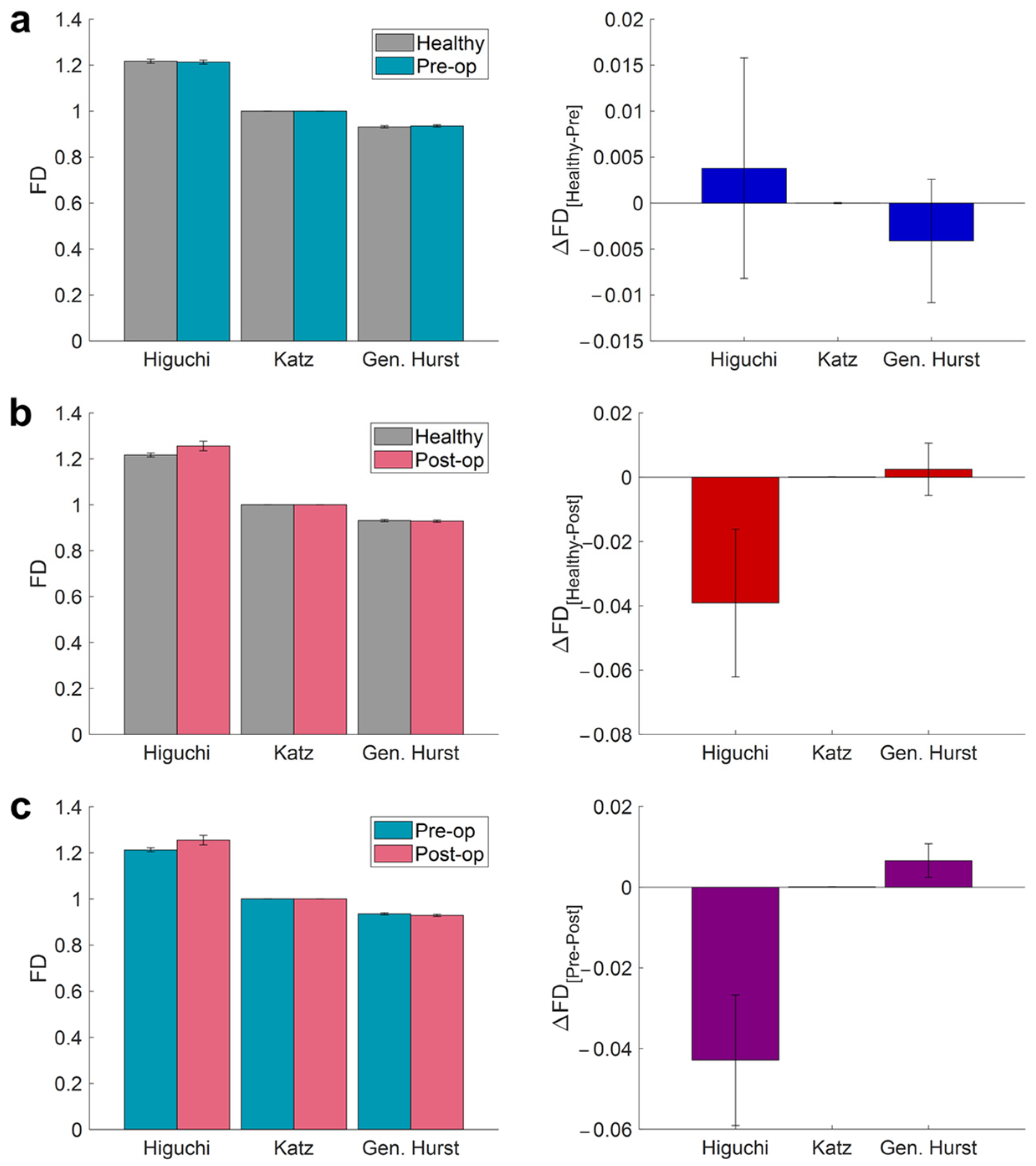
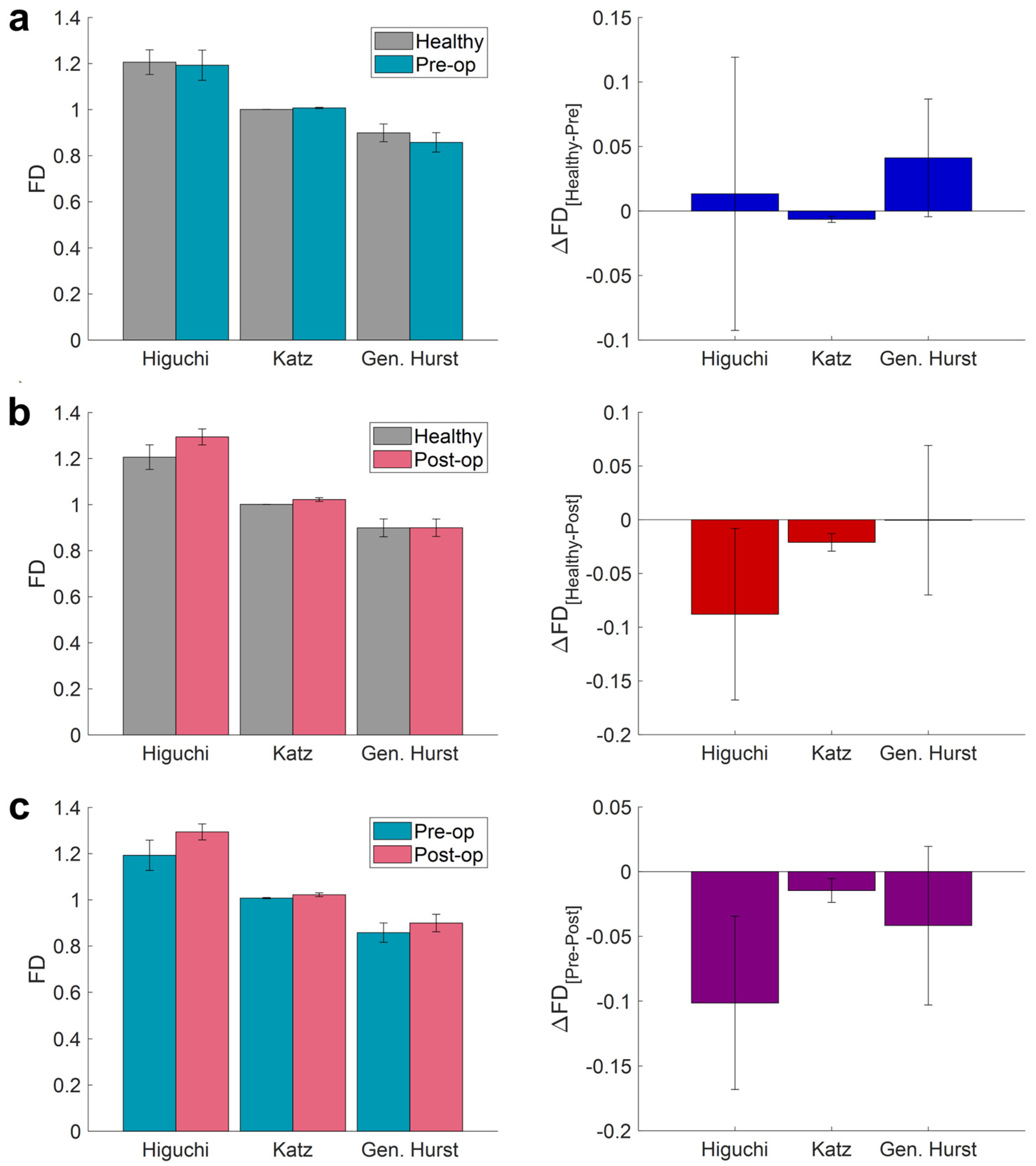
3. Results
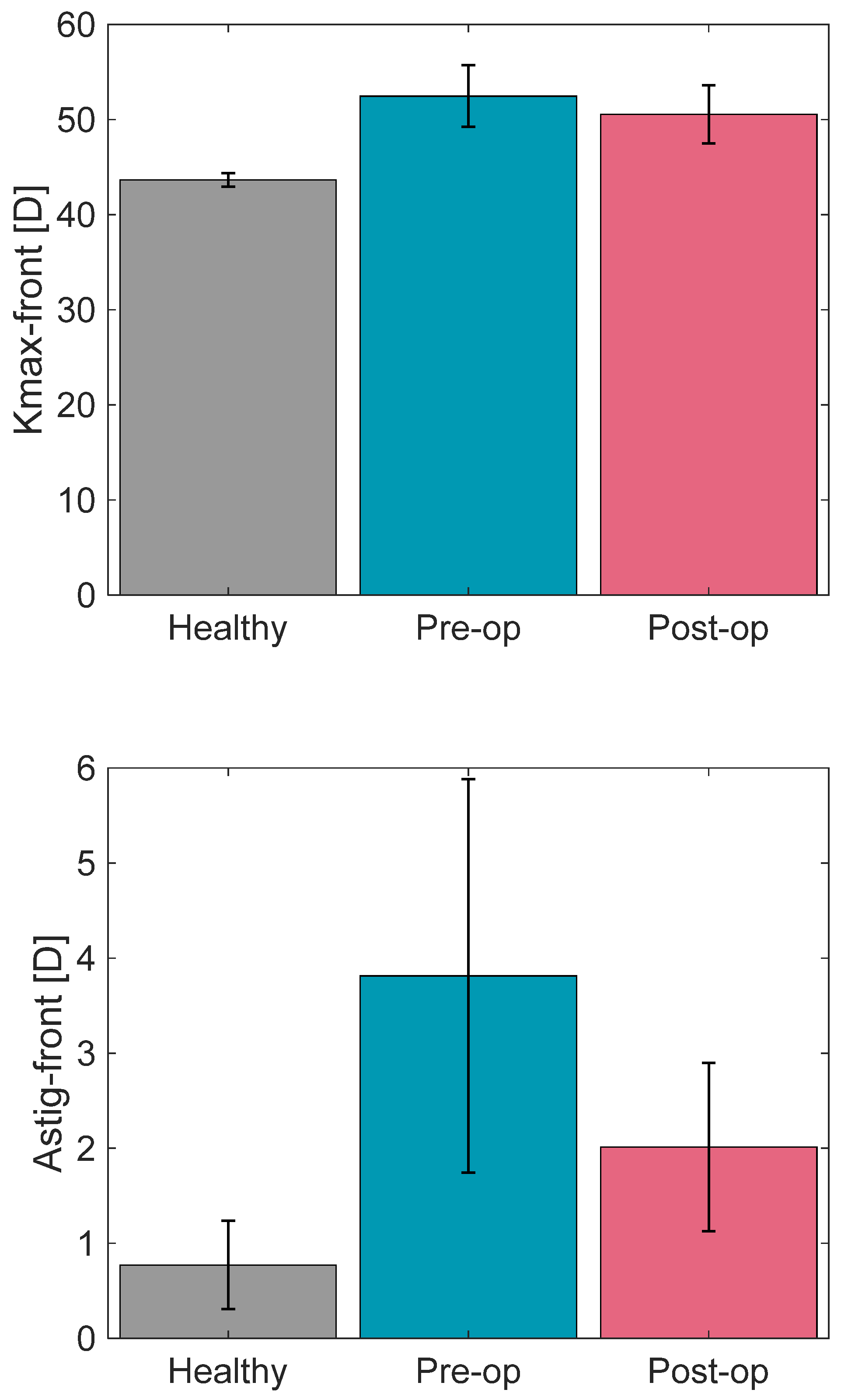
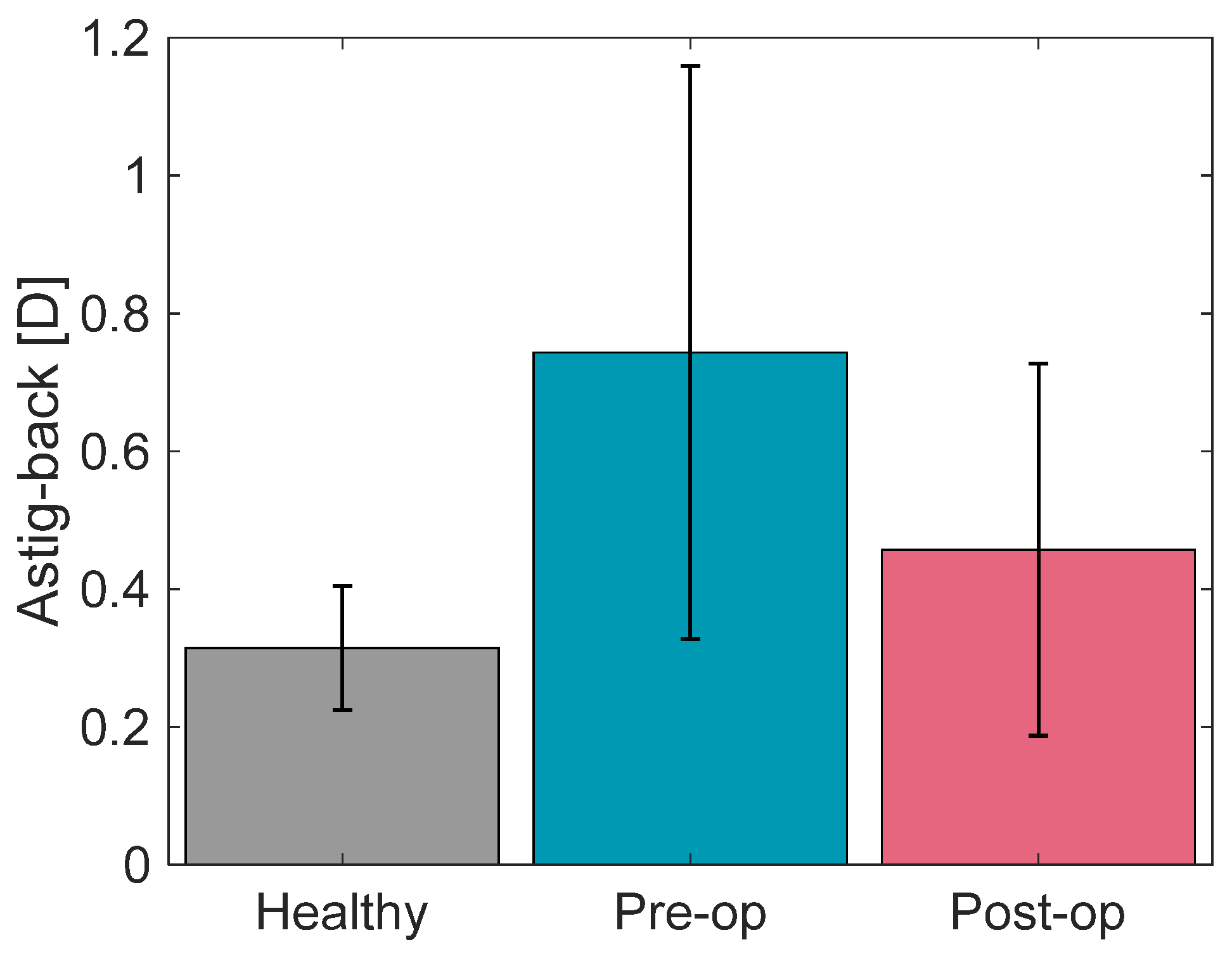
3.1. Pentacam-Derived Topometric Data
3.2. Fractal Dimensions
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Namazi, H.; Jafari, S. Decoding of wrist movements direction by fractal analysis of Magnetoen-cephalography (MEG) signal. Fractals 2019, 27, 1950001. [Google Scholar] [CrossRef]
- Namazi, H.; Akhavan Farid, A.; Seng, C.T. Complexity-based analysis of of the influence of tool geometry on cutting forces in rough end milling. Fractals 2018, 26, 1850078. [Google Scholar] [CrossRef]
- Namazi, H.; Kiminezhadmalaie, M. Diagnosis of lung cancer by fractal analysis of damaged DNA. Comput. Math. Methods Med. 2015, 2015, 242695. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Meyer, M.; Stiedl, O. Self-affine fractal variability of human heartbeat interval dynamics in health and disease. Eur. J. Appl. Physiol. 2003, 90, 305–316. [Google Scholar] [CrossRef]
- Smits, F.M.; Porcaro, C.; Cottone, C.; Cancelli, A. Electroencephalographic Fractal Dimension in Healthy Ageing and Alzheimer’s Disease. PLoS ONE 2016, 11, e0149587. [Google Scholar] [CrossRef]
- Peng, C.; Havlin, S.; Hausdorff, J.-J.M.; Mietus, J.E.; Stanley, H.E.; Goldberger, A.L. Fractal Mechanisms and Heart Rate Dynamics Long-range Correlations and Their Breakdown With Disease. J. Electrocardiol. 1995, 28, 59–65. [Google Scholar] [CrossRef]
- Goldberger, A.L.; Amaral, L.A.N.; Hausdorff, J.M.; Ivanov, P.C.; Peng, C.; Stanley, H.E. Fractal dynamics in physiology: Alterations with disease and aging. Proc. Natl. Acad. Sci. USA 2002, 99, 2466–2472. [Google Scholar] [CrossRef]
- Firtion, E.; Peng, C.K.; Hausdorff, J.M.; Mitchell, S.L.; Cudkowicz, M.E.; Wei, J.Y.; Goldberger, A.R.Y.L.; Jeffrey, M.; Mitchell, S.L. Altered fractal dynamics of gait: Reduced stride-interval correlations with aging and Huntington’s disease. J. Appl. Physiol. 1997, 82, 262–269. [Google Scholar] [CrossRef]
- Nayak, S.R.; Mishra, J.; Khandual, A.; Palai, G. Fractal dimension of RGB color images. Opt. Int. J. Light Electron. Opt. 2018, 162, 196–205. [Google Scholar] [CrossRef]
- Swartz, T.; Marten, L.; Wang, M. Measuring the cornea: The latest developments in corneal topography. Curr. Opin. Ophthalmol. 2007, 18, 325–333. [Google Scholar] [CrossRef]
- de Sanctis, U.; Loiacono, C.; Richiardi, L.; Turco, D.; Mutani, B.; Grignolo, F.M. Sensitivity and Specificity of Posterior Corneal Elevation Measured by Pentacam in Discriminating Keratoconus/Subclinical Keratoconus. Ophthalmology 2008, 115, 1534–1539. [Google Scholar] [CrossRef] [PubMed]
- Flecha-Lescún, J.; Calvo, B.; Zurita, J.; Ariza-Gracia, M.Á. Template-based methodology for the simulation of intracorneal segment ring implantation in human corneas. Biomech. Model. Mechanobiol. 2018, 17, 923–938. [Google Scholar] [CrossRef] [PubMed]
- Godefrooij, D.A.; de Wit, G.A.; Uiterwaal, C.S.; Imhof, S.M.; Wisse, R.P.L. Age-specific Incidence and Prevalence of Keratoconus: A Nationwide Registration Study. Am. J. Ophthalmol. 2017, 175, 169–172. [Google Scholar] [CrossRef] [PubMed]
- Rabinowitz, Y.S. Keratoconus. Surv. Ophthalmol. 1998, 42, 297–319. [Google Scholar] [CrossRef]
- Andreassen, T.T.; Simonsen, A.H.; Oxlund, H. Biomechanical Properties of Keratoconus and Normal Corneas. Exp. Eye Res. 1980, 31, 435–441. [Google Scholar] [CrossRef]
- Torres Netto, E.A.; Al-Otaibi, W.M.; Hafezi, N.L.; Kling, S.; Al-Farhan, H.M.; Randleman, J.B.; Hafezi, F. Prevalence of keratoconus in paediatric patients in Riyadh, Saudi Arabia. Br. J. Ophthalmol. 2018, 102, 1436–1441. [Google Scholar] [CrossRef]
- Kennedy, R.H.; Bourne, W.M.; Dyer, J.A. A 48-year clinical and epidemiologic study of keratoconus. Am. J. Ophthalmol. 1986, 101, 267–273. [Google Scholar] [CrossRef]
- Alió, J.L. What Is Keratoconus? A New Approach to a Not So Rare Disease. In Keratoconus; Springer: Cham, Switzerland, 2017; pp. 3–5. [Google Scholar]
- Khan, S.N.; Shiakolas, P.S. To study the effects of intrastromal corneal ring geometry and surgical conditions on the postsurgical outcomes through finite element analysis. J. Mech. Med. Biol. 2016, 16, 1650101. [Google Scholar] [CrossRef]
- Piñero, D.P.; Alio, J.L.; Teus, M.A.; Barraquer, R.I.; Uceda-Montañés, A. Modeling the intracorneal ring segment effect in keratoconus using refractive, Keratometric, and corneal aberrometric data. Investig. Ophthalmol. Vis. Sci. 2010, 51, 5583–5591. [Google Scholar] [CrossRef]
- Vinciguerra, R.; Fernández-Vega-Cueto, L.; Poo-Lopez, A.; Eliasy, A.; Merayo-Lloves, J.; Elsheikh, A.; Madrid-Costa, D.; Lisa, C.; Alfonso, J.F. The Effect of Intracorneal Ring Segments Implantation for Keratoconus on In Vivo Corneal Biomechanics Assessed With the Corvis ST. J. Refract. Surg. 2022, 38, 264–269. [Google Scholar] [CrossRef]
- Ariza-Gracia, M.Á.; Flecha-Lescún, J.; Büchler, P.; Calvo, B. Corneal biomechanics after intrastromal ring surgery: Optomechanical in silico assessment. Transl. Vis. Sci. Technol. 2020, 9, 26. [Google Scholar] [CrossRef] [PubMed]
- Nicula, C.; Pop, R.N.; Nicula, D.V. Comparative Results in a Combined Procedure of Intrastromal Corneal Rings Implantation and Cross-linking in Patients with Keratoconus: A Retrospective Study. Ophthalmol. Ther. 2017, 6, 313–321. [Google Scholar] [CrossRef] [PubMed]
- Piñero, D.P.; Alio, J.L. Intracorneal ring segments in ectatic corneal disease—A review. Clin. Exp. Ophthalmol. 2010, 38, 154–167. [Google Scholar] [CrossRef] [PubMed]
- Piñero, D.P.; Nieto, J.C.; Lopez-Miguel, A. Characterization of corneal structure in keratoconus. J. Cataract. Refract. Surg. 2012, 38, 2167–2183. [Google Scholar] [CrossRef]
- Higuchi, T. Approach to an irregular time series on the basis of the Fractal Theory. Phys. D Nonlinear Phenom. 1988, 31, 277–283. [Google Scholar] [CrossRef]
- Ţălu, Ş.; Stach, S.; Sueiras, V.; Ziebarth, N.M. Fractal Analysis of AFM Images of the Surface of Bowman’s Membrane of the Human Cornea. Ann. Biomed. Eng. 2015, 43, 906–916. [Google Scholar] [CrossRef]
- Stach, S.; Ţălu, Ş.; Trabattoni, S.; Tavazzi, S.; Głuchaczka, A.; Siek, P.; Zając, J.; Giovanzana, S. Morphological Properties of Siloxane-Hydrogel Contact Lens Surfaces. Curr. Eye Res. 2017, 42, 498–505. [Google Scholar] [CrossRef]
- Ţălu, Ş.; Bramowicz, M.; Kulesza, S.; Fiorillo, I. Fractal Features and Surface Micromorphology of Unworn Surfaces of Rigid Gas Permeable Contact Lenses Fractal Features and Surface Micromorphology of Unworn Surfaces of Rigid Gas. Curr. Eye Res. 2017, 42, 1118–1123. [Google Scholar] [CrossRef]
- Katz, M.J. Fractals and the analysis of waveforms. Comput. Biol. Med. 1988, 18, 145–156. [Google Scholar] [CrossRef]
- Matteo, T.D.I. Multi-scaling in finance. Quant. Financ. 2007, 7, 21–36. [Google Scholar] [CrossRef]
- Kisan, S.; Mishra, S.; Rout, S.B. Fractal dimension in medical imaging: A review. Int. Res. J. Eng. Technol. 2017, 4, 1102–1106. [Google Scholar]
- Ambrósio, R.; Belin, M.; Conrad-Hengerer, C.; Dhubhghaill, S.; Gilg, A.-N.; Grewal, D.; Gulani, A.; Holladay, J.; Iwanczuk, J.; Kohnen, T.; et al. Interpretation Guide: Pentacam (2016; p. 216). Available online: https://www.pentacam.com/fileadmin/user_upload/pentacam.de/downloads/interpretations-leitfaden/Pentacam_Guideline_3rd_0218_k.pdf (accessed on 26 May 2022).
- Kim, J.T.; Zloty, P. Simplified Nomenclature for describing Keratoconus. Int. J. Keratoconus Ectatic Corneal Dis. 2012, 1, 31–35. [Google Scholar] [CrossRef]
- Duncan, J.K.; Belin, M.W.; Borgstrom, M. Assessing progression of keratoconus: Novel tomographic determinants. Eye Vis. 2016, 3, 6. [Google Scholar] [CrossRef] [PubMed]
- Ahammer, H. Higuchi Dimension of Digital Images. PLoS ONE 2011, 6, e24796. [Google Scholar] [CrossRef] [PubMed]
- Alessio, G.; Boscia, F.; La Tegola, M.G.; Sborgia, C. Topography-driven excimer laser for the retreatment of decentralized myopic photorefractive keratectomy. Ophthalmology 2001, 108, 1695–1703. [Google Scholar] [CrossRef]
- Shabayek, M.H.; Alió, J.L. Intrastromal Corneal Ring Segment Implantation by Femtosecond Laser for Keratoconus Correction. Ophthalmology 2007, 114, 1643–1652. [Google Scholar] [CrossRef]
- Alio, J.L.; Vega-Estrada, A.; Esperanza, S.; Barraquer, R.; Teus, M.; Murta, J. Intrastromal corneal ring segments: How successful is the surgical treatment of keratoconus? Middle East Afr. J. Ophthalmol. 2014, 21, 3–9. [Google Scholar] [CrossRef]
- Sögütlü, E.; Piñero, D.P.; Kubaloglu, A.; Alio, J.L.; Cinar, Y. Elevation changes of central posterior corneal surface after intracorneal ring segment implantation in keratoconus. Cornea 2012, 31, 387–395. [Google Scholar] [CrossRef]
- Piñero, D.P.; Alió, J.L.; Alesón, A.; Vergara, M.E.; Miranda, M. Corneal volume, pachymetry, and correlation of anterior and posterior corneal shape in subclinical and different stages of clinical keratoconus. J. Cataract Refract. Surg. 2010, 36, 814–825. [Google Scholar] [CrossRef]
- Tomidokoro, A.; Oshika, T.; Amano, S.; Higaki, S.; Maeda, N.; Miyata, K. Changes in anterior and posterior corneal curvatures in keratoconus. Ophthalmology 2000, 107, 1328–1332. [Google Scholar] [CrossRef]
- Mahmoud, A.M.; Nuñez, M.X.; Blanco, C.; Koch, D.D.; Wang, L.; Weikert, M.P.; Frueh, B.E.; Tappeiner, C.; Twa, M.D.; Roberts, C.J. Expanding the cone location and magnitude index to include corneal thickness and posterior surface information for the detection of keratoconus. Am. J. Ophthalmol. 2013, 156, 1102–1111. [Google Scholar] [CrossRef] [PubMed]
- Khachikian, S.S.; Belin, M.W. Posterior Elevation in Keratoconus. Ophthalmology 2009, 116, 816–816.e1. [Google Scholar] [CrossRef] [PubMed]
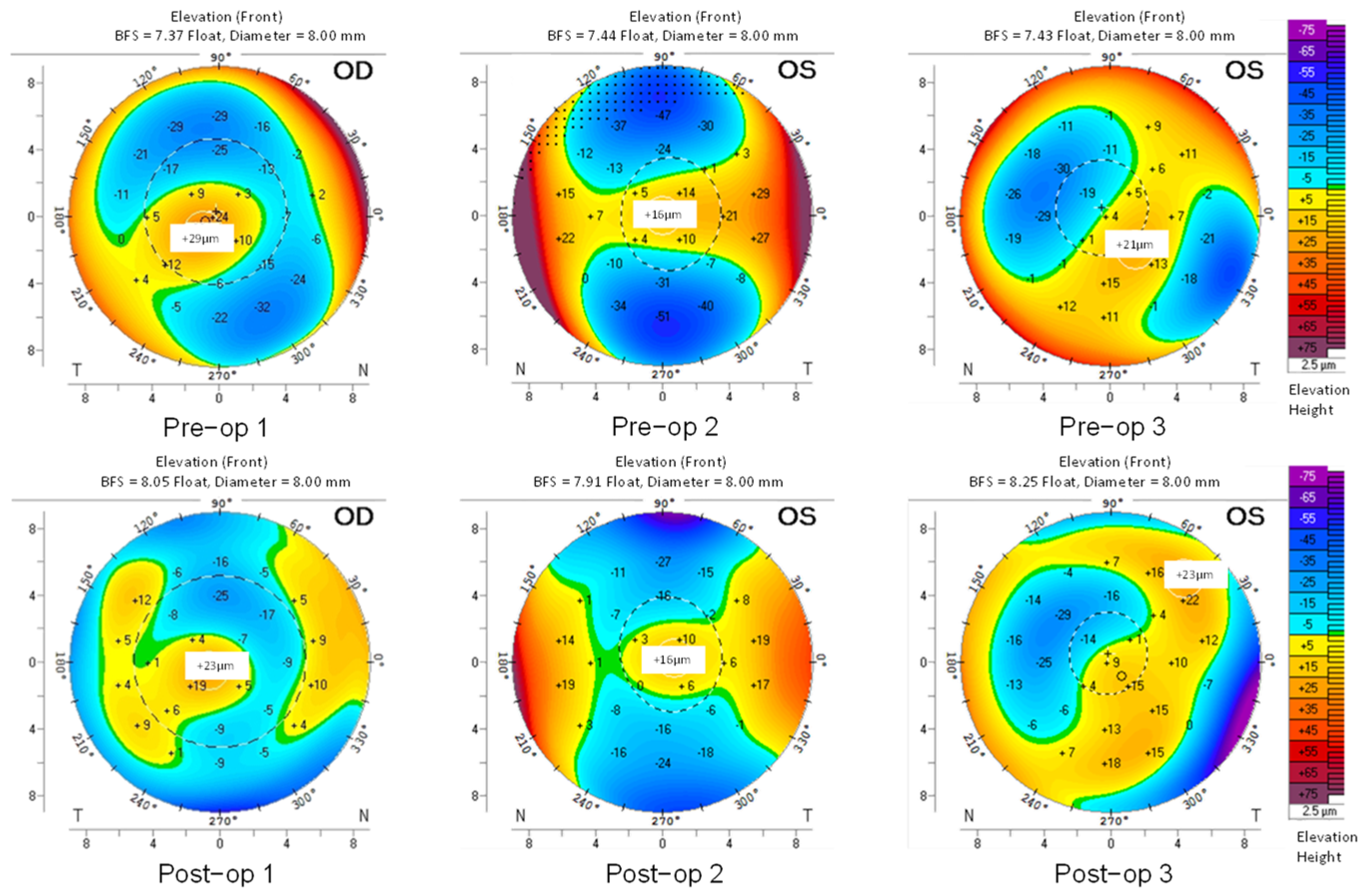
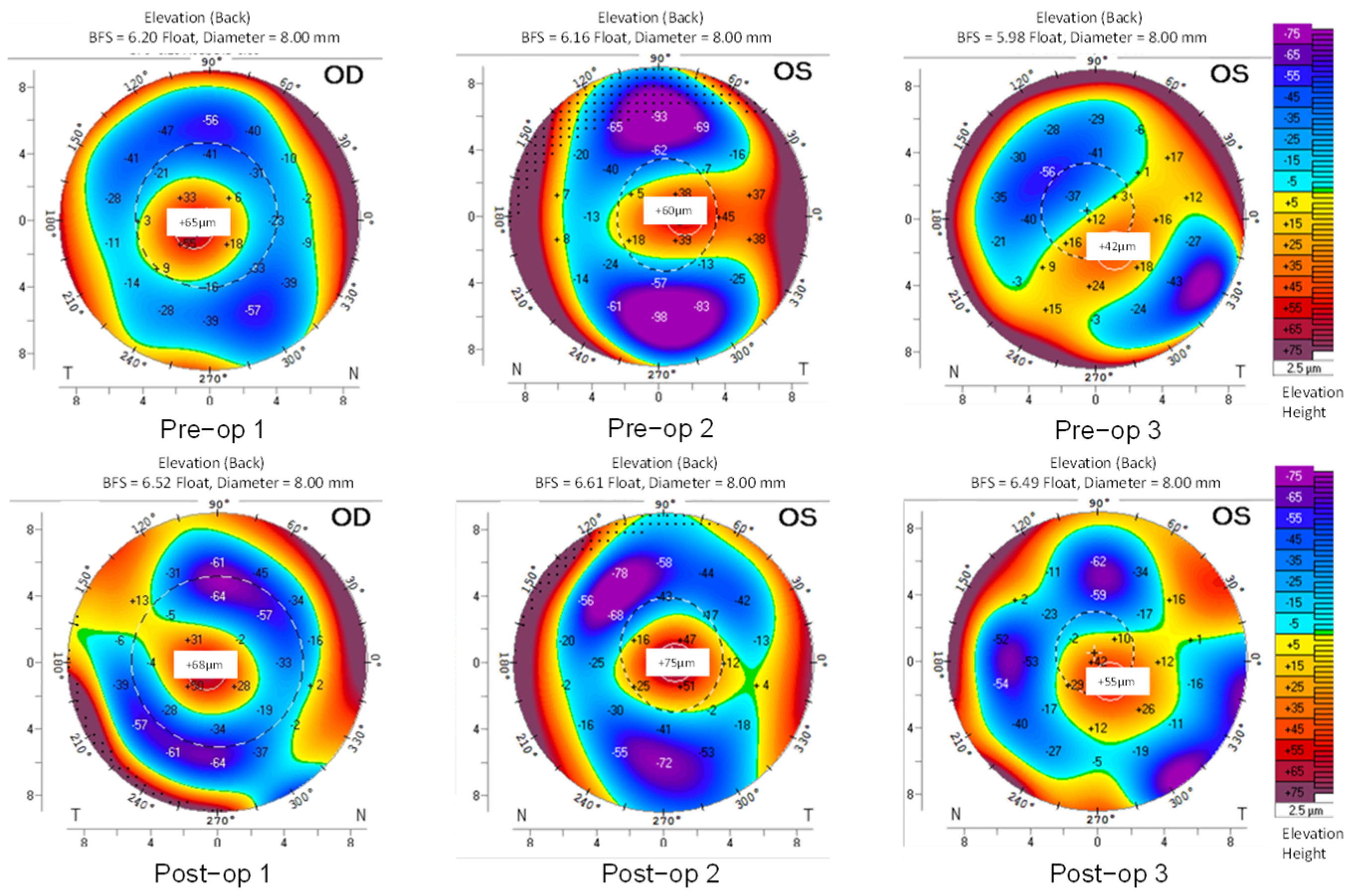


| Subjects | Sex (m/f) | Age (Yr, Mean ± Std) | OS/OD Eye * |
|---|---|---|---|
| Healthy | 4/3 | 29.5 ± 3.5 | 3 OS/4 OD |
| KCN | 4/3 | 39.25 ± 7.1 | 5 OS/2 OD |
| Subjects | Optical Outcome | Kmax-Front (D) | Astig-Front (D) | Astig-Back (D) |
|---|---|---|---|---|
| Healthy | Status | 43.66 ± 0.7 | 0.77 ± 0.46 | 0.31 ± 0.09 |
| KCN | Pre-op * | 52.47 ± 3.24 | 3.81 ± 2.07 | 0.74 ± 0.42 |
| Post-op ** | 50.56 ± 3.04 | 2.01± 0.89 | 0.46 ± 0.27 |
| Subjects | Kmax-Front (D) | Astig-Front (D) | Astig-Back (D) | |||
|---|---|---|---|---|---|---|
| Pre-op * | Post-op ** | Pre-op | Post-op | Pre-op | Post-op | |
| Healthy | 0.002 | 0.268 | 0.003 | 0.021 | 0.016 | 0.002 |
| Pre-op | N/A | 0.078 | N/A | 0.059 | N/A | 0.55 |
| Subjects | Pre-op | Post-op |
|---|---|---|
| Higuchi fractal dimension | ||
| Healthy | 0.710 | 0.007 |
| Pre-op | N/A | 0.018 |
| Katz fractal dimension | ||
| Healthy | 0.620 | 0.017 |
| Pre-op | N/A | 0.028 |
| Generalized Hurst exponent | ||
| Healthy | 0.535 | 0.383 |
| Pre-op | N/A | 0.091 |
| Subjects | Pre-op | Post-op |
|---|---|---|
| Higuchi fractal dimension | ||
| Healthy | 0.535 | 0.001 |
| Pre-op | N/A | 0.018 |
| Katz fractal dimension | ||
| Healthy | 0.535 | 0.002 |
| Pre-op | N/A | 0.018 |
| Generalized Hurst exponent | ||
| Healthy | 0.318 | 0.620 |
| Pre-op | N/A | 0.028 |
| Subjects | Pre-op | Post-op |
|---|---|---|
| Higuchi fractal dimension | ||
| Healthy | 0.805 | 0.002 |
| Pre-op | N/A | 0.028 |
| Katz fractal dimension | ||
| Healthy | 0.001 | 0.001 |
| Pre-op | N/A | 0.028 |
| Generalized Hurst exponent | ||
| Healthy | 0.456 | 0.805 |
| Pre-op | N/A | 0.128 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bahramizadeh-Sajadi, S.; Katoozian, H.R.; Mehrabbeik, M.; Baradaran-Rafii, A.; Jadidi, K.; Jafari, S. A Fractal Approach to Nonlinear Topographical Features of Healthy and Keratoconus Corneas Pre- and Post-Operation of Intracorneal Implants. Fractal Fract. 2022, 6, 688. https://doi.org/10.3390/fractalfract6110688
Bahramizadeh-Sajadi S, Katoozian HR, Mehrabbeik M, Baradaran-Rafii A, Jadidi K, Jafari S. A Fractal Approach to Nonlinear Topographical Features of Healthy and Keratoconus Corneas Pre- and Post-Operation of Intracorneal Implants. Fractal and Fractional. 2022; 6(11):688. https://doi.org/10.3390/fractalfract6110688
Chicago/Turabian StyleBahramizadeh-Sajadi, Shima, Hamid Reza Katoozian, Mahtab Mehrabbeik, Alireza Baradaran-Rafii, Khosrow Jadidi, and Sajad Jafari. 2022. "A Fractal Approach to Nonlinear Topographical Features of Healthy and Keratoconus Corneas Pre- and Post-Operation of Intracorneal Implants" Fractal and Fractional 6, no. 11: 688. https://doi.org/10.3390/fractalfract6110688
APA StyleBahramizadeh-Sajadi, S., Katoozian, H. R., Mehrabbeik, M., Baradaran-Rafii, A., Jadidi, K., & Jafari, S. (2022). A Fractal Approach to Nonlinear Topographical Features of Healthy and Keratoconus Corneas Pre- and Post-Operation of Intracorneal Implants. Fractal and Fractional, 6(11), 688. https://doi.org/10.3390/fractalfract6110688






