Elastoplastic Analysis of Circular Steel Tube of CFT Stub Columns under Axial Compression
Abstract
1. Introduction
2. Experimental Program
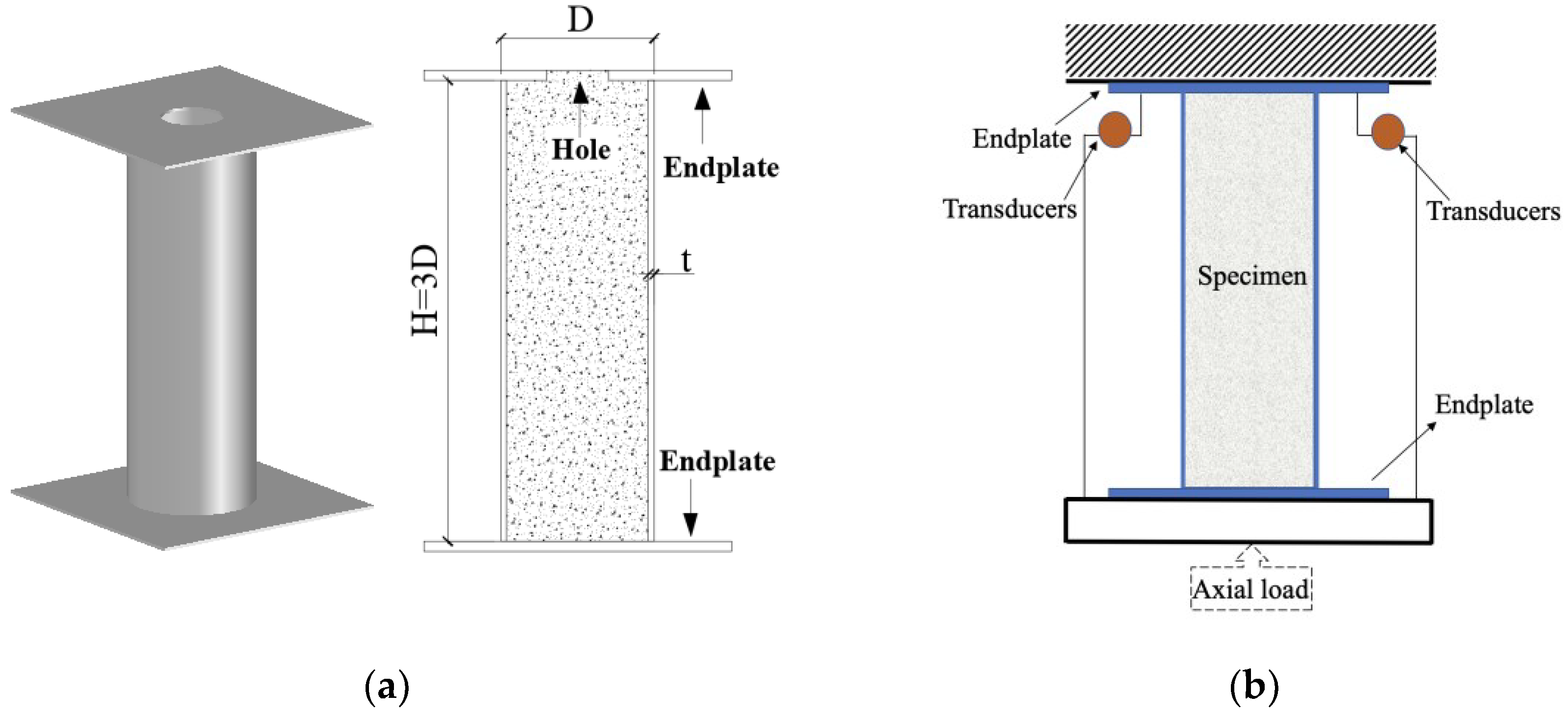
2.1. Details of the Specimens
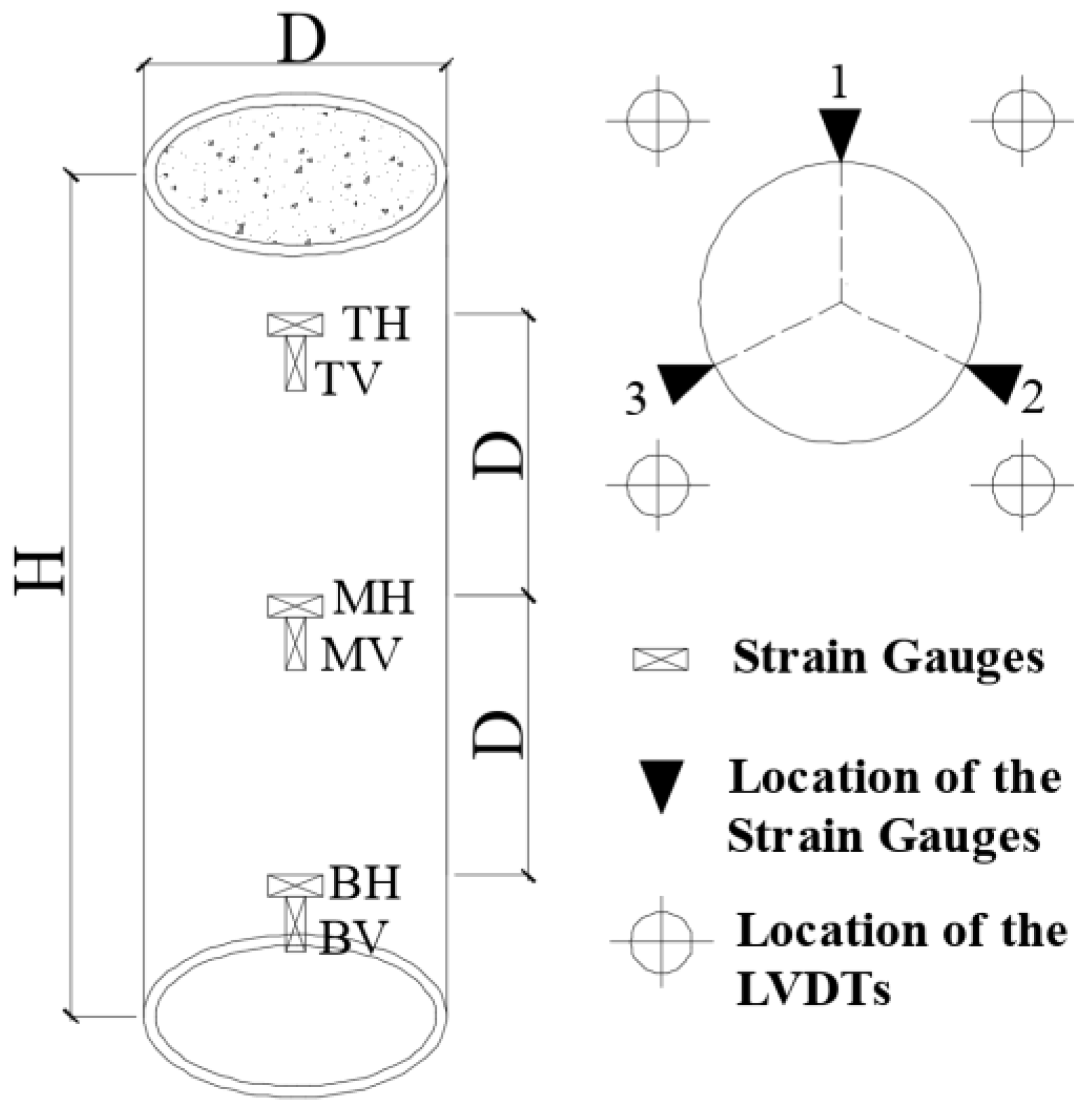
2.2. Test Apparatus and Instrumentations
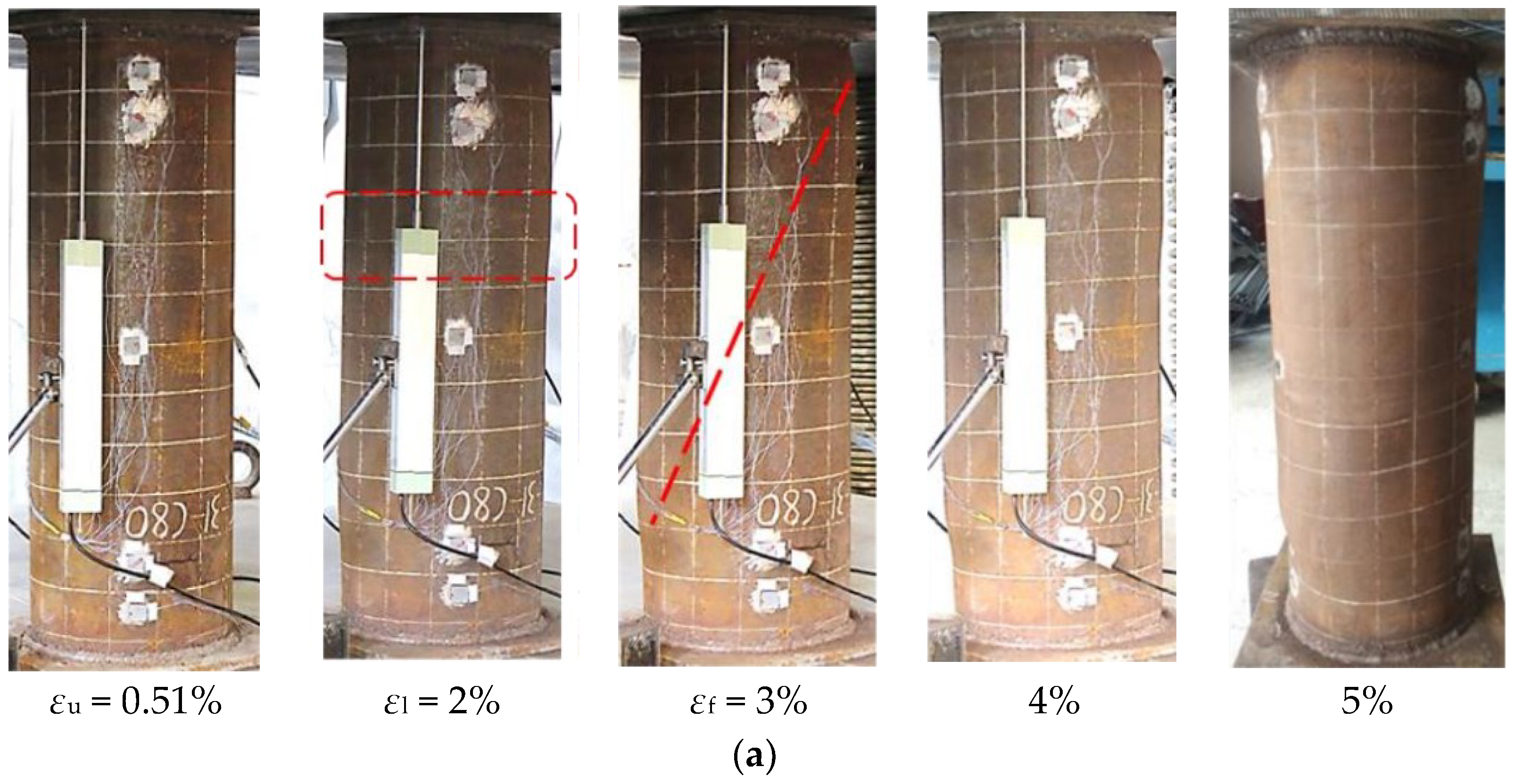
3. Test results and Discussions
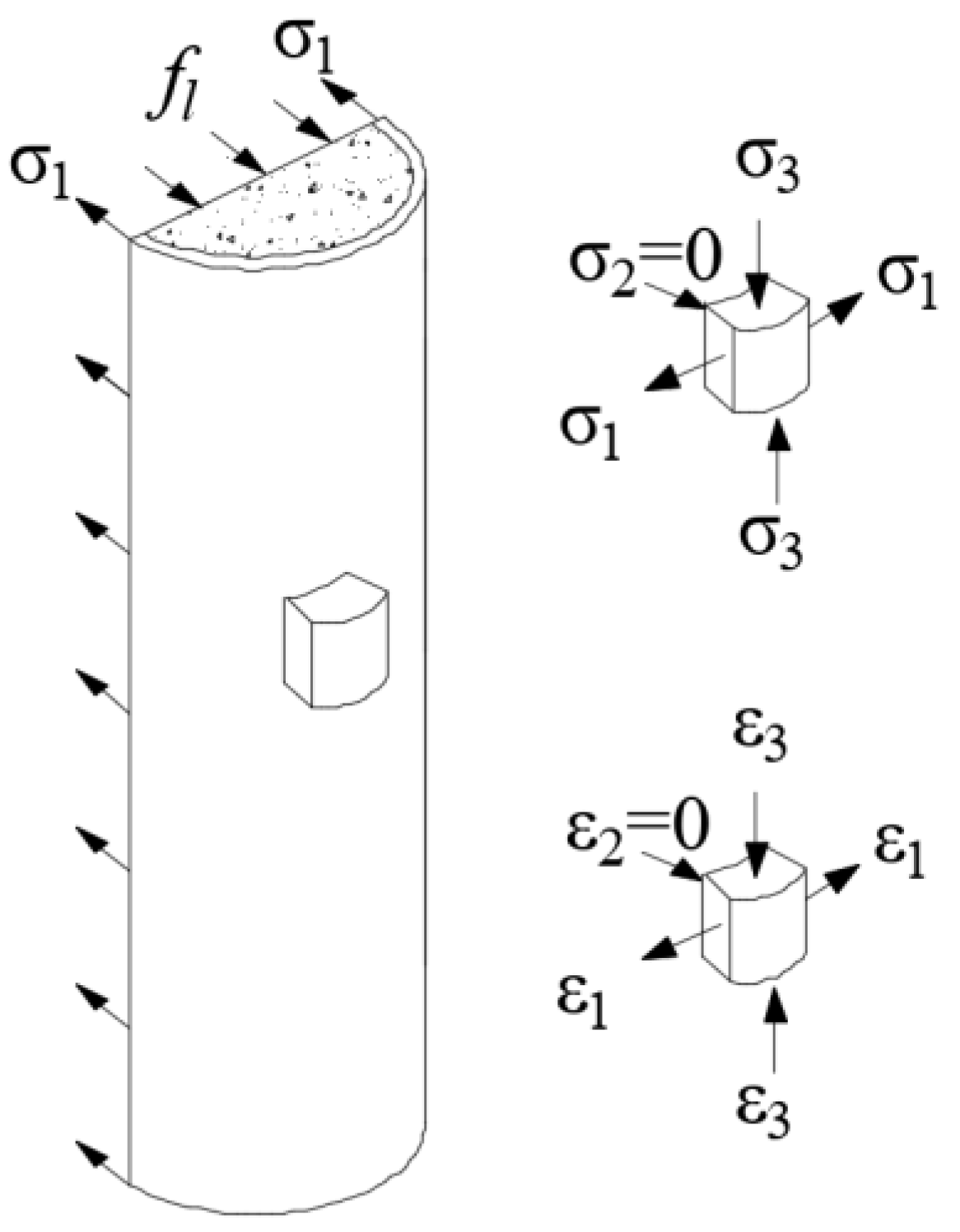
4. Theoretical Relation of the Axial Stress–Strain between CHS and Steel Tube of CFT
5. Analytical Results
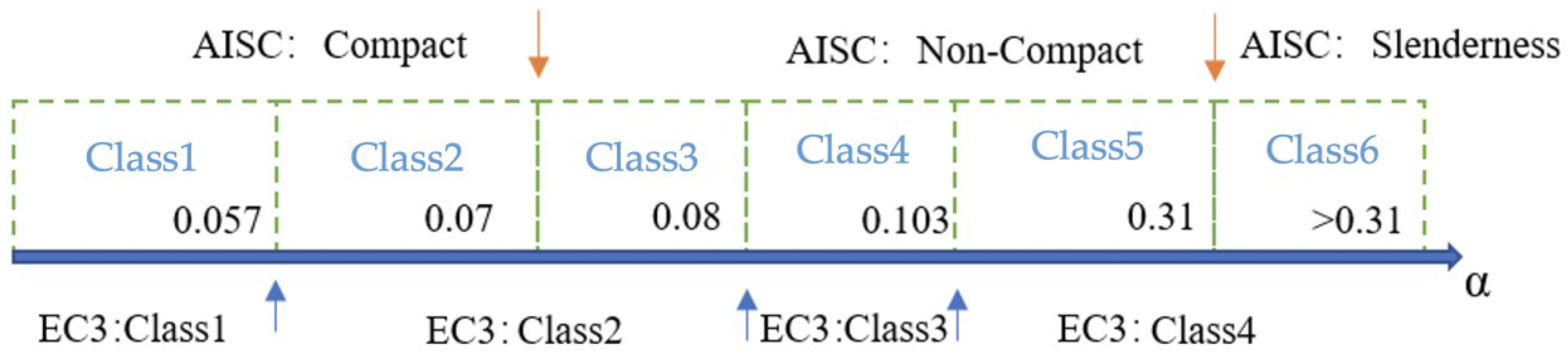
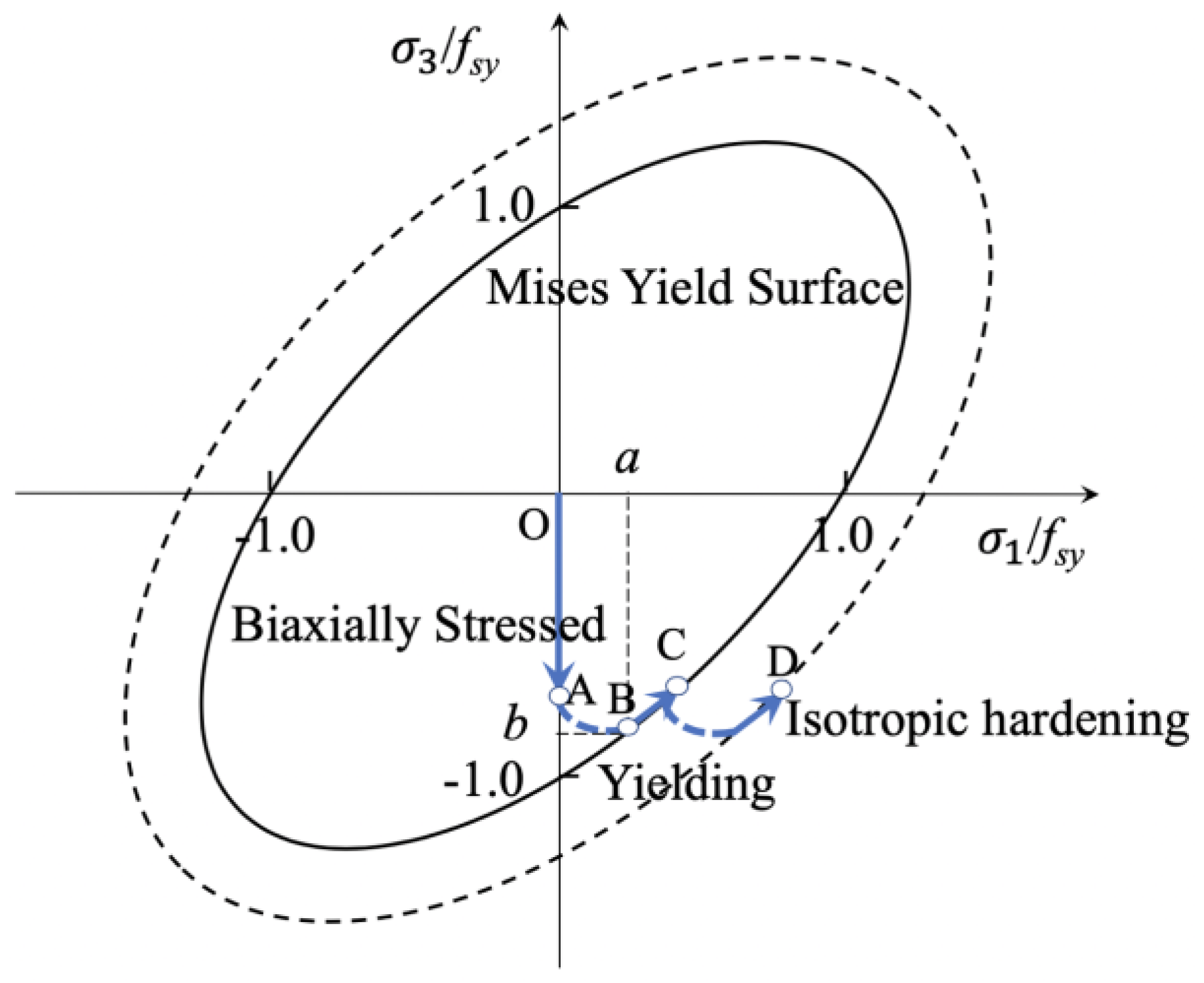
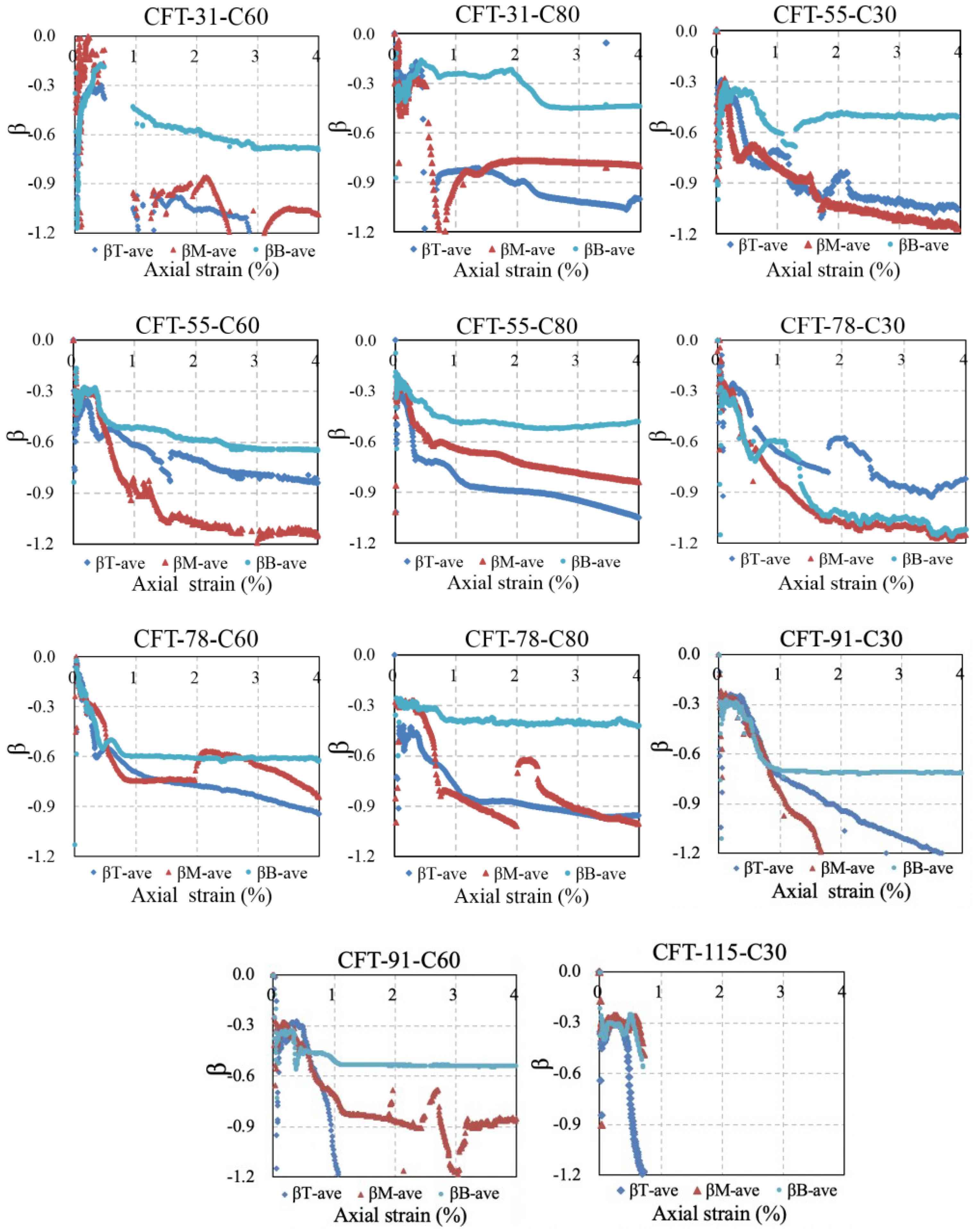
5.1. Ratios β1 of Hoop Strain to the Vertical Strain of the Measured Point
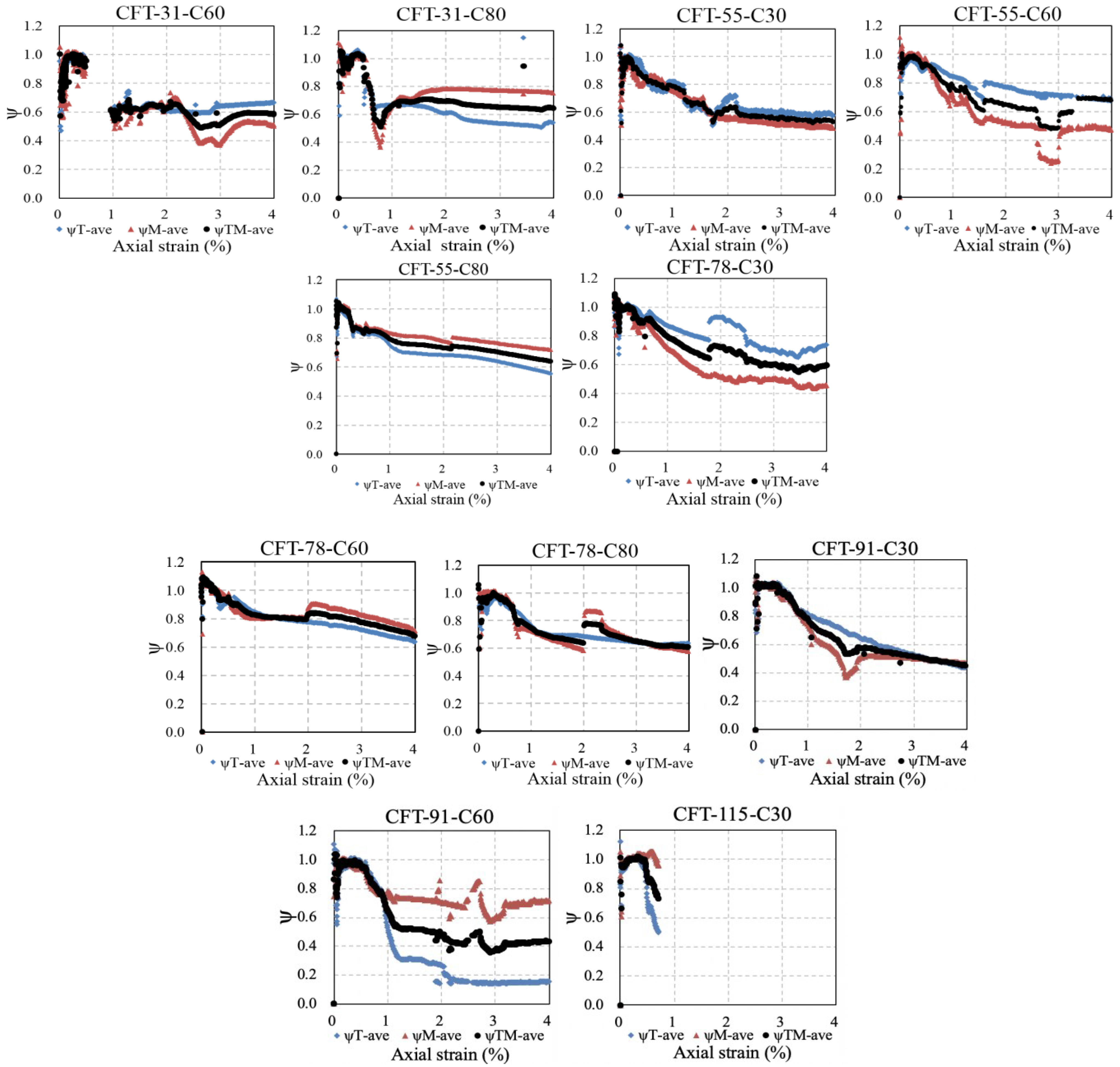
5.2. Real-Time Reduction Factor ψ
5.3. The Constitutive Model of CHS
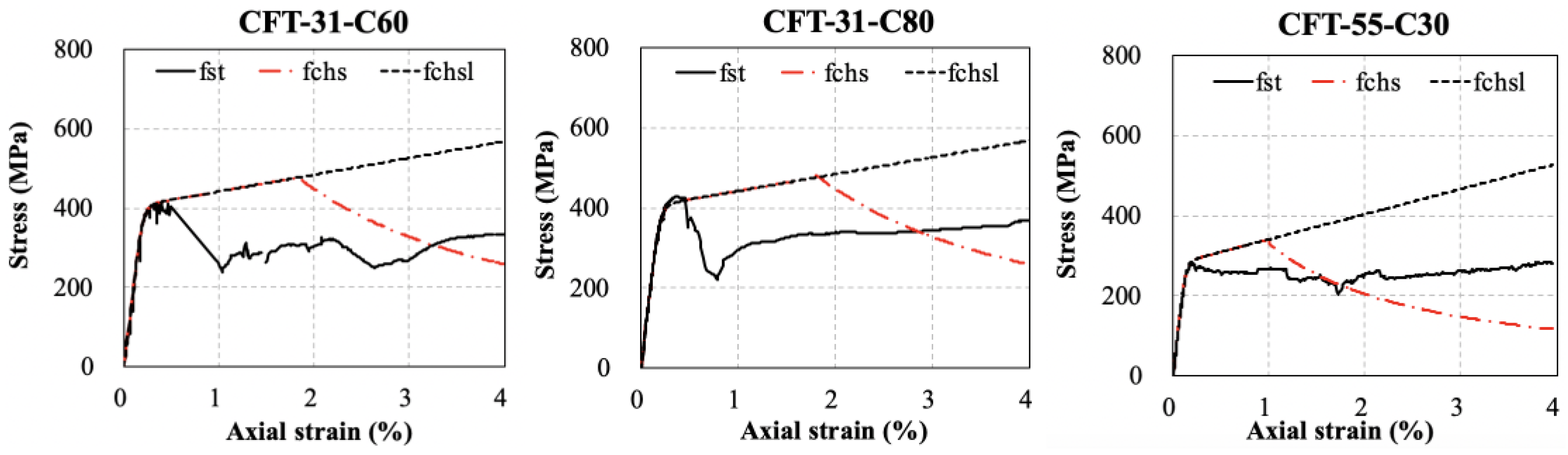
5.4. Stress–Strain Curves of the Steel Tube of the Specimens
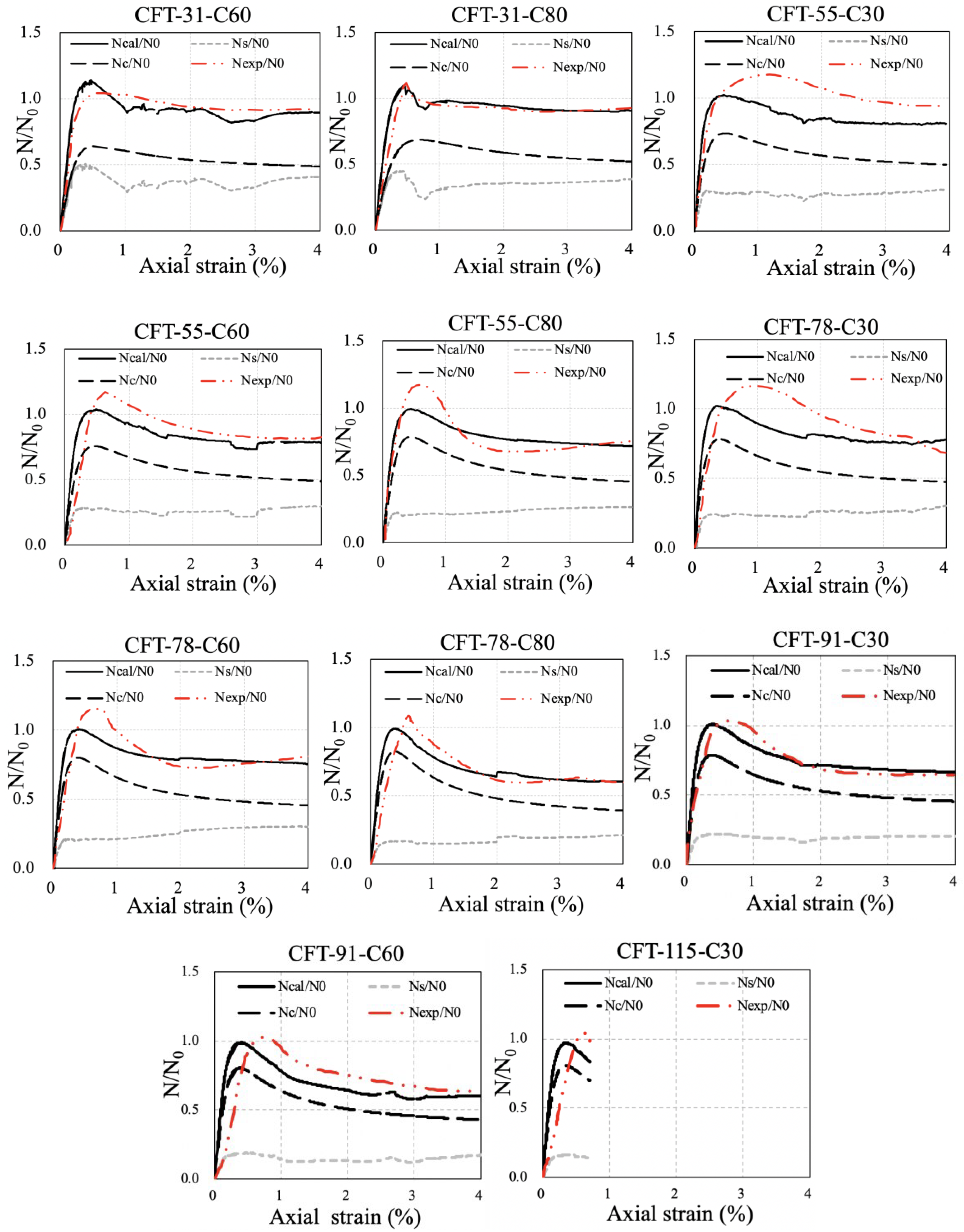
6. Verification
7. Conclusions
- (1)
- The proposed method provides an effective way to analyze the measure strain history to obtain the experimental stress–strain curve of the steel tube of CFT considering biaxial stress state and local buckling, which is critical to comprehend the elastoplastic behavior of the steel tube interacted with the core concrete.
- (2)
- Comparisons of the stress–strain curves of the steel tube and CHS indicated that the infilled concrete indeed helpfully improved the residual stress of the steel tube, but it would cause an earlier strength degradation of the steel tube. This feature is suggested to be considered in finite simulation work on CFT columns.
- (3)
- Different from the specimens of Class 2 and Class 4, local buckling of the specimens of Class 5 was prior to the ultimate strength, which indicated that the influence of local buckling was greater on the columns with thinner-walled steel tube. To reduce the local buckling effect on the behavior of CFT columns, the thin-walled steel tube is suggested to be used carefully.
- (4)
- Crushing of concrete in shear failure and local buckling of the steel tube were observed in all the specimens. The shear failed concrete caused secondary local buckling of the steel tube, which dominated buckle deformation of the steel tube with increasing axial compression.
- (5)
- Higher strength concrete of specimens showed earlier shear failure due to less ductility. With increasing concrete strength and ratio of D/t, the strength degradation of the columns was steeper.
8. Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Johansson, M.; Gylltoft, K. Mechanical Behavior of Circular Steel–Concrete Composite Stub Columns. J. Struct. Eng. 2002, 128, 1073–1081. [Google Scholar] [CrossRef]
- Zeghiche, J.; Chaoui, K. An experimental behaviour of concrete-filled steel tubular columns. J. Constr. Steel Res. 2005, 61, 53–66. [Google Scholar] [CrossRef]
- Yu, Z.W.; Ding, F.X.; Cai, C.S. Experimental behavior of circular concrete-filled steel tube stub columns. J. Constr. Steel Res. 2007, 63, 165–174. [Google Scholar] [CrossRef]
- Ekmekyapar, T.; Al-Eliwi, B.J.M. Experimental behaviour of circular concrete filled steel tube columns and design specifications. Thin Wall Struct. 2016, 105, 220–230. [Google Scholar] [CrossRef]
- Dundu, M. Compressive strength of circular concrete filled steel tube columns. Thin Wall Struct. 2012, 56, 62–70. [Google Scholar] [CrossRef]
- Oliveira, W.L.D.; Nardin, S.D.; de Cresce El, A.L.H.; El Debs, M.K. Influence of concrete strength and length/diameter on the axial capacity of CFT columns. J. Constr. Steel Res. 2009, 65, 2103–2110. [Google Scholar] [CrossRef]
- Abed, F.; AlHamaydeh, M.; Abdalla, S. Experimental and numerical investigations of the compressive behavior of concrete filled steel tubes (CFTs). J. Constr. Steel Res. 2013, 80, 429–439. [Google Scholar] [CrossRef]
- Giakoumelis, G.; Lam, D. Axial capacity of circular concrete-filled tube columns. J. Constr. Steel Res. 2004, 60, 1049–1068. [Google Scholar] [CrossRef]
- Guler, S.; Copur, A.; Aydogan, M. Axial capacity and ductility of circular UHPC-filled steel tube columns. Mag. Concr. Res. 2013, 65, 898–905. [Google Scholar] [CrossRef]
- Gupta, P.K.; Sarda, S.M.; Kumar, M.S. Experimental and computational study of concrete filled steel tubular columns under axial loads. J. Constr. Steel Res. 2007, 63, 182–193. [Google Scholar] [CrossRef]
- Wang, F.C.; Xie, W.Q.; Li, B.; Han, L.H. Experimental study and design of bond behavior in concrete-filled steel tubes (CFST). Eng. Struct. 2022, 268, 114750. [Google Scholar] [CrossRef]
- Han, L.H.; Xu, C.Y.; Hou, C. Axial compression and bond behaviour of recycled aggregate concrete-filled stainless steel tubular stub columns. Eng. Struct. 2022, 262, 114306. [Google Scholar] [CrossRef]
- Ci, J.; Ahmed, M.; Liang, Q.Q.; Chen, S.; Chen, W.; Sennah, K.; Hamoda, A. Experimental and numerical investigations into the behavior of circular concrete-filled double steel tubular slender columns. Eng. Struct. 2022, 267, 114644. [Google Scholar] [CrossRef]
- Ahmed, M.; Liang, Q.Q.; Patel, V.I.; Hadi, M.N. Behavior of circular concrete-filled double steel tubular slender beam-columns including preload effects. Eng. Struct. 2020, 220, 111010. [Google Scholar] [CrossRef]
- Zhang, S.; Li, X.; Li, J.; Lu, W.; Chen, J. Behavior comparison of seven-types of steel–concrete composite stub columns under axial compression. Eng. Struct. 2022, 252, 113637. [Google Scholar] [CrossRef]
- Richart, F.E.; Brandtzaeg, A.; Brown, R.L. A Study of the Failure of Concrete under Combined Compressive Stresses; University of Illinois at Urbana Champaign, College of Engineering. Engineering Experiment Station: Chicago, IL, USA, 1928. [Google Scholar]
- Gardner, N.J. Triaxial behavior of concrete. J. Proc. 1969, 66, 136–158. [Google Scholar]
- Jamet, P.; Millard, A.; Nahas, G. Triaxial Behaviour of a Micro-Concrete Complete Stress-Strain Curves for Confining Pressures Ranging from 0 to 100 MPa; CEA Centre d’Etudes Nucleaires de Saclay: Gif-sur-Yvette, France, 1984. [Google Scholar]
- Ansari, F.; Li, Q. High-strength concrete subjected to triaxial compression. ACI Mater. J. 1998, 95, 747–755. [Google Scholar]
- Li, Q.; Ansari, F. Mechanics of damage and constitutive relationships for high-strength concrete in triaxial compression. J. Eng. Mech. ASCE 1999, 125, 1–10. [Google Scholar] [CrossRef]
- Mander, J.B.; Chang, G.A. Seismic Energy Based Fatigue Damage Analysis of Bridge Columns: Part 1—Evaluation of Seismic Capacity; Technical Report; NCEER: Buffalo, NY, USA, 1994; p. 0006. [Google Scholar]
- Sakino, K.; Nakahara, H.; Morino, S.; Nishiyama, I. Behavior of centrally loaded concrete-filled steel-tube short columns. J. Struct. Eng. 2004, 130, 180–188. [Google Scholar] [CrossRef]
- Sakino, K. Stress-Strain Curve of Concrete Confined by Rectilinear Steels. J. Struct. Constr. Eng. AIJ 1994, 461, 95–104. [Google Scholar] [CrossRef]
- Sun, Y.P.; Sakino, K. Modelling for the axial behaviour of high strength CFT columns. In Proceedings of the 23rd Conference on Our World in Concrete & Structures, Singapore, 25–26 August 1998. [Google Scholar]
- Lai, Z.; Varma, A.H. Effective stress-strain relationships for analysis of noncompact and slender filled composite (CFST) members. Eng. Struct. 2016, 124, 457–472. [Google Scholar] [CrossRef]
- Hatzigeorgiou, G.D. Numerical model for the behavior and capacity of circular CFT columns, Part I: Theory. Eng. Struct. 2008, 30, 1573–1578. [Google Scholar] [CrossRef]
- Liang, Q.Q.; Fragomeni, S. Nonlinear analysis of circular concrete-filled steel tubular short columns under axial loading. J. Constr. Steel Res. 2009, 65, 2186–2196. [Google Scholar] [CrossRef]
- Tao, Z.; Wang, Z.B.; Yu, Q. Finite element modelling of concrete-filled steel stub columns under axial compression. J. Constr. Steel Res. 2013, 89, 121–131. [Google Scholar] [CrossRef]
- Katwal, U.; Tao, Z.; Hassan, M.K.; Wang, W.-D. Simplified numerical modeling of axially loaded circular concrete-filled steel stub columns. J. Struct. Eng. 2017, 143, 04017169. [Google Scholar] [CrossRef]
- Shea, M.D.O.; Bridge, R.Q. Local buckling of thin-walled circular steel sections with or without internal restraint. J. Constr. Steel Res. 1997, 41, 137–157. [Google Scholar] [CrossRef]
- Elchalakani, M.; Zhao, X.L.; Grzebieta, R. Tests on concrete filled double-skin (CHS outer and SHS inner) composite short columns under axial compression. Thin Wall Struct. 2002, 40, 415–441. [Google Scholar] [CrossRef]
- Zhao, X.L.; Grzebieta, R.; Elchalakani, M. Tests of concrete-filled double skin CHS composite stub column. Steel Compos. Struct. 2002, 2, 129–146. [Google Scholar] [CrossRef]
- Fujimoto, T.; Mukai, A.; Nishiyama, I. Axial compression behavior of concrete filled steel tubular stub columns using high strength materials. J. Struct. Constr. Eng. AIJ 1997, 62, 161–168. [Google Scholar] [CrossRef][Green Version]
- Lin, B.Z. Research on the Circular Transversely Confined Concrete under Uniaxial Compression. Master’s Dissertation, Kyushu University, Fukuoka, Japan, 1990. [Google Scholar]
- Lai, M.H.; Ho JC, M. A theoretical axial stress-strain model for circular concrete-filled-steel-tube columns. Eng. Struct. 2016, 125, 124–143. [Google Scholar] [CrossRef]
- Li, Y.L.; Zhao, X.L.; Raman RK, S. Theoretical model for concrete-filled stainless steel circular stub columns under axial compression. J. Constr. Steel Res. 2019, 157, 426–439. [Google Scholar] [CrossRef]
- Ore, E.; Durban, D. Elastoplastic buckling of axially compressed circular cylindrical shells. Int. J. Mech. Sci. 1992, 34, 727–742. [Google Scholar] [CrossRef]
- Venkata Deepthi, T.; Sridhar Reddy, C.; Satyadevi, A. Elastic-Plastic Analysis Under Proportional and Non-Proportional Loading Paths Using Deformation Plasticity Theory. Int. J. Comput. Methods Eng. Sci. Mech. 2018, 19, 412–418. [Google Scholar] [CrossRef]
- Durban, D.; Zuckerman, Z. Elastoplastic buckling of rectangular plates in biaxial compression/tension. Int. J. Mech. Sci. 1999, 41, 751–765. [Google Scholar] [CrossRef]
- Paquette, J.A.; Kyriakides, S. Plastic buckling of tubes under axial compression and internal pressure. Int. J. Mech. Sci. 2006, 48, 855–867. [Google Scholar] [CrossRef]
- Grigoryan, M.N.; Urvachev, P.M.; Chepurnenko, A.S.; Polyakova, T.V. Determination of the ultimate load for centrally compressed concrete filled steel tubular columns based on the deformation theory of plasticity. IOP Conf. Ser. Mater. Sci. Eng. 2020, 913, 022069. [Google Scholar] [CrossRef]
- Yang, C.; Zhao, H.; Sun, Y.; Zhao, S. Compressive stress-strain model of cold-formed circular hollow section stub columns considering local buckling. Thin Walled Struct. 2017, 120, 495–505. [Google Scholar] [CrossRef]
- Architecture Institute of Japan (AIJ). Design Standard for Steel Structures; Marauzen: Tokyo, Japan, 1993. (In Japanese) [Google Scholar]
- GB/T 50081-2002; Standard for Test Method of Mechanical Properties on Ordinary Concrete. China Academy of Building Research: Beijing, China, 2002. (In Chinese)
- Yang, C.; Yu, Z.X.; Sun, Y.P.; Zhao, L.; Zhao, H. Axial Residual Capacity of Circular Concrete-Filled Steel Tube Stub Columns Considering Lo-Cal Buckling. Adv. Steel Constr. Int. J. 2018, 14, 496–513. [Google Scholar]
- GB50936-2014; Technical Code for Concrete Filled Steel Tubular Structures. China Academy of Building Research: Beijing, China, 2014. (In Chinese)
- Hosford, W.F. Solid Mechanics; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Valluri, S.R. Fracture under Biaxial Conditions in the Presence of a Crack. In Proceedings of the First International Conference on Fracture, Sendai, Japan, 12–17 September 1965. [Google Scholar]
- He, Y.T.; Fu, X.J.; Chen, R.F. Stress-strain curve under triaxial stress state and its applications. J. NPU 1998, 16, 610–615. (In Chinese) [Google Scholar]
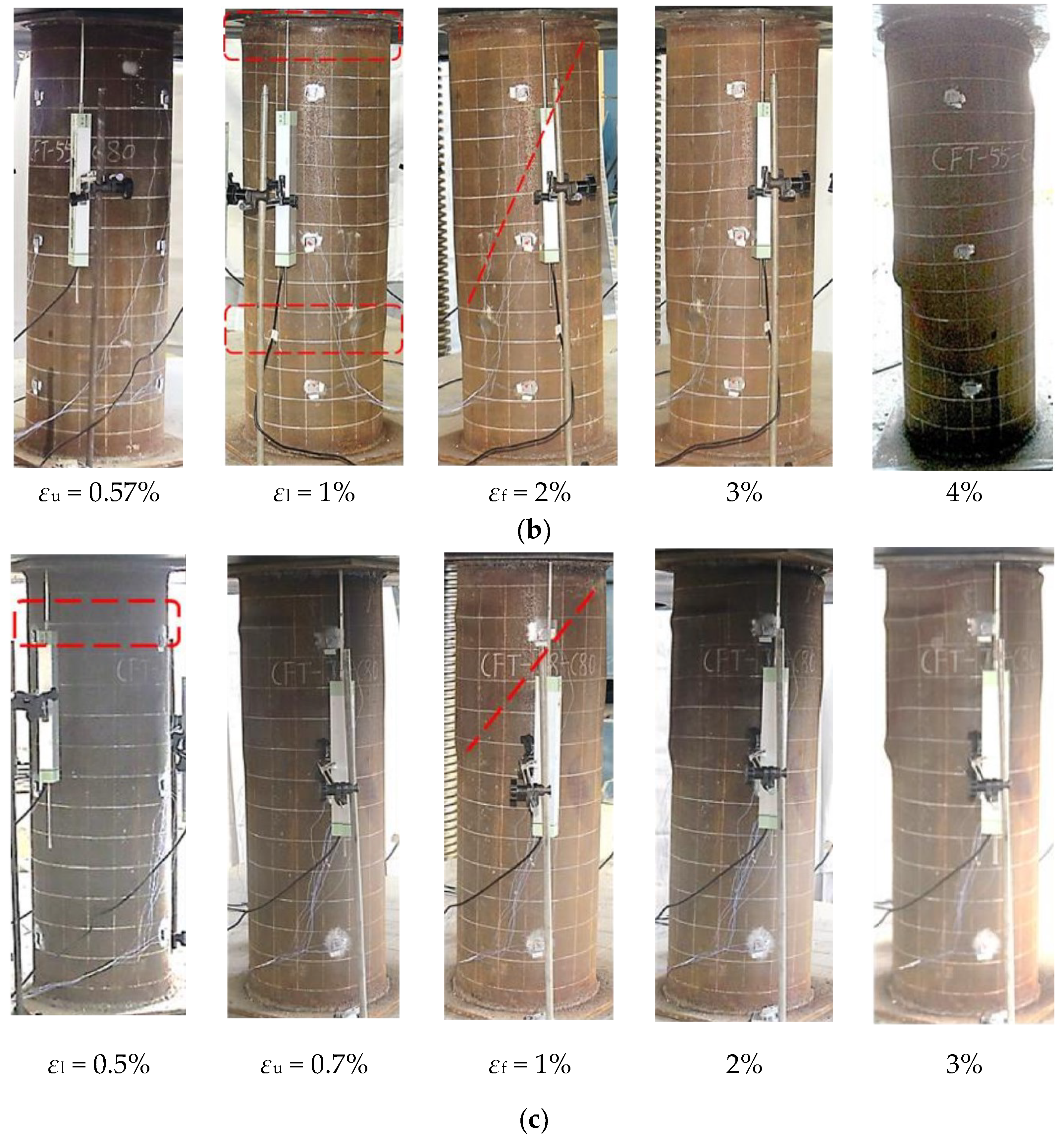

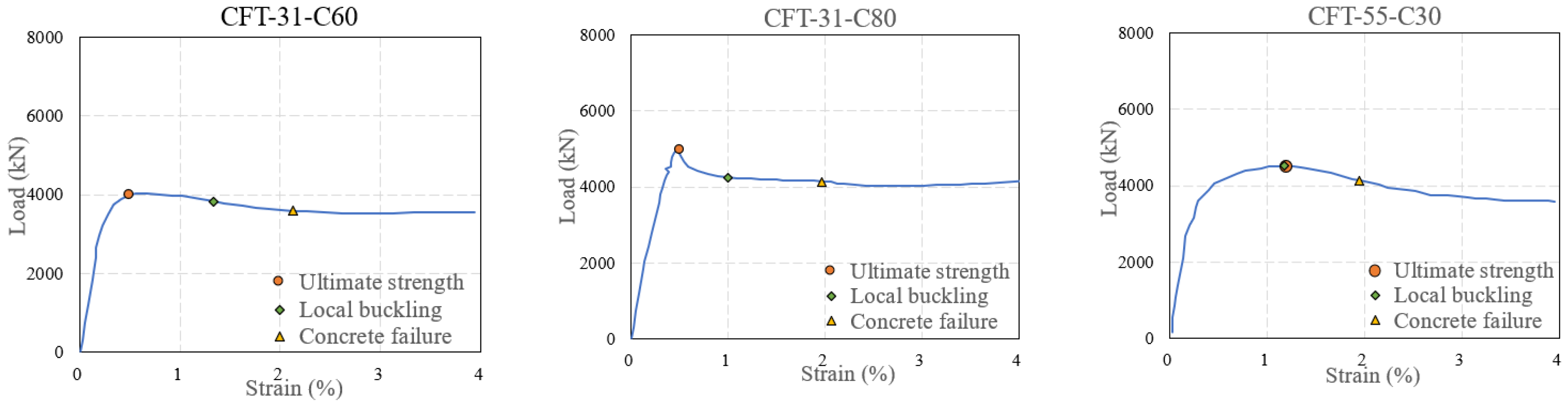


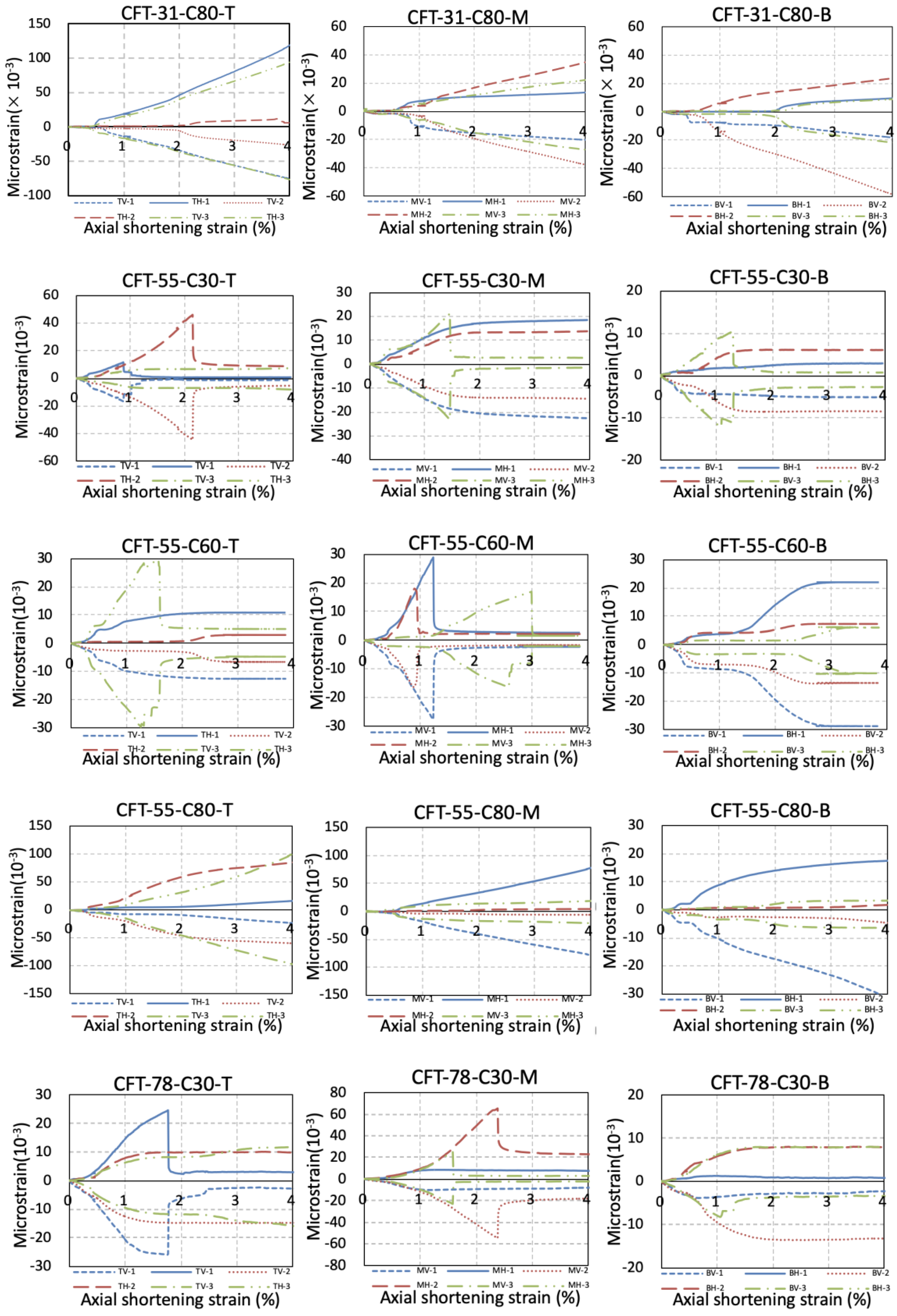
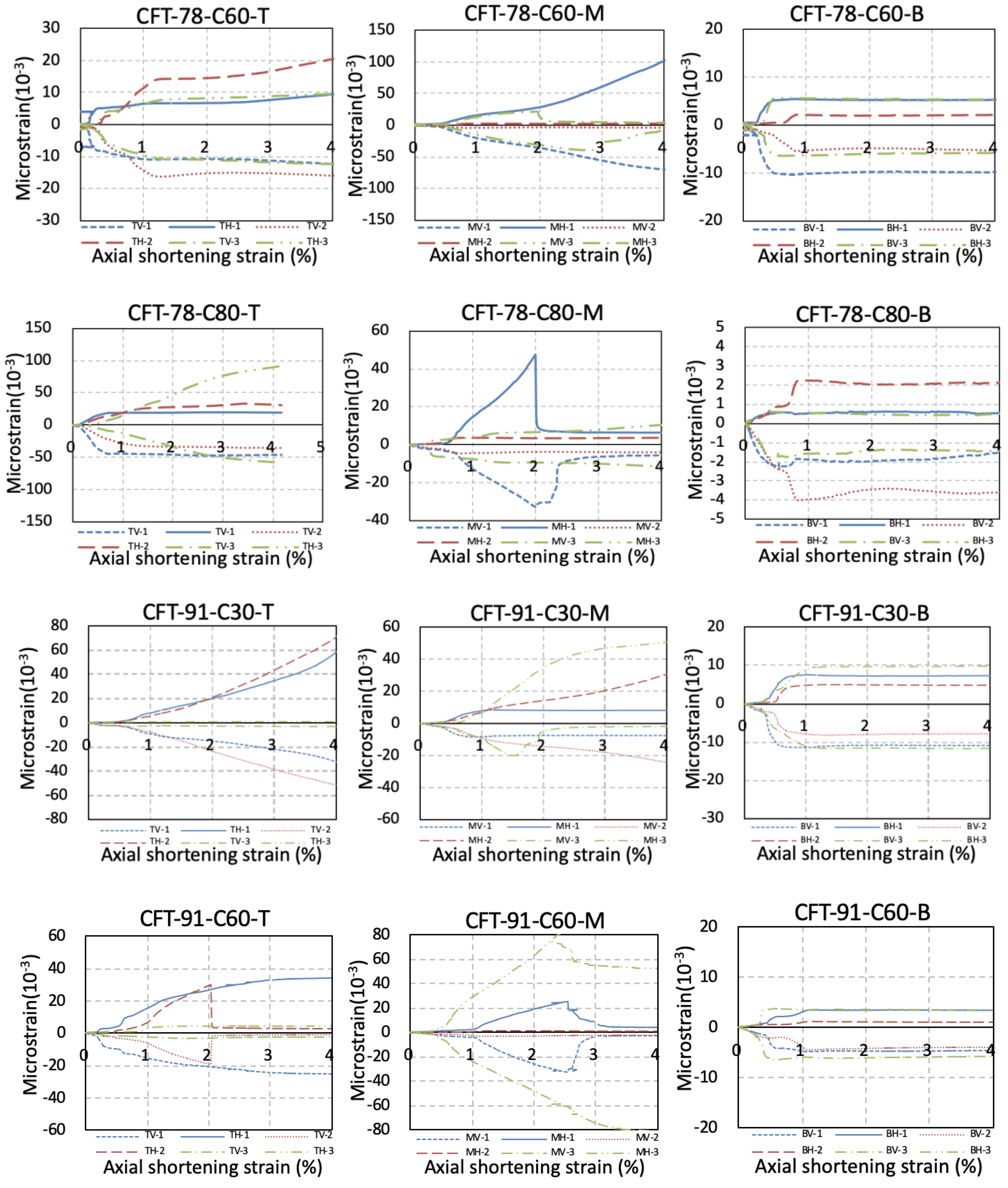



| Specimens | D (mm) | t (mm) | H(mm) | fcu,k(MPa) | fcu,kcy(MPa) | fsy(MPa) | fsu(MPa) | A (%) | Es (GPa) | α | Type |
|---|---|---|---|---|---|---|---|---|---|---|---|
| CFT-31-C60 | 219 | 7.06 | 659 | 70 | 60 | 429 | 663 | 25 | 205 | 0.06 | Class2 |
| CFT-31-C80 | 219 | 7.06 | 659 | 93 | 81 | 429 | 663 | 25 | 205 | 0.06 | Class 2 |
| CFT-55-C30 | 273 | 5.00 | 816 | 58 | 50 | 312 | 452 | 35 | 202 | 0.08 | Class 4 |
| CFT-55-C60 | 273 | 5.00 | 816 | 70 | 60 | 312 | 452 | 35 | 202 | 0.08 | Class 4 |
| CFT-55-C80 | 273 | 5.00 | 816 | 93 | 81 | 312 | 452 | 35 | 202 | 0.08 | Class 4 |
| CFT-78-C30 | 273 | 3.50 | 813 | 58 | 50 | 305 | 444 | 36 | 205 | 0.12 | Class 5 |
| CFT-78-C60 | 273 | 3.50 | 813 | 70 | 60 | 305 | 444 | 36 | 205 | 0.12 | Class 5 |
| CFT-78-C80 | 273 | 3.50 | 818 | 93 | 81 | 305 | 444 | 36 | 205 | 0.12 | Class 5 |
| CFT-93-C30 | 325 | 3.57 | 971 | 58 | 50 | 329 | 453 | 33 | 206 | 0.15 | Class 5 |
| CFT-93-C60 | 325 | 3.57 | 971 | 70 | 60 | 329 | 453 | 33 | 206 | 0.15 | Class 5 |
| CFT-115-C30 | 376 | 3.28 | 1125 | 58 | 50 | 305 | 435 | 37 | 181 | 0.17 | Class 5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Han, R.; Yuan, W.; Zhao, S.; Sun, Y. Elastoplastic Analysis of Circular Steel Tube of CFT Stub Columns under Axial Compression. Materials 2022, 15, 8275. https://doi.org/10.3390/ma15228275
Zhao H, Han R, Yuan W, Zhao S, Sun Y. Elastoplastic Analysis of Circular Steel Tube of CFT Stub Columns under Axial Compression. Materials. 2022; 15(22):8275. https://doi.org/10.3390/ma15228275
Chicago/Turabian StyleZhao, Hua, Rui Han, Weiguang Yuan, Shichun Zhao, and Yuping Sun. 2022. "Elastoplastic Analysis of Circular Steel Tube of CFT Stub Columns under Axial Compression" Materials 15, no. 22: 8275. https://doi.org/10.3390/ma15228275
APA StyleZhao, H., Han, R., Yuan, W., Zhao, S., & Sun, Y. (2022). Elastoplastic Analysis of Circular Steel Tube of CFT Stub Columns under Axial Compression. Materials, 15(22), 8275. https://doi.org/10.3390/ma15228275






