Regenerative Braking Control Strategy of Electric-Hydraulic Hybrid (EHH) Vehicle
Abstract
:1. Introduction
2. Structure of the Electric-Hydraulic Hybrid System
3. Models of the Main Components
3.1. Electric Motor Model
3.2. Battery Character
3.3. Secondary Component (Hydraulic Pump/Motor) Model
3.4. Hydraulic Accumulator Model
3.5. Vehicle Braking Dynamic Model
4. Control Strategy Design
4.1. Braking Mode Judgment
4.2. Control Strategy Based on Maximum Energy Recovery Rate
- (1)
- According to the braking demand from the driver, the distribution ratio β is designated. If a/g < 0.1 and β = 1, the front wheels alone provide the braking force; if a/g > 0.1, β = 0.689, the braking force is distributed between the front and rear axles in the ratio β.
- (2)
- If the pressure of the accumulator is higher than its maximum allowed value, the hydraulic regenerative braking system is not activated.
- (3)
- If the hydraulic regenerative braking force can cover the braking demand in the front axle, the braking force in the front is completely provided by the hydraulic system (for example, point A in Figure 4b); otherwise, the hydraulic system provides its maximum allowed value.
- (4)
- If the electric regenerative braking force can cover the insufficient force, the insufficient force is provided by electric braking; otherwise, the electric system provides its maximum.
- (5)
- If the hydraulic braking force and electric braking force together are insufficient, the friction force contributes the insufficient part (for example, point C in Figure 4b).
4.3. Control Strategy Based on Minimum Current Impact
- (1)
- According to the braking demand from the driver, the distribution ratio β is designated. When a/g < 0.1 and β = 1, the front wheels alone provide the braking force; when a/g > 0.1 and β = 0.689, the braking force is distributed between the front and rear axles in the ratio β.
- (2)
- According to the braking force required by the front axle, the current required by the electric motor Ichrg is calculated. If Ichrg < Imax, the braking force generated by the motor completely provides the braking force required by the front axle; Fmreg = βFtotal (for example, point A in Figure 4b). If Ichrg > Imax, the braking force generated by the motor is restricted by the current; Fmreg = f(Imax).
- (3)
- When the generated current is restricted, the braking force generated by the electric motor is insufficient. If the hydraulic regenerative braking force could cover the remaining part of the requirement, the insufficient part is provided by the hydraulic system (for example, point B in Figure 5b). Otherwise, the hydraulic system provides its maximum.
- (4)
- If the hydraulic braking force and electric braking force together are insufficient, notwithstanding the hydraulic system providing its maximum, the friction force contributes the insufficient part (for example, point C in Figure 5b).
4.4. ABS Control Strategy
5. Modeling
5.1. Basic Parameters
5.2. Simulation Models
6. Simulation Results
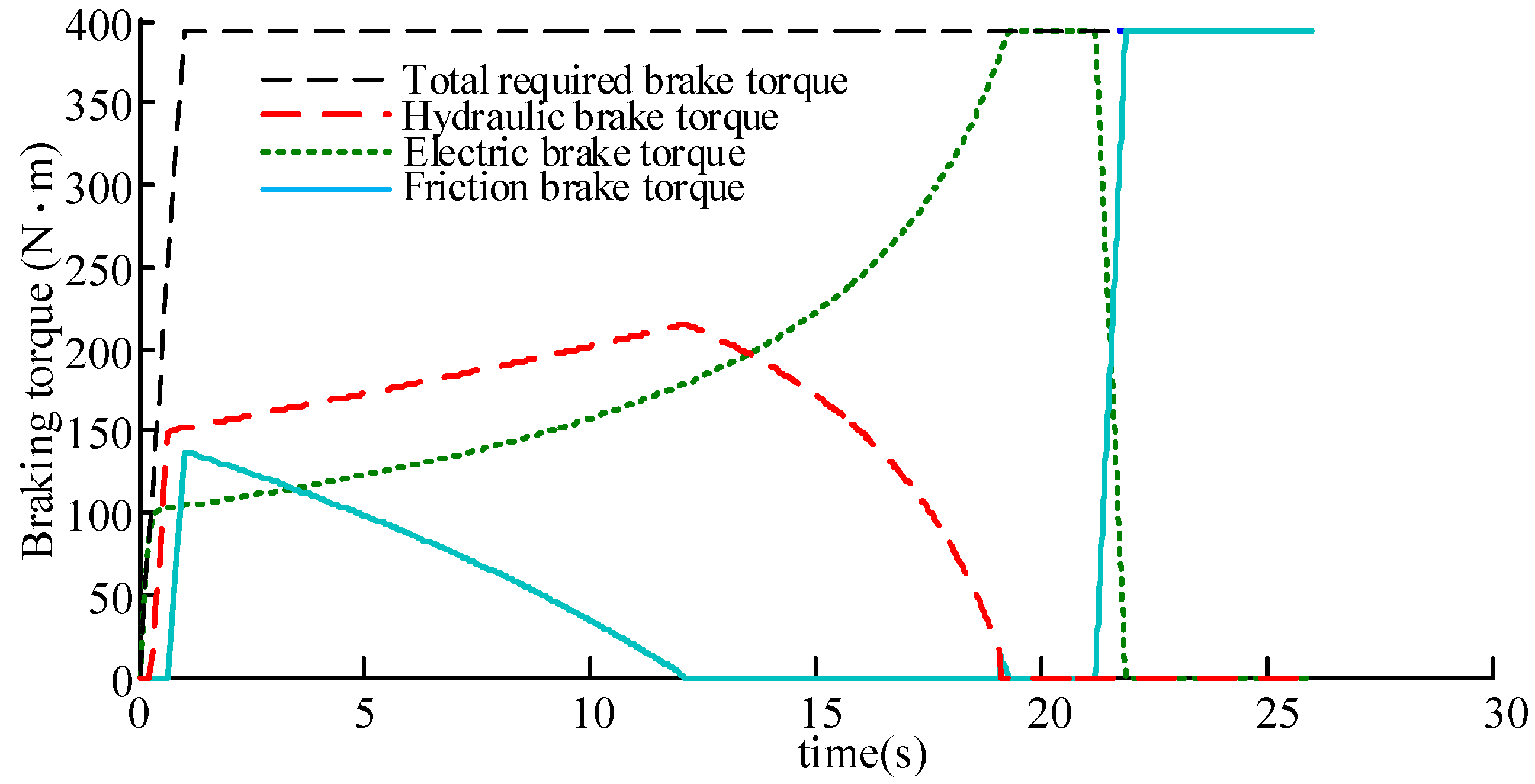
6.1. Simulation Results by Following Maximum Energy Recovery Rate
6.2. Simulation Results by Following Minimum Current Impact
6.3. Changing Deceleration and ABS Response
7. Summary
- (1)
- By incorporating a hydraulic energy storage system into a pure electric prototype, a novel electro-hydraulic hybrid system was designed. This system is aimed at absorbing high power braking energy as well as suppressing the impact of current on the battery.
- (2)
- The simulation models of the components in the powertrain are constructed to form a combined simulation model. Two control strategies, which are based on two separate rules, the maximum energy recovery rate and the minimum charge current impact, are designed and compared. The simulation results indicated the effectiveness of the two strategies; the energy recovery rate is higher when the principle of the maximum energy recovery rate is followed. The charging current is gentle and without fluctuation when the principle of minimum current impact is followed, although the energy recovery rate is lower.
- (3)
- The ABS control strategy is simulated with variable braking deceleration intent. The result demonstrates that the ABS is triggered as soon as the slip ratio crosses the threshold; the wheel slip ratio can then be regulated to around the optimal value to ensure braking safety.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Borba, B.S.M.C.; Szklo, A.; Schaeffer, R. Plug-in hybrid electric vehicles as a way to maximize the integration of variable renewable energy in power systems: The case of wind generation in northeastern Brazil. Energy 2012, 37, 469–481. [Google Scholar] [CrossRef]
- Lu, L.; Han, X.; Li, J.; Hua, J.; Ouyang, M. A review on the key issues for lithium-ion battery management in electric vehicles. J. Power Sources 2013, 226, 272–288. [Google Scholar] [CrossRef]
- Onda, K.; Ohshima, T.; Nakayama, M. Thermal behavior of small lithium-ion battery during rapid charge and discharge cycles. J. Power Sources 2006, 158, 535–542. [Google Scholar] [CrossRef]
- Schaltz, E.; Khaligh, A.; Rasmussen, P.O. Investigation of battery/ultracapacitor energy storage rating for a fuel cell hybrid electric vehicle. In Proceedings of the IEEE Vehicle Power and Propulsion Conference, Harbin, China, 3–5 September 2008; pp. 1–6. [Google Scholar]
- Wu, Y.; Jiang, X.; Xie, J. The reasons of rapid decline in cycle life of Li-ion battery. Batter. Bimon. 2009, 4, 80–82. [Google Scholar]
- Wang, B.; Zhang, J.; Luo, Y. A study on regenerative braking for parallel hybird electric bus. Automot. Eng. 2005, 27, 649–651. [Google Scholar]
- Amjad, S.; Rudramoorthy, R.; Neelakrishnan, S.; Varmana, K.S.R.; Arjunan, T.V. Evaluation of energy requirements for all-electric range of plug-in hybrid electric two-wheeler. Energy 2011, 36, 1623–1629. [Google Scholar] [CrossRef]
- Niu, G.; Shang, F.; Krishnamurthy, M.; Garcia, J.M. Evaluation and selection of accumulator size in electric-hydraulic hybrid (EH2) powertrain. In Proceedings of the IEEE Transportation Electrification Conference and Expo (ITEC), Dearborn, MI, USA, 27–29 June 2016; pp. 1–6. [Google Scholar]
- World’s First Full Hydraulic Hybrid SUV Presented at 2004 SAE World Congress. United States Environmental Protection Agency: Washington, DC, USA, 2004. Available online: https://permanent.access.gpo.gov/gpo60682/P1001T88.PDF (accessed on 11 July 2017).
- PSA Group. Hybrid Air: An Innovative Full-Hybrid Petrol Solution for the Car of the Future. Available online: https://www.groupe-psa.com/en/newsroom/automotive-innovation/hybrid-air/ (accessed on 11 July 2017).
- Luo, C.; Yang, Y.; Li, P. Design and Parameter Matching of a New Electro hydraulic Hybrid Transmission System. In Proceedings of the International Conference on Motion and Power Transmissions, Kyoto, Japan, 1–3 March 2017. [Google Scholar]
- Li, J. Modeling and Simulation on Regenerative Braking System of Hydraulic Hybrid Vehicle Based on AMESim-Simulink. Master’s Thesis, Chongqing University, Chongqing, China, 2013. [Google Scholar]
- Chen, H.; Yuan, S. Design of Hydraulic System for Braking Energy Recovery of Urban Vehicle. Hydraul. Pneum. 2003, 49, 1–3. [Google Scholar]
- Takahiro, N.; Setsuko, K.; Hirobumi, A. Development of a New Regenerative Braking System; SAE International: Warrendale, PA, USA, 2013. [Google Scholar]
- Regulation No 13 of the Economic Commission for Europe of the United Nations (UN/ECE)—Uniform Provisions Concerning the Approval of Vehicles of Categories M, N and O with Regard to Braking [2016/194]. Available online: http://eur-lex.europa.eu/legal-content/EN/TXT/?uri=CELEX:42016X0218(01) (accessed on 11 July 2017).
- Yang, Y. New Electro-hydraulic Braking System for Pure Electric Vehicle. Master’s Thesis, Chongqing University, Chongqing, China, 2012. [Google Scholar]
- Jiang, X. Study on Battery Management System of Lithium-Ion Batteries. Ph.D. Thesis, Graduate University of Chinese Academy of Sciences, Shanghai, China, 2007. [Google Scholar]
- Gao, Y.; Mehrdad, E. Electronic Braking System of EV and HEV—Integration of Regenerative Braking Automatic Braking Force Control and ABS; SAE International: Warrendale, PA, USA, 2001. [Google Scholar]
- Tawadros, P.; Zhang, N. Integration and performance of regenerative braking and energy recovery technologies in vehicles. In Alternative Fuels and Advanced Vehicle Technologies for Improved Enviromental Performance: Towards Zero Carbon Transportation; Woodhead Publishing: Cambridge, UK, 2014; pp. 541–563. [Google Scholar]
- Ko, J.W.; KO, S.Y.; Kim, I.S.; Hyun, D.Y.; Kim, H.S. Co-operative Control for Regenerative Braking and Friction Braking to Increase Energy Recovery without Wheel Lock. Int. J. Automot. Technol. 2014, 15, 253–262. [Google Scholar] [CrossRef]
- Lynn, A.; Smid, E.; Eshraghi, M.; Caldwell, N.; Woody, D. Modeling Hydraulic Regenerative Hybrid Vehicles Using AMESim and Matlab/Simulink. Proc. SPIE 2005, 5805. [Google Scholar] [CrossRef]






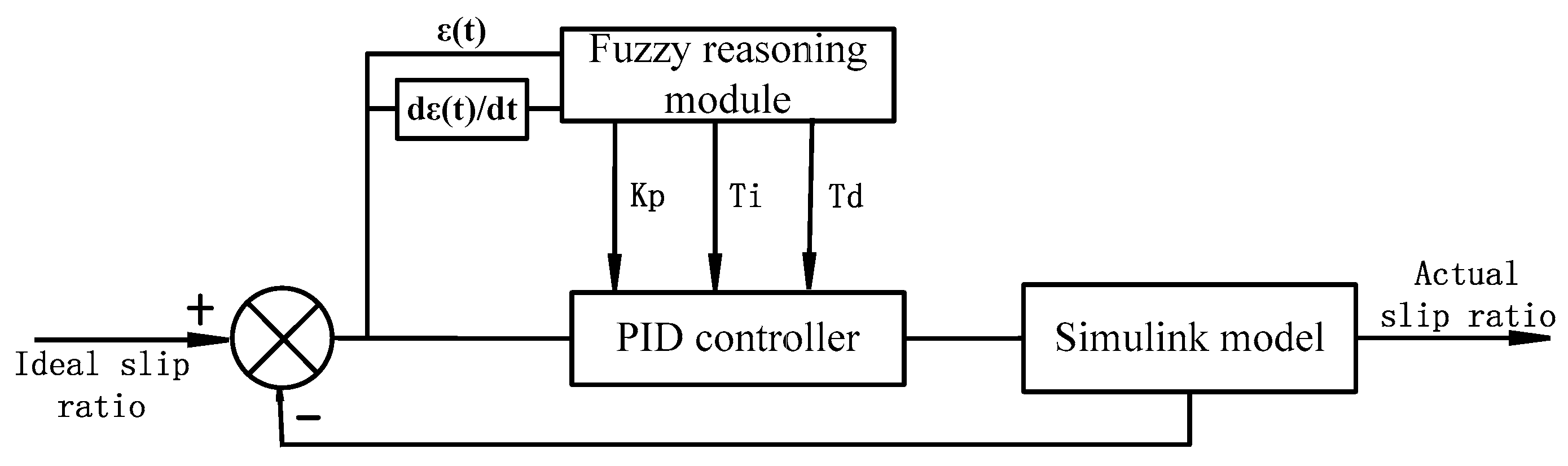












| Judgement Conditions | Results |
|---|---|
| (1) if (E is NB) and (EC is NB) | then (Kp is PB), (Ti is N), (Td is PS) |
| (2) if (E is NB) and (EC is NM) | then (Kp is PB), (Ti is N), (Td is NS) |
| (3) if (E is NB) and (EC is NS) | then (Kp is PM), (Ti is N), (Td is NB) |
| (4) if (E is NB) and (EC is Z) | then (Kp is PM), (Ti is N), (Td is NB) |
| (5) if (E is NB) and (EC is PS) | then (Kp is PS), (Ti is N), (Td is NB) |
| (6) if (E is NB) and (EC is PM) | then (Kp is Z), (Ti is Z), (Td is NM) |
| (7) if (E is NB) and (EC is PB) | then (Kp is Z), (Ti is Z), (Td is PS) |
| ▪ ▪ ▪ ▪ ▪ ▪ | ▪ ▪ ▪ ▪ ▪ ▪ |
| (43) if (E is PB) and (EC is NB) | then (Kp is Z), (Ti is Z), (Td is PB) |
| (44) if (E is PB) and (EC is NM) | then (Kp is Z), (Ti is Z), (Td is PM) |
| (45) if (E is PB) and (EC is NS) | then (Kp is NM), (Ti is NM), (Td is PM) |
| (46) if (E is PB) and (EC is Z) | then (Kp is NM), (Ti is PM), (Td is PM) |
| (47) if (E is PB) and (EC is PS) | then (Kp is NM), (Ti is PM), (Td is PS) |
| (48) if (E is PB) and (EC is PM) | then (Kp is NB), (Ti is PB), (Td is PS) |
| (49) if (E is PB) and (EC is PB) | then (Kp is N), (Ti is PB), (Td is PB) |
| Components | Parameters | Value |
|---|---|---|
| Basic parameters of the vehicle | Loaded mass (m) | 1825 kg |
| Wheel rolling radius (r) | 0.308 m | |
| Wheelbase (L) | 2.28 m | |
| Distance from front axle to mass center (L1) | 1.25 m | |
| Height of the mass center (hg) | 0.585 m | |
| Motor | Rated power (Pr) (kw) | 35 kw |
| Rated rotational speed (nr) | 3000 rpm | |
| Rated torque (Tr) | 111.4 Nm | |
| Battery | Rated voltage (U) | 336 V |
| Capacity | 70 Ah |
| Components | Parameters | Value |
|---|---|---|
| Pump/motor (secondary component) | Maximum displacement (Vp/m max) | 18 cm3 |
| Maximum rotational speed (np/m max) | 3300 rpm | |
| Peak power (Pp/m max) | 30 kw | |
| Peak torque (Tp/m max) | 80 Nm | |
| Accumulator | Volume (Va) | 10 L |
| Working pressure (Pa) | 330 Bar |
| Braking Force Distribution Principle | Energy Recovery Rate of Hydraulic System | Energy Recovery Rate of Electric System | Total Energy Recovery Rate |
|---|---|---|---|
| Maximum energy recovery rate | 43.4% | 50.8% | 94.2% |
| Minimum current impact | 37.6% | 38.9% | 76.5% |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Luo, C.; Li, P. Regenerative Braking Control Strategy of Electric-Hydraulic Hybrid (EHH) Vehicle. Energies 2017, 10, 1038. https://doi.org/10.3390/en10071038
Yang Y, Luo C, Li P. Regenerative Braking Control Strategy of Electric-Hydraulic Hybrid (EHH) Vehicle. Energies. 2017; 10(7):1038. https://doi.org/10.3390/en10071038
Chicago/Turabian StyleYang, Yang, Chang Luo, and Pengxi Li. 2017. "Regenerative Braking Control Strategy of Electric-Hydraulic Hybrid (EHH) Vehicle" Energies 10, no. 7: 1038. https://doi.org/10.3390/en10071038
APA StyleYang, Y., Luo, C., & Li, P. (2017). Regenerative Braking Control Strategy of Electric-Hydraulic Hybrid (EHH) Vehicle. Energies, 10(7), 1038. https://doi.org/10.3390/en10071038




