Economic Analysis for Residential Solar PV Systems Based on Different Demand Charge Tariffs
Abstract
:1. Introduction
2. Methodology
2.1. Simulator Software
2.2. Case Studies
2.3. Price Plans
2.4. PV System
2.5. Evaluation Methods
2.6. Simple Payback Period (PP)
2.7. Net Present Value (NPV)
2.8. The Internal Rate of Return (IRR)
2.9. Profitability Index (PI)
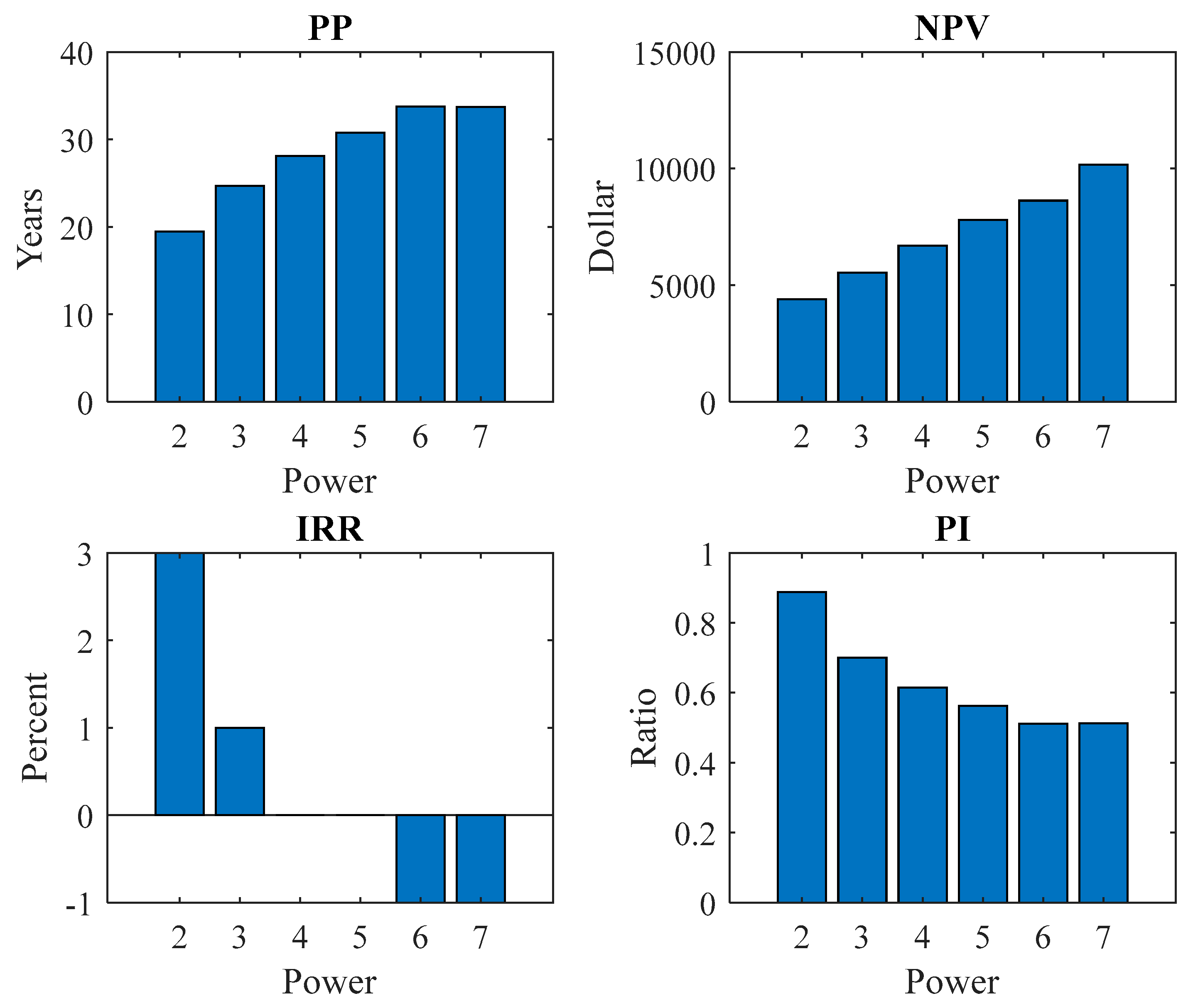
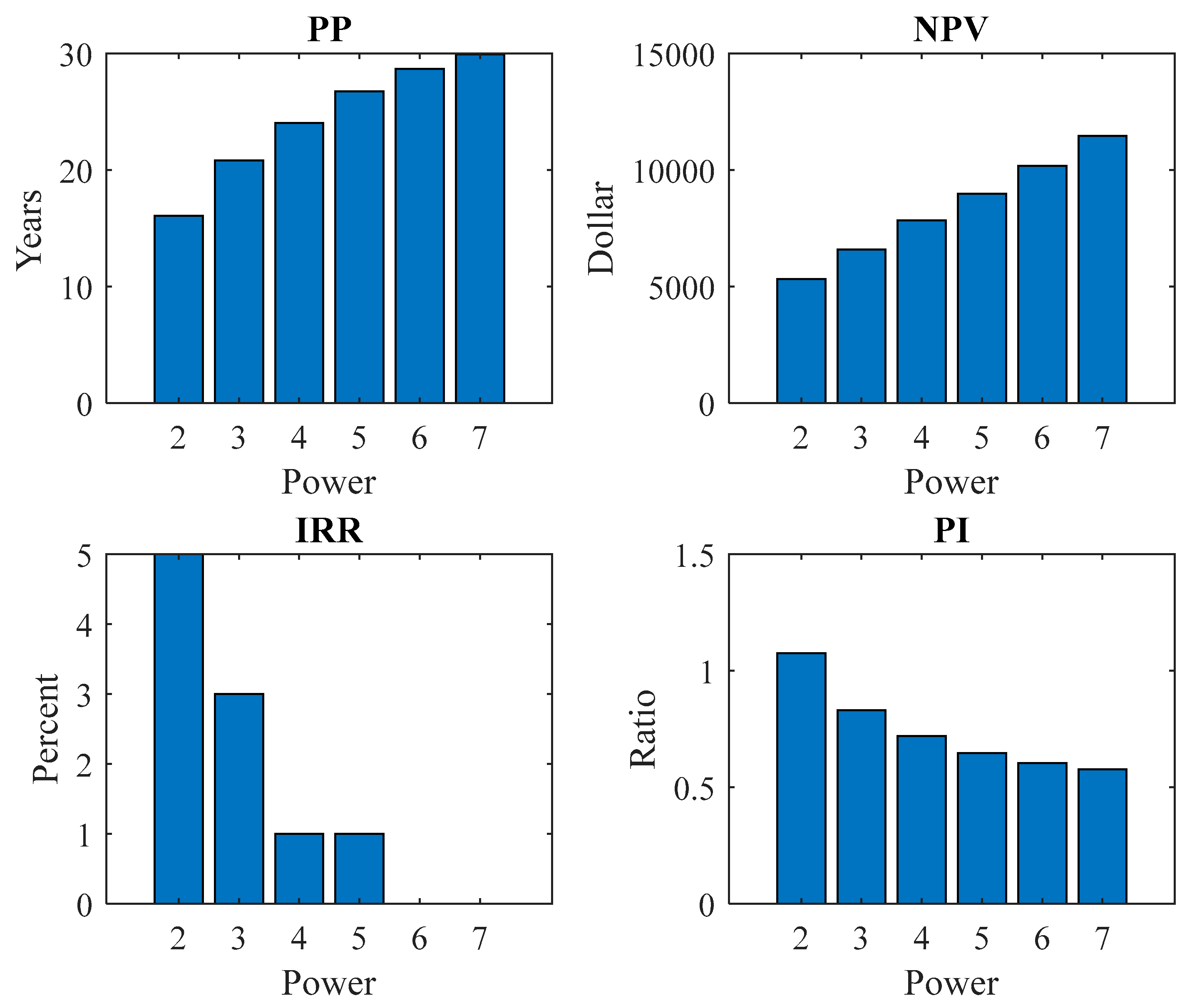
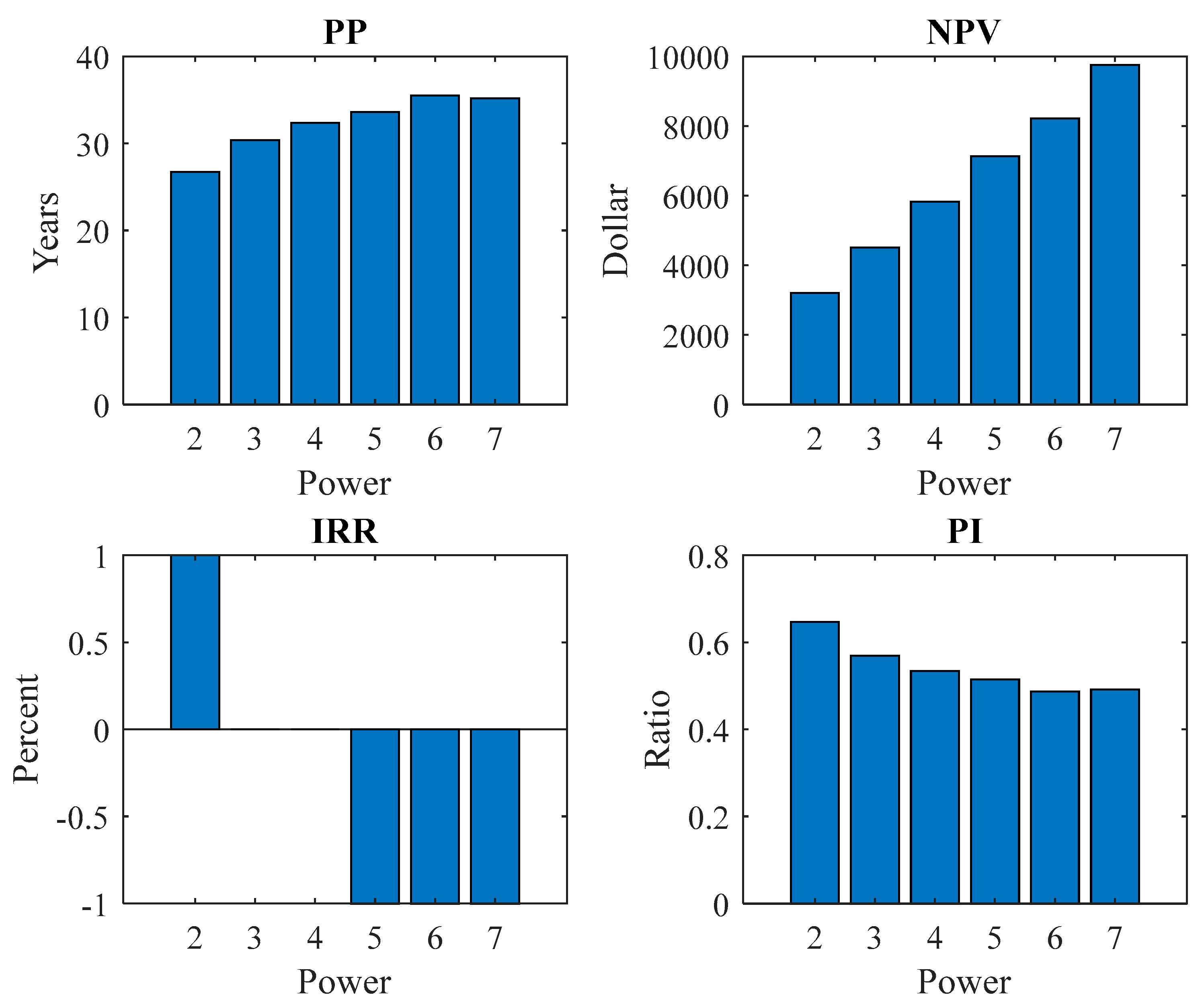
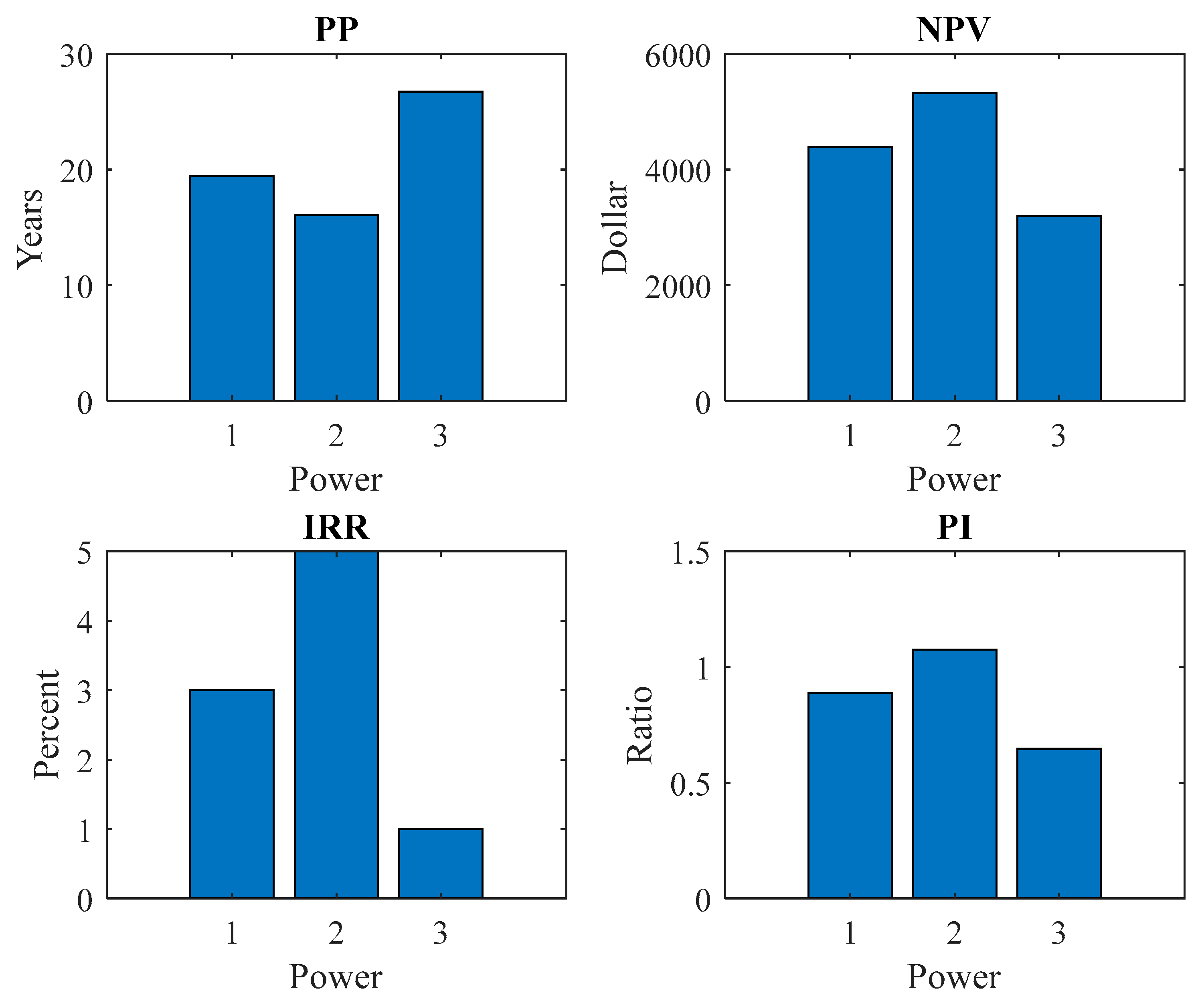
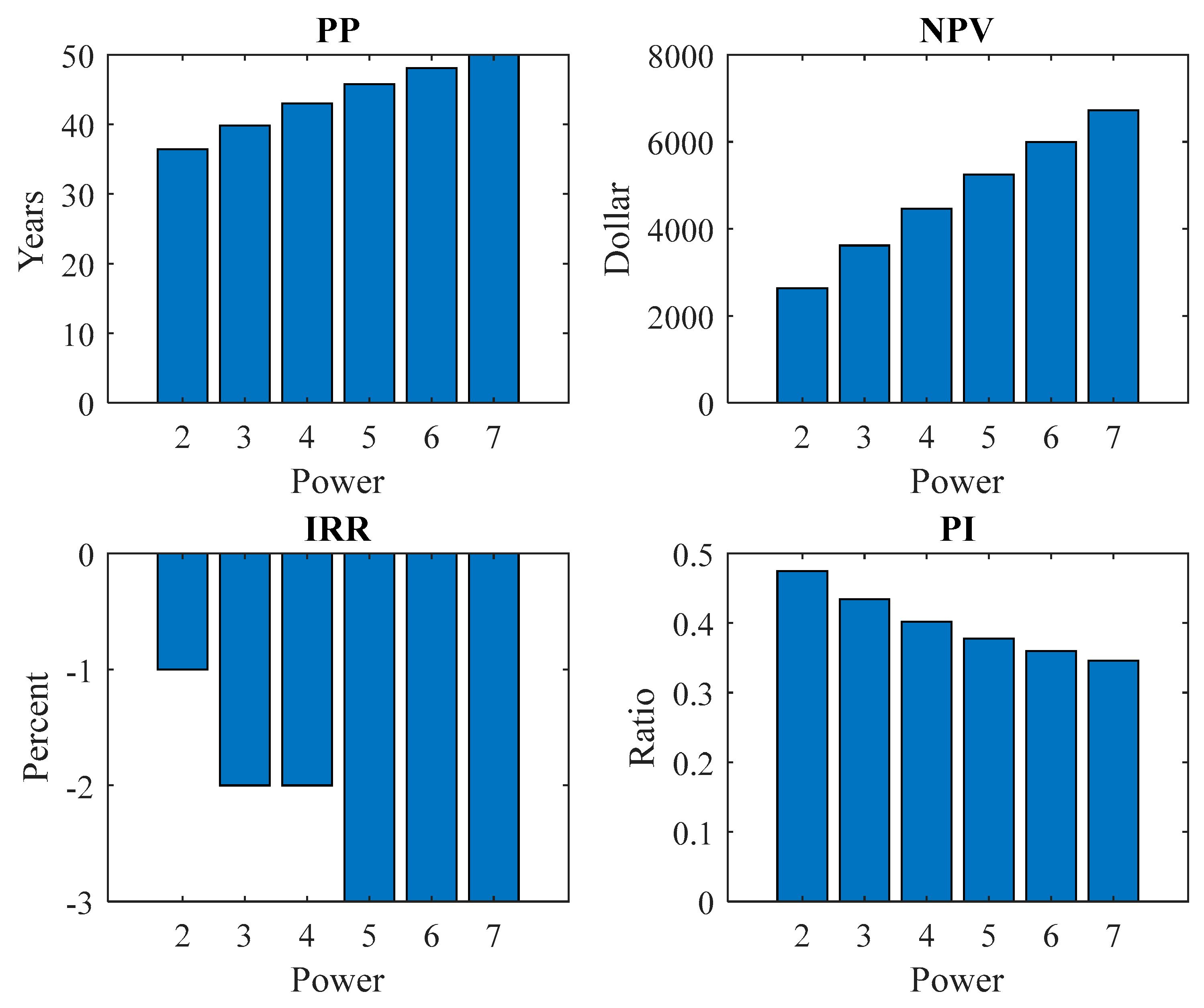
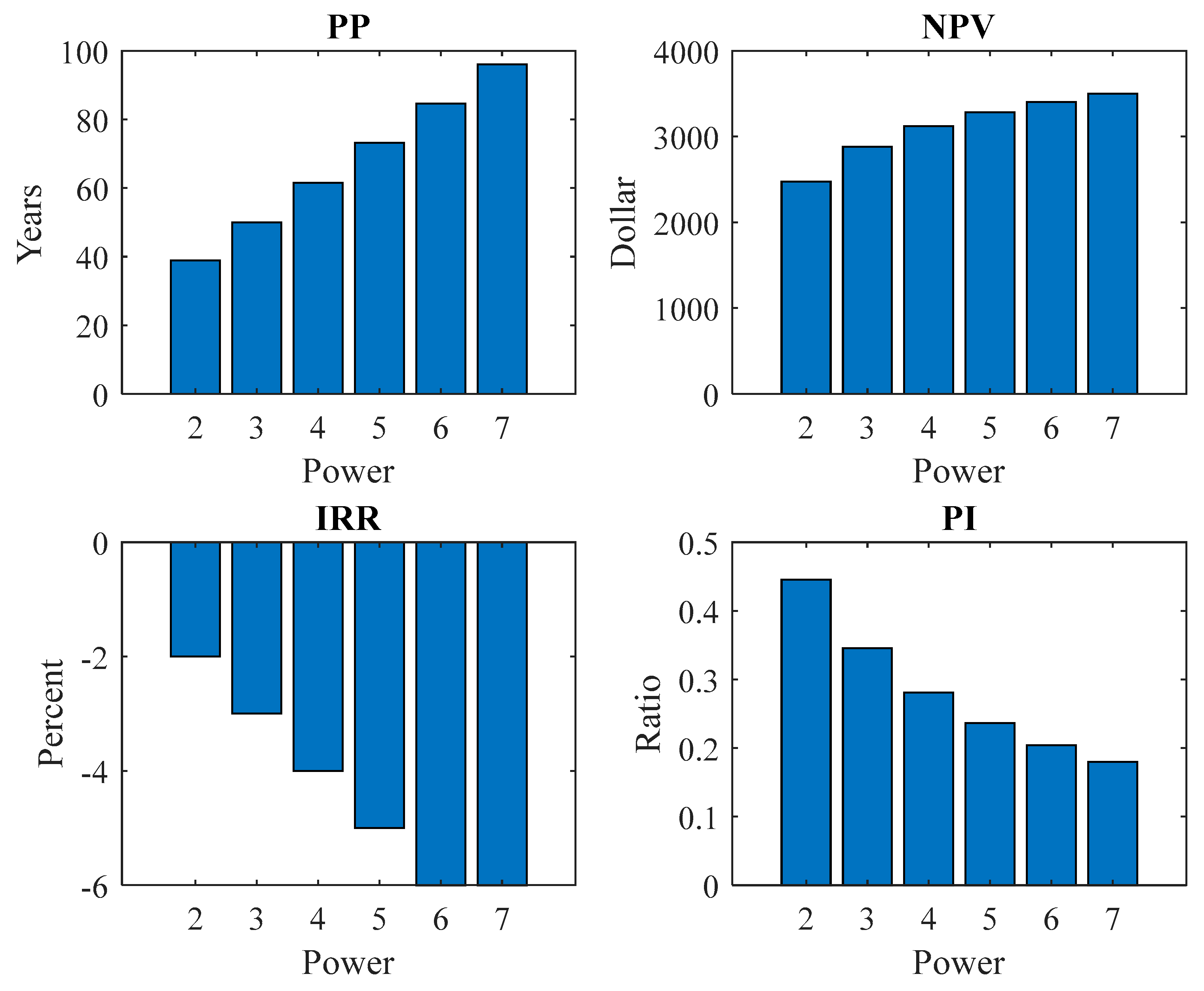
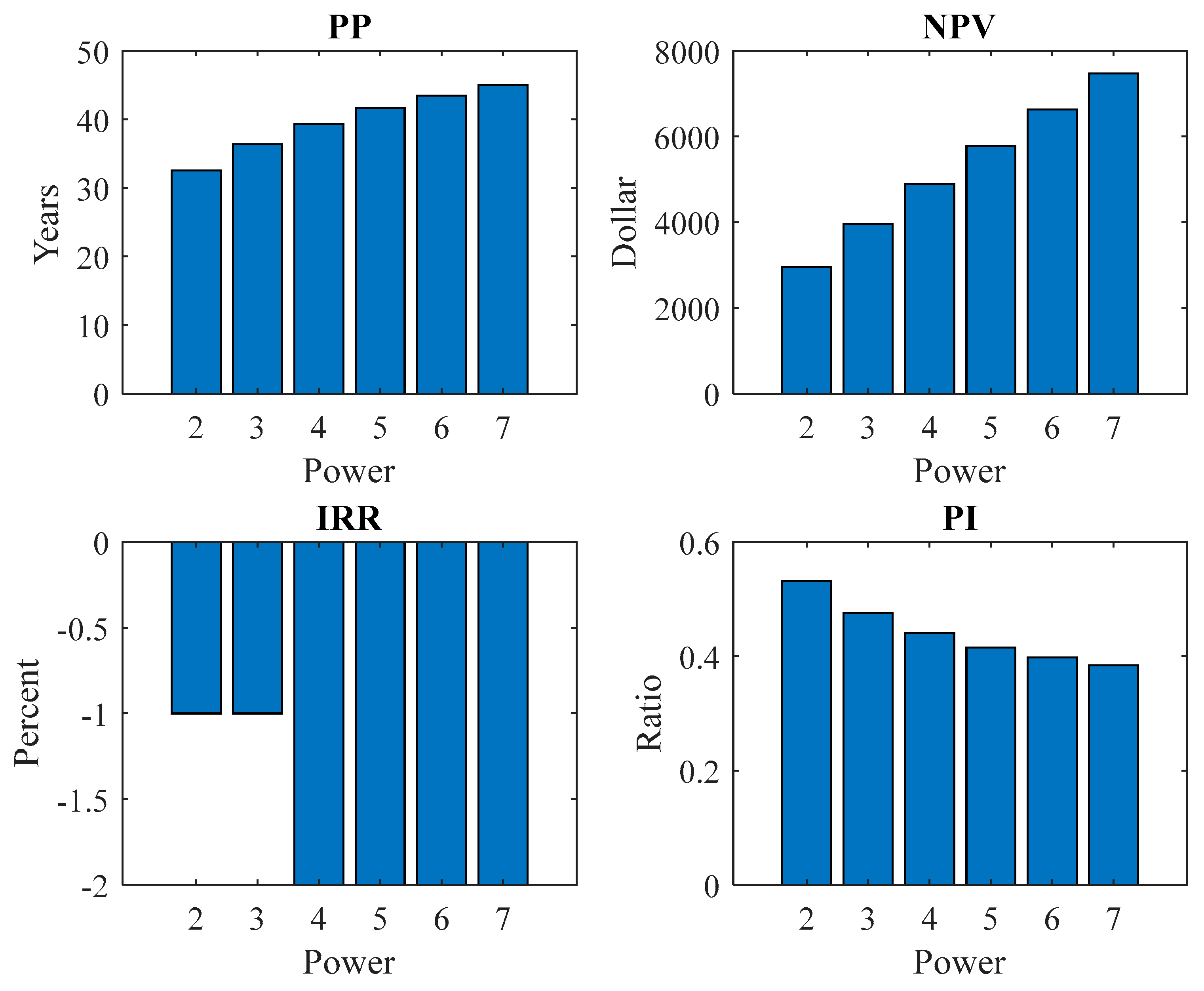
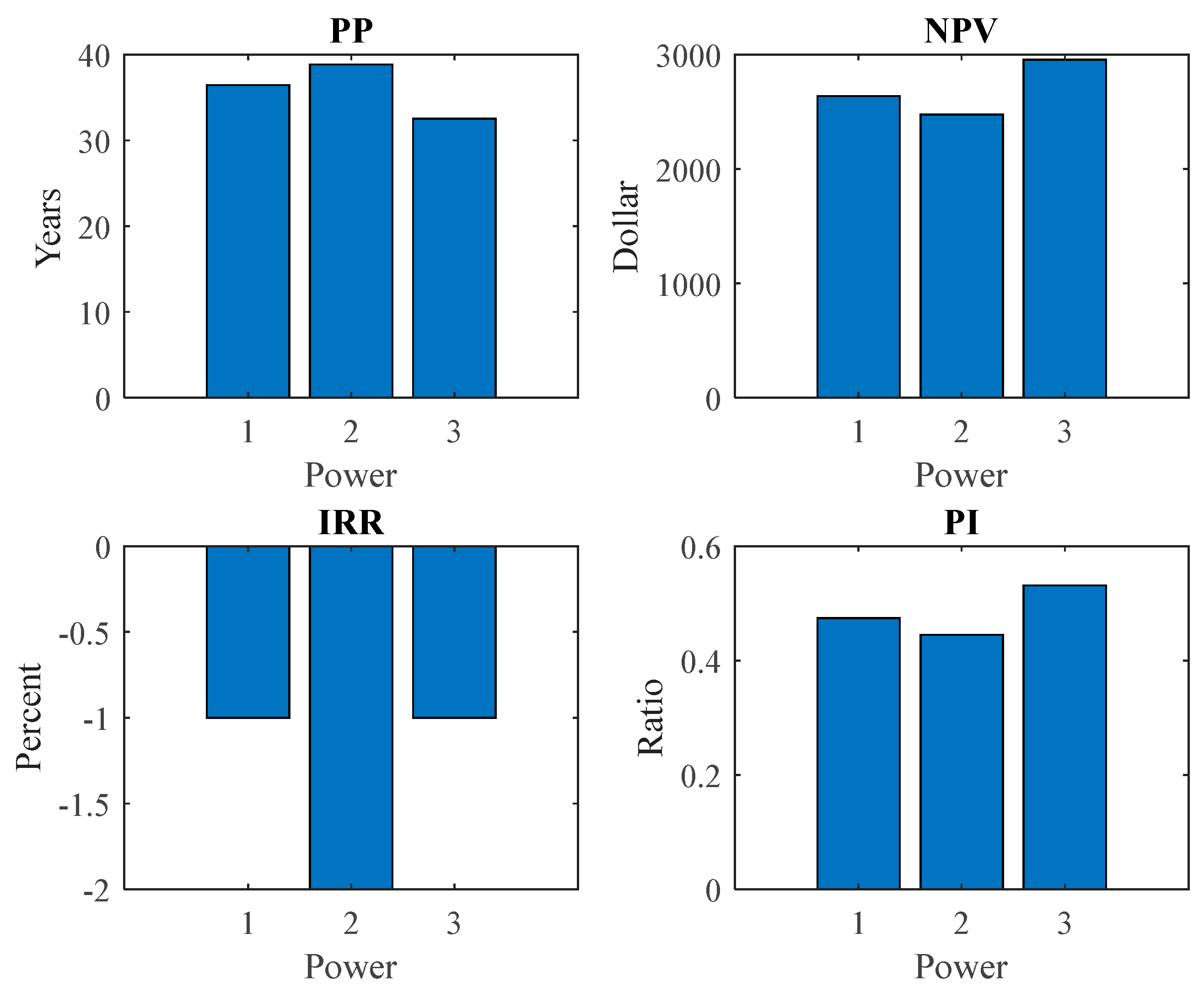
3. Results and Discussion
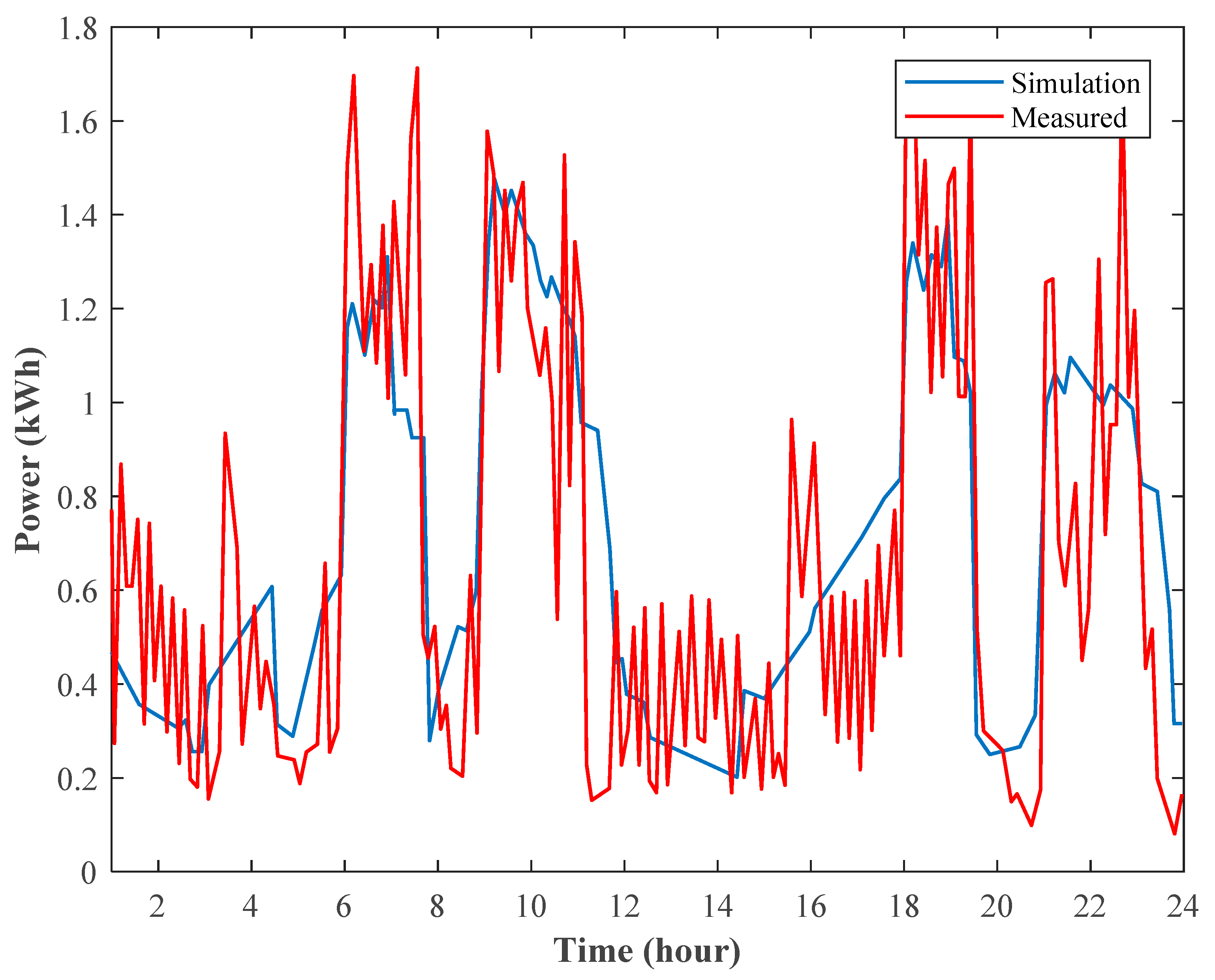
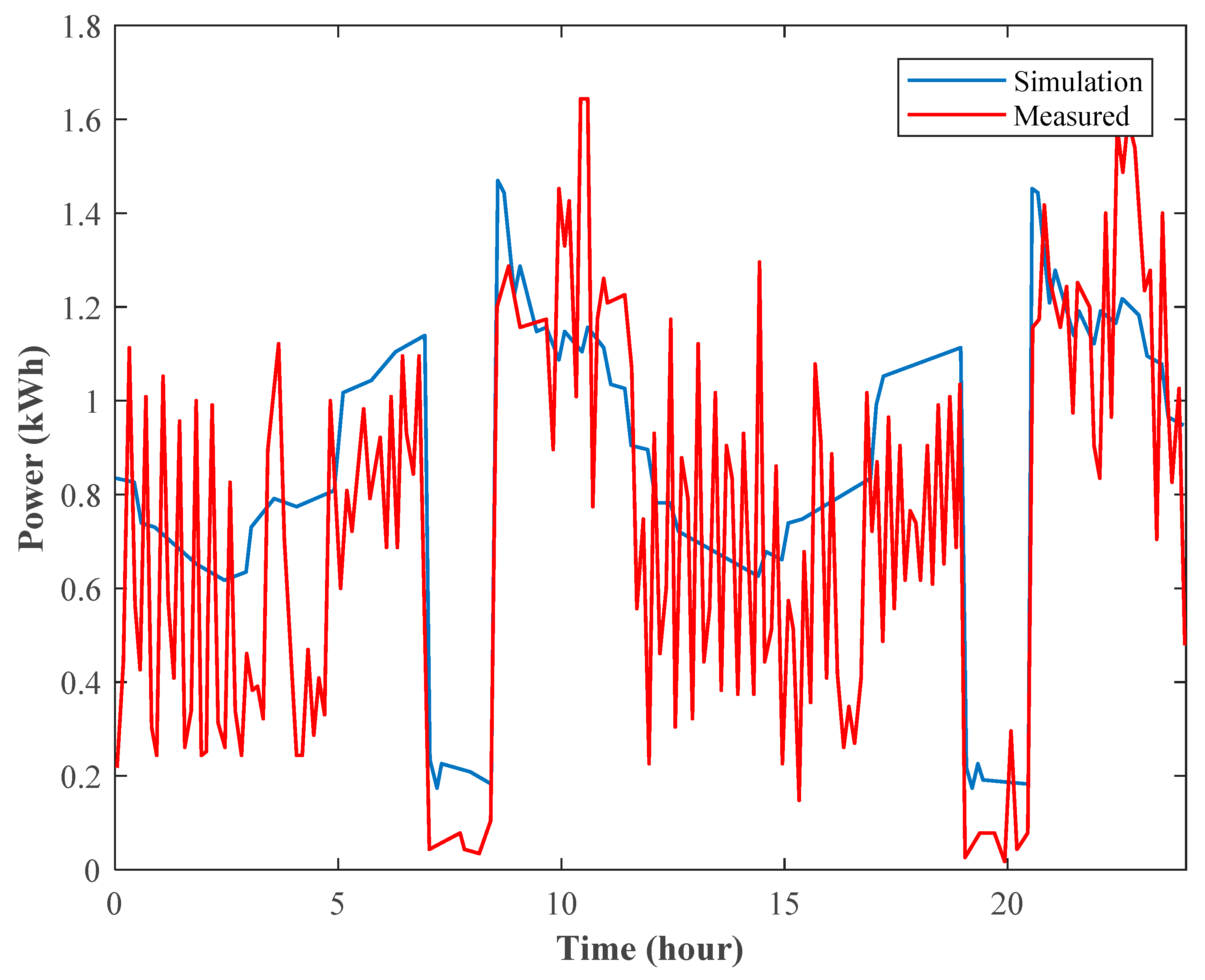
3.1. Validation
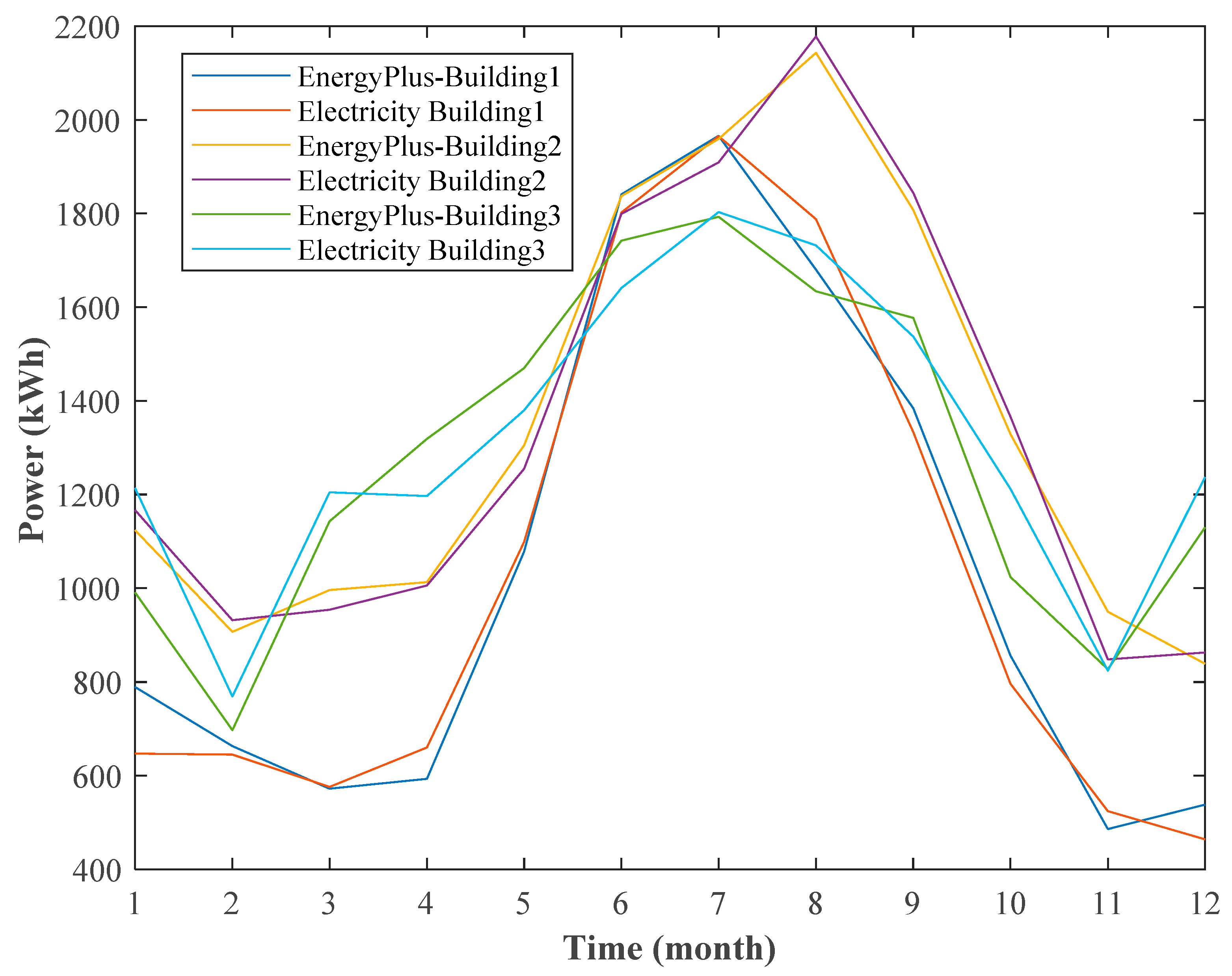
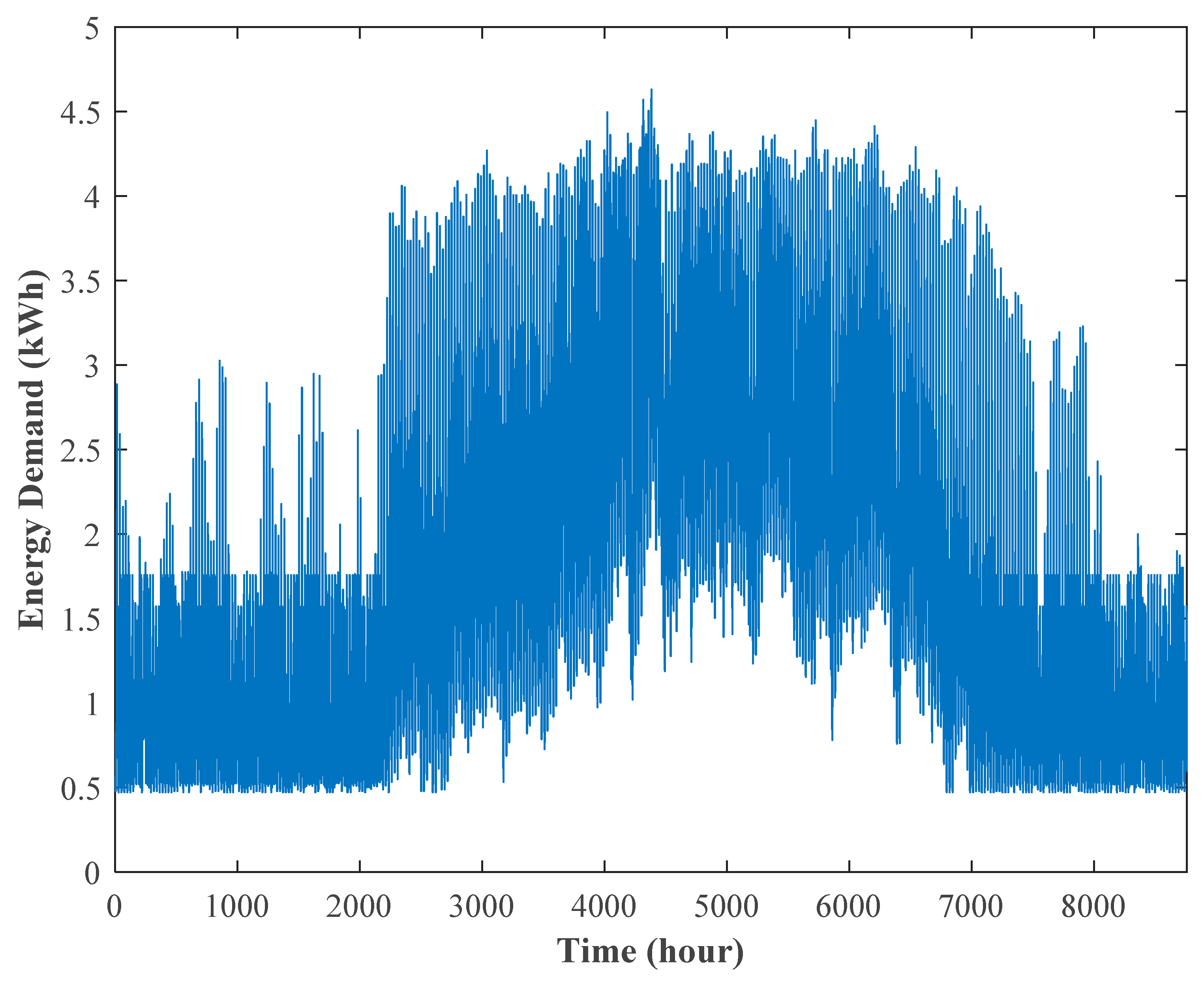
3.2. EnergyPlus Simulation Results
3.3. Economical Comparison
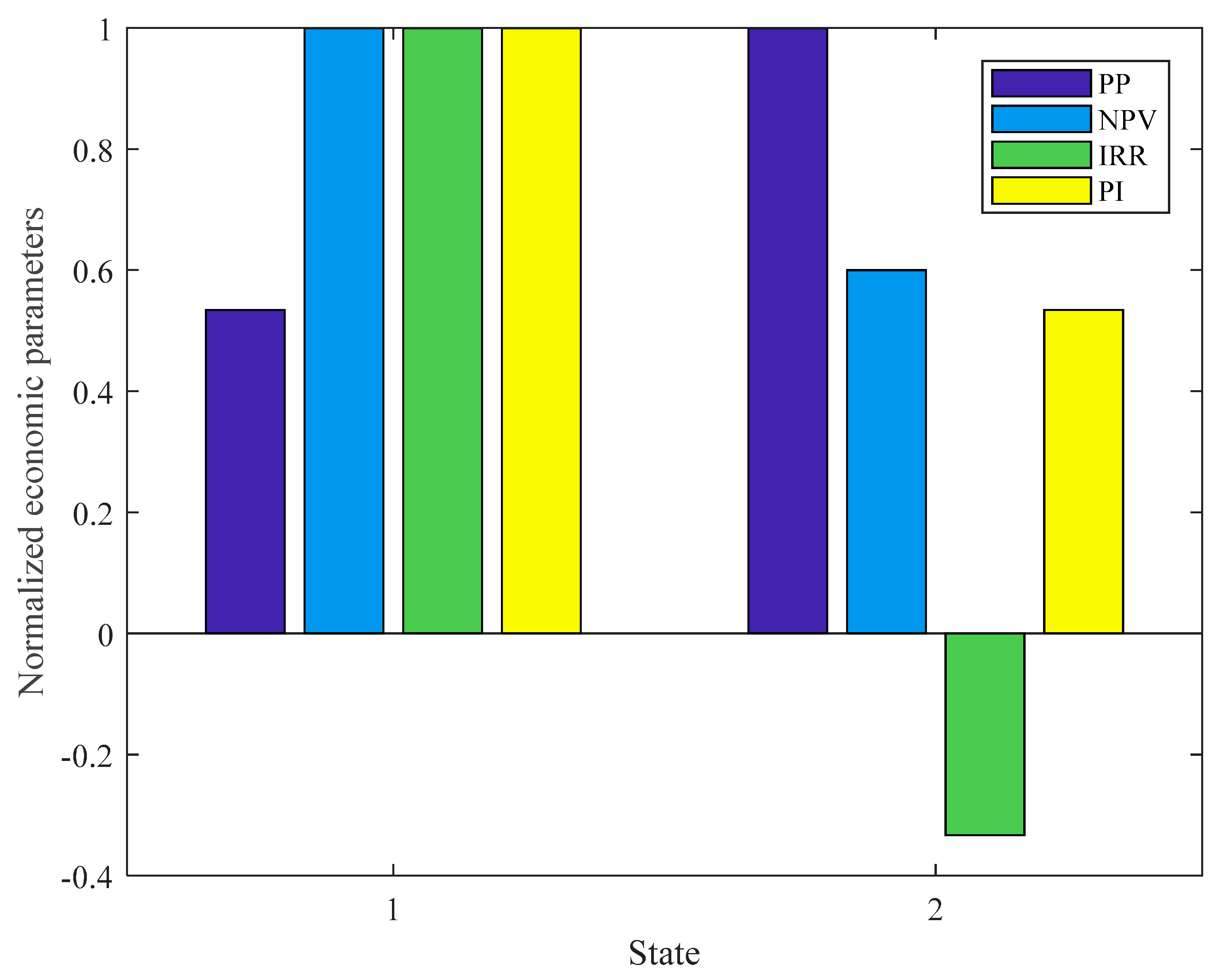
3.4. State A
3.5. State B
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Horrigan, L.; Robert, S.L.; Walker, P. How sustainable agriculture can address the environmental and human health harms of industrial agriculture. Environ. Health Perspect. 2002, 110, 445–456. [Google Scholar] [CrossRef] [PubMed]
- Chu, S.; Cui, Y.; Liu, N. The path towards sustainable energy. Nat. Mater. 2017, 16, 16. [Google Scholar] [CrossRef] [PubMed]
- Solomon, S.; Qin, D.; Manning, M.; Averyt, K.; Marquis, M. Climate Change 2007—The Physical Science Basis: Working Group I Contribution to the Fourth Assessment Report of the IPCC; Cambridge University Press: Cambridge, UK, 2007; Volume 4. [Google Scholar]
- Berinstein, P. Alternative Energy: Facts, Statistics, and Issues; Oryx Press: Westport, CT, USA, 2001; pp. 1–190. [Google Scholar]
- Foster, R.; Ghassemi, M.; Cota, A. Solar Energy: Renewable Energy and the Environment; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2010; pp. 233–248. [Google Scholar]
- Masschelein, W.J.; Rice, R.G. Ultraviolet Light in Water and Wastewater Sanitation; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Renewable Energy Policy Network for the 21st Centry. Global Status Report; REN21 Secretariat: Paris, France, 2016. [Google Scholar]
- Breyer, C.; Bogdanov, D.; Gulagi, A.; Aghahosseini, A.; Barbosa, L.S.; Koskinen, O.; Barasa, M.; Caldera, U.; Afanasyeva, S.; Child, M. On the role of solar photovoltaics in global energy transition scenarios. Progr. Photovolt. Res. Appl. 2017, 25, 727–745. [Google Scholar] [CrossRef]
- Solangi, K.; Islam, M.; Saidur, R.; Rahim, N.; Fayaz, H. A review on global solar energy policy. Renew. Sustain. Energy Rev. 2011, 15, 2149–2163. [Google Scholar] [CrossRef]
- Brito, M.; Gomes, N.; Santos, T.; Tenedório, J. Photovoltaic potential in a lisbon suburb using lidar data. Sol. Energy 2012, 86, 283288. [Google Scholar] [CrossRef]
- Grätzel, M. Photoelectrochemical cells. Nature 2001, 414, 338. [Google Scholar] [CrossRef] [PubMed]
- Grubišić-Čabo, F.; Nižetić, S.; Giuseppe Marco, T.J.T.O.F. Photovoltaic panels: A review of the cooling techniques. Trans. FAMENA 2016, 40, 63–74. [Google Scholar]
- Das, N.; Wongsodihardjo, H.; Islam, S.J.R.E. Modeling of multi-junction photovoltaic cell using matlab/simulink to improve the conversion efficiency. Renew. Energy 2015, 74, 917–924. [Google Scholar] [CrossRef]
- Das, N.; Wongsodihardjo, H.; Islam, S. A preliminary study on conversion efficiency improvement of a multi-junction pv cell with mppt. In Smart Power Systems and Renewable Energy System Integration; Springer: Berlin, Germany, 2016; pp. 49–73. [Google Scholar]
- Hammonds, M. Getting power from the sun. Chem. Ind. 1998, 6, 219. [Google Scholar]
- Burns, J.E.; Kang, J.-S. Comparative economic analysis of supporting policies for residential solar pv in the united states: Solar renewable energy credit (srec) potential. Energy Policy 2012, 44, 217–225. [Google Scholar] [CrossRef]
- Nejat, P.; Jomehzadeh, F.; Taheri, M.M.; Gohari, M.; Majid, M.Z.A. A global review of energy consumption, CO2 emissions and policy in the residential sector (with an overview of the top ten CO2 emitting countries). Renew. Sustain. Energy Rev. 2015, 43, 843–862. [Google Scholar] [CrossRef]
- Nižetić, S.; Papadopoulos, A.; Tina, G.; Rosa-Clot, M.J.E. Hybrid energy scenarios for residential applications based on the heat pump split air-conditioning units for operation in the mediterranean climate conditions. Energy Build. 2017, 140, 110–120. [Google Scholar] [CrossRef]
- Bernal-Agustín, J.L.; Dufo-López, R. Economical and environmental analysis of grid connected photovoltaic systems in spain. Renew. Energy 2006, 31, 1107–1128. [Google Scholar] [CrossRef]
- Oliver, M.; Jackson, T. Energy and economic evaluation of building-integrated photovoltaics. Energy 2001, 26, 431–439. [Google Scholar] [CrossRef]
- Shaw-Williams, D.; Susilawati, C.; Walker, G. Value of residential investment in photovoltaics and batteries in networks: A techno-economic analysis. Energies 2018, 11, 1022. [Google Scholar] [CrossRef]
- Mahmud, M.; Huda, N.; Farjana, S.; Lang, C. Environmental impacts of solar-photovoltaic and solar-thermal systems with life-cycle assessment. Energies 2018, 11, 2346. [Google Scholar] [CrossRef]
- Shah, S.; Valasai, G.; Memon, A.; Laghari, A.; Jalbani, N.; Strait, J. Techno-economic analysis of solar pv electricity supply to rural areas of Balochistan, Pakistan. Energies 2018, 11, 1777. [Google Scholar] [CrossRef]
- Zsiborács, H.; Baranyai, N.H.; Vincze, A.; Háber, I.; Pintér, G. Economic and technical aspects of flexible storage photovoltaic systems in Europe. Energies 2018, 11, 1445. [Google Scholar] [CrossRef]
- Kolhe, M.; Kolhe, S.; Joshi, J. Economic viability of stand-alone solar photovoltaic system in comparison with diesel-powered system for India. Energy Econ. 2002, 24, 155–165. [Google Scholar] [CrossRef]
- Ajan, C.W.; Ahmed, S.S.; Ahmad, H.B.; Taha, F.; Zin, A.A.B.M. On the policy of photovoltaic and diesel generation mix for an off-grid site: East malaysian perspectives. Sol. Energy 2003, 74, 453–467. [Google Scholar] [CrossRef]
- Nafeh, E.-S.A. Design and economic analysis of a stand-alone PV system to electrify a remote area household in Egypt. Open Renew. Energy J. 2009, 2, 33–37. [Google Scholar] [CrossRef]
- Ojosu, J. The iso-radiation map for Nigeria. Sol. Wind Technol. 1990, 7, 563–575. [Google Scholar] [CrossRef]
- Rodrigues, S.; Chen, X.; Morgado-Dias, F.J.E.P. Economic analysis of photovoltaic systems for the residential market under China’s new regulation. Energy Policy 2017, 101, 467–472. [Google Scholar] [CrossRef]
- Rodrigues, S.; Faria, F.; Cafôfo, N.; Chen, X.; Mata-Lima, H.; Morgado-Dias, F.J.J.C.E.T. Analysis of the self-consumption regulation for photovoltaic systems with battery banks in the portuguese residential sector. J. Clean. Energy Technol. 2017, 5, 52–59. [Google Scholar] [CrossRef]
- Rodrigues, S.; Torabikalaki, R.; Faria, F.; Cafôfo, N.; Chen, X.; Ivaki, A.R.; Mata-Lima, H.; Morgado-Dias, F.J.S.E. Economic feasibility analysis of small scale PV systems in different countries. Sol. Energy 2016, 131, 81–95. [Google Scholar] [CrossRef]
- Arababadi, R.; Parrish, K. Modeling and testing multiple precooling strategies in three residential building types in the phoenix climate. ASHRAE Trans. 2016, 122, 202–214. [Google Scholar]
- Arababadi, R.; Parrish, K. Reducing the need for electrical storage by coupling solar pvs and precooling in three residential building types in the phoenix climate. ASHRAE Trans. 2017, 123, 279–290. [Google Scholar]
- Griffith, B.T.; Ellis, P. Photovoltaic and Solar Thermal Modeling with the Energyplus Calculation Engine; National Renewable Energy Lab.: Golden, CO, USA, 2004. [Google Scholar]



| Input Parameters | Building 1 | Building 2 | Building 3 |
|---|---|---|---|
| Total floor area | 147 m2 (1584 ft2) | 183 m2 (1978 ft2) | 143 m2 (1540 ft2) |
| Floor height | 3.92 m (9.6 ft) | 2.7m (9 ft) | 2.4 m (8 ft) |
| Window-wall ratio, % | 11.2 | 12.33 | 9.35 |
| Exterior wall U-factor | 0.75 (W/m2·K) (0.1321 Btu/h·ft2·°F) | 0.429 (W/m2·K) (0.0755 Btu/h·ft2·°F) | 0.85 (W/m2·K) (0.1497 Btu/h·ft2·°F) |
| Floor U-factor | 0.31 (W/m2·K) (0.0546 Btu/h·ft2·°F) | 0.51 (W/m2·K) (0.0898 Btu/h·ft2·°F) | 1.17 (W/m2·K) (0.2060 Btu/h·ft2·°F) |
| Roof U-factor | 0.21 (W/m2·K) (0.0370 Btu/h·ft2·°F) | 0.12 (W/m2·K) (0.0211 Btu/h·ft2·°F) | 0.15 (W/m2·K) (0.0264 Btu/h·ft2·°F) |
| Window U-factor | 0.75 (W/m2·K) (0.1321 Btu/h·ft2·°F) | 2.72 (W/m2·K) (0.4790 Btu/h·ft2·°F) | 1.57 (W/m2·K) (0.2765 Btu/h·ft2·°F) |
| Infiltration | 0.53 ach | 0.53 ach | 0.53 ach |
| HVAC coefficient of performance | 2.45 | 3.63 | 2.43 |
| HVAC energy efficiency ratio | 8.37 | 2.38 | 8.29 |
| Lighting power density | 13 W/m2 (1.2 W/ft2) | 14 W/m2 (1.3 W/ft2) | 9.6 W/m2 (0.9 W/ft2) |
| Plug loads power density | 5.3 W/m2 (0.5 W/ft2) | 4 W/m2 (0.4 W/ft2) | 3.4 W/m2 (0.3 W/ft2) |
| People | 49 m2/person (527 ft2/person) | 46 m2/person (495 ft2/person) | 35 m2/person (376 ft2/person) |
| Month | On-Peak Hours | First 3 kW | Next 7 kW | All Add’1 kW | On-Peak kWh | Off-Peak kWh | Monthly Service Charge |
|---|---|---|---|---|---|---|---|
| Jan. | 5 a.m. to 9 a.m. and from 5 p.m. to 9 p.m. | 3.55 | 5.68 | 9.74 | 0.043 | 0.0390 | 32.44 |
| Feb. | 5 a.m. to 9 a.m. and from 5 p.m. to 9 p.m. | 3.55 | 5.68 | 9.74 | 0.043 | 0.0390 | 32.44 |
| Mar. | 5 a.m. to 9 a.m. and from 5 p.m. to 9 p.m. | 3.55 | 5.68 | 9.74 | 0.043 | 0.0390 | 32.44 |
| Apr. | 5 a.m. to 9 a.m. and from 5 p.m. to 9 p.m. | 3.55 | 5.68 | 9.74 | 0.043 | 0.0390 | 32.44 |
| May | 1 p.m. to 8 p.m. | 8.03 | 14.63 | 27.77 | 0.0486 | 0.0360 | 30.94 |
| Jun. | 1 p.m. to 8 p.m. | 8.03 | 14.63 | 27.77 | 0.0486 | 0.0360 | 30.94 |
| Jul. | 1 p.m. to 8 p.m. | 9.59 | 17.82 | 34.19 | 0.0633 | 0.0412 | 30.94 |
| Aug. | 1 p.m. to 8 p.m. | 9.59 | 17.82 | 34.19 | 0.0633 | 0.0412 | 30.94 |
| Sep. | 1 p.m. to 8 p.m. | 8.03 | 14.63 | 27.77 | 0.0486 | 0.0360 | 32.44 |
| Oct. | 1 p.m. to 8 p.m. | 8.03 | 14.63 | 27.77 | 0.0486 | 0.0360 | 32.44 |
| Nov. | 5 a.m. to 9 a.m. and from 5 p.m. to 9 p.m. | 3.55 | 5.68 | 9.74 | 0.043 | 0.0390 | 32.44 |
| Dec. | 5 a.m. to 9 a.m. and from 5 p.m. to 9 p.m. | 3.55 | 5.68 | 9.74 | 0.043 | 0.0390 | 32.44 |
| Month | $/kW | $/kWh Off-Peak | $/kWh On-Peak | Monthly Service Charge |
|---|---|---|---|---|
| Jan. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Feb. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Mar. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Apr. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| May | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Jun. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Jul. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Aug. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Sep. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Oct. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Nov. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| Dec. | 8.25 | 0.02934 | 0.0643 | 15.5 |
| PV System Power (kW) | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|
| Solar system cost | 8500 | 12,750 | 17,000 | 21,250 | 25,500 | 29,750 |
| Total rebate | 3550 | 4825 | 6100 | 7375 | 8650 | 9925 |
| Total cost | 4950 | 7925 | 10,900 | 13,875 | 16,850 | 19,850 |
| Sample Building | NMBE | CVRMSE |
|---|---|---|
| 1 | −2.6 | 8.7 |
| 2 | 5.0 | 3.4 |
| 3 | 1.2 | 6.3 |
| PV Power (kW) | 0 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| Annual Electricity costs Building 1-case 1 ($) | 1579.28 | 1325.14 | 1258.42 | 1191.75 | 1128.41 | 1080.45 | 991.51 |
| Annual Electricity costs Building 1-case 2 ($) | 109272 | 940.25 | 883.39 | 834.47 | 789.21 | 745.87 | 703.62 |
| Annual Electricity costs Building 2-case 1 ($) | 1585.31 | 1277.44 | 1204.60 | 1131.94 | 1066.36 | 997.35 | 922.69 |
| Annual Electricity costs Building 2-case 2 ($) | 897.04 | 753.97 | 730.39 | 716.55 | 707.21 | 700.13 | 694.53 |
| Annual Electricity costs Building 3-case 1 ($) | 1229.03 | 1043.89 | 968.19 | 892.41 | 816.34 | 754.16 | 665.24 |
| Annual Electricity costs Building 3-case 2 ($) | 995.14 | 824.26 | 765.89 | 712.34 | 661.29 | 611.70 | 563.08 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeraatpisheh, M.; Arababadi, R.; Saffari Pour, M. Economic Analysis for Residential Solar PV Systems Based on Different Demand Charge Tariffs. Energies 2018, 11, 3271. https://doi.org/10.3390/en11123271
Zeraatpisheh M, Arababadi R, Saffari Pour M. Economic Analysis for Residential Solar PV Systems Based on Different Demand Charge Tariffs. Energies. 2018; 11(12):3271. https://doi.org/10.3390/en11123271
Chicago/Turabian StyleZeraatpisheh, Milad, Reza Arababadi, and Mohsen Saffari Pour. 2018. "Economic Analysis for Residential Solar PV Systems Based on Different Demand Charge Tariffs" Energies 11, no. 12: 3271. https://doi.org/10.3390/en11123271
APA StyleZeraatpisheh, M., Arababadi, R., & Saffari Pour, M. (2018). Economic Analysis for Residential Solar PV Systems Based on Different Demand Charge Tariffs. Energies, 11(12), 3271. https://doi.org/10.3390/en11123271






