A Thermoelectric Performance Study of Layered Bi2TeI Weak Topological Insulator Materials
Abstract
:1. Introduction
2. Experimental
3. Results and Discussion
3.1. Synthesis and TE Properties of Bi2TeI
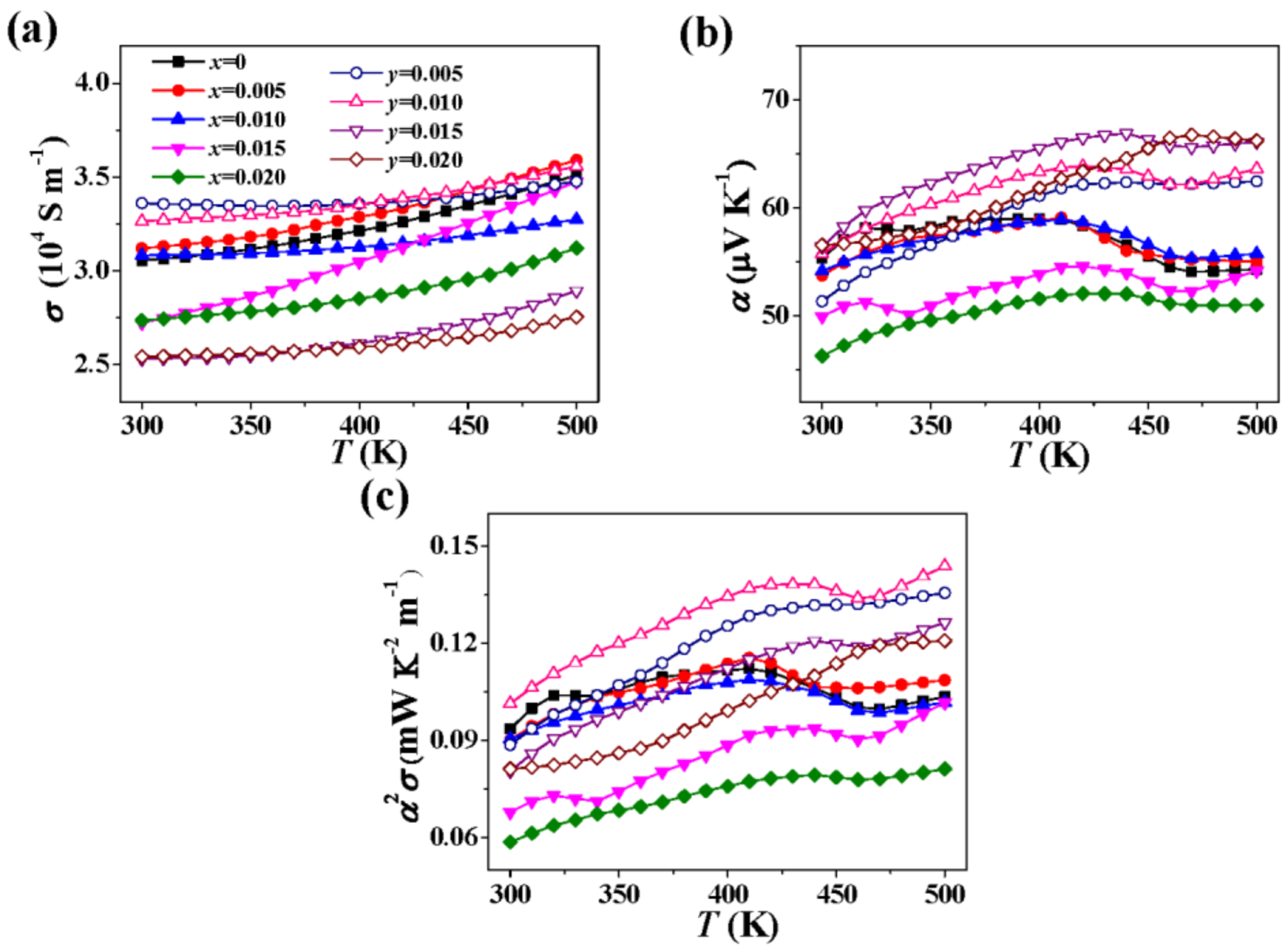
3.2. TE Properties Optimization of Doped Bi2TeI
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Snyder, G.J.; Toberer, E.S. Complex thermoelectric materials. Nature Mater. 2008, 7, 105–114. [Google Scholar] [CrossRef] [PubMed]
- Zhu, T.J.; Liu, Y.T.; Fu, C.G.; Heremans, J.P.; Snyder, J.G.; Zhao, X.B. Compromise and synergy in high-efficiency thermoelectric materials. Adv. Mater. 2017, 29, 1–26. [Google Scholar] [CrossRef] [PubMed]
- Shi, X.; Chen, L.; Uher, C. Recent advances in high-performance bulk thermoelectric materials. Inter. Mater. Rev. 2016, 61, 379–415. [Google Scholar] [CrossRef]
- Sootsman, J.R.; Chung, D.Y.; Kanatzidis, M.G. New and old concepts in thermoelectric materials. Angew. Chem. Int. Ed. 2009, 48, 8616–8939. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Yip, H.-L.; Jen, A.K.-Y. Rational design of advanced thermoelectric materials. Adv. Energy Mater. 2013, 3, 549–565. [Google Scholar] [CrossRef]
- Su, X.L.; Wei, P.; Li, H.; Liu, W.; Yan, Y.G.; Li, P.; Su, C.Q.; Xie, C.J.; Zhao, W.Y.; Zhai, P.C.; et al. Multi-scale microstructural thermoelectric materials: Transport behavior, non-equilibrium preparation, and applications. Adv. Mater. 2017, 29, 1602013. [Google Scholar] [CrossRef] [PubMed]
- Zhao, W.Y.; Liu, Z.Y.; Wei, P.; Zhang, Q.J.; Zhu, W.T.; Tang, X.F.; Yang, J.H.; Liu, Y.; Shi, J.; Chao, Y.M.; et al. Magnetoelectric interaction and transport behaviours in magnetic nanocomposite thermoelectric materials. Nature Nanotechnol. 2017, 12, 55–60. [Google Scholar] [CrossRef] [PubMed]
- Zhao, W.Y.; Liu, Z.Y.; Sun, Z.G.; Zhang, Q.J.; Wei, P.; Mu, X.; Zhou, H.Y.; Li, C.C.; Ma, S.F.; He, D.Q.; et al. Superparamagnetic enhancement of thermoelectric performance. Nature 2017, 549, 247–251. [Google Scholar] [CrossRef] [PubMed]
- Fu, L.; Kane, C.L.; Mele, E.J. Topological insulators in three dimensions. Phys. Rev. Lett. 2007, 98, 106803. [Google Scholar] [CrossRef] [PubMed]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological Insulators. Rev. Mod. Phys. 2010, 82, 3045–3067. [Google Scholar] [CrossRef]
- Kong, D.; Cui, Y. Opportunities in chemistry and materials science for topological insulators and their nanostructures. Nature Chem. 2011, 3, 845–849. [Google Scholar] [CrossRef] [PubMed]
- Müchler, L.; Casper, F.; Yan, B.; Chadov, S.; Felser, C. Topological insulators and thermoelectric materials. Phys. Status Solidi RRL 2013, 7, 91–100. [Google Scholar] [CrossRef]
- Ghaemi, P.; Mong, R.S.K.; Moore, J.E. In-plane transport and enhanced thermoelectric performance in thin films of the topological insulators Bi2Te3 and Bi2Se3. Phys. Rev. Lett. 2010, 105, 166603. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.; Gan, Z.; Zhang, S.-C. Enhanced thermoelectric performance and anomalous Seebeck effects in topological insulators. Phys. Rev. Lett. 2014, 112, 226801. [Google Scholar] [CrossRef] [PubMed]
- Rasche, B.; Isaeva, A.; Ruck, M.; Borisenko, S.; Zabolotnyy, V.; Büchner, B.; Koepernik, K.; Ortix, C.; Richter, M.; van den Brink, J. Stacked topological insulator built from bismuth-based graphene sheet analogues. Nature Mater. 2013, 12, 422–425. [Google Scholar] [CrossRef] [PubMed]
- Dresselhaus, M.S.; Chen, G.; Tang, M.Y.; Yang, R.; Lee, H.; Wang, D.; Ren, Z.; Fleurial, J.-P.; Gogna, P. New directions for low-dimensional thermoelectric materials. Adv. Mater. 2007, 19, 1043–1053. [Google Scholar] [CrossRef]
- Venkatasubramanian, R.; Siivola, E.; Colpitts, T.; O’Quinn, B. Thin-film thermoelectric devices with high room-temperature figures of merit. Nature 2001, 413, 597–602. [Google Scholar] [CrossRef] [PubMed]
- Wei, P.; Yang, J.; Guo, L.; Wang, S.Y.; Wu, L.H.; Xu, X.F.; Zhao, W.Y.; Zhang, Q.J.; Zhang, W.Q.; Dresselhaus, M.S.; et al. Minimum thermal conductivity in weak topological insulators with bismuth-based stack structure. Adv. Funct. Mater. 2016, 26, 5360–5367. [Google Scholar] [CrossRef]
- Savilov, S.V.; Khrustalev, V.N.; Kuznetsov, A.N.; Popovkin, B.A.; Antipin, M.Y. New subvalent bismuth telluroiodides incorporating Bi2, layers: The crystal and electronic structure of Bi2TeI. Russ. Chem. Bull. 2005, 54, 87–92. [Google Scholar] [CrossRef]
- Tang, P.; Yan, B.; Cao, W.; Wu, S.; Felser, C.; Duan, W. Weak topological insulators induced by the inter-layer coupling: A first-principles study of stacked Bi2TeI. Phys. Rev. B 2014, 89, 041409(R). [Google Scholar] [CrossRef]
- Rusinov, I.P.; Menshchikova, T.V.; Isaeva, A.; Eremeev, S.V.; Koroteev, Y.M.; Vergniory, M.G.; Echenique, P.M.; Chulkov, E.V. Mirror-symmetry protected non-TRIM surface state in the weak topological insulator Bi2TeI. Sci. Rep. 2016, 6, 20734. [Google Scholar] [CrossRef] [PubMed]
- Moroz, M.V.; Prokhorenko, M.V. Phase equilibria and the thermodynamic properties of saturated solid solutions of BiTeI, Bi2TeI, and Bi4TeI1.25, compounds of the AgI-Bi-Bi2Te3-BiTeI system. Russ. J. Phys. Chem. A 2016, 90, 1301–1305. [Google Scholar] [CrossRef]
- Ryu, G.; Son, K.; Schütz, G. Growth and characterization of large weak topological insulator Bi2TeI single crystal by Bismuth self-flux method. J. Crystal Growth 2016, 440, 26–30. [Google Scholar] [CrossRef]
- Zeugner, A.; Kaiser, M.; Schmidt, P.; Menshchikova, T.V.; Rusinov, I.P.; Markelov, A.V.; van den Broek, W.; Chulkov, E.V.; Doert, T.; Ruck, M.; et al. Modular design with 2D topological-insulator building blocks: Optimized synthesis and crystal growth, crystal and electronic structures of BixTeI (x = 2, 3). Chem. Mater. 2017, 29, 1321–1337. [Google Scholar] [CrossRef]
- Tomokiyo, A.; Okada, T.; Kawano, S. Phase diagram of system (Bi2Te3)-(BiI3) and crystal structure of BiTeI. Jpn. J. Appl. Phys. 1977, 16, 291–298. [Google Scholar] [CrossRef]
- Aliyev, Z.S.; Babanly, M.B. Solid-state equilibria and thermodynamic properties of compounds in the Bi-Te-I system. Inorg. Mater. 2008, 44, 1076–1080. [Google Scholar] [CrossRef]
- Babanly, M.B.; Tedenac, J.; Aliyev, Z.S.; Balitsky, D.V. Phase equilibriums and thermodynamic properties of the system Bi-Te-I. J. Alloys Compd. 2009, 481, 349–353. [Google Scholar] [CrossRef]
- Wu, L.; Yang, J.; Chi, M.; Wang, S.; Wei, P.; Zhang, W.; Chen, L.; Yang, J. Enhanced thermoelectric performance in Cu-intercalated BiTeI by compensation weakening induced mobility improvement. Sci. Rep. 2015, 5, 14319. [Google Scholar] [CrossRef] [PubMed]
- Ishizaka, K.; Bahramy, M.S.; Murakawa, H.; Sakano, M.; Shimojima, T.; Sonobe, T.; Koizumi, K.; Shin, S.; Miyahara, H.; Kimura, A.; et al. Giant Rashba-type spin splitting in bulk BiTeI. Nature Mater. 2011, 10, 521–526. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.S.; Zhang, Q.Y.; Lan, Y.C.; Chen, S.; Yan, X.; Zhang, Q.; Wang, H.; Wang, D.Z.; Chen, G.; Ren, Z.F. Thermoelectric property studies on Cu-doped n-type CuxBi2Te2.7Se0.3 nanocomposites. Adv. Energy Mater. 2011, 1, 577–587. [Google Scholar] [CrossRef]
- Wang, S.Y.; Li, H.; Lu, R.M.; Zheng, G.; Tang, X.F. Metal nanoparticle decorated n-type Bi2Te3-based materials with enhanced thermoelectric performances. Nanotechnology 2013, 24, 285702. [Google Scholar] [CrossRef] [PubMed]
- Cui, J.L.; Mao, L.D.; Yang, W.; Xu, X.B.; Chen, D.Y.; Xiu, W.J. Thermoelectric properties of Cu-doped n-type (Bi2Te3)0.9–(Bi2−xCuxSe3)0.1(x = 0–0.2) alloys. J. Solid State Chem. 2007, 180, 3583–3587. [Google Scholar] [CrossRef]

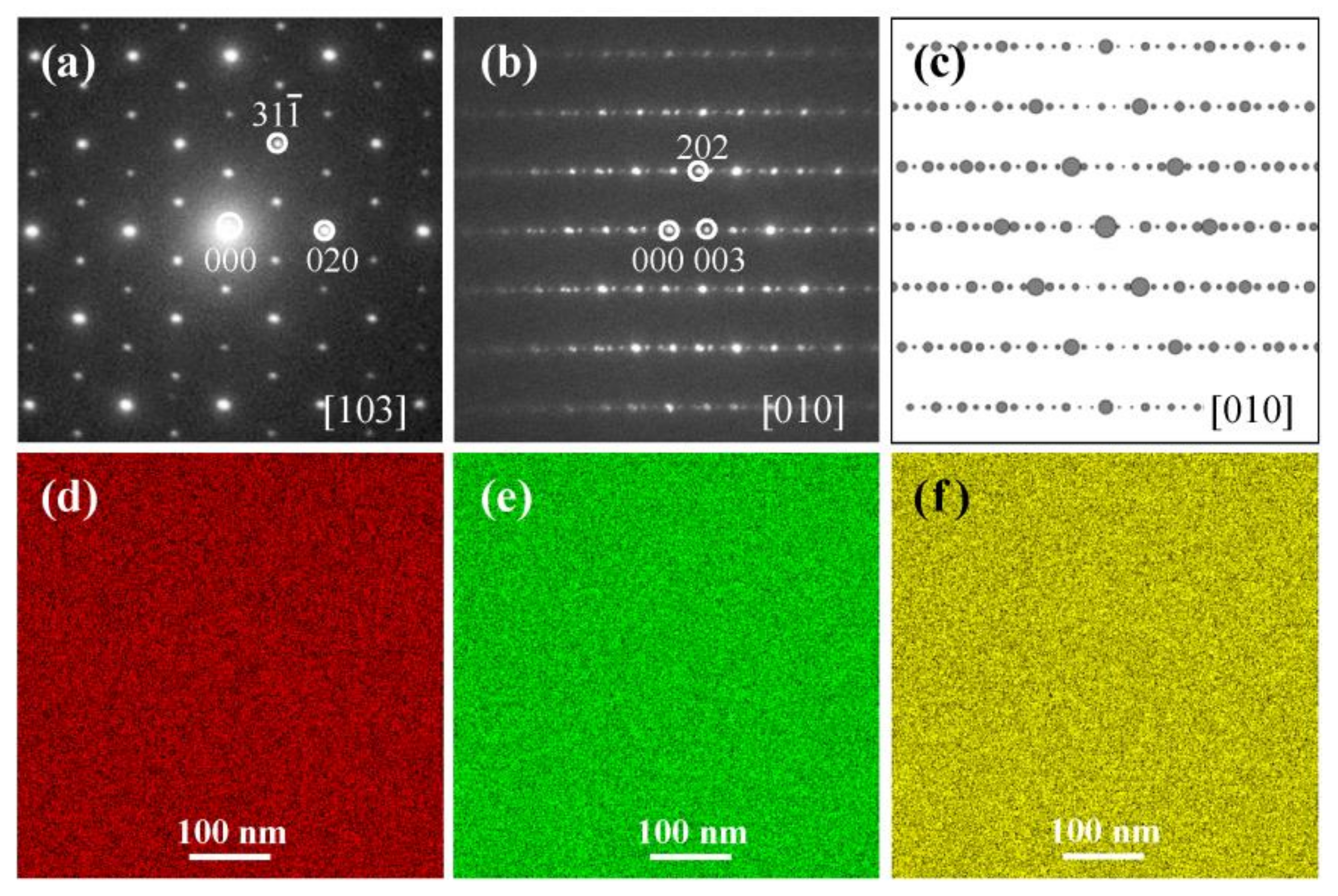
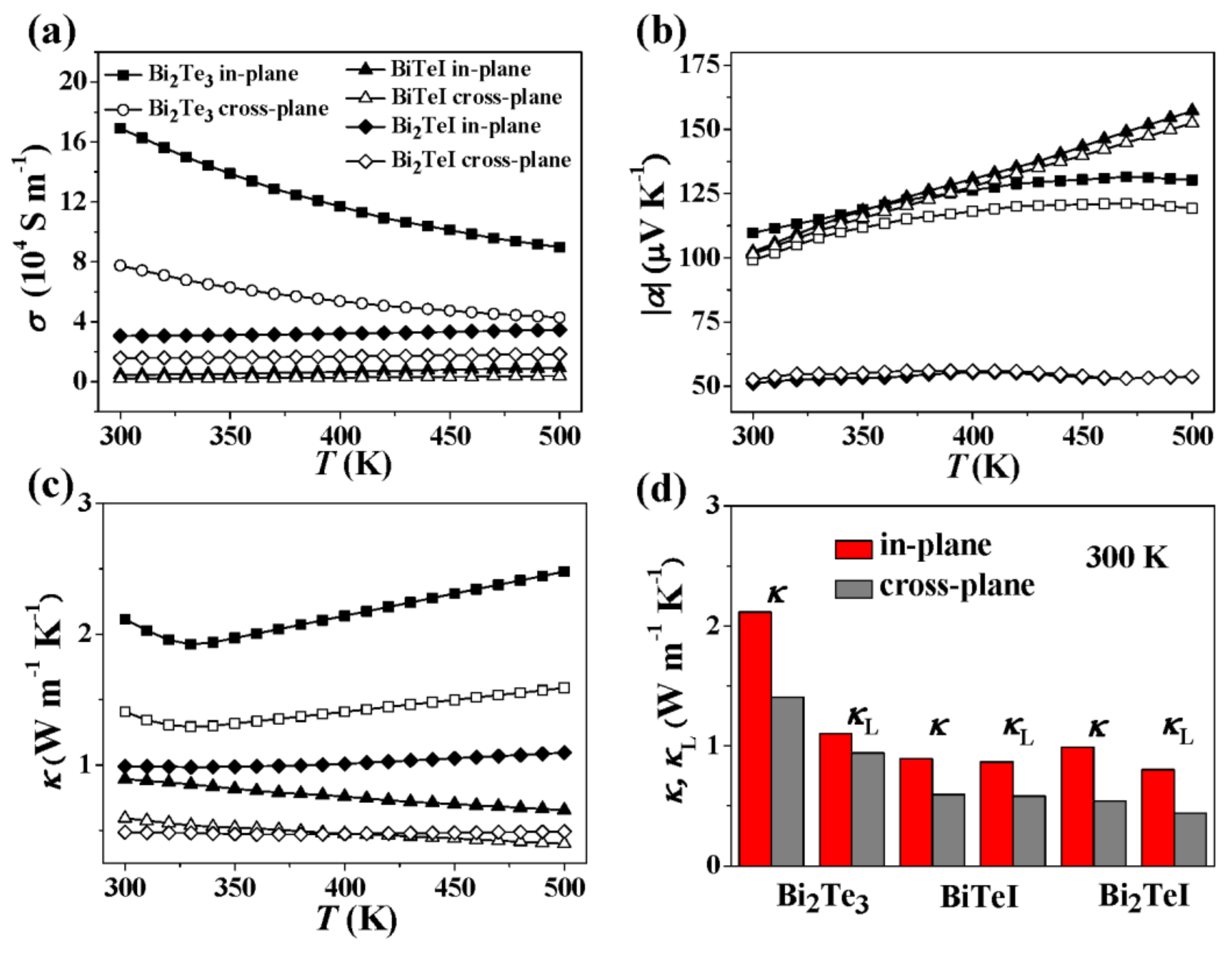
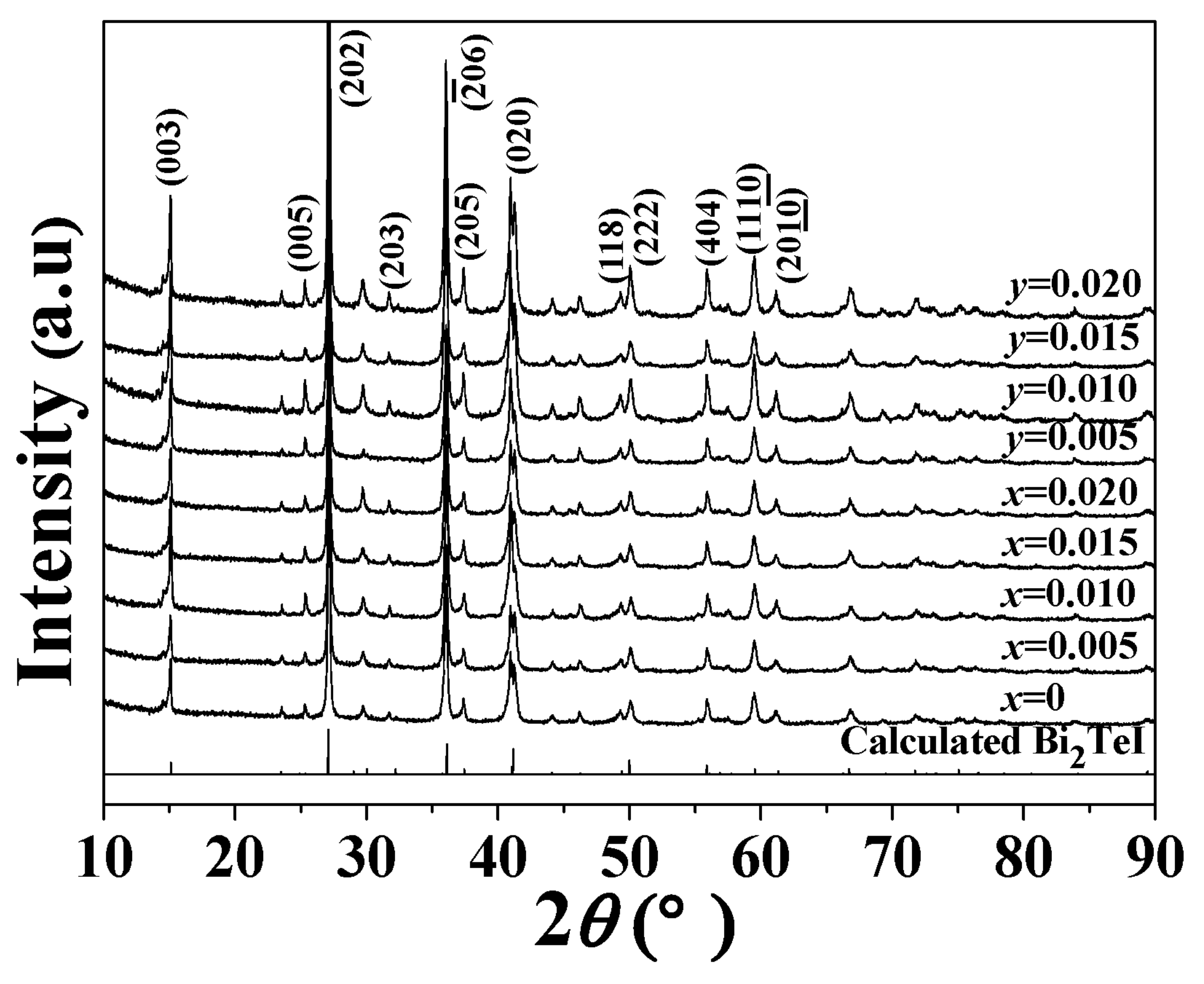
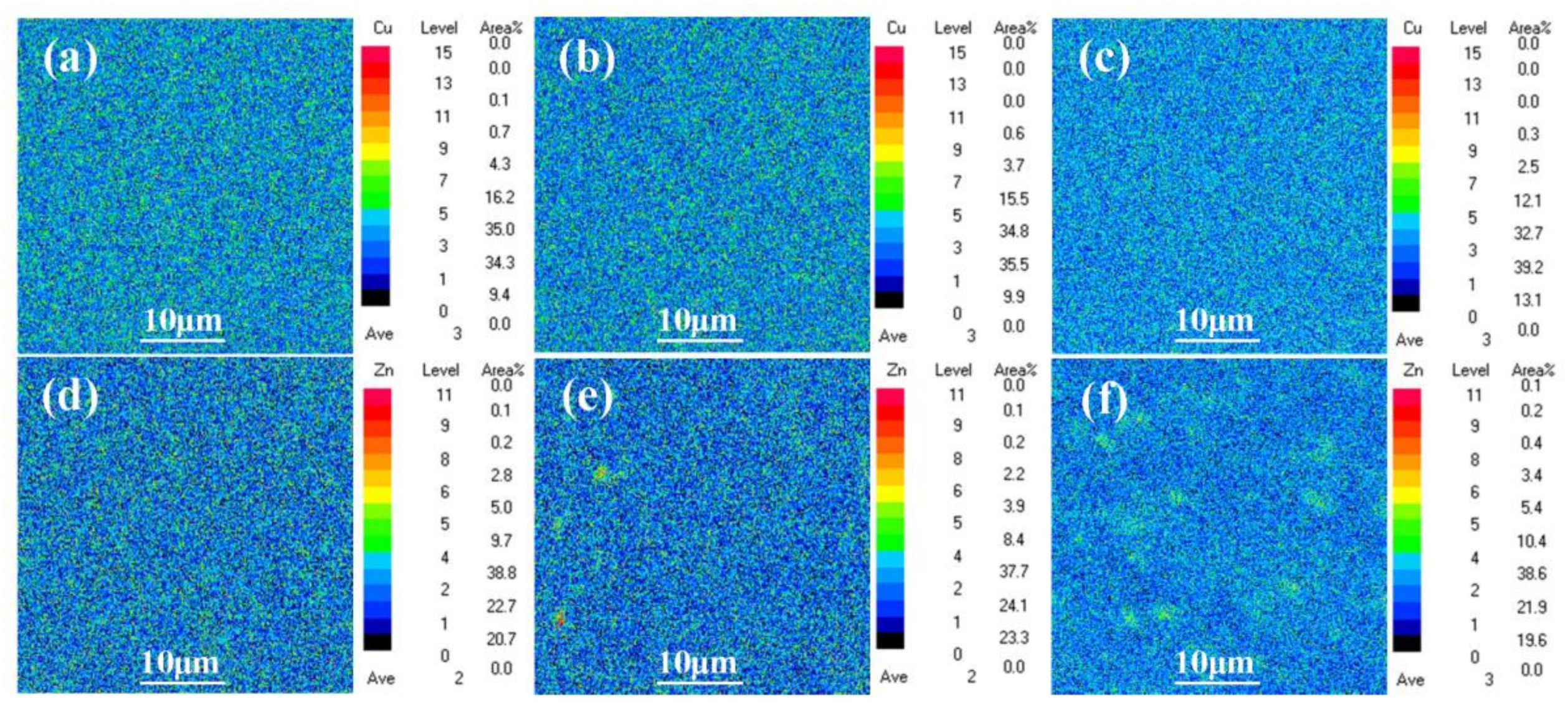


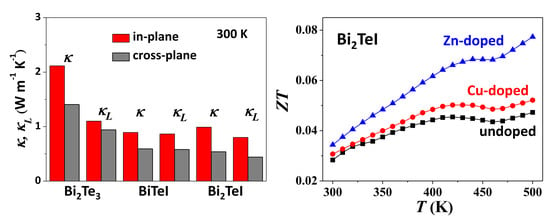
| Samples | N (1020 cm−3) | µH (cm2 V−1 s−1) | σ (104 S m−1) | α (µV K−1) | κL (W m−1 K−1) |
|---|---|---|---|---|---|
| Bi2Te3 (in-plane) | 0.72 | 150 | 16.9 | −111 | 1.10 |
| Bi2Te3 (cross-plane) | 0.53 | 92 | 7.75 | −102 | 0.94 |
| BiTeI (in-plane) | 0.19 | 15 | 0.45 | −106 | 0.86 |
| BiTeI (cross-plane) | 0.14 | 8.6 | 0.20 | −102 | 0.58 |
| Bi2TeI (in-plane) | 8.1 | 2.3 | 3.07 | 53.6 | 0.80 |
| Bi2TeI (cross-plane) | 7.5 | 1.3 | 1.59 | 53.8 | 0.44 |
| Samples | n (1020 cm−3) | µH (cm2 V−1 s−1) | σ (104 S m−1) | α (µV K−1) | α2σ (mW m−1 K−2) | κ (W m−1 K−1) | κL (W m−1 K−1) |
|---|---|---|---|---|---|---|---|
| Bi2TeI | 8.1 | 2.3 | 3.07 | 55.3 | 0.093 | 0.99 | 0.80 |
| Cu0.005Bi2TeI | 7.9 | 2.4 | 3.11 | 53.7 | 0.090 | 0.91 | 0.72 |
| Cu0.01Bi2TeI | 7.1 | 2.7 | 3.08 | 54.1 | 0.090 | 0.88 | 0.70 |
| Cu0.015Bi2TeI | 5.2 | 3.2 | 2.70 | 49.9 | 0.068 | 0.91 | 0.75 |
| Cu0.02Bi2TeI | 4.2 | 4.0 | 2.73 | 46.2 | 0.058 | 0.92 | 0.75 |
| Zn0.005Bi2TeI | 11.2 | 1.9 | 3.36 | 51.3 | 0.088 | 0.90 | 0.70 |
| Zn0.01Bi2TeI | 14.8 | 1.4 | 3.26 | 55.7 | 0.101 | 0.88 | 0.69 |
| Zn0.015Bi2TeI | 15.8 | 1.0 | 2.53 | 56.4 | 0.080 | 0.94 | 0.78 |
| Zn0.02Bi2TeI | 14.9 | 1.1 | 2.54 | 56.5 | 0.081 | 0.97 | 0.82 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tu, K.; Wei, P.; Zhou, H.; Mu, X.; Zhu, W.; Nie, X.; Zhao, W. A Thermoelectric Performance Study of Layered Bi2TeI Weak Topological Insulator Materials. Energies 2018, 11, 891. https://doi.org/10.3390/en11040891
Tu K, Wei P, Zhou H, Mu X, Zhu W, Nie X, Zhao W. A Thermoelectric Performance Study of Layered Bi2TeI Weak Topological Insulator Materials. Energies. 2018; 11(4):891. https://doi.org/10.3390/en11040891
Chicago/Turabian StyleTu, Kaihua, Ping Wei, Hongyu Zhou, Xin Mu, Wanting Zhu, Xiaolei Nie, and Wenyu Zhao. 2018. "A Thermoelectric Performance Study of Layered Bi2TeI Weak Topological Insulator Materials" Energies 11, no. 4: 891. https://doi.org/10.3390/en11040891
APA StyleTu, K., Wei, P., Zhou, H., Mu, X., Zhu, W., Nie, X., & Zhao, W. (2018). A Thermoelectric Performance Study of Layered Bi2TeI Weak Topological Insulator Materials. Energies, 11(4), 891. https://doi.org/10.3390/en11040891