Analyzing the Interaction of Vortex and Gas–Liquid Interface Dynamics in Fuel Spray Nozzles by Means of Lagrangian-Coherent Structures (2D)
Abstract
1. Introduction
2. Fundamentals
2.1. SPH—Schemes
2.2. LCS and FTLE
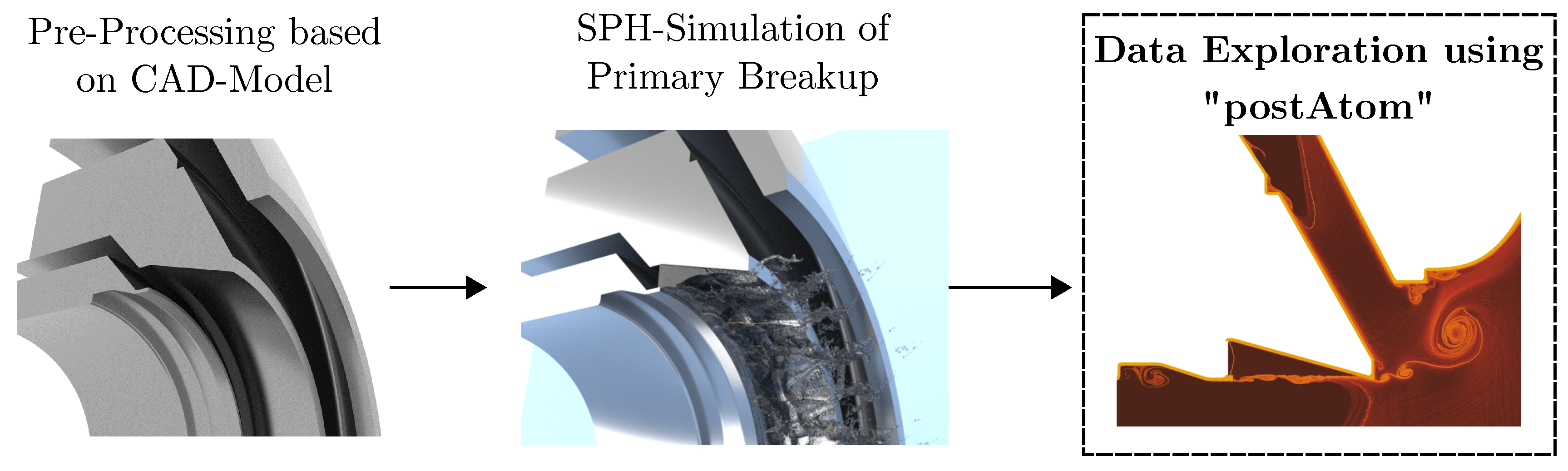
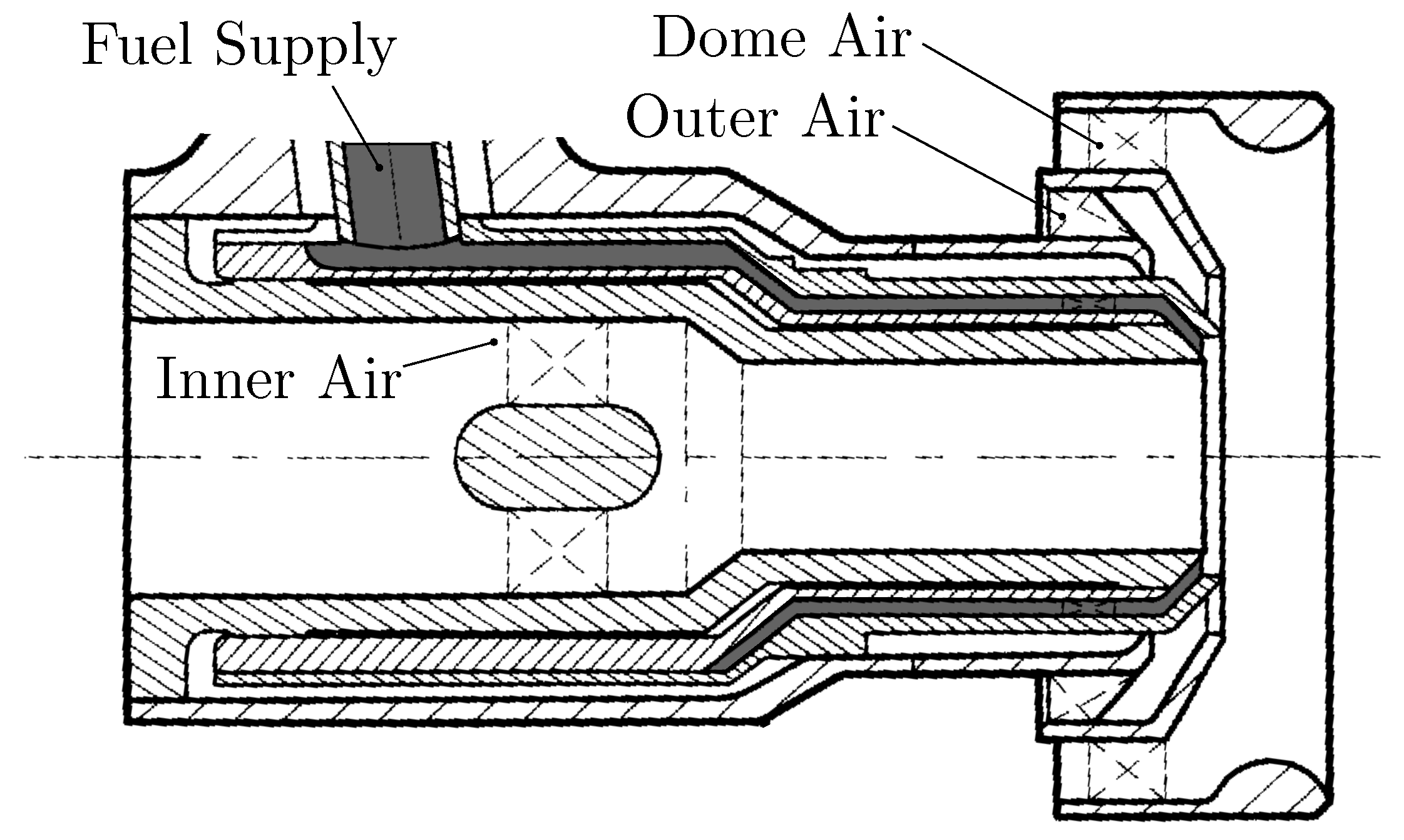
3. Computational Setup (2D)
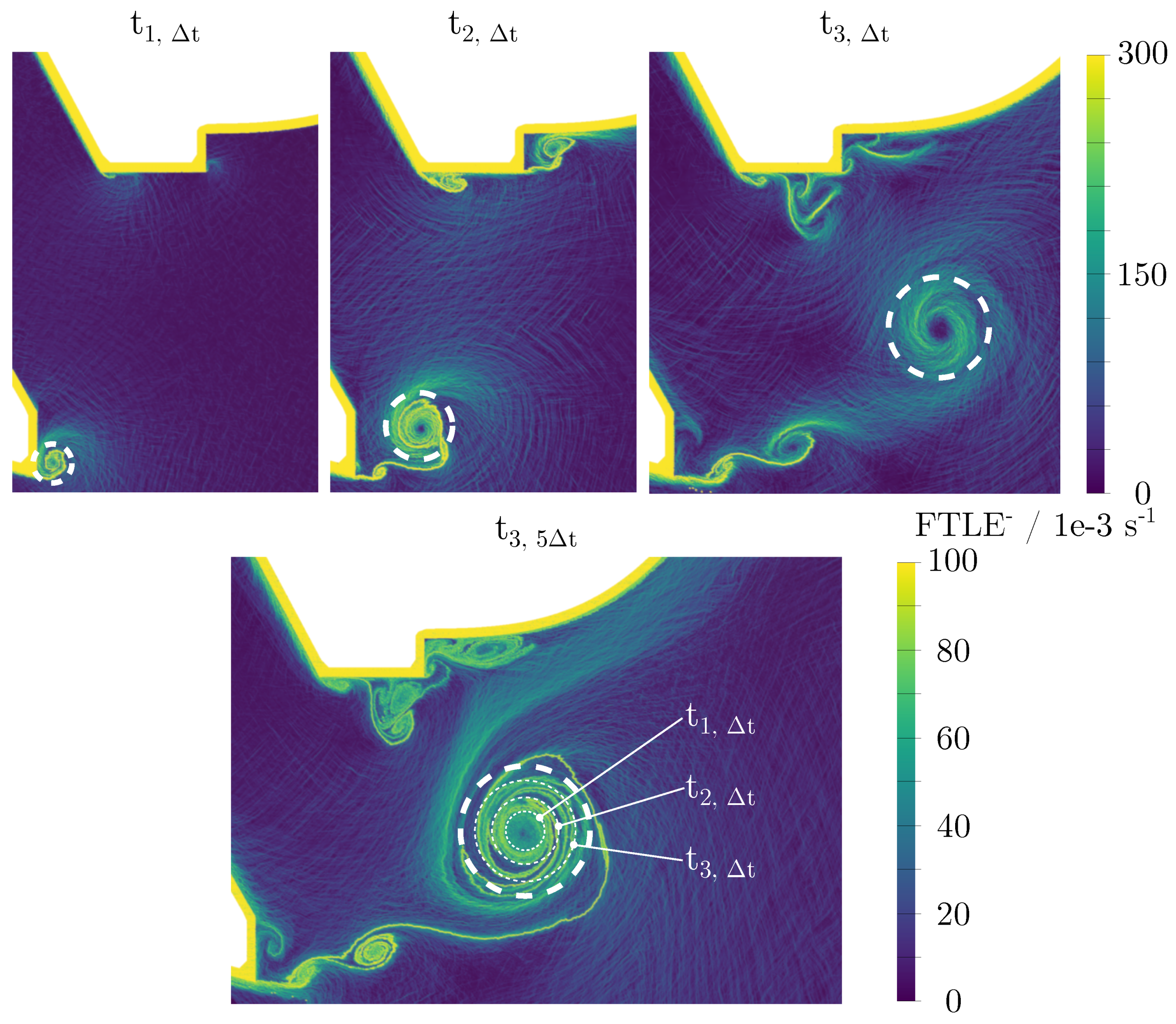
4. Results and Discussion
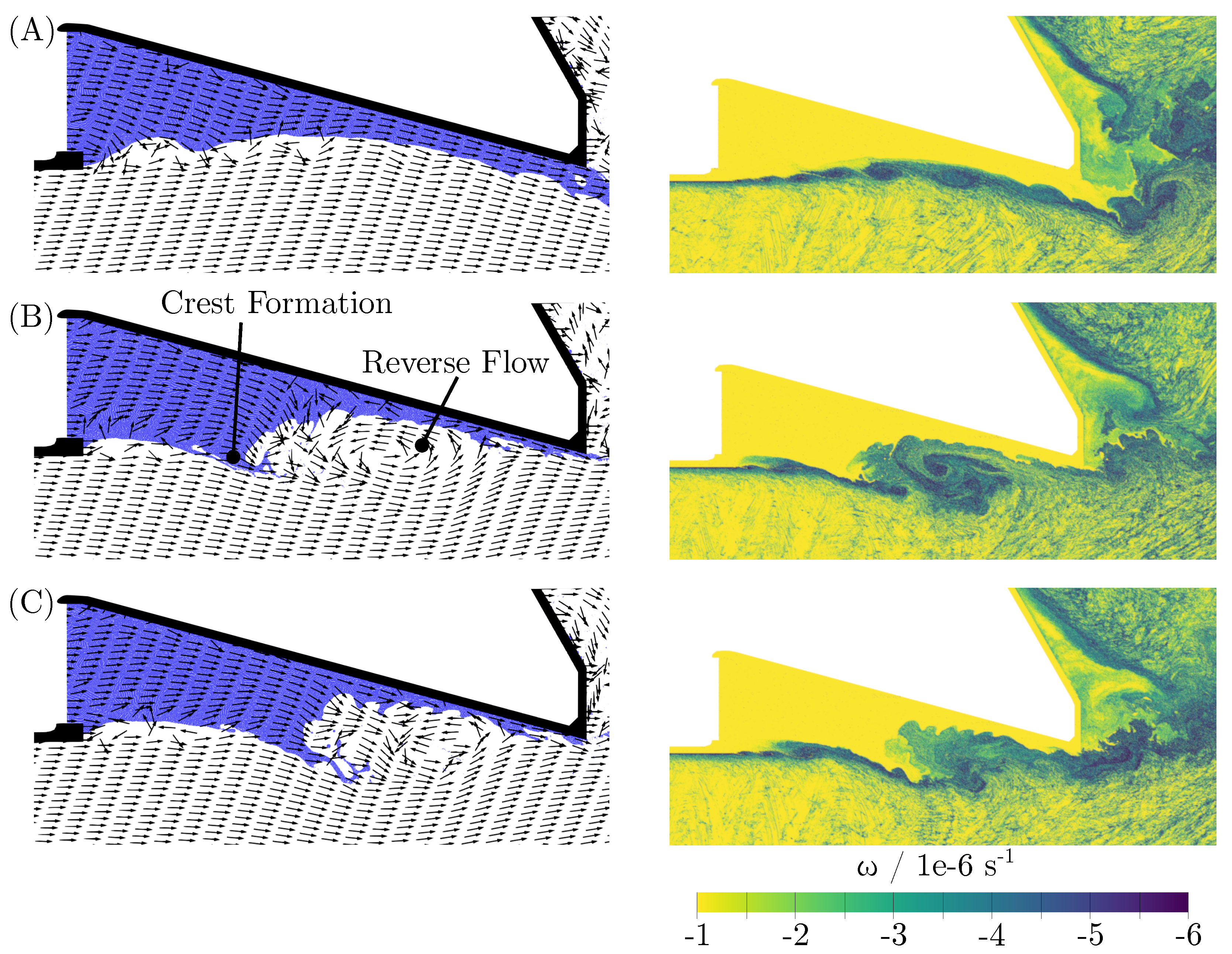
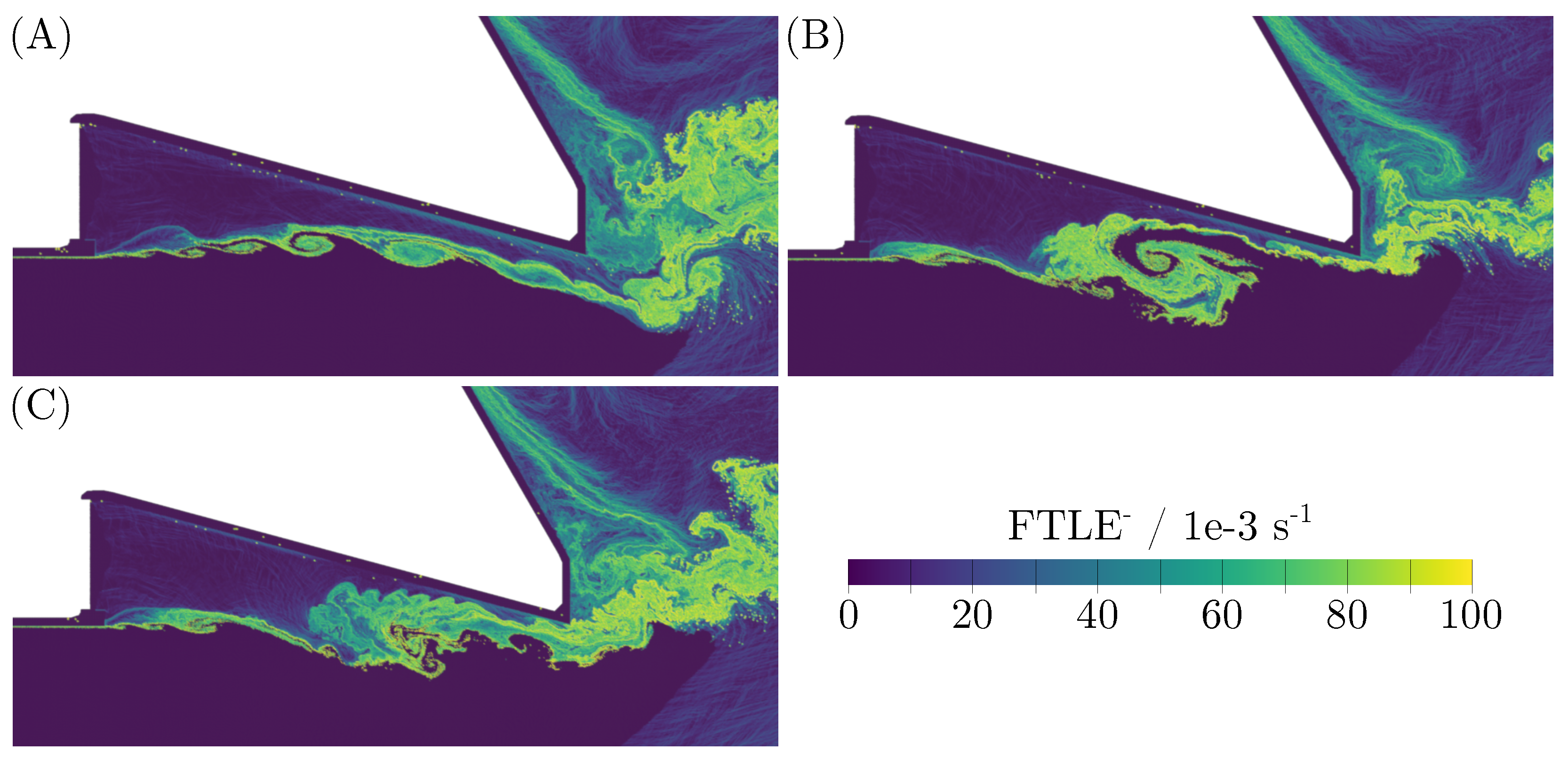
4.1. Interaction of Vortices and the Phase Interface
4.2. Sensitivity of -Variations of
4.3. Influence of Nozzle Geometry on Primary Breakup
5. Conclusions
- The transport of LCSs can effectively be captured by SPH and identified by means of fields at a fixed integration time .
- Investigating the dynamics of LCSs in the gaseous phase helps to understand the momentum transfer from the gas to the liquid phase, leading to disintegration.
- Suppressing LCSs by a modification of the fuel spray nozzle geometry leads to a suppression of time fluctuations in the fuel flow emerging from the nozzle.
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| CFD | Computational Fluid Dynamics |
| FTLE | Finite-Time Lyapunov Exponent |
| ITS | Institut für Thermische Strömungsmaschinen |
| IVD | Institut für Visualisierung und Datenanalyse |
| LCS | Lagrangian-Coherent Structure |
| SPH | Smoothed Particle Hydrodynamics |
| Nomenclature | |
| Regularizing parameter | |
| Isentropic coefficient | |
| Eigenvalues | |
| Kinematic viscosity | |
| Density | |
| Shear stress | |
| Flow map | |
| Right Cauchy–Green tensor | |
| Gradient of flowmap | |
| Tensor for Kernel correction | |
| b | Arbitrary vector |
| c | Speed of sound |
| Volume forces | |
| h | Smoothing length |
| m | Mass |
| n | Dimension |
| p | Pressure |
| Q | Arbitrary matrix |
| Distance vector | |
| t | Time |
| V | Volume |
| Velocity | |
| W | Kernel |
| Position | |
| Operators | |
| ∇ | Gradient |
| Difference | |
| ⊗ | Dyadic product |
| SPH discretization | |
| Subscripts | |
| a | Particle a |
| b | Particle b |
| Background | |
| Difference between particles a and b | |
| Property of gas | |
| Property of liquid | |
| Reference for EOS | |
| Optimal value for integration time | |
| Reference value for integration time | |
| 0 | Reference point in time |
| Indices of certain times | |
References
- Lefebvre, A.; Ballal, D.R. Gas Turbine Combustion, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Jones, W.P.; Lyra, S.; Navarro-Martinez, S. Numerical investigation of swirling kerosene spray flames using Large Eddy Simulation. Combust. Flame 2012, 159, 1539–1561. [Google Scholar] [CrossRef]
- Knudsen, E.; Shashank; Pitsch, H. Modeling partially premixed combustion behavior in multiphase LES. Combust. Flame 2015, 162, 159–180. [Google Scholar] [CrossRef]
- Braun, S.; Wieth, L.; Dauch, T.; Keller, M.; Chaussonnet, G.; Höfler, C.; Koch, R.; Bauer, H.J. HPC Predictions of Primary Atomization with SPH: Challenges and Lessons Learned. In Proceedings of the 11th International SPHERIC Workshop, Munich, Germany, 13–16 June 2016. [Google Scholar]
- Koch, R.; Braun, S.; Wieth, L.; Chaussonnet, G.; Dauch, T.F.; Bauer, H.J. Prediction of primary atomization using Smoothed Particle Hydrodynamics. Eur. J. Mech. B/Fluids 2017, 61 Pt 2, 271–278. [Google Scholar] [CrossRef]
- Nagel, W.E.; Kröner, D.H.; Resch, M.M. (Eds.) High Performance Computing in Science and Engineering ’16; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Dauch, T.F.; Braun, S.; Wieth, L.; Chaussonnet, G.; Keller, M.C.; Koch, R.; Bauer, H.J. Computation of Liquid Fuel Atomization and Mixing by Means of the SPH Method: Application to a Jet Engine Fuel Nozzle. In Proceedings of the ASME Turbo Expo 2016: Turbine Technical Conference and Exposition (GT2016-56023), Seoul, Korea, 13–17 June 2016. [Google Scholar] [CrossRef]
- Dauch, T.F.; Braun, S.; Wieth, L.; Chaussonnet, G.; Keller, M.C.; Koch, R.; Bauer, H.J. Computational Prediction of Primary Breakup in Fuel Spray Nozzles for Aero-Engine Combustors. In Proceedings of the ILASS Europe, 29th Annual Conference on Liquid Atomization and Spray Systems, Valencia, Spain, 6–8 September 2017. [Google Scholar] [CrossRef]
- Chaussonnet, G.; Braun, S.; Wieth, L.; Koch, R.; Bauer, H.J.; Sänger, A.; Jakobs, T.; Djordjevic, N.; Kolb, T. SPH Simulation of a Twin-Fluid Atomizer Operating with a High Viscosity Liquid. In Proceedings of the ICLASS 2015, 13th Triennial International Conference on Liquid Atomization and Spray Systems, Tainan, Taiwan, 23–27 August 2015. [Google Scholar]
- Zandian, A.; Sirignano, W.A.; Hussain, F. Understanding liquid-jet atomization cascades via vortex dynamics. J. Fluid Mech. 2018, 843, 293–354. [Google Scholar] [CrossRef]
- Dauch, T.; Rapp, T.; Chaussonnet, G.; Braun, S.; Keller, M.; Kaden, J.; Koch, R.; Dachsbacher, C.; Bauer, H.J. Highly efficient computation of Finite-Time Lyapunov Exponents (FTLE) on GPUs based on three-dimensional SPH datasets. Comput. Fluids 2018, 175, 129–141. [Google Scholar] [CrossRef]
- Dauch, T.F.; Okraschevski, M.; Keller, M.C.; Braun, S.; Wieth, L.; Chaussonnet, G.; Koch, R.; Bauer, H.J. Preprocessing Workflow for the Initialization of SPH Predictions based on Arbitrary CAD Models. In Proceedings of the 12th International SPHERIC Workshop, Ourense, Spain, 12–15 June 2017. [Google Scholar]
- Braun, S.; Wieth, L.; Holz, S.; Dauch, T.F.; Keller, M.C.; Chaussonnet, G.; Gepperth, S.; Koch, R.; Bauer, H.J. Numerical prediction of air-assisted primary atomization using Smoothed Particle Hydrodynamics. Int. J. Multiph. Flow 2019, 114, 303–315. [Google Scholar] [CrossRef]
- Chaussonnet, G.; Braun, S.; Dauch, T.; Keller, M.; Sänger, A.; Jakobs, T.; Koch, R.; Kolb, T.; Bauer, H.J. Toward the development of a virtual spray test-rig using the Smoothed Particle Hydrodynamics method. Comput. Fluids 2019, 180, 68–81. [Google Scholar] [CrossRef]
- Español, P.; Revenga, M. Smoothed dissipative particle dynamics. Phys. Rev. E 2003, 67, 026705. [Google Scholar] [CrossRef] [PubMed]
- Hu, X.Y.; Adams, N.A. A multi-phase SPH method for macroscopic and mesoscopic flows. J. Comput. Phys. 2006, 213, 844–861. [Google Scholar] [CrossRef]
- Monaghan, J. Smoothed Particle Hydrodynamics. Ann. Rev. Astron. Astrophys. 1992, 30, 543–574. [Google Scholar] [CrossRef]
- Szewc, K.; Pozorski, J.; Minier, J.P. Analysis of the incompressibility constraint in the smoothed particle hydrodynamics method. Int. J. Numer. Methods Eng. 2012, 92, 343–369. [Google Scholar] [CrossRef]
- Cleary, P.W. Modelling confined multi-material heat and mass flows using SPH. Appl. Math. Model. 1998, 22, 981–993. [Google Scholar] [CrossRef]
- Cole, R.H.; Weller, R. Underwater Explosions. Phys. Today 1948, 1, 35. [Google Scholar] [CrossRef]
- Liu, M.B.; Liu, G.R. Smoothed Particle Hydrodynamics (SPH): An Overview and Recent Developments. Arch. Comput. Methods Eng. 2010, 17, 25–76. [Google Scholar] [CrossRef]
- Gorokhovski, M.; Herrmann, M. Modeling Primary Atomization. Ann. Rev. Fluid Mech. 2008, 40, 343–366. [Google Scholar] [CrossRef]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Hunt, J.C.R. Vorticity and vortex dynamics in complex turbulent flows. In Canadian Society for Mechanical Engineering; NRC Research Press: Ottawa, ON, Canada, 1987; Volume 11, pp. 21–35. ISSN 0315-8977. [Google Scholar]
- Haller, G. An objective definition of a vortex. J. Fluid Mech. 2005, 525, 1–26. [Google Scholar] [CrossRef]
- Sun, P.N.; Colagrossi, A.; Marrone, S.; Zhang, A.M. Detection of Lagrangian Coherent Structures in the SPH framework. Comput. Methods Appl. Mech. Eng. 2016, 305, 849–868. [Google Scholar] [CrossRef]
- Bonet, J.; Lok, T.S.L. Variational and momentum preservation aspects of Smooth Particle Hydrodynamic formulations. Comput. Methods Appl. Mech. Eng. 1999, 180, 97–115. [Google Scholar] [CrossRef]
- Steinthorsson, E.; Benjamin, M.A.; Barnhart, D.R. Fuel Nozzle for Turbine Combustion Engines Having Aerodynamic Turning Vanes. U.S. Patent No. 6883332 B2, 26 April 2005. [Google Scholar]
- Mansour, A.; Benjamin, M. Pure Airblast Nozzle. U.S. Patent No. 6622488 B2, 23 September 2003. [Google Scholar]
- Zandian, A.; Sirignano, W.; Hussain, F. Mechanisms of Liquid Stream Breakup: Vorticity and Time and Length Scales. In Proceedings of the ILASS Europe, 29th Annual Conference on Liquid Atomization and Spray Systems, Valencia, Spain, 6–8 September 2017. Editorial Universitat Politècnica de València. [Google Scholar] [CrossRef]





© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dauch, T.F.; Ates, C.; Rapp, T.; Keller, M.C.; Chaussonnet, G.; Kaden, J.; Okraschevski, M.; Koch, R.; Dachsbacher, C.; Bauer, H.-J. Analyzing the Interaction of Vortex and Gas–Liquid Interface Dynamics in Fuel Spray Nozzles by Means of Lagrangian-Coherent Structures (2D). Energies 2019, 12, 2552. https://doi.org/10.3390/en12132552
Dauch TF, Ates C, Rapp T, Keller MC, Chaussonnet G, Kaden J, Okraschevski M, Koch R, Dachsbacher C, Bauer H-J. Analyzing the Interaction of Vortex and Gas–Liquid Interface Dynamics in Fuel Spray Nozzles by Means of Lagrangian-Coherent Structures (2D). Energies. 2019; 12(13):2552. https://doi.org/10.3390/en12132552
Chicago/Turabian StyleDauch, Thilo F., Cihan Ates, Tobias Rapp, Marc C. Keller, Geoffroy Chaussonnet, Johannes Kaden, Max Okraschevski, Rainer Koch, Carsten Dachsbacher, and Hans-Jörg Bauer. 2019. "Analyzing the Interaction of Vortex and Gas–Liquid Interface Dynamics in Fuel Spray Nozzles by Means of Lagrangian-Coherent Structures (2D)" Energies 12, no. 13: 2552. https://doi.org/10.3390/en12132552
APA StyleDauch, T. F., Ates, C., Rapp, T., Keller, M. C., Chaussonnet, G., Kaden, J., Okraschevski, M., Koch, R., Dachsbacher, C., & Bauer, H.-J. (2019). Analyzing the Interaction of Vortex and Gas–Liquid Interface Dynamics in Fuel Spray Nozzles by Means of Lagrangian-Coherent Structures (2D). Energies, 12(13), 2552. https://doi.org/10.3390/en12132552




