Heat Pipe Thermal Management Based on High-Rate Discharge and Pulse Cycle Tests for Lithium-Ion Batteries
Abstract
:1. Introduction
2. Introduction of the Experimental Methods
2.1. The A123-8Ah Battery
2.2. Cooling System
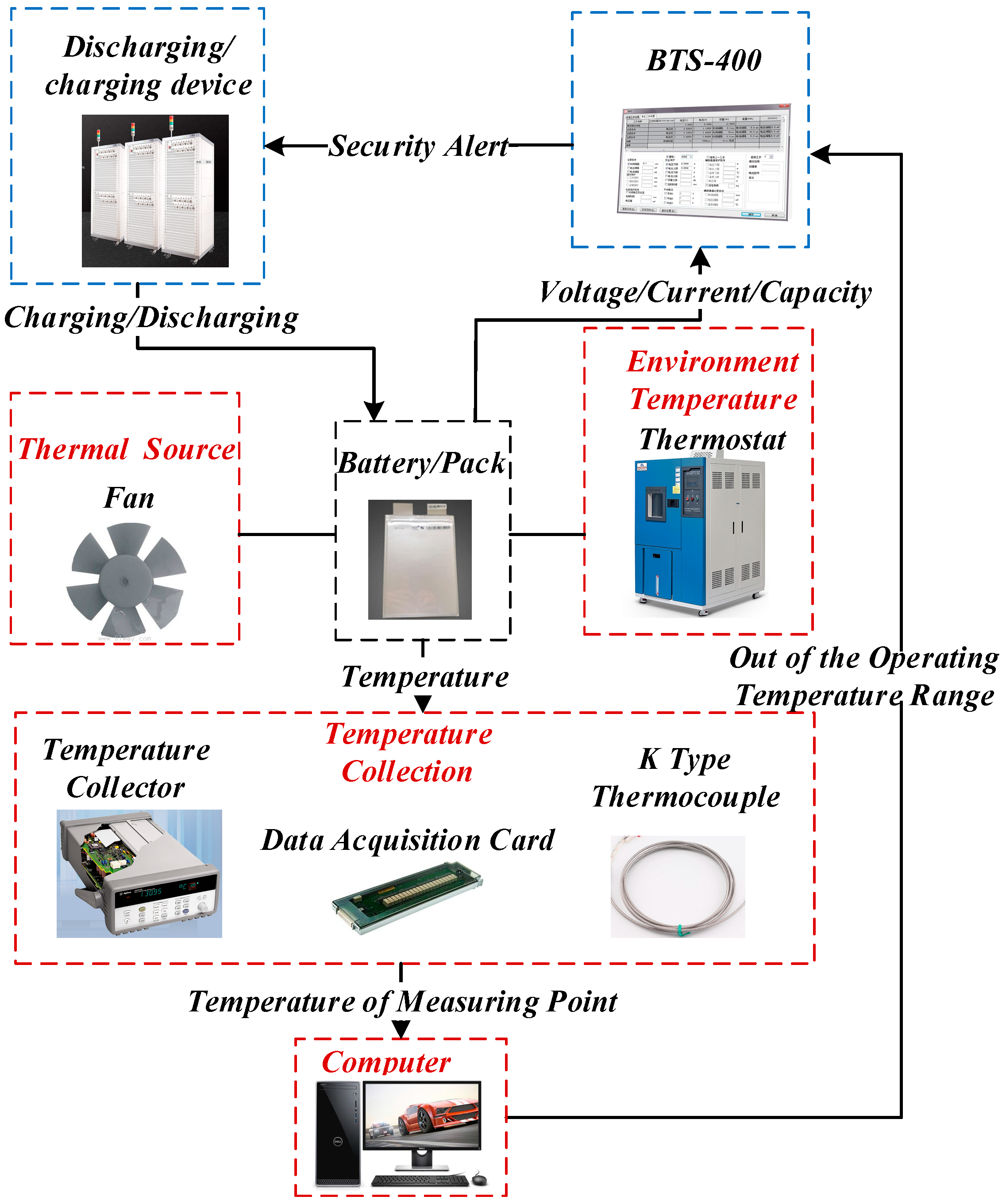
2.3. The Equipment of the Experiments
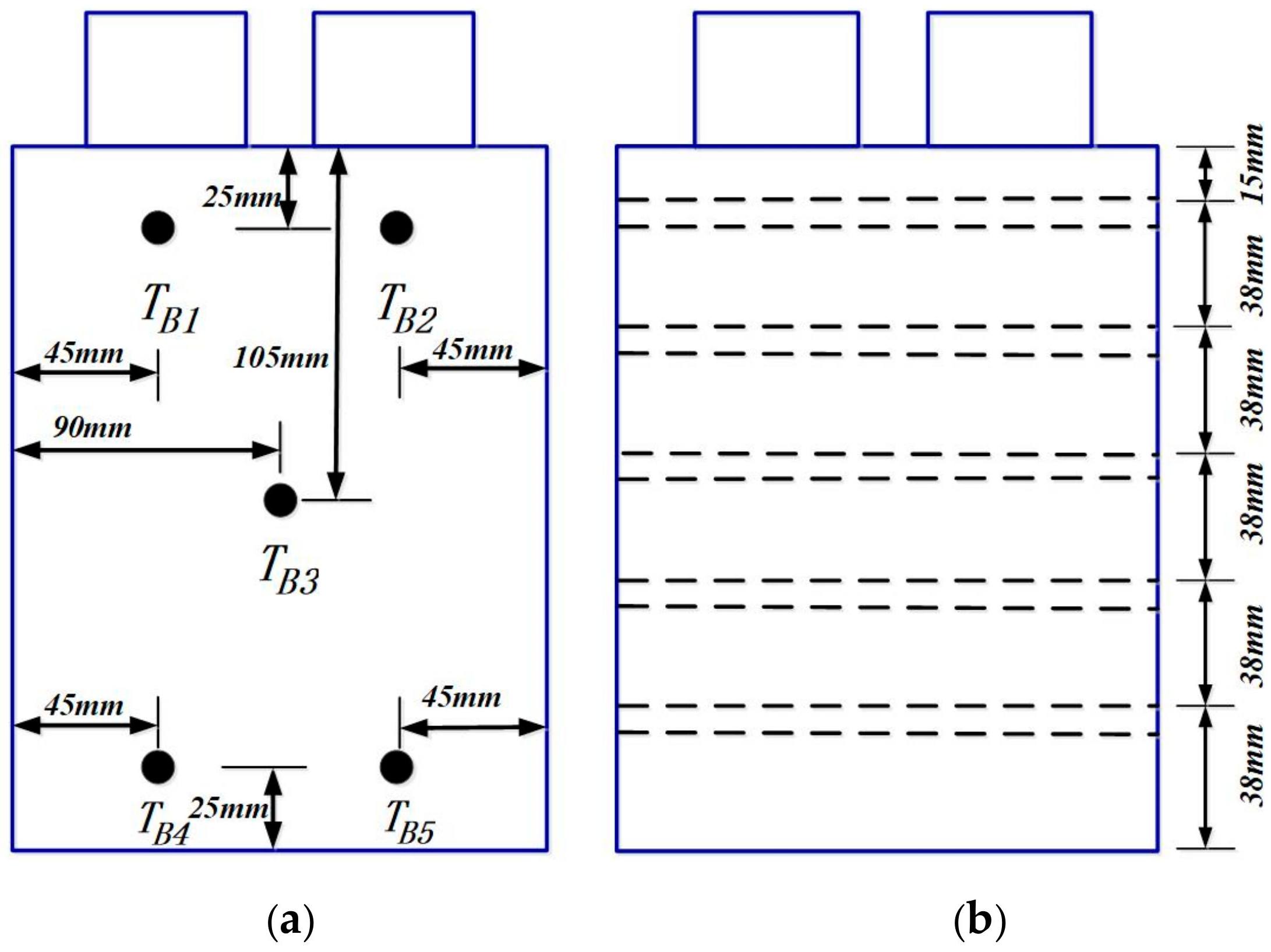
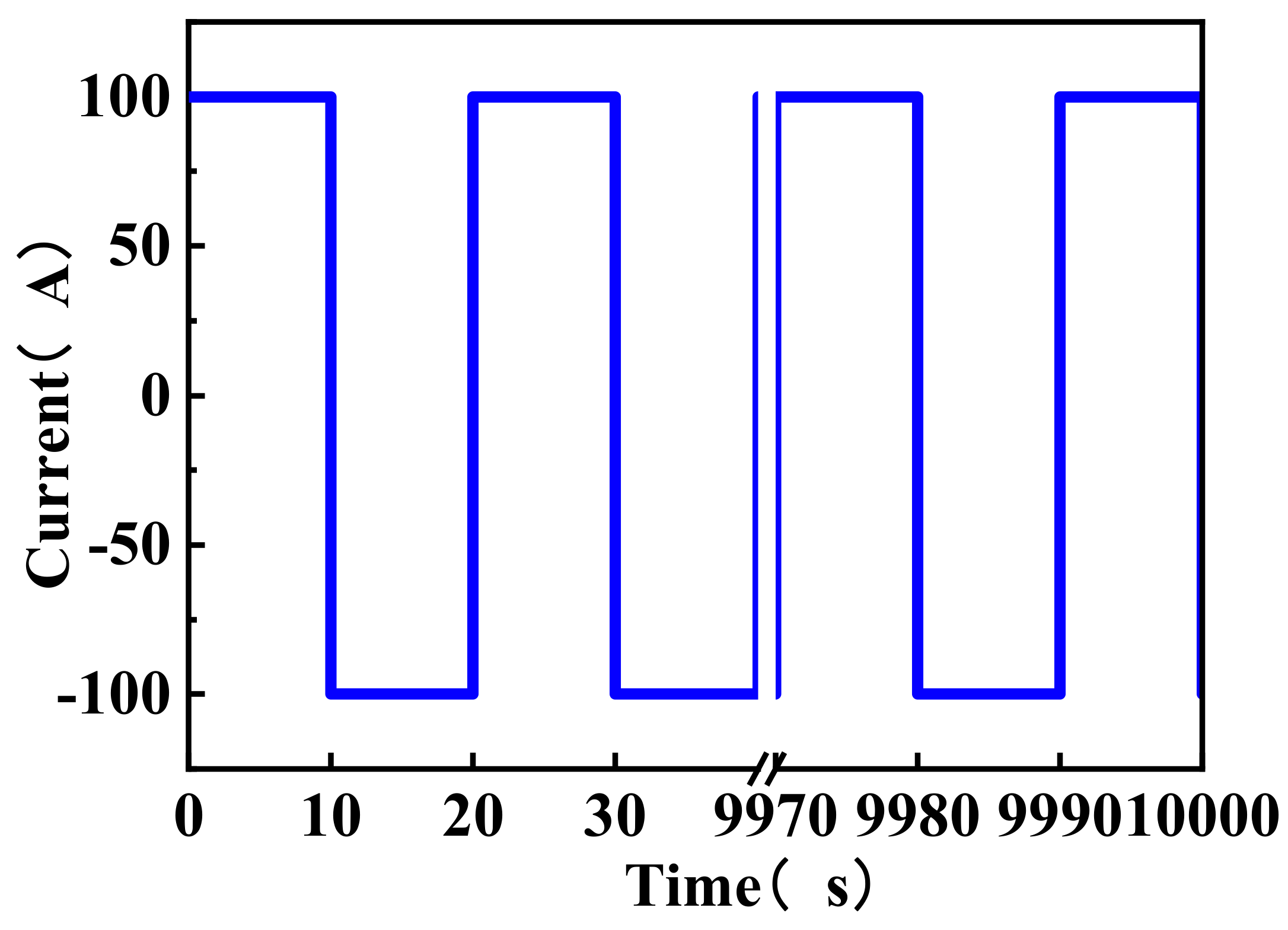
2.4. Content of the Experiment
3. Analysis of Results
3.1. Analysis of Discharge Experiment
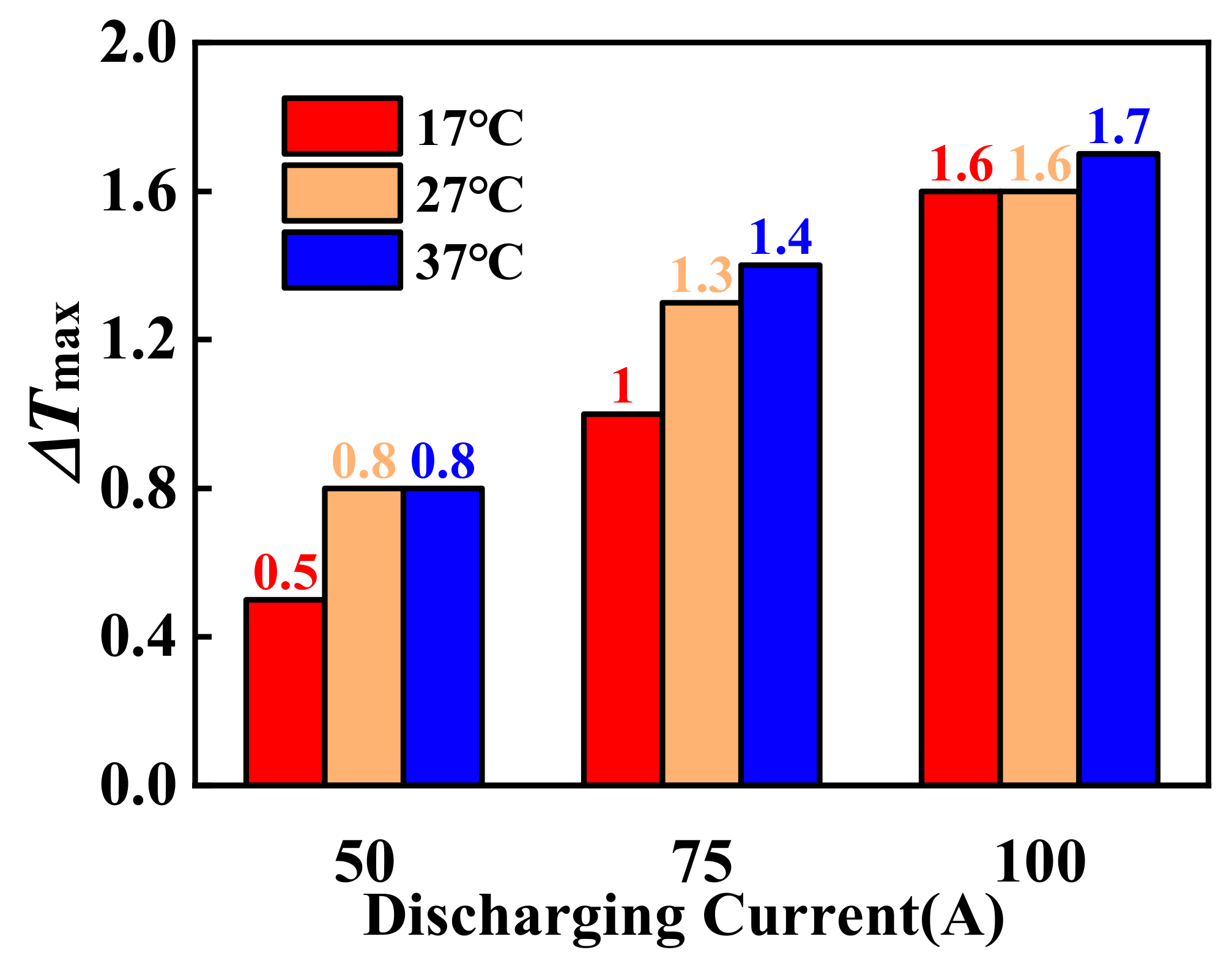
3.1.1. Effects of Discharge Rate on the Experiment
3.1.2. Effects of Heat Dissipation on the Experiment
3.2. Experimental Analysis of Pulse Cycling Process
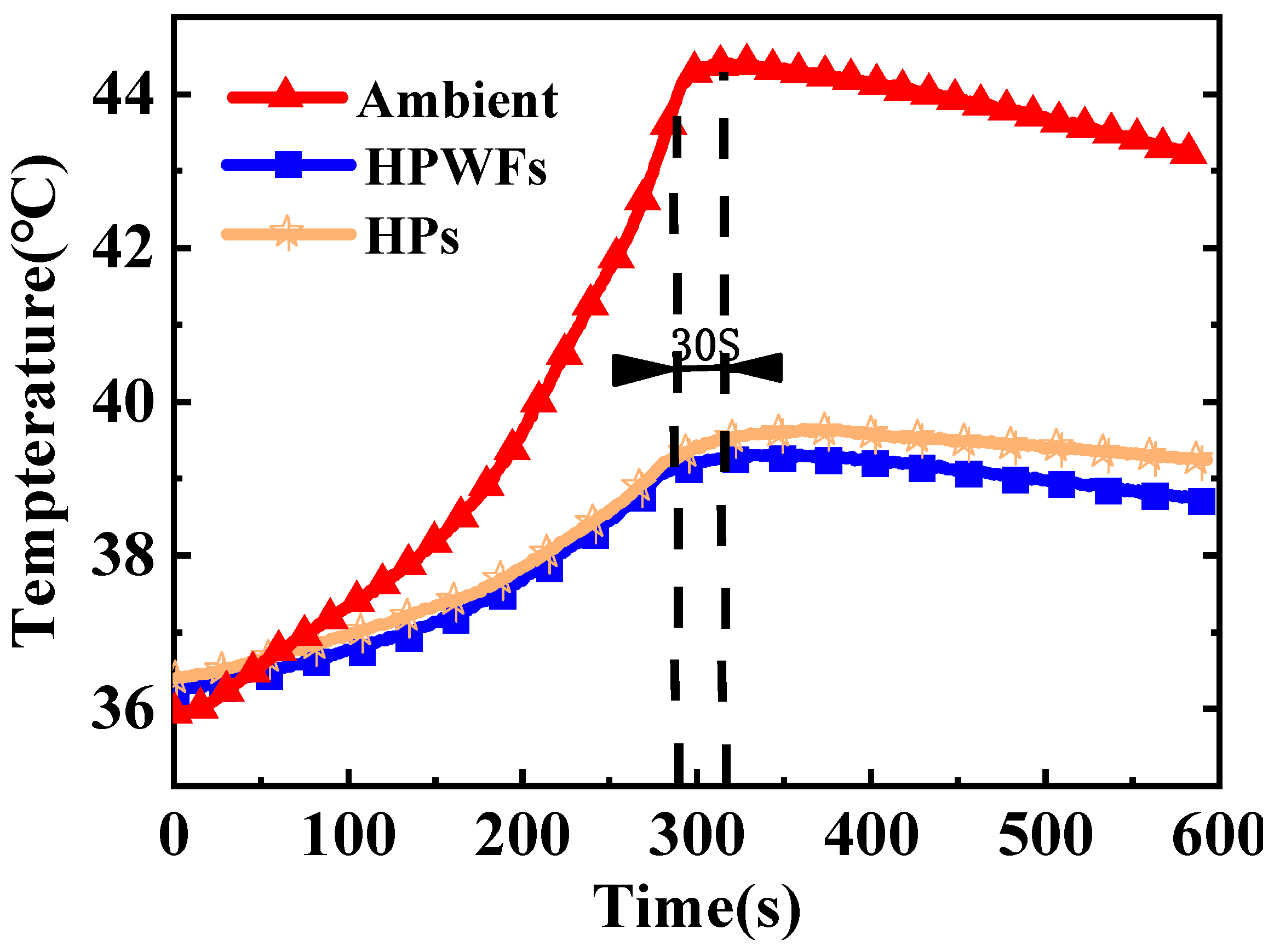
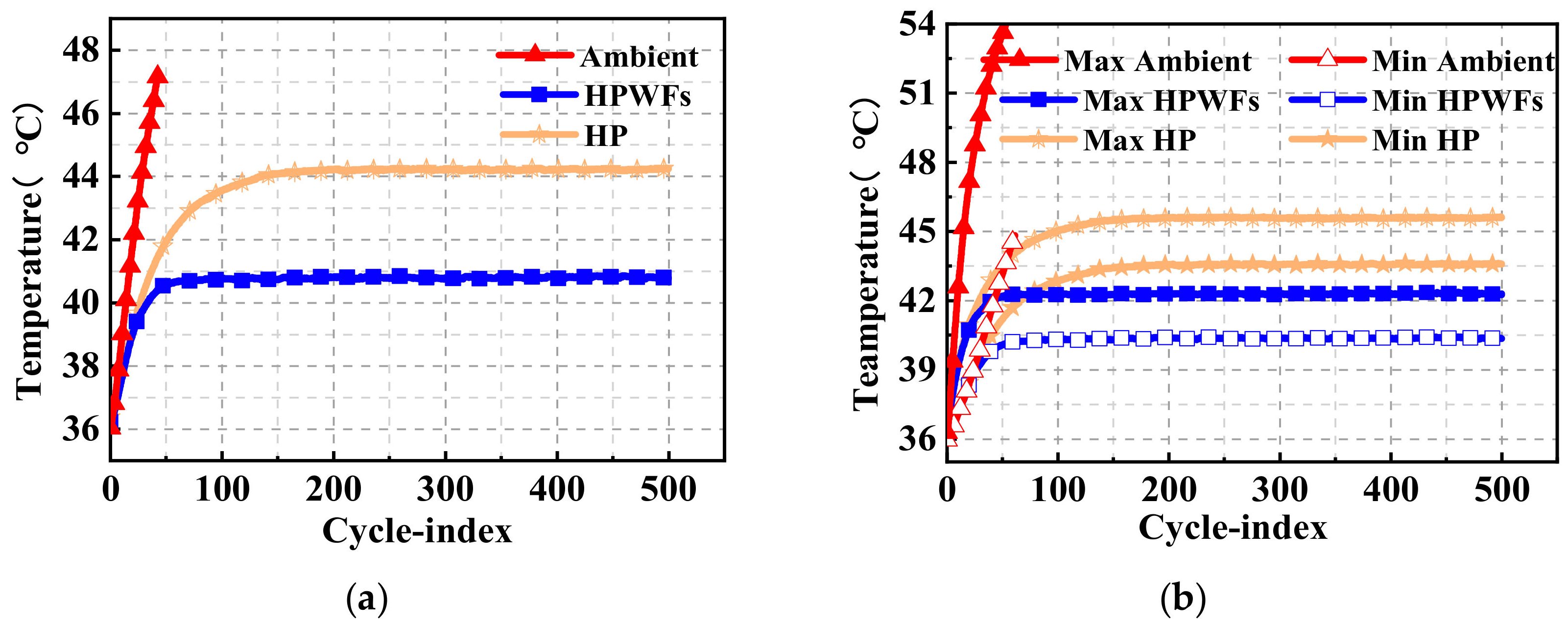
3.2.1. Effects of Heat Dissipation on Pulse Circulation
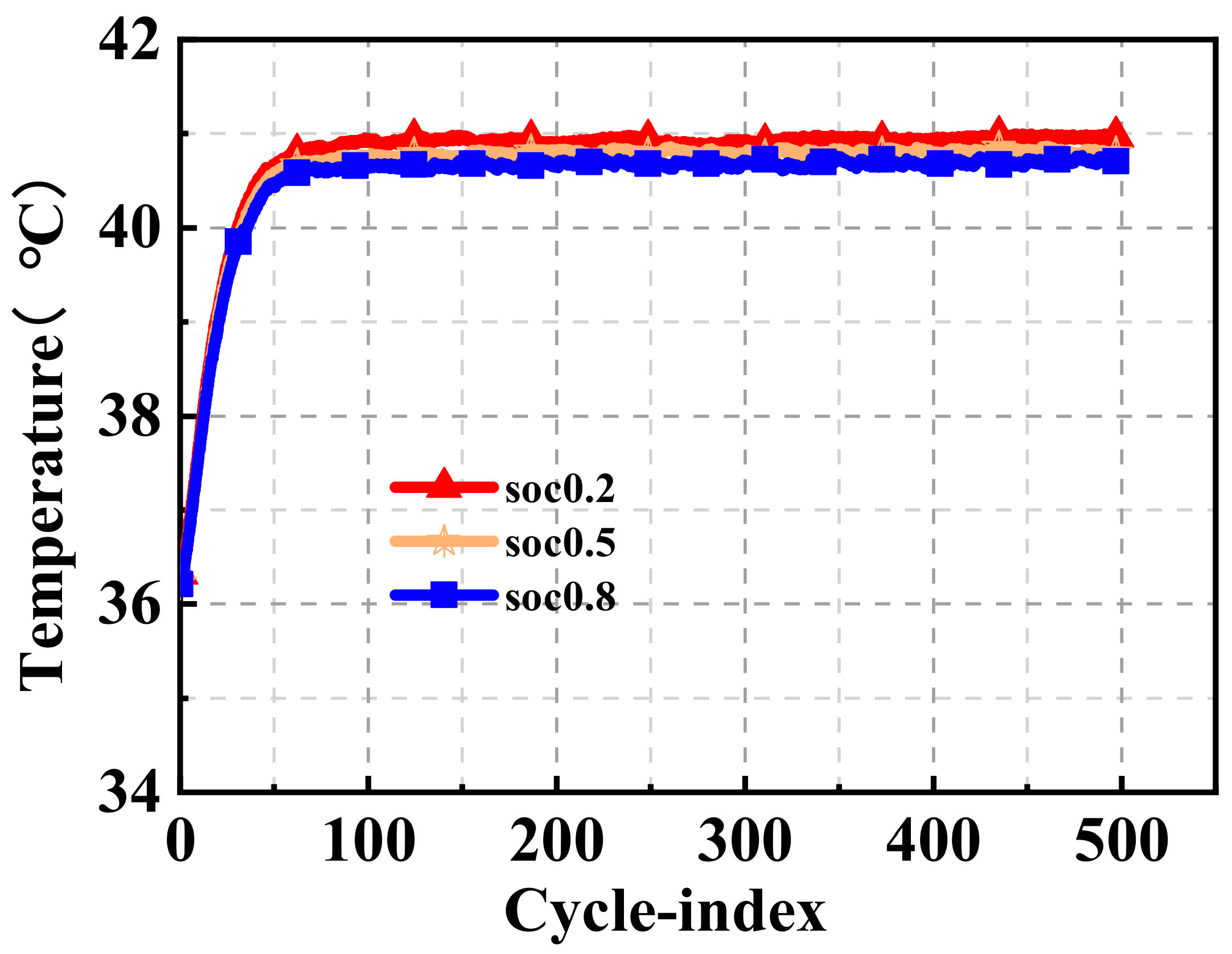
3.2.2. Effects of the Discharge Depth on Pulse Circulation
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| BTMS | battery thermal management system |
| TMS | thermal management system |
| HP | heat pipe |
| SOC | state of charge |
| PCM | phase change material |
| CFD | computed fluid dynamics |
| HPWF | heat pipe with fins |
| beg | the beginning of battery |
| end | the finial of battery |
| e | evaporation |
| c | condensation |
| B | battery |
| battery heat; | |
| working current; | |
| Ohmic internal resistance; | |
| polarized internal resistance; | |
| battery temperature; °C | |
| battery temperature influence factor |
References
- Scrosati, B.; Garche, J. Lithium batteries: Status, prospects and future. J. Power Sources 2010, 195, 2419–2430. [Google Scholar] [CrossRef]
- Nieto, N.; Díaz, L.; Gastelurrutia, J.; Blanco, F.; Ramos, J.C.; Rivas, A. Novel thermal management system design methodology for power lithium-ion battery. J. Power Sources 2014, 272, 291–302. [Google Scholar] [CrossRef]
- E, J.Q.; Yue, M.; Chen, J.W.; Zhu, H.; Deng, Y.W.; Zhu, Y.; Zhang, F.; Wen, M.; Zhang, B.; Kang, S.Y. Effects of the different air cooling strategies on cooling performance of a lithium-ion battery module with baffle. Appl. Therm. Eng. 2018, 144, 231–241. [Google Scholar] [CrossRef]
- De Simón-Martín, M.; de la Puente-Gil, Á.; Borge-Diez, D.; Ciria-Garcés, T.; González-Martínez, A.J.E.P. Wind energy planning for a sustainable transition to a decarbonized generation scenario based on the opportunity cost of the wind energy: Spanish Iberian Peninsula as case study. Energy Procedia 2019, 157, 1144–1163. [Google Scholar] [CrossRef]
- Shoaib, M.; Siddiqui, I.; Rehman, S.; Khan, S.; Alhems, L.M. Assessment of wind energy potential using wind energy conversion system. J. Clean. Prod. 2019, 216, 346–360. [Google Scholar] [CrossRef]
- Fathi Nassar, Y.; Yassin Alsadi, S. Assessment of solar energy potential in Gaza Strip-Palestine. Sustain. Energy Technol. Assess. 2019, 31, 318–328. [Google Scholar] [CrossRef]
- López-Lapeña, O.; Pallas-Areny, R. Solar energy radiation measurement with a low–power solar energy harvester. Comput. Electron. Agric. 2018, 151, 150–155. [Google Scholar] [CrossRef]
- Gourdo, L.; Fatnassi, H.; Tiskatine, R.; Wifaya, A.; Demrati, H.; Aharoune, A.; Bouirden, L. Solar energy storing rock-bed to heat an agricultural greenhouse. Energy 2019, 169, 206–212. [Google Scholar] [CrossRef]
- Cetin, T.H.; Kanoglu, M.; Yanikomer, N. Cryogenic energy storage powered by geothermal energy. Geothermics 2019, 77, 34–40. [Google Scholar] [CrossRef]
- Bao, T.; Meldrum, J.; Green, C.; Vitton, S.; Liu, Z.; Bird, K. Geothermal energy recovery from deep flooded copper mines for heating. Energy Convers. Manag. 2019, 183, 604–616. [Google Scholar] [CrossRef]
- Guerra, M.; Cienfuegos, R.; Thomson, J.; Suarez, L. Tidal energy resource characterization in Chacao Channel, Chile. Int. J. Mar. Energy 2017, 20, 1–16. [Google Scholar] [CrossRef]
- Chawla, N.; Bharti, N.; Singh, S. Recent Advances in Non-Flammable Electrolytes for Safer Lithium-Ion Batteries. Batteries 2019, 5, 19. [Google Scholar] [CrossRef]
- Safa, M.; Adelowo, E.; Chamaani, A.; Chawla, N.; Baboukani, A.R.; Herndon, M.; Wang, C.; El-Zahab, B. Poly(Ionic Liquid)-Based Composite Gel Electrolyte for Lithium Batteries. ChemElectroChem 2019, 6, 3319–3326. [Google Scholar] [CrossRef]
- Ianniciello, L.; Biwolé, P.H.; Achard, P. Electric vehicles batteries thermal management systems employing phase change materials. J. Power Sources 2018, 378, 383–403. [Google Scholar] [CrossRef]
- Safa, M.; Chamaani, A.; Chawla, N.; El-Zahab, B. Polymeric Ionic Liquid Gel Electrolyte for Room Temperature Lithium Battery Applications. Electrochim. Acta 2016, 213, 587–593. [Google Scholar] [CrossRef]
- Huang, Q.; Li, X.; Zhang, G.; Zhang, J.; He, F.; Li, Y. Experimental investigation of the thermal performance of heat pipe assisted phase change material for battery thermal management system. Appl. Therm. Eng. 2018, 141, 1092–1100. [Google Scholar] [CrossRef]
- Liu, F.; Lan, F.; Chen, J. Dynamic thermal characteristics of heat pipe via segmented thermal resistance model for electric vehicle battery cooling. J. Power Sources 2016, 321, 57–70. [Google Scholar] [CrossRef]
- Mahamud, R.; Park, C. Reciprocating air flow for Li-ion battery thermal management to improve temperature uniformity. J. Power Sources 2011, 196, 5685–5696. [Google Scholar] [CrossRef]
- Yang, Y.; Hu, X.; Qing, D.; Chen, F. Arrhenius Equation-Based Cell-Health Assessment: Application to Thermal Energy Management Design of a HEV NiMH Battery Pack. Energies 2013, 6, 2709–2725. [Google Scholar] [CrossRef] [Green Version]
- Demircalı, A.; Sergeant, P.; Koroglu, S.; Kesler, S.; Öztürk, E.; Tumbek, M. Influence of the temperature on energy management in battery-ultracapacitor electric vehicles. J. Clean. Prod. 2018, 176, 716–725. [Google Scholar] [CrossRef]
- Chiu, K.C.; Lin, C.H.; Yeh, S.F.; Lin, Y.H.; Huang, C.S.; Chen, K.C. Cycle life analysis of series connected lithium-ion batteries with temperature difference. J. Power Sources 2014, 263, 75–84. [Google Scholar] [CrossRef]
- Pesaran, A.A. Battery thermal models for hybrid vehicle simulations. J. Power Sources 2002, 110, 377–382. [Google Scholar] [CrossRef]
- Al-Zareer, M.; Dincer, I.; Rosen, M.A. Heat and mass transfer modeling and assessment of a new battery cooling system. Int. J. Heat Mass Transf. 2018, 126, 765–778. [Google Scholar] [CrossRef]
- Giuliano, M.R.; Prasad, A.K.; Advani, S.G. Experimental study of an air-cooled thermal management system for high capacity lithium–titanate batteries. J. Power Sources 2012, 216, 345–352. [Google Scholar] [CrossRef]
- Wang, H.; Ma, L. Thermal management of a large prismatic battery pack based on reciprocating flow and active control. Int. J. Heat Mass Transf. 2017, 115, 296–303. [Google Scholar] [CrossRef]
- Arya, A.; Sarafraz, M.M.; Shahmiri, S.; Madani, S.A.H.; Nikkhah, V.; Nakhjavani, S.M. Thermal performance analysis of a flat heat pipe working with carbon nanotube-water nanofluid for cooling of a high heat flux heater. Heat Mass Transf. 2017, 54, 985–997. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Hormozi, F. Experimental study on the thermal performance and efficiency of a copper made thermosyphon heat pipe charged with alumina–glycol based nanofluids. Powder Technol. 2014, 266, 378–387. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Hormozi, F.; Peyghambarzadeh, S.M. Thermal performance and efficiency of a thermosyphon heat pipe working with a biologically ecofriendly nanofluid. Int. Commun. Heat Mass Transf. 2014, 57, 297–303. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Pourmehran, O.; Yang, B.; Arjomandi, M. Assessment of the thermal performance of a thermosyphon heat pipe using zirconia-acetone nanofluids. Renew. Energy 2019, 136, 884–895. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Tian, Z.; Tlili, I.; Kazi, S.; Goodarzi, M. Thermal evaluation of a heat pipe working with n-pentane-acetone and n-pentane-methanol binary mixtures. J. Therm. Anal. Calorim. 2019, 1–11. [Google Scholar] [CrossRef]
- Shah, K.; McKee, C.; Chalise, D.; Jain, A. Experimental and numerical investigation of core cooling of Li-ion cells using heat pipes. Energy 2016, 113, 852–860. [Google Scholar] [CrossRef] [Green Version]
- Putra, N.; Ariantara, B.; Pamungkas, R.A. Experimental investigation on performance of lithium-ion battery thermal management system using flat plate loop heat pipe for electric vehicle application. Appl. Therm. Eng. 2016, 99, 784–789. [Google Scholar] [CrossRef]
- Wang, J.C.; Transfer, M. Thermal investigations on LED vapor chamber-based plates. Int. Commun. Heat Mass Transf. 2011, 38, 1206–1212. [Google Scholar] [CrossRef]
- Yang, K.S.; Yang, T.Y.; Tu, C.W.; Yeh, C.T.; Lee, M.T. A novel flat polymer heat pipe with thermal via for cooling electronic devices. Energy Convers. Manag. 2015, 100, 37–44. [Google Scholar] [CrossRef]
- Naphon, P.; Wongwises, S.; Wiriyasart, S. Application of two-phase vapor chamber technique for hard disk drive cooling of PCs. Int. Commun. Heat Mass Transf. 2013, 40, 32–35. [Google Scholar] [CrossRef]
- Wang, R.T.; Wang, J.C.; Chang, T.L. Technology Experimental analysis for thermal performance of a vapor chamber applied to high-performance servers. Int. Commun. Heat Mass Transf. 2011, 19, 353–360. [Google Scholar]
- Jouhara, H.; Meskimmon, R. Heat pipe based thermal management systems for energy-efficient data centres. Energy 2014, 77, 265–270. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.C. Development of vapour chamber-based VGA thermal module. Int. J. Numer. Methods Heat Fluid Flow 2010, 20, 416–428. [Google Scholar] [CrossRef]
- Zeng, J.; Zhang, S.; Chen, G.; Lin, L.; Sun, Y.; Chuai, L.; Yuan, W. Experimental investigation on thermal performance of aluminum vapor chamber using micro-grooved wick with reentrant cavity array. Appl. Therm. Eng. 2018, 130, 185–194. [Google Scholar] [CrossRef]
- Chi, R.G.; Rhi, S.H. Oscillating Heat Pipe Cooling System of Electric Vehicle’s Li-Ion Batteries with Direct Contact Bottom Cooling Mode. Energies 2019, 12, 1698. [Google Scholar] [CrossRef]
- Tran, T.H.; Harmand, S.; Sahut, B. Experimental investigation on heat pipe cooling for Hybrid Electric Vehicle and Electric Vehicle lithium-ion battery. J. Power Sources 2014, 265, 262–272. [Google Scholar] [CrossRef]
- Zhao, R.; Gu, J.; Liu, J. An experimental study of heat pipe thermal management system with wet cooling method for lithium ion batteries. J. Power Sources 2015, 273, 1089–1097. [Google Scholar] [CrossRef]
- Rao, Z.; Wang, S.; Wu, M.; Lin, Z.; Li, F. Experimental investigation on thermal management of electric vehicle battery with heat pipe. Energy Convers. Manag. 2013, 65, 92–97. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, B.; Xue, Q.F.; Sun, H.L.; Li, B.; Zou, H.M.; Yan, Y.Y. Experimental investigation on EV battery cooling and heating by heat pipes. Appl. Therm. Eng. 2015, 88, 54–60. [Google Scholar] [CrossRef]
- Fully Integrated Solutions. Available online: http://www.a123systems.com/automotive/products./systems/ (accessed on 24 April 2019).
- Jung, E.G.; Boo, J.H. Thermal numerical model of a high temperature heat pipe heat exchanger under radiation. Appl. Energy 2014, 135, 586–596. [Google Scholar] [CrossRef]
- Kline, S.J.; McClintock, F.A. Describing Uncertainties in Single-Sample Experiments. Mech. Eng. 1953, 75, 3–8. [Google Scholar]


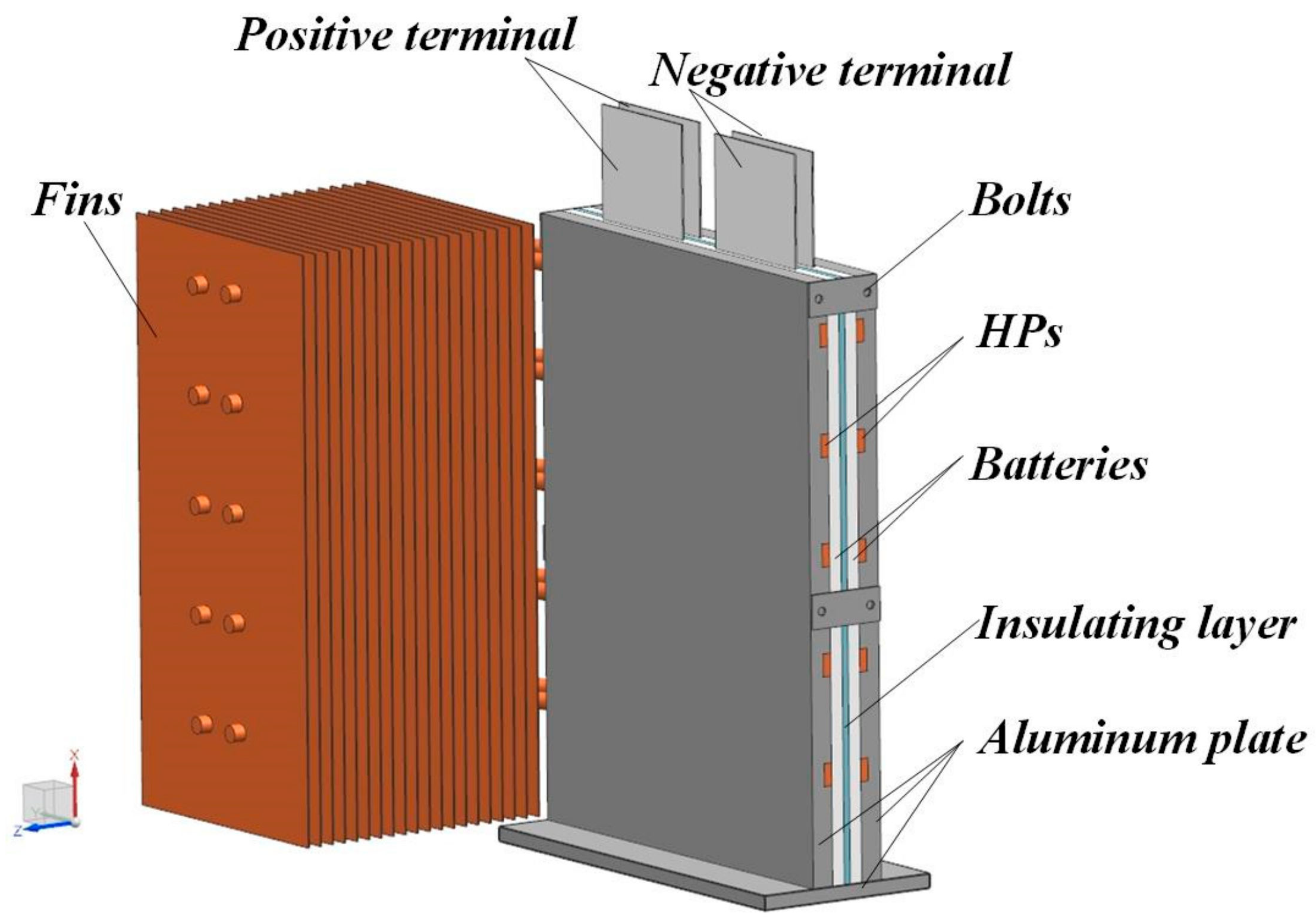
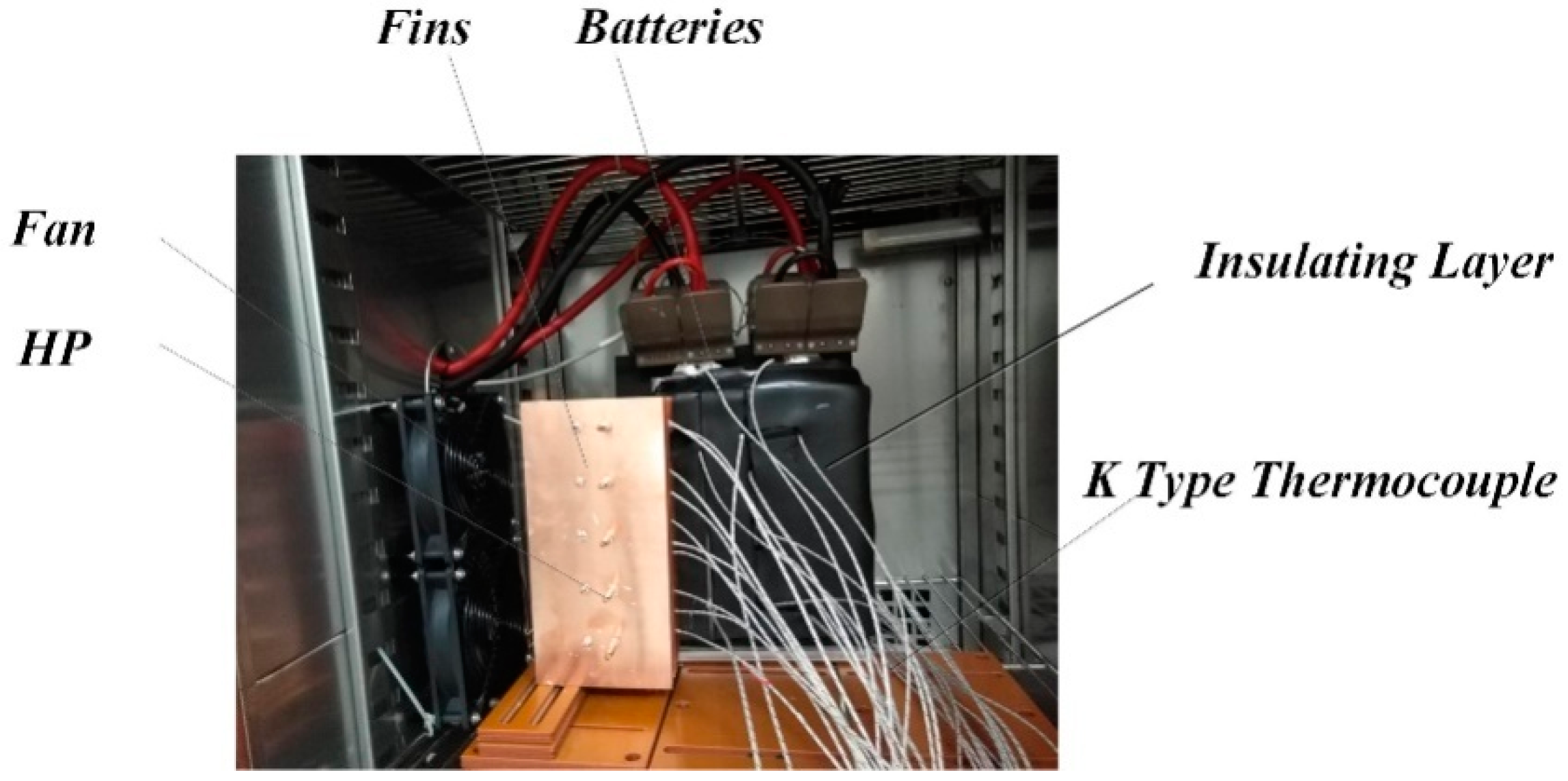


| Cell Dimensions (mm) | |
|---|---|
| Voltage (V) | 3.3 |
| Capacity (Ah) | 8-LFP |
| Operating Temperature (°C) | −30 to 50 |
| Storage Temperature (°C) | −40 to 60 |
| Cell Weight (g) | 330 |
| Cutoff Voltage During Charging (V) | 3.85 |
| Cutoff Voltage During Discharging (V) | 1.7 |
| Name | |
|---|---|
| Aluminum plate | |
| Groove | |
| Fin | |
| Fin aperture |
| Device | Accuracy |
|---|---|
| thermostatic test chamber | ±0.5 °C |
| Agilent Model 34972 | ±0.004% |
| Type K Thermocouple | ±0.75% |
| Heat Dissipation Methods | Discharge Current (A) | ||||
|---|---|---|---|---|---|
| HP | 50 | 36.3 | 38.3 | 2.0 | 1.0 |
| 75 | 36.3 | 38.9 | 2.6 | 1.5 | |
| 100 | 36.3 | 39.3 | 3.0 | 1.8 | |
| HP with Fins | 50 | 36.2 | 38.1 | 1.9 | 0.8 |
| 75 | 36.2 | 38.6 | 2.4 | 1.4 | |
| 100 | 36.2 | 39.0 | 2.8 | 1.7 | |
| Ambient | 50 | 36.1 | 41.0 | 4.9 | 3.8 |
| 75 | 36.1 | 42.7 | 6.6 | 5.0 | |
| 100 | 35.9 | 43.8 | 7.9 | 6.0 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, S.; Li, K.; Xie, Y.; Wu, C.; Wang, P.; Yu, M.; Li, B.; Zheng, J. Heat Pipe Thermal Management Based on High-Rate Discharge and Pulse Cycle Tests for Lithium-Ion Batteries. Energies 2019, 12, 3143. https://doi.org/10.3390/en12163143
Deng S, Li K, Xie Y, Wu C, Wang P, Yu M, Li B, Zheng J. Heat Pipe Thermal Management Based on High-Rate Discharge and Pulse Cycle Tests for Lithium-Ion Batteries. Energies. 2019; 12(16):3143. https://doi.org/10.3390/en12163143
Chicago/Turabian StyleDeng, Shasha, Kuining Li, Yi Xie, Cunxue Wu, Pingzhong Wang, Miao Yu, Bo Li, and Jintao Zheng. 2019. "Heat Pipe Thermal Management Based on High-Rate Discharge and Pulse Cycle Tests for Lithium-Ion Batteries" Energies 12, no. 16: 3143. https://doi.org/10.3390/en12163143
APA StyleDeng, S., Li, K., Xie, Y., Wu, C., Wang, P., Yu, M., Li, B., & Zheng, J. (2019). Heat Pipe Thermal Management Based on High-Rate Discharge and Pulse Cycle Tests for Lithium-Ion Batteries. Energies, 12(16), 3143. https://doi.org/10.3390/en12163143






