The Investigation of Permeability Calculation Using Digital Core Simulation Technology
Abstract
:1. Introduction
2. Mathematical Method
2.1. Direct Simulation of Navier-Stokes Equations
2.2. Pore Network Model Simulation
2.3. Lattice Boltzmann Method
3. Numerical Simulation and Analysis
3.1. Physical Models
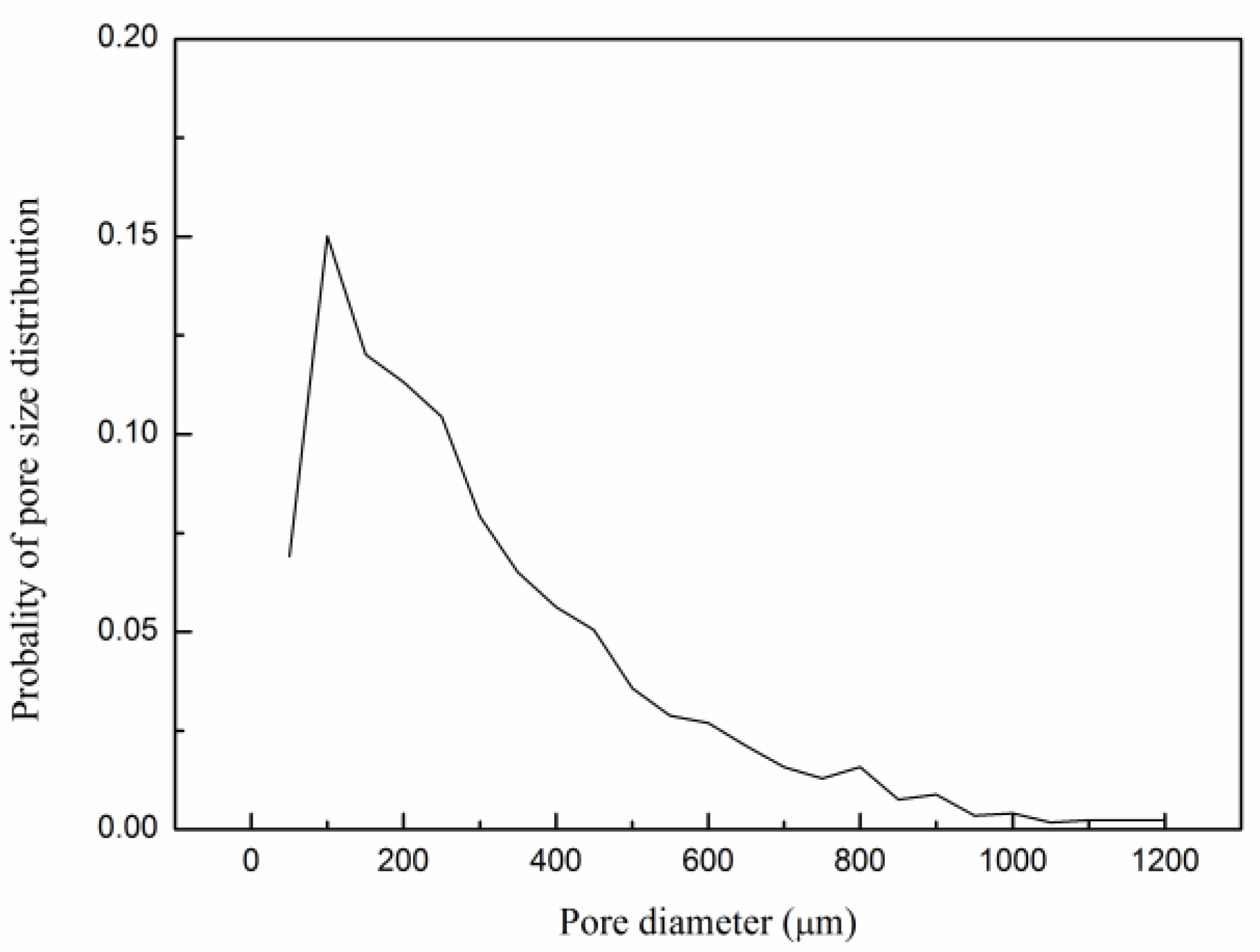
3.1.1. X-Ray CT Scanning Test
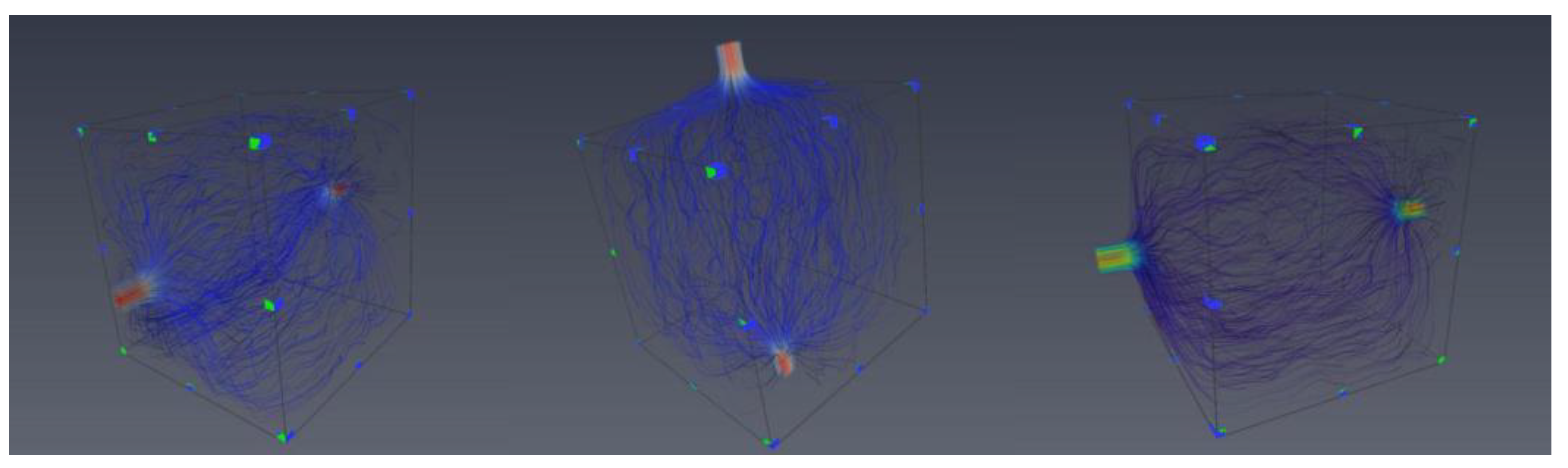
3.1.2. Permeability Calculation Based on Direct Solving of N-S Equations in Avizo
3.1.3. Permeability Calculation Based on Pore Network Model Simulation
3.1.4. Permeability Calculation Based on the Lattice Boltzmann Method
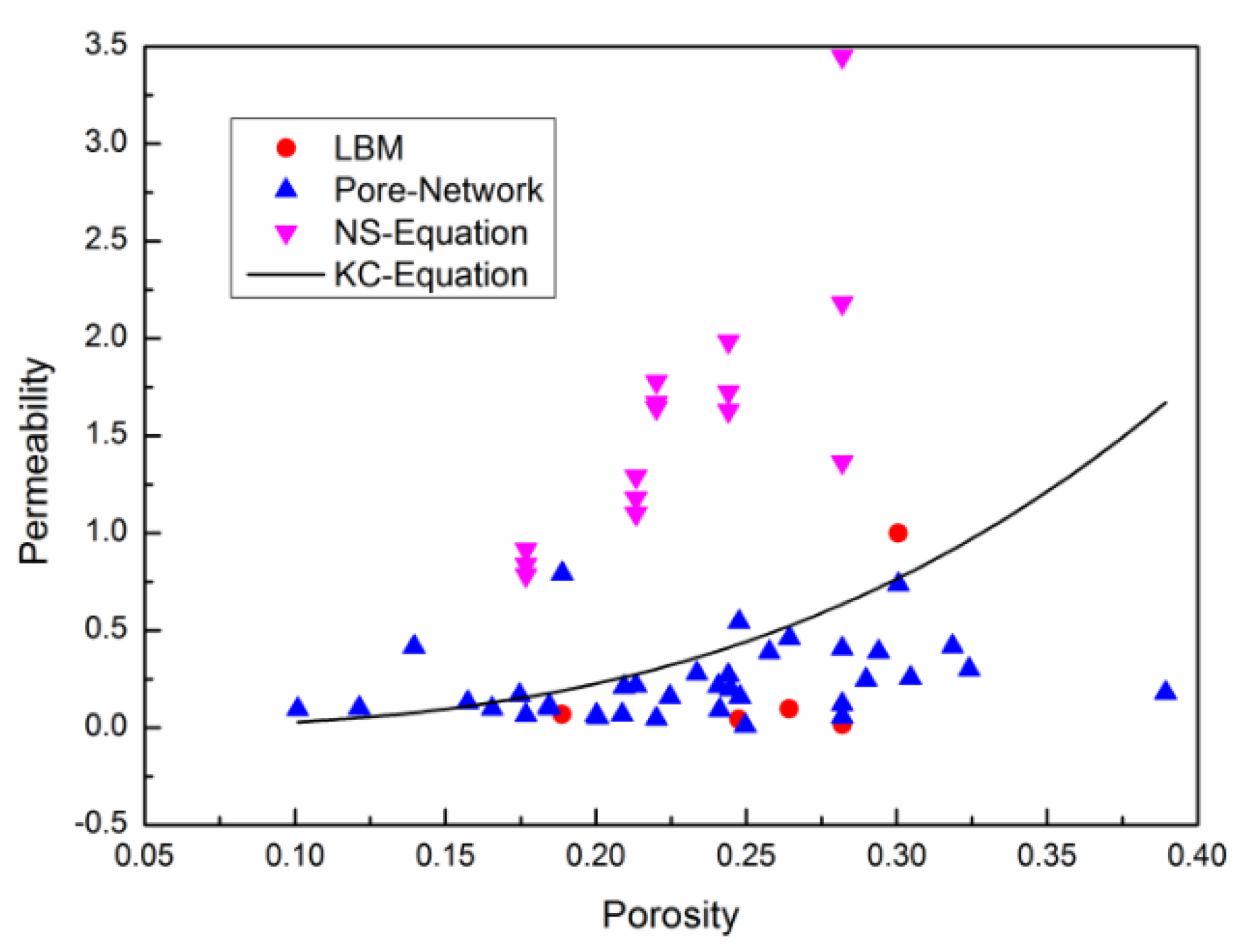
3.2. Results Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Knackstedt, M.A.; Sok, R.; Adrian, S.; Arns, C.H. 3D Pore Scale Characterisation of Carbonate Core: Relating pore types and interconnectivity to petrophysical and multiphase flow properties. In Proceedings of the International Petroleum Technology Conference, Dubai, UAE, 4–6 December 2007; p. 6. [Google Scholar]
- Cipolla, C.L.; Lolon, E.; Mayerhofer, M.J.; Warpinski, N.R. Fracture Design Considerations in Horizontal Wells Drilled in Unconventional Gas Reservoirs. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, The Woodlands, TX, USA, 19–21 January 2009; p. 10. [Google Scholar]
- Wildenschild, D.; Sheppard, A.P. X-ray imaging and analysis techniques for quantifying pore-scale structure and processes in subsurface porous medium systems. Adv. Water Resour. 2013, 51, 217–246. [Google Scholar] [CrossRef]
- Joshi, M.Y. A Class of Stochastic Models for Porous Media; University Microfilms: Ann Arbor, MI, USA, 1975. [Google Scholar]
- Hazlett, R. Statistical characterization and stochastic modeling of pore networks in relation to fluid flow. Math. Geol. 1997, 29, 801–822. [Google Scholar] [CrossRef]
- Yeong, C.; Torquato, S. Reconstructing random media. Phys. Rev. 1998, 57, 495. [Google Scholar] [CrossRef]
- Bryant, S.; Blunt, M. Prediction of relative permeability in simple porous media. Phys. Rev. 1992, 46, 2004. [Google Scholar] [CrossRef] [PubMed]
- Okabe, H.; Blunt, M.J. Prediction of permeability for porous media reconstructed using multiple-point statistics. Phys. Rev. 2004, 70, 066135. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, K.; Van Dijke, M.I.; Couples, G.D.; Jiang, Z.; Ma, J.; Sorbie, K.S.; Crawford, J.; Young, I.; Zhang, X. 3D stochastic modelling of heterogeneous porous media–applications to reservoir rocks. Transp. Porous Media 2006, 65, 443–467. [Google Scholar] [CrossRef]
- Montemagno, C.; Pyrak-Nolte, L. Fracture network versus single fractures: Measurement of fracture geometry with X-ray tomography. Phys. Chem. Earth A Solid Earth Geod. 1999, 24, 575–579. [Google Scholar] [CrossRef]
- Wolf, K.-H.A.; van Bergen, F.; Ephraim, R.; Pagnier, H. Determination of the cleat angle distribution of the RECOPOL coal seams, using CT-scans and image analysis on drilling cuttings and coal blocks. Int. J. Coal Geol. 2008, 73, 259–272. [Google Scholar] [CrossRef]
- Cai, Y.; Liu, D.; Mathews, J.P.; Pan, Z.; Elsworth, D.; Yao, Y.; Li, J.; Guo, X. Permeability evolution in fractured coal—Combining triaxial confinement with X-ray computed tomography, acoustic emission and ultrasonic techniques. Int. J. Coal Geol. 2014, 122, 91–104. [Google Scholar] [CrossRef]
- Watanabe, N.; Hirano, N.; Tsuchiya, N. Determination of aperture structure and fluid flow in a rock fracture by high-resolution numerical modeling on the basis of a flow-through experiment under confining pressure. Water Resour. Res. 2008, 44. [Google Scholar] [CrossRef]
- Nemoto, K.; Watanabe, N.; Hirano, N.; Tsuchiya, N. Direct measurement of contact area and stress dependence of anisotropic flow through rock fracture with heterogeneous aperture distribution. Earth Planet. Sci. Lett. 2009, 281, 81–87. [Google Scholar] [CrossRef]
- Zhu, G.; Chen, H.; Yao, J.; Sun, S. Efficient energy-stable schemes for the hydrodynamics coupled phase-field model. Appl. Math. Model. 2019, 70, 82–108. [Google Scholar] [CrossRef]
- Blunt, M.; King, P. Relative permeabilities from two-and three-dimensional pore-scale network modelling. Transport. Porous Media 1991, 6, 407–433. [Google Scholar] [CrossRef]
- Qian, Y.-H.; d’Humières, D.; Lallemand, P. Lattice BGK models for Navier-Stokes equation. EPL Europhys. Lett. 1992, 17, 479. [Google Scholar] [CrossRef]
- Zhang, L.; Kang, Q.; Yao, J.; Gao, Y.; Sun, Z.; Liu, H.; Valocchi, A.J. Pore scale simulation of liquid and gas two-phase flow based on digital core technology. Sci. China Technol. Sci. 2015, 58, 1375–1384. [Google Scholar] [CrossRef]
- Chen, L.; Kang, Q.; He, Y.-L.; Tao, W.-Q. Pore-scale simulation of coupled multiple physicochemical thermal processes in micro reactor for hydrogen production using lattice Boltzmann method. Int. J. Hydrog. Energy 2012, 37, 13943–13957. [Google Scholar] [CrossRef]
- Kang, Q.; Zhang, D.; Chen, S.; He, X. Lattice Boltzmann simulation of chemical dissolution in porous media. Phys. Rev. 2002, 65, 036318. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, L.; Kang, Q.; Mu, Y.; He, Y.-L.; Tao, W.-Q. A critical review of the pseudopotential multiphase lattice Boltzmann model: Methods and applications. Int. J. Heat Mass Transf. 2014, 76, 210–236. [Google Scholar] [CrossRef]
- Javanbakht, G.; Arshadi, M.; Qin, T.; Goual, L. Micro-scale displacement of NAPL by surfactant and microemulsion in heterogeneous porous media. Adv. Water Resour. 2017, 105, 173–187. [Google Scholar] [CrossRef]
- Qin, T.; Javanbakht, G.; Goual, L.; Piri, M.; Towler, B. Microemulsion-enhanced displacement of oil in porous media containing carbonate cements. Coll. Surf. A Physicochem. Eng. Asp. 2017, 530, 60–71. [Google Scholar] [CrossRef] [Green Version]



| Sample Number | Diameter/mm | Length/mm | Pixel Size/μm |
|---|---|---|---|
| No. 1 | 38 | 48 | 19.8502 |
| No. 2 | 38 | 49 | 19.8502 |
| No. 3 | 38 | 48 | 19.8502 |
| No. 4 | 38 | 47 | 19.8502 |
| No. 5 | 38 | 39 | 19.8502 |
| Direction | No. 1/μm2 | No. 2/μm2 | No. 3/μm2 | No. 4/μm2 | No. 5/μm2 |
|---|---|---|---|---|---|
| X | 0.84 | 1.37 | 1.29 | 1.98 | 1.67 |
| Y | 0.92 | 2.18 | 1.18 | 1.63 | 1.64 |
| Z | 0.78 | 3.45 | 1.10 | 1.73 | 1.78 |
| Sub-Region | No. 1/μm2 | No. 2/μm2 | No. 3/μm2 | No. 4/μm2 | No. 5/μm2 |
|---|---|---|---|---|---|
| 1 | 0.065 | 0.41 | 0.22 | 0.27 | 0.05 |
| 2 | 0.10 | 0.46 | 0.16 | 0.39 | 0.16 |
| 3 | 0.10 | 0.54 | 0.21 | 0.28 | 0.07 |
| 4 | 0.05 | 0.73 | 0.20 | 0.22 | 0.07 |
| 5 | 0.13 | 0.30 | 0.11 | 0.25 | 0.09 |
| 6 | 0.09 | 0.18 | 0.25 | 0.39 | 0.42 |
| 7 | 0.01 | 0.79 | 0.10 | 0.17 | 0.42 |
| Sample Number | Permeability/μm2 |
|---|---|
| No. 1 | 0.27 |
| No. 2 | 0.18 |
| No. 3 | 0.24 |
| No. 4 | 0.28 |
| No. 5 | 0.39 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Jing, W.; Yang, Y.; Yang, H.; Guo, Y.; Sun, H.; Zhao, J.; Yao, J. The Investigation of Permeability Calculation Using Digital Core Simulation Technology. Energies 2019, 12, 3273. https://doi.org/10.3390/en12173273
Zhang L, Jing W, Yang Y, Yang H, Guo Y, Sun H, Zhao J, Yao J. The Investigation of Permeability Calculation Using Digital Core Simulation Technology. Energies. 2019; 12(17):3273. https://doi.org/10.3390/en12173273
Chicago/Turabian StyleZhang, Lei, Wenlong Jing, Yongfei Yang, Hainan Yang, Yaohao Guo, Hai Sun, Jianlin Zhao, and Jun Yao. 2019. "The Investigation of Permeability Calculation Using Digital Core Simulation Technology" Energies 12, no. 17: 3273. https://doi.org/10.3390/en12173273
APA StyleZhang, L., Jing, W., Yang, Y., Yang, H., Guo, Y., Sun, H., Zhao, J., & Yao, J. (2019). The Investigation of Permeability Calculation Using Digital Core Simulation Technology. Energies, 12(17), 3273. https://doi.org/10.3390/en12173273







