Optimal Configuration of a Gas Expansion Process in a Piston-Type Cylinder with Generalized Convective Heat Transfer Law
Abstract
:1. Introduction
2. Modeling
3. Optimal Solutions
3.1. Arc with
3.2. Arc with
3.3. Arc with
4. Numerical Examples
4.1. Calculation Example for
4.2. Calculation Example for
4.3. Calculation Example for
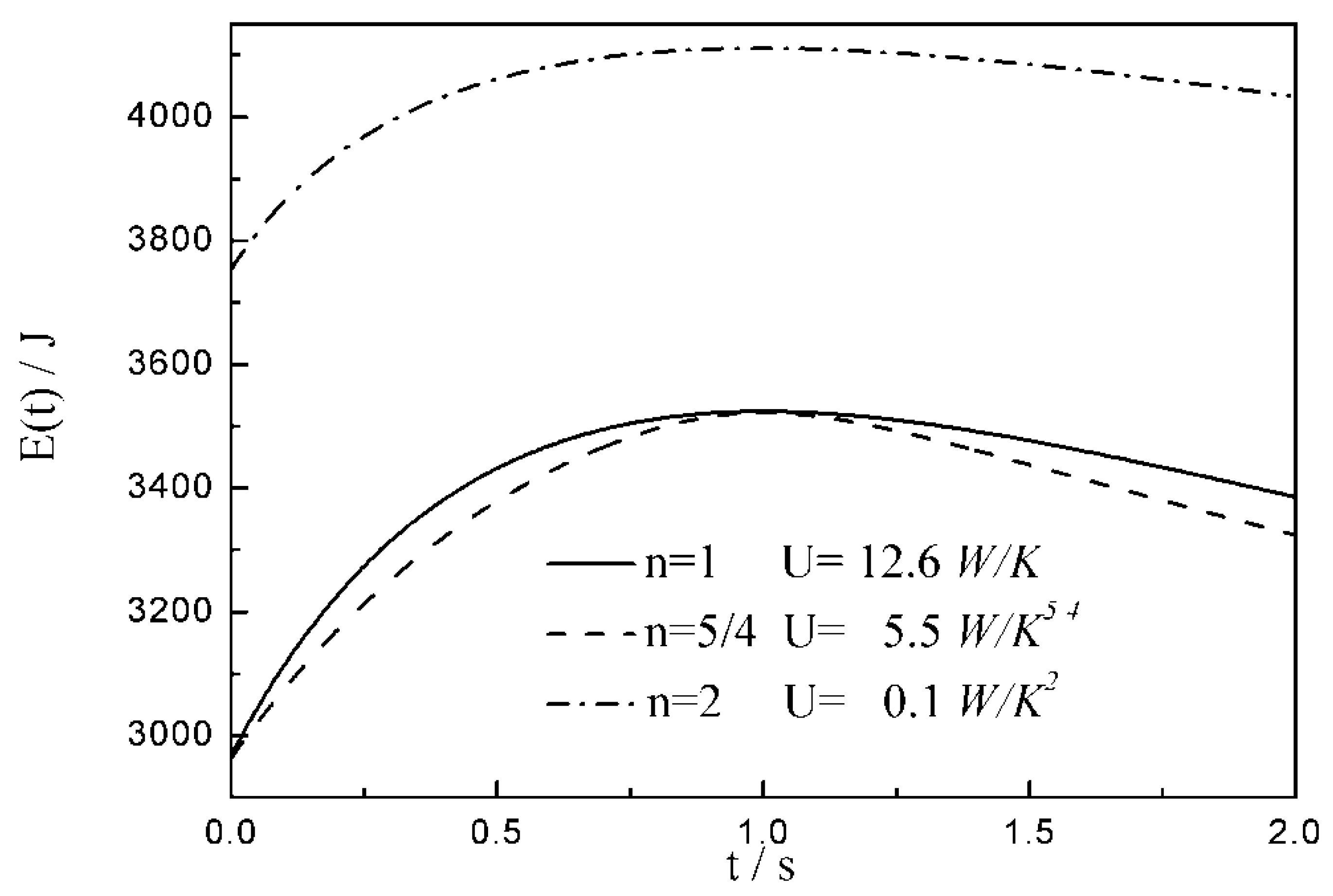
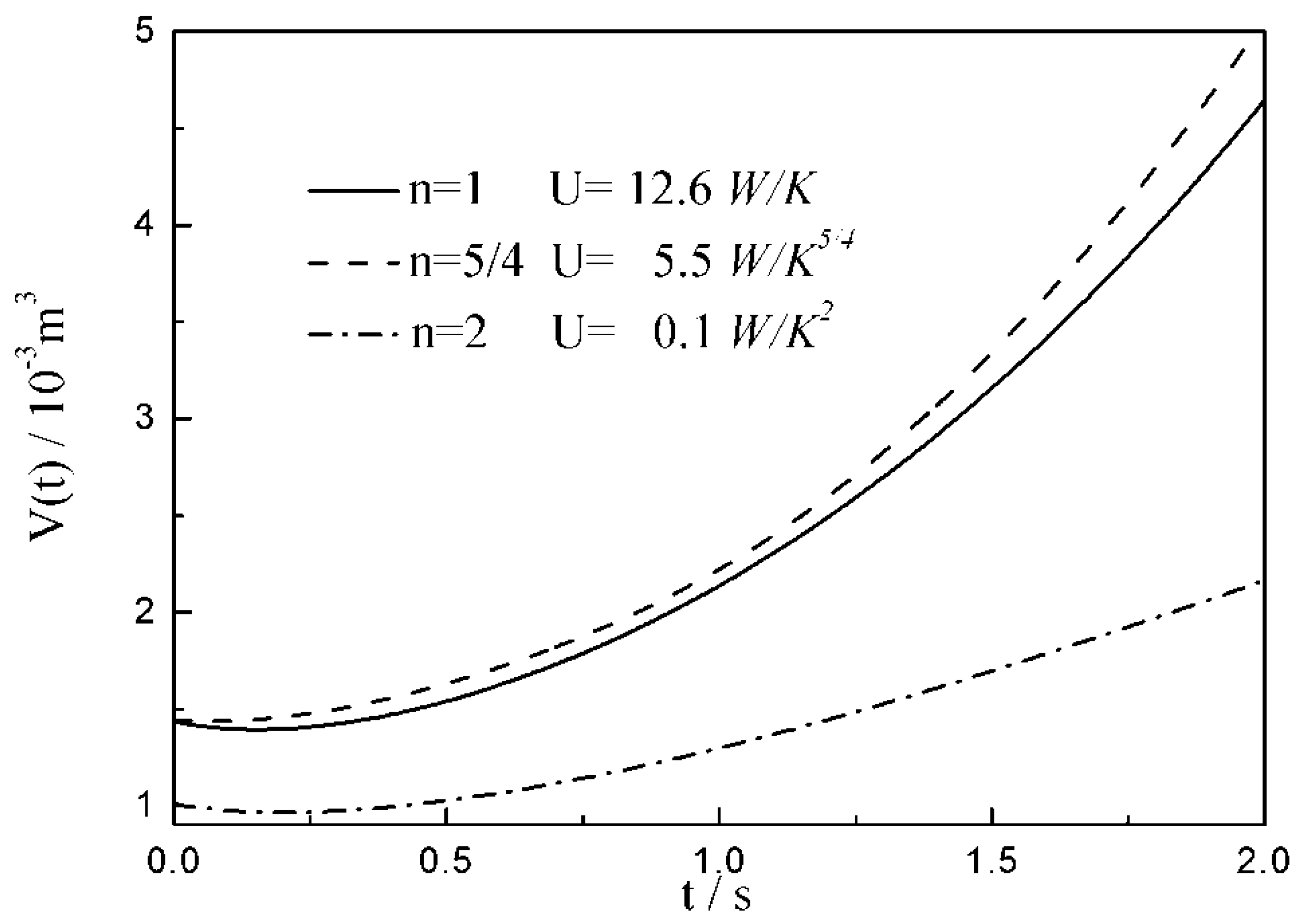
4.4. Performance Comparisons for Three Special HTLs
5. Conclusions
- (1)
- The relationships between the and time are similar under the conditions of three special heat transfer laws; namely, the increases to the peak before decreasing with the increase in time, and there is a maximum . For all of three special heat transfer laws, the working fluid is compressed slightly in the initial arc, and then monotonically expands until the end of expansion process. with the augmentation of the , of working fluid during the arc increases.
- (2)
- There are differences among the optimal configurations with three different heat transfer laws. In the cases of and , the temperature at which the whole arc occurs should be below the external heat bath temperature, namely less than ; with the augmentation of , the decreases, and the , maximum work output and increase. While for , the temperature at which the whole arc occurs should be above the external heat bath temperature, namely more than . This indicates that the working fluid does not absorb heat from the external heat bath, but releases heat to the external heat bath during the whole arc. With the augmentation of , the increases, and the , maximum work output and decrease. The results obtained with are not only different from those with and , but also from those with square , cubic and radiative heat transfer laws obtained in the previous studies. Moreover, compared with 1 and , the maximum work output and with 2 are smaller. It can be concluded that the heat transfer law has both quantitative and qualitative influences on the optimal configurations of the expansion process.
- (3)
- The generalized convective heat transfer law is introduced into the theoretical model of an ideal gas irreversible expansion process in a piston-type cylinder, so the results obtained are universal and fully reveal the effect of heat transfer laws. The work in this paper can enrich FTT theory.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
| Abbreviations | |
| DPHTL | Dulong–Petit heat transfer law |
| EHB | external heat bath |
| E-L | Euler–Lagrange |
| EP | expansion process |
| FTT | finite time thermodynamics |
| GCHTL | generalized convective heat transfer law |
| GRHTL | generalized radiative heat transfer law |
| HE | heat engine |
| HTL | heat transfer law |
| ICE | internal combustion engine |
| LPHTL | the linear phenomenological heat transfer law |
| MWP | maximum work output |
| NHTL | Newton’s heat transfer law |
| OC | optimal configuration |
| OPM | optimal piston motion |
| SCHTL | square convective heat transfer law |
| WF | working fluid |
| Nomenclature | |
| coefficient of the heat absorption rate | |
| exponent of the heat absorption rate | |
| mole specific heat | |
| internal energy | |
| heat absorption rate | |
| modified Lagrangian | |
| heat transfer index | |
| pressure | |
| heat transfer rate | |
| gas constant | |
| sign function | |
| temperature | |
| time | |
| heat conductance | |
| volume | |
| work output | |
| Greek symbol | |
| efficiency | |
| Lagrange multiplier | |
| Subscripts | |
| external heat bath | |
| final state of expansion process | |
| ambient or reference |
References
- Andresen, B.; Berry, R.S.; Ondrechen, M.J.; Salamon, P. Thermodynamics for processes in finite time. Acc. Chem. Res. 1984, 17, 266–271. [Google Scholar] [CrossRef]
- Berry, R.S.; Kazakov, V.A.; Sieniutycz, S.; Szwast, Z.; Tsirlin, A.M. Thermodynamic Optimization of Finite Time Processes; Wiley: Chichester, UK, 1999. [Google Scholar]
- Chen, L.G.; Wu, C.; Sun, F.R. Finite time thermodynamic optimization or entropy generation minimization of energy systems. J. Non-Equilib. Thermodyn. 1999, 24, 327–359. [Google Scholar] [CrossRef]
- Hoffmann, K.H.; Burzler, J.; Fischer, A.; Schaller, M.; Schubert, S. Optimal process paths for endoreversible systems. J. Non-Equilib. Thermodyn. 2003, 28, 233–268. [Google Scholar] [CrossRef]
- Chen, L.G.; Sun, F.R. Advances in Finite Time Thermodynamics: Analysis and Optimization; Nova Science Publishers: New York, NY, USA, 2004. [Google Scholar]
- Sieniutycz, S. Thermodynamic Approaches in Engineering Systems; Elsevier: Oxford, UK, 2016. [Google Scholar]
- Feidt, M. Finite Physical Dimensions Optimal Thermodynamics 1. Fundamental; ISTE Press and Elsevier: London, UK, 2017. [Google Scholar]
- Badescu, V. Optimal Control in Thermal Engineering; Springer: New York, NY, USA, 2017. [Google Scholar]
- Chen, L.G.; Xia, S.J. Generalized Thermodynamic Dynamic-Optimization for Irreversible Cycles- Thermodynamic and Chemical Theoretical Cycles; Science Press: Beijing, China, 2018. (In Chinese) [Google Scholar]
- Chen, L.G.; Xia, S.J. Generalized Thermodynamic Dynamic-Optimization for Irreversible Cycles–Engineering Thermodynamic Plants and Generalized Engine Cycles; Science Press: Beijing, China, 2018. (In Chinese) [Google Scholar]
- Chen, L.G.; Xia, S.J. Progresses in generalized thermodynamic dynamic-optimization of irreversible processes. Sci. Sin. Technol. 2019, 49, 981–1022. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Xia, S.J.; Feng, H.J. Progress in generalized thermodynamic dynamic-optimization of irreversible cycles. Sci. Sin. Technol. 2019, 49, 1223–1267. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Li, J. Thermodynamic Optimization Theory for Two-Heat-Reservoir Cycles; Science Press: Beijing, China, 2020. [Google Scholar]
- Boikov, S.Y.; Andresen, B.; Akhremenkov, A.A.; Tsirlin, A.M. Evaluation of irreversibility and optimal organization of an integrated multi-stream heat exchange system. J. Non-Equilib. Thermodyn. 2020, 45, 155–171. [Google Scholar] [CrossRef]
- Li, P.L.; Chen, L.G.; Xia, S.J.; Zhang, L. Entropy generation rate minimization for in methanol synthesis via CO2 hydrogenation reactor. Entropy 2019, 21, 174. [Google Scholar] [CrossRef] [Green Version]
- Zhang, L.; Chen, L.G.; Xia, S.J.; Ge, Y.L.; Wang, C.; Feng, H.J. Multi-objective optimization for helium-heated reverse water gas shift reactor by using NSGA-II. Int. J. Heat Mass Transfer 2020, 148, 119025. [Google Scholar] [CrossRef]
- Kingston, D.; Razzitte, A.C. Entropy generation minimization in Dimethyl Ether synthesis: A case study. J. Non-Equilib. Thermodyn. 2018, 43, 111–120. [Google Scholar] [CrossRef]
- Li, P.L.; Chen, L.G.; Xia, S.J.; Zhang, L.; Kong, R.; Ge, Y.L.; Feng, H.J. Entropy generation rate minimization for steam methane reforming reactor heated by molten salt. Energy Rep. 2020, 6, 685–697. [Google Scholar] [CrossRef]
- Marsik, F.; Weigand, B.; Tomas, M.; Tucek, O.; Novotny, P. On the efficiency of electrochemical devices from the perspective of endoreversible thermodynamics. J. Non-Equilib. Thermodyn. 2019, 44, 425–438. [Google Scholar] [CrossRef]
- Roach, T.N.F.; Salamon, P.; Nulton, J.; Andresen, B.; Felts, B.; Haas, A.; Calhoun, S.; Robinett, N.; Rohwer, F. Application of finite-time and control thermodynamics to biological processes at multiple scales. J. Non-Equilib. Thermodyn. 2018, 43, 193–210. [Google Scholar] [CrossRef] [Green Version]
- Barranco-Jimenez, M.A.; Ramos-Gayosso, I.; Rosales, M.A.; Angulo-Brown, F. A proposal of ecologic taxes based on thermoeconomic performance of heat engine models. Energies 2009, 2, 1042–1056. [Google Scholar] [CrossRef]
- Schwalbe, K.; Hoffmann, K.H. Novikov engine with fluctuating heat bath temperature. J. Non-Equilib. Thermodyn. 2018, 43, 141–150. [Google Scholar] [CrossRef]
- Schwalbe, K.; Hoffmann, K.H. Stochastic Novikov engine with Fourier heat transport. J. Non-Equilib. Thermodyn. 2019, 44, 417–424. [Google Scholar] [CrossRef]
- Paéz-Hernández, R.T.; Chimal-Eguía, J.C.; Sánchez-Salas, N.; Ladino-Luna, D. General properties for an Agrawal thermal engine. J. Non-Equilib. Thermodyn. 2018, 43, 131–140. [Google Scholar] [CrossRef]
- Feidt, M.; Costea, M. From finite time to finite physical dimensions thermodynamics: The Carnot engine and Onsager’s relations revisited. J. Non-Equilib. Thermodyn. 2018, 43, 151–162. [Google Scholar] [CrossRef]
- Zaeva, M.A.; Tsirlin, A.M.; Didina, O.V. Finite time thermodynamics: Realizability domain of heat to work converters. J. Non-Equilib. Thermodyn. 2019, 44, 181–191. [Google Scholar] [CrossRef]
- Schwalbe, K.; Hoffmann, K.H. Optimal control of an endoreversible solar power plant. J. Non-Equilib. Thermodyn. 2018, 43, 255–272. [Google Scholar] [CrossRef]
- Wu, Z.X.; Chen, L.G.; Feng, H.J. Thermodynamic optimization for an endoreversible Dual-Miller cycle (DMC) with finite speed of piston. Entropy 2018, 20, 165. [Google Scholar] [CrossRef] [Green Version]
- You, J.; Chen, L.G.; Wu, Z.X.; Sun, F.R. Thermodynamic performance of Dual-Miller cycle (DMC) with polytropic processes based on power output, thermal efficiency and ecological function. Sci. China Technol. Sci. 2018, 61, 453–463. [Google Scholar] [CrossRef]
- Raman, R.; Kumar, N. Performance analysis of Diesel cycle under efficient power density condition with variable specific heat of working fluid. J. Non-Equilib. Thermodyn. 2019, 44, 405–416. [Google Scholar] [CrossRef]
- Abedinnezhad, S.; Ahmadi, M.H.; Pourkiaei, S.M.; Pourfayaz, F.; Mosavi, A.; Feidt, M.; Shamshirband, S. Thermodynamic assessment and multi-objective optimization of performance of irreversible Dual-Miller cycle. Energies 2019, 12, 4000. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Ge, Y.L.; Liu, C.; Feng, H.J.; Lorenzini, G. Performance of universal reciprocating heat-engine cycle with variable specific heats ratio of working fluid. Entropy 2020, 22, 397. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Meng, F.K.; Sun, F.R. Thermodynamic analyses and optimizations for thermoelectric devices: The state of the arts. Sci. China: Technol. Sci. 2016, 59, 442–455. [Google Scholar] [CrossRef]
- Feng, Y.L.; Chen, L.G.; Meng, F.K.; Sun, F.R. Influences of external heat transfer and Thomson effect on performance of TEG-TEC combined thermoelectric device. Sci. China: Technol. Sci. 2018, 61, 1600–1610. [Google Scholar] [CrossRef]
- Li, G.; Wang, Z.C.; Wang, F.; Wang, X.Z.; Li, S.B.; Xue, M.S. Experimental and numerical study on the effect of interfacial heat transfer on performance of thermoelectric generators. Energies 2019, 12, 3797. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Meng, F.K.; Ge, Y.L.; Feng, H.J.; Xia, S.J. Performance optimization of a class of combined thermoelectric heating devices. Sci. China: Technol. Sci. 2020, 63. [Google Scholar] [CrossRef]
- Dumitrascu, G.; Feidt, M.; Popescu, A.; Grigorean, S. Endoreversible trigeneration cycle design based on finite physical dimensions thermodynamics. Energies 2019, 12, 3165. [Google Scholar]
- Feng, H.J.; Tao, G.S.; Tang, C.Q.; Ge, Y.L.; Chen, L.G.; Xia, S.J. Exergoeconomic performance optimization for a regenerative gas turbine closed-cycle heat and power cogeneration plant. Energy Rep. 2019, 5, 1525–1531. [Google Scholar] [CrossRef]
- Chen, L.G.; Yang, B.; Feng, H.J.; Ge, Y.L.; Xia, S.J. Performance optimization of an open simple-cycle gas turbine combined cooling, heating and power plant driven by basic oxygen furnace gas in China’s steelmaking plants. Energy 2020, 203, 117791. [Google Scholar] [CrossRef]
- Fontaine, K.; Yasunaga, T.; Ikegami, Y. OTEC maximum net power output using Carnot cycle and application to simplify heat exchanger selection. Entropy 2019, 21, 1143. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.X.; Feng, H.J.; Chen, L.G.; Tang, W.; Shi, J.Z.; Ge, Y.L. Constructal thermodynamic optimization for ocean thermal energy conversion system with dual-pressure organic Rankine cycle. Energy Convers. Manag. 2020, 210, 112727. [Google Scholar] [CrossRef]
- Yasunaga, T.; Ikegami, Y. Finite-time thermodynamic model for evaluating heat engines in ocean thermal energy conversion. Entropy 2020, 22, 211. [Google Scholar] [CrossRef] [Green Version]
- Feng, H.J.; Qin, W.X.; Chen, L.G.; Cai, C.G.; Ge, Y.L.; Xia, S.J. Power output, thermal efficiency and exergy-based ecological performance optimizations of an irreversible KCS-34 coupled to variable temperature heat reservoirs. Energy Convers. Manag. 2020, 205, 112424. [Google Scholar] [CrossRef]
- Chen, W.J.; Feng, H.J.; Chen, L.G.; Xia, S.J. Optimal performance characteristics of subcritical simple irreversible organic Rankine cycle. J. Therm. Sci. 2018, 27, 555–562. [Google Scholar] [CrossRef]
- Wang, S.; Zhang, W.; Feng, Y.Q.; Wang, X.; Wang, Q.; Liu, Y.Z.; Wang, Y.; Yao, L. Entropy, entransy and exergy analysis of a dual-loop organic Rankine cycle (DORC) using mixture working fluids for engine waste heat recovery. Energies 2020, 13, 1301. [Google Scholar] [CrossRef] [Green Version]
- Feng, H.J.; Chen, W.J.; Chen, L.G.; Tang, W. Power and efficiency optimizations of an irreversible regenerative organic Rankine cycle. Energy Convers. Manag. 2020, 220, 113079. [Google Scholar] [CrossRef]
- Tang, C.Q.; Feng, H.J.; Chen, L.G.; Wang, W.H. Power density analysis and multi-objective optimization for a modified endoreversible simple closed Brayton cycle with one isothermal heating process. Energy Rep. 2020, 6. in pres. [Google Scholar]
- Chen, L.G.; Feng, H.J.; Ge, Y.L. Power and efficiency optimization for open combined regenerative Brayton and inverse Brayton cycles with regeneration before the inverse cycle. Entropy 2020, 22, 677. [Google Scholar] [CrossRef]
- Tang, C.Q.; Chen, L.G.; Feng, H.J.; Wang, W.H.; Ge, Y.L. Power optimization of a closed binary Brayton cycle with isothermal heating processes and coupled to variable-temperature reservoirs. Energies 2020, 13, 3212. [Google Scholar]
- Zhu, F.L.; Chen, L.G.; Wang, W.H. Thermodynamic analysis of an irreversible Maisotsenko reciprocating Brayton cycle. Entropy 2018, 20, 167. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.G.; Shen, J.F.; Ge, Y.L.; Wu, Z.X.; Wang, W.H.; Zhu, F.L.; Feng, H.J. Power and efficiency optimization of open Maisotsenko-Brayton cycle and performance comparison with traditional open regenerated Brayton cycle. Energy Convers. Manag. 2020, 217, 113001. [Google Scholar] [CrossRef]
- Ding, Z.M.; Ge, Y.L.; Chen, L.G.; Feng, H.J.; Xia, S.J. Optimal performance regions of Feynman’s ratchet engine with different optimization criteria. J. Non-Equilib. Thermodyn. 2020, 45, 191–207. [Google Scholar] [CrossRef]
- Ding, Z.M.; Chen, L.G.; Ge, Y.L.; Xie, Z.H. Optimal performance regions of an irreversible energy selective electron heat engine with double resonance. Sci. China: Technol. Sci. 2019, 62, 397–405. [Google Scholar] [CrossRef]
- Meng, Z.W.; Chen, L.G.; Wu, F. Optimal power and efficiency of multi-stage endoreversible quantum Carnot heat engine with harmonic oscillators at the classical limit. Entropy 2020, 22, 457. [Google Scholar] [CrossRef] [Green Version]
- Rubin, M.H. Optimal configuration of a class of irreversible heat engines. Phys. Rev. A 1979, 19, 1272–1287. [Google Scholar] [CrossRef]
- Rubin, M.H. Optimal configuration of an irreversible heat engine with fixed compression ratio. Phys. Rev. A 1980, 22, 1741–1752. [Google Scholar] [CrossRef]
- Ondrechen, M.J.; Rubin, M.H.; Band, Y.B. The generalized Carnot cycles: A working fluid operating in finite-time between finite heat sources and sinks. J. Chem. Phys. 1983, 78, 4721–4727. [Google Scholar] [CrossRef]
- Chen, L.G.; Zhou, S.B.; Sun, F.R.; Wu, C. Optimal configuration and performance of heat engines with heat leak and finite heat capacity. Open Syst. Inf. Dyn. 2002, 9, 85–96. [Google Scholar] [CrossRef]
- Mozurkewich, M.; Berry, R.S. Finite-time thermodynamics: Engine performance improved by optimized piston motion. Proc. Natl. Acad. Sci. USA 1981, 78, 1986–1988. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mozurkewich, M.; Berry, R.S. Optimal paths for thermodynamic systems. The ideal Otto cycle. J. Appl. Phys. 1982, 53, 34–42. [Google Scholar] [CrossRef]
- Hoffmann, K.H.; Watowich, S.J.; Berry, R.S. Optimal paths for thermodynamic systems. The ideal Diesel cycle. J. Appl. Phys. 1985, 58, 2125–2134. [Google Scholar] [CrossRef]
- Blaudeck, P.; Hoffmann, K.H. Optimization of the power output for the compression and power stroke of the Diesel engine. In Proceedings of the International Conference ECOS’95, Istanbul, Turkey, 11–14 July 1995; Volume 2, p. 754. [Google Scholar]
- Watowich, S.J.; Hoffmann, K.H.; Berry, R.S. Intrinsically irreversible light-driven engine. J. Appl. Phys. 1985, 58, 2893–2901. [Google Scholar] [CrossRef]
- Watowich, S.J.; Hoffmann, K.H.; Berry, R.S. Optimal path for a bimolecular, light –driven engine. IL Nuovo Cimento B 1989, 104B, 131–147. [Google Scholar] [CrossRef]
- Teh, K.Y.; Miller, S.L.; Edwards, C.F. Thermodynamic requirements for maximum internal combustion engine cycle efficiency Part 1: Optimal combustion strategy. Int. J. Engine Res. 2008, 9, 449–465. [Google Scholar] [CrossRef]
- Teh, K.Y.; Miller, S.L.; Edwards, C.F. Thermodynamic requirements for maximum internal combustion engine cycle efficiency Part 2: Work extraction and reactant preparation strategies. Int. J. Engine Res. 2008, 9, 467–481. [Google Scholar] [CrossRef]
- Bi, Y.H.; Guo, T.; Zhang, L.; Chen, L.G.; Sun, F.R. Entropy generation minimization for charging and discharging processes in a gas hydrate cool storage system. Appl. Energy 2010, 87, 1149–1157. [Google Scholar] [CrossRef]
- Band, Y.B.; Kafri, O.; Salamon, P. Maximum work production from a heated gas in a cylinder with piston. Chem. Phys. Lett. 1980, 72, 127–130. [Google Scholar] [CrossRef]
- Band, Y.B.; Kafri, O.; Salamon, P. Finite time thermodynamics: Optimal expansion of a heated working fluid. J. Appl. Phys. 1982, 53, 8–28. [Google Scholar] [CrossRef]
- Band, Y.B.; Kafri, O.; Salamon, P. Optimization of a model external combustion engine. J. Appl. Phys. 1982, 53, 29–33. [Google Scholar] [CrossRef]
- Aizenbud, B.M.; Band, Y.B. Power considerations in the operation of a piston fitted inside a cylinder containing a dynamically heated working fluid. J. Appl. Phys. 1981, 52, 3742–3744. [Google Scholar] [CrossRef]
- Salamon, P.; Band, Y.B.; Kafri, O. Maximum power from a cycling working fluid. J. Appl. Phys. 1982, 53, 197–202. [Google Scholar] [CrossRef]
- Aizenbud, B.M.; Band, Y.B.; Kafri, O. Optimization of a model internal combustion engine. J. Appl. Phys. 1982, 53, 1277–1282. [Google Scholar] [CrossRef]
- Song, H.J.; Chen, L.G.; Li, J.; Sun, F.R.; Wu, C. Optimal configuration of a class of endoreversible heat engines with linear phenomenological heat transfer law []. J. Appl. Phys. 2006, 100, 124907. [Google Scholar] [CrossRef]
- Song, H.J.; Chen, L.G.; Sun, F.R. Endoreversible heat engines for maximum power output with fixed duration and radiative heat-transfer law. Appl. Energy 2007, 84, 374–388. [Google Scholar] [CrossRef]
- Song, H.J.; Chen, L.G.; Sun, F.R.; Wang, S.B. Configuration of heat engines for maximum power output with fixed compression ratio and generalized radiative heat transfer law. J. Non-Equilib. Thermodyn. 2008, 33, 275–295. [Google Scholar] [CrossRef]
- Chen, L.G.; Song, H.J.; Sun, F.R.; Wang, S.B. Optimal configuration of heat engines for maximum efficiency with generalized radiative heat transfer law. Rev. Mexi. Fis. 2009, 55, 55–67. [Google Scholar]
- Chen, L.G.; Song, H.J.; Sun, F.R.; Wu, C. Optimal configuration of heat engines for maximum power with generalized radiative heat transfer law. Int. J. Ambient Energy 2009, 30, 137–160. [Google Scholar] [CrossRef]
- Chen, L.G.; Song, H.B.; Sun, F.R. Endoreversible radiative heat engines for maximum efficiency. Appl. Math. Modell. 2010, 34, 1710–1720. [Google Scholar] [CrossRef]
- Yan, Z.J.; Chen, J.C. Optimal performance of a generalized Carnot cycle for another linear heat transfer law. J. Chem. Phys. 1990, 92, 1994–1998. [Google Scholar] [CrossRef]
- Xiong, G.; Chen, J.C.; Yan, Z.J. The effect of heat transfer law on the performance of a generalized Carnot cycle. J. Xiamen Univ. 1989, 28, 489–494. (In Chinese) [Google Scholar]
- Chen, L.G.; Zhu, X.; Sun, F.R.; Wu, C. Optimal configurations and performance for a generalized Carnot cycle assuming the heat transfer law . Appl. Energy 2004, 78, 305–313. [Google Scholar] [CrossRef]
- Li, J.; Chen, L.G.; Sun, F.R. Optimal configuration for a finite high-temperature source heat engine cycle with complex heat transfer law. Sci. China Ser. G. 2009, 52, 587–592. [Google Scholar] [CrossRef]
- Burzler, M.J.; Hoffmann, K.H. Optimal piston paths for Diesel engines. In Thermodynamics of Energy Conversion and Transport; Sienuitycz, S., de Vos, A., Eds.; Springer: New York, NY, USA, 2000; Chapter 7. [Google Scholar]
- Burzler, J.M. Performance Optima for Endoreversible Systems. Ph.D. Thesis, University of Chemnitz, Chemnitz, Germany, 2002. [Google Scholar]
- Xia, S.J.; Chen, L.G.; Sun, F.R. Optimal path of piston motion for Otto cycle with linear phenomenological heat transfer law. Sci. China Ser. G 2009, 52, 708–719. [Google Scholar] [CrossRef]
- Ge, Y.L.; Chen, L.G.; Sun, F.R. Optimal paths of piston motion of irreversible Otto cycle heat engines for minimum entropy generation. Sci. China, Phys. Mech. Astron. 2010, 40, 1115–1129. [Google Scholar]
- Ma, K.; Chen, L.G.; Sun, F.R. Optimal paths for a light-driven engine with linear phenomenological heat transfer law. Sci. China, Chem. 2010, 53, 917–926. [Google Scholar] [CrossRef]
- Chen, L.G.; Sun, F.R.; Wu, C. Optimal expansion of a heated working fluid with phenomenological heat transfer. Energy Convers. Mgmt. 1998, 39, 149–156. [Google Scholar] [CrossRef]
- Song, H.J.; Chen, L.G.; Sun, F.R. Optimal expansion of a heated working fluid for maximum work output with generalized radiative heat transfer law. J. Appl. Phys. 2007, 102, 094901. [Google Scholar] [CrossRef]
- Chen, L.G.; Song, H.J.; Sun, F.R.; Wu, C. Optimal expansion of a heated working fluid with convective- radiative heat transfer law. Int. J. Ambient Energy 2010, 31, 81–90. [Google Scholar] [CrossRef]
- Chen, L.G.; Ma, K.; Ge, Y.L.; Feng, H.J. Re-optimization of expansion work of a heated working fluid with generalized radiative heat transfer law. Entropy 2020, 22. in press. [Google Scholar]
- Song, H.J.; Chen, L.G.; Sun, F.R. Optimization of a model external combustion engine with linear phenomenological heat transfer law. J. Energy Inst. 2009, 82, 180–183. [Google Scholar] [CrossRef]
- Chen, L.G.; Song, H.J.; Sun, F.R.; Wu, C. Optimization of a model internal combustion engine with linear phenomenological heat transfer law. Int. J. Ambient Energy 2010, 31, 13–22. [Google Scholar] [CrossRef]
- Gutowicz-Krusin, D.; Procaccia, J.; Ross, J. On the efficiency of rate processes: Power and efficiency of heat engines. J. Chem. Phys. 1978, 69, 3898–3906. [Google Scholar] [CrossRef]
- Angulo-Brown, F.; Paez-Hernandez, R. Endoreversible thermal cycle with a nonlinear heat transfer law. J. Appl. Phys. 1993, 74, 2216–2219. [Google Scholar] [CrossRef]
- Chen, L.G.; Sun, F.R.; Wu, C. Influence of heat transfer law on the performance of a Carnot engine. Appl. Thermal Eng. 1997, 17, 277–282. [Google Scholar] [CrossRef]
- Zhou, S.B.; Chen, L.G.; Sun, F.R. Optimal performance of a generalized irreversible Carnot engine. Appl. Energy 2005, 81, 376–387. [Google Scholar] [CrossRef]
- Huleihil, M.; Andresen, B. Convective heat transfer law for an endoreversible engine. J. Appl. Phys. 2006, 100, 014911. [Google Scholar] [CrossRef] [Green Version]
- O’Sullivan, C.T. Newton’s law of cooling-A critical assessment. Am. J. Phys. 1990, 58, 956–960. [Google Scholar] [CrossRef]

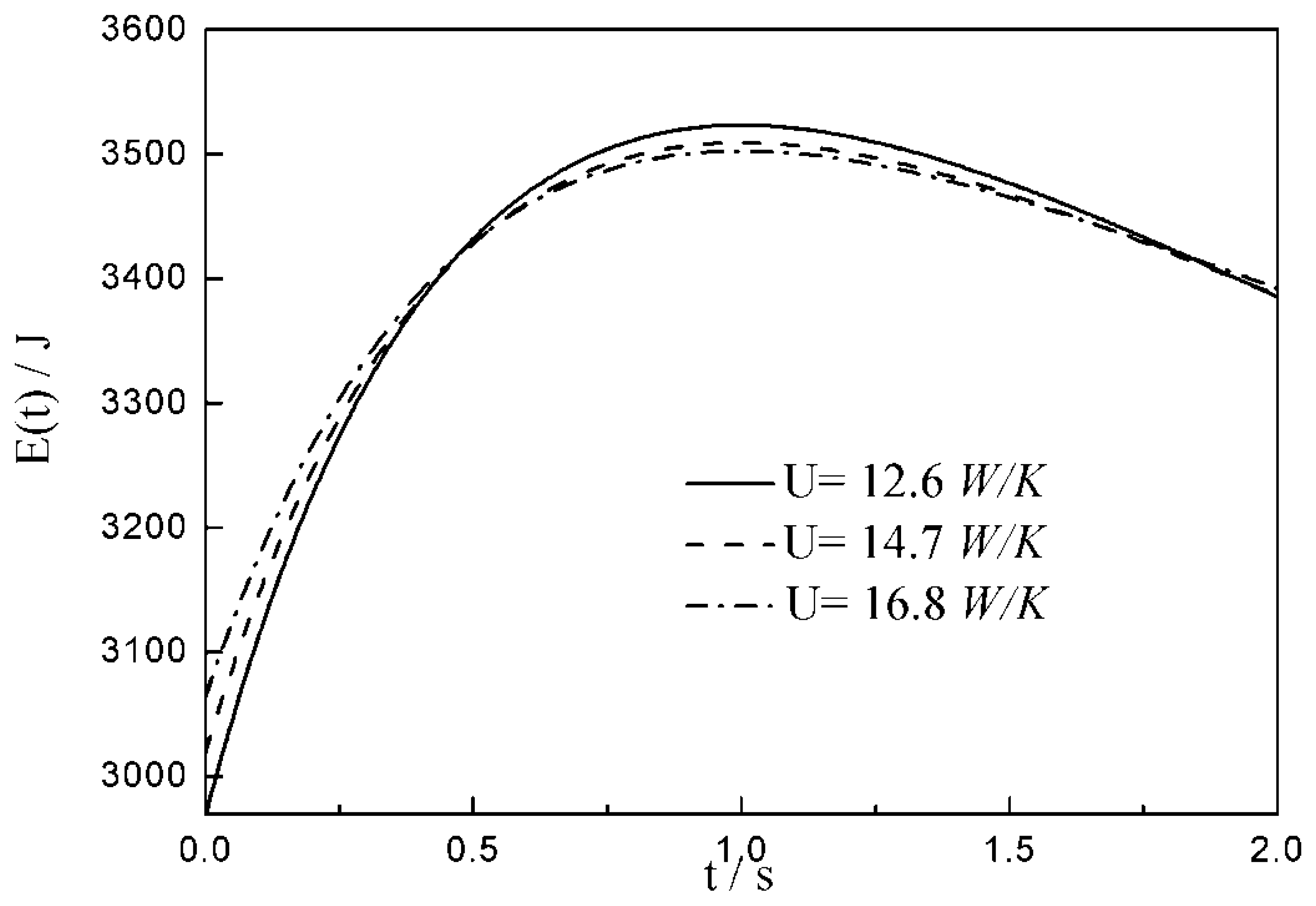
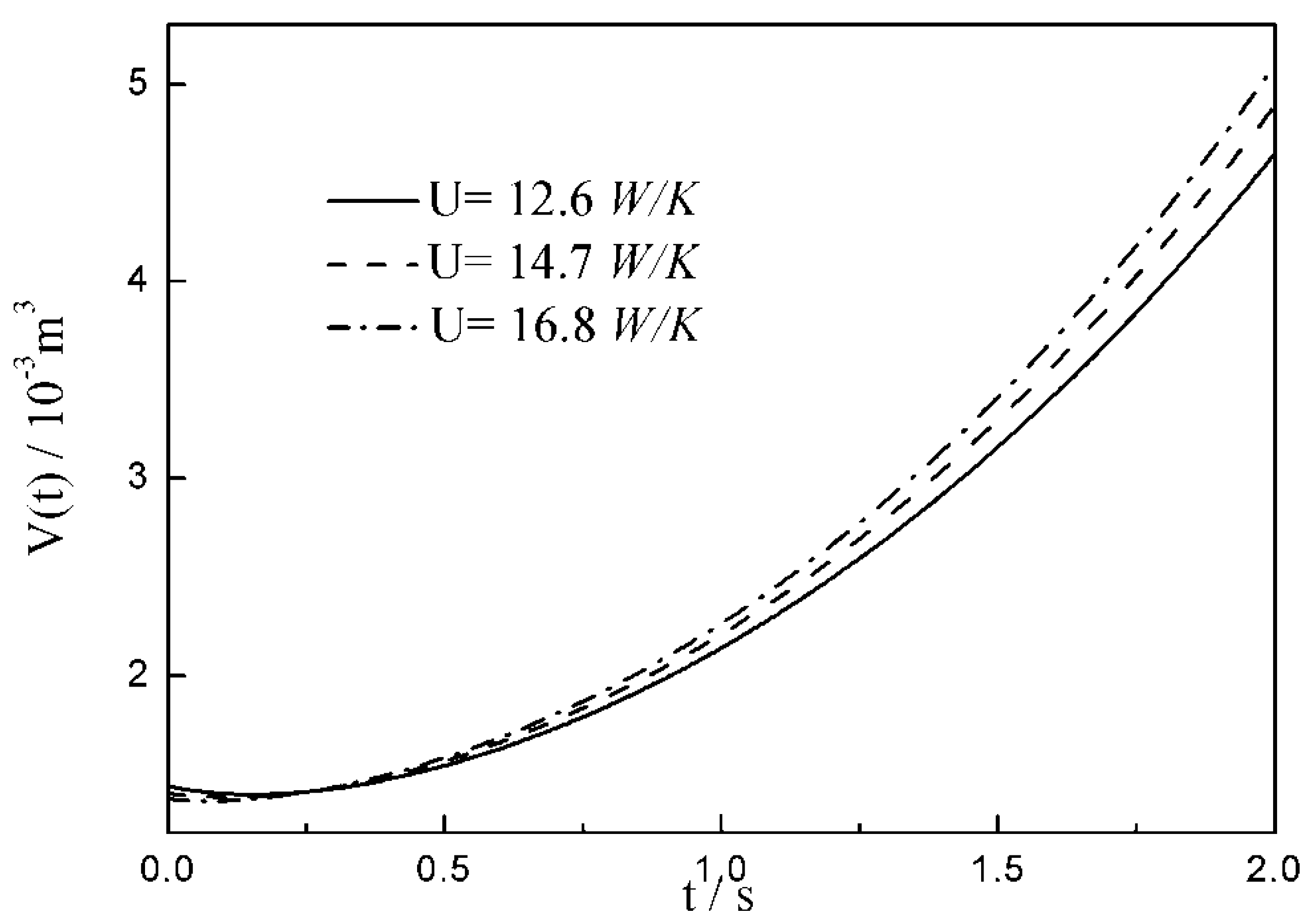
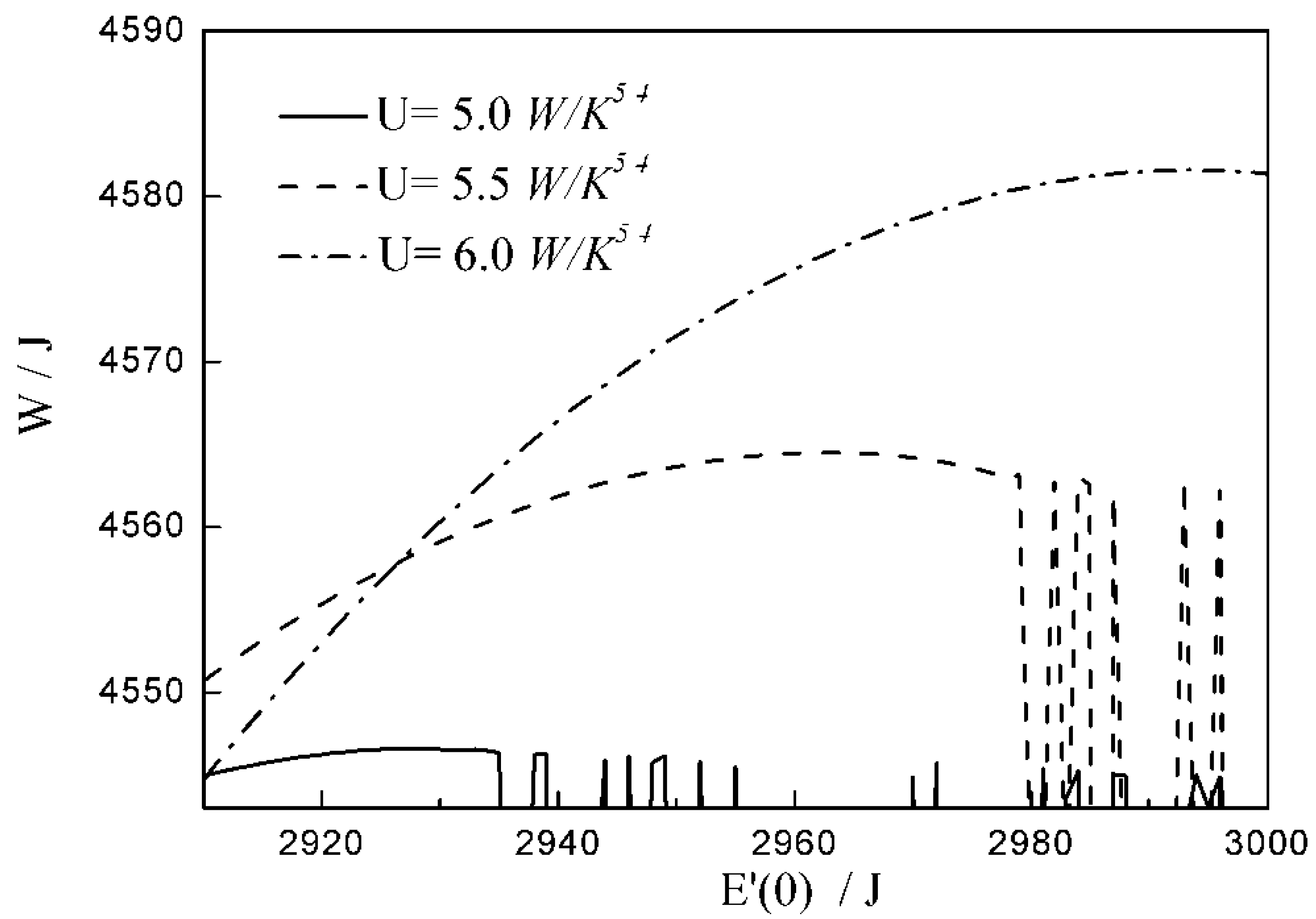
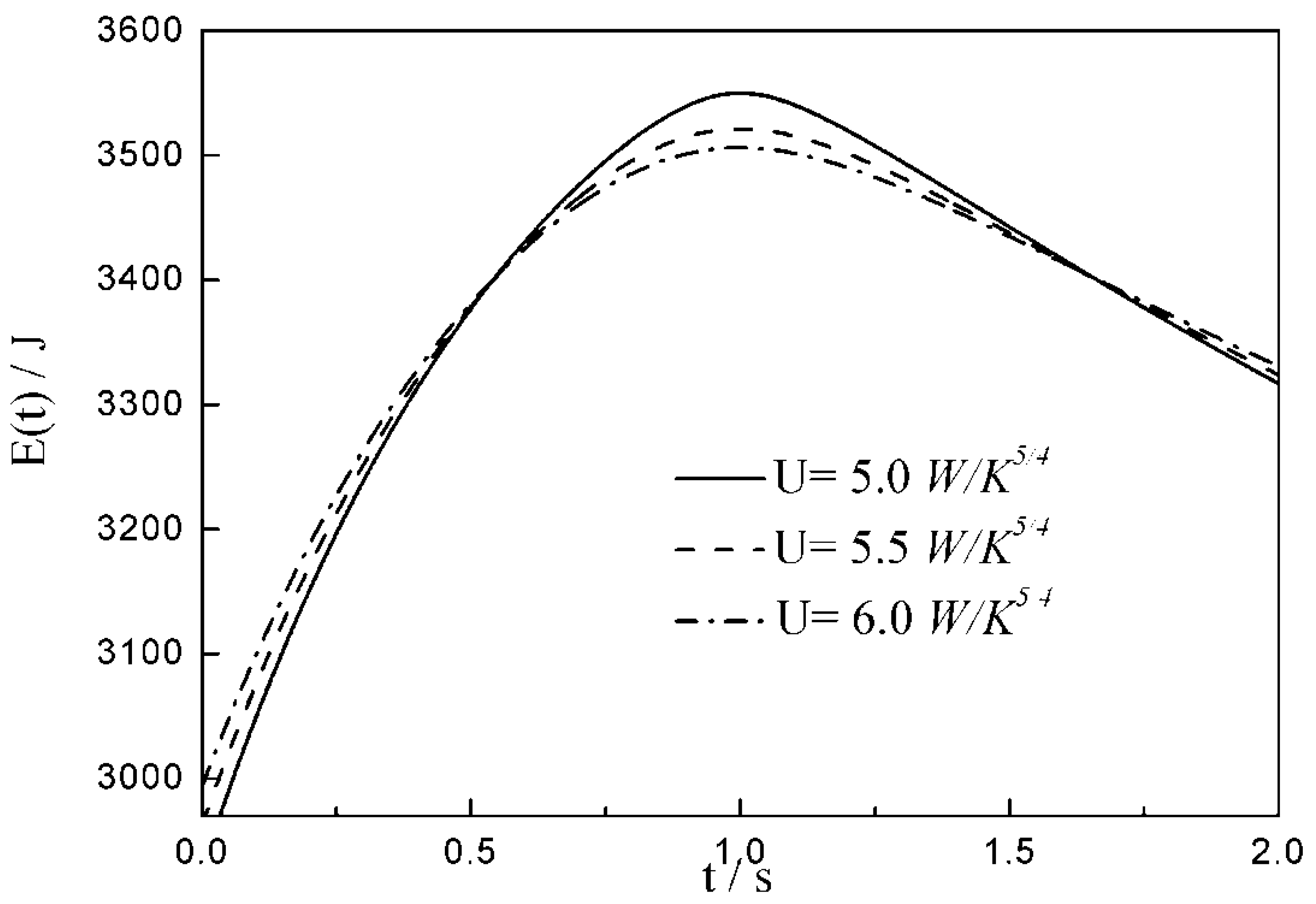


| 12.6 | 14.7 | 16.8 | |
| 1.4370 | 1.4005 | 1.3702 | |
| 2968.3 | 3019.7 | 3064.2 | |
| 4.6451 | 4.8877 | 5.0944 | |
| 3385.4 | 3386.6 | 3392.2 | |
| 2356.2 | 2438.43 | 2510.8 | |
| 4562.5 | 4593.6 | 4622.5 | |
| 0.5942 | 0.5982 | 0.6020 |
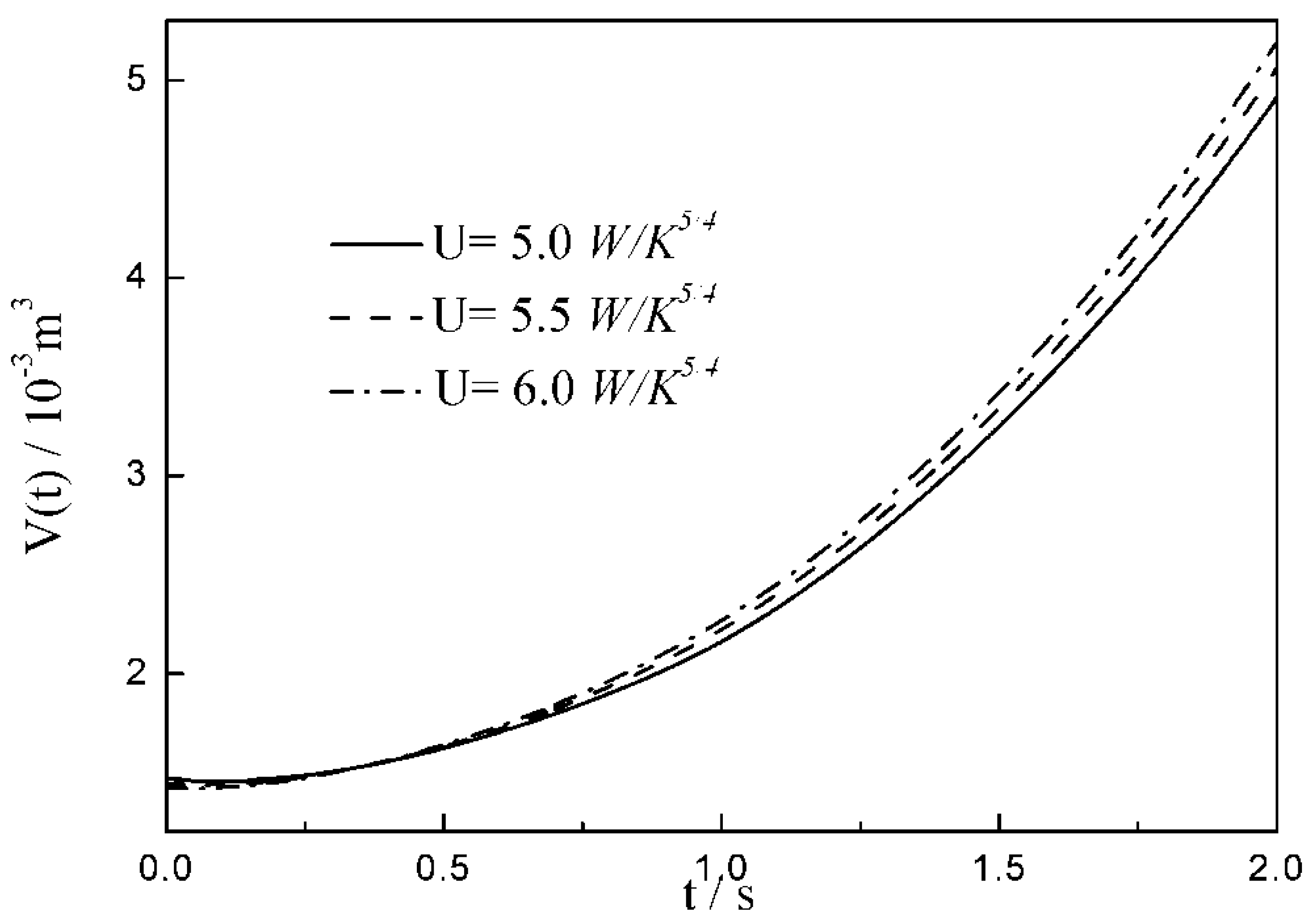
| 5.0 | 5.5 | 6.0 | |
| 1.4670 | 1.4411 | 1.4189 | |
| 2927.78 | 2962.71 | 2993.55 | |
| 4.9076 | 5.0552 | 5.1856 | |
| 3317.32 | 3324.24 | 3331.11 | |
| 2395.02 | 2447.90 | 2494.78 | |
| 4546.61 | 4564.50 | 4581.58 | |
| 0.5921 | 0.5945 | 0.5967 |
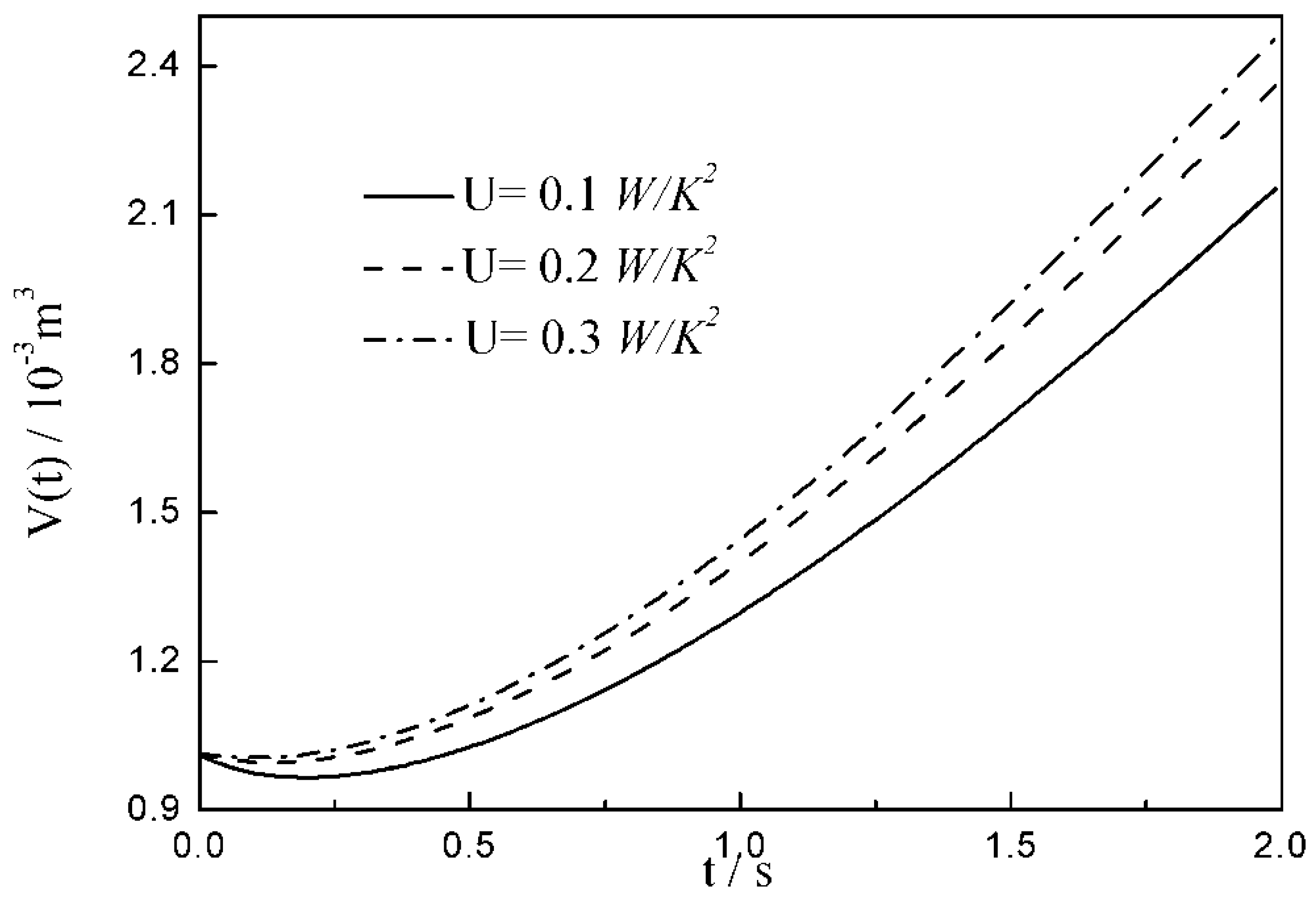
| 0.1 | 0.2 | 0.3 | |
| 1.0108 | 1.0134 | 1.0143 | |
| 3753.00 | 3746.61 | 3744.34 | |
| 2.1527 | 2.3598 | 2.4566 | |
| 4033.61 | 3910.56 | 3861.22 | |
| 1681.22 | 1732.87 | 1757.48 | |
| 4461.83 | 4450.59 | 4447.02 | |
| 0.5811 | 0.5796 | 0.5792 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Ma, K.; Feng, H.; Ge, Y. Optimal Configuration of a Gas Expansion Process in a Piston-Type Cylinder with Generalized Convective Heat Transfer Law. Energies 2020, 13, 3229. https://doi.org/10.3390/en13123229
Chen L, Ma K, Feng H, Ge Y. Optimal Configuration of a Gas Expansion Process in a Piston-Type Cylinder with Generalized Convective Heat Transfer Law. Energies. 2020; 13(12):3229. https://doi.org/10.3390/en13123229
Chicago/Turabian StyleChen, Lingen, Kang Ma, Huijun Feng, and Yanlin Ge. 2020. "Optimal Configuration of a Gas Expansion Process in a Piston-Type Cylinder with Generalized Convective Heat Transfer Law" Energies 13, no. 12: 3229. https://doi.org/10.3390/en13123229





