Mathematical Modeling and Performance Analysis of a New Hybrid Solar Dryer of Lemon Slices for Controlling Drying Temperature
Abstract
1. Introduction
2. Materials and Methods
2.1. Description of System
2.2. Sample Preparation
2.3. Experimental Procedure
2.4. Uncertainty Analysis
3. Performance Analysis
3.1. Drying Analysis
3.2. Energy Analysis
3.3. Exergy Analysis
- The flow of drying air can be assumed to be steady.
- The exergy loss of the product was neglected due to the type of chamber.
- The variation of drying air moisture content was neglected [22].
- The change of pressure in the inlet and outlet of drying chamber was neglected.
3.4. Economic Analysis
3.5. Environmental Analysis
4. Results and Discussion
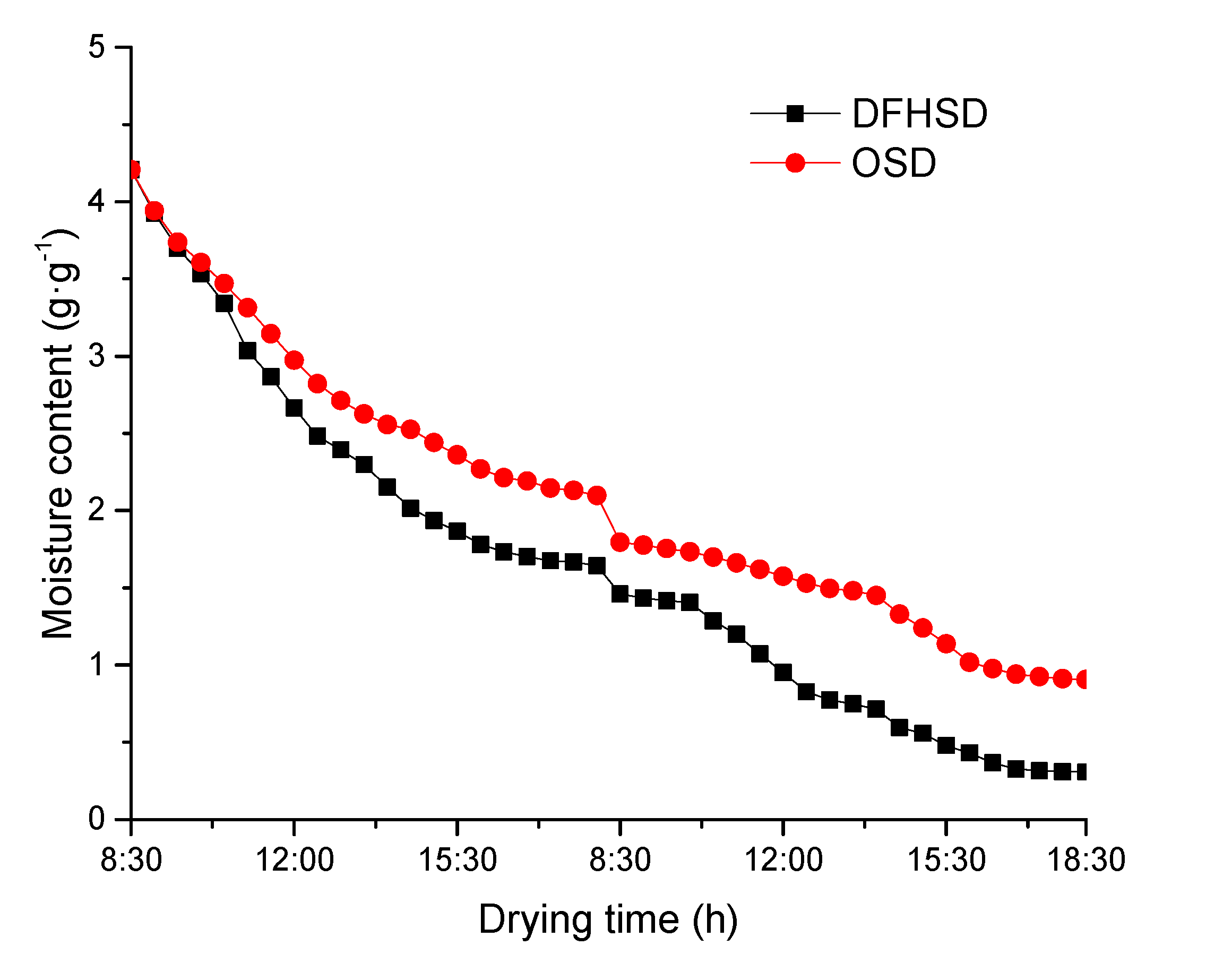
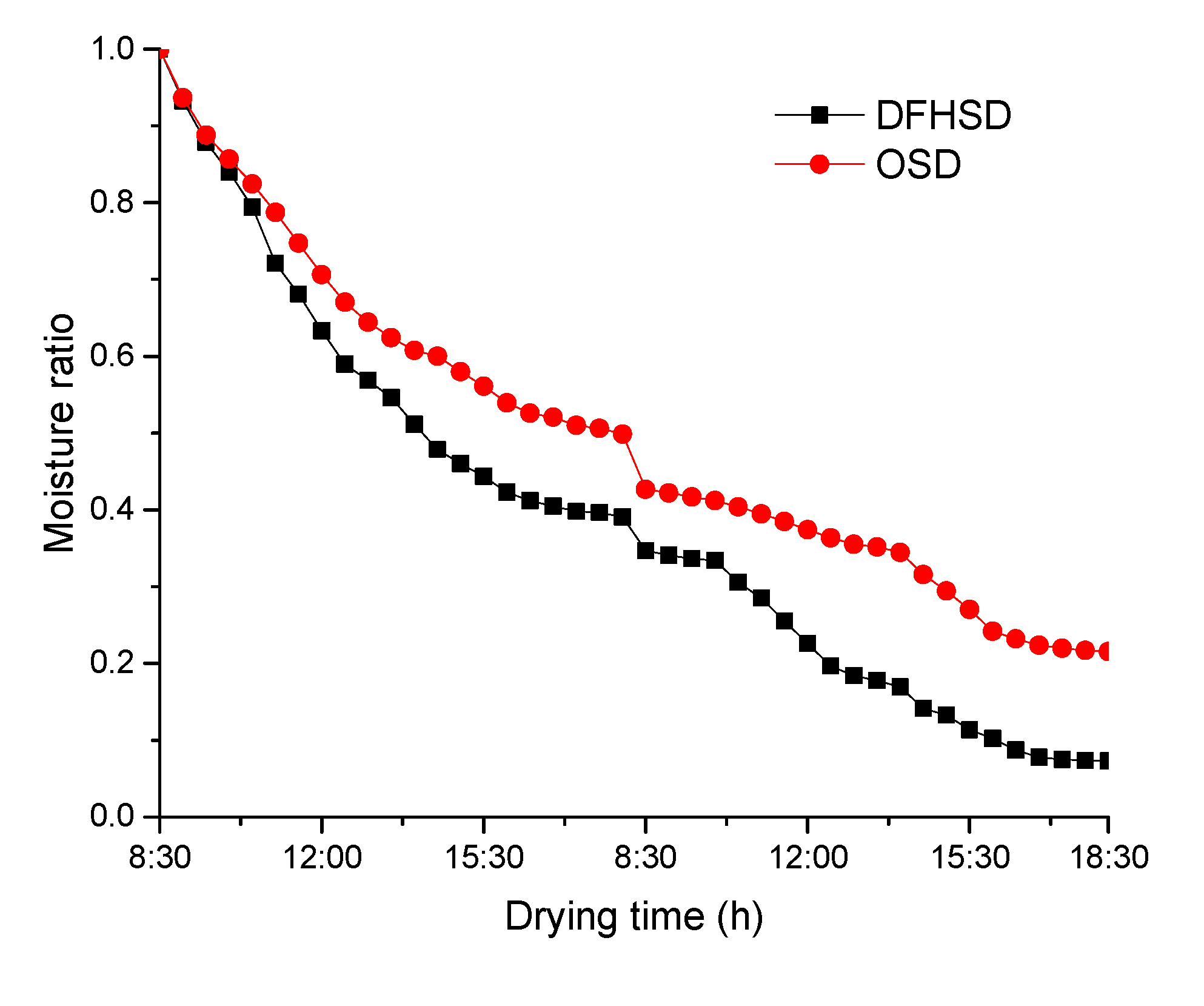
4.1. Drying Characteristics
4.1.1. Drying Kinetics
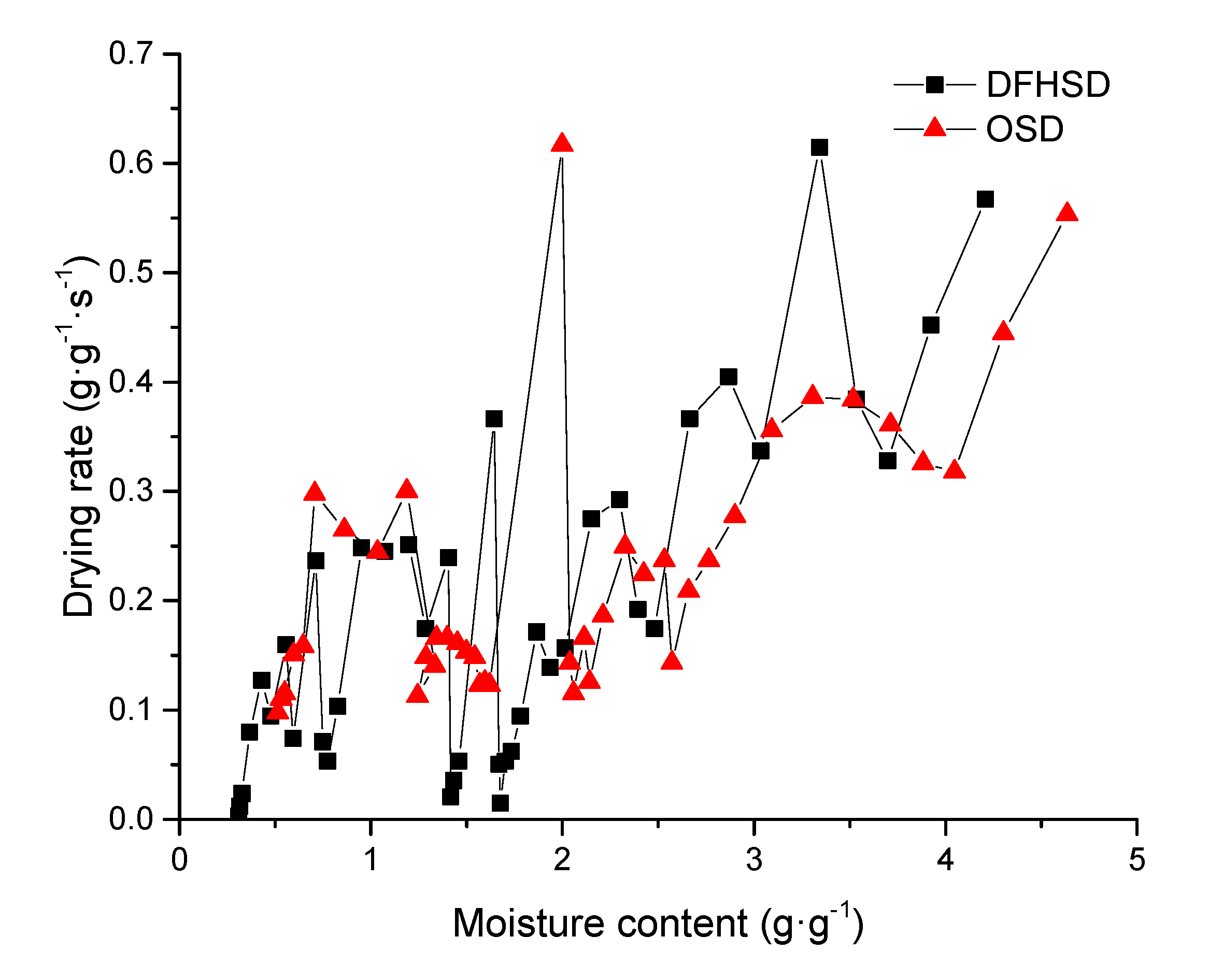
4.1.2. Drying Rates Curves
4.1.3. Fitting of Drying Model
4.2. Energy and Exergy Analysis
4.3. Economic and Environment Analysis
4.4. Analysis of Hybrid Solar Dryer Operation Mode
5. Conclusions
- Under the same drying time condition, the drying capacity of the hybrid solar dryer is better than OSD. Meanwhile, it was found that the Two term model and Wang and Singh model were the best suitable models to describe the lemon slices drying characteristics for hybrid solar dryer. The Two term model was only the best model for OSD.
- The efficiency range of DF-FPSC was from 2% to 69.52% with an average of 44.6%. The exergy flow of the drying cabinet inlet and outlet ranged from 6.46 W to 359.34 W and 0.68 W to 149.42 W, respectively. The exergy efficiency range of the drying cabinet was from 39.38% to 71.7%, respectively. The improvement potential range of the drying cabinet was from 0.65 W to 85.56 W. It can be concluded that the outlet of the drying chamber lost considerable energy.
- It was found that the pay-back period was 3.63 years. The CO2 reduction per batch based on the results of this experiment was 21.79 kg/batch. The revenue of CO2 abatement per batch was 1.61 RMB/batch. The system has good economic and environmental benefits.
- During the period of hybrid solar dryer operation, the maximum drying air temperature can be controlled under 60.2 °C in the cabinet. The start-up time of the water pump was 12:51, 12:19, respectively, for two days. This was because the dry cabinet air temperature was higher than the suitable product drying temperature. The experimental results showed the feasibility and validity of the proposed hybrid solar dryer with DF-FPSC.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Nomenclature | |
| Wd | Final mass of products (g) |
| Md,0 | Initial moisture content on dry basis (g g−1) |
| w | Uncertainty |
| IP | Improvement potential (W) |
| M | Moisture content (g g−1) |
| i | Year |
| Wt | Evaporate quantity of water at t time (g) |
| Me | Equilibrium moisture content (g g−1) |
| MR | Moisture ratio |
| R2 | Regression coefficient |
| Ex | Exergy (W) |
| SEC | Specify energy consumption (kW·h/kg) |
| DR | Drying rate of products (g g−1 s−1) |
| Ct | Capital cost (RMB) |
| RMSE | Root mean square error |
| a,b,k,n,k0,k1 | Constant used in models |
| m | Mass flow (m3/h) |
| Qu | Useful energy (J) |
| I(t) | Solar radiation intensity (W/m2) |
| Ac | Collector area (m2) |
| Mw,0 | Initial moisture content on wet basis (g g−1) |
| Cm | Material cost (RMB) |
| Cc | Construction cost (RMB) |
| Ca | Annual cost (RMB) |
| t | Time (s) |
| PBP | Payback period (year) |
| P | Cost per unit of drying materials (RMB/kg) |
| R | CO2 reductions (kg CO2) |
| EM | CO2 emission (kg CO2) |
| CP | International carbon price (RMB/ton) |
| Y | Renue of CO2 abatement (RMB) |
| Cp | Specific heat capacity (kJ/(kg °C)) |
| T | Temperature (°C) |
| Greek symbols | |
| δ | Saving-time percentage of drying |
| Average CO2 equivalent intensity for electricity generation from coal (kg CO2/kW·h) | |
| Absorptivity | |
| Transmissivity | |
| Relative humidity (%) | |
| η | Thermal efficiency of collector |
| Subscripts and abbreviations | |
| 0 | Initial time |
| a | Air |
| mp | Moisture of product |
| exp | Experimental |
| pre | Predicted |
| sat | Saturated |
| w | Water |
| i | Number |
| ou | Outlet |
| in | Inlet |
| ev | Evaporate |
| th | Theoretical thermal efficiency |
| dc | Drying chamber |
| ref | Reference value |
| c | Collector |
| f | Final time |
References
- Pirasteh, G.; Saidur, R.; Rahman, S.M.A.; Rahim, N.A. A review on development of solar drying applications. Renew. Sustain. Energy Rev. 2014, 31, 133–148. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Bakhtyar, B.; Gabbasa, M.; Othman, M.Y.; Ruslan, M.H. Review of solar drying systems with air based solar collectors in Malaysia. Renew. Sustain. Energy Rev. 2015, 51, 1191–1204. [Google Scholar] [CrossRef]
- Mustayen, A.G.M.B.; Mekhilef, S.; Saidur, R. Performance study of different solar dryers: A review. Renew. Sustain. Energy Rev. 2014, 34, 463–470. [Google Scholar] [CrossRef]
- Goh, L.J.; Othman, M.Y.; Mat, S.; Ruslan, H.; Sopian, K. Review of heat pump systems for drying application. Renew. Sustain. Energy Rev. 2011, 15, 4788–4796. [Google Scholar] [CrossRef]
- Fadhel, M.I.; Sopian, K.; Daud, W.R.W.; Alghoul, M.A. Review on advanced of solar assisted chemical heat pump dryer for agriculture produce. Renew. Sustain. Energy Rev. 2011, 15, 1152–1168. [Google Scholar] [CrossRef]
- El-Sebaii, A.A.; Shalaby, S.M. Solar drying of agricultural products: A review. Renew. Sustain. Energy Rev. 2012, 16, 37–43. [Google Scholar] [CrossRef]
- Kumar, M.; Sansaniwal, S.K.; Khatak, P. Progress in solar dryers for drying various commodities. Renew. Sustain. Energy Rev. 2016, 55, 346–360. [Google Scholar] [CrossRef]
- Banout, J.; Ehl, P.; Havlik, J.; Lojka, B.; Polesny, Z.; Verner, V. Design and performance evaluation of a Double-pass solar drier for drying of red chilli (Capsicum annum L.). Sol. Energy 2011, 85, 506–515. [Google Scholar] [CrossRef]
- Tiwari, S.; Tiwari, G.N.; Al-Helal, I.M. Performance analysis of photovoltaic-thermal (PVT) mixed mode greenhouse solar dryer. Sol. Energy 2016, 133, 421–428. [Google Scholar] [CrossRef]
- Elkhadraoui, A.; Kooli, S.; Hamdi, I.; Farhat, A. Experimental investigation and economic evaluation of a new mixed-mode solar greenhouse dryer for drying of red pepper and grape. Renew. Energy 2015, 77, 1–8. [Google Scholar] [CrossRef]
- Kabeel, A.E.; Abdelgaied, M. Performance of novel solar dryer. Process Saf. Environ. 2016, 102, 183–189. [Google Scholar] [CrossRef]
- Janjai, S.; Lamlert, N.; Intawee, P.; Mahayothee, B.; Bala, B.K.; Nagle, M.; Müller, J. Experimental and simulated performance of a PV-ventilated solar greenhouse dryer for drying of peeled longan and banana. Sol. Energy 2009, 83, 1550–1565. [Google Scholar] [CrossRef]
- Aktaş, M.; Şevik, S.; Amini, A.; Khanlari, A. Analysis of drying of melon in a solar-heat recovery assisted infrared dryer. Sol. Energy 2016, 137, 500–515. [Google Scholar] [CrossRef]
- Sonthikun, S.; Chairat, P.; Fardsin, K.; Kirirat, P.; Kumar, A.; Tekasakul, P. Computational fluid dynamic analysis of innovative design of solar-biomass hybrid dryer: An experimental validation. Renew. Energy 2016, 92, 185–191. [Google Scholar] [CrossRef]
- Can, A. Drying kinetics of pumpkinseeds. Int. J. Energy Res. 2000, 24, 965–975. [Google Scholar] [CrossRef]
- Gulcimen, F.; Karakaya, H.; Durmus, A. Drying of sweet basil with solar air collectors. Renew. Energy 2016, 93, 77–86. [Google Scholar] [CrossRef]
- Ali, I.; Abdelkader, L.; El Houssayne, B.; Mohamed, K.; El Khadir, L. Solar convective drying in thin layers and modeling of municipal waste at three temperatures. Appl. Therm. Eng. 2016, 108, 41–47. [Google Scholar] [CrossRef]
- Dejchanchaiwong, R.; Arkasuwan, A.; Kumar, A.; Tekasakul, P. Mathematical modeling and performance investigation of mixed-mode and indirect solar dryers for natural rubber sheet drying. Energy Sustain. Dev. 2016, 34, 44–53. [Google Scholar] [CrossRef]
- Kooli, S.; Fadhel, A.; Farhat, A.; Belghith, A. Drying of red pepper in open sun and greenhouse conditions. Mathematical modeling and experimental validation. J. Food Eng. 2007, 79, 1094–1103. [Google Scholar] [CrossRef]
- Bennamoun, L.; Belhamri, A. Numerical simulation of drying under variable external conditions: Application to solar drying of seedless grapes. J. Food Eng. 2006, 76, 179–187. [Google Scholar] [CrossRef]
- El-Sebaii, A.A.; Shalaby, S.M. Experimental investigation of an indirect-mode forced convection solar dryer for drying thymus and mint. Energy Convers. Manag. 2013, 74, 109–116. [Google Scholar] [CrossRef]
- Rabha, D.K.; Muthukumar, P.; Somayaji, C. Energy and exergy analyses of the solar drying processes of ghost chilli pepper and ginger. Renew. Energy 2017, 105, 764–773. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Othman, M.Y.; Ruslan, M.H. Energy and exergy analyses of solar drying system of red seaweed. Energy Build. 2014, 68, 121–129. [Google Scholar] [CrossRef]
- Akbulut, A.; Durmuş, A. Energy and exergy analyses of thin layer drying of mulberry in a forced solar dryer. Energy 2010, 35, 1754–1763. [Google Scholar] [CrossRef]
- Midilli, A.; Kucuk, H. Energy and exergy analyses of solar drying process of pistachio. Energy 2003, 28, 539–556. [Google Scholar] [CrossRef]
- Nayak, S.; Tiwari, G.N. Energy and exergy analysis of photovoltaic/thermal integrated with a solar greenhouse. Energy Build. 2008, 40, 2015–2021. [Google Scholar] [CrossRef]
- Sarhaddi, F.; Farahat, S.; Ajam, H.; Behzadmehr, A. Exergetic performance assessment of a solar photovoltaic thermal (PV/T) air collector. Energy Build. 2010, 42, 2184–2199. [Google Scholar] [CrossRef]
- Prakash, O.; Kumar, A. Environomical analysis and mathematical modelling for tomato flakes drying in a modified greenhouse dryer under active mode. Int. J. Food Eng. 2014, 10, 669–681. [Google Scholar] [CrossRef]
- Chen, H.H.; Hernandez, C.E.; Huang, T.C. A study of the drying effect on lemon slices using a closed-type solar dryer. Sol. Energy 2005, 78, 97–103. [Google Scholar] [CrossRef]
- Wang, J.; Law, C.L.; Nema, P.K.; Zhao, J.H.; Liu, Z.L.; Deng, L.Z.; Gao, Z.J.; Xiao, H.W. Pulsed vacuum drying enhances drying kinetics and quality of lemon slices. J. Food Eng. 2018, 224, 129–138. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Ruslan, M.H.; Alghoul, M.A.; Sulaiman, M.Y. Review of solar dryers for agricultural and marine products. Renew. Sustain. Energy Rev. 2010, 14, 1–30. [Google Scholar] [CrossRef]
- Hao, W.; Lu, Y.; Lai, Y.; Yu, H.; Lyu, M. Research on operation strategy and performance prediction of flat plate solar collector with dual-function for drying agricultural products. Renew. Energy 2018, 127, 685–696. [Google Scholar] [CrossRef]
- El-Sebaii, A.A.; Aboul-Enein, S.; Ramadan, M.R.I.; El-Gohary, H.G. Empirical correlations for drying kinetics of some fruits and vegetables. Energy 2002, 27, 845–859. [Google Scholar] [CrossRef]
- Jayas, D.S.; Cenkowski, S.; Pabis, S.; Muir, W.E. Review of thin-layer drying and wetting equations. Dry Technol. 1991, 9, 551–588. [Google Scholar] [CrossRef]
- Page, G.E. Factors Influencing the Maximum Rates of Air Drying Shelled Corn in Thin Layers. Master’s Thesis, Purdue University, Purdue, IN, USA, 1949. [Google Scholar]
- Henderson, S.M.; Pabis, S. Grain drying theory. J. Agric. Eng. Res. 1961, 6, 169–174. [Google Scholar]
- Yagcioglu, A.; Degirmencioglu, A.; Cagatay, F. Drying characteristic of laurel leaves under different conditions. In Proceedings of the 7th International Congress on Agricultural Mechanization and Energy, Adana, Turkey, 26–27 May 1999; pp. 565–569. [Google Scholar]
- Henderson, S.M. Progress in developing the thin layer drying equation. Trans. ASAE 1974, 17, 1167–1168. [Google Scholar] [CrossRef]
- Wang, C.Y.; Singh, R.P. A Single Layer Drying Equation for Rough Rice; ASAE: St. Joseph, MI, USA, 1978; pp. 78–3001. [Google Scholar]
- White, G.M.; Bridges, T.C.; Loewer, O.J.; Ross, I.J. Seed Coat Damage in Thin Layer Drying of Soybeans as Affected by Drying Conditions; ASAE: St. Joseph, MI, USA, 1978; p. 3052. [Google Scholar]
- Thompson, T.L. Predicted Performances and Optimal Designs of Convection Grain Dryers. Ph.D. Thesis, Purdue University, Lafayette, IN, USA, 1967. [Google Scholar]
- Audsley, E.W.J. The annual cost of machinery calculated using actual cash flows. J. Agric. Eng. Res. 1978, 23, 189–201. [Google Scholar] [CrossRef]
- Tripathi, R.; Tiwari, G.N.; Dwivedi, V.K. Overall energy, exergy and carbon credit analysis of N partially covered Photovoltaic Thermal (PVT) concentrating collector connected in series. Sol. Energy 2016, 136, 260–267. [Google Scholar] [CrossRef]
- Mghazli, S.; Ouhammou, M.; Hidar, N.; Lahnine, L.; Idlimam, A.; Mahrouz, M. Drying characteristics and kinetics solar drying of Moroccan rosemary leaves. Renew. Energy 2017, 108, 303–310. [Google Scholar] [CrossRef]
- De Lima, A.G.B.; Queiroz, M.R.; Nebra, S.A. Simultaneous moisture transport and shrinkage during drying of solids with ellipsoidal configuration. Chem. Eng. J. 2002, 86, 85–93. [Google Scholar] [CrossRef]
- Kaewkiew, J.; Nabnean, S.; Janjai, S. Experimental investigation of the performance of a large-scale greenhouse type solar dryer for drying chilli in Thailand. Procedia Eng. 2012, 32, 433–439. [Google Scholar] [CrossRef]
- Hamdi, I.; Elkhadraoui, A.; Kooli, S.; Farhat, A.; Guizani, A. Drying of red pepper slices in a solar greenhouse dryer and under open sun: Experimental and mathematical investigations. Innov. Food Sci. Emerg. 2019, 52, 262–270. [Google Scholar]
- Argyropoulos, D.; Müllera, J. Effect of Convective Drying on Quality of Lemon Balm (Melissa Officinalis L.). Procedia Food Sci. 2011, 1, 1932–1939. [Google Scholar] [CrossRef]
- Boulemtafes-Boukadoum, A.; Benzaoui, A. Energy and exergy analysis of solar drying process of Mint. Energy Procedia 2011, 6, 583–591. [Google Scholar] [CrossRef]
- Sami, S.; Etesami, N.; Rahimi, A. Energy and exergy analysis of an indirect solar cabinet dryer based on mathematical modeling results. Energy 2011, 36, 2847–2855. [Google Scholar] [CrossRef]
- Kesavan, S.; Arjunan, T.V.; Vijayan, S. Thermodynamic analysis of a triple-pass solar dryer for drying potato slices. J. Therm. Anal. Calorim. 2018, 136, 159–171. [Google Scholar] [CrossRef]
- Akpinar, E.K. Drying of mint leaves in a solar dryer and under open sun: Modelling, performance analyses. Energy Convers. Manag. 2010, 51, 2407–2418. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Yazdi, M.H.; Ruslan, M.H.; Gabbasa, M.; Kazem, H.A. Performance analysis of solar drying system for red chili. Sol. Energy 2014, 99, 47–54. [Google Scholar] [CrossRef]
- Vega, A.; Fito, P.; Andrés, A.; Lemus, R. Mathematical modeling of hot-air drying kinetics of red bell pepper. J. Food Eng. 2007, 79, 1460–1466. [Google Scholar] [CrossRef]














| Component Name | Function | Characteristic Parameters |
|---|---|---|
| Flat-plate solar collector with dual-function (DF-FPSC) | Converting solar energy into hot air or hot water | Dual air and water channel. Size: 2 m × 1 m × 0.15 m (L × W × H). |
| Cabinet | Drying sample material | Total volume: 0.77 m2. Inclination: of 26.7° |
| Fan | Driving air flow | Fan power of 80 W |
| Water tank | Storing hot water | Water tank bulk of 120 mL |
| Water pump | Driving water flow | Pump power of 200 W |
| Auxiliary system | Providing extra heating energy | Electric heating power of 800 W |
| Tray | Placing the drying material | Size: 0.6 m × 0.3 m (L × W). |
| Instruments Name | Measuring Parameters | Range | Accuracy |
|---|---|---|---|
| TBQ-2 Pyranometer | Solar radiation intensity | 0~2000 W/m2 | 2% |
| T type thermocouple | temperature | −200~350 °C | ±0.5 °C |
| Temperature and humidity tester | Air relative humidity | 0~100% | 3% |
| Air flow meter | Mass flow rate of air | 0~500 m3/h | 1% |
| Water flow meter | Mass flow rate of water | 0~15 m3/h | 1% |
| Electronic balance | Samples mass | 0~10 kg | ±0.1 g |
| Clamp meter Testo 770-3 | Power of fan and pump | Alternating/direct current (0~600 A) Alternating/direct voltage (0~600 V) | Alternating/direct current (± 2%) Alternating/direct voltage (± 1%) |
| Name | Value |
|---|---|
| Solar radiation | 2.8 W/m2 |
| Temperature | 1 °C |
| Mass flow rate (air and water) | 1.4 m3/h |
| Power | 0.04 W |
| Air relative humidity | 4.24% |
| Sample mass | 0.52 kg |
| Thermal efficiency of collector | 3.44% |
| Drying system thermal efficiency | 2% |
| Drying system exergy efficiency | 2.43% |
| Number | Model Name | Model Equation | Ref. |
|---|---|---|---|
| 1 | Newton | [34] | |
| 2 | Page | [35] | |
| 3 | Henderson and pabis | [36] | |
| 4 | Logarithmic | [37] | |
| 5 | Two term | [38] | |
| 6 | Wang and Singh | [39] | |
| 7 | Modified Page | [40] | |
| 8 | Thompson | [41] |
| Name of Model | Hybrid Solar Dryer | Open Sun Drying (OSD) | ||||
|---|---|---|---|---|---|---|
| Constant Coefficients | R2 | RMSE | Constant Coefficients | R2 | RMSE | |
| Newton | k = 0.116 | 0.973 | 0.0617 | k = 0.083 | 0.956 | 0.0316 |
| Page | k = 0.156 n = 0.834 | 0.989 | 0.0742 | k = 0.128 n = 0.767 | 0.992 | 0.0138 |
| Henderson and pabis | a = 0.965 k = 0.109 | 0.979 | 0.0276 | a = 0.953 k = 0.075 | 0.974 | 0.0239 |
| Logarithmic | a = 0.735 k = 0.208 c = 0.277 | 0.996 | 0.0120 | a = 0.596 k = 0.192 c = 0.404 | 0.997 | 0.0069 |
| Two term | a = 0.031 b = 0.976 k0 = −0.168 k1 = 0.15 | 0.998 | 0.0069 | a = 0.87 b = 0.126 k0 = 0.133 k1 = −0.075 | 0.998 | 0.0069 |
| Wang and Singh | a = −0.127 b = 0.007 | 0.998 | 0.0069 | a = −0.099 b = 0.005 | 0.997 | 0.0098 |
| Modified Page | k = 0.108 n = 0.834 | 0.989 | 0.0195 | k = 0.071 n = 0.773 | 0.986 | 0.0183 |
| Thompson | a = −5.36 b = 4.412 | 0.985 | 0.3727 | a = −6.424 b = 10.215 | 0.992 | 0.2661 |
| Performance Parameters | Value | Unit |
|---|---|---|
| Initial mass (lemon slices) | 4.12 | kg |
| Final mass (lemon slices) | 0.55 | kg |
| Total drying time | 27 | h |
| Specific energy consumption | 4.05 | kW·h/kg |
| Initial moisture content (w.b) | 83.6 | % |
| Electrical consumption of fan | 2.28 | kW·h |
| Electrical consumption of pump | 0.75 | kW·h |
| Final moisture content (w.b) | 10.3 | % |
| Thermal efficiency of collector | 2~69.52 | % |
| Thermal efficiency of hybrid solar dryer | 9.5 | % |
| Exergy inflow of hybrid solar dryer | 6.46~359.34 | W |
| Exergy outflow of hybrid solar dryer | 0.68~149.42 | W |
| Exergy efficiency of hybrid solar dryer | 39.38~71.7 | % |
| Parameters | Value | Unit |
|---|---|---|
| Material cost (collector, fan, pipe etc.) | 3500 | RMB |
| Construction cost | 2200 | RMB |
| Maintenance cost | 57 | RMB |
| Electricity price | 0.8 | RMB/kW·h |
| Fresh lemon price | 22.9 | RMB/kg |
| Dried lemon price | 169 | RMB/kg |
| Lifetime | 5 | Year |
| Interest rate | 5.63 | % |
| Inflation rate | 2.5 | % |
| Pay-back period | 3.63 | Year |
| Annual cost calculation | 1177 | RMB |
| Cost per unit of drying materials | 5.89 | RMB/kg |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hao, W.; Liu, S.; Mi, B.; Lai, Y. Mathematical Modeling and Performance Analysis of a New Hybrid Solar Dryer of Lemon Slices for Controlling Drying Temperature. Energies 2020, 13, 350. https://doi.org/10.3390/en13020350
Hao W, Liu S, Mi B, Lai Y. Mathematical Modeling and Performance Analysis of a New Hybrid Solar Dryer of Lemon Slices for Controlling Drying Temperature. Energies. 2020; 13(2):350. https://doi.org/10.3390/en13020350
Chicago/Turabian StyleHao, Wengang, Shuonan Liu, Baoqi Mi, and Yanhua Lai. 2020. "Mathematical Modeling and Performance Analysis of a New Hybrid Solar Dryer of Lemon Slices for Controlling Drying Temperature" Energies 13, no. 2: 350. https://doi.org/10.3390/en13020350
APA StyleHao, W., Liu, S., Mi, B., & Lai, Y. (2020). Mathematical Modeling and Performance Analysis of a New Hybrid Solar Dryer of Lemon Slices for Controlling Drying Temperature. Energies, 13(2), 350. https://doi.org/10.3390/en13020350




