Flexible Kinetic Energy Release Controllers for a Wind Farm in an Islanding System
Abstract
:1. Introduction
2. Test System Model
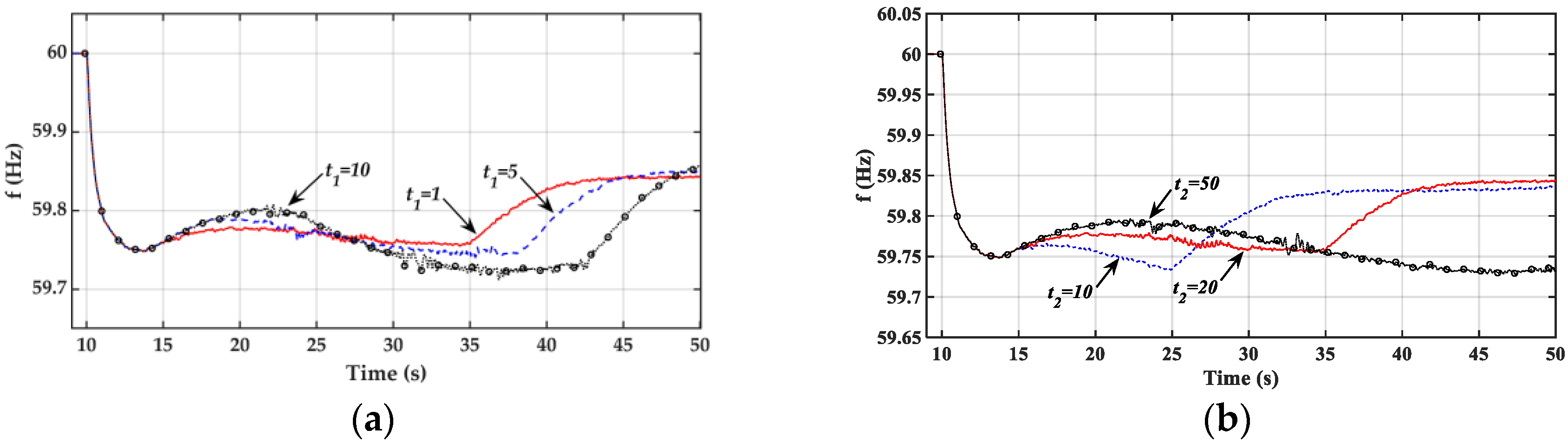
3. Type I Flexible Kinetic Energy Release Controller Using the Deactivation Function Based Integral Controller
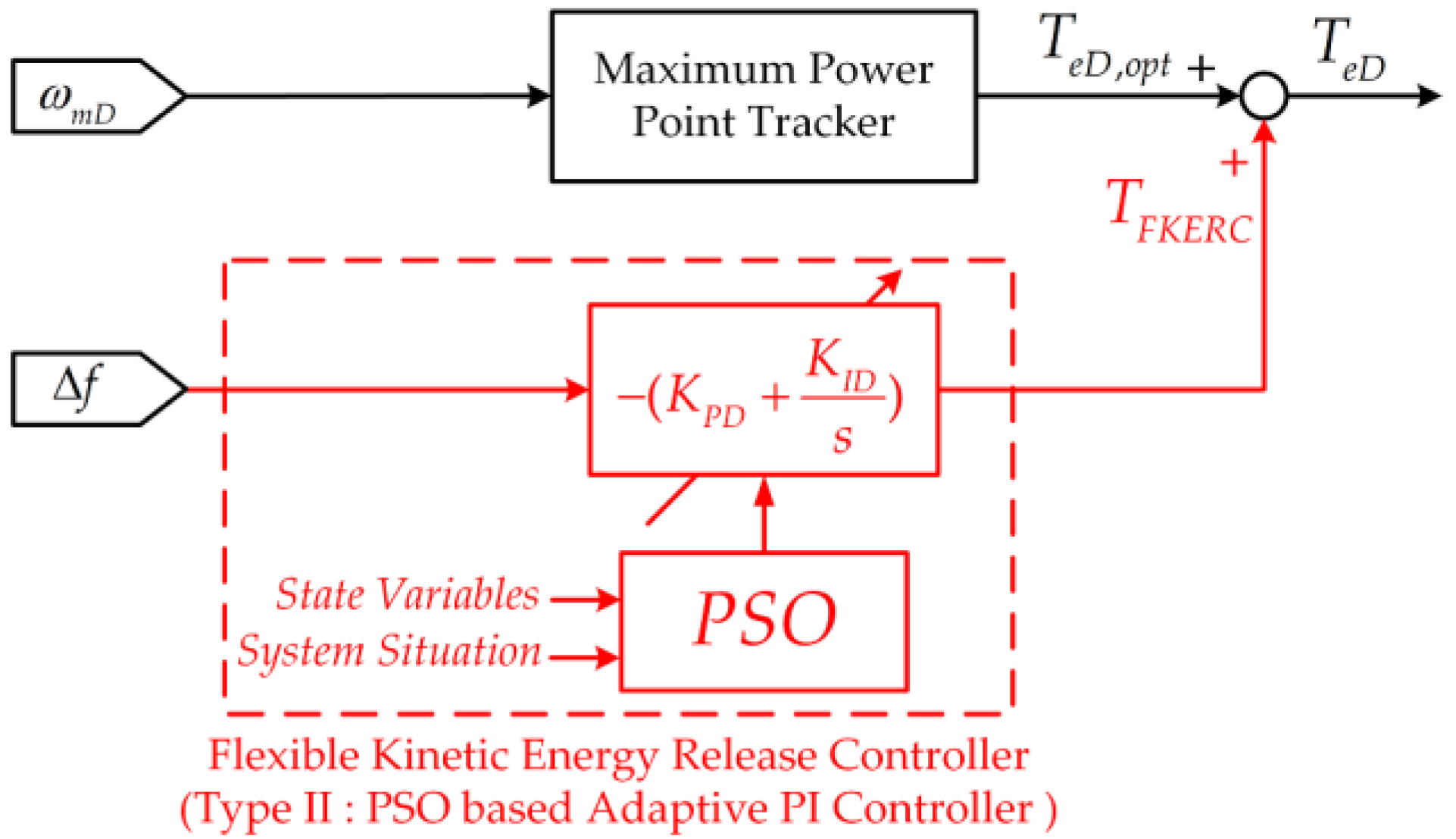
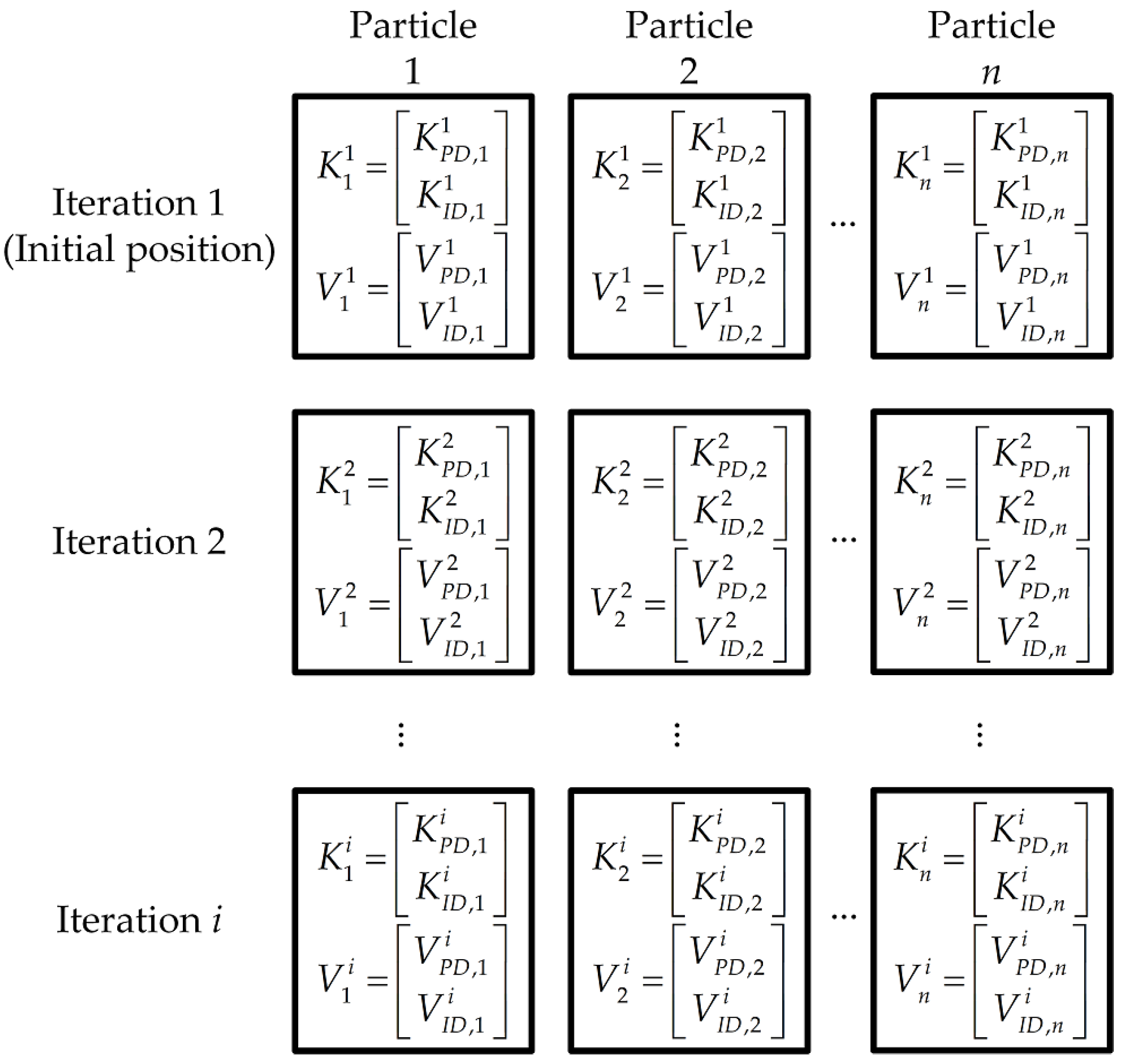
4. Type II Flexible Kinetic Energy Release Controller Using PSO
5. Simulation Results
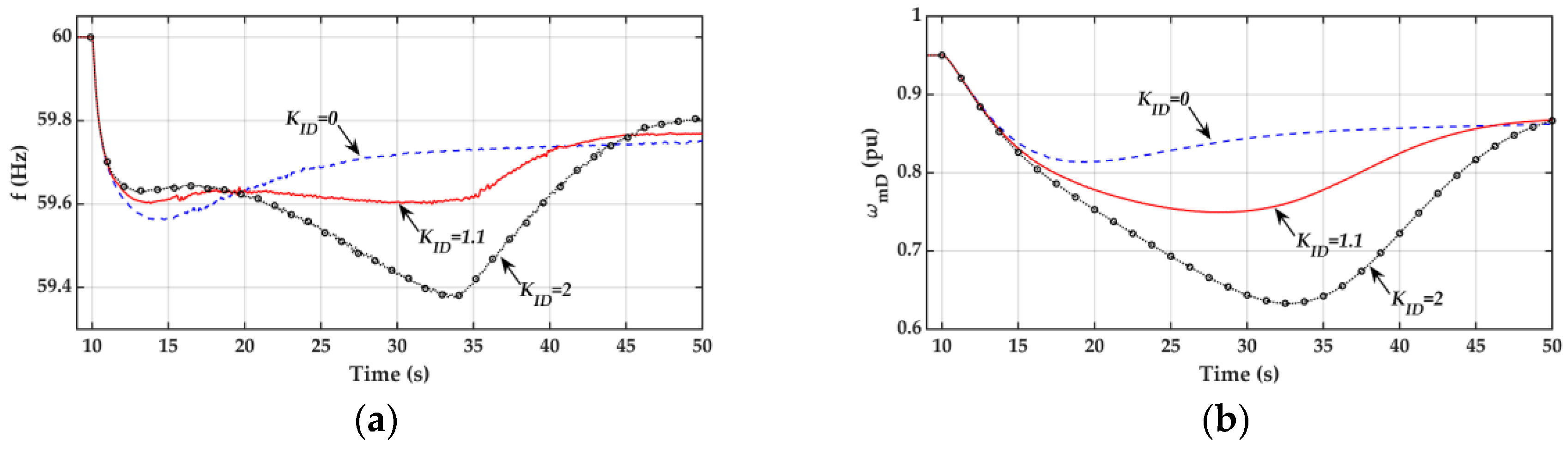
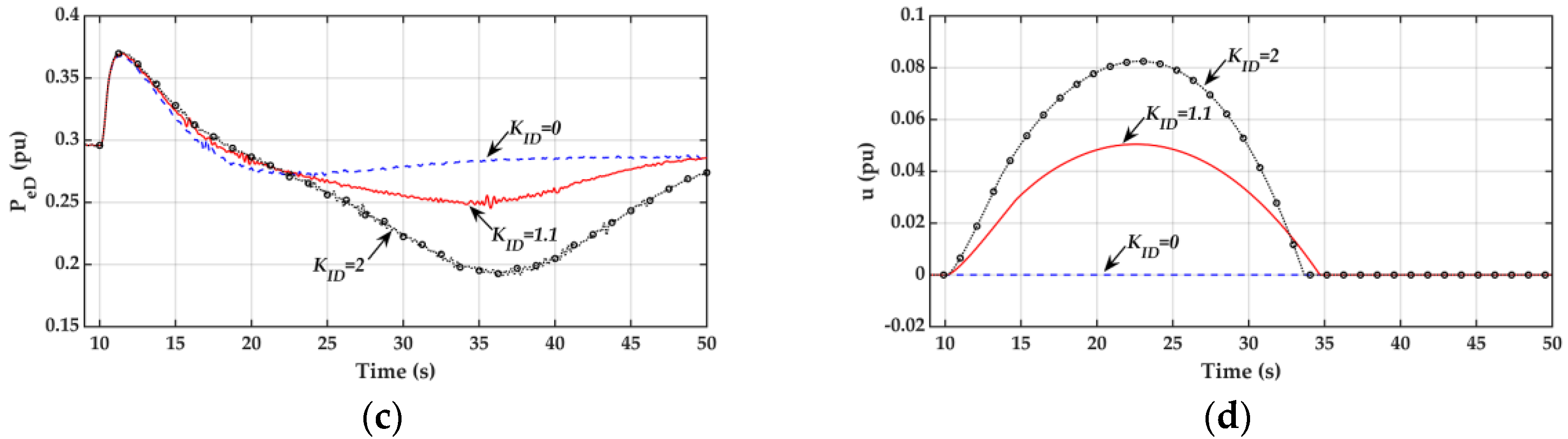
5.1. Dynamic Performance of Type I Flexible Kinetic Energy Release Controller
- Case 1: Droop control only (KID = 0).
- Case 2: Type I controller with KID = 1.1.
- Case 3: Type I controller with KID = 2.
- The frequency response curves for the three cases are essentially the same during the inertia period (1–2 s after the disturbance or 10 s ≤ t ≤ 11–12 s) since the frequency nadir is of major concern and inertia control is not considered in this work.
- The frequency nadir for Case 1 with only a droop controller is lower than the prespecified threshold of 59.6 Hz.
- With the proposed Type I flexible kinetic energy release controller, the frequency nadir is improved to a value higher than the threshold of 59.6 Hz. This is achieved through the addition of an integral controller with a gain of KID = 1.1. As shown in Figure 9c,d, both the DFIG electrical power PeD and control signal are increased by the proposed Type I flexible kinetic energy release controller during the first few seconds in the primary frequency regulation period (12 s ≤ t ≤ 22 s)
- As evidenced by the response curve in Figure 9d, the control signal u from the proposed Type I flexible kinetic energy release controller is gradually increased when the system frequency drops to 59.9 Hz at t = 10.5 s. The deactivation function in Figure 4 begins to work at t = 13 s when the frequency nadir is detected. Due to the action of deactivation function, the rate of change of control signal u is gradually decreased and the control signal u is decreased to zero in a very smooth manner in order to avoid the second frequency nadir.
- Although the DFIG output power PeD can be further increased and the first frequency nadir at around t = 13 s can be improved further using a higher integral gain of KID = 2 for the Type I flexible kinetic energy release controller, the DFIG speed will drop significantly and the DFIG kinetic energy will be exhausted in the primary frequency regulation period. As a result, a very low second frequency nadir (59.4 Hz) will be observed at t = 34 s. A moderate gain of KID = 1.1 seems to be a good choice for the study system.
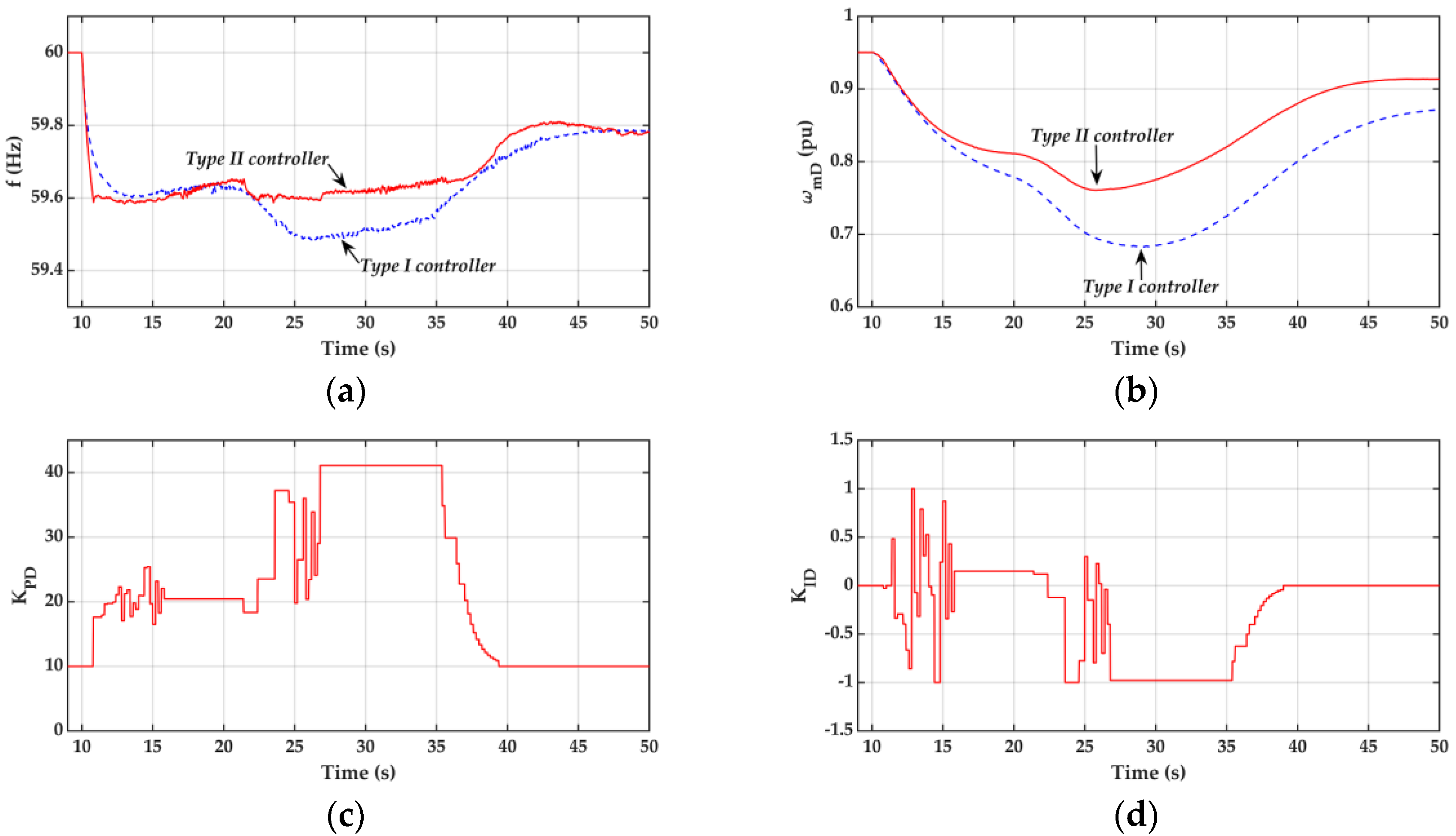
5.2. Dynamic Performance of Type II Flexible Kinetic Energy Release Controller
5.2.1. Dynamic Response Curves under Uncertainties in System Parameters Servo-Motor Time Constant Tsm and Reheater Time Constant Tt4 (Pgrid = 45 MW, Vw = 11 m/s, Tsm = 0.375 s, Tt4 = 6.625 s)
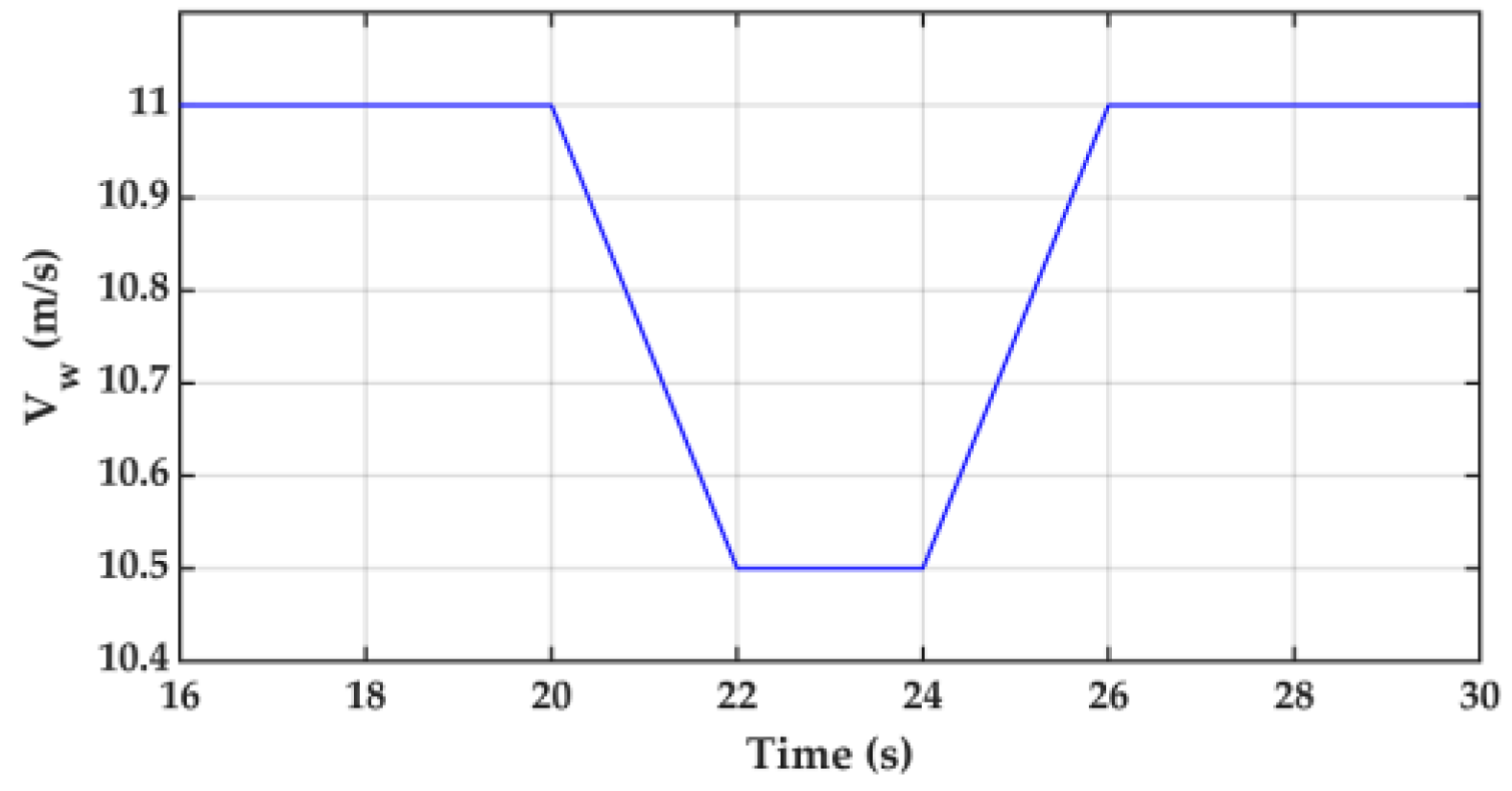
5.2.2. Dynamic Response Curves under Change of Wind Speed
5.2.3. Dynamic Response Curves under Uncertainties in System Parameters and Fluctuation of Wind Speed in Different Pgrid
- When the deficit power Pgrid is 30 MW, the PSO algorithm will not be initiated since the frequency response during the entire post-fault period is higher than the threshold of 59.6 Hz. Therefore, the controller gains for the Type II controller will remain at the initial values (KPD = 10, KID = 0).
- As the deficit power Pgrid is increased to 45 MW, the controller gains for the Type II controller can be adjusted online to improve the frequency response, even though the PSO algorithm is unaware of the change in SG parameters and the fluctuations in wind speed.
- When the system encounters a large deficit power, e.g., Pgrid= 50 MW, the system frequency can be kept around 59.6 Hz in the first few seconds after disturbance using the proposed Type II controller. However, the DFIG stall and frequency collapse are observed afterwards since the large deficit power exceeds the upper limit of the DFIG stored kinetic energy, which can be released to the power system under disturbance conditions.
6. Conclusions
- The Type II flexible kinetic energy release controller with the controller gains being adapted in real-time, using the PSO technique, has been found to be able to offer a better dynamic frequency response than the Type I controller when the system is subject to external disturbances or parameter variations.
- In this paper, the pitch angle is set to be zero to avoid considerable revenue losses. However, the de-loaded operation may be used to improve the system frequency at the price of revenue loss when the deficit power exceeds the DFIG kinetic energy limit.
- Although the dynamic frequency response can be improved by the proposed Type I and Type II controllers, the delay time and decay time in the Type I controller must be properly designed in order to achieve good performance. In addition, it takes a long time for the PSO algorithm to reach the desired optimal gains of KPD and KID. In order to reduce the computational burden, it is necessary to limit the number of particles per iteration. However, the local minimum may be experienced as a result of an insufficient number of particles.
- Future work will be devoted to the implementation and field test of the proposed controllers. Furthermore, the coordination between the pitch angle controller and the proposed controller will be investigated.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| load damping | |
| (t) | deactivation function |
| equivalent inertia time constants of synchronous machine and DFIG | |
| system frequency | |
| DFIG supplementary proportional and integral controller gain | |
| grid power | |
| electromagnetic power of DFIG | |
| mechanical power of synchronous machine | |
| mechanical torque and electromagnetic torque of DFIG | |
| electromagnetic torque command of DFIG for MPPT | |
| flexible kinetic energy release control signal | |
| ,, | decay time, delay time, and nadir frequency time |
| DFIG speed | |
| wind speed | |
| incremental quantity |
Appendix A
References
- Shahabi, M.; Haghifam, M.R.; Mohamadian, M.; Nabavi-Niaki, S.A. Microgrid dynamic performance improvement using a doubly fed induction wind generator. IEEE Trans. Energy Convers. 2009, 24, 137–145. [Google Scholar] [CrossRef]
- Mauricio, J.M.; Marano, A.; Gomez-Exposito, A.; Ramos, J.L.M. Frequency regulation contribution through variable-speed wind energy conversion systems. IEEE Trans. Power Syst. 2009, 24, 173–180. [Google Scholar]
- Arani, M.F.M.; Mohamed, Y.A.I. Analysis and impacts of implementing droop control in DFIG-based wind turbines on microgrid/weak-grid stability. IEEE Trans. Power Syst. 2015, 30, 385–396. [Google Scholar]
- Hwang, M.; Muljadi, E.; Park, J.; Sørensen, P.; Kang, Y.C. Dynamic droop–based inertial control of a doubly-fed induction generator. IEEE Trans. Sustain. Energy 2016, 7, 924–933. [Google Scholar]
- Lee, J.; Jang, G.; Muljadi, E.; Blaabjerg, F.; Chen, Z.; Kang, Y.C. Stable short-term frequency support using adaptive gains for a DFIG-based wind power plant. IEEE Trans. Energy Convers. 2016, 31, 1068–1079. [Google Scholar]
- Arani, M.F.M.; Mohamed, Y.A.I. Dynamic droop control for wind turbines participating in primary frequency regulation in microgrids. IEEE Trans. Smart Grid 2018, 9, 5742–5751. [Google Scholar]
- Garmroodi, M.; Verbič, G.; Hill, D.J. Frequency support from wind turbine generators with a time-variable droop characteristic. IEEE Trans. Sustain. Energy 2018, 9, 676–684. [Google Scholar] [CrossRef]
- Yang, J.; Chen, Y.; Hsu, Y. Small-signal stability analysis and particle swarm optimisation self-tuning frequency control for an islanding system with DFIG wind farm. IET Gener. Transm. Distrib. 2019, 13, 563–574. [Google Scholar]
- Li, Y.; Xu, Z.; Zhang, J.; Wong, K.P. Variable gain control scheme of DFIG-based wind farm for over-frequency support. Renew. Energy 2018, 120, 379–391. [Google Scholar] [CrossRef]
- Hwang, M.; Muljadi, E.; Jang, G.; Kang, Y.C. Disturbance-adaptive short-term frequency support of a DFIG associated with the variable gain based on the ROCOF and rotor speed. IEEE Trans. Power Syst. 2017, 32, 1873–1881. [Google Scholar] [CrossRef]
- Hafiz, F.; Abdennour, A. An adaptive neuro-fuzzy inertia controller for variable-speed wind turbines. Renew. Energy 2016, 92, 136–146. [Google Scholar] [CrossRef]
- Qudaih, Y.S.; Bernard, M.; Mitani, Y.; Mohamed, T.H. Model predictive based load frequency control design in the presence of DFIG wind turbine. In Proceedings of the 2011 2nd International Conference on Electric Power and Energy Conversion Systems (EPECS), Sharjah, UAE, 15–17 November 2011; pp. 1–5. [Google Scholar]
- Mohamed, T.H.; Morel, J.; Bevrani, H.; Hiyama, T. Model predictive based load frequency control_design concerning wind turbines. Int. J. Electr. Power Energy Syst. 2012, 43, 859–867. [Google Scholar] [CrossRef]
- Tielens, P.; Hertem, D.V. Receding horizon control of wind power to provide frequency regulation. IEEE Trans. Power Syst. 2017, 32, 2663–2672. [Google Scholar] [CrossRef]
- Wang, H.; Yang, J.; Chen, Z.; Ge, W.; Ma, Y.; Xing, Z.; Yang, L. Model predictive control of PMSG-based wind turbines for frequency regulation in an isolated grid. IEEE Trans. Ind. Appl. 2018, 54, 3077–3089. [Google Scholar] [CrossRef]
- Zhang, S.; Mishra, Y.; Shahidehpour, M. Fuzzy-logic based frequency controller for wind farms augmented with energy storage systems. IEEE Trans. Power Syst. 2016, 31, 1595–1603. [Google Scholar] [CrossRef]
- Ghafouri, A.; Milimonfared, J.; Gharehpetian, G.B. Fuzzy-adaptive frequency control of power system including microgrids, wind farms, and conventional power plants. IEEE Syst. J. 2018, 12, 2772–2781. [Google Scholar] [CrossRef]
- Abazari, A.; Monsef, H.; Wu, B. Load frequency control by de-loaded wind farm using the optimal fuzzy-based PID droop controller. IET Renew. Power Gener. 2019, 13, 180–190. [Google Scholar] [CrossRef]
- Wu, Y.; Yang, W.; Hu, Y.; Dzung, P.Q. Frequency regulation at a wind farm using time-varying inertia and droop controls. IEEE Trans. Ind. Appl. 2019, 55, 213–224. [Google Scholar] [CrossRef]
- Geng, H.; Xi, X.; Yang, G. Small-signal stability of power system integrated with ancillary-controlled large-scale DFIG-based wind farm. IET Renew. Power Gener. 2017, 11, 1191–1198. [Google Scholar] [CrossRef]
- Lee, J.; Muljadi, E.; Srensen, P.; Kang, Y.C. Releasable kinetic energy-based inertial control of a DFIG wind power plant. IEEE Trans. Sustain. Energy 2016, 7, 279–288. [Google Scholar] [CrossRef]
- Vyver, J.V.d.; Kooning, J.D.M.D.; Meersman, B.; Vandevelde, L.; Vandoorn, T.L. Droop control as an alternative inertial response strategy for the synthetic inertia on wind turbines. IEEE Trans. Power Syst. 2016, 31, 1129–1138. [Google Scholar] [CrossRef]
- Vidyanandan, K.V.; Senroy, N. Primary frequency regulation by deloaded wind turbines using variable droop. IEEE Trans. Power Syst. 2013, 28, 837–846. [Google Scholar] [CrossRef]
- Kayikci, M.; Milanovic, J.V. Dynamic contribution of DFIG-based wind plants to system frequency disturbances. IEEE Trans. Power Syst. 2009, 24, 859–867. [Google Scholar]
- Ramtharan, G.; Ekanayake, J.B.; Jenkins, N. Frequency support from doubly fed induction generator wind turbines. IET Renew. Power Gener. 2007, 1, 3–9. [Google Scholar]
- Morren, J.; Pierik, J.; De Haan, S.W.H. Inertial response of variable speed wind turbines. Electr. Power Syst. Res. 2006, 76, 980–987. [Google Scholar] [CrossRef]
- Morren, J.; Haan, S.W.H.d.; Kling, W.L.; Ferreira, J.A. Wind turbines emulating inertia and supporting primary frequency control. IEEE Trans. Power Syst. 2006, 21, 433–434. [Google Scholar] [CrossRef]
- Zhang, X.; Zhu, Z.; Fu, Y.; Li, L. Optimized virtual inertia of wind turbine for rotor angle stability in interconnected power systems. Electr. Power Syst. Res. 2020, 180, 106157. [Google Scholar] [CrossRef]
- Kundur, P. Power System Stability and Control; McGraw-Hill Education: New York, NY, USA, 1994. [Google Scholar]
- Eberhart, R.; Kennedy, J. A new optimizer using particle swarm theory. In MHS’95. Proceedings of the Sixth International Symposium on Micro Machine and Human Science, Nagoya, Japan, 4–6 October 1995; IEEE: New York, NY, USA; pp. 39–43.
- Kennedy, J.; Eberhart, R. Particle swarm optimization. In Proceedings of the ICNN’95—International Conference on Neural Networks, Perth, WA, Australia, 27 November–1 December 1995; Volume 1944, pp. 1942–1948. [Google Scholar]








| Controller/Algorithm | Operating Conditions Dependent | Needs Training | Needs Rule Base | Needs Evaluation Function | Computational Burden |
|---|---|---|---|---|---|
| PID [1,2,3,4,5,6,7,8,9,10,19,20,21,22,23,24,25,26,27,28] | YES | NO | NO | NO | LOW |
| ANN [11] | NO | YES | NO | NO | LOW |
| MPC [12,13,14,15] | NO | NO | NO | YES | HIGH |
| Fuzzy [16,17,18] | NO | NO | YES | NO | LOW |
| Type I | YES | NO | NO | NO | LOW |
| Type II | NO | NO | NO | YES | HIGH |
| KPD | KID | FN | |
|---|---|---|---|
| Pgrid = 30 MW | 5 | 5.5 | 59.63 |
| 10 | 3.7 | 59.70 | |
| 15 | 2.3 | 59.75 | |
| 20 | 1.3 | 59.78 | |
| Pgrid = 35 MW | 5 | 5.4 | 59.56 |
| 10 | 3.6 | 59.65 | |
| 15 | 2.2 | 59.71 | |
| 20 | 1.2 | 59.74 | |
| Pgrid = 40 MW | 5 | 5.3 | 59.50 |
| 10 | 3.5 | 59.60 | |
| 15 | 2.1 | 59.67 | |
| 20 | 1.1 | 59.70 | |
| Pgrid = 45 MW | 5 | 5.1 | 59.43 |
| 10 | 3.4 | 59.55 | |
| 15 | 2 | 59.62 | |
| 20 | 1 | 59.66 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.-W.; Hsu, Y.-Y. Flexible Kinetic Energy Release Controllers for a Wind Farm in an Islanding System. Energies 2020, 13, 6135. https://doi.org/10.3390/en13226135
Chen Y-W, Hsu Y-Y. Flexible Kinetic Energy Release Controllers for a Wind Farm in an Islanding System. Energies. 2020; 13(22):6135. https://doi.org/10.3390/en13226135
Chicago/Turabian StyleChen, Yi-Wei, and Yuan-Yih Hsu. 2020. "Flexible Kinetic Energy Release Controllers for a Wind Farm in an Islanding System" Energies 13, no. 22: 6135. https://doi.org/10.3390/en13226135
APA StyleChen, Y.-W., & Hsu, Y.-Y. (2020). Flexible Kinetic Energy Release Controllers for a Wind Farm in an Islanding System. Energies, 13(22), 6135. https://doi.org/10.3390/en13226135






