Utilization of CO2 as Cushion Gas for Depleted Gas Reservoir Transformed Gas Storage Reservoir
Abstract
:1. Introduction
2. Simulation System
2.1. Governing Equations
2.2. Geological Model
2.3. Operation Scenarios
3. Results and Discussion
3.1. Properties and Behavior of the Gases in a UGSR
3.1.1. Physical Properties of the Mixed Gases
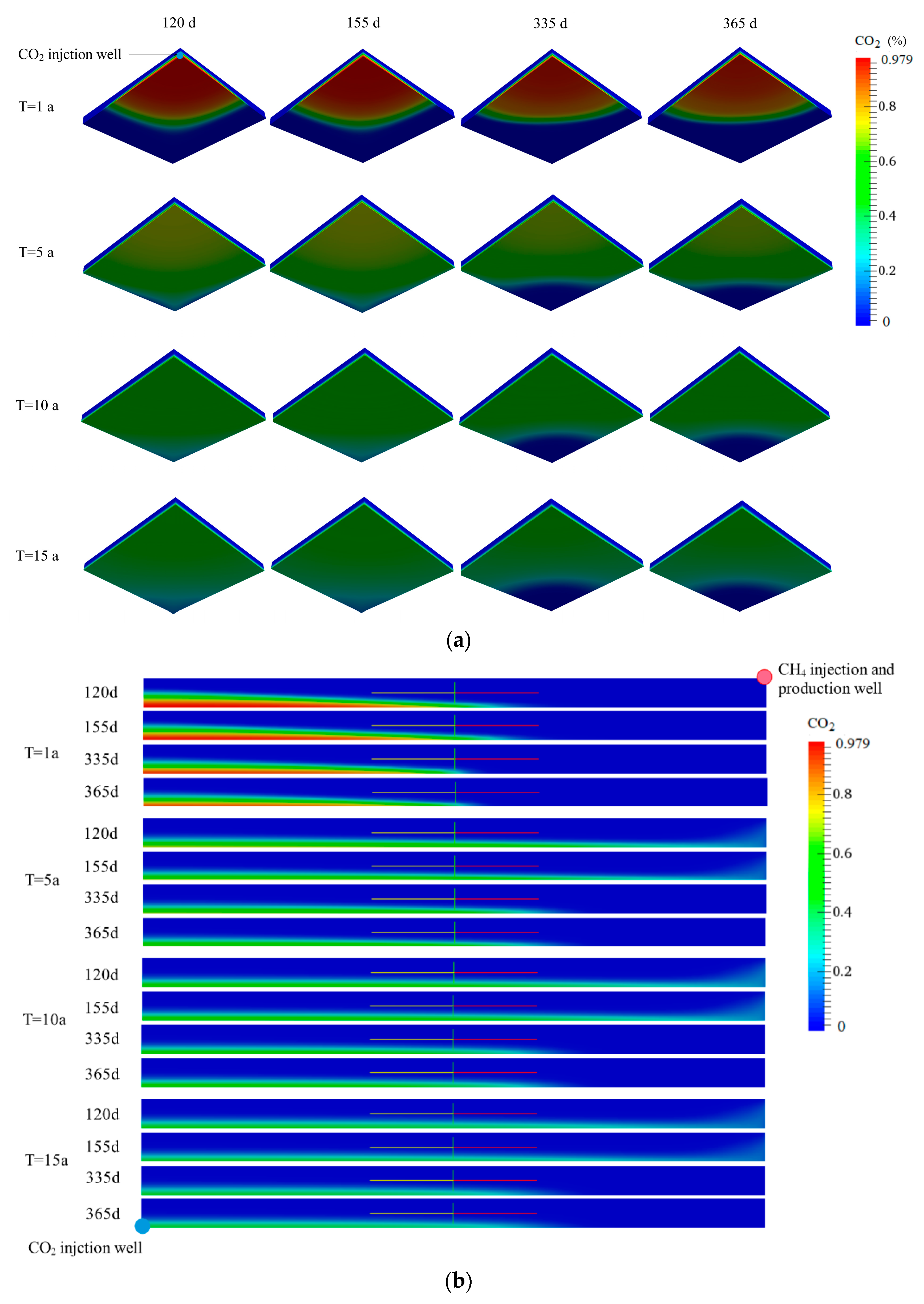
3.1.2. Spatial Distribution of CO2 and CH4
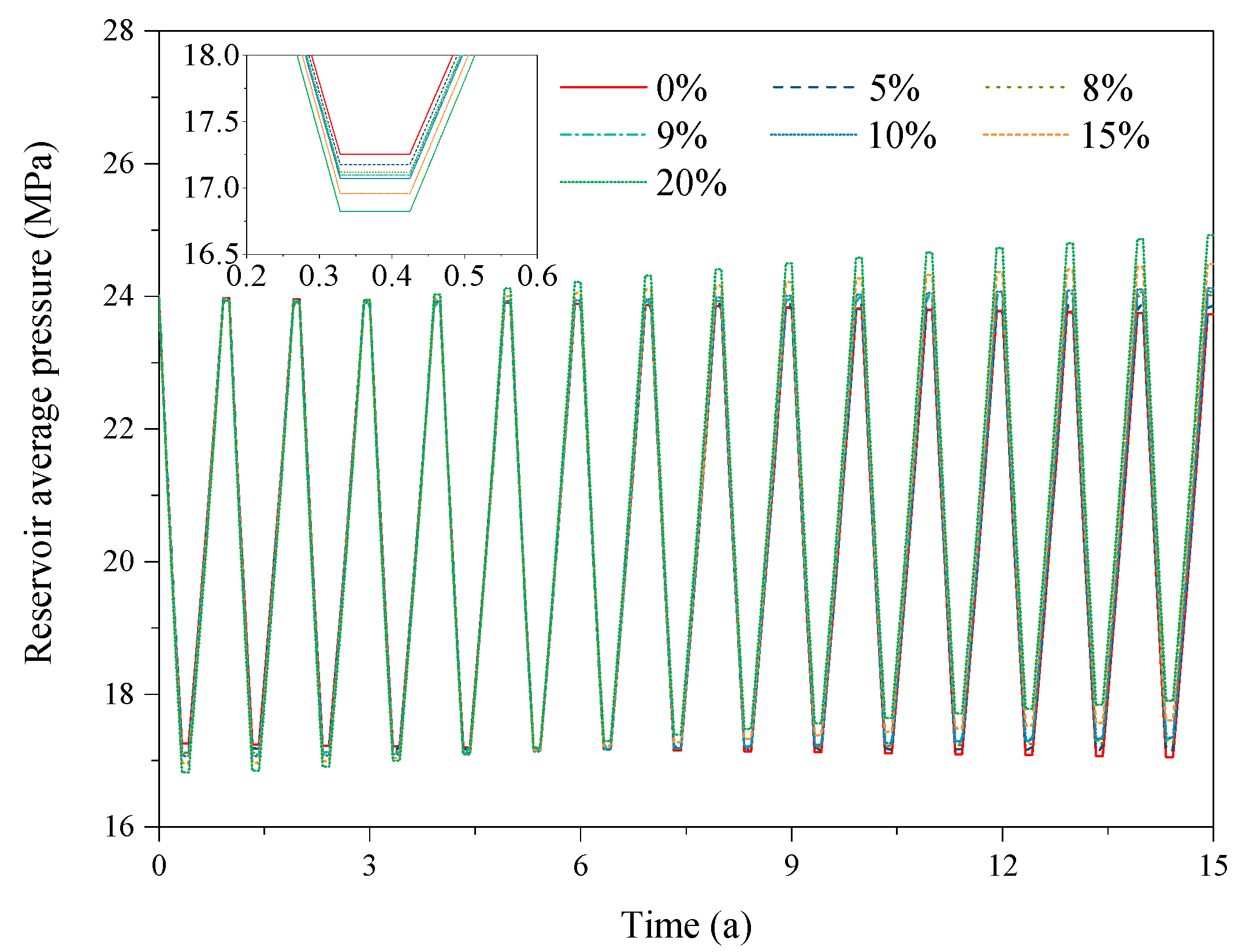
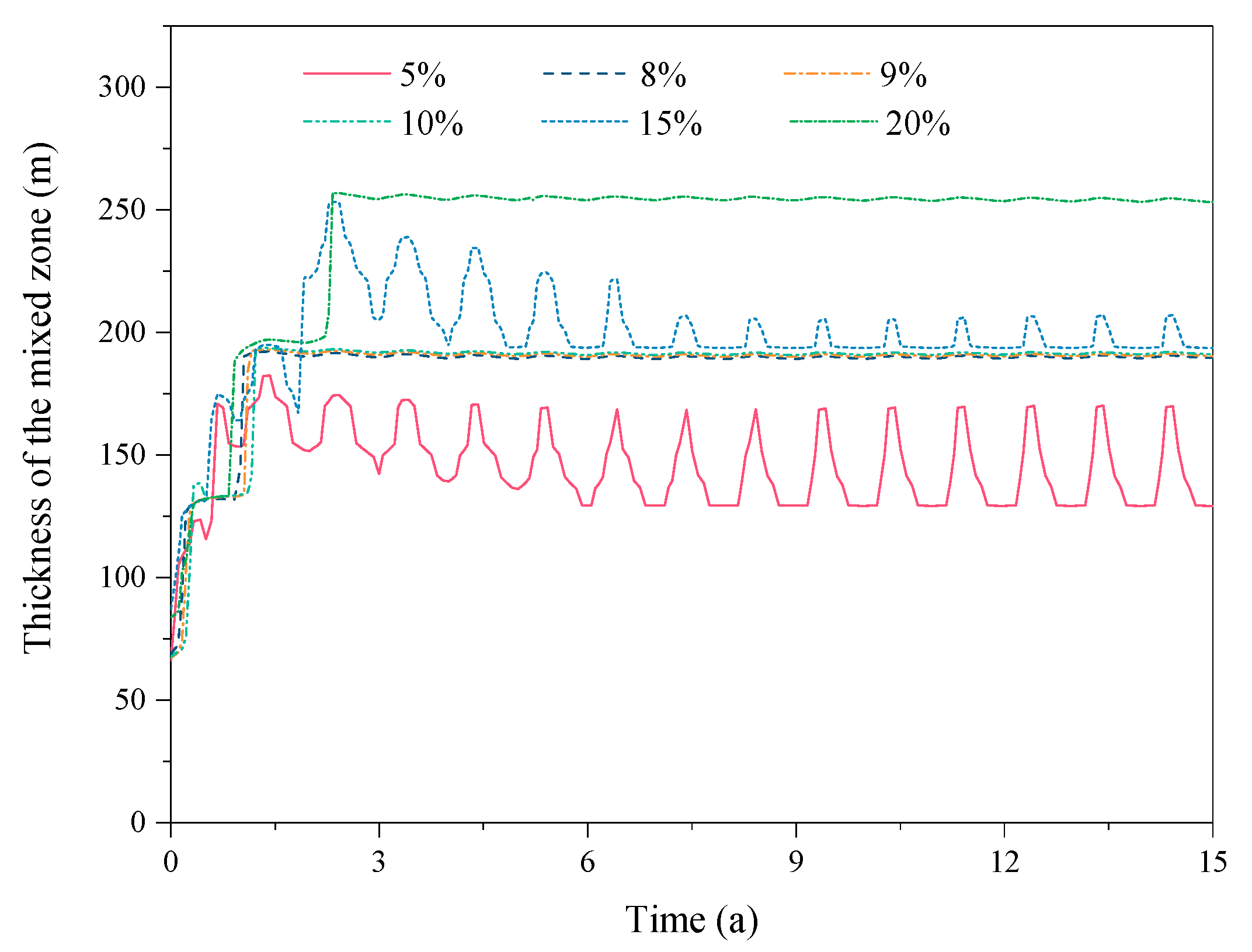
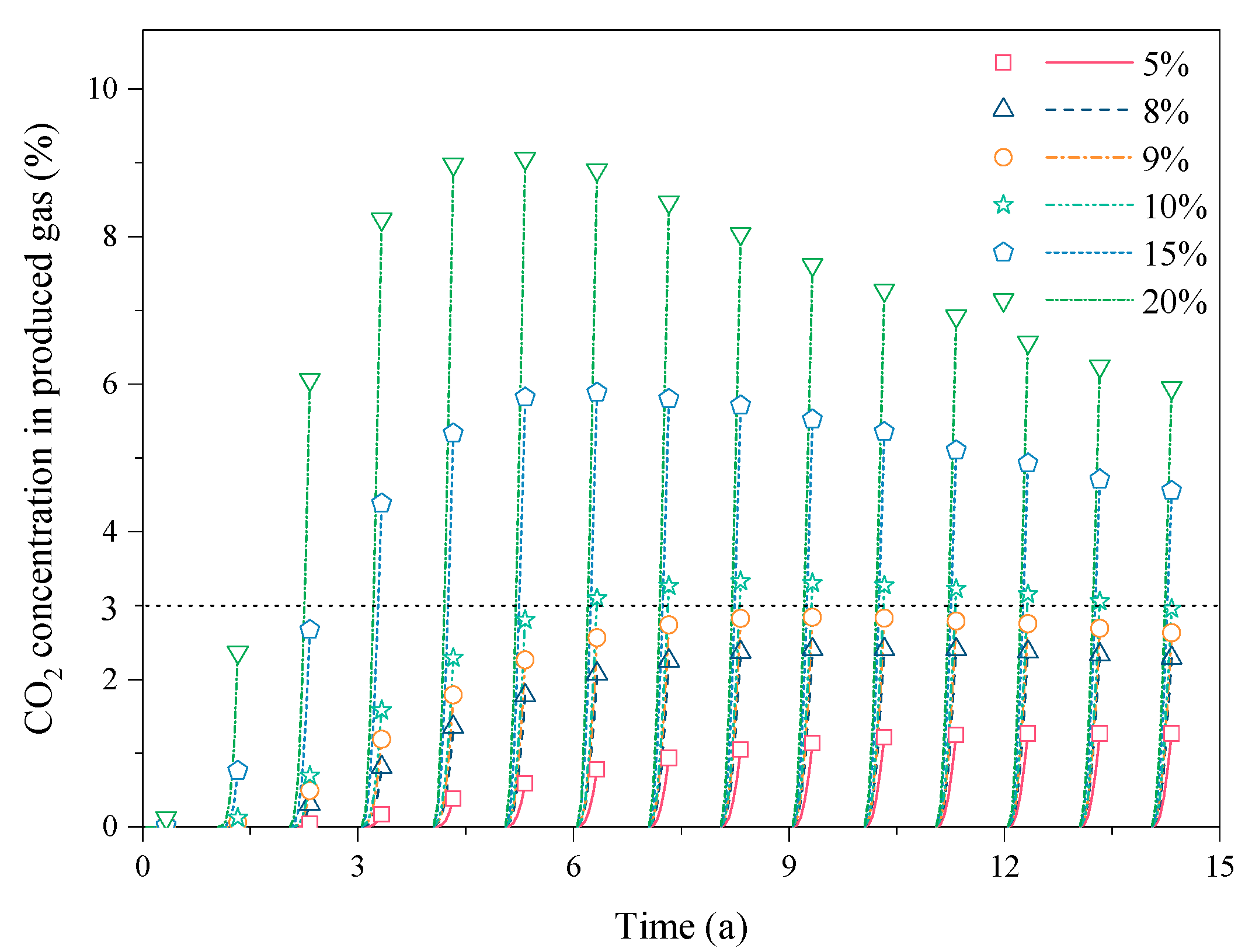
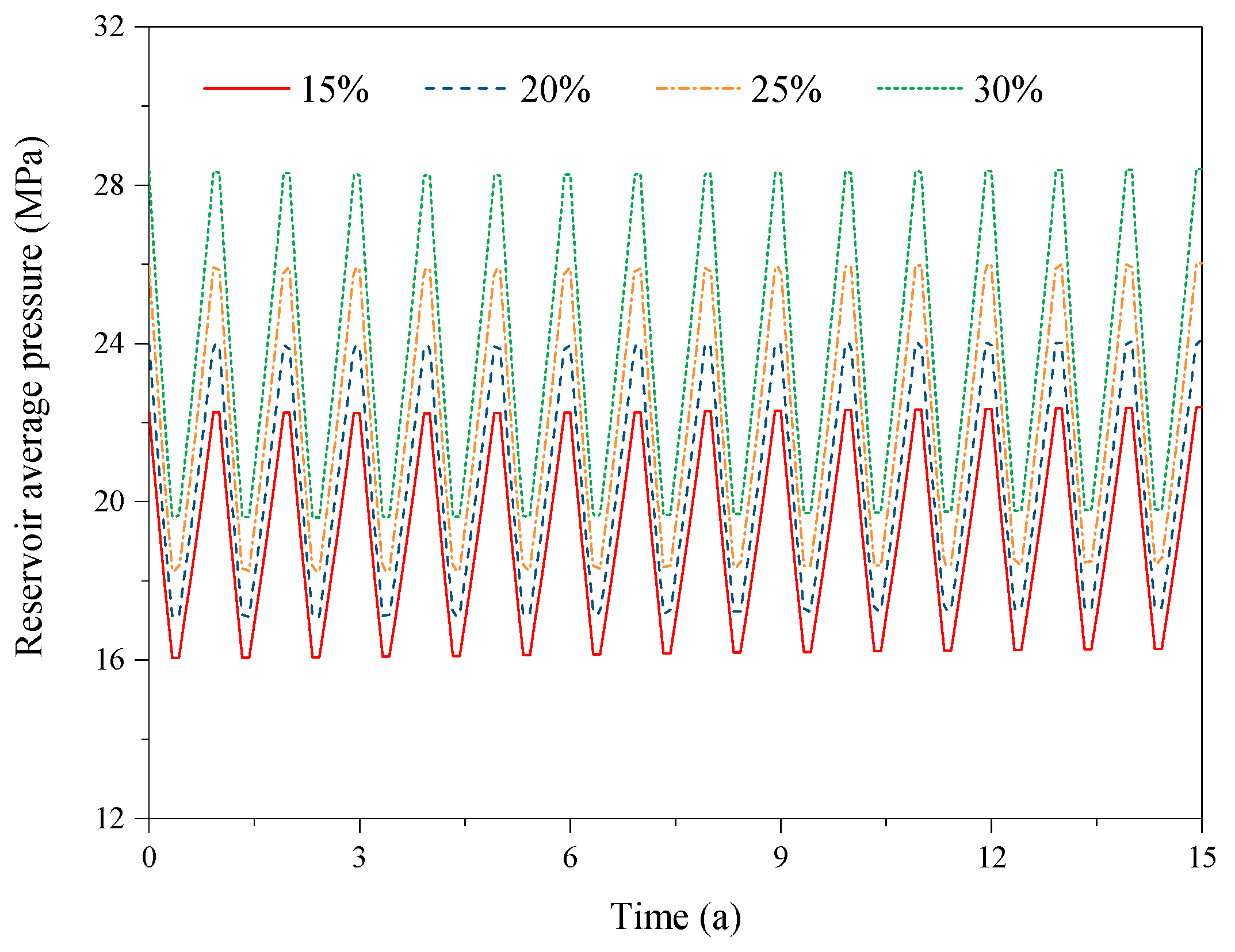
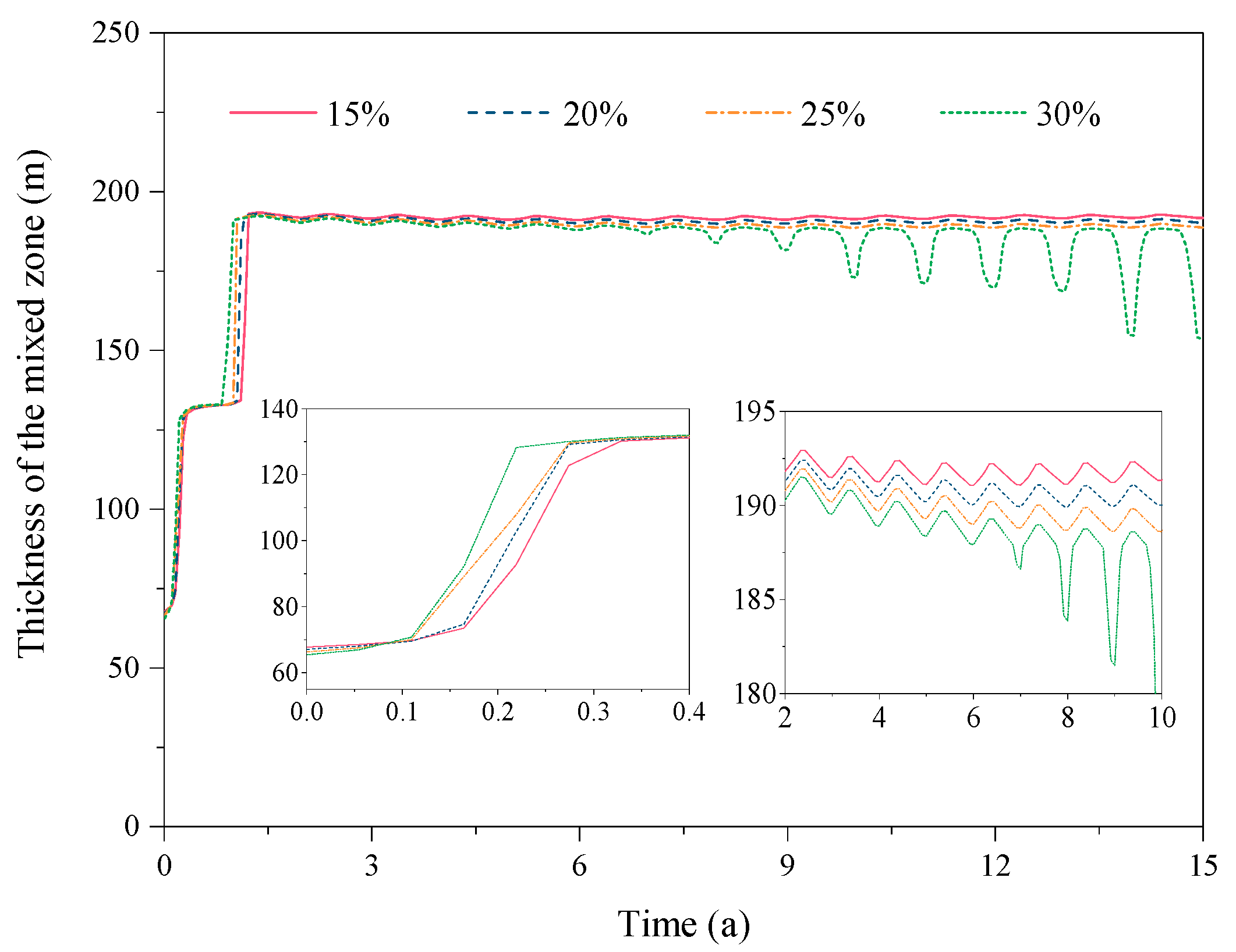
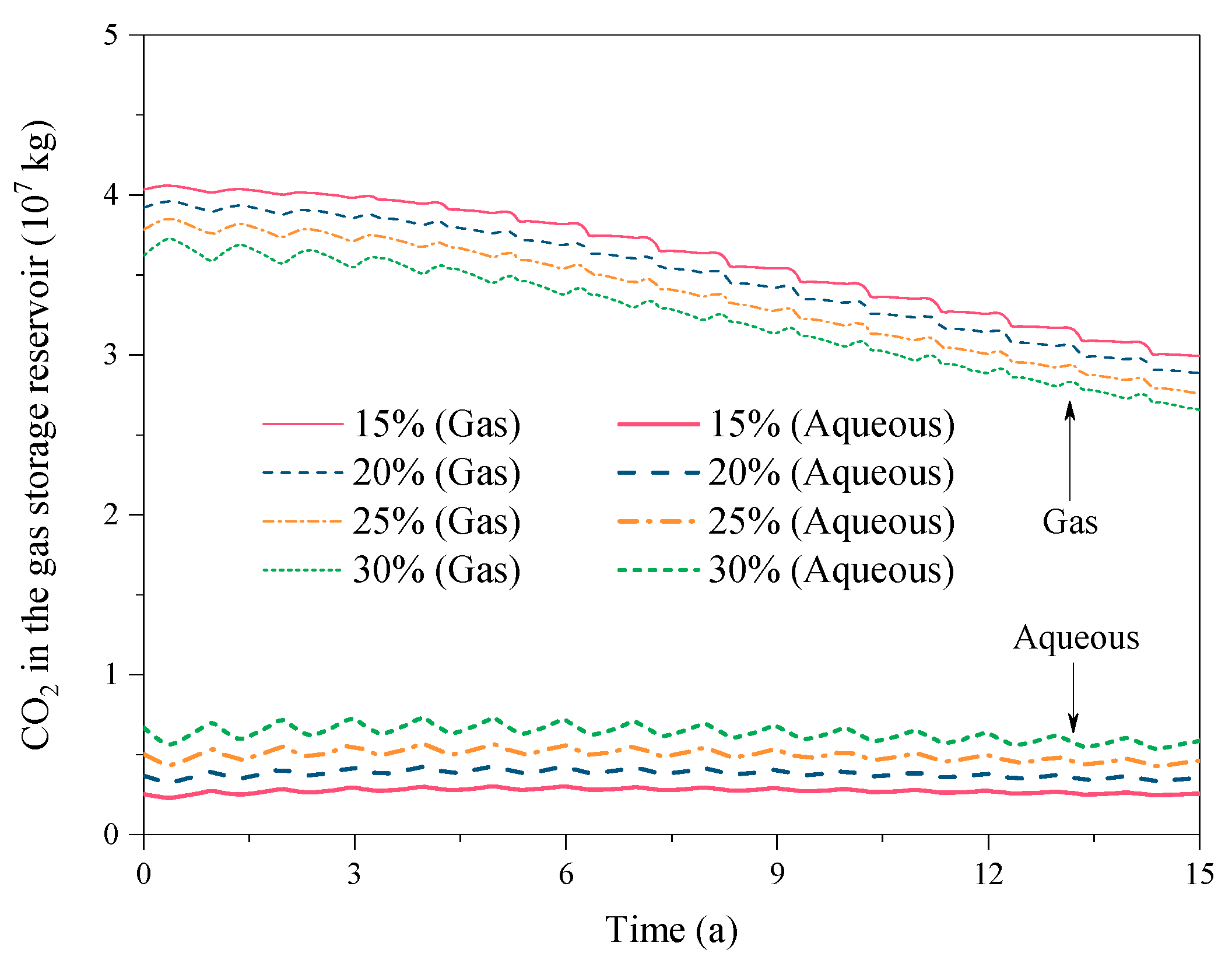
3.2. Effect of the CO2 Fraction
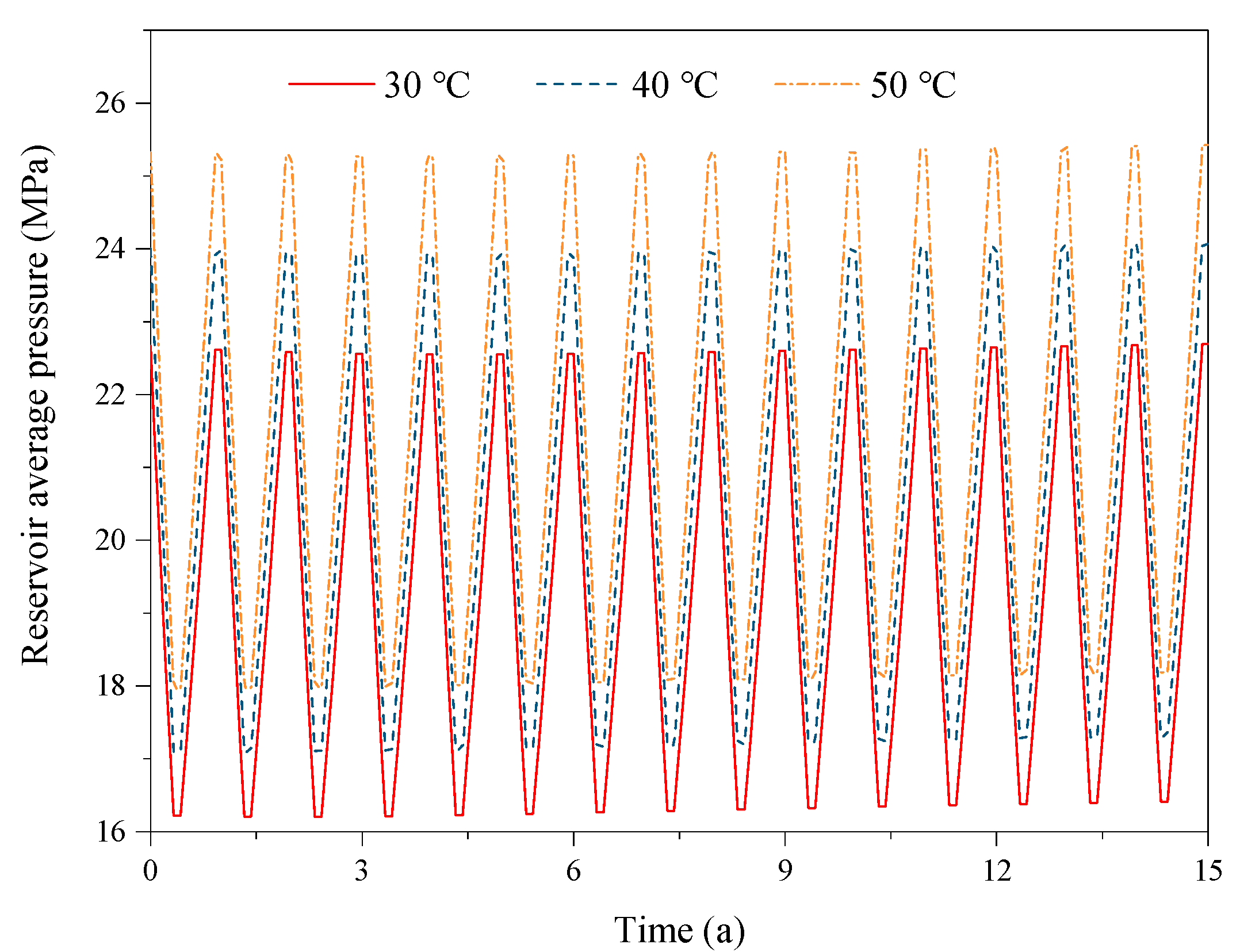
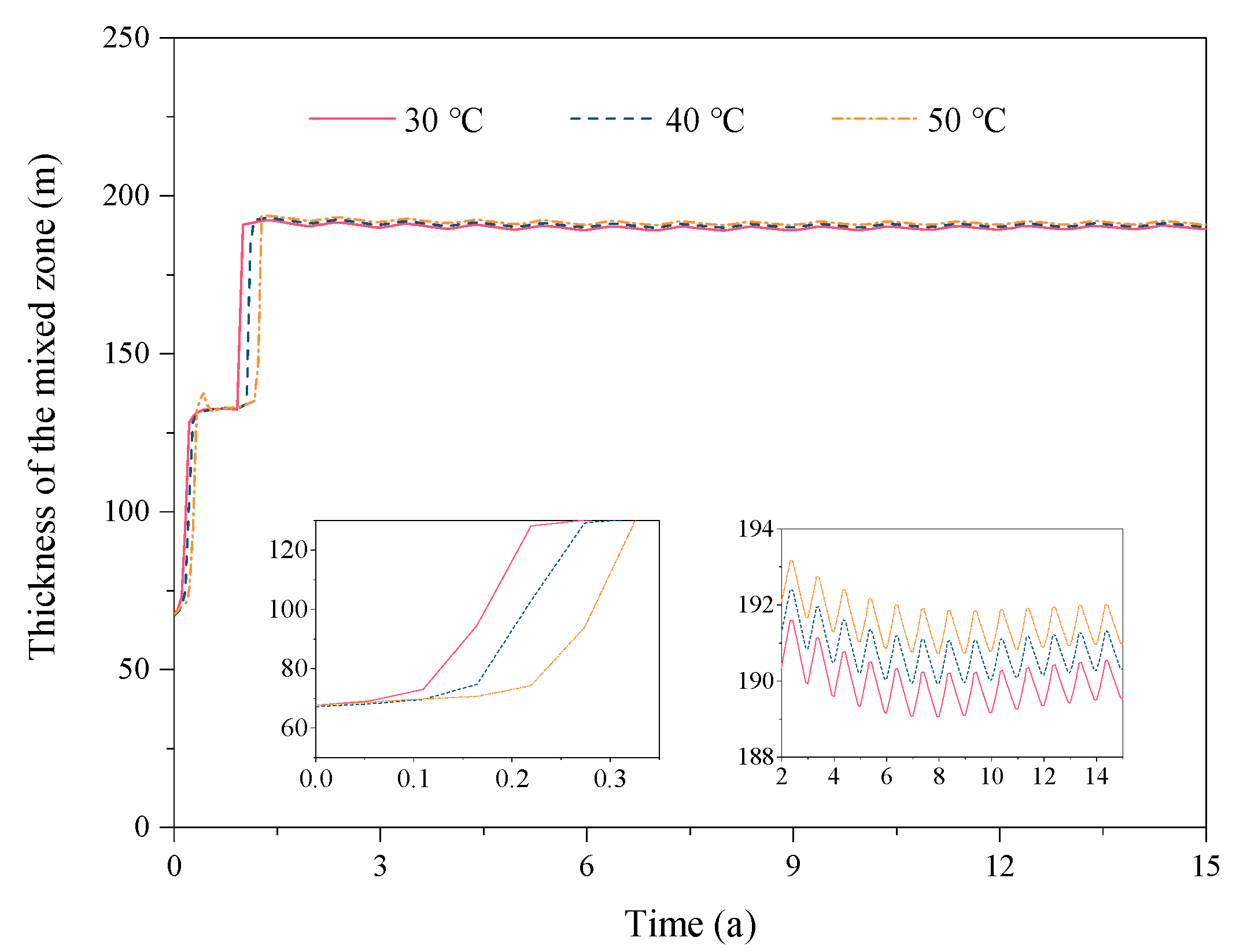
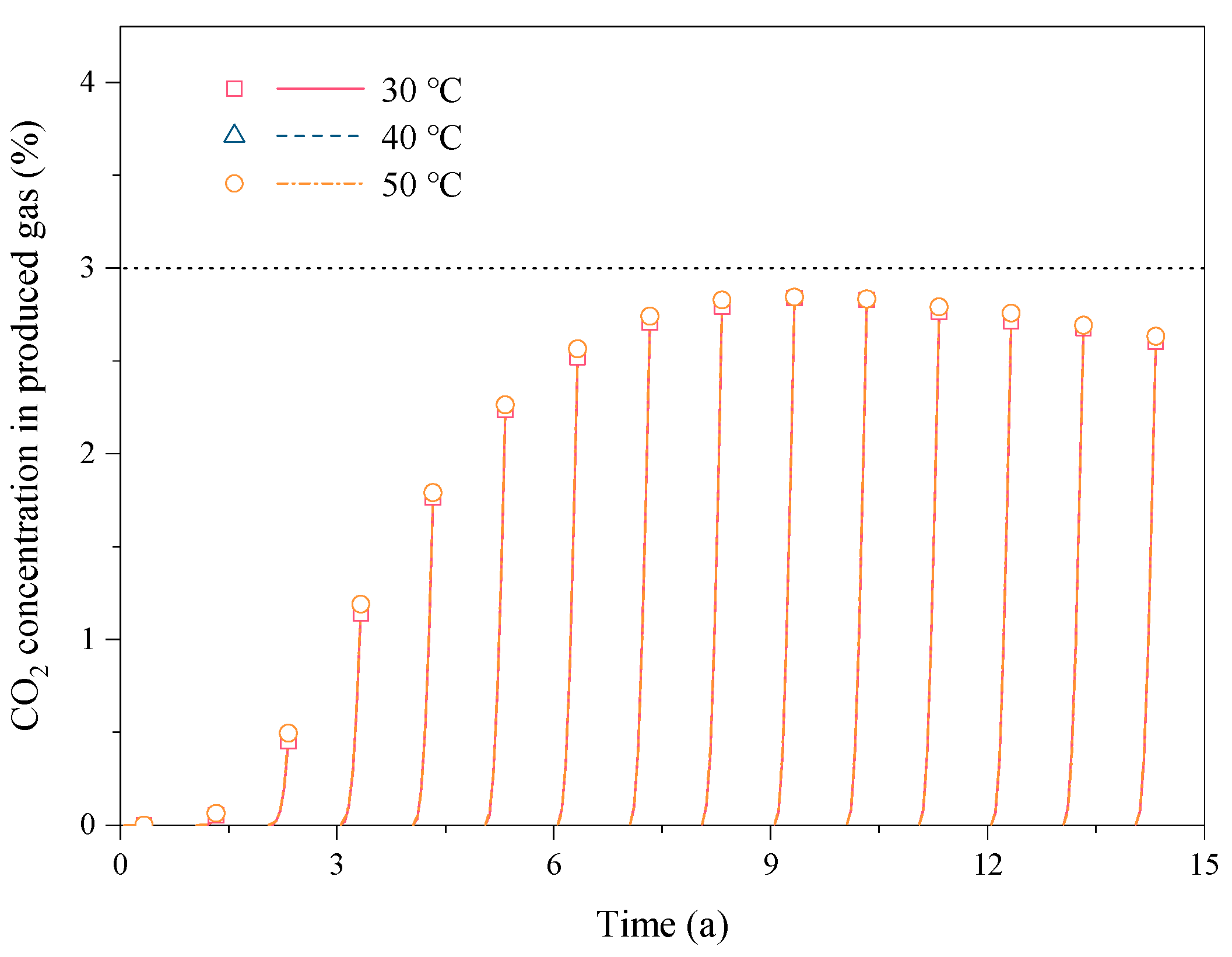
3.3. Effect of the Reservoir Temperature
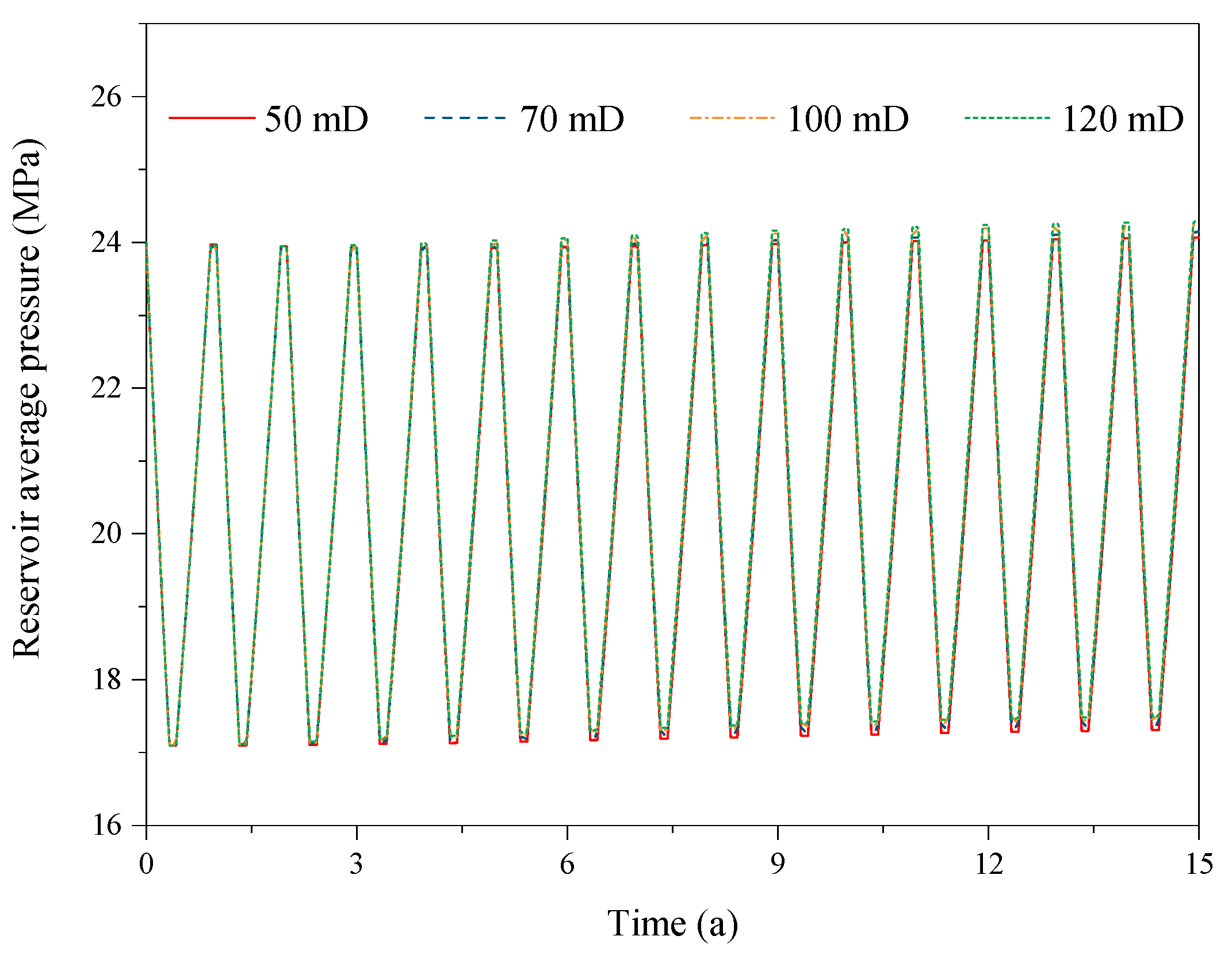
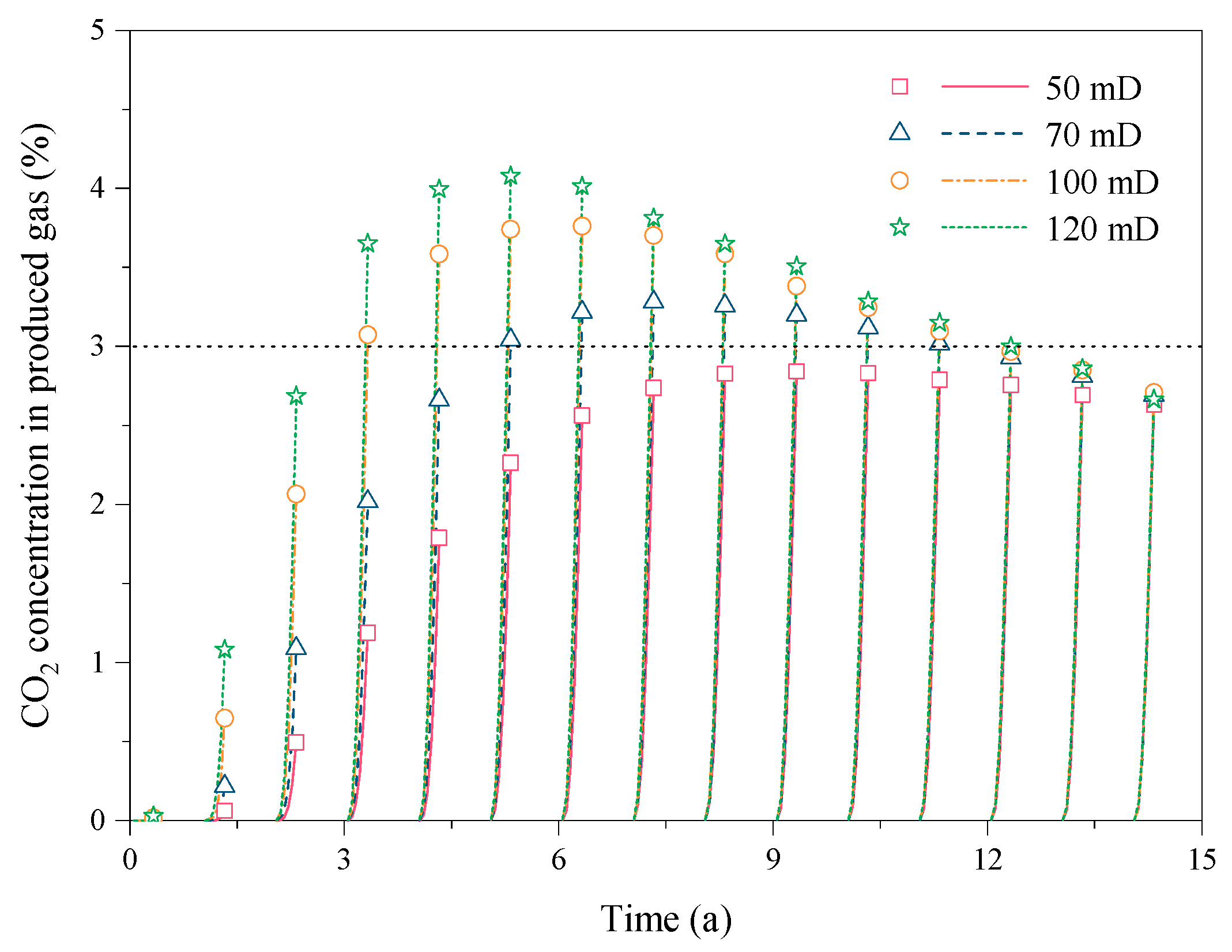
3.4. Effect of the Reservoir Permeability
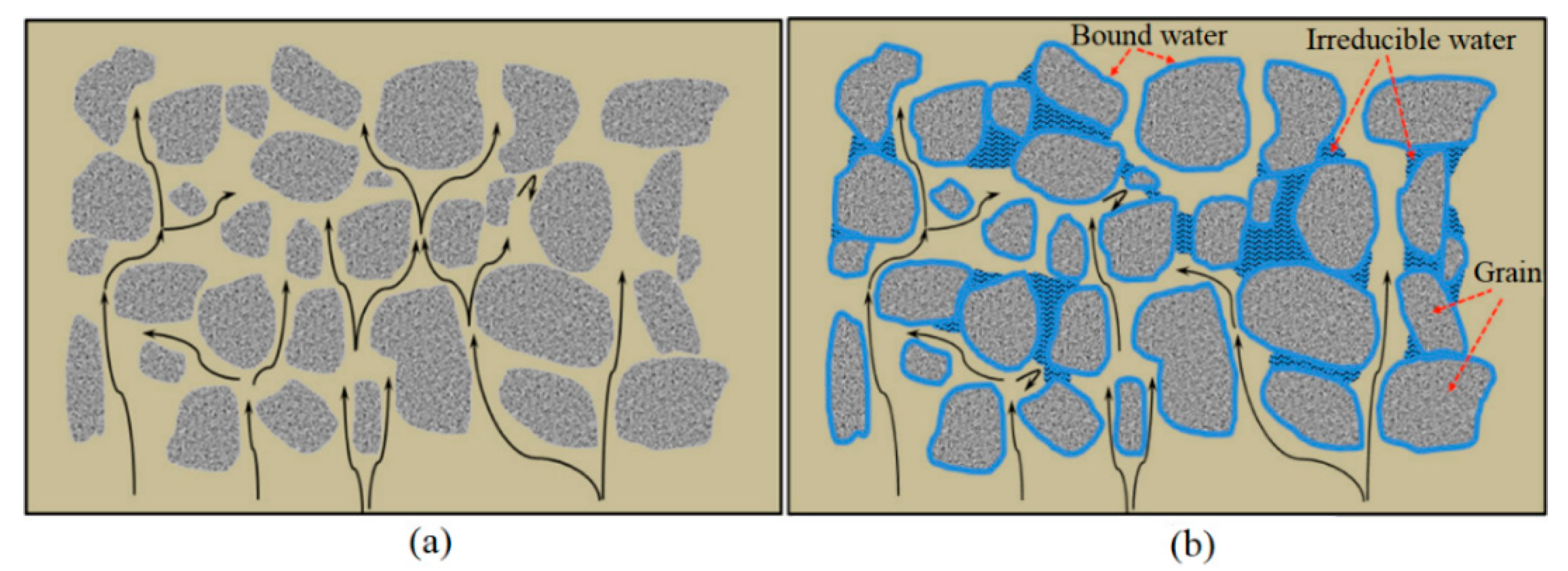
3.5. Effect of the Residual Water
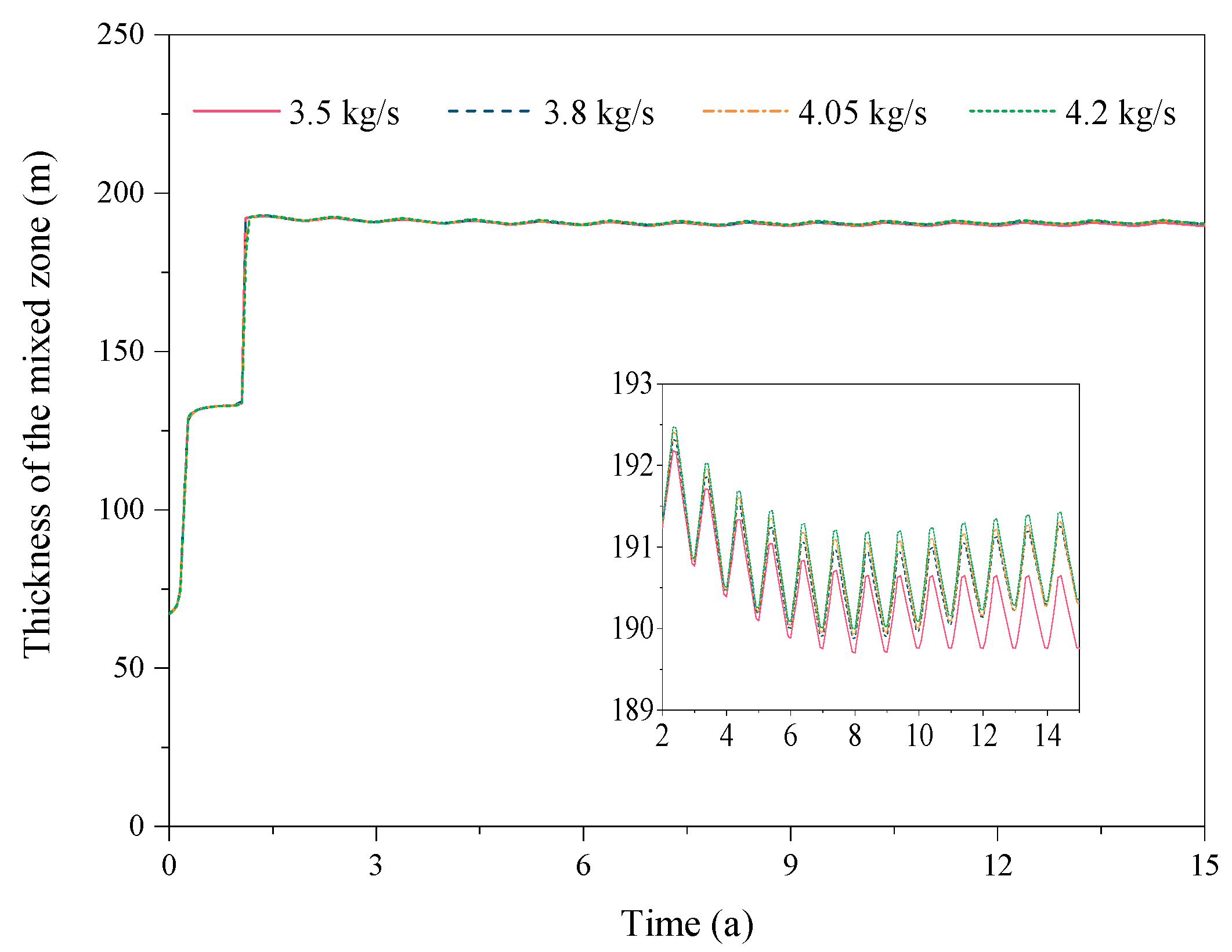
3.6. Effect of the Production Rate
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Katz, D.L.; Tek, M.R. Overview on underground storage of natural gas. J. Petrol. Technol. 1981, 33, 943–951. [Google Scholar] [CrossRef]
- Tek, M.R. Underground Storage of Natural Gas: Theory and Practice; Springer Science & Business Media: Berlin, Germany, 1989; Volume 171. [Google Scholar]
- Demirel, N.; Demirel, T.; Deveci, M.; Vardar, G. Location selection for underground natural gas storage using Choquet integral. J. Nat. Gas Sci. Eng. 2017, 45, 368–379. [Google Scholar] [CrossRef]
- Speight, J.G. Natural Gas: A Basic Handbook; Gulf Publishing Company: Houston, TX, USA, 2007. [Google Scholar]
- Wang, X.; Economides, M.J. Advanced Natural Gas Engineering; Gulf Publishing Company: Houston, TX, USA, 2009. [Google Scholar]
- Evans, D.J.; Chadwick, R.A. Underground Gas Storage: Worldwide Experiences and Future Development in the UK and Europe; Geological Society of London: London, UK, 2009. [Google Scholar]
- Laier, T. Results of monitoring groundwater above the natural gas underground storage at Stenlille, Denmark. Geol. Surv. Den. Greenl. Bull. 2012, 26, 45–48. [Google Scholar]
- Wang, X.; Economides, M.J. Purposefully built underground natural gas storage. J. Nat. Gas Sci. Eng. 2012, 9, 130–137. [Google Scholar] [CrossRef]
- Juez-Larré, J.; Remmelts, G.; Breunese, J.N.; van Gessel, S.F.; Leeuwenburgh, O. Using underground gas storage to replace the swing capacity of the giant natural gas field of Groningen in the Netherlands. A reservoir performance feasibility study. J. Pet. Sci. Eng. 2016, 145, 34–53. [Google Scholar] [CrossRef]
- Zhang, G.; Li, B.; Zheng, D.; Ding, G.; Wei, H.; Qian, P.; Li, C. Challenges to and proposals for underground gas storage (UGS) business in China. Nat. Gas Ind. B 2017, 4, 231–237. [Google Scholar] [CrossRef]
- Teatini, P.; Castelletto, N.; Ferronato, M.; Gambolati, G.; Janna, C.; Cairo, E.; Marzorati, D.; Colombo, D.; Ferretti, A.; Bagliani, A.; et al. Geomechanical response to seasonal gas storage in depleted reservoirs: A case study in the Po River basin, Italy. J. Geophys. Res. 2011, 116. [Google Scholar] [CrossRef]
- Oldenburg, C.M. Carbon dioxide as cushion gas for natural gas storage. Energy Fuels 2003, 17, 240–246. [Google Scholar] [CrossRef] [Green Version]
- Misra, B.R.; Foh, S.E.; Shikari, Y.A.; Berry, R.M.; Labaune, F. The use of inert base gas in underground natural gas storage. In Proceedings of the SPE Gas Technology Symposium, Dallas, TX, USA, 13–15 June 1988. [Google Scholar]
- Imre, A.R.; Ramboz, C.; Deiters, U.K.; Kraska, T. Anomalous fluid properties of carbon dioxide in the supercritical region: Application to geological CO2 storage and related hazards. Environ. Earth Sci. 2014, 73, 4373–4384. [Google Scholar] [CrossRef] [Green Version]
- Imre, A.R.; Groniewsky, A.; Györke, G.; Katona, A.; Velmovszki, D. Anomalous properties of some fluids—With high relevance in energy engineering—In their pseudo-critical (Widom) region. Period. Polytech. Chem. Eng. 2019, 63, 276–285. [Google Scholar] [CrossRef]
- Raju, M.; Banuti, D.T.; Ma, P.C.; Ihme, M. Widom lines in binary mixtures of supercritical fluids. Sci. Rep. 2017, 7, 3027. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ma, J.; Li, Q.; Kempka, T.; Kuehn, M. Hydromechanical response and impact of gas mixing behavior in subsurface CH4 storage with CO2-based cushion gas. Energy Fuels 2019, 33, 6527–6541. [Google Scholar] [CrossRef]
- Niu, C.; Tan, Y. Numerical simulation and analysis of migration law of gas mixture using carbon dioxide as cushion gas in underground gas storage reservoir. J. Harbin Inst. Technol. 2014, 21, 121–128. [Google Scholar]
- Oldenburg, C.M.; Pan, L. Utilization of CO2 as cushion gas for porous media compressed air energy storage. Greenh. Gases Sci. Technol. 2013, 3, 124–135. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.; Choi, J.; Park, K. Comparison of nitrogen and carbon dioxide as cushion gas for underground gas storage reservoir. Geosyst. Eng. 2015, 18, 163–167. [Google Scholar] [CrossRef]
- Pruess, K.; Battistelli, A. TMVOC, A Numerical Simulator for Three-Phase Non-Isothermal Flows of Multicomponent Hydrocarbon Mixtures in Saturated-Unsaturated Heterogeneous Media; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 2002.
- Pruess, K.; Oldenburg, C.M.; Moridis, G.J. TOUGH2 User’s Guide Version 2; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 1999.
- Honari, A.; Bijeljic, B.; Johns, M.L.; May, E.F. Enhanced gas recovery with CO2 sequestration: The effect of medium heterogeneity on the dispersion of supercritical CO2-CH4. Int. J. Greenh. Gas Control 2015, 39, 39–50. [Google Scholar] [CrossRef] [Green Version]
- Lee, J.H.; Park, Y.C.; Sung, W.M.; Lee, Y.S. A Simulation of a trap mechanism for the sequestration of CO2 into Gorae, V.; Aquifer, Korea. Energy Sour. Part A 2010, 32, 796–808. [Google Scholar] [CrossRef]
- Reagan, M. WebGasEOS v. 2.01; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 2012. Available online: http://esdtools.lbl.gov/gaseos/gaseos.html (accessed on 19 September 2019).
- Oldenburg, C.; Pruess, K.; Benson, S.M. Process modeling of CO2 injection into natural gas reservoirs for carbon sequestration and enhanced gas recovery. Energy Fuels 2001, 15, 293–298. [Google Scholar] [CrossRef]
- Seo, J.G. Experimental and Simulation Studies of Sequestration of Supercritical Carbon Dioxide in Depleted Gas Reservoirs; Texas A & M University: College Station, TX, USA, 2004. [Google Scholar]
- General Administration of Quality Supervision, Inspection and Quarantine of the China. Natural Gas, GB 17820—2012; Standardization Administration of the China: Beijing, China, 2012.
- Honari, A.; Zecca, M.; Vogt, S.J.; Iglauer, S.; Bijeljic, B.; Johns, M.L.; May, E.F. The impact of residual water on CH4-CO2 dispersion in consolidated rock cores. Int. J. Greenh. Gas Control 2016, 50, 100–111. [Google Scholar] [CrossRef]












| Parameters | Value |
|---|---|
| Porosity | 0.2 |
| Horizontal permeability (mD) | 50 |
| Vertical permeability (mD) | 10 |
| Gas saturation | 80% |
| Initial pressure (MPa) | 5.17 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, C.; Liao, J.; Hou, Z.; Xu, H.; Mehmood, F.; Wu, X. Utilization of CO2 as Cushion Gas for Depleted Gas Reservoir Transformed Gas Storage Reservoir. Energies 2020, 13, 576. https://doi.org/10.3390/en13030576
Cao C, Liao J, Hou Z, Xu H, Mehmood F, Wu X. Utilization of CO2 as Cushion Gas for Depleted Gas Reservoir Transformed Gas Storage Reservoir. Energies. 2020; 13(3):576. https://doi.org/10.3390/en13030576
Chicago/Turabian StyleCao, Cheng, Jianxing Liao, Zhengmeng Hou, Hongcheng Xu, Faisal Mehmood, and Xuning Wu. 2020. "Utilization of CO2 as Cushion Gas for Depleted Gas Reservoir Transformed Gas Storage Reservoir" Energies 13, no. 3: 576. https://doi.org/10.3390/en13030576
APA StyleCao, C., Liao, J., Hou, Z., Xu, H., Mehmood, F., & Wu, X. (2020). Utilization of CO2 as Cushion Gas for Depleted Gas Reservoir Transformed Gas Storage Reservoir. Energies, 13(3), 576. https://doi.org/10.3390/en13030576







