Performance Analysis of a Stirling Engine Hybrid Power System
Abstract
1. Introduction
2. Literature Review
2.1. Overview of the Stirling Engine Technology
- Operating conditions (pressure and rotational speed) and performance characteristics (overall efficiency, power, heat exchangers effectiveness, heat losses, and energy dissipation) [34,35], and other studies have focused on additional parameters such as concentration ratio, hot and cold temperature, working fluid, and absorber temperature in order to analyze a solar dish solution [36,37,38,39];
- Combined methodologies using the three parameters previously mention such as the optimization of geometric and operating parameters of a beta Stirling engine [40], the development of an experimental free-piston engine used in a micro combined heat and power system [41], and the implementation of a new control method related to a variable-speed operation of a dish Stirling system [42].
2.2. Utilization of Alternative Renewable Resources to Fuel Stirling Engines
2.3. Stirling Engine Modeling Approaches
2.4. Hybrid Power Systems Base on Stirling Engine Technology
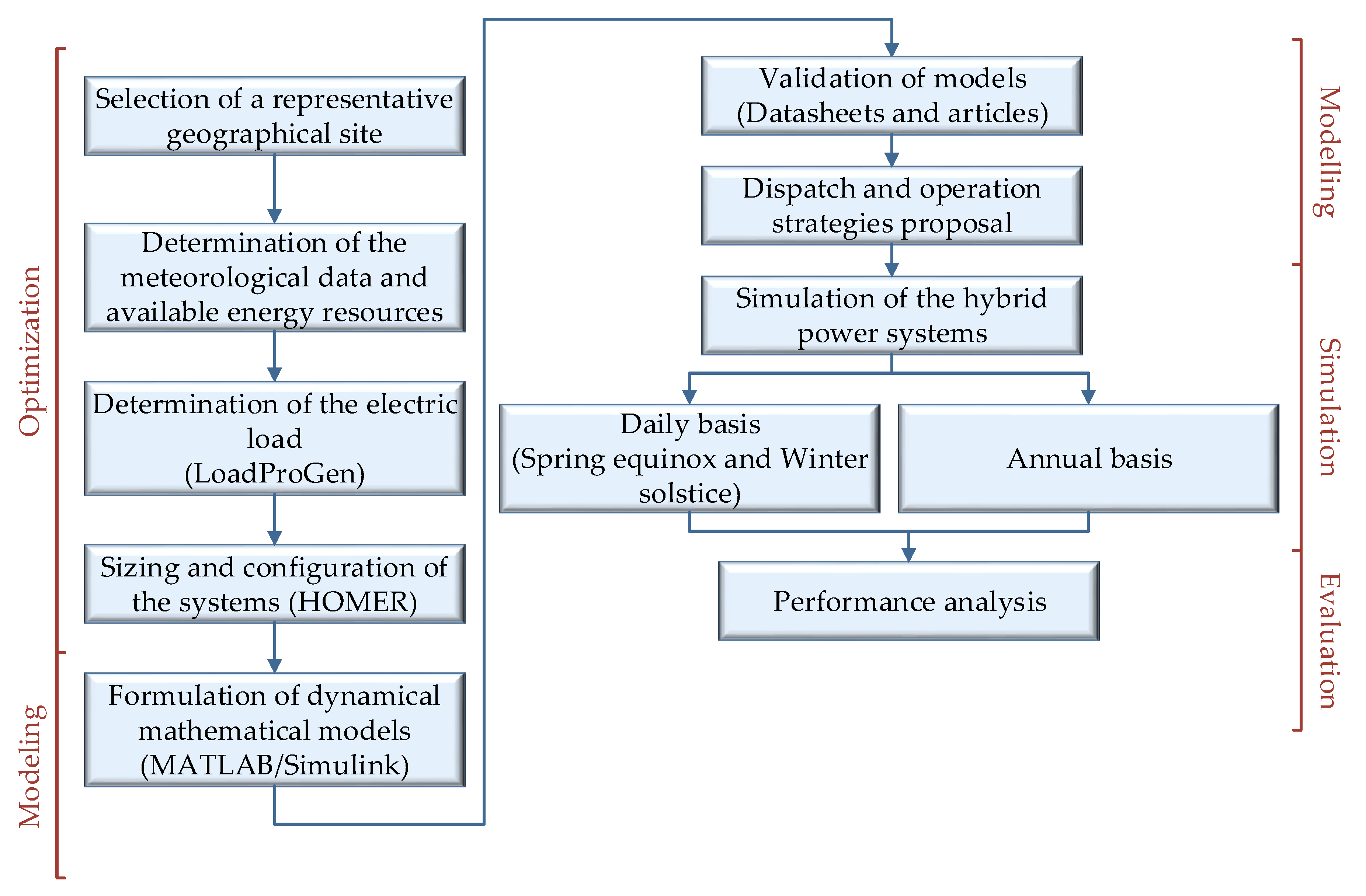
3. Objectives and Methodology
4. Economic Optimization
4.1. Geographical Site Selection
4.2. Primary Energy Sources
4.2.1. Solar Energy
4.2.2. Biomass Resources
4.2.3. Temperature
4.3. Load Characteristics
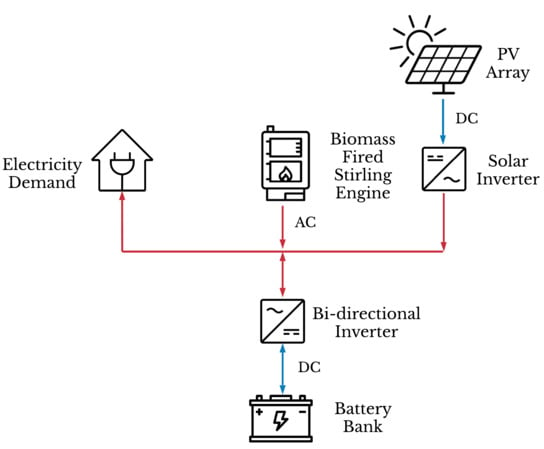
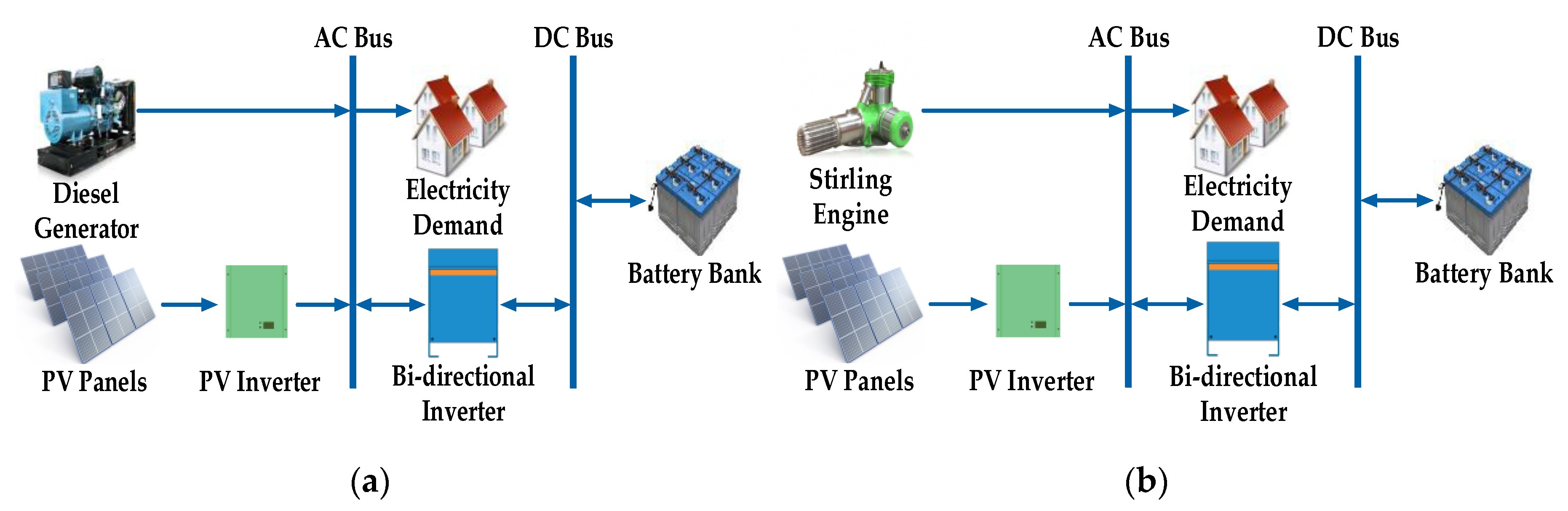
4.4. Configuration
4.5. Component Specifications and Inputs to HOMER
4.5.1. PV Array
4.5.2. Battery Bank
4.5.3. Converter
4.5.4. Diesel Engine Genset
4.5.5. CHP Plant with a Stirling Engine
4.5.6. Other Parameters
4.5.7. System Control
5. Modeling
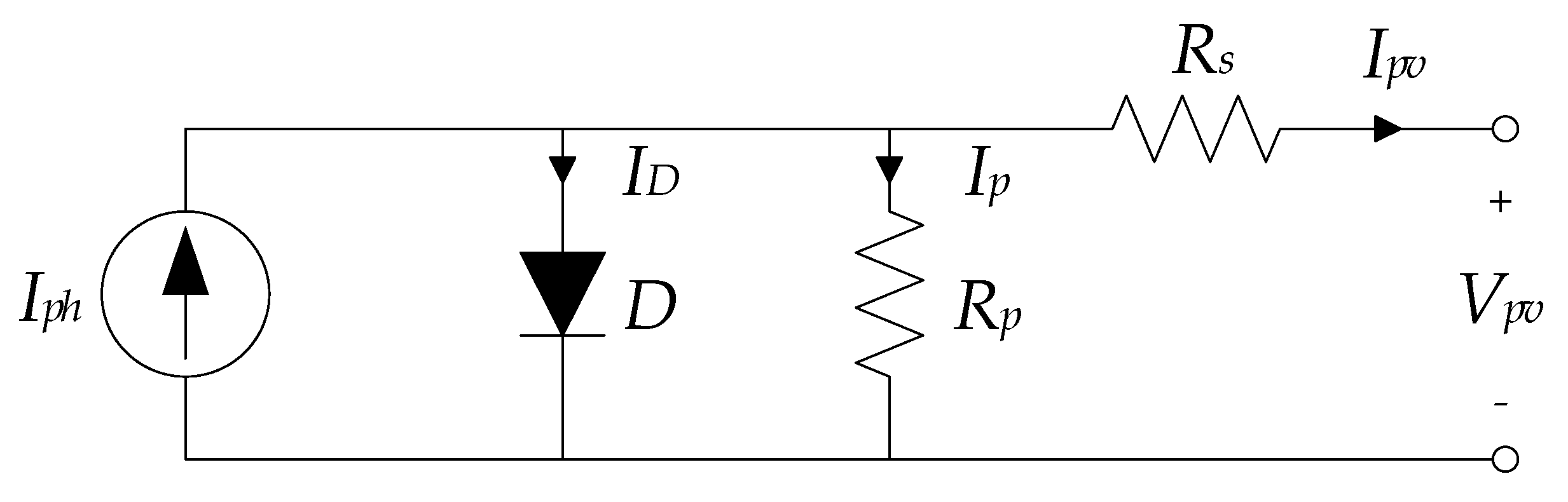
5.1. Photovoltaic Array
5.2. Battery Bank
5.3. Converter
5.4. Diesel Generator
5.4.1. Operation Modes
5.4.2. Fuel Consumption
5.4.3. Emissions
5.4.4. Simulation Parameters
5.5. Stirling Engine
5.5.1. Operation Modes
5.5.2. Fuel Consumption
5.5.3. Emissions
5.5.4. Simulation Parameters
5.6. Control Strategy
5.6.1. Load Following-Frugal Strategy
5.6.2. Cycle Charging-Frugal-SOC Setpoint Strategy
6. Performance Indicators
6.1. Energy Efficiency Evaluation
6.2. Economic Feasibility Evaluation
6.3. Environmental Sustainability Evaluation
6.4. Weighting Factor Method
7. Results and Discussion
7.1. Optimization Results
7.2. Dynamic Analysis
7.2.1. Simulation of PV/Diesel/Battery System during Winter Solstice
7.2.2. Simulation of PV/Stirling/Battery System during Winter Solstice
7.2.3. Comparison between MATLAB/Simulink and HOMER Models
7.3. Performance Analysis
8. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Nomenclature
| a | Ideality factor of the diode | Pgd_nom | Nominal power of diesel generator (W) |
| A | Exponential zone amplitude of battery (V) | Pgen_min | Minimum power recommended by manufacturer of diesel generator or Stirling engine (W) |
| ATCSR | Annualized total cost saving ratio (%) | Pgen_nom | Nominal power of diesel generator or Stirling engine (W) |
| ATCref_tot | Annualized total cost of reference system (USD/year) | Pinv_ac | Inverter output power (W) |
| ATCsis_tot | Annualized total cost of studied system (USD/year) | Pinv_dc | Inverter input power (W) |
| b0–b26 | Empirical coefficients related to the thermal efficiency of the Stirling engine | Pinv_nom | Inverter nominal power (W) |
| B | Exponential zone time constant inverse of battery (1/Ah) | Pmax_car | Maximum charge rate by batteries (W) |
| c0–c8 | Empirical coefficients for the mass flow of cooling water | Pmax_des | Maximum discharge rate by batteries (W) |
| cp | Specific heat of water (J/kg·K) | Pnet | Difference between PV power generated and demand of power (W) |
| CO2ERR | CO2 emission reduction ratio (%) | PSt | Output power of Stirling engine (W) |
| CO2ref_tot | Emissions from reference system (kg/year) | PSt_ee | Steady-state net electrical output of Stirling engine (W) |
| CO2sis_tot | Emissions from studied system (kg/year) | PST_nom | Stirling engine nominal electrical power (W) |
| CHX | Thermal capacitance of the cooling water control volume (J/K) | qgen | Gross heat rate of Stirling engine (J/s) |
| CSt | Thermal capacitance of Stirling engine (J/K) | qloss | Heat loss rate of Stirling engine (J/s) |
| Exp(t) | Voltage function of battery exponential zone (V) | qrec | Heat recovery rate of Stirling engine (J/s) |
| E0 | Battery voltage constant (V) | qSt_ee | Steady-state rate of heat generation related to Stirling engine (J/s) |
| FSR | Fuel Saving Ratio (%) | R | Drop of speed governor (Hz/pu) |
| Fref_tot | Equivalent energy related to fuel consumption by reference system (kWh) | Rbat | Internal resistance of battery (Ω) |
| Fsis_tot | Equivalent energy related to fuel consumption by studied system (kWh) | Rp | Shunt resistance of PV cell (Ω) |
| HD | Inertial constant of the rotor (pu s/Hz) | Rs | Series resistance of PV cell (Ω) |
| i* | Filtered battery current (A) | SOC | State of charge of batteries (%) |
| it | Current battery charge (Ah) | SOCmin | Minimum state of charge of batteries (%) |
| Ibat | Battery current (A) | SOCstp | SOC setpoint (%) |
| Io | Diode reverse saturation current (A) | Tamb | Ambient temperature (°C) |
| Iph | Photoelectric currtent (A) | Tcw_i | Inlet temperature of cooling water (°C) |
| Ipv | Output current of PV panel (A) | Tcw_o | Outlet temperature of cooling water (°C) |
| ISR | Integrated saving ratio (%) | TD | First order lag of diesel engine (s) |
| k0–k2 | Constants of efficiency of converter | TSM | First order lag of servomechanism of the valve (s) |
| kA–kB | Constants of the diesel consumption (L/kWh) | TST | Average temperature of Stirling engine (°C) |
| kp | Sensitivity coefficient of the result electrical power to Tst | TST_nom | Stirling engine nominal temperature of operation (°C) |
| K | Polarization constant of battery (V/Ah) | UAHX | Heat transfer coefficient of heat recovery (W/K) |
| KD | Damping coefficient of diesel generator | UAloss | Heat transfer coefficient of heat loss (W/K) |
| KI | Integral control gain of diesel generator | u(t) | Process of charge or discharge of battery |
| KS | Synchronization coefficient of diesel generator | Vbat | Battery voltage (V) |
| Ld | Critical discharge power (W) | Vth | Thermal voltage of PV cell (V) |
| LHVbiomass | Lower heating value of wood pellets (J/kg) | ηe | Electric efficiency of the Stirling engine in steady state mode |
| ṁdiesel | Diesel consumption flow (L/s) | ηinv | Inverter efficiency |
| ṁbiomass | Pellets consumption flow (kg/s) | ηq | Thermal efficiency of the Stirling engine in steady state mode |
| ṁcw | Mass flow of cooling water (kg/s) | w1–w3 | Weighted factors |
| Ncel | Number of cells in PV module | ωd | Generator velocity (rad/s) |
| Pdef | Deficit power (W) | xB | Temperature function of B |
| Pexc | Surplus power (W) | xE0 | Temperature function of E0 |
| Pgd | Power supplied by diesel generator (W) | xK | Temperature function of K |
References
- Khatib, H. Electricity in the global energy scene. IEE Proc. A Sci. Meas. Technol. 1993, 140, 24–28. [Google Scholar] [CrossRef]
- International Energy Agency (IEA) World Energy Outlook 2019—Analysis. Available online: https://www.iea.org/reports/world-energy-outlook-2019 (accessed on 25 December 2019).
- Agrawal, A.; Wies, R.; Johnson, R. Hybrid Electric Power Systems: Modeling, Optimization and Control; Omniscriptum Gmbh & Company: Saarbrücken, Germany, 2007; ISBN 978-3-8364-1966-6. [Google Scholar]
- Sigarchian, S.G.; Paleta, R.; Malmquist, A.; Pina, A. Feasibility study of using a biogas engine as backup in a decentralized hybrid (PV/wind/battery) power generation system–Case study Kenya. Energy 2015, 90, 1830–1841. [Google Scholar] [CrossRef]
- Balderrama, S.; Lombardi, F.; Riva, F.; Canedo, W.; Colombo, E.; Quoilin, S. A two-stage linear programming optimization framework fsor isolated hybrid microgrids in a rural context: The case study of the “El Espino” community. Energy 2019, 188, 116073. [Google Scholar] [CrossRef]
- Murphy, J.T. Making the energy transition in rural East Africa: Is leapfrogging an alternative? Technol. Forecast. Soc. Chang. 2001, 68, 173–193. [Google Scholar] [CrossRef]
- Pickering, B.; Choudhary, R. District energy system optimisation under uncertain demand: Handling data-driven stochastic profiles. Appl. Energy 2019, 236, 1138–1157. [Google Scholar] [CrossRef]
- World Bank. Sustainable Energy for All (SE4ALL) Access to Electricity, Rural Population—Bolivia; World Bank: Washington, DC, USA, 2017. [Google Scholar]
- Autoridad de Fiscalización y Control Social de Electricidad (AE). Anuario Estadístico. 2018. Available online: https://sawi.ae.gob.bo/docfly/app/webroot/uploads/IMG-ANUARIO-cpelaez-2019-09-16-Libro%20Anuario%20AE%202018%20FINAL_web.pdf (accessed on 19 December 2019).
- Inter-American Development Bank (IDB). Evaluación Financiera y Económica del Proyecto Electrificación Rural Con Energίa Renovable; Inter-American Development Bank (IDB): Washington, DC, USA, 2013. [Google Scholar]
- ENDE Guaracachi. Planta Solar Cobija. Available online: http://guaracachi.com.bo/index.php?option=com_content&view=article&id=48&Itemid=251 (accessed on 25 December 2019).
- Ministerio de Comunicación de Bolivia. Gobierno Lleva Energía eléctrica a Comunidad Guaraní “El Espino”, en el Chaco Cruceño. Available online: https://www.comunicacion.gob.bo/?q=20151022/19658 (accessed on 25 December 2019).
- SMA Energy Systems. Hybrid Energy Supply for the city of Cobija, Bolivia. Available online: https://www.sma.de/es/productos/referencias/cobija-bolivia.html (accessed on 19 December 2019).
- ENDE Corporación. Inauguración: Planta Solar Fotovoltaica de Cobija. Available online: https://www.ende.bo/noticia/noticia/7 (accessed on 25 December 2019).
- ENERSOL. Planta Solar el Espino. Available online: http://www.enersol-sa.com/planta-solar-el-espino (accessed on 20 December 2019).
- Ministerio de Hidrocarburos. Inaguración: Planta Híbrida Solar-Diésel “El Espino”. Available online: https://www3.hidrocarburos.gob.bo/index.php/comunicaci%C3%B3n/prensa/3418-presidente-morales-inaugura-planta-h%C3%ADbrida-solar-di%C3%A9sel-%E2%80%9Cel-espino%E2%80%9D-con-capacidad-de-60-kw.html (accessed on 25 December 2019).
- Corria, M.E.; Cobas, V.M.; Lora, E.S. Perspectives of Stirling engines use for distributed generation in Brazil. Energy Policy 2006, 34, 3402–3408. [Google Scholar] [CrossRef]
- Cardozo, E. Combustion of Agricultural Residues: Application for Stirling Micro-combined Heat and Power. Ph.D. Thesis, KTH Royal Institute of Technology, Stockholm, Sweden, 2014. [Google Scholar]
- Ljunggren Falk, H.; Berg, S. Implementation of a Stirling Engine Generation System for Residential Use in Rural Areas of Beni Department of Bolivia. Bachelor’s Thesis, KTH, School of Industrial Engineering and Management (ITM), Stockholm, Sweden, 2014. [Google Scholar]
- Arco Sola, J.; Nelson, O. Applications of Biomass Stirling Engines for Electrification-A Case Study of Rural Areas in Bolivia. Bachelor’s Thesis, KTH, School of Industrial Engineering and Management (ITM), Stockholm, Sweden, 2014. [Google Scholar]
- Kylili, A.; Christoforou, E.; Fokaides, P.A. Environmental evaluation of biomass pelleting using life cycle assessment. Biomass Bioenergy 2016, 84, 107–117. [Google Scholar] [CrossRef]
- Núñez, C.A.F.; Fajardo, C.A.G.; Vargas, F.E.S. Producción y uso de pellets de biomasa para la generación de energía térmica: Una revisión a los modelos del proceso de gasificación. Iteckne 2012, 9, 21–30. [Google Scholar]
- Walker, G. Stirling Engines; Clarendon Press: Oxford, UK, 1980; ISBN 978-0-19-856209-2. [Google Scholar]
- Martini, W.R. Stirling Engine Design Manual; University Press of the Pacific: Honolulu, Hawaii, 2004; ISBN 978-1-4102-1604-5. [Google Scholar]
- Iwamoto, S.; Hirata, K.; Toda, F. Performance of Stirling Engines. JSME Int. J. Ser. B 2001, 44, 140–147. [Google Scholar] [CrossRef]
- Thombare, D.G.; Verma, S.K. Technological development in the Stirling cycle engines. Renew. Sustain. Energy Rev. 2008, 12, 1–38. [Google Scholar] [CrossRef]
- Staffell, I.; Brett, D.J.L.; Brandon, N.P.; Hawkes, A.D. Domestic Microgeneration: Renewable and Distributed Energy Technologies, Policies and Economics; Routledge: Abingdon, UK, 2015; ISBN 978-1-317-44884-6. [Google Scholar]
- Sultan, U. Stirling Engine Design Methodology with Advanced Optimization Models: Thermodynamics and CFD Analysis; LAP LAMBERT Academic Publishing: Saarbrücken, Germany, 2017. [Google Scholar]
- Valenti, G.; Silva, P.; Fergnani, N.; Campanari, S.; Ravidà, A.; Di Marcoberardino, G.; Macchi, E. Experimental and numerical study of a micro-cogeneration Stirling unit under diverse conditions of the working fluid. Appl. Energy 2015, 160, 920–929. [Google Scholar] [CrossRef]
- Shaneb, O.A.; Coates, G.; Taylor, P.C. Sizing of residential μCHP systems. Energy Build. 2011, 43, 1991–2001. [Google Scholar] [CrossRef]
- Zarinchang, J.; Yarmahmoudi, A. Optimization of thermal components in a Stirling engine. WSEA Trans. Heat Mass Transf. 2009, 1, 1–10. [Google Scholar]
- Kongtragool, B.; Wongwises, S. Thermodynamic analysis of a Stirling engine including dead volumes of hot space, cold space and regenerator. Renew. Energy 2006, 31, 345–359. [Google Scholar] [CrossRef]
- Costa, S.-C.; Tutar, M.; Barreno, I.; Esnaola, J.-A.; Barrutia, H.; García, D.; González, M.-A.; Prieto, J.-I. Experimental and numerical flow investigation of Stirling engine regenerator. Energy 2014, 72, 800–812. [Google Scholar] [CrossRef]
- Rogdakis, E.D.; Antonakos, G.D.; Koronaki, I.P. Thermodynamic analysis and experimental investigation of a Solo V161 Stirling cogeneration unit. Energy 2012, 45, 503–511. [Google Scholar] [CrossRef]
- Puech, P.; Tishkova, V. Thermodynamic analysis of a Stirling engine including regenerator dead volume. Renew. Energy 2011, 36, 872–878. [Google Scholar] [CrossRef]
- Ferreira, A.C.; Nunes, M.L.; Teixeira, J.C.; Martins, L.A.; Teixeira, S.F. Thermodynamic and economic optimization of a solar-powered Stirling engine for micro-cogeneration purposes. Energy 2016, 111, 1–17. [Google Scholar] [CrossRef]
- Ferreira, A.C.; Teixeira, S.; Teixeira, J.C.; Martins, L.B. Design optimization of a solar dish collector for its application with stirling engines. In Proceedings of the ASME 2015 International Mechanical Engineering Congress and Exposition; American Society of Mechanical Engineers Digital Collection, Houston, TX, USA, 13–19 November 2015. [Google Scholar]
- Arora, R.; Kaushik, S.C.; Kumar, R.; Arora, R. Multi-objective thermo-economic optimization of solar parabolic dish Stirling heat engine with regenerative losses using NSGA-II and decision making. Int. J. Electr. Power Energy Syst. 2016, 74, 25–35. [Google Scholar] [CrossRef]
- Barreto, G.; Canhoto, P. Modelling of a Stirling engine with parabolic dish for thermal to electric conversion of solar energy. Energy Convers. Manag. 2017, 132, 119–135. [Google Scholar] [CrossRef]
- Ahmed, F.; Hulin, H.; Khan, A.M. Numerical modeling and optimization of beta-type Stirling engine. Appl. Therm. Eng. 2019, 149, 385–400. [Google Scholar] [CrossRef]
- Zhu, S.; Yu, G.; Jongmin, O.; Xu, T.; Wu, Z.; Dai, W.; Luo, E. Modeling and experimental investigation of a free-piston Stirling engine-based micro-combined heat and power system. Appl. Energy 2018, 226, 522–533. [Google Scholar] [CrossRef]
- Li, Y.; Xiong, B.; Su, Y.; Tang, J.; Leng, Z. Particle swarm optimization-based power and temperature control scheme for grid-connected DFIG-based dish-stirling solar-thermal system. Energies 2019, 12, 1300. [Google Scholar] [CrossRef]
- Ferreira, A.C.; Silva, J.; Teixeira, S.; Teixeira, J.C.; Nebra, S. Analysis of the different renewable energy sources in the performance of a Stirling engine. In Proceedings of the 32nd International Conference on Efficiency, Cost, Optimization, Simulation and Environmental Impact of Energy Systems, Institute of Thermal Technology. Wroclaw, Poland, 23–28 June 2019; pp. 2367–2379. [Google Scholar]
- Hachem, H.; Gheith, R.; Aloui, F.; Nasrallah, S.B. Technological challenges and optimization efforts of the Stirling machine: A review. Energy Convers. Manag. 2018, 171, 1365–1387. [Google Scholar] [CrossRef]
- Bataineh, K.M. Optimization analysis of solar-powered average temperature Stirling heat engine. J. Energy S. Afr. 2015, 26, 55–66. [Google Scholar] [CrossRef]
- Grasse, W.; Hertlein, H.P.; Winter, C.-J.; Braun, G.W. Thermal solar power plants experience. In Solar Power Plants; Springer: Berlin, Germany, 1991; pp. 215–282. [Google Scholar]
- Entchev, E.; Gusdorf, J.; Swinton, M.; Bell, M.; Szadkowski, F.; Kalbfleisch, W.; Marchand, R. Micro-generation technology assessment for housing technology. Energy Build. 2004, 36, 925–931. [Google Scholar] [CrossRef]
- Cardozo, E.; Erlich, C.; Malmquist, A.; Alejo, L. Integration of a wood pellet burner and a Stirling engine to produce residential heat and power. Appl. Therm. Eng. 2014, 73, 671–680. [Google Scholar] [CrossRef]
- Dong, L.; Liu, H.; Riffat, S. Development of small-scale and micro-scale biomass-fuelled CHP systems–A literature review. Appl. Therm. Eng. 2009, 29, 2119–2126. [Google Scholar] [CrossRef]
- Maraver, D.; Sin, A.; Royo, J.; Sebastián, F. Assessment of CCHP systems based on biomass combustion for small-scale applications through a review of the technology and analysis of energy efficiency parameters. Appl. Energy 2013, 102, 1303–1313. [Google Scholar] [CrossRef]
- Obernberger, I. Trends and opportunities of micro-CHP technologies based on biomass combustion. In Proceedings of the 18th European Biomass Conference, Lyon, France, 3–7 May 2010; pp. 1–9. [Google Scholar]
- Liu, H. Biomass fuels for small and micro combined heat and power (CHP) systems: Resources, conversion and applications. In Small and Micro Combined Heat and Power (CHP) Systems; Elsevier: Amsterdam, The Netherlands, 2011; pp. 88–122. [Google Scholar]
- Gliński, M.; Bojesen, C.; Rybiński, W.; Bykuć, S. Modelling of the Biomass mCHP Unit for Power Peak Shaving in the Local Electrical Grid. Energies 2019, 12, 458. [Google Scholar] [CrossRef]
- ÖkoFEN. Pellematic Condens_e Project. Available online: http://www.okofen-e.com/en/pellematic_condens_e/ (accessed on 31 January 2020).
- Inresol, A.B. Company Introduction and Current V2-6 Products. Available online: https://energiforskmedia.blob.core.windows.net/media/22643/4-inresol-introduction-and-products.pdf (accessed on 31 January 2020).
- Araoz, J.; Platell, P.; Larsson-Mastonstråle, S. Design Analysis and Control of the V2-6 Stirling Engine. Available online: https://www.researchgate.net/publication/338431073_Design_analysis_and_control_of_the_V2-6_Stirling_engine (accessed on 25 December 2019).
- Beausoleil-Morrison, I.; Kelly, N. Specifications for Modelling Fuel Cell and Combustion-Based Residential Cogeneration Device within Whole-Building Simulation Programs; International Energy Agency: Paris, France, 2007; ISBN 978-0-662-47116-5.
- Thiers, S.; Aoun, B.; Peuportier, B. Experimental characterization, modeling and simulation of a wood pellet micro-combined heat and power unit used as a heat source for a residential building. Energy Build. 2010, 42, 896–903. [Google Scholar] [CrossRef]
- Veitch, D.; Mahkamov, K. Assessment of economical and ecological benefits from deployment of a domestic combined heat and power unit based on its experimental performance. Proc. Inst. Mech. Eng. A J. Power 2009, 223, 783–798. [Google Scholar] [CrossRef]
- Alexakis, A.; Gounis, G.; Mahkamov, K.; Davis, J. Experimental and Theoretical Evaluation of the Performance of a Whispergen Mk Vb micro CHP Unit in Typical UK House Conditions. In Proceedings of the World Renewable Energy Congress 2011, World Renewable Energy Congress 2011, Linköping, Sweden, 8–13 May 2011; pp. 810–817. [Google Scholar]
- Conroy, G.; Duffy, A.; Ayompe, L.M. Validated dynamic energy model for a Stirling engine μ-CHP unit using field trial data from a domestic dwelling. Energy Build. 2013, 62, 18–26. [Google Scholar] [CrossRef]
- Ferguson, A.; Kelly, N.; Weber, A.; Griffith, B. Modelling residential-scale combustion-based cogeneration in building simulation. J. Build. Perform. Simul. 2009, 2, 1–14. [Google Scholar] [CrossRef]
- Lombardi, K.; Ugursal, V.I.; Beausoleil-Morrison, I. Proposed improvements to a model for characterizing the electrical and thermal energy performance of Stirling engine micro-cogeneration devices based upon experimental observations. Appl. Energy 2010, 87, 3271–3282. [Google Scholar] [CrossRef]
- Ulloa, C.; Míguez, J.L.; Porteiro, J.; Eguía, P.; Cacabelos, A. Development of a Transient Model of a Stirling-Based CHP System. Energies 2013, 6, 3115–3133. [Google Scholar] [CrossRef]
- Schulz, S.; Schwendig, F. A General Simulation Model for Stirling Cycles. J. Eng. Gas Turbines Power 1996, 118, 1–7. [Google Scholar] [CrossRef]
- Kongtragool, B.; Wongwises, S. A review of solar-powered Stirling engines and low temperature differential Stirling engines. Renew. Sustain. Energy Rev. 2003, 7, 131–154. [Google Scholar] [CrossRef]
- Organ, A.J. The Regenerator and the Stirling Engine; Wiley: Hoboken, NJ, USA, 1997; ISBN 978-1-86058-010-9. [Google Scholar]
- Mahkamov, K. Design improvements to a biomass stirling engine using mathematical analysis and 3D CFD modeling. J. Energy Resour. Technol. Trans. ASME 2006, 128, 203–215. [Google Scholar] [CrossRef]
- Shih, H.-J. An analysis model combining gamma-type stirling engine and power converter. Energies 2019, 12, 1322. [Google Scholar] [CrossRef]
- Bouvenot, J.-B. Etudes Expérimentales et Numériques de Systèmes de Micro Cogénération Couplés Aux Bâtiments D’habitation et au Réseau Électrique. Ph.D. Thesis, Université de Strasbourg, Strasbourg, France, 2015. [Google Scholar]
- Balcombe, P.; Rigby, D.; Azapagic, A. Environmental impacts of microgeneration: Integrating solar PV, Stirling engine CHP and battery storage. Appl. Energy 2015, 139, 245–259. [Google Scholar] [CrossRef]
- Balcombe, P.; Rigby, D.; Azapagic, A. Energy self-sufficiency, grid demand variability and consumer costs: Integrating solar PV, Stirling engine CHP and battery storage. Appl. Energy 2015, 155, 393–408. [Google Scholar] [CrossRef]
- Shariatpanah, H.; Zareian Jahromi, M.; Fadaeinedjad, R. Simulation of a new grid-connected hybrid generation system with Stirling engine and wind turbine. J. Renew. Sustain. Energy 2013, 5, 063128. [Google Scholar] [CrossRef]
- Kadri, Y.; Abdallah, H.H. New grid connected hybrid generation system using wind turbine and solar Dish/Stirling engine. In Proceedings of the 2017 International Conference on Green Energy Conversion Systems (GECS), Hammamet, Tunisia, 23–25 March 2017; pp. 1–7. [Google Scholar]
- Rahman, A.; Saikia, L.C.; Sinha, N. Automatic generation control of an interconnected two-area hybrid thermal system considering dish-stirling solar thermal and wind turbine system. Renew. Energy 2017, 105, 41–54. [Google Scholar] [CrossRef]
- Jimenez Zabalaga, P.; Choque, L.; Cardozo, E.; Araoz, A. Performance Analysis of a Stirling Engine Hybrid Power System. In Proceedings of the 32nd International Conference on Efficiency, Cost, Optimization, Simulation and Environmental Impact of Energy Systems, ECOS 2019, Wroclaw, Poland, 23–28 June 2019; pp. 1095–1114. [Google Scholar]
- Mandelli, S.; Merlo, M.; Colombo, E. Novel procedure to formulate load profiles for off-grid rural areas. Energy Sustain. Dev. 2016, 31, 130–142. [Google Scholar] [CrossRef]
- Farret, F.A.; Simoes, M.G. Integration of Renewable Sources of Energy; John Wiley & Sons: Hoboken, NJ, USA, 2017; ISBN 978-1-119-13736-8. [Google Scholar]
- HOMER Energy LLC Welcome to HOMER. Available online: https://www.homerenergy.com/products/pro/docs/latest/index.html (accessed on 21 December 2019).
- Lambert, T.; Gilman, P.; Lilienthal, P. Micropower system modeling with HOMER. In Integration Alternative Sources Energy; John Wiley & Sons: Hoboken, NJ, USA, 2006; Volume 1, pp. 379–418. ISBN 978-0-471-75562-3. [Google Scholar]
- Engineers in Action. Active Projects. Available online: https://engineersinaction.org/2016/08/25/tacuaral-del-matto/ (accessed on 10 December 2019).
- Instituto Nacional de Estadística (INE). Censo Nacional de Población y Vivienda 2012. Available online: http://datos.ine.gob.bo/binbol/RpWebEngine.exe/Portal?BASE=CPV2012COM&lang=ESP (accessed on 12 December 2019).
- Meteotest. Meteonorm v.7.1.3. Available online: https://meteonorm.com/en/changelog (accessed on 12 December 2019).
- Lucano, M.; Fuentes, I.; Avilés, S. Mapa Solar de Bolivia. Available online: http://www.energetica.org.bo/energetica/publicacionesd.asp?d=278 (accessed on 18 December 2019).
- United Nations Department of Economic and Social Affairs (UNDESA); Fernández, M.; Rodriguez, G.; Orellano, R.; Terrazas, E. Cambio Climático, Agua y Energía en Bolivia. Sustainable Development Knowledge Platform. Available online: https://sustainabledevelopment.un.org/index.php?page=view&type=400&nr=1626&menu=1515 (accessed on 18 December 2019).
- Plan Para el Desarrollo de Las Energías Alternativas 2025. Available online: https://www.bivica.org/file/view/id/4689 (accessed on 19 December 2019).
- Fernández, M.; Saavedra, M. Bolivia. Available online: http://www.fao.org/3/T2363s/t2363s0q.htm (accessed on 19 December 2019).
- Gobierno Municipal Autónomo de San Borja. Plan de Desarrollo Municipal (PDM) 2007–2011; CICO-Beni: Beni, Bolivia, 2006.
- Hallberg, M.; Hallme, E. Energy Demand in Different Topographical Zones: A Field Study about the Domestic Energy Demand in the Rural Areas of Bolivia. Bachelor’s Thesis, KTH, School of Industrial Engineering and Management (ITM), Stockholm, Sweden, 2015. [Google Scholar]
- Subieta, S.L.B.; Tarantino, A.; Sabatini, S.; Riva, F.; Bonamini, G.; Quoilin, S. Feasibility Study of PV & Li-Ion Battery Based Micro-Grids for Bolivian Off-Grid Communities; EUROSOLAR: Düsseldorf, Germany, 2017. [Google Scholar]
- Toledo, E.; Arteaga, J.L. Proyecto de Electrificación Rural “Central Caranavi; Gobierno Municipal de Caranavi: La Paz, Bolivia, 2008.
- Reddy, Y.J.; Kumar, Y.V.P.; Raju, K.P.; Ramsesh, A. Retrofitted hybrid power system design with renewable energy sources for buildings. IEEE Trans. Smart Grid 2012, 3, 2174–2187. [Google Scholar] [CrossRef]
- Palizban, O.; Mekhilef, S. Hybrid Systems Control: With Renewable Energy Sources; LAP LAMBERT Academic Publishing: Saarbrücken, Germany, 2012; ISBN 978-3-659-15846-9. [Google Scholar]
- TRINA SOLAR. Módulo TSM-PD14. Available online: http://static.trinasolar.com/sites/default/files/LA_TSM_PD14_datasheet.pdf (accessed on 20 December 2019).
- Balfour, J.R.; Shaw, M.; Nash, N.B. Advanced Photovoltaic Installations; Jones & Bartlett Publishers: Burlington, MA, USA, 2011; ISBN 978-1-4496-2471-2. [Google Scholar]
- Birhuett García, E. Aprovechamiento de la Energía Solar Entre los Trópicos: Energía Fotovoltaica Para Ingenieros; Energética: Cochabamba, Bolivia, 2016. [Google Scholar]
- HOMER Energy LCC. PV Derating Factor. Available online: https://www.homerenergy.com/products/pro/docs/3.10/pv_derating_factor.html (accessed on 26 December 2019).
- Victron Energy. Gel and AGM Batteries. Available online: https://www.victronenergy.com/upload/documents/Datasheet-GEL-and-AGM-Batteries-EN.pdf (accessed on 20 December 2019).
- Hernández Arrondo, M. Análisis de Factibilidad de la Implantación de Sistemas Aislados Híbridos Eólicos-Diesel en Comunidades Rurales Remotas de Bolivia. Diseño de un Sistema de Este tipo en Una Comunidad Boliviana en la Que Resulte Factible. Master’s Thesis, Universidad Pública de Navarra, Pamplona, España, 2012. [Google Scholar]
- HOMER Energy LCC. Converter. Available online: https://www.homerenergy.com/products/pro/docs/3.10/converter.html (accessed on 27 December 2019).
- Victron Energy. MultiPlus Inverter/Chargers 2 kVA/3 kVA. Available online: https://www.victronenergy.com/upload/documents/Datasheet-Multiplus-inverter-charger_2kVA-and-3kVA-120V-US-EN.pdf (accessed on 20 December 2019).
- Finning International Inc. Technical Information C1.5 Diesel Generator Set 2017; Finning International Inc.: Vancouver, BC, Canada, 2017. [Google Scholar]
- KIPOR. Diesel Generator Maintenance Manual. Available online: http://kipor.es/uploads/_productos_kipor/id37/MANUAL_KDE7000.pdf (accessed on 30 November 2019).
- HOMER Energy LCC Fuels. Available online: https://www.homerenergy.com/products/pro/docs/3.10/fuels.html (accessed on 28 December 2019).
- Swaminathan, R. Cost effective, low capacity, biomass fired power plant. Energy Power 2013, 3, 1–6. [Google Scholar]
- Sugden, B.; Drury, T. Investigation into the viability of replacing internal combustion diesel generators with diesel fired stirling engine generators for remote microwave radio telecommunication applications. In Proceedings of the IEEE Intelec 2012, Scottsdale, AZ, USA, 30 September–4 October 2012; pp. 1–8. [Google Scholar]
- Inresol AB GENIUS: 10 kW PEAK POWER CHP MULTI FUEL STIRLING. Available online: http://www.skargardarnasriksforbund.se/document/2015-05-12_14314317931.pdf (accessed on 20 December 2019).
- Hawkes, A.; Leach, M. Comparison of Fuel Cell and Combustion Micro-CHP under Future Residential Energy Demand Scenarios; Imperial College Centre for Energy Policy and Technology (ICEPT): London, UK, 2007. [Google Scholar]
- Walker, S. Potential for Microgeneration: Study and Analysis; Department of Trade and Industry: London, UK, 2005.
- Beith, R. Small and Micro Combined Heat and Power (CHP) Systems: Advanced Design, Performance, Materials and Applications; Elsevier: Amsterdam, The Netherlands, 2011; ISBN 978-0-85709-275-5. [Google Scholar]
- Inresol, A.B. Data sheet: V2-6 Stirling engine. Available online: www.inresol.se (accessed on 22 December 2018).
- Cardozo, E.; Erlich, C.; Alejo, L.; Fransson, T.H. Combustion of agricultural residues: An experimental study for small-scale applications. Fuel 2014, 115, 778–787. [Google Scholar] [CrossRef]
- Vargas, N. Emprendimientos: Los Magos de la Cáscara de Castaña. Available online: https://mydokument.com/editor-marco-zelaya.html (accessed on 12 December 2019).
- Viceministerio de Electricidad y Energías Alternativas (VMEEA). Manual de Elaboración y Evaluación de Proyectos de Electrificación Rural; Imprenta Sagitario: La Paz, Bolivia, 2014. [Google Scholar]
- Banco Central de Bolivia (BCB). Indicadores de Inflación Anual. Available online: https://www.bcb.gob.bo/?q=indicadores_inflacion (accessed on 20 December 2019).
- HOMER Energy LCC. Cycle Charging. Available online: https://www.homerenergy.com/products/pro/docs/3.10/cycle_charging.html (accessed on 29 December 2019).
- HOMER Energy LCC. Load Following. Available online: https://www.homerenergy.com/products/pro/docs/3.10/load_following.html (accessed on 29 December 2019).
- Duffie, J.A.; Beckman, W.A. Solar Engineering of Thermal Processes; John Wiley & Sons: Hoboken, NJ, USA, 2013; ISBN 0-470-87366-3. [Google Scholar]
- Villalva, M.G.; Gazoli, J.R.; Ruppert Filho, E. Comprehensive approach to modeling and simulation of photovoltaic arrays. IEEE Trans. Power Electron. 2009, 24, 1198–1208. [Google Scholar] [CrossRef]
- Virtuani, A.; Lotter, E.; Powalla, M. Performance of Cu (In, Ga) Se2 solar cells under low irradiance. Thin Solid Film. 2003, 431, 443–447. [Google Scholar] [CrossRef]
- Jena, D.; Ramana, V.V. An accurate modeling of photovoltaic system for uniform and non-uniform irradiance. Int. J. Renew. Energy Res. 2015, 5, 29–40. [Google Scholar]
- Tremblay, O.; Dessaint, L.-A. Experimental validation of a battery dynamic model for EV applications. World Electr. Veh. J. 2009, 3, 289–298. [Google Scholar] [CrossRef]
- MathWorks, Inc. Generic Battery Model—Simulink. Available online: https://www.mathworks.com/help/physmod/sps/powersys/ref/battery.html (accessed on 29 December 2019).
- DiOrio, N.; Dobos, A.; Janzou, S.; Nelson, A.; Lundstrom, B. Technoeconomic Modeling of Battery Energy Storage in SAM; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2015.
- Wijewardana, S.M. New dynamic battery model for hybrid vehicles and dynamic model analysis using simulink. Eng. J. Inst. Eng. Sri Lanka 2014, 47, 53–61. [Google Scholar] [CrossRef]
- Tan, Y.K.; Mao, J.C.; Tseng, K.J. Modelling of battery temperature effect on electrical characteristics of Li-ion battery in hybrid electric vehicle. In Proceedings of the 2011 IEEE Ninth International Conference on Power Electronics and Drive Systems, Singapore, 5–8 December 2011; pp. 637–642. [Google Scholar]
- Panasonic. VRLA: Technical Handbook Industrial Batteries for Professionals. Available online: https://eu.industrial.panasonic.com/sites/default/pidseu/files/downloads/files/panasonic-batteries-vrla-for-professionals_interactive.pdf (accessed on 12 December 2019).
- Monteiro, L.; Finelli, I.; Quinan, A.; Macêdo, W.N.; Torres, P.; Pinho, J.T.; Nohme, E.; Marciano, B.; Silva, S.R. Implementation and validation of energy conversion efficiency inverter models for small PV systems in the north of Brazil. In Renewable Energy in the Service of Mankind Vol II; Springer: Berlin, Germany, 2016; pp. 93–102. [Google Scholar]
- Juárez, R.T.; Fuerte-Esquivel, C.R.; Espinosa-Juárez, E.; Sandoval, U. Steady-state model of grid-connected photovoltaic generation for power flow analysis. IEEE Trans. Power Syst. 2018, 33, 5727–5737. [Google Scholar] [CrossRef]
- Victron Energy. Invesor Red BlueSolar. Available online: https://www.victronenergy.com.es/upload/documents/Datasheet%20-%20BlueSolar%20Grid%20Inverter%20-%20rev%2005%20-%20ES.pdf (accessed on 12 December 2019).
- Datta, M.; Senjyu, T.; Yona, A.; Funabashi, T.; Kim, C.-H. A frequency-control approach by photovoltaic generator in a PV–diesel hybrid power system. IEEE Trans. Energy Convers. 2010, 26, 559–571. [Google Scholar] [CrossRef]
- United Nations Refugee Agency (UNHCR). Diesel Generators: Technical Specification 2017; UNHCR: Geneva, Switzerland, 2017.
- Skarstein, Ø.; Uhlen, K. Design considerations with respect to long-term diesel saving in wind/diesel plants. Wind Eng. 1989, 13, 72–87. [Google Scholar]
- Holmes, K.; Hughes, M.; Mair, J.; Carlsen, J. Events and Sustainability; Routledge: Abingdon, UK, 2015; ISBN 978-1-317-80027-9. [Google Scholar]
- Papathanassiou, S.; Papadopoulos, M. Dynamic characteristics of autonomous wind–diesel systems. Renew. Energy 2001, 23, 293–311. [Google Scholar] [CrossRef]
- Beausoleil-Morrison, I. An Experimental and Simulation-Based Investigation of the Performance of Small-scale Fuel Cell and Combustion-Based Cogeneration Devices Serving Residential Buildings: Final Report of Annex 42 of the International Energy Agency’s Energy Conservation in Buildings and Community Systems Programme; Natural Resources Canada: Ottawa, ON, Canada, 2008; ISBN 0-662-47923-8.
- Alanne, K.; Söderholm, N.; Sirén, K. Implementation and validation of combustion engine micro-cogeneration routine for the simulation program IDA-ICE. In Proceedings of the Conference Building Simulation, Glasgow, Scotland, 27–30 July 2009. [Google Scholar]
- Ribberink, H.; Lombardi, K.; Yang, L.; Entchev, E. Investigation of a hybrid renewable–microgeneration energy system for power and thermal generation with reduced emissions. Proc. Inst. Mech. Eng. Part A J. Power Energy 2013, 227, 62–72. [Google Scholar] [CrossRef]
- Barley, C.D.; Winn, C.B.; Flowers, L.; Green, H.J. Optimal Control of Remote Hybrid Power Systems. Part 1: Simplified Model; National Renewable Energy Lab.: Golden, CO, USA, 1995.
- Dufo-López, R.; Bernal-Agustín, J.L. Generación de energía eléctrica con fuentes renovables. Optimización de Sistemas Híbridos Renovables Con Almacenamiento Energético Mediante Algoritmos Genéticos; Editorial Académica Española. LAP Lambert Academic Publishing GMBH & Co. KG: Saarbrücken, Germany, 2011. [Google Scholar]
- Barbieri, E.S.; Spina, P.R.; Venturini, M. Analysis of innovative micro-CHP systems to meet household energy demands. Appl. Energy 2012, 97, 723–733. [Google Scholar] [CrossRef]
- Bianchi, M.; De Pascale, A.; Spina, P.R. Guidelines for residential micro-CHP systems design. Appl. Energy 2012, 97, 673–685. [Google Scholar] [CrossRef]
- Sigarchian, S.G.; Malmquist, A.; Martin, V. Design optimization of a complex polygeneration system for a hospital. Energies 2018, 11, 1071. [Google Scholar] [CrossRef]
- Wegener, M.; Isalgué, A.; Malmquist, A.; Martin, A. 3E-analysis of a bio-solar CCHP system for the Andaman Islands, India—A case study. Energies 2019, 12, 1113. [Google Scholar] [CrossRef]
- Sigarchian, S.G.; Malmquist, A.; Martin, V. The choice of operating strategy for a complex polygeneration system: A case study for a residential building in Italy. Energy Convers. Manag. 2018, 163, 278–291. [Google Scholar] [CrossRef]
- Wang, J.; Zhai, Z.J.; Jing, Y.; Zhang, C. Particle swarm optimization for redundant building cooling heating and power system. Appl. Energy 2010, 87, 3668–3679. [Google Scholar] [CrossRef]
- Li, L.; Mu, H.; Gao, W.; Li, M. Optimization and analysis of CCHP system based on energy loads coupling of residential and office buildings. Appl. Energy 2014, 136, 206–216. [Google Scholar] [CrossRef]
- Zheng, C.Y.; Wu, J.Y.; Zhai, X.Q. A novel operation strategy for CCHP systems based on minimum distance. Appl. Energy 2014, 128, 325–335. [Google Scholar] [CrossRef]
- HOMER Energy LCC Simulation Time Step. Available online: https://www.homerenergy.com/products/pro/docs/3.10/simulation_time_step.html (accessed on 22 December 2019).
- HOMER Energy LCC How HOMER Calculates Emissions. Available online: https://www.homerenergy.com/products/pro/docs/3.10/how_homer_calculates_emissions.html (accessed on 29 December 2019).
- Genoa Stirling Engine ML1000. Available online: https://genoastirling.com/eng/engine-ml1000.php (accessed on 28 December 2019).
- Araoz Ramos, J.A. Thermodynamic Analysis of Stirling Engine Systems: Applications for Combined Heat and Power. Ph.D. Thesis, KTH, School of Industrial Engineering and Management (ITM), Stockholm, Sweden, 2015. [Google Scholar]
- Momoh, J.A. Energy Processing and Smart Grid; John Wiley & Sons: Hoboken, NJ, USA, 2018; ISBN 978-1-119-37614-9. [Google Scholar]
- Srinivasan, K.K.; Agarwal, A.K.; Krishnan, S.R.; Mulone, V. Natural Gas Engines: For Transportation and Power Generation; Springer: Berlin, Germany, 2018; ISBN 9811333076. [Google Scholar]
- Genoa Stirling Price List. Available online: https://genoastirling.com/eng/price-list.php (accessed on 30 December 2019).
- Ashurst, S. ARPA-E GENSETS Annual Review. Available online: https://arpa-e.energy.gov/sites/default/files/Ashurst_DeltaEE.pdf (accessed on 12 December 2019).
- De Paepe, M.; D’Herdt, P.; Mertens, D. Micro-CHP systems for residential applications. Energy Convers. Manag. 2006, 47, 3435–3446. [Google Scholar] [CrossRef]
- Zabalaga, J.P. Performance Analysis of a Stirling Engine Hybrid Power System—OpenEI Datasets. Available online: https://openei.org/datasets/dataset/performance-analysis-of-a-stirling-engine-hybrid-power-system (accessed on 31 December 2019).




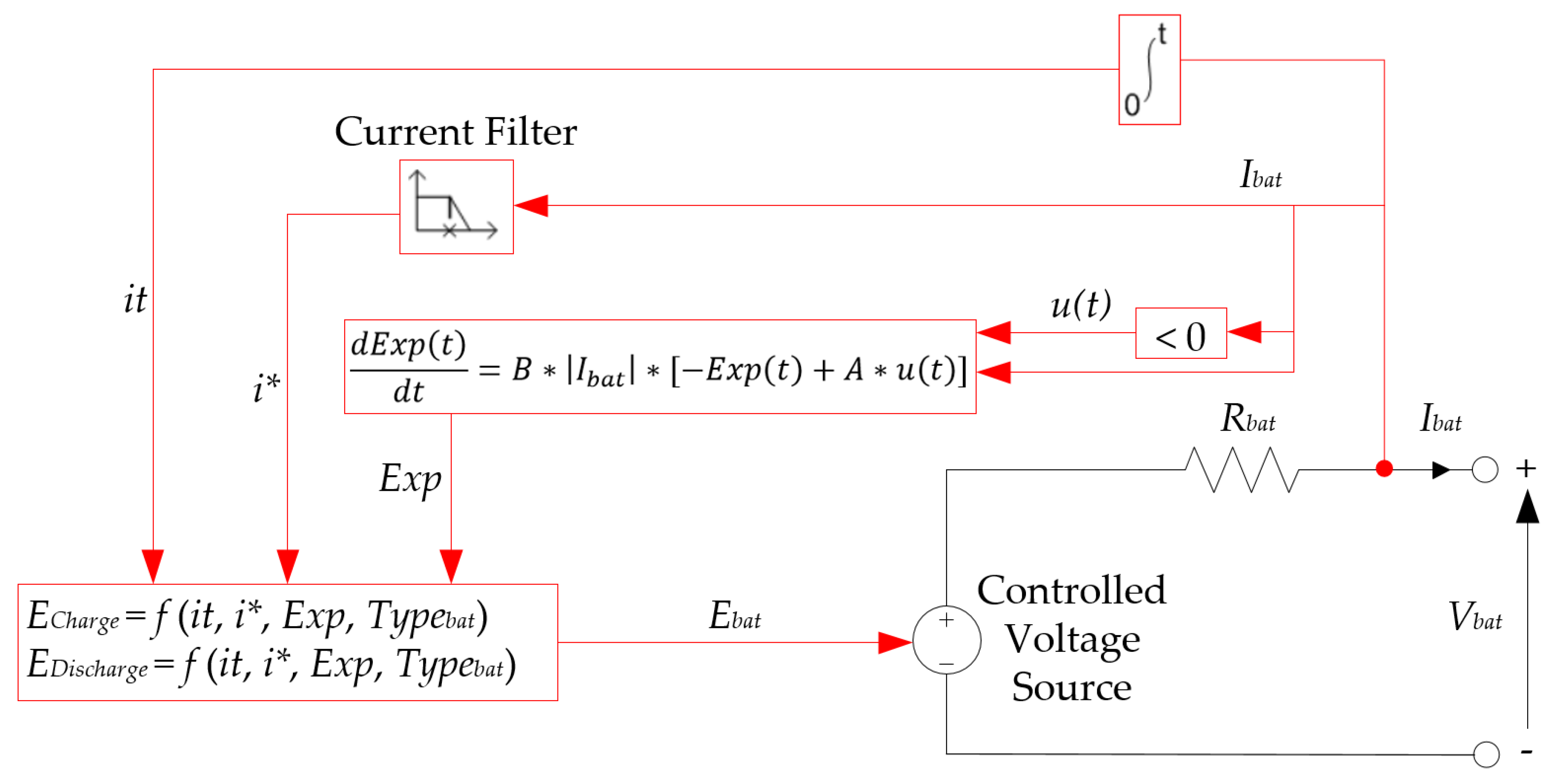

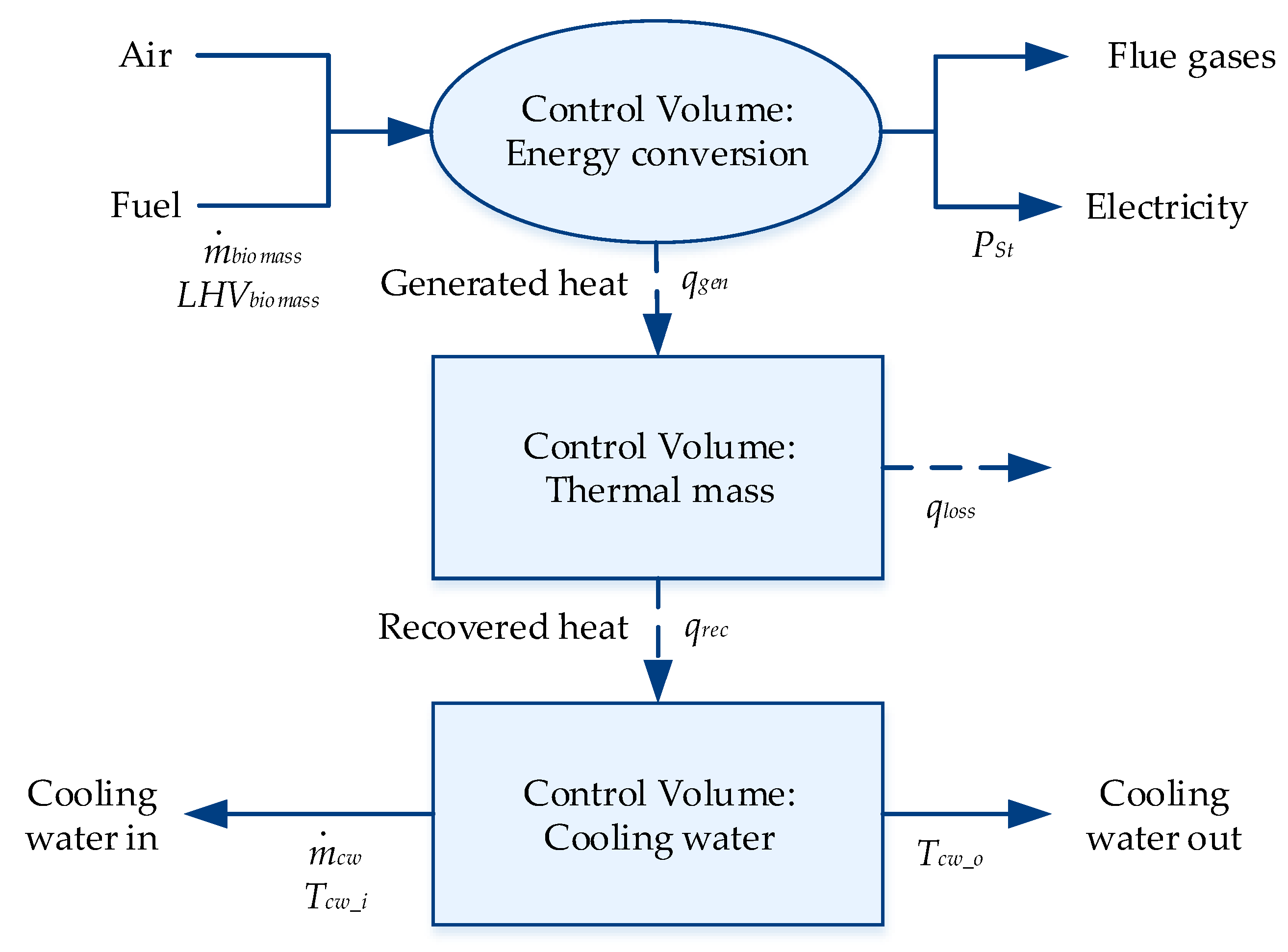


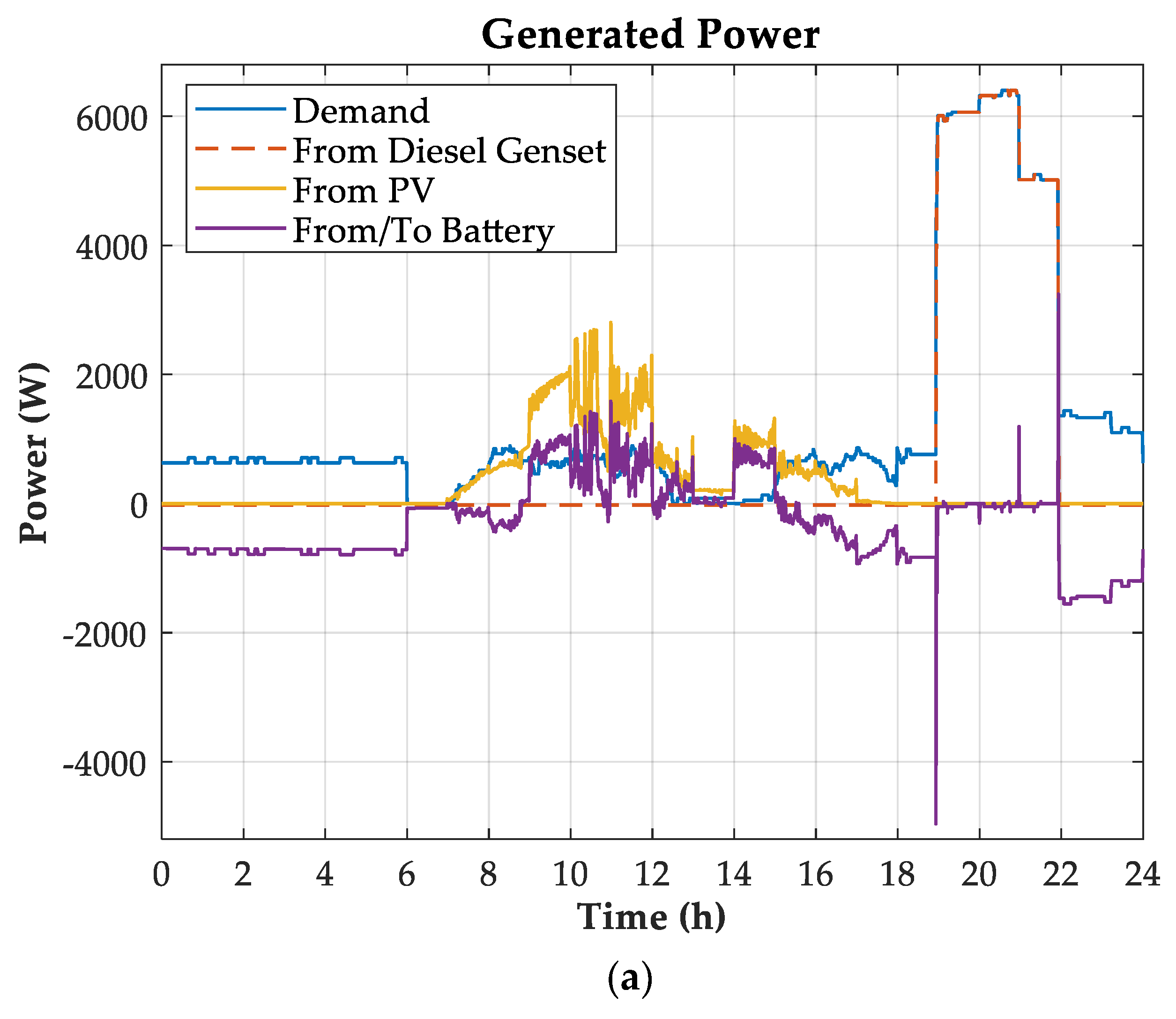
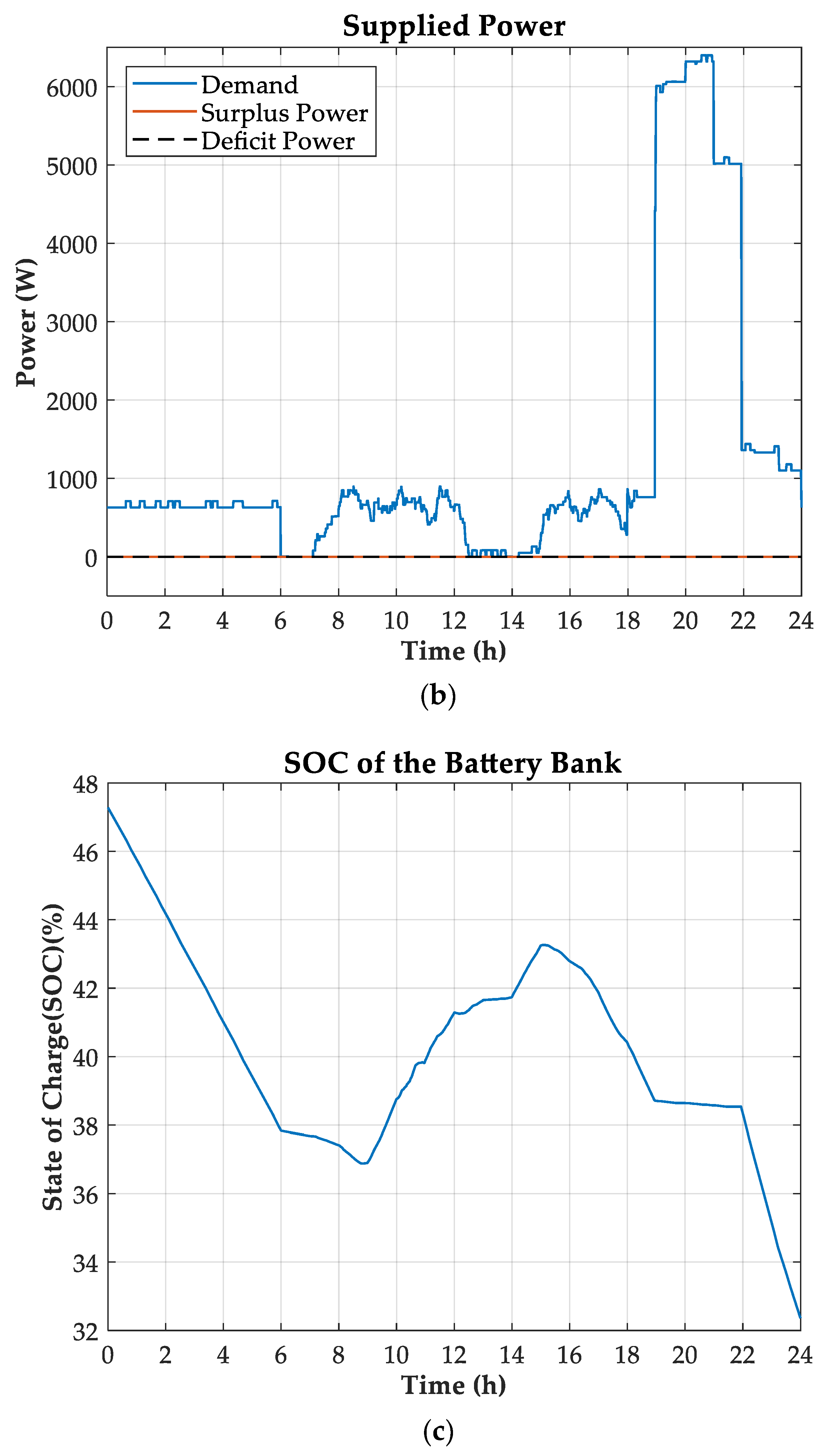
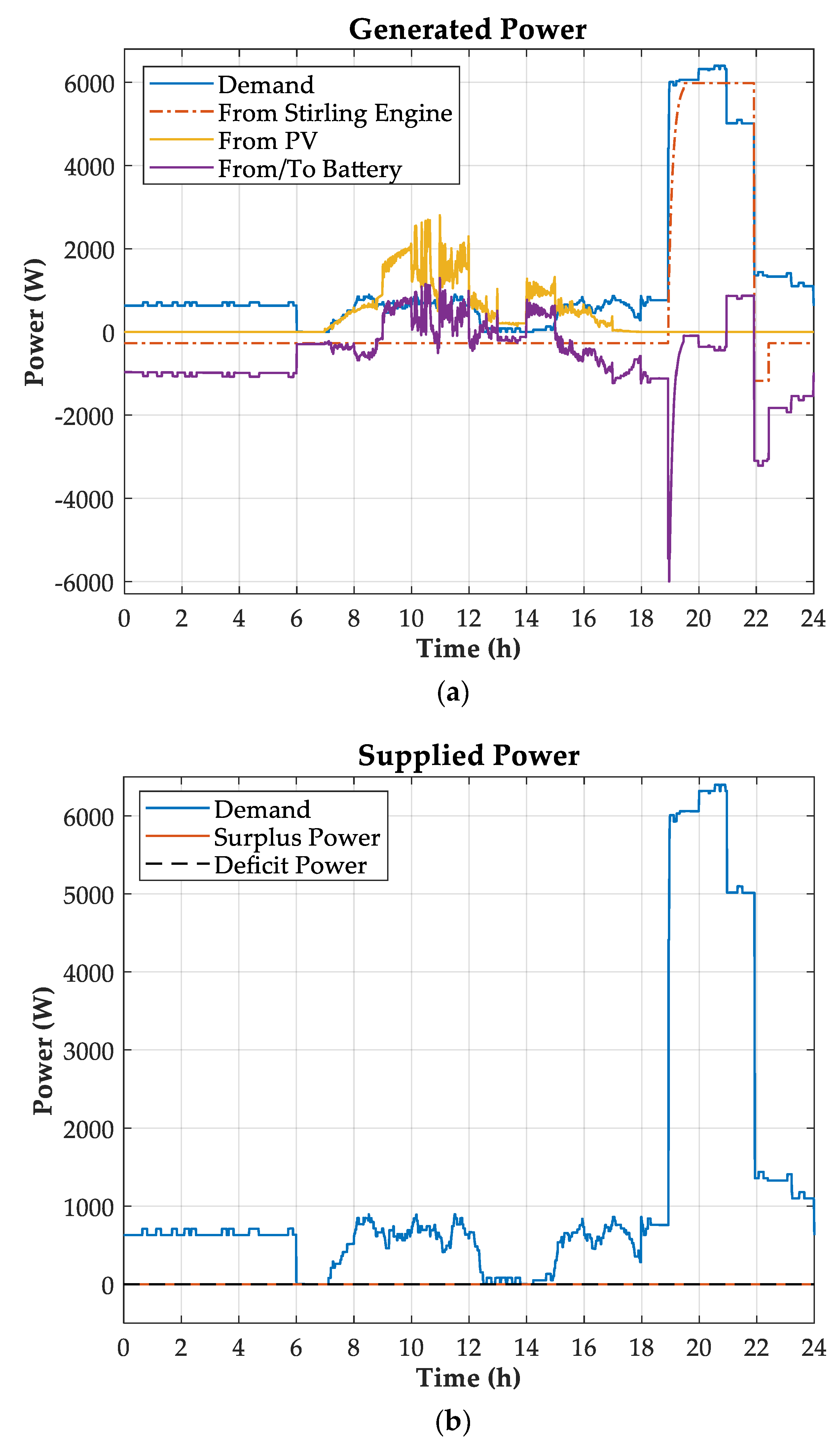
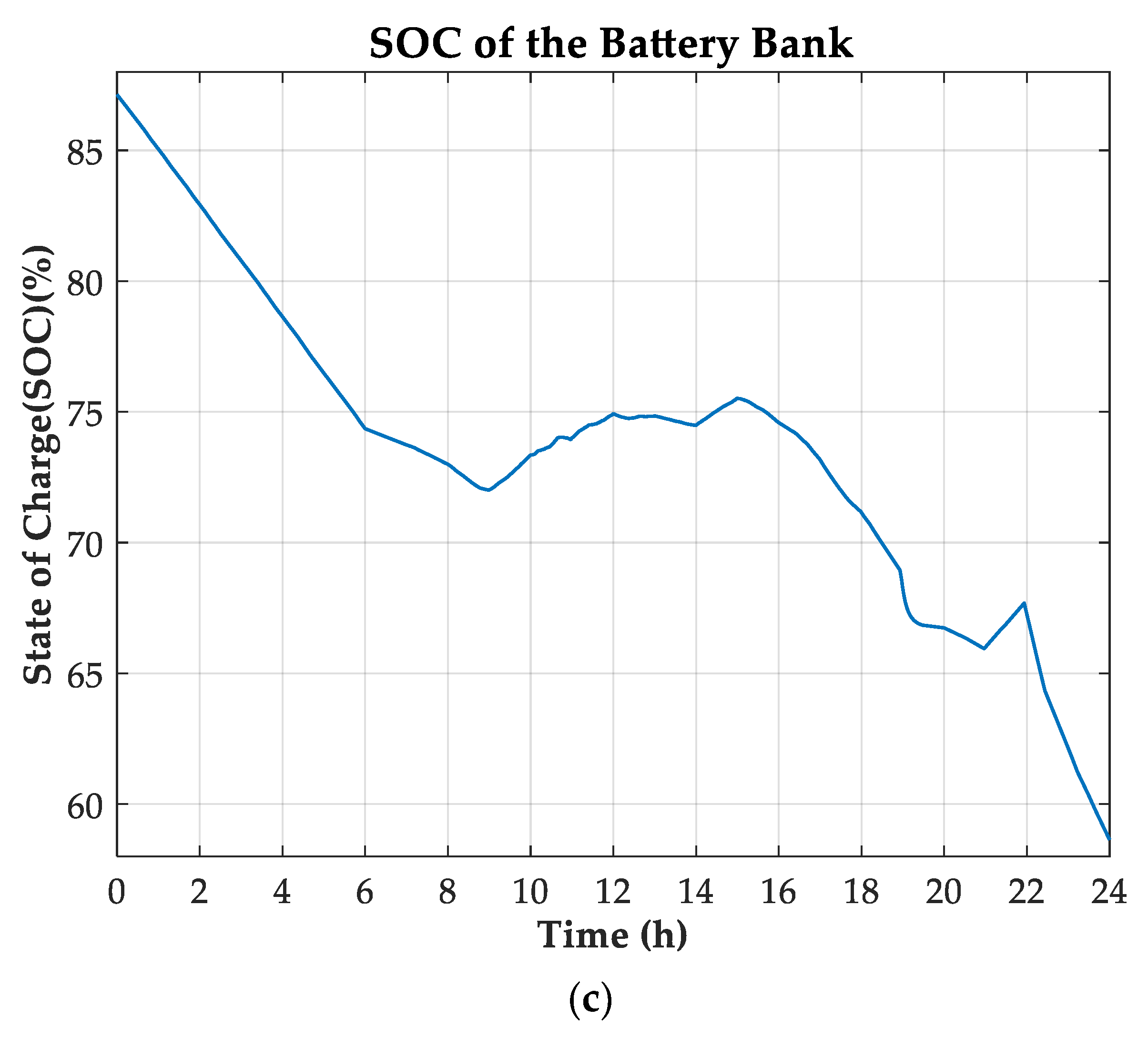
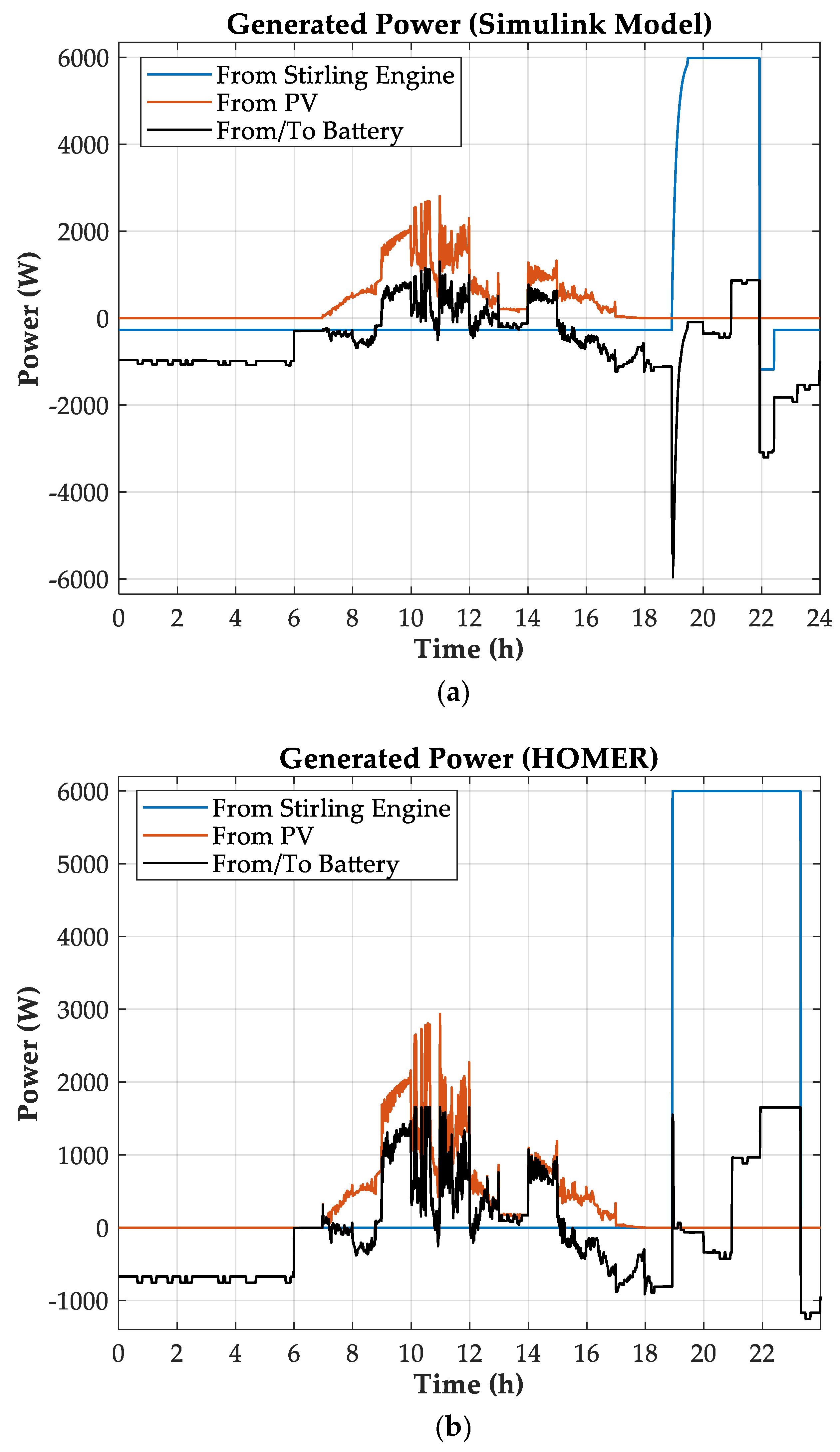

| Type of Load | Active Power | Duration |
|---|---|---|
| Household | ||
| Lighting | 3 × 14 W | 19:00–22:00 |
| Mobile charging | 3 × 5 W | 19:00–21:00 |
| Each of both schools | ||
| Computer | 9 × 50 W | 07:30–12:30 15:00–18:00 |
| Printer | 20 W | |
| Mobile charging | 5 × 2 W | |
| Church | ||
| Lighting | 10 × 26 W | 20:00–00:00 |
| External Lighting | 7 × 30 W | |
| Speaker | 100 W | 20:00–22:30 |
| Primary healthcare facility | ||
| Lighting | 5 × 26 W | 08:00–12:00 19:00–22:30 |
| External Lighting | 30 W | 18:30–22:30 |
| TV | 80 W | 07:00–12:00 |
| Mobile charging | 2 × 2 W | 05:00–23:00 |
| Small fridge | 80 W | Whole day |
| Computer | 50 W | Whole day |
| Streetlight | ||
| Streetlight | 9 × 70 W | 19:00–06:00 |
| Component | Size | Capital Cost (USD) | Replacement Cost (USD) | O&M Cost | Lifetime |
|---|---|---|---|---|---|
| PV panel | 0.32 kW | 747 | 344 | USD $18/year | 25 years |
| Battery | 110 Ah | 432 | 376 | USD $8/year | 500 cycles (70% DOD) |
| Bidirectional Inverter | 1.6 kW | 1764 | 1604 | USD $160.40/year | 15 years |
| Diesel genset | 1 kW | 925 | 804 | USD $0.0130/h | 20,000 h |
| Stirling engine | 1 kW | 4200 | 4200 | USD $0.0118/h | 25,000 h |
| Parameter | Value | Unit |
|---|---|---|
| Maximum power point | 37.1 | V |
| Maximum power point current | 8.63 | A |
| Open circuit voltage | 45.8 | V |
| Short circuit current | 9.10 | A |
| Number of cells in the module | 72 | − |
| Temperature coefficient of short circuit current | 0.00455 | A/°C |
| Temperature coefficient of open circuit voltage | −0.14656 | V/°C |
| Normal operating cell temperature (NOCT) | 44 | °C |
| Ideality factor of diode | 1 | − |
| Temperature coefficient of power | −0.41 | %/°C |
| Solar irradiation coefficient | 0.12 | − |
| Panel electrical efficiency | 16.5 | % |
| Parameter | Value | Unit |
|---|---|---|
| Battery capacity | 110 | Ah |
| Nonimal voltage | 12 | V |
| Battery voltage constant | 12.8632 | V |
| Internal resistance | 0.004 | Ω |
| Polarization constant | 0.0028319 | V/Ah |
| Exponential zone amplitude | 0.15876 | V |
| Exponential zone time constant inverse | 32.7154 | 1/Ah |
| Minimum state of charge | 30 | % |
| Reference battery temperature | 20 | °C |
| Battery roundtrip efficiency | 80 | % |
| Maximum charge power | 10,326 | W |
| Maximum discharge power | 20,955 | W |
| Number of equivalent full cycles | 500 | − |
| Parameter | Value | Unit |
|---|---|---|
| Converter efficiency at 100% of its nominal value | 86.53 | % |
| Converter efficiency at 50% of its nominal value | 91.50 | % |
| Converter efficiency at 10% of its nominal value | 90.00 | % |
| Parameter | Value | Unit |
|---|---|---|
| TD | 0.5 | s |
| TSM | 0.05 | s |
| HD | 1.5 | − |
| KI | 4 | − |
| R | 0.05 | − |
| KS | 4 | − |
| KD | 0.075 | − |
| Ancillaries’ consumption | −3.091 | Wconsumption/kWrated power |
| Parameter | Value | Unit |
|---|---|---|
| Maximum net power of Stirling engine from experimental calibration | 780 | W |
| Engine nominal temperature from experimental calibration | 465 | °C |
| CSt | 10,950 | J/K |
| CHX | 25,200 | J/K |
| UAHX | 18.9 | W/K |
| UAloss | 0.35 | W/K |
| a0 | 0.0999959 | − |
| a4 | −0.0937248 | − |
| a5 | −1.58009 × 10−6 | − |
| a6 | −2.71098 × 10−4 | − |
| a16 | 1.13114 × 10−3 | − |
| a1–a3; a7–a15; a17–a26 | 0 | − |
| b0 | 0.415454 | − |
| b3 | −5.61372 | − |
| b4 | 3.38032 | − |
| b5 | 4.23782 × 10−5 | − |
| b6 | −1.94283 × 10−3 | − |
| b16 | −0.01455 | − |
| c0 | 0.266 | − |
| c1–c8 | 0 | − |
| kp | 1 | − |
| Power consumption during standby mode | −157 | W |
| Power consumption during cool-down mode | −36 | W |
| Cool-down time | 1800 | s |
| Cooling water temperature | 55 | °C |
| Architecture | PV/Diesel/Battery System | PV/Stirling/Battery System |
|---|---|---|
| PV panels (kW) | 3.54 | 3.56 |
| Stirling CHP module (kW) | − | 6.00 |
| Diesel genset (kW) | 7.10 | − |
| Batteries | 34 | 34 |
| Bidirectional inverter (kW) | 4.62 | 1.65 |
| COE (USD/kWh) | 0.778 | 0.726 |
| Parameter | Simulink Models | HOMER | ||
|---|---|---|---|---|
| PV/Diesel/Battery System | PV/Stirling/Battery System | PV/Diesel/Battery System | PV/Stirling/Battery System | |
| Electricity generated by PV array (kWh/year) | 6013 | 6013 | 5297 | 5518 |
| Electricity generated by Stirling engine (kWh/year) | 0 | 8955 | 0 | 7282 |
| Electricity generated by Diesel Genset (kWh/year) | 6361 | 0 | 6432 | 0 |
| Biomass consumption (kg/year) | 0 | 4264 | 0 | 3467 |
| Diesel consumption (L/year) | 2,395 | 0 | 2,060 | 0 |
| Annualized cost of the system (USD/year) | 13,760.88 | 12,249.66 | 8314.55 | 7758.63 |
| CO2 emissions (kg/year) | 6300 | 1970 | 5392 | − |
| Performance Criterions | Rate (%) |
|---|---|
| FSR | 5 |
| CO2ERR | 69 |
| ATCSR | 11 |
| ISR | 28 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jimenez Zabalaga, P.; Cardozo, E.; Choque Campero, L.A.; Araoz Ramos, J.A. Performance Analysis of a Stirling Engine Hybrid Power System. Energies 2020, 13, 980. https://doi.org/10.3390/en13040980
Jimenez Zabalaga P, Cardozo E, Choque Campero LA, Araoz Ramos JA. Performance Analysis of a Stirling Engine Hybrid Power System. Energies. 2020; 13(4):980. https://doi.org/10.3390/en13040980
Chicago/Turabian StyleJimenez Zabalaga, Pablo, Evelyn Cardozo, Luis A. Choque Campero, and Joseph Adhemar Araoz Ramos. 2020. "Performance Analysis of a Stirling Engine Hybrid Power System" Energies 13, no. 4: 980. https://doi.org/10.3390/en13040980
APA StyleJimenez Zabalaga, P., Cardozo, E., Choque Campero, L. A., & Araoz Ramos, J. A. (2020). Performance Analysis of a Stirling Engine Hybrid Power System. Energies, 13(4), 980. https://doi.org/10.3390/en13040980