Data-Driven Modeling of Fuel Consumption for Turboprop-Powered Civil Airliners
Abstract
1. Introduction
2. Database and Methods
2.1. Data Set
2.2. Weights Breakdown and Weight of Fuel Burned
- The Weight of Fuel Reserves (WFRes) is taken as a fixed percentage of one of the aircraft’s representative masses, in the present case, the Maximum Take-Off Weight (MTOW). For civil airliners, a survey of the required reserves to satisfy the Airworthiness Authorities provisions (missed approach, hold, and diversion) among the training set led to a safe value of 3.5%. This value is less cautious than a flat 8% of the zero-fuel weight [7,25], but exceeds the 6% of the total fuel consumption taken as tolerance for reserves and trapped fuel [12].
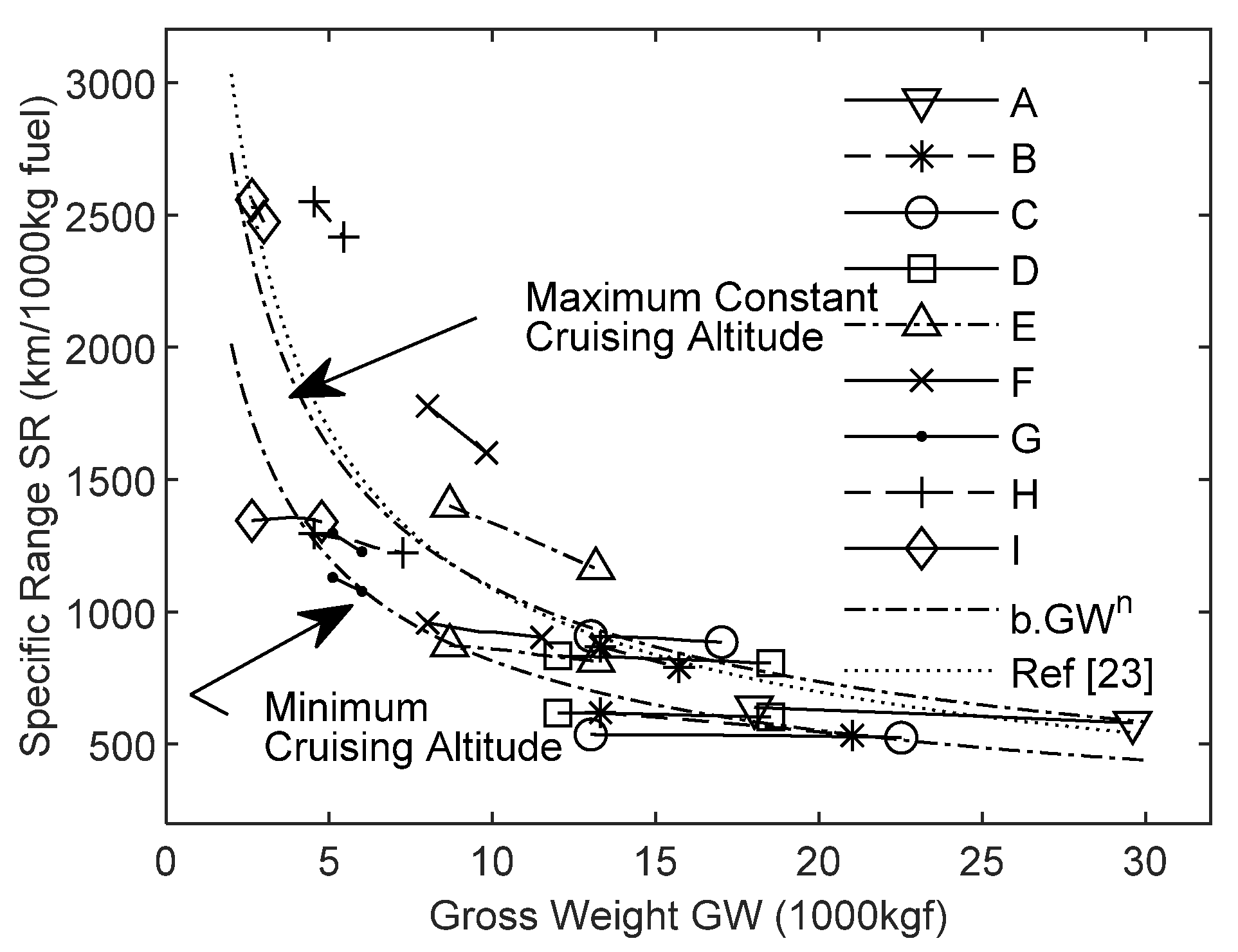
- The gross weight is used for a linear interpolation of the specific range for different altitudes (6 samples), although better interpolation was obtained with a quadratic regression for 3 samples. In all cases, the goodness of fit R2 exceeded 0.99. The maximum relative errors computed after the interpolation process did not exceed 0.8%.
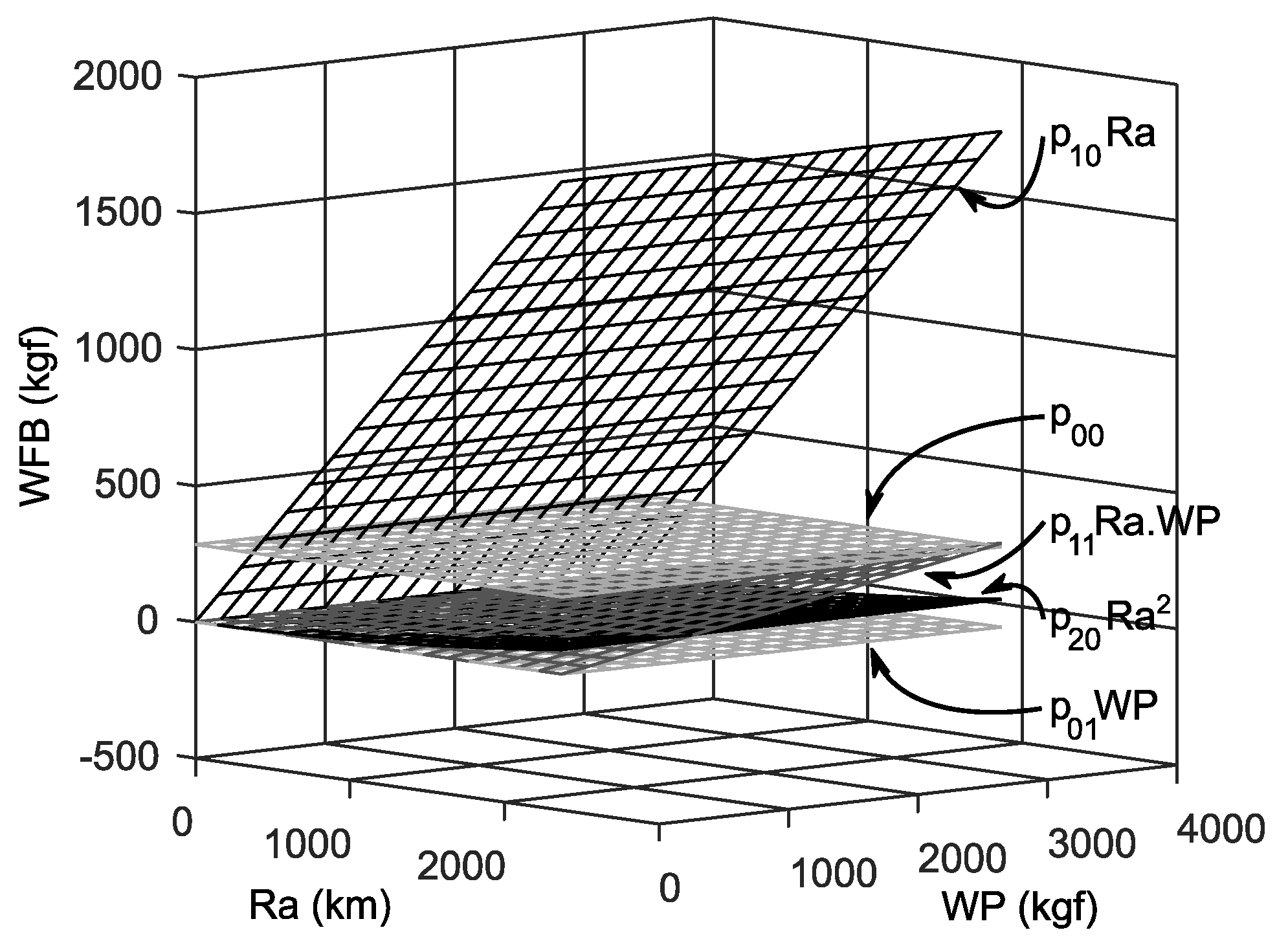
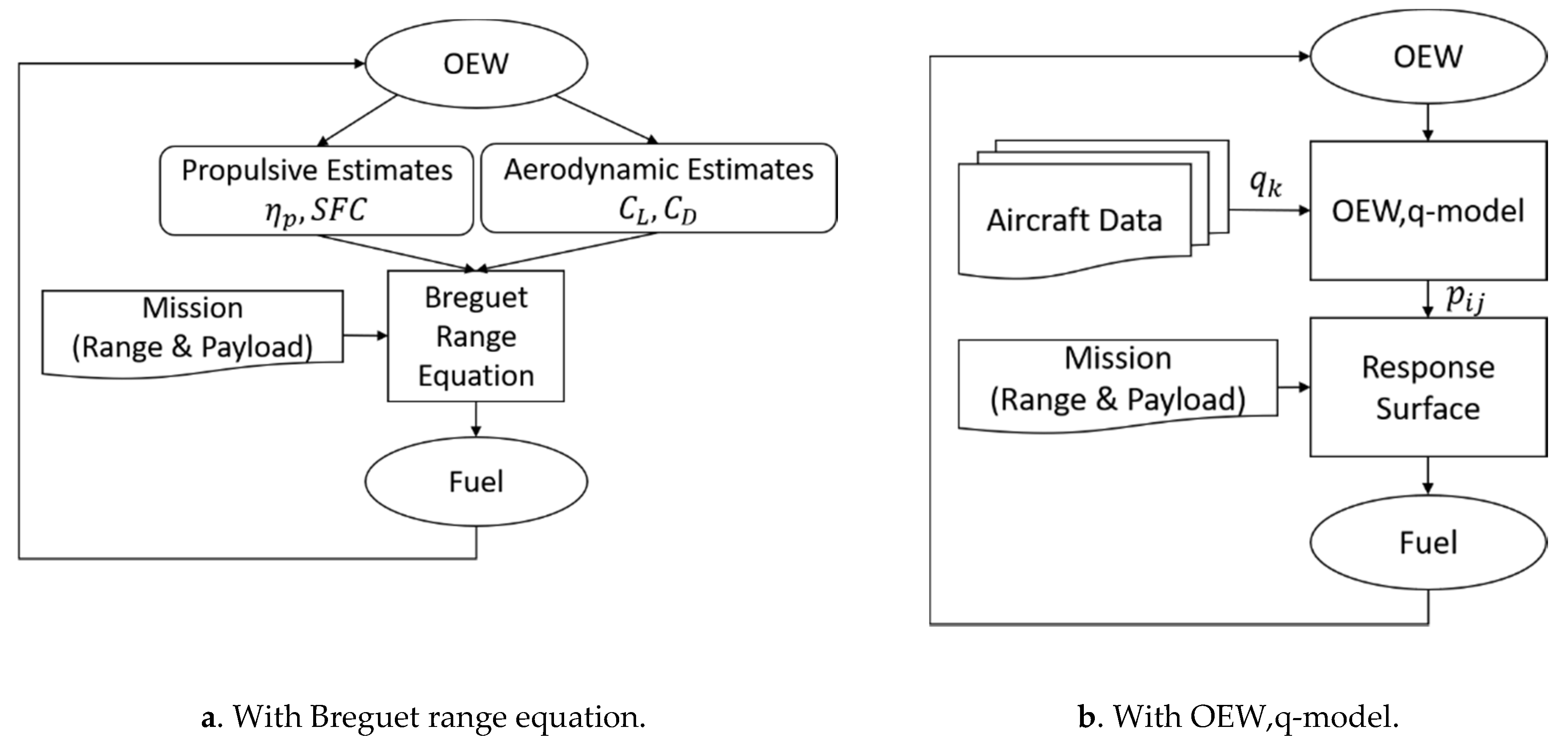
2.3. Fuel Estimate Model Based on OEW
3. Results, Validation of the OEW,q-Model, and Discussion
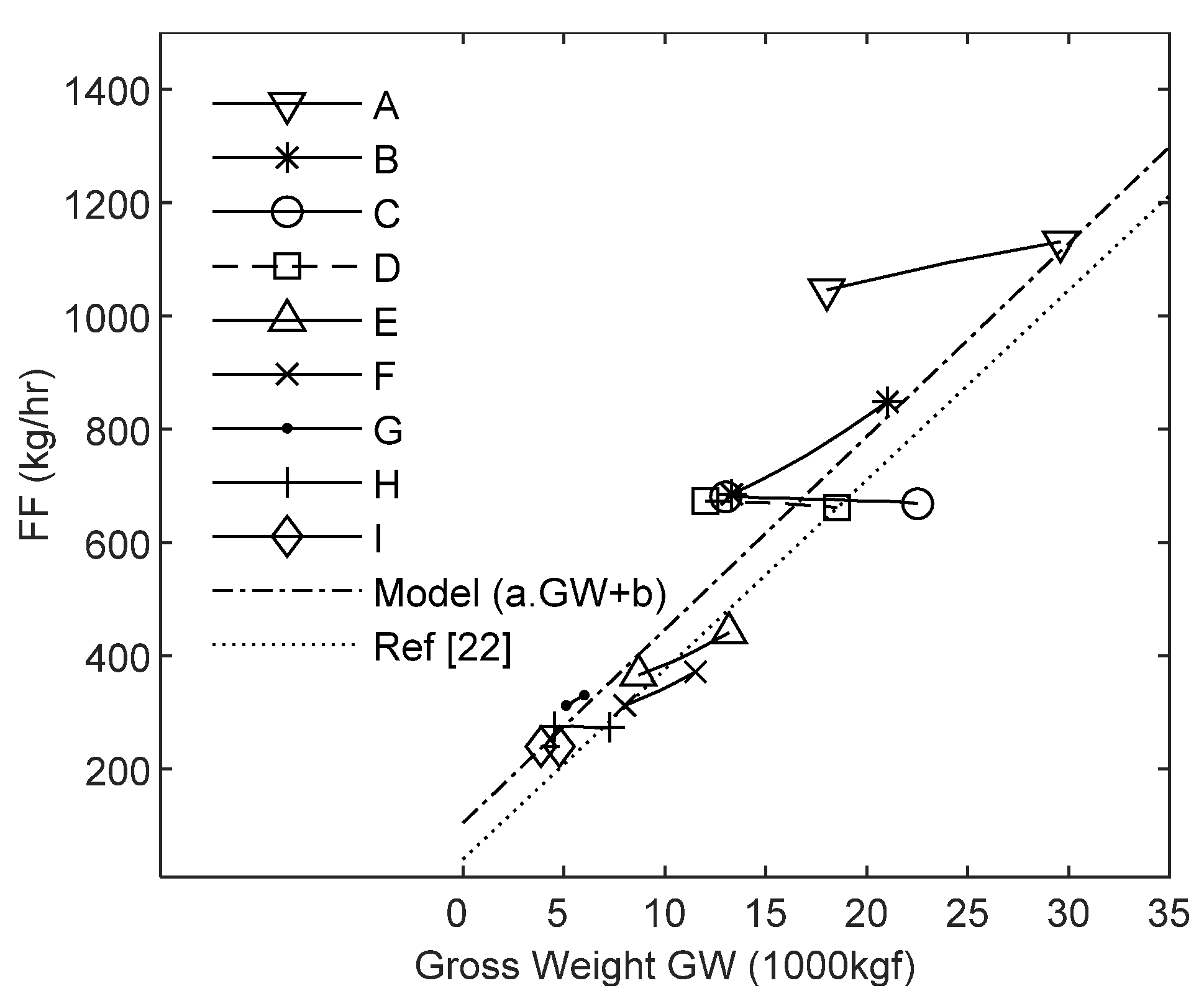
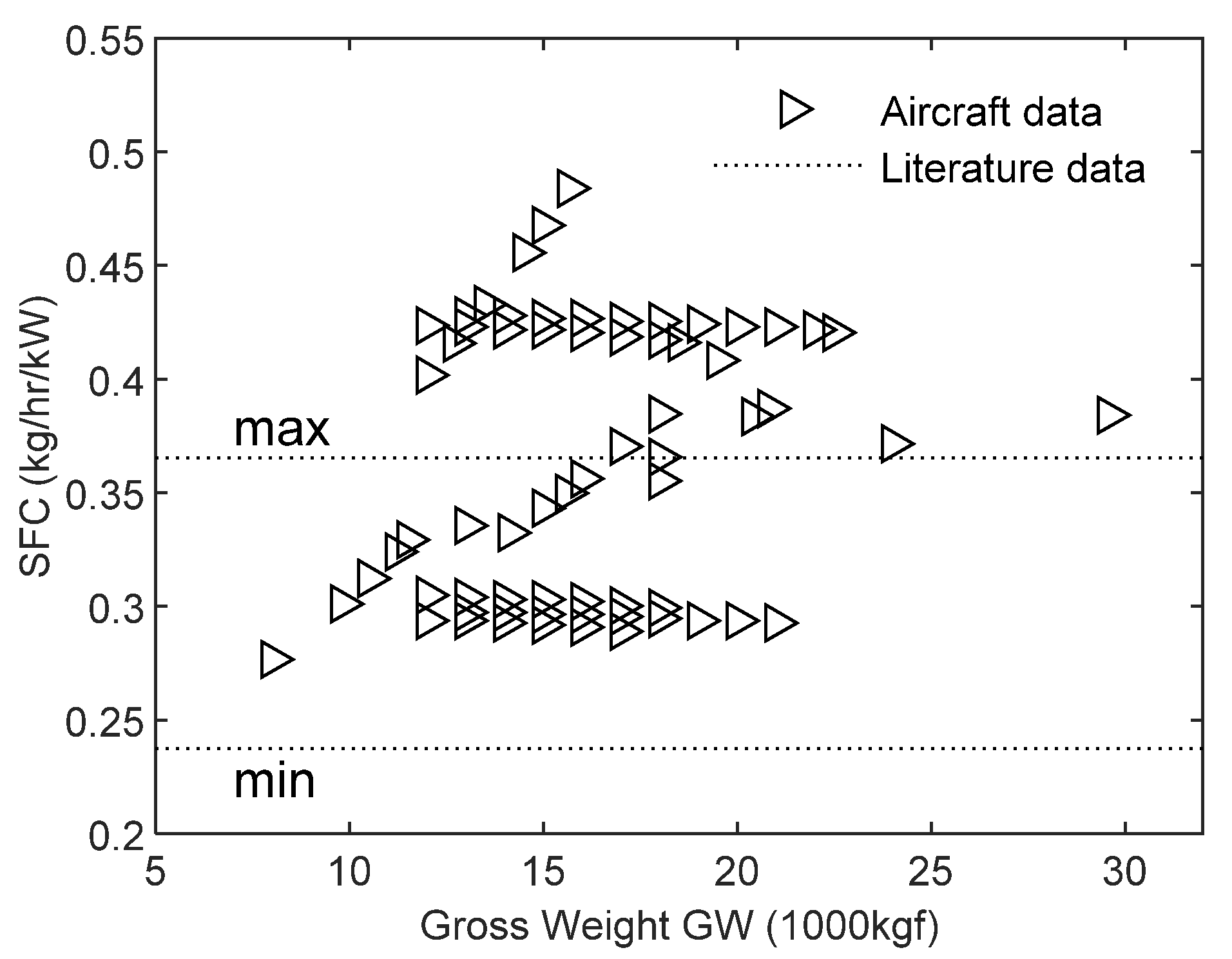
3.1. Instantaneous Fuel Consumption Figure of Merits
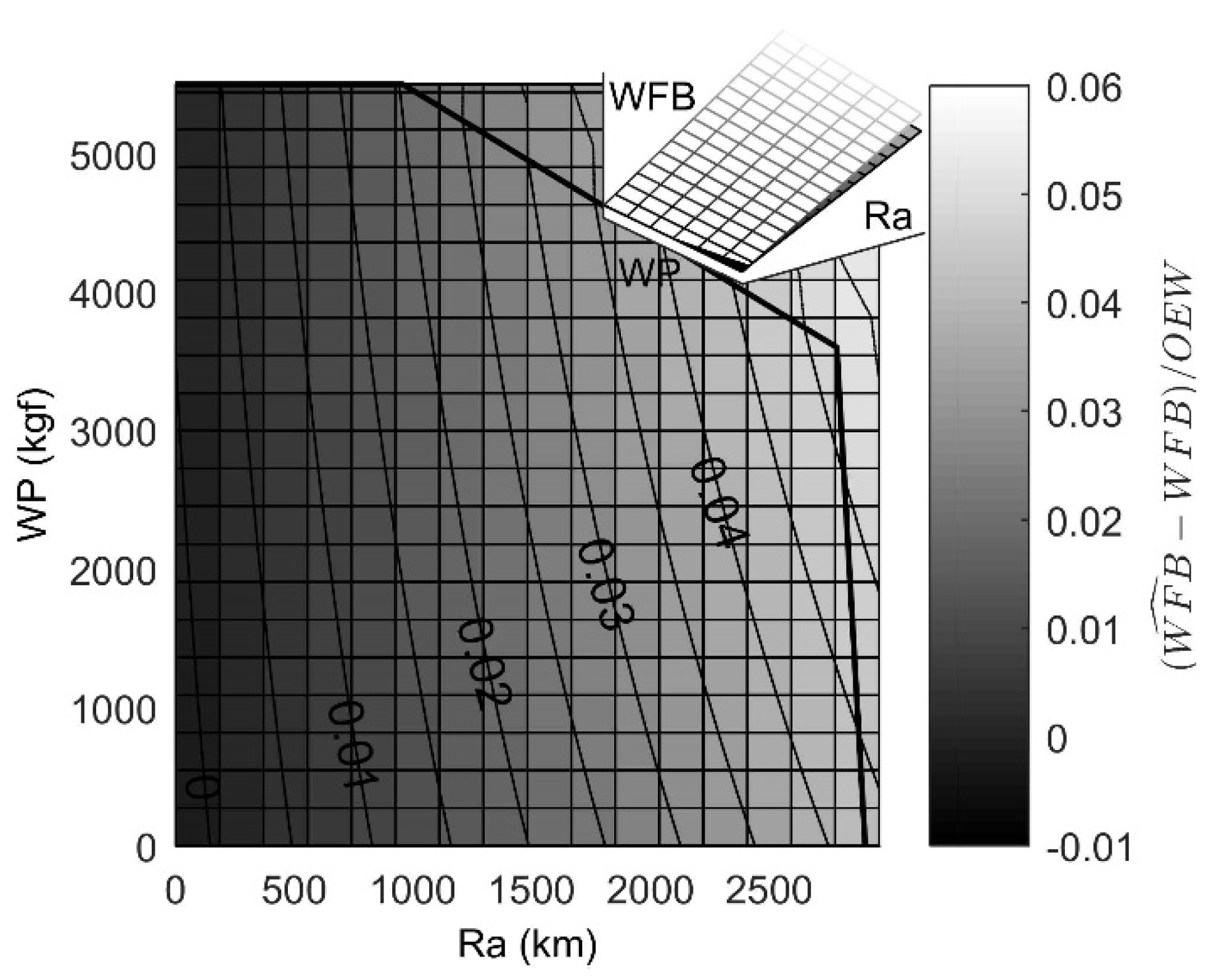
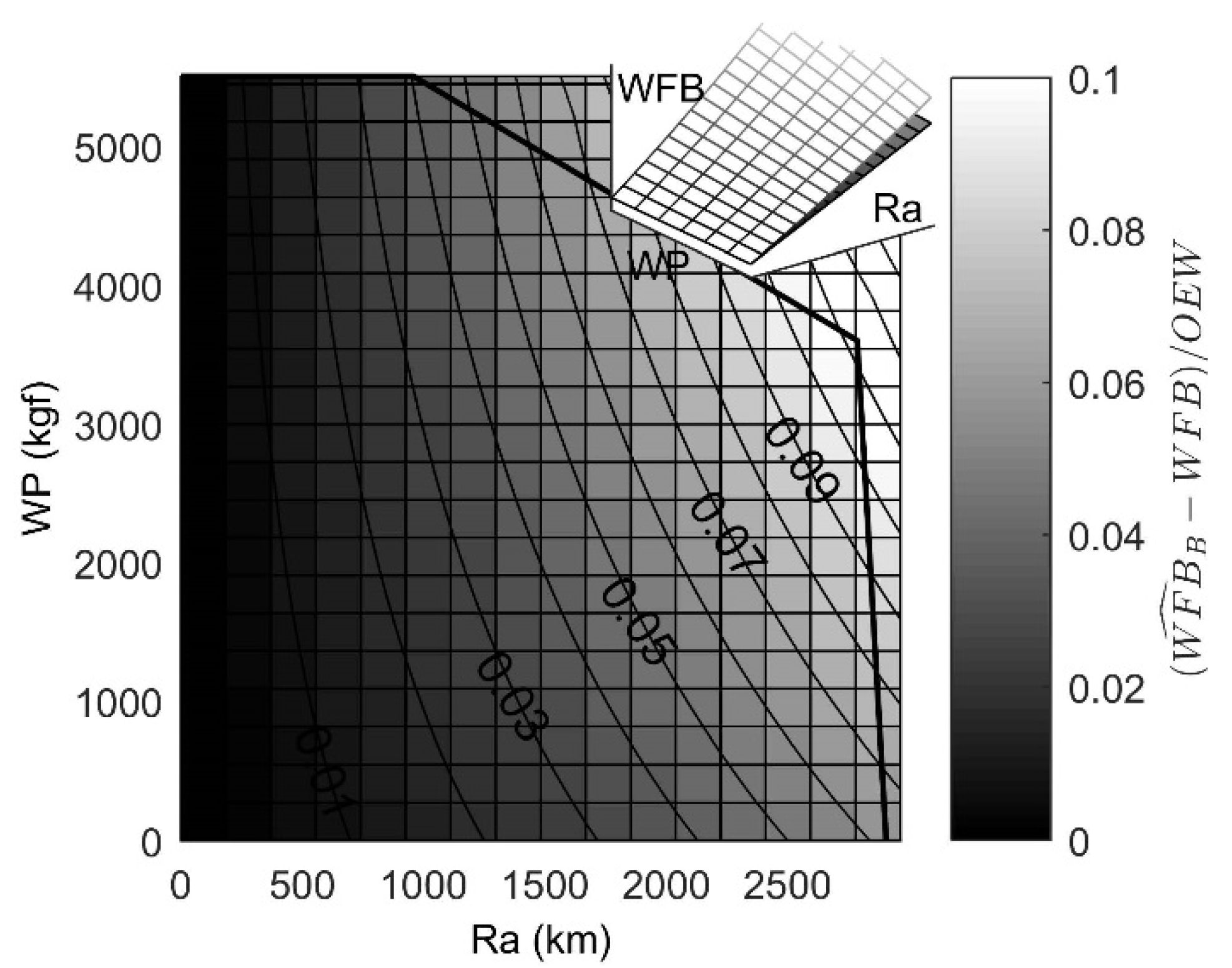
3.2. WFB-Predictions and Validation of the OEW,q-Model
3.3. Influence of Altitude on the OEW,q-Model
3.4. Influence of Temperature on the OEW,q-Model
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| a,b | Regression coefficients |
| Alt | Altitude |
| D | Drag (N) |
| FF | Fuel Flow rate (kg/hr) |
| g | Gravitational acceleration (m/s2) |
| GW | Gross Weight |
| L | Lift (N) |
| MTOW | Maximum Take-Off Weight (kgf) |
| n | Regression exponent |
| OEW | Operating Empty Weight (kgf) |
| p,q | Polynomial coefficients |
| Ra | Range (km) |
| SFC | Power specific fuel consumption (kg/kW/hr) |
| SR | Specific Range (km/1000kg of fuel) |
| TOW | Take-Off Weight (kgf) |
| WF | Weight of Fuel (kgf) |
| WFB | Weight of Fuel Burned (kgf) |
| WFBC | Weight of Fuel Burned for Cruise (kgf) |
| WFBS | Weight of Fuel Burned for Starting (kgf) |
| WFRes | Weight of Fuel Reserves (kgf) |
| ZFW | Zero Fuel Weight (kgf) |
| Propeller propulsive efficiency | |
| Predicted value | |
| Breguet estimate | |
| Note: Altitudes are expressed in hectofeet (hft) according to common aeronautical practice. | |
| Note: Kilogram-force units (kgf) are used for weights that are otherwise expressed in mass units in standard aeronautical practice. | |
References
- Babikian, R.; Lukachko, S.; Waitz, I. The historical fuel efficiency characteristics of regional aircraft from technological, operational, and cost perspectives. J. Air Transp. Manag. 2002, 8, 389–400. [Google Scholar] [CrossRef]
- Corke, T.C. Design of Aircraft; Prentice Hall: Upper Saddle River, NJ, USA, 2003. [Google Scholar]
- Filippone, A. Data and performances of selected aircraft and rotorcraft. Prog. Aerosp. Sci. 2000, 36, 629–654. [Google Scholar] [CrossRef]
- Howe, D. Aircraft Conceptual Design Synthesis; Professional Engineering Publishing: London, UK, 2000. [Google Scholar]
- Ibrahim, K. Selecting principal parameters of baseline design configuration for twin turboprop transport aircraft. In Proceedings of the 22nd Applied Aerodynamics Conference and Exhibit, Providence, RI, USA, 16–19 August 2004. Number AIAA 2004–5069. [Google Scholar] [CrossRef]
- Kundu, A.K. Aircraft Design; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Lee, H.-T.; Chatterji, G.B. Closed-form takeoff weight estimation model for air transportation simulation. In Proceedings of the 10th AIAA Aviation Technology, Integration and Operations Conference, Ft. Worth, TX, USA, 13–15 September 2010; Volume 2. [Google Scholar] [CrossRef]
- Liem, R.P.; Mader, C.A.; Martins, J.R. Surrogate models and mixtures of experts in aerodynamic performance prediction for aircraft mission analysis. Aerosp. Sci. Technol. 2015, 43, 126–151. [Google Scholar] [CrossRef]
- Marinus, B.G.; Poppe, J. Data and design models for military turbo-propeller aircraft. Aerosp. Sci. Technol. 2015, 41, 63–80. [Google Scholar] [CrossRef]
- Nightingale, W.I.M. Aeroplane weight analysis. Aircr. Eng. Aerosp. Technol. 1945, 17, 250–253. [Google Scholar] [CrossRef]
- Obert, E.; Slingerland, R. Aerodynamic Design of Transport Aircraft; IOS Press BV: Amsterdam, The Netherlands, 2009. [Google Scholar]
- Raymer, D.P. Aircraft Design: A Conceptual Approach; AIAA: Reston, VA, USA, 2006. [Google Scholar] [CrossRef]
- Roskam, J. Part 1: Preliminary sizing of airplanes. In Airplane Design; DARcorporation: Lawrence, KS, USA, 2002; ISBN 9781884885556. [Google Scholar]
- Roskam, J.; Lan, C.-T.E. Airplane Aerodynamics and Performance; DARcorporation: Lawrence, KS, USA, 1997. [Google Scholar]
- Schaufele, R.D. The Elements of Aircraft Preliminary Design; Aries Publications: Santa Ana, CA, USA, 2000. [Google Scholar]
- Stinton, D. The Design of the Airplane; BSP Professional Books: Oxford, UK, 1983. [Google Scholar]
- Torenbeek, E. Synthesis of Subsonic Airplane Design; Delft University Press-Kluwer Academic Publishers: Delft, The Netherlands, 1982. [Google Scholar]
- Torenbeek, E. Prediction of wing group weight for preliminary design. Aircr. Eng. Aerosp. Technol. 1971, 43, 16–21. [Google Scholar] [CrossRef]
- Vouvakos, X.; Kallinderis, Y.; Menounou, P. Preliminary design correlations for twin civil turboprops and comparison with jet aircraft. Aircr. Eng. Aerosp. Technol. 2010, 82, 126–133. [Google Scholar] [CrossRef]
- Breguet, L. Calcul du poids de combustible consommé par un avion en vol ascendant. In Comptes Rendus Hebdomadaires des Séances de l’Académie des Sciences; Académie des Sciences: Paris, France, 1923; pp. 870–872. [Google Scholar]
- Randle, W.E.; Hall, C.A.; Vera-Morales, M. Improved range equation based on aircraft flight data. J. Aircr. 2011, 48, 1291–1298. [Google Scholar] [CrossRef]
- Torenbeek, E. Cruise performance and range prediction reconsidered. Prog. Aerosp. Sci. 1997, 33, 285–321. [Google Scholar] [CrossRef]
- Marinus, B.G.; Maison, J. Fuel weight estimates of military turbo-propeller transport aircraft. Aerosp. Sci. Technol. 2016, 55, 458–464. [Google Scholar] [CrossRef]
- BADA v3.13. Base of Aircraft Data, March 10 2017. Available online: www.eurocontrol.int/services/bada (accessed on 15 May 2017).
- Kroo, I. Aircraft Design: Synthesis and Analysis; Desktop Aeronautics, Inc.: Stanford, CA, USA, 2001. [Google Scholar]
- Fielding, J.P. Introduction to Aircraft Design; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar] [CrossRef]
- Cavcar, A.; Cavcar, M. Approximate solutions of range for constant altitude-constant high subsonic speed flight of transport aircraft. Aerosp. Sci. Technol. 2004, 8, 557–567. [Google Scholar] [CrossRef]





| Set | Sample | OEW (kgf) | OEW/MTOW | WPmax/OEW | WFmax/OEW |
|---|---|---|---|---|---|
| Training | A | 17,830 | 0.60 | 0.48 | 0.30 |
| B | 13,940 | 0.66 | 0.39 | 0.32 | |
| C | 13,610 | 0.60 | 0.53 | 0.37 | |
| D | 11,700 | 0.63 | 0.45 | 0.38 | |
| E | 8620 | 0.66 | 0.39 | 0.30 | |
| F | 7230 | 0.63 | 0.45 | 0.36 | |
| G | 4350 | 0.66 | 0.39 | 0.30 | |
| H | 4180 | 0.58 | 0.51 | 0.47 | |
| I | 2630 | 0.53 | 0.74 | 0.43 | |
| Testing | J | 14,510 | 0.63 | 0.38 | 0.29 |
| K | 13,110 | 0.63 | 0.42 | 0.31 | |
| L | 11,800 | 0.60 | 0.52 | 0.22 | |
| M | 10,440 | 0.67 | 0.39 | 0.25 | |
| N | 4850 | 0.62 | 0.42 | 0.42 | |
| O | 4322 | 0.63 | 0.31 | 0.38 |
| Hypothesis | p-term | q2 | q1 | q0 |
|---|---|---|---|---|
| Constant Altitude | p00 | 0 | 3.24 × 10−2 | 4.79 |
| p10 | 1.69 × 10−9 | 4.74 × 10−5 | 2.29 × 10−1 | |
| p01 | 1.68 × 10−11 | −1.83 × 10−7 | 3.33 × 10−3 | |
| p11 | 9.96 × 10−14 | −4.42 × 10−9 | 6.76 × 10−5 | |
| p20 | −4.54 × 10−14 | 1.30 × 10−9 | 8.33 × 10−6 | |
| Variable Altitude | p00 | 0 | 2.94 × 10−2 | 4.36 |
| p10 | 4.44 × 10−9 | 2.45 × 10−6 | 0.361 | |
| p01 | 1.37 × 10−10 | −2.89 × 10−6 | 0.022 | |
| p11 | 8.64 × 10−13 | −1.68e × 10−8 | 1.05 × 10−4 | |
| p20 | 3.84 × 10−13 | −5.93 × 10−9 | 2.60 × 10−5 |
| Altitude | n | b | R2 |
|---|---|---|---|
| Maximum cruise | −0.57 | 2.070 × 105 | 0.70 |
| Minimum Cruise | −0.56 | 1.435 × 105 | 0.88 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marinus, B.G.; Hauglustaine, A. Data-Driven Modeling of Fuel Consumption for Turboprop-Powered Civil Airliners. Energies 2020, 13, 1695. https://doi.org/10.3390/en13071695
Marinus BG, Hauglustaine A. Data-Driven Modeling of Fuel Consumption for Turboprop-Powered Civil Airliners. Energies. 2020; 13(7):1695. https://doi.org/10.3390/en13071695
Chicago/Turabian StyleMarinus, Benoit G., and Antoine Hauglustaine. 2020. "Data-Driven Modeling of Fuel Consumption for Turboprop-Powered Civil Airliners" Energies 13, no. 7: 1695. https://doi.org/10.3390/en13071695
APA StyleMarinus, B. G., & Hauglustaine, A. (2020). Data-Driven Modeling of Fuel Consumption for Turboprop-Powered Civil Airliners. Energies, 13(7), 1695. https://doi.org/10.3390/en13071695






