Transmission Lines in Poland and Space Weather Effects
Abstract
1. Introduction
2. Geomagnetic Conditions in Poland in 2010 and 2014
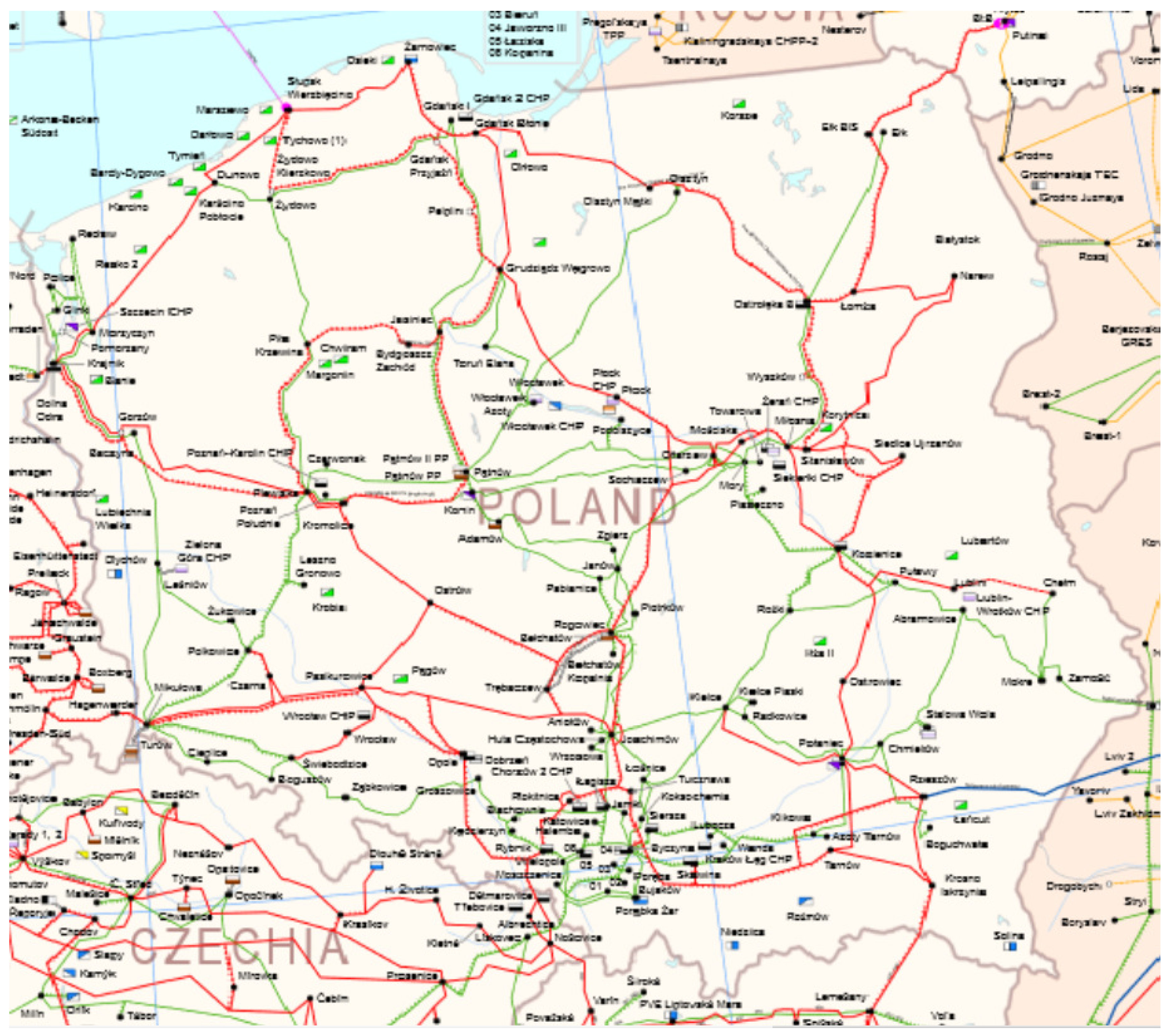
3. Characteristic Features of Transmission Lines in South Poland and General Reasons of Their Failures
Electrical Grids Failures’ Classification
4. Results and Discussion
5. Conclusions
- The assessment of the electrical grid failures’ total number in the categories of failures, which might be connected to space weather effects near the solar minimum, i.e., in 2010 and around the sunspot maximum, January–July 2014, shows that the number of failures is twice larger in January–July 2014 than in 2010. Thus, it can be an indication of solar cycle phase dependency.
- The presented rapid growth of the number of electrical grids failures coincides in time (mostly with some delay) with an increase of geomagnetic activity mirrored in the increase of geoelectric field’ disturbances reflected in GICs. This suggests a link to the space weather effects.
- The delay in EGFs emergence may be connected to some cumulative effect due to the result of transient states and their propagation in the distribution network.
- Performed analysis implies that prominent phenomena having a solar origin had an impact on the efficiency of the transmission lines in southern Poland in 2010 and January–July 2014.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| CME | Coronal Mass Ejection |
| DSO | Distribution System Operators |
| EGF | Electrical Grid Failure |
| GIC | Geomagnetically Induced Current |
| IMGW-PIB | The Institute Of Meteorology And Water Management-National Research Institute |
| NOAA | National Oceanic and Atmospheric Administration |
| SA | Solar Activity |
References
- Szmitkowski, P.; Zakrzewska, S.; Gil, A.; Swiderski, P. Capabilities of Polish power plants-advantages and threats. Przeglad Elektrotech. 2019, 95, 188–193. [Google Scholar] [CrossRef]
- Zakrzewska, S.; Gil-Swiderska, A. Energetic critical infrastructure in Poland—Perspectives and threats. Rynek Energii 2012, 5, 55–64. [Google Scholar]
- Zbunjak, Z.; Kuzle, I. System Integrity Protection Scheme (SIPS) Development and an Optimal Bus-Splitting Scheme Supported by Phasor Measurement Units (PMUs). Energies 2019, 12, 3404. [Google Scholar] [CrossRef]
- Lanzerotti, L.J. Space Weather: Historical and Contemporary Perspectives. Space Sci. Rev. 2017, 212, 1253–1270. [Google Scholar] [CrossRef]
- Lindborg, L.; Bartlett, D.; Beck, P.; McAulay, I.; Schnuer, K.; Schraube, H.; Spurny, F. Cosmic radiation exposure of aircraft crew: Compilation of measured and calculated data. Radiat. Protect. Dosim. 2004, 110, 417–422. [Google Scholar] [CrossRef]
- Sigurdson, A.J.; Ron, E. Cosmic radiation exposure and cancer risk among flight crew. Cancer Investig. 2004, 22, 743–761. [Google Scholar] [CrossRef]
- Hathaway, D.H. The Solar Cycle. Living Rev. Sol. Phys. 2015, 12. [Google Scholar] [CrossRef]
- Pirjola, R. Geomagnetically induced currents during magnetic storms. IEEE Trans. Plasma Sci. 2000, 28, 1867–1873. [Google Scholar] [CrossRef]
- Viljanen, A. Relation of Geomagnetically Induced Currents and Local Geomagnetic 700 Variations. IEEE Trans. Power Deliv. 1998, 13, 1285–1290. [Google Scholar] [CrossRef]
- Eastwood, J.P.; Biffis, E.; Hapgood, M.A.; Green, L.; Bisi, M.M.; Bentley, R.D.; Wicks, R.; McKinnell, L.A.; Gibbs, M.; Burnett, C. The Economic Impact of Space Weather: Where Do We Stand? Risk Anal. 2017, 37, 206–218. [Google Scholar] [CrossRef]
- Gaunt, C.T. Reducing uncertainty—Responses for electricity utilities to severe solar storms. J. Space Weather Space Clim. 2014. [Google Scholar] [CrossRef]
- Gaunt, C.T.; Coetzee, G. Transformer failures in regions incorrectly considered to have low GIC-risk. In Proceedings of the 2007 IEEE Lausanne Power Tech, Lausanne, Switzerland, 1–5 July 2007; pp. 807–812. [Google Scholar]
- Carter, B.A.; Yizengaw, E.; Pradipta, R.; Halford, A.J.; Norman, R.; Zhang, K. Interplanetary shocks and the resulting geomagnetically induced currents at the equator. Geophys. Res. Lett. 2015, 42, 6554–6559. [Google Scholar] [CrossRef]
- Carter, B.A.; Yizengaw, E.; Pradipta, R.; Weygand, J.M.; Piersanti, M.; Pulkkinen, A.; Moldwin, M.B.; Norman, R.; Zhang, K. Geomagnetically induced currents around the world during the 17 March 2015 storm. J. Geophys. Res. Space Phys. 2016, 121, 496–507. [Google Scholar] [CrossRef]
- Anuar, N.M.; Kasran, F.A.M.; Zatul, I.A.L.; Ab Rahim, S.A.E.; Manut, A.; Jusoh, M.H.; Hadi, N.A.; Yoshikawa, A. Estimation of Time Derivative of Horizontal Geomagnetic Component for GIC Assesment in Malaysia during Quiet Period. In Proceedings of the 8th International Conference on System Engineering and Technology (ICSET), Bandung, Indonesia, 15–16 October 2018; Institute of Electrical and Electronics Engineers Inc.: Bandung, Indonesia, 2018; pp. 118–122. [Google Scholar]
- Gil, A.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Wawrzynczak, A.; Zakrzewska, S. Does time series analysis confirms the relationship between space weather effects and the failures of electrical grids in South Poland? J. Math. Ind. 2019, 9. [Google Scholar] [CrossRef]
- Vybostokova, T.; Svanda, M. Statistical Analysis of the Correlation between Anomalies in the Czech Electric Power Grid and Geomagnetic Activity. Space Weather 2019, 17, 1208–1218. [Google Scholar] [CrossRef]
- Zois, J.P. Solar activity and transformer failures in the Greek national electric grid. J. Space Weather Space Clim. 2013. [Google Scholar] [CrossRef]
- Tozzi, R.; De Michelis, P.; Coco, I.; Giannattasio, F. A preliminary risk assessment of geomagnetically induced currents over the Italian territory. Space Weather 2019, 17, 46–58. [Google Scholar] [CrossRef]
- Clilverd, M.A.; Rodger, C.J.; Brundell, J.B.; Dalzell, M.; Martin, I.; Mac Manus, D.H.; Thomson, N.R.; Petersen, T.; Obana, Y. Long-lasting geomagnetically induced currents and harmonic distortion observed in New Zealand during the 7–8 September 2017 disturbed period. Space Weather 2018, 16, 704–717. [Google Scholar] [CrossRef]
- Bolduc, L. GIC observations and studies in the Hydro Qubec power system. J. Atmos. Sol. Terr. Phys. 2002, 64, 1793–1802. [Google Scholar] [CrossRef]
- Boteler, D.H.; Pirola, R.J.; Nevanlinna, H. The effects of geomagnetic disturbances on electrical systems at the Earth’s surface. Adv. Space Res. 1998, 22, 17–27. [Google Scholar] [CrossRef]
- Makinen, T. Geomagnetically Induced Currents in the Finnish Power Transmission System; Finnish Meteorological Institute, Geophysical Publications: Helsinki, Finland, 1993. [Google Scholar]
- Watari, S.; Kunitake, M.; Kitamura, K.; Hori, T.; Kikuchi, T.; Shiokawa, K.; Nishitani, N.; Kataoka, R.; Kamide, Y.; Aso, T.; et al. Measurements of geomagnetically induced current in a power grid in Hokkaido, Japan. Space Weather 2009. [Google Scholar] [CrossRef]
- Mac Manus, D.H.; Rodger, C.J.; Dalzell, M.; Thomson, A.W.P.; Clilverd, M.A.; Petersen, T.; Wolf, M.M.; Thomson, N.R.; Divett, T. Long term Geomagnetically Induced Current Observations in New Zealand: Earth return Corrections and Geomagnetic Field Driver. Space Weather 2017, 15, 1020–1038. [Google Scholar] [CrossRef]
- Rodger, C.J.; Mac Manus, D.H.; Dalzell, M.; Thomson, A.W.P.; Clarke, E.; Petersen, T.; Divett, T. Longterm geomagnetically induced current observations from New Zealand: Peak current estimates for extreme geomagnetic storms. Space Weather 2017, 15, 1447–1460. [Google Scholar] [CrossRef]
- Pulkkinen, A.; Lindahl, S.; Viljanen, A.; Pirjola, R. Geomagnetic storm of 29–31 October 2003: Geomagnetically induced currents and their relation to problems in the Swedish high-voltage power transmission system. Space Weather 2005. [Google Scholar] [CrossRef]
- Thorberg, R. Risk Analysis of Geomagnetically Induced Currents in Power Systems. Available online: http://www.iea.lth.se/publications/MS-Theses/Fulldocument/5296$_{}$full$_{}$document$_{}$GIC.pdf (accessed on 1 March 2020).
- Wik, M. The Sun, Space Weather and Effects. Ph.D. Thesis, Department of Physics, Lund University, Lund, Sweden, 2008. [Google Scholar]
- Marusek, J.A. Solar Storm Threat Analysis. Impact. Indiana. Available online: https://pdfs.semanticscholar.org/9fa3/16d0b9bb073d9826d9d4295b36e0f282d3eb.pdf (accessed on 26 November 2019).
- Ramsingh, S.S.; SrLeekumar, S.; Banola, S.; Emperumal, K.; Tiwari, P.; Kumar, B.S. Low-latitude ionosphere response to super geomagnetic storm of 17/18 March 2015: Results from a chain of ground-based observations over Indian sector. J. Geophys. Res. Space Phys. 2015, 120, 10864–10882. [Google Scholar] [CrossRef]
- Kozyreva, O.V.; Pilipenko, V.A.; Belakhovsky, V.B.; Sakharov, Y.A. Ground geomagnetic field and GIC response to March 17, 2015, storm. Earth Planets Space 2018. [Google Scholar] [CrossRef]
- Kamide, Y.; Kusano, K. No major solar flares but the largest geomagnetic storm in the present solar cycle. Space Weather 2015, 13, 365–367. [Google Scholar] [CrossRef]
- Cannon, P.S. Extreme Space Weather: Impacts on Engineered Systems and Infrastructure; Royal Academy of Engineering: London, UK, 2013; Available online: http://www.raeng.org.uk/publications/reports/space-weather-full-report (accessed on 26 November 2019).
- Juusola, L.; Viljanen, A.; Van de Kamp, M.; Tanskanen, E.I.; Vanhamaki, H.; Partamies, N.; Kauristie, K. High-latitude ionospheric equivalent currents during strong space storms: Regional perspective. Space Weather 2015, 13, 49–60. [Google Scholar] [CrossRef]
- Oughton, E.J.; Skelton, A.; Horne, R.B.; Thomson, A.W.P.; Gaunt, C.T. Quantifying the daily economic impact of extreme space weather due to failure in electricity transmission infrastructure. Space Weather 2017, 15, 65–83. [Google Scholar] [CrossRef]
- Pulkkinen, A.; Viljanen, A.; Pirjola, R. Estimation of geomagnetically induced current levels from different input data. Space Weather 2006. [Google Scholar] [CrossRef]
- Schulte in den Bäumen, H.; Moran, D.; Lenzen, M.; Cairns, I.; Steenge, A. How severe space weather can disrupt global supply chains. Nat. Hazards Earth Syst. Sci. 2014, 14, 2749–2759. [Google Scholar] [CrossRef]
- Schrijver, C.J.; Dobbins, R.; Murtagh, W.; Petrinec, S.M. Assessing the impact of space weather on the electric power grid based on insurance claims for industrial electrical equipment. Space Weather 2014, 12, 487–498. [Google Scholar] [CrossRef]
- Menvielle, M.; Iyemori, T.; Marchaudon, A.; Nosé, M. Geomagnetic Indices. In Geomagnetic Observations and Models; Mandea, M., Korte, M., Eds.; IAGA Special Sopron Book Series; Springer: Dordrecht, The Netherlands, 2011; Volume 5. [Google Scholar]
- Jankowski, J.; Sucksdorff, C. Guide for Magnetic Measurements and Observatory Practice; PAN Press: Warsaw, Poland, 1996. [Google Scholar]
- Love, J.J. Magnetic monitoring of Earth and space. Phys. Today 2008, 61, 31–37. [Google Scholar] [CrossRef]
- Bartels, J.; Heck, N.H.; Johnston, H.F. The three-hour-range index measuring geomagnetic activity. Terr. Magnet. Atmos. Electr. 1939, 44, 411–454. [Google Scholar] [CrossRef]
- Rasson, J.J. Observatories, instrumentation. In Encyclopedia of Geomagnetism and Paleomagnetism; Gubbins, D., Herrero-Bervera, E., Eds.; Springer: New York, NY, USA, 2007; pp. 711–713. [Google Scholar]
- Love, J.J.; Chulliat, A. An International Network of Magnetic Observatories. EOS 2013, 94, 373–374. [Google Scholar] [CrossRef]
- Nowozynski, K.; Ernst, T.; Jankowski, J. Adaptive smoothing method for computer derivation of K-indices. Geophys. J. Int. 1991, 104, 85–93. [Google Scholar] [CrossRef]
- Szmitkowski, P.; Gil-Swiderska, A.; Zakrzewska, S. Electrical energy infrastructure in Poland and its sensitivity to failures as part of the energy security system. Energy Policy J. 2019, 22, 59–80. [Google Scholar] [CrossRef]
- Adam, A.; Pracser, E.; Wesztergom, V. Estimation of the electric resistivity distribution (EURHOM) in the European lithosphere in the frame of the EURISGIC WP2 project. Acta Geod. Geophys. Hung. 2012, 47, 377–387. [Google Scholar] [CrossRef]
- Viljanen, A.; Pirjola, R.; Wik, M.; Adam, A.; Pracser, E.; Sakharov, Y.; Katkalov, J. Continental scale modelling of geomagnetically induced currents. J. Space Weather Space Clim. 2012. [Google Scholar] [CrossRef]
- Viljanen, A.; Pirjola, R. Statistics on geomagnetically-induced currents in the Finnish 400 kV power system based on recordings of geomagnetic variations. J. Geomagnet. Geoelectr. 1989, 41, 411–420. [Google Scholar] [CrossRef]
- Demetrescu, C.; Dobrica, V.; Greculeasa, R.; Stefan, C. The induced surface electric response in Europe to 2015 St. Patrick’s Day geomagnetic storm. J. Atmos. Sol. Terr. Phys. 2018, 180, 106–115. [Google Scholar] [CrossRef]
- Pirjola, R.; Lehtinen, M. Currents produced in the Finnish 400 kV power transmission grid and in the Finnish natural gas pipeline by geomagnetically induced electric fields. Ann. Geophys. 1985, 3, 485–491. [Google Scholar]
- Wik, M.; Viljanen, A.; Pirjola, R.; Pulkkinen, A.; Wintoft, P.; Lundstedt, H. Calculation of geomagnetically induced currents in the 400 kV power grid in southern Sweden. Space Weather 2008, 6, 7. [Google Scholar] [CrossRef]



| Main Groups | Number in 2010 | % in 2010 | Number in 2014 | % in 2014 | |
|---|---|---|---|---|---|
| A | meteorological effects | 3653 | 14.3 | 9004 | 29.9 |
| B | operational shutdowns | 16,614 | 64.9 | 9703 | 32.2 |
| C | vandalism | 824 | 3.2 | 792 | 2.6 |
| D | aging | 1917 | 7.5 | 6209 | 20.6 |
| E | electronics devices | 32 | 0.1 | 1181 | 3.9 |
| F | unidentified | 2576 | 10 | 3266 | 10.8 |
| TOTAL | 25,616 | 100.0 | 30,155 | 100.0 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gil, A.; Modzelewska, R.; Moskwa, S.; Siluszyk, A.; Siluszyk, M.; Wawrzynczak, A.; Pozoga, M.; Domijanski, S. Transmission Lines in Poland and Space Weather Effects. Energies 2020, 13, 2359. https://doi.org/10.3390/en13092359
Gil A, Modzelewska R, Moskwa S, Siluszyk A, Siluszyk M, Wawrzynczak A, Pozoga M, Domijanski S. Transmission Lines in Poland and Space Weather Effects. Energies. 2020; 13(9):2359. https://doi.org/10.3390/en13092359
Chicago/Turabian StyleGil, Agnieszka, Renata Modzelewska, Szczepan Moskwa, Agnieszka Siluszyk, Marek Siluszyk, Anna Wawrzynczak, Mariusz Pozoga, and Sebastian Domijanski. 2020. "Transmission Lines in Poland and Space Weather Effects" Energies 13, no. 9: 2359. https://doi.org/10.3390/en13092359
APA StyleGil, A., Modzelewska, R., Moskwa, S., Siluszyk, A., Siluszyk, M., Wawrzynczak, A., Pozoga, M., & Domijanski, S. (2020). Transmission Lines in Poland and Space Weather Effects. Energies, 13(9), 2359. https://doi.org/10.3390/en13092359





