Experimental Study of Single Taylor Bubble Rising in Stagnant and Downward Flowing Non-Newtonian Fluids in Inclined Pipes
Abstract
:1. Introduction
2. Literature Review
2.1. Drift Velocity Correlation
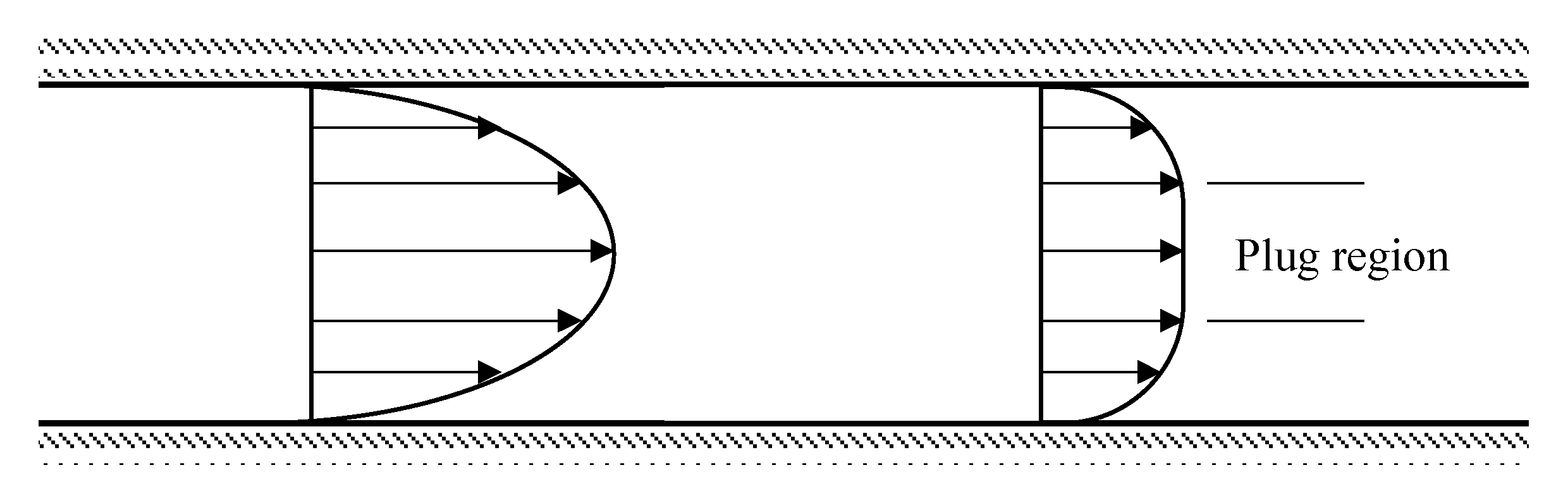
2.2. Theoretical Part
3. Experimental Work
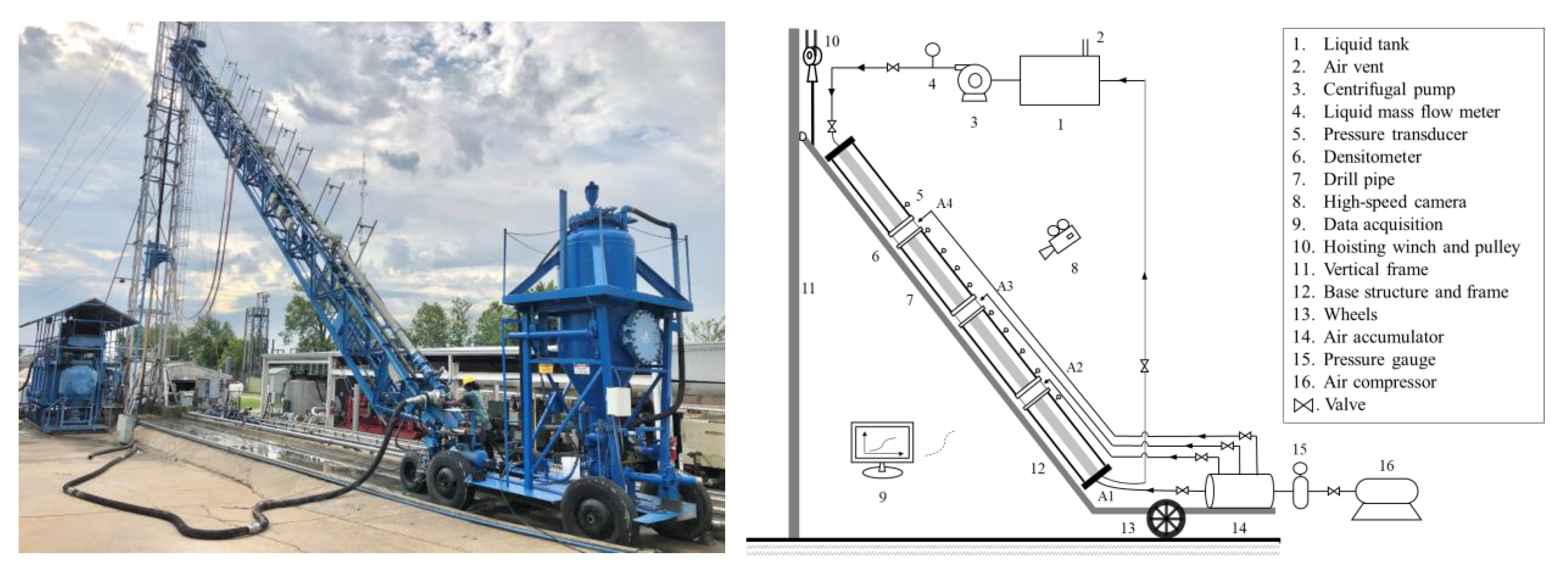
3.1. Experimental Facility
- Ten fiber optic pressure transducers, sensitive enough to detect small pressure variations with an accuracy of ±0.1%, were installed approximately 5 ft apart for differential pressure and velocity measurements. The pressure and time resolutions of the sensors were 0.001 psi and 0.1 sec, respectively.
- Three non-contact nuclear densitometers (X96S: Valencia, CA, USA) using a gamma radiation technique, which covered the entire pipe cross section area, for holdup and velocity measurements, were located approximately 20 ft from each end of the test section, and in its middle (spaced about 21 ft apart). The resolutions in density and time were 0.001 ppg and 0.1 sec, respectively. All densitometers were pre-calibrated by the full liquid/gas phase based on two-phase flow systems.
- Eight high-speed Amcrest security video cameras (Houston, TX, USA) were used for visualization. The non-Newtonian fluid applied in the experiment was opaque; therefore, cameras were only used in the water test.
3.2. Experimental Procedure and Test Matrix
3.3. Determination of Bubble Velocity and Length
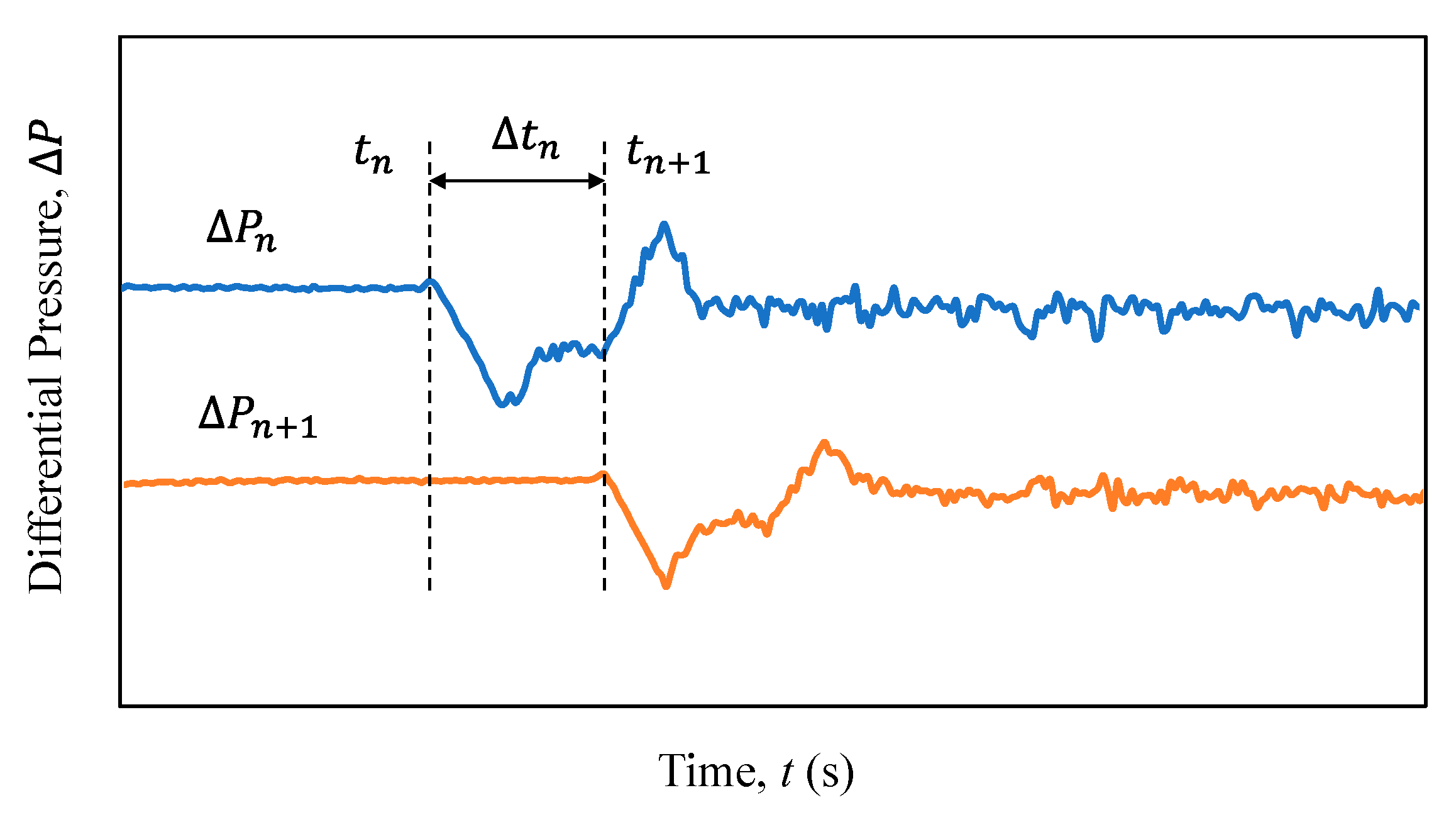
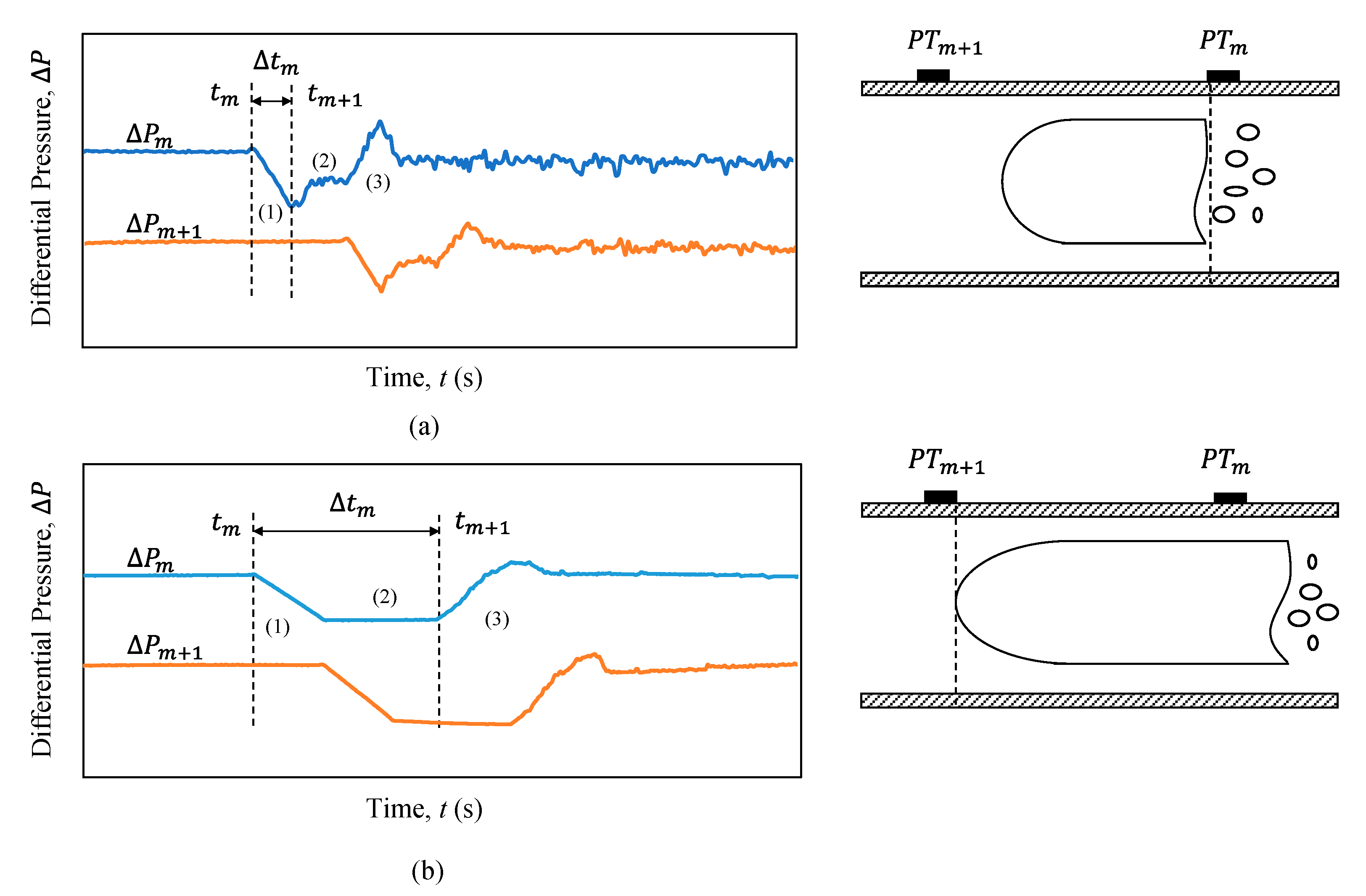
3.3.1. Velocity of Taylor Bubbles
3.3.2. Length of Taylor Bubbles
4. Results and Discussion
4.1. Static Tests
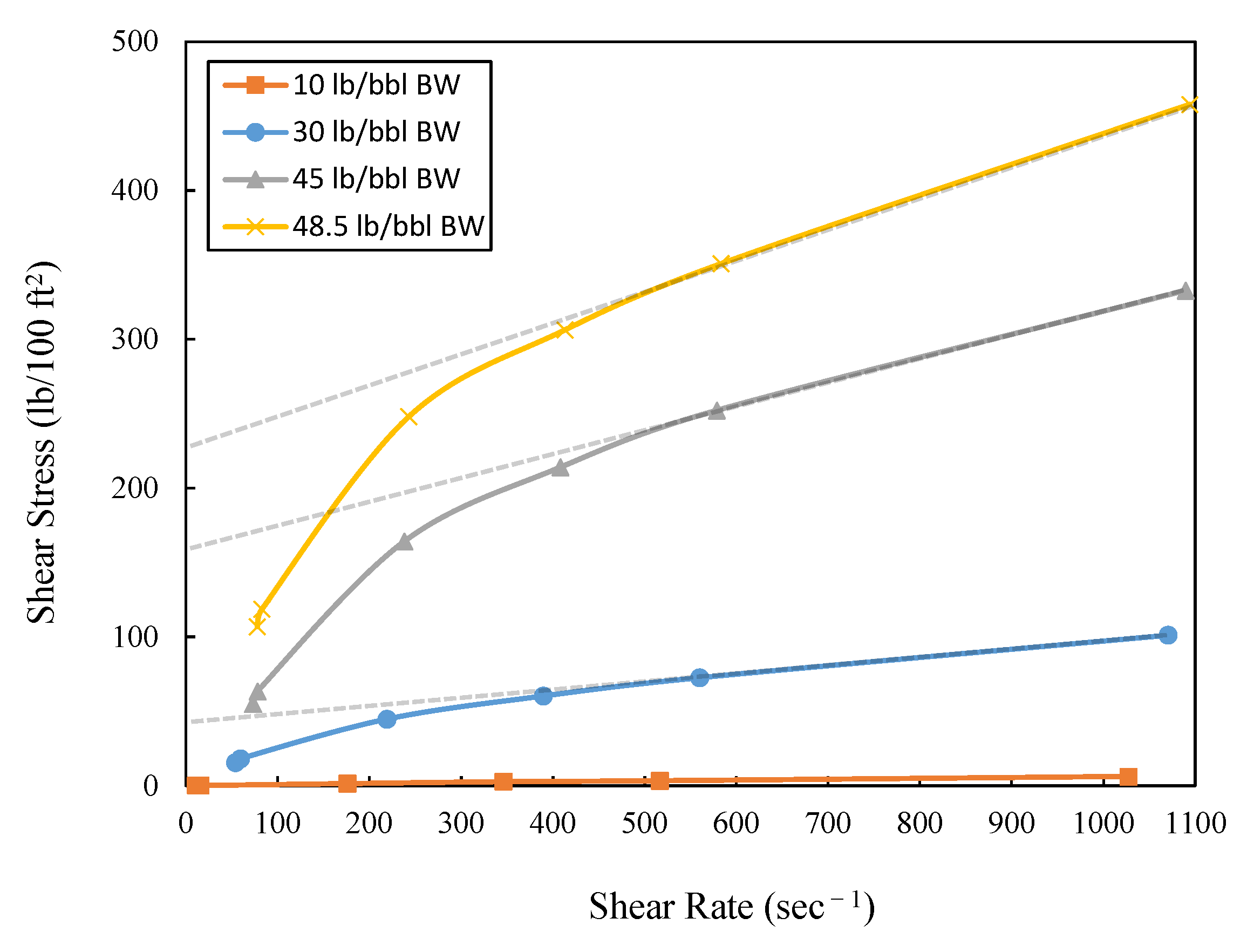
4.1.1. Influence of and on
4.1.2. Proposed Drift Velocity Correlation
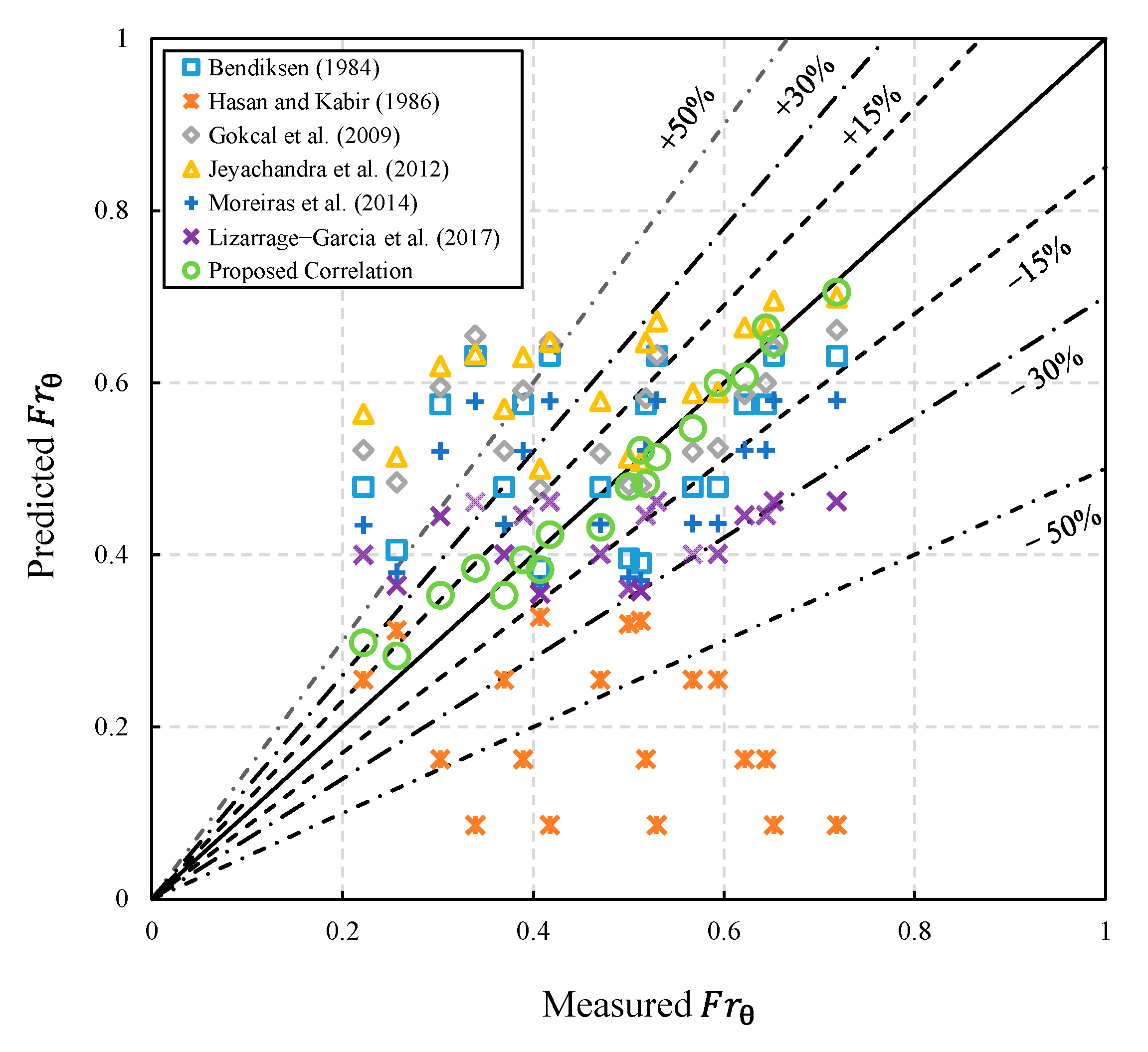
Performance of Proposed Correlation
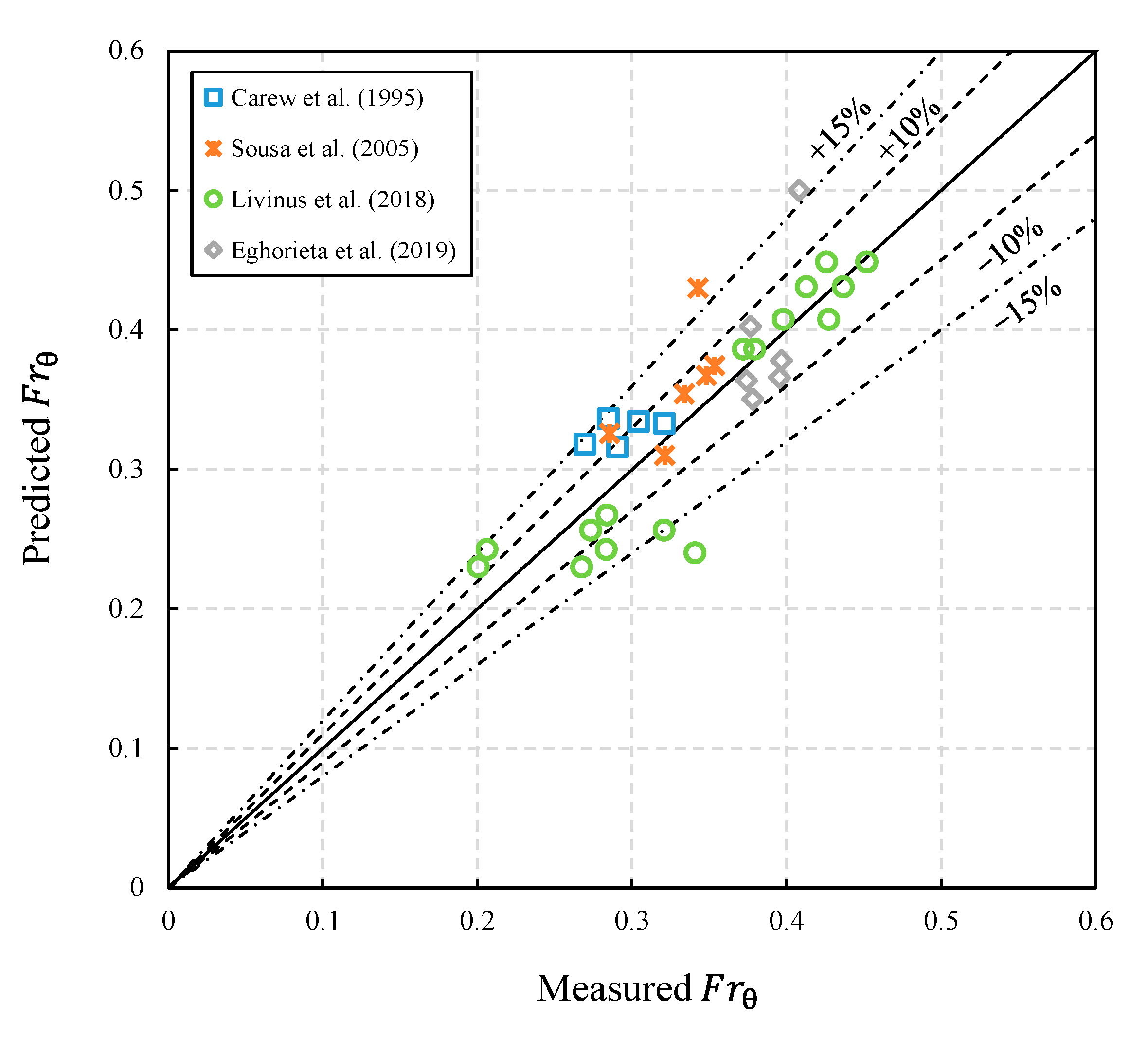
Validation of the Proposed Correlation
4.2. Countercurrent Flow Tests
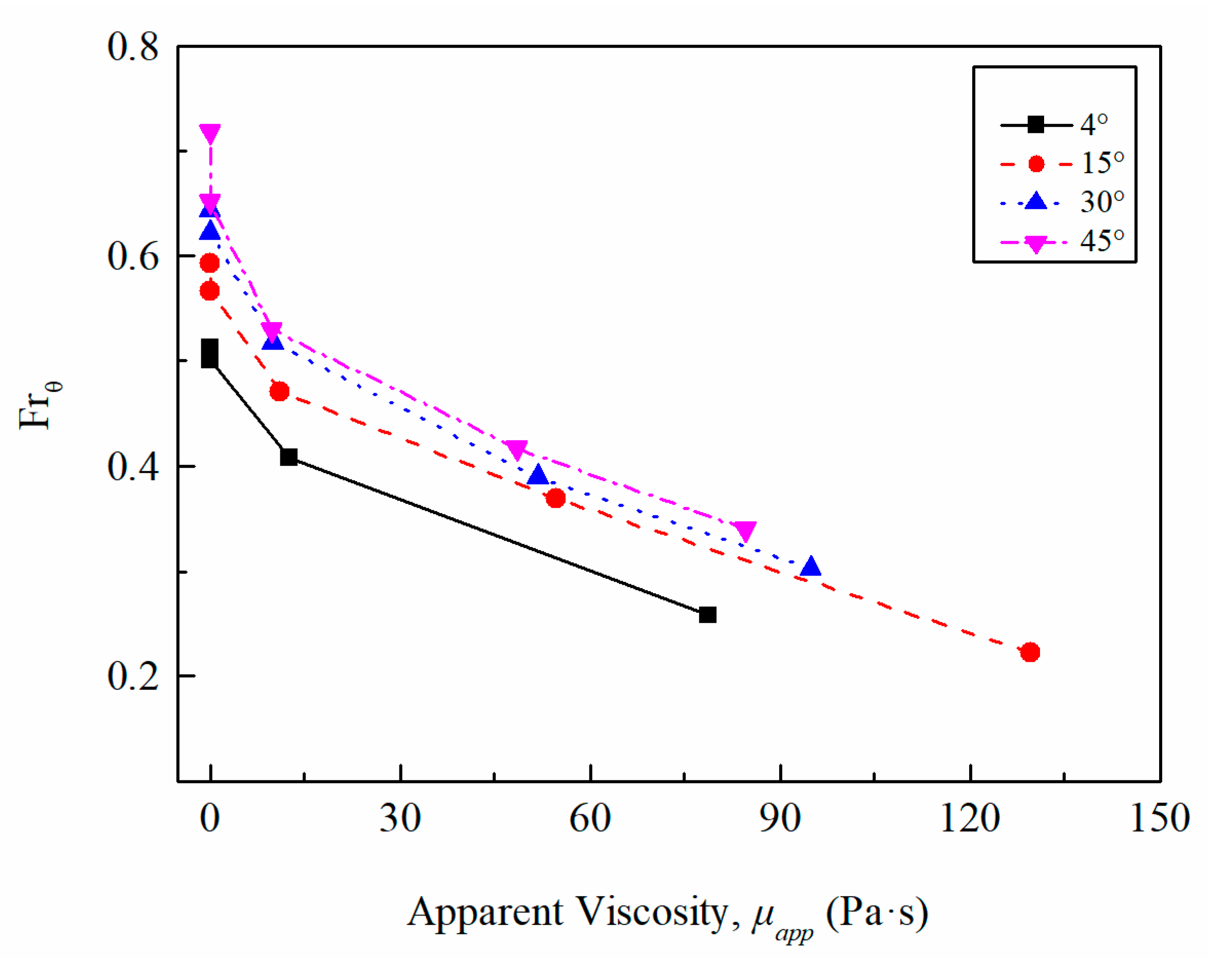
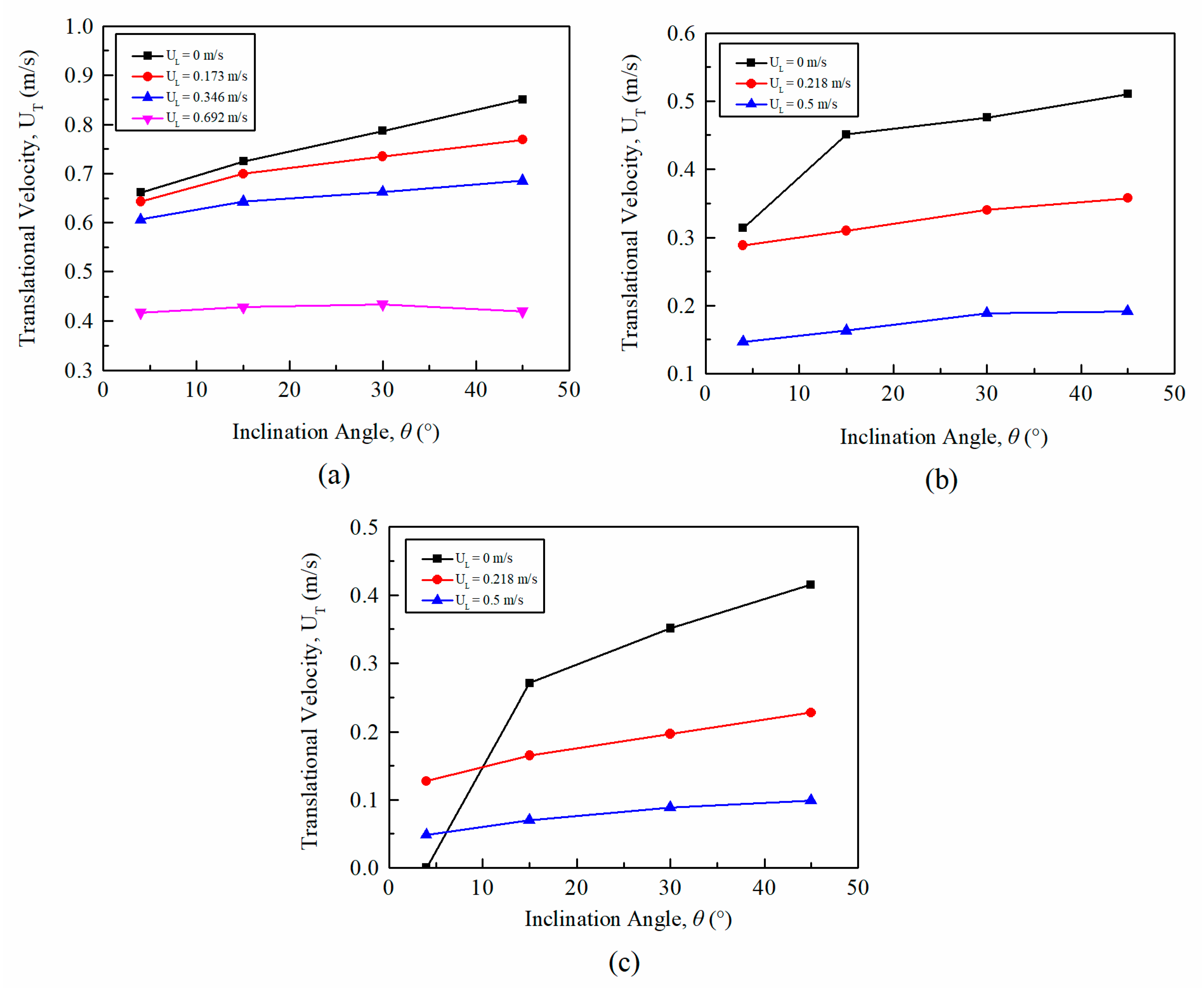
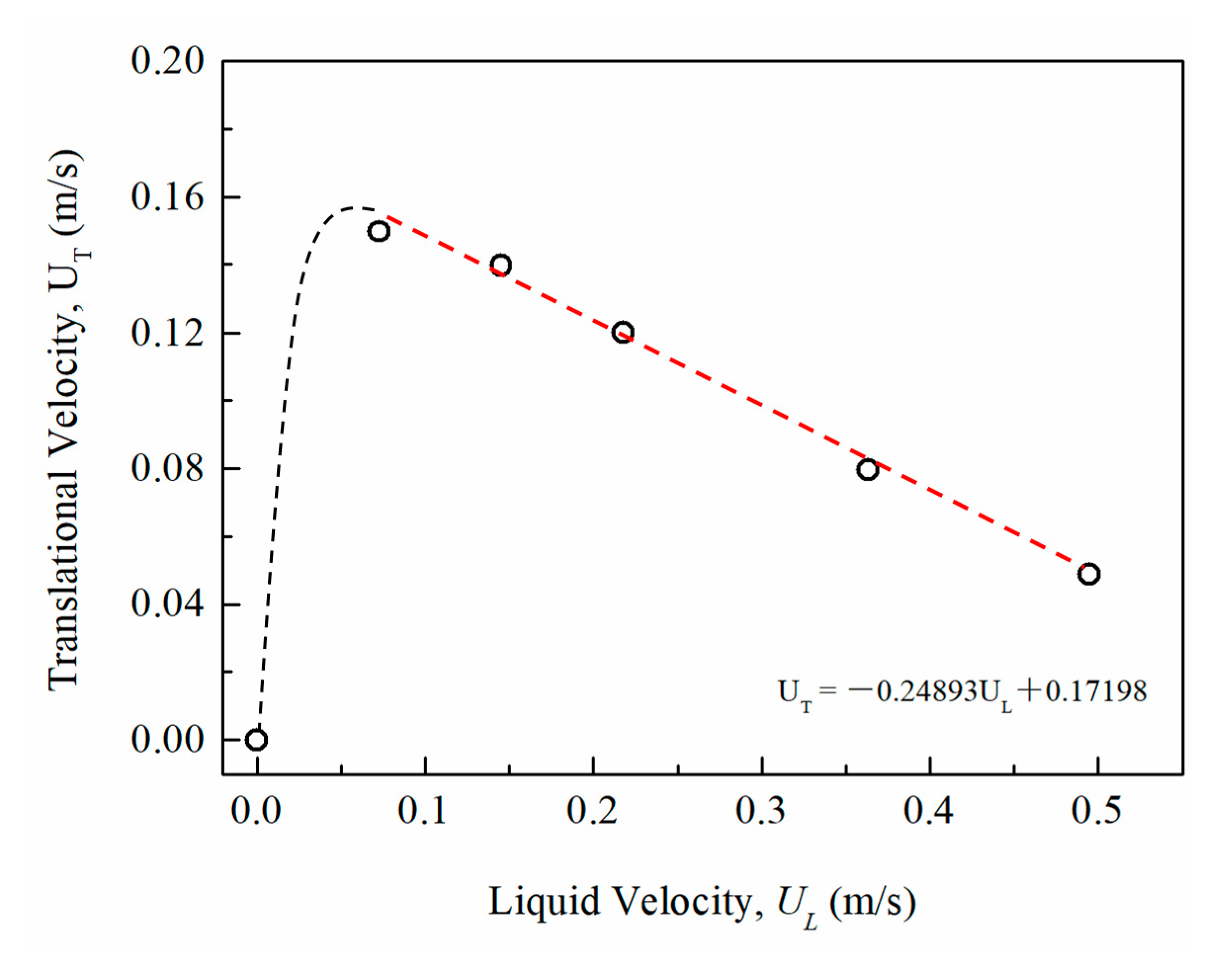
4.2.1. Influence of on
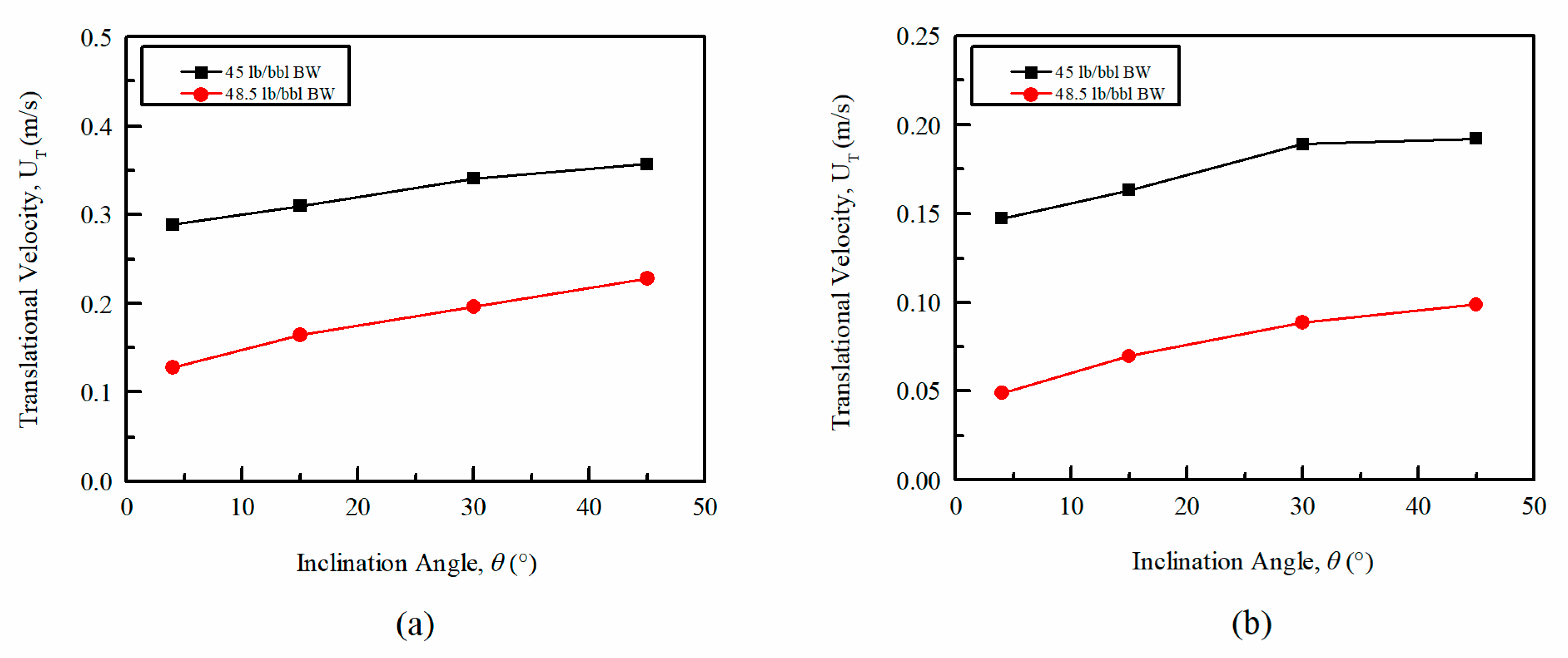
4.2.2. Influence of Liquid Viscosity on
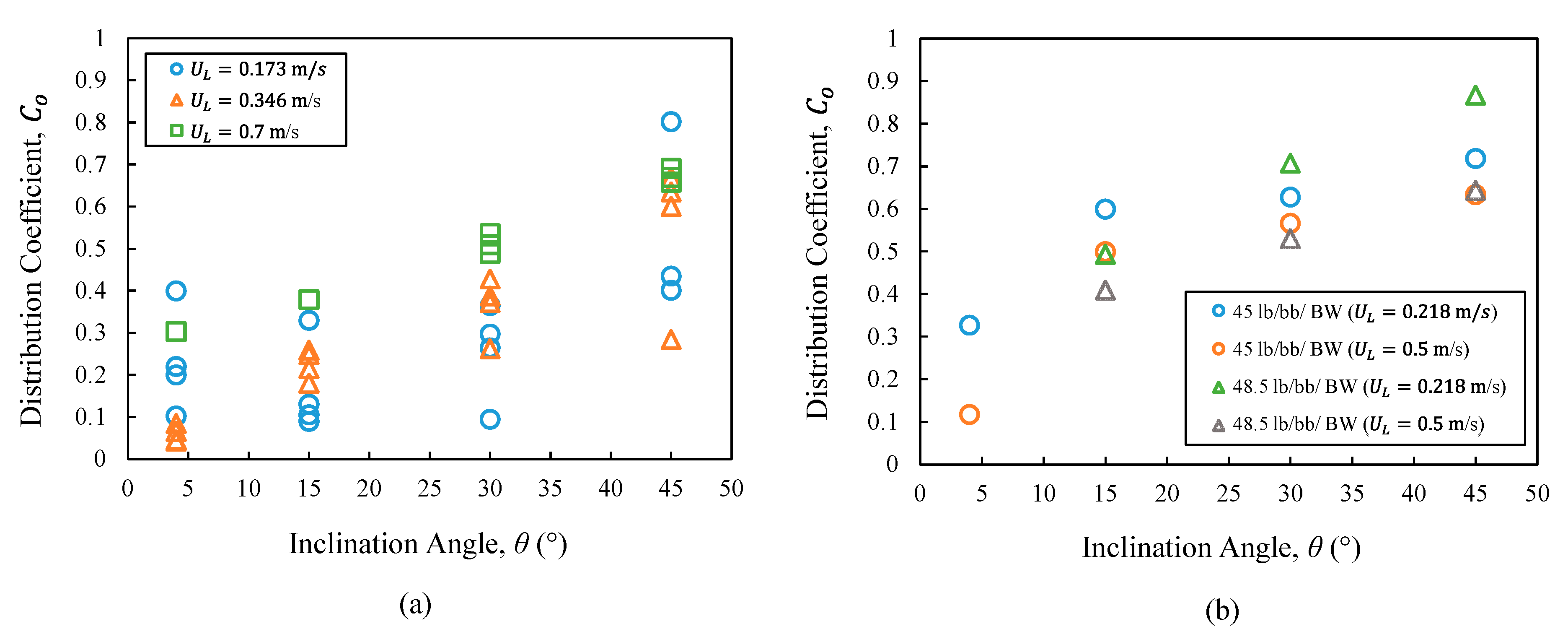
4.2.3. Distribution coefficient,
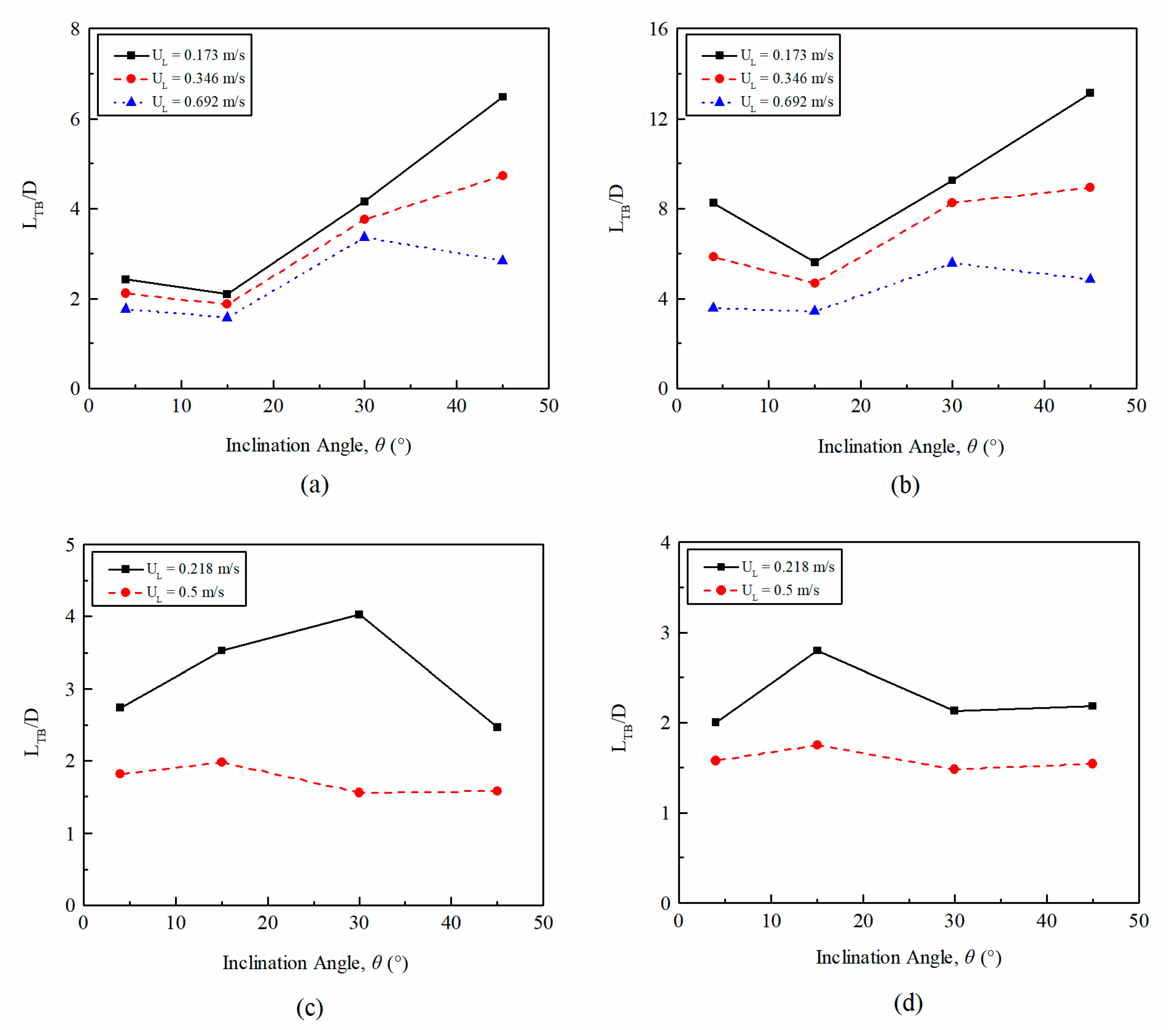
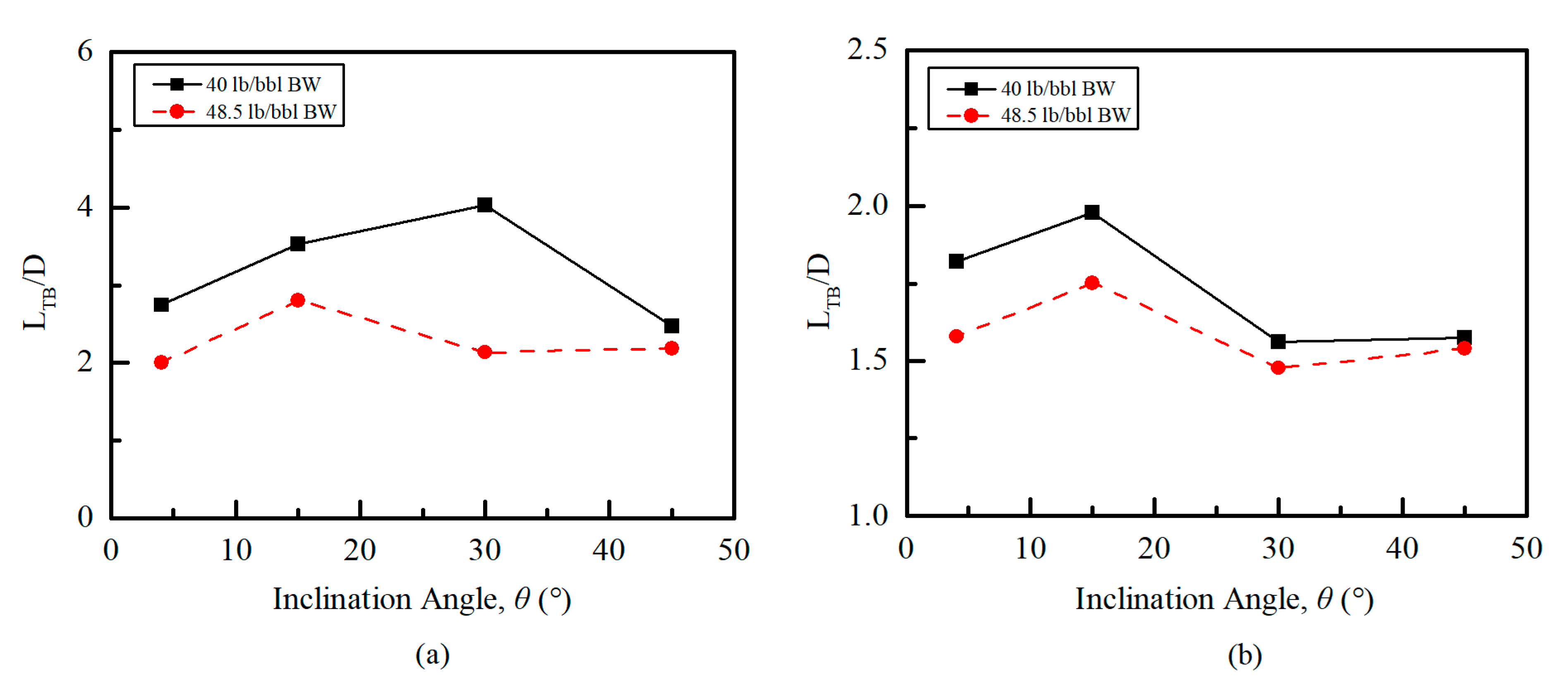
4.2.4. Length of Taylor Bubbles
Influence of and on
Influence of Liquid Viscosity on
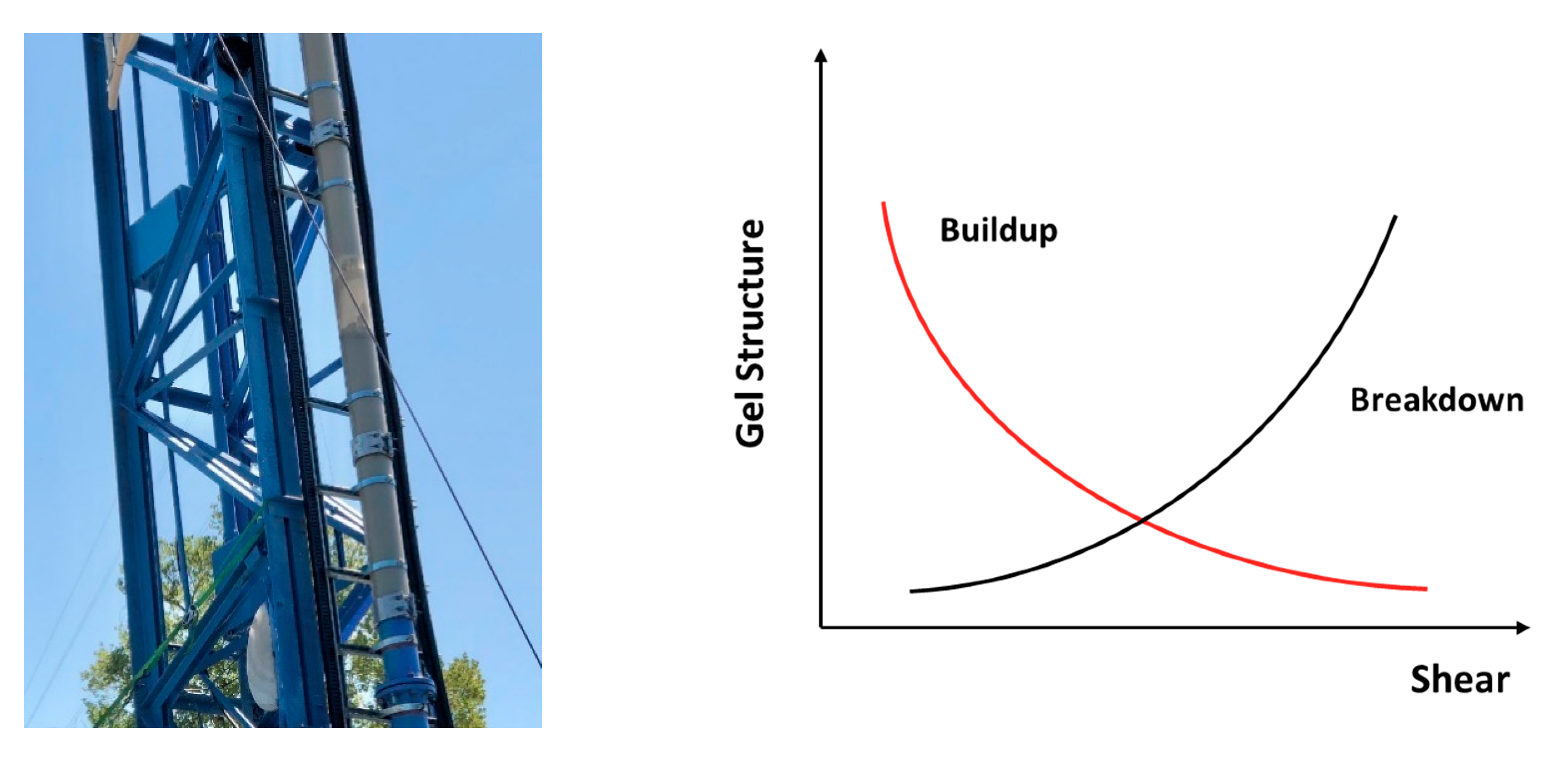
4.3. Unexpected Case: The Stagnant Taylor Bubble
5. Conclusions
- 1.
- A flow rig has been built for the purpose of investigating the movement of a single Taylor bubble rising in stagnant and downward flowing bentonite–water mixture in inclined pipes (4° < < 45° from vertical).
- 2.
- The measured drift velocity () data presented in this study can contribute to improve the knowledge of a single Taylor bubble rising in stagnant non-Newtonian fluids having a yield point.
- 3.
- The experimental results indicate that for all fluids tested, the bubble velocity increased as the inclination angles () were increased, while velocity decreased with an increase in plastic viscosity () and yield point (). The length of the Taylor bubble () decreased as the downward flowing liquid velocity () and were increased. The bubble velocity was found to be independent of the .
- 4.
- A reduction in drift velocity along its migration was detected, because increasing the concentration of bentonite results in a strong gel strength buildup. Eventually, a stagnant Taylor bubble can occur.
- 5.
- The drift velocity is formulated by combining a function of and a yield–power-law correlation in terms of apparent viscosity (), making it applicable for non-Newtonian fluids with the Eötvös number () ranging from 3212 to 3405 and apparent viscosity () ranging from 0.001 to 129 . The proposed correlation shows good performance for predicting drift velocity from both the present study (mean absolute relative difference is 0.0702) and a new database (mean absolute relative difference is 0.09614).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Fluid | (°) | ID (m) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Water | 4 | 0.152 | 998 | 0.001 | 0 | 0.001 | 3240 | 0.661 | 0.541 |
| 15 | 0.152 | 998 | 0.001 | 0 | 0.001 | 3240 | 0.725 | 0.593 | |
| 30 | 0.152 | 998 | 0.001 | 0 | 0.001 | 3240 | 0.787 | 0.644 | |
| 45 | 0.152 | 998 | 0.001 | 0 | 0.001 | 3240 | 0.850 | 0.695 | |
| 10 lb/bbl BW | 4 | 0.152 | 1015 | 0.002 | 0.75 | 0.189 | 3212 | 0.627 | 0.513 |
| 15 | 0.152 | 1015 | 0.002 | 0.75 | 0.167 | 3212 | 0.693 | 0.567 | |
| 30 | 0.152 | 1015 | 0.002 | 0.75 | 0.153 | 3212 | 0.756 | 0.618 | |
| 45 | 0.152 | 1015 | 0.002 | 0.75 | 0.146 | 3212 | 0.792 | 0.648 | |
| 30 lb/bbl BW | 4 | 0.152 | 1047 | 0.027 | 41.6 | 12.765 | 3314 | 0.540 | 0.442 |
| 15 | 0.152 | 1047 | 0.027 | 41.6 | 11.045 | 3314 | 0.573 | 0.469 | |
| 30 | 0.152 | 1047 | 0.027 | 41.6 | 10.042 | 3314 | 0.633 | 0.518 | |
| 45 | 0.152 | 1047 | 0.027 | 41.6 | 9.816 | 3314 | 0.660 | 0.540 | |
| 45 lb/bbl BW | 6 | 0.152 | 1063 | 0.076 | 162 | 78.666 | 3364 | 0.314 | 0.257 |
| 15 | 0.152 | 1063 | 0.076 | 162 | 54.734 | 3364 | 0.451 | 0.369 | |
| 30 | 0.152 | 1063 | 0.076 | 162 | 51.963 | 3364 | 0.476 | 0.389 | |
| 45 | 0.152 | 1063 | 0.076 | 162 | 48.471 | 3364 | 0.510 | 0.417 | |
| 48.5 lb/bbl BW | 4 | 0.152 | 1076 | 0.101 | 230 | undefined | 3405 | 0 | 0 |
| 15 | 0.152 | 1076 | 0.101 | 230 | 129.443 | 3405 | 0.271 | 0.222 | |
| 30 | 0.152 | 1076 | 0.101 | 230 | 94.803 | 3405 | 0.351 | 0.287 | |
| 45 | 0.152 | 1076 | 0.101 | 230 | 84.606 | 3405 | 0.415 | 0.339 |
Appendix B
References
- Evje, S.; Fjelde, K.K. Hybrid Flux-Splitting Schemes for a Two-Phase Flow Model. J. Comput. Phys. 2002, 175, 674–701. [Google Scholar] [CrossRef]
- Fabre, J.; Figueroa-Espinoza, B. Taylor bubble rising in a vertical pipe against laminar or turbulent downward flow: Symmetric to asymmetric shape transition. J. Fluid Mech. 2014, 755, 485–502. [Google Scholar] [CrossRef] [Green Version]
- Fershtman, A.; Babin, V.; Barnea, D.; Shemer, L. On shapes and motion of an elongated bubble in downward liquid pipe flow. Phys. Fluids 2017, 29, 112103. [Google Scholar] [CrossRef]
- Kelessidis, V.C.; Dukler, A. Motion of large gas bubbles through liquids in vertical concentric and eccentric annuli. Int. J. Multiph. Flow 1990, 16, 375–390. [Google Scholar] [CrossRef]
- Daş, G.; Das, P.; Purohit, N.; Mitra, A. Rise velocity of a Taylor bubble through concentric annulus. Chem. Eng. Sci. 1998, 53, 977–993. [Google Scholar] [CrossRef]
- Agarwal, V.; Jana, A.K.; Daş, G.; Das, P.K. Taylor bubbles in liquid filled annuli: Some new observations. Phys. Fluids 2007, 19, 108105. [Google Scholar] [CrossRef]
- Lei, Q.; Xie, Z.; Pavlidis, D.; Salinas, P.; Veltin, J.; Matar, O.K.; Pain, C.C.; Muggeridge, A.H.; Gyllensten, A.J.; Jackson, M.D. The shape and motion of gas bubbles in a liquid flowing through a thin annulus. J. Fluid Mech. 2018, 855, 1017–1039. [Google Scholar] [CrossRef]
- Nicklin, D.J. Two-phase flow in vertical tubes. Trans. Inst. Chem. Eng. 1962, 40, 61–68. [Google Scholar]
- Choi, J.; Pereyra, E.; Sarica, C.; Park, C.; Kang, J.M. An Efficient Drift-Flux Closure Relationship to Estimate Liquid Holdups of Gas-Liquid Two-Phase Flow in Pipes. Energies 2012, 5, 5294–5306. [Google Scholar] [CrossRef] [Green Version]
- Bhagwat, S.M.; Ghajar, A.J. A flow pattern independent drift flux model based void fraction correlation for a wide range of gas–liquid two phase flow. Int. J. Multiph. Flow 2014, 59, 186–205. [Google Scholar] [CrossRef]
- Tang, H.; Bailey, W.; Stone, T.; Killough, J. A Unified Gas-Liquid Drift-Flux Model for Coupled Wellbore-Reservoir Simulation. In Proceedings of the SPE Annual Technical Conference and Exhibition, Calgary, AB, Canada, 30 September–2 October 2019. [Google Scholar]
- Liu, Y.; Tong, T.A.; Ozbayoglu, E.; Yu, M.; Upchurch, E. An improved drift-flux correlation for gas-liquid two-phase flow in hori-zontal and vertical upward inclined wells. J. Pet. Sci. Eng. 2020, 195, 107881. [Google Scholar] [CrossRef]
- Dumitrescue, D.T. Strömung an einer Luftblase im senkrechten Rohr. ZAMM 1943, 23, 139–149. [Google Scholar] [CrossRef]
- Davies, R.M.; Taylor, G.I. The mechanics of large bubbles rising through extended liquids and through liquids in tubes. In Proceedings of the Royal Society of London. Series A. Mathematical and Physical Sciences; The Royal Society: London, UK, 1950; Volume 200, pp. 375–390. [Google Scholar]
- Viana, F.; Pardo, R.; Yánez, R.; Trallero, J.L.; Joseph, D.D. Universal correlation for the rise velocity of long gas bubbles in round pipes. J. Fluid Mech. 2003, 494, 379–398. [Google Scholar] [CrossRef] [Green Version]
- Morgado, A.O.; Miranda, J.M.; Araújo, J.D.; Campos, J.B. Review on vertical gas–liquid slug flow. Int. J. Multiph. Flow 2016, 85, 348–368. [Google Scholar] [CrossRef]
- Bendiksen, K.H. An experimental investigation of the motion of long bubbles in inclined tubes. Int. J. Multiph. Flow 1984, 10, 467–483. [Google Scholar] [CrossRef] [Green Version]
- Hasan, A.R.; Kabir, C.S. Predicting Multiphase Flow Behavior in a Deviated Well. SPE Prod. Eng. 1988, 3, 474–482. [Google Scholar] [CrossRef]
- Gokcal, B.; Al-Sarkhi, A.; Sarica, C. Effects of High Oil Viscosity on Drift Velocity for Horizontal and Upward Inclined Pipes. SPE Proj. Facil. Constr. 2009, 4, 32–40. [Google Scholar] [CrossRef]
- Benjamin, T.B. Gravity currents and related phenomena. J. Fluid Mech. 1968, 31, 209–248. [Google Scholar] [CrossRef]
- Jeyachandra, B.C.; Gokcal, B.; Al-Sarkhi, A.; Sarica, C.; Sharma, A.K. Drift-Velocity Closure Relationships for Slug Two-Phase High-Viscosity Oil Flow in Pipes. SPE J. 2012, 17, 593–601. [Google Scholar] [CrossRef]
- Moreiras, J.; Pereyra, E.; Sarica, C.; Torres, C.F. Unified drift velocity closure relationship for large bubbles rising in stagnant vis-cous fluids in pipes. J. Pet. Sci. Eng. 2014, 124, 359–366. [Google Scholar] [CrossRef]
- Lizarraga-Garcia, E.; Buongiorno, J.; Al-Safran, E.; Lakehal, D. A broadly-applicable unified closure relation for Taylor bubble rise velocity in pipes with stagnant liquid. Int. J. Multiph. Flow 2017, 89, 345–358. [Google Scholar] [CrossRef] [Green Version]
- Carew, P.; Thomas, N.; Johnson, A. A physically based correlation for the effects of power law rheology and inclination on slug bubble rise velocity. Int. J. Multiph. Flow 1995, 21, 1091–1106. [Google Scholar] [CrossRef]
- Majumdar, A.; Das, P.K. Rise of Taylor bubbles through power law fluids–Analytical modelling and numerical simulation. Chem. Eng. Sci. 2019, 205, 83–93. [Google Scholar] [CrossRef]
- Shosho, C.E.; Ryan, M.E. An experimental study of the motion of long bubbles in inclined tubes. Chem. Eng. Sci. 2001, 56, 2191–2204. [Google Scholar] [CrossRef]
- Kamışlı, F. Free coating of a non-Newtonian liquid onto walls of a vertical and inclined tube. Chem. Eng. Process. Process Intensif. 2003, 42, 569–581. [Google Scholar] [CrossRef]
- Sousa, R.; Riethmuller, M.; Pinto, A.; Campos, J.B.L.M. Flow around individual Taylor bubbles rising in stagnant CMC solutions: PIV measurements. Chem. Eng. Sci. 2005, 60, 1859–1873. [Google Scholar] [CrossRef] [Green Version]
- Sousa, R.G.; Riethmuller, M.; Pinto, A.; Campos, J.B.L.M. Flow around individual Taylor bubbles rising in stagnant polyacrylamide (PAA) solutions. J. Non-Newtonian Fluid Mech. 2006, 135, 16–31. [Google Scholar] [CrossRef]
- Rabenjafimanantsoa, A.H.; Time, R.W.; Paz, T. Dynamics of expanding slug flow bubbles in non-Newtonian drilling fluids. Ann. Trans. Nord. Rheol. Soc. 2011, 19, 1–8. [Google Scholar]
- Araújo, J.D.P.; Miranda, J.M.; Campos, J.B.L.M. Taylor bubbles rising through flowing non-Newtonian inelastic fluids. J. Non-Newtonian Fluid Mech. 2017, 245, 49–66. [Google Scholar] [CrossRef]
- Griffith, P.; Wallis, G.B. Two-Phase Slug Flow. J. Heat Transf. 1961, 83, 307–318. [Google Scholar] [CrossRef]
- Lu, X.; Prosperetti, A. Axial stability of Taylor bubbles. J. Fluid Mech. 2006, 568, 173. [Google Scholar] [CrossRef] [Green Version]
- Ozbayoglu, M.; Kuru, E.; Miska, S.; Takach, N. A Comparative Study of Hydraulic Models for Foam Drilling. J. Can. Pet. Technol. 2002, 41. [Google Scholar] [CrossRef]
- Ozbayoglu, M.E.; Miska, S.Z.; Reed, T.; Takach, N. Analysis of the effects of major drilling parameters on cuttings transport effi-ciency for high-angle wells in coiled tubing drilling operations. In Proceedings of the SPE/ICoTa Coiled Tubing Conference and Exhibition, Houston, TX, USA, 23–24 March 2004. [Google Scholar]
- Liu, Y.; Luo, C.; Zhang, L.; Liu, Z.; Xie, C.; Wu, P. Experimental and modeling studies on the prediction of liquid loading onset in gas wells. J. Nat. Gas Sci. Eng. 2018, 57, 349–358. [Google Scholar] [CrossRef]
- Tong, T.A.; Liu, Y.; Ozbayoglu, E.; Yu, M.; Ettehadi, R.; May, R. Threshold velocity of non-Newtonian fluids to initiate solids bed erosion in horizontal conduits. J. Pet. Sci. Eng. 2021, 199, 108256. [Google Scholar] [CrossRef]
- Dadashev, R.K.; Mezhidov, V.K.; Dzhambulatov, R.S.; Elimkhanov, D.Z. Features of isotherms of the surface tension of a bentonite water suspension. Bull. Russ. Acad. Sci. Phys. 2014, 78, 288–290. [Google Scholar] [CrossRef]
- Bourgoyne, A.T., Jr.; Millheim, K.K.; Chenevert, M.E.; Young, F.S., Jr. Applied Drilling Engineering; Society of Petroleum Engineers: Richardson, TX, USA, 1991. [Google Scholar]
- Zukoski, E.E. Influence of viscosity, surface tension, and inclination angle on motion of long bubbles in closed tubes. J. Fluid Mech. 1966, 25, 821–837. [Google Scholar] [CrossRef] [Green Version]
- Tong, T.A.; Yu, M.; Ozbayoglu, E.; Takach, N. Numerical simulation of non-Newtonian fluid flow in partially blocked eccentric annuli. J. Pet. Sci. Eng. 2020, 193, 107368. [Google Scholar] [CrossRef]
- Luo, C.; Zhang, L.; Liu, Y.; Zhao, Y.; Xie, C.; Wang, L.; Wu, P. An improved model to predict liquid holdup in vertical gas wells. J. Pet. Sci. Eng. 2020, 184, 106491. [Google Scholar] [CrossRef]
- Eghorieta, R.A.; Pugliese, V.; Panacharoensawad, E. Experimental and Numerical Studies on the Drift Velocity of Two-Phase Gas and High-Viscosity-Liquid Slug Flow in Pipelines. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 6–9 May 2019. [Google Scholar]
- Livinus, A.; Verdin, P.; Lao, L.; Nossen, J.; Langsholt, M.; Sleipnæs, H.G. Simplified generalised drift velocity correlation for elon-gated bubbles in liquid in pipes. J. Pet. Sci. Eng. 2018, 160, 106–118. [Google Scholar] [CrossRef] [Green Version]
- Zuber, N.; Findlay, J.A. Average Volumetric Concentration in Two-Phase Flow Systems. J. Heat Transf. 1965, 87, 453–468. [Google Scholar] [CrossRef]






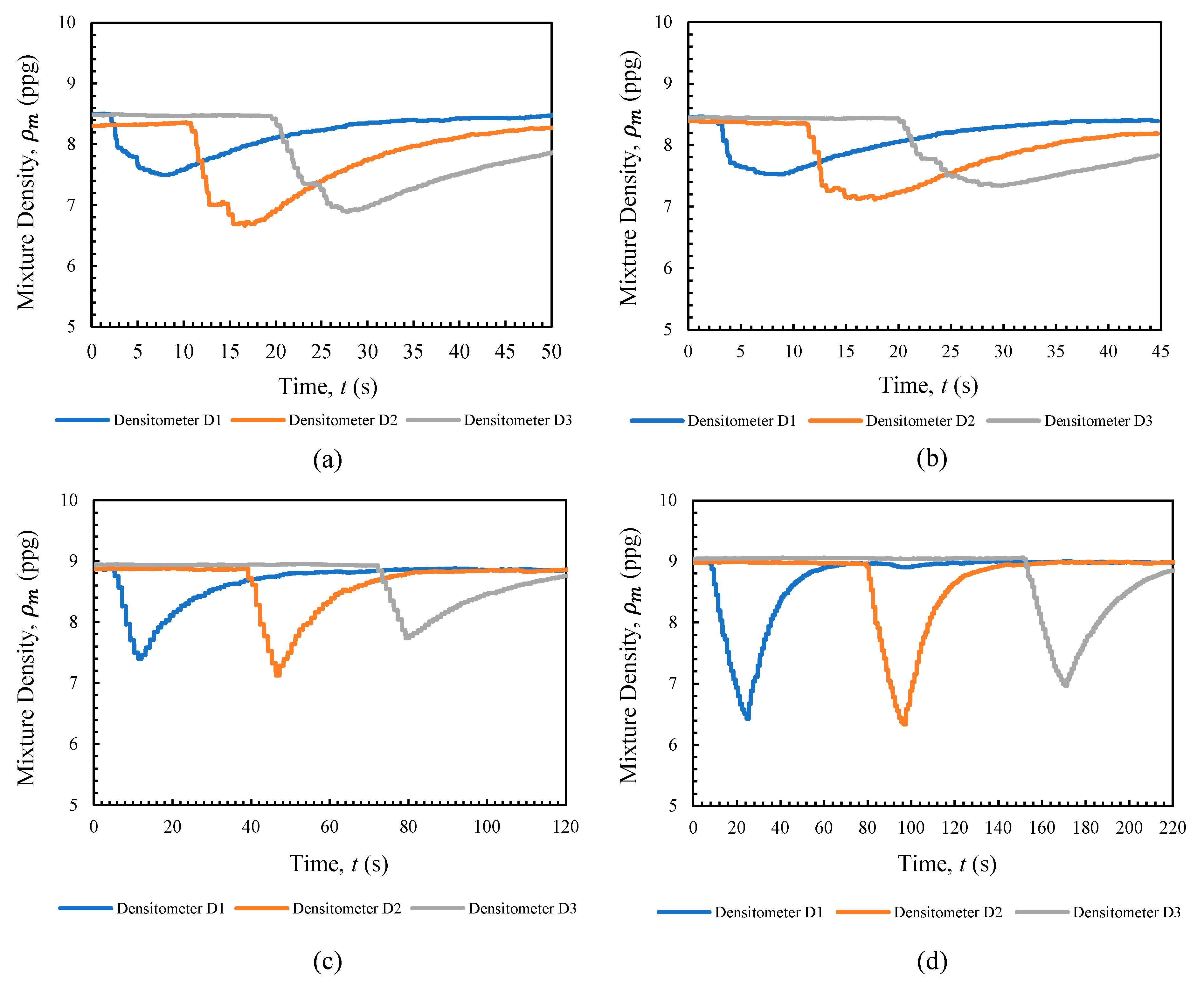


| Liquid | Condition | ID (m) | (°) | (kg/m3) | (cp) | (lb/100 ft2) | (m/s) | |
|---|---|---|---|---|---|---|---|---|
| Water | S/C | 0.1524 | 4, 15, 30, 45 | 998 | 1 | - | 0.173~0.7 | 26,306~105,227 |
| 10 lb/bbl BW | S | 0.1524 | 4, 15, 30, 45 | 1014 | 2.4 | 0.75 | 0 | - |
| 30 lb/bbl BW | S | 0.1524 | 4, 15, 30, 45 | 1047 | 27 | 41.6 | 0 | - |
| 45 lb/bbl BW | S/C | 0.1524 | 4, 15, 30, 45 | 1063 | 76 | 162 | 0.22~0.7 | 477~1113 |
| 48.5 lb/bbl BW | S/C | 0.1524 | 4, 15, 30, 45 | 1076 | 101 | 230 | 0.073~0.7 | 118~803 |
| Correlations | 15% | 10% | Statistical Parameter | ||
|---|---|---|---|---|---|
| Bendiksen | 42.10% | 21.05% | 0.06619 | 0.3391 | 0.1782 |
| Hasan and Kabir | 5.26% | 0.00% | −0.247 | 0.5575 | 0.2242 |
| Gokcal | 57.89% | 36.84% | 0.1079 | 0.35831 | 0.1988 |
| Jeyachandra | 47.37% | 42.10% | 0.1465 | 0.3903 | 0.2132 |
| Moreiras | 21% | 16% | 0.02351 | 0.3078 | 0.1622 |
| Lizarraga-Garcia | 31.58% | 10.53% | −0.03655 | 0.2907 | 0.1628 |
| Proposed correlation | 90% | 80% | 0.00201 | 0.0702 | 0.093 |
| Source | Fluids | Pipe Diameter (mm) | Inclination Angle (°) | n | k | |
|---|---|---|---|---|---|---|
| Carew et al. | Carbopol 981 | 25, 45, 70 | 0 | - | 0.386, 0.485 | 1.016, 2.927 |
| Sousa et al. | CMC | 32 | 0 | - | 0.437~0.772 | 0.079~4.189 |
| Eghorieta et al. | Water | 50.8 | 80, 83, 85, 87, 89, 90 | 0.001 | - | - |
| Livinus et al. | Oil | 57, 99 | 82.5, 85, 87.5, 89 | 0.16, 1.14 | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Upchurch, E.R.; Ozbayoglu, E.M. Experimental Study of Single Taylor Bubble Rising in Stagnant and Downward Flowing Non-Newtonian Fluids in Inclined Pipes. Energies 2021, 14, 578. https://doi.org/10.3390/en14030578
Liu Y, Upchurch ER, Ozbayoglu EM. Experimental Study of Single Taylor Bubble Rising in Stagnant and Downward Flowing Non-Newtonian Fluids in Inclined Pipes. Energies. 2021; 14(3):578. https://doi.org/10.3390/en14030578
Chicago/Turabian StyleLiu, Yaxin, Eric R. Upchurch, and Evren M. Ozbayoglu. 2021. "Experimental Study of Single Taylor Bubble Rising in Stagnant and Downward Flowing Non-Newtonian Fluids in Inclined Pipes" Energies 14, no. 3: 578. https://doi.org/10.3390/en14030578
APA StyleLiu, Y., Upchurch, E. R., & Ozbayoglu, E. M. (2021). Experimental Study of Single Taylor Bubble Rising in Stagnant and Downward Flowing Non-Newtonian Fluids in Inclined Pipes. Energies, 14(3), 578. https://doi.org/10.3390/en14030578





