Effect of Foaming Temperature on Microstructure, Mechanical Properties and Flame Spread Rate in PET–PEN Copolymer
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
2.2.1. Sample Preparation
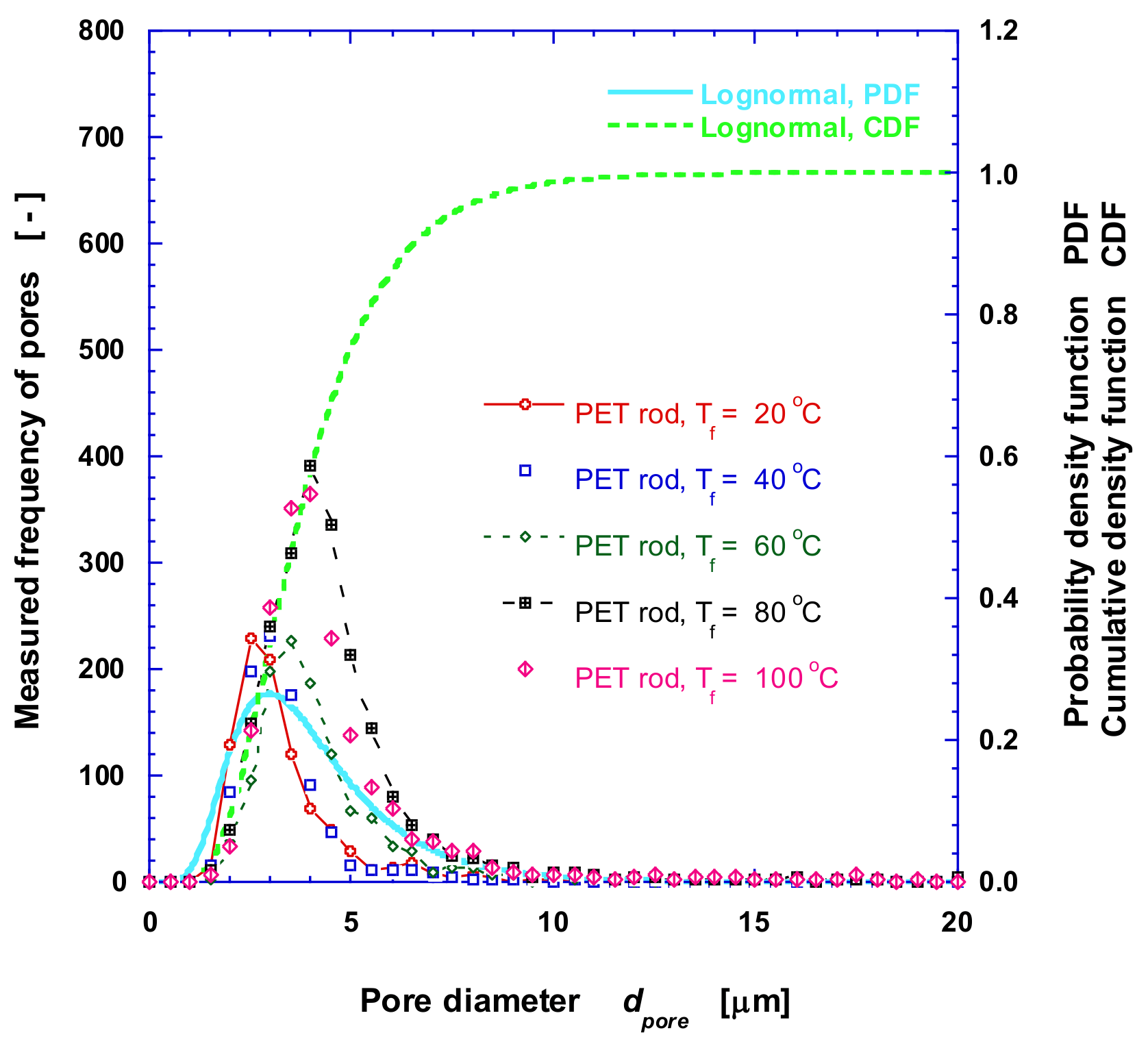
2.2.2. Measurement of Pore Diameter and Distribution
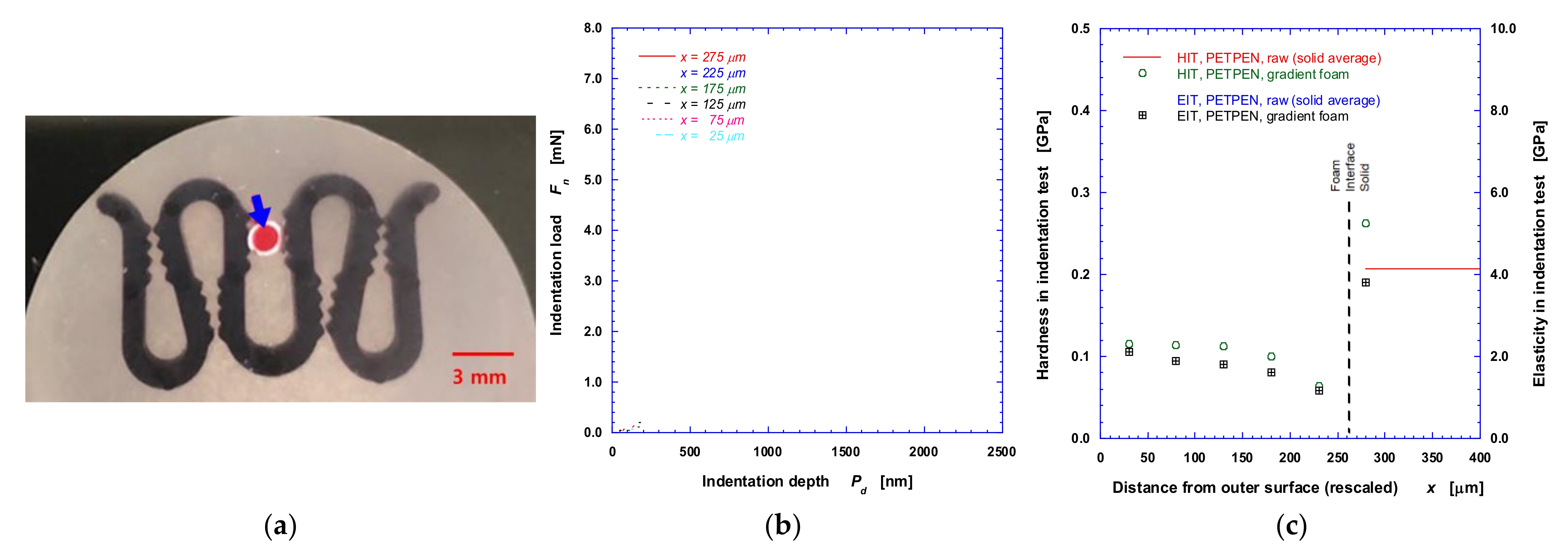
2.2.3. Micromechanical Property Measurement
2.2.4. Measurement of Simple Extension Behavior
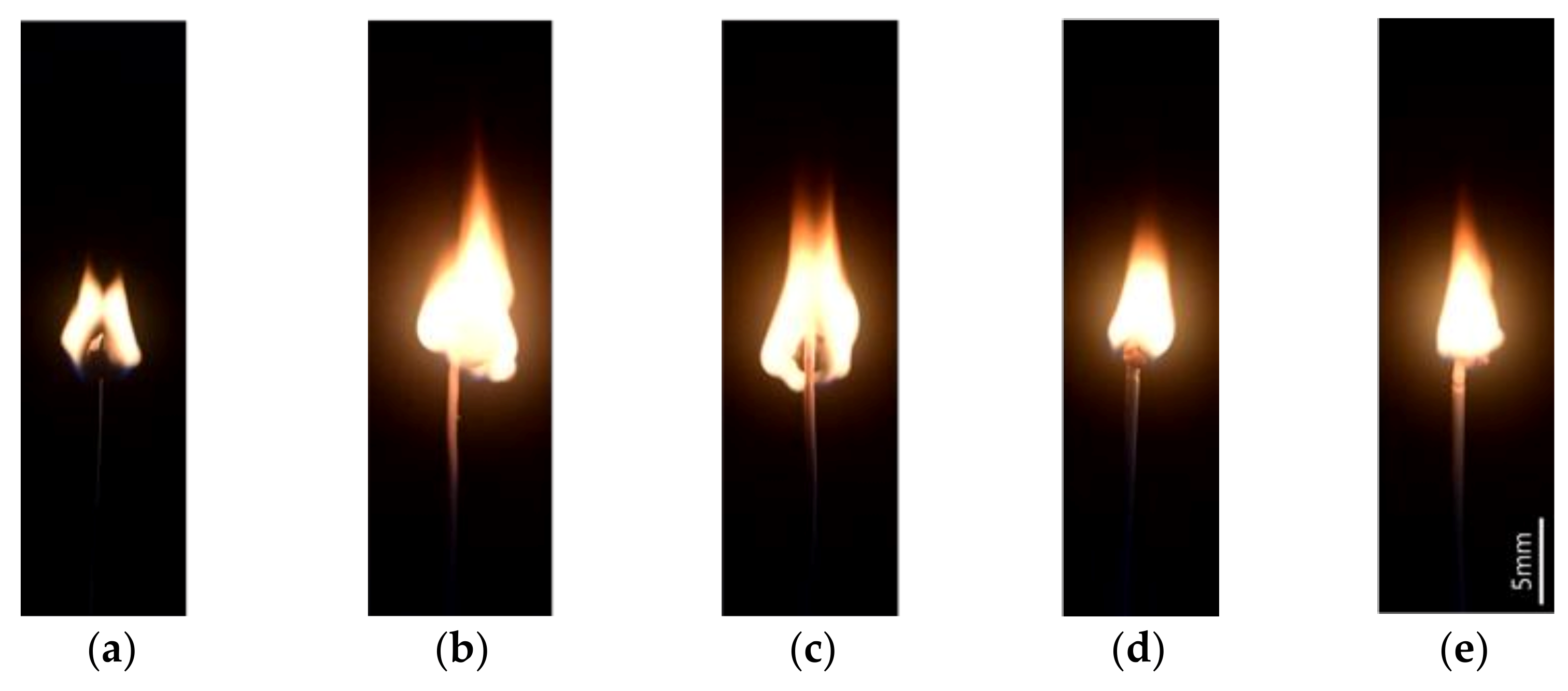
2.2.5. Measurement of Flame Spread Rate
3. Results and Discussion
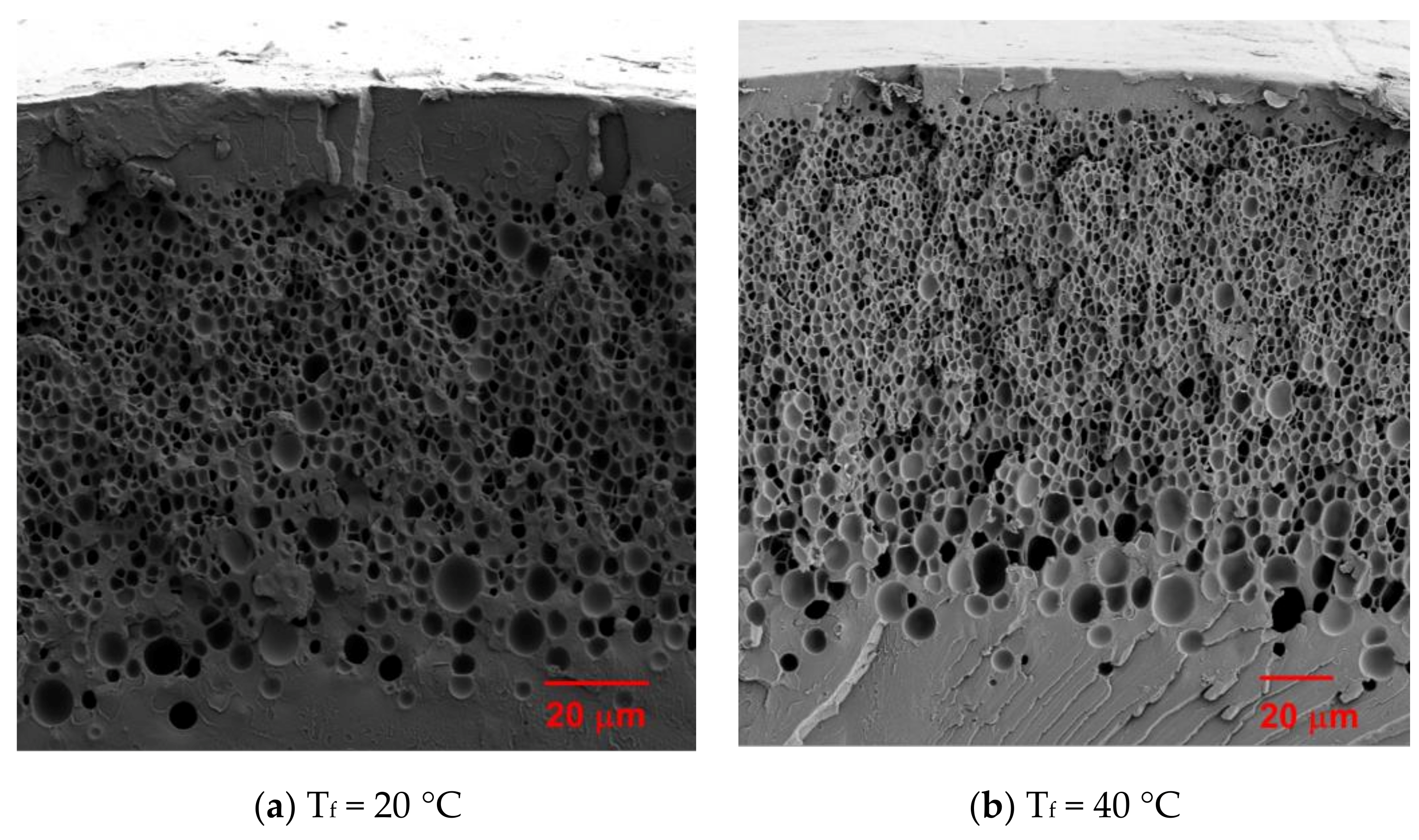
3.1. Microstructure
3.2. Mechanical Properties—Hardness and Elasticity
3.3. Simple Extension Behavior
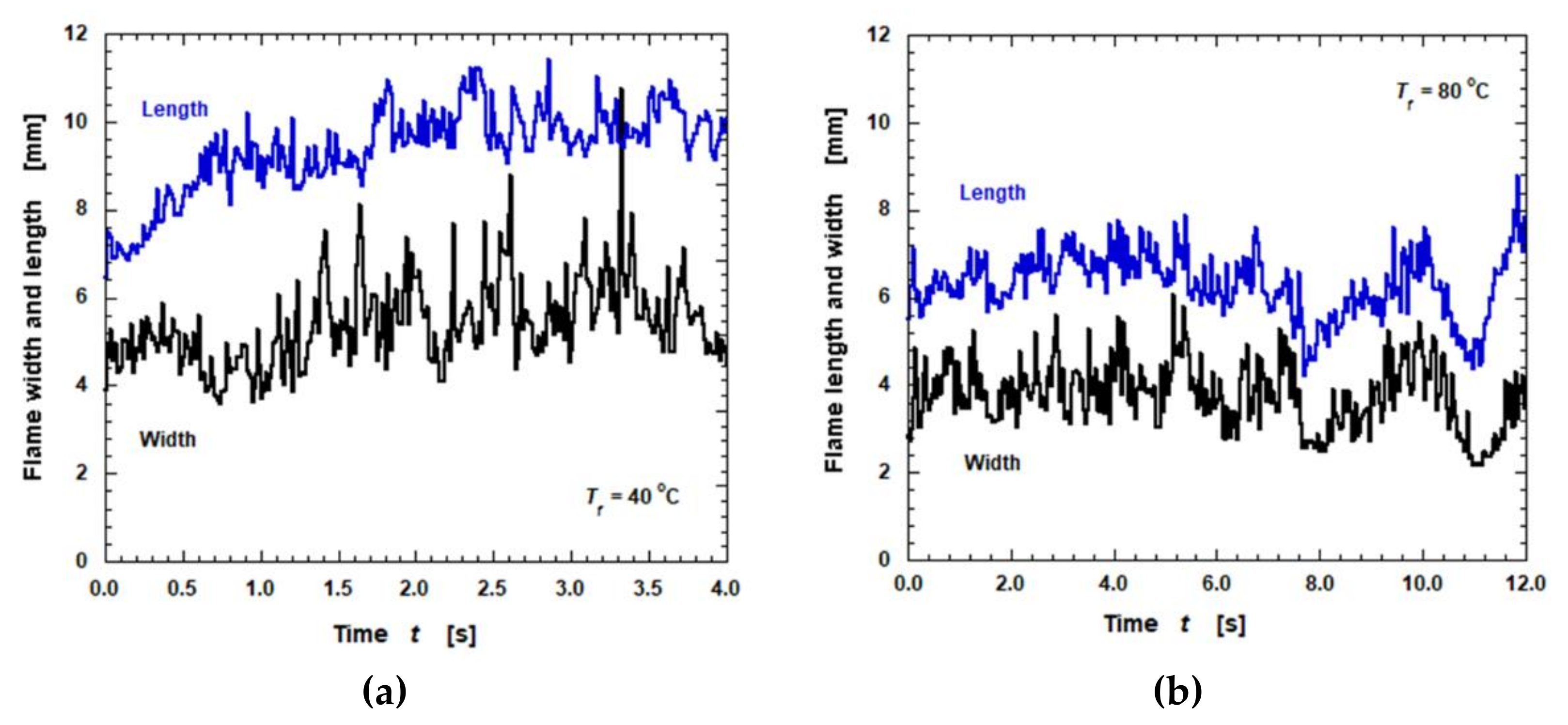
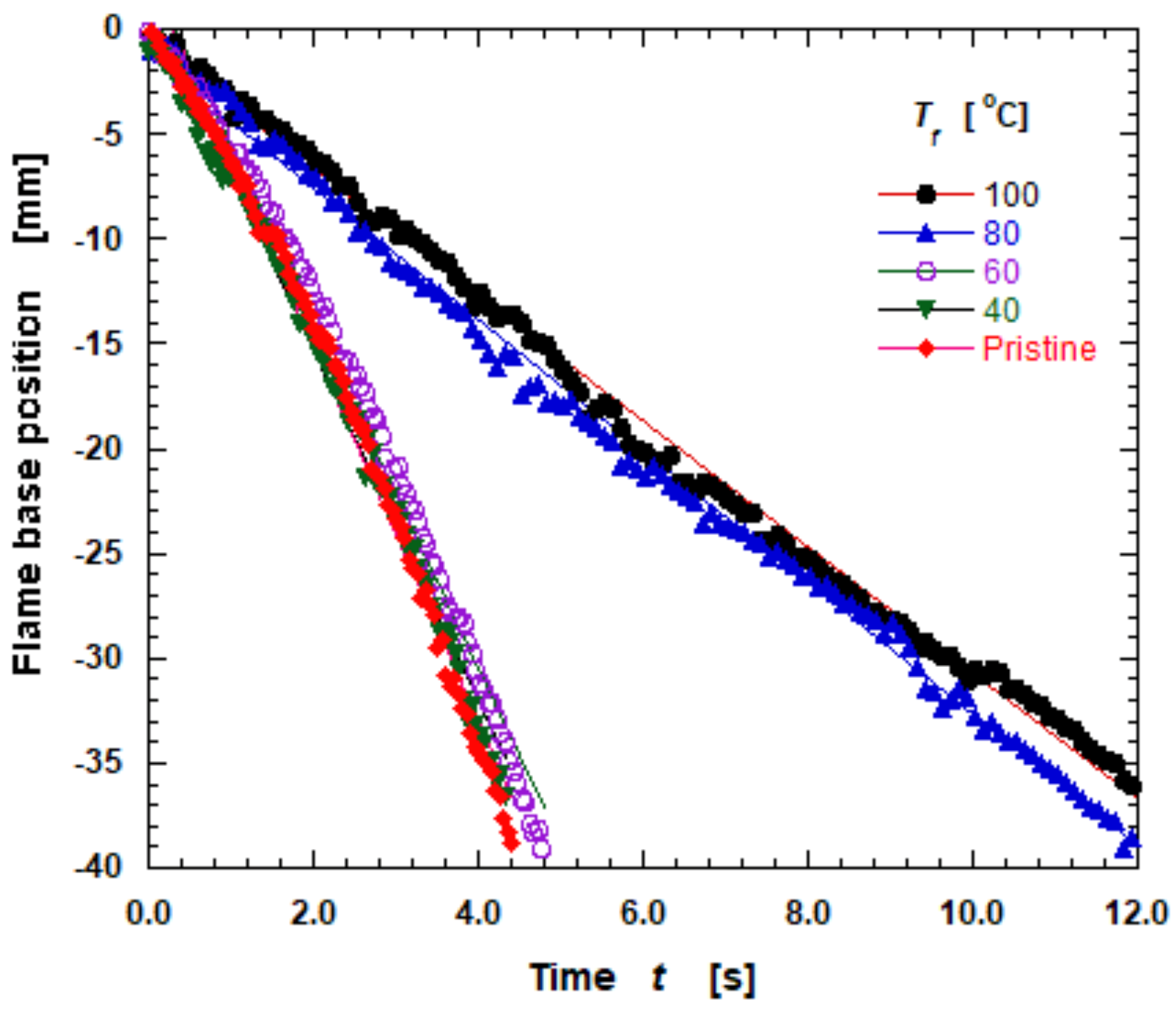
3.4. Flame Spread Rate
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gonçalvesa, M.; Simõesa, N.; Serraa, C.; Flores-Colen, I. A review of the challenges posed by the use of vacuum panels in external insulation finishing systems. Appl. Energy 2020, 257, 114028. [Google Scholar]
- Choi, B.; Yeo, I.; Lee, J.; Kang, W.K.; Song, T.H. Pillar-supported vacuum insulation panel with multi-layered filler material. Int. J. Heat Mass Transf. 2016, 102, 902–910. [Google Scholar] [CrossRef]
- Kwon, J.S.; Jang, C.H.; Jung, H.; Song, T.H. Vacuum maintenance in vacuum insulation panels exemplified with a staggered beam VIP. Energy Build. 2010, 42, 590–597. [Google Scholar] [CrossRef]
- Lee, S.T. Polymeric Foams: Innovations in Process, Technologies and Products; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Maio, E.D.; Kiran, E. Foaming of polymers with supercritical fluids and perspectives on the current knowledge gaps and challenges. J. Supercrit. Fluids 2018, 134, 157–166. [Google Scholar] [CrossRef]
- Martini, J.E.; Waldman, F.A.; Suh, N.P. The Production and analysis of microcellular thermoplastic foams. SPE Tech. Pap. 1982, 28, 674. [Google Scholar]
- Guo, H.; Kumar, V. Some thermodynamic and kinetic low-temperature properties of the PC-CO2 system and morphological characteristics of solid-state PC nanofoams produced with liquid CO2. Polymer 2015, 56, 46–56. [Google Scholar] [CrossRef]
- Xia, T.; Xi, Z.; Tao Liu, T.; Zhao, L. Solid state foaming of poly(ethylene terephthalate) based on periodical CO2-renewing sorption process. Chem. Eng. Sci. 2017, 168, 124–136. [Google Scholar] [CrossRef]
- Yu, J.; Song, L.; Chen, F.; Fan, P.; Sun, L.; Zhong, M.; Yang, J. Preparation of polymer foams with a gradient of cell size: Further exploring the nucleation effect of porous inorganic materials in polymer foaming. Mater. Today Commun. 2016, 9, 1–6. [Google Scholar] [CrossRef]
- Yoon, T.J.; Kong, W.; Kwon, D.E.; Park, B.K.; Lee, Y.-W. Preparation of solid-state micro- and nanocellular acrylonitrile-butadiene-styrene (ABS) foams using sub- and supercritical CO2 as blowing agents. J. Supercrit. Fluids 2017, 124, 30–37. [Google Scholar] [CrossRef]
- Ono, T.; Wu, X.; Horiuchi, S.; Furuya, T.; Yoda, T. Two-step foaming process for production of PMMA nanocellular polymer foams via ultra-high pressure and rapid depressurization. J. Supercrit. Fluids 2020, 165, 104963. [Google Scholar] [CrossRef]
- Martin-de Leon, J.; Bernardo, V.; Rodriguez-Perez, M.A. Key production parameters to obtain transparent nanocellular PMMA. Macromol. Mater. Eng. 2017, 302, 1700343. [Google Scholar] [CrossRef]
- Sałasińska, K.; Borucka, M.; Leszczyńska, M.; Zatorski, W.; Celiński, M.; Gajek, A.; Ryszkowska, J. Analysis of flammability and smoke emission of rigid polyurethane foams modified with nanoparticles and halogen-free fire retardants. J. Therm. Anal. Calorim. 2017, 130, 131–141. [Google Scholar] [CrossRef] [Green Version]
- Szabó, V.A.; Dogossy, G. Flame retardancy of recycled PET foam. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2020; Volume 903. [Google Scholar] [CrossRef]
- Yuan, Q.; Huang, D.; Hu, Y.; Shen, L.; Shi, L.; Zhang, M. Comparison of Fire Behavior of Thermally Thin and Thick Latex Foam Under Bottom Ventilation. Polymers 2019, 11, 88. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Babu, K.; Rend, G.; Mensah, R.A.; Kim, N.K.; Jiang, L.; Xu, Q.; Restas, A.; Neisiany, R.E.; Hedenqvist, M.S.; Försth, M.; et al. A Review on the Flammability Properties of Carbon-Based Polymeric Composites: State-of-the-Art and Future Trends. Polymers 2020, 12, 1518–1536. [Google Scholar] [CrossRef]
- Jasinski, E.; Bounor-Legaré, V.; Taguet, A.; Beyou, E. Influence of halloysite nanotubes onto the fire properties of polymer based composites: A review. Polymer Degrad. Stab. 2021, 183. [Google Scholar] [CrossRef]
- Delichatsios, M. Effects of material thickness on ignition times and creeping flame spread in the thermal regime: Theory, analytical solution and experimental justification. Fire Saf. J. 2020, 116, 103204. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Huang, X.; Nakaya, S.; Tsue, M.; Fernandez-Pello, C. Flame Spread over Horizontal and Vertical Wires: The Role of Dripping and Core. Fire Saf. J. 2017, 91, 112–122. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Konno, Y.; Huang, X.; Nakaya, S.; Tsue, M.; Hashimoto, N.; Fujita, O.; Fernandez-Pello, C. Effect of insulation melting and dripping on opposed flame spread over laboratory simulated electrical wires. Fire Saf. J. 2018, 95, 1–10. [Google Scholar] [CrossRef]
- Park, S.H.; Lim, S.J.; Cha, M.S.; Park, J.; Chung, S.H. Effect of AC electric field on flame spread in electrical wire: Variation in polyethylene insulation thickness and di-electrophoresis phenomenon. Combust. Flame 2019, 202, 107–118. [Google Scholar] [CrossRef]
- Park, S.H.; Kang, M.S.; Cha, M.S.; Park, J.; Chung, S.H. Flame spread over twin electrical wires with applied DC electric fields. Combust. Flame 2019, 210, 350–359. [Google Scholar] [CrossRef] [Green Version]
- Rybińskia, P.; Bradłob, D.; Żukowskib, W.; Syreka, B. Determination of toxic products emissions of polymers thermal decomposition using fluidised bed reactor and FTIR analysis. Polym. Test. 2019, 79, 106040. [Google Scholar]
- Godey, F.; Fleury, A.; Soldera, A. Local dynamics within the glass transition domain. Sci. Rep. 2019, 9, 9638. [Google Scholar] [CrossRef] [Green Version]
- Tetlow, D. Cellulosic-crystals as a fumed-silica substitute in vacuum insulated panel technology used in building construction and retrofit applications. Energy Build. 2017, 156, 187–196. [Google Scholar] [CrossRef]
- Park, B.K.; Kim, C.-J.; Kwon, D.E.; Lee, Y.-W. Design and Fabrication of of Partially Foamed Grid Structure Using Additive Manufacturing and Solid State Foaming. Processes 2020, 8, 1594. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. An improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 1992, 7, 1564–1583. [Google Scholar] [CrossRef]
- Baylakoglu, I.; Hillman, C.; Pecht, M. Characterization of Some Commercial Thermally-Cured Potting Materials. In Proceedings of the International IEEE Conference on the Business of Electronic Product Reliability and Liability, Hong Kong & Shenzhen, China, 13–14 January 2003; Available online: https://www.researchgate.net/publication/265869710 (accessed on 20 December 2020).
- Kiran, E. Morphological modifications and formation of morphologically-gradient polymers in dense fluids. In Proceedings of the 5th International Symposium on High Pressure Process Technology and Chemical Engineering, Segovia, Spain, 24–27 June 2007; pp. 1–10. [Google Scholar]
- Park, B.K.; Kim, C.-J.; Lee, Y.W. Characterization of Gradient Foam Using Additive Manufacturing and Gas Foaming Technologies. In Proceedings of the 4th International Conference on Manufacturing Technologies (ICMT 2020), Seattle, DC, USA, 17–20 January 2020; Agarwal, R.K., Ed.; Abstract MC20-321-A. Available online: http://icmt.org/icmt2020.html (accessed on 16 October 2020).
- Jin, T.; Niu, X.; Xiao, G.; Wang, Z.; Zhou, Z. Effects of experimental variables on PMMA nano-indentation measurements. Polym. Test. 2015, 41, 1–6. [Google Scholar]





| Standards and Options | Acquisition Rate: 10.0 [Hz], Linear Loading, Max load: 5.00 mN, Loading rate: 10.00 mN/min, Unloading Rate: 10.00 mN/min, Pause: 1.0 s |
|---|---|
| Fn contact: 0.3 mN, Approach distance: 2000 nm, Approach speed: 2000 nm/min, Retract speed: 2000 nm/min, Stiffness Threshold: 150 μN/μm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, B.K.; Kim, C.-J.; Lee, B.J. Effect of Foaming Temperature on Microstructure, Mechanical Properties and Flame Spread Rate in PET–PEN Copolymer. Energies 2021, 14, 957. https://doi.org/10.3390/en14040957
Park BK, Kim C-J, Lee BJ. Effect of Foaming Temperature on Microstructure, Mechanical Properties and Flame Spread Rate in PET–PEN Copolymer. Energies. 2021; 14(4):957. https://doi.org/10.3390/en14040957
Chicago/Turabian StylePark, Byung Kyu, Charn-Jung Kim, and Byeong Jun Lee. 2021. "Effect of Foaming Temperature on Microstructure, Mechanical Properties and Flame Spread Rate in PET–PEN Copolymer" Energies 14, no. 4: 957. https://doi.org/10.3390/en14040957
APA StylePark, B. K., Kim, C.-J., & Lee, B. J. (2021). Effect of Foaming Temperature on Microstructure, Mechanical Properties and Flame Spread Rate in PET–PEN Copolymer. Energies, 14(4), 957. https://doi.org/10.3390/en14040957







