Analysis of the Dynamic Characteristics of the Pump Valve System of an Ultra-High Pressure Liquid Hydrogen Reciprocating Pump
Abstract
:1. Introduction
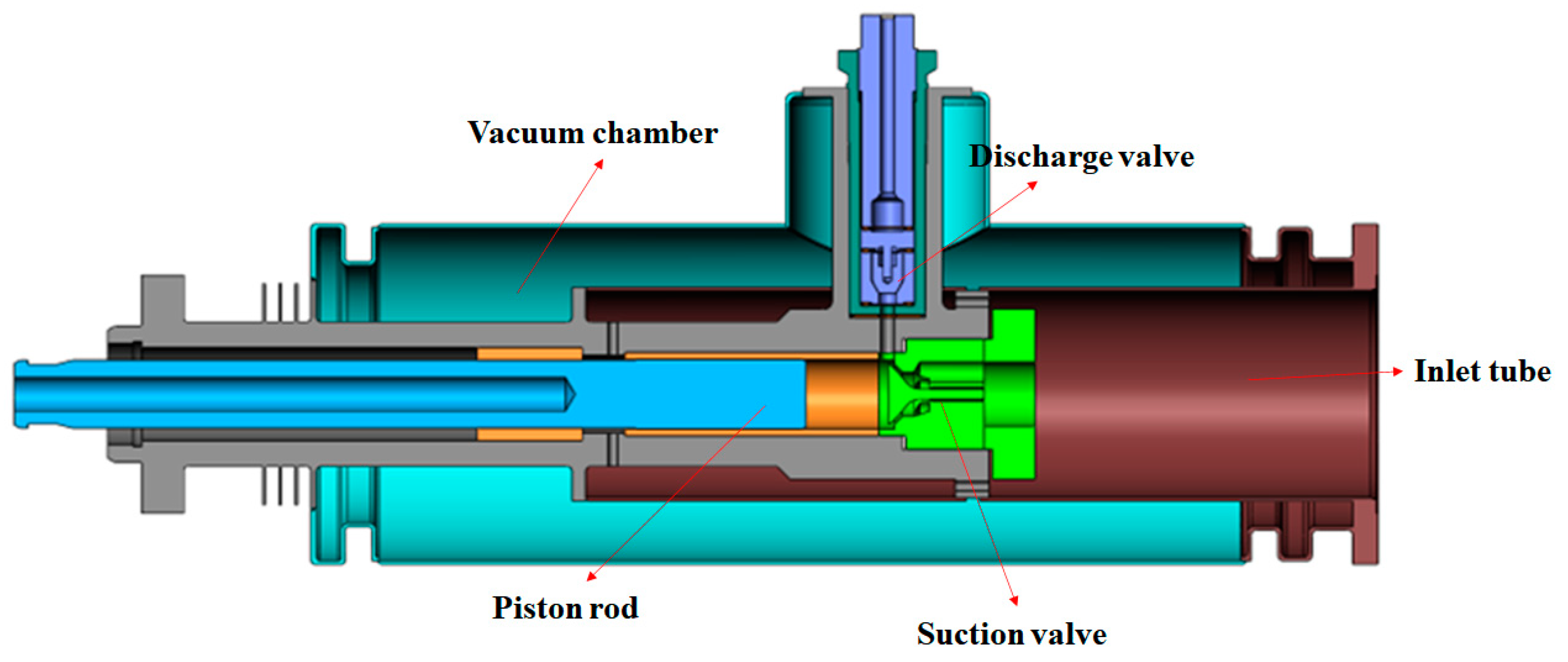
2. Coupling Model of Valve Spool Motion
2.1. Mathematical Model of Piston and Valve Motion
2.1.1. Motion of the Piston
2.1.2. Movement of the Valve
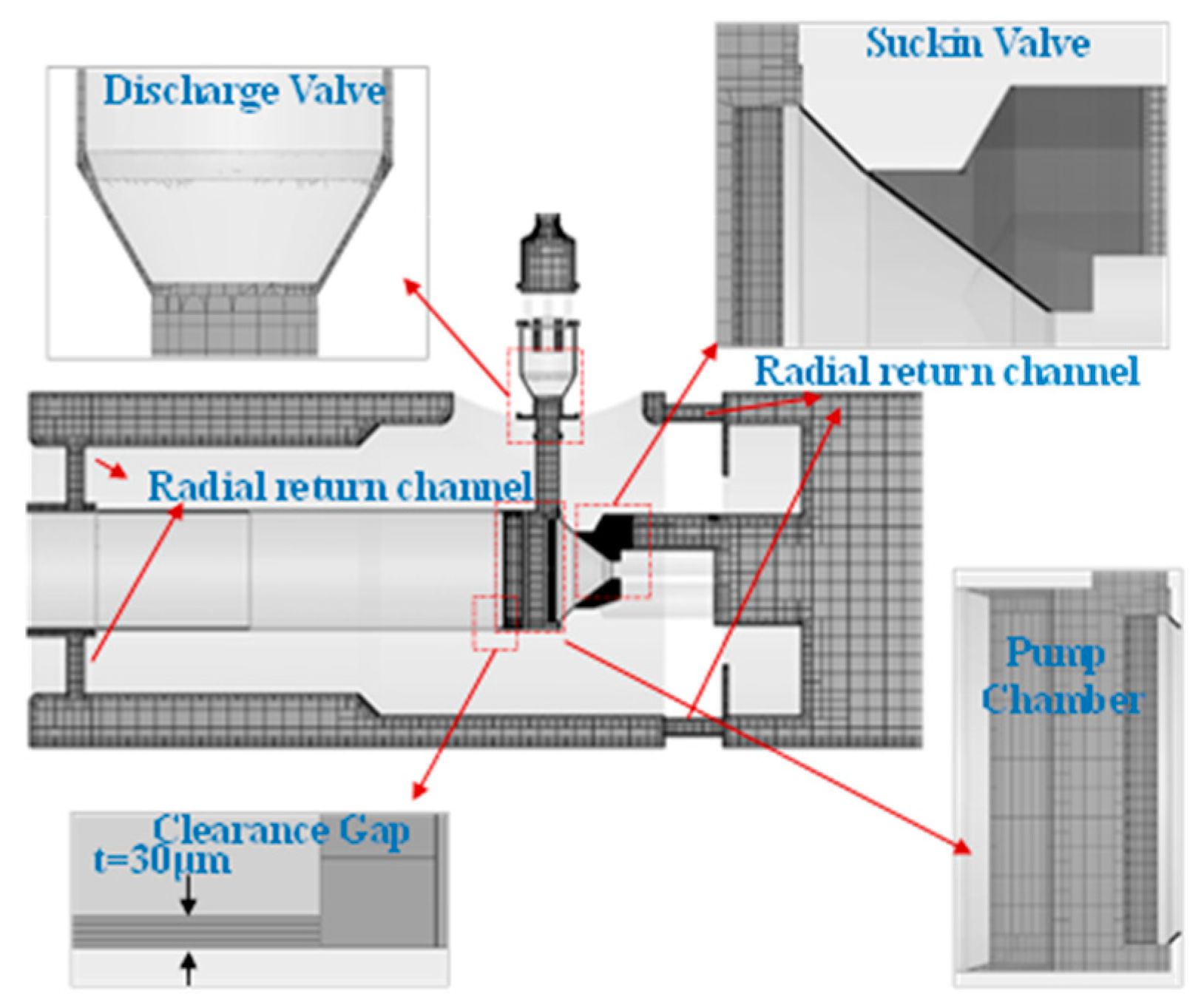
2.2. Calculation of Domain and Grid
2.3. Numerical Methods and Boundary Conditions
2.4. Simulation Study Content and Valve Parameter Setting
2.4.1. Simulation Research Content
2.4.2. Valve Parameter Setting
- (1)
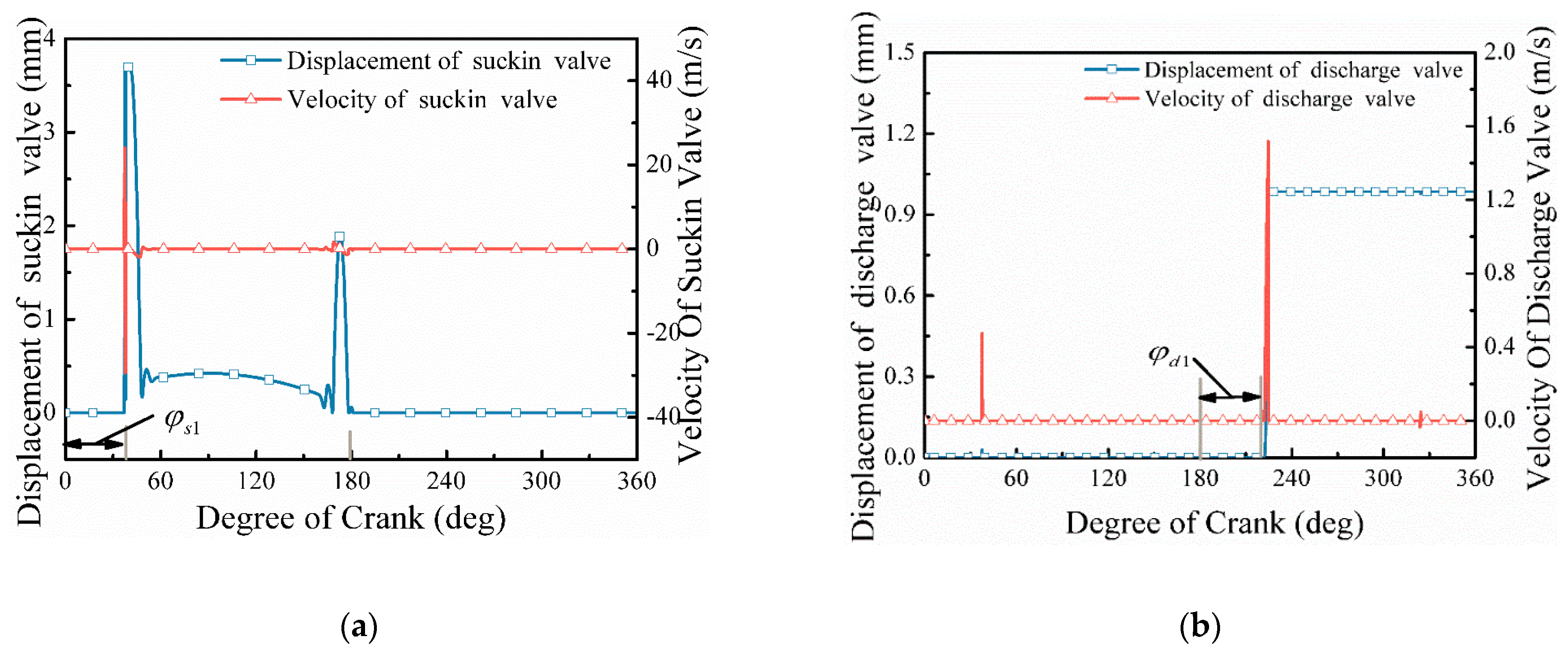
- In the expansion stroke, both the suction valve and the discharge valve are closed, and the pressure of liquid hydrogen in the compression chamber needs to be lowered to below the inlet pressure. When the sum of the pressure in the compression chamber and the spring preload is balanced with the inlet pressure, the suction valve will be opened; when the pressure in the compression chamber is too large, it will increase the opening hysteresis angle of the suction valve and affect the normal inlet; when the pressure in the compression chamber is too small, it will easily lead to the vaporization of liquid hydrogen.
- (2)
- In the discharge stroke, the suction valve is closed, the discharge valve is opened, and the discharge valve is balanced by the spring force and the hydraulic force. At the end of the discharge stroke, if the spring force of the discharge valve is too small, it will reduce the closing speed of the discharge valve and lead to an increase in the closing hysteresis angle, which will increase the amount of liquid hydrogen in the compression chamber when the discharge valve is completely closed and then reduce the amount of liquid hydrogen feeding during the weekly period.
3. Discussion of Results
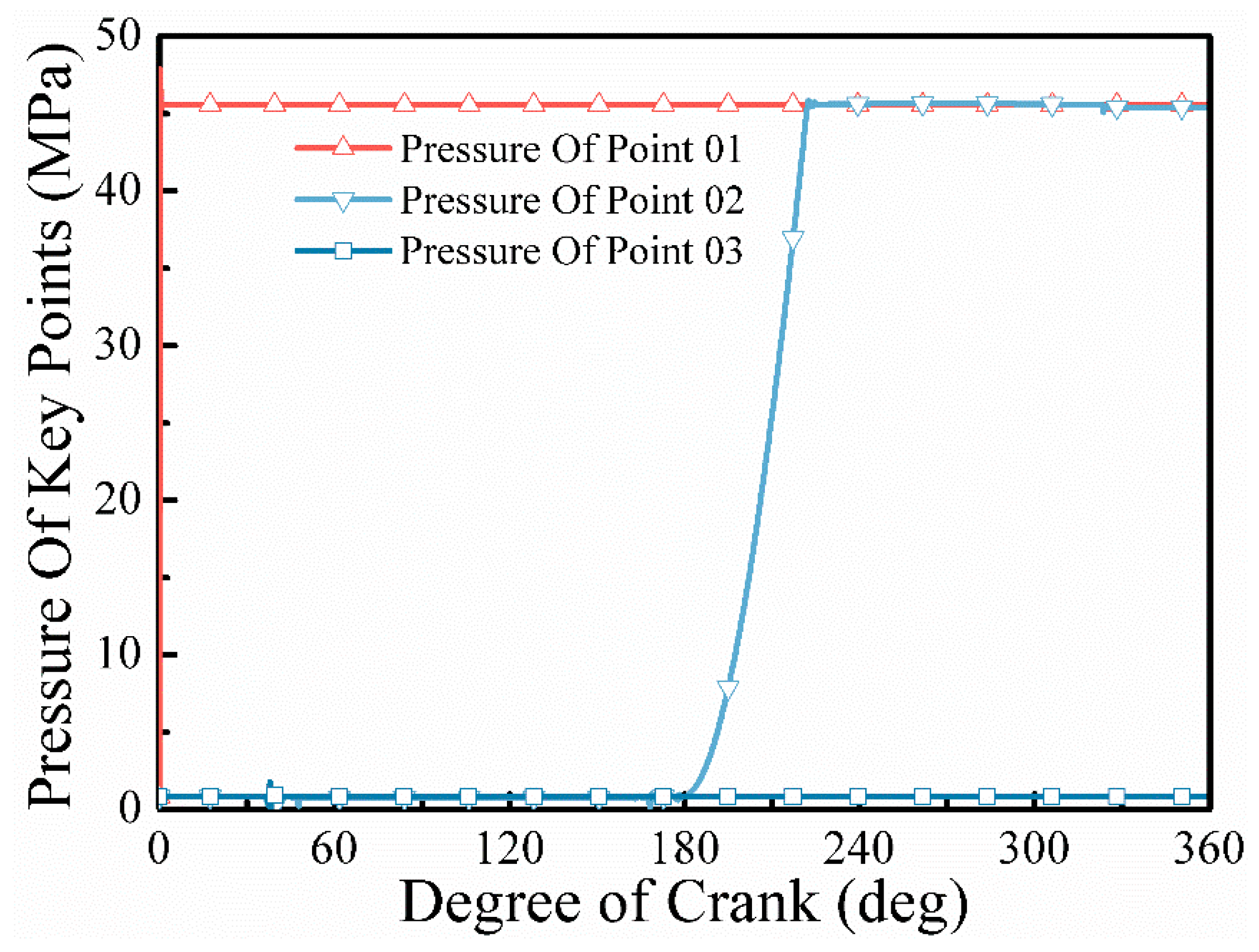
3.1. Coupling Analysis of Pump and Valve Dynamic Characteristics
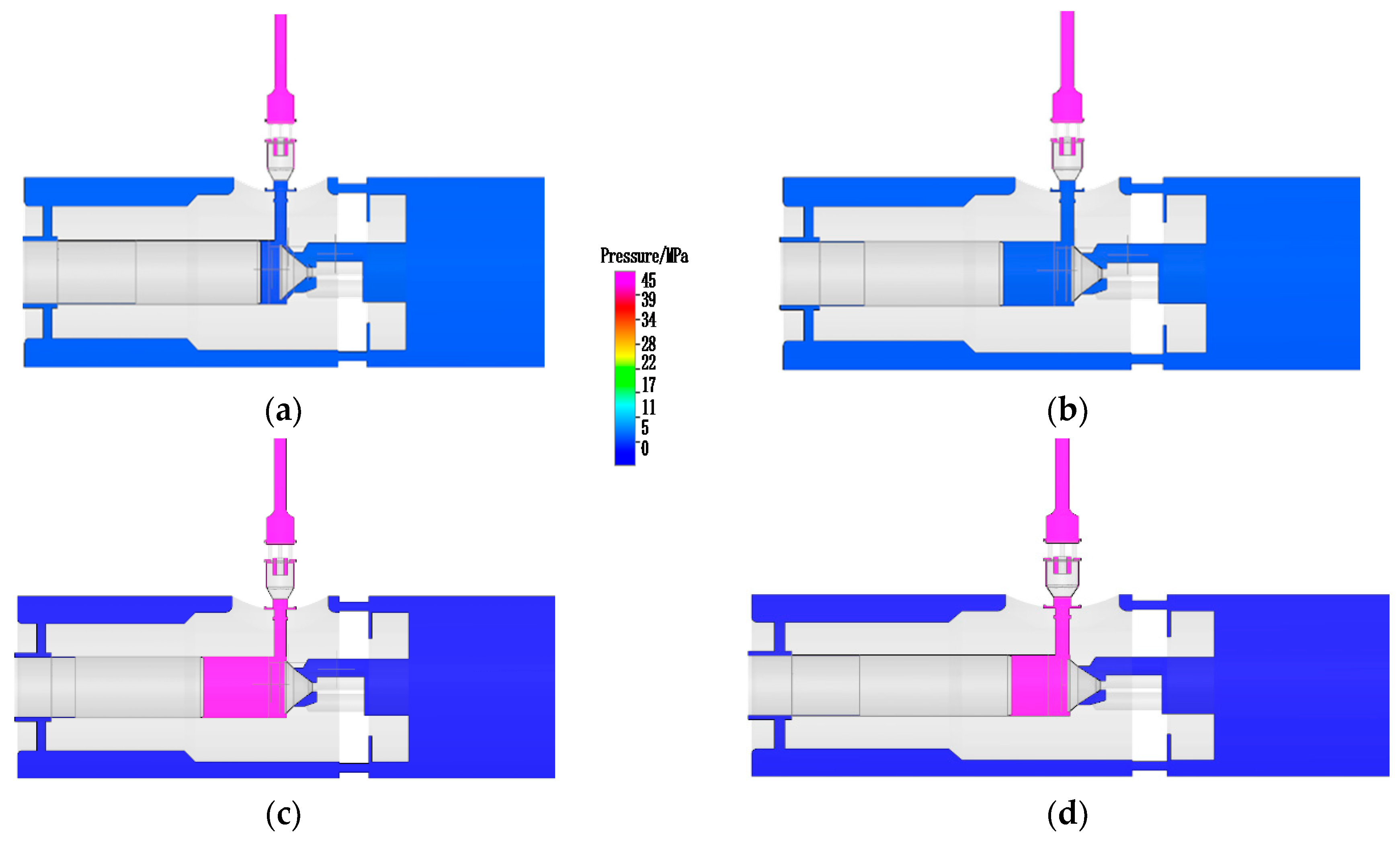
3.1.1. Flowfield Analysis of the Pressurization Process
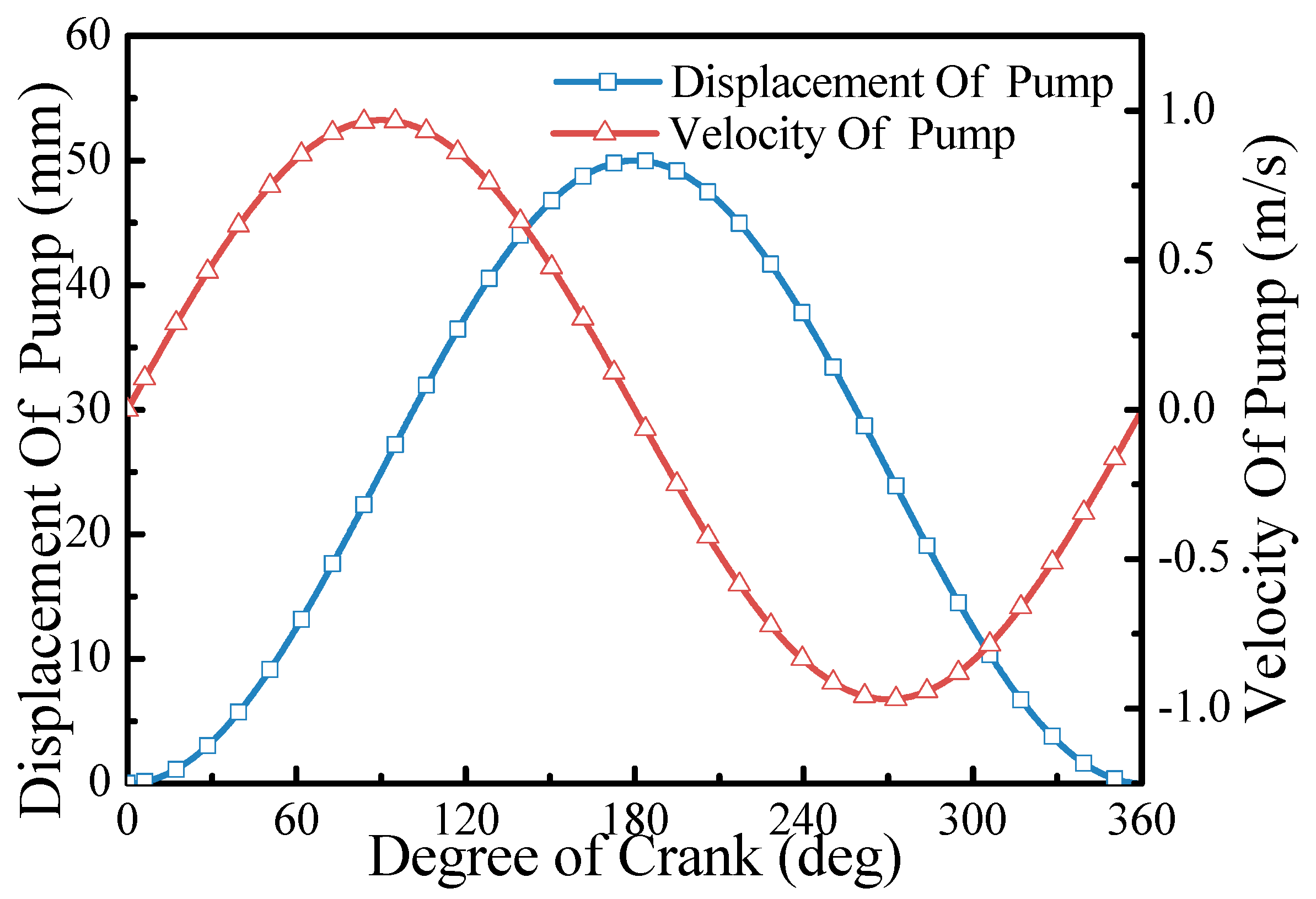
3.1.2. Coupling Analysis of Pump Valve Displacement and Piston Displacement
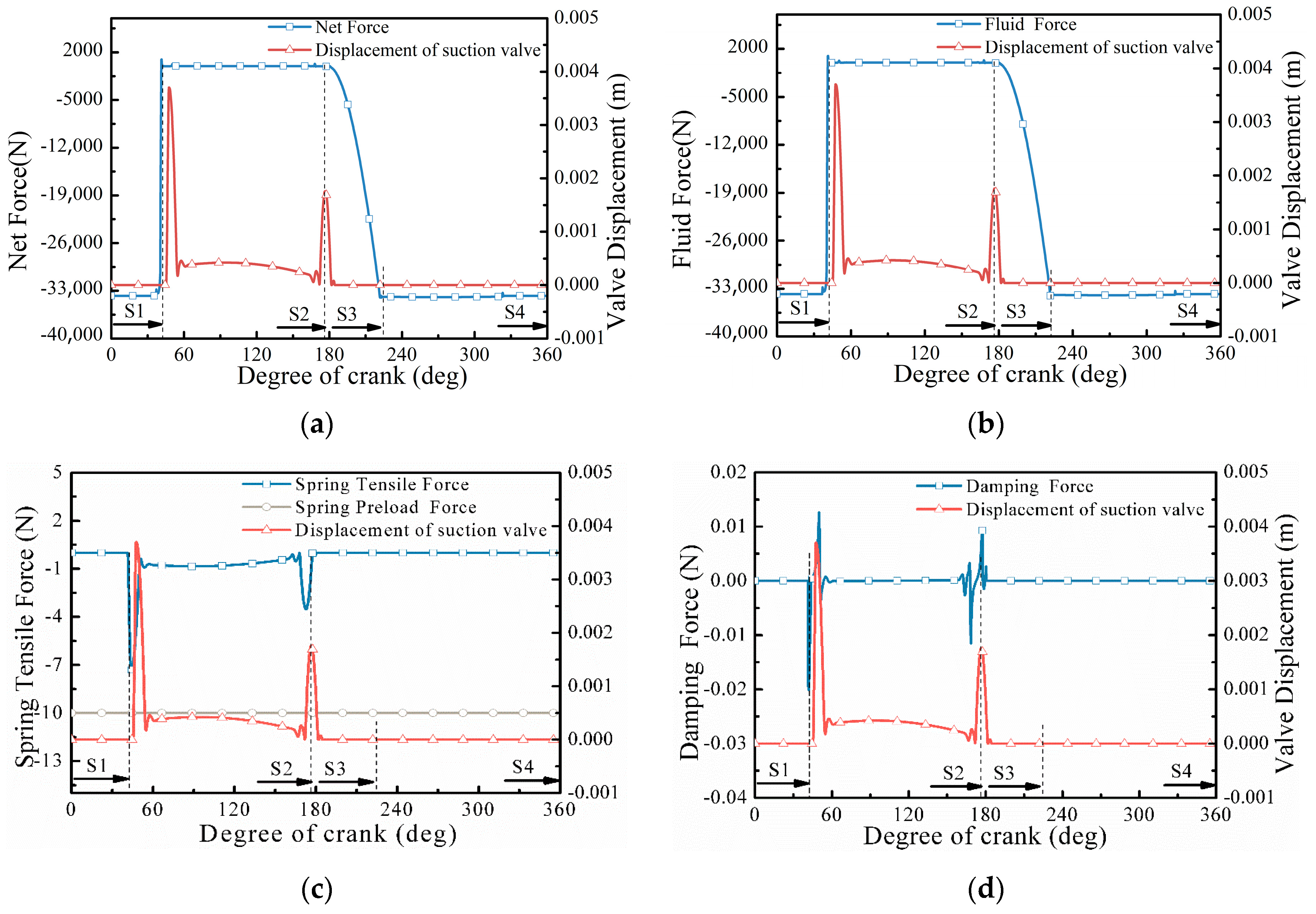
3.1.3. Coupling Analysis of Pump Valve Dynamic Characteristics and Forces
3.2. Effect of Pump Valve Spring Stiffness and Preload Force
3.2.1. Effect of Suction Valve Spring
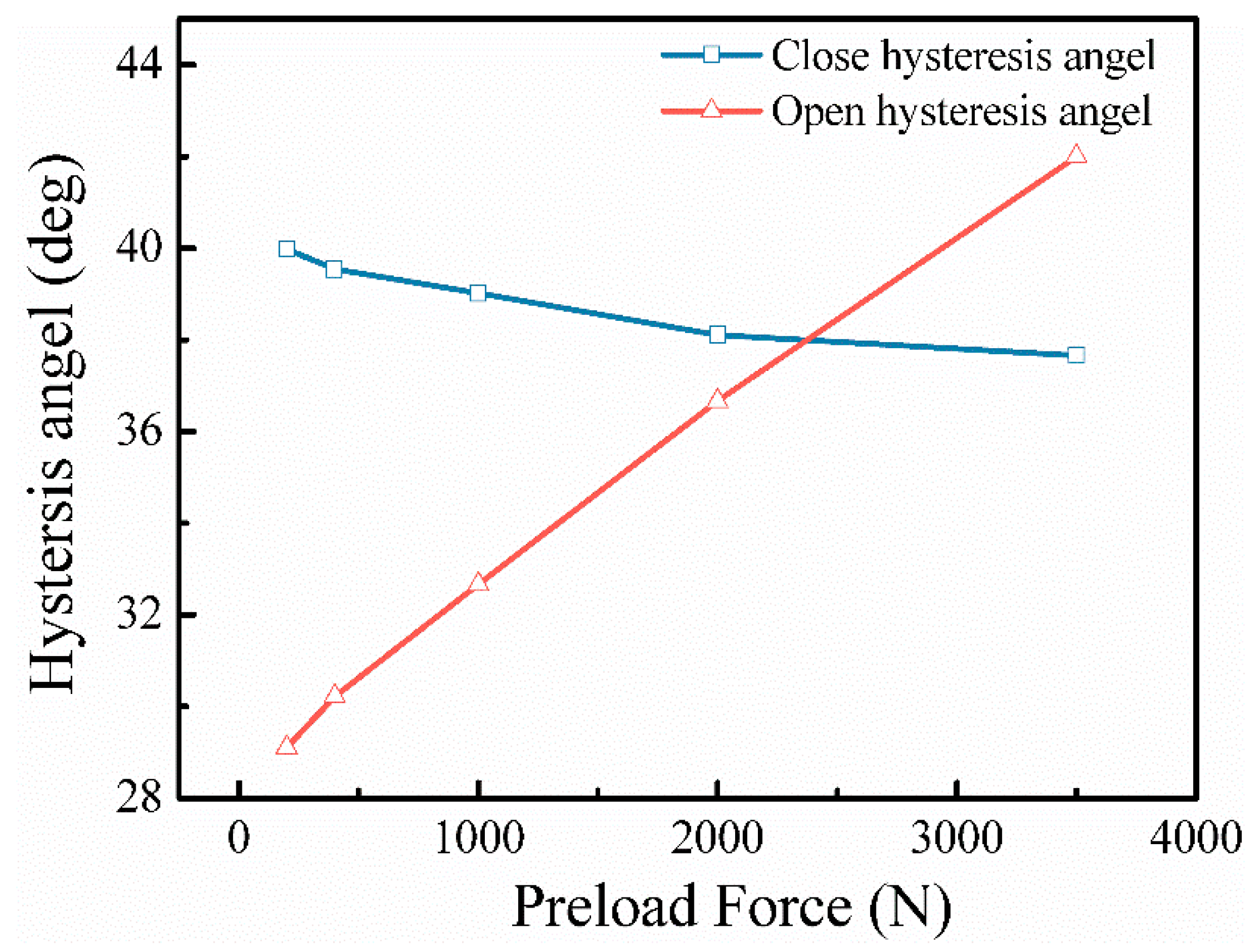
3.2.2. Effect of Discharge Valve Preload Force
4. Experimental Design and Results Validation
4.1. Test System and Results
4.2. Simulation and Results of Pressurization Process
4.2.1. Analysis of Experimental Pressure Increase Results
4.2.2. Calculation of Pressure Rise Due to Heat Absorption
5. Conclusions
- (1)
- By considering the leakage between the piston and the cylinder wall and the valve closure model, an unsteady simulation model of the reciprocating pump valve fluid end system was established, which can simulate the motion law of the suction and discharge valves more accurately.
- (2)
- The opening hysteresis angle is about 40° for both the suction and discharge valves, and the opening hysteresis effect is obvious. In the four strokes of reciprocating pump operation, the hydrodynamic force on the surface of the suction spool gradually decreases to zero in the expansion stroke, after which the suction valve opens, and the spring force gradually increases. In the suction stroke, the suction spool hydraulic force and spring force remain unchanged, and the valve opening degree remains unchanged. In the compression stroke, the suction valve closes first, and its surface hydrodynamic force gradually increases; meanwhile, the surface hydrodynamic force of the discharge valve gradually increases. In the discharge stroke, the spring force and hydrodynamic force on the valve spool remain unchanged.
- (3)
- For the suction valve stiffness in the range of 2000–20,000 N/m, as the stiffness increases, the maximum lift of the stable opening stage of the suction valve is smaller. The preload force of the suction valve mainly affects the degree of oscillation in the opening and closing stages of the suction valve. In the range of 10–60 N for the preload force of the suction valve, the larger the preload force is, the more obvious the effect of oscillation of the suction valve is. As the preload force of the discharge valve increases, the opening hysteresis angle of the discharge valve increases significantly, and the closing hysteresis angle decreases.
- (4)
- By comparing the simulation model with the test, the error between the boosting speed of the simulation model and the test boosting speed is 7.4%, which verifies the effectiveness of the method in this paper and can meet the accuracy of engineering applications.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, H. Reflections on Hydrogen Energy Development and Hydrogen Refueling Station Construction. Oil Depots Gas Stn. 2019, 28, 21–24. [Google Scholar]
- Brunner, T.; Kampitsch, M.; Kircher, O. Cryo-compressed hydrogen storage. Fuel Cells Data Facts Fig. 2016, 17, 162–172. [Google Scholar]
- Zhu, Q.; Zhu, J. The development and prospect of domestic liquid hydrogen hydrogen refueling station. Gas Heat 2020, 40, 15–19. [Google Scholar]
- Aceves, S.M.; Espinosa-Loza, F.; Ledesma-Orozco, E.; Ross, T.O.; Weisberg, A.H.; Brunner, T.C.; Kircher, O. High-density automotive hydrogen storage with cryogenic capable pressure vessels. Int. J. Hydrog. Energy 2010, 35, 1219–1226. [Google Scholar] [CrossRef]
- Petitpas, G. Simulation of boil-off losses during transfer at a LH2 based hydrogen refueling station. Int. J. Hydrog. Energy 2018, 43, 21451–21463. [Google Scholar] [CrossRef]
- Petitpas, G.; Simon, A.; Moreno-Blanco, J.; Aceves, S.M. Liquid Hydrogen Infrastructure Analysis; Lawrence Livermore National Laboratory: Livermore, CA, USA, 2017. [Google Scholar]
- Qin, N.; Brooker, P.; Srinivasan, S. Hydrogen Fueling Stations Infrastructure; Research and Innovative Technology Administration: Orlando, FL, USA, 2014. [Google Scholar]
- Xian, J.; Lin, Z.; Lai, Y. Hydrogen refueling station process and operational safety. Gas Heat 2017, 37, B01–B06. [Google Scholar]
- Furuhama, S.; Kobayashi, Y. A liquid hydrogen car with a two-stroke direct injection engine and LH2-pump. Int. J. Hydrog. Energy 1982, 7, 809–820. [Google Scholar] [CrossRef]
- Petitpas, G.; Moreno-Blanco, J.; Espinosa-Loza, F.; Aceves, S.M. Rapid high density cryogenic pressure vessel filling to 345 bar with a liquid hydrogen pump. Int. J. Hydrog. Energy 2018, 43, 19547–19558. [Google Scholar] [CrossRef]
- Gao, J.; Li, Z.; Luo, X.; Zhang, H. Numerical simulation study of the operating characteristics of axial flow control valve. Fluid Mach. 2020, 48, 12–16. [Google Scholar]
- Yang, Z.; Zhang, S.; Wang, M.; Zhang, D. Numerical simulation of steady-state flow field of shuttle valve based on FLUENT. Hydraul. Pneum. Seals 2017, 09, 29–32. [Google Scholar]
- Wu, D.; Li, S.; Wu, P. CFD simulation of flow-pressure characteristics of a pressure control valve for automotive fuel supply system. Energy Convers. Manag. 2015, 101, 658–665. [Google Scholar] [CrossRef]
- Zhang, M.; Feng, J.; Zhou, Z. Dynamic numerical simulation study of reciprocating pump drawing process. China Pet. Mach. 2009, 37, 42–45. [Google Scholar]
- Zhang, R.; Sun, P.; Fu, Y. Dynamic coupling characteristics of the flow field and spool motion of reciprocating pump valves. J. Irrig. Drain. Mach. Eng. 2021, 39, 217–223. [Google Scholar]
- Zhu, G.; Dong, S.; Zhang, W.; Zhang, J.; Zhang, C. Simulation of variable stiffness spring reciprocating pump cone valve and its dynamic characteristics. China Mech. Eng. 2018, 29, 2912–2916. [Google Scholar]
- Iannetti, A.; Stickland, M.T.; Dempster, W.M. A computational fluid dynamics model to evaluate the inlet stroke performance of a positive displacement reciprocating plunger pump. Proc. Inst. Mech. Eng. Part A J. Power Energy 2014, 228, 574–584. [Google Scholar] [CrossRef]
- Menéndez-Blanco, A.; Oro, J.M.F.; Meana-Fernández, A. Unsteady three-dimensional modeling of the Fluid–Structure Interaction in the check valves of diaphragm volumetric pumps. J. Fluids Struct. 2019, 90, 432–449. [Google Scholar] [CrossRef]
- Woo, J.; Sohn, D.K.; Ko, H.S. Analysis of stiffness effect on valve behavior of a reciprocating pump operated by piezoelectric elements. Micromachines 2020, 11, 894. [Google Scholar] [CrossRef]
- Yan, G.; Zhao, J.; Dong, Y. Study of reciprocating pump valve motion law. China Mech. Eng. 2004, 15, 1617–1619. [Google Scholar]
- Ma, Y.; Ni, Y.; Zhang, H.; Zhou, S.; Deng, H. Influence of valve’s lag characteristic on pressure pulsation and performance of reciprocating multiphase pump. J. Pet. Sci. Eng. 2018, 164, 584–594. [Google Scholar] [CrossRef]
- Bergmann, C.M.; Ormiston, S.J.; Chatoorgoon, V. A Comparative Study of Turbulence Models for Prediction of Upward Supercritical Fluid Flow in Vertical Rod Beam Subchannels. Nuclear Eng. Des. 2017, 322, 177–191. [Google Scholar] [CrossRef]
- Sharma, G.; Ghosh, S.; Karmakar, S. Computational fluid dynamic simulation of single and two-phase vortex flow—A comparison of flow field and energy separation. J. Heat Transf. 2016, 138, 082003. [Google Scholar] [CrossRef]





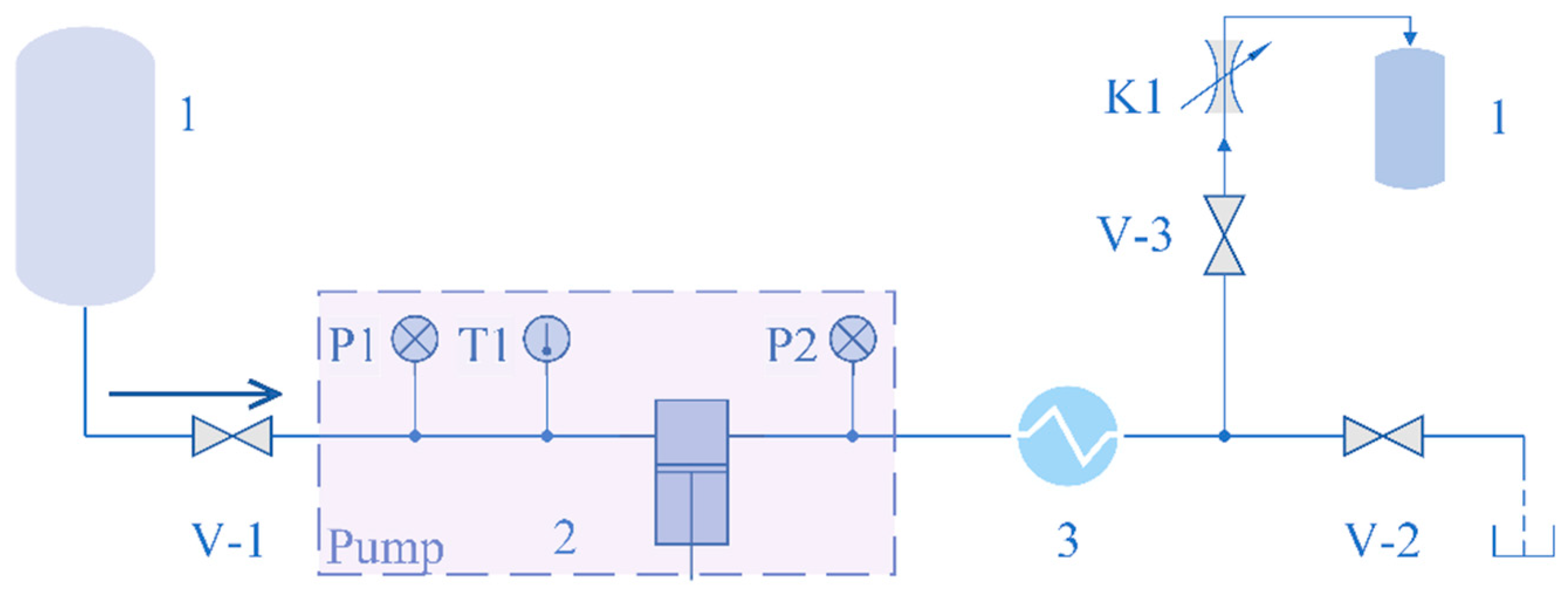

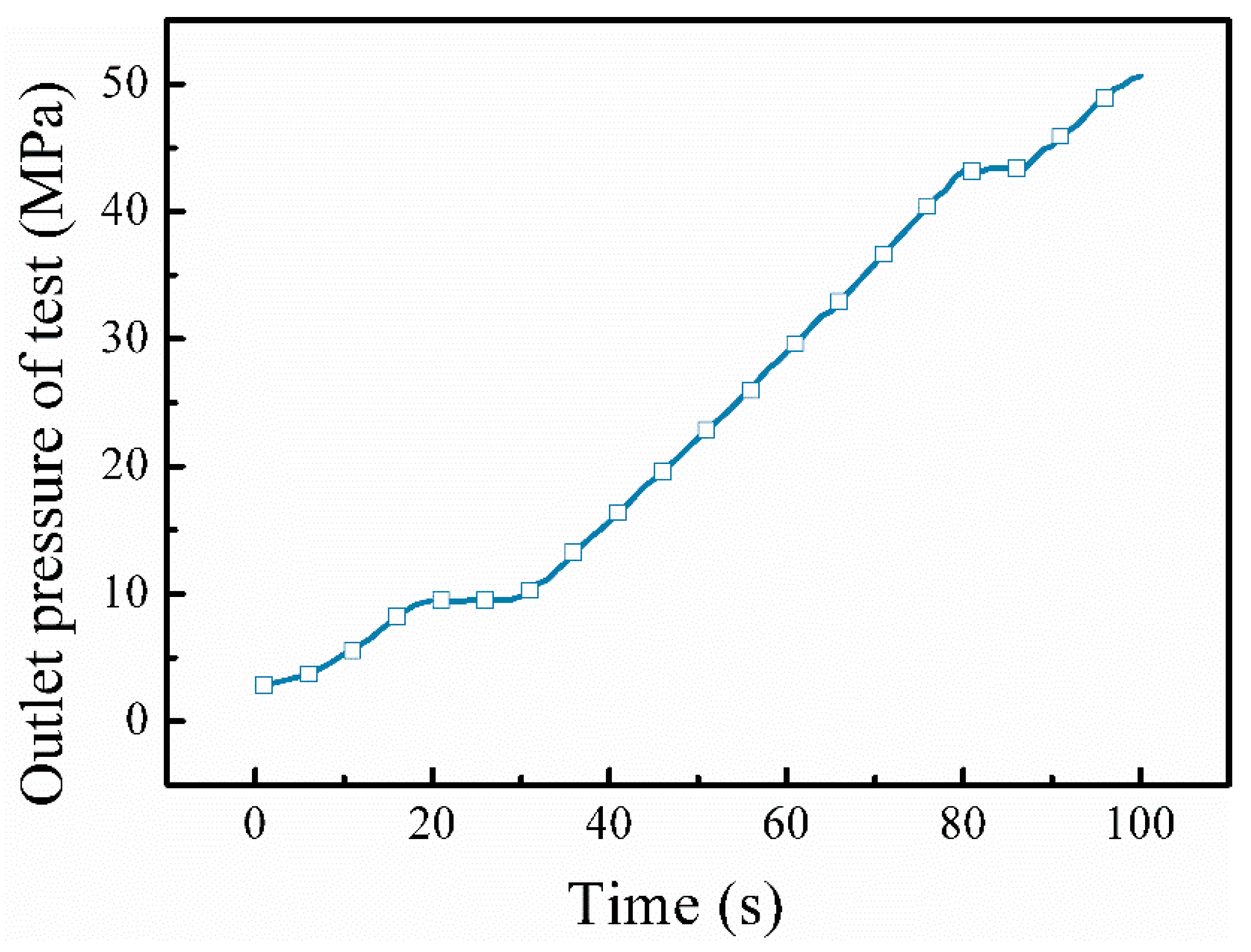
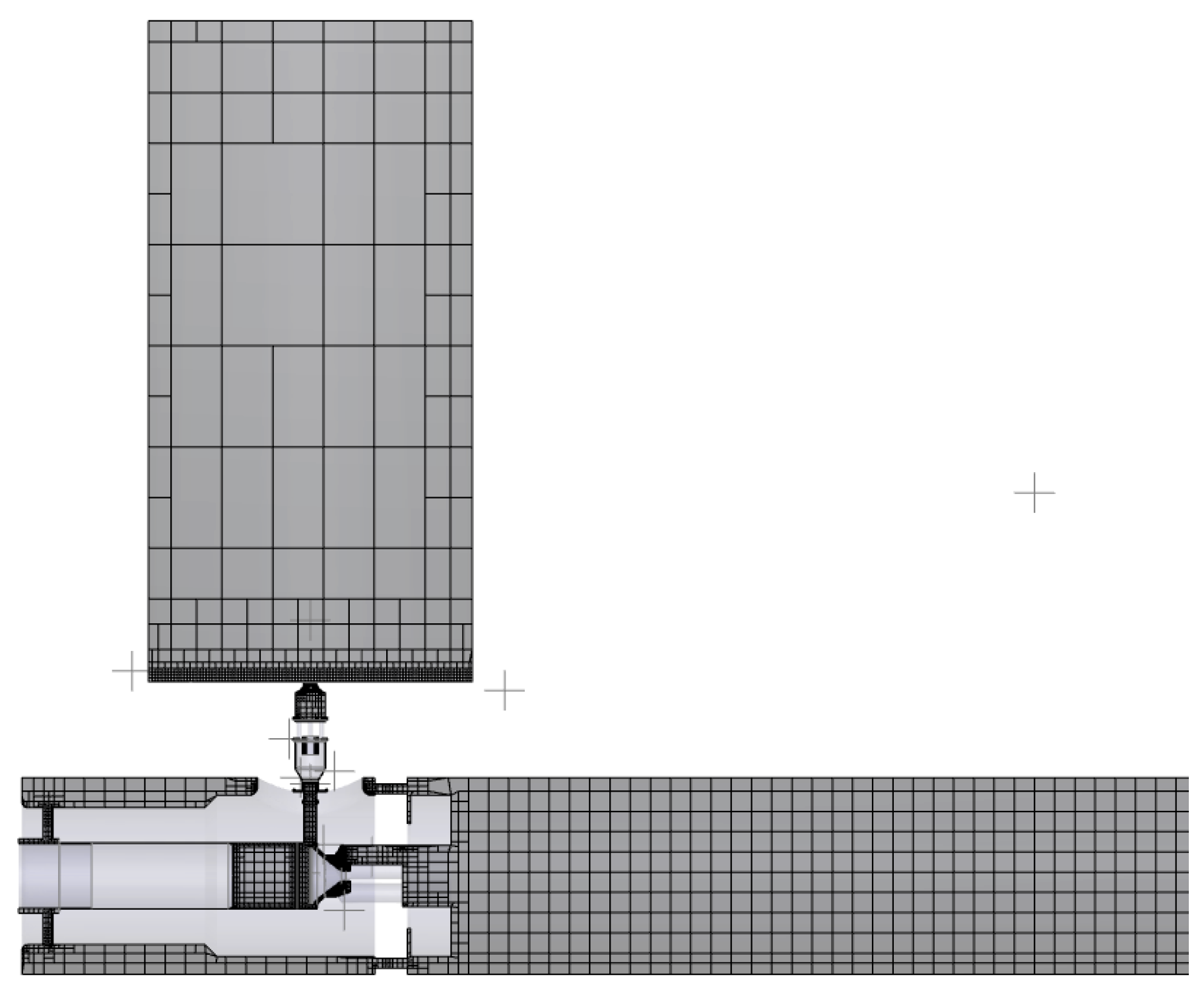


| Parameter Name | Unit | Indicators |
|---|---|---|
| Flow | L/h | 1170 |
| Discharge pressure | MPa | 45 |
| Rotational speed | rpm | 370 |
| Crank length | mm | 25 |
| Crank linkage ratio | 10.8 | |
| Discharge valve sealing width | mm | 6.00 |
| Discharge valve inner diameter | mm | 8.00 |
| Outside diameter of discharge valve | mm | 19.00 |
| Research Content | Spring Stiffness of the Suction Valve (N/m) | Spring Preload of the Suction Valve (N) | Spring Stiffness of the Discharge Valve (N/mm) | Spring Preload of the Discharge Valve (N) | |
|---|---|---|---|---|---|
| Research Content 1 | Case1.1 | 2000 | 10 | 200 | 2000 |
| Research Content 2 | Case2.1 | 2000/6000/10,000/20,000 | 10 | 200 | 2000 |
| Case2.2 | 2000 | 10/60 | 200 | 2000 | |
| Case2.3 | 2000 | 10 | 200 | 200/400/1000/2000/3500 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, N.; Shang, X.; Liu, R.; Jin, P.; Gao, W. Analysis of the Dynamic Characteristics of the Pump Valve System of an Ultra-High Pressure Liquid Hydrogen Reciprocating Pump. Energies 2022, 15, 4255. https://doi.org/10.3390/en15124255
Qiu N, Shang X, Liu R, Jin P, Gao W. Analysis of the Dynamic Characteristics of the Pump Valve System of an Ultra-High Pressure Liquid Hydrogen Reciprocating Pump. Energies. 2022; 15(12):4255. https://doi.org/10.3390/en15124255
Chicago/Turabian StyleQiu, Nanbin, Xianwei Shang, Ruimin Liu, Ping Jin, and Wanli Gao. 2022. "Analysis of the Dynamic Characteristics of the Pump Valve System of an Ultra-High Pressure Liquid Hydrogen Reciprocating Pump" Energies 15, no. 12: 4255. https://doi.org/10.3390/en15124255
APA StyleQiu, N., Shang, X., Liu, R., Jin, P., & Gao, W. (2022). Analysis of the Dynamic Characteristics of the Pump Valve System of an Ultra-High Pressure Liquid Hydrogen Reciprocating Pump. Energies, 15(12), 4255. https://doi.org/10.3390/en15124255






