Design and Analysis of Optimal Current Vector for HTS-Based Multi-Input Wireless Power Transfer Systems
Abstract
:1. Introduction
1.1. Related Surveys
1.2. Contributions of This Study
1.3. Organization of This Paper
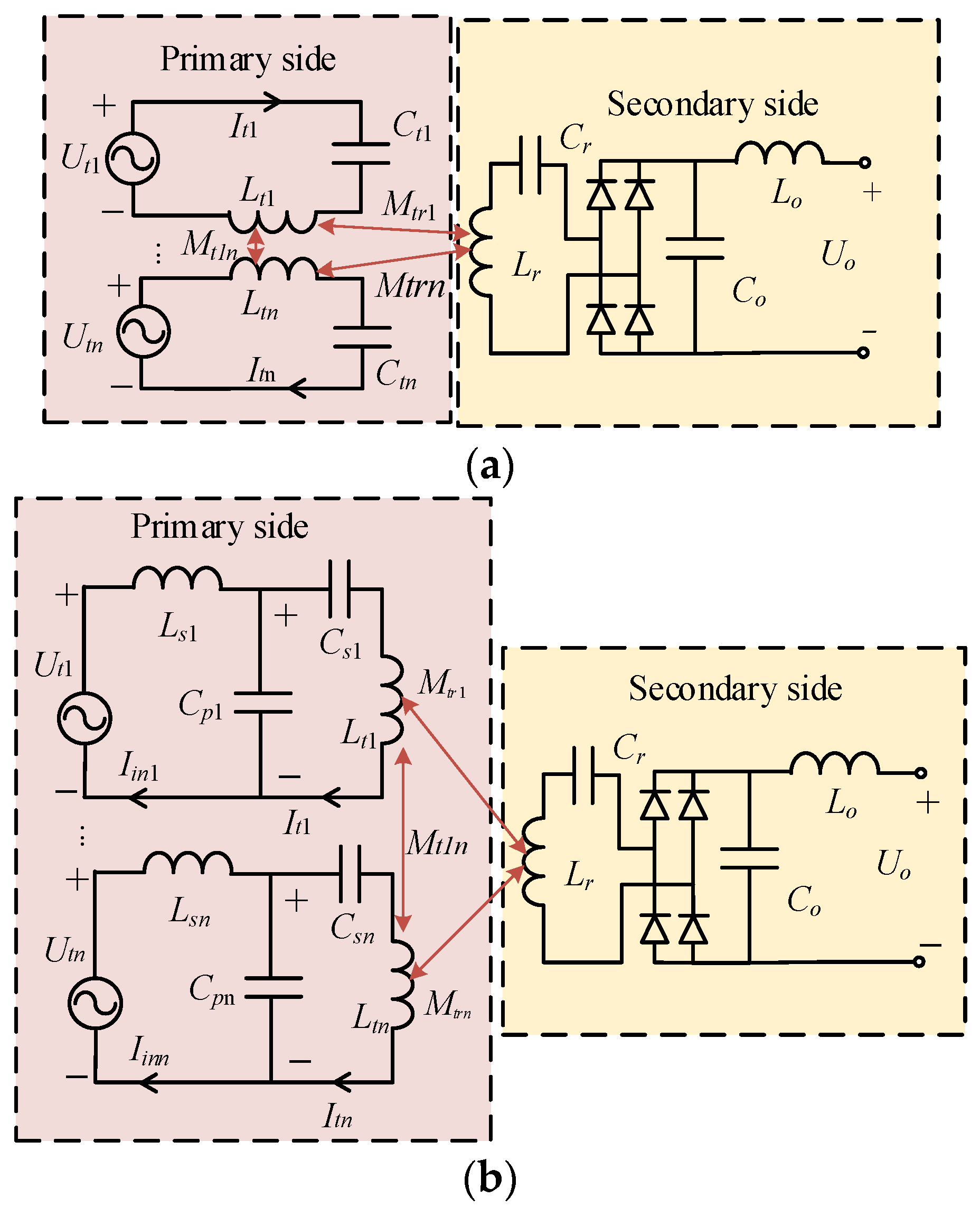
2. Design Scheme
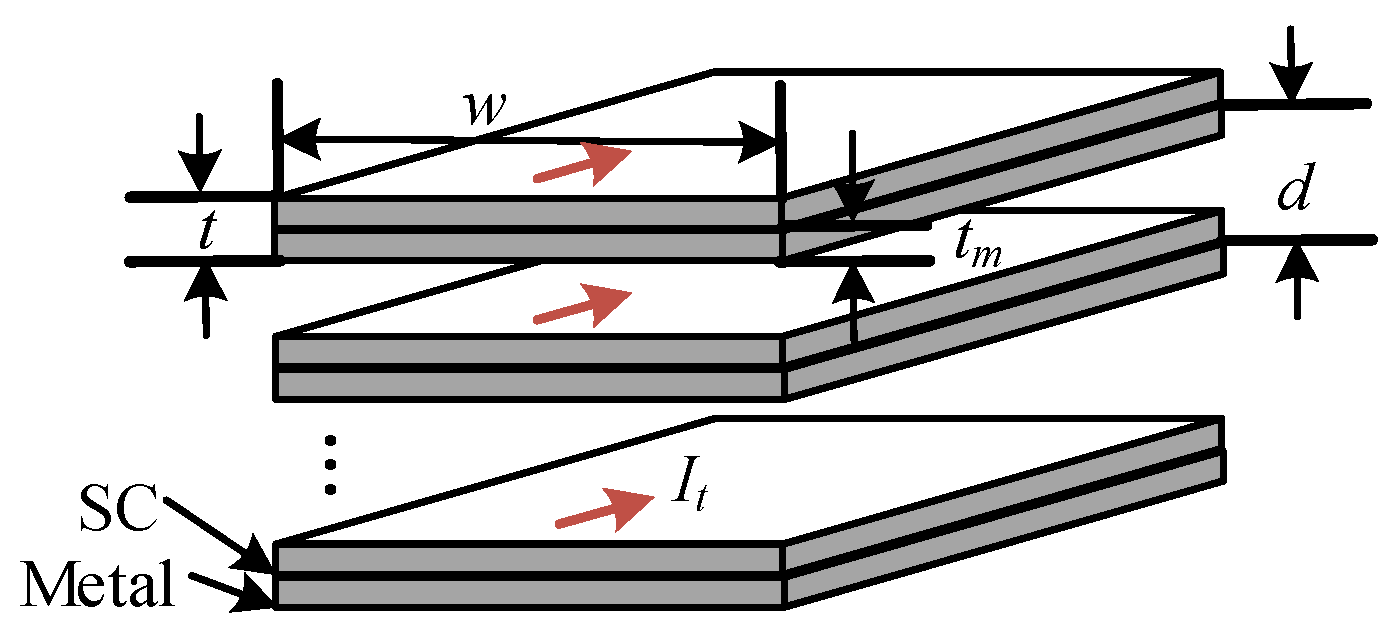
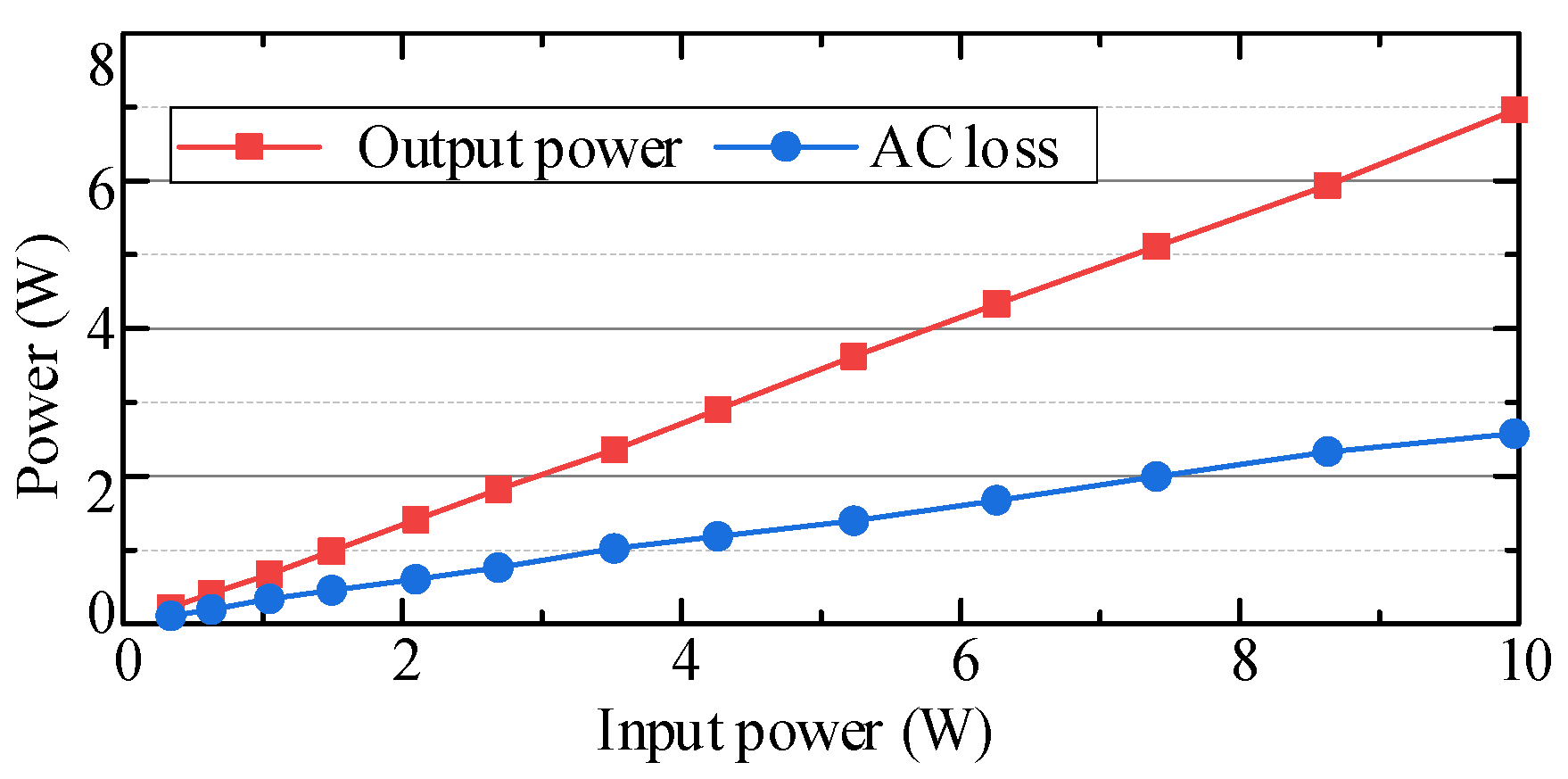
2.1. AC Loss Modelling for the HTS Winding
2.2. Optimal Current Algorithm
2.3. Coil Design
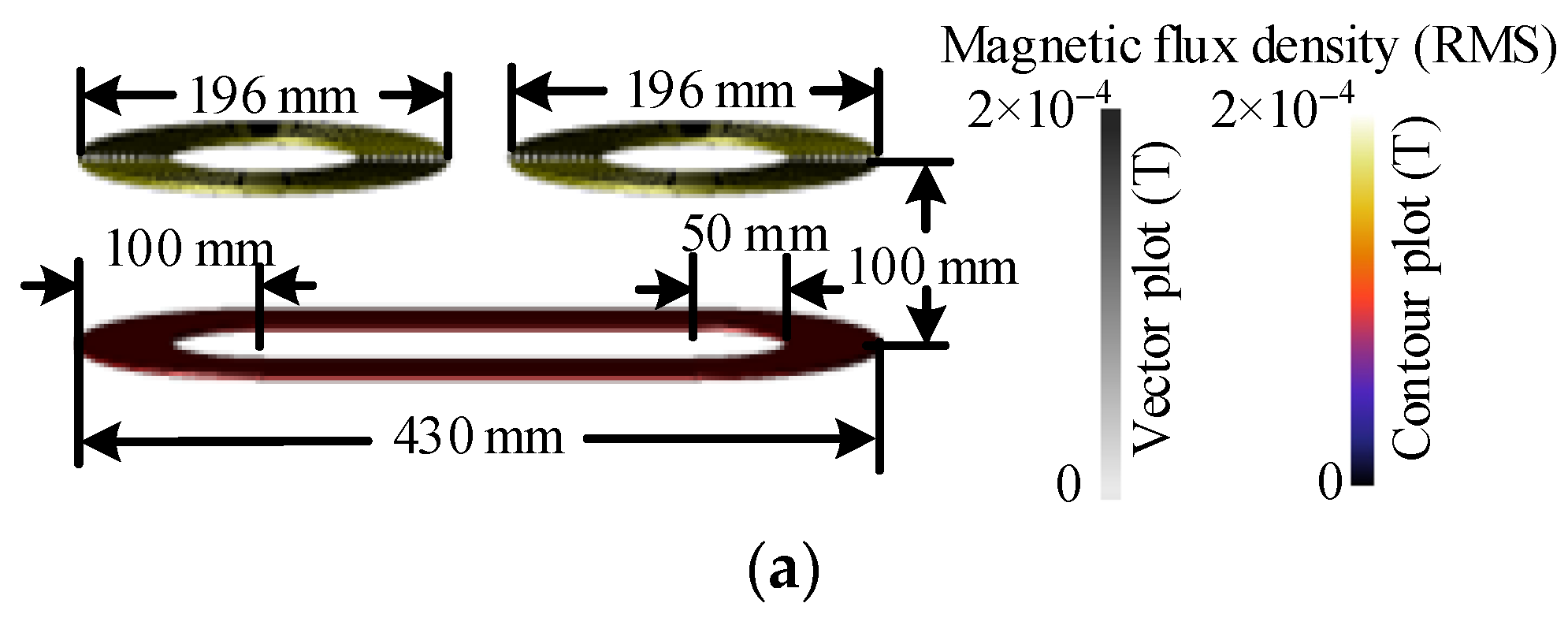
3. System Performance
4. Experimental Validation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Qhyst | Hysteresis loss per cycle |
| Qeddy | Eddy current loss per cycle |
| t | Tape thickness |
| tm | Metal layer thickness of the tape |
| w | Tape width |
| d | Innerturn gap |
| It | Transport current |
| Ic | Critical current |
| fc | Critical frequency |
| Chyst | Constant coefficient for hysteresis loss estimation |
| ω | System angular frequency |
| Ut | Input voltage |
| Mtpi | Mutual inductance between the pth and ith transmitter coils |
| Mrp | Mutual inductance between the receiver coil and the pth transmitter coil |
| Lt | Transmitter inductance |
| Ct | Compensated capacitor for a transmitter in series resonance |
| Ls | Series-connected inductor in the LCC-S topology |
| Cp | Parallel-connected capacitor in the LCC-S topology |
| Ct | Series-connected capacitor in the LCC-S topology |
| Lr | Receiver inductance |
| Cr | Compensated capacitor for the receiver |
| Ir | Compensated capacitor for the receiver |
| Zr | Secondary impedance |
| Rl | Equivalent load resistance |
| γ | Signal noise ratio |
| Ibase | Base current for the optimal current vector |
| φtp | Phase correction angle for the pth input signal in the SS topology |
References
- Clerckx, B.; Huang, K.; Varshney, L.R.; Ulukus, S.; Alouini, M.-S. Wireless power transfer for future networks: Signal processing, machine learning, computing, and sensing. IEEE J. Sel. Top. Signal Process. 2021, 15, 1060–1094. [Google Scholar] [CrossRef]
- Jiang, C.; Chau, K.T.; Leung, Y.Y.; Liu, C.; Lee, C.H.T.; Han, W. Design and analysis of wireless ballastless fluorescent lighting. IEEE Trans. Ind. Electron. 2019, 66, 4065–4074. [Google Scholar] [CrossRef]
- Han, W.; Chau, K.T.; Zhang, Z. Flexible induction heating using magnetic resonant coupling. IEEE Trans. Ind. Electron. 2017, 64, 1982–1992. [Google Scholar] [CrossRef]
- Tian, X.; Chau, K.T.; Han, W.; Lee, C.H.T. Design and analysis of double-layer electromagnetic field limiter for wireless rechargeable medical implants. IEEE Trans. Magn. 2021, 57, 5100206. [Google Scholar] [CrossRef]
- Liu, W.; Chau, K.T.; Chow, C.C.T.; Lee, C.H.T. Wireless energy trading in traffic internet. IEEE Trans. Power Electron. 2022, 37, 4831–4841. [Google Scholar] [CrossRef]
- Huang, Y.; Liu, C.; Xiao, Y.; Liu, S. Separate power allocation and control method based on multiple power channels for wireless power transfer. IEEE Trans. Power Electron. 2020, 35, 9046–9056. [Google Scholar] [CrossRef]
- Lee, H.-S.; Lee, J.-W. Adaptive wireless power transfer beam scheduling for non-static IoT devices using deep reinforcement learning. IEEE Access 2020, 8, 206659–206673. [Google Scholar] [CrossRef]
- Tian, X.; Chau, K.T.; Han, W.; Lee, C.H.T. Analysis of multi-coil omnidirectional energy harvester. IEEE Trans. Magn. 2021, 57, 8000806. [Google Scholar] [CrossRef]
- Wen, F.; Chu, X.; Li, Q.; Zhao, W.; Zhu, X.; Wu, Y. Receiver localization strategy of wireless charging system based on mutual inductance disturbance. IEEE Trans. Appl. Supercond. 2021, 31, 0600604. [Google Scholar] [CrossRef]
- Qian, L.; Chen, M.; Cui, K.; Shi, G.; Wang, J.; Xia, Y. Modeling of mutual inductance between two misalignment planar coils in wireless power transfer. IEEE Microw. Wirel. Compon. Lett. 2020, 30, 814–817. [Google Scholar] [CrossRef]
- Shu, X.; Zhang, B.; Wei, Z.; Rong, C.; Sun, S. Extended-distance wireless power transfer system with constant output power and transfer efficiency based on parity-time-symmetric principle. IEEE Trans. Power Electron. 2021, 36, 8861–8871. [Google Scholar] [CrossRef]
- Dong, Z.; Liu, S.; Li, X.; Xu, Z.; Yang, L. A novel long-distance wireless power transfer system with constant current output based on domino-resonator. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 2343–2355. [Google Scholar] [CrossRef]
- Fujita, T.; Yasuda, T.; Akagi, H. A dynamic wireless power transfer system applicable to a stationary system. IEEE Trans. Ind. Appl. 2017, 53, 3748–3757. [Google Scholar] [CrossRef]
- Zhang, Z.; Chau, K.T. Homogeneous wireless power transfer for move-and-charge. IEEE Trans. Power Electron. 2015, 30, 6213–6220. [Google Scholar] [CrossRef]
- Dai, X.; Jiang, J.; Wu, J. Charging area determining and power enhancement method for multiexcitation unit configuration of wirelessly dynamic charging EV system. IEEE Trans. Ind. Electron. 2019, 66, 4086–4096. [Google Scholar] [CrossRef]
- Tian, X.; Chau, K.T.; Pang, H.; Liu, W. Power adaption design for multifrequency wireless power transfer system. IEEE Trans. Magn. 2021. [Google Scholar] [CrossRef]
- Tian, X.; Chau, K.T.; Hua, Z.; Han, W. Design and analysis of demand-customized selective wireless power transfer system. IEEE Trans. Ind. Electron. 2022. [Google Scholar] [CrossRef]
- Zhong, W.; Hui, S.Y.R. Auxiliary circuits for power flow control in multifrequency wireless power transfer systems with multiple receivers. IEEE Trans. Power Electron. 2015, 30, 5902–5910. [Google Scholar] [CrossRef]
- Kim, M.W.; Kim, J.H.; Cho, Y.; Kim, M.; Choi, B.H.; Lee, K.; Kim, J.; Cho, G.H.; Rim, C.T. High-resolution synthesized magnetic field focusing for RF barcode applications. IEEE Trans. Ind. Electron. 2018, 65, 597–607. [Google Scholar] [CrossRef]
- Kim, J.H.; Choi, B.H.; Kit, H.R.; Rim, C.T. 2-D synthesized magnetic field focusing technology with loop coils distributed in a rectangular formulation. IEEE Trans. Power Electron. 2019, 66, 5558–5566. [Google Scholar] [CrossRef]
- Asiful Huda, S.M.; Arafat, M.Y.; Moh, S. Wireless power transfer in wirelessly powered sensor networks: A Review of recent progress. Sensors 2022, 22, 2952. [Google Scholar] [CrossRef] [PubMed]
- Jung, H.; Lee, B. Optimization of magnetic field focusing and null steering for selective wireless power transfer. IEEE Trans. Power Electron. 2020, 35, 4622–4633. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, X.; Ji, Y.; Xu, C. Random energy beamforming for magnetic MIMO wireless power transfer system. IEEE Internet Things J. 2020, 7, 1773–1787. [Google Scholar] [CrossRef]
- Yang, G.; Moghadam, M.R.V.; Zhang, R. Magnetic MIMO signal processing and optimization for wireless power transfer. IEEE Trans. Signal Process. 2017, 65, 2860–2874. [Google Scholar] [CrossRef] [Green Version]
- Tang, W.; Zhu, Q.; Yang, J.; Song, D.; Su, M.; Zou, R. Simultaneous 3-D wireless power transfer to multiple moving devices with different power demands. IEEE Trans. Power Electron. 2020, 35, 4533–4546. [Google Scholar] [CrossRef]
- Lu, Z.; Zhao, Y.; Liu, D. Adaptive impedance matching scheme for magnetic MIMO wireless power transfer system. Electronics 2021, 10, 2788. [Google Scholar] [CrossRef]
- Hwang, Y.J.; Jang, J.Y. Design and characteristic analysis of an inductive link for wireless current charging of a HTS magnet. IEEE Trans. Appl. Supercond. 2022, 32, 4600905. [Google Scholar] [CrossRef]
- Philip, M.; Zhang, H.; Kails, K.; Li, Q. Loss characteristics of superconducting pancake, solenoid and spiral coils for wireless power transfer. Supercond. Sci. Technol. 2020, 33, 074008. [Google Scholar]
- Chung, Y.D.; Lee, C.Y.; Kang, H.K.; Park, Y.G. Design consideration and efficiency comparison of wireless power transfer with HTS and cooled copper antennas for electric vehicle. IEEE Trans. Appl. Supercond. 2015, 25, 5000205. [Google Scholar] [CrossRef]
- Grilli, F.; Pardo, E.; Stenvall, A.; Nguyen, D.N.; Yuan, W.; Gömöry, F. Computation of losses in HTS under the action of varying magnetic fields and currents. IEEE Trans. Appl. Supercond. 2014, 24, 78–110. [Google Scholar] [CrossRef]
- Müller, K.-H. AC losses in stacks and arrays of YBCO/Hastelloy and monofilamentary Bi-2223/Ag tapes. Phys. C Supercond. 1999, 312, 149–167. [Google Scholar] [CrossRef]
- Utschick, C.; Som, C.; Šouc, J.; Große, V.; Gömöry, F.; Gross, R. Superconducting wireless power transfer beyond 5 kW at high power density for industrial applications and fast battery charging. IEEE Trans. Appl. Supercond. 2021, 31, 5500110. [Google Scholar] [CrossRef]
- Jiang, Z.; Long, N.J.; Staines, M.; Li, Q.; Slade, R.A.; Amemiya, N.; Caplin, A.D. Transport AC loss measurements in single- and two-layer parallel coated conductor arrays with low turn numbers. IEEE Trans. Appl. Supercond. 2012, 32, 8200306. [Google Scholar] [CrossRef]
- Tian, X.; Chau, K.T.; Liu, W.; Lee, C.H.T. Selective wireless power transfer using magnetic field editing. IEEE Trans. Power Electron. 2019, 66, 245–254. [Google Scholar] [CrossRef]
- Zhu, Q.; Wang, L.; Guo, Y.; Liao, C.; Li, F. Applying LCC compensation network to dynamic wireless EV charging system. IEEE Trans. Ind. Electron. 2016, 63, 6557–6567. [Google Scholar] [CrossRef]
- Tian, X.; Chau, K.T.; Liu, W.; Pang, H.; Lee, C.H.T. Maximum power tracking for magnetic field editing based omnidirectional wireless power transfer. IEEE Trans. Power Electronics. 2022. [Google Scholar] [CrossRef]
- Aslam, N.; Xia, K.; Hadi, M.U. Optimal wireless charging inclusive of intellectual routing based on SARSA learning in renewable wireless sensor networks. IEEE Sens. J. 2019, 19, 8340–8351. [Google Scholar] [CrossRef]
- Chen, J.C.; Kan, P.; Yu, Z.; Alrashdan, F.; Garcia, R.; Singer, A.; Lai, C.S.; Avants, B.; Crosby, S.; Li, Z.; et al. A wireless millimetric magnetoelectric implant for the endovascular stimulation of peripheral nerves. Nat. Biomed. Eng. 2022. [Google Scholar] [CrossRef]
- He, Y.; Wang, Y.; Nie, X.; Chen, W.; Yan, Z. High-temperature superconducting capacitor and its application to a superconducting wireless power transfer system. IEEE Trans. Appl. Supercond. 2019, 29, 5000207. [Google Scholar] [CrossRef]
- Chung, Y.D.; Lee, C.Y.; Kim, D.W.; Kang, H.; Park, Y.G.; Yoon, Y.S. Conceptual design and operating characteristics of multi-resonance antennas in the wireless power charging system for superconducting MAGLEV train. IEEE Trans. Appl. Supercond. 2017, 27, 3601805. [Google Scholar] [CrossRef]
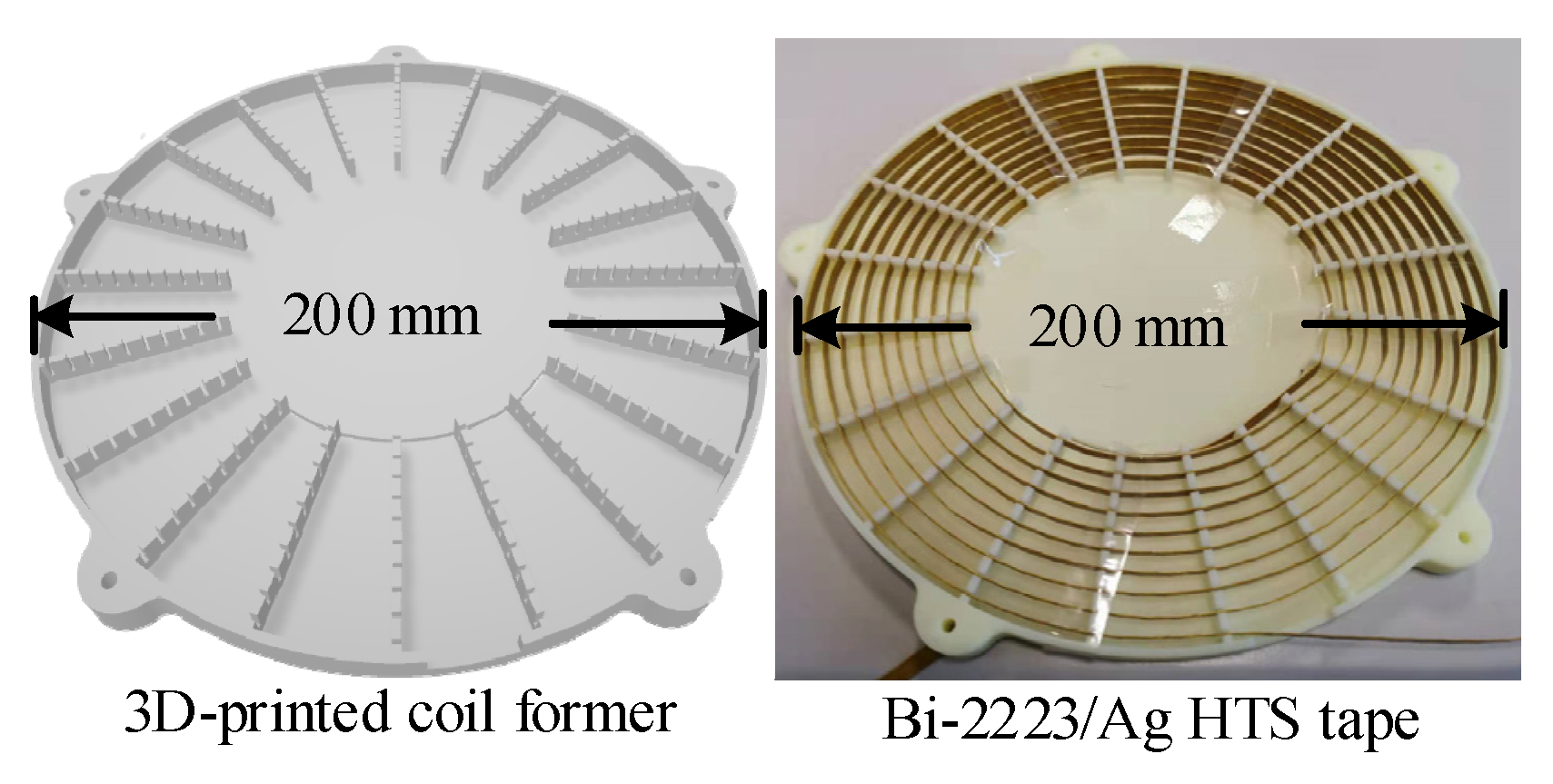


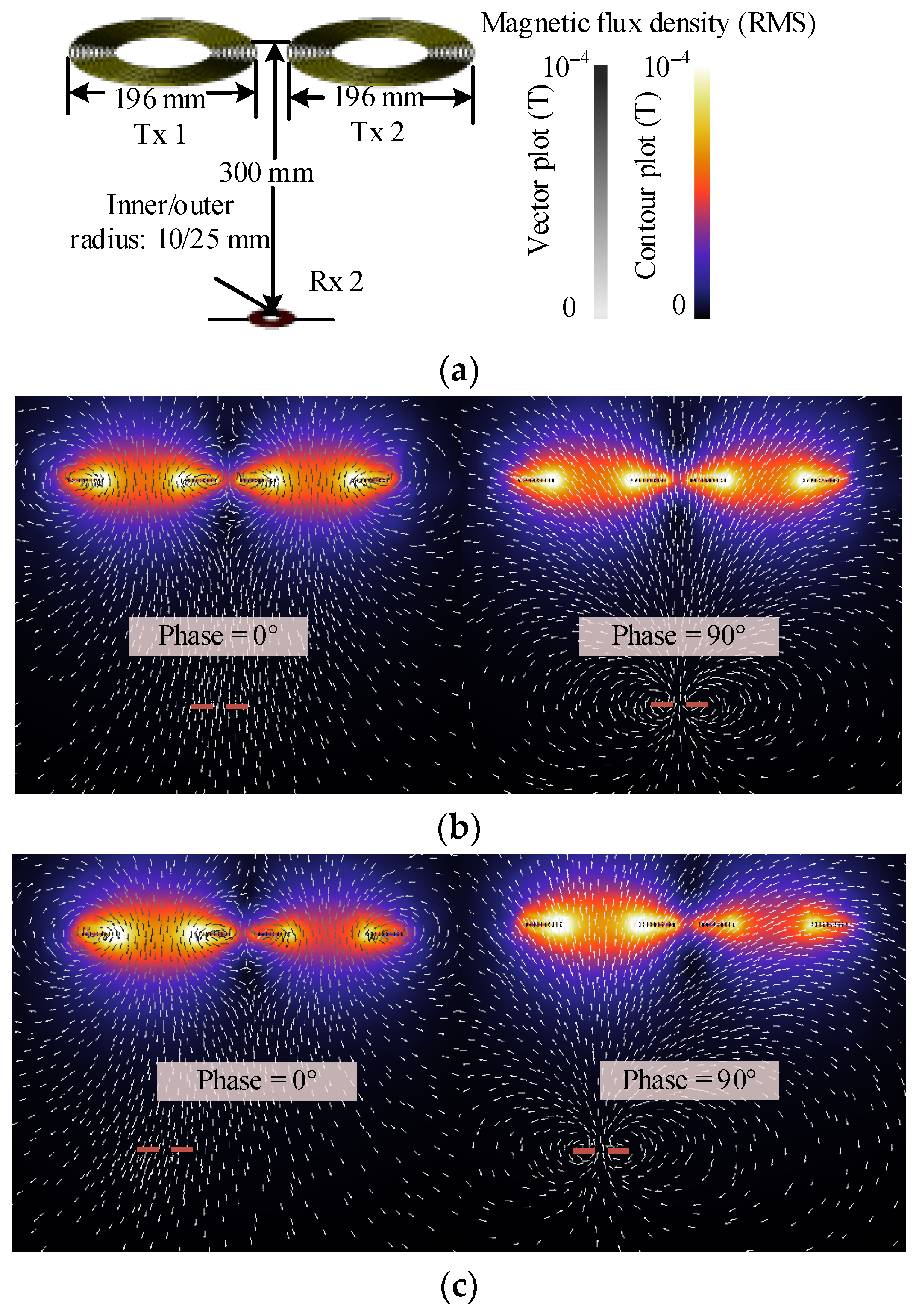
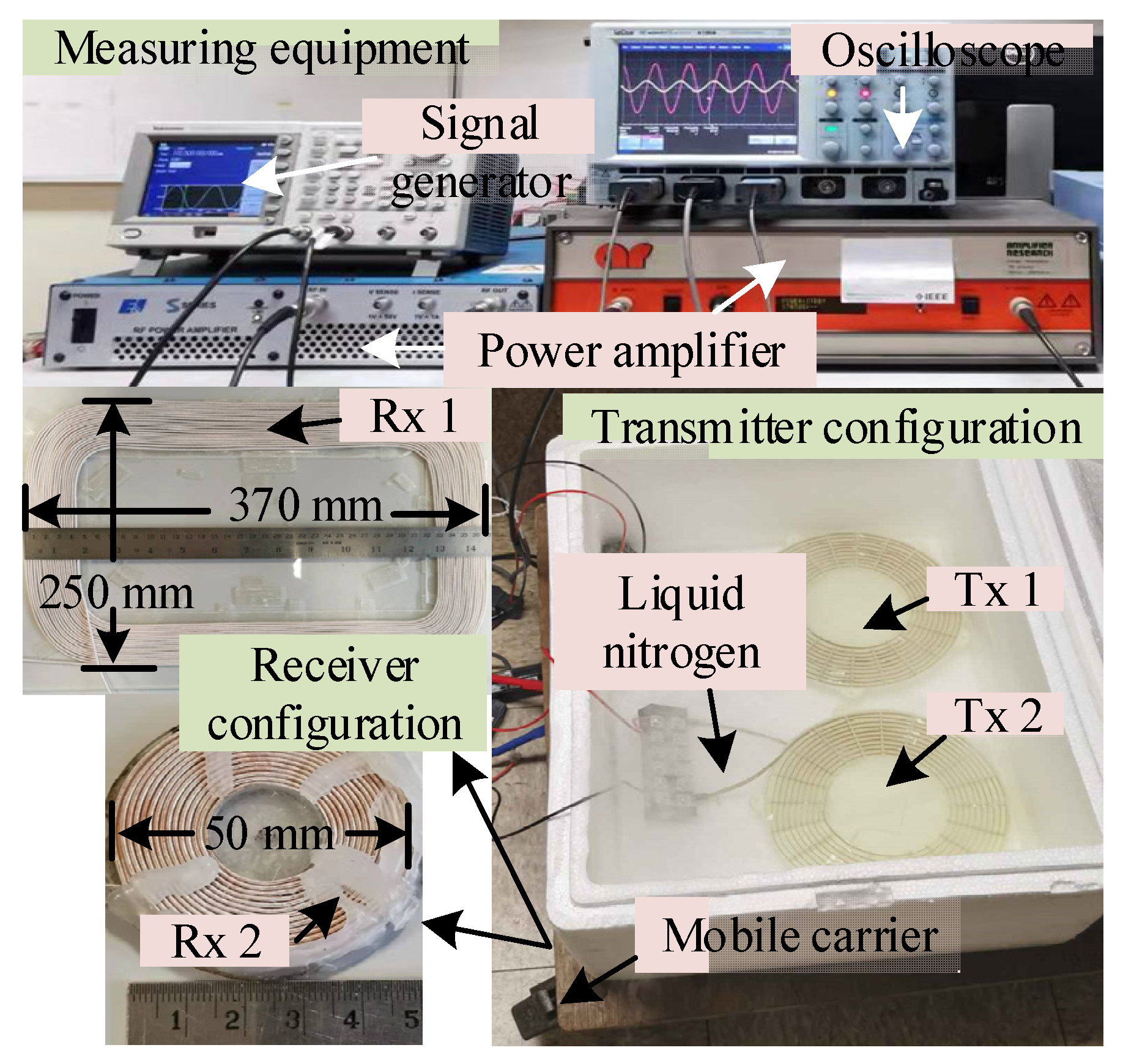
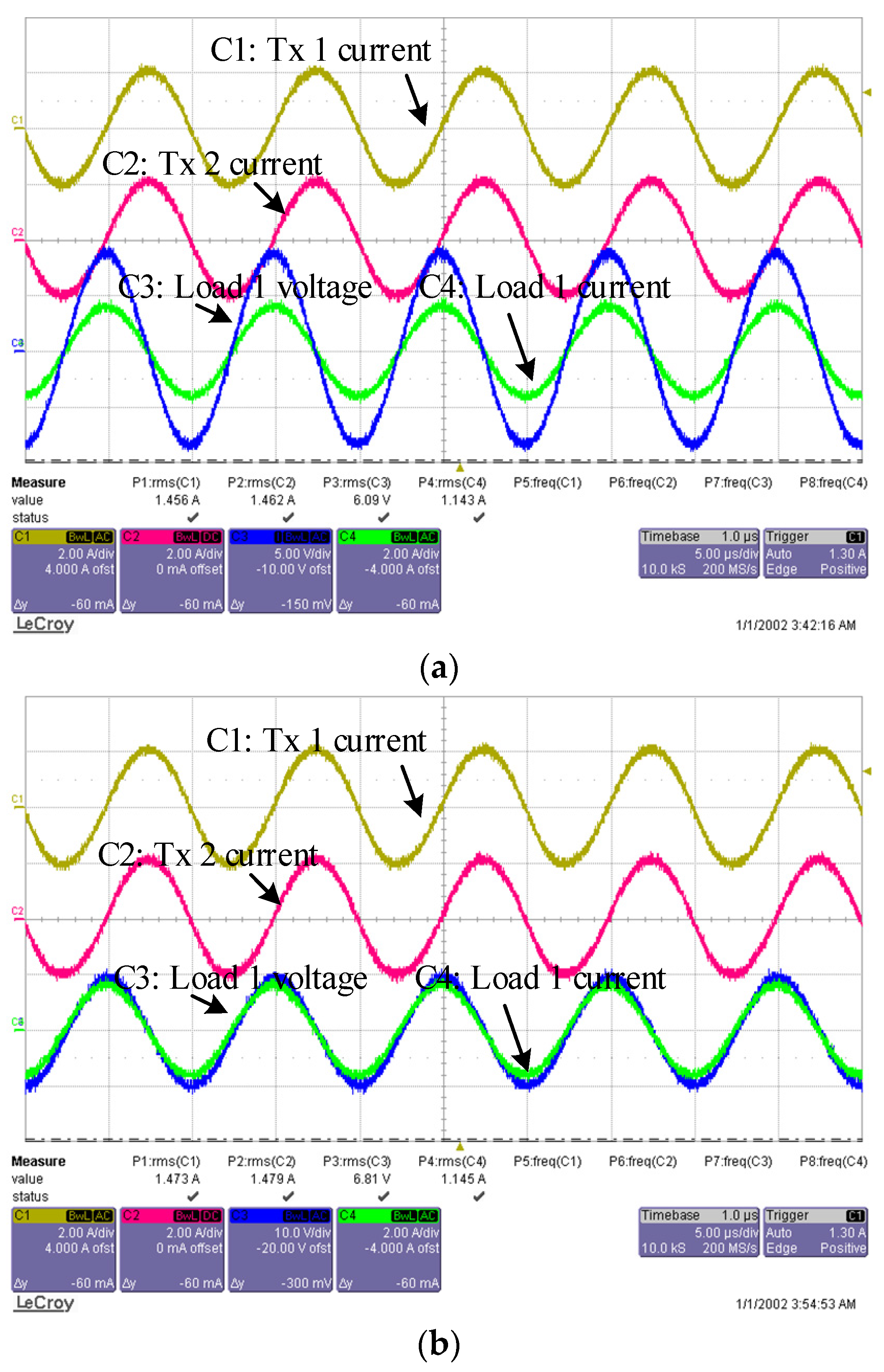
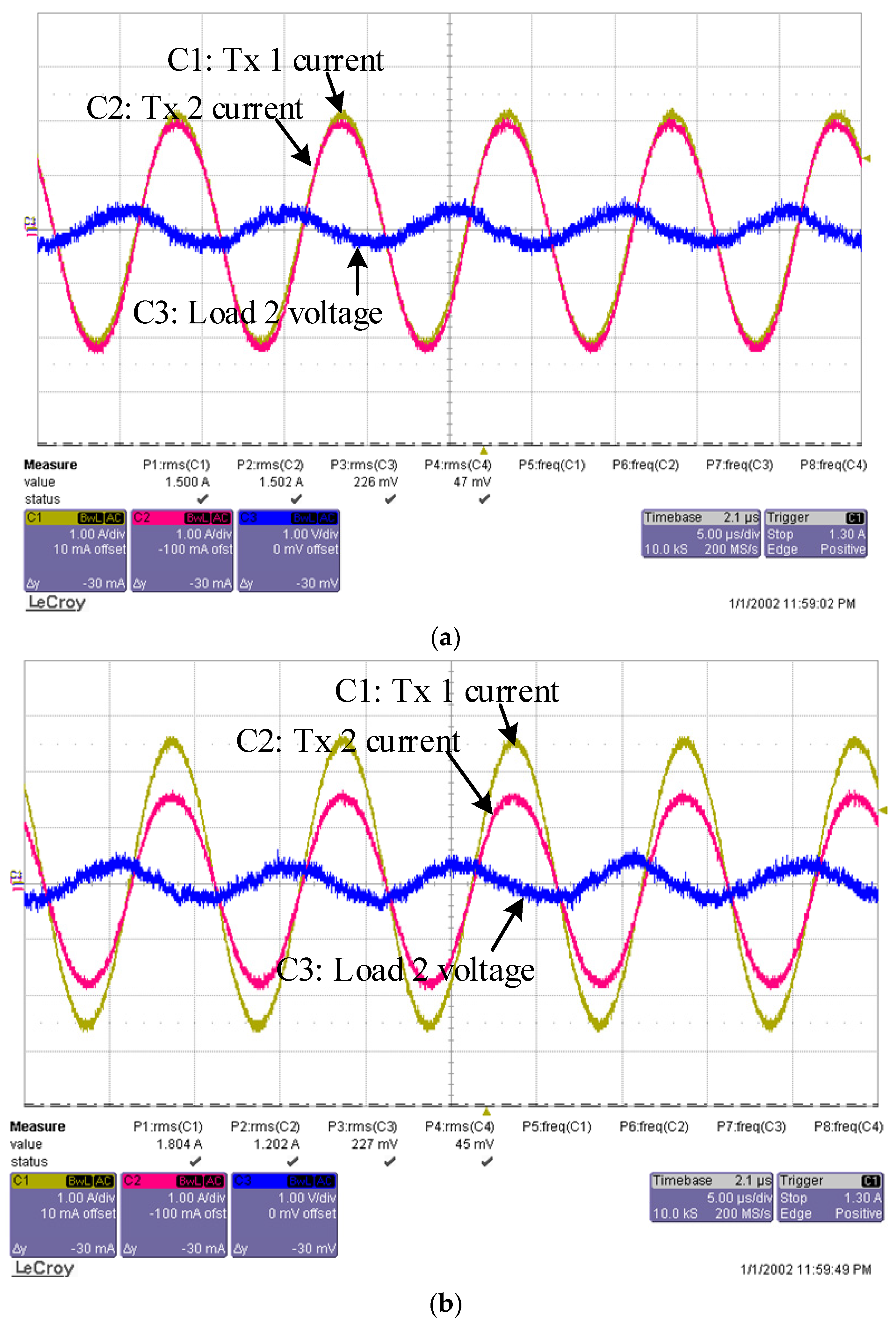
| Items | Value |
|---|---|
| Material (SC/metal) | Bi-2223/Ag |
| Thickness × width (t × w) | 0.5 × 4 mm |
| Number of turns | 10 |
| Inner radius | 53 mm |
| Interturn gap (d) | 4.5 mm |
| Outer radius | 98 mm |
| Total length | 4.2 m |
| Inductance (averaged) | 20.4 μH |
| Items | Value |
|---|---|
| Inductance of Rx 1 | 208.5 μH |
| Compensated capacitor for Rx 1 | 12.1 nF |
| Resistance of Rx 1 | 0.3 Ω |
| Load resistor 1 | 5 Ω |
| Inductance of Rx 2 | 36.6 μH |
| Compensated capacitor for Rx 2 | 69.2 nF |
| Resistance of Rx 2 | 0.5 Ω |
| Load resistor 2 | 50 Ω |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, X.; Chau, K.T.; Liu, W. Design and Analysis of Optimal Current Vector for HTS-Based Multi-Input Wireless Power Transfer Systems. Energies 2022, 15, 4337. https://doi.org/10.3390/en15124337
Tian X, Chau KT, Liu W. Design and Analysis of Optimal Current Vector for HTS-Based Multi-Input Wireless Power Transfer Systems. Energies. 2022; 15(12):4337. https://doi.org/10.3390/en15124337
Chicago/Turabian StyleTian, Xiaoyang, Kwok Tong Chau, and Wei Liu. 2022. "Design and Analysis of Optimal Current Vector for HTS-Based Multi-Input Wireless Power Transfer Systems" Energies 15, no. 12: 4337. https://doi.org/10.3390/en15124337
APA StyleTian, X., Chau, K. T., & Liu, W. (2022). Design and Analysis of Optimal Current Vector for HTS-Based Multi-Input Wireless Power Transfer Systems. Energies, 15(12), 4337. https://doi.org/10.3390/en15124337






