Investigating Drillstring Vibration and Stability in Coring Drilling
Abstract
:1. Introduction
2. Model Development
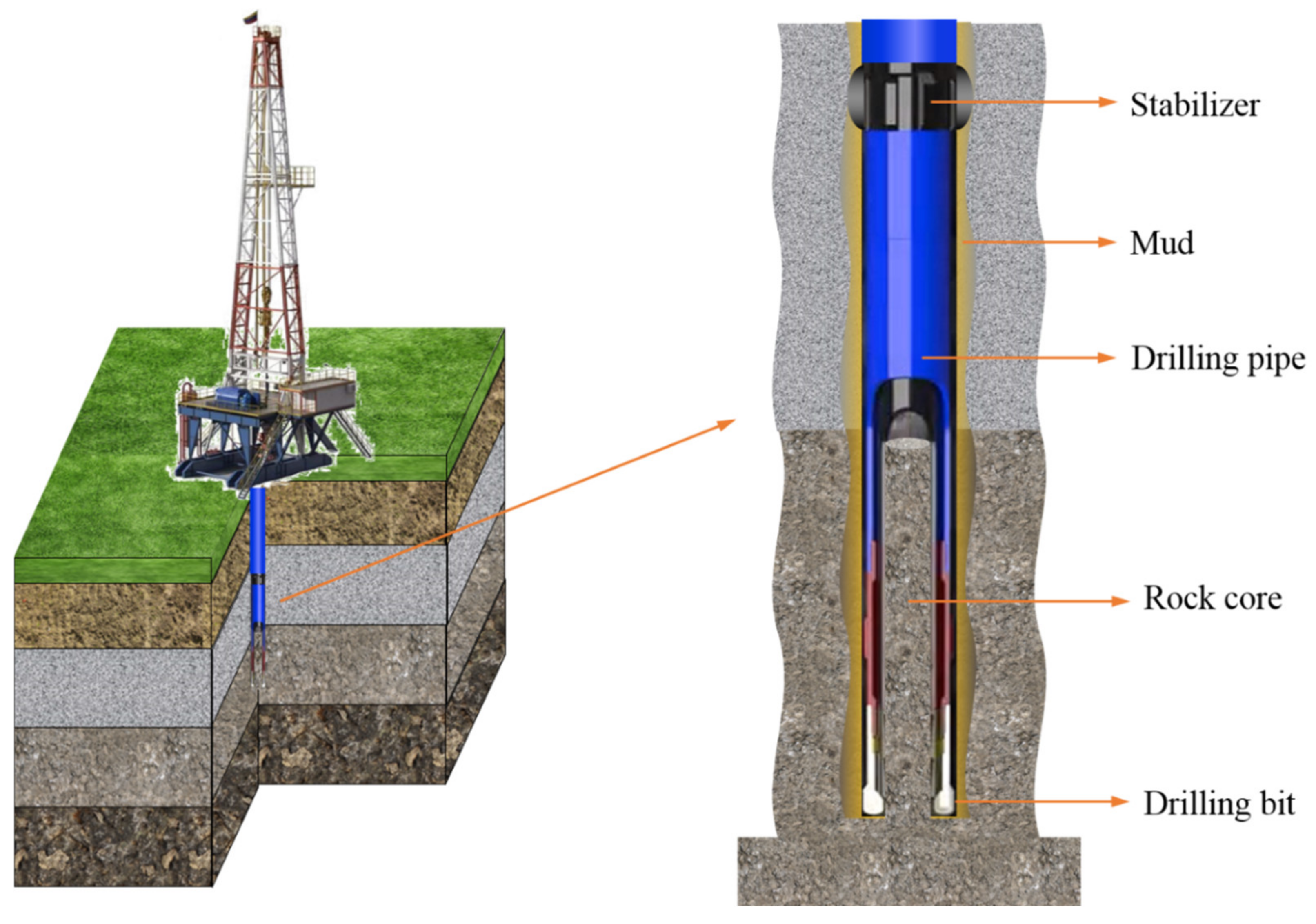
2.1. Simplified Model
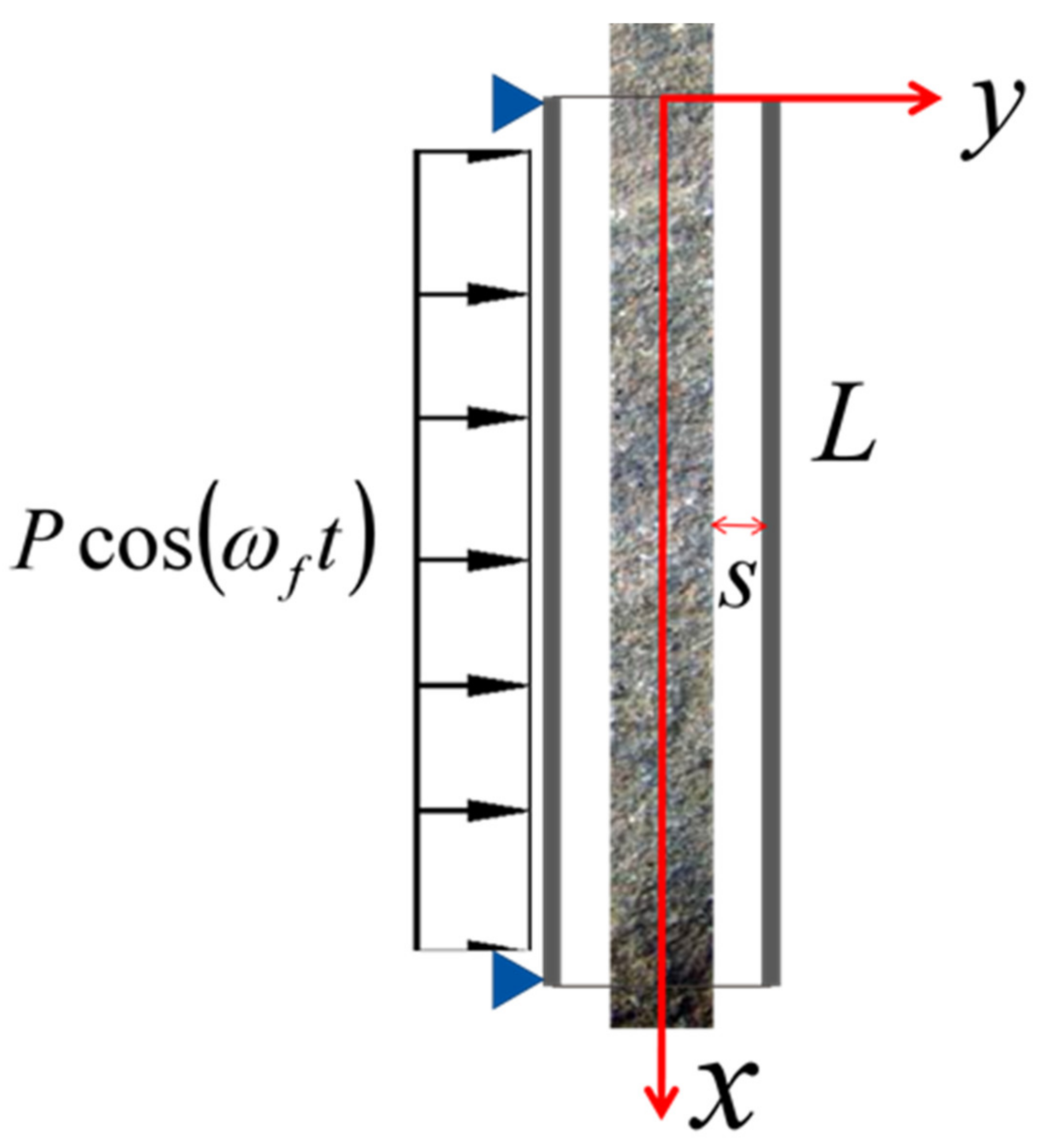
2.2. Governing Equations
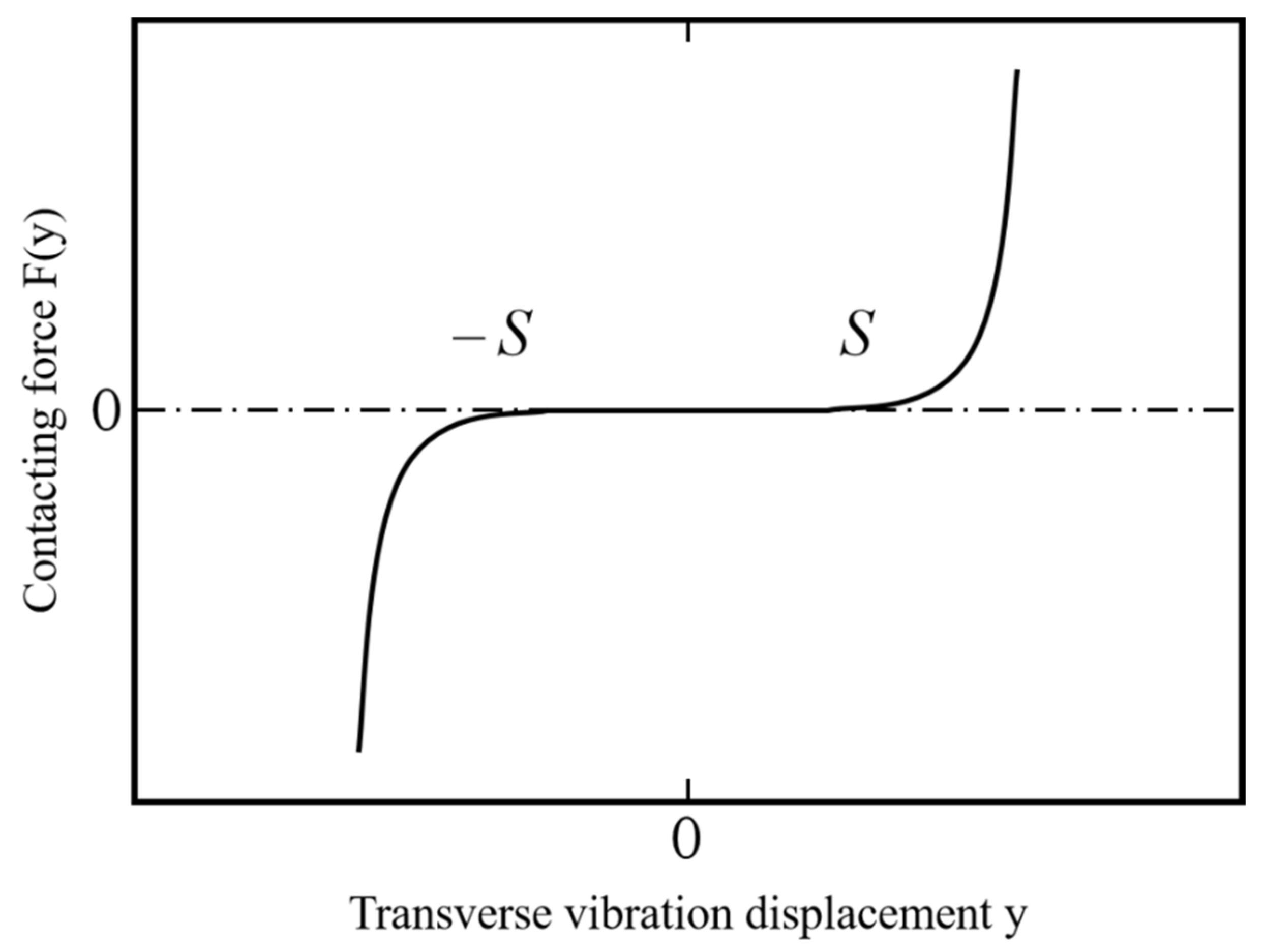
2.3. Discretization, Linear System, and Nonlinear Systems
3. Perturbation Solution for Response
3.1. Primary Resonance
3.2. Secondary Resonances
3.2.1. Subharmonic Resonance
3.2.2. Superharmonic Resonance
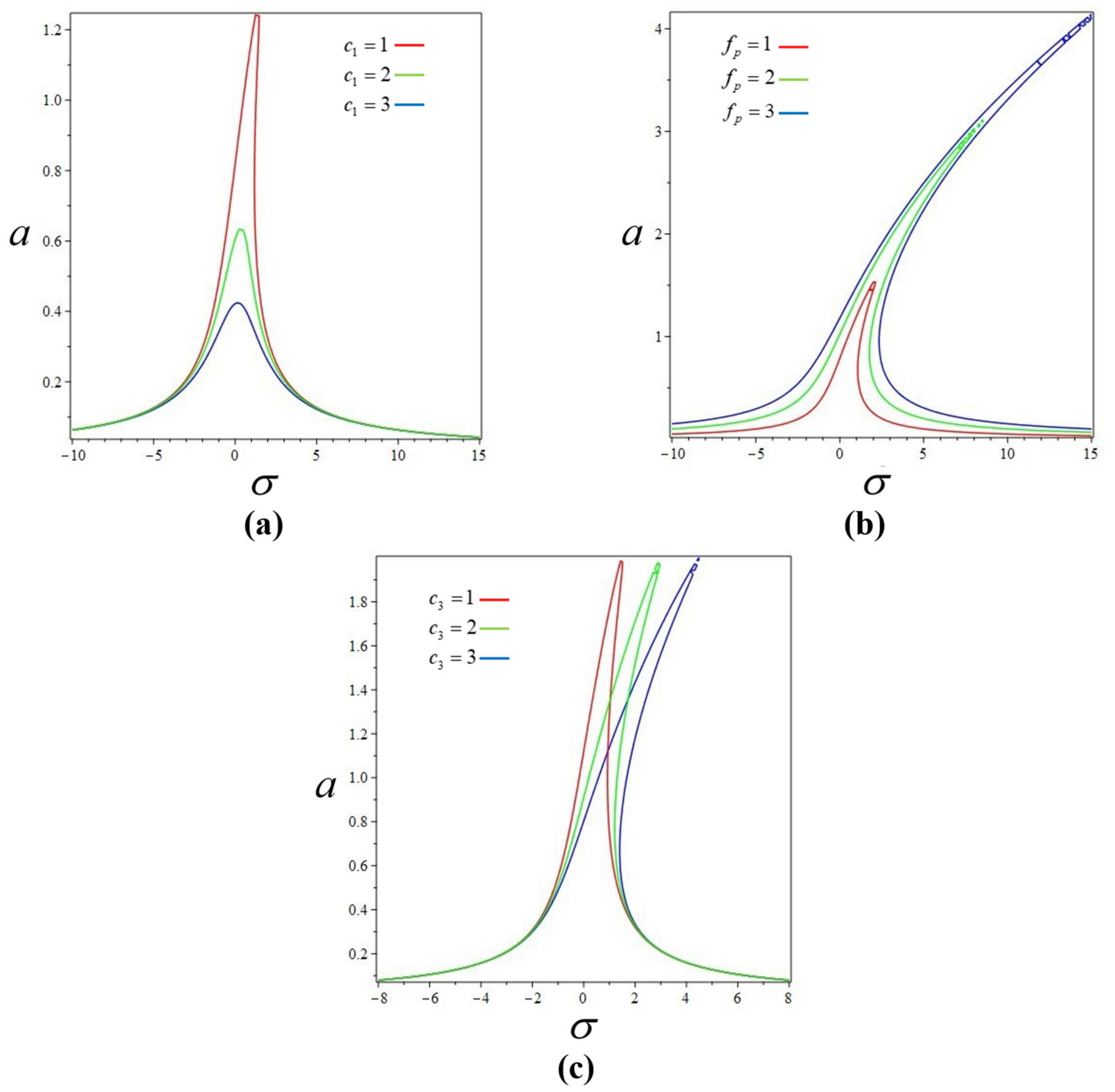
4. Amplitude-Frequency Response Analysis
4.1. Principal Resonance Response
4.2. Secondary Resonances Response
4.2.1. Subharmonic Resonance Response
4.2.2. Superharmonic Resonance Response
5. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahmed, U.; Meehan, D.N. Unconventional Oil and Gas Resources: Exploitation and Development; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Teodoriu, C.; Bello, O. An Outlook of Drilling Technologies and Innovations: Present Status and Future Trends. Energies 2021, 14, 4499. [Google Scholar] [CrossRef]
- Nikolaos, P.P. An Approach for Efficient Analysis of Drill-String Random Vibrations; Dissertation, Taxes; Rice University: Houston, TX, USA, 2002. [Google Scholar]
- Ritto, T.G.; Soize, C.; Sampaio, R. Non-linear dynamics of a drill-string with uncertain model of the bit-rock interaction. Int. J. Non-Linear Mech. 2009, 44, 865–876. [Google Scholar] [CrossRef] [Green Version]
- Apostal, M.C.; Haduch, G.A.; Williams, J.B. A Study to Determine the Effect of Damping on Finite-Element-Based, Forced-Frequency-Response Models for Bottomhole Assembly Vibration Analysis. In Proceedings of the SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 23–26 September 1990. [Google Scholar]
- Jansen, J.D. Non-Linear Rotor Dynamics as Applied to Oilwell Drillstring Vibrations. J. Sound Vib. 1991, 147, 115–135. [Google Scholar] [CrossRef]
- Vaz, M.A.; Patel, M.H. Analysis of Drill Strings in Vertical and Deviated Holes Using the Galerkin Technique. Eng. Struct. 1995, 17, 437–442. [Google Scholar] [CrossRef]
- Khulief, Y.A.; Al-Naser, H. Finite Element Dynamic Analysis of Drillstrings. Finite Elem. Anal. Des. 2005, 41, 1270–1288. [Google Scholar] [CrossRef]
- Omojuwa, E.O.; Osisanya, S.; Ahmed, R. Influence of Dynamic Drilling Parameters on Axial Load and Torque Transfer in Extended-Reach Horizontal Wells. In Proceedings of the SPE Annual Technical Conference and Exhibition, ATCE 2014, Amsterdam, The Netherlands, 27–29 October 2014. [Google Scholar]
- Zhao, Z.B.; Qiu, X.Q.; Xu, J.L. Finite element analysis of modal vibrations of drill strings in deep-sea natural gas hydrate coring/drilling. Nat. Gas Ind. 2011, 1, 73–76. [Google Scholar]
- Liang, J.; Guo, B.K.; Wang, Z.G.; Sun, J.H.; Li, X.M.; Yin, H. Dynamics Behavior of Compound Drill String for Wire-line Coring. Explor. Eng. 2017, 44, 34–40. [Google Scholar]
- Liu, Y.S.; Gao, D.L.; Vipin, A. Drillstring Oscillations: The Influence of Fluid Loading and Stabilizer Effects. Shock Vib. 2021, 2021, 8888837. [Google Scholar] [CrossRef]
- Kamgue, L.; Arnaud, R.; Deng, J.; Feng, Y.C.; Li, H.T.; Oloruntoba, A.; Songwe, S.; Naomie, B.; Marembo, M.; Sun, Y.X.; et al. Numerical Investigation of the Influence of the Drill String Vibration Cyclic Loads on the Development of the Wellbore Natural Fracture. Energies 2021, 14, 2015. [Google Scholar] [CrossRef]
- Païdoussis, M.P.; Li, G.X. Cross-flow-induced chaotic vibrations of heat-exchanger tubes impacting on loose supports. J. Sound Vib. 1992, 152, 305–326. [Google Scholar] [CrossRef] [Green Version]

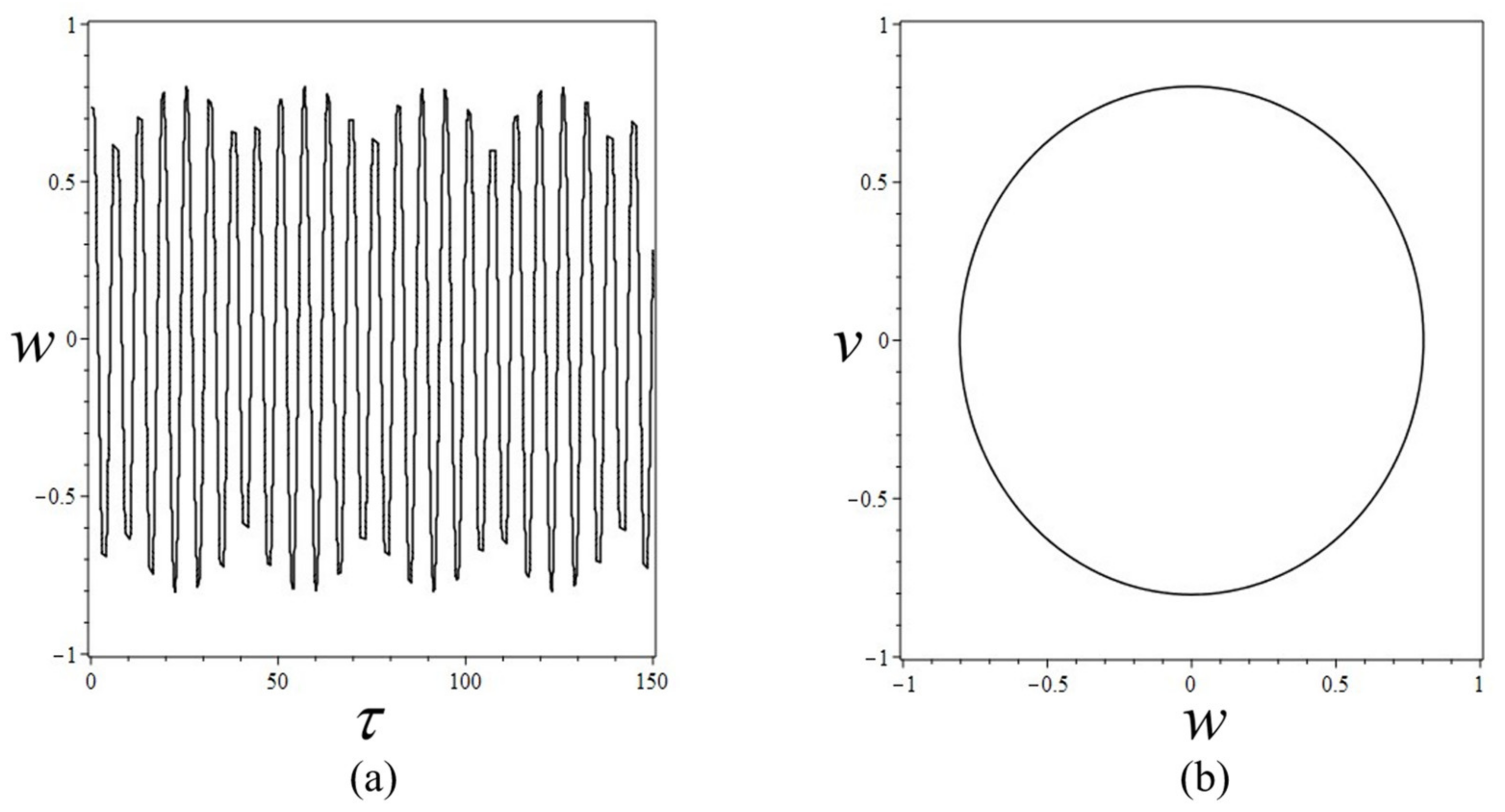
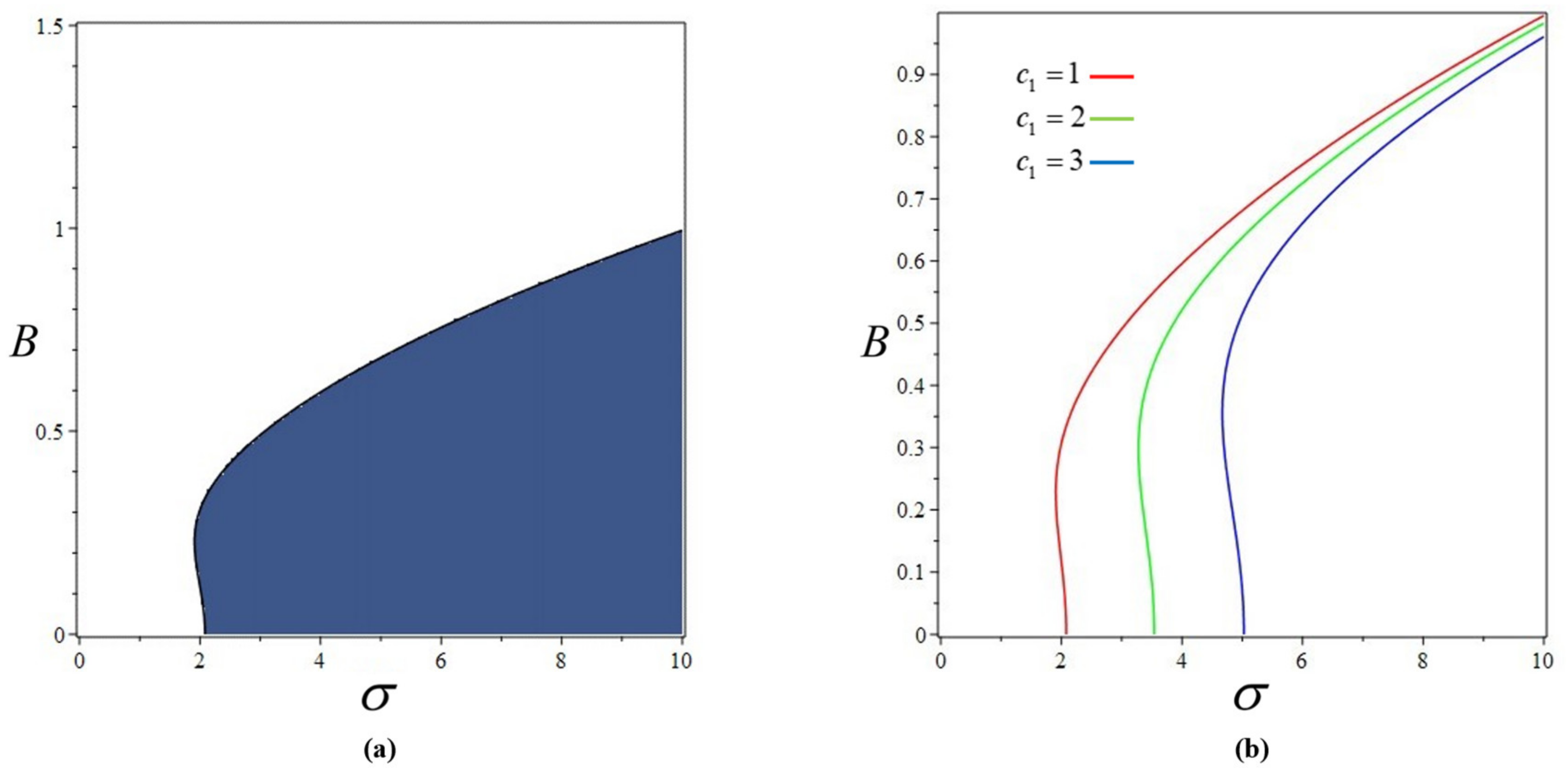

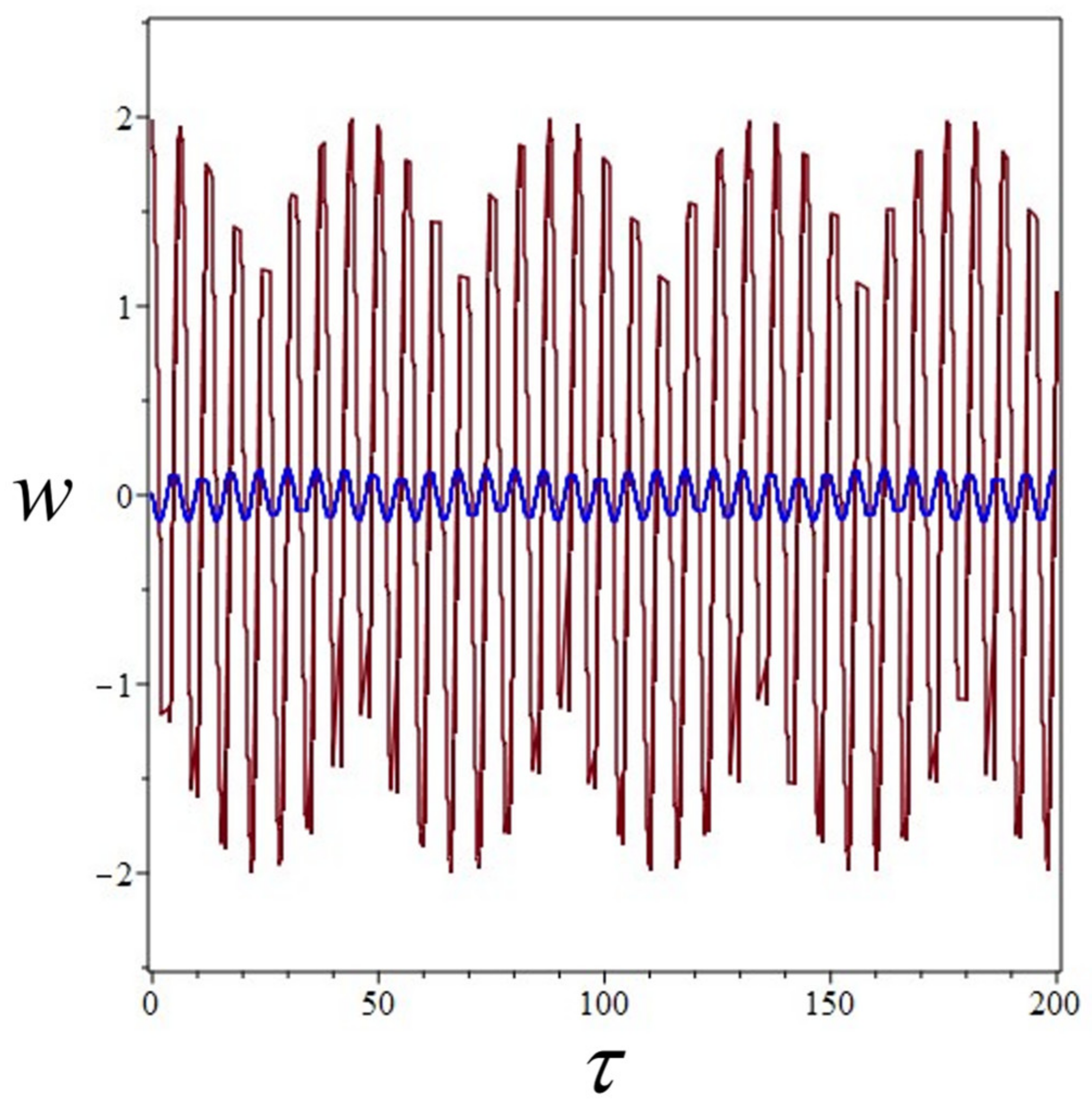
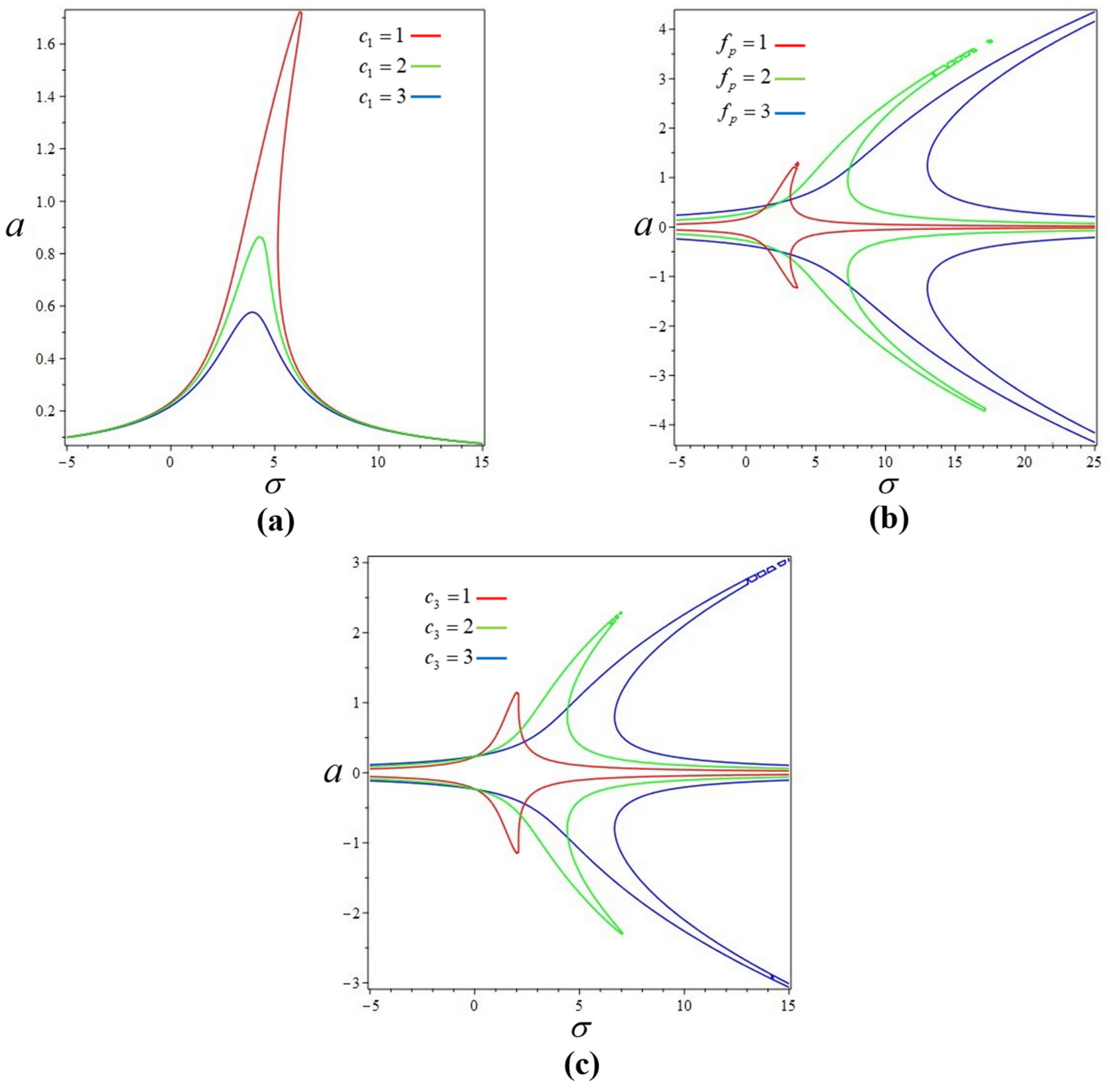

| 0.5 | 0.5 | 2.3562 | 1 | 0.8032 | −0.4133 |
| 0.5 | 0.5 | 2.3562 | 16 | 0.1418 | 1.5026 |
| 1.9950 | 0.0710 |
| 0.5 | 0.5 | 2.3562 | 1 | 0.1839 | 0.1099 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Y.; Liu, Y.; Qin, X.; Dou, Z.; Feng, Z.; Yang, G. Investigating Drillstring Vibration and Stability in Coring Drilling. Energies 2022, 15, 5234. https://doi.org/10.3390/en15145234
Sun Y, Liu Y, Qin X, Dou Z, Feng Z, Yang G. Investigating Drillstring Vibration and Stability in Coring Drilling. Energies. 2022; 15(14):5234. https://doi.org/10.3390/en15145234
Chicago/Turabian StyleSun, Yuchen, Yongsheng Liu, Xing Qin, Zijun Dou, Zibo Feng, and Gansheng Yang. 2022. "Investigating Drillstring Vibration and Stability in Coring Drilling" Energies 15, no. 14: 5234. https://doi.org/10.3390/en15145234
APA StyleSun, Y., Liu, Y., Qin, X., Dou, Z., Feng, Z., & Yang, G. (2022). Investigating Drillstring Vibration and Stability in Coring Drilling. Energies, 15(14), 5234. https://doi.org/10.3390/en15145234






