A High-Power-Density Active-Clamp Converter with Integrated Planar Transformer
Abstract
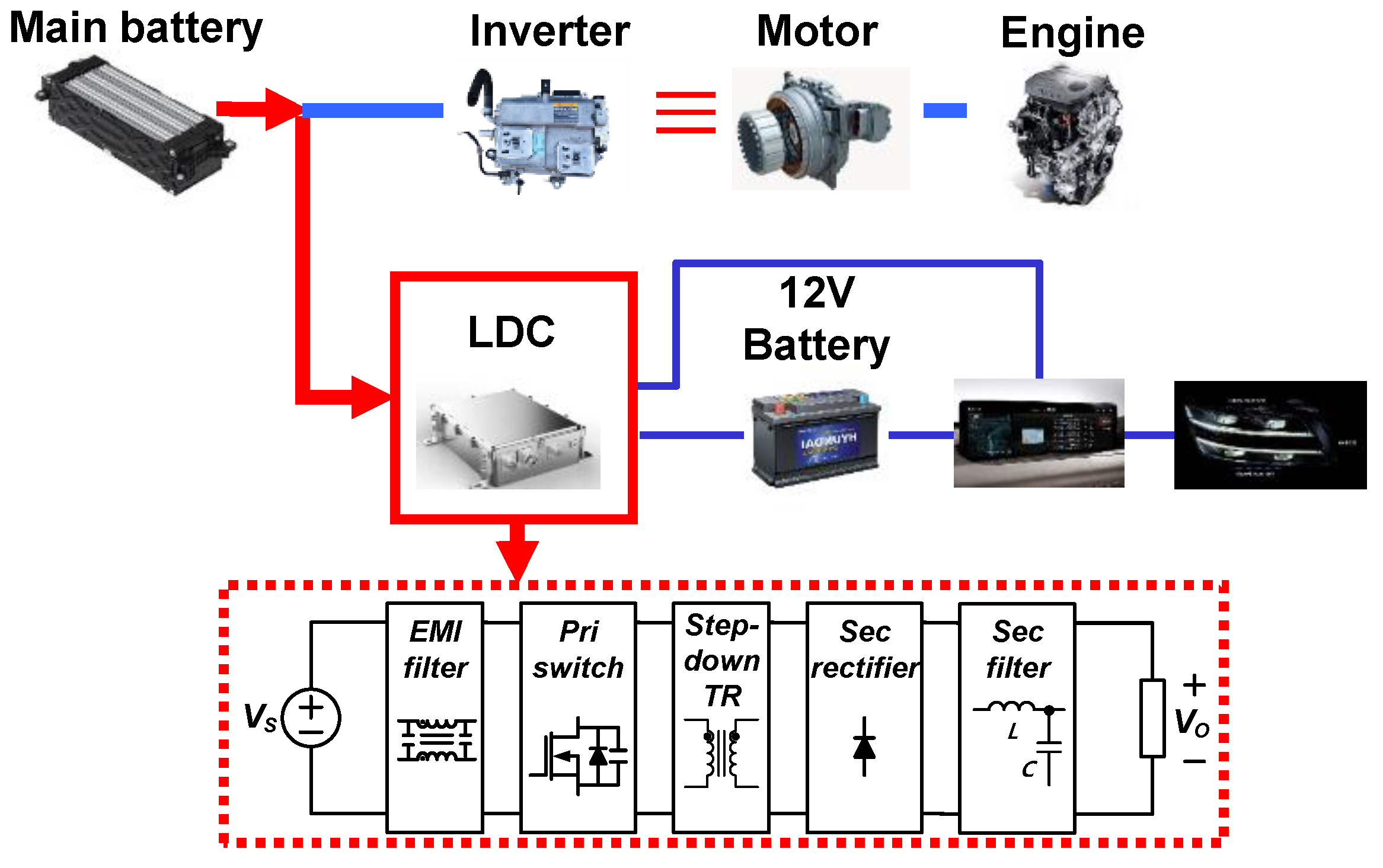
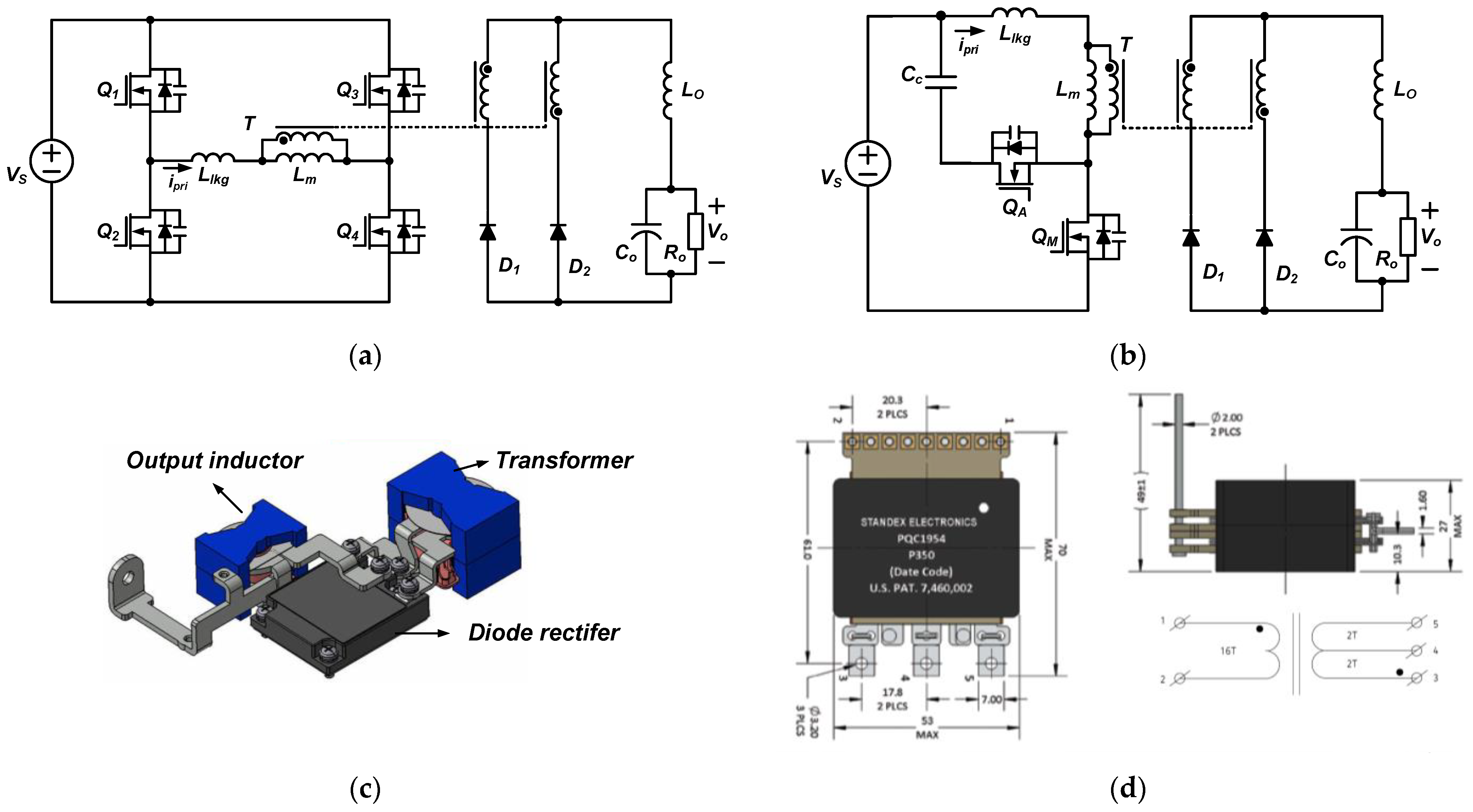
:1. Introduction
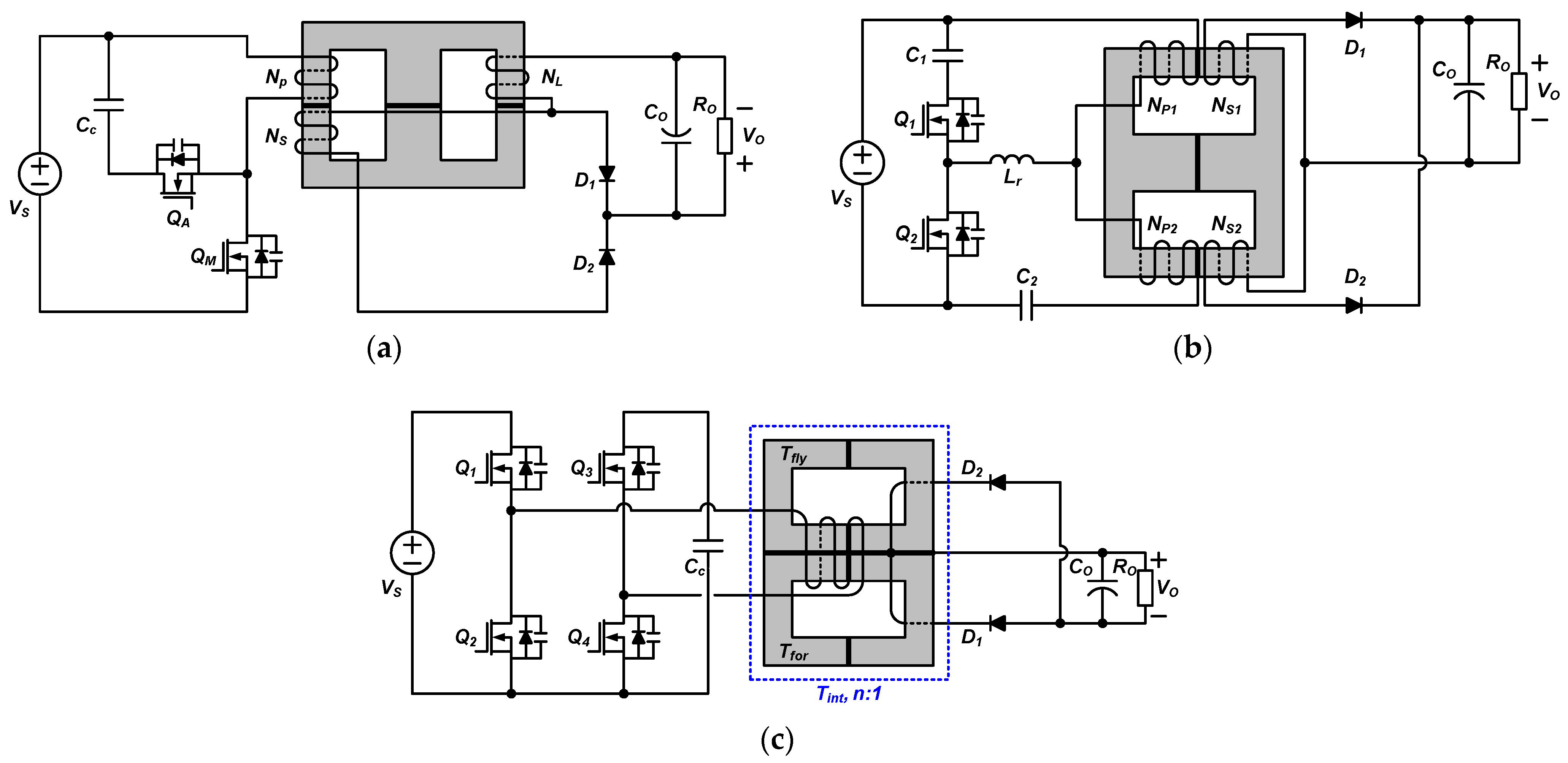
2. Features of the Proposed Converter
2.1. Descriptions of the Proposed Converter
- (1)
- The power density of the proposed converter is similar to that of high-frequency converters, which have over 500 kHz operating frequency.
- (2)
- Input/output voltage range characteristics of the proposed converter are similar to conventional PWM isolated converters with an output inductor.
- (3)
- The high price competitiveness and low cost can be achieved by eliminating the output inductor, reducing the winding configuration cost, and simplifying the structure of the rectifier structure.
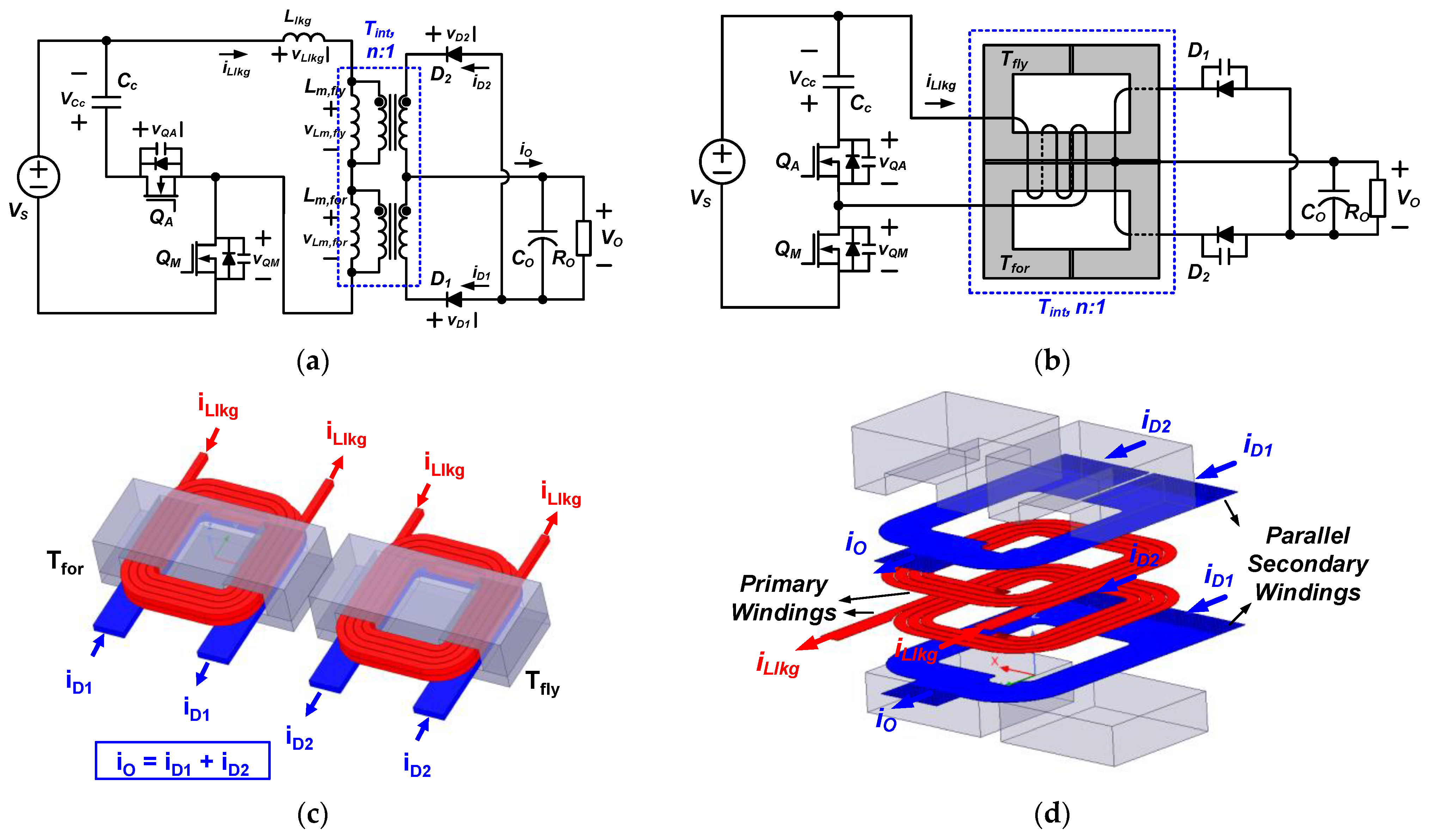
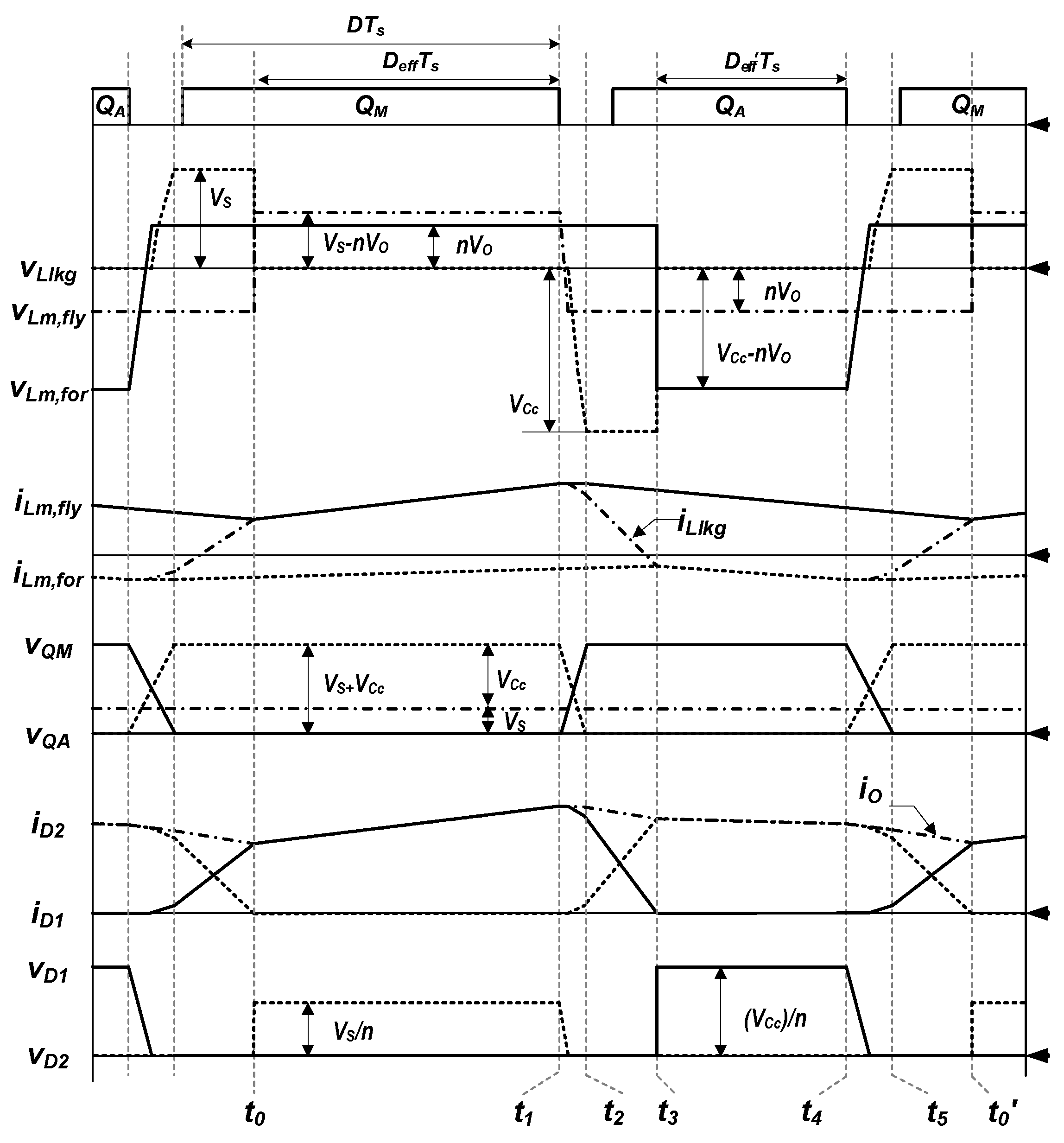
2.2. Operational Principles
- (1)
- All parasitic components are ignored except for parasitic components in Figure 4;
- (2)
- A clamp capacitor (CC) is large enough to be considered as a constant voltage source (VCc);
- (3)
- The output voltage (VO) is constant since the output is connected to the LV battery;
- (4)
- The transformer turns ratio (n) of the forward and flyback transformers (Tfor and Tfly) is N/1, where N is a number of the primary winding.
3. Analysis of Proposed Converter
3.1. DC Conversion Ratio
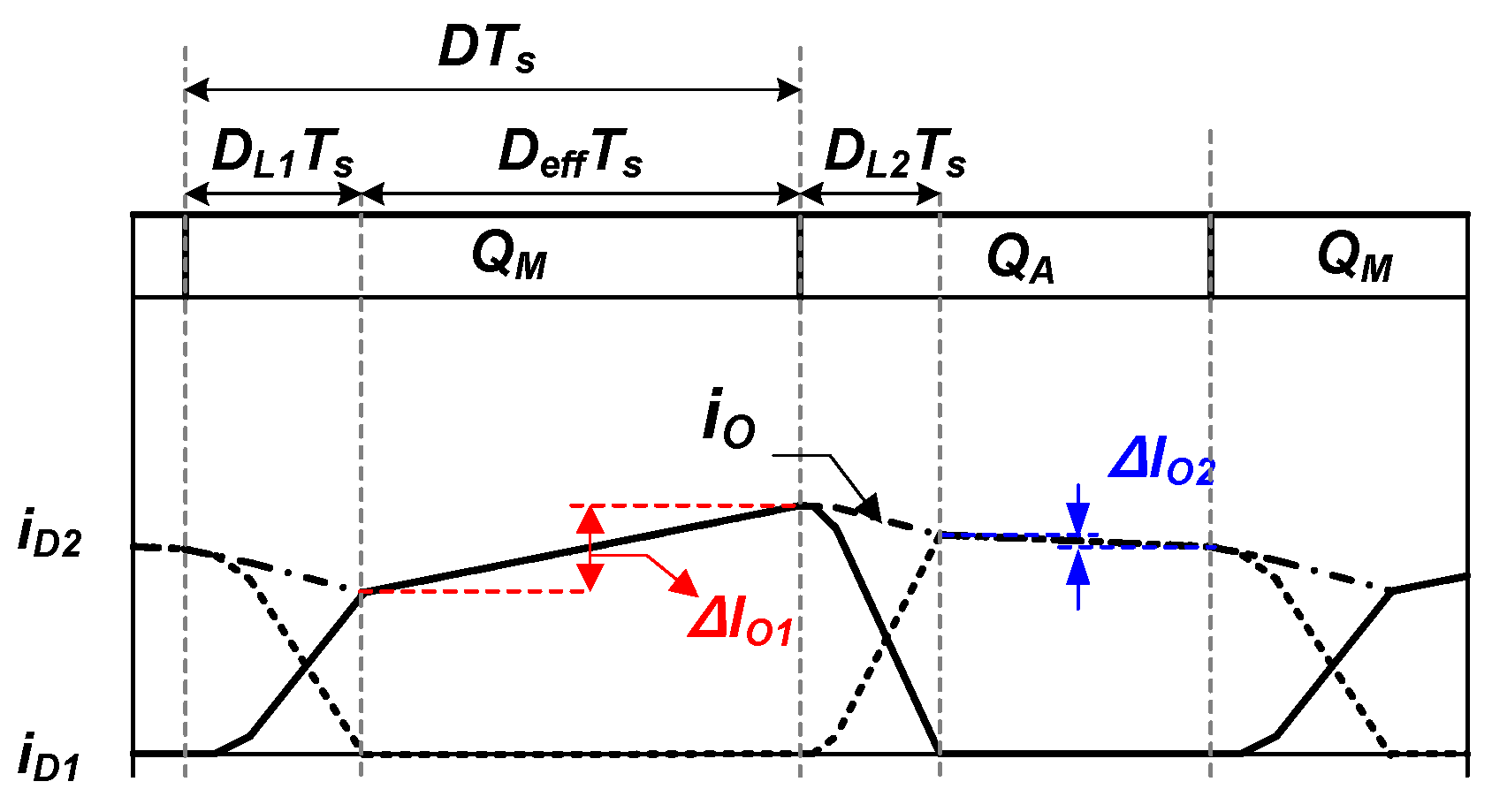
3.2. Design Consideration of Transformer
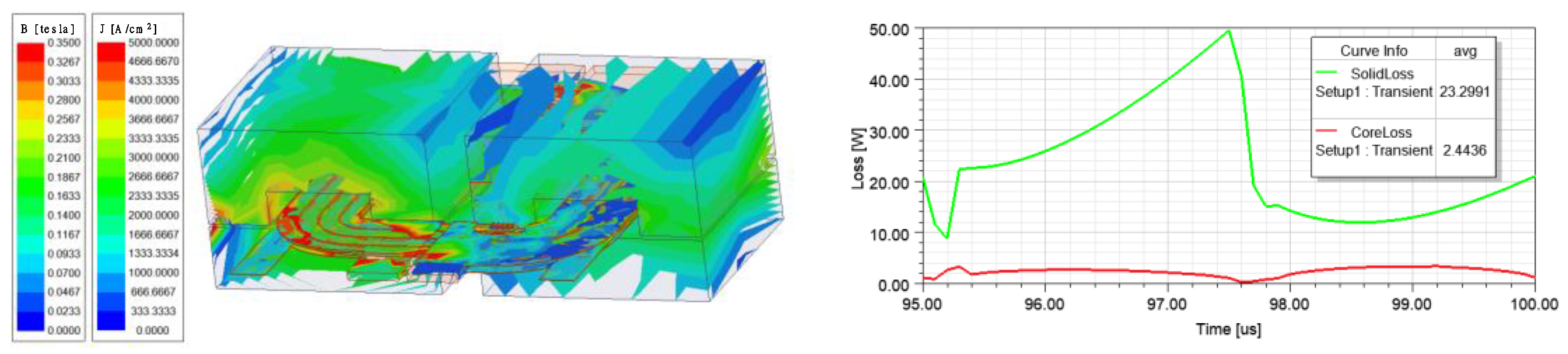
3.3. Loss Analysis of Proposed Converter
- (1)
- The magnetizing inductances of the transformer are large enough, so the magnetizing current ripples of the transformer are small enough to be ignorable.
- (2)
- The leakage inductance of the transformer is small enough to be negligible, and because of that, the commutation periods can be ignorable.
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Greenhouse Gas Emissions from a Typical Passenger Vehicle. Available online: https://www.epa.gov/greenvehicles/greenhouse-gas-emissions-typical-passenger-vehicle (accessed on 28 June 2022).
- Europe’s CO2 Emission Performance Standards for New Passenger Cars: Lessons from 2020 and Future Prospects. Available online: https://theicct.org/publication/europes-co2-emission-per-formance-standards-for-new-passenger-cars-lessons-from-2020-and-future-prospects (accessed on 28 June 2022).
- Global EV Outlook 2021. Available online: https://www.iea.org/reports/global-ev-outlook-2022 (accessed on 28 June 2022).
- Evolution of Vehicle Architecture. Available online: https://www.aptiv.com/newsroom/article/evolution-of-vehicle-architecture (accessed on 28 June 2022).
- Emadi, A.; Lee, Y.J.; Rajashekara, K. Power Electronics and Motor Drives in Electric, Hybrid Electric, and Plug-In Hybrid Electric Vehicles. IEEE Trans. Ind. Electron. 2008, 55, 2237–2245. [Google Scholar] [CrossRef]
- Noh, Y.S.; Hyon, B.J.; Park, J.S.; Kim, J.H.; Choi, J.H. Development of Low Voltage DC/DC Converter for Electric Vehicle using SiC MOSFET. In Proceedings of the 2019 International Symposium on Electrical and Electronics Engineering (ISEE), Ho Chi Minh City, Vietnam, 10–12 October 2019; pp. 237–240. [Google Scholar]
- Gu, B.; Lin, C.Y.; Chen, B.; Dominic, J.; Lai, J.S. Zero-Voltage-Switching PWM Resonant Full-Bridge Converter With Minimized Circulating Losses and Minimal Voltage Stresses of Bridge Rectifiers for Electric Vehicle Battery Chargers. IEEE Trans. Power Electron. 2013, 28, 4657–4667. [Google Scholar] [CrossRef]
- Kim, Y.D.; Lee, I.O.; Cho, I.H.; Moon, G.W. Hybrid Dual Full-Bridge DC–DC Converter With Reduced Circulating Current, Output Filter, and Conduction Loss of Rectifier Stage for RF Power Generator Application. IEEE Trans. Power Electron. 2014, 29, 1069–1081. [Google Scholar]
- Safaee, A.; Jain, P.; Bakhshai, A. A ZVS Pulsewidth Modulation Full-Bridge Converter with a Low-RMS-Current Resonant Auxiliary Circuit. IEEE Trans. Power Electron. 2016, 31, 4031–4047. [Google Scholar] [CrossRef]
- Zhao, L.; Li, H.; Wu, X.; Zhang, J. An Improved Phase-Shifted Full-Bridge Converter with Wide-Range ZVS and Reduced Filter Requirement. IEEE Trans. Ind. Electron. 2018, 65, 2167–2176. [Google Scholar] [CrossRef]
- Han, J.; Moon, G. High-Efficiency Phase-Shifted Full-Bridge Converter with a New Coupled Inductor Rectifier (CIR). IEEE Trans. Power Electron. 2019, 34, 8468–8480. [Google Scholar] [CrossRef]
- Park, K.; Kim, C.; Moon, G.; Youn, M. Three-Switch Active-Clamp Forward Converter with Low Switch Voltage Stress and Wide ZVS Range for High-Input-Voltage Applications. IEEE Trans. Power Electron. 2010, 25, 889–898. [Google Scholar] [CrossRef]
- Kim, C.; Baek, J.; Lee, J. Improved Three Switch-Active Clamp Forward Converter with Low Switching and Conduction Losses. IEEE Trans. Power Electron. 2019, 34, 5209–5216. [Google Scholar] [CrossRef]
- Li, W.; Li, P.; Yang, H.; He, X. Three-Level Forward–Flyback Phase-Shift ZVS Converter with Integrated Series-Connected Coupled Inductors. IEEE Trans. Power Electron. 2012, 27, 2846–2856. [Google Scholar] [CrossRef]
- Moon, S.; Park, K.; Moon, G.; Youn, M. Analysis and design of active clamp forward DC/DC converter with asymmetric two transformers. In Proceedings of the 8th International Conference on Power Electronics—ECCE Asia, Jeju, Korea, 30 May–3 June 2011; pp. 599–606. [Google Scholar]
- Lee, J.; Park, J.; Jeon, J.H. Series-Connected Forward–Flyback Converter for High Step-Up Power Conversion. IEEE Trans. Power Electron. 2011, 26, 3629–3641. [Google Scholar] [CrossRef]
- Lee, J.H.; Park, J.H.; Jeon, J.H. Series connected forward-flyback converter for high step-up power conversion. In Proceedings of the 8th International Conference on Power Electronics—ECCE Asia, Jeju, Korea, 30 May–3 June 2011; pp. 3037–3040. [Google Scholar]
- Chen, Q.; Feng, Y.; Zhou, L.; Wang, J.; Ruan, X. An improved Active Clamp Forward Converter with Integrated Magnetics. In Proceedings of the IEEE Power Electronics Specialists Conference, Orlando, FL, USA, 17–21 June 2007; pp. 377–382. [Google Scholar]
- Lin, J.Y.; Lo, Y.K.; Chiu, H.J.; Wang, C.F.; Lin, C.Y. An active-clamping ZVS flyback converter with integrated transformer. In Proceedings of the 2014 International Power Electronics Conference (IPEC-Hiroshima 2014—ECCE ASIA), Hiroshima, Japan, 18–21 May 2014; pp. 172–176. [Google Scholar]
- Baek, J.-I.; Youn, H.-S. Full-Bridge Active-Clamp Forward-Flyback Converter with an Integrated Transformer for High-Performance and Low Cost Low-Voltage DC Converter of Vehicle Applications. Energies 2020, 13, 863. [Google Scholar] [CrossRef] [Green Version]
- Planar Transformers for EV On-Board Chargers & DC-DC Converter Applications. Available online: https://standexelectronics.com/planar-magnetics/planar-transformers-for-ev-on-board-chargers-dc-dc-converter-applications/ (accessed on 14 July 2022).
- Naradhipa, A.M.; Kim, S.; Yang, D.; Choi, S.; Yeo, I.; Lee, Y. Power Density Optimization of 700 kHz GaN-Based Auxiliary Power Module for Electric Vehicles. IEEE Trans. Power Electron. 2022, 36, 5610–5621. [Google Scholar] [CrossRef]
- Kim, K.-W.; Jeong, Y.; Kim, J.-S.; Moon, G.-W. Low Common-Mode Noise LLC Resonant Converter With Static-Point-Connected Transformer. IEEE Trans. Power Electron. 2021, 36, 401–408. [Google Scholar] [CrossRef]
- Reinert, J.; Brockmeyer, A.; De Doncker, R.W. Calculation of losses in ferro- and ferrimagnetic materials based on the modified Steinmetz equation. IEEE Trans. Ind. Appl. 2001, 37, 1055–1061. [Google Scholar] [CrossRef]


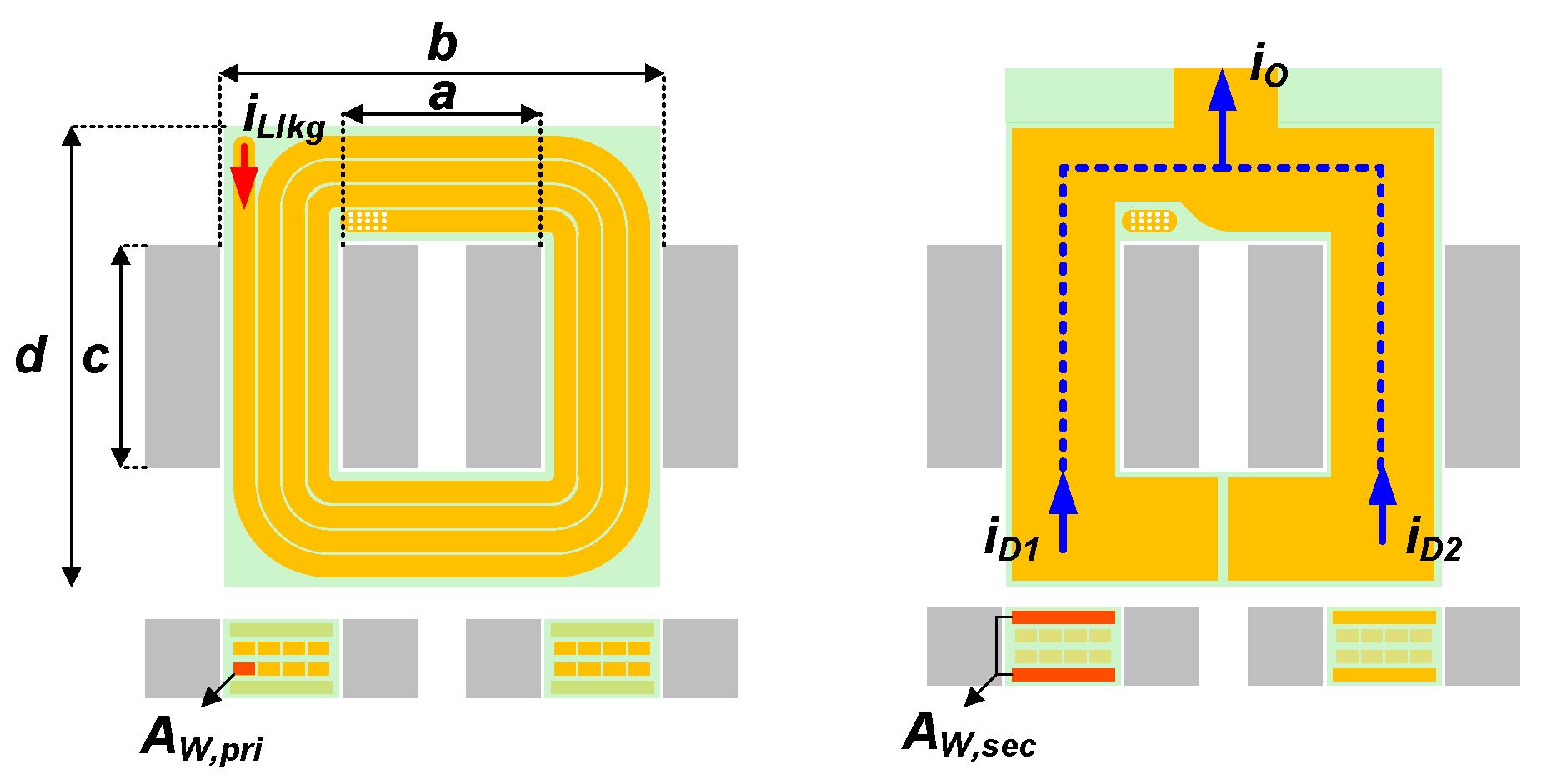


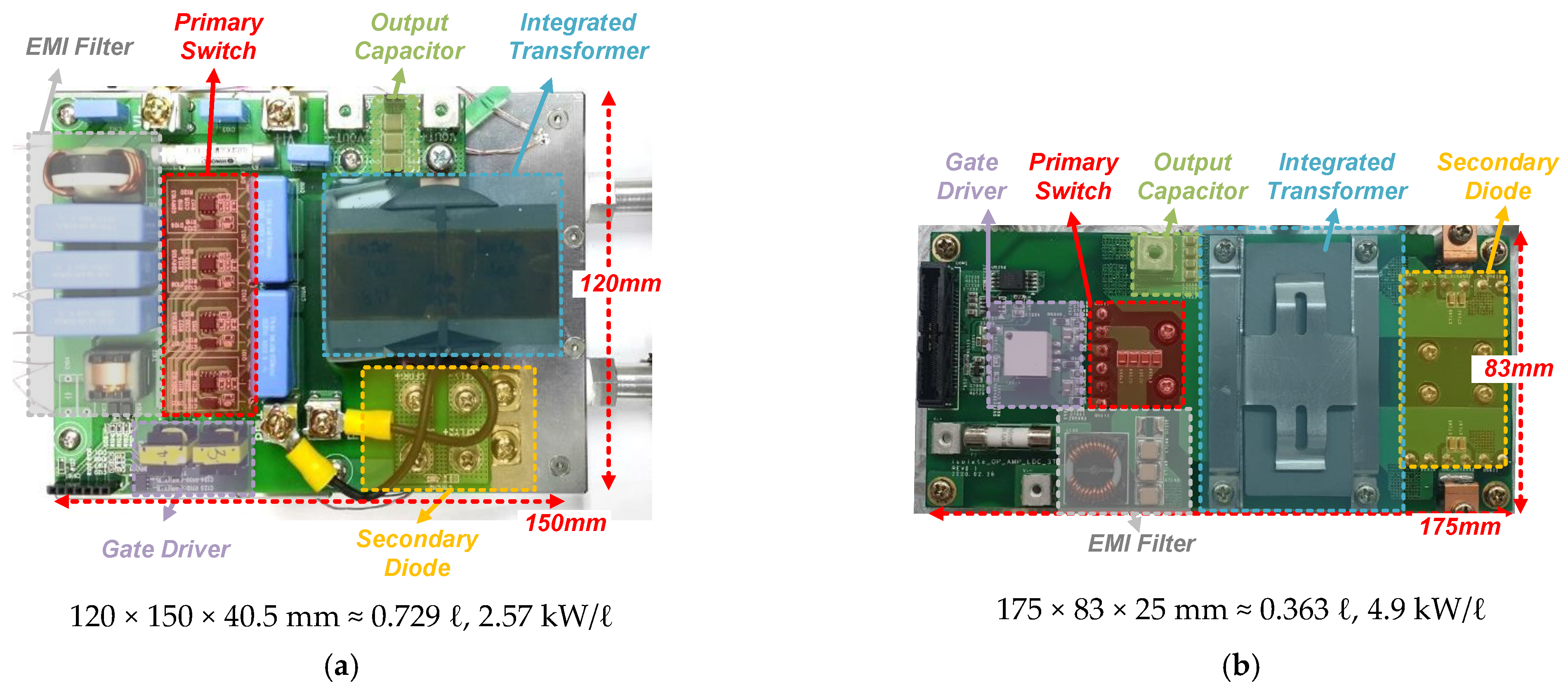


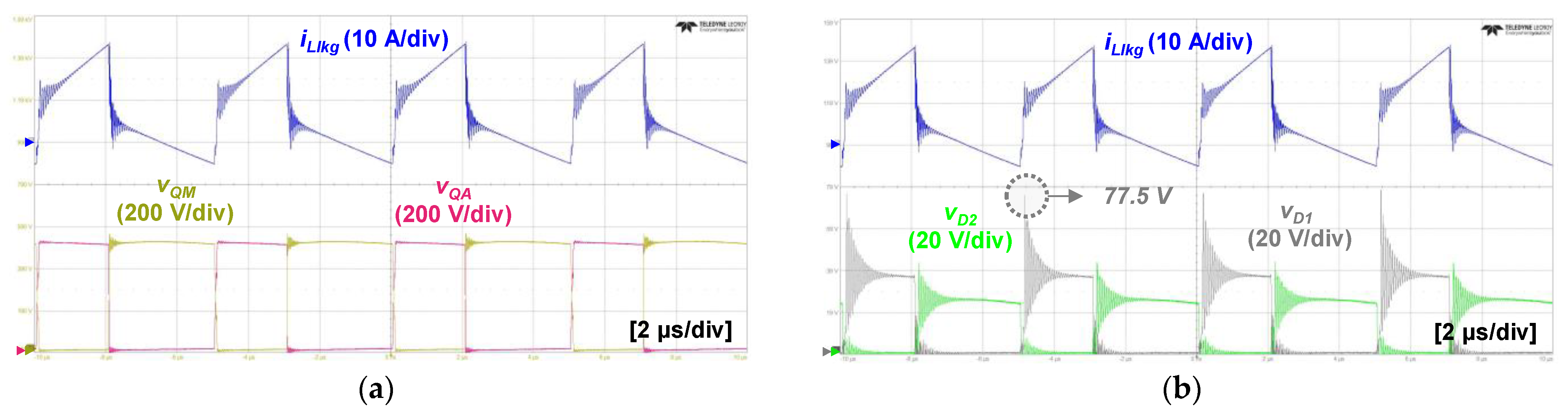
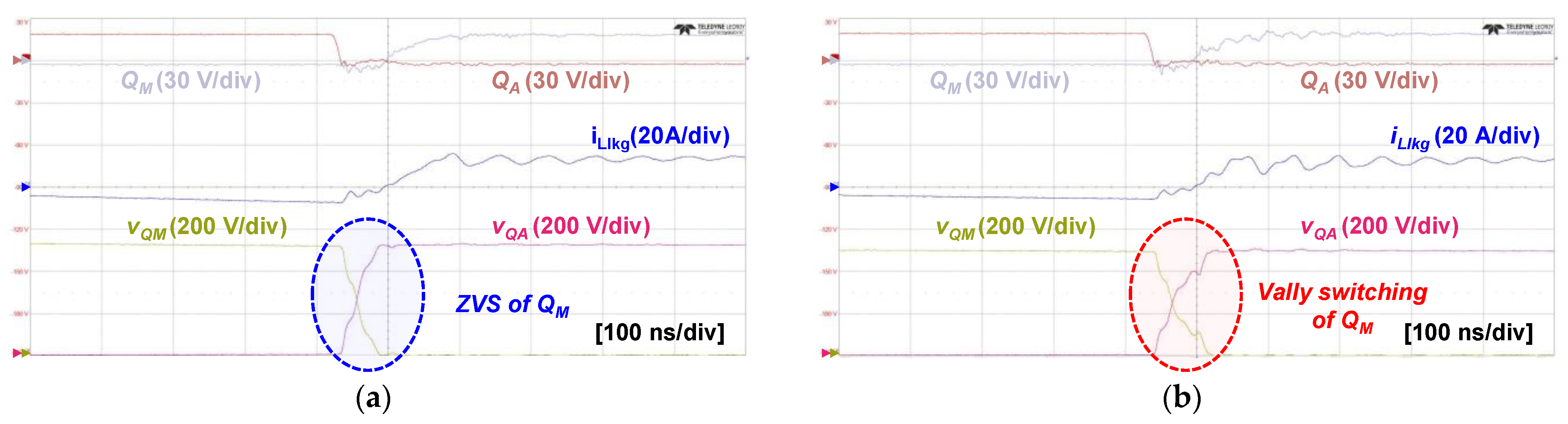
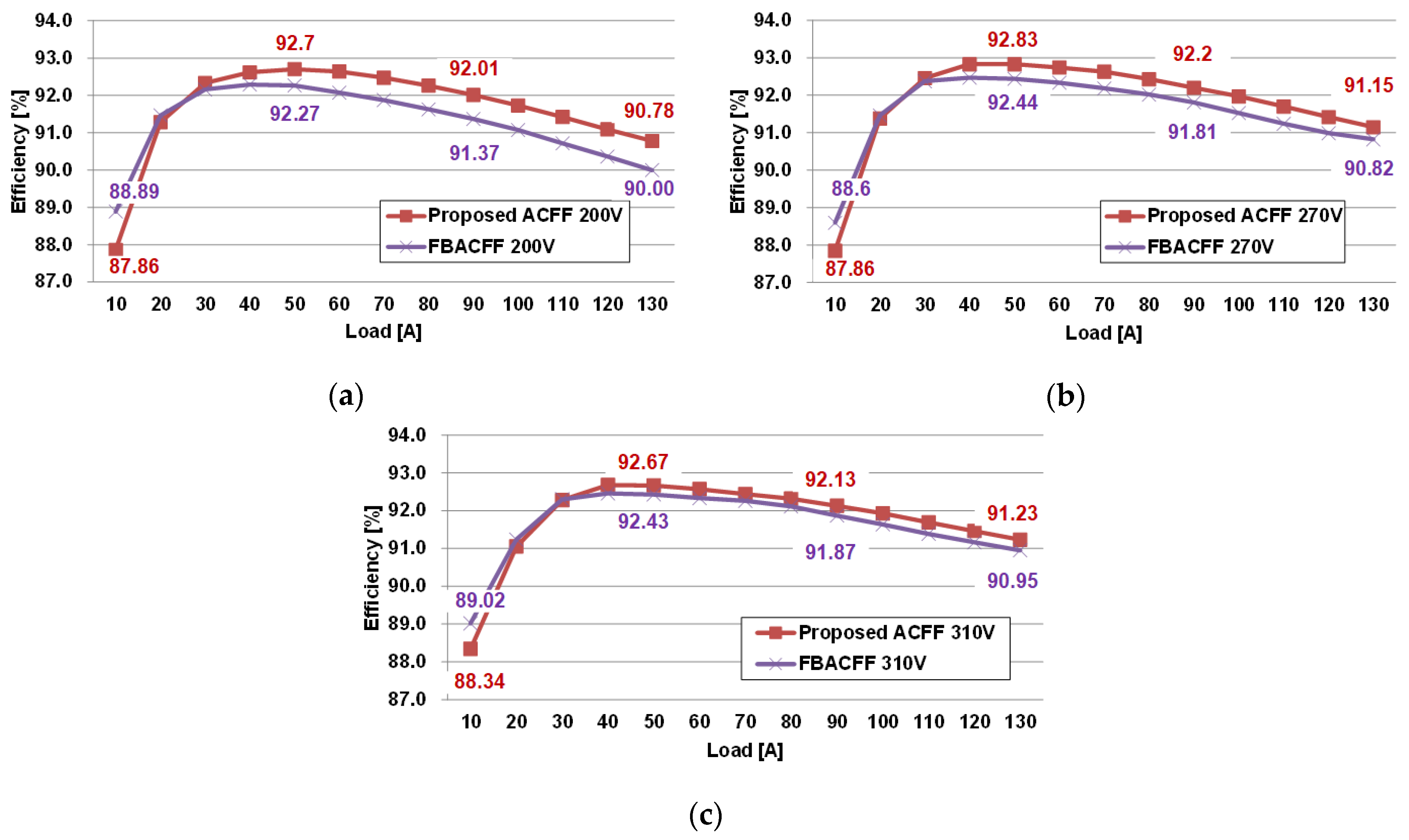
| Parameter | Conventional Integrated Transformer | Proposed Integrated Planar Transformer |
|---|---|---|
| V/VO, PO | 270 V/13.9 V, 1.8 kW | |
| Switching frequency | 150 kHz | 200 kHz |
| Core | PQ6640 custom | UI3323 × 2EA |
| Flux variation (ΔBfor/ΔBfly) | 0.16 T | 0.157 T/0.143 T |
| Core volume | 71,396 mm4 | 42,268 mm4 |
| Primary windings area/current density (A/cm2) | 0.1Φ 250 strands/559 A/cm2 | 0.105 mm × 2.6 mm/4046 A/cm2 |
| Resistance of primary winding | 0.014 Ω | 0.1 Ω |
| Secondary winding area/current density (A/cm2) | 2 mm × 10 mm/456 A/cm2 | 0.105 mm × 11.3 mm × 2/3737 A/cm2 |
| Resistance of primary winding | 0.1 mΩ | 0.6 mΩ |
| Magnetizing inductance (Lm,for/Lm,fly) | 50 μH/40 μH | 30 μH/30 μH |
| Leakage inductance | 5 μH | 2 μH |
| Core loss | 4.28 W | 2.66 W |
| Primary winding loss | 1.34 W | 13.76 W |
| Secondary winding loss | 3.27 W | 13.33 W |
| Total loss of transformer | 8.89 W | 29.75 W |
| Parameter | PSFB | FBACFF | Proposed ACFF |
|---|---|---|---|
| RMS currents on switches (A) | , Q2, Q3 : – | , QA : – | |
| Voltage stress on switches (V) | |||
| Voltage stresses on diodes (V) |
| Parameter | PSFB | FBACFF | Proposed ACFF |
|---|---|---|---|
| Primary switch Part number/Price ($) | Q1–Q4: IPW65R115/4.636 | Q1, Q4: IPW65R115/4.636, Q2, Q3: IPW65R190/2.69 | QM: C3M0120090/7.47 QA: C3M0280090/4.03 |
| Total price of primary switches ($) | 18.544 | 14.652 | 11.5 |
| Transformer/inductor | EER4730 /EER6025 | EE6630 | UI3323 × 2 EA |
| Price of magnetics($) | 20 | 12 | 5 |
| Total Price ($) | 38.544 | 26.625 | 16.5 |
| Components | Conventional FBACFF with Integrated Transformer | Proposed ACF with Integrated Planar Transformer |
|---|---|---|
| Primary switch | Q1, Q4: IPW60R080 Q2, Q3: IPW60R190 | QM: C3M0120090 QA: C3M0280090 |
| Secondary diode | M80QZ12N, 2 EA | VS-63CPQ100, 4 EA |
| Clamp cap | Film: 630 V, 1 uF | MLCC: 150 nF, 4 EA |
| Transformer | Core: PQ6640, Lm,for = 50 μH, Lm,fly = 40 μH Llkg = 5 μH, Np:Ns = 8:1 | Core: UI3323, 2 EA, Lm,for = Lm,fly = 30 μH, Llkg = 2 μH, Np:Ns = 8:1 |
| Output cap | MLCC: 22 μF × 6 EA | MLCC: 10 μF, 8 EA |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, D.-W.; Lim, J.-H.; Lee, D.-I.; Youn, H.-S. A High-Power-Density Active-Clamp Converter with Integrated Planar Transformer. Energies 2022, 15, 5609. https://doi.org/10.3390/en15155609
Lee D-W, Lim J-H, Lee D-I, Youn H-S. A High-Power-Density Active-Clamp Converter with Integrated Planar Transformer. Energies. 2022; 15(15):5609. https://doi.org/10.3390/en15155609
Chicago/Turabian StyleLee, Dae-Woo, Ji-Hoon Lim, Dong-In Lee, and Han-Shin Youn. 2022. "A High-Power-Density Active-Clamp Converter with Integrated Planar Transformer" Energies 15, no. 15: 5609. https://doi.org/10.3390/en15155609
APA StyleLee, D.-W., Lim, J.-H., Lee, D.-I., & Youn, H.-S. (2022). A High-Power-Density Active-Clamp Converter with Integrated Planar Transformer. Energies, 15(15), 5609. https://doi.org/10.3390/en15155609






