Adsorption Solar Air Conditioning System for Singapore Climate
Abstract
:1. Background and Introduction
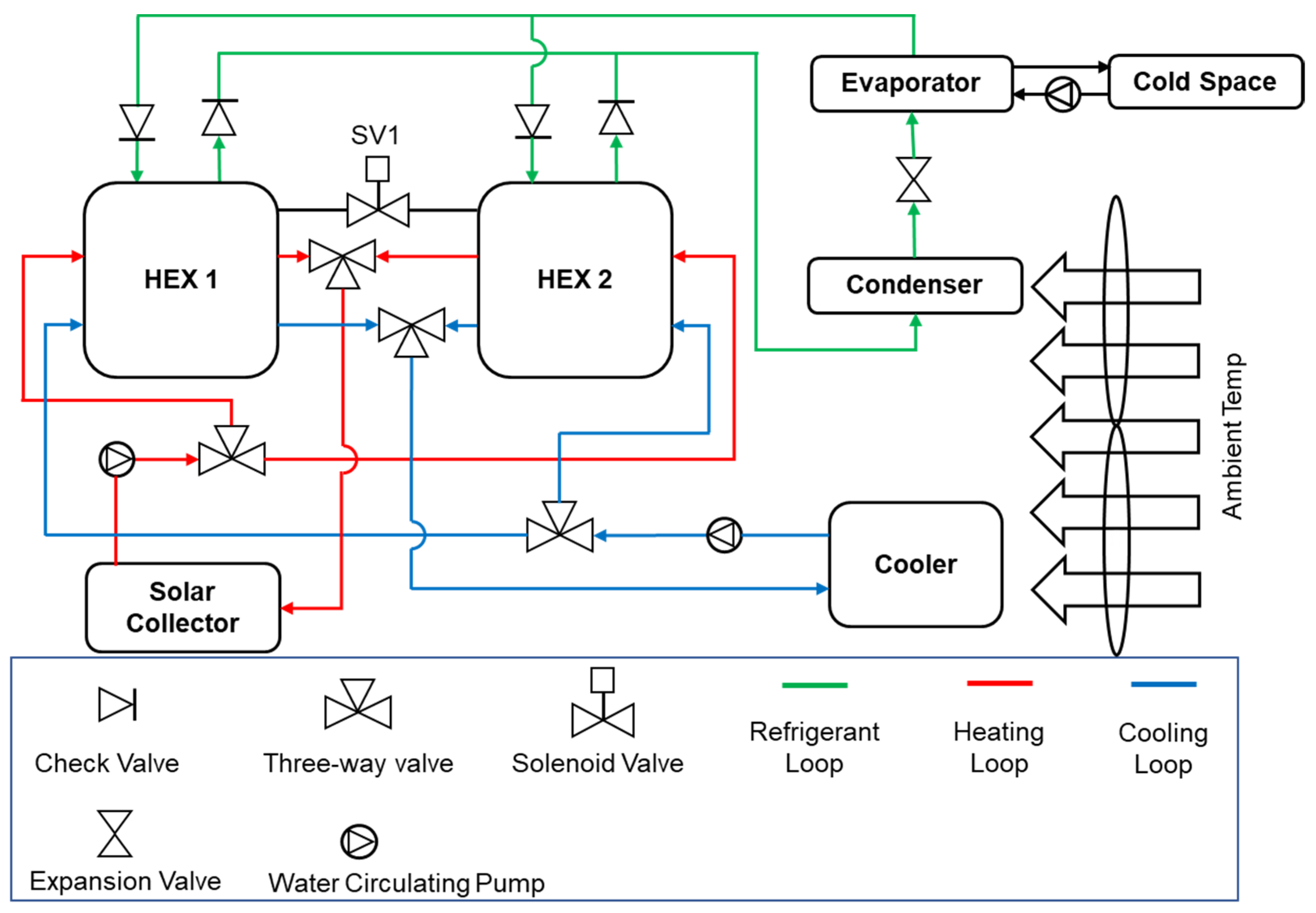
2. Adsorption Air Conditioning Model
2.1. Thermal Compressor
2.2. Evaporator
2.3. System Coefficient of Performance (COP)
2.4. Solar Collector Integration
3. Simulation Operating Conditions
3.1. Solar Operating Conditions
3.2. Air Conditioning Operating Temperatures
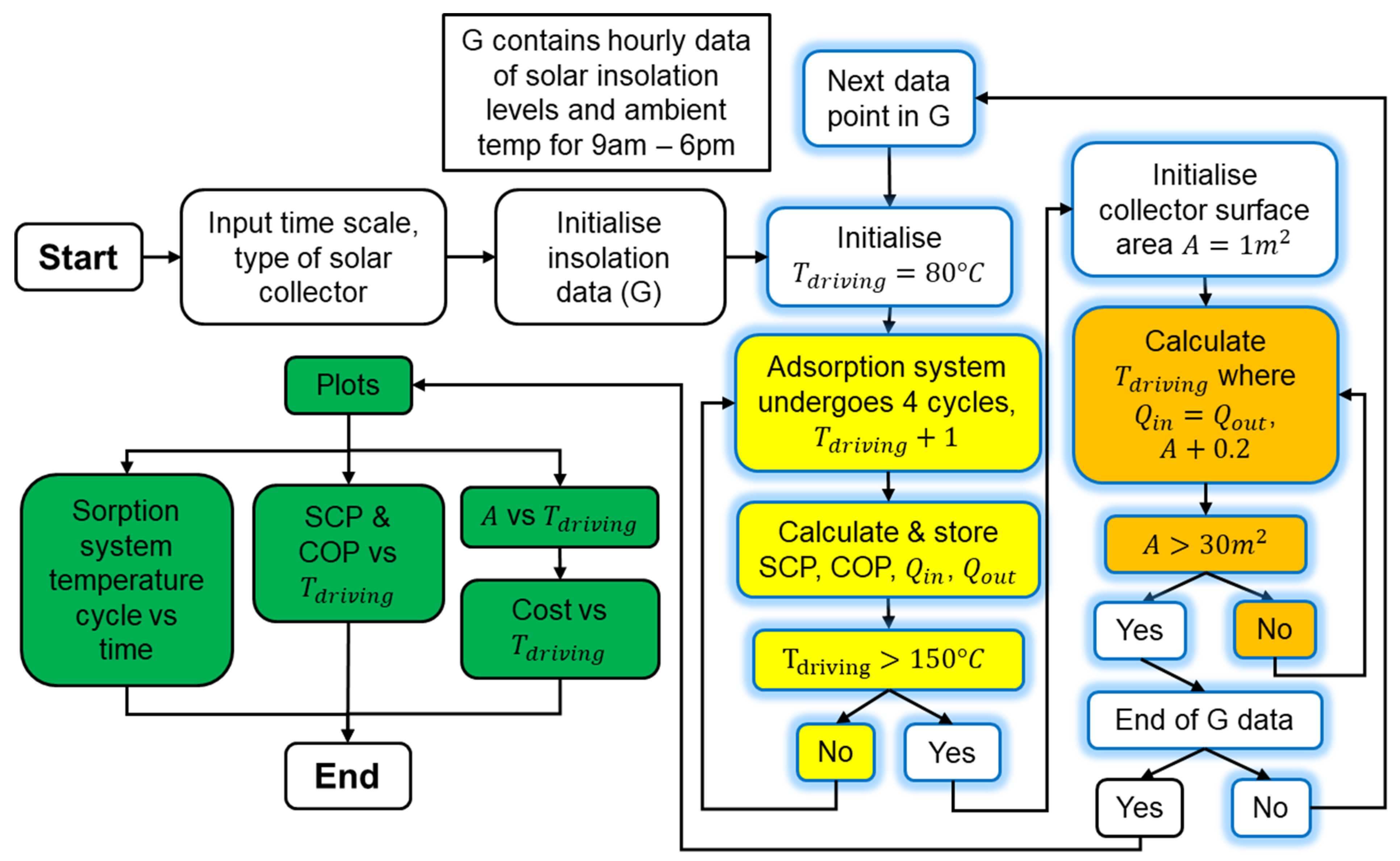
4. Results Analysis and Discussion
5. Conclusions
- A physical description of the adsorption reactor (thermal compressor), which is a shell (154.4 mm OD × 1.63 mm wall thickness in stainless steel), and the micro-tube (800 micro-tubes of 1.2 mm OD × 2 mm wall thickness in stainless steel with about 2 mm layer of activated carbon and methanol refrigerant) type of heat exchanger.
- An elaboration of finite difference modelling of the adsorption reactor based on both mass and heat balances leading to the establishment of the intrinsic performance of the thermal compressor, namely, the specific cooling power (SCP) and coefficient of performance (COP) as a function of the driving temperature.
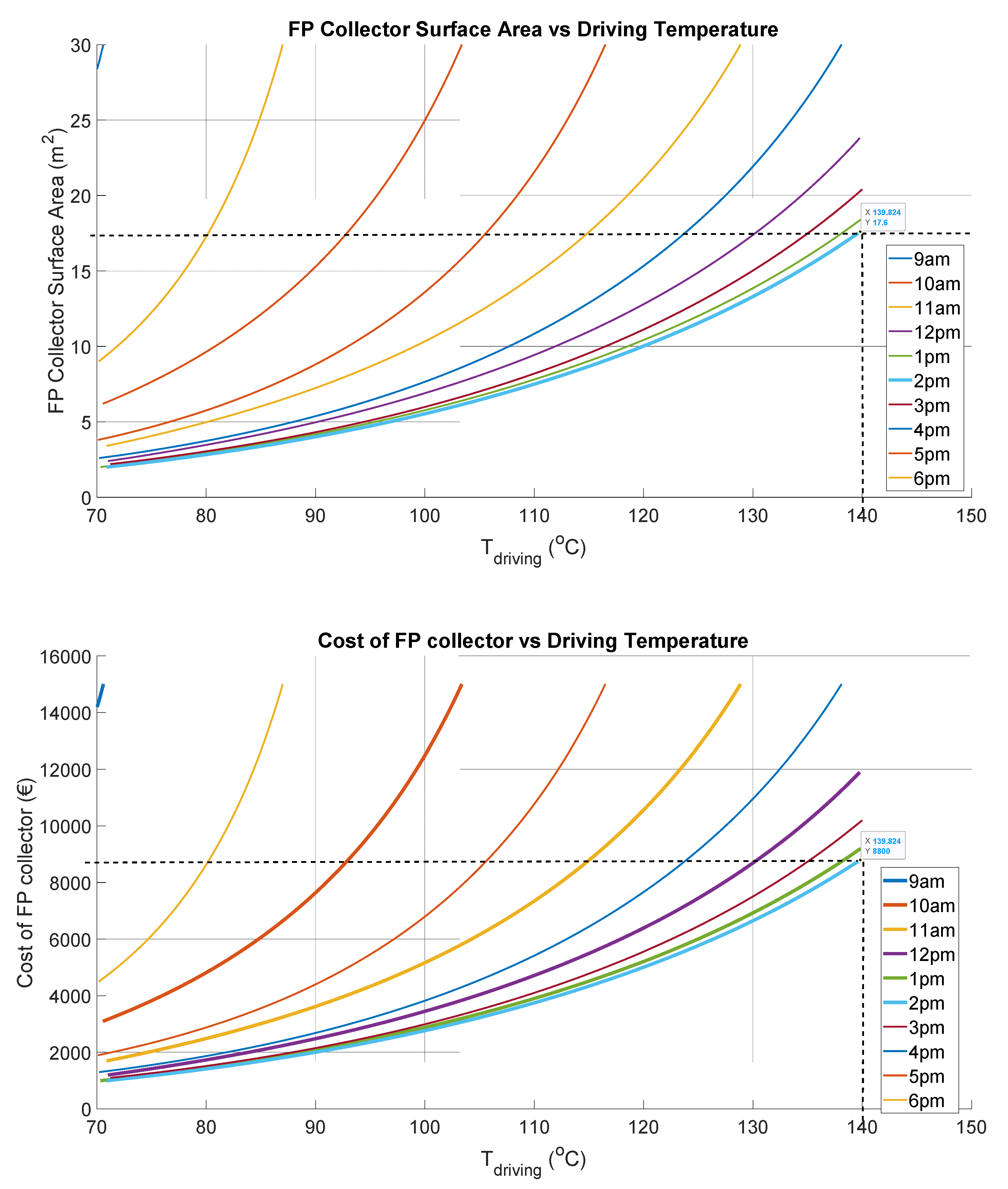
- Thermal solar collector area and cost mapping for Singapore hourly weather data on the hottest day of the year (5 April), leading to sizing and costing of the required solar collector.
- A comparison of both the flat plate solar collector (FPSC) and the evacuated tube solar collector (ETSC), leading to ETSC being identified as the most cost coeffective (19% cheaper) option with 9.8 m2 area vs. 17.6 m2 to achieve similar nominal cooling capacity (2.6 kW).
- An exploitation of the elaborated model with a 9.8 m2 ETSC to predict the hourly, daily and annual performance of air conditioning.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Acronyms | |
| ANSI | American National Standards Institute |
| ASHRAE | American Society of Heating, Refrigerating and Air-Conditioning Engineers |
| COP | Coefficient of Performance |
| D–A | Dubinin–Astakhov |
| ETSC | Evacuated Tube Solar collector |
| FPSC | Flat Plate Solar collector |
| HEX | Heat Exchanger |
| HTF | Heat Transfer Fluid |
| ID | Inner Diameter (m or mm) |
| kWc | Kilowatt Cooling |
| LPM | Liter per minute |
| MT | Micro-Tube or Miniaturized Tube |
| OD | Outer Diameter (m or mm) |
| SCP | Specific Cooling Power (W kg−1 or kW kg−1) |
| S$ | Singapore Dollar currency |
| SS | Stainless Steel |
| SV | Solenoid Valve |
| TREX | Tubular Reactor Heat Exchanger |
| WHO | World Health Organization |
| Latin Symbols | |
| A | Area (m2) |
| c | Specific heat capacity (J kg−1 K−1) |
| D | Diameter (m) |
| G | Solar Insolation (W m−2) |
| h | Convection heat transfer coefficient (W m−2 K−1) |
| h | Specific enthalpy of refrigerant (J kg−1) |
| H | Specific enthalpy of sorption (J kg−1) |
| k1 | Linear loss coefficient (W m−2 K−1) |
| k2 | Quadratic loss coefficient (W m−2 K−2) |
| k | D–A parameter |
| KPI | Key Performance Indicator |
| L | Length (m) |
| m | Mass (kg) |
| n | D–A parameter |
| Nu | Nusselt Number |
| q | Heat rate (W) |
| Q | Heat energy (J) |
| Re | Reynolds Number |
| th | Thickness (m or mm) |
| T | Temperature ( or K) |
| UA | Overall Heat Transfer Coefficient (W K−1) |
| v | Velocity (m s−1) |
| Volumetric flow rate (m−3 s−1) | |
| x | Refrigerant uptake (kg Methanol kg−1 Carbon) |
| xo | Maximum Refrigerant uptake (kg Methanol kg−1 Carbon) |
| Greek symbols | |
| ∆ | Difference |
| € | Euro currency |
| η | Efficiency of solar collector |
| λ | Thermal conductivity (W m−1 K−1) |
| µ | Dynamic viscosity (kg m−1 s−1) |
| ρ | Density (kg m−3) |
| Subscripts | |
| amb | Ambient |
| c | Carbon |
| con | Condensation or Condenser |
| col | Solar collector |
| cool | Cooling |
| driving | Driving temperature of adsorption system |
| evap | Evaporation or Evaporator |
| f | Fluid in liquid form |
| HP | High Pressure |
| i | Inner |
| in | Into HEX |
| m | Methanol |
| LP | Low Pressure |
| o | Outer |
| opt | Optimum |
| out | Out of solar collector |
| p | Pressure |
| sat | Saturation |
| sup | Superheated vapor |
| t | Tube or Micro-Tube |
| v | Volume |
| w | Stainless Steel wall between carbon and HTF |
References
- Asia, C.N. Air Conditioning for All? Hotter World Faces Risk of ‘Cooling Poverty’. Available online: https://www.channelnewsasia.com/world/climate-change-air-conditioning-hotter-world-risk-8603062019 (accessed on 13 September 2019).
- Singapore, E.M.A. Singapore Energy Statistics 2018. Available online: https://www.ema.gov.sg/Singapore_Energy_Statistics.aspx (accessed on 13 September 2019).
- Chua, K.J.; Chou, K.S.; Yang, W.M.; Yan, J. Achieving better energy-efficient air conditioning—A review of technologies and strategies. Appl. Energy 2013, 104, 87–104. [Google Scholar] [CrossRef]
- Electricity Tariff Revision for the Period of 1 July to September 2019. Available online: https://www.spgroup.com.sg/wcm/connect/spgrp/ (accessed on 4 August 2021).
- Smart ENVi Series, Daikin Split Air Conditioning Units, Catalogue 2019; Daikin: Osaka, Japan, 2019.
- Air-Con-System-Efficiency-Primer-A-Summary. Available online: https://www.nccs.gov.sg/files/docs/default-source/default-document-library/air-con-system-efficiency-primer-a-summary.pdf (accessed on 4 August 2021).
- Chapter 14 climatic design information. In ASHRAE-Handbook-Fundamentals; ASHRAE: Atlanta, GA, USA, 2019.
- Kalkan, N.; Young, E.; Celiktas, A. Solar thermal air conditioning technology reducing the footprint of solar thermal air conditioning. Renew. Sustain. Energy Rev. 2012, 16, 6352–6383. [Google Scholar] [CrossRef]
- Weiss, W.; Spörk-Dür, M. Global Market Development and Trends 2021: Detailed Market Figures 2022; Report—IEA Solar Heating & Cooling Programme; SHC: Cedar, MI, USA, 2022. [Google Scholar]
- Yande, N.N.G. Thermal Adsorption Air Conditioning: Case Study of Singapore; Project Report; Nanyang Technological University: Singapore; University of Warwick: Coventry, UK, 2019. [Google Scholar]
- Metcalf, S.J.; Tamainot-Telto, Z.; Critoph, R.E. Novel compact sorption generators for heat pump applications, paper no. P5-49. In Proceedings of the IEA—Heat Pump Conference, Zurich, Switzerland, 22 May 2008. [Google Scholar]
- Metcalf, S.; Tamainot-Telto, Z.; Critoph, R. Application of a compact sorption generator to solar refrigeration: Case study of Dakar (Senegal). Appl. Therm. Eng. 2011, 31, 2197–2204. [Google Scholar] [CrossRef]
- Metcalf, S.; Critoph, R.; Tamainot-Telto, Z. Optimal cycle selection in carbon-ammonia adsorption cycles. Int. J. Refrig. 2012, 35, 571–580. [Google Scholar] [CrossRef]
- WKays, M.; London, A.L. Compact Heat Exchangers, 3rd ed.; McGraw-Hill: New York, NY, USA, 1984; ISBN 0-07-033418-8. [Google Scholar]
- Holman, J. Heat Transfer, 10th ed.; McGraw-Hill: New York, NY, USA, 2010; ISBN 978-007-126769-4. [Google Scholar]
- Reuck, K.M.D.; Craven, R.J.B. Methanol; Blackwell Scientific: Oxford, UK, 1993; ISBN 9780632023790. [Google Scholar]
- Turner, L.H. Improvement of Activated Charcoal-Ammonia Adsorption Heat Pumping/Refrigeration Cycles: Investigation of Porosity and Heat/Mass Transfer Chacteristics. Ph.D. Thesis, University of Warwick, Coventry, UK, 1992. [Google Scholar]
- Critoph, R.E. Evaluation of alternative refrigerant—Adsorbent pairs for refrigeration cycles. Appl. Therm. Eng. 1996, 16, 891–900. [Google Scholar] [CrossRef]
- Critoph, R.E. Adsorption refrigerators and heat pumps. In Carbon Materials for Advanced Technologies; Burchell, T.D., Ed.; Elsevier: Amsterdam, The Netherlands, 1999; Chapter 10; pp. 303–340. ISBN 0-08-042683-2. [Google Scholar]
- SolarBayer. 2018. Available online: https://www.solarbayer.com/ (accessed on 17 August 2019).
- WHO. Health impact of low indoor temperatures. In Proceedings of the WHO Meeting, Copenhagen, Denmark, 11–14 November 1985. [Google Scholar]
- WHO Housing and Health Guidelines 2018; WHO: Geneva, Switzerland, 2018; ISBN 978-92-4-155037-6.
- ANSI/ASHRAE Standard 55-2017; Thermal Environmental Conditions for Human Occupancy. ASHRAE: Atlanta, GA, USA, 2017; ISSN 1041-2336.
- ANSI/ASHRAE Addendum d to ANSI/ASHRAE Standard 55-2017; Thermal Environmental Conditions for Human Occupancy. ASHRAE: Atlanta, GA, USA, 2020; ISSN 1041-2336.
- Wang, R.Z. Performance improvement of adsorption cooling by heat and mass recovery operation. Int. J. Refrig. 2001, 2, 602–611. [Google Scholar] [CrossRef]
- Critoph, R.E.; Metcalf, S.J. Specific cooling power intensification limits in ammonia–carbon adsorption refrigeration systems. Appl. Therm. Eng. 2004, 24, 661–678. [Google Scholar] [CrossRef]
- Habib, K.; Saha, B.B.; Koyama, S. Study of various adsorbent–refrigerant pairs for the application of solar driven adsorption cooling in tropical climates. Appl. Therm. Eng. 2014, 72, 266–274. [Google Scholar] [CrossRef]
- Henning, H.; Häberle, A.; Guerra, M.; Motta, M. Solar Cooling and Refrigeration with High Temperature Lifts—Thermodynamic Background and Technical Solution. Available online: https://www.researchgate.net/publication/268359467 (accessed on 30 July 2022).
- Renewables 2015 Global Statue Report, REN21. Available online: https://www.ren21.net/wp-content/uploads/2019/05/GSR2015_FullReport_English.pdf (accessed on 4 August 2021).
- Roumpedakis, T.C.; Vasta, S.; Sapienza, A.; Kallis, G.; Karellas, S.; Wittstadt, U.; Tanne, M.; Harborth, N.; Sonnenfeld, U. Performance Results of a Solar Adsorption Cooling and Heating Unit. Energies 2020, 13, 1630. [Google Scholar] [CrossRef] [Green Version]
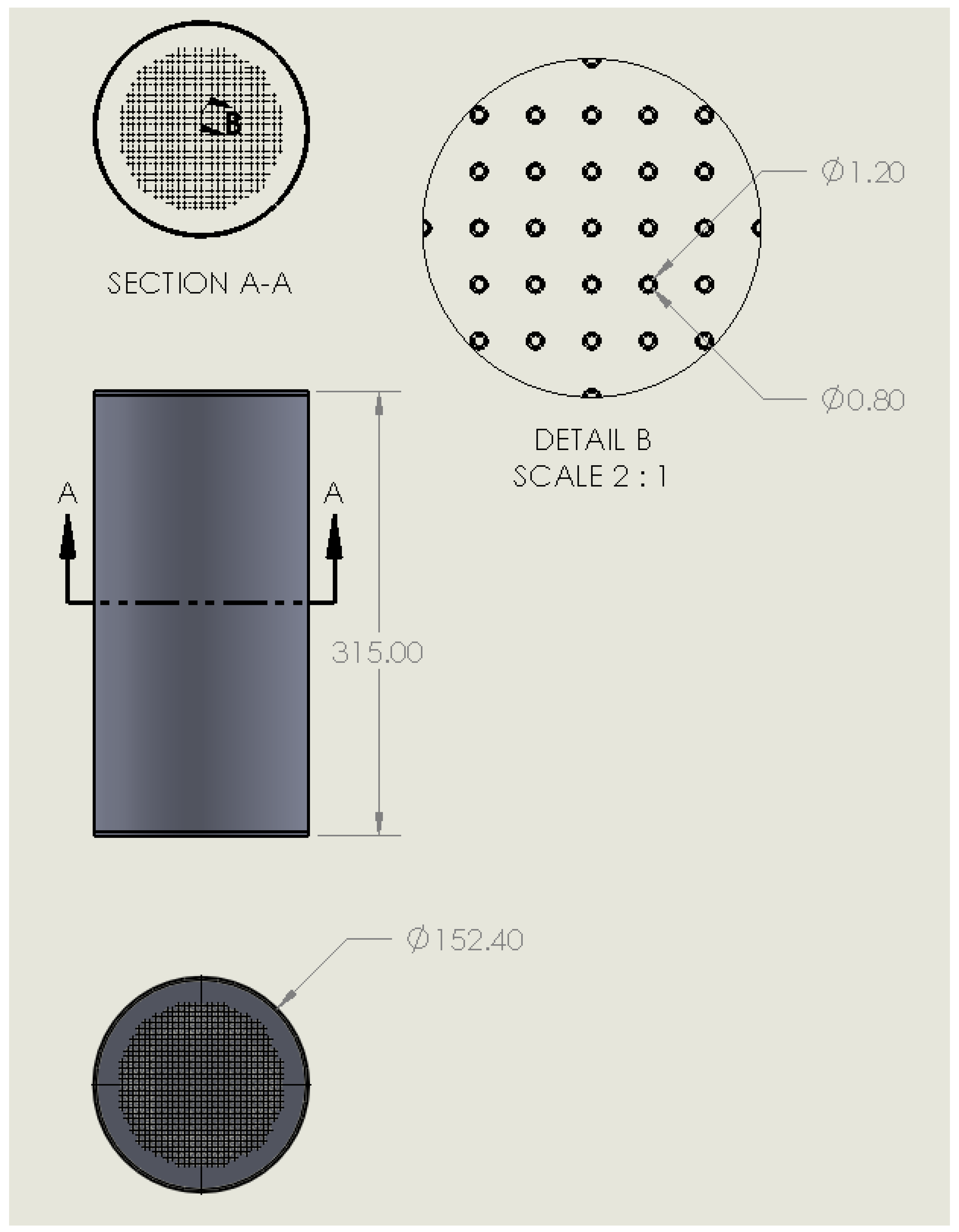


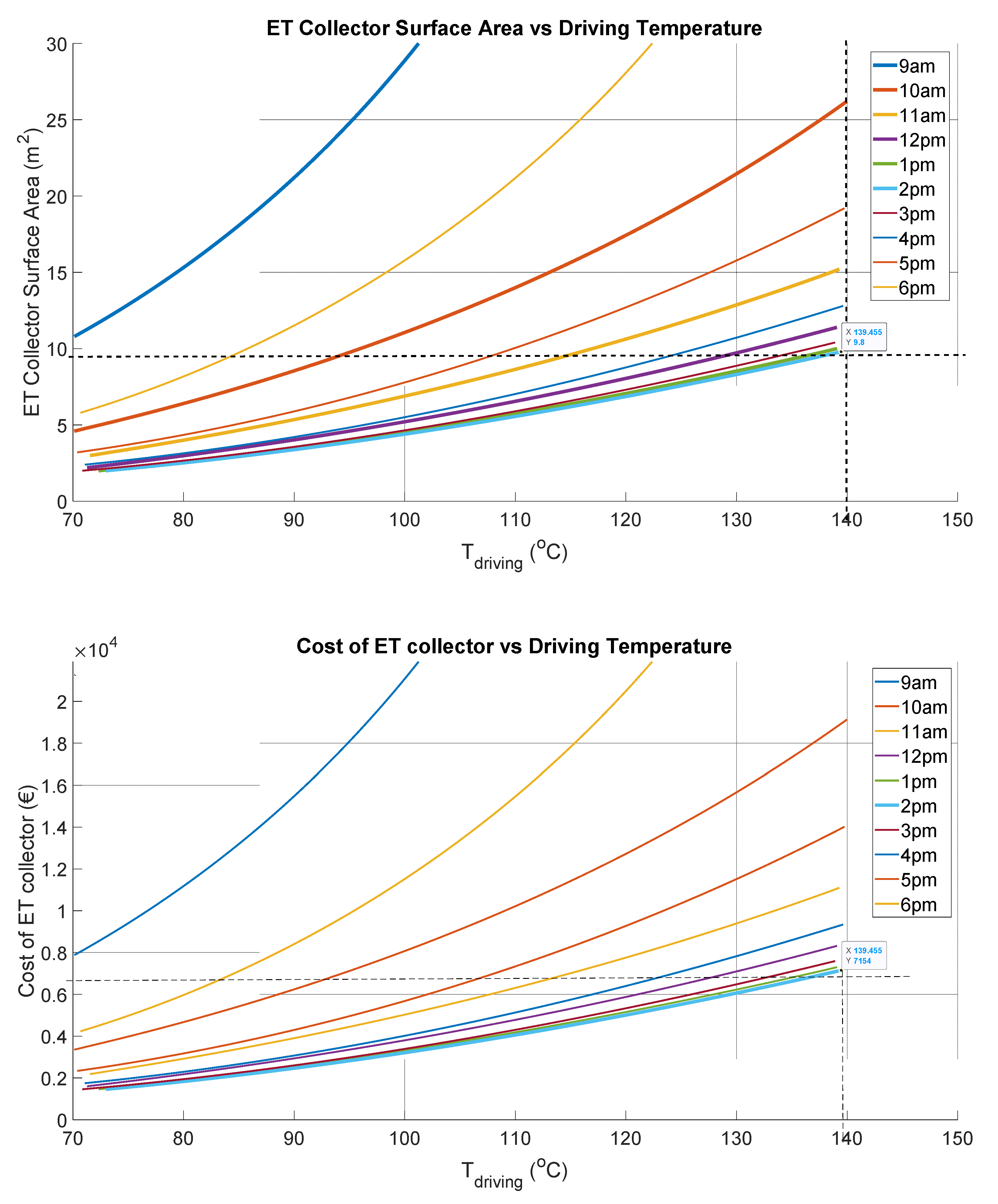
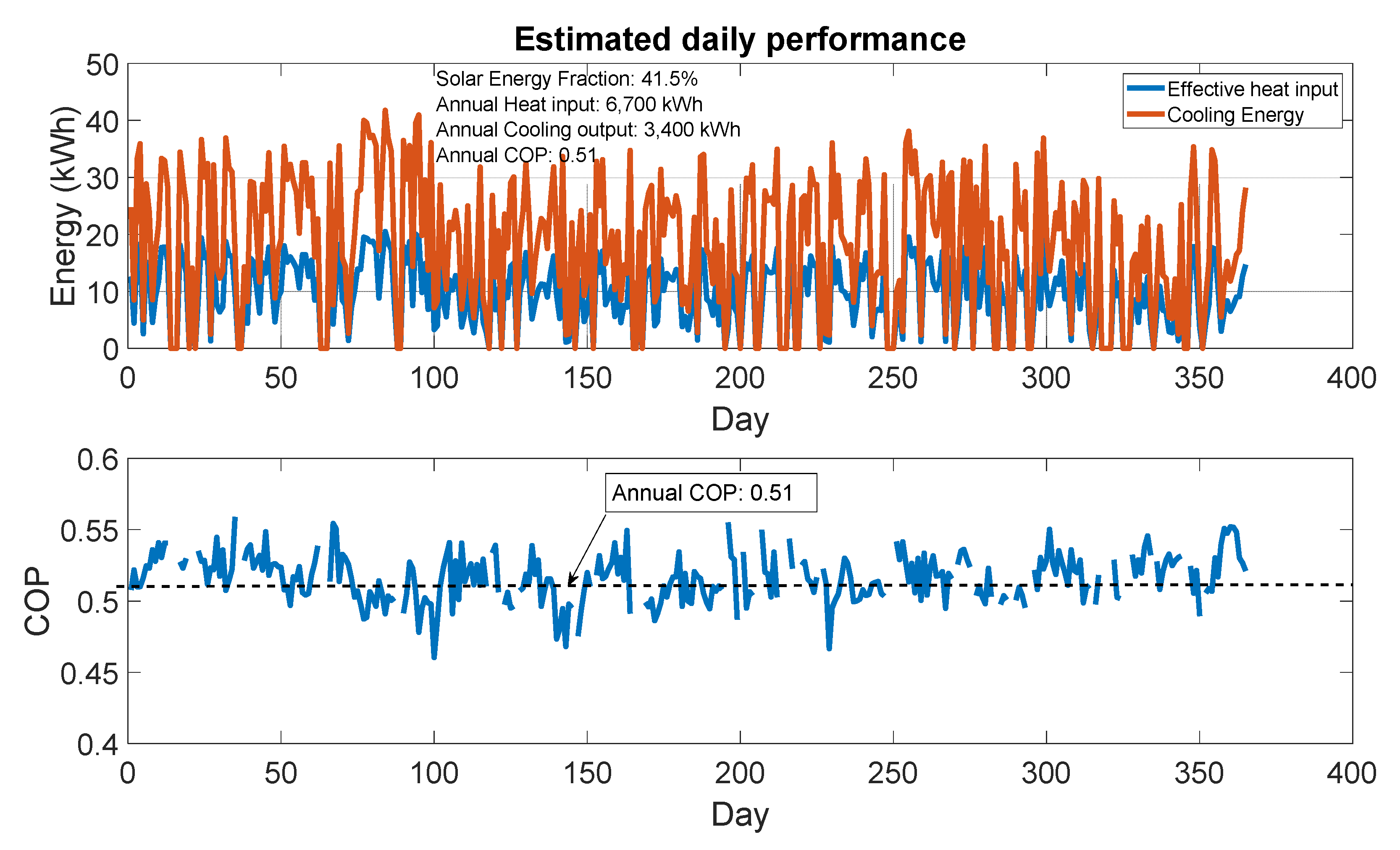
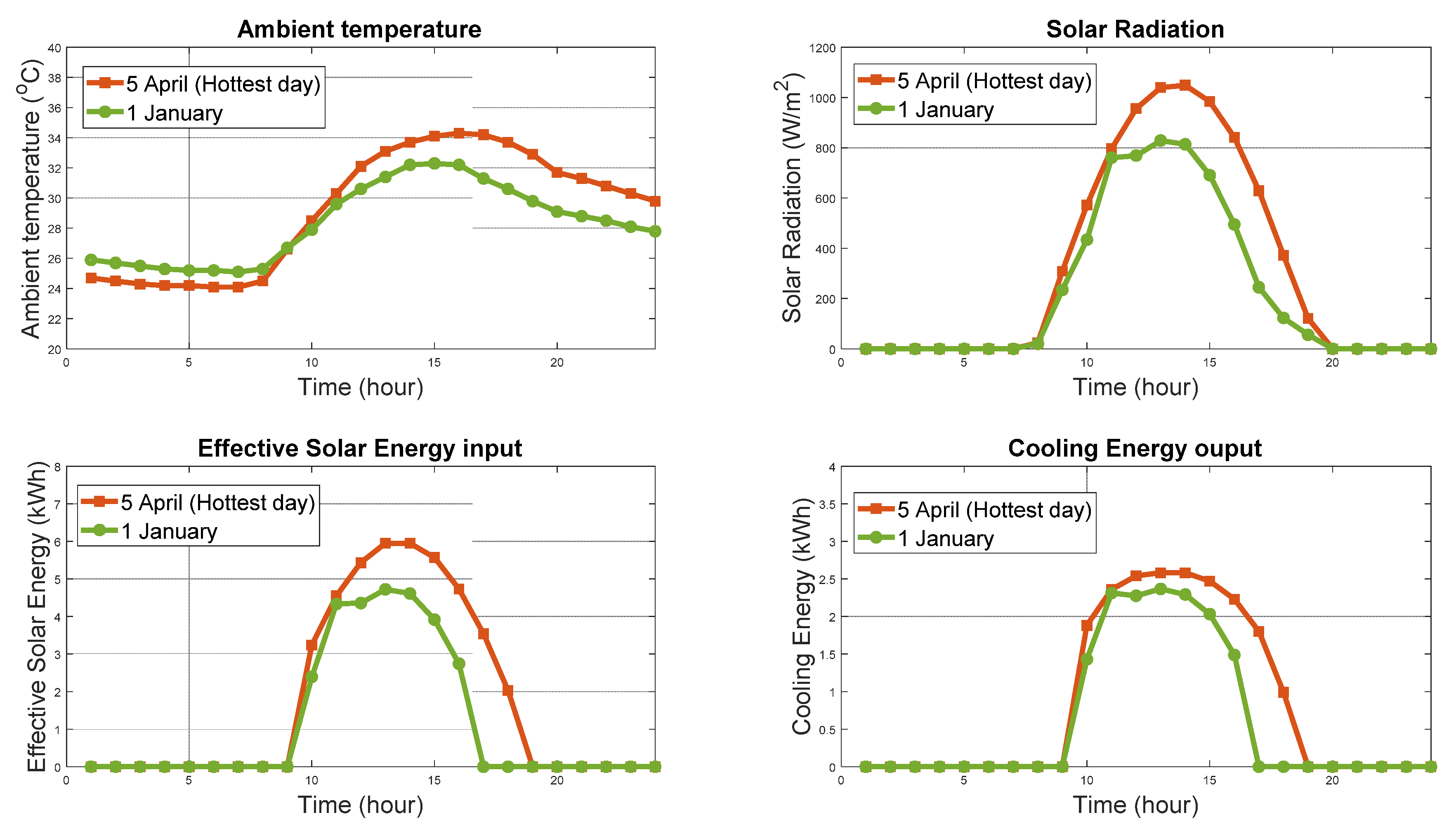
| Carbon Type | Compacted SRD1352/3 |
|---|---|
| Carbon thickness around each micro-tube (mm) | 1.97 |
| Carbon effective thermal conductivity (W m−1 K−1) | 0.42 |
| Carbon density ρc (kg m−3) | 435 |
| Total mass of carbon (kg) | 2.27 |
| Carbon UA value (W K−1) | 457.47 |
| D–A maximum uptake Xo (kg methanol kg−1 carbon) | 0.637 |
| D–A parameter | 5.0775 |
| D–A parameter | 0.8529 |
| Material of cylinder shell and miniaturized tubes | 316 SS |
| Length of cylinder and tubes (mm) | 315 |
| OD of cylinder (mm) | 152.4 |
| Thickness of cylinder wall (mm) | 1.63 |
| Number of micro-tubes | 800 |
| OD of tubes (mm) | 1.2 |
| ID of tubes (mm) | 0.8 |
| Heat transfer fluid HTF | Water |
| HTF density ρf (kg m−3) | 1000 |
| HTF thermal conductivity (W m−1 K−1) | 0.63 |
| HTF specific heat capacity (J kg−1 K−1) | 4200 |
| HTF flow rate in a single MT (m3 s−1) | 0.000333 |
| Reynolds number (Re) | 1002 |
| Nusselt number (Nu) | 4 |
| HTF convective heat transfer coefficient (W m−2 K−1) | 3150 |
| HTF UA value (W K−1) | 1995 |
| Solar Collector Reference | SolarBayer PremiumPlus 2.86 | SolarBayer CPC Nero 12 |
|---|---|---|
| Type | Flat Plate | Evacuated Tube |
| Cost (EUR/m2) | 500 | 730 |
| Optical efficiency | 0.773 | 0.719 |
| Linear loss coefficient (W m−2 K−1) | 3.675 | 0.93 |
| Quadratic loss coefficient (W m−2 K−2) | 0.007 | 0.004 |
| Time | Tamb (°C) | Solar Radiation G (W m−2) |
|---|---|---|
| 9 (9 am) | 26.6 | 309 |
| 10 (10 am) | 28.5 | 572 |
| 11 (11 am) | 30.3 | 797 |
| 12 (12 pm) | 32.1 | 956 |
| 13 (1 pm) | 33.1 | 1040 |
| 14 (2 pm) | 33.7 | 1049 |
| 15 (3 pm) | 34.1 | 984 |
| 16 (4 pm) | 34.3 | 841 |
| 17 (5 pm) | 34.2 | 629 |
| 18 (6 pm) | 33.7 | 372 |
| Month | Tamb (°C) | Solar Energy (kWh m−2) | Sunlight Hours | Solar Radiation G (W m−2) |
|---|---|---|---|---|
| January | 28.2 | 145 | 380 | 382 |
| February | 28.9 | 145 | 358 | 405 |
| March | 29.4 | 156 | 380 | 411 |
| April | 29.5 | 140 | 362 | 387 |
| May | 30.4 | 136 | 372 | 366 |
| June | 29.7 | 130 | 360 | 361 |
| July | 29.7 | 136 | 383 | 355 |
| August | 29.6 | 139 | 375 | 371 |
| September | 29.3 | 136 | 360 | 378 |
| October | 29.7 | 136 | 375 | 363 |
| November | 28.6 | 118 | 360 | 328 |
| December | 28.5 | 126 | 372 | 339 |
| Time | Tamb (°C) | G (W m−2) | Tdriving (°C) | SCP (kW/kg) | COP | Capacity (kW) | Cooling Energy (kWh) |
|---|---|---|---|---|---|---|---|
| 9 (9 am) | 26.6 | 309 | - | - | - | - | - |
| 10 (10 am) | 28.5 | 572 | 93 | 0.79 | 0.58 | 1.81 | 1.81 |
| 11 (11 am) | 30.3 | 797 | 115 | 1.03 | 0.52 | 2.33 | 2.33 |
| 12 (12 pm) | 32.1 | 956 | 130 | 1.12 | 0.47 | 2.54 | 2.54 |
| 13 (1 pm) | 33.1 | 1040 | 138 | 1.15 | 0.44 | 2.62 | 2.62 |
| 14 (2 pm) | 33.7 | 1049 | 140 | 1.15 | 0.43 | 2.60 | 2.60 |
| 15 (3 pm) | 34.1 | 984 | 135 | 1.09 | 0.44 | 2.47 | 2.47 |
| 16 (4 pm) | 34.3 | 841 | 124 | 0.97 | 0.47 | 2.21 | 2.21 |
| 17 (5 pm) | 34.2 | 629 | 106 | 0.75 | 0.51 | 1.70 | 1.70 |
| 18 (6 pm) | 33.7 | 372 | 80 | 0.33 | 0.41 | 0.74 | 0.74 |
| Time | Tamb (°C) | G (W m−2) | Tdriving (°C) | SCP (kW/kg) | COP | Capacity (kW) | Cooling Energy (kWh) |
|---|---|---|---|---|---|---|---|
| 9 (9 am) | 26.6 | 309 | - | - | - | - | - |
| 10 (10 am) | 28.5 | 572 | 95 | 0.82 | 0.58 | 1.88 | 1.88 |
| 11 (11 am) | 30.3 | 797 | 116 | 1.04 | 0.52 | 2.36 | 2.36 |
| 12 (12 pm) | 32.1 | 956 | 130 | 1.12 | 0.47 | 2.54 | 2.54 |
| 13 (1 pm) | 33.1 | 1040 | 138 | 1.14 | 0.43 | 2.58 | 2.58 |
| 14 (2 pm) | 33.7 | 1049 | 139 | 1.14 | 0.43 | 2.58 | 2.58 |
| 15 (3 pm) | 34.1 | 984 | 135 | 1.09 | 0.44 | 2.47 | 2.47 |
| 16 (4 pm) | 34.3 | 841 | 125 | 0.98 | 0.47 | 2.23 | 2.23 |
| 17 (5 pm) | 34.2 | 629 | 109 | 0.79 | 0.51 | 1.80 | 1.80 |
| 18 (6 pm) | 33.7 | 372 | 85 | 0.44 | 0.49 | 0.99 | 0.99 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tamainot-Telto, Z.; Metcalf, S.J.; Yande, N.N. Adsorption Solar Air Conditioning System for Singapore Climate. Energies 2022, 15, 6537. https://doi.org/10.3390/en15186537
Tamainot-Telto Z, Metcalf SJ, Yande NN. Adsorption Solar Air Conditioning System for Singapore Climate. Energies. 2022; 15(18):6537. https://doi.org/10.3390/en15186537
Chicago/Turabian StyleTamainot-Telto, Zacharie, Stephen John Metcalf, and Neilson Ng Yande. 2022. "Adsorption Solar Air Conditioning System for Singapore Climate" Energies 15, no. 18: 6537. https://doi.org/10.3390/en15186537
APA StyleTamainot-Telto, Z., Metcalf, S. J., & Yande, N. N. (2022). Adsorption Solar Air Conditioning System for Singapore Climate. Energies, 15(18), 6537. https://doi.org/10.3390/en15186537







