Computational Fluid Dynamics and Experimental Analysis of a Wind Turbine Blade’s Frontal Section with and without Arrays of Dimpled Structures
Abstract
:1. Introduction
2. Design of the Turbine Blade
2.1. Original Frontal Section
2.2. Proposed Frontal Section
2.3. Experimental Investigation
2.4. Numerical Simulation Models
- Dhk = value of manometer;
- ρk = air density;
- g = acceleration due to gravity;
- P = pressure.
- FL = lift force;
- FD = drag force;
- V = free stream velocity;
- ρ = density of air;
- dP = difference between ambient and static pressure;
- ATotal = Total Present Projected Area (A1 + A2 + A3 +………+ An).
- An upwind-based multidimensional linear construction approach is used.
- Settings are in a default solver mode to solve a steady state problem.
- For the solution of equations of pressure, kinetic energy and turbulence dissipation, the upwind discretization scheme is utilized.
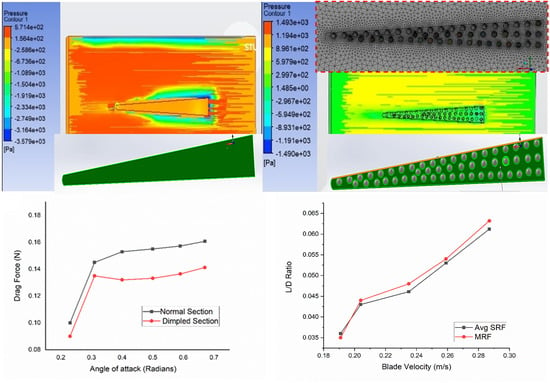
3. Results and Discussion
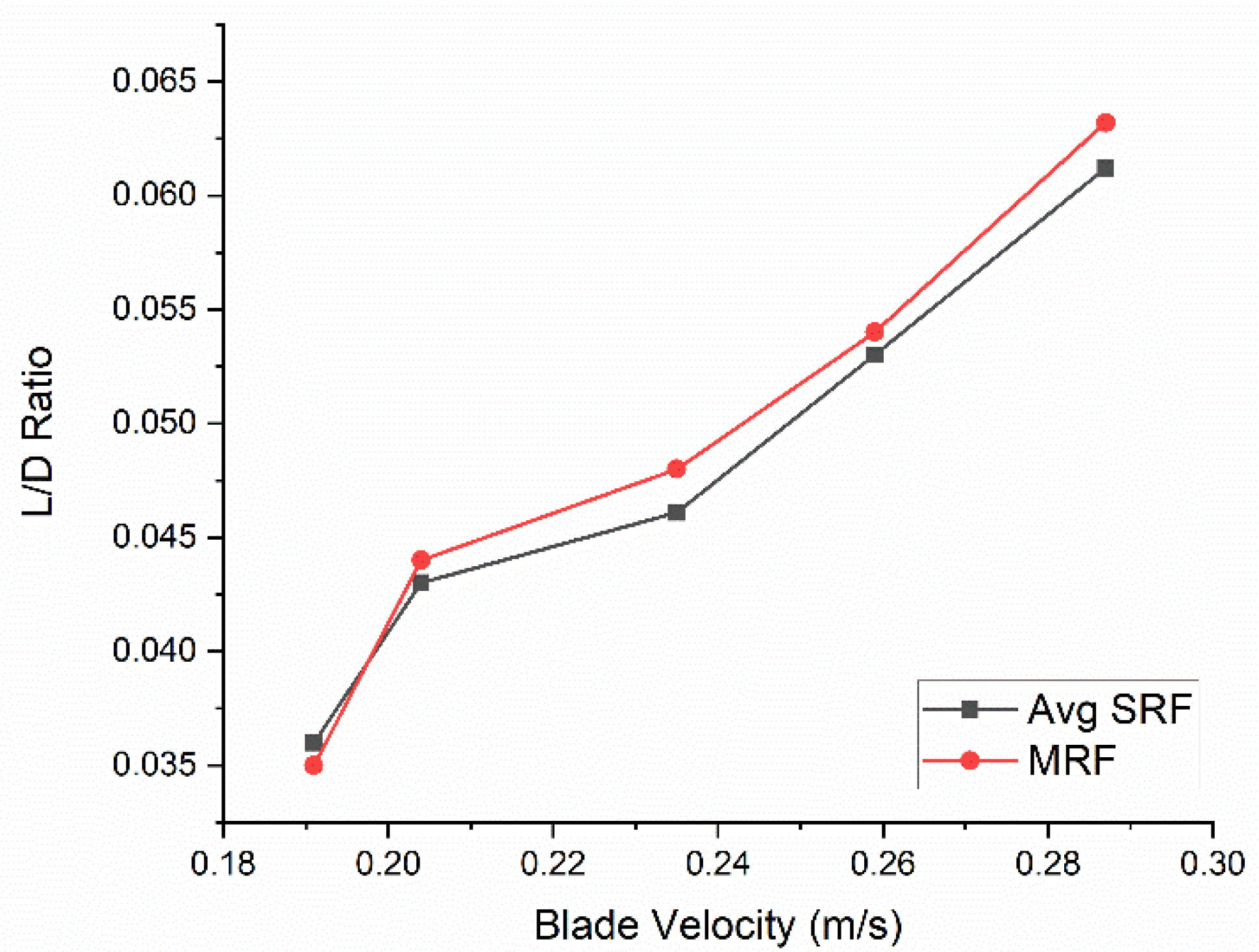
3.1. Single Reference Frame (SRF) Approach
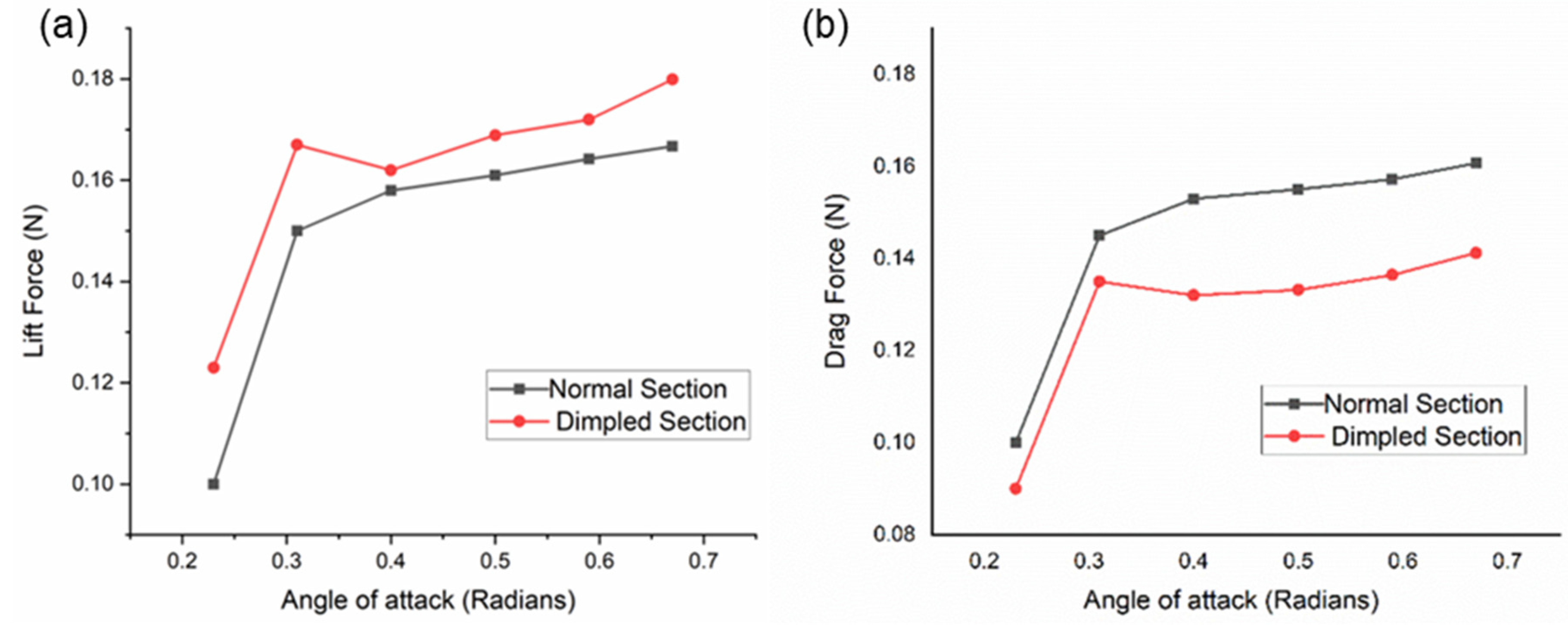
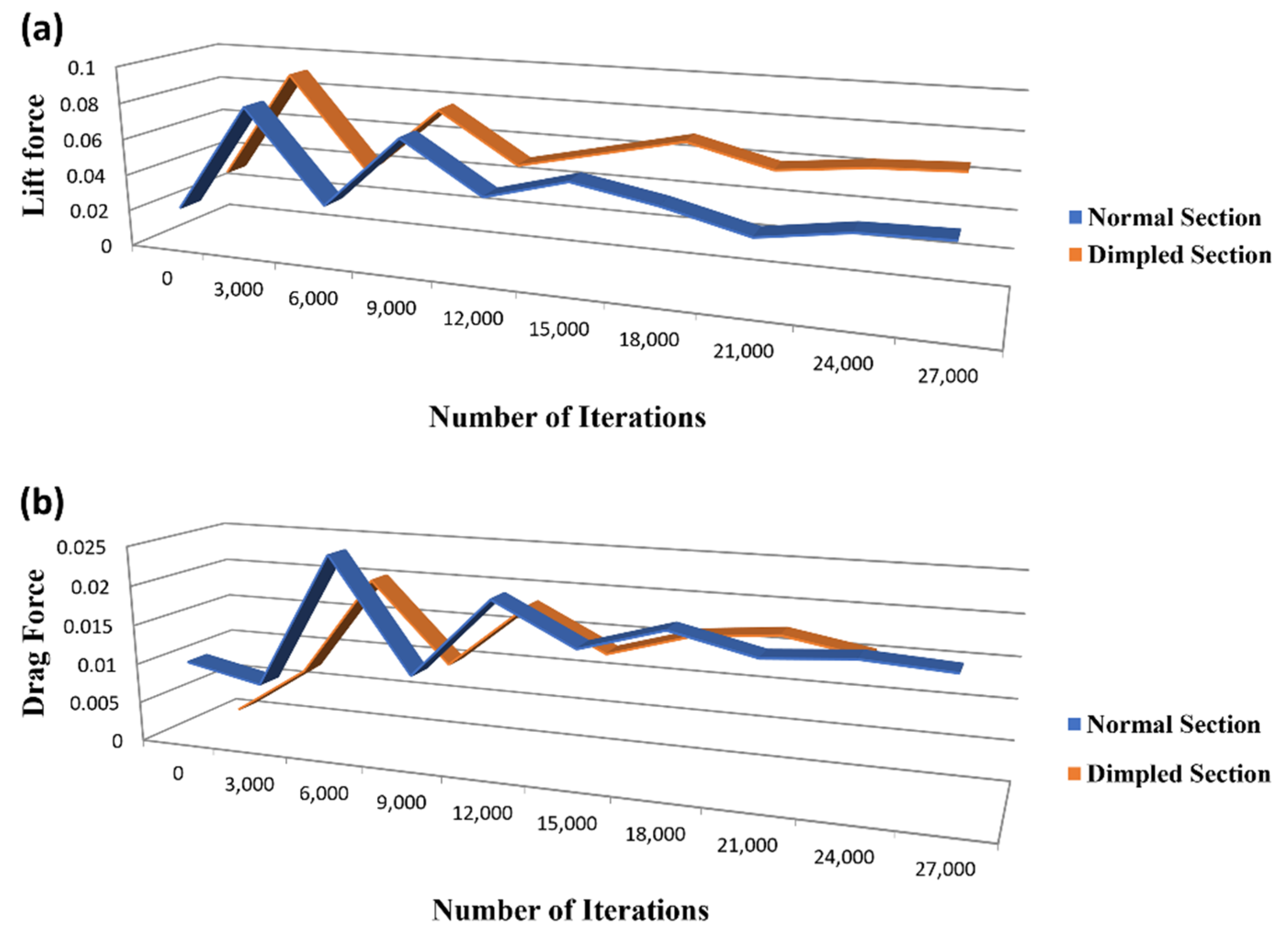
3.2. Lift and Drag Curves
3.3. Uncertainty Calculation for Lift and Drag Forces
3.4. Moving Reference Frame (MRF) Approach
3.5. Lift and Drag Curves
- The difference between the constant boundary conditions in CFD and the actual (variable) boundary conditions in the experiments. These boundary conditions are assumed to be constant in CFD and the experiments.
- The wind tunnel may have some systematic errors that cause the difference between the CL (EXP) and CL (CFD).
- The wind tunnel may have blockage effects at the inlet or exit that cause the difference between the experimental and CFD coefficients.
3.6. Experimental Investigation of Lift and Drag Coefficients
4. Conclusions
- In this research work, the investigation is carried out at angles of attack from 0° to 360°, at a Reynolds number 10,000 and at an inlet velocity 0.17 m/s.
- The lift force and drag force acting on a turbine blade section are directly proportional to the AOA, whereas the optimum AOA where the maximum L/D ratio is attained is 25°.
- The efficiency of a turbine blade can be increased by optimizing the design of a turbine blade and by increasing the L/D ratio.
- The number of velocity streamlines is inversely proportional to the drag coefficient, as a smaller number of velocity streamlines appear in the dimpled section of a turbine blade as compared to the original section of a turbine blade.
- Dimpled structures on the turbine blade surface cause the air to flow more smoothly; thus, a wake region is decreased, creating a low-pressure area behind the blade surface due to which less drag is generated there. Dimpled structures on the turbine blade surface cause the air to flow smoother, reducing the wake region and creating a low-pressure area behind the blade surface, hence reducing the drag force acting on the blade. They also cause the air on the top surface of the turbine blade to move faster; as a result, the pressure decreases, and more lift force is generated.
- The results from SRF and MRF are in good agreement with the experimental results.
Author Contributions
Funding
Conflicts of Interest
References
- Adeyeye, K.A.; Ijumba, N.; Colton, J. The Effect of the Number of Blades on the Efficiency of A Wind Turbine. IOP Conf. Ser. Earth Environ. Sci. 2021, 801, 012020. [Google Scholar] [CrossRef]
- Kamalasree, H.; Rao, A.V.L. Design & Manufacturing ofsteam turbine blade. Int. J. Sci. Eng. Res. 2017, 8, 373–384. [Google Scholar]
- Pigott, R. Turbine blade vibration due to partial admission. Int. J. Mech. Sci. 1980, 22, 247–264. [Google Scholar] [CrossRef]
- Najar, F.A.; Harmain, G.A. Blade Design and Performance Analysis of Wind Turbine. Int. J. ChemTech Res. 2013, 5, 1054–1061. [Google Scholar] [CrossRef]
- Rehman, S.; Mahbub Alam, M.; Alhems, L.M.; Mujahid Rafique, M. Horizontal Axis Wind Turbine Blade Design Methodologies for Efficiency Enhancement A Review. Energies 2018, 11, 506. [Google Scholar] [CrossRef]
- Abid, M.A.R.; Sarwar, M.I.; Tahir, A.; Shah, S.M.; Shehryar, M. Gas Turbine Blade Flow Analysis Comparison Using CFD and Wind Tunnel. In Proceedings of the 9th International Bhurban Conference on Applied Sciences & Technology (IBCAST), Islamabad, Pakistan, 9–12 January 2012; pp. 203–207. [Google Scholar] [CrossRef]
- Oukassou, K.; El Mouhsine, S.; El Hajjaji, A.; Kharbouch, B. Comparison of the Power, Lift and Drag Coefficients of Wind Turbine Blade from Aerodynamics Characteristics of Naca0012 and Naca2412. Procedia Manuf. 2019, 32, 983–990. [Google Scholar] [CrossRef]
- Sakthivel, P.; Rajamani, G.P. Design and Analysis of Modified Wind Turbine Blades. Asian J. Res. Soc. Sci. Humanit. 2017, 7, 166. [Google Scholar] [CrossRef]
- Saraf, A.K.; Singh, D.M.P.; Chouhan, D.T.S. Effect of Dimple on Aerodynamic Behaviour of Airfoil. Int. J. Eng. Technol. 2017, 9, 2268–2277. [Google Scholar] [CrossRef]
- Sourav, A.P.; Koly, F.A. A review of different shaped dimple effects on aerofoil surfaces. In Proceeding of the International Conference on Mechanical Engineering and Renewable Energy 2019 (ICMERE 2019), Chittagong, Bangladesh, 11–13 December 2019. [Google Scholar]
- Kumar Shaw, K.; Kesarwani, Y.; Chakravarty, P. Study of Dimple Effect on Aerodynamic Drag Characteristics of a Car. Prepr. Int. J. Innov. Res. Sci. Eng. Technol. 2020, 9, 4628–4637. [Google Scholar] [CrossRef]
- Zhou, D.; Wu, L.; Tan, C.; Hu, T. Study on the Effect of Dimple Position on Drag Reduction of High-Speed Maglev Train. Transp. Saf. Environ. 2021, 3, tdab027. [Google Scholar] [CrossRef]
- Thabet, S.; Thabit, T.H. Computational Fluid Dynamics: Science of the Future. Int. J. Res. Eng. 2018, 5, 430–433. [Google Scholar] [CrossRef]
- Settar, A.N.; Sarip, S.; Kaidi, H.M. Computational Fluid Dynamics Model of Wells Turbine for Oscillating Water Column System: A Review. J. Phys. Conf. Ser. 2021, 2053, 012013. [Google Scholar] [CrossRef]
- Kalvig, S.; Manger, E.; Hjertager, B. Comparing Different CFD Wind Turbine Modelling Approaches with Wind Tunnel Measurements. J. Phys. Conf. Ser. 2014, 555, 012056. [Google Scholar] [CrossRef]
- Li, Q.; Jia, H.; Qiu, Q.; Lu, Y.; Zhang, J.; Mao, J.; Fan, W.; Huang, M. Typhoon-Induced Fragility Analysis of Transmission Tower in Ningbo Area Considering the Effect of Long-Term Corrosion. Appl. Sci. 2022, 12, 4774. [Google Scholar] [CrossRef]
- Ismail, M.F.; Vijayaraghavan, K. The Effects of Aerofoil Profile Modification on a Vertical Axis Wind Turbine Performance. Energy 2015, 80, 20–31. [Google Scholar] [CrossRef]
- Sunada, S.; Sakaguchi, A.; Kawachi, K. Airfoil Section Characteristics at a Low Reynolds Number. J. Fluids Eng. Trans. ASME 1997, 119, 129–135. [Google Scholar] [CrossRef]
- Sunada, S.; Yasuda, T.; Yasuda, K.; Kawachi, K. Comparison of Wing Characteristics at an Ultralow Reynolds Number. J. Aircr. 2002, 39, 331–338. [Google Scholar] [CrossRef]
- Haque, M.N.; Ali, M.; Ara, I. Experimental Investigation on the Performance of NACA 4412 Aerofoil with Curved Leading Edge Planform. Procedia Eng. 2015, 105, 232–240. [Google Scholar] [CrossRef]
- Kroo, I.; Prinz, F.; Shantz, M.; Kunz, P.; Fay, G.; Cheng, S.; Fabian, T.; Partridge, C. The Mesicopter: A Miniature Rotorcraft Concept; Stanford University: Stanford, CA, USA, 2000. [Google Scholar]
- Viieru, D.; Tang, J.; Lian, Y.; Liu, H.; Shyy, W. Flapping and Flexible Wing Aerodynamics of Low Reynolds Number Flight Vehicles. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit 2006, Reno, Nevada, 9–12 January 2006; Volume 9, pp. 6087–6104, Collected Technical Paper. [Google Scholar] [CrossRef]
- Rainbird, J.M.; Peiró, J.; Graham, J.M.R. Blockage-Tolerant Wind Tunnel Measurements for a NACA 0012 at High Angles of Attack. J. Wind Eng. Ind. Aerodyn. 2015, 145, 209–218. [Google Scholar] [CrossRef]
- Schubel, P.J.; Crossley, R.J. Wind Turbine Blade Design. Energies 2012, 5, 3425–3449. [Google Scholar] [CrossRef]
- Bardakjian, A.T.; Mandadakis, P.P.; Tingle, A. Efficiency Comparison of Horizontal Axis Wind Turbines and Bladeless Turbines. PAM Rev. Energy Sci. Technol. 2017, 4, 59–75. [Google Scholar] [CrossRef]
- Ahmed, Z.U.; Mashud, M.; Joty, S.M. Assessment of Viscous Models on the Simulation of Low Cut-Off Wind Turbine Blade. ARPN J. Eng. Appl. Sci. 2021, 16, 1954–1966. [Google Scholar]
- Ciappi, L.; Stebel, M.; Smolka, J.; Cappietti, L.; Manfrida, G. Analytical and Computational Fluid Dynamics Models of Wells Turbines for Oscillating Water Column Systems. J. Energy Resour. Technol. Trans. ASME 2022, 144, 050903. [Google Scholar] [CrossRef]
- Zongheng, H.; Tao, Y.; Guanyu, C.; Xiangrui, L.; Yu, H. Simulation Analysis on the Blade Airfoil of Small Wind Turbine. IOP Conf. Ser. Earth Environ. Sci. 2019, 295, 012079. [Google Scholar] [CrossRef]
- Uruba, V. Reynolds Number in Laminar Flows and in Turbulence. AIP Conf. Proc. 2019, 2118, 020003. [Google Scholar] [CrossRef]
- Michna, J.; Rogowski, K. Numerical Study of the Effect of the Reynolds Number and the Turbulence Intensity on the Performance of the NACA 0018 Airfoil at the Low Reynolds Number Regime. Processes 2022, 10, 1004. [Google Scholar] [CrossRef]





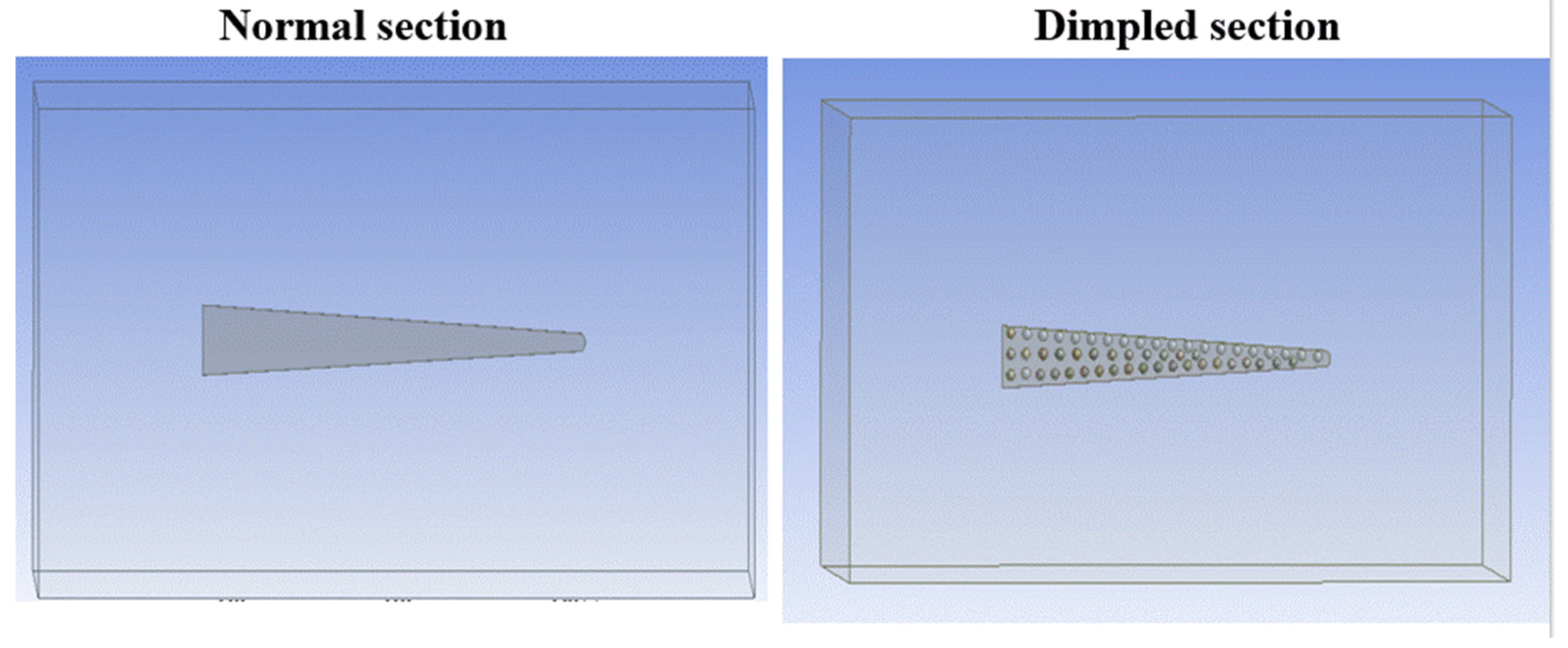





| Blade Frontal Section Surface | AOA (°) | Air Velocity (m/s) | Tapping Points (mm) | Surface Area of a Turbine Blade (mm2) |
|---|---|---|---|---|
| Plain surface | 0° to 50° | 0.17 | 52 | 85,872.2 mm2 |
| Extruded dimpled surface | 0° to 360° | 0.17 | 52 | 54,908 mm2 |
| Normal Section | Dimpled Section |
Lift Coefficient (CL) = 2FL/(p × A × V2)
CL = 0.22125 Drag Coefficient (CD) = 2 × FD/(p × A × V2) FD = 0.146 N CD = 2 × 0.146/1.225 × 0.0061948 × 92 CD = 0.48798 | Lift Coefficient (CL) = 2FL/(p × A × V2)
CL = 0.2425 Drag Coefficient (CD) = 2 × FD/(p × A × V2) FD = 0.142 N CD = 2 × 0.142/1.225 × 0.0061948 × 92 CD = 0.46698 |
| SRF | |
|---|---|
| Normal Section | Dimpled Section |
| CFD CL = 0.307 CD = 0.47 Experimental CL = 0.247 CD = 0.46 Uncertainty Lift Coefficient Uncertainty = (0.28260–0.251925)/0.251925 = 12% Drag Coefficient Uncertainty = (0.493665–0.484355)/0.484355 = 2% | CFD CL = 0.307 CD = 0.465 Experimental CL = 0.247 CD = 0.45 Uncertainty Lift Coefficient Uncertainty = (0.307260–0.277925)/0.2221925 = 8.5% Drag Coefficient Uncertainty = (0.447665–0.484355)/0.484355 = 5% |
| MRF | |
|---|---|
| Normal Section | Dimpled Section |
| CFD CL = 0.285 CD = 0.479 Experimental CL = 0.247 CD = 0.462 Uncertainty Lift Coefficient Uncertainty = (0.28260–0.251925)/0.251925 = 10% Drag Coefficient Uncertainty = (0.493665–0.484355)/0.484355 = 2% | CFD CL = 0.308 CD = 0.472 Experimental CL = 0.247 CD = 0.455 Uncertainty Lift Coefficient Uncertainty = (0.307260–0.277925)/0.2221925 = 8.2% Drag Coefficient Uncertainty = (0.447665–0.484355)/0.484355 = 5.8% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aziz, S.; Khan, A.; Shah, I.; Khan, T.A.; Ali, Y.; Sohail, M.U.; Rashid, B.; Jung, D.W. Computational Fluid Dynamics and Experimental Analysis of a Wind Turbine Blade’s Frontal Section with and without Arrays of Dimpled Structures. Energies 2022, 15, 7108. https://doi.org/10.3390/en15197108
Aziz S, Khan A, Shah I, Khan TA, Ali Y, Sohail MU, Rashid B, Jung DW. Computational Fluid Dynamics and Experimental Analysis of a Wind Turbine Blade’s Frontal Section with and without Arrays of Dimpled Structures. Energies. 2022; 15(19):7108. https://doi.org/10.3390/en15197108
Chicago/Turabian StyleAziz, Shahid, Abdullah Khan, Imran Shah, Tariq Amin Khan, Yasir Ali, Muhammad Umer Sohail, Badar Rashid, and Dong Won Jung. 2022. "Computational Fluid Dynamics and Experimental Analysis of a Wind Turbine Blade’s Frontal Section with and without Arrays of Dimpled Structures" Energies 15, no. 19: 7108. https://doi.org/10.3390/en15197108
APA StyleAziz, S., Khan, A., Shah, I., Khan, T. A., Ali, Y., Sohail, M. U., Rashid, B., & Jung, D. W. (2022). Computational Fluid Dynamics and Experimental Analysis of a Wind Turbine Blade’s Frontal Section with and without Arrays of Dimpled Structures. Energies, 15(19), 7108. https://doi.org/10.3390/en15197108