A Review on Power Electronic Topologies and Control for Wave Energy Converters
Abstract
14. Introduction
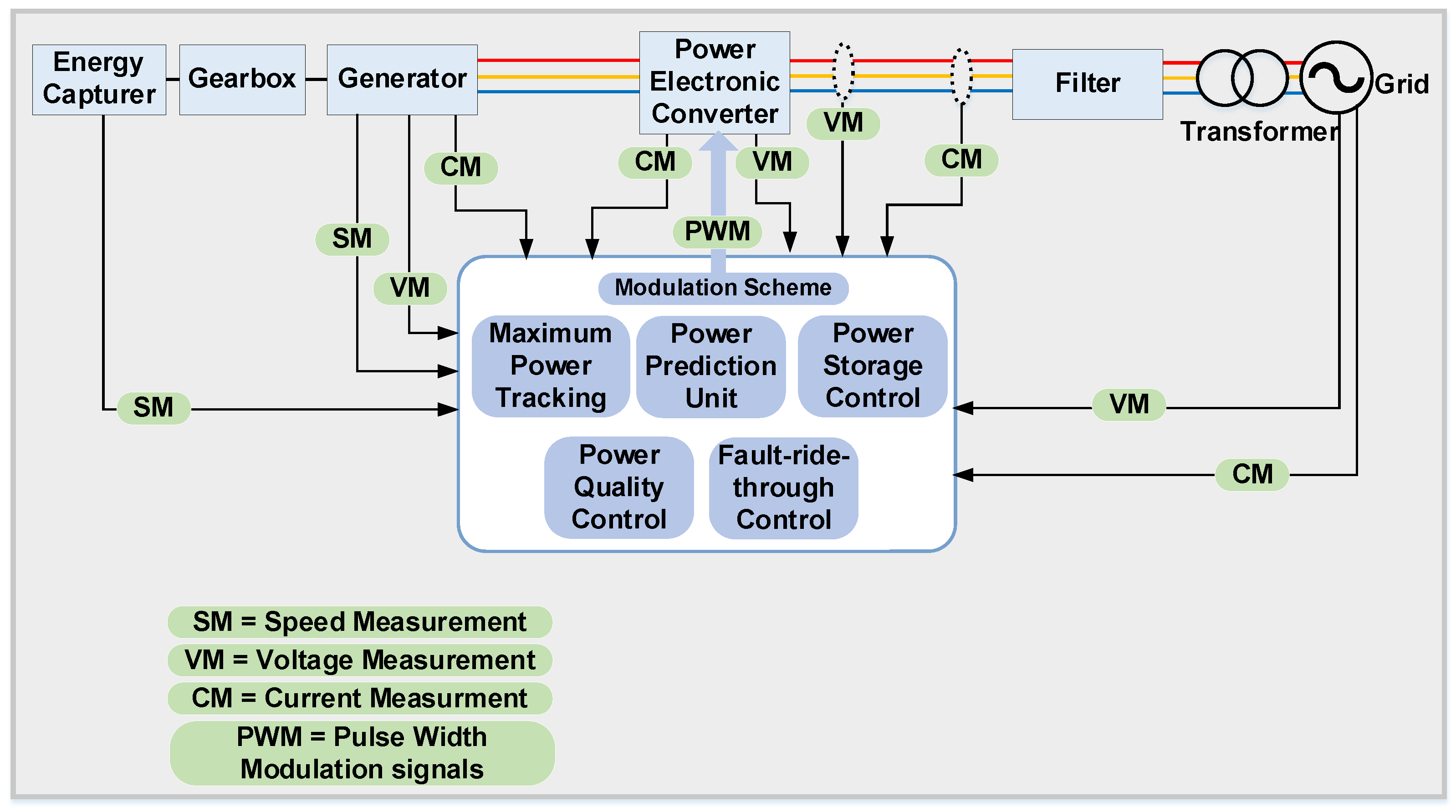
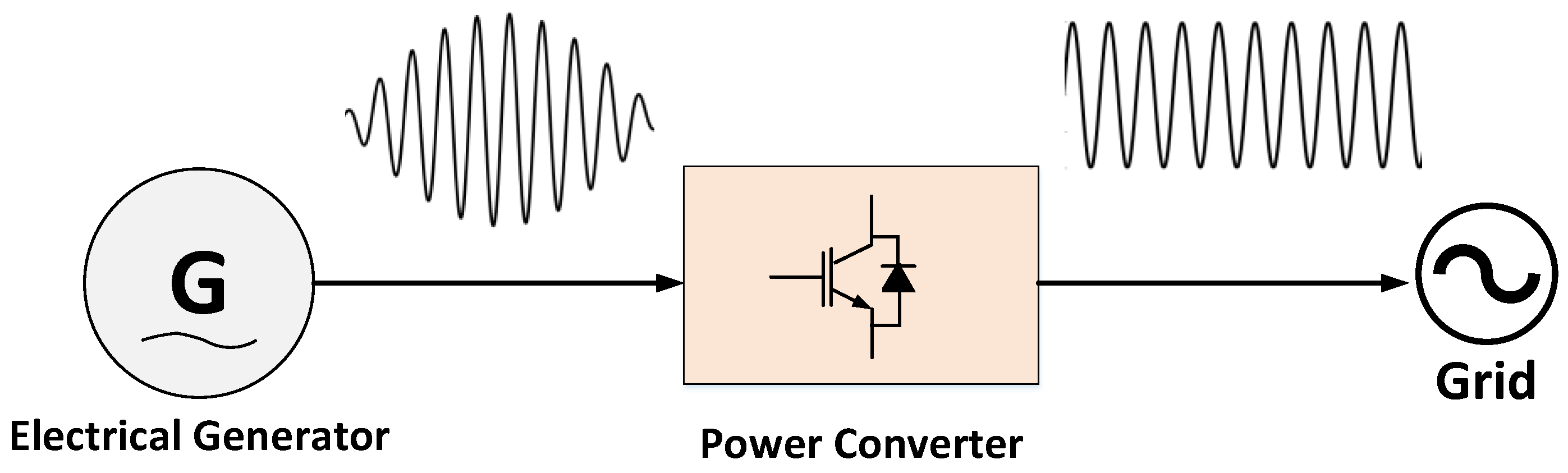
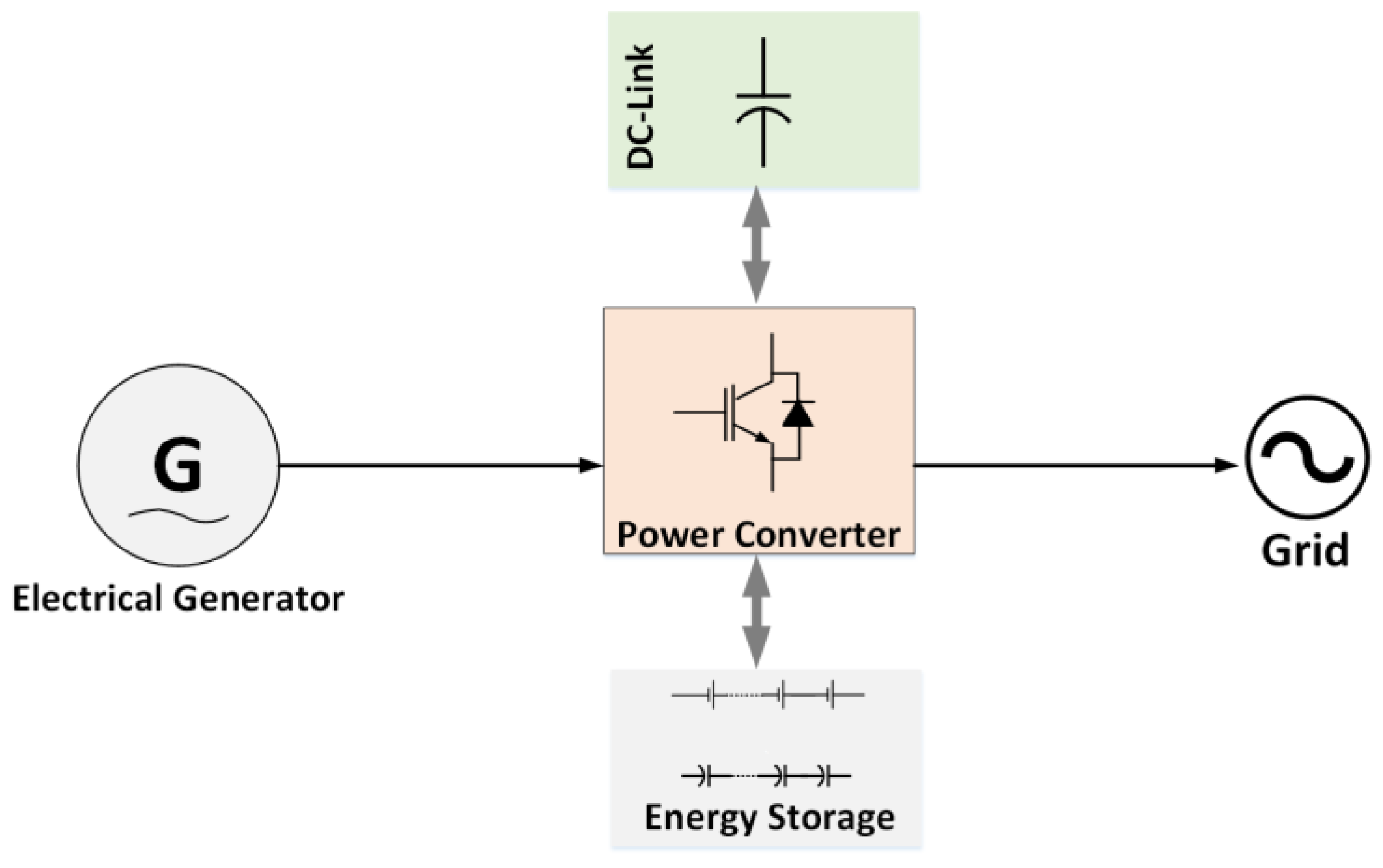
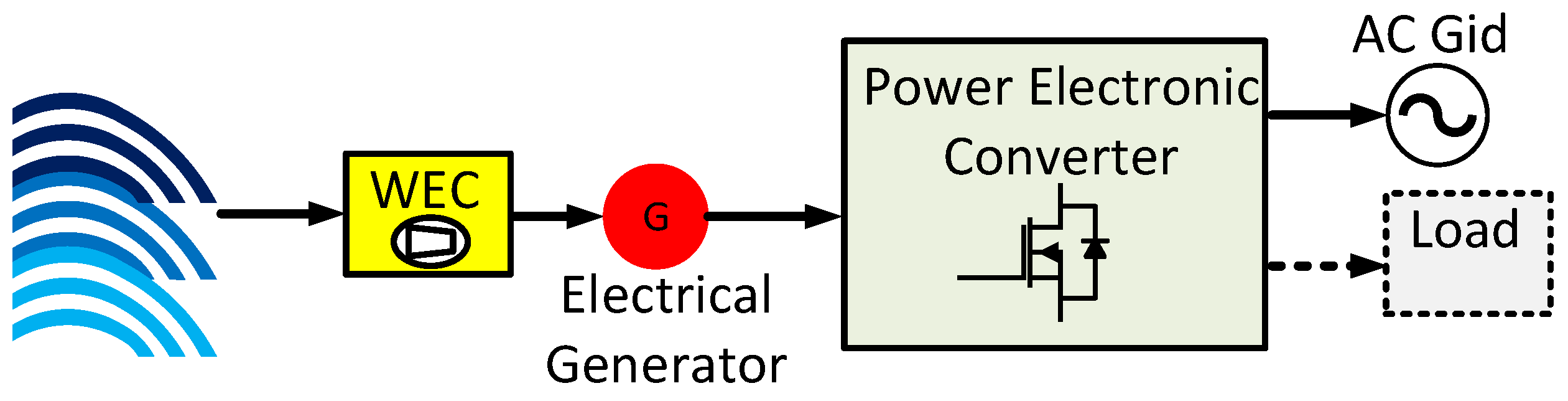
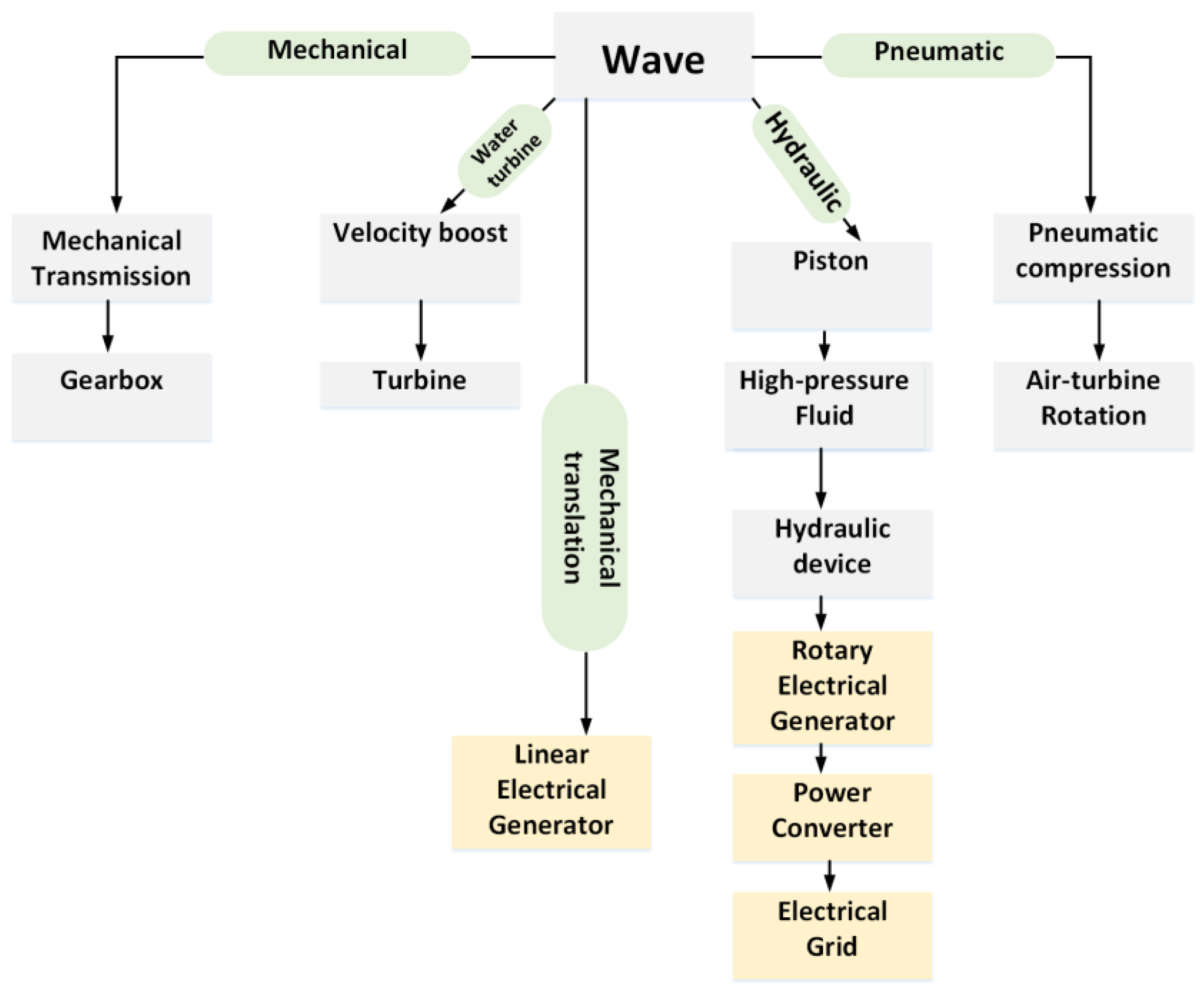
2. WEC Electrical Considerations
3. Electrical System of the WECs
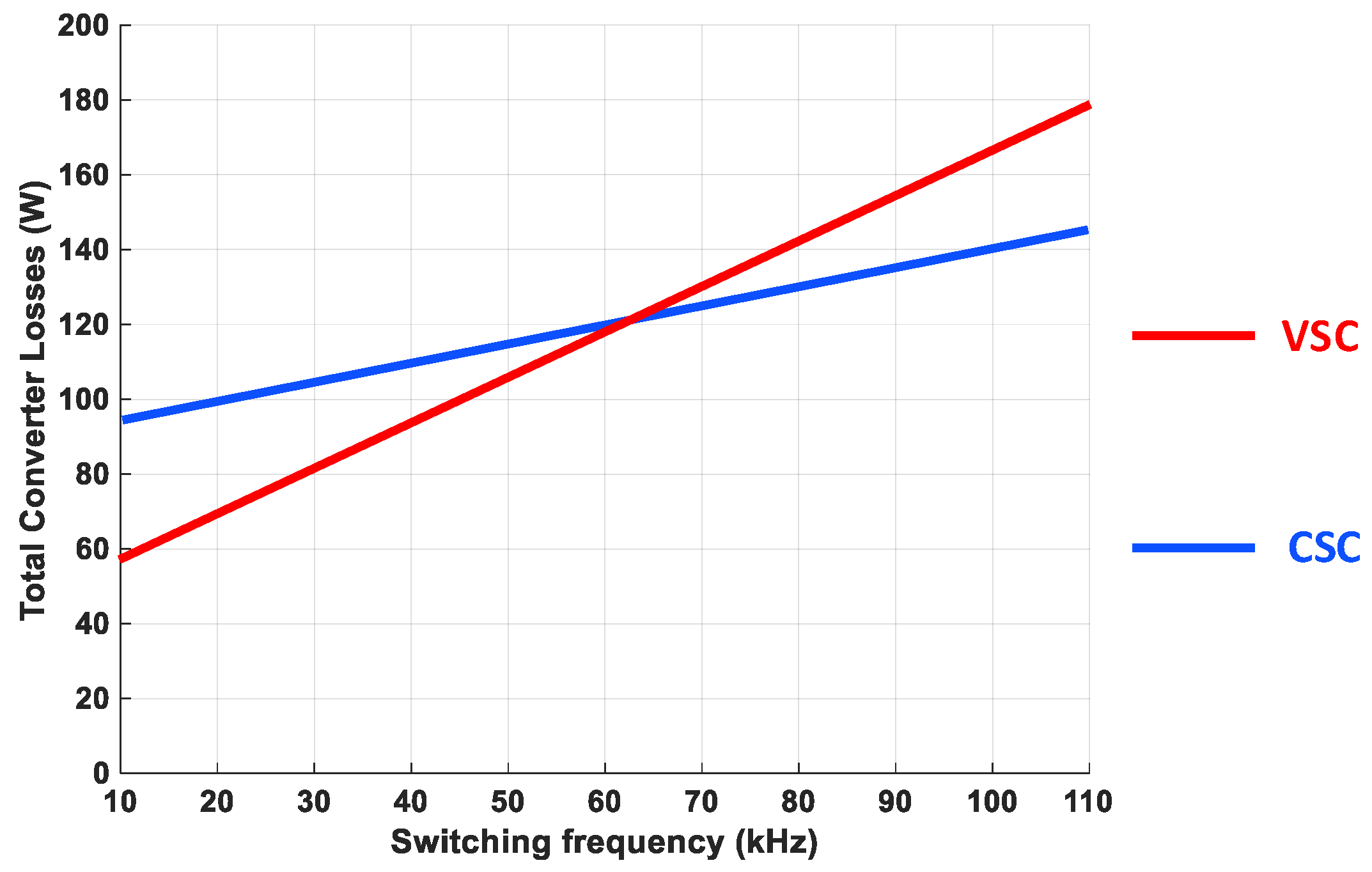
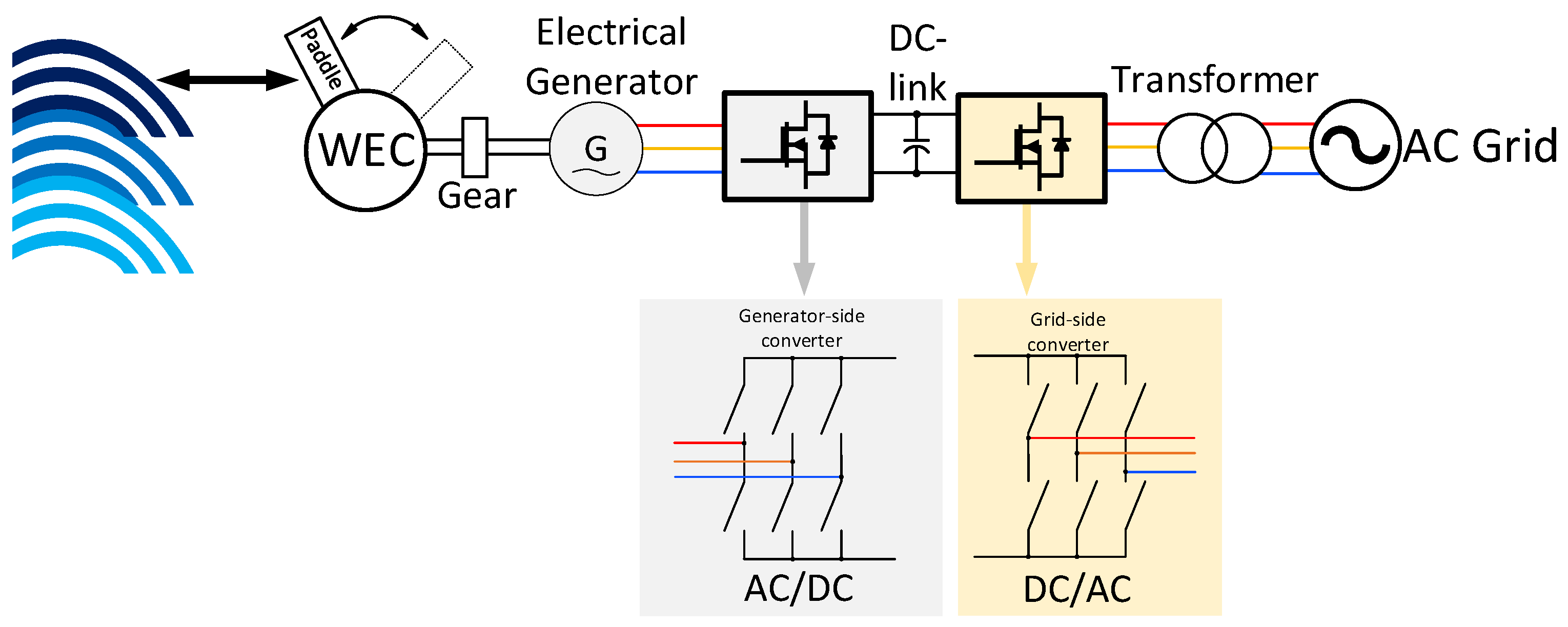
3.1. AC/DC/AC Back-to-Back Converters
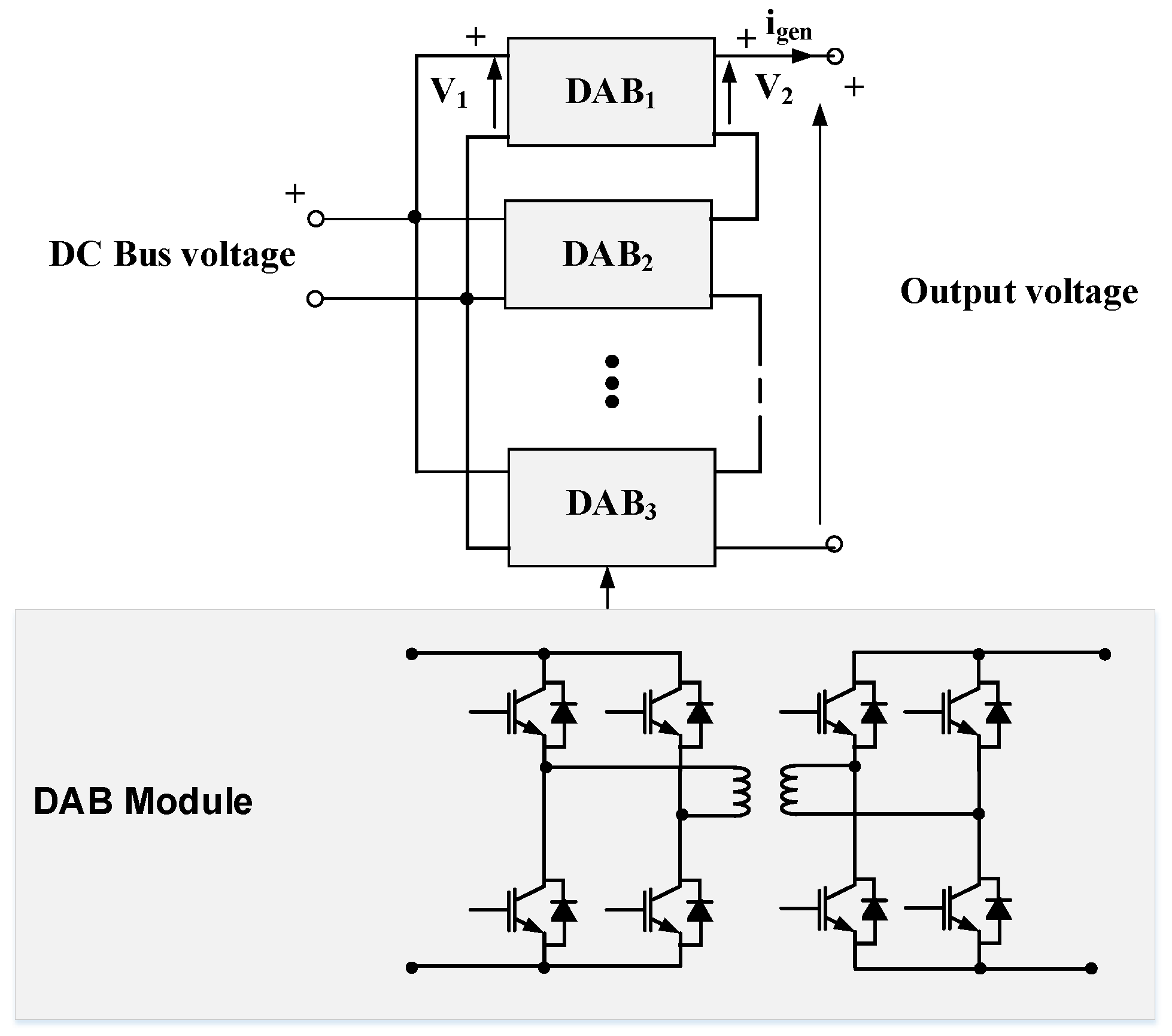
3.2. Modular and Cascaded Configurations
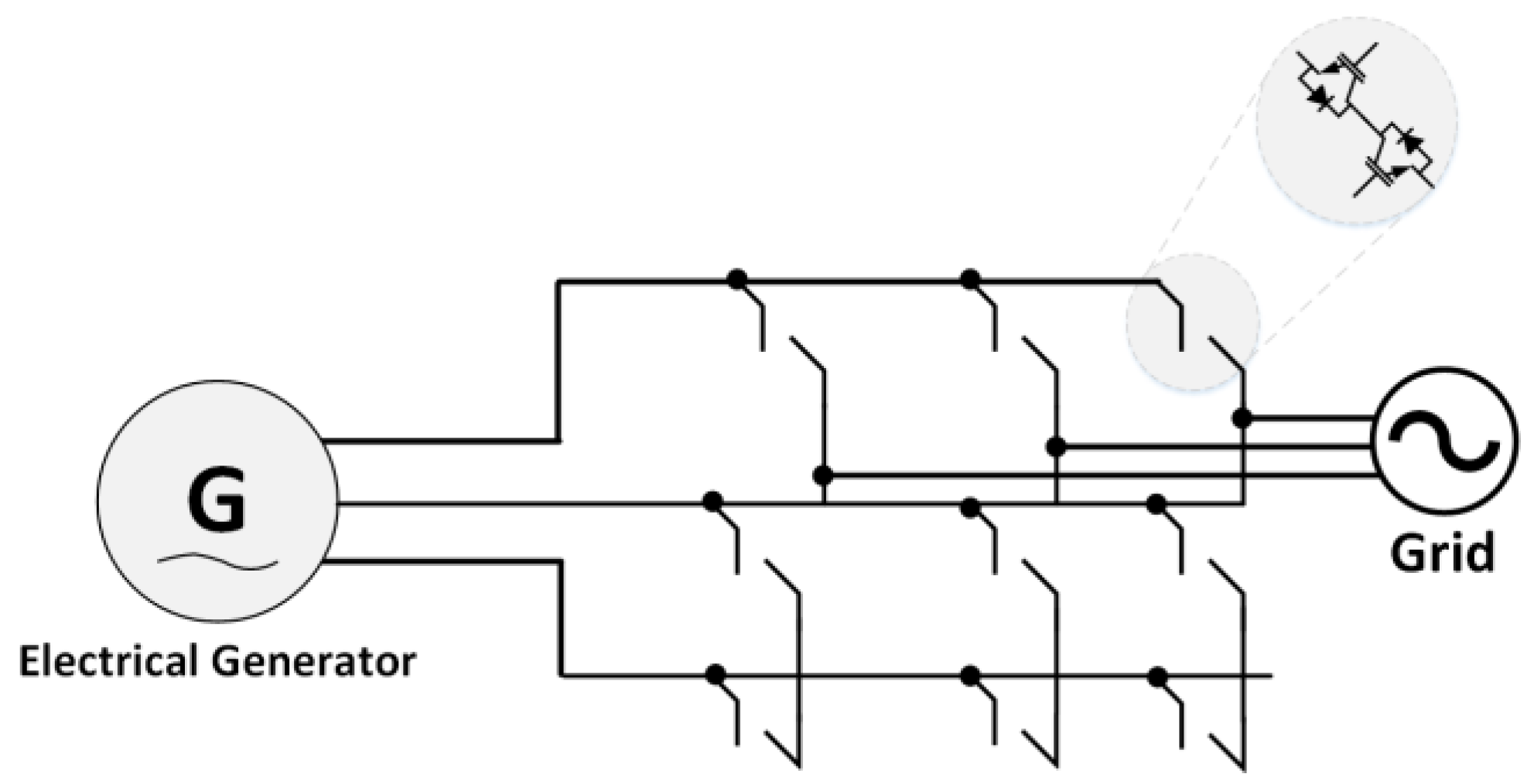
3.3. Matrix Converter
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- von Jouanne, A.; Brekken, T.K.A. Ocean and Geothermal Energy Systems. Proc. IEEE 2017, 105, 2147–2165. [Google Scholar] [CrossRef]
- Tousif, S.M.R.; Taslim, S.M.B. Producing electricity from geothermal energy. In Proceedings of the 2011 10th International Conference on Environment and Electrical Engineering, Rome, Italy, 8–11 May 2011; pp. 1–4. [Google Scholar]
- Burhanudin, J.; Abu Hasim, A.S.; Ishak, A.M.; Dardin, S.S. A Review of Power Electronics for Nearshore Wave Energy Converter Applications. IEEE Access 2022, 10, 16670–16680. [Google Scholar] [CrossRef]
- CC Energy. CETO. Available online: https://www.carnegiece.com/wave/ (accessed on 24 May 2019).
- Blaabjerg, F.; Liu, H.; Loh, P.C. Marine energy generation systems and related monitoring and control. IEEE Instrum. Meas. Mag. 2014, 17, 27–32. [Google Scholar] [CrossRef]
- IRENA. Future of Solar Photovoltaic: Deployment, Investment, Technology, Grid Integration and Socio-Economic Aspects (A Global Energy Transformation: Paper). 2019. Available online: https://www.irena.org/-/media/Files/IRENA/Agency/Publication/2019/Nov/IRENA_Future_of_Solar_PV_2019.pdf (accessed on 16 October 2022).
- IREA. Renewable Power Generation Costs in 2020. 2020. Available online: https://www.irena.org/publications/2021/Jun/Renewable-Power-Costs-in-2020 (accessed on 16 October 2022).
- Vining, J.G.; Muetze, A. Economic factors and incentives for ocean wave energy conversion. IEEE Trans. Ind. Appl. 2009, 45, 547–554. [Google Scholar] [CrossRef]
- Teillant, B.; Costello, R.; Weber, J.; Ringwood, J. Productivity and economic assessment of wave energy projects through operational simulations. Renew. Energy 2012, 48, 220–230. [Google Scholar] [CrossRef]
- DiPippo, R. Geothermal Power Plants, Principles, Applications, Case Studies and Environmental Impact, 2nd ed.; Elsevier: Butterworth-Heinemann, Oxford, UK, 2008. [Google Scholar]
- Schmela, M.; Döring, T. Global Market Outlook for Solar Power 2017–2021; Solar-Power Europe: Brussels, Belgium, 2017. [Google Scholar]
- REN21. Renewables 2021 Global Status Report. Available online: https://www.ren21.net/wp-content/uploads/2019/05/gsr_2020_full_report_en.pdf (accessed on 16 October 2022).
- Kai, L.Y.; Sarip, S.; Kaidi, H.M.; Ardila-Rey, J.A.; Samsuddin, N.M.; Muhtazaruddin, M.N.; Muhammad-Sukki, F.; Aziz, S.A. Current Status and Possible Future Applications of Marine Current Energy Devices in Malaysia: A Review. IEEE Access 2021, 9, 86869–86888. [Google Scholar] [CrossRef]
- Zhou, Z.; Scuiller, F.; Charpentier, J.F.; Benbouzid, M.; Tang, T. An up-to-date review of large marine tidal current turbine technologies. In Proceedings of the 2014 International Power Electronics and Application Conference and Exposition, Shanghai, China, 5–8 November 2014; pp. 480–484. [Google Scholar] [CrossRef]
- Chappell, B. Greece Says Its Entire Electrical Grid Ran on 100% Renewables for the First Time. Npr, 13 October 2022. Available online: https://www.npr.org/2022/10/13/1128737392/greece-renewable-energy-electrical-grid (accessed on 16 October 2022).
- Shek, J.K.H.; Macpherson, D.E.; Mueller, M.A. Power conversion for wave energy applications. In Proceedings of the 5th IET International Conference on Power Electronics, Machines and Drives (PEMD 2010), Brighton, UK, 19–21 April 2010; pp. 1–6. [Google Scholar] [CrossRef]
- IEA. Key World Energy statistics 2021. Available online: https://iea.blob.core.windows.net/assets/52f66a88-0b63-4ad2-94a5-29d36e864b82/KeyWorldEnergyStatistics2021.pdf (accessed on 16 October 2022).
- International Energy Agency-Ocean Energy Systems (IEA-OES) Annual Report. 2021. Available online: https://www.ocean-energy-systems.org/about-us/annual-report/ (accessed on 16 October 2022).
- Halamay, D.A.; Brekken, T.K.A.; Simmons, A.; McArthur, S. Reserve requirement impacts of large-scale integration of wind, solar, and ocean wave power generation. IEEE Trans. Sustain. Energy 2011, 2, 321–328. [Google Scholar] [CrossRef]
- García-Medina, G.; Özkan-Haller, H.T.; Ruggiero, P. Wave resource assessment in Oregon and southwest Washington, USA. Renew. Energy 2014, 64, 203–214. [Google Scholar] [CrossRef]
- Electric Power Research Institute. Mapping and Assessment of the United States Ocean Wave Energy Resource; Electric Power Research Institute: Washington, DC, USA, 2011; p. 1024637. Available online: https://www.osti.gov/servlets/purl/1060943 (accessed on 16 October 2022).
- Energy Information Administration. Annual Energy Outlook 2016 with Projections to 2040; Energy Information Administration: Washington, DC, USA, 2016. [Google Scholar]
- Gunn, K.; Stock-Williams, C. Quantifying the global wave power resource. Renew. Energy 2012, 44, 294–304. [Google Scholar] [CrossRef]
- Pelc, R.; Fujita, R.M. Renewable energy from the ocean. Mar. Policy 2022, 26, 471–479. [Google Scholar] [CrossRef]
- Grotelüschen, F. Scientists Race to Develop Floating Wind Farms. Deutsche Welle. Available online: http://www.dw.de/scientists-race-to-develop-floating-wind-farms/a-16540081 (accessed on 16 October 2022).
- Boake, C.B.; Whittaker, T.J.T.; Folley, M.; Ellen, H. Overview and initial operational experience of the LIMPET wave energy plant. In Proceedings of the Twelfth International Offshore and Polar Engineering Conference, Kitakyushu, Japan, 26–31 May 2002; Volume 3, p. 586594. [Google Scholar]
- De Falcão, A.F.O. Wave energy utilization: A review of the technologies. Renew. Sustain. Energy Rev. 2010, 14, 899–918. [Google Scholar] [CrossRef]
- Dalton, G.J.; Alcorn, R.; Lewis, T. Case study feasibility analysis of the pelamis wave energy convertor in Ireland, Portugal and North America. Renew. Energy 2010, 35, 443–455. [Google Scholar] [CrossRef]
- The European Commission. Islay Limpet Wave Power Plant; Queen’s University Belfast: Belfast, UK, 2002. Available online: https://tethys.pnnl.gov/sites/default/files/publications/Islay_LIMPET_Report.pdf (accessed on 16 October 2022).
- Penalba, M.; Ringwood, J.V. A Review of Wave-to-Wire Models for Wave Energy Converters. Energies 2016, 9, 506. [Google Scholar] [CrossRef]
- Amundarain, M.; Alberdi, M.; Garrido, A.J.; Garrido, I.; Maseda, J. Wave energy plants: Control strategies for avoiding the stalling behaviour in the Wells turbine. Renew. Energy 2010, 35, 2639–2648. [Google Scholar] [CrossRef]
- Carroll, C.; and Bell, M. Wave Energy Converters Utilizing Pressure Differences. U.S. Patent 6 933 623, 23 August 2005. [Google Scholar]
- Jarocki, D. Wave energy converter performance modeling and cost of electricity asessment. In Proceedings of the ASME 2010 International Mechanical Engineering Congress and Exposition, Vancouver, BC, Canada, 12–18 November 2010. [Google Scholar]
- Dewhurst, T.; Sivakumar, S. Wave-to-wire modeling and simulation of a wave energy converter for off-grid and micro-grid applications. In Proceedings of the OCEANS 2018 MTS/IEEE Charleston, Charleston, SC, USA, 22–25 October 2018; p. 18. [Google Scholar]
- Multon, B. Marine Renewable Energy Handbook; Wiley: New York, NY, USA, 2013. [Google Scholar]
- Drew, B.; Plummer, A.; Sahinkaya, M.N. A review of wave energy converter technology. Proc. Inst. Mech. Eng. A J. Power Energy 2009, 223, 887–902. [Google Scholar] [CrossRef]
- Edenhofer, O.; Pichs-Madruga, R.; Sokona, Y.; Seyboth, K.; Kadner, S.; Zwickel, T.; Hansen, G.; Schlömer, S.; von Stechow, C.; Matschoss, P.; et al. Renewable Energy Sources and Climate Change Mitigation: Special Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Delmonte, N.; Barater, D.; Giuliani, F.; Cova, P.; Buticchi, G. Review of Oscillating Water Column Converters. IEEE Trans. Ind. Appl. 2016, 52, 1698–1710. [Google Scholar] [CrossRef]
- Markets and Martekts. Wave Energy Converter Market by Technology; Market Research Report. Available online: https://www.marketsandmarkets.com/Market-Reports/wave-energy-market-217091216.html (accessed on 16 October 2022).
- Chen, Z.; Guerrero, J.M.; Blaabjerg, F. A review of the state of the art of power electronics for wind turbines. IEEE Trans. Power Electron. 2009, 24, 1859–1875. [Google Scholar] [CrossRef]
- Bediaga, I.; Mendizabal, X.; Arnaiz, A.; Munoa, J. Ball bearing damage detection using traditional signal processing algorithms. IEEE Instrum. Meas. Mag. 2013, 16, 20–25. [Google Scholar] [CrossRef]
- Darwish, A.; Abdelsalam, A.K.; Massoud, A.M.; Ahmed, S. Single phase grid connected curent source inverter: Mitigation of oscillating power effect on the grid current. In Proceedings of the IET Conference on Renewable Power Generation (RPG 2011), Edinburgh, UK, 6–8 September 2011; pp. 1–7. [Google Scholar] [CrossRef]
- Darwish, A.; Holliday, D.; Finney, S. Operation and control design of an input-series–input-parallel–output-series conversion scheme for offshore DC wind systems. IET Power Electron. 2017, 10, 2092–2103. [Google Scholar] [CrossRef]
- Darwish, A.; Elserougi, A.; Abdel-Khalik, A.S.; Ahmed, S.; Massoud, A.; Holliday, D.; Williams, B.W. A single-stage three-phase DC/AC inverter based on Cuk converter for PV application. In Proceedings of the 2013 7th IEEE GCC Conference and Exhibition (GCC), Doha, Qatar, 17–20 November 2013; pp. 384–389. [Google Scholar] [CrossRef]
- Blaabjerg, F.; Teodorescu, R.; Liserre, M.; Timbus, A.V. Overview of control and grid synchronization for distributed power generation systems. IEEE Trans. Ind. Electron. 2006, 53, 1398–1409. [Google Scholar] [CrossRef]
- Badawy, A.D. Current Source dc-dc and dc-ac Converters with Continuous Energy Flow. Ph.D. Thesis, University of Strathclyde, Glasgow, UK, 2015. [Google Scholar]
- Darwish, A.; Abdel-Khalik, A.; Elserougi, A.; Ahmed, S.; Massoud, A. Fault current contribution scenarios for grid-connected voltage source inverter-based distributed generation with an LCL filter. Electr. Power Syst. Res. 2013, 104, 93–103. [Google Scholar] [CrossRef]
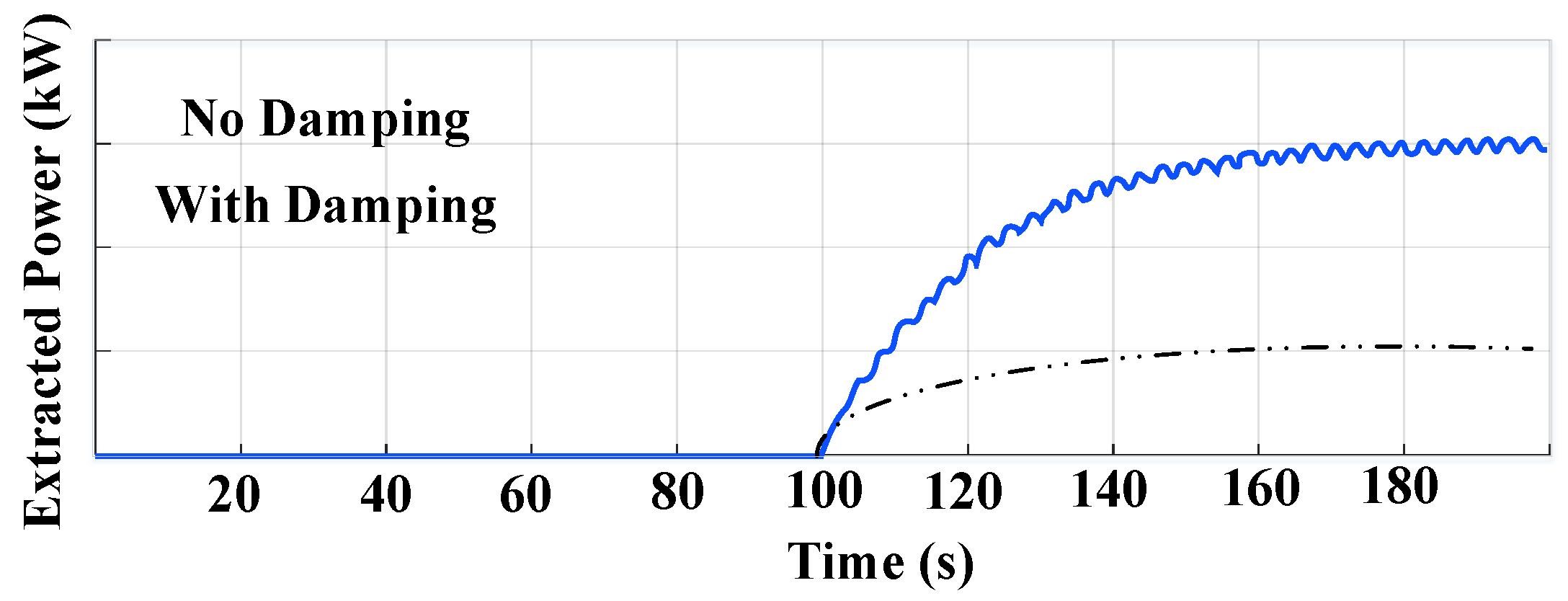
- Salter, S.H.; Taylor, J.R.M.; Caldwell, N.J. Power conversion mechanisms for wave energy. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2002, 216, 1–27. [Google Scholar] [CrossRef]
- EAmon, A.; Brekken, T.K.A.; Schacher, A.A. Maximum Power Point Tracking for Ocean Wave Energy Conversion. IEEE Trans. Ind. Appl. 2012, 48, 1079–1086. [Google Scholar] [CrossRef]
- Darwish, A.; Elgenedy, M.A. Current-Source Modular Medium-Voltage Grid-Connected System with High-Frequency Isolation for Photovoltaic Applications. IEEE Trans. Energy Convers. 2019, 34, 255–266. [Google Scholar] [CrossRef]
- Ibrahim, A.; Philip, A.G.; Derrick, H.; Williams, B.W. Modified back-to-back current source converter and its application to wind energy conversion systems. IET Power Electron. 2015, 8, 103–111, ISSN 1755-4535. [Google Scholar]
- Simonetti, D.S.L.; Amorim, A.E.A.; Oliveira, F.D.C. Chapter 15—Doubly Fed Induction Generator in Wind Energy Conversion Systems. In Advances in Renewable Energies and Power Technologies; Yahyaoui, I., Ed.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 461–490. [Google Scholar]
- Hazra, S.; Bhattacharya, S. Modeling and emulation of a rotating paddle typewave energy converter. IEEE Trans. Energy Convers. 2018, 33, 594–604. [Google Scholar] [CrossRef]
- M’zoughi, F.; Bouallegue, S.; Garrido, A.J.; Garrido, I.; Ayadi, M. Stalling-free control strategies for power generation plants. IEEE Trans. Energy Convers. 2018, 33, 209–222. [Google Scholar] [CrossRef]
- Zarei, M.E.; Ramirez, D.; Nicolas, C.V.; Arribas, J.R. Three-Phase Four-Switch Converter for SPMS Generators Based on Model Predictive Current Control for Wave Energy Applications. IEEE Trans. Power Electron. 2020, 35, 289–302. [Google Scholar] [CrossRef]
- McDonald, S.P.; Baker, N.J.; Espinoza, M.; Pickert, V. Power-takeoff topology comparison for a wave energy converter. J. Eng. 2019, 2019, 5012–5017. [Google Scholar] [CrossRef]
- McDonald, S.P.; Baker, N.J.; Pickert, V. High-frequency current source converter for a direct drive powertrain in a wave energy converter. J. Eng. 2019, 2019, 3629–3633. [Google Scholar] [CrossRef]
- Hillis, A.; Whitlam, C.; Brask, A.; Chapman, J.; Plummer, A. Active control for multi-degree-of-freedom wave energy converters with load limiting. Renew. Energy 2020, 159, 1177–1187. [Google Scholar] [CrossRef]
- Jama, M.; Wahyudie, A.; Mekhilef, S. Wave excitation force estimation using an electrical-based extended Kalman lter for point absorber wave energy converters. IEEE Access 2020, 8, 49823–49836. [Google Scholar] [CrossRef]
- Hasanien, H.M. Transient Stability Augmentation of a Wave Energy Conversion System Using a Water Cycle Algorithm-Based Multiobjective Optimal Control Strategy. IEEE Trans. Ind. Inform. 2019, 15, 3411–3419. [Google Scholar] [CrossRef]
- Zarei, M.E.; Ramirez, D.; Veganzones, C.; Rodriguez, J. Predictive Direct Control of SPMS Generators Applied to the Machine Side Converter of an OWC Power Plant. IEEE Trans. Power Electron. 2020, 35, 6719–6731. [Google Scholar] [CrossRef]
- Ramirez, D.; Mendonça, H.; Blanco, M.; Martinez, F. Non-linear vector current source for the control of permanent magnet synchronous generators in wave energy applications. IET Renew. Power Gener. 2019, 13, 2409–2417. [Google Scholar] [CrossRef]
- Huang, W.; Yang, J. A novel piecewise velocity control method using passivity-based controller for wave energy conversion. IEEE Access 2020, 8, 59029–59043. [Google Scholar] [CrossRef]
- Oh, Y.J.; Park, J.S.; Hyon, B.J.; Lee, J. Novel Control Strategy of Wave Energy Converter Using Linear Permanent Magnet Synchronous Generator. IEEE Trans. Appl. Supercond. 2018, 28, 26. [Google Scholar] [CrossRef]
- O’Sullivan, A.C.M.; Sheng, W.; Lightbody, G. An Analysis of the Potential Benefits of Centralised Predictive Control for Optimal Electrical Power Generation from Wave Energy Arrays. IEEE Trans. Sustain. Energy 2018, 9, 1761–1771. [Google Scholar] [CrossRef]
- Andrade, D.; Jaen, A.G.S.l. Considering linear generator copper losses on model predictive control for a point absorber wave energy converter. Energy Convers. Manag. 2014, 78, 173–183. [Google Scholar] [CrossRef]
- De La Villa-Jaén, A.; El Montoya-Andrade, D.; García-Santana, A. Control strategies for point absorbers considering linear generator copper losses and maximum excursion constraints. IEEE Trans. Sustain. Energy 2018, 9, 433–442. [Google Scholar] [CrossRef]
- Kazmierkowski, M.P.; Jasinski, M. Power electronics for renewable sea wave energy. In Proceedings of the 2010 12th International Conference on Optimization of Electrical and Electronic Equipment, Brasov, Romania, 20–22 May 2010. [Google Scholar]
- Islam, R.; Guo, Y.; Zhu, J. A High-Frequency Link Multilevel Cascaded Medium-Voltage Converter for Direct Grid Integration of Renewable Energy Systems. IEEE Trans. Power Electron. 2014, 29, 4167–4182. [Google Scholar] [CrossRef]
- Darwish, A.; Alotaibi, S.; Elgenedy, M.A. Current-Source Single-Phase Module Integrated Inverters for PV Grid-Connected Applications. IEEE Access 2020, 8, 53082–53096. [Google Scholar] [CrossRef]
- Niroomand, M.; Esfahani, F.N. Converter technologies for PV systems: A comprehensive review. In Energy Conversion Systems: An Overview; Nova Science Publishers: Hauppauge, NY, USA, 2021. [Google Scholar]
- Islam, R.; Mahfuz-Ur-Rahman, A.M.; Muttaqi, K.M.; Sutanto, D. State-of-the-Art of the Medium-Voltage Power Converter Technologies for Grid Integration of Solar Photovoltaic Power Plants. IEEE Trans. Energy Convers. 2019, 34, 372–384. [Google Scholar] [CrossRef]
- Bughneda, A.; Salem, M.; Richelli, A.; Ishak, D.; Alatai, S. Review of Multilevel Inverters for PV Energy System Applications. Energies 2021, 14, 1585. [Google Scholar] [CrossRef]
- Zhang, X.; Zhao, T.; Mao, W.; Tan, D.; Chang, L. Multilevel Inverters for Grid-Connected Photovoltaic Applications: Examining Emerging Trends. IEEE Power Electron. Mag. 2018, 5, 32–41. [Google Scholar] [CrossRef]
- Alotaibi, S.; Darwish, A. Modular Multilevel Converters for Large-Scale Grid-Connected Photovoltaic Systems: A Review. Energies 2021, 14, 6213. [Google Scholar] [CrossRef]
- Noman, A.M.; Addoweesh, K.E.; Alabduljabbar, A.A.; Alolah, A.I. Cascaded H-Bridge MLI and Three-Phase Cascaded VSI Topologies for Grid-Connected PV Systems with Distributed MPPT. Int. J. Photoenergy 2019, 2019, 7642919. [Google Scholar] [CrossRef]
- Stynski, S.; Grzegorczyk, M.; Sobol, C.; Kot, R. Current Control Strategies for a Star Connected Cascaded H-Bridge Converter Operating as MV-AC to MV-DC Stage of a Solid State Transformer. Energies 2021, 14, 4607. [Google Scholar] [CrossRef]
- Lumbreras, D.; Barrios, E.L.; Balda, J.; Navarrete, M.; Gonzalez, R.; Sanchis, P. Medium-Voltage Cascaded Sequential Topology for Large-Scale PV Plants. IEEE Access 2021, 9, 130601–130614. [Google Scholar] [CrossRef]
- Gunsal, I.; Stone, D.A.; Foster, M.P. Suppressing Leakage Current for Cascaded H-Bridge Inverters in Renewable Energy and Storage Systems. IEEE Trans. Ind. Electron. 2021, 68, 11035–11043. [Google Scholar] [CrossRef]
- Amaral, F.V.; Parreiras, T.M.; Lobato, G.C.; Machado, A.A.P.; Pires, I.A.; Filho, B.D.J.C. Operation of a Grid-Tied Cascaded Multilevel Converter Based on a Forward Solid-State Transformer Under Unbalanced PV Power Generation. IEEE Trans. Ind. Appl. 2018, 54, 5493–5503. [Google Scholar] [CrossRef]
- Muller, N.; Kouro, S.; Malinowski, M.; Rojas, C.A.; Jasinski, M.; Estay, G. Medium-Voltage Power Converter Interface for Multigenerator Marine Energy Conversion Systems. IEEE Trans. Ind. Electron. 2017, 64, 1061–1070. [Google Scholar] [CrossRef]
- Müller, N.; Kouro, S.; Malinowski, M.; Rivera, S.; Wu, B. Cascaded H-bridge multilevel converter interface for Wave Dragon energy conversion system. In Proceedings of the IECON 2013-39th Annual Conference of the IEEE Industrial Electronics Society, Vienna, Austria, 10–13 November 2013; pp. 6201–6206. [Google Scholar] [CrossRef]
- Todorčević, T.; van Kessel, R.; Bauer, P.; Ferreira, J.A. A Modulation Strategy for Wide Voltage Output in DAB-Based DC–DC Modular Multilevel Converter for DEAP Wave Energy Conversion. IEEE J. Emerg. Sel. Top. Power Electron. 2013, 3, 1171–1181. [Google Scholar] [CrossRef]
- Khwan-On, S.; De Lillo, L.; Empringham, L.; Wheeler, P.; Gerada, C. Fault-tolerant, matrix converter, permanent magnet synchronous motor drive for open-circuit failures. IET Electr. Power Appl. 2011, 5, 654–667. [Google Scholar] [CrossRef]
- Lee, M.J.; Wheeler, P.; Klumpner, C. Space-vector modulated multilevel matrix converter. IEEE Trans. Ind. Electron. 2010, 57, 3385–3394. [Google Scholar] [CrossRef]
- Muller, S.; Deicke, M.; De Doncker, R. Doubly fed induction generator systems for wind turbines. IEEE Ind. Appl. Mag. 2002, 8, 26–33. [Google Scholar] [CrossRef]
- Alotaibi, S.; Darwish, A.; Ma, X.; Williams, B.W. A new modular three-phase inverter based on sepic-cuk combination converter for photovoltaic applications. In Proceedings of the 9th Renewable Power Generation Conference (RPG Dublin Online 2021), Online Conference, 1–2 March 2021; pp. 259–264. [Google Scholar] [CrossRef]








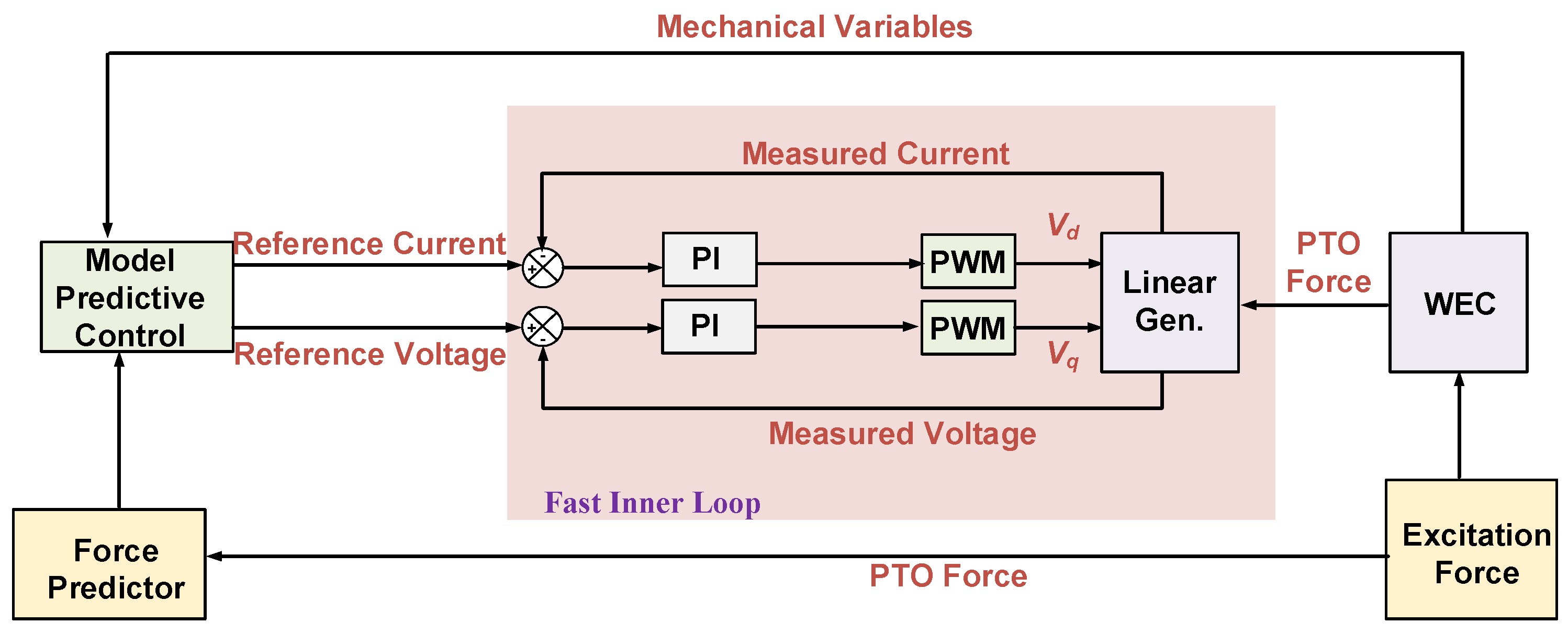

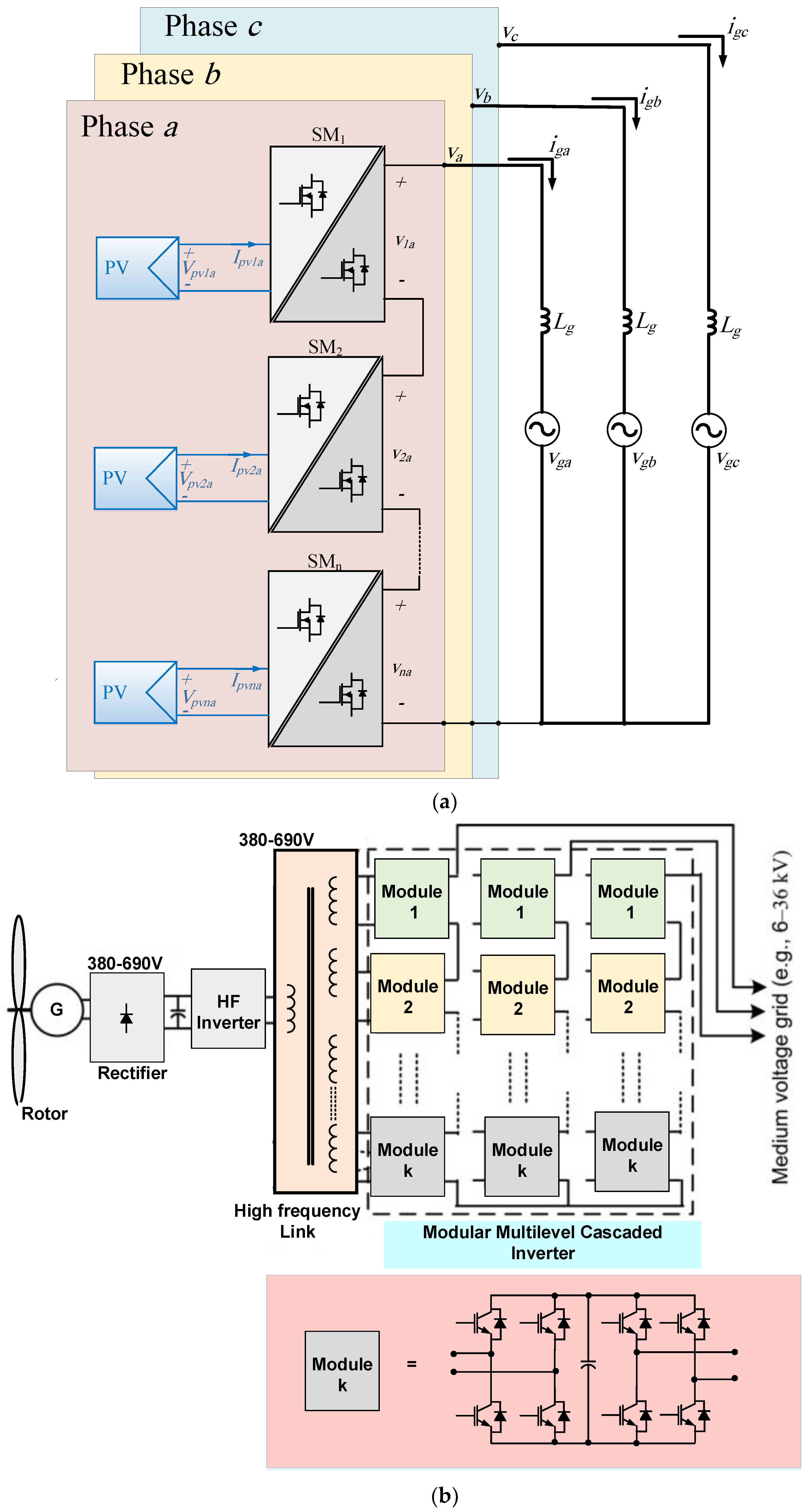
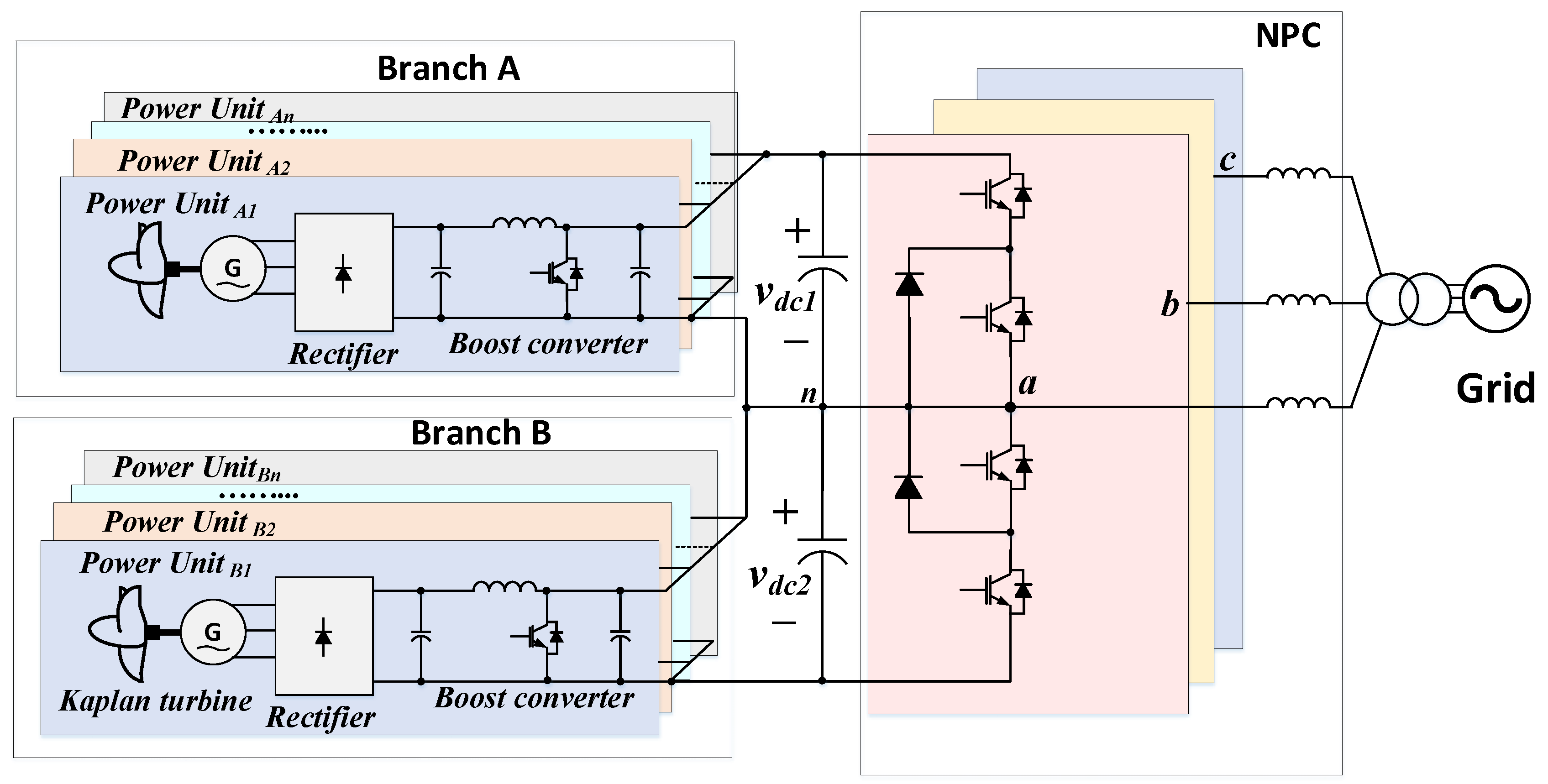
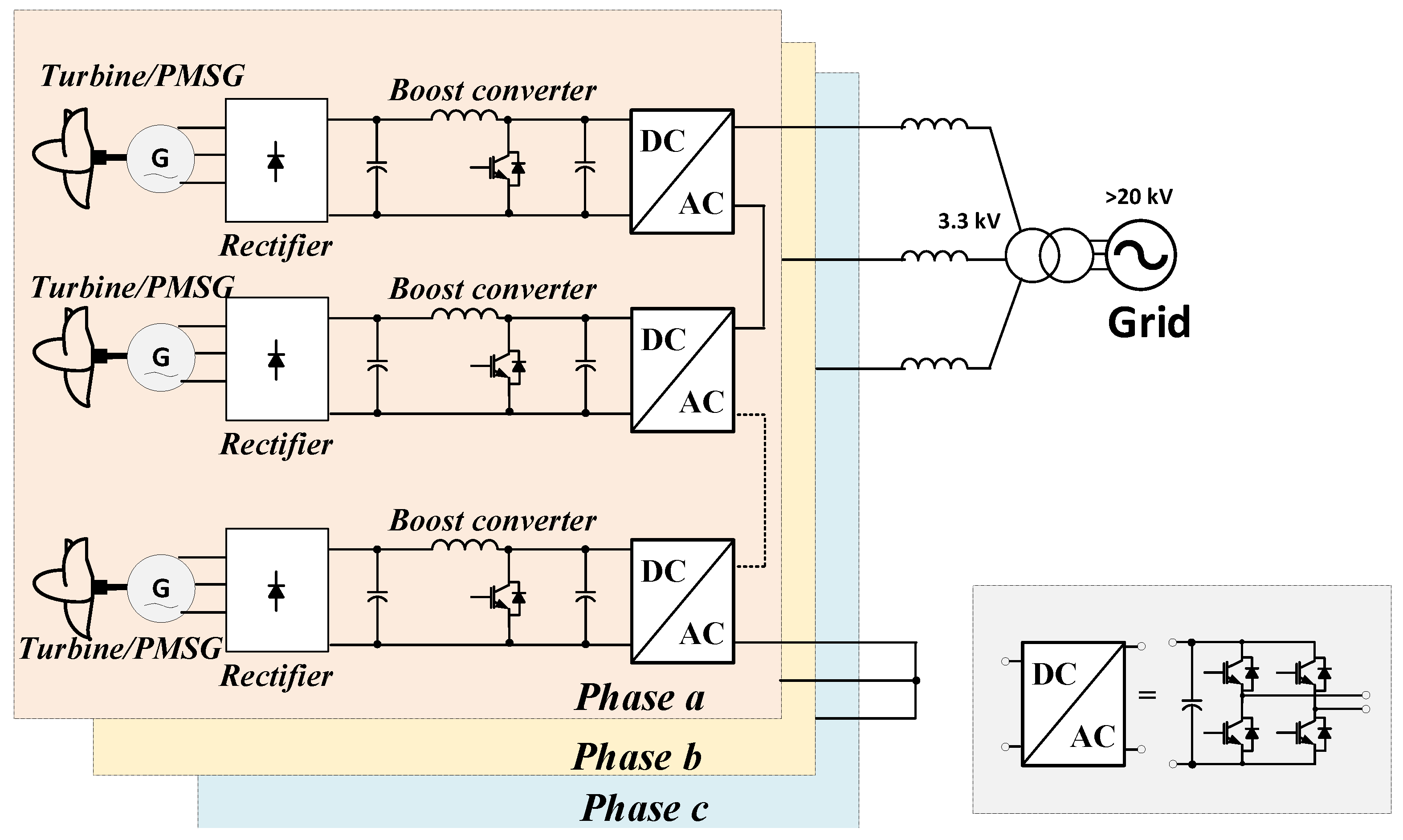
| Ref. | Contribution | Remarks |
|---|---|---|
| [53] |
|
|
| [54] |
|
|
| [55] |
|
|
| [56] |
|
|
| [57] |
|
|
| [58] |
|
|
| [59] |
|
|
| [60] |
|
|
| [61] |
|
|
| [62] |
|
|
| [63] |
|
|
| [64] |
|
|
| [65] |
|
|
| [66] |
|
|
| [67] |
|
|
| Ref. | Contribution | Remarks |
|---|---|---|
| [81] |
|
|
| [82] |
|
|
| [83] |
|
|
| Ref | WEC Type | Electric Generator | Power Converter | Control Algorithm |
|---|---|---|---|---|
| [53] | OWC | PMSG | AC/DC/AC | Open-loop Control |
| [54] | OWC | DFIG | AC/DC/AC | Two-loop PI Control |
| [55] | OWC | PMSG | AC/DC/AC | Model Predictive Current Control |
| [56] | OWC | PMLG | Current source back-to-back | Not considered |
| [57] | OWC | PMLG | Current source back-to-back | Open-loop based on a new SVM |
| [58] | WAB | Not considered | AC/DC/AC | Multi-degree-of-freedom active control |
| [59] | WAB | PMLG | AC/DC/AC | Electrical-based extended Kalman filter |
| [60] | WAB | PMSG | AC/DC/AC | Two-loop PI Control |
| [61] | OWC | PMSG | AC/DC/AC | Predictive Direct Control |
| [62] | OWC | PMSG | AC/DC/AC | Two-loop PI Control |
| [63] | WAB | PMLG | AC/DC | Piecewise Velocity Control |
| [64] | WAB | PMSG | AC/DC/AC | Damping control strategy |
| [65] | WAB | PMLG | AC/DC/AC | Model predictive control |
| [66] | OT | PMLG | AC/DC/AC | Model predictive control |
| [67] | OT | PMLG | AC/DC/AC | Model predictive control |
| [81] | OT | PMSG | Modular back-to-back voltage source converter | Two-loop PI Control |
| [82] | OT | PMSG | Modular Cascaded H-bridge | Two-loop PI Control |
| [83] | DEAP | Not considered | Modular DAB | PI Control |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Darwish, A.; Aggidis, G.A. A Review on Power Electronic Topologies and Control for Wave Energy Converters. Energies 2022, 15, 9174. https://doi.org/10.3390/en15239174
Darwish A, Aggidis GA. A Review on Power Electronic Topologies and Control for Wave Energy Converters. Energies. 2022; 15(23):9174. https://doi.org/10.3390/en15239174
Chicago/Turabian StyleDarwish, Ahmed, and George A. Aggidis. 2022. "A Review on Power Electronic Topologies and Control for Wave Energy Converters" Energies 15, no. 23: 9174. https://doi.org/10.3390/en15239174
APA StyleDarwish, A., & Aggidis, G. A. (2022). A Review on Power Electronic Topologies and Control for Wave Energy Converters. Energies, 15(23), 9174. https://doi.org/10.3390/en15239174