Analysis of Controlled Driving and Spreading Behavior of Molten Pool in Cold Metal Transfer
Abstract
:1. Introduction
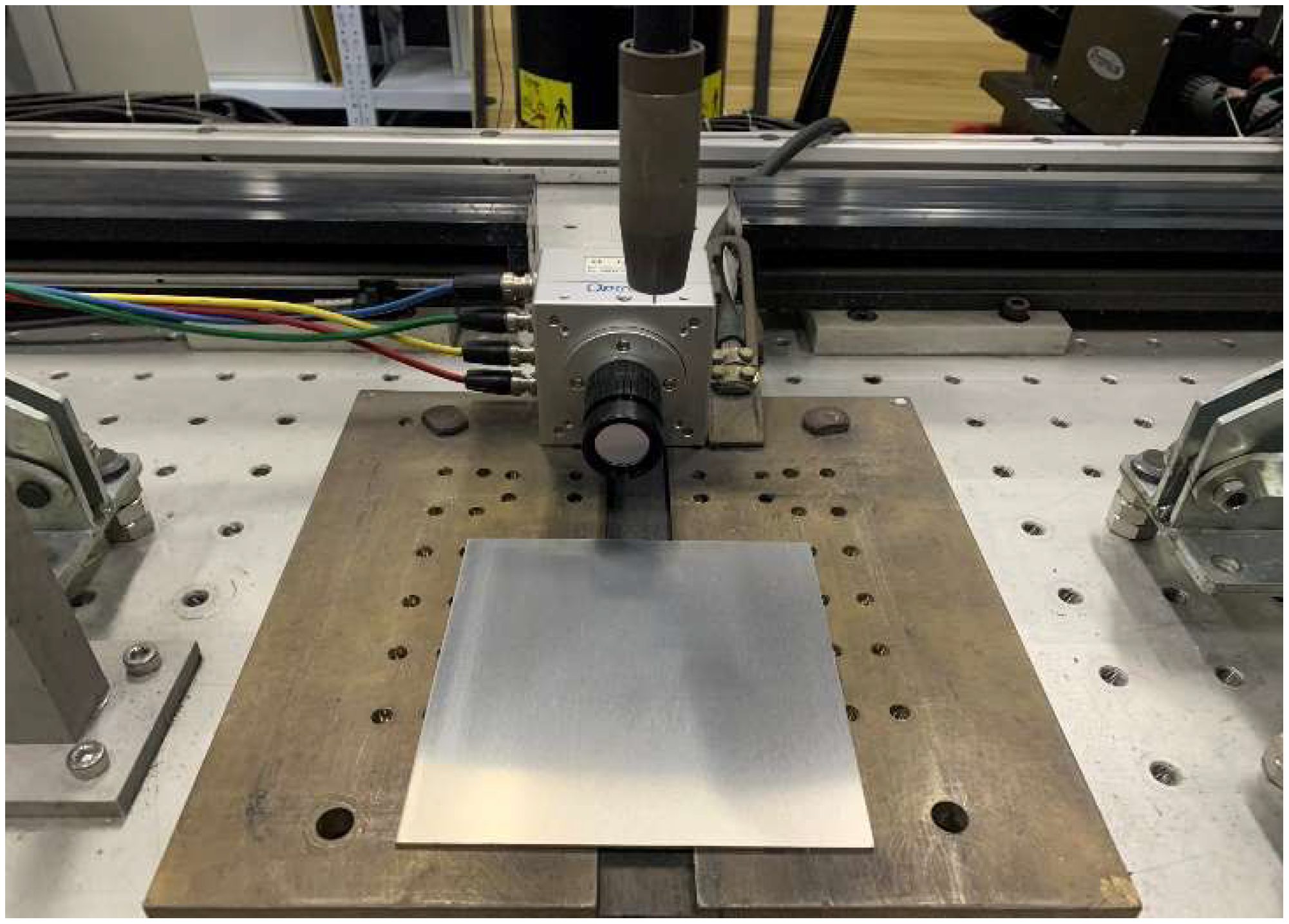
2. Materials and Methods
3. Analytical Model of Controlled Driving and Spreading
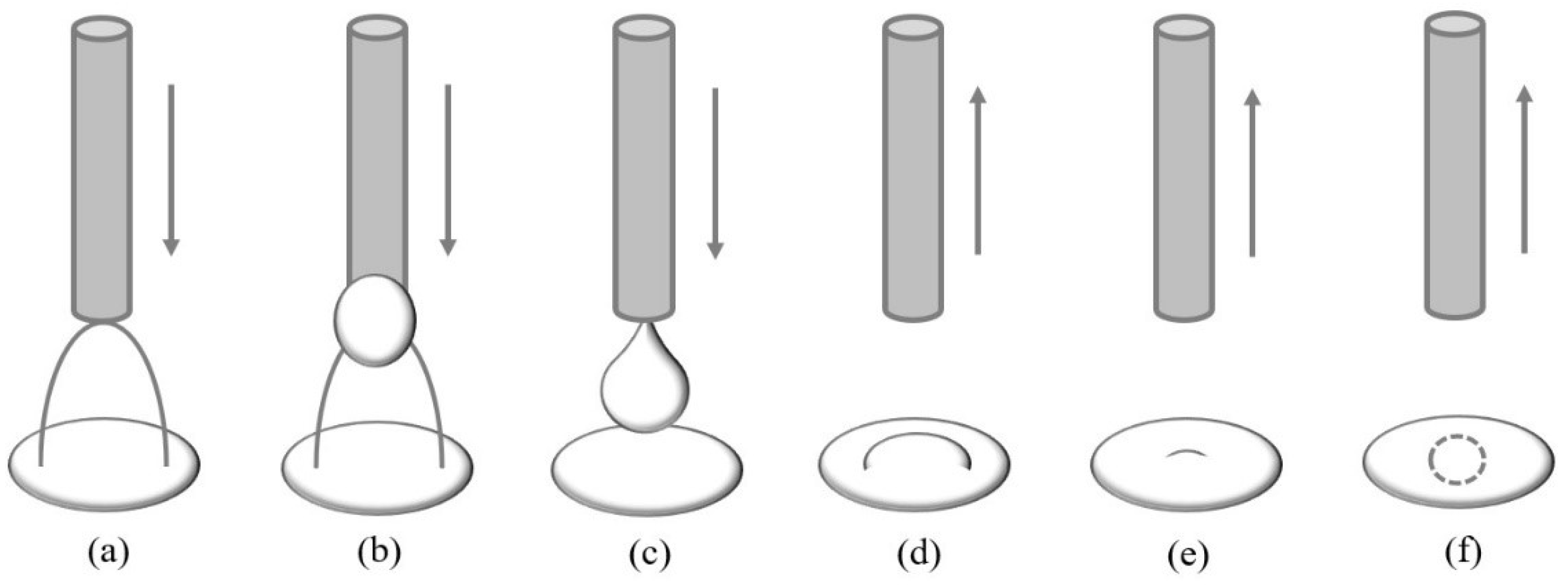
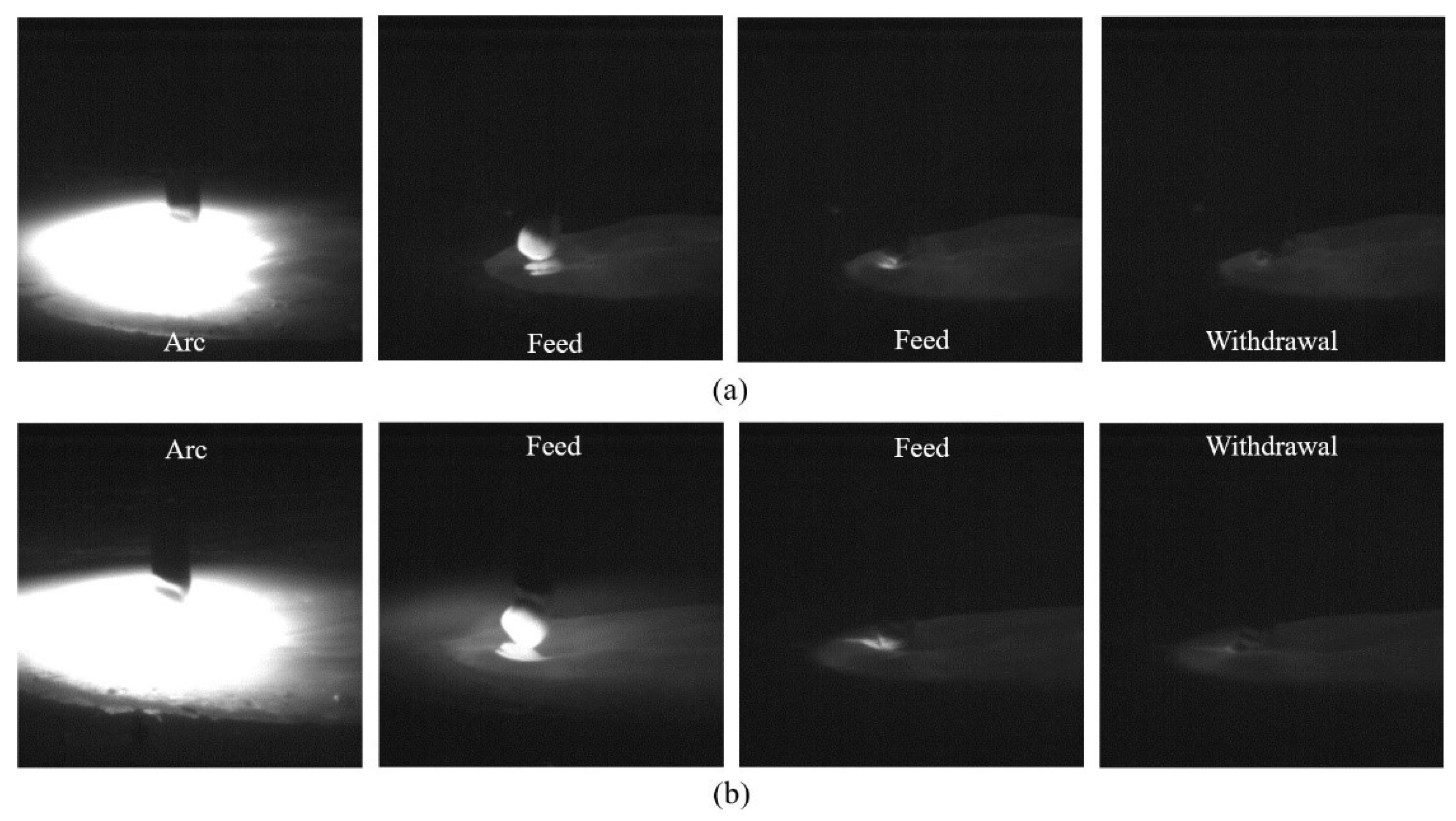
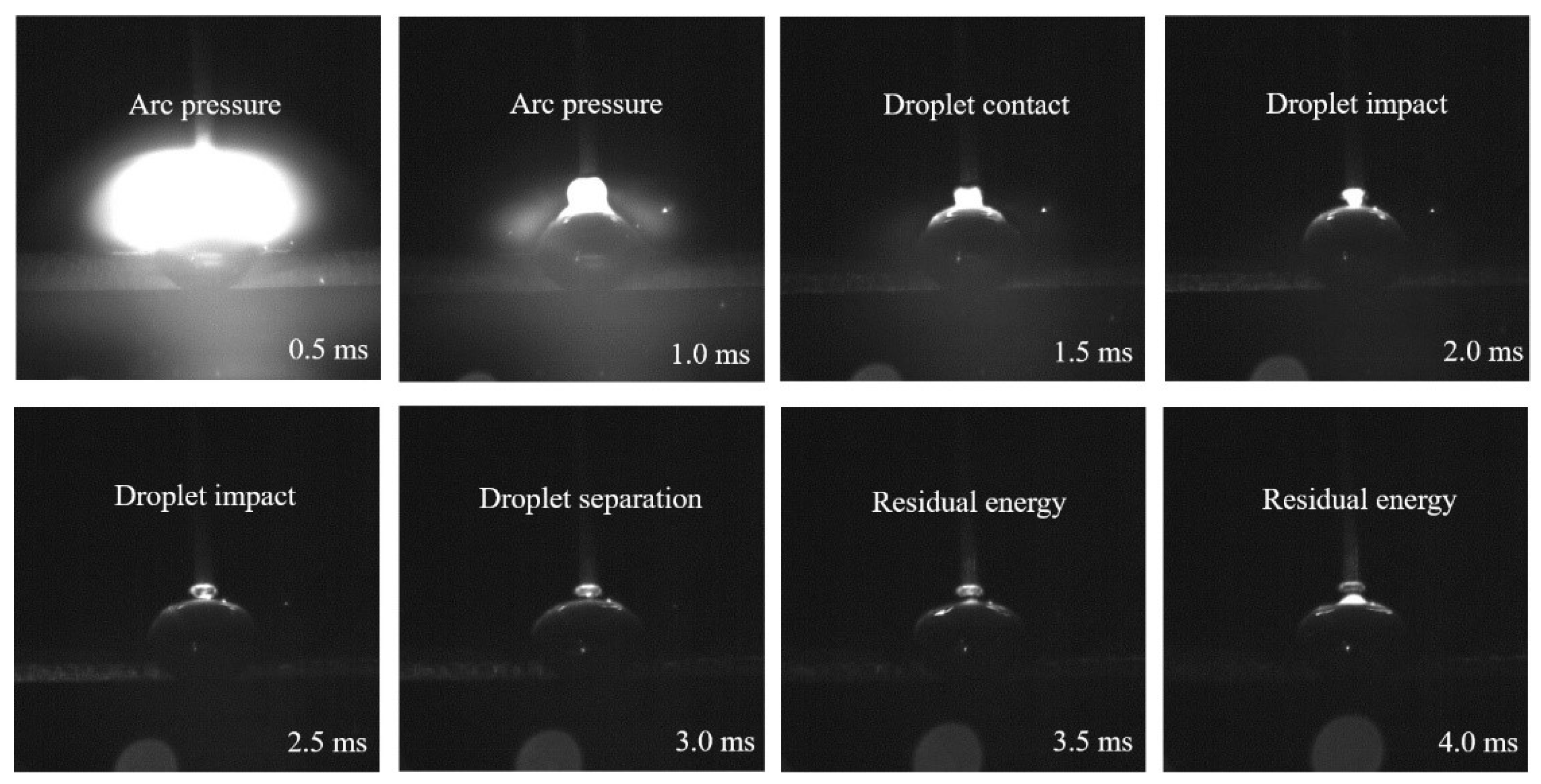
3.1. Controlled Driving and Spreading Process
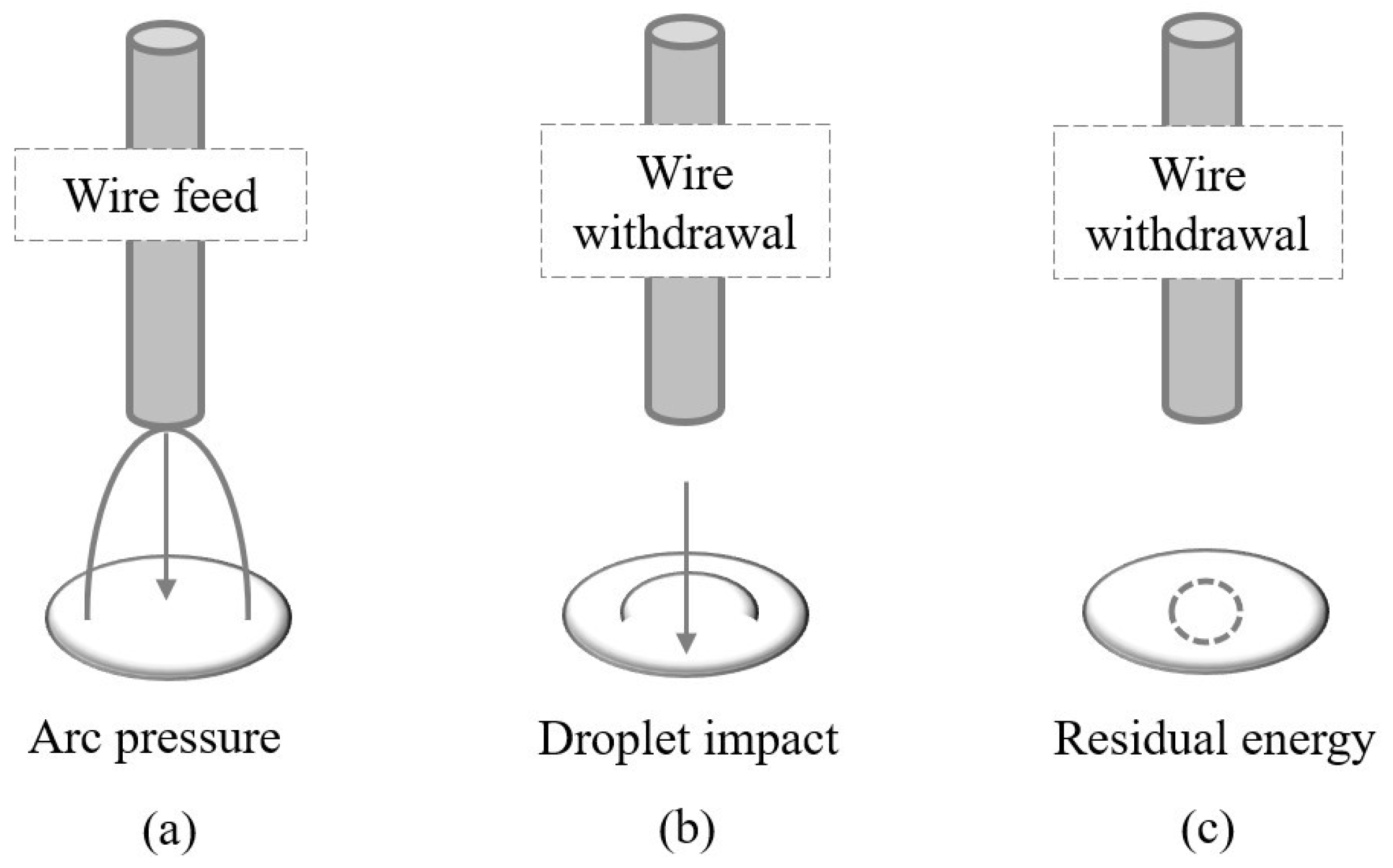
3.2. Arc Pressure Drive on the Surface of the Molten Pool
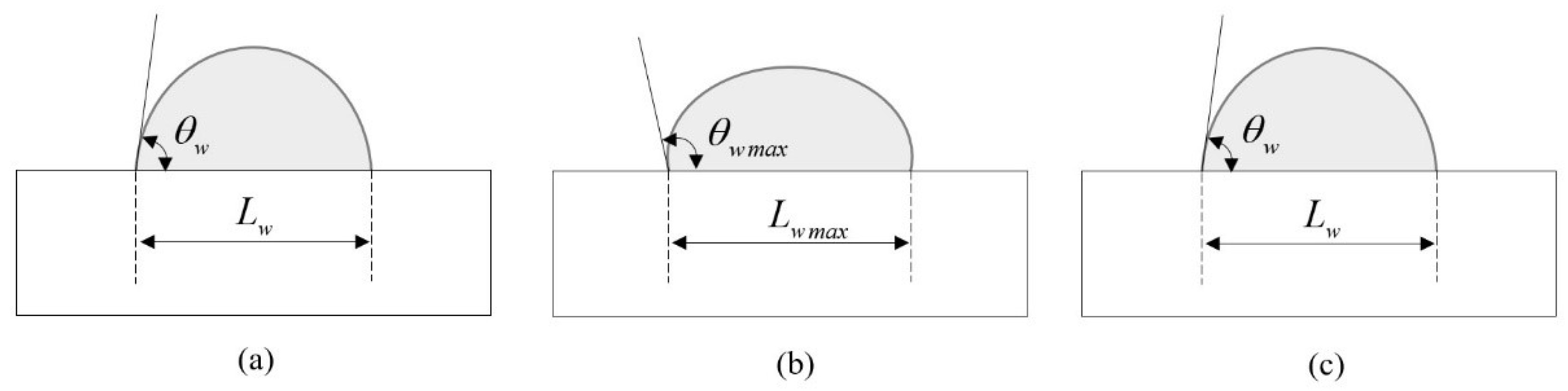
3.3. Droplet Impact
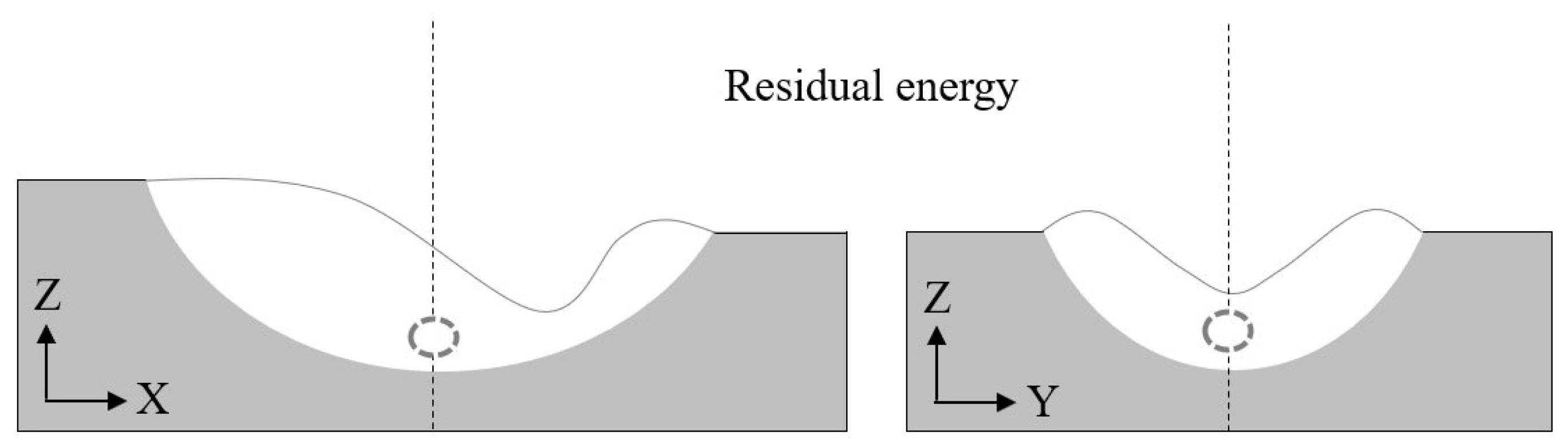
3.4. Residual Energy Transfer
3.5. Theoretical Model of Controlled Drive and Spreading
4. Analysis and Discussion
4.1. Molten Pool Transition Method
4.2. The Controlled Driving Process of the Molten Pool
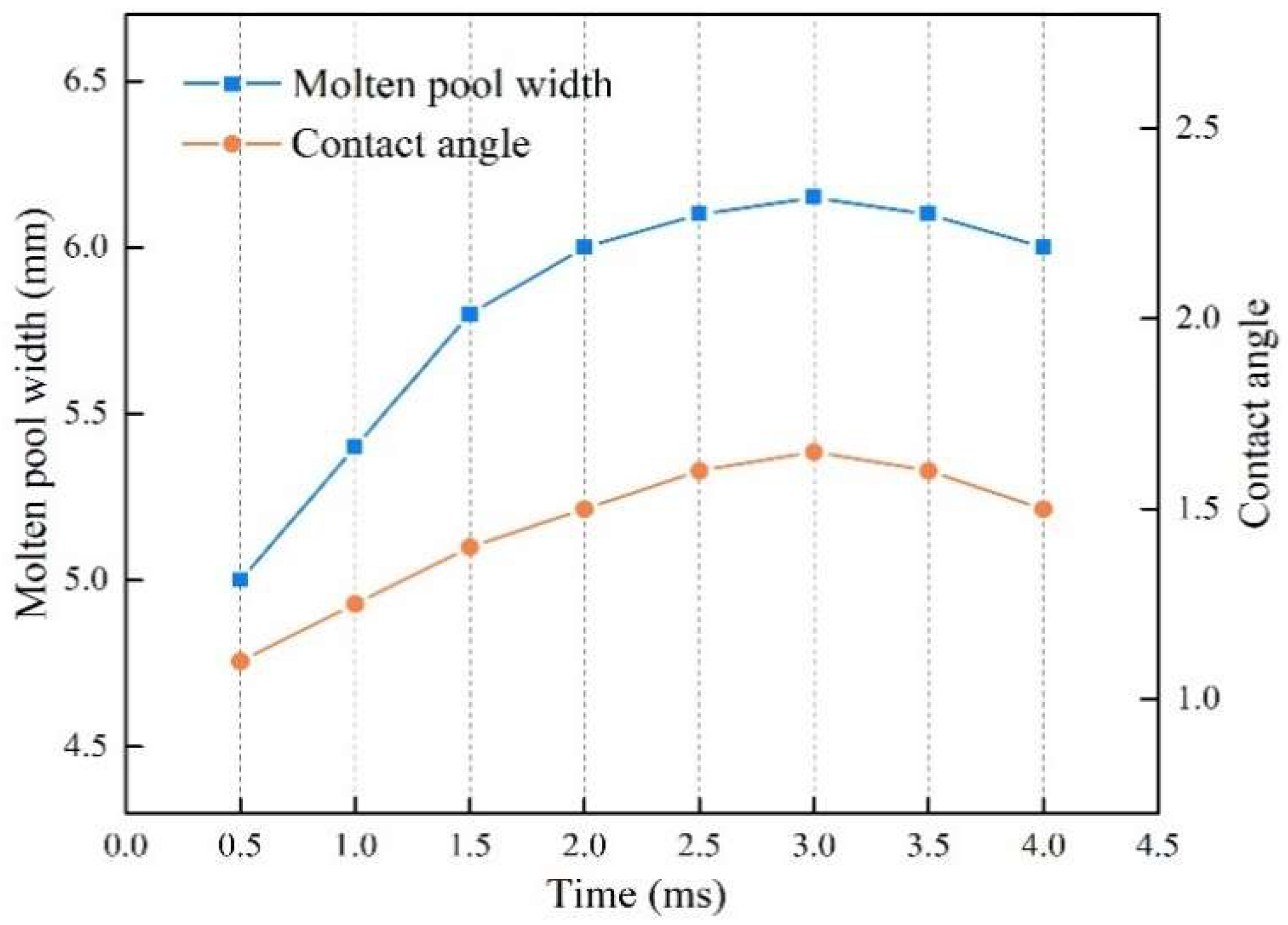
4.3. Controlled Driving and Spreading Process
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wu, F.; Falch, K.; Guo, D.; English, P.; Drakopoulos, M.; Mirihanage, W. Time evolved force domination in arc weld pools. Mater. Des. 2020, 190, 108534. [Google Scholar] [CrossRef]
- Shi, Y.; Li, C.; Du, L.; Gu, Y.; Zhu, M. Frequency characteristics of weld pool oscillation in pulsed gas tungsten arc welding. J. Manuf. Processes 2016, 24, 145–151. [Google Scholar]
- Meng, X.; Qin, G.; Zou, Z. Characterization of molten pool behavior and humping formation tendency in high-speed gas tungsten arc welding. Int. J. Heat Mass Transf. 2018, 117, 508–516. [Google Scholar] [CrossRef]
- Cai, J.; Feng, Y.; Zhou, J.; Li, Y.; Zhang, X.; Wu, C. Numerical analysis of weld pool behaviors in plasma arc welding with the lattice Boltzmann method. Int. J. Therm. Sci. 2018, 124, 447–458. [Google Scholar] [CrossRef]
- Huang, J.; Pan, W.; Chen, J.; Shao, Y.; Yang, M.; Zhang, Y. The transient behaviours of free surface in a fully penetrated weld pool in gas tungsten arc welding. J. Manuf. Processes 2018, 36, 405–416. [Google Scholar] [CrossRef]
- Liu, X.; Wu, C.; Jia, C.; Zhang, G. Visual sensing of the weld pool geometry from the topside view in keyhole plasma arc welding. J. Manuf. Processes 2017, 26, 74–83. [Google Scholar] [CrossRef]
- Nguyen, A.; Tashiro, S.; Ngo, M.; Bui, H.; Tanaka, M. Effect of the eddies formed inside a weld pool on welding defects during plasma keyhole arc welding. J. Manuf. Processes 2020, 59, 649–657. [Google Scholar] [CrossRef]
- Li, Y.; Tian, S.; Wu, C.; Tanaka, M. Experimental sensing of molten flow velocity, weld pool and keyhole geometries in ultrasonic-assisted plasma arc welding. J. Manuf. Processes 2021, 64, 1412–1419. [Google Scholar] [CrossRef]
- Wu, D.; Tashiro, S.; Hua, X.; Tanaka, M. Analysis of the energy propagation in the keyhole plasma arc welding using a novel fully coupled plasma arc-keyhole-weld pool model. Int. J. Heat Mass Transf. 2019, 141, 604–614. [Google Scholar] [CrossRef]
- Ning, J.; Zhang, L.; Zhang, L.; Long, J.; Yin, X.; Zhang, J.; Na, S. Effects of power modulation on behaviours of molten pool and keyhole during laser–arc hybrid welding of pure copper. Mater. Des. 2020, 194, 108829. [Google Scholar] [CrossRef]
- Wu, D.; Nguyen, A.; Tashiro, S.; Hua, X.; Tanaka, M. Elucidation of the weld pool convection and keyhole formation mechanism in the keyhole plasma arc welding. Int. J. Heat Mass Transf. 2019, 131, 920–931. [Google Scholar] [CrossRef]
- Yang, M.; Liu, H.; Qi, B. The surface depression and temperatures in molten pool with pulsed arc welding. J. Manuf. Processes 2019, 37, 130–138. [Google Scholar] [CrossRef]
- Meng, X.; Qin, G.; Zou, Z. Sensitivity of driving forces on molten pool behavior and defect formation in high-speed gas tungsten arc welding. Int. J. Heat Mass Transf. 2017, 107, 1119–1128. [Google Scholar] [CrossRef]
- Meng, X.; Qin, G. A theoretical study of molten pool behavior and humping formation in full penetration high-speed gas tungsten arc welding. Int. J. Heat Mass Transf. 2019, 132, 143–153. [Google Scholar] [CrossRef]
- Wang, D.; Lu, H. Numerical analysis of internal flow of molten pool in pulsed gas tungsten arc welding using a fully coupled model with free surface. Int. J. Heat Mass Transf. 2021, 165, 120572. [Google Scholar] [CrossRef]
- Chen, C.; Wei, X.; Zhao, Y.; Yan, K.; Jia, Z.; He, Y. Effects of helium gas flow rate on arc shape, molten pool behavior and penetration in aluminum alloy DCEN TIG welding. J. Mater. Process. Technol. 2018, 255, 696–702. [Google Scholar] [CrossRef]
- Eda, S.; Ogino, Y.; Asai, S.; Hirata, Y. Numerical study of the influence of gap between plates on weld pool formation in arc spot welding process. Weld. World 2018, 62, 1021–1030. [Google Scholar] [CrossRef]
- Zong, R.; Chen, J.; Wu, C. A comparison of TIG-MIG hybrid welding with conventional MIG welding in the behaviors of arc, droplet and weld pool. J. Mater. Process. Technol. 2019, 270, 345–355. [Google Scholar] [CrossRef]
- Murphy, A.; Thomas, D. Prediction of arc, weld pool and weld properties with a desktop computer model of metal–inert-gas welding. Weld. World 2017, 61, 623–633. [Google Scholar] [CrossRef]
- Ikram, A.; Chung, H. Numerical simulation of arc, metal transfer and its impingement on weld pool in variable polarity gas metal arc welding. J. Manuf. Processes 2021, 64, 1529–1543. [Google Scholar] [CrossRef]
- Cho, W.; Na, S. Impact of driving forces on molten pool in gas metal arc welding. Weld. World 2021, 65, 1735–1747. [Google Scholar] [CrossRef]
- Komen, H.; Shigeta, M.; Tanaka, M. Numerical simulation of molten metal droplet transfer and weld pool convection during gas metal arc welding using incompressible smoothed particle hydrodynamics method. Int. J. Heat Mass Transf. 2018, 121, 978–985. [Google Scholar] [CrossRef]
- Wang, Y.; Tsai, H. Effect of surface active elements on weld pool fluid flow and weld penetration in gas metal arc welding. Metall. Mater. Trans. B 2001, 32, 501–515. [Google Scholar] [CrossRef]
- Kim, C.; Zhang, W.; Debroy, T. Modeling of temperature field and solidified surface profile during gas metal arc fillet welding. J. Appl. Phys. 2003, 94, 2667–2669. [Google Scholar] [CrossRef] [Green Version]
- Esser, W.; Walter, R. Heat transfer and penetration mechanisms with GMA and plasma-GMA welding. Weld. J. 1981, 60, 37–42. [Google Scholar]
- Ushio, M.; Wu, C. Mathematical modeling of three-dimensional heat and fluid flow in a moving gas metal arc weld pool. Metall. Mater. Trans. B 1997, 28, 509–516. [Google Scholar] [CrossRef]
- Tsao, K.; Wu, C. Fluid flow and heat transfer in GMA weld pools. Weld. J. 1988, 67, 70–75. [Google Scholar]
- Kim, Y.; Eagar, T. Analysis of metal transfer in gas metal arc welding. Weld. J. 1993, 72, 269–277. [Google Scholar]
- Ghosh, K. Chemical Engineering. In ASM Handbook of Engineering Mathematics; ASM: Geauga County, OH, USA, 1983; Volume 90, p. 75. [Google Scholar]
- Ketter, R.; Pravel, S. Modern Methods of Engineering Computation; McGraw-Hill Book Company: New York, NY, USA, 1971; Volume 66, p. 660. [Google Scholar]






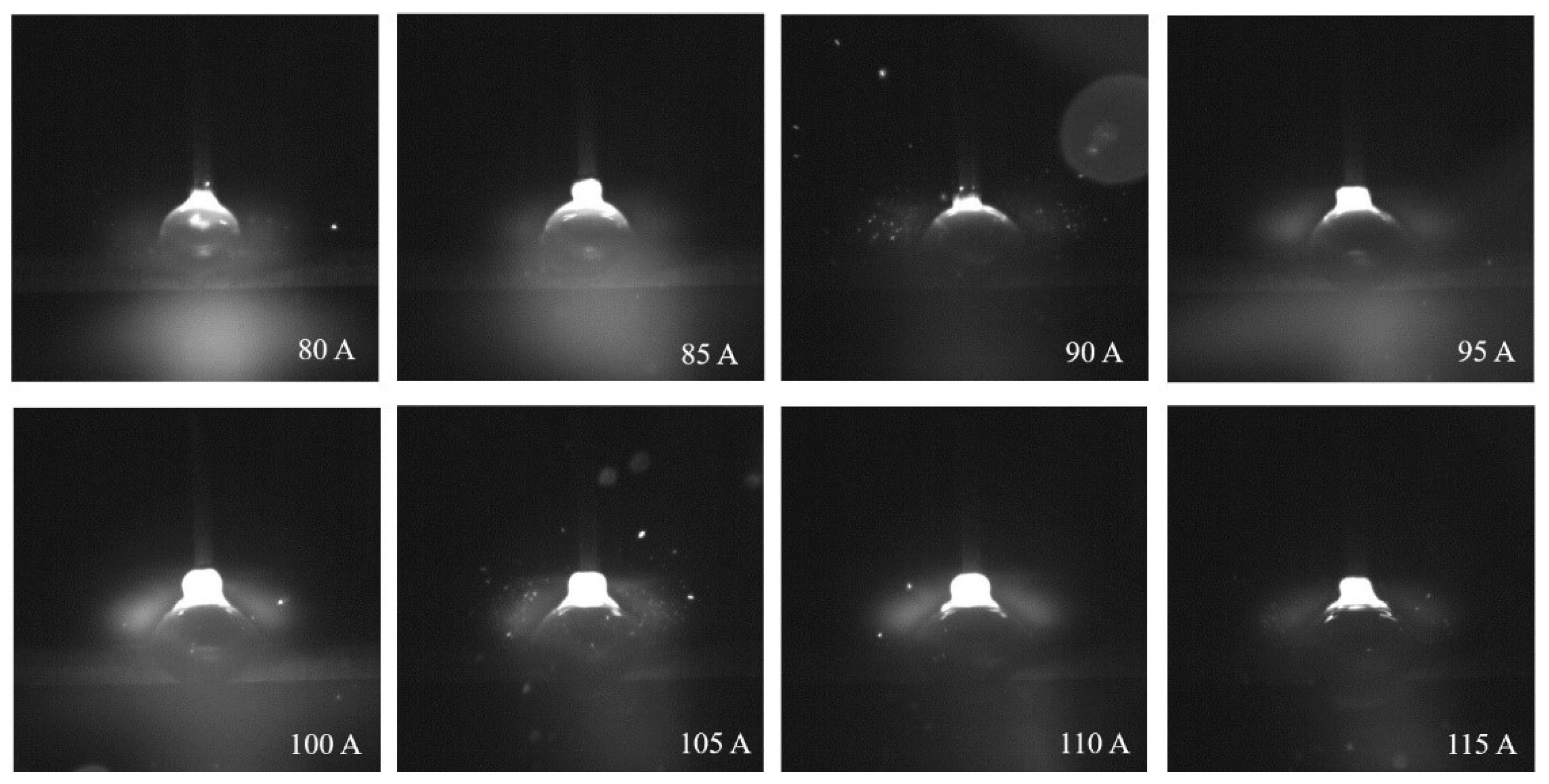
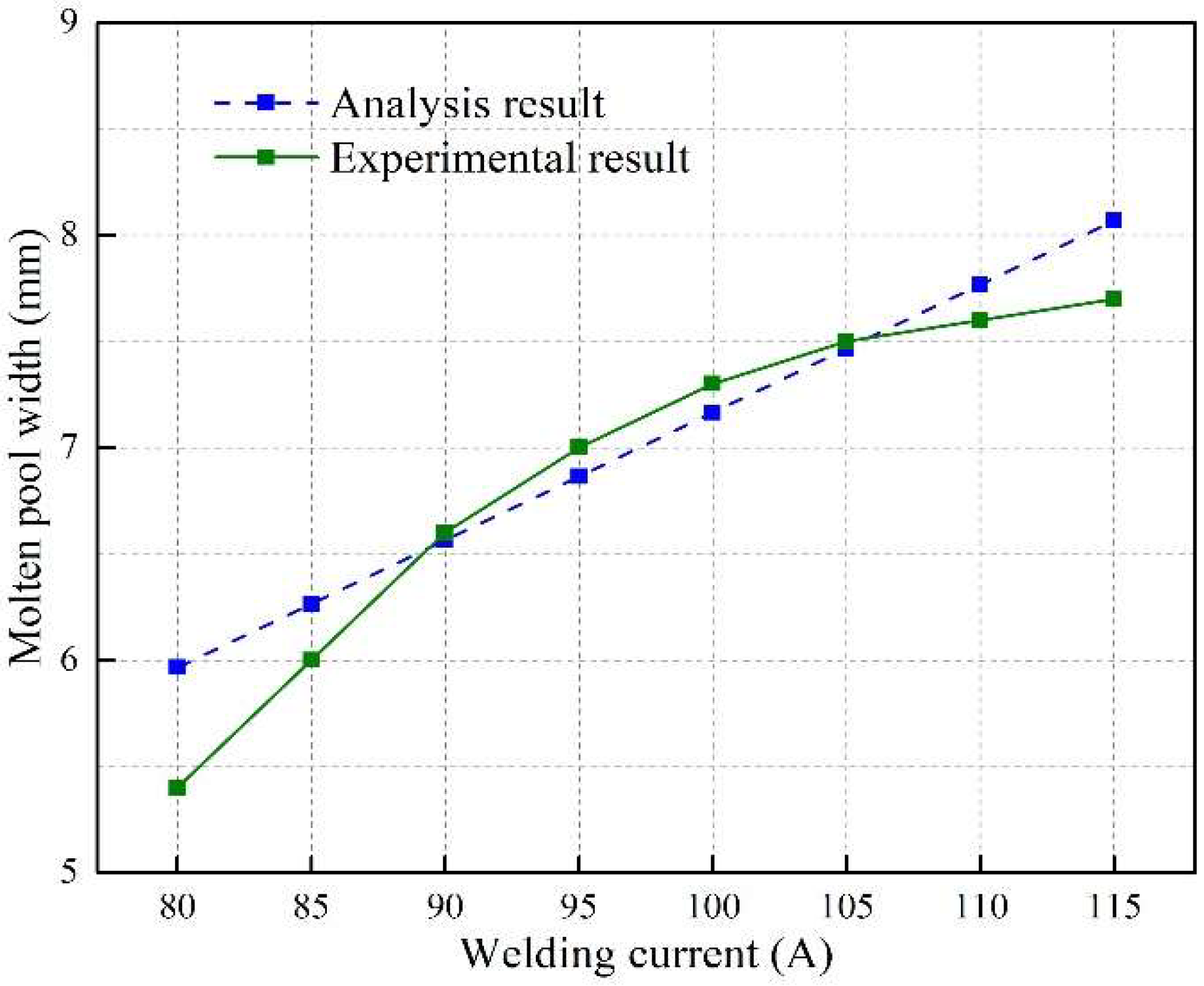
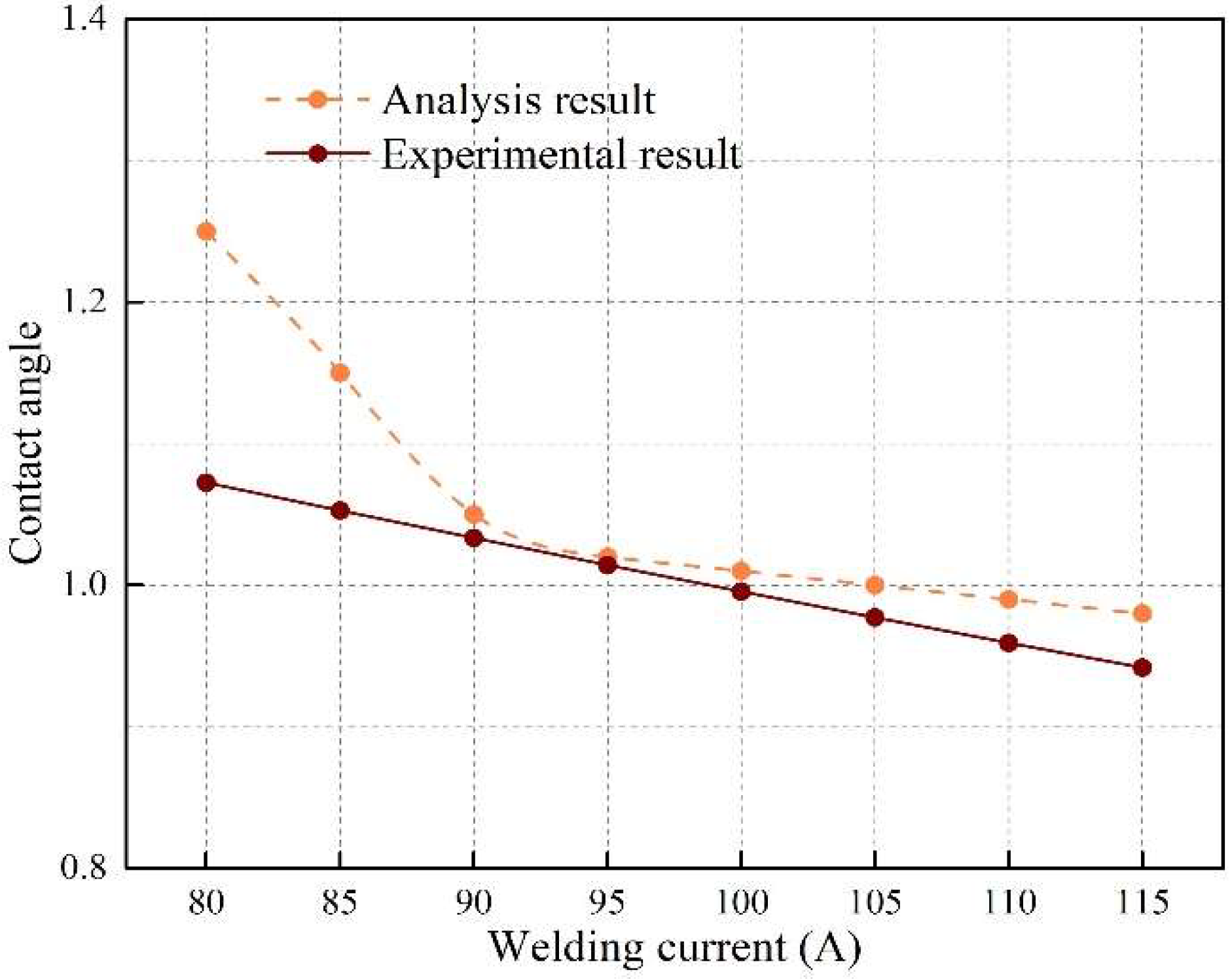
| Test Code | Welding Current | Welding Speed |
|---|---|---|
| 1 | 80 A | 0.48 m/min |
| 2 | 85 A | 0.48 m/min |
| 3 | 90 A | 0.48 m/min |
| 4 | 95 A | 0.48 m/min |
| 5 | 100 A | 0.48 m/min |
| 6 | 105 A | 0.48 m/min |
| 7 | 110 A | 0.48 m/min |
| 8 | 115 A | 0.48 m/min |
| Properties | Symbol | Value |
|---|---|---|
| Density | 2.7 × 106 g/m3 | |
| Arc heating parameter | 7.5 × 10−5 g/As | |
| Joule heating parameter | 2.5 × 10−3 g/mA2s | |
| Extension length | 1.2 × 10−2 m | |
| Transfer distance | 1.0 × 10−2 m | |
| Transfer time constant | 0.4 | |
| Impact time constant | 0.08 | |
| Surface tension | 1.2 N/m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, A.; Xing, Y.; Zhang, X.; Yang, F.; Cao, J. Analysis of Controlled Driving and Spreading Behavior of Molten Pool in Cold Metal Transfer. Energies 2022, 15, 1575. https://doi.org/10.3390/en15041575
Zhang A, Xing Y, Zhang X, Yang F, Cao J. Analysis of Controlled Driving and Spreading Behavior of Molten Pool in Cold Metal Transfer. Energies. 2022; 15(4):1575. https://doi.org/10.3390/en15041575
Chicago/Turabian StyleZhang, An, Yanfeng Xing, Xiaobing Zhang, Fuyong Yang, and Juyong Cao. 2022. "Analysis of Controlled Driving and Spreading Behavior of Molten Pool in Cold Metal Transfer" Energies 15, no. 4: 1575. https://doi.org/10.3390/en15041575
APA StyleZhang, A., Xing, Y., Zhang, X., Yang, F., & Cao, J. (2022). Analysis of Controlled Driving and Spreading Behavior of Molten Pool in Cold Metal Transfer. Energies, 15(4), 1575. https://doi.org/10.3390/en15041575





