Accurate Calculation of Iron Loss of High-Temperature and High-Speed Permanent Magnet Synchronous Generator under the Conditions of SVPWM Modulation
Abstract
:1. Introduction
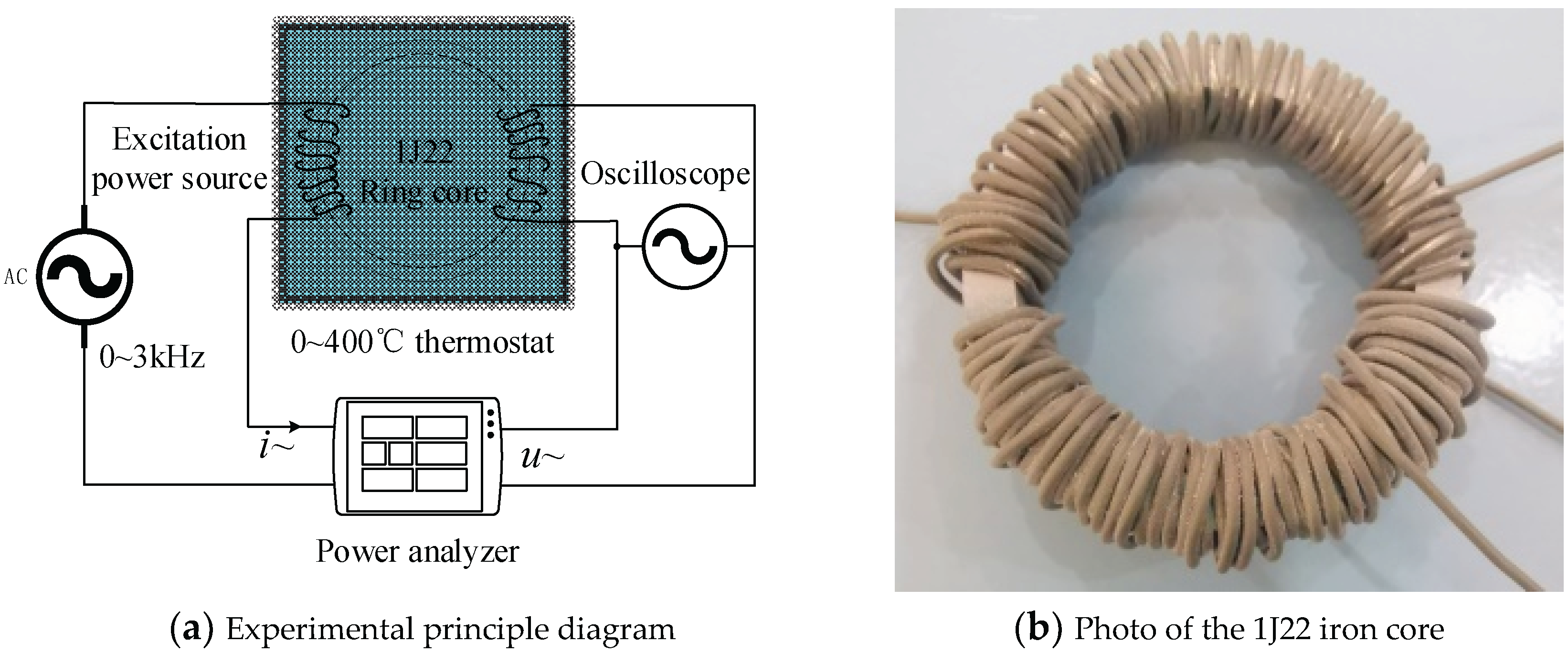
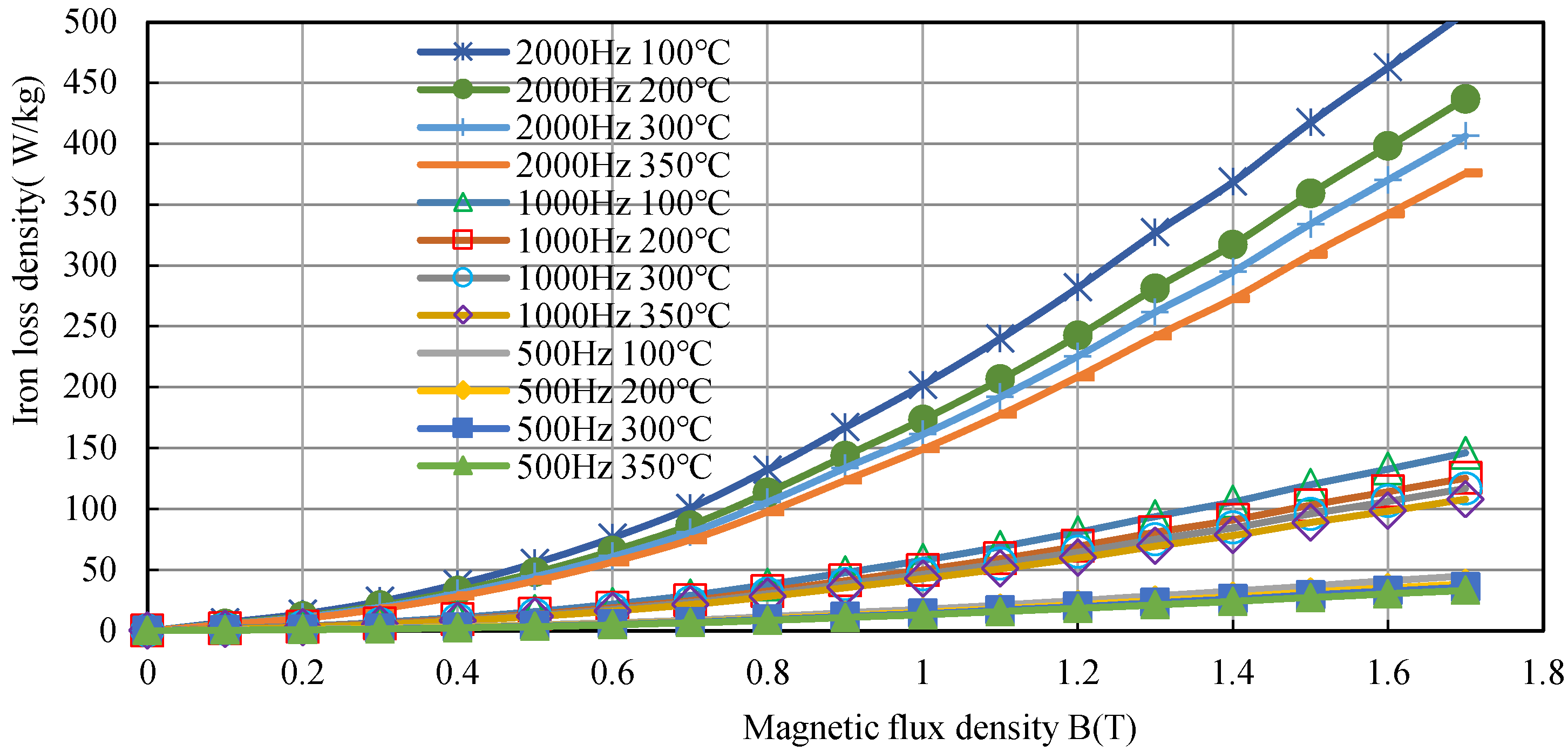
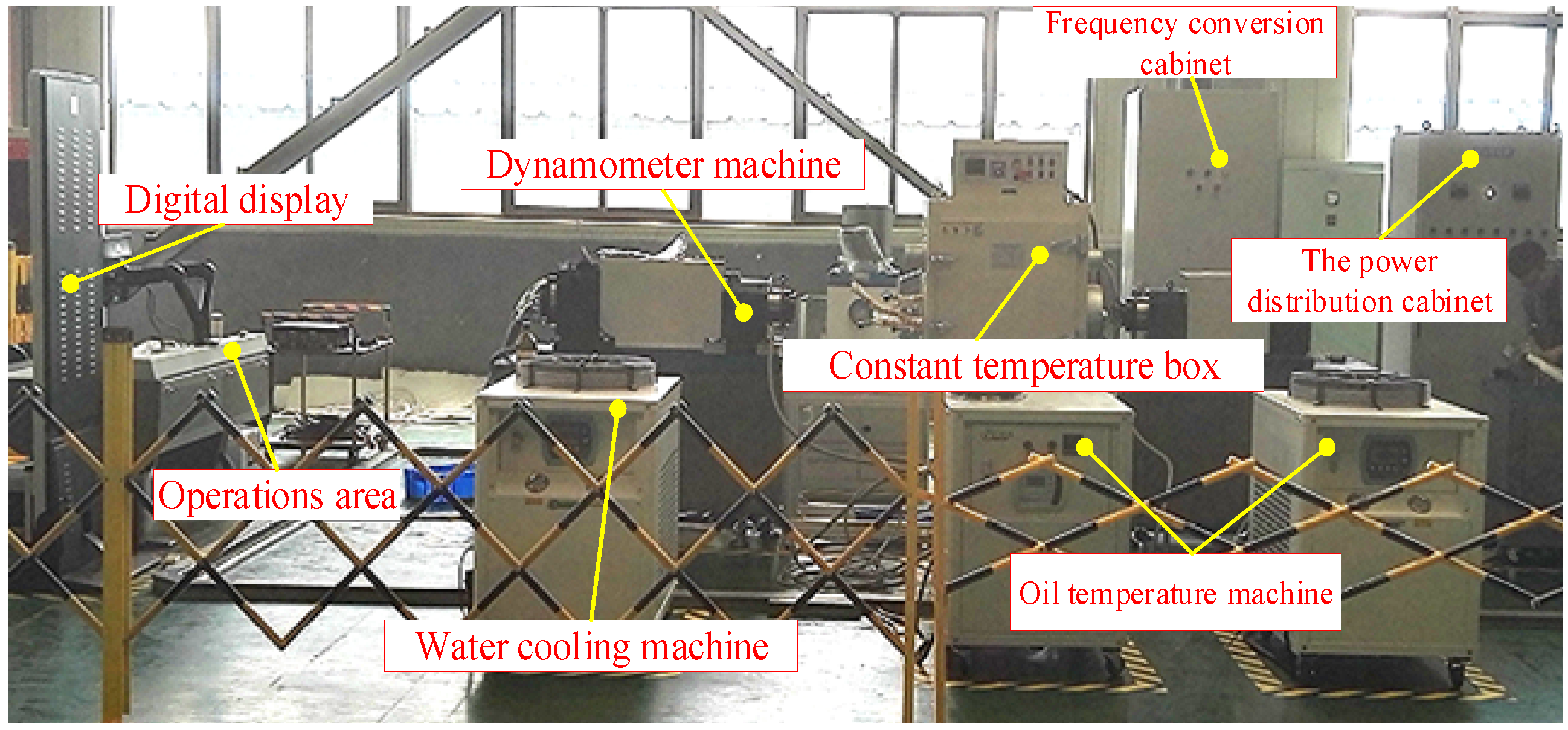
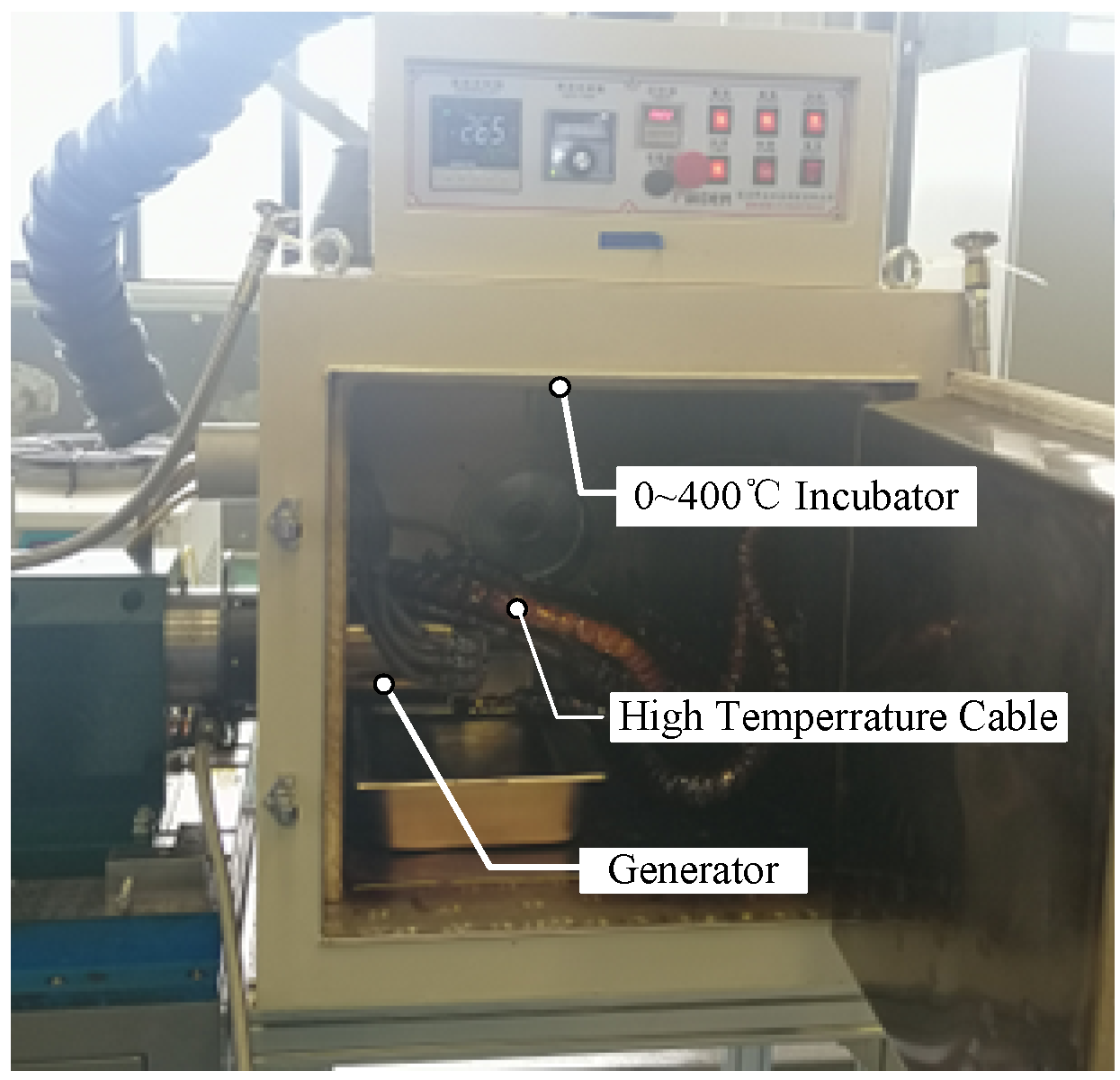
2. Test of Core Loss Characteristics under HTHS
3. Mathematical Calculation Model of Iron Loss
4. Loss Analysis of the HTHSPMSG
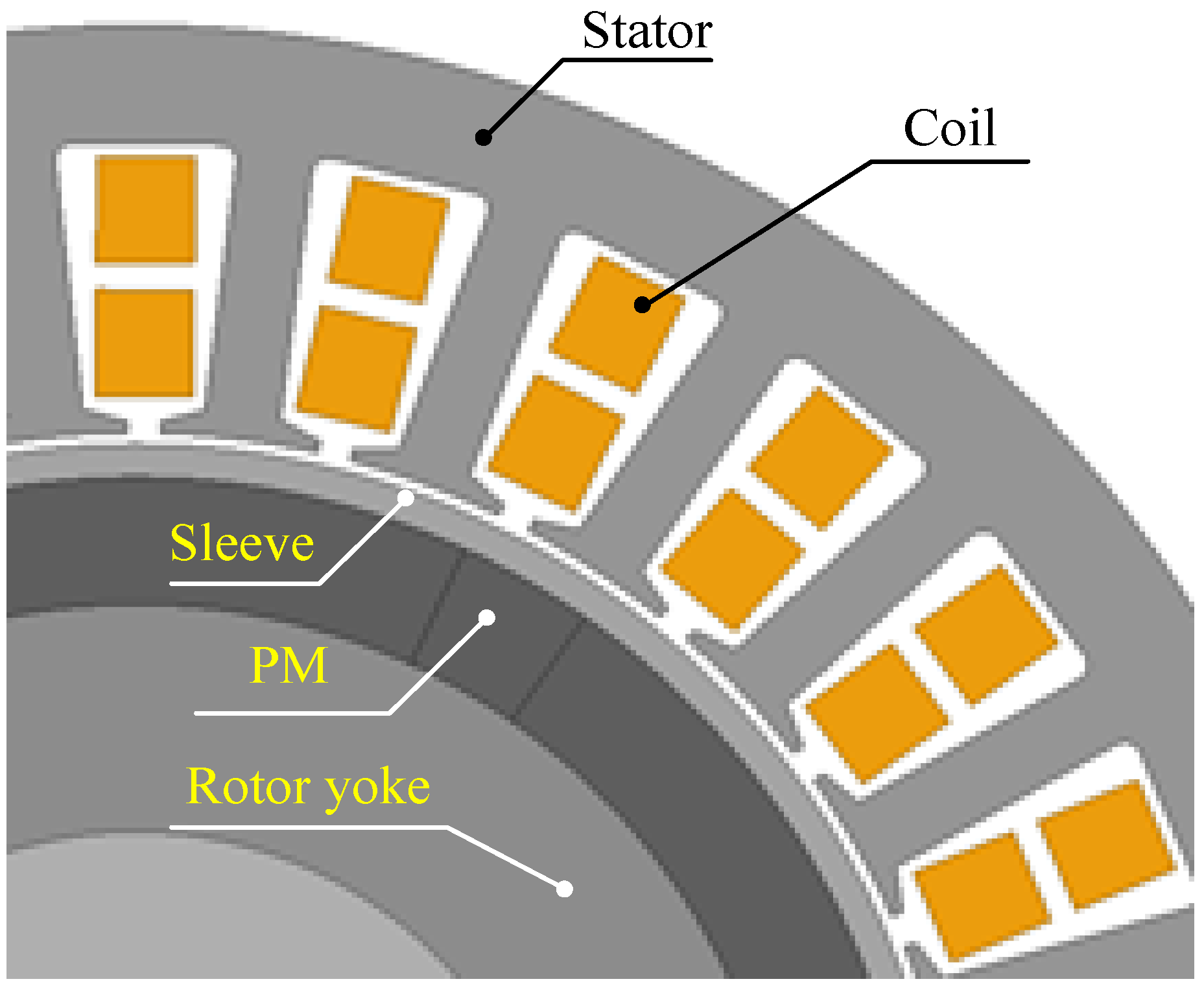
4.1. Parameters and Configuration of the HTHSPMSG
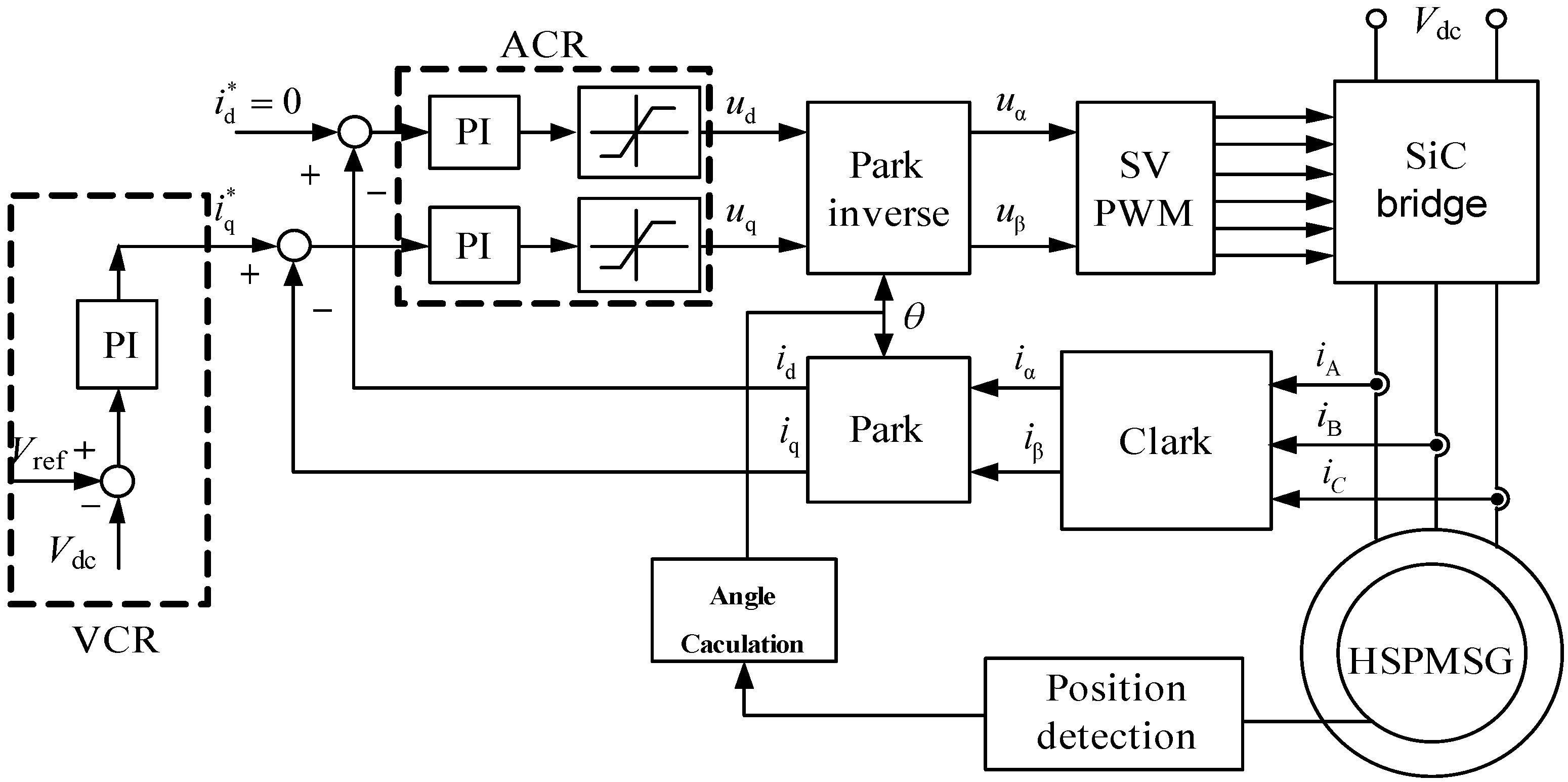
4.2. Power Generation System Control Strategy
4.3. Iron Consumption Calculation Program of HTHS PMSG
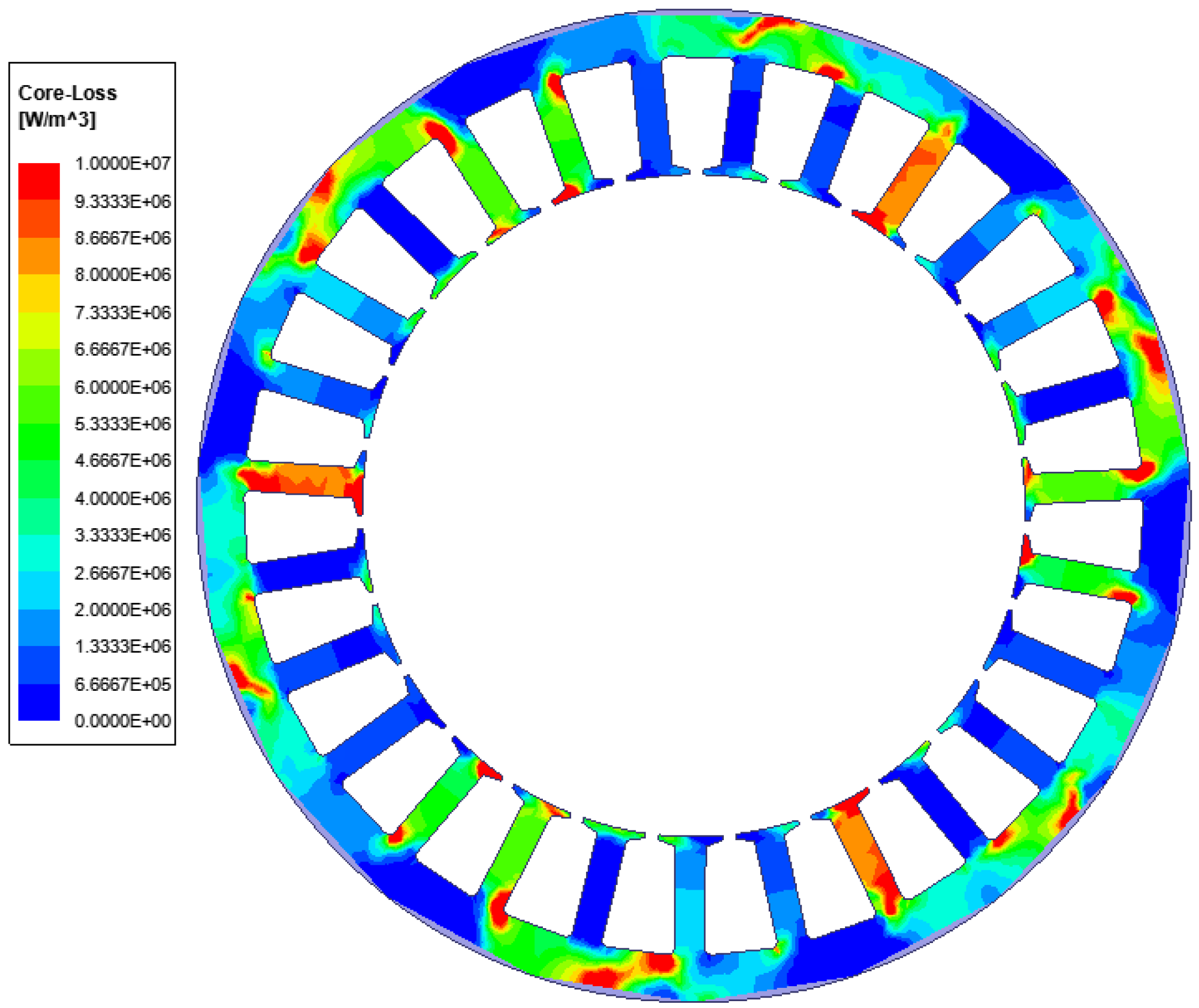
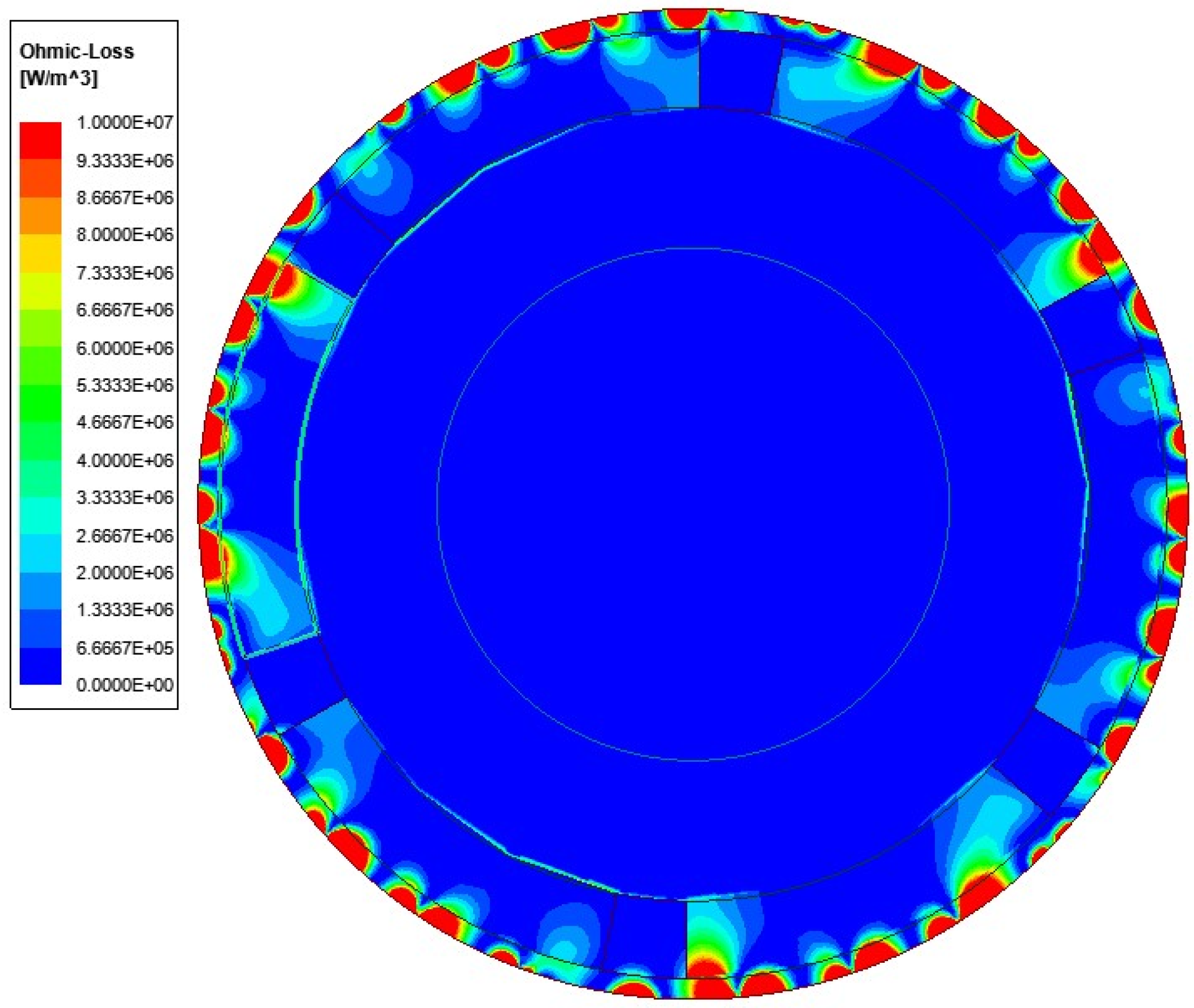
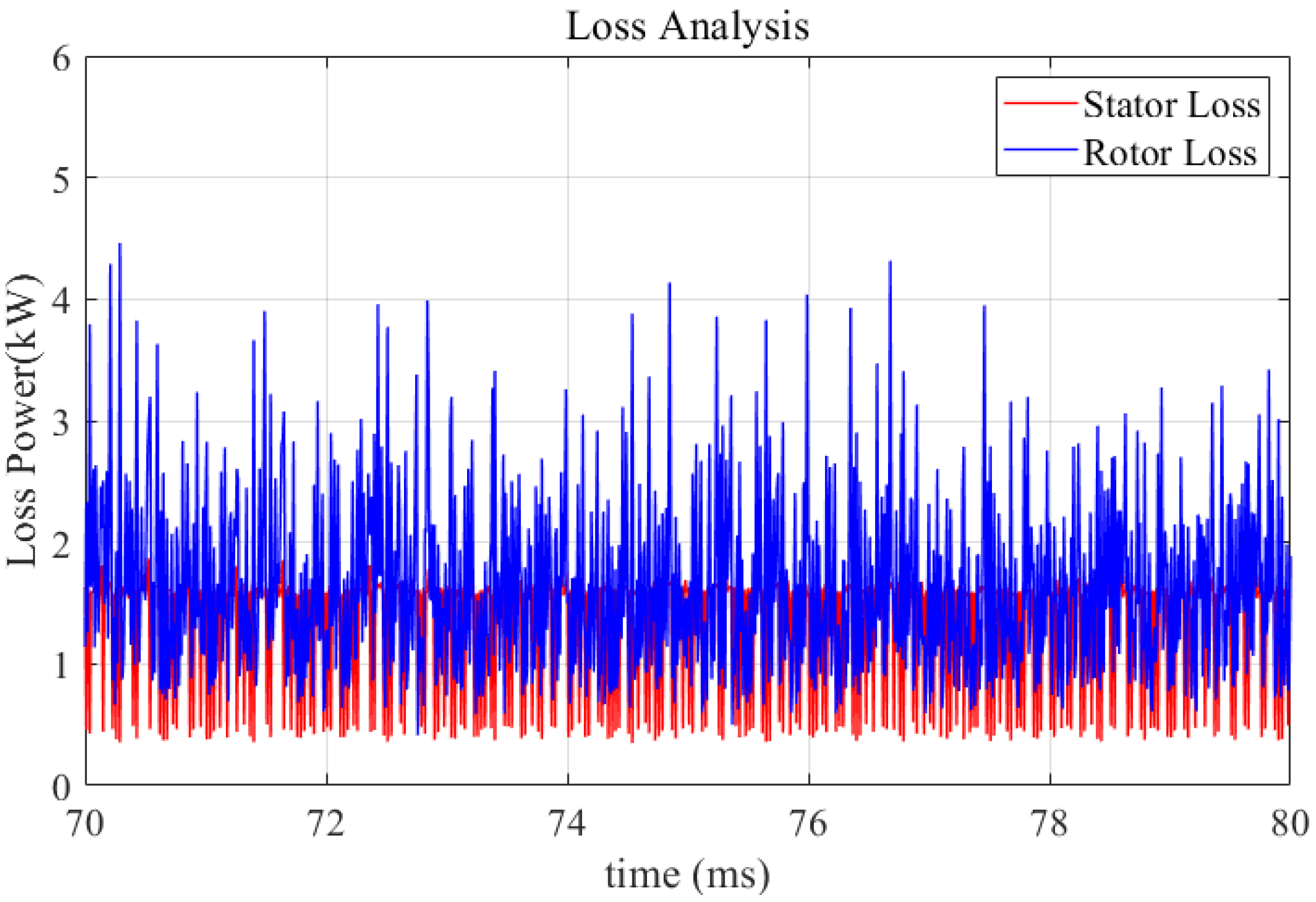
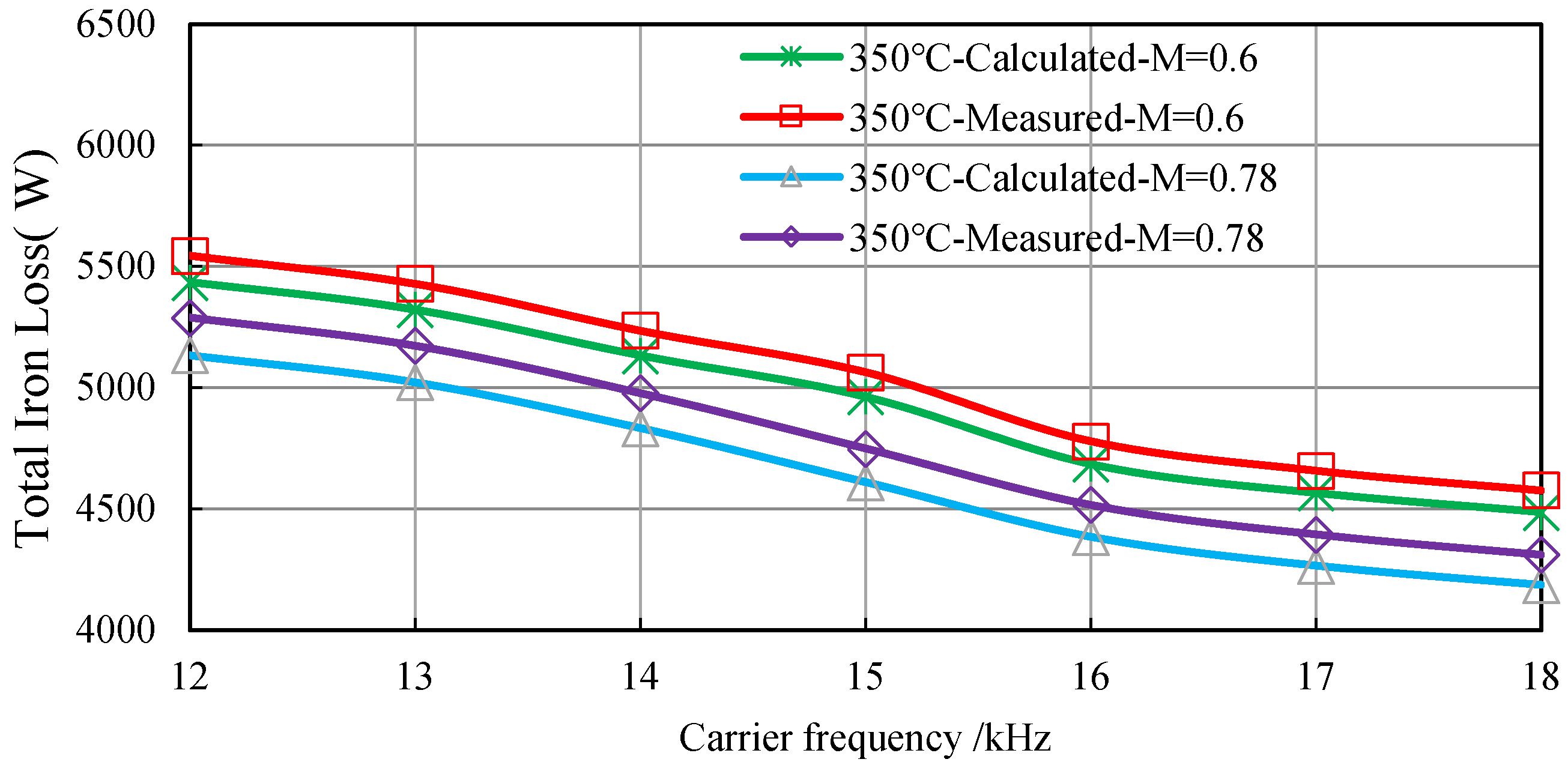
4.4. Calculation and Analysis of Iron Loss of Generators
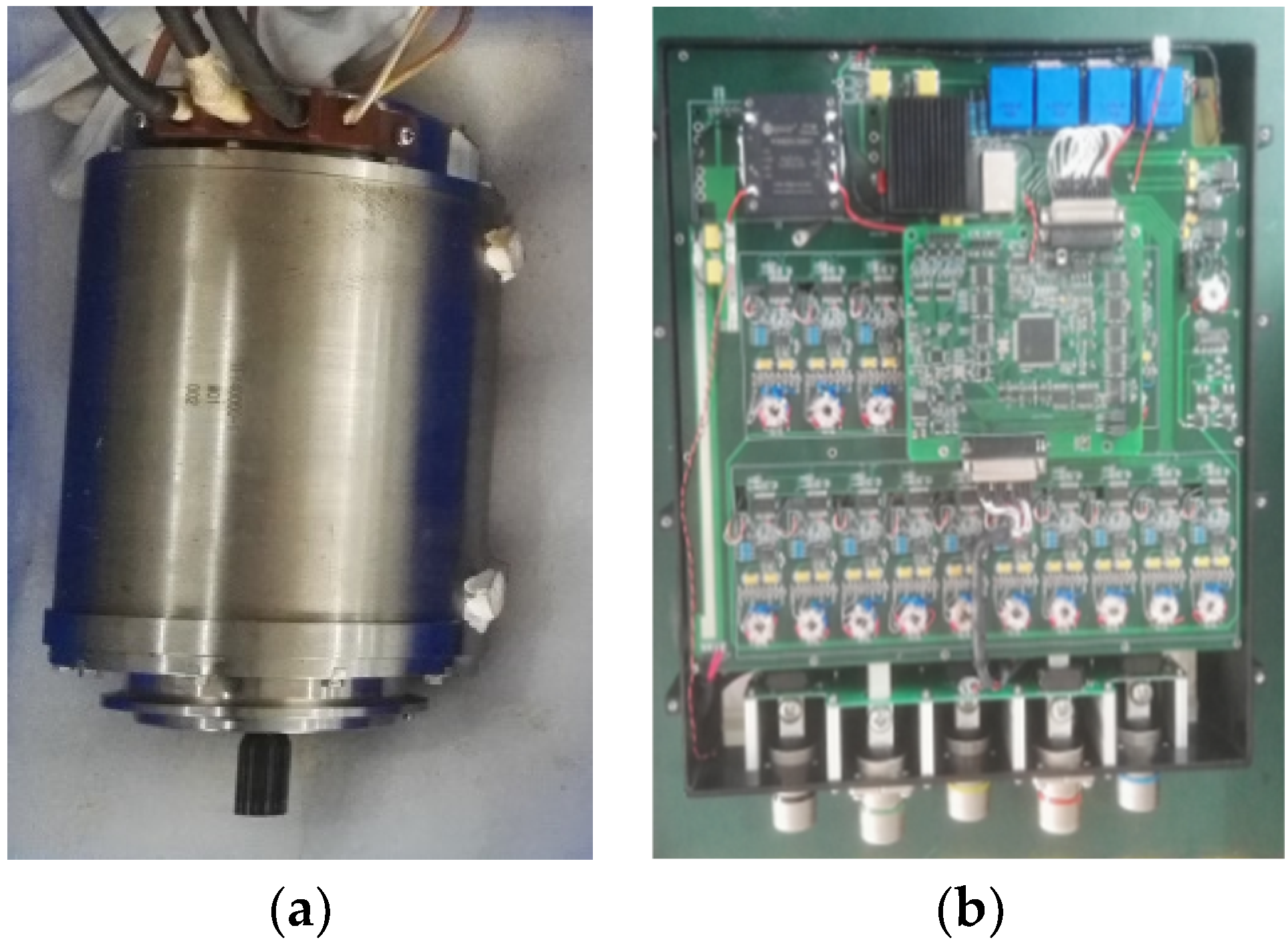
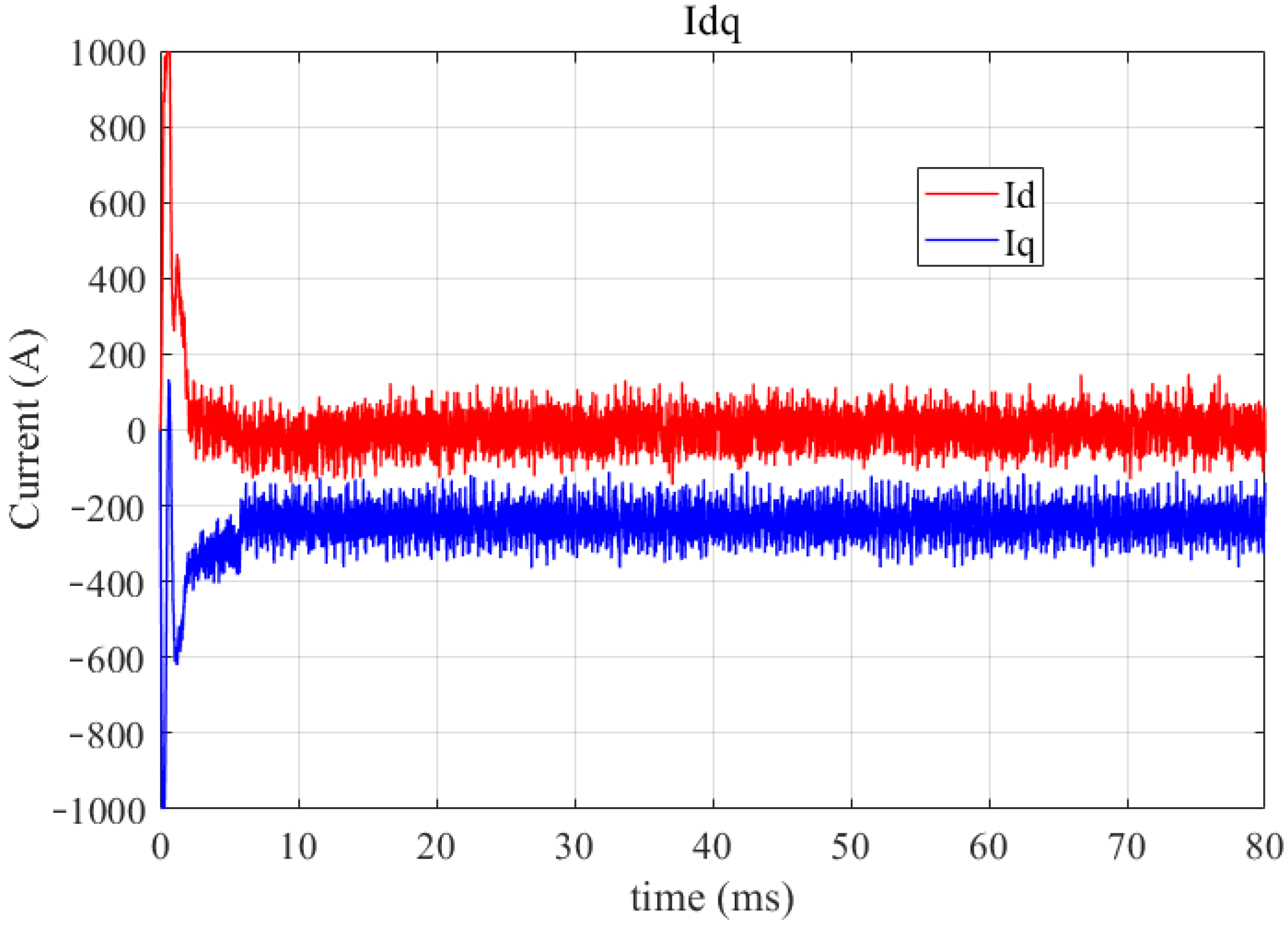
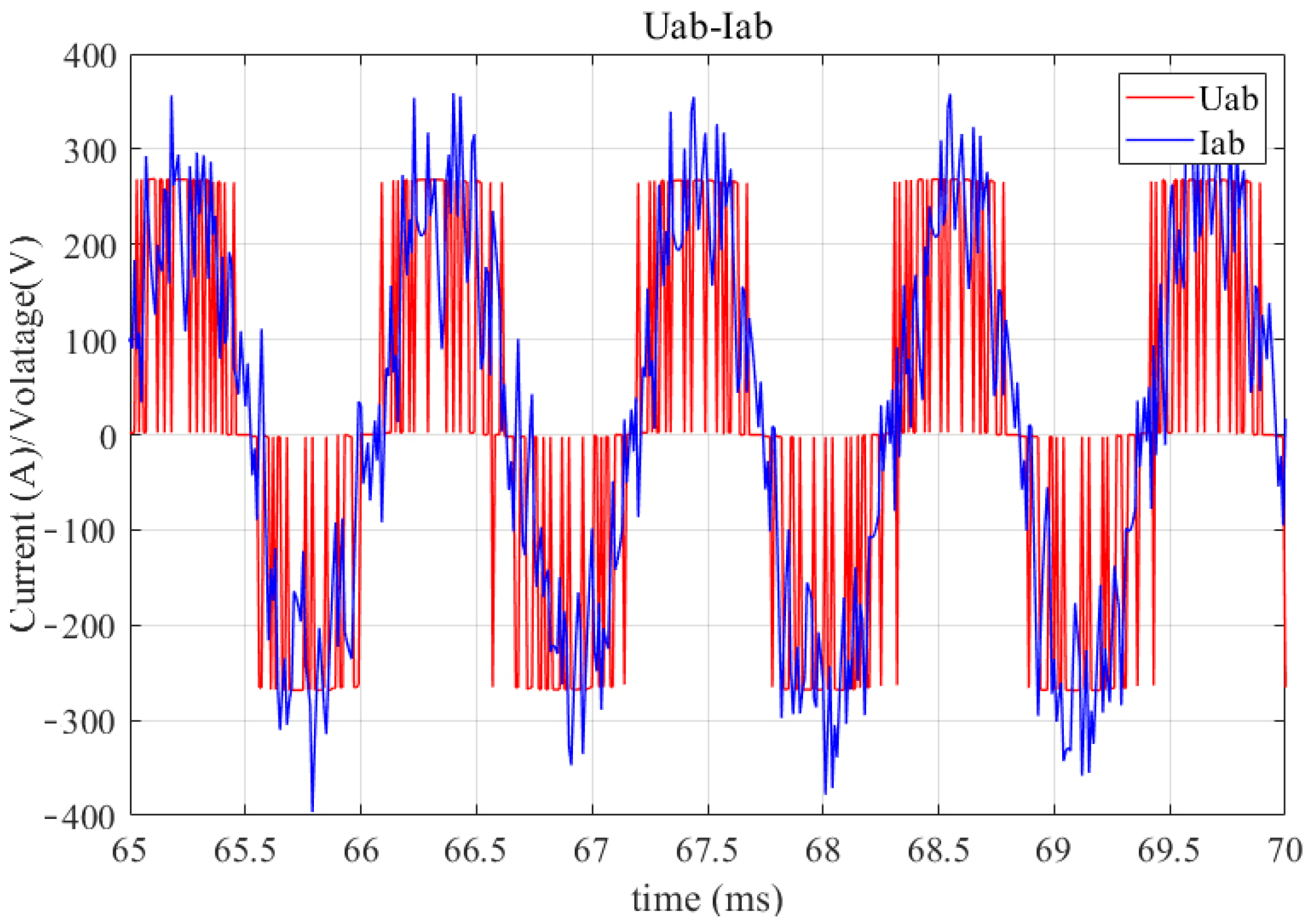
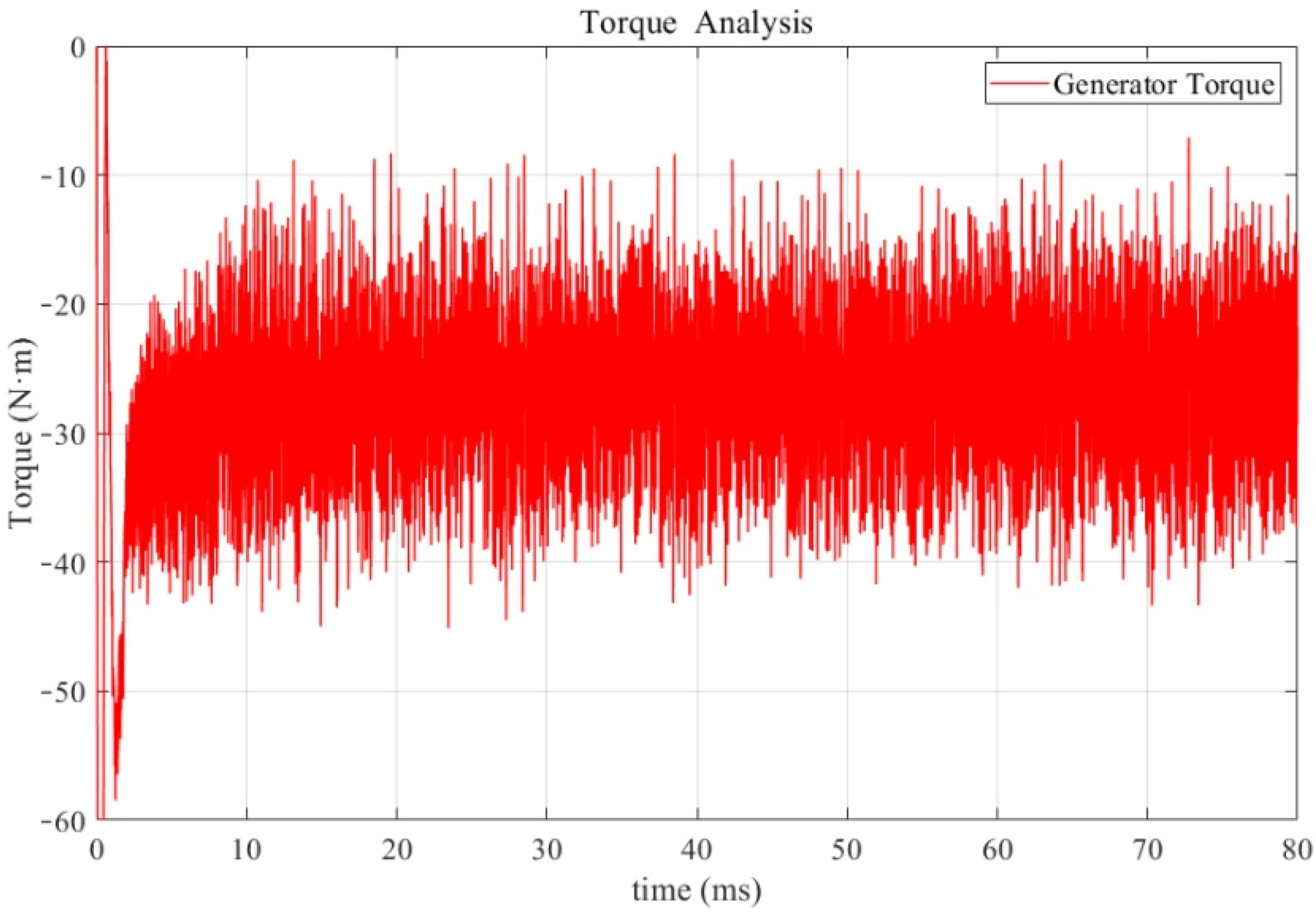
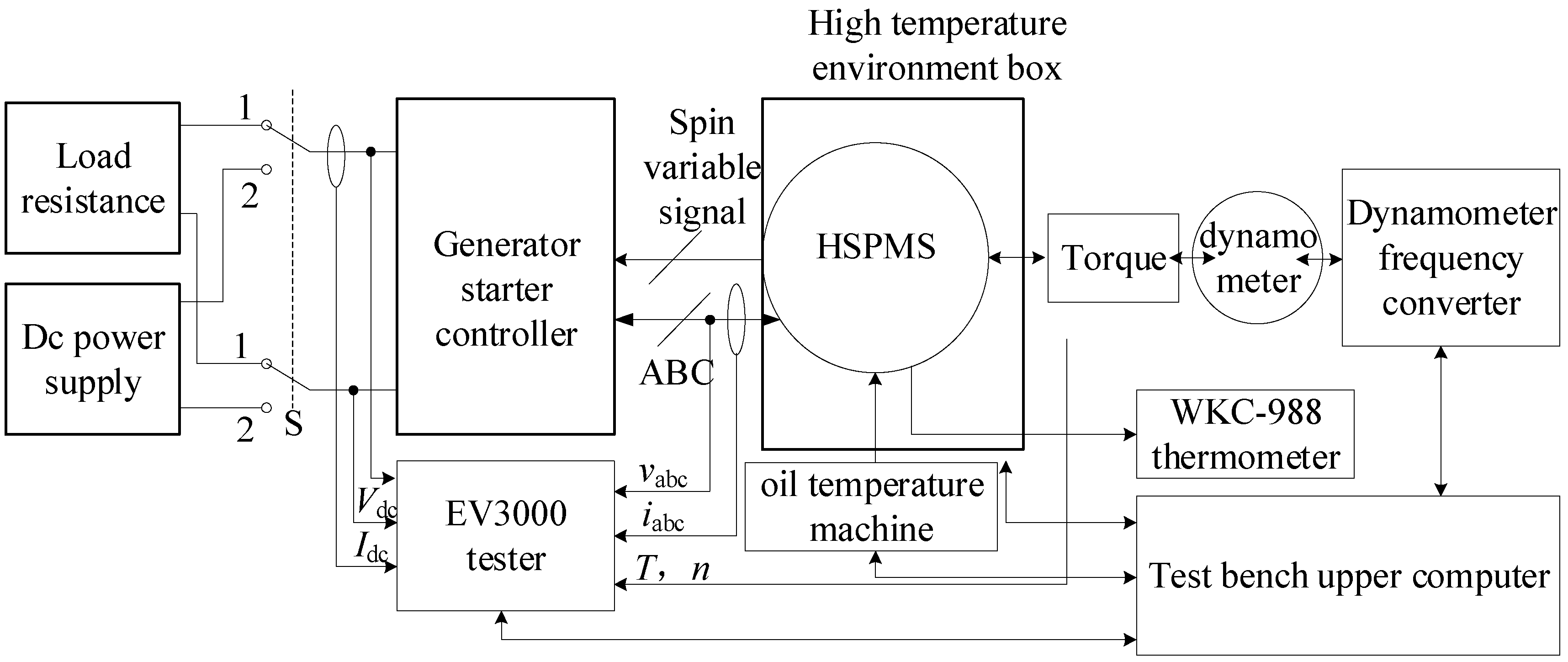
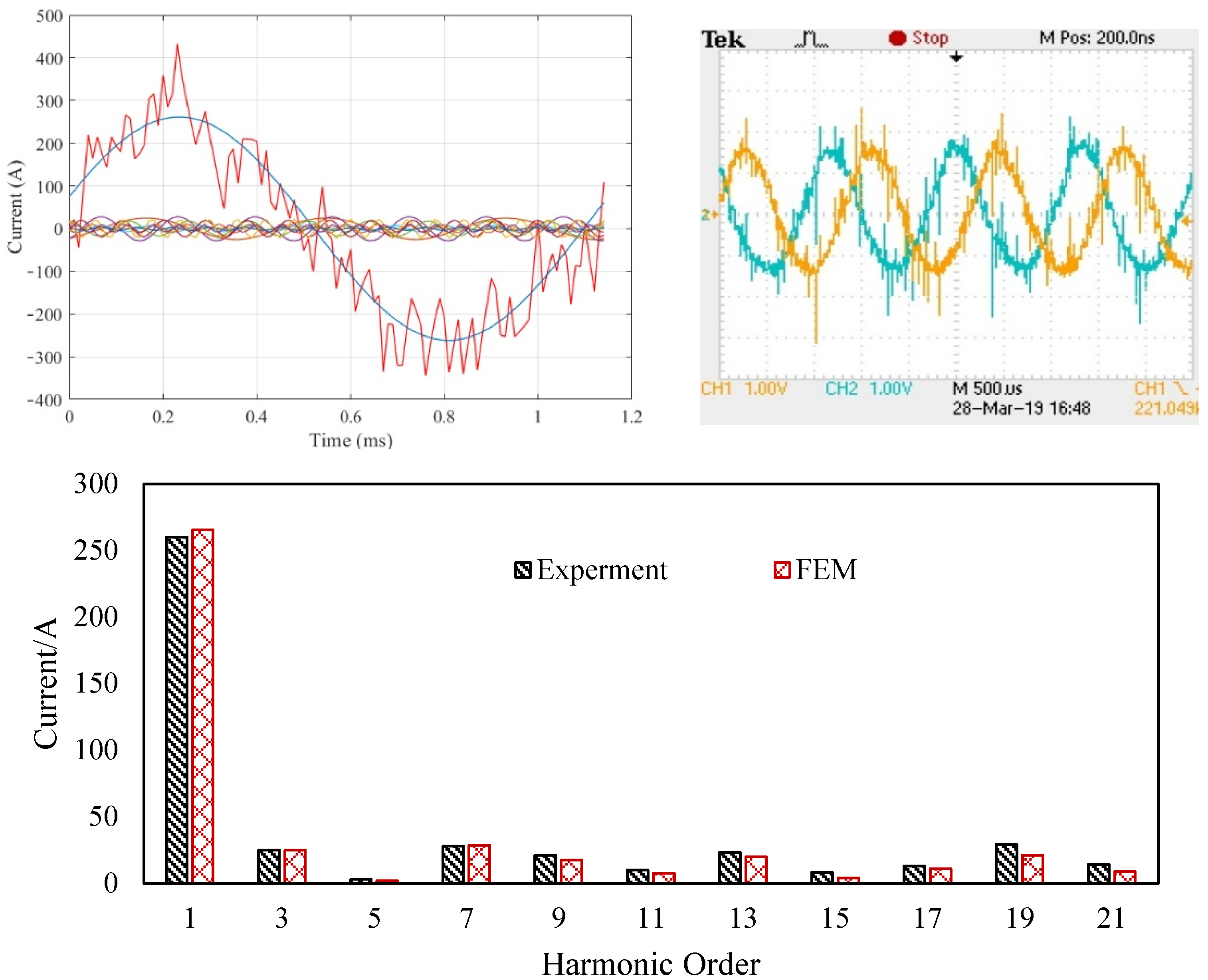
5. Experiment and Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lee, J.-H.; Kim, W.-J.; Jung, S.-Y. Analysis and Verification of Traction Motor Iron Loss for Hybrid Electric Vehicles Based on Current Source Analysis Considering Inverter Switching Carrier Frequency. Electronics 2021, 10, 2714. [Google Scholar] [CrossRef]
- Du, J.; Li, C.; Zhao, J.; Ren, H.; Zhang, K.; Song, X.; Chen, L.; Yu, S.; Mi, Y. Investigation of Eddy Current Loss and Structure Design with Magnetic-Thermal Coupling for Toothless BLDC High-Speed PM Motor. Machines 2022, 10, 118. [Google Scholar] [CrossRef]
- Huang, Y.; Dong, J.; Zhu, J.; Guo, Y. Core Loss Modeling for Permanent-Magnet Motor Based on Flux Variation Locus and Finite-Element Method. IEEE Trans. Magn. 2012, 48, 1023–1026. [Google Scholar] [CrossRef]
- Xue, S.; Feng, J.; Guo, S.; Peng, J.; Chu, W.Q.; Zhu, Z.Q. A New Iron Loss Model for Temperature Dependencies of Hysteresis and Eddy Current Losses in Electrical Machines. IEEE Trans. Magn. 2018, 54, 1–10. [Google Scholar] [CrossRef]
- Fujisaki, K.; Hirayama, R.; Kawachi, T.; Satou, S.; Kaidou, C.; Yabumoto, M.; Kubota, T. Motor core iron loss analysis evaluating shrink fitting and stamping by finite-element method. IEEE Trans. Magn. 2007, 43, 1950–1954. [Google Scholar] [CrossRef]
- Liu, G.; Liu, M.; Zhang, Y.; Wang, H.; Gerada, C. High-Speed Permanent Magnet Synchronous Motor Iron Loss Calculation Method Considering Multiphysics Factors. IEEE Trans. Ind. Electron. 2019, 67, 5360–5368. [Google Scholar] [CrossRef]
- Yamazaki, K.; Watari, S. Loss analysis of permanent-magnet motor considering carrier harmonics of PWM inverter using combination of 2-D and 3-D finite-element method. IEEE Trans. Magn. 2005, 41, 1980–1983. [Google Scholar] [CrossRef]
- Yamazaki, K.; Abe, A. Loss Analysis of Interior Permanent Magnet Motors Considering Carrier Harmonics and Magnet Eddy Currents Using 3-D FEM. In Proceedings of the 2007 IEEE International Electric Machines & Drives Conference, Antalya, Turkey, 3–5 May 2007; Volume 2, pp. 904–909. [Google Scholar]
- Boglietti, A.; Cavagnino, A.; Ionel, D.M.; Popescu, M.; Staton, D.A.; Vaschetto, S. A General Model to Predict the Iron Losses in PWM Inverter-Fed Induction Motors. IEEE Trans. Ind. Appl. 2010, 46, 1882–1890. [Google Scholar] [CrossRef]
- Dubas, F.; Rahideh, A. Two-Dimensional Analytical Permanent-Magnet Eddy-Current Loss Calculations in Slotless PMSM Equipped with Surface-Inset Magnets. IEEE Trans. Magn. 2013, 50, 54–73. [Google Scholar] [CrossRef]
- Huang, Z.; Fang, J.; Liu, X.; Han, B. Loss Calculation and Thermal Analysis of Rotors supported by Active Magnetic Bearings for High-Speed Permanent Magnet Electrical Machines. IEEE Trans. Ind. Electron. 2015, 63, 1. [Google Scholar] [CrossRef]
- Roy, R.; Dalal, A.; Kumar, P. Prediction of high frequency core loss for electrical steel using the data provided by manufacturer. J. Magn. Magn. Mater. 2016, 410, 248–256. [Google Scholar] [CrossRef]
- Chen, J.; Wang, D.; Cheng, S.; Wang, Y.; Zhu, Y.; Liu, Q. Modeling of temperature effects on magnetic property of nonoriented silicon steel lamination. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Yokoyama, D.; Sato, T.; Todaka, T.; Enokizono, M. Comparison of Iron Loss Characteristics of Divided Cores Considering Vector Magnetic Properties. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]




| Composition | C | Mn | Si | Co | Cu | Ni | Fe |
|---|---|---|---|---|---|---|---|
| Content | ≤0.04% | ≤0.30% | ≤0.30% | 49.0–51.0% | ≤0.20% | ≤0.50% | other |
| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Rated power (kW) | 40 | Rated speed (rpm) | 18,000 |
| Poles/slots | 6/27 | Rated output voltage (V) | 270 DC |
| Stator outer diameter (mm) | 160 | Air gap length (mm) | 2 |
| Rotor outer diameter (mm) | 95 | Lamination length (mm) | 240 |
| Sleeve thickness (mm) | 1 | Magnet thickness (mm) | 7.6 |
| Measurement | FEM-Frequency/Temperature | FEM-Frequency | |
|---|---|---|---|
| Output power P2 (W) | 40,561 | 40,044 | 40,785 |
| Input mechanical Torque T1 (N·m) | −25.6 | −25.2 | −26.9 |
| Input power P1 (W) | 48,230 | 47,497 | 49,879 |
| Total loss PL (W) | 7669 | 7453 | 9094 |
| Efficiency η | 84.1% | 84.3% | 81.7% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhuo, L.; Yang, D.; Sun, R.; Sun, L.; Zou, J. Accurate Calculation of Iron Loss of High-Temperature and High-Speed Permanent Magnet Synchronous Generator under the Conditions of SVPWM Modulation. Energies 2022, 15, 2315. https://doi.org/10.3390/en15072315
Zhuo L, Yang D, Sun R, Sun L, Zou J. Accurate Calculation of Iron Loss of High-Temperature and High-Speed Permanent Magnet Synchronous Generator under the Conditions of SVPWM Modulation. Energies. 2022; 15(7):2315. https://doi.org/10.3390/en15072315
Chicago/Turabian StyleZhuo, Liang, Du Yang, Ruolan Sun, Lu Sun, and Jibin Zou. 2022. "Accurate Calculation of Iron Loss of High-Temperature and High-Speed Permanent Magnet Synchronous Generator under the Conditions of SVPWM Modulation" Energies 15, no. 7: 2315. https://doi.org/10.3390/en15072315
APA StyleZhuo, L., Yang, D., Sun, R., Sun, L., & Zou, J. (2022). Accurate Calculation of Iron Loss of High-Temperature and High-Speed Permanent Magnet Synchronous Generator under the Conditions of SVPWM Modulation. Energies, 15(7), 2315. https://doi.org/10.3390/en15072315





