Analysis of a Two-Stage Magnetic Precession Gear Dynamics
Abstract
:1. Introduction
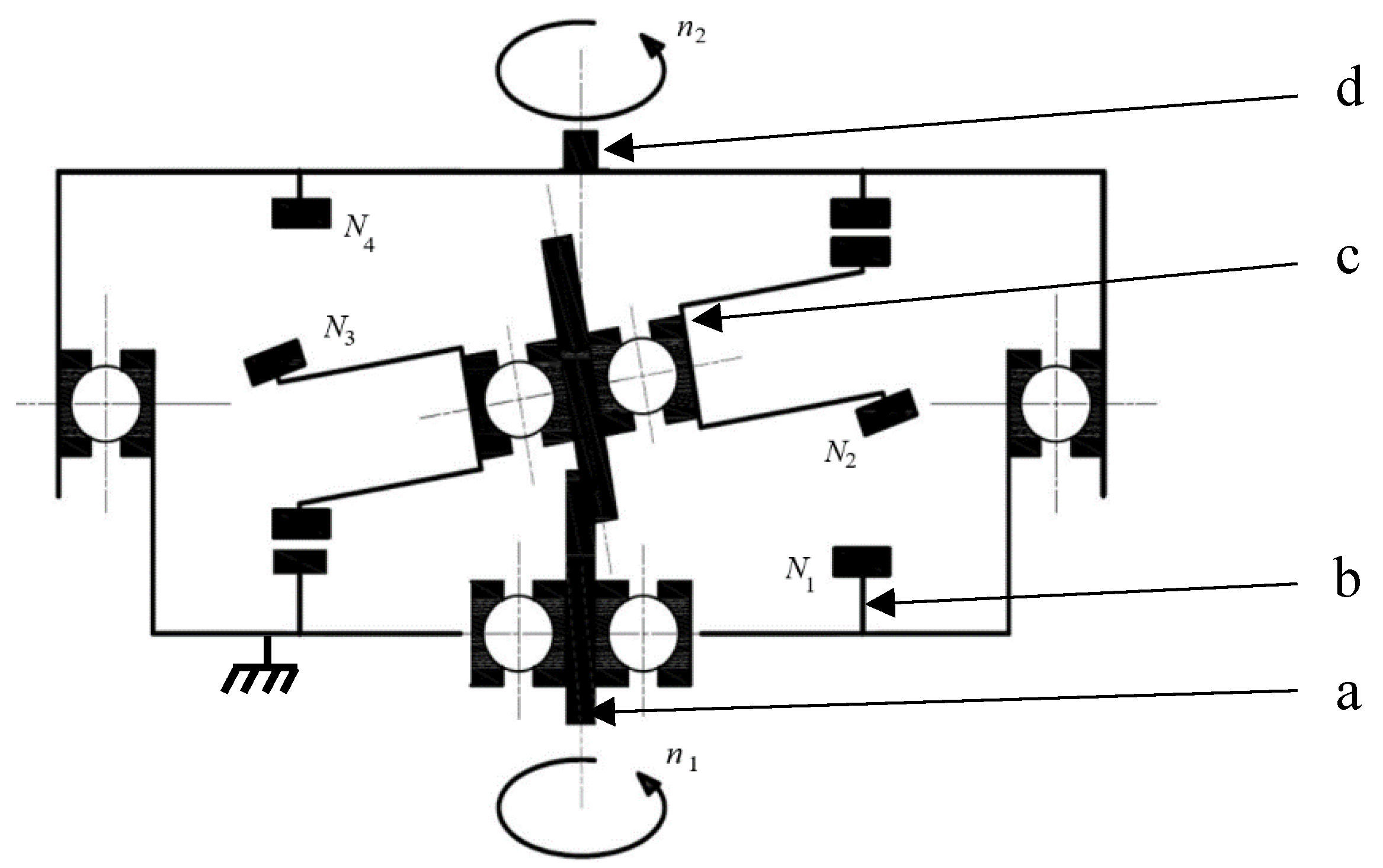
2. Design and 3D FEM Model of a Two-Stage Magnetic Precession Gear
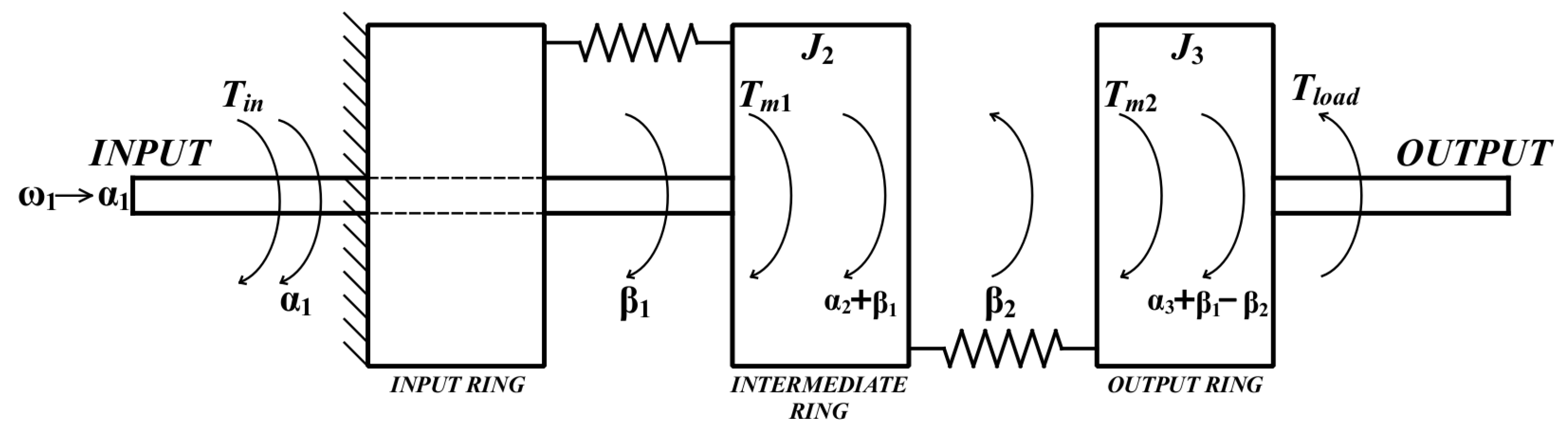
3. Model of the MPG Dynamics
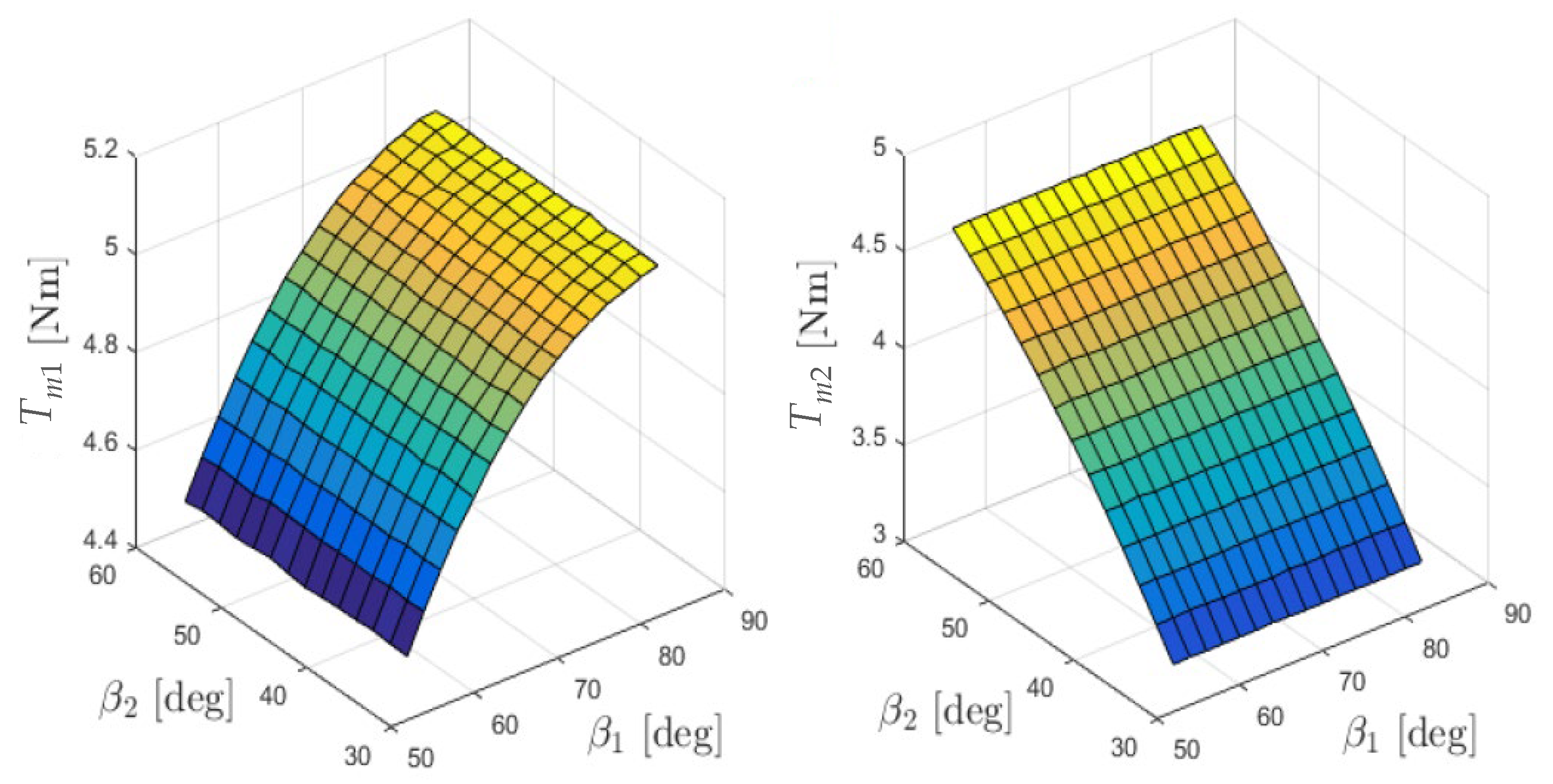
4. Numerical Model of the MPG Dynamics
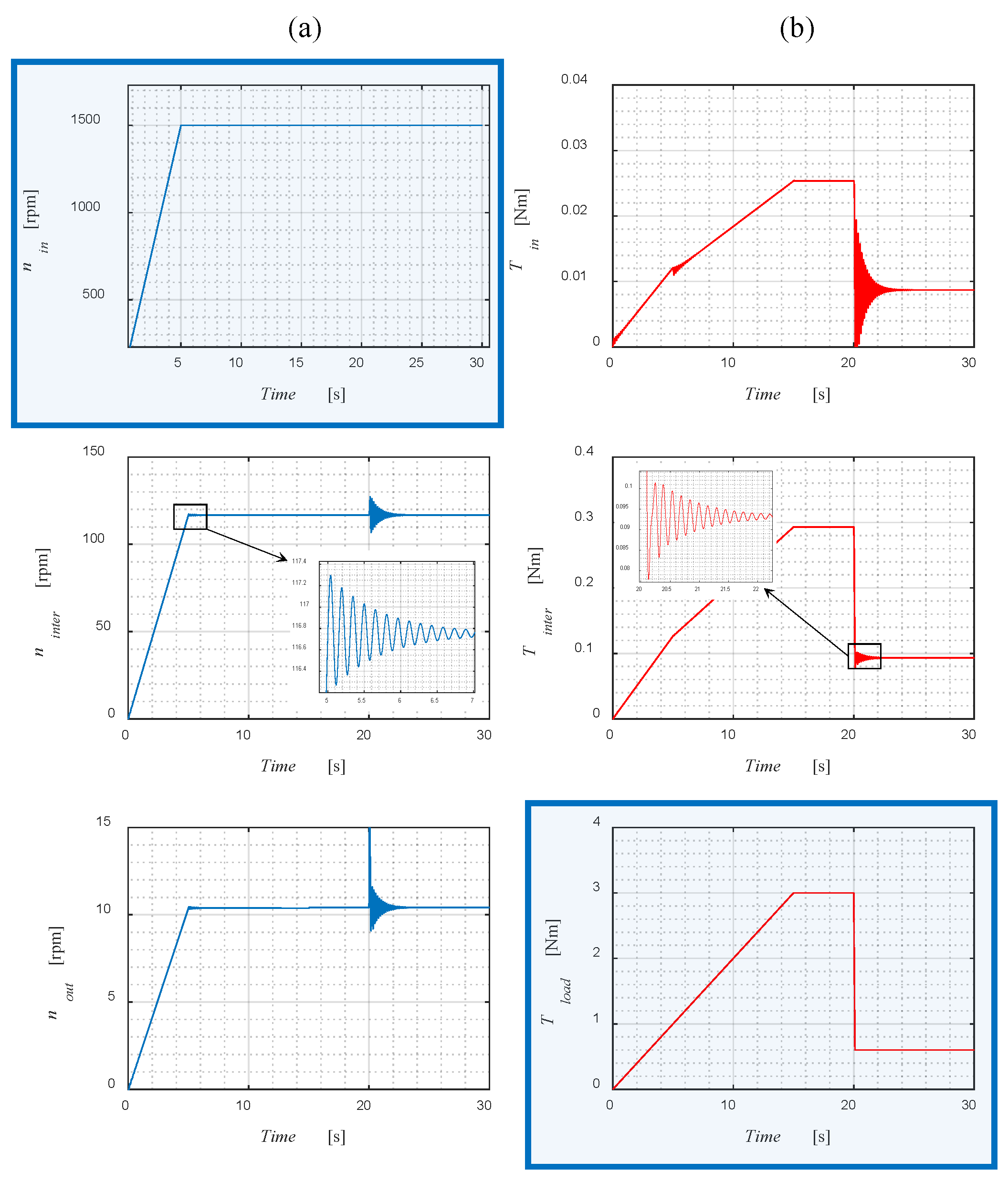
5. Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| n1 | input rotational speed |
| n2 | output rotational speed |
| Ni | number of neodymium magnets on the i-th ring |
| β1m | mechanical angle between the immovable ring and the intermediate ring |
| β2m | mechanical angle between the intermediate ring and the output ring |
| β1 | load angle between the immovable ring and the intermediate ring |
| β2 | load angle between the intermediate ring and the output ring |
| Tm1 | magnetic torque resulting from interaction between the magnetic field in the air gaps excited by the magnets between immovable and intermediate rings |
| Tm1max | maximal value of magnetic torque resulting from interaction between the magnetic field in the air gaps excited by the magnets between immovable and intermediate rings |
| Tm2 | magnetic torque resulting from interaction between the magnetic field in the air gaps excited by the magnets between intermediate and output rings |
| Tm2max | maximal value of magnetic torque resulting from interaction between the magnetic field in the air gaps excited by the magnets between intermediate and output rings |
| J2 | moments of inertia of the intermediate ring |
| J3 | moments of inertia of the output ring |
| Tload | load torque |
| kf2 | coefficient representing mechanical friction and losses in the magnetic circuit caused by eddy currents and magnetic hysteresis phenomena in the intermediate ring |
| kf3 | coefficient representing mechanical friction and losses in the magnetic circuit caused by eddy currents and magnetic hysteresis phenomena in the output ring |
| ki1 | gear ratio at the first stage |
| ki2 | gear ratio at the second stage |
| pi | number of pole pairs on each ring |
| γ | precession angle |
| β0 | angle corresponding to the specific load condition |
| β01 | angle corresponding to the specific load condition for the intermediate ring |
| β02 | angle corresponding to the specific load condition for the output ring |
| α2 | angular position of the intermediate ring |
| α3 | angular position of the output ring |
| Wt1 | function describing the stiffness coefficients of the intermediate ring |
| Wt1_0 | stiffness coefficient of the intermediate ring simplified by introducing linearity with consideration of the center of the coordinate system |
| Wt2 | functions describing the stiffness coefficients of the output ring |
| Wt2_0 | stiffness coefficient of the output ring simplified by introducing linearity with consideration of the center of the coordinate system |
| ξ1 | damping factor for the intermediate ring |
| ξ2 | damping factor for the output ring |
| ωt1 | resonance frequency for the intermediate ring |
| ωt2 | resonance frequency for the output ring |
| ω2 | angular velocity of the intermediate ring |
| ω3 | angular velocity of the output ring |
| fr | frequency of load torque ripples |
References
- Sun, K.; Wang, G.; Lu, Y. Optimization Method of Bevel Gear Reliability Based on Genetic Algorithm and Discrete Element. Eksploat. Niezawodn. Maint. Reliab. 2019, 21, 186–196. [Google Scholar] [CrossRef]
- He, Z.; Zhang, T.; Lin, T. Novel Mathematical Modelling Method for Meshing Impact of Helical Gear. Mech. Mach. Theory 2020, 152, 103949. [Google Scholar] [CrossRef]
- Karabacak, Y.E.; Özmen, N.G.; Gümüşel, L. Worm Gear Condition Monitoring and Fault Detection from Thermal Images via Deep Learning Method. Eksploat. Niezawodn. Maint. Reliab. 2020, 22, 544–556. [Google Scholar] [CrossRef]
- Ramadani, R.; Belsak, A.; Kegl, M.; Predan, J.; Pehan, S. Topology Optimization Based Design of Lightweight and Low Vibration Gear Bodies. Int. J. Simul. Model. 2018, 17, 92–104. [Google Scholar] [CrossRef] [PubMed]
- Yuan, B.; Chang, L.; Liu, G.; Chang, S.; Liu, L.; Shen, Y. An Efficient Three-Dimensional Dynamic Contact Model for Cylindrical Gear Pairs with Distributed Tooth Flank Errors. Mech. Mach. Theory 2020, 152, 103930. [Google Scholar] [CrossRef]
- Stetter, R.; Göser, R.; Gresser, S.; Till, M.; Witczak, M. Fault-Tolerant Design for Increasing the Reliability of an Autonomous Driving Gear Shifting System. Eksploat. Niezawodn. Maint. Reliab. 2020, 22, 482–492. [Google Scholar] [CrossRef]
- Yuan, W.; Wang, H.; Guo, Q.; Wang, W.; Zhu, Y.; Yu, J.; Yang, X. Study on Wear Mechanism of Helical Gear by Three-Body Abrasive Based on Impact Load. Materials 2022, 15, 4135. [Google Scholar] [CrossRef]
- Miladinović, S.; Ivanovic, L.; Blagojevic, M.; Stojanovic, B. The development of magnetic gears for transportation applications. Mobil. Veh. Mech. 2017, 43, 41–55. [Google Scholar] [CrossRef]
- Tlali, P.M.; Wang, R.; Gerber, S. Magnetic Gear Technologies: A Review. In Proceedings of the 2014 International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 544–550. [Google Scholar]
- Armstrong, C.G. Power-Transmitting Device. US Patent 687292A, 26 November 1901. [Google Scholar]
- Li, X.; Chau, K.-T.; Cheng, M.; Hua, W. Comparison of Magnetic-Geared Permanent-Magnet Machines. Prog. Electromagn. Res. 2013, 133, 177–198. [Google Scholar] [CrossRef]
- Tsurumoto, K.; Kikuchi, S. A New Magnetic Gear Using Permanent Magnet. IEEE Trans. Magn. 1987, 23, 3622–3624. [Google Scholar] [CrossRef]
- Park, E.-J.; Jung, S.-Y.; Kim, Y.-J. Comparison of Magnetic Gear Characteristics Using Different Permanent Magnet Materials. IEEE Trans. Appl. Supercond. 2020, 30, 5200704. [Google Scholar] [CrossRef]
- Derbas, H.; Williams, J.; Koenig, A.; Pekarek, S. A Comparison of Nodal- and Mesh-Based Magnetic Equivalent Circuit Models. Energy Convers. IEEE Trans. 2009, 24, 388–396. [Google Scholar] [CrossRef]
- Boules, N. Two-Dimensional Field Analysis of Cylindrical Machines with Permanent Magnet Excitation. IEEE Trans. Ind. Appl. 1984, IA-20, 1267–1277. [Google Scholar] [CrossRef]
- Furlani, E. Analytical Analysis of Magnetically Coupled Multipole Cylinders. J. Phys. D Appl. Phys. 1999, 33, 28. [Google Scholar] [CrossRef]
- Wu, Y.-C.; Wang, C.-W. Transmitted Torque Analysis of a Magnetic Gear Mechanism with Rectangular Magnets. Appl. Math. Inf. Sci. 2015, 9, 1059–1065. [Google Scholar] [CrossRef]
- Wu, Y.-C.; Jian, B.-S. Magnetic Field Analysis of a Coaxial Magnetic Gear Mechanism by Two-Dimensional Equivalent Magnetic Circuit Network Method and Finite-Element Method. Appl. Math. Model. 2015, 39, 5746–5758. [Google Scholar] [CrossRef]
- Kołodziej, J.; Kowol, M.; Mynarek, P.; Gabor, R.; Łukaniszyn, M. Forces in Axial Flux Magnetic Gears with Integer and Fractional Gear Ratios. Energies 2021, 14, 855. [Google Scholar] [CrossRef]
- Wang, Y.; Filippini, M.; Bianchi, N.; Alotto, P. A Review on Magnetic Gears: Topologies, Computational Models, and Design Aspects. IEEE Trans. Ind. Applicat. 2019, 55, 4557–4566. [Google Scholar] [CrossRef]
- Ruiz-Ponce, G.; Arjona, M.A.; Hernandez, C.; Escarela-Perez, R. Design Optimization of an Axial Flux Magnetic Gear by Using Reluctance Network Modeling and Genetic Algorithm. Energies 2023, 16, 1852. [Google Scholar] [CrossRef]
- Ionel, D.M.; Eastham, J.F.; Miller, T.J.E.; Demeter, E. Design Considerations for Permanent Magnet Synchronous Motors for Flux Weakening Applications. IEE Proc. Electr. Power Appl. 1998, 145, 435–440. [Google Scholar] [CrossRef]
- Stumberger, B.; Stumberger, G.; Hadziselimovic, M.; Marcic, T.; Virtic, P.; Trlep, M.; Gorican, V. Design and Finite-Element Analysis of Interior Permanent Magnet Synchronous Motor With Flux Barriers. IEEE Trans. Magn. 2008, 44, 4389–4392. [Google Scholar] [CrossRef]
- Desvaux, M.; Latimier, R.L.G.; Multon, B.; Sire, S.; Ahmed, H.B. Analysis of the Dynamic Behaviour of Magnetic Gear with Nonlinear Modelling for Large Wind Turbines. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016; pp. 1332–1338. [Google Scholar]
- Hartung, S.; Rehberg, I. Dynamics of a Magnetic Gear with Two Cogging-Free Operation Modes. Arch. Appl. Mech. 2021, 91, 1423–1435. [Google Scholar] [CrossRef]
- Kołodziej, J. Analiza własności dynamicznych współosiowej przekładni magnetycznej. Electrotech. Rev. 2019, 1, 248–251. [Google Scholar] [CrossRef]
- Montague, R.; Bingham, C.; Atallah, K. Servo Control of Magnetic Gears. IEEE/ASME Trans. Mechatron. 2012, 17, 269–278. [Google Scholar] [CrossRef]
- Vournas, D. Modeling and Control of Magnetic Gear Dynamics in a Wind Turbine Drivetrain. Ph.D. Thesis, Portland State University, Portland, OR, USA, 2000. [Google Scholar]
- Macyszyn, Ł.; Staniek, R.; Myszkowski, A. Przekładnia Magnetyczna Precesyjna. PL Patent 229502, 2 March 2018. [Google Scholar]
- Macyszyn, L.; Jedryczka, C.; Myszkowski, A. Simulation and Experimental Investigation of a Two-Stage Magnetic Precession Gear. Energies 2021, 14, 1838. [Google Scholar] [CrossRef]
- Macyszyn, Ł.; Jedryczka, C.; Staniek, R. Design and Finite Element Analysis of Novel Two-Stage Magnetic Precession Gear. Int. J. Simul. Model. 2019, 18, 586–595. [Google Scholar] [CrossRef]
- Macyszyn, Ł.; Myszkowski, A.; Staniek, R.; Pabiszczak, S. Two-Stage Precession Type Gear: Design, Geometric and Kinematic Analysis. In Proceedings of the American Society of Mechanical Engineers Digital Collection, Tampa, FL, USA, 3–9 November 2017. [Google Scholar]
- Coulomb, J. A Methodology for the Determination of Global Electromechanical Quantities from a Finite Element Analysis and Its Application to the Evaluation of Magnetic Forces, Torques and Stiffness. IEEE Trans. Magn. 1983, 19, 2514–2519. [Google Scholar] [CrossRef]






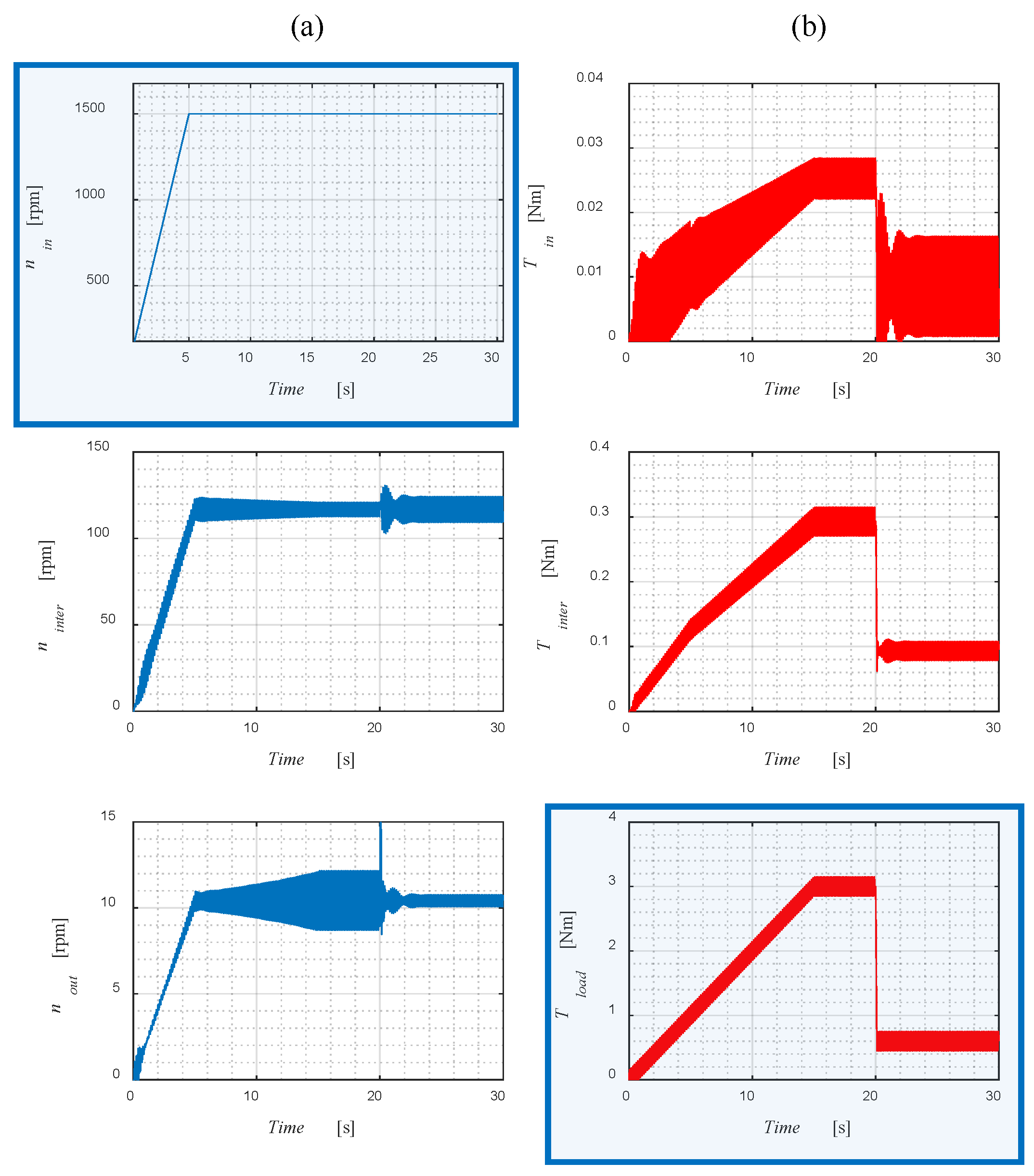
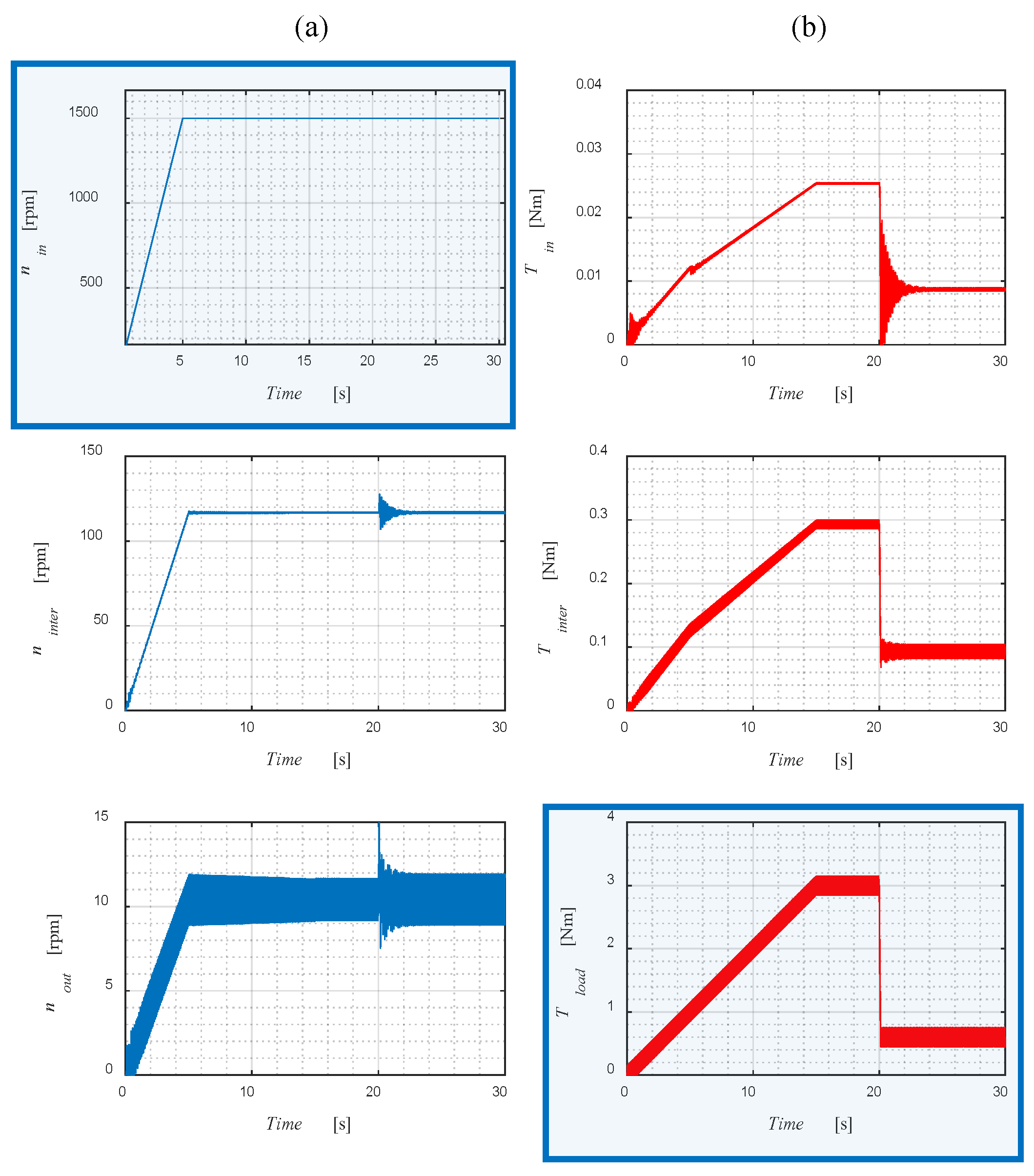
| fr [Hz] | [Nm] | Torque Ripple Factor | [rpm] | Speed Ripple Factor |
|---|---|---|---|---|
| 1 | 0.0253 | 8.70 | 10.35 | 3.86 |
| 7 | 0.0253 | 25.69 | 10.35 | 32.85 |
| 15 | 0.0253 | 1.58 | 10.35 | 24.54 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Macyszyn, L.; Jedryczka, C.; Mysinski, M. Analysis of a Two-Stage Magnetic Precession Gear Dynamics. Energies 2023, 16, 4484. https://doi.org/10.3390/en16114484
Macyszyn L, Jedryczka C, Mysinski M. Analysis of a Two-Stage Magnetic Precession Gear Dynamics. Energies. 2023; 16(11):4484. https://doi.org/10.3390/en16114484
Chicago/Turabian StyleMacyszyn, Lukasz, Cezary Jedryczka, and Michal Mysinski. 2023. "Analysis of a Two-Stage Magnetic Precession Gear Dynamics" Energies 16, no. 11: 4484. https://doi.org/10.3390/en16114484
APA StyleMacyszyn, L., Jedryczka, C., & Mysinski, M. (2023). Analysis of a Two-Stage Magnetic Precession Gear Dynamics. Energies, 16(11), 4484. https://doi.org/10.3390/en16114484








