Research on the Dynamic Response of a Steel Catenary Riser in the Touchdown Zone under Pigging Conditions
Abstract
:1. Introduction
2. Materials and Methods
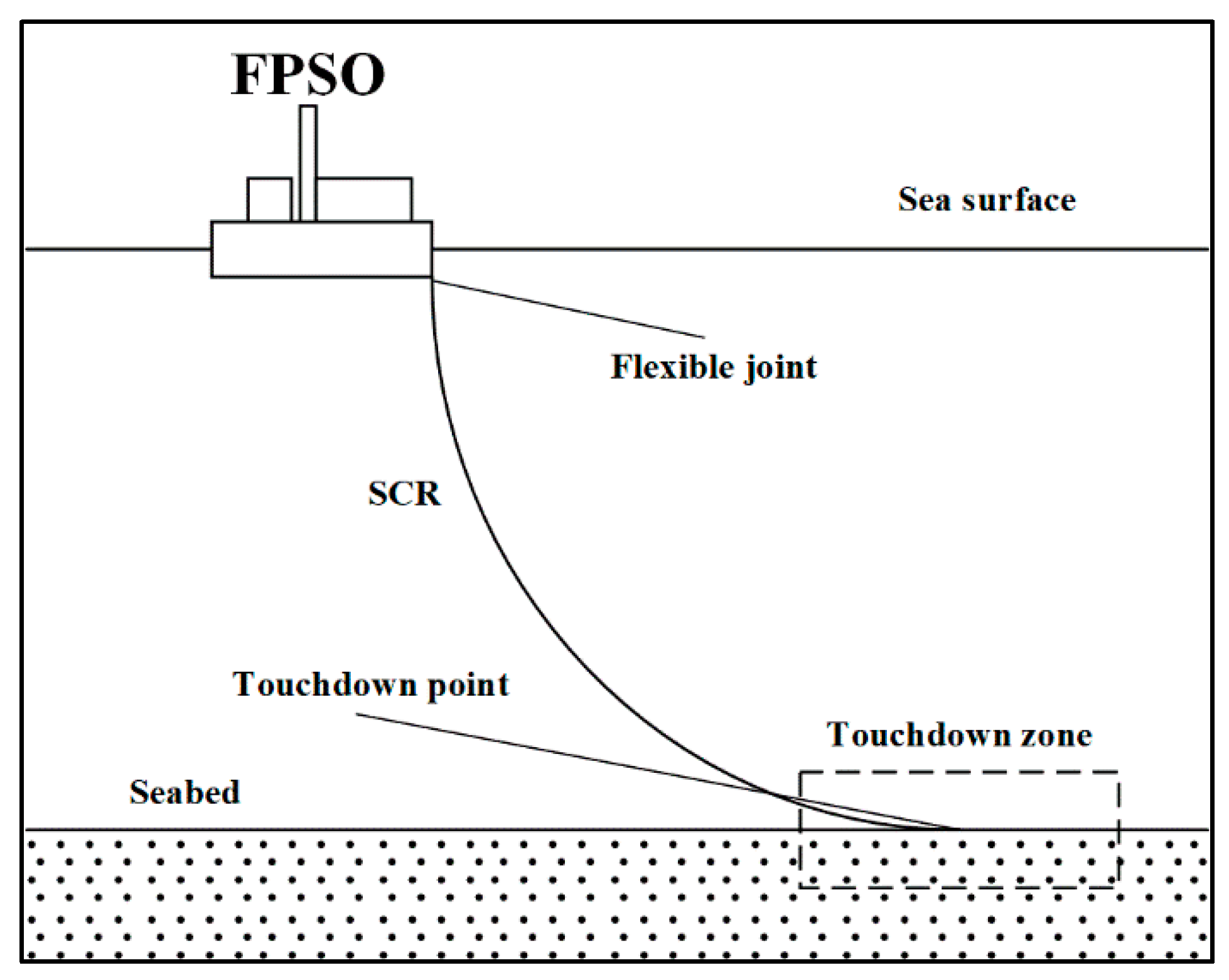
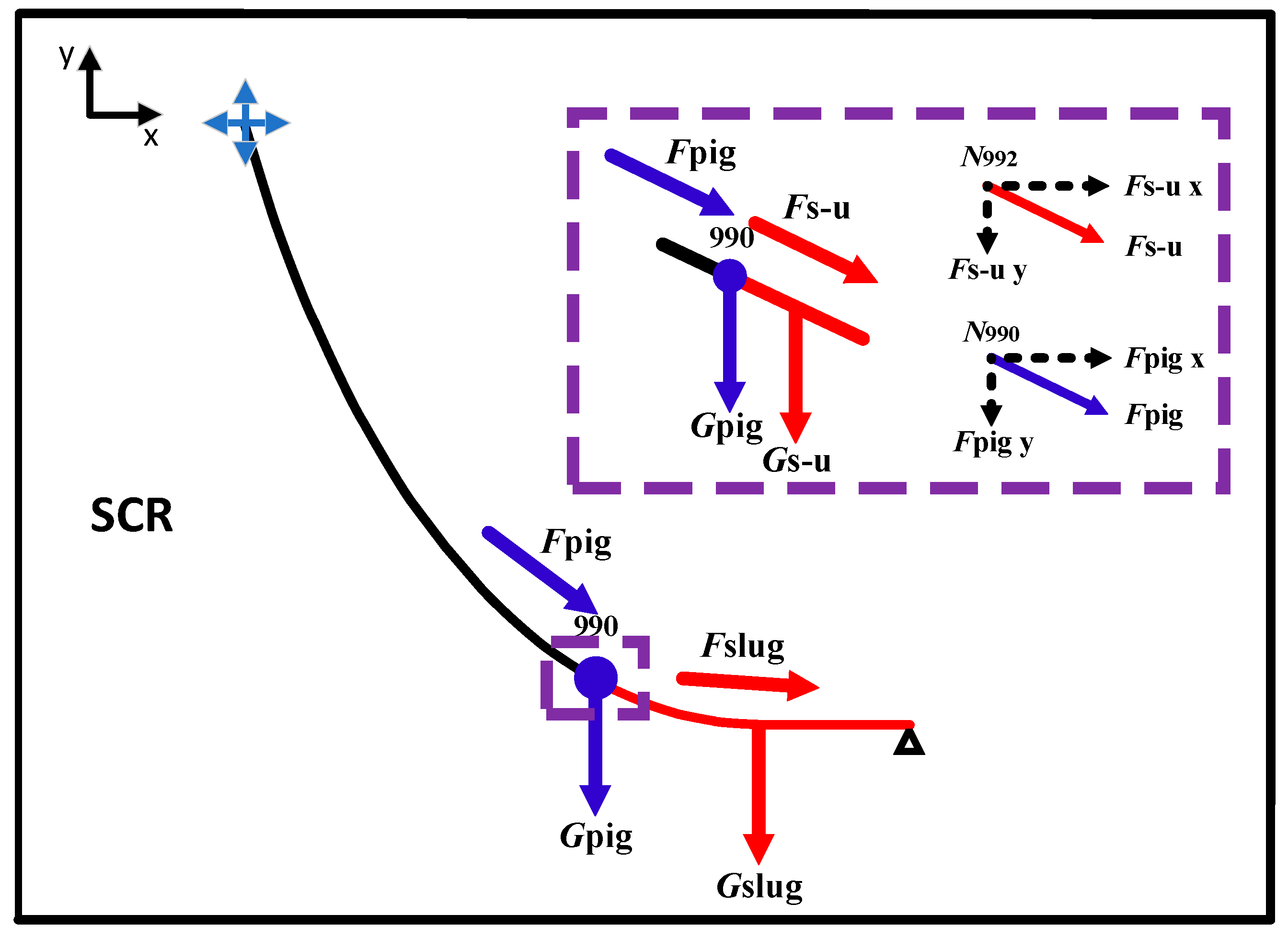
2.1. Model Introduction
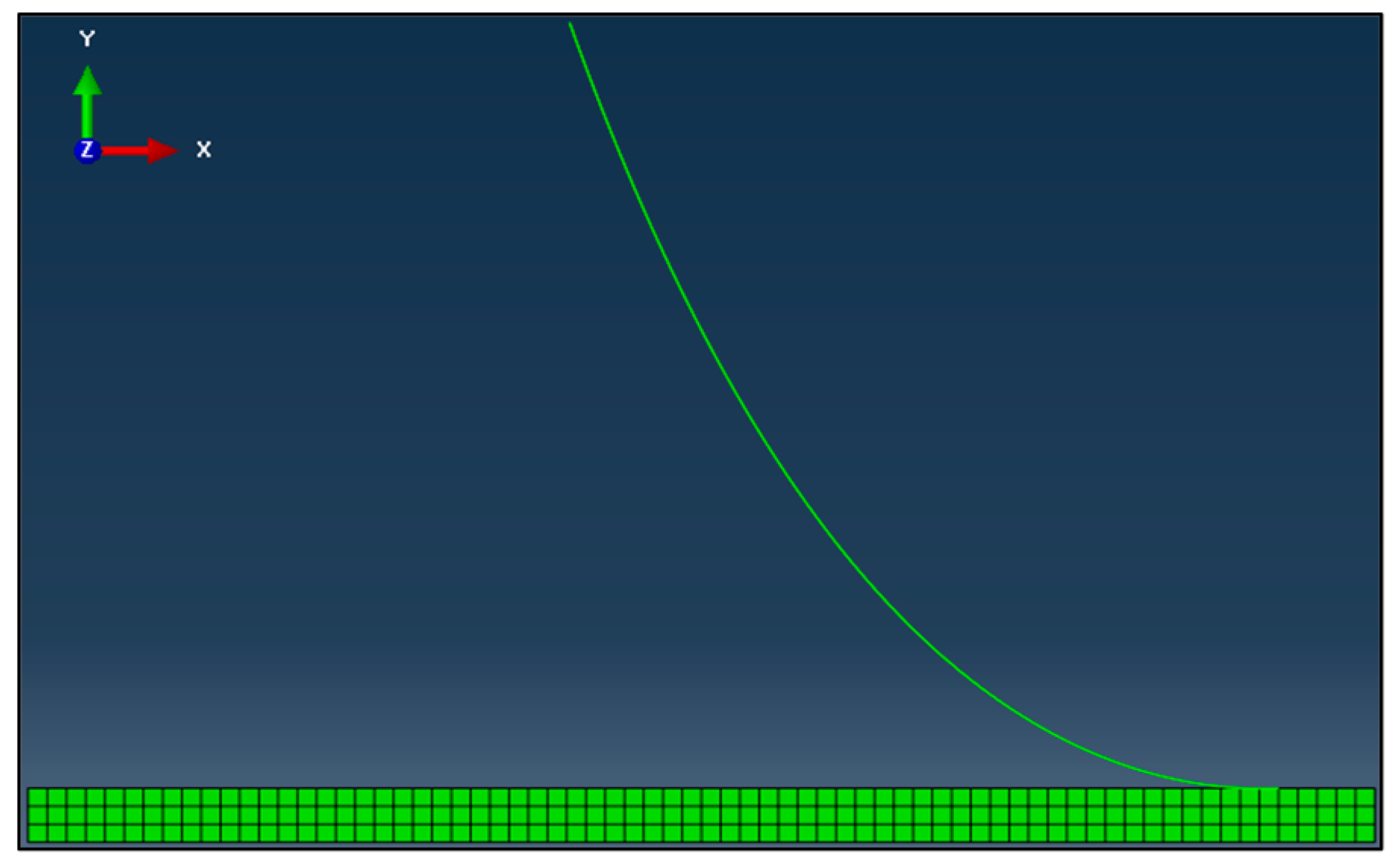
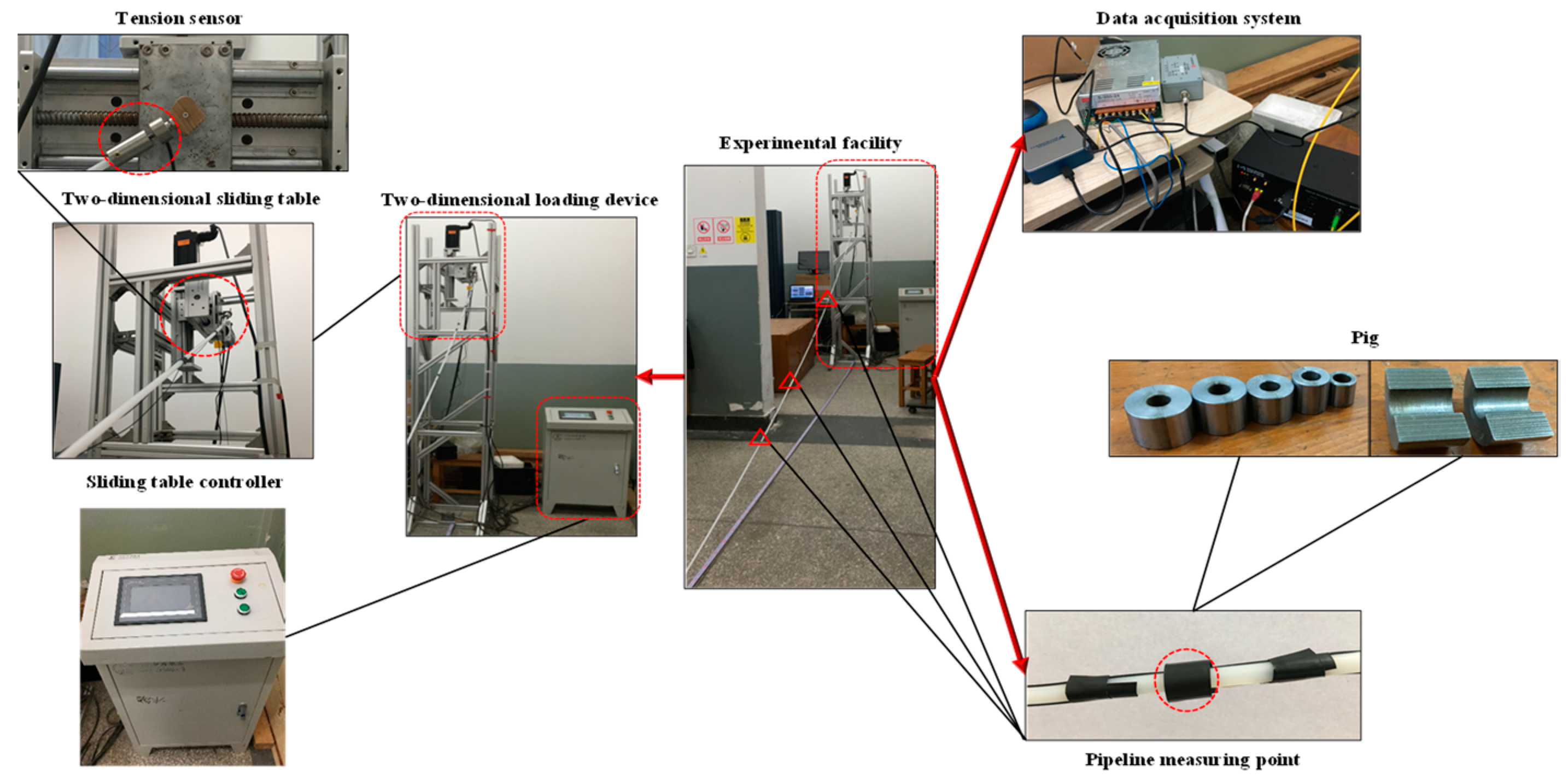
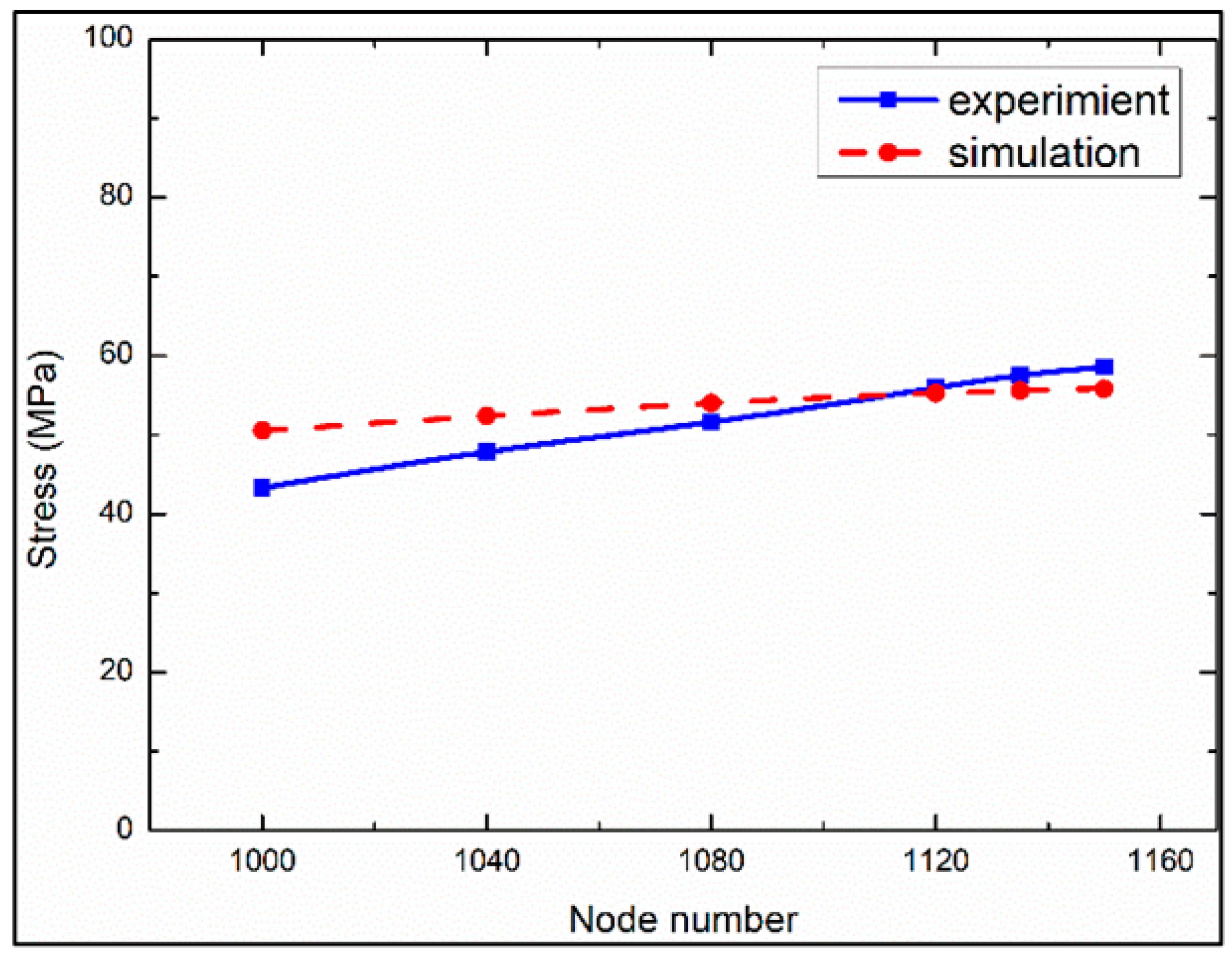
2.2. Model Validation
3. Simulation Results
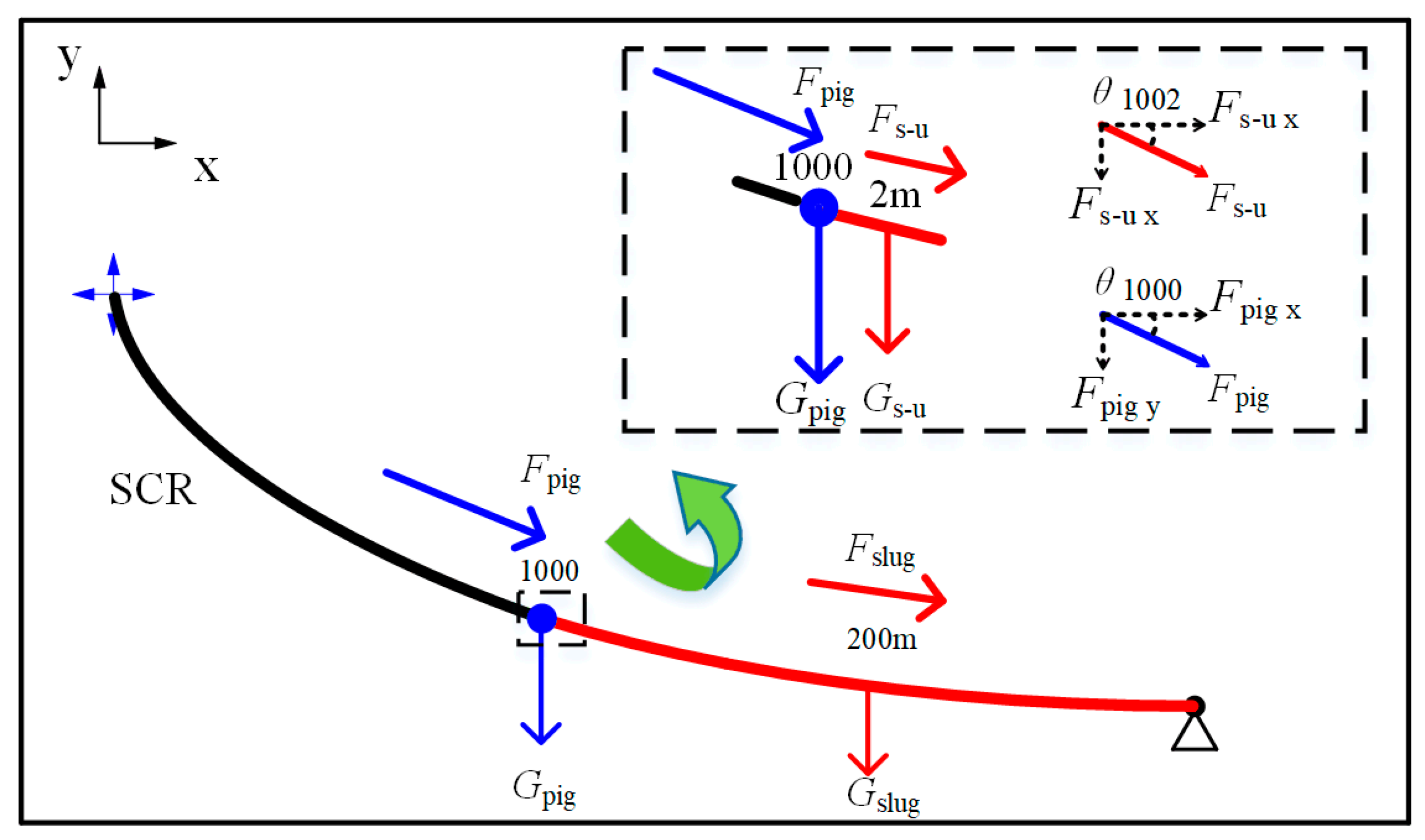
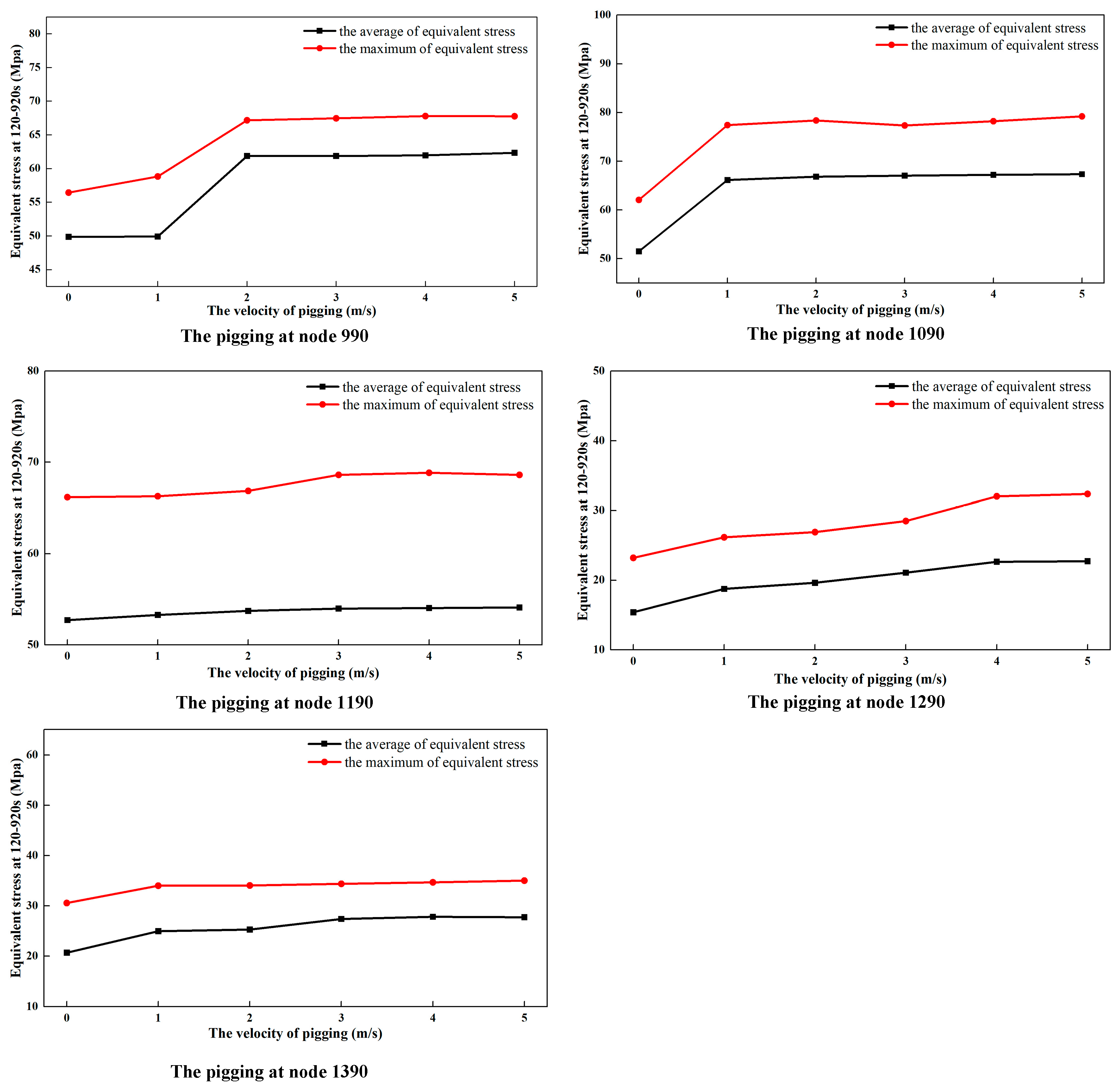
3.1. The Effect of the Pigging Impact Load on the SCR Stress Variation with no Pigging-Induced Slug Load
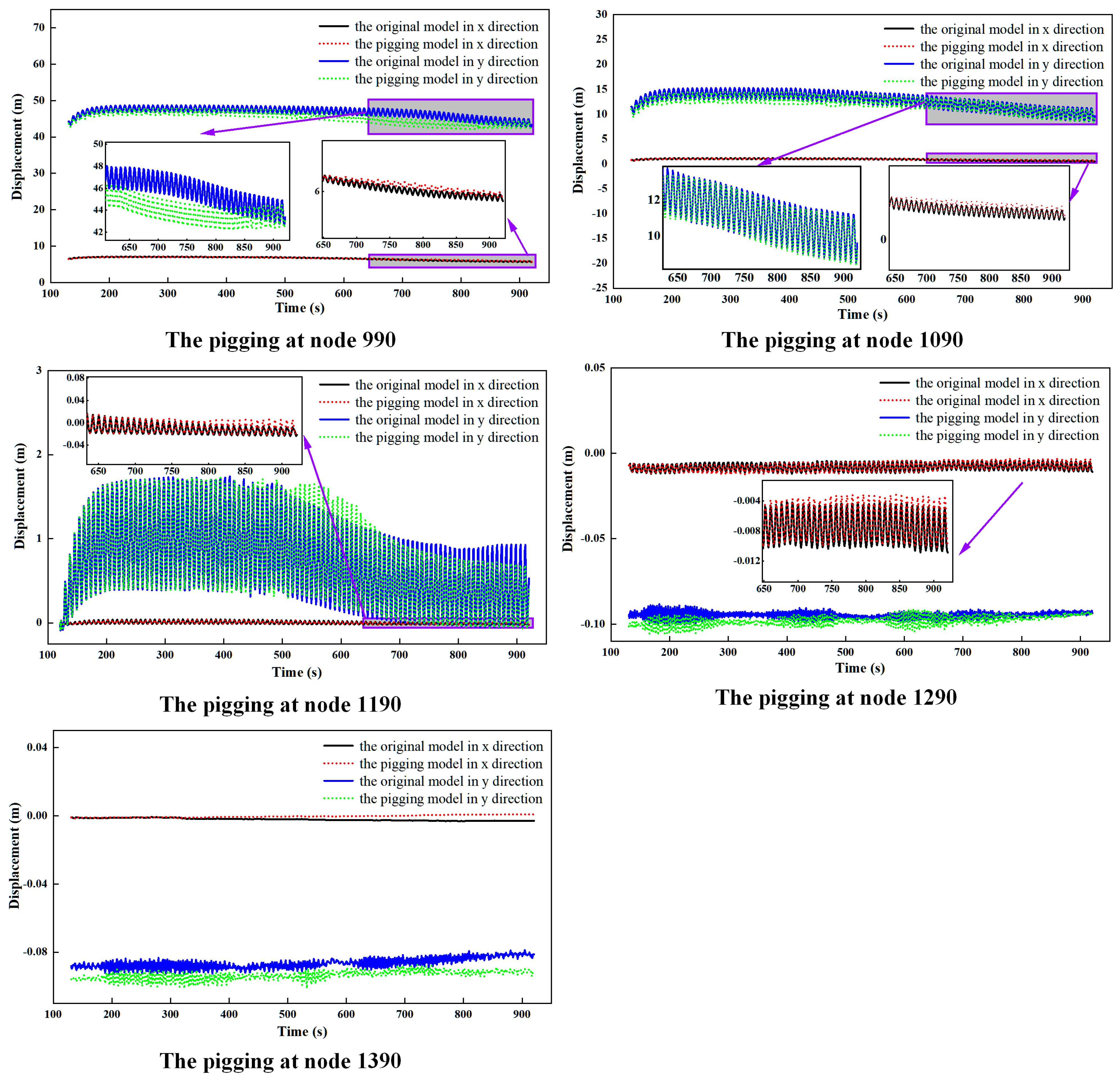
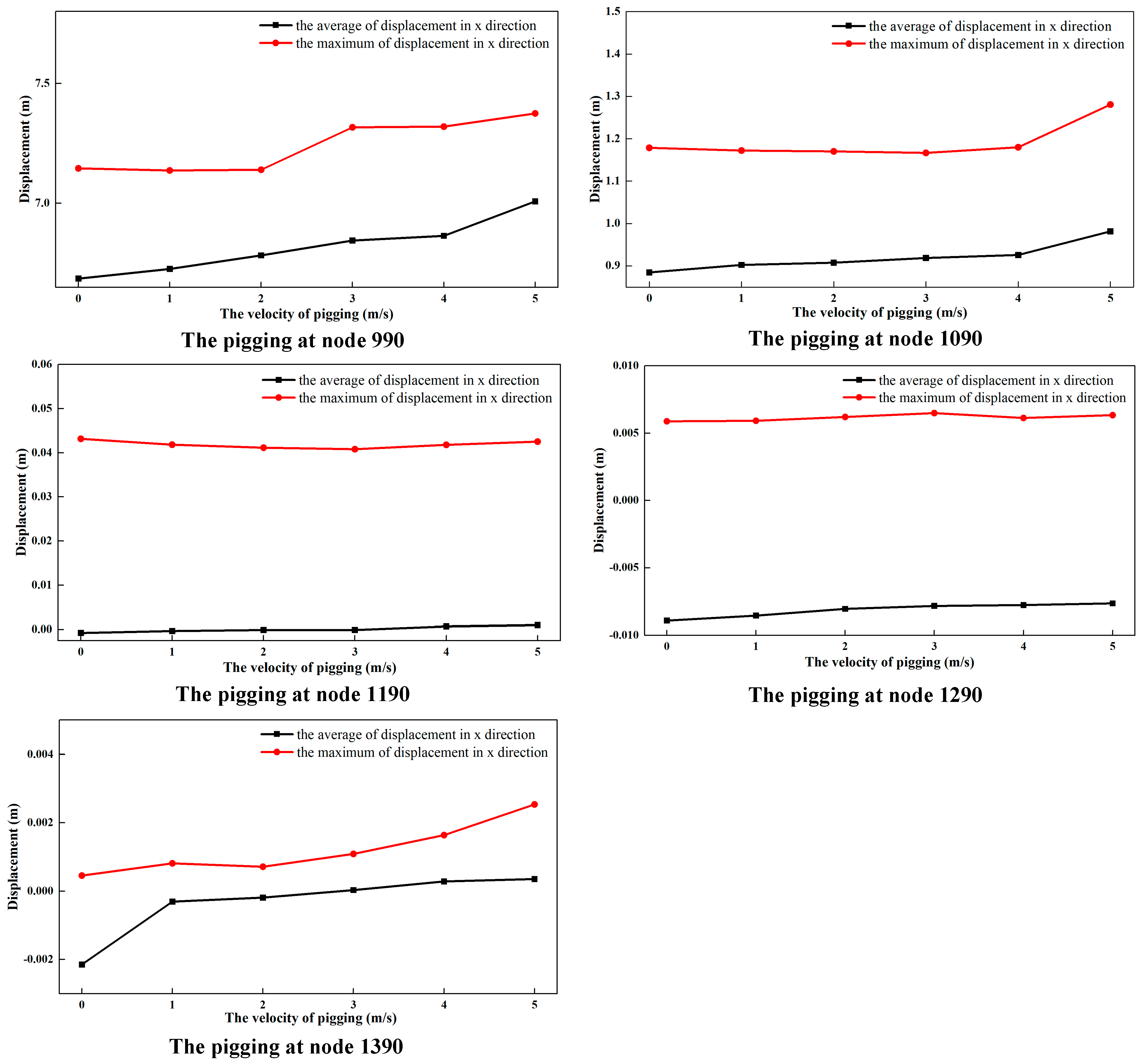
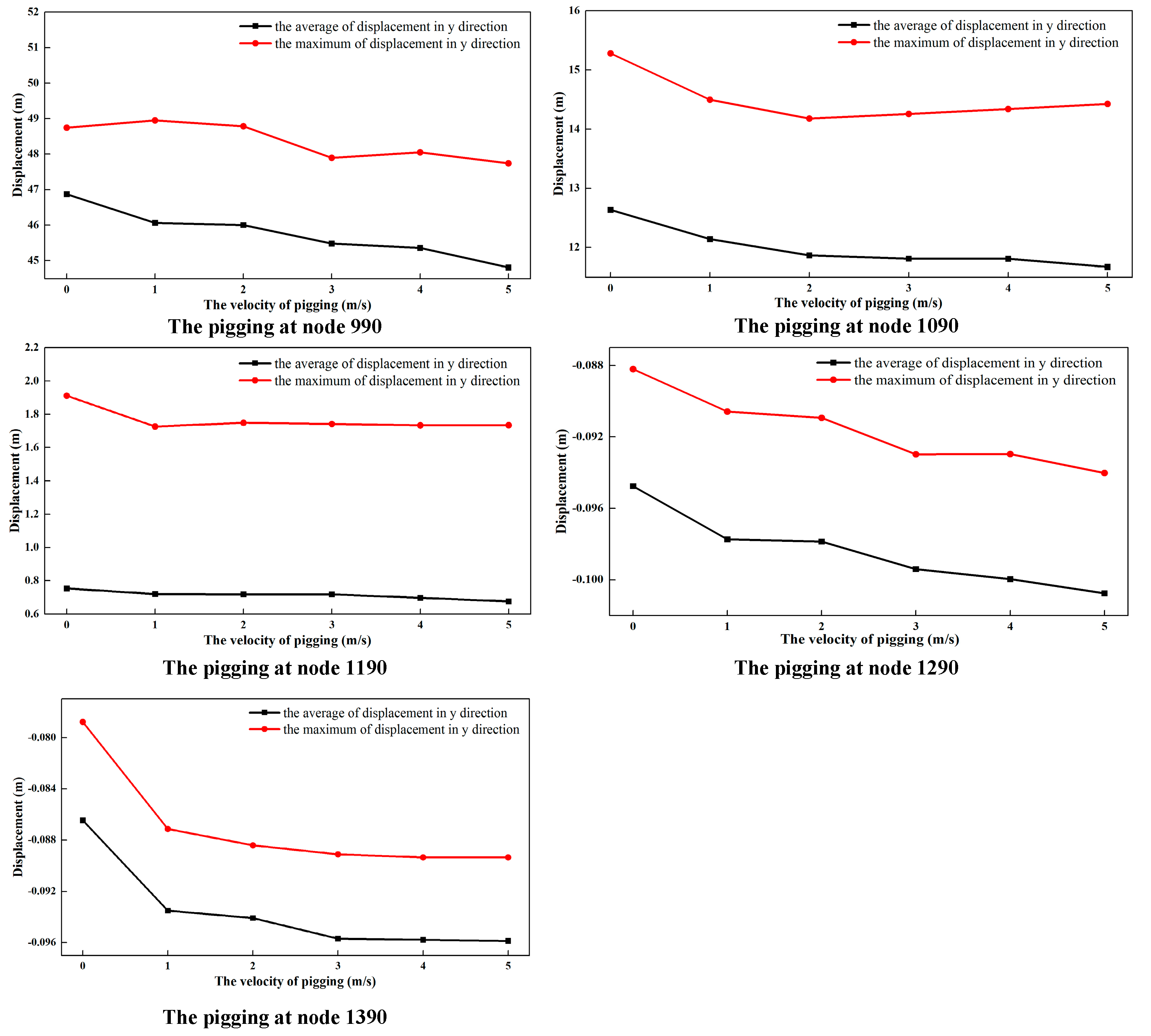
3.2. The Effect of the Pigging Impact Load on the SCR Displacement Variation with no Pigging-Induced Slug Load
3.3. The Effect of Multiple Loads on the SCR Stress Variation, Considering the Pigging-Induced Slug Load
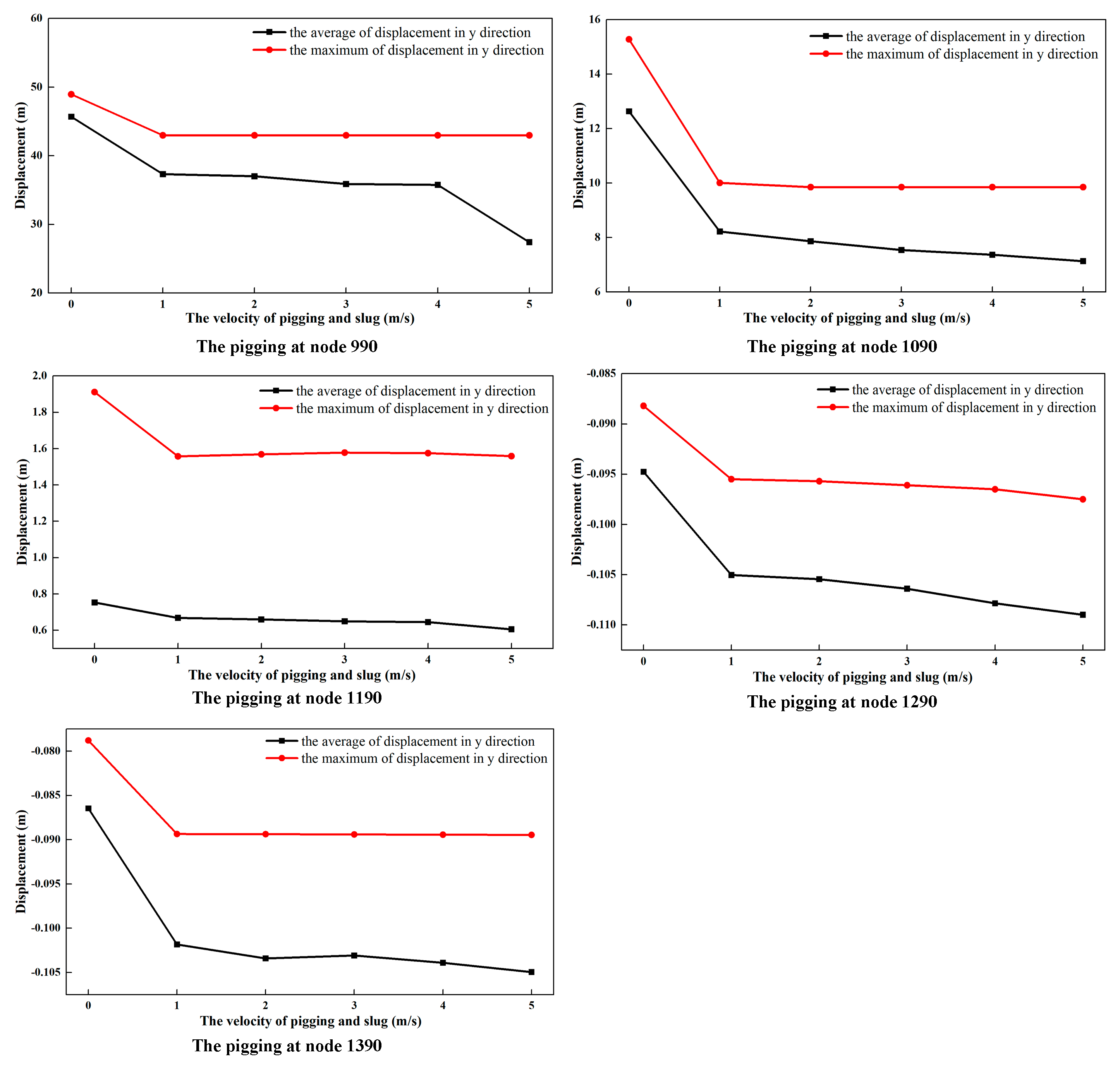
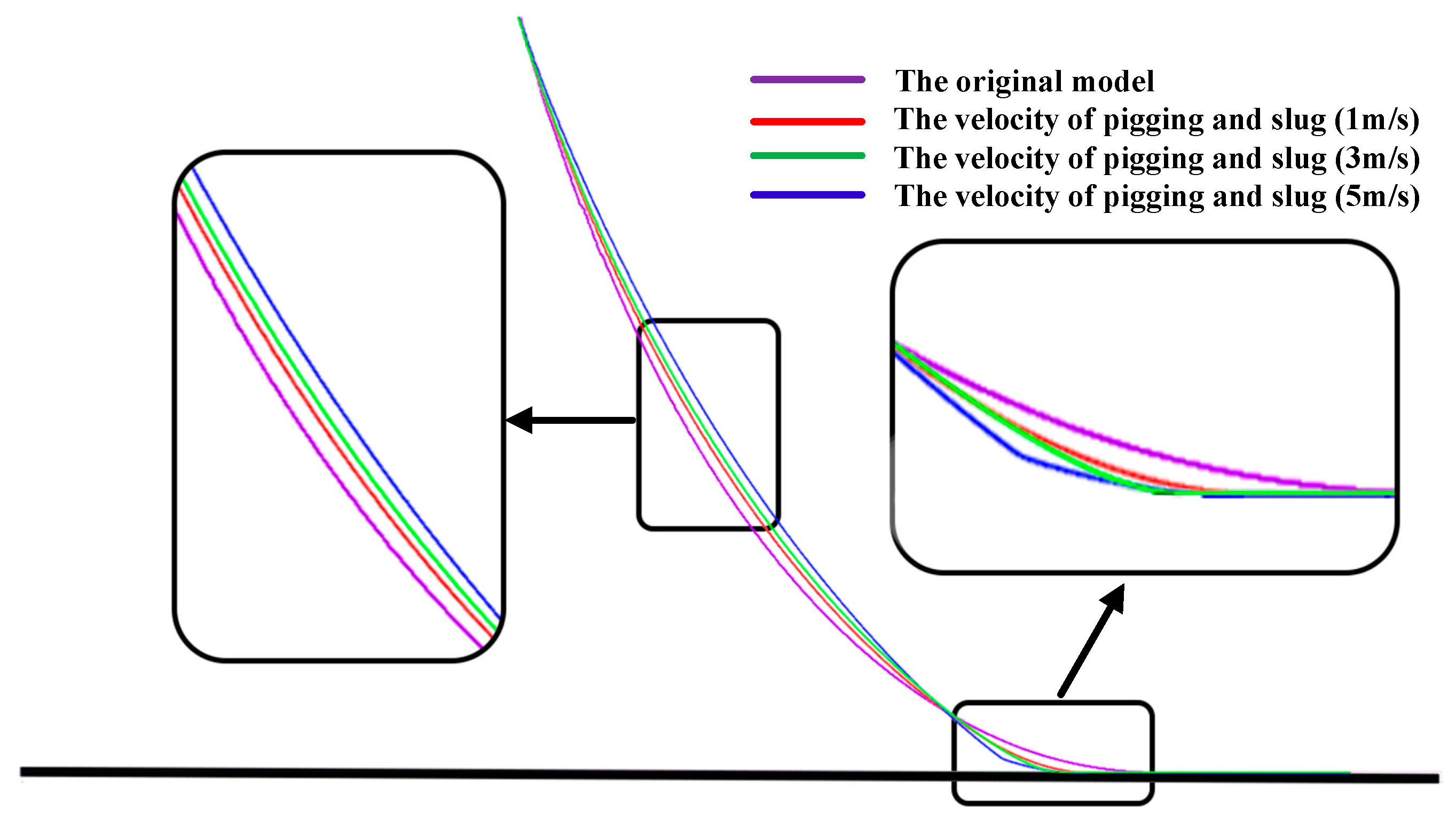
3.4. The Effects of Multiple Loads on the SCR Displacement Variation, Considering the Pigging-Induced Slug Load
4. Conclusions
- (1)
- During the pigging of the SCR TDZ, the amplitude of the equivalent stress increased due to the pigging load, and a 106.76% maximum increment was observed. The closer to the touchdown point, the more obvious the trend. However, the periodic variation law of the equivalent stress is not affected by the pigging impact load, which is mainly determined by the effects of the waves, currents, and floating platform movements.
- (2)
- The displacement of the SCR TDZ in the Y direction was significantly larger than that in the X direction due to the catenary properties. The displacement of the nodes on the left part of the TDP underwent a large offset and fluctuated regularly. The displacement of the right part of the TDP was quite small due to the support of the seabed.
- (3)
- When the slug flow was considered in the pigging of the SCR TDZ, the displacement of the node on the left the TDP in the X direction experienced an offset to the left, while the nodes on the right of the TDP underwent a right offset. Downward displacements in the Y direction was found for all the nodes and was positively correlated with the initial velocity of the pig. Here, a 2.51 m absolute displacement in the X direction and a 18.29 m absolute displacement in the Y direction were observed at node 990 when the pigging velocity increased from 0 m/s to 5 m/s. The research results indicate that the potential damage of the pigging to the riser was limited, compared with the fatigue failure due to the periodic motion of the SCR.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Y.B.; Gao, D.L.; Fang, J. Static analysis of deep-water marine riser subjected to both axial and lateral forces in its installation. J. Nat. Gas Sci. Eng. 2014, 19, 84–90. [Google Scholar] [CrossRef]
- Bai, X.L.; Chen, K.; Xie, Y.H. Experimental study on the pipe-soil interaction in the contact zone of steel catenary riser. Shipbuild. China 2016, 57, 112–119. [Google Scholar]
- Wang, J.; Fu, S.; Baarholm, R.; Wu, J.; Larsen, C.M. Out-of-plane vortex-induced vibration of a steel catenary riser caused by vessel motions. Ocean Eng. 2015, 109, 389–400. [Google Scholar]
- Mekha, B.B. New Frontiers in the Design of Steel Catenary Risers for Floating Production Systems. J. Offshore Mech. Arct. Eng. 2001, 123, 153–158. [Google Scholar]
- Willis, N.R.T.; Thethi, K.S.; 2H Offshore Engineering Ltd. Stride JIP: Steel risers in deepwater environments—Progress summary. Offshore Technol. Conf. 1999, OTC 10974. [Google Scholar] [CrossRef]
- Hodder, M.S.; Byrne, B.W. 3D experiments investigating the interaction of a model SCR with the seabed. Appl. Ocean Res. 2010, 32, 146–157. [Google Scholar]
- Elliott, B.J.; Zakeri, A.; Barrett, J.; Hawlader, B.; Li, G.; Clukey, E.C. Centrifuge modeling of steel catenary risers at touchdown zone part II: Assessment of centrifuge test results using kaolin clay. Ocean Eng. 2013, 60, 208–218. [Google Scholar]
- Elliott, B.J.; Zakeri, A.; Macneill, A.; Phillips, R.; Clukey, E.C.; Li, G. Centrifuge modeling of steel catenary risers at touchdown zone part I: Development of novel centrifuge experimental apparatus. Ocean Eng. 2013, 60, 200–207. [Google Scholar]
- Gao, Y.; Fu, S.; Ma, L.; Chen, Y. Experimental investigation of the response performance of VIV on a flexible riser with helical strakes. Ships Offshore Struct. 2014, 11, 113–128. [Google Scholar]
- Wang, Y.; Duan, M.L.; Zhang, Y. Experimental and numerical investigation of vertical pipe–soil interaction considering pipe velocity. Ships Offshore Struct. 2015, 12, 77–85. [Google Scholar] [CrossRef]
- Yao, R.; Bai, X.L.; Xie, Y.H. Small size three-dimensional experimental study of interaction between steel catenary riser and seabed. Ocean Eng. 2015, 33, 93–99. [Google Scholar]
- Bai, X.; Vaz, M.A.; Morooka, C.K.; Xie, Y. Dynamic tests in a steel catenary riser reduced scale model. Ships Offshore Struct. 2017, 12, 1064–1076. [Google Scholar]
- Yu, Y.; Xu, S.; Yu, J.; Xu, L.; Liu, X.; Liu, P. Experimental Study of the Dynamic Performance of Steel Catenary Riser within the Touchdown Zone. J. Mar. Sci. Eng. 2023, 11, 151. [Google Scholar] [CrossRef]
- Li, F.Z.; Low, Y.M. Influence of low-frequency vessel motions on the fatigue response of steel catenary risers at the touchdown point. Ships Offshore Struct. 2012, 9, 134–148. [Google Scholar]
- Guo, L.; Duan, M.; Wang, Y.; Yu, F. Experimental investigation on dynamic model testing of a deep-water riser support by truncated hybrid method. Ships Offshore Struct. 2013, 9, 344–353. [Google Scholar] [CrossRef]
- Shiri, H. Influence of seabed trench formation on fatigue performance of steel catenary risers in touchdown zone. Mar. Struct. 2014, 36, 1–20. [Google Scholar] [CrossRef]
- Yang, H.Z.; Xiao, F. Instability analyses of a top-tensioned riser under combined vortex and multi-frequency parametric excitations. Ocean Eng. 2014, 81, 12–28. [Google Scholar] [CrossRef]
- Elosta, H.; Huang, S.; Incecik, A. Trenching effects on structural safety assessment of integrated riser/semisubmersible in cohesive soil. Eng. Struct. 2014, 77, 57–64. [Google Scholar] [CrossRef]
- Dong, Y.; Sun, L. Fatigue analysis of steel catenary risers based on a plasticity model. J. Mar. Sci. Appl. 2015, 14, 76–82. [Google Scholar]
- Thorsen, M.J.; Savik, S.; Larsen, C.M. Fatigue damage from time domain simulation of combined in-line and cross-flow vortex-induced vibrations. Mar. Struct. 2015, 41, 200–222. [Google Scholar] [CrossRef]
- Bai, X.; Huang, W.; Vaz, M.A.; Yang, C.; Duan, M. Riser-soil interaction model effects on the dynamic behavior of a steel catenary riser. Mar. Struct. 2015, 41, 53–76. [Google Scholar] [CrossRef]
- Kim, S.; Kim, M.-H. Dynamic behaviors of conventional SCR and lazy-wave SCR for FPSOs in deepwater. Ocean Eng. 2015, 106, 396–414. [Google Scholar]
- Zhang, J.; Tang, Y. Parametric instability analysis of deepwater top-tensioned risers considering variable tension along the length. J. Ocean Univ. China 2015, 14, 59–64. [Google Scholar]
- Gong, S.; Xu, P. Influences of pipe–soil interaction on dynamic behaviour of deepwater S-lay pipeline under random sea states. Ships Offshore Struct. 2016, 12, 370–387. [Google Scholar]
- Wang, K.; Ji, C.; Xue, H.; Tang, W. Fatigue sensitivity analysis of steel catenary riser near touchdown point. J. Shanghai Jiaotong Univ. (Sci.) 2017, 22, 570–576. [Google Scholar] [CrossRef]
- Sun, L.; Zhang, Y.; Ma, G.; Wang, H. Nonlinear dynamic analysis of deepwater risers with the irregular seabed. Offshore Arct. Eng. 2017, OMAE-62531. [Google Scholar] [CrossRef]
- Dong, X.; Shiri, H. Performance of non-linear seabed interaction models for steel catenary risers, part I: Nodal response. Ocean Eng. 2018, 154, 153–166. [Google Scholar]
- Dong, X.; Shiri, H. Performance of non-linear seabed interaction models for steel catenary risers, part II: Global response. Appl. Ocean Res. 2019, 82, 158–174. [Google Scholar]
- Liu, Z.; Guo, H.Y. Dynamic response study of steel catenary riser based on slender rod model. China Ocean Eng. 2019, 33, 57–64. [Google Scholar] [CrossRef]
- Liu, Y.; Zhu, X.; Song, J.; Wu, H.; Zhang, S.; Zhang, S. Research on bypass pigging in offshore riser system to mitigate severe slugging. Ocean Eng. 2022, 246, 110606. [Google Scholar]
- Queau, L.M.; Kimiaei, M.; Randolph, M.F. Dimensionless groups governing response of steel catenary risers. Ocean Eng. 2013, 74, 247–259. [Google Scholar] [CrossRef]
- Jayawardena, S.; Dykhno, L.; Hudson, J. Challenges in pigging subsea gas flowlines. Soc. Pet. Eng. 2002, SPE-77576. [Google Scholar] [CrossRef]








| Physical Model | Physical Properties | Value |
|---|---|---|
| SCR | Length/m | 1200 |
| Outer diameter/m | 0.1684 | |
| Wall thickness/m | 0.0069 | |
| Density/(kg/m3) | 7850 | |
| Young’s modulus/GPa | 200.0 | |
| Poisson’s ratio | 0.3 | |
| Yield strength/MPa | 448 | |
| Ocean environment | Depth of sea water/m | 850 |
| Density of sea water/(kg/m3) | 1020 | |
| Seabed stiffness/(kN/m2) | 200 |
| Unit Size of SCR (m) | Unit Size of the Seabed (m) | Grid Numbers | Stress (MPa) |
|---|---|---|---|
| 2 | 10 | 740 | 63.56 |
| 2 | 5 | 1160 | 63.43 |
| 1 | 3 | 2601 | 51.35 |
| 1 | 2.5 | 3240 | 57.46 |
| 1 | 2 | 4700 | 55.54 |
| 1 | 1.5 | 7731 | 55.75 |
| Pig Weight (kg) | Pigging Velocity (m/s) | Pigging Impact Load (m × a, kg × m/s2) | Number of Nodes Applied on |
|---|---|---|---|
| 80 | 1 | 80 × 1 × 9.8/0.1 | 990, 1090, 1190, 1290, and 1390 |
| 2 | 80 × 2 × 9.8/0.1 | ||
| 3 | 80 × 3 × 9.8/0.1 | ||
| 4 | 80 × 4 × 9.8/0.1 | ||
| 5 | 80 × 5 × 9.8/0.1 |
| Pig Weight (m, kg) | Slugging Length (m) | Velocity of Pig and Slugging (m/s) | Pigging Impact Load (m × a, kg × m/s2) | Slugging Impact Load (m × a, kg × m/s2) | Number of Nodes Applied on | |
|---|---|---|---|---|---|---|
| 80 | 410 | 1 | 80 × 9.8 × 1/0.1 | m × 9.8 × 1/0.1 | 990, 1090, 1190, 1290 and 1390 | |
| 310 | ||||||
| 110 | ||||||
| 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, X.; Fu, Y.; Wang, Y.; Wang, L.; Lao, L. Research on the Dynamic Response of a Steel Catenary Riser in the Touchdown Zone under Pigging Conditions. Energies 2023, 16, 5832. https://doi.org/10.3390/en16155832
Zhu X, Fu Y, Wang Y, Wang L, Lao L. Research on the Dynamic Response of a Steel Catenary Riser in the Touchdown Zone under Pigging Conditions. Energies. 2023; 16(15):5832. https://doi.org/10.3390/en16155832
Chicago/Turabian StyleZhu, Xiaoxiao, Yunlei Fu, Yutao Wang, Lulu Wang, and Liyun Lao. 2023. "Research on the Dynamic Response of a Steel Catenary Riser in the Touchdown Zone under Pigging Conditions" Energies 16, no. 15: 5832. https://doi.org/10.3390/en16155832
APA StyleZhu, X., Fu, Y., Wang, Y., Wang, L., & Lao, L. (2023). Research on the Dynamic Response of a Steel Catenary Riser in the Touchdown Zone under Pigging Conditions. Energies, 16(15), 5832. https://doi.org/10.3390/en16155832






