Identification of Lossy Y-Type Two-Port Circuit Models under Measurement Uncertainties: Closed-Form Solution and Statistical–Perturbative Characterization

Abstract

The present paper treats a black-box estimation of the three independent parameters of a reciprocal lossy two-port network whose terminals are supposed to be accessible to an impedance measurement device. The discussed estimation method is based on the availability of a number of data pairs made of external load admittances paired to equivalent external admittances affected by measurement errors. The proposed method is framed as a squared-estimation-error minimization problem that leads to a system of three nonlinear equations in the three unknown parameters. A key observation is, however, that a core subsystem of two equations may be turned exactly to a linear form and hence may be solved in closed form. The purely real-valued case is treated first since it serves to clarify the optimization problem at hand and the structure of its solution. In the purely real-valued case, a statistical analysis is carried out as well, which affords the evaluation of the effects of the measurement errors. The results of the statistical analysis afford quantifying the dependence of the estimation errors from the number of samples and from the variance of the measurement errors. Subsequently, the full complex-valued case is treated. Results of numerical simulations complement and illustrate the theoretical findings. The obtained numerical results confirm the statistical analysis and that the proposed external identification method is effective.

1. Introduction

Linear two-port and multiport networks have found widespread application in engineering and in applied sciences to model multivariable interaction phenomena. A recent reference on multiport networks and on their energy-exchange properties published on Energies is [1], while a general-purpose textbook that includes multiport elements is [2].

Multiport networks have been used in the modeling of complex mechanical systems [3], in waveguides modeling and calibration [4] as well as in antennas modeling and optimization [5], in modeling bioheat transfers in lungs (in particular during thoracic and open-heart surgery) [6], in the analysis of power delivery in electrical railways systems [7], in modeling low dropout voltage regulators [8] as well as in modeling and analyzing multiple grid-connected voltage source converter systems [9], in the modeling of electrochemical transport processes through biological membranes [10], as well as in the modeling and simulation of acoustic systems [11] even by two-port circuit models derived from the linearized Navier–Stokes equations [12]. In addition, nonlinear multiterminal electronic devices or portions of electronic circuits may be represented, in the small-signal hypothesis, as linear multiport networks.

Most black-box modeling techniques of linear interaction phenomena involve two-port circuits. However, multiport circuit models are of prime importance when a large number of variables are involved in an interaction phenomenon. As a specific application to exemplify the usefulness of multiport identification, consider the electronic chip package/connector de-embedding problem tackled in [13]. The plastic package of an electronic chip embeds a number of highly conductive wires whose function is to connect the internal electronic chip to the external pins. At very high frequencies (in the range of GHz), a number of parasitic effects show up and, in particular, the connecting wires interact to each other electromagnetically in such a way that they may no longer be regarded as simple wires. In [13], the package is modeled as a reciprocal, linear, time-invariant multiport network, which is supposed to be accessible by n internal electrical ports and n external electrical ports. The identification of the circuit model, represented through its $2n\times 2n$ admittance matrix, is carried out by measuring the admittance of the external ports by a vector network analyzer. Once identified, the model is used to de-embed the internal chip, namely, to decouple the signals to/from the chip’s pins which become mixed up by the connector. A further example concerns modeling microvascular networks [14], where two-port models are used to compute biohydraulic quantities related to fluid circulation in thin blood vessels. In such an application, hence, neither the modeled phenomenon nor the variables of interest are of electric type.

Two-port networks turn out to be useful tools that afford analyzing the behavior and performance of linear networks that may be accessed by two pairs of terminals. Two-port network models are electrical circuits that embody a mathematical representation of the relationship occurring between the two independent voltages and two independent currents at their terminals. There exist six common types of two-port network models, classified as impedance, admittance, hybrid, and transmission type [15,16].

One of the challenges of using two-port network models is identifying the values of their independent parameters. There exist different methods to accomplish such a task, such as short-circuit, open-circuit, and known-load tests [2]. Each method implies applying known voltages and currents to the terminals and measuring the resulting voltages and currents. The choice of one specific method depends on the feasibility and accuracy of the available measurement devices. In the present research work, we deal specifically with the problem of the external identification of the three independent parameters of a reciprocal, lossy Y-type two-port network. Lossy networks characterize, among others, transmission media that entail some form of energy dissipation, such as energy loss due to nonideal conducting walls [17,18].

A basic assumption behind the present research is that the terminals of the two-port network are accessible to an impedance-measurement analyzer. Through such device, a number of test loads may be subjected to measurement of admittance. Next, such known loads may be connected to one of the ports of the network, and the equivalent admittance at the other port may be measured. In this way, a dataset may be collected, made of external load admittance values paired to equivalent external admittance values.

The collected data pairs are supposed to be affected by measurement errors whose entity depends on the quality of the measurement device and on the competence in the investigation. Whenever the measurement errors affecting the data available to accomplish the identification task are not negligible, the classical identification methods may be severely affected in a negative way. (In dimensions higher than two, such problem is even more severe, as illustrated, for instance, in [1].) In these instances, alternative identification methods may, hence, prove viable. A summary of three methodologies to accomplish identification are summarized in

Table 1. Advantages and drawbacks of three categories of external identification methods.

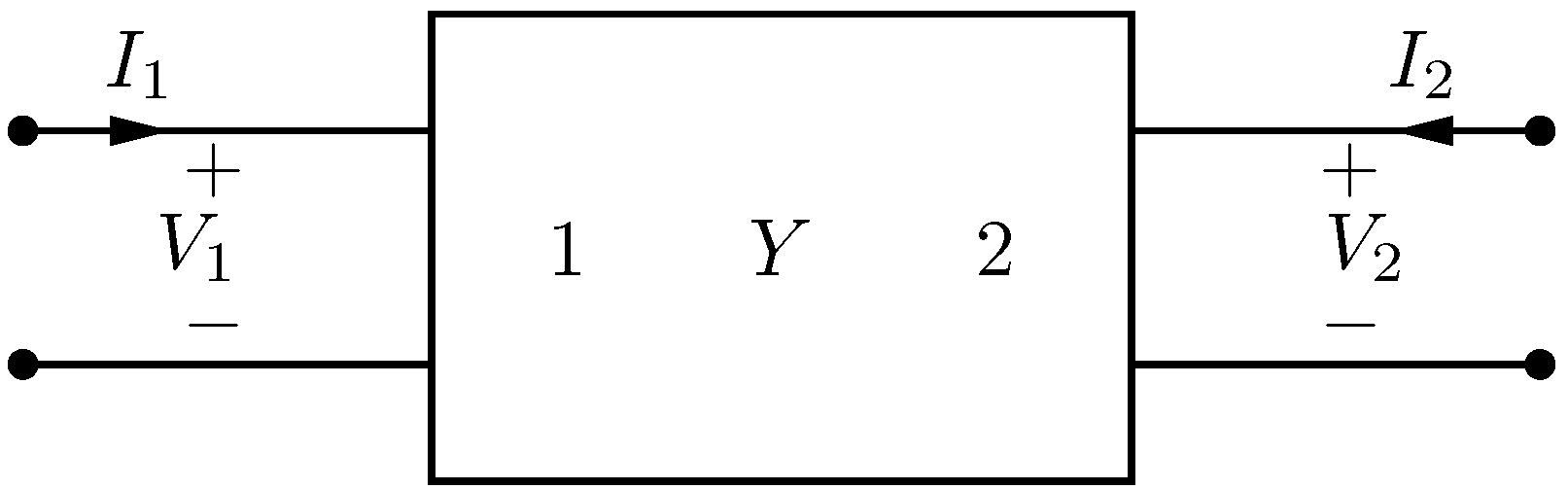
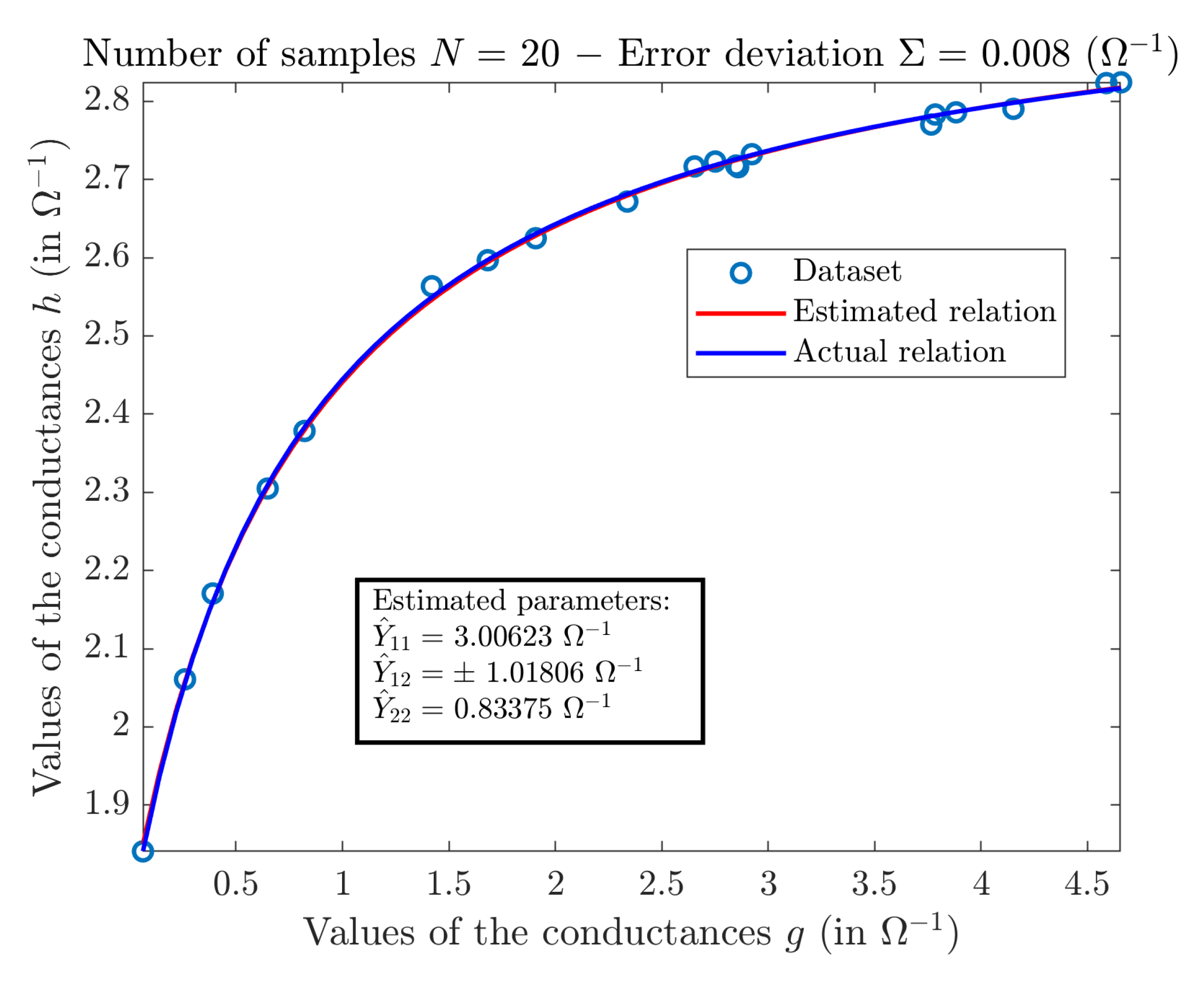
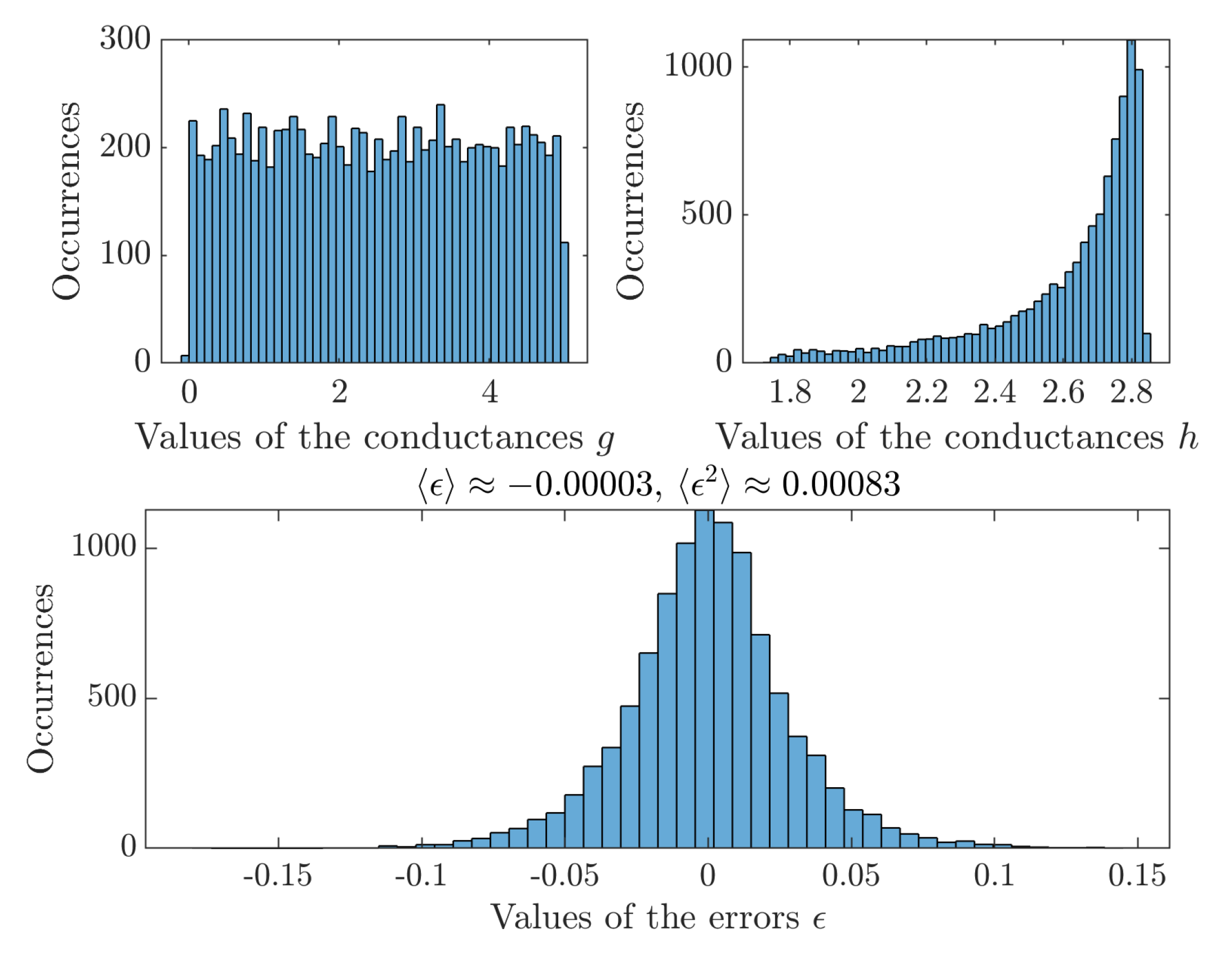
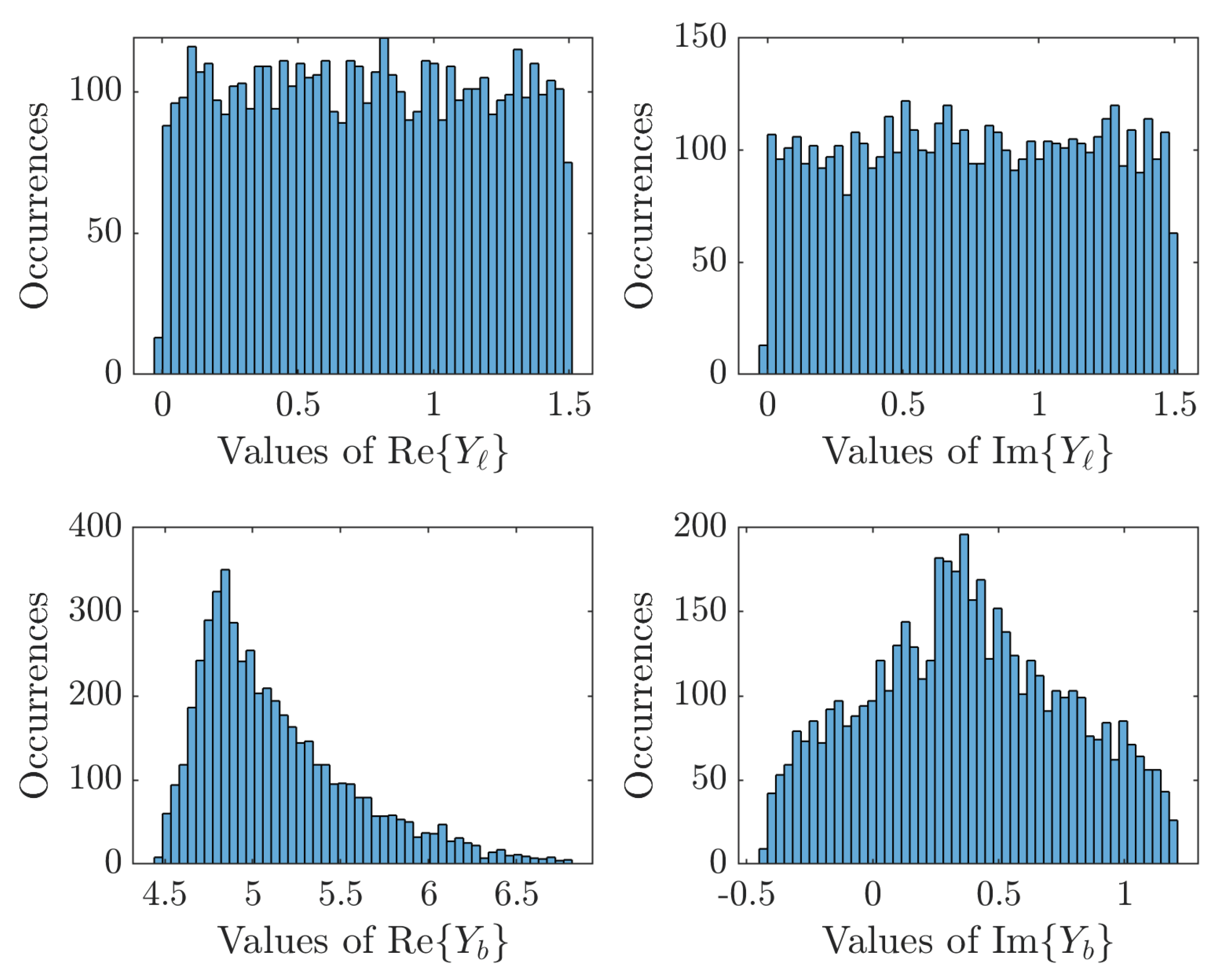
Method	Advantages	Drawbacks
Classical [2]	Requires a minimal number of measurements; requires minimal computational efforts.	Assumes errorless measurements and presents a lack of resiliency to measurement errors.
Iterative [1]	Deals with measurement errors by an iterative least-squared error approach.	Requires a larger number of measurements (from dozens to hundreds, depending on the size of the multiport network) which may be effected through an inexpensive instrumentation; requires a powerful computation platform.
Nonclassical, closed form (proposed)	Deals with measurement errors through a least-squares-error approach; does not requires a powerful computation platform.	Requires a larger number of measurements which may be effected through an inexpensive instrumentation.

.

The method utilized to estimate the values of the parameters is based on a squared-estimation-error minimization procedure. Such formulation leads to a system of three nonlinear equations in the three (independent) unknown parameters of the Y-type two-port network model. A subsystem of two equations is then isolated, and it will be shown that such a subsystem may be turned exactly (i.e., without any approximation) to linear by an appropriate ansatz and may hence be solved in closed form.

The first part of the present paper deals with the purely real-valued case that serves to clarify the problem and the found solution. The relative simplicity of the equations pertaining to the purely real case affords a statistical analysis to quantify the effects of the measurement errors on the quality of the solution, under the form of the mean values and the variances of the deviations of the estimates to the actual values, evaluated to lowest order of approximation. In the second part of the paper, the full complex-valued case is treated, although with fewer details compared to the real-valued case since the equations are similar to the ones found for the purely real case.

The present analysis is of methodological value and does not imply any in-lab experimentation. Results of numerical simulations are hence displayed and discussed to complement and illustrate the theoretical findings throughout the paper.

2. Nomenclature Summary

The present paper includes several variables and constants.

Table 2. List of the principal symbols used within this manuscript and their description.

Symbols	Description
$x,y,z$	Independent parameters of the two-port network to be identified.
$(h_k,g_k)$	Dataset pair to conduct identification.
N	Number of dataset pairs.
${ϵ}_k,E_k,J$	Specific and global modeling error.
$A,B,C,D,E,F,G,H,K$	Correlations between data entries to be used in the resolvent system and in the statistical analysis.
$M,d$	Coefficient matrix and vector of the resolvent system.
t	Estimated closed-form solution.
${\mathsf{\Sigma }}^2$	Variance of the measurement error.
$\langle \mathsf{\Delta }t\rangle ,C_t$	Mean and variance of estimation mismatch.
$V,L,R,S,P,W$	Higher-order correlations between data entries to be used in the statistical analysis.

summarizes the principal symbols used in the following sections and is meant as a guide for readers throughout the extensive notation used.

3. Problem Formulation for the Real-Valued Case

In the present work, we shall assume that a two-port system is represented by a $2\times 2$ impedance matrix $Y=\left(\begin{array}{cc}{Y}_{11}& Y_{12}\\ Y_{21}& Y_{22}\end{array}\right)$. Since the working hypotheses are that the system being modeled is lossy, reciprocal, and memoryless, we shall assume that the matrix Y is symmetric, real-valued, and positive definite, namely, that it possesses the structure $Y:=\left(\begin{array}{cc}x& y\\ y& z\end{array}\right)$ and that the following constraints on the three independent parameters $x,y,z\in \mathbb{R}$ hold:

$$\left\{\begin{array}{c}x>0,\hfill \\ xz-y^2>0,\hfill \end{array}\right.$$

(1)

which inherently imply that $z>0$ as well. A diagram of a two-port is shown in Figure 1.

Upon loading the second port by a resistor of conductance $g>0$, the equivalent conductance h at the first port reads

$$h=x-\frac{y^2}{z+g}.$$

(2)

Notice that $h=\frac{xz-y^2}{z+g}+\frac{xg}{z+g}$; therefore, by definition of the conductance g and by virtue of the constraints (1), it holds that $h>0$, namely, the whole bipole behaves as a passive resistor. Since $z+g\ne 0$, the above relationship may be rewritten as the following constraint on the unknown triple $(x,y,z):$

$$(h-x)(z+g)+y^2=0.$$

(3)

Clearly one single measurement testing is not enough to determine three unknowns, nor would be three tests, because of inherent measurement errors. We shall henceforth admit a number $N>3$ of independent tests, which provide a set of pairs $(h_k,g_k)$, with $k=1,2,\dots ,N$. Due to measurement errors, none of such pairs exactly meet the relationship (3). The discrepancy of the left-hand side to zero will be denoted as modeling error and defined as

$${ϵ}_k(x,y,z):=(h_k-x)(z+g_k)+y^2,$$

(4)

where each $g_k>0$ and each $h_k>0$. As a global model-to-data mismatch figure, we defined the index

$$J(x,y,z):=\frac{1}{2}\sum _{k=1}^N{ϵ}_k^2(x,y,z).$$

(5)

Optimal estimation consists in determining the values of the two-port parameters $(x,y,z)$ that minimize the quadratic error J and that meet the constrains (1).

4. Closed-Form Solution to the Optimization Problem for the Real-Valued Case

The first fundamental observation is that the pairwise modeling error appears as a polynomial in the three variables x, y, and z. As a consequence, the global mismatch figure J also takes the form of a polynomial in such three variables. In fact, it takes the expression

$$\begin{array}{cc}\hfill J=& \frac{1}{2}(N{x}^2{z}^2+2D{x}^{2}z+C{x}^2-2Nx{y}^{2}z-2Dx{y}^2-2Ex{z}^2-4Bxz-2Ax\hfill \\ \hfill & +N{y}^4+2E{y}^{2}z+2B{y}^2+G{z}^2+2Fz+H),\hfill \end{array}$$

(6)

where we introduce the following constants that depend only on the dataset $\{(h_1,g_1),(h_2,g_2),(h_3,g_3),\dots ,(h_N,g_N)\}$:

$$\left\{\begin{array}{c}A:=\sum _k{h}_k{g}_k^2,B:=\sum _k{h}_k{g}_k,C:=\sum _k{g}_k^2,D:=\sum _k{g}_k,\hfill \\ E:=\sum _k{h}_k,F:=\sum _k{h}_k^2{g}_k,G:=\sum _k{h}_k^2,H:=\sum _k{h}_k^2{g}_k^2.\hfill \end{array}\right.$$

(7)

It is interesting to underline that the constants D and E appear to be scaled versions of the sample mean value of the datasets $g_k$ and $h_k$, respectively, and the constants C and G appear as scaled versions of the variances of the two sets, while the constant B appears as a correlation coefficient between the data sequences (up to scaling and shifting). Such observations will become useful in evaluating the positive definiteness of the Hessian matrix associated with the error function J.

It is also useful to highlight that, since any value $g_k>0$ and $h_k>0$, any coefficient in the list (7) is also strictly positive. Such observation will turn out to be worthy in the evaluation of the shape of the feasible region.

In order to determine the critical points of the global error, it is necessary to compute the partial derivatives of the function J with respect to its three variables and to set them to zero. In order to confirm that such critical points correspond to minima of the criterion function, it will also be necessary to compute the Hessian matrix of the mismatch function J and to make sure that it is positive definite. The sought partial derivatives read

$$\begin{array}{c}\frac{\partial J}{\partial x}=-(A+2Bz-Cx+D{y}^2+E{z}^2-Nx{z}^2+N{y}^{2}z-2Dxz),\hfill \end{array}$$

(8)

$$\begin{array}{c}\frac{\partial J}{\partial y}=2y(N{y}^2+B+Ez-Dx-Nxz),\hfill \end{array}$$

(9)

$$\begin{array}{c}\frac{\partial J}{\partial z}=Gz+N{x}^{2}z+F-2Bx-2Exz+E{y}^2+D{x}^2-Nx{y}^2.\hfill \end{array}$$

(10)

Some observations are worth highlighting. The first observation is that one trivial solution arising by setting the partial derivative $\frac{\partial J}{\partial y}$ to zero would be $y=0$; we shall, however, rule out such a solution that would imply the absence of interaction between the two ports, hence making the problem essentially unworthy examining. A second observation is that the variable y appears always at the power of two; such observation leads to the conclusion that, within the present framework, it is impossible to determine the right sign of the parameter y and, hence, that this method always produces two possible solutions, namely, $(x,y,z)$ and $(x,-y,z)$.

A third observation of interest is that from the equation $N{y}^2+B+Ez-Dx-Nxz=0$, arising from the relation (9), we may directly express the unknown y in terms of the unknowns $(x,z)$ as

$$y^2=\frac{1}{N}(Dx+Nxz-B-Ez).$$

(11)

By replacing such a result back into the first equation of the system, (8), and into the third Equation (10), we may simplify the identification problem to a two-variable optimization problem. But there is more to that, since the resulting system of equations turns out to be linear and may hence be solved in closed form. In fact, the equations $\frac{\partial J}{\partial x}=0$ and $\frac{\partial J}{\partial z}=0$ lead to the linear system

$$\underset{M:=}{\underbrace{\left(\begin{array}{cc}NC-D^2& DE-NB\\ DE-NB& NG-E^2\end{array}\right)}} \underset{t:=}{\underbrace{\left(\begin{array}{c}x\\ z\end{array}\right)}}=\underset{d:=}{\underbrace{\left(\begin{array}{c}NA-DB\\ BE-NF\end{array}\right)}},$$

(12)

where we have defined the two-variable $2\times 1$ unknown array t, the $2\times 2$ system matrix M, and the $2\times 1$ coefficients array d. Notice that the matrix M results to be symmetric.

The solution of the linear system (12) reads $t=M^{-1}d$, where the inverse of the coefficient matrix M takes the form

$$M^{-1}=\frac{1}{\mathcal{D}}\left(\begin{array}{cc}NG-E^2& NB-DE\\ NB-DE& NC-D^2\end{array}\right),$$

(13)

with $\mathcal{D}:=det\left(M\right)=N\left(2BED-G{D}^2-C{E}^2+NCG-N{B}^2\right)$. Henceforth, the solution in closed form reads

$$t=\frac{N}{\mathcal{D}}\left(\begin{array}{c}AGN-A{E}^2+B^{2}E-BDG-BFN+DEF\\ ABN-ADE-B^{2}D+BCE-CFN+D^{2}F\end{array}\right).$$

(14)

The Hessian matrix of the global modeling error J with respect to the variables $(x,z)$ reads $\frac{M}{N}$, hence the solution to the above linear system of equations represents a minimum of the global error only if the matrix M is positive definite. Positive definiteness of such Hessian matrix holds only if

$$\left\{\begin{array}{c}NC-D^2>0,\hfill \\ (NC-D^2)(NG-E^2)-{(DE-NB)}^2>0.\hfill \end{array}\right.$$

(15)

We will verify that such constraints are, in fact, always met. The first constraint, $NC>D^2$, may be recast explicitly as $N{\sum }_k{g}_k^2>{\left({\sum }_k{g}_k\right)}^2$. Upon defining

$${\mu }_g:=\frac{1}{N}\sum _k{g}_k,{\sigma }_g^2:=\frac{1}{N}\sum _k{(g_k-{\mu }_g)}^2,$$

(16)

simple calculations show that $NC-D^2=N^2{\sigma }_g^2>0$. Analogously, upon defining

$${\mu }_h:=\frac{1}{N}\sum _k{h}_k,{\sigma }_h^2:=\frac{1}{N}\sum _k{(h_k-{\mu }_h)}^2,R_{hg}:=\frac{1}{N}\sum _k(h_k-{\mu }_h)(g_k-{\mu }_g),$$

(17)

the last one being a correlation coefficient, it turns out that $NG-E^2=N^2{\sigma }_h^2$ and that ${(DE-NB)}^2=N^4{R}_{gh}^2$; therefore,

$$(NC-D^2)(NG-E^2)-{(DE-NB)}^2=N^4({\sigma }_g^2{\sigma }_h^2-R_{gh}^2).$$

(18)

Since the value of the correlation coefficient $R_{gh}/\left({\sigma }_g{\sigma }_h\right)$ is strictly less than 1, the second constraint is unconditionally met as well.

Notice that the positive definiteness of the matrix $\frac{M}{N}$ implies the invertibility of the matrix M which, in turn, implies that linear system of Equation (12) certainly admits a solution.

Figure 2 shows the result of a numerical test. In this test, the actual values of the parameters are $Y_{11}=3{\mathsf{\Omega }}^{-1}$, $Y_{12}=-1{\mathsf{\Omega }}^{-1}$, and $Y_{22}=0.8{\mathsf{\Omega }}^{-1}$, and the values of g are drawn randomly from a uniform distribution in $[0,5]{\mathsf{\Omega }}^{-1}$. The measurement error is herewith assumed to be a random noise with normal distribution. Namely, we shall assume that each true value $g_k$ is affected by an additive normal measurement error, such that the value that is effectively collected is $g_k+v_k$, with $v_k\sim \mathcal{N}(0,{\mathsf{\Sigma }}^2)$ and that each true value $h_k$ is affected by an additive normal measurement error, such that the collected value is $h_k+w_k$, where $w_k\sim \mathcal{N}(0,{\mathsf{\Sigma }}^2)$. The quantity ${\mathsf{\Sigma }}^2>0$ denotes the variance of the zero-mean measurement error.

The achieved minimal squared error is $J_{\min }\approx 0.00546{\mathsf{\Omega }}^{-4}$.

We further notice that the definiteness constraint $xz-y^2>0$ may be rewritten in terms of the variables $(x,z)$ only, through the relationship (11), as

$$B+Ez-Dx>0.$$

(19)

Such inequality, together with the further definiteness constraint $x>0$, individuates the feasible set of acceptable solutions situated in the upper-right quadrant of the plane ${\mathbb{R}}^2$, as shown in Figure 3. The feasible region is characterized by the axes intercepts $\overline{x}:=\frac{B}{D}>0$ and $\overline{z}:=-\frac{B}{E}<0$ (since $B,D,E>0$, as recalled at the beginning of the present section). We shall remark that, in particular, whenever the actual value of z is approximately zero (yet being positive), the feasible solution for x is approximately $\overline{x}$.

The proposed external identification approach, resulting from the above mathematical developments, is summarized by the flowchart displayed in Figure 4.

5. Special Case of the Parameter ${\mathit{Y}}_{\mathbf{11}}$ Known in the Real-Valued Case

A special measurement setting may be exploited to obtain an approximation of the parameter $Y_{11}$ of the two-port. In the following, we make a number of assumptions to formalize such a special case:

• The second port of the two-port network may be closed by a short-circuit, which corresponds to assuming $g=\infty$; notice that this is an assumption that does not necessarily correspond to a real-world case since, at high frequency, a short-circuit is not necessarily so.
• The equivalent conductance h, in this particular measurement setting, may be measured with negligible measurement error; even this assumption will, most likely, appear just as a case study.

Under the above assumptions, the fundamental relationship (2) returns $h\equiv x$, namely, the value of the variable x may be measured directly and hence is to be considered, in principle, as known.

Now, before presenting the resulting closed-form expression of the variable z, it pays to define a reduced error function that is obtained by plugging the relation (11) into the error function (6) to obtain

$$\overline{J}(x,z):=\frac{1}{2N}[(NG-E^2)z^2+(NC-D^2)x^2+2(DE-NB)xz+2(DB-NA)x+NH-B^2].$$

(20)

If the value of the parameter x is known, the reduced error function is a function of the variable z only, whose optimal value is obtained by setting $0={\left.\frac{d\overline{J}}{dz}\right|}_{x=\mathrm{known}}$, which gives

$${\widehat{Y}}_{22}=\frac{BE-NF+(BN-DE)x}{NG-E^2}.$$

(21)

Aside, we deem it quite interesting the observation that the sharpness of the paraboloid described by the function $\overline{J}(x,z)$ along both axes is determined by the two coefficients $NG-E^2$ and $NC-D^2$ that are proportional to the sample variance of the data subsets $\{h_1,h_2,\dots ,h_N\}$ and $\{g_1,g_2,\dots ,g_N\}$.

Moreover, we notice that if the physical system that is being modeled by a two-port network allows swapping of the first port with the second port, namely, the first port may be loaded on a known load and the second port is accessible for conductance measurement, then the above considerations may be repeated with the assumption that the parameter $Y_{22}$ be known. In this way, both parameters $Y_{11}$ and $Y_{22}$ may be measured directly and the remaining parameter $Y_{12}$, the transfer admittance, may be estimated through a series of further measurements.

6. Statistical Analysis for the Real-Valued Case

The present analysis is based on the assumption that the measurements $g_k$ are the realizations of a random variable g and that the measurements $h_k$ are the realizations of a random variable h. We may then define a new error-type random variable

$$ϵ=(h-x)(g+z)+y^2.$$

(22)

On the basis of such assumption, we shall be able to estimate a number of statistical characteristics of the modeling error $\epsilon$ and of the deviations of the parameters estimations to their actual values.

6.1. Statistical Analysis of the Modeling Error $ϵ$

It is instrumental to estimate the average value and the variance of the error variable $ϵ$.

With regard to the average value, it holds that

$$\langle ϵ\rangle =\langle hg\rangle +\langle h\rangle z-\langle g\rangle x-xz+y^2,$$

(23)

where we make use of the statistical expectation operator $\langle ·\rangle$.

The actual average values may be estimated through the relevant coefficients (7) normalized by the integer N, namely, to first order

$$\langle ϵ\rangle \approx \frac{B+Ez-Dx}{N}+y^2-xz.$$

(24)

We already showed in Section 4 that the quantity $B+Ez-Dx$ equals $N(xz-y^2)$ when the triple $(x,y,z)$ is the one estimated by the devised optimization algorithm; therefore, it holds that $\langle ϵ\rangle =0$.

Likewise, the variance of the error-type random variable may be approximated, to first order, as

$$\langle {ϵ}^2\rangle -{\langle ϵ\rangle }^2\approx \frac{2J}{N}.$$

(25)

Figure 5 shows the probabilistic distributions of the random variables g, h, and $ϵ$ and correspond to the numerical test whose results were illustrated in Figure 2, except that, to obtain a meaningful statistical result, an enlarged dataset of 10,000 samples was generated.

It is immediately visible how the statistical distribution of the random variable $ϵ$ is centered at 0 within a good approximation range, as predicted.

6.2. Statistical Analysis of the Perturbations of the System (12)

In order to evaluate the effect of the measurement errors on the estimation of the parameters of the two-port, we shall perform a perturbation analysis on the system (12) written in short as $Mt=d$ and we shall further conduct a statistical analysis on the perturbations.

The seven coefficients A, B, C, D, E, F, and G that enter the linear system $Mt=d$ depend entirely on the dataset $\{(h_1,g_1),(h_2,g_2),\dots ,(h_N,g_N)\}$. Whenever the dataset is affected by measurement noise, such seven coefficients are subjected to deviations that may be assumed to be small enough to legitimate a low-order perturbation analysis.

In order to perform a perturbation analysis, we shall assume that the matrix M changes to $M+\mathsf{\Delta }M$ and that the vector d changes to $d+\mathsf{\Delta }d$. Accordingly, the solution t deviates to $t+\mathsf{\Delta }t$. For first order, we hence obtain that $\left(\mathsf{\Delta }M\right)t+M\mathsf{\Delta }t=\mathsf{\Delta }d$; therefore,

$$\mathsf{\Delta }t=M^{-1}\mathsf{\Delta }d-M^{-1}\left(\mathsf{\Delta }M\right)t,$$

(26)

where the deviations of the coefficient vector d and of the coefficient matrix M take the expressions

$$\left\{\begin{array}{c}\mathsf{\Delta }d=\left(\begin{array}{c}N\mathsf{\Delta }A-D\mathsf{\Delta }B-B\mathsf{\Delta }D\\ B\mathsf{\Delta }E+E\mathsf{\Delta }B-N\mathsf{\Delta }F\end{array}\right),\hfill \\ \mathsf{\Delta }M=\left(\begin{array}{cc}N\mathsf{\Delta }C-2D\mathsf{\Delta }D& D\mathsf{\Delta }E+E\mathsf{\Delta }D-N\mathsf{\Delta }B\\ D\mathsf{\Delta }E+E\mathsf{\Delta }D-N\mathsf{\Delta }B& N\mathsf{\Delta }G-2E\mathsf{\Delta }E\end{array}\right).\hfill \end{array}\right.$$

(27)

Notice that the matrix deviation $\mathsf{\Delta }M$ is symmetric. We remark that, in the above and following expressions of the present section, the coefficients A, B, C, D, E, F, G, and the matrix M, as well as the arrays t and d, refer to the errorless case, namely, to the case that the measurements of the conductances $g_k$ and $h_k$ are unaffected by measurement errors. The measurement errors are accounted for by the random variables $v_k$ and $w_k$ already introduced at the end of Section 4.

We shall make the following reasonable assumptions on the zero-mean Gaussian measurement errors of standard deviation $\mathsf{\Sigma }$:

• The sequence $k\mapsto v_k$ of random variables is uncorrelated, namely, $\langle v_k{v}_j\rangle ={\delta }_{kj}{\mathsf{\Sigma }}^2$, where $\delta$ denotes the Kronecker’s delta;
• The sequence $k\mapsto w_k$ is uncorrelated, namely, $\langle w_k{w}_j\rangle ={\delta }_{kj}{\mathsf{\Sigma }}^2$;
• The two sequences $k\mapsto v_k$ and $k\mapsto w_k$ are statistically independent, hence $\langle v_k{w}_j\rangle =0$, since they were supposed to be zero-mean.

Notice that both random errors are assumed to have the same variance because both measurements are supposed to be taken through the same measurement device.

On the basis of such assumption, it is possible to evaluate the deviation of each coefficient from its errorless value. With regard to the calculations about the deviation $\mathsf{\Delta }A$ of the coefficient A, we have that $A+\mathsf{\Delta }A={\sum }_k(h_k+w_k){(g_k+v_k)}^2$ from which, by expanding the multiplications, we may obtain the expression of the deviation $\mathsf{\Delta }A$, and likewise for the remaining deviations. The obtained results are summarized as follows:

$$\left\{\begin{array}{c}\mathsf{\Delta }A=2\sum _k{h}_k{g}_k{v}_k+\sum _k{h}_k{v}_k^2+\sum _k{g}_k^2{w}_k+2\sum _k{g}_k{v}_k{w}_k+\sum _k{v}_k^2{w}_k,\hfill \\ \mathsf{\Delta }B=\sum _k{h}_k{v}_k+\sum _k{g}_k{w}_k+\sum _k{v}_k{w}_k,\hfill \\ \mathsf{\Delta }C=2\sum _k{g}_k{v}_k+\sum _k{v}_k^2,\mathsf{\Delta }D=\sum _k{v}_k,\mathsf{\Delta }E=\sum _k{w}_k,\hfill \\ \mathsf{\Delta }F=\sum _k{h}_k^2{v}_k+2\sum _k{h}_k{g}_k{w}_k+2\sum _k{h}_k{w}_k{v}_k+\sum _k{g}_k{w}_k^2+\sum _k{w}_k^2{v}_k,\hfill \\ \mathsf{\Delta }G=2\sum _k{h}_k{w}_k+\sum _k{w}_k^2.\hfill \end{array}\right.$$

(28)

Applying the statistical expectation operator $\langle ·\rangle$ to both sides of the above expressions yields, at the lowest degree of approximation,

$$\left\{\begin{array}{c}\langle \mathsf{\Delta }A\rangle =E{\mathsf{\Sigma }}^2,\hfill \\ \langle \mathsf{\Delta }B\rangle =\langle \mathsf{\Delta }D\rangle =\langle \mathsf{\Delta }E\rangle =0,\hfill \\ \langle \mathsf{\Delta }C\rangle =\langle \mathsf{\Delta }G\rangle =N{\mathsf{\Sigma }}^2,\hfill \\ \langle \mathsf{\Delta }F\rangle =D{\mathsf{\Sigma }}^2,\hfill \end{array}\right.$$

(29)

thanks to the assumption on the measurement errors being statistically independent and zero-mean.

From the above results and from the relationships in (27), it turns out that the average deviation in the array d and in the matrix M take the expressions

$$\left\{\begin{array}{c}\langle \mathsf{\Delta }d\rangle =N{\mathsf{\Sigma }}^2\left(\begin{array}{c}E\\ -D\end{array}\right),\hfill \\ \langle \mathsf{\Delta }M\rangle =N^2{\mathsf{\Sigma }}^2{I}_2.\hfill \end{array}\right.$$

(30)

Through these quantities, it is possible to evaluate the average mismatch in the solution array that reads

$$\langle \mathsf{\Delta }t\rangle =M^{-1}\langle \mathsf{\Delta }d\rangle -M^{-1}\langle \mathsf{\Delta }M\rangle t.$$

(31)

The closed-form expressions of the average deviations of the single parameters, to the lowest degree of approximation, may hence be computed as

$$\left(\begin{array}{c}\langle \mathsf{\Delta }x\rangle \\ \langle \mathsf{\Delta }z\rangle \end{array}\right)=M^{-1}\left(\begin{array}{c}E-Nx\\ -D-Nz\end{array}\right)N{\mathsf{\Sigma }}^2.$$

(32)

Notice that, although the matrix M is certainly positive definite, as shown in Section 4, it might happen to be close to singularity, in which case the inverse $M^{-1}$ might amplify the deviation in the parameters. The larger the norm $\parallel M^{-1}\parallel$, the larger the amplification of the deviations.

Notice that the average deviation $\langle \mathsf{\Delta }t\rangle$ only depends on the coefficients $B,C,D,E,G$. A further observation is that, although it is not surprising that the average deviations $\langle \mathsf{\Delta }x\rangle$ and $\langle \mathsf{\Delta }z\rangle$ depend linearly on the actual values x and z (given that a first-order analysis is being carried out), it is interesting to notice that the deviation $\langle \mathsf{\Delta }x\rangle$ only depends on the value of x, while the deviation $\langle \mathsf{\Delta }z\rangle$ only depends on the value of z.

Along with the mean value of the mismatch in the estimation of the parameters x and z, the covariance matrix of such mismatch may be computed, which is defined as

$$C_t:=\langle (\mathsf{\Delta }t-\langle \mathsf{\Delta }t\rangle ){(\mathsf{\Delta }t-\langle \mathsf{\Delta }t\rangle )}^{\top }\rangle =\langle \left(\mathsf{\Delta }t\right){\left(\mathsf{\Delta }t\right)}^{\top }\rangle -\langle \mathsf{\Delta }t\rangle {\langle \mathsf{\Delta }t\rangle }^{\top }.$$

(33)

While the mean value of the mismatch fixes a reference for the estimation errors, the covariance quantifies the extent of the deviation around such reference.

The value of the entries of the covariance matrix $C_t$ may be evaluated in closed form provided some assumptions and simplifications are made. In particular, besides the assumptions on their statistical distributions and mutual uncorrelation, we shall also ignore all terms in the expressions that are proportional to a power of the measurement errors’ standard deviation, ${\mathsf{\Sigma }}^p$, with $p>2$. For instance, we notice from the expression (32) that $\langle \mathsf{\Delta }t\rangle {\langle \mathsf{\Delta }t\rangle }^{\top }\propto {\mathsf{\Sigma }}^4$; therefore, we shall neglect such a term in the computation of the entries of the matrix $C_t$. As a consequence, we only need to evaluate the $2\times 2$ (symmetric) matrix $\langle \left(\mathsf{\Delta }t\right){\left(\mathsf{\Delta }t\right)}^{\top }\rangle$.

From the expression (26), we obtain that the uncentered component of the sought covariance matrix takes the expression

$$\langle \left(\mathsf{\Delta }t\right){\left(\mathsf{\Delta }t\right)}^{\top }\rangle =M^{-1}(\langle \left(\mathsf{\Delta }d\right){\left(\mathsf{\Delta }d\right)}^{\top }-\left(\mathsf{\Delta }d\right)t^{\top }\left(\mathsf{\Delta }M\right)-\left(\mathsf{\Delta }M\right)t{\left(\mathsf{\Delta }d\right)}^{\top }+\left(\mathsf{\Delta }M\right)t{t}^{\top }\left(\mathsf{\Delta }M\right))M^{-1},$$

(34)

to come to which we make use repeatedly of the property of the matrix M (and hence of the matrix $\mathsf{\Delta }M$) to be symmetric. In order to facilitate the evaluation of the above expression, it pays to define a vector of coefficient deviations as

$$q:={\left(\begin{array}{ccccccc}\mathsf{\Delta }A& \mathsf{\Delta }B& \mathsf{\Delta }C& \mathsf{\Delta }D& \mathsf{\Delta }E& \mathsf{\Delta }F& \mathsf{\Delta }G\end{array}\right)}^{\top }.$$

(35)

Then, the vector-valued quantities $\mathsf{\Delta }d$ and $\left(\mathsf{\Delta }M\right)t$ may be expressed as

$$\left\{\begin{array}{c}\mathsf{\Delta }d=Uq,\hfill \\ \left(\mathsf{\Delta }M\right)t=Kq,\hfill \end{array}\right.$$

(36)

where we have introduced the following two matrices that help link the deviations in the coefficients A, B, C, …, G to the deviations $\mathsf{\Delta }d$ and $\left(\mathsf{\Delta }M\right)t$:

$$\left\{\begin{array}{c}U:=\left(\begin{array}{ccccccc}N& -D& 0& -B& 0& 0& 0\\ 0& E& 0& 0& B& -N& 0\end{array}\right),\hfill \\ K:=\left(\begin{array}{ccccccc}0& -Nz& Nx& Ez-2Dx& Dz& 0& 0\\ 0& -Nx& 0& Ex& Dx-2Ez& 0& Nz\end{array}\right).\hfill \end{array}\right.$$

(37)

Consequently, the expression (34) may be recast more conveniently through the relation

$$\langle \left(\mathsf{\Delta }t\right){\left(\mathsf{\Delta }t\right)}^{\top }\rangle =M^{-1}(UQ{U}^{\top }-UQ{K}^{\top }-KQ{U}^{\top }+KQ{K}^{\top })M^{-1},$$

(38)

where we use the $7\times 7$ inner covariance matrix $Q:=\langle q{q}^{\top }\rangle$, to be evaluated, which describes the dispersion of the random variables A, B, …, G around their errorless values.

The covariance matrix Q includes the auto- and cross-correlation values of all coefficient variations due to measurement errors. In the following, we shall present a detailed derivation of the expression of one of them and then, directly, the results of such calculations about every entry of the matrix Q (a total of 28 independent values). As we shall see, every entry of the covariance matrix Q is proportional to ${\mathsf{\Sigma }}^2$, where the proportionality coefficients are combinations of the quantities A, B, C, …, G plus some ad hoc new coefficients to be defined that depend on higher-order powers of the measurement errors and of their products (which may be read as sorts of higher-order correlation expressions).

We start by examining the detailed derivation of the expression of $\langle {\left(\mathsf{\Delta }B\right)}^2\rangle$. By definition, it holds that

$$\begin{array}{cc}\hfill \langle {\left(\mathsf{\Delta }B\right)}^2\rangle =& \langle (\sum _k{h}_k{v}_k+\sum _k{g}_k{w}_k+\sum _k{v}_k{w}_k)(\sum _k+j{h}_j{v}_j+\sum _j{g}_j{w}_j+\sum _j{v}_j{w}_j)\rangle \hfill \\ \hfill =& \sum _k\sum _j{g}_k{g}_j\langle w_k{w}_j\rangle +\sum _k\sum _j{g}_k{h}_j\langle w_k{v}_j\rangle +\sum _k\sum _j{g}_k\langle w_k{v}_j{w}_j\rangle +\hfill \\ \hfill & \sum _k\sum _j{h}_k{g}_j\langle v_k{w}_j\rangle +\sum _k\sum _j{h}_k{h}_j\langle v_k{v}_j\rangle +\sum _k\sum _j{h}_k\langle v_k{v}_j{w}_j\rangle +\hfill \\ \hfill & \sum _k\sum _j{g}_j\langle v_k{w}_k{w}_j\rangle +\sum _k\sum _j{h}_j\langle v_k{w}_k{v}_j\rangle +\sum _k\sum _j\langle v_k{v}_j{w}_k{w}_j\rangle \hfill \\ \hfill =& \sum _k\sum _j{g}_k{g}_j{\delta }_{kj}{\mathsf{\Sigma }}^2+\sum _k\sum _j{g}_k{h}_j·0+\sum _k\sum _j{g}_k{\delta }_{kj}{\mathsf{\Sigma }}^2·0+\hfill \\ \hfill & \sum _k\sum _j{h}_k{g}_j·0+\sum _k\sum _j{h}_k{h}_j{\delta }_{kj}{\mathsf{\Sigma }}^2+\sum _k\sum _j{h}_k·0+\hfill \\ \hfill & \sum _k\sum _j{g}_j{\delta }_{kj}{\mathsf{\Sigma }}^2·0+\sum _k\sum _j{h}_j{\delta }_{kj}{\mathsf{\Sigma }}^2·0\rangle +\sum _k\sum _j{\delta }_{kj}{\mathsf{\Sigma }}^4\hfill \\ \hfill =& \left(\sum _k{g}_k^2\right){\mathsf{\Sigma }}^2+\left(\sum _k{h}_k^2\right){\mathsf{\Sigma }}^2+\mathcal{O}\left({\mathsf{\Sigma }}^4\right).\hfill \end{array}$$

(39)

In the above equations chain, we make use repeatedly of the statistical properties of the involved random variables as, for instance, the property that $\langle w_k{v}_j{w}_j\rangle =\langle v_j\rangle \langle w_k{w}_j\rangle =0·{\delta }_{jk}{\mathsf{\Sigma }}^2=0$. Also, $\mathcal{O}$ denotes a Landau symbol. In addition, we may notice that the sum ${\sum }_k{g}_k^2$ coincides with the coefficient C and the quantity ${\sum }_k{h}_k^2$ coincides with the coefficient G, as defined in (7).

Gathered together, the seven coefficients in the first row of the matrix Q read, up to higher-order terms in the variance ${\mathsf{\Sigma }}^2$:

$$\left\{\begin{array}{c}\langle {\left(\mathsf{\Delta }A\right)}^2\rangle =(4H+V){\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }A\right)\left(\mathsf{\Delta }B\right)\rangle =(2F+L){\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }A\right)\left(\mathsf{\Delta }C\right)\rangle =4A{\mathsf{\Sigma }}^2\hfill \\ \langle \left(\mathsf{\Delta }A\right)\left(\mathsf{\Delta }D\right)\rangle =2D{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }A\right)\left(\mathsf{\Delta }E\right)\rangle =C{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }A\right)\left(\mathsf{\Delta }F\right)\rangle =2(R+S){\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }A\right)\left(\mathsf{\Delta }G\right)\rangle =2A{\mathsf{\Sigma }}^2,\hfill \end{array}\right.$$

(40)

where we made use of the following additional coefficients:

$$V:=\sum _k{g}_k^4,L:=\sum _k{g}_k^3,R:=\sum _k{h}_k^3{g}_k,S:=\sum _k{h}_k{g}_k^3.$$

(41)

The six independent coefficients in the second row of the matrix Q, up to higher-order terms in the variance ${\mathsf{\Sigma }}^2$, take the expressions

$$\left\{\begin{array}{c}\langle {\left(\mathsf{\Delta }B\right)}^2\rangle =(C+G){\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }B\right)\left(\mathsf{\Delta }C\right)\rangle =2B{\mathsf{\Sigma }}^2\hfill \\ \langle \left(\mathsf{\Delta }B\right)\left(\mathsf{\Delta }D\right)\rangle =E{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }B\right)\left(\mathsf{\Delta }E\right)\rangle =D{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }B\right)\left(\mathsf{\Delta }F\right)\rangle =(2A+P){\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }B\right)\left(\mathsf{\Delta }G\right)\rangle =2B{\mathsf{\Sigma }}^2,\hfill \end{array}\right.$$

(42)

where we made use of the additional coefficient $P:={\sum }_k{h}_k^3$.

The five independent coefficients in the third row of the matrix Q, up to higher-order terms in the variance ${\mathsf{\Sigma }}^2$, read

$$\left\{\begin{array}{c}\langle {\left(\mathsf{\Delta }C\right)}^2\rangle =4C{\mathsf{\Sigma }}^2\hfill \\ \langle \left(\mathsf{\Delta }C\right)\left(\mathsf{\Delta }D\right)\rangle =2D{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }C\right)\left(\mathsf{\Delta }E\right)\rangle =0,\hfill \\ \langle \left(\mathsf{\Delta }C\right)\left(\mathsf{\Delta }F\right)\rangle =2F{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }C\right)\left(\mathsf{\Delta }G\right)\rangle =0.\hfill \end{array}\right.$$

(43)

Continuing with the evaluation of the entries of the matrix Q, the four independent coefficients in its fourth row read, up to higher-order terms in the variance ${\mathsf{\Sigma }}^2$:

$$\left\{\begin{array}{c}\langle {\left(\mathsf{\Delta }D\right)}^2\rangle =N{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }D\right)\left(\mathsf{\Delta }E\right)\rangle =0,\hfill \\ \langle \left(\mathsf{\Delta }D\right)\left(\mathsf{\Delta }F\right)\rangle =G{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }D\right)\left(\mathsf{\Delta }G\right)\rangle =0.\hfill \end{array}\right.$$

(44)

Likewise, up to higher-order terms in the variance ${\mathsf{\Sigma }}^2$, the three independent coefficients in the fifth row of the covariance matrix Q read

$$\left\{\begin{array}{c}\langle {\left(\mathsf{\Delta }E\right)}^2\rangle =N{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }E\right)\left(\mathsf{\Delta }F\right)\rangle =2B{\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }E\right)\left(\mathsf{\Delta }G\right)\rangle =2E{\mathsf{\Sigma }}^2.\hfill \end{array}\right.$$

(45)

The last three independent coefficients from the sixth and seventh rows of the matrix Q are expressed, up to higher-order terms in the variance ${\mathsf{\Sigma }}^2$, as follows:

$$\left\{\begin{array}{c}\langle {\left(\mathsf{\Delta }F\right)}^2\rangle =(4H+W){\mathsf{\Sigma }}^2,\hfill \\ \langle \left(\mathsf{\Delta }F\right)\left(\mathsf{\Delta }G\right)\rangle =4F{\mathsf{\Sigma }}^2,\hfill \\ \langle {\left(\mathsf{\Delta }G\right)}^2\rangle =4G{\mathsf{\Sigma }}^2,\hfill \end{array}\right.$$

(46)

where we have defined the further coefficient $W:={\sum }_k{h}_k^4$.

By chaining the above relations and operating the indicated matrix calculations, the sought-after covariance matrix may be obtained. Although such covariance matrix is of size $2\times 2$, its entries are too complicated to be expressed in closed form. Nevertheless, we observe that such covariance matrix may be broken down as

$$C_t=\left(\begin{array}{cc}{\sigma }_x^2& ★\\ ★& {\sigma }_z^2\end{array}\right),$$

(47)

where the cross-covariance terms are neglected. We should perhaps remark that, due to the limited number N of samples and of the simplifications performed, the numerically estimated covariance matrix $C_t$ might result as only positive semidefinite. The quantities ${\sigma }_x^2$ and ${\sigma }_z^2$ represent the variances of the deviations of the two parameters of the admittance matrix Y (on the main diagonal).

On the basis of the estimated average deviations and of their variances, the statistical estimations of the actual (errorless) values of the parameters x and z may be presented as

$$\left\{\begin{array}{c}\widehat{x}=x+\langle \mathsf{\Delta }x\rangle \pm {\sigma }_x,\hfill \\ \widehat{z}=z+\langle \mathsf{\Delta }z\rangle \pm {\sigma }_z,\hfill \end{array}\right.$$

(48)

where the confidence intervals are assumed to be as large as $[-{\sigma }_x,{\sigma }_x]$ and $[-{\sigma }_z,{\sigma }_z]$.

As a safety-check test, the external identification method summarized in the flowchart of Figure 4 was applied to a dataset composed of $N=10$ randomly generated data-pairs with $\mathsf{\Sigma }=0{\mathsf{\Omega }}^{-1}$, namely, when no measurement errors are present. The actual admittance matrix was taken as $Y=\left(\begin{array}{cc}3& 1.5\\ 1.5& 1\end{array}\right){\mathsf{\Omega }}^{-1}$. The estimation algorithm returns the two possible solutions $\widehat{Y}=\left(\begin{array}{cc}3& \pm 1.5\\ \pm 1.5& 1\end{array}\right){\mathsf{\Omega }}^{-1}$ and the statistical estimation returns $\widehat{x}=3.00000\pm 0.00000{\mathsf{\Omega }}^{-1}$ and $\widehat{z}=1.00000\pm 0.00000{\mathsf{\Omega }}^{-1}$, with $C_t=0{\mathsf{\Omega }}^{-2}$.

As a numerical example, on the basis of the simulation conditions described at the end of Section 4, the average estimation error reads

$$\langle \mathsf{\Delta }t\rangle \approx \left(\begin{array}{c}-0.00324\\ -0.02644\end{array}\right){\mathsf{\Omega }}^{-1}$$

(49)

and the inner covariance matrix reads

$$Q\approx \left(\begin{array}{ccccccc}0.59597& 0.10457& 0.14207& 0.02006& 0.01281& 0.42734& 0.07104\\ 0.10457& 0.02194& 0.02006& 0.00341& 0.00366& 0.09561& 0.02006\\ 0.14207& 0.02006& 0.05123& 0.00731& 0.00000& 0.05512& 0.00000\\ 0.02006& 0.00341& 0.00731& 0.00128& 0.00000& 0.00914& 0.00000\\ 0.01281& 0.00366& 0.00000& 0.00000& 0.00128& 0.02006& 0.00682\\ 0.42734& 0.09561& 0.05512& 0.00914& 0.02006& 0.46054& 0.11023\\ 0.07104& 0.02006& 0.00000& 0.00000& 0.00682& 0.11023& 0.03654\end{array}\right),$$

(50)

which leads to the covariance of the deviation of the solutions

$$C_t\approx \left(\begin{array}{cc}0.00010& ★\\ ★& 0.00239\end{array}\right){\mathsf{\Omega }}^{-2}.$$

(51)

The results predicted by the statistical analysis are, hence,

$$\left\{\begin{array}{c}{\widehat{Y}}_{11}=2.99676\pm 0.00996{\mathsf{\Omega }}^{-1},\hfill \\ {\widehat{Y}}_{22}=0.77356\pm 0.04885{\mathsf{\Omega }}^{-1},\hfill \end{array}\right.$$

(52)

that, in fact, agree with the results presented in Figure 2.

7. Covering of the Complex-Valued Case

In the most general case, which also covers the frequency-domain representation of a two-port network, the involved quantities (i.e., the known loads and the parameters of the two-port circuit model) are complex-valued. Since the two-port network is supposed to be reciprocal and lossy, its admittance matrix reads

$$Y:=\left(\begin{array}{cc}{Y}_{11}& Y_{12}\\ Y_{12}& Y_{22}\end{array}\right),$$

(53)

with $Y_{11}$, $Y_{12}$, and $Y_{22}$ being three complex-valued parameters and the constraint $\Re \left\{Y\right\}>0$ must hold, which means that the conductive component of the admittance matrix must be positive definite (besides being symmetric). The susceptive component of the matrix Y just needs to be symmetric without any further constraints.

In order to estimate the unknown values of the three complex-valued parameters $Y_{11}$, $Y_{12}$, and $Y_{22}$, in the same spirit of the real-valued case, N independent tests are supposed to have been conducted by loading the second port with a series of known impedances of admittance $Y_{𝓁,k}$, and by collecting as many equivalent admittance values $Y_{b,k}$. Such values are related by

$$Y_{b,k}\approx Y_{11}-\frac{Y_{12}^2}{Y_{22}+Y_{𝓁,k}}.$$

(54)

The approximation is due, once again, to unknown measurement errors. The pairwise estimation error may be defined, in this case, as

$$E_k:=(Y_{b,k}-Y_{11})(Y_{22}+Y_{𝓁,k})+Y_{12}^2$$

(55)

on the basis of which a global squared estimation error may be defined as

$$J:={\displaystyle \frac{1}{2}}\sum _k{\left|E_k\right|}^2,$$

(56)

where the notation $|·|$ denotes the modulus of a complex number. The best estimation of the three coefficients arises from the minimization of the global squared estimation error.

In the present complex-domain instance, the minimization of the criterion J may still be achieved by setting its “derivative” to zero, as long as a notion of derivative with respect to a complex-valued variable is defined properly. We define the derivative (or “gradient” or “del operator”) $\nabla J$ with respect to one of its arguments $Y_{ab}$ as (see, e.g., [19])

$${\nabla }_{Y_{ab}}J:=\frac{\partial J}{\partial \Re \left\{Y_{ab}\right\}}+i\frac{\partial J}{\partial \Im \left\{Y_{ab}\right\}},$$

(57)

where i denotes the imaginary unit. Calculations lead to the following expressions of the complex derivatives

$$\left\{\begin{array}{c}{\nabla }_{Y_{11}}J=-\sum _k{Y}_{𝓁,k}^{*}{E}_k-Y_{22}^{*}\sum _k{E}_k,\hfill \\ {\nabla }_{Y_{12}}J=2Y_{12}^{*}\sum _k{E}_k,\hfill \\ {\nabla }_{Y_{22}}J=\sum _k{Y}_{b,k}^{★}{E}_k-Y_{11}^{★}\sum _k{E}_k,\hfill \end{array}\right.$$

(58)

to be set to zero, where the superscript ${}^{*}$ denotes complex conjugation. As for the real-valued case, we shall discard the trivial solution $Y_{12}=0$ that would imply a null transfer admittance; hence, the second equation would be ${\sum }_k{E}_k=0$. This condition, however, implies that the second sum on the right-hand side of the first and of the third relations are identically zero. Therefore, the system of equations to solve reads

$$\left\{\begin{array}{c}\sum _k{Y}_{𝓁,k}^{*}{E}_k=0,\hfill \\ \sum _k{E}_k=0,\hfill \\ \sum _k{Y}_{b,k}^{★}{E}_k=0.\hfill \end{array}\right.$$

(59)

Interestingly, such expressions appear as sums of errors—weighted by data—set to zero.

Upon plugging-in the expression of the error $E_k=Y_{b,k}{Y}_{𝓁,k}+Y_{b,k}{Y}_{22}-Y_{𝓁,k}{Y}_{11}+Y_{12}^2-Y_{11}{Y}_{22}$, we obtain the following system of polynomial equations:

$$\left\{\begin{array}{c}A+B{Y}_{22}-C{Y}_{11}+D^{*}(Y_{12}^2-Y_{11}{Y}_{22})=0,\hfill \\ F+G{Y}_{22}-D{Y}_{11}+N(Y_{12}^2-Y_{11}{Y}_{22})=0,\hfill \\ H+K{Y}_{22}-B^{*}{Y}_{11}+G^{*}(Y_{12}^2-Y_{11}{Y}_{22})=0,\hfill \end{array}\right.$$

(60)

where the constant coefficients of the systems are defined as

$$\left\{\begin{array}{c}A:=\sum _k|Y_{𝓁,k}{|}^2{Y}_{b,k},B:=\sum _k{Y}_{𝓁,k}^{*}{Y}_{b,k},C:=\sum _k{\left|Y_{𝓁,k}\right|}^2,D:=\sum _k{Y}_{𝓁,k},\hfill \\ F:=\sum _k{Y}_{b,k}{Y}_{𝓁,k},G:=\sum _k{Y}_{b,k},H:=\sum _k|Y_{b,k}{|}^2{Y}_{𝓁,k},K:=\sum _k{\left|Y_{b,k}\right|}^2.\hfill \end{array}\right.$$

(61)

The system (60) appears as a set of three nonlinear equations, which may, however, be rendered in linear form. In fact, from the second equation, one may infer that

$$Y_{12}^2=Y_{11}{Y}_{22}+\frac{1}{N}(D{Y}_{11}-G{Y}_{22}-F);$$

(62)

henceforth, the first and third equations in (60) may be rewritten as the linear system

$$\underset{M:=}{\underbrace{\left(\begin{array}{cc}NC-{\left|D\right|}^2& G{D}^{*}-NB\\ N{B}^{*}-D{G}^{*}& {\left|G\right|}^2-NK\end{array}\right)}} \underset{t:=}{\underbrace{\left(\begin{array}{c}{Y}_{11}\\ Y_{22}\end{array}\right)}}=\underset{d:=}{\underbrace{\left(\begin{array}{c}NA-F{D}^{*}\\ NH-F{G}^{*}\end{array}\right)}}.$$

(63)

The solution to such system of equations may be written again as $t=M^{-1}d$, to determine the optimal values of the parameters $Y_{11}$ and $Y_{22}$. In addition, the relationship

$$Y_{12}=\pm \sqrt{Y_{11}{Y}_{22}+\frac{1}{N}(D{Y}_{11}-G{Y}_{22}-F)},$$

(64)

involving complex-valued square-rooting, may be used to determine two (indistinguishable, in fact) optimal values of the parameter $Y_{12}$.

As a numerical result, taking as actual value of the admittance matrix

$$Y=\left(\begin{array}{cc}3.0+0.2i& -1.0+2.0i\\ -1.0+2.0i& 0.8+1.0i\end{array}\right){\mathsf{\Omega }}^{-1}$$

(65)

and setting a value for the standard deviation of the measurement error $\mathsf{\Sigma }={10}^{-2}{\mathsf{\Omega }}^{-1}$, the optimal values obtained through the optimization-based estimation method are

$$\widehat{Y}\approx \left(\begin{array}{cc}3.0012+0.2176i& 0.9821-2.0057i\\ 0.9821-2.0057i& 0.8127+0.9846i\end{array}\right){\mathsf{\Omega }}^{-1},$$

(66)

which is, of course, in excellent agreement with the actual value (notice the sign flip in the off-diagonal entries). Figure 6 shows the statistical distribution of the real and imaginary part of the dataset enlarged to $10,000$ samples to obtain a meaningful representation (since the samples are generated randomly by a computer code, the number N of samples may be inflated at will).

8. Conclusions

The problem of the estimation of the three independent parameters of a reciprocal, lossy Y-type two-port network was dealt with, under the assumption that its terminals are accessible to an impedance-measurement analyzer. A number of test loads are first subjected to measurement of admittance; next, such loads are connected to one of the ports of the network, and the equivalent admittance seen from the other port is collected.

The data pairs collected during the execution of such procedure are affected by measurement errors; therefore, the optimal parameter estimation method utilized is based on a squared-estimation-error minimization. Such formulation leads to a system of three nonlinear equations in the three unknown parameters of the Y-type two-port network model. A subsystem of two equations is then isolated, which may be turned exactly to linear, and may be solved in closed form.

The first part of the paper dealt with the purely real-valued case that served to clarify the problem and its solution. The purely real-valued case also affords a statistical analysis that enables an analytic, as well as a numerical, evaluation of the effects of the measurement errors on the quality of the solution. Such statistical analysis yields, as a result, the mean values and the variances of the deviations of the estimates to the actual values. The full complex-valued case was treated in the second part of the paper with fewer details since the equations are similar to the ones found for the purely-real case.

The results of some numerical experiments, both for the real-valued and for the complex-valued cases, were displayed and discussed to complement and illustrate the theoretical findings.

The present research work constitutes the first step in the external characterization by multiport networks on the basis of a black-box modeling procedure. The full case of a $2n$-terminal network will be investigated in forthcoming research endeavors.